Analysis of Overturning and Vibration during Field Operation of a Tractor-Mounted 4-Row Radish Collector toward Ensuring User Safety
Abstract
1. Introduction
2. Materials and Methods
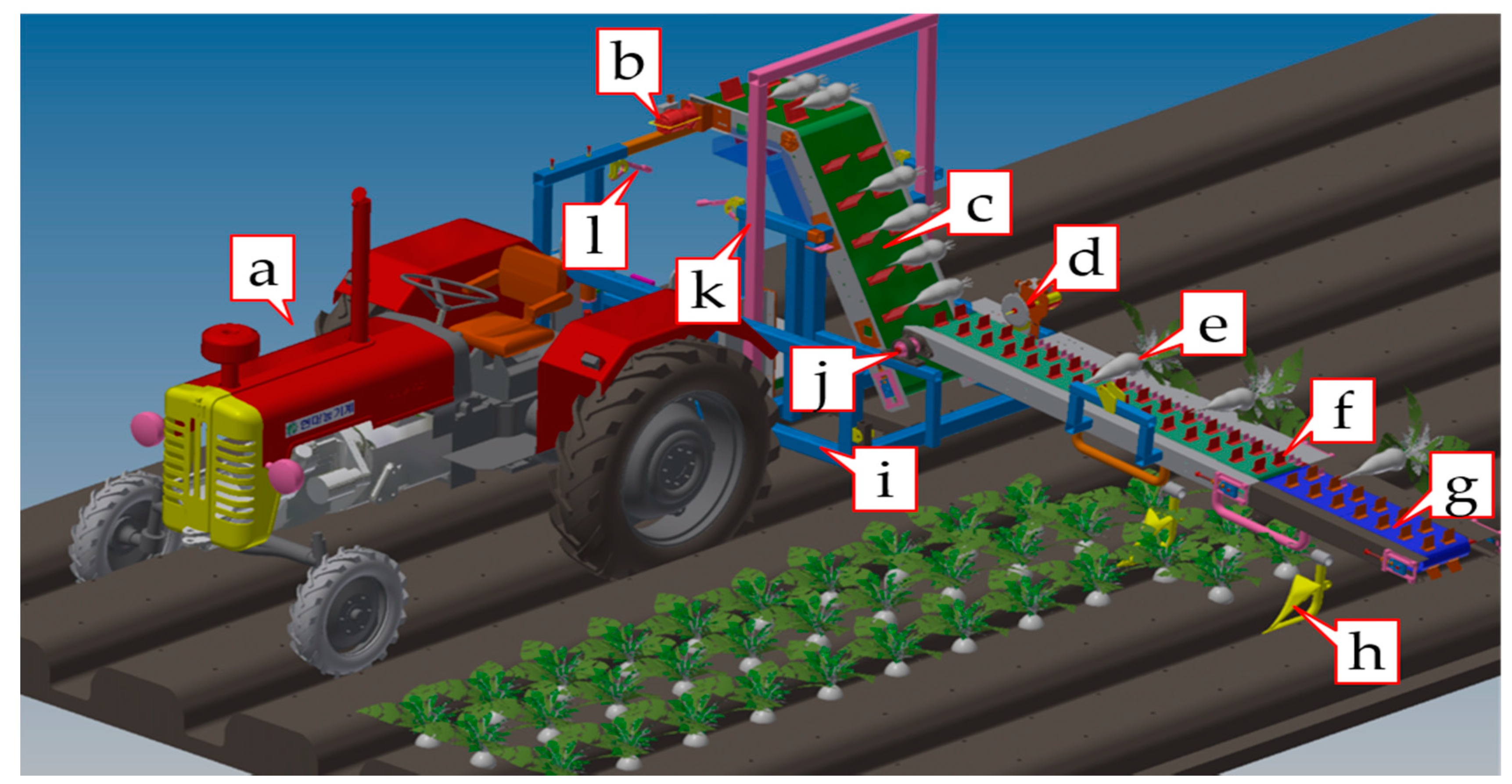
2.1. Structure and Working Principle of the Radish Collector
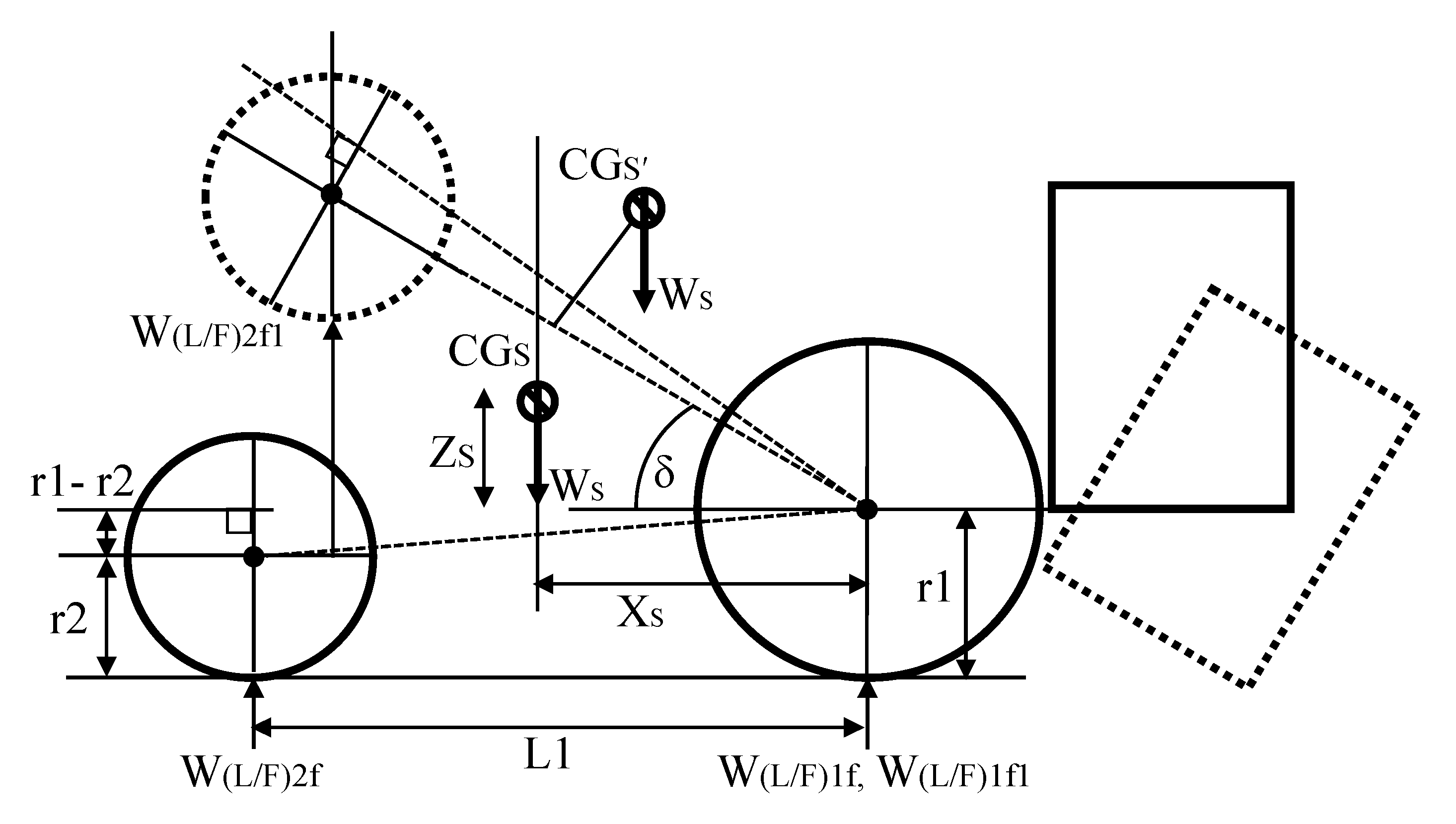
2.2. Overturning Stability of the Tractor–Collector System
2.2.1. Determination of the Center of Gravity
2.2.2. Estimation of the Transverse Overturning Angle
2.2.3. Identification of the Lateral Overturning Angles through Simulation and Validation
2.3. Vibration Measurement of the Tractor–Collector System
2.3.1. Fast Fourier Transform (FFT)
2.3.2. Power Spectral Density (PSD)
2.4. Statistical Analysis
3. Results
3.1. Overturning Stability
3.1.1. Center of Gravity
3.1.2. Transverse Overturning Angle
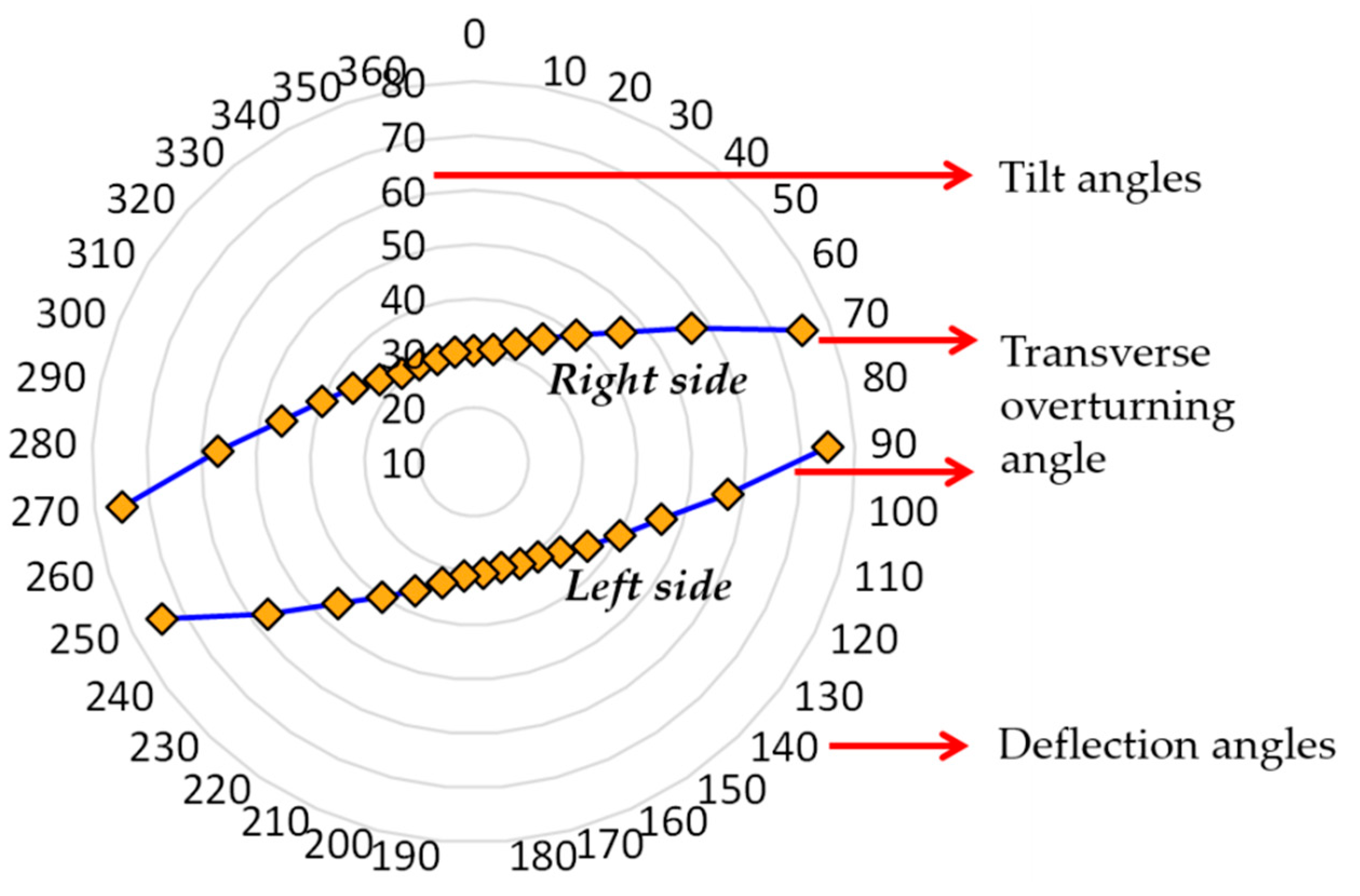
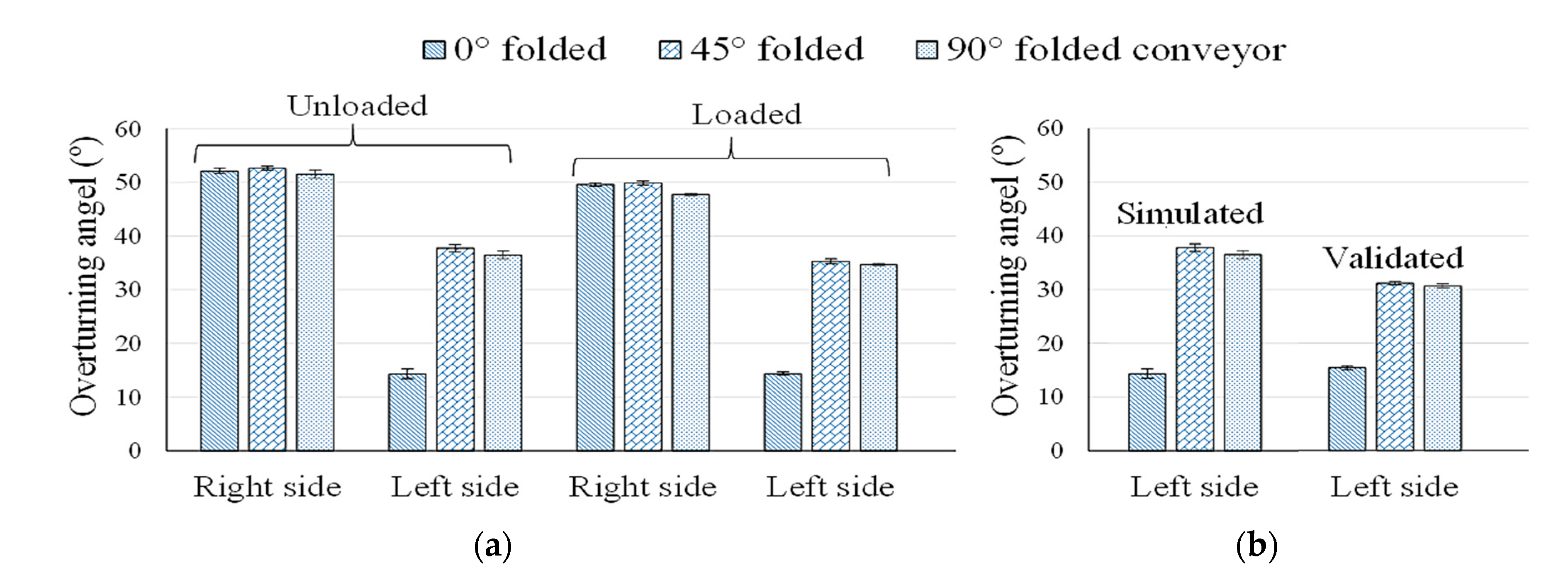
3.1.3. Lateral Overturning Angle
3.2. Vibration Evaluation of the Tractor–Collector System
4. Discussion
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Zhang, J.; He, P.; Ding, W.; Xu, X.; Ullah, S.; Abbas, T.; Ai, C.; Li, M.; Cui, R.; Jin, C.; et al. Estimating nutrient uptake requirements for radish in China based on QUEFTS model. Sci. Rep. 2019, 9, 11663. [Google Scholar] [CrossRef]
- LLC, A. Daikon Radish—Japanese Agriculture|Japan CROPs. Available online: https://japancrops.com/en/crops/daikon-radish/ (accessed on 11 November 2020).
- Cultivated Area of Autumn Cabbages and Radishes in 2019. Available online: http://kostat.go.kr/portal/eng/pressReleases/2/2/index.board?bmode=read&aSeq=378915&pageNo=&rowNum=10&amSeq=&sTarget=&sTxt= (accessed on 24 September 2020).
- Banihani, S.A. Radish (Raphanus sativus) and Diabetes. Nutrients 2017, 9, 1014. [Google Scholar] [CrossRef] [PubMed]
- Ishida, M.; Kakizaki, T.; Morimitsu, Y.; Ohara, T.; Hatakeyama, K.; Yoshiaki, H.; Kohori, J.; Nishio, T. Novel glucosinolate composition lacking 4-methylthio-3-butenyl glucosinolate in Japanese white radish (Raphanus sativus L.). Theor. Appl. Genet. 2015, 128, 2037–2046. [Google Scholar] [CrossRef] [PubMed]
- Malik, M.S.; Riley, M.B.; Norsworthy, J.K.; Bridges, W. Variation of glucosinolates in wild radish (Raphanus raphanistrum) accessions. J. Agric. Food Chem. 2010, 58, 11626–11632. [Google Scholar] [CrossRef] [PubMed]
- Sakuragawa, M.; Makino, T. Labor Force Ageing and Economic Growth in Japan. In Ageing and the Labor Market in Japan; Koichi, H., Hiromi, K., Eds.; Edward Elgar Publishing: Cheltenham, UK, 2007; pp. 57–74. [Google Scholar]
- Oshio, T.; Usui, E.; Shimizutani, S. Labor Force Participation of the Elderly in Japan; National Bureau of Economic Research: Cambridge, MA, USA, 2018; p. w24614. [Google Scholar]
- Shin, S.Y.; Kang, C.H.; Yu, S.C.; Kim, Y.Y.; Noh, J.S. Criteria for determining working area and operating cost for long-term lease of agricultural machinery. J. Biosyst. Eng. 2015, 40, 178–185. [Google Scholar] [CrossRef][Green Version]
- Jung, Y.; Jeon, H.H.; Jung, H.J.; Choi, C.H.; Yong Joo, K. Finite element analysis of radish harvesting part. In Proceedings of the ASABE Annual International Meeting, Detroit, MI, USA, 29 July–1 August 2018. [Google Scholar]
- Ali, M.; Lee, Y.S.; Kabir, M.S.N.; Kang, T.K.; Lee, S.H.; Chung, S.O. Kinematic analysis for design of the transportation part of a tractor-mounted Chinese cabbage collector. J. Biosyst. Eng. 2019, 44, 226–235. [Google Scholar] [CrossRef]
- Liu, H.C.; Sung, W.P.; Yao, W. Current status and future strategy for the application of new technologies in agricultural mechanization. In Proceedings of the International Conference on Information Technology and Computer Application Engineering Press, Hong Kong, China, 12 June 2018. [Google Scholar]
- Cuddihy, W. Agricultural prices, farm mechanization, and the demand for labor. In Migration, Mechanization, and Agricultural Labor Markets in Egypt; Richards, A., Martin, P.L., Eds.; Routledge: Abingdon, UK, 2019; pp. 225–236. ISBN 978-0-429-04710-7. [Google Scholar]
- Hong, S.; Lee, K.; Kang, D.; Park, W. Analysis of static lateral stability using mathematical simulations for 3-axis tractor-baler system. J. Biosyst. Eng. 2017, 42, 86–97. [Google Scholar] [CrossRef]
- Paul, A.; John, C.; Rob, C.; Pau, T. Stability analysis of agricultural off-road vehicles. J. Agric. Saf. Health 2018, 24, 167–182. [Google Scholar] [CrossRef]
- Liu, B.; Koc, A.B. Field tests of a tractor rollover detection and emergency notification system. J. Agric. Saf. Health 2015, 113–127. [Google Scholar] [CrossRef]
- ISO 16231-2:2015 Self-Propelled Agricultural Machinery—Assessment of Stability—Part 2: Determination of Static Stability and Test Procedures. Available online: https://www.iso.org/cms/render/live/en/sites/isoorg/contents/data/standard/06/15/61583.html (accessed on 24 September 2020).
- ANSI/OPEI B71.4-2017—Commercial Turf Care Equipment—Safety Specifications. Available online: https://webstore.ansi.org/standards/opei/ansiopeib712017-1654115 (accessed on 24 September 2020).
- Wang, X. The influence of the lift angle on the center of gravity: Measurements for zero turning radius mowers. Appl. Eng. Agric. 2016, 32, 189–199. [Google Scholar] [CrossRef]
- Khorsandi, F.; Ayers, P.D.; Freeland, R.S.; Wang, X. Modeling the effect of liquid movement on the center of gravity calculation of agricultural vehicles. J. Terramech. 2018, 75, 37–48. [Google Scholar] [CrossRef]
- Rabbani, M.A.; Tsujimoto, T.; Mitsuoka, M.; Inoue, E.; Okayasu, T. Prediction of the vibration characteristics of half-track tractor considering a three-dimensional dynamic model. Biosyst. Eng. 2011, 110, 178–188. [Google Scholar] [CrossRef]
- Scarlett, A.J.; Price, J.S.; Stayner, R.M. Whole-body vibration: Evaluation of emission and exposure levels arising from agricultural tractors. J. Terramech. 2007, 44, 65–73. [Google Scholar] [CrossRef]
- Cutini, M.; Costa, C.; Bisaglia, C. Development of a simplified method for evaluating agricultural tractor’s operator whole body vibration. J. Terramech. 2016, 63, 23–32. [Google Scholar] [CrossRef]
- Deboli, R.; Calvo, A.; Preti, C. Comparison between ISO 5008 and field whole body vibration tractor values. J. Agric. Eng. 2012, 43, e8. [Google Scholar] [CrossRef]
- Gao, Z.; Xu, L.; Li, Y.; Wang, Y.; Sun, P. Vibration measure and analysis of crawler-type rice and wheat combine harvester in field harvesting condition. Trans. Chin. Soc. Agric. Eng. 2017, 33, 48–55. [Google Scholar]
- Xu, L.; Li, Y.; Sun, P.; Pang, J. Vibration measurement and analysis of tracked-whole feeding rice combine harvester. Trans. Trans. Chin. Soc. Agric. Eng. 2014, 30, 49–55. [Google Scholar]
- Krajnak, K. Health effects associated with occupational exposure to hand-arm or whole body vibration. J. Toxic. Environ. Health Part B 2018, 21, 320–334. [Google Scholar] [CrossRef]
- Gialamas, T.; Gravalos, I.; Kateris, D.; Xyradakis, P.; Dimitriadis, C. Vibration analysis on driver’s seat of agricultural tractors during tillage tests. Spanish J. Agric. Res. 2016, 14, 5. [Google Scholar] [CrossRef]
- Pang, J.; Li, Y.; Ji, J.; Xu, L. Vibration excitation identification and control of the cutter of a combine harvester using triaxial accelerometers and partial coherence sorting. Biosyst. Eng. 2019, 185, 25–34. [Google Scholar] [CrossRef]
- ISO 5008:2002—Agricultural Wheeled Tractors and Field Machinery. Measurement of Whole-Body Vibration of the Operator. Available online: https://shop.bsigroup.com/en/ProductDetail/?pid=000000000030142859&_ga=2.150491198.943613003.1601766954-1138060861.1601766954 (accessed on 4 October 2020).
- Choi, K.; Kim, S.M.; Hong, S. Analysis of static stability by modified mathematical model for asymmetric tractor-harvester system: Changes in lateral overturning angle by movement of center of gravity coordinates. J. Biosyst. Eng. 2017, 42, 127–135. [Google Scholar] [CrossRef]
- Kabir, M.S.N.; Chung, S.O.; Kim, Y.J.; Sung, N.S.; Hong, S.J. Measurement and evaluation of whole body vibration of agricultural tractor operator. Int. J. Agric. Biol. Eng. 2017, 10, 248–255. [Google Scholar] [CrossRef]
- Nakai, D.; Saito, K. A method for generating random vibration using acceleration kurtosis and velocity kurtosis. J. Appl. Pack. Res. 2019, 11, 64–74. [Google Scholar]
- Heidarbeigi, K.; Ahmadi, H.; Omid, M. Fault diagnosis of Massey Ferguson gearbox using power spectral density. In Proceedings of the 18th International Conference on Electrical Machines, Vilamoura, Portugal, 6–9 September 2008. [Google Scholar]
- Bracewell, R.N.; Bracewell, R.N. The Fourier Transform and Its Applications; McGraw-Hill: New York, NY, USA, 1986; Volume 31999, pp. 127–134. [Google Scholar]
- Khaksar, Z.; Ahmadi, H.; Mohtasebi, S.S. Whole body vibration analysis of tractor operators using power spectral density. J. Mech. Eng. Technol. 2013, 1, 6–12. [Google Scholar] [CrossRef]
- Rahman, M.M.; Ariffin, A.K.; Jamaludin, N.; Haron, C.H.C. Finite element based vibration fatigue analysis for a new free piston engine components. Arabian J. Sci. Eng. 2009, 34, 231–246. [Google Scholar]
- Irvine, T. Power Spectral Density Units: [ G^2 / Hz]. Revision B 2007. Available online: http://vibrationdata.com/tutorials2/psd.pdf (accessed on 24 September 2020).
- Howard, R.M. Principles of Random Signal Analysis and Low Noise Design: The Power Spectral Density and Its Applications; John Wiley & Sons: Perth, Australia, 2004; ISBN 978-0-471-46083-1. [Google Scholar]
- Fargnoli, M.; Lombardi, M. Safety vision of agricultural tractors: An engineering perspective based on recent studies (2009–2019). Safety 2020, 6, 1. [Google Scholar] [CrossRef]
- Reński, A. Investigation of the influence of the center of gravity position on the course of vehicle rollover. In Proceedings of the 24th International Technical Conference on the Enhanced Safety of Vehicles (ESV) National Highway Traffic Safety Administration, Gothenburg, Sweden, 8–11 June 2015. [Google Scholar]
- Hostens, I.; Ramon, H. Descriptive analysis of combine cabin vibrations and their effect on the human body. J. Sound Vib. 2003, 266, 453–464. [Google Scholar] [CrossRef]
- Kabir, S.N.; Song, M.Z.; Chung, S.O.; Kim, Y.J.; Kim, S.C.; Ha, J.K. Visibility evaluation for agricultural tractor operators according to ISO 5006 and 5721-1 standards. J. Biosyst. Eng. 2015, 40, 19–27. [Google Scholar] [CrossRef][Green Version]




| Specification of Tractor | Specification of Radish Collector | ||
|---|---|---|---|
| Model | Luxen 74 | - | |
| Mass (kg) | 3052 | Mass (kg) | 460 |
| Overall length (mm) | 4117 | Overall length (mm) | 4210 |
| Overall width (mm) | 1893 | Overall width (mm) | 1200 |
| Overall height (mm) | 2605 | Overall height (mm) | 2000 |
| Ground clearance (mm) | 488 | Ground clearance (mm) | 600 |
| Wheelbase (mm) | 2250 | Polymer bit (mm) | 50.44 |
| Wheel track (mm) | 1650 | 1st conveyor belt length (mm) | 2950 |
| Front wheel radius (mm) | 530 | 2nd conveyor belt length (mm) | 1400 |
| Rear wheel radius (mm) | 720 | Radish collecting capacity | 30/bag |
| Mass of Tractor (kg) | Mass of Tractor with Radish Collector (kg) | ||
|---|---|---|---|
| Mass of left front wheel (WTF1) | 714 | Mass of left front wheel (WSF1) | 531 |
| Mass of right front wheel (WTF2) | 718 | Mass of right front wheel (WSF2) | 520 |
| Mass of left rear wheel (WTF1) | 814 | Mass of left rear wheel (WSR1) | 1322 |
| Mass of right rear wheel (WTF2) | 806 | Mass of right rear wheel (WSR2) | 1268 |
| Total mass of the tractor (WT) | 3052 | Total mass of the system (WS) | 3641 |
| Mass of tractor operator | 83 | ||
| Mass of harvested radish | 45 | ||
| Factor | Condition |
|---|---|
| Folding condition of the conveyor belt | 0, 45, and 90° |
| Load condition | Loaded, unloaded |
| Overturning side | Left, right |
| Sensor Location | Conveyor Speed (ms−1) | |||||
|---|---|---|---|---|---|---|
| 0.10 | 0.16 | 0.20 | 0.10 | 0.16 | 0.20 | |
| Unloaded Condition | Loaded Condition | |||||
| Collector base | 1.03 a | 1.27 a | 1.66 a | 0.41 a | 0.37 a | 0.41 a |
| 1st conveyor belt | 2.41 a | 2.24 a | 1.59 a | 4.34 b | 4.27 b | 4.43 b |
| 2nd conveyor belt | 1.01 a | 1.19 a | 1.43 a | 0.41 c | 0.37 c | 0.48 c |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chowdhury, M.; Islam, M.N.; Iqbal, M.Z.; Islam, S.; Lee, D.-H.; Kim, D.-G.; Jun, H.-J.; Chung, S.-O. Analysis of Overturning and Vibration during Field Operation of a Tractor-Mounted 4-Row Radish Collector toward Ensuring User Safety. Machines 2020, 8, 77. https://doi.org/10.3390/machines8040077
Chowdhury M, Islam MN, Iqbal MZ, Islam S, Lee D-H, Kim D-G, Jun H-J, Chung S-O. Analysis of Overturning and Vibration during Field Operation of a Tractor-Mounted 4-Row Radish Collector toward Ensuring User Safety. Machines. 2020; 8(4):77. https://doi.org/10.3390/machines8040077
Chicago/Turabian StyleChowdhury, Milon, Md Nafiul Islam, Md Zafar Iqbal, Sumaiya Islam, Dae-Hyun Lee, Dae-Geon Kim, Hyeon-Jong Jun, and Sun-Ok Chung. 2020. "Analysis of Overturning and Vibration during Field Operation of a Tractor-Mounted 4-Row Radish Collector toward Ensuring User Safety" Machines 8, no. 4: 77. https://doi.org/10.3390/machines8040077
APA StyleChowdhury, M., Islam, M. N., Iqbal, M. Z., Islam, S., Lee, D.-H., Kim, D.-G., Jun, H.-J., & Chung, S.-O. (2020). Analysis of Overturning and Vibration during Field Operation of a Tractor-Mounted 4-Row Radish Collector toward Ensuring User Safety. Machines, 8(4), 77. https://doi.org/10.3390/machines8040077









