Design of a Large Deployable Reflector Opening System
Abstract
1. Introduction
- Mesh antennas
- Solid surface antennas
- Inflatable antennas
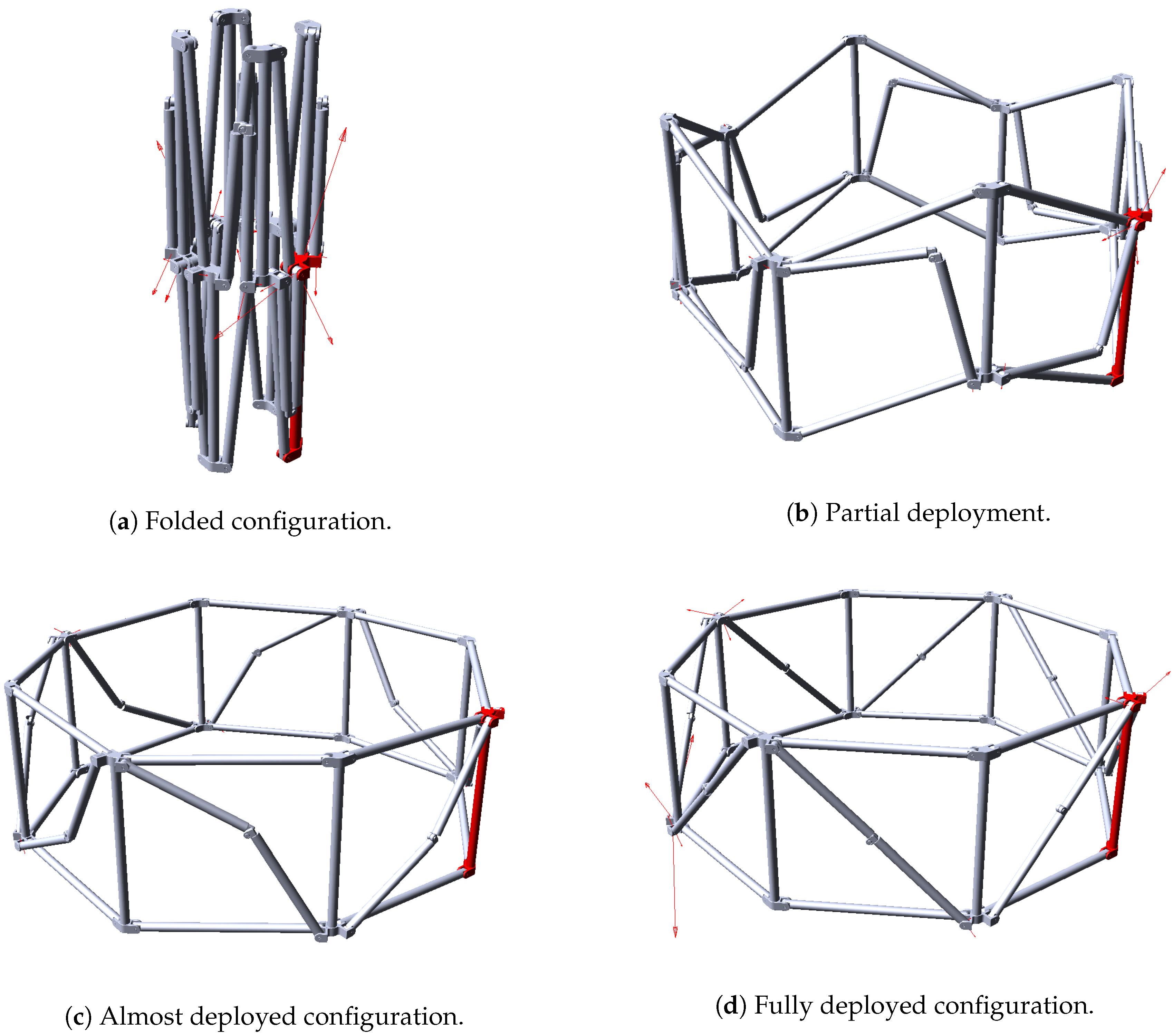
2. Kinematic Design
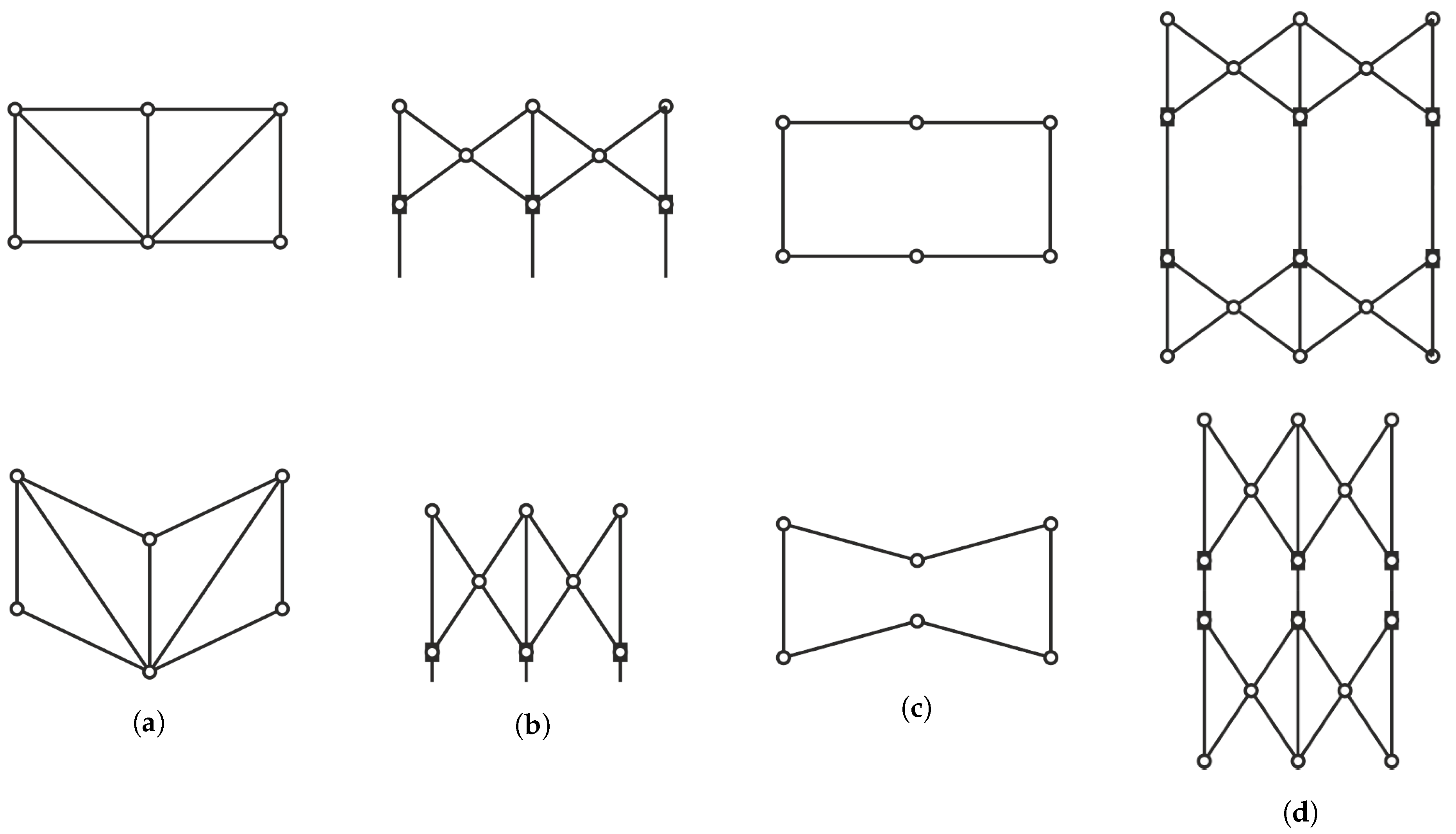
2.1. Features of an LDR
- Parallelogram with bar or diagonal cable
- Pantograph
- V-folding rectangle
- Double vertical pantograph
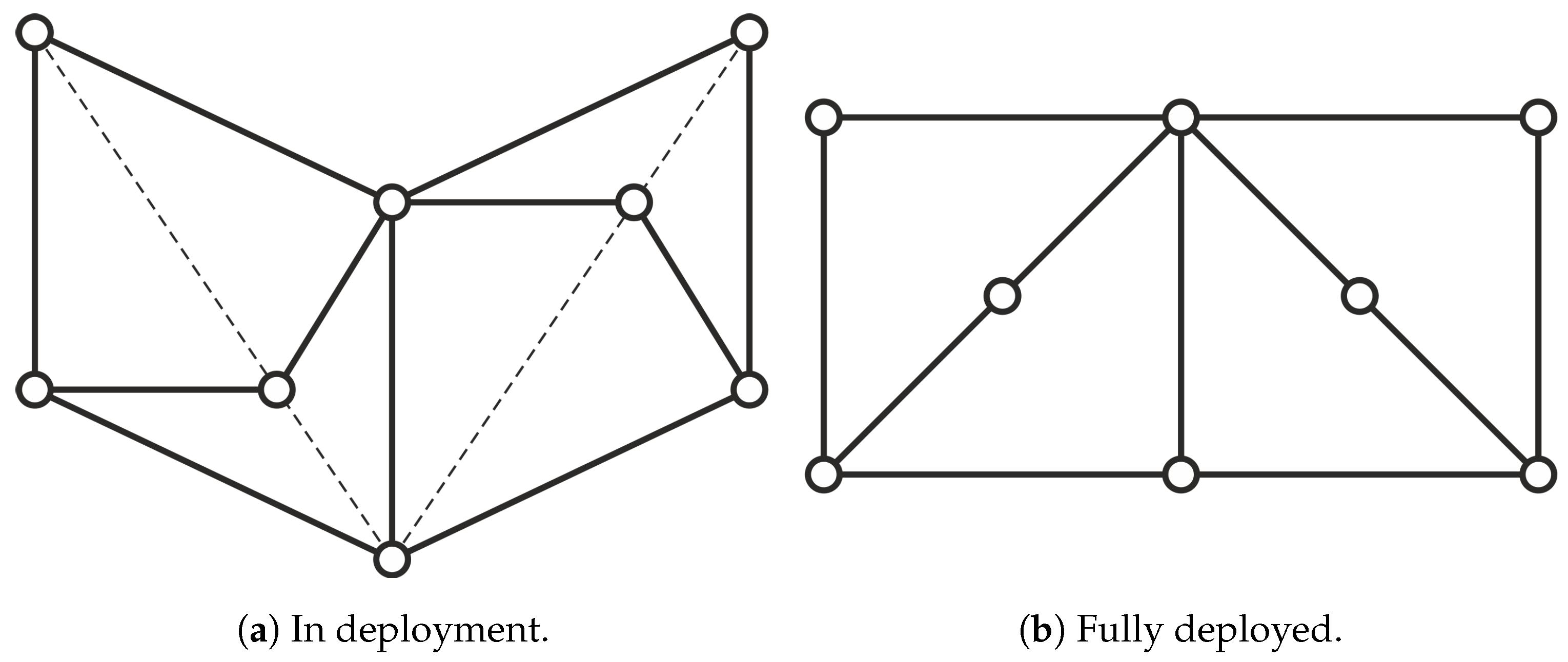
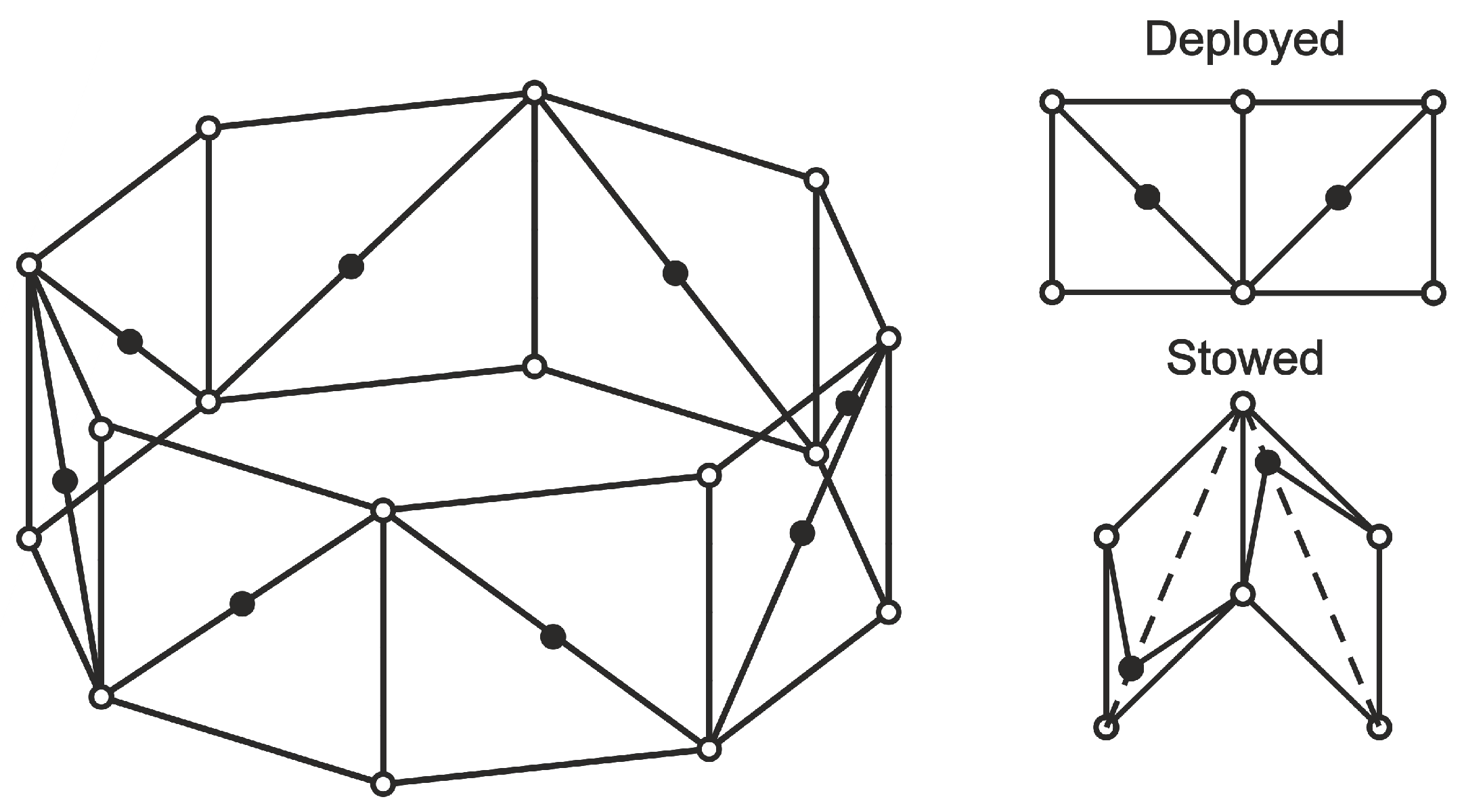
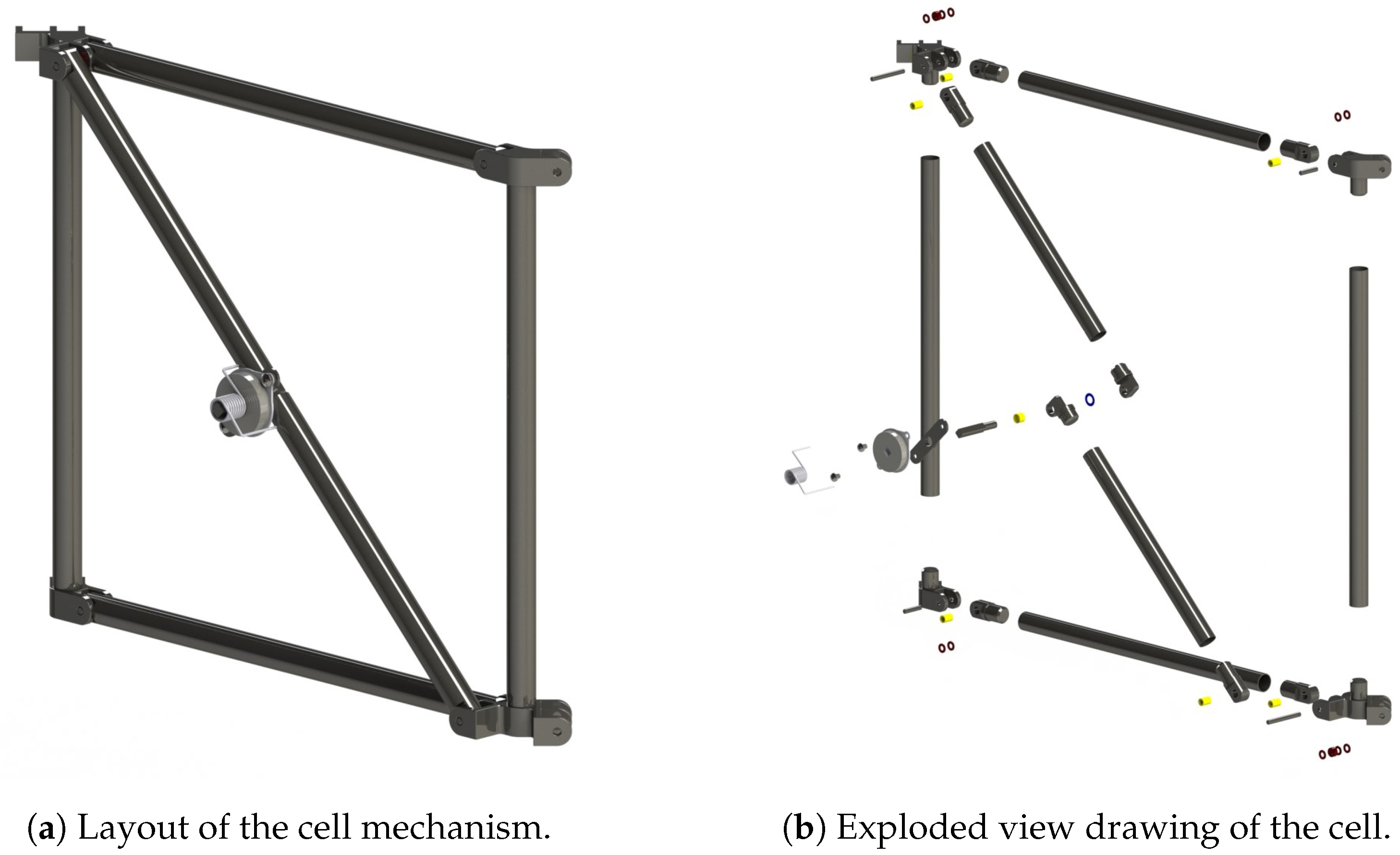
2.2. Elementary Cell and Opening Mechanism
2.3. Dynamic Analysis
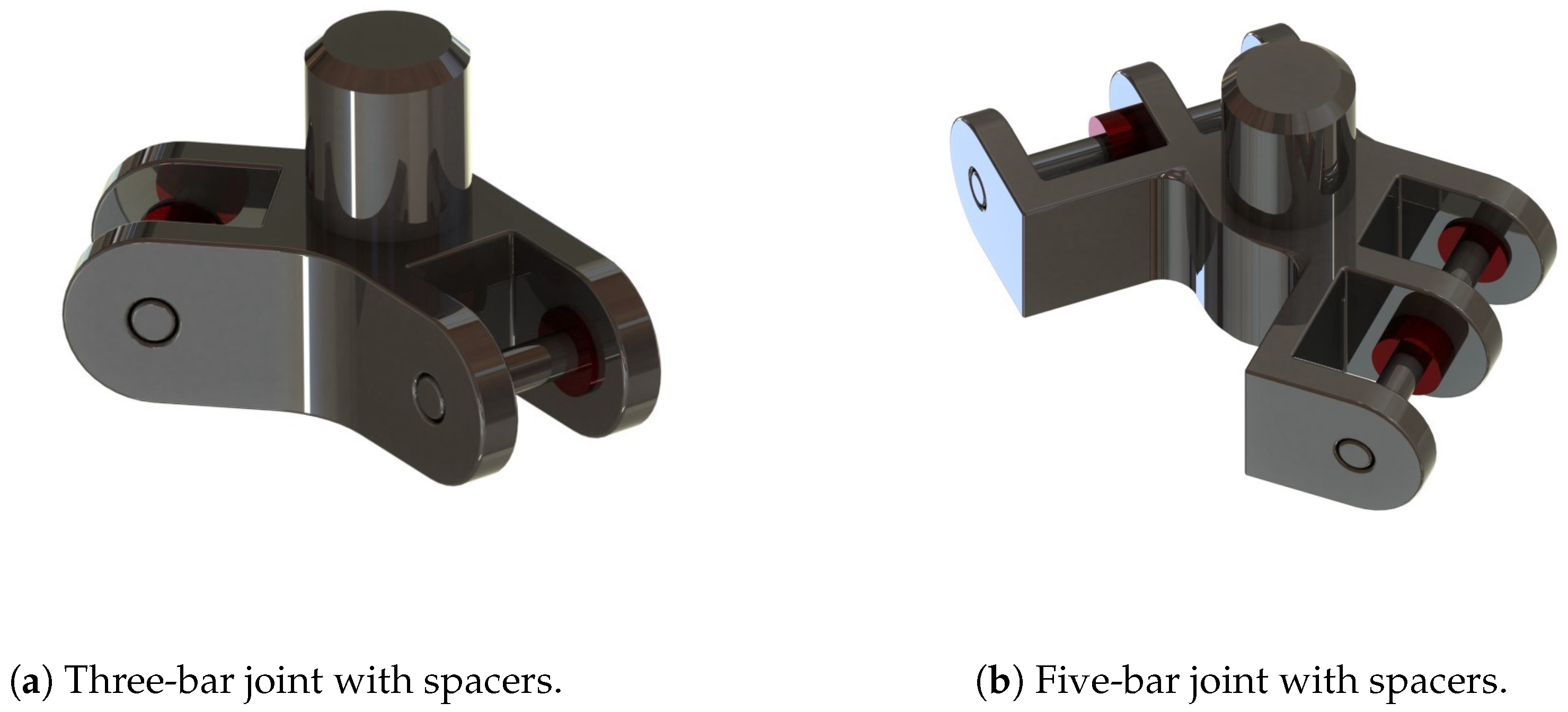
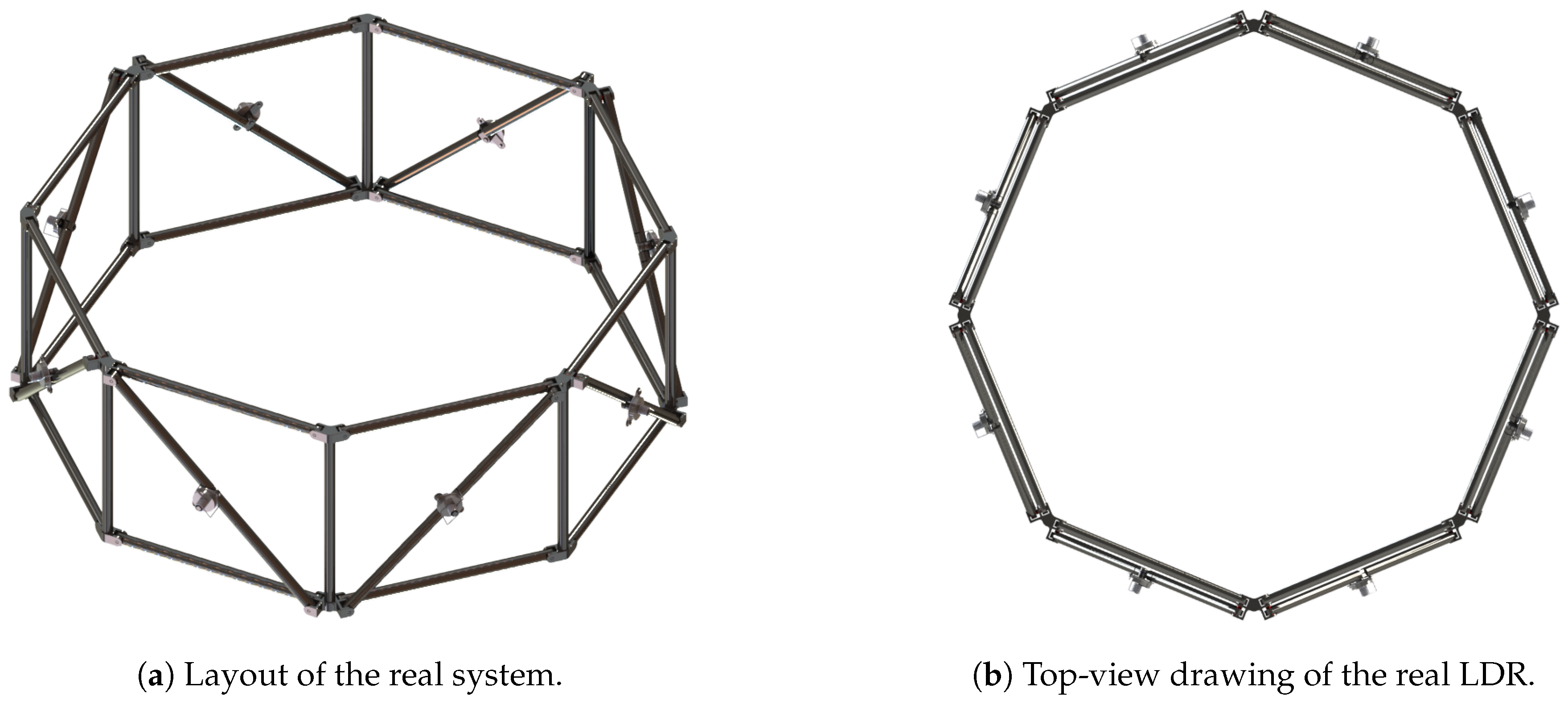
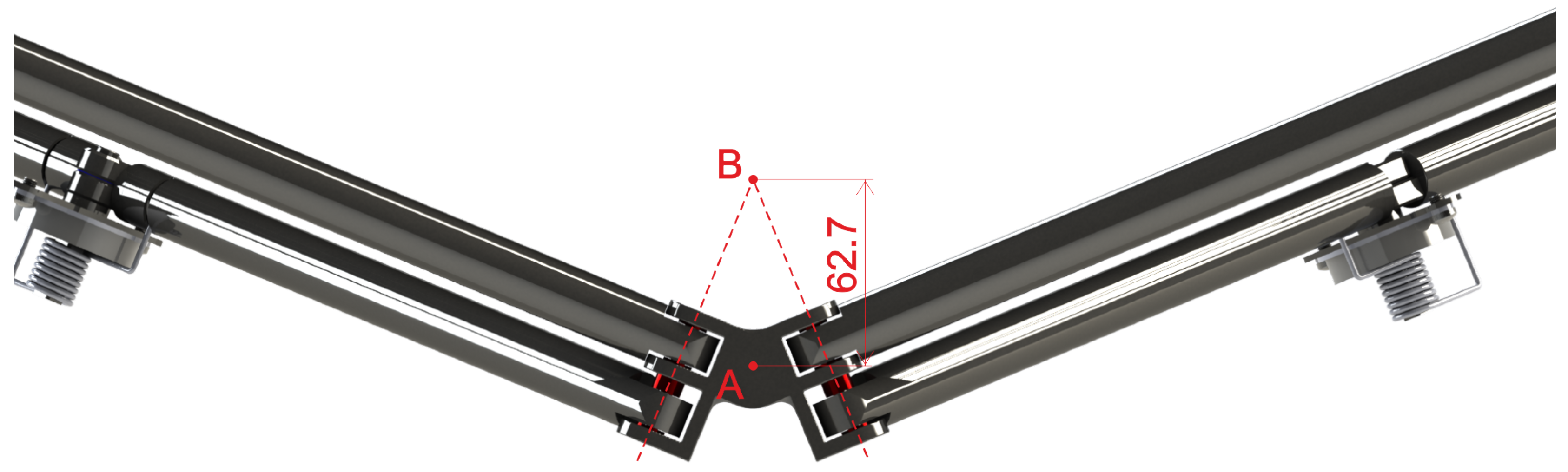
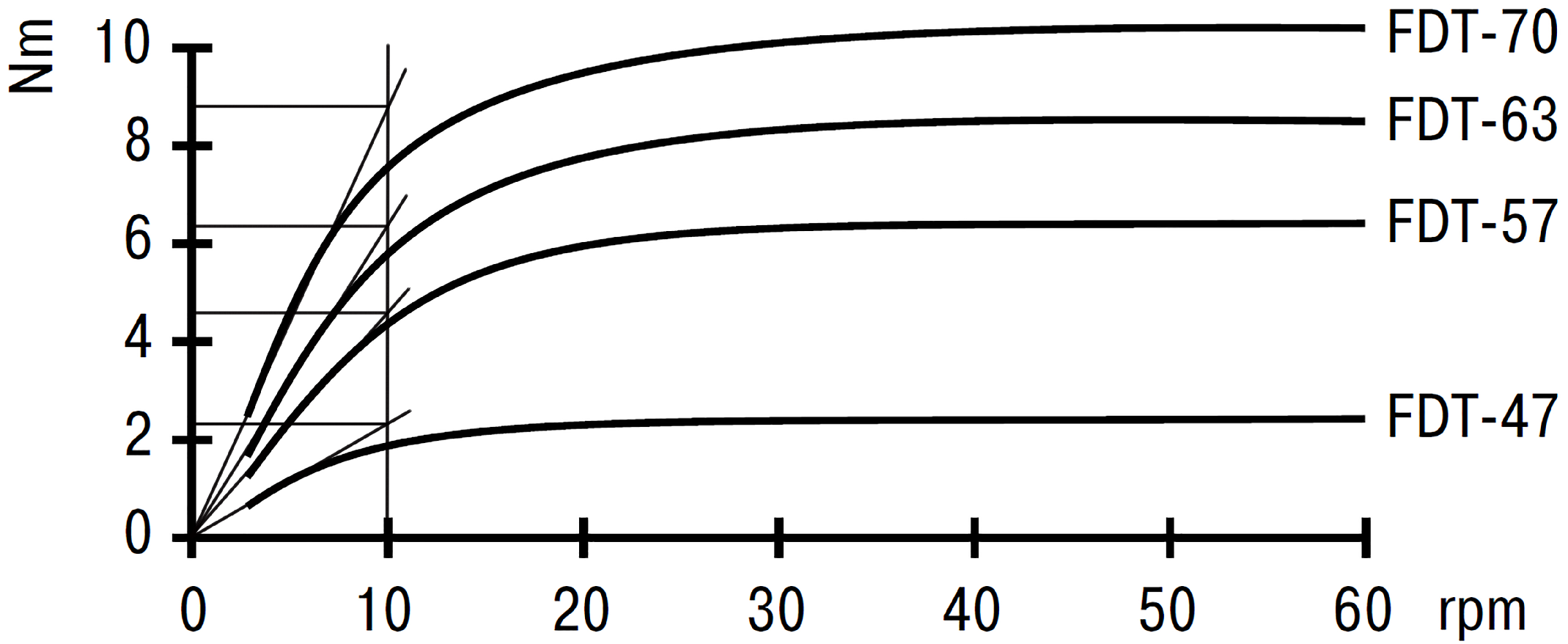
3. Design of the Real System
4. Numerical Simulations
4.1. Contact Model
4.2. Friction Model
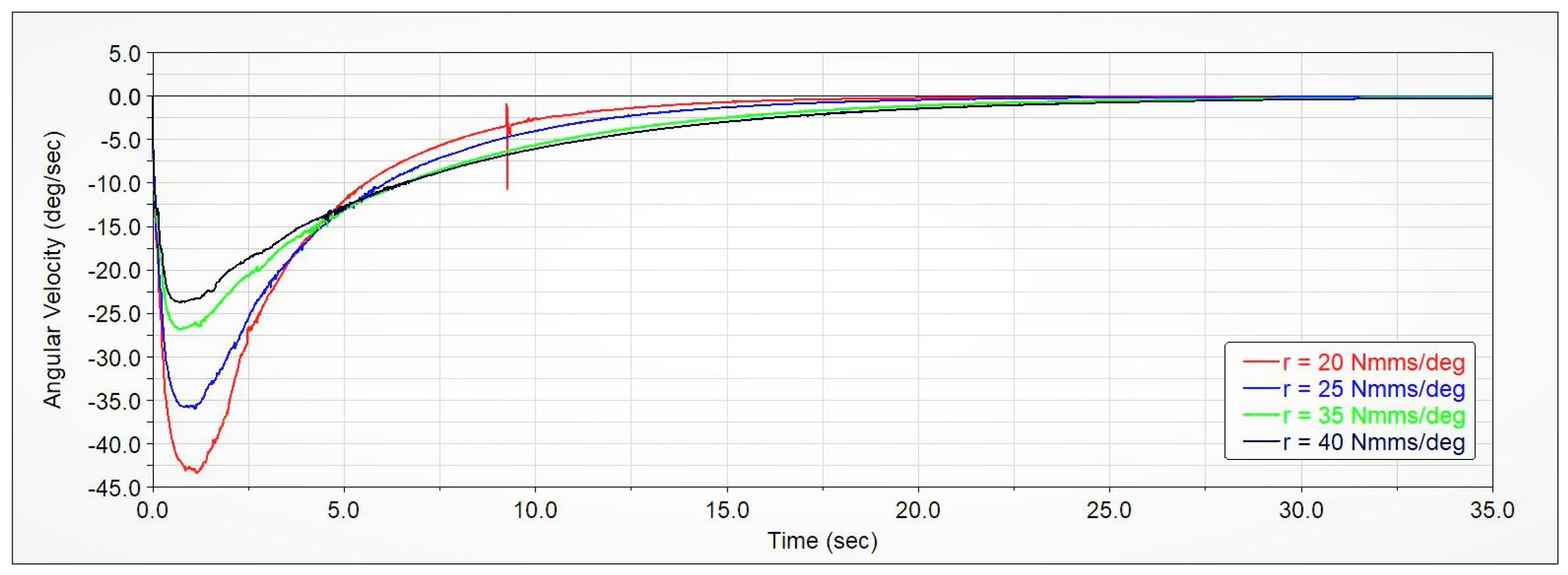
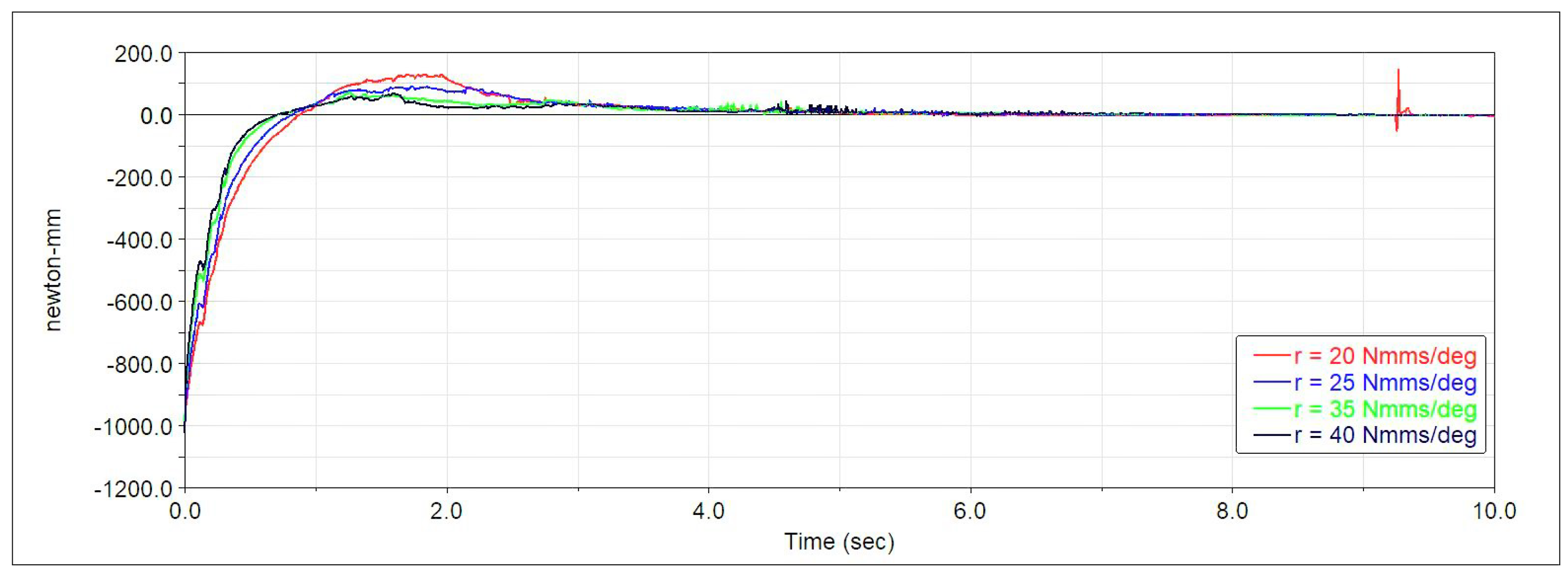
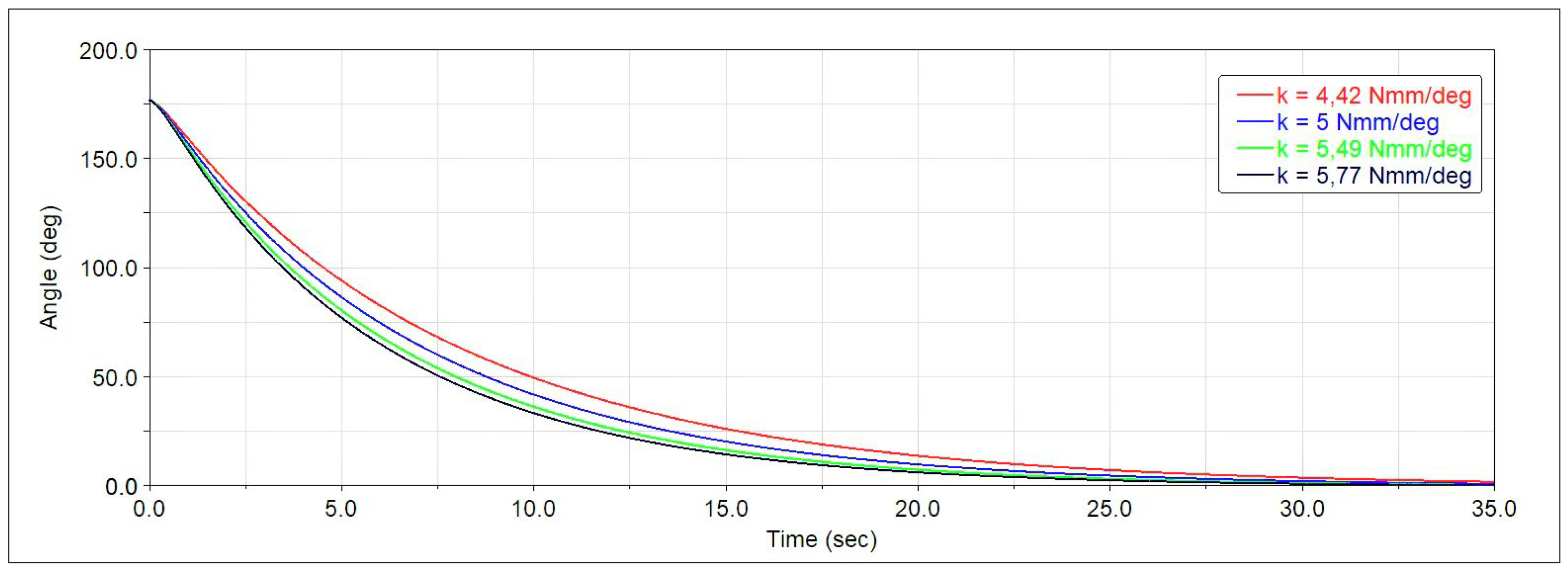
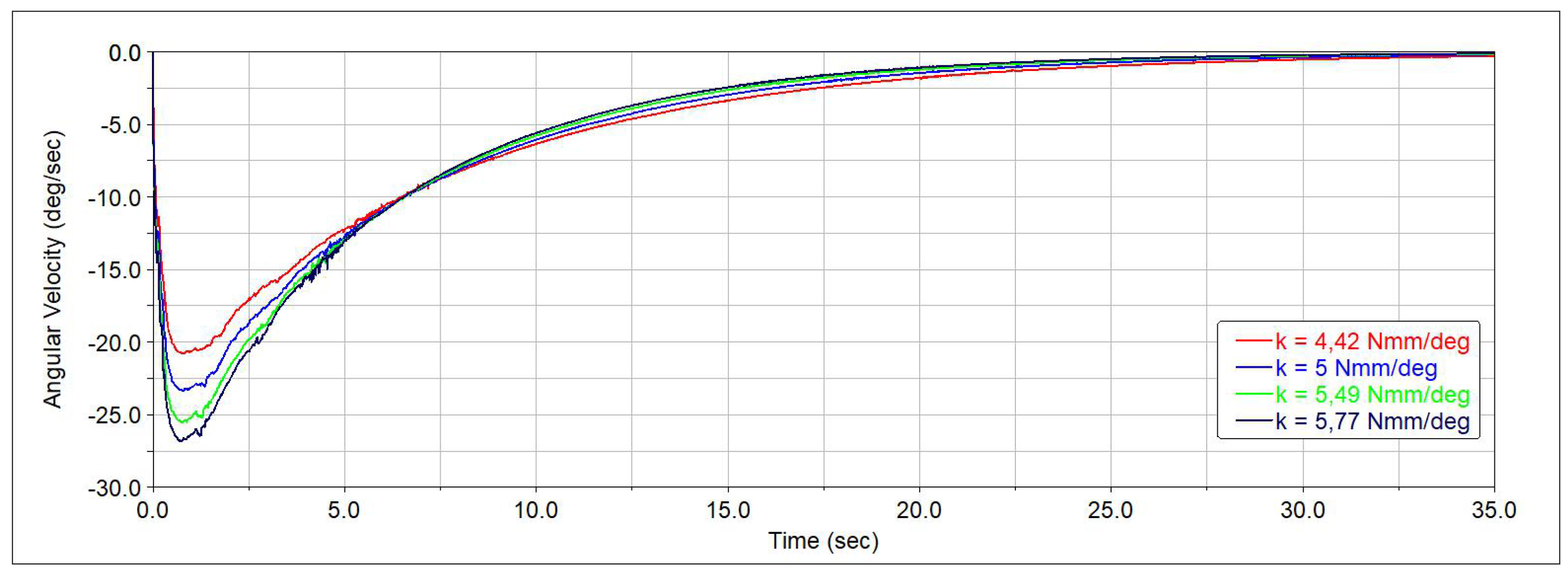
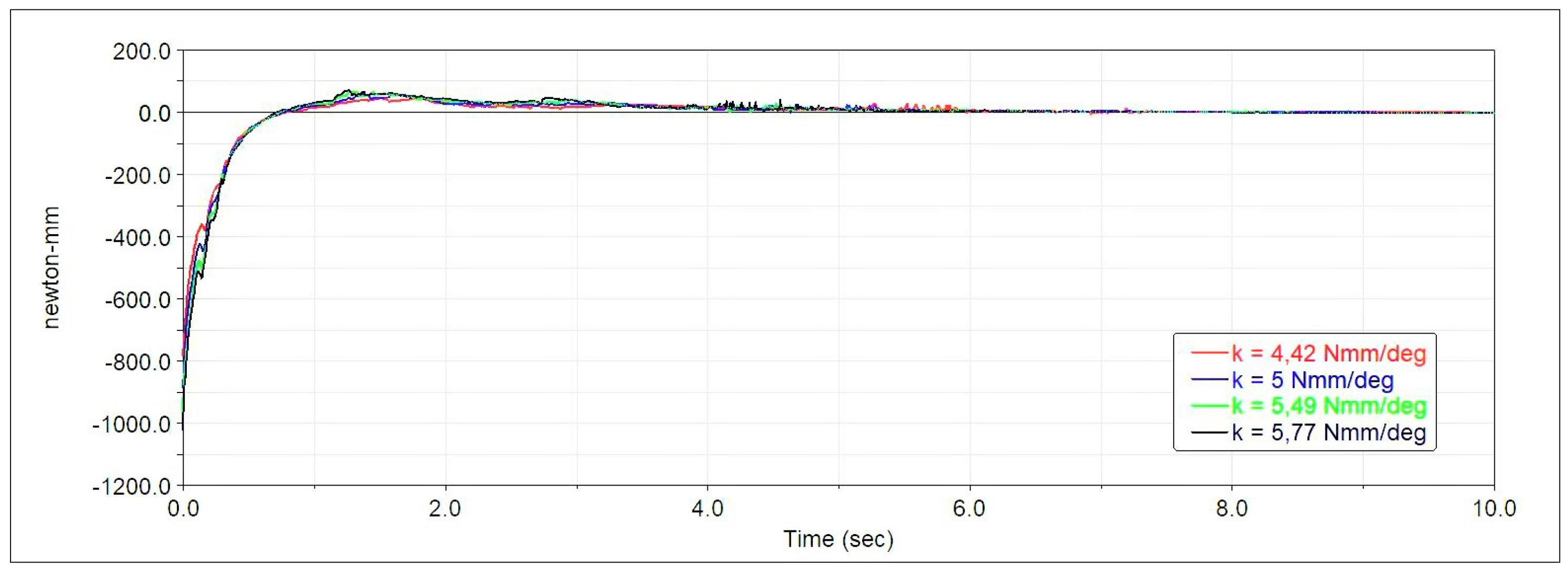
4.3. Spring-Damper System
- the curve of the opening angle becomes flat: the mechanism opens more slowly and therefore within a longer time,
- the angular velocity decreases, and
- the initial slope of the torque increases while the overshoot decreases.
- the curve of the opening angle becomes sharp,
- the angular velocity increases, and
- the initial slope of the torque decreases while the overshoot increases.
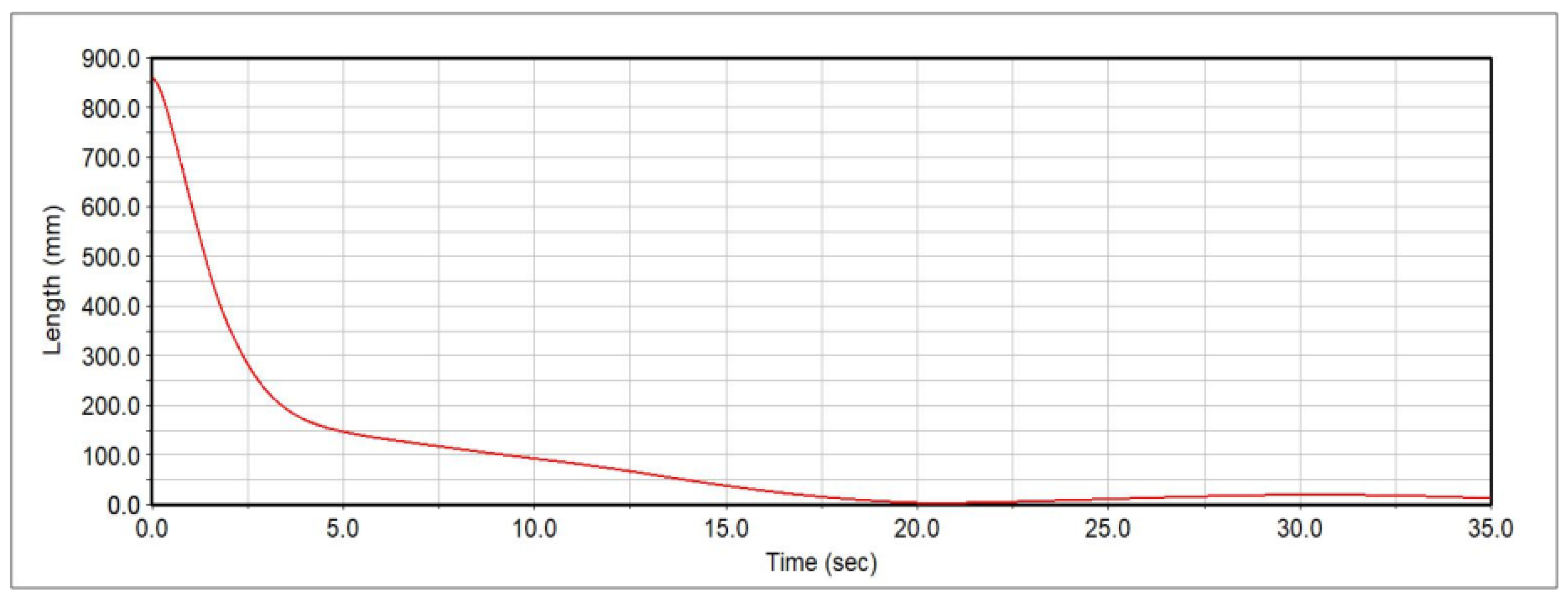
4.4. Deployment Time
5. Conclusions
Author Contributions
Acknowledgments
Conflicts of Interest
Abbreviations
| LDR | Large Deployable Reflector |
References
- Freebury, G.E.; Beidleman, N.J. Deployable Reflector. U.S. Patent 10,256,530 B2, 9 April 2019. [Google Scholar]
- Maddio, P.; Meschini, A.; Sinatra, R.; Cammarata, A. An optimized form-finding method of an asymmetric large deployable reflector. Eng. Struct. 2019, 181, 27–34. [Google Scholar] [CrossRef]
- Henriksen, T.; Mangenot, C. Large deployable antennas. CEAS Space J. 2013, 5, 87–88. [Google Scholar] [CrossRef]
- Freeland, R.; Bilyeu, G.; Veal, G.; Steiner, M.; Carson, D. Large inflatable deployable antenna flight experiment results. Acta Astronaut. 1997, 41, 267–277. [Google Scholar] [CrossRef]
- Morterolle, S.; Maurin, B.; Quirant, J.; Dupuy, C. Numerical form-finding of geotensoid tension truss for mesh reflector. Acta Astronaut. 2012, 76, 154–163. [Google Scholar] [CrossRef]
- Thomson, M. AstroMesh deployable reflectors for ku and ka band commercial satellites. In Proceedings of the 20th AIAA international communication satellite systems conference and exhibit, Montreal, QC, Canada, 12–15 May 2002. [Google Scholar]
- Mobrem, M.; Kuehn, S.; Spier, C.; Slimko, E. Design and performance of astromesh reflector onboard soil moisture active passive spacecraft. In Proceedings of the 2012 IEEE Aerospace Conference, Big Sky, MT, USA, 3–10 March 2012. [Google Scholar]
- Ryan, R. ADAMS—Multibody system analysis software. In Multibody Systems Handbook; Springer: Berlin/Heidelberg, Germany, 1990; pp. 361–402. [Google Scholar]
- Pappalardo, C.M.; Guida, D. A time-domain system identification numerical procedure for obtaining linear dynamical models of multibody mechanical systems. Arch. Appl. Mech. 2018, 88, 1325–1347. [Google Scholar] [CrossRef]
- Pappalardo, C.M. A natural absolute coordinate formulation for the kinematic and dynamic analysis of rigid multibody systems. Nonlinear Dyn. 2015, 81, 1841–1869. [Google Scholar] [CrossRef]
- Cammarata, A. Optimized design of a large-workspace 2-DOF parallel robot for solar tracking systems. Mech. Mach. Theory 2015, 83, 175–186. [Google Scholar] [CrossRef]
- Barbagallo, R.; Sequenzia, G.; Cammarata, A.; Oliveri, S. An integrated approach to design an innovative motorcycle rear suspension with eccentric mechanism. In Advances on Mechanics, Design Engineering and Manufacturing; Springer: New York, NY, USA, 2017; pp. 609–619. [Google Scholar]
- Callegari, M.; Cammarata, A.; Gabrielli, A.; Sinatra, R. Kinematics and dynamics of a 3-CRU spherical parallel robot. In Proceedings of the ASME 2007 International Design Engineering Technical Conferences and Computers and Information in Engineering Conference, Las Vegas, NV, USA, 4–7 September 2007. [Google Scholar]
- Cammarata, A.; Sinatra, R. On the elastostatics of spherical parallel machines with curved links. In Recent Advances in Mechanism Design for Robotics; Springer: New York, NY, USA, 2015; pp. 347–356. [Google Scholar]
- Cammarata, A.; Caliò, I.; D’Urso, D.; Greco, A.; Lacagnina, M.; Fichera, G. Dynamic stiffness model of spherical parallel robots. J. Sound Vib. 2016, 384, 312–324. [Google Scholar] [CrossRef]
- Villecco, F. On the evaluation of errors in the virtual design of mechanical systems. Machines 2018, 6, 36. [Google Scholar] [CrossRef]
- Cammarata, A.; Lacagnina, M.; Sinatra, R. Closed-form solutions for the inverse kinematics of the Agile Eye with constraint errors on the revolute joint axes. In Proceedings of the 2016 IEEE/RSJ International Conference on Intelligent Robots and Systems, Daejeon, Korea, 9–14 October 2016. [Google Scholar]
- Cammarata, A. A novel method to determine position and orientation errors in clearance-affected overconstrained mechanisms. Mech. Mach. Theory 2017, 118, 247–264. [Google Scholar] [CrossRef]
- Giesbers, J. Contact Mechanics in MSC Adams-A technical evaluation of the contact models in multibody dynamics software MSC Adams. Bachelor’s Thesis, University of Twente, Enschede, The Netherlands, 2012. [Google Scholar]
- Guida, D.; Pappalardo, C.M. Control design of an active suspension system for a quarter-car model with hysteresis. J. Vibr. Eng. Technol. 2015, 3, 277–299. [Google Scholar]
- Pirrotta, S.; Sinatra, R.; Meschini, A. A novel simulation model for ring type ultrasonic motor. Meccanica 2007, 42, 127–139. [Google Scholar] [CrossRef]
- Pappalardo, C.M.; Guida, D. Adjoint-based optimization procedure for active vibration control of nonlinear mechanical systems. J. Dyn. Syst. Meas. Contr. 2017, 139, 081010. [Google Scholar] [CrossRef]
- Negrut, D.; Harris, B. ADAMS Theory in a Nutshell. Available online: https://www.me.ua.edu/me364/Student_Version/Digital_Appendix/adamsUofM.pdf (accessed on 13 February 2020).
- Pappalardo, C.M.; Guida, D. System Identification Algorithm for Computing the Modal Parameters of Linear Mechanical Systems. Machines 2018, 6, 12. [Google Scholar] [CrossRef]
- Cavacece, M.; Pennestri, E.; Sinatra, R. Experiences in teaching multibody dynamics. Multibody Sys. Dyn. 2005, 13, 363–369. [Google Scholar] [CrossRef]
- Barbagallo, R.; Sequenzia, G.; Oliveri, S.; Cammarata, A. Dynamics of a high-performance motorcycle by an advanced multibody/control co-simulation. Proc. Inst. Mech. Eng. Part K J. Multibody Dyn. 2016, 230, 207–221. [Google Scholar] [CrossRef]

© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cammarata, A.; Sinatra, R.; Rigano, A.; Lombardo, M.; Maddio, P.D. Design of a Large Deployable Reflector Opening System. Machines 2020, 8, 7. https://doi.org/10.3390/machines8010007
Cammarata A, Sinatra R, Rigano A, Lombardo M, Maddio PD. Design of a Large Deployable Reflector Opening System. Machines. 2020; 8(1):7. https://doi.org/10.3390/machines8010007
Chicago/Turabian StyleCammarata, Alessandro, Rosario Sinatra, Alessio Rigano, Mattia Lombardo, and Pietro Davide Maddio. 2020. "Design of a Large Deployable Reflector Opening System" Machines 8, no. 1: 7. https://doi.org/10.3390/machines8010007
APA StyleCammarata, A., Sinatra, R., Rigano, A., Lombardo, M., & Maddio, P. D. (2020). Design of a Large Deployable Reflector Opening System. Machines, 8(1), 7. https://doi.org/10.3390/machines8010007




