Design and Development of a New Press for Grape Marc

Abstract

The purpose of this research was to determine the optimal geometry of a variable pitch conical helicoid to be used in a pressing machine for grape pomace, also known as grape marc. This study attempted to understand if the optimized geometry of the considered helicoid after every pitch resulted in volume decrease DV_c, equal to that obtained during the pressing phase of grape pomace DV_p, using an optimized membrane press. The conical helicoid with variable pitch was replaced in a machine that offered continuous pressing of grape pomace using a cylindrical helicoid with constant pitch (constant pressure distribution, not optimized, along the cochlea axis). As this was a machine already available in the market, the overall dimensions were already established—5.95 m in length and 1.5 m in width. The pressure distribution p₁ and volume change DV_p, obtained during the grape pomace pressing phase in the optimized membrane press (producing high-quality wine) was taken into consideration in this research. Furthermore, the optimized pressure distribution p₁ was applied in seven phases during the pressing process, and a consequent volume change value DV_p was obtained for each phase. Therefore, this study determined the geometry of the variable pitch conical helicoid, which, after every pitch, resulted in volume changing DV_c that was similar to the volume changing DV_p obtained by the optimized membrane press. For this scope, calculations were realized using the Mathematica 10 program code, which, on being assigned the overall dimensions, slope angle of the helicoid, and volume for the first pitch value, determined the radius and pitch values of the helicoid, total volume, and volume change DV_c. It was also noted that by appropriately varying the geometric parameters (taper and pitch of the helicoid), different options of pressure distribution on grape pomace can be obtained, thus enabling improvement and optimization of product quality.

1. Introduction

In the wine production process, specialized machines are used to extract wine that is relatively virgin or fermented grape pomace [1,2,3]. The pressing operation is aimed at extracting, by mean pressure, the juice retained in grape pomace. Different devices called presses are used in the process; they function on a continuous or discontinuous mechanism. The pressing is performed by gradually increasing pressure, alternating with pauses such that the juice is extracted from the mass and skin until exhausted. To obtain a high-quality product, the solid part of the grapes has to maintain its integrity. There are various types of pressing machines widely used; they include the membrane press, which has produced good results. Although optimized pressing processes are in use, most of them employ a discontinuous process, which is not favored in the industrial ambit.

A continuous process is a method that is used to produce the greatest quantity in the shortest possible time. A continuous process is constant and uninterrupted, in contrast to the batch method, wherein a batch (determined quantity) is subjected to a process that needs completion before the subsequent one is initiated. A continuous process plant is designed such that there is uninterrupted flow of the product from one machine to another throughout the production process. Complex software and equipment programs monitor the process and regulate the flow. The production phases in continuous manufacturing follow one after the other, without interruptions, with shorter production times, without intermediate storage, lower production costs, and greater flexibility. This offers remarkable economic and technical benefits.

Presses that operate continuously are commercially available; however, they have not been optimized. They are able to obtain complete exhaustion of the skins, while respecting the peel and the stalk. A large loading hopper is constructed with perforated stainless steel sheets for separation of the must. This type of apparatus essentially consists of a perforated cylindrical compression chamber, fitted at one end with a feeding hopper and at the other with a hinged door, held in place with a counterweight. An Archimedes’ screw with a constant pitch ensures compression of the grape pomaces. Unfortunately, these types of presses are not optimized because they use cylindrical cochlea with constant pitch that allows only a constant pressure distribution during the pressing phase. Nevertheless, interest in this type of machine is still high because they are able to perform the pressing process in a truly continuous way, which is what the winemaking industry seeks. However, a disastrous flaw of these machines is that it does not allow the control and management of the pressure at which a given volume of pressed grape pomace is submitted, or pressure distribution along the cochlea axis.

Previous studies on different type of cochlea [4,5,6,7,8,9] and the effect of particle shapes [10,11,12,13] have been taken into consideration. These studies have investigated the effect on total mass flow rate, mass flow rate distribution from different regions of the hopper [4,14,15,16,17], draw down patterns and power consumption arising from a conical helicoidal screw with variable pitch [18,19,20,21]. The screws in these studies cover a wide range of commonly found designs, including variations in outer helicoid diameter, inner core taper and screw pitch spacing, [22,23,24,25,26], as reported in

Table 1. Solutions proposed to perform grape pomace pressing.

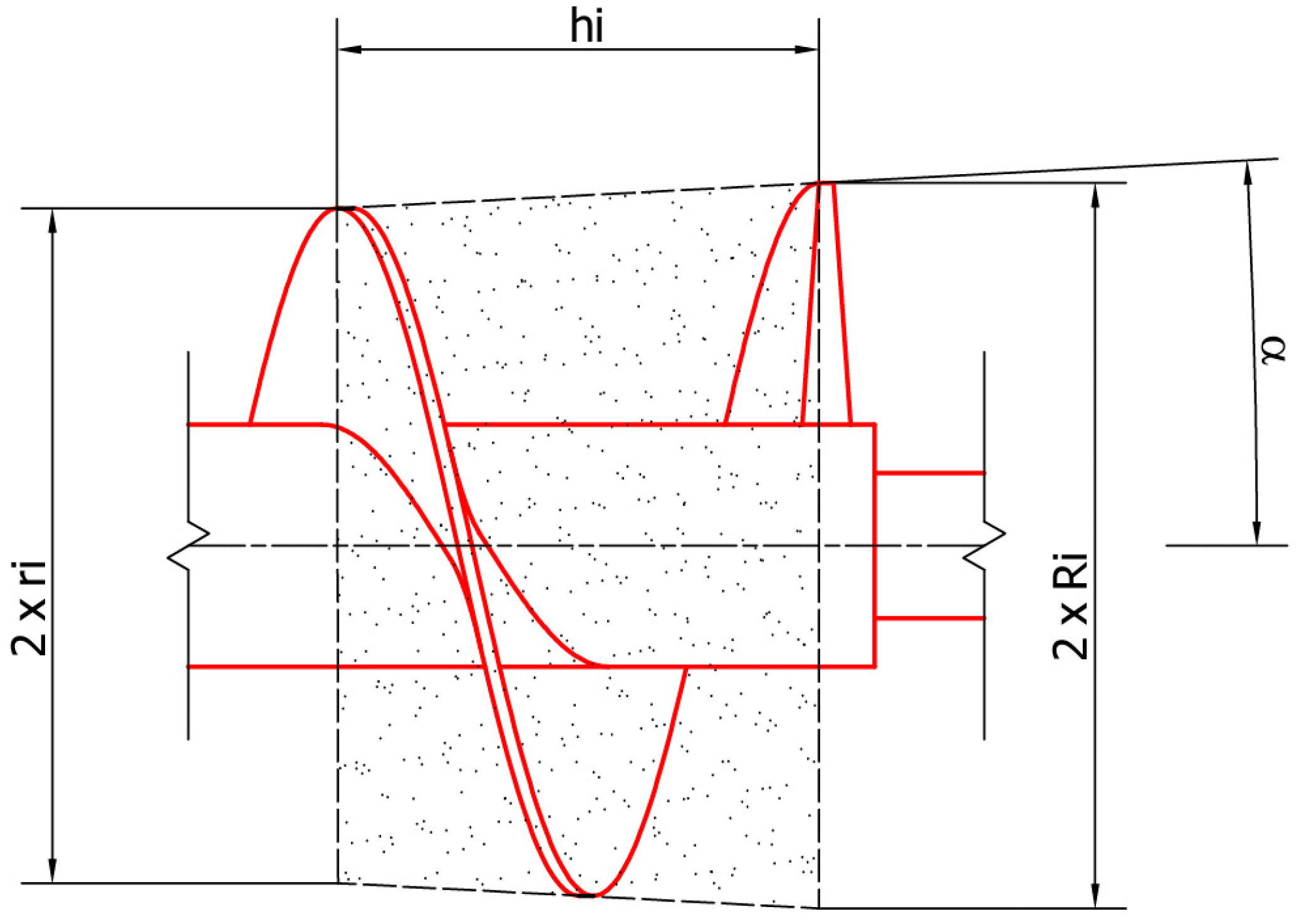
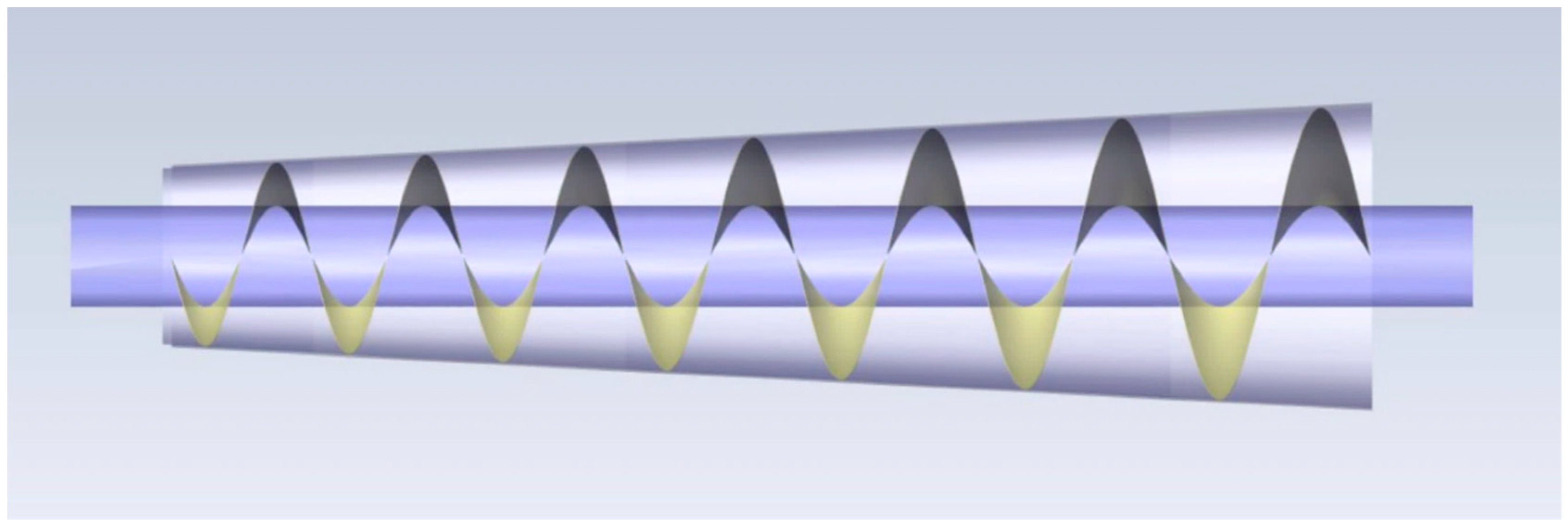
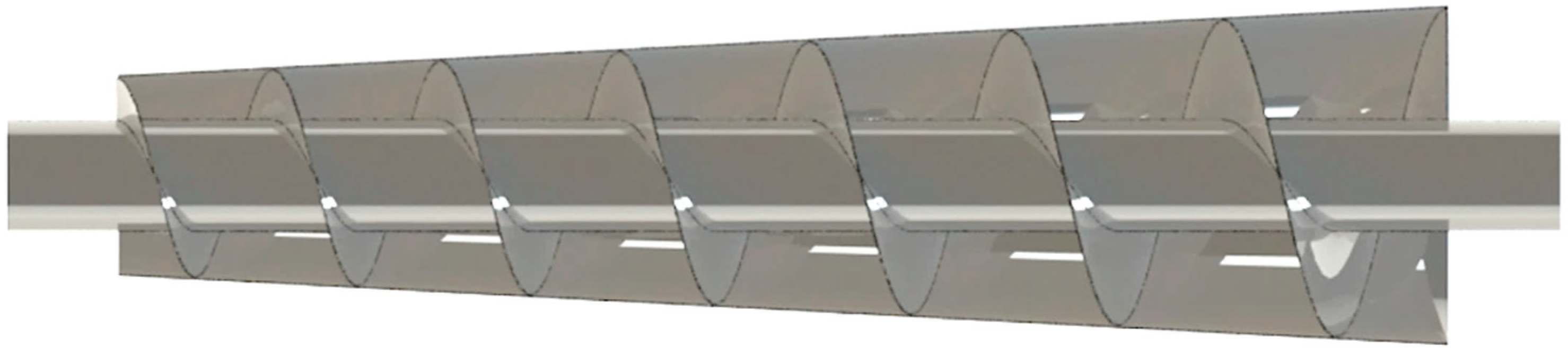
Solutions Proposed	Advantages	Disadvantages
Membrane press	Optimized pressing process	Non-continuous process
Press with Archimedes’ screw	Continuous process	Non-optimized pressing process
Press with conical helicoid with variable pitch	Continuous process optimized pressing process	More studies to optimize the pressing process for different types of marc

.

This research, therefore, seeks to optimize an existing continuous press by replacing the cylindrical helicoid (with a constant pitch) in the machine considered with a designed variable pitch conical helicoid that allows the researchers to obtain, after every pitch, the same volume changing DV_P obtained for the optimized membrane presses—a variable pitch conical helicoid that obtains volume change DV_c along the axis of the cochlea in an optimized membrane press, thus improving the quality of the product obtained. This research identified a variable pitch conical helicoid, which is able to perform the pressing process in a continuous and optimized way [8,27,28,29].

2. Materials and Methods

In this paper, a variable pitch conical screw that is able to carry out the extracting process of grape pomace liquid, similar to that of an optimized membrane press, is studied. The study seeks to realize, through the use of a variable pitch conical screw, volume change DV_c after each pitch, equal to volume change DV_p realized by optimized membrane presses, in the corresponding pressing phase. As a result, the variable pitch conical screw realizes the same liquid phase extraction process performed with an optimized membrane press. The membrane presses realize the extracting process in phases, with pressure increases for each phase not exceeding 0.20–0.30 bar, performing a drainage DV_p of the liquid phase, following the scheme reported in

Table 2. Optimized pressure distribution p₁ and consequent volume reduction DV_p detected, % respect to the initial value V₀, during pressing process performed with membrane press.

n. phase	p1 (105 Pa)	DVp (%)
1	0.20	4
2	0.40	16
3	0.80	26
4	1.20	34
5	1.50	39
6	1.80	45
7	2.0	51

.

Therefore, a variable pitch conical screw was designed, which aimed to replace a constant pitch cylindrical screw in a continuous press machine. The objective of this step is reduction in volume DV_c after each round of the screw (with variable pitch). Further, a hypothesis is made that this volume change that the mass of grape pomace undergoes is equal to the drained liquid phase; therefore DV_p is the volume of liquid extracted.

The conical helicoid screw system with variable pitch induces a volume change, which depends on the geometry of the helicoidal surface considered [13,30,31,32]. Therefore, the study of helicoidal surfaces interacting with the grape pomace volume, as well as how they change along the axis of the machine, is important and necessary [14,33,34,35,36]. Further, we have hypothesized that the product DV_p is constant for each cone volume considered, after each variable pitch value. Indeed, it is necessary to evaluate the characteristic geometric parameters of the variable pitch conical screw, such that the volume change DV_c after each pitch value is equal to DV_p detected for the optimized membrane press.

2.1. Experimental Tests

For the pressing process of grape pomace, optimized pressure distribution p₁ and consequent volume changing DV_p performed during the pressing process with membrane press is considered. They are generally used during pressing done with a pneumatic press, which has a seven-phase process. The optimized pressure distribution and consequent volume change DV_p for each considered phase is reported in Table 2, and have been considered in determining the geometry of the conical helicoid with variable pitch. The geometrical shape of the conical helicoid that produced the volume change equal to DV_p for the considered phases of 1, 2, 3, 4, 5, 6, 7 had to be determined. We assumed that each considered phase is representative of a cochlea portion after pitch value h_i.

The bulk density of the considered grape pomace was around 500 kg m⁻³ and initial humidity was around 60%.

2.2. Helicoidal Screw Press Geometry

The proposed structure includes a central frame with suction and delivery connections obtained at the opposite ends, which enclose the helical screw. During the working process, the grape pomaces are moved to the opposite end along the external cavities between the screws and the frame. This helps avoid noise and pulsation. The main components are: the “rotor”, a metal conical helicoid screw that rotates inside the “stator”, a fixed part, creating a hermetic contact line between the two elements that allows the displacement of the grape pomace. As the elementary volume decreases, it is subjected to pressure that increases in proportion to the volume decrease.

The grape pomaces were contained in cylindrical volume with diameter of 1.50 m and height of 1 m; the initial volume V₀ = 1.766 m³.

The volume enclosed by the conical cochlea with variable pitch, after the distance equal to pitch h_i, is given by the initial value of the cochlea height (R_i) and the value of the cochlea height (r_i) after pitch hi (Figure 1); whereby the volume enclosed by the cochlea tract considered after pitch h is the one enclosed in the truncated cone, if R = r_i; r = r_i

$$V=\frac{\pi h}{3}\left(R^2+Rr+r^2\right)$$

(1)

where V is the volume enclosed by the conical cochlea after pitch h. The volume of the cochlea central axle must be subtracted from it. Further, if we consider the cross-section of the cochlea, considering only the cochlea radius initial and final for every segment h_i, we can note a succession of isosceles trapezius in which 2R is the major basis value, and 2r is the minor basis value. Further, the minor basis of a previous trapezius is the major basis of the following trapezius, for which we can write:

$$R_i=r_{i-1}$$

(2)

The initial volume V₀ and liquid volume extract DV is known, because we suppose that the volume extract is equal to the volume reduction for each phase considered; for every phase i, volume V_i is:

$$V_i=\frac{\pi h_i}{3}\left(R_i{}^2+R_i{r}_i+r_i{}^2\right)$$

(3)

The angle α formed by the cochlea axis and oblique side of the isosceles trapezius is given by:

$$tan\alpha =\frac{\left(\frac{R_i-r_i}{2}\right)}{h_i}$$

(4)

For the following isosceles trapezius, after the first one, the unknown variables are r_i and h_i, which are determined by solving Formulae (3) and (4) and considering the other parameters examined. Taking into account the fact that we intend to replace the constant pitch cylindrical helicoid (not optimized) installed in the press machine with the optimized variable pitch conical helicoid screw, we are required to respect the overall dimensions. This resulted in a conical cochlea with variable pitch 5.95 m long and external maximum radius of 1.5 m.

The variable pitch conical helicoid allows submission of the grape pomace to variable pressures, so as to be able to realize the pressing process by applying an optimized pressure distribution. This kind of pressure distribution has been detected in membrane presses. Therefore, the overall dimensions of the machine to be optimized have been used. The following are known: the initial value of the input section and therefore the value of the major base R₁ of the first isosceles trapezoid, the value of the height of the trapezoid, which is equal to step h₁ = 1 m. Thus, we need to find out α ed r₁. Once the initial volume V₁, which is related to the capacity of the machine, is set based on Formula (3), we can calculate r₁, since R₁ and h₁ are known, and α is obtained from Formula (4).

Subsequently we can proceed assuming α to be constant and then imposing the same volume decrease for the optimized process considered. The volume of the next cell is known, and therefore, it is possible to calculate the subsequent new h_i and r_i values, considering the same value of α. Keep in mind that the current R_i value is equal to the r_i value of the previous trapezoid.

Therefore, the testing conditions are: V₁ = 1.72 m³; h₁ = 1 m; R₁ = 0.75 m; α = 3°. It is also possible to assign different α values. As a result of the above analysis, a program code realized in Mathematica was set up to determine the characteristic geometric parameters of the optimized conical helicoid with variable pitch. As first pitch value, h₁ = 1 m was considered. The program code was able to evaluate, for every assigned angle α and pitch value, the volume values V_i of each sector of the cochlea considered. V_i value is reduced with respect to the V₀ value of quantity DV_c (%), which is compared with the optimized volume changing DV_p reported in Table 2. The evaluation was stopped when the differences were minimum, and the obtained values were angle α = 3° and pitch decrease of 5 cm along the cochlea axis. In

Table 3. Pitches and radius helicoid values determined.

h	Pitch (m)	i	Radius Ri (m)
1	1	1	0.75
2	0.95	2	0.73
3	0.90	3	0.70
4	0.85	4	0.68
5	0.80	5	0.67
6	0.75	6	0.66
7	0.70	7	0.65
		8	0.64

, the determined pitches and radius values are reported.

Table 4. DV_c and DV_p volume changing evaluated, in % respect to the initial value V₀, respectively, for the cochlea after every pitch and for the optimized membrane press after every phase.

Cochlea after Pitch h	DVc %	Membrane Press Phase	Optimized Volume Changing DVp %
h1	5	1	4
h2	14	2	16
h3	24	3	26
h4	32	4	34
h5	39	5	39
h6	47	6	45
h7	51	7	51

reported the DV_c volume decrease, after every pitch h_i, evaluated with respect to initial volume V₀. These DV_c values obtained have been compared with the DV_p values obtained with an optimized membrane press after every phase [37,38,39,40]. The maximum percentual difference was around 2%.

It was, thus, possible to design an optimized cochlea and identify volume changing DV_c after every pitch, similar to DV_p realized by an optimized membrane press. The optimized cochlea is reported in Figure 2 and Figure 3 [41,42,43].

3. Discussion

Table 4 notes that with the considered geometry for the variable pitch conical helicoid, the DV_c volume change obtained is similar to the DV_p volume change obtained with an optimized membrane press. In this case, we considered a constant α angle. However, it is possible to design another optimized variable pitch conical helicoid for the same optimized pressure distribution by using a variable α angle. In this case, we have chosen to keep angle α constant, because it is a simple solution.

3.1. Specific Energy Consumption

Specific energy consumption is the main technical and economic symbol of machinery equipment. It is the ratio of the driving power of the motor to the production capacity. The specific energy consumption of the screw press machine is:

$$H=\frac{N}{Q}$$

(5)

where H is the specific energy consumption in kilowatt hours per ton, N is the driving power of the motor in kilowatts, and Q is the production capacity in tons per hour. At present, the screw press machine has no formula to calculate production capacity. Since the working principle of the screw press machine is similar to that of the screw conveyor, the formula of the production capacity of the screw conveyor is used to calculate the production capacity of the screw press machine, as given by:

$$Q=3600A_g\nu \gamma$$

(6)

where A_g is the section area of grape pomace in square meters, ν is the conveying velocity of grape pomace in meters per second, γ is the material accumulation density in tons per meter cubed. In the considered case, Q = 12 ton/h, resulting in A_g = 1.2 m²; ν = 0.05 m/s.

3.2. Quality of Wine

The final quality of the wine depends on many other factors and is still under study and experimental tests. However, the organoleptic characteristics of the musts obtained with a continuous press with a variable pitch conical screw, and an optimized membrane press were similar. However, the strategic and important fact remains that the variable pitch conical screw press performs a continuous process, while the optimized membrane press performs a non-continuous process, and this makes the comparison of consumption between the two types of machines viable in the second order, which was not carried out.

4. Conclusions

In this study, the geometry of a variable pitch conical helicoid screw to perform the optimized pressing process for grape pomaces was determined. It was found to be able to replace the constant pitch cylindrical helicoid screw installed in a press machine and perform a continuous pressing operation in oenological processes [44,45].

Therefore, the machine thus modified is able to carry out the process of grape pomace pressing in a continuous and optimized way; the optimized cochlea performs a volume changing DV_c after every pitch, equal to the volume changing DV_p realized by an optimized membrane press during the grape pomace pressing phase.

The designed machine is very efficient, from an operational point of view, with high work capacity compared to other optimized machines (operating with the discontinuous method) of equal power already available in the market. The examined geometry of the conical helicoid offers the same volume change as the optimized membrane press, following widely diffused enological regulations. The optimized geometry was determined by program code realized in Mathematica 10. The program continuously compared the calculated DV_c values, for each pitch considered, with the assigned DV_p, obtained by an optimized membrane press. When this difference was found to be less than 5%, the program provided the final results. By means of this program code, the radius and pitch for each phase was obtained. The variable pitch conical helicoid geometry acting on the grape pomace, generating the assigned volume variation DV_p during the operative phase considered, was thus been determined. We considered a constant α angle; however, it is possible to design another optimized variable pitch conical helicoid for the same optimized pressure distribution by using a variable α angle. In this study, we choose to keep the angle α constant because it is a simple solution [46].

Further studies are needed to identify optimal pressure distributions for different grape pomace varieties.