A Novel Modelling Approach for Condensing Boilers Based on Hybrid Dynamical Systems
Abstract
:1. Introduction
- Thermal dynamics are either neglected or oversimplified with a nonlinear efficiency curve followed by two point thermal nodes. It is important to point out that the efficiency curve is always calculated by installers and specifiers of domestic/commercial heating systems, at steady-state. Therefore, it might not be able to represent the dynamics of the boiler.
- The condensing/noncondensing behavior is either neglected or oversimplified with two heat exchangers with a fixed proportion of dry/wet heat exchange. Required is a model that is capable of capturing possible time-varying proportions of dry/wet heat exchange occurring during dynamical behavior.
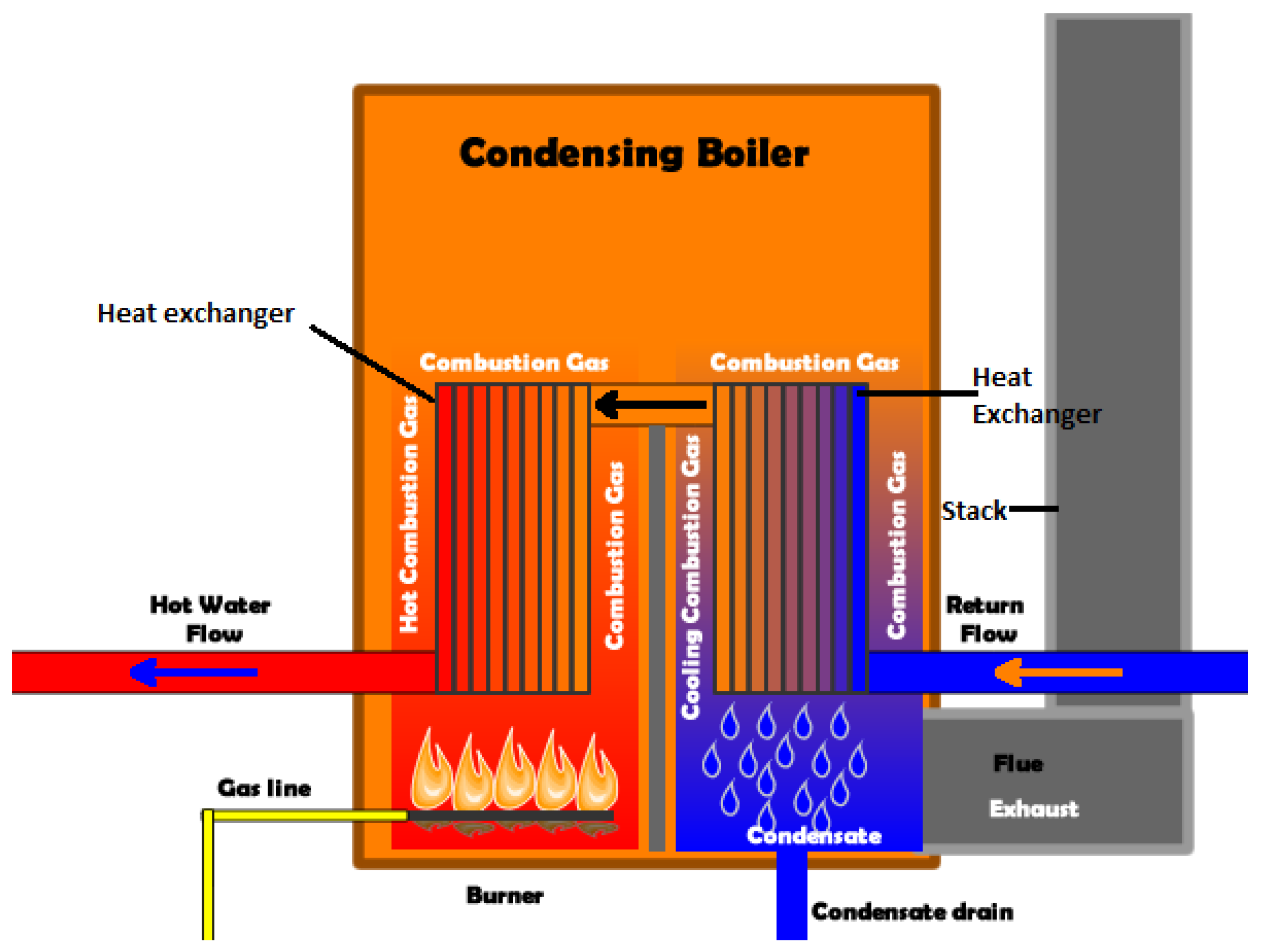
2. Boiler Operation and Involved Heat Exchanges
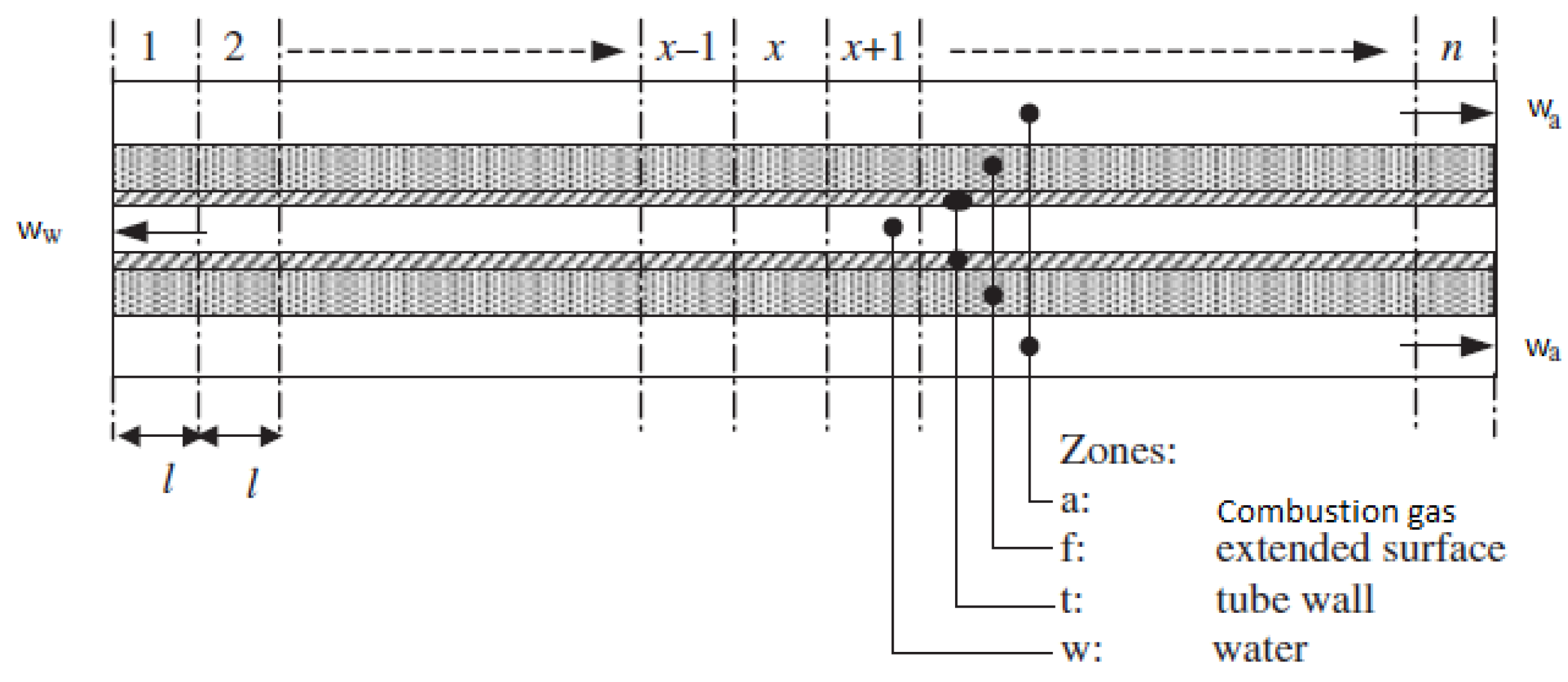
3. Dynamic Thermal Modeling
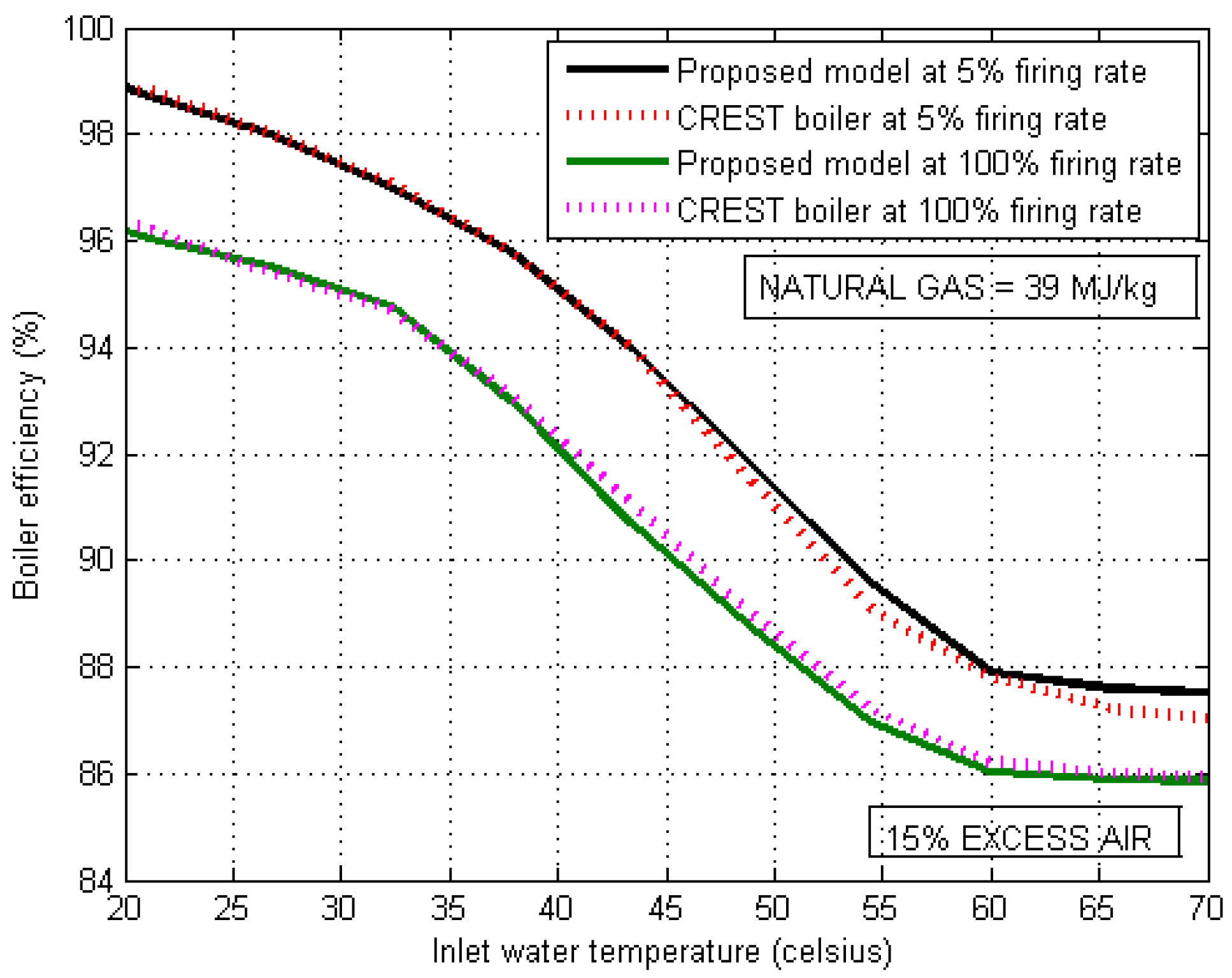
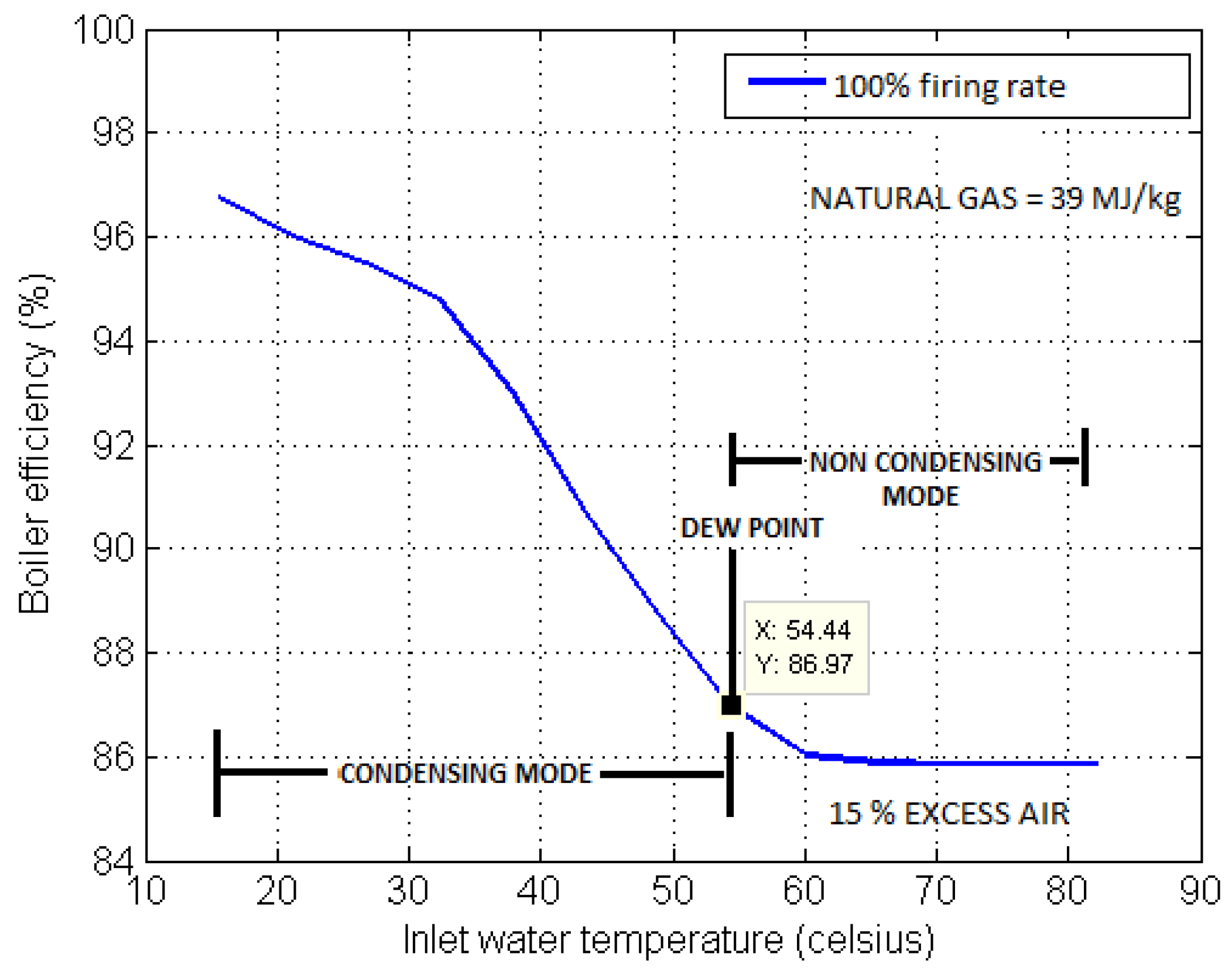
4. Simulation Results and Analysis
5. Conclusions
Author Contributions
Conflicts of Interest
References
- Pérez-Lombard, L.; Ortiz, J.; Pout, C. A review on buildings energy consumption information. Energy Build. 2008, 40, 394–398. [Google Scholar] [CrossRef]
- Cockroft, J.; Samuel, A.; Tuohy, P. Development of a Methodology for the Evaluation of Domestic Heating Controls; Phase 2 of a DEFRA Market Transformation Programme Project, Carried Out Under Contract to BRE Environment; University of Strathclyde, Energy Systems Research Unit: Glasgow, UK, 2007. [Google Scholar]
- Energy Saving Trust. Domestic Heating by Gas: Boiler Systems—Guidance For Installers and Specifiers; Central Heating System Specifications: England, UK, 2008; Available online: http://bpec.org.uk/downloads/CE30%20-%20Domestic%20heating%20by%20gas.pdf (accessed on 5 April 2016).
- Lee, S.; Kum, S.M.; Lee, C.E. Performances of a heat exchanger and pilot boiler for the development of a condensing gas boiler. Energy 2011, 36, 3945–3951. [Google Scholar] [CrossRef]
- Rosa, L.; Tosato, R. Experimental evaluation of seasonal efficiency of condensing boilers. Energy Build. 1990, 14, 237–241. [Google Scholar] [CrossRef]
- Jeong, K.; Kessen, M.J.; Bilirgen, H.; Levy, E.K. Analytical modeling of water condensation in condensing heat exchanger. Int. J. Heat Mass Transf. 2010, 53, 2361–2368. [Google Scholar] [CrossRef]
- Lazzarin, R.M.; Schibuola, L. Performance analysis of heating plants equipped with condensing boilers. Heat Recover. Syst. 1986, 6, 269–276. [Google Scholar] [CrossRef]
- Ternoveanu, A.; Ngendakumana, P. Dynamic model of a hot water boiler. In Proceedings of the Climate 2000 Conference, Brussels, Belgium, 30 August 1997.
- Lemort, V.; Rodriguez, A.; Lebrun, J. Simulation of HVAC Components with the Help of an Equation Solver; Solar Heating and Cooling Programme International Energy Agency: Liege, Belgium, 2008. [Google Scholar]
- Makaire, D.; Ngendakumana, P. Thermal performance of condensing boilers. In Proceedings of the 32nd TLM-IEA Energy Conservation and Emission reduction in Combustion, Nara, Japan, 26–29 July 2010; pp. 1–11.
- Aganovic, A. Analysis of Dynamical Behavior of the Boiler Room at Mechanical Engineering Faculty in Sarajevo in Standard Exploitation Conditions; Department of Energy and Process Engineering: Trondheim, Norway, 2013. [Google Scholar]
- Chen, Q.; Finney, K.; Li, H.; Zhang, X.; Zhou, J.; Sharifi, V.; Swithenbank, J. Condensing boiler applications in the process industry. Energy Build. 2012, 89, 30–36. [Google Scholar] [CrossRef]
- Michailidis, I.; Baldi, S.; Pichler, M.F.; Kosmatopoulos, E.B.; Santiago, J.R. Proactive control for solar energy exploitation: A german high-inertia building case study. Appl. Energy 2015, 155, 409–420. [Google Scholar] [CrossRef]
- Lee, S.; Kum, S.M.; Lee, C.E. An experimental study of a cylindrical multi-hole premixed burner for the development of a condensing gas boiler. Energy 2011, 36, 4150–4157. [Google Scholar] [CrossRef]
- Korkas, C.D.; Baldi, S.; Michailidis, I.; Kosmatopoulos, E.B. Intelligent energy and thermal comfort management in grid-connected microgrids with heterogeneous occupancy schedule. Appl. Energy 2015, 149, 194–203. [Google Scholar] [CrossRef]
- Michailidis, I.; Baldi, S.; Kosmatopoulos, E.B.; Boutalis, Y.S. Optimization-based active techniques for energy efficient building control part II: Real-life experimental results. Available online: http://simonebaldi.my-board.org/wp-content/uploads/2014/06/BEE-RES2014PARTB.pdf (accessed on 5 April 2016).
- Bojica, M.; Dragicevic, S. MILP optimization of energy supply by using a boiler, a condensing turbine and a heat pump. Energy Convers. Manag. 2002, 43, 591–608. [Google Scholar] [CrossRef]
- Baldi, S.; Michailidis, I.; Ravanis, C.; Kosmatopoulos, E.B. Model-based and Model-free “Plug-and-Play” Building Energy Efficient Control. Appl. Energy 2015, 154, 829–841. [Google Scholar] [CrossRef]
- Verhelst, C.; Logist, F.; Van Impe, J.; Helsen, L. Study of the optimal control problem formulation for modulating air-to-water heat pumps connected to a residential floor heating system. Energy Build. 2012, 45, 43–53. [Google Scholar] [CrossRef]
- Baldi, S.; Karagevrekis, A.; Michailidis, I.; Kosmatopoulos, E.B. Joint energy demand and thermal comfort optimization in photovoltaic-equipped interconnected microgrids. Energy Convers. Manag. 2015, 101, 352–363. [Google Scholar] [CrossRef]
- Tzivanidis, C.; Antonopoulos, K.; Gioti, F. Numerical simulation of cooling energy consumption in connection with thermostat operation mode and comfort requirements for the Athens buildings. Appl. Energy 2011, 88, 2871–2884. [Google Scholar] [CrossRef]
- Korkas, C.D.; Baldi, S.; Michailidis, I.; Kosmatopoulos, E.B. Occupancy-based demand response and thermal comfort optimization in microgrids with renewable energy sources and energy storage. Appl. Energy 2016, 163, 93–104. [Google Scholar] [CrossRef]
- Satyavada, H.; Babuska, R.; Baldi, S. Integrated dynamic modelling and multivariable control of HVAC components. In Proceedings of the 15th European Control Conference, Aalborg, Denmark, 29 June–1 July 2016. submitted.
- Underwood, C.; Yik, F. Modelling Methods for Energy in Buildings; Blackwell Publishing Ltd.: Hoboken, NJ, USA, 2004. [Google Scholar]
- BetterBricks. Boilers Introduction How Boilers Work. Available online: http://betterbricks.com/sites/default/files/operations/om_of_boilers_final.pdf (accessed on 29 December 2015).
- Riverside Hydronics. Condensing Boiler Operation and Appropriate Use. Available online: http://www.riversidehydronics.com/pdf%20documents/Tech%20Papers/condensing%20boiler%20operation%20and%20use.pdf (accessed on 29 December 2015).
- Riverside Hydronics. First Law of Thermodynamics Summary. Available online: https://www.phy.duke.edu/rgb/Class/phy51/phy51/node59.html (accessed on 30 December 2015).
- Green Shoots Controls. Available online: http://www.greenshootscontrols.net/?p=153 (accessed on 30 December 2015).
- Lochinvar. CREST Condesing Boiler. Available online: http://www.lochinvar.com/products/default.aspx?type=productline&lineid=177 (accessed on 31 December 2015).

© 2016 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons by Attribution (CC-BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Satyavada, H.; Baldi, S. A Novel Modelling Approach for Condensing Boilers Based on Hybrid Dynamical Systems. Machines 2016, 4, 10. https://doi.org/10.3390/machines4020010
Satyavada H, Baldi S. A Novel Modelling Approach for Condensing Boilers Based on Hybrid Dynamical Systems. Machines. 2016; 4(2):10. https://doi.org/10.3390/machines4020010
Chicago/Turabian StyleSatyavada, Harish, and Simone Baldi. 2016. "A Novel Modelling Approach for Condensing Boilers Based on Hybrid Dynamical Systems" Machines 4, no. 2: 10. https://doi.org/10.3390/machines4020010
APA StyleSatyavada, H., & Baldi, S. (2016). A Novel Modelling Approach for Condensing Boilers Based on Hybrid Dynamical Systems. Machines, 4(2), 10. https://doi.org/10.3390/machines4020010





