Impact of Double-Suction Pump Eye Diameter Variation on Cavitation Phenomena

Abstract

Cavitation phenomena in pumps are major determinants of the lifespan of both the impeller and the pump itself, causing significant vibration and noise, which are critical concerns for pump designers. This study focuses on the influence of various geometric factors of the impeller, including the shape of the blade leading edge, blade inlet angle, number and thickness of blades, surface roughness, wrap angle, impeller outlet width, inlet hub diameter, and tip clearance. The pump analyzed in this study, which exhibited issues of vibration and noise in actual industrial settings, was evaluated by varying only the shroud diameter based on Gulich’s theory, while keeping other parameters constant, to assess the effects on cavitation phenomena across five different impellers. Single-phase analysis was initially conducted to evaluate the performance of each pump model, with the reliability of the numerical analysis methods validated by comparison with experimental data. Furthermore, to analyze cavitation phenomena, a multiphase flow analysis was performed using the Rayleigh–Plesset model within a computational fluid dynamics framework. Quantitative analysis of cavitation occurrence, NPSH3% head-drop performance, and bubble volume was conducted. The results confirmed that the M1 model, featuring a shroud diameter of 560 mm, exhibited superior cavitation resistance. Variations in cavitation occurrence observed under three different flow conditions demonstrated a nonlinear trend, but overall, improvements were noted within a specific diameter range. This study offers valuable insights and data for pump design applicable in real-world industrial settings.

1. Introduction

A centrifugal pump is a mechanical device that transfers fluid from one location to another within a system. Recent industrial advancements have escalated the demand for high-performance and efficient pumps. The performance of a pump is contingent on various factors, notably the impeller and casing, which are essential components. The impeller, in particular, continuously induces a vacuum through blade rotation, invariably leading to cavitation. Cavitation occurs when the pressure at the pump inlet falls below the saturation vapor pressure, causing bubbles to form and block the impeller’s flow path. The subsequent collapse of these bubbles decreases efficiency, head, and power, and inflicts damage on the impeller and casing [1,2], resulting in vibrations and noise [3,4,5]. Therefore, understanding and mitigating cavitation is crucial.

Recent advances in computational fluid dynamics (CFD) have increased interest in the numerical analysis of cavitation, a method previously constrained to experimental approaches. Numerous studies have validated the accuracy of numerical simulations in analyzing cavitation, demonstrating that these analyses, which were once costly and labor-intensive, can now be performed economically and efficiently [6,7,8,9].

The expanding body of literature includes studies such as those by Zhu et al. [10], who applied the diffusion-angle integral design method, genetic algorithms, and CFD to minimize pressure drop in mixed-flow pumps by modifying the leading edge (LE) shape of the impeller. Their optimization efforts resulted in an optimized impeller design. Sano et al. [11] examined the impact of variations in the ellipse ratio at the LE of a circulation pump impeller on cavitation, concluding that increases in flow rate and ellipse ratio mitigated cavitation. Tao et al. [12] explored various LE shapes—initially blunt, sharp, round, and elliptical—and their effects on cavitation, head, and flow separation, finding that smooth transitions in LE geometry significantly influence cavitation and overall pump performance. Liu et al. [13] analyzed the effects of changes in the angle and wrap angle of the centrifugal pump impeller’s LE, identifying that these modifications significantly impact the severity of cavitation.

Several studies have explored the effect of alterations in impeller blade shapes on cavitation, consistently highlighting that the geometry of the impeller’s LE crucially influences suction performance. Additionally, the integration of inducers within impellers as a preventative measure against cavitation is a focal area of ongoing research. For instance, Huan et al. [14] conducted both experimental and numerical analyses on cavitation phenomena in a centrifugal pump equipped with a two-blade inducer. Guo et al. [15] assessed the impact of varying the number of inducer blades on cavitation resistance and overall performance, finding that an optimal blade count enhances the net positive suction head required (NPSH_re), thereby improving cavitation resistance although at the expense of reduced head and efficiency. Campos-Amezcua et al. [16] explored the effects of radial tip clearance on pump performance and cavitation behavior in an inducer. They found that numerical analysis of models considering clearance was more accurate and consistent with experimental results compared to models without clearance. The study emphasizes the important role of considering clearance in optimizing the performance of axial inducers. Moreover, they identified that local cavitation flow instabilities in the inducer result from interactions with recirculating flow [17].

However, the universal application of inducers is constrained by environmental and economic factors, limiting their effectiveness in fundamentally addressing cavitation. Research aimed at diminishing recirculating flow and cavitation by modifying the impeller outlet width is also prevalent. Shi et al. [18] conducted an extensive study—experimental, theoretical, and numerical—on the performance of a multi-stage centrifugal pump with varied impeller outlet widths, observing that increased widths enhance head and shift efficiency to higher flow points, though excessive width may lead to overloads and vortices owing to increased shaft power, thus necessitating a balance. Tan et al. [19] used CFD to investigate the impact of changes in impeller outlet width on vortices and recirculating flow, noting that narrower widths increase vortex formation at the outlet, while wider widths are more prone to backflow into the impeller owing to recirculating flow.

Recirculating flow not only induces vibrations but also generates high-pitched noises that could cause severe pump damage due to cavitation [20]. Shim et al. [21] employed a multi-objective genetic algorithm combined with CFD to optimize pump performance by reducing suction recirculating flow and cavitation. Subsequent research [22] focused on the effects of adjusting the volute cross-sectional area, while Kim et al. [23] demonstrated that reducing the impeller inlet diameter effectively mitigates recirculating flow and enhances pump performance. However, the study by Schiavello et al. [24] assessed that the effects of cavitation and recirculation worsen as pump size (impeller eye diameter) and design capacity increase. This contrasts with the findings of Kim et al. [23] and many other researchers, suggesting that the results may vary depending on the pump’s geometry and operating conditions.

Extensive research has been conducted on pump performance, recirculation flow, and cavitation, but studies related to cavitation concerning impeller inlet diameter vary depending on the pump’s geometry and operating conditions. This study aims to address the gap in research that has been primarily focused on single-suction pumps, and it discusses the geometric design and procedures of the pump along with numerical validation. The research findings are discussed in Section 3, where the flow characteristics of each model are analyzed, and the onset of cavitation is described. The primary objective is to computationally analyze changes in the inlet shape that can improve the performance and cavitation resistance of pumps, particularly those experiencing issues with vibration and noise in the field.

2. Study Design

2.1. Research Subject

The subject of this study is a double-suction, single-stage centrifugal pump with a specific speed of 260, currently encountering issues related to vibration and noise in industrial settings. This pump comprises an impeller, suction, volute, and wearing parts. The three-dimensional configuration of the pump is shown in Figure 1, and the analysis includes five impeller blades per side (a total of 10 blades).

Key design specifications of the analysis model are detailed in

Table 1. Design specifications.

Volume flow	Q	7500 m3/h
Efficiency	Ŋ	80%
Head	H	60 m
Revolutions	N	710 rpm
Specific speed	ns	260.4
Suction specific speed	nss	1573.6
Number of blades		10
Stage		1

, and the blade angle for each model is specified in detail in

Table 2. Impeller blade angle data.

Model	Inlet Blade Angle (°)	Outlet Blade Angle (°)	Wrap Angle (°)
O1	45.80	64.58	16.9
M1, M2, M3, M4	45.84	64.61	16.9

. Aside from minor variations due to changes in inlet diameter, the angle differences are negligible. The specific speed (ns), suction specific speed (nss), and efficiency (η) are calculated using the following equations:

$$ns={\displaystyle \frac{N\sqrt{\frac{Q}{60\times f_q}}}{H^{0.75}}},$$

(1)

$$nss={\displaystyle \frac{N\sqrt{Q}}{{NPSH}^{0.75}}},$$

(2)

$$\eta ={\displaystyle \frac{\rho gHQ}{T\omega }},$$

(3)

where N is the rotational speed (rev/min), f_q is the number of impeller eyes per impeller (single-entry f_q = 1; double-entry f_q = 2), Q is the flow rate (m³/h), H is the head (m), NPSH is the net positive suction head (m), ρ is the liquid density (kg/m³), g is gravitational acceleration (m/s²), T is torque (Nm), and ω is angular velocity (rad/s). Modifications to the impeller design focused solely on the inlet diameter of the shroud while the hub dimensions remained constant. The design equation utilized, proposed by Gulich [25], is expressed as follows:

$$d_{1a}=2.9\sqrt[3]{{\displaystyle \frac{Q_{La}}{f_{q}n{k}_n\tan {\beta }_1}}\left(1+{\displaystyle \frac{\tan {\beta }_1}{\tan {\alpha }_1}}\right)},$$

(4)

where Q_La denotes the flow rate through the impeller (m³/s), k_n represents the blockage caused by the hub (kn =1 − d_n²/d₁², d_n = hub diameter), α₁ is the angle between the direction of circumferential and absolute velocity (°), and β₁ is the angle between the relative velocity vector and the negative direction of circumferential velocity (°). Figure 2 shows the side view of the impeller models used in this study based on this formula and experiential design, labeled O1 (original impeller), M1 (modified impeller), M2, M3, and M4, with diameters of 586, 560, 550, 530, and 520 mm, respectively.

2.2. Governing Equations

In CFD, the methods for simulating turbulent flow are primarily classified into three types: direct numerical simulation (DNS), large eddy simulation (LES), and Reynolds-averaged Navier–Stokes (RANS). DNS provides detailed calculations of vortices of all sizes, whereas LES focuses on large vortices, offering an accurate analysis of turbulent flows. However, owing to their high computational costs and extensive time requirements, both DNS and LES are often impractical for routine studies [26,27,28]. Therefore, this study utilizes the three-dimensional incompressible RANS equations, known for their cost-effectiveness and numerical stability. The continuity and momentum equations, incorporating time-averaged variables, are represented as follows:

$${\displaystyle \frac{\partial }{\partial x_j}}\left(\rho U\right)=0,$$

(5)

$${\displaystyle \frac{\partial }{\partial x_j}}\left(\rho U_i{U}_j\right)=-{\displaystyle \frac{\partial p}{\partial x_i}}+{\displaystyle \frac{\partial }{\partial x_j}}\left({\tau }_{ij}-\overline{\rho u_i{u}_j}\right)+S_M.$$

(6)

Here, the term $\overline{\rho u_i{u}_j}$ in the momentum equation is treated as the Reynolds stress, which models the mean flow in regions affected by turbulence.

2.3. Cavitation Model

For cavitation analysis, the Rayleigh–Plesset model is employed, as shown in Equation (7):

$$R_B{\displaystyle \frac{d^2{R}_B}{d{t}^2}}+{\displaystyle \frac{3}{2}}{\left({\displaystyle \frac{d{R}_B}{dt}}\right)}^2+{\displaystyle \frac{2\sigma }{{\rho }_f{R}_B}}={\displaystyle \frac{P_v-P}{{\rho }_f}}.$$

(7)

Here, R_B is the bubble radius (m), P_v is the pressure inside the bubble (Pa), and P is the external liquid pressure (Pa). The equation is simplified by ignoring the second-order term and surface tension results in Equation (8). Franc et al. [29] stated in their paper that this equation is applicable when the bubble radius is sufficiently large or when the bubble oscillates at low frequencies. They also indicated that this simplification can be an appropriate approximation in scenarios where the bubble radius is large or where pressure differences are the dominant factors. The rate of change of bubble volume and mass are described in Equations (9) and (10), respectively.

$${\displaystyle \frac{d{R}_B}{dt}}=\sqrt{{\displaystyle \frac{2}{3}}{\displaystyle \frac{P_v-P}{{\rho }_f}}}$$

(8)

$${\displaystyle \frac{d{V}_B}{dt}}={\displaystyle \frac{d}{dt}}\left({\displaystyle \frac{4}{3}}\pi {R_B}^3\right)=4\pi {R_B}^2\sqrt{{\displaystyle \frac{2}{3}}{\displaystyle \frac{P_v-P}{{\rho }_f}}}$$

(9)

$${\displaystyle \frac{d{m}_B}{dt}}={\rho }_g{\displaystyle \frac{d{V}_B}{dt}}=4\pi {R_B}^2{\rho }_g\sqrt{{\displaystyle \frac{2}{3}}{\displaystyle \frac{P_v-P}{{\rho }_f}}}$$

(10)

Incorporating the number of nuclei per unit volume (N_B), the vapor volume fraction is expressed in Equation (11). The general formula for evaporation and condensation, considering bubble growth and collapse, is given in Equation (12), with evaporation initiated from nuclei as described in Equation (13).

$$r_g=V_B{N}_B={\displaystyle \frac{4}{3}}\pi {R_B}^3{N}_B$$

(11)

$$\dot{m_{fg}}=F{\displaystyle \frac{3r_g{\rho }_g}{R_B}}\sqrt{{\displaystyle \frac{2}{3}}{\displaystyle \frac{\left|P_v-P\right|}{{\rho }_f}}}sgn(P_v-P)$$

(12)

$$\dot{m_{fg}}=F{\displaystyle \frac{3r_{nc}(1-r_g){\rho }_g}{R_{nc}}}\sqrt{{\displaystyle \frac{2}{3}}{\displaystyle \frac{\left|P_v-P\right|}{{\rho }_f}}}sgn(P_v-P)$$

(13)

Here, r_g is the vapor volume fraction, r_nc is the volume fraction of nucleation sites, and F is the empirical coefficient accounting for the phase change time disparity between condensation and evaporation. F_cond = 0.01 and F_vap = 50 are applied for condensation and evaporation, respectively. The default values for the volume fraction of nucleation sites and bubble nuclei radius used in this study are r_nc = 5 × 10⁻⁴ and R_nc = 1 μm, respectively [30].

2.4. Mesh Generation

Table 3. Mesh type and number of elements.

Parts	Mesh Type	Number of Elements
Suction	Tetra-prism	10,917,647
Volute	Tetra-prism	1,292,388
Wearing	Hexahedral	2,745,984
Impeller	Hexahedral	8,622,150
Total		23,579,169

details the grid configurations used in the domain for this analysis. For the blades, the flow field was initially generated using Blade Editor and subsequently constructed with hexahedral grids using TurboGrid. All blades were modeled considering symmetry and replication techniques. The meshes for the suction, volute, and wearing parts were developed using the ICEM-CFD grid generation program provided by ANSYS, incorporating both tetra-prism and hexahedral grids, as shown in Figure 3. Figure 4 presents the results of a mesh independence test conducted to verify the reliability of the simulations, indicating that variations in pump head were negligible beyond 800,000 grids per impeller. The head difference between the maximum grid count and 800,000 grids is approximately 0.61%. Therefore, the mesh was optimized to approximately 800,000 grids.

Turbulence modeling plays a crucial role in numerical analysis, particularly owing to the effects of the wall’s physical properties and the fluid’s viscosity, which significantly influence the flow near the wall. To accurately simulate this interaction, the dimensionless length concept y+ is utilized, combining the fluid’s viscosity and the wall shear stress at a specified distance from the wall; y+ is calculated as shown in Equation (14):

$$y^{+}={\displaystyle \frac{u_{\tau }y}{\nu }},$$

(14)

where u_τ represents the friction velocity (m/s), y is the absolute distance from the wall (m), and ν is the kinematic viscosity (m²/s). In CFD simulations, y+ is critical for adjusting grid density near the wall to ensure accurate flow predictions. The impeller, a crucial component in pump analysis, necessitates precise implementation of wall flow within the domain. Research has indicated that a y+ value below 5 is generally appropriate for rotating machinery [31,32,33]. This study adhered to this standard and maintained it consistently.

2.5. Analysis Method

The analysis in this study was conducted using the commercial CFD software ANSYS CFX-21.2. The entire flow field was modeled as an incompressible three-dimensional steady state.

The boundary conditions are summarized in

Table 4. Boundary conditions.

Working Fluid	Water at 25 °C
	Water vapor at 25 °C
Interface Area	Frozen-rotor
	Stage mix
Inlet	Opening (total pressure)
Outlet	Mass flow rate
Wall	No slip
Mesh Connection	GGI

. The nominal rotational speed of the pump was set at 710 rpm. To account for the backflow phenomenon in the pump, the inlet condition was configured as an opening with a total pressure condition, and the outlet was set to manage a specified mass flow rate. The components such as the suction, volute, wearing parts, and the impeller’s hub, shroud, and blades were defined as walls to inhibit fluid flow. For the analysis of the rotating impeller domain and the stationary domains of the suction, volute, and wearing parts, the multiple reference frame model was utilized. The wearing-impeller interface, where fluid enters the suction domain, was modeled with the frozen-rotor method, and the impeller-wearing interface, where fluid exits into the volute, was handled with the stage mix method. The mesh’s connectivity between the rotating and stationary domains was facilitated by the General Grid Interface (GGI). The shear stress transport (SST) turbulence model, well-suited for rotating fluid machinery, was employed [34,35,36]. To closely simulate the actual pump environment, a surface roughness [37,38,39] of 30 microns was applied. The working fluid was water at 25 °C, with properties such as a density of 997 kg/m³ and a saturation vapor pressure of 3170 Pa.

2.6. Reliability Verification

The reliability of the CFD analysis results was substantiated using experimental data provided by the pump manufacturer. Figure 5 shows a schematic of the experimental setup employed to evaluate the performance of the O1 model, one of the five impeller models tested. Flow measurements were obtained at the pump outlet using an electronic flowmeter (KTM-800, Korea Flowmeter Co., Ltd., Incheon, Republic of Korea), and head measurements were conducted at points three diameters from the pump casing inlet and five diameters from the outlet. Pressure was measured using a pressure transmitter (266GST, ABB Asea Brown Boveri Ltd., Zurich, Switzerland), with a maximum error margin of 0.5% for flow and 0.04% for pressure.

Figure 6 presents a comparison between the experimental results and the numerical values obtained from CFD analysis. The analysis was performed for the O1 model across various flow rates, specifically at 5220, 6000, 6750, 7500, 8250, and 9000 m³/h. At the designated flow rate of 7500 m³/h, discrepancies between the experimental outcomes and numerical predictions were 6.22% for efficiency and 3.63% for head. At the best efficiency point (BEP), the deviations were noted as 5.27% for efficiency and 6.01% for head. The overall errors were approximately 6.88% for head and 5.65% for efficiency, indicating significant accuracy considering the potential leakage losses from bearings and piping, as well as losses attributable to surface roughness observed in actual experimental conditions.

3. Results

3.1. Performance Analysis

Performance analysis was conducted by observing changes in the inlet diameter of each impeller. Initially, the BEP flow rate was set at 8250 m³/h for the original impeller model, O1, and then adjusted by ±10%. The resultant head and efficiency metrics at varying flow rates are shown in Figure 7. The flow rate criteria for this study adhere to the preferred operating region (POR), defined as 70% to 120% of the BEP according to API Standard 610 [40]. Numerical analysis was performed for each impeller model, and results were non-dimensionalized by dividing by the flow rate at the peak efficiency point. Figure 7a shows the variations in head across different flow rates. The difference in head between the impeller with the largest inlet diameter (O1) and the smallest inlet diameter (M4) is 0.3% at a flow rate of 0.7 and −0.81% at a flow rate of 1.2, indicating that minor adjustments in shroud diameter do not substantially impact head performance. Figure 7b shows the efficiency changes across impeller models. As the shroud diameter decreases, efficiency improves across all flow rates, with the gap narrowing at higher flows. This occurs because changes in inlet diameter increase torque and energy consumption due to flow characteristics like recirculation at the impeller inlet. These findings indicate that inlet diameter significantly affects efficiency.

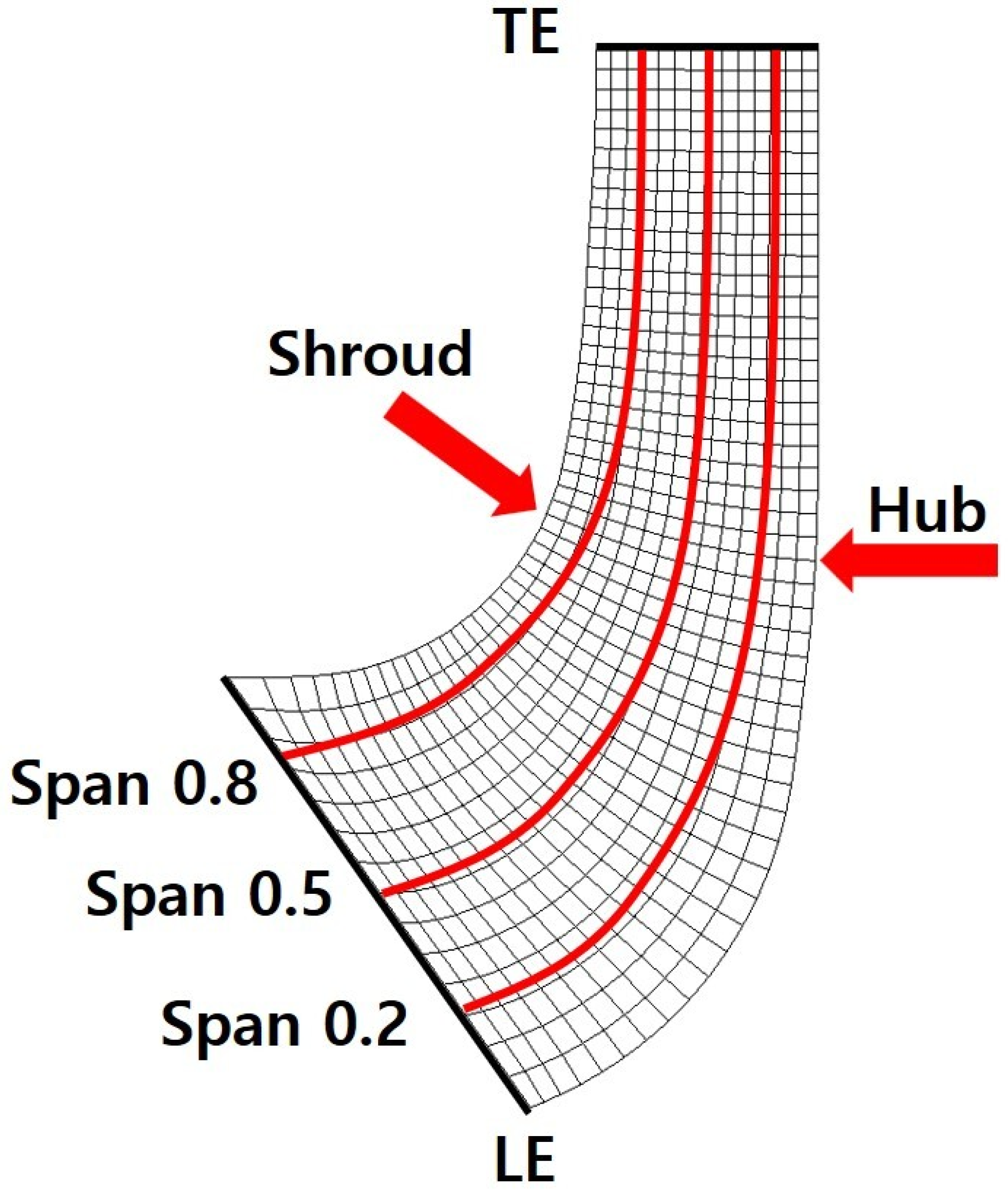
Figure 8 shows the meridional plane geometry of the impeller, including the leading edge (LE), trailing edge (TE), shroud, hub, and the spanwise direction. This diagram visually represents the key geometrical features of the impeller, providing a comprehensive view of its design characteristics.

Figure 9 provides an in-depth examination of the pressure field at the impeller’s LE for each model at a flow rate of 1.0, depicted through pressure contours calculated using the pressure coefficient in Equation (15):

$$C_p={\displaystyle \frac{P-P_{inlet}}{\rho u_2^2/2}},$$

(15)

where P is the static pressure at the pump (Pa), P_inlet is the static pressure at the pump inlet (Pa), and u₂ is the peripheral speed at the impeller exit (m/s). Negative pressure indicates a pressure distribution lower than the pump inlet pressure, which increases the likelihood of cavitation in those areas. The centrifugal force generated by impeller rotation creates a positive pressure gradient in the radial direction. However, the local pressure reduction near the shroud can be attributed to factors beyond centrifugal force, such as the complex flow patterns between the impeller and the shroud. These factors may collectively contribute to pressure reductions near the shroud, as well as near the hub.

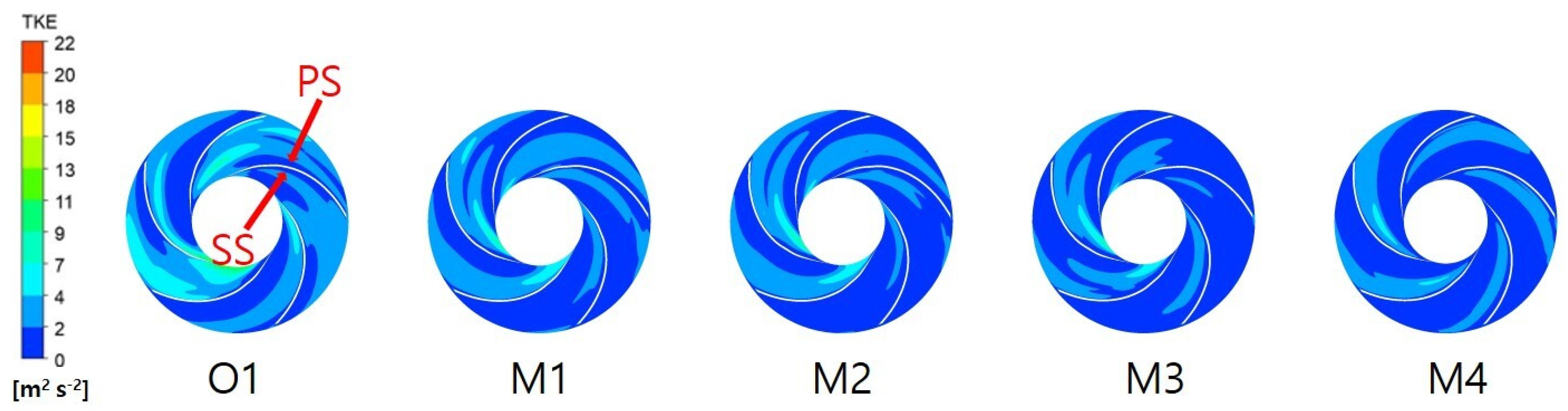
Turbulence kinetic energy (TKE), which represents turbulence intensity, is calculated as the mean of the squared turbulent velocity components. Although directly correlating TKE with efficiency and pump performance is challenging, it offers indirect insights into fluid behavior. Figure 10 presents the TKE values at a flow rate of 1.0 for each impeller model at a spanwise position of 0.5. As the impeller inlet diameter decreases, the TKE correspondingly decreases, indicating that the increased flow velocity results in relatively reduced recirculating flow, which consequently induces turbulence. Analysis from Figure 8 indicates that across all impeller models, turbulence intensity and pressure drops are more pronounced on the suction side (SS) than on the pressure side (PS), providing valuable insights into potential bubble formation sites during cavitation analysis.

3.2. Suction Performance Analysis

To assess the potential for cavitation in a double-suction pump, it is crucial to quantify the cavitation characteristics of the rotating machinery. In this study, the pump suction analysis was performed by progressively reducing the suction pressure while maintaining a constant speed in the simulation. The NPSH_3% head, which is the head at which a 3% head drop due to cavitation occurs at flow rates of 0.7, 1.0, and 1.2, was measured and non-dimensionalized as the cavitation number ($\sigma$). The fluid dynamic cavitation number (${\sigma }_{hyd}$) is defined by Equation (16):

$${\sigma }_{hyd}={\displaystyle \frac{P-P_{vap}}{\frac{1}{2}\rho V^2}}.$$

(16)

Here, P is the fluid pressure (Pa), and V is the fluid velocity (m/s), illustrating the relationship between the fluid pressure, saturation vapor pressure, and the kinetic energy per unit volume. A lower cavitation number indicates a greater likelihood of cavitation, while a higher number suggests reduced susceptibility to cavitation. In this study, Thoma’s cavitation number (σ) was utilized to predict and analyze cavitation during pump fluid suction, as shown in Equation (17):

$$\sigma ={\displaystyle \frac{NPSH}{H}}.$$

(17)

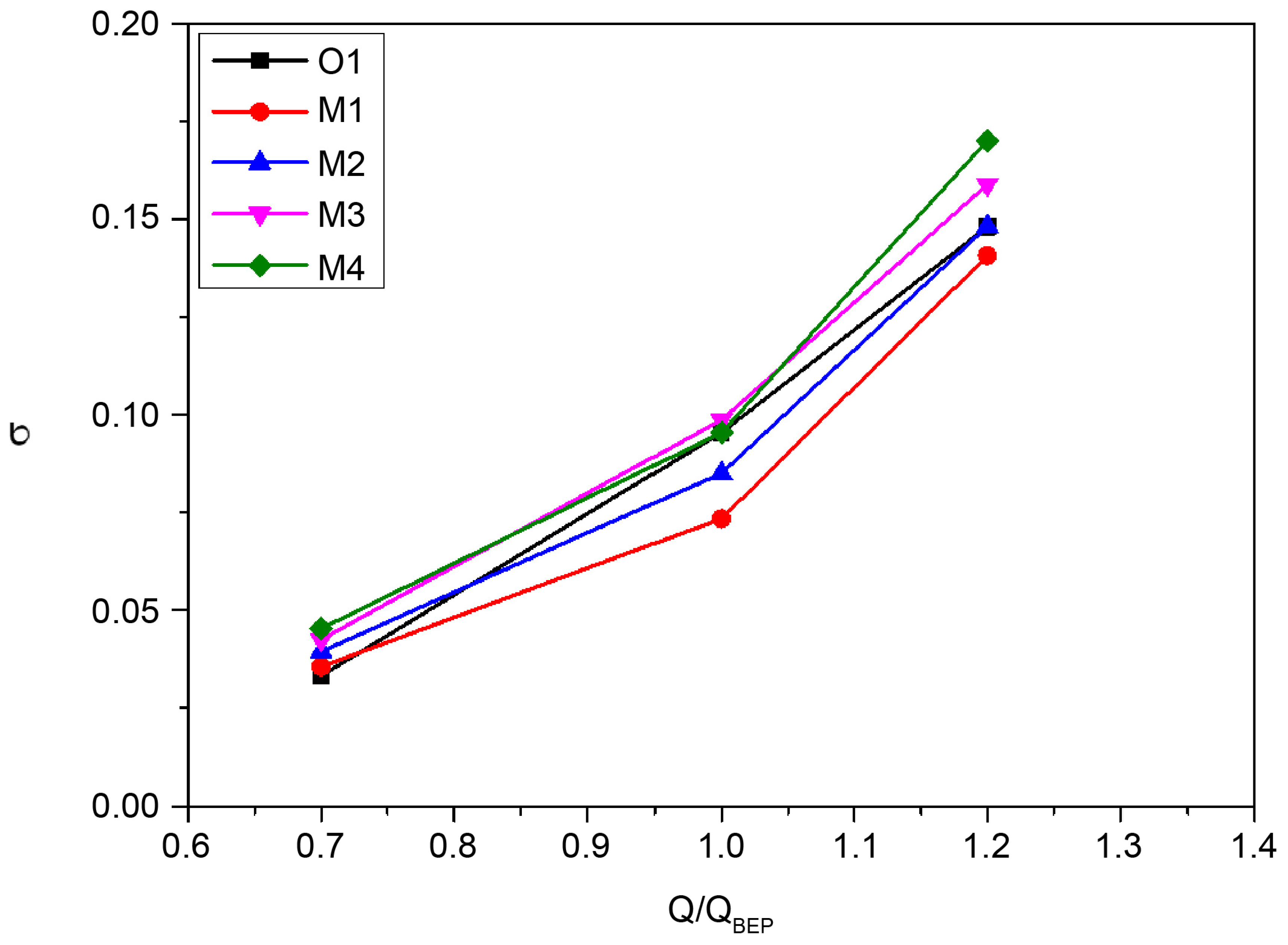
The head was non-dimensionalized by dividing it by the head under conditions where cavitation does not occur for each impeller model. Figure 11a shows the head drop curve at a flow rate of 0.7. The values corresponding to σ_3% in the low-flow region range from 0.03 to 0.045, with σ_3% values of 0.033 for O1, 0.036 for M1, 0.039 for M2, 0.042 for M3, and 0.045 for M4. This indicates that the cavitation number increased by 9%, 18%, 27%, and 39%, respectively, compared to the O1 impeller, suggesting that suction performance decreases as the inlet diameter decreases in the low-flow region.

Figure 11b shows the head drop curve at a flow rate of 1.0. The σ_3% values at this flow rate range from 0.073 to 0.095. As the flow rate increases, the velocity increases, and the pressure decreases. Consequently, cavitation occurs earlier than in the low-flow region. The σ_3% values are 0.095 for O1, 0.073 for M1, 0.085 for M2, 0.099 for M3, and 0.095 for M4. As the inlet diameter decreases, the changes in cavitation number are −23.1%, −10.5%, 4.2%, and 0%, respectively. Under BEP flow conditions, the suction performance of the M1 and M2 impellers is significantly improved compared to the O1 impeller.

Figure 11c shows the head drop curve at a flow rate of 1.2, with σ_3% values ranging from 0.141 to 0.170. The σ_3% values are 0.148 for O1, 0.141 for M1, 0.148 for M2, 0.159 for M3, and 0.170 for M4. As the inlet diameter decreases, the changes in cavitation number are −4.7%, 0%, 7.4%, and 14.8%, respectively. According to referenced studies, there is generally a tendency for cavitation to occur earlier as the pump inlet diameter decreases and the flow rate increases. Even under these conditions, the M1 model still exhibits better suction performance than the O1 model. The analysis results at a flow rate of 1.2 indicate that the suction performance of the M1 impeller improves compared to the O1 impeller, while the suction performance of the M3 and M4 impellers decreases compared to the BEP flow. The σ_3% values for each model at varying flow rates are summarized in

Table 5. Cavitation number (σ_3%) for each flow rate (Q).

	O1	M1	M2	M3	M4
Q = 0.7
σ3%	0.033	0.036	0.039	0.042	0.045
Q = 1.0
σ3%	0.095	0.073	0.085	0.099	0.095
Q = 1.2
σ3%	0.148	0.141	0.148	0.159	0.170

.

Figure 12 shows the cavitation numbers corresponding to NPSH_3% at flow rates of 0.7, 1.0, and 1.2 for each impeller type. As the flow rate increases, the suction velocity of the pump increases at higher flow rates, causing cavitation to occur earlier than in the low-flow region.

3.3. Bubble Volume Analysis

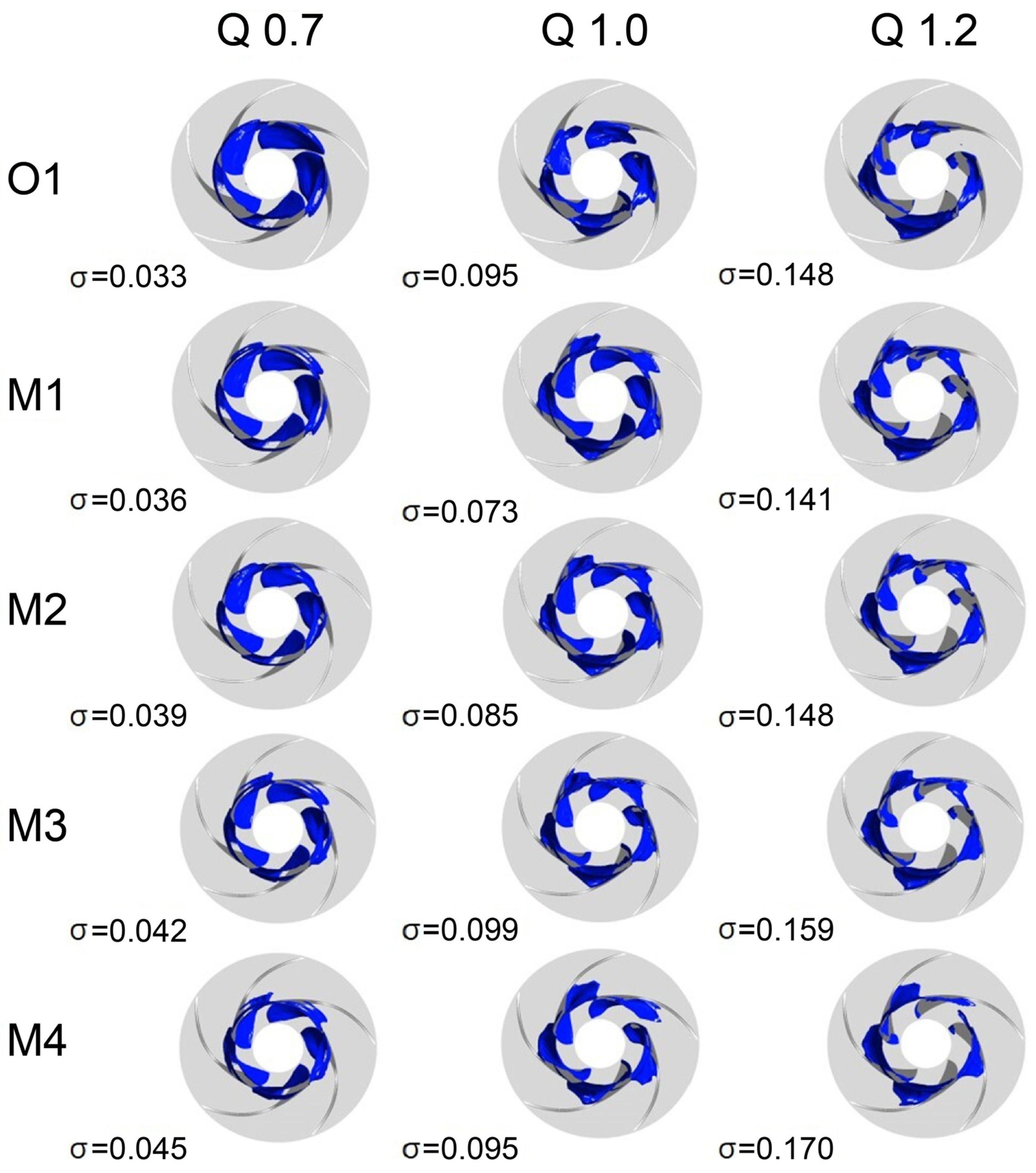
This section examines cavitation phenomena within each impeller model just before the onset of head collapse at various flow rates. Figure 13 presents iso-surface contours that illustrate these dynamics. Across all models, as the flow rate increases, the point of head collapse is reached as the number and size of bubbles decrease. Cavitation typically involves the formation and collapse of bubbles; while many studies have visually examined bubble development and used indirect metrics such as efficiency and head to quantify this, these methods provide useful trends but have limitations in quantitatively assessing cavitation itself.

To quantitatively analyze the bubble volume within the impeller at the same cavitation number, Figure 14 shows the bubble volume relative to the impeller volume. Here, V_vap represents the total vapor volume within the impeller, and V_imp is the impeller volume. The ratio V_vap/V_imp is non-dimensionalized. At a flow rate of 0.7 and a σ of 0.0333, V_vap/V_imp for O1 is 0.129; V_vap/V_imp for M1, M2, M3, and M4 are 0.132, 0.152, 0.140, and 0.174, respectively, indicating corresponding increases of 2.33%, 17.8%, 8.5%, and 34.9% compared to the O1 impeller. This trend suggests that the O1 model exhibits the smallest relative bubble volume at low flow rates, correlating to the later occurrence of the NPSH3% point among all impellers.

At a flow rate of 1.0 and a σ of 0.095, the V_vap/V_imp values are 0.054 for O1, 0.046 for M1, 0.050 for M2, 0.055 for M3, and 0.067 for M4, showing decreases of −14.8% and −7.4% for M1 and M2, and increases of 1.9% and 24.1% for M3 and M4, respectively, compared to the O1 impeller.

At a flow rate of 1.2 and a σ of 0.148, the V_vap/V_imp values are 0.048 for O1, 0.036 for M1, 0.038 for M2, 0.053 for M3, and 0.105 for M4, indicating decreases of −25.0% and −20.8% for M1 and M2, and increases of 10.4% and 118.8% for M3 and M4, respectively, compared to the O1 impeller. Notably, the M1 impeller demonstrates a significant increase in suction performance, while the M2 impeller shows a comparable occurrence of NPSH3% but with fewer bubbles, suggesting better resistance to impeller damage and vibration. The M4 impeller exhibits the largest increase in V_vap/V_imp at a flow rate of 1.2. These results are summarized in

Table 6. V_vap/V_imp and compared ratio to O1 impeller.

	O1	M1	M2	M3	M4
σ = 0.033
Vvap/Vimp	0.129	0.132	0.152	0.140	0.174
Ratio [%]	-	2.33	17.8	8.5	34.9
σ = 0.095
Vvap/Vimp	0.054	0.046	0.050	0.055	0.067
Ratio [%]	-	−14.8	−7.4	1.9	24.1
σ = 0.148
Vvap/Vimp	0.048	0.036	0.038	0.053	0.105
Ratio [%]	-	−25.0	−20.8	10.4	118.8

.

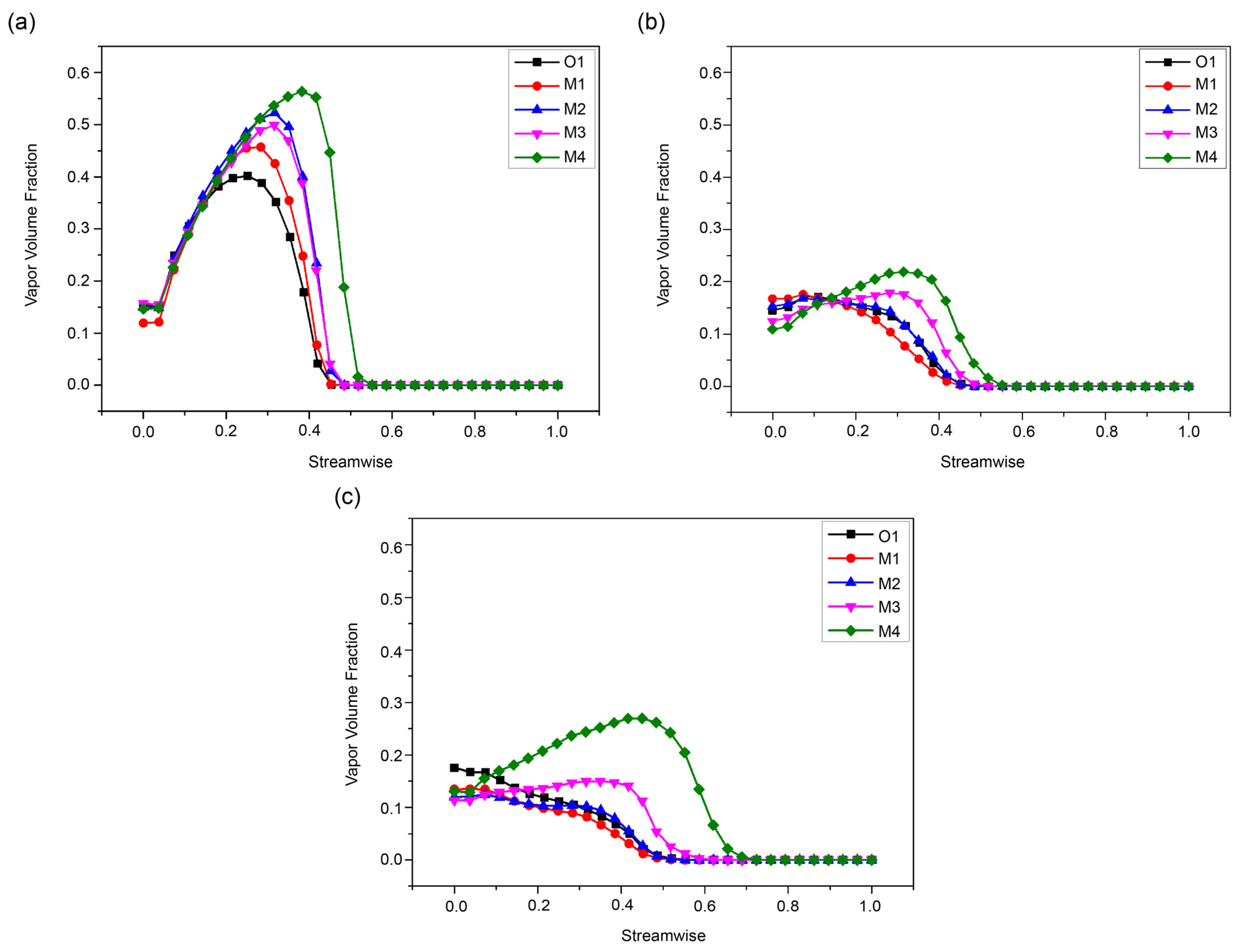
To further explore the impact of cavitation on impeller blades, Figure 15 shows graphs of the vapor volume fraction in the streamwise direction at σ_3% for each model under different flow rates. Here, 0 represents the LE, and 1 represents the trailing edge.

Figure 15a shows the vapor volume fraction in the streamwise direction at a σ of 0.033. The initial bubble development at the LE shows values of 0.154 for O1, 0.119 for M1, 0.150 for M2, 0.157 for M3, and 0.146 for M4. This indicates slower initial development in the M1 impeller. Up to approximately 0.2 streamwise, the development is consistent across models; however, beyond this point, bubble development at the trailing edge increases with reduced inlet diameter at low flow rates.

Figure 15b shows the vapor volume fraction in the streamwise direction at a σ of 0.095. Bubble development at the LE is recorded as 0.145 for O1, 0.167 for M1, 0.153 for M2, 0.125 for M3, and 0.109 for M4, indicating slower initial development in the M3 impeller. The development and decay patterns of O1 and M2 are notably similar.

Figure 15c shows the vapor volume fraction in the streamwise direction at a σ of 0.148. Bubble development at the LE is 0.176 for O1, 0.135 for M1, 0.119 for M2, 0.113 for M3, and 0.131 for M4, indicating the slowest initial development in the M3 impeller and the highest initial development in the O1 impeller. Under high-flow conditions, the least bubble development is observed in the M1 impeller, with the most active development towards the TE observed in the M3 and M4 impellers.

However, it is important to note that in actual operation, cavitation phenomena within the impeller are influenced by various complex factors such as surface roughness, site conditions, operator skill, and fluid temperature, among others. These factors make it challenging to precisely predict cavitation through simulation alone. Therefore, discrepancies may exist between the simulation results and actual cavitation experiments.

4. Conclusions

Geometric factors at the impeller eye significantly influence the suction performance of pumps. While various factors such as hub and shroud diameters, blade angle and thickness, number of blades, surface roughness, and LE profile play a role, this study specifically investigates cavitation phenomena based on variations in shroud diameter, with all other variables held constant. Utilizing impeller designs derived from Gulich’s equations and the designer’s experience, five impeller models, including the original, were examined. The performance regarding efficiency, head, and cavitation resistance at different flow rates was analyzed through CFD using a multiphase model.

The accuracy of the numerical analyses was validated by comparing experimental values with numerical results. It was confirmed that operating the pump in a cavitation-free environment could enhance efficiency without significant alterations in head. However, achieving such ideal conditions poses substantial challenges in actual industrial applications. To create models and numerical analyses applicable to real-world scenarios, cavitation numerical analysis was conducted using widely referenced theories and commercial codes. The findings indicate that the M1 model exhibited the best overall suction performance. Given that the impeller eye diameter linearly affects recirculation flow in the performance analysis, the optimal d1a value for the pump used in this study is determined to be between 560–550 mm, balancing both cavitation resistance performance and recirculation flow.

The improved NPSH3% values were demonstrated in the head drop curves at flow points commonly referenced by pump users. By comparing the bubble volume to the impeller volume under cavitation conditions, the potential for bubble volume was quantified. Results indicate that the M1 model exhibited the least bubble growth and occurrence, with V_vap/V_imp values of 0.046 at BEP flow and 0.036 at high flow, suggesting that this model could extend impeller lifespan under consistent operating conditions.

In summary, this study confirms that the design of the impeller inlet diameter in double-suction single-stage pumps significantly affects recirculation flow, which can cause vibration and noise, as well as impact cavitation resistance. Based on the numerical analysis of five impeller models, it is recommended that a moderate reduction in inlet diameter can reduce recirculation flow. However, excessive reduction may decrease cavitation resistance, indicating the necessity for a balanced approach in impeller inlet diameter design to optimize efficiency and minimize cavitation in industrial settings. Building on the M1 model, future research will involve experimental validation incorporating variations in additional parameters such as surface roughness and throat area, to improve cavitation phenomena and optimize pump performance under various operating conditions.