Research on Suppressing Commutation Torque Ripple of BLDCM Based on Zeta Converter
Abstract
1. Introduction
2. Analysis of Commutation Torque Ripple in BLDCM
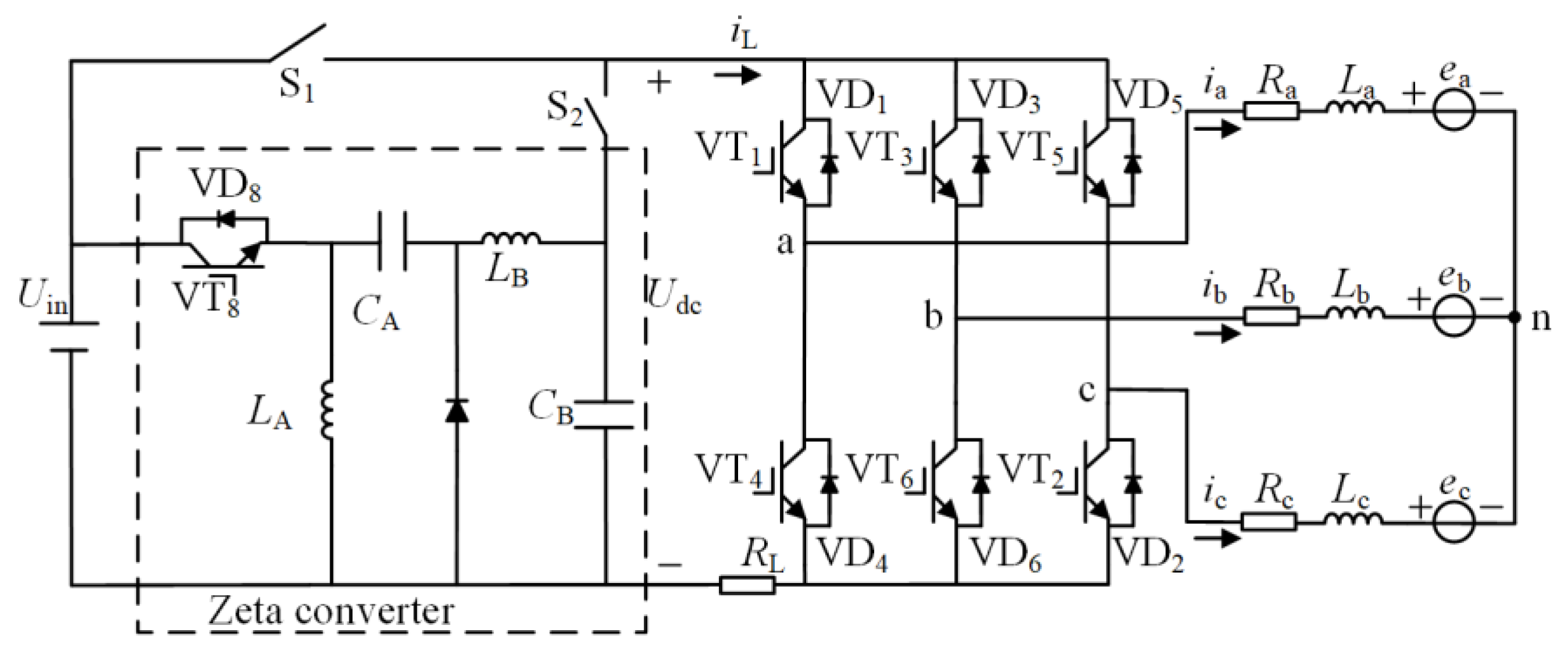
2.1. Equivalent Model of BLDCM with Zeta Converter
2.2. Analysis of Commutation Torque Ripple Based on Zeta Converter
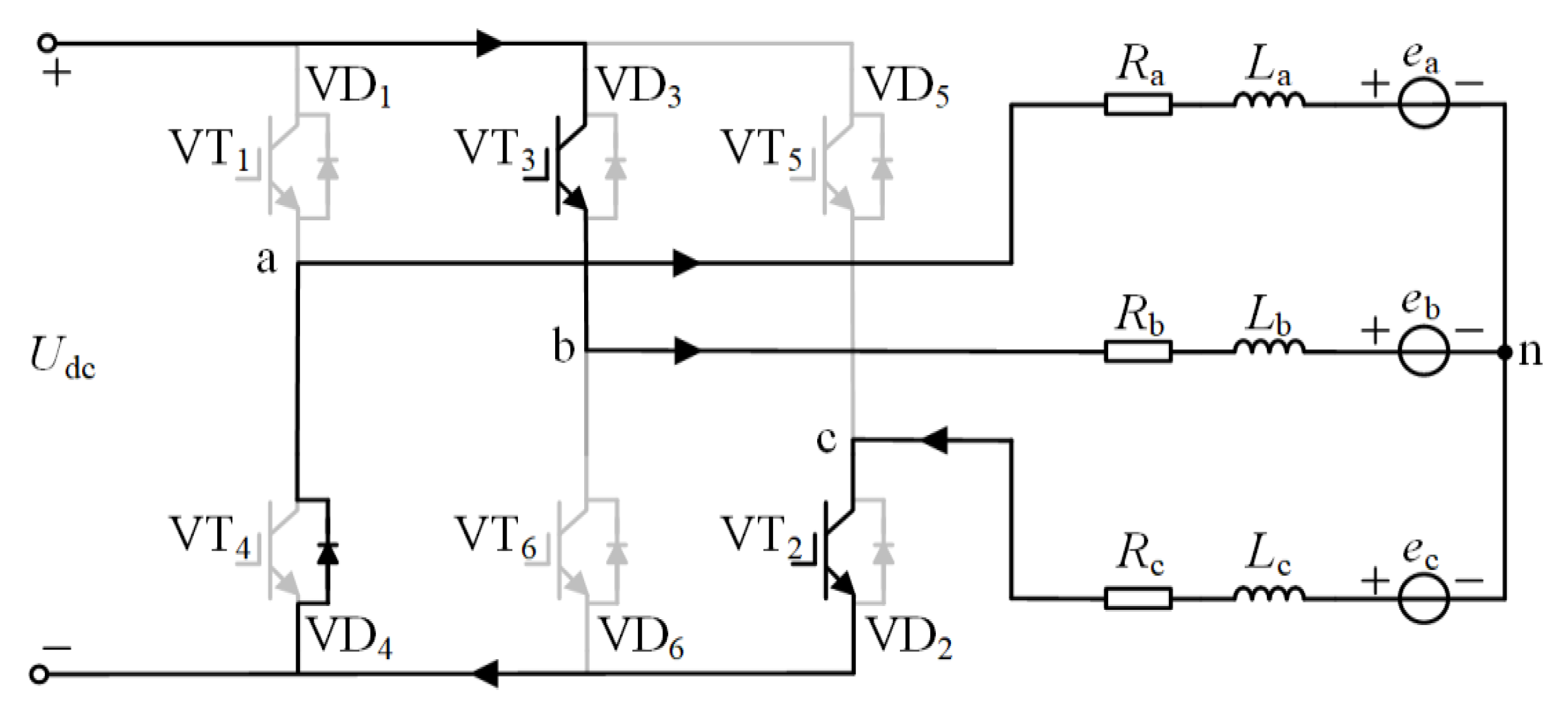
3. Strategy of Suppressing Commutation Torque Ripple Based on Zeta Converter
3.1. Conditions for Suppressing Commutation Torque Ripple in BLDCM
3.2. Design of Back-EMF Observer for Brushless DC Motor
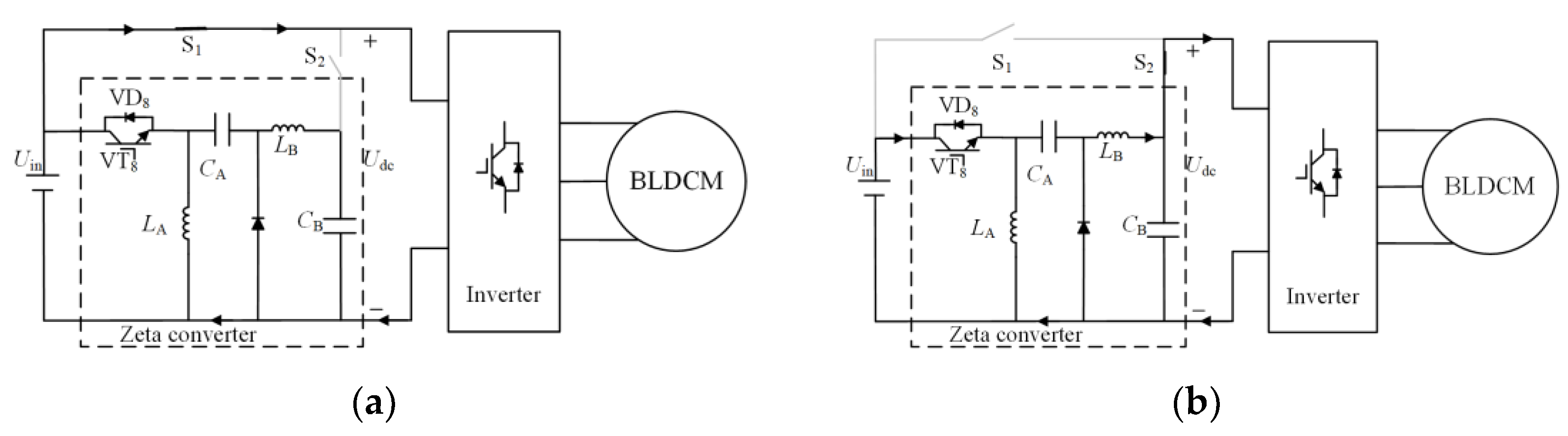
3.3. Analysis of the Dynamic Response of the Zeta Converter
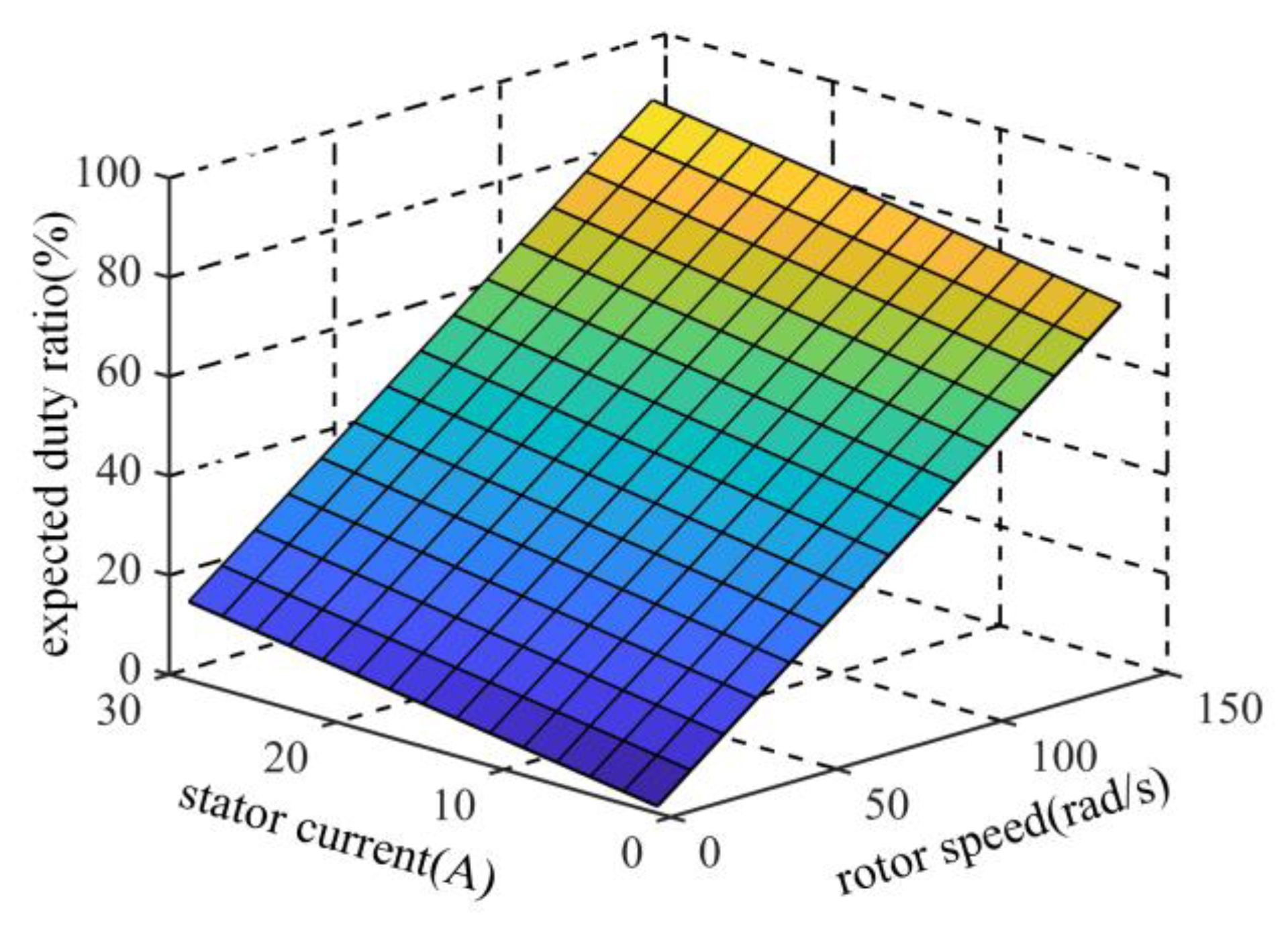
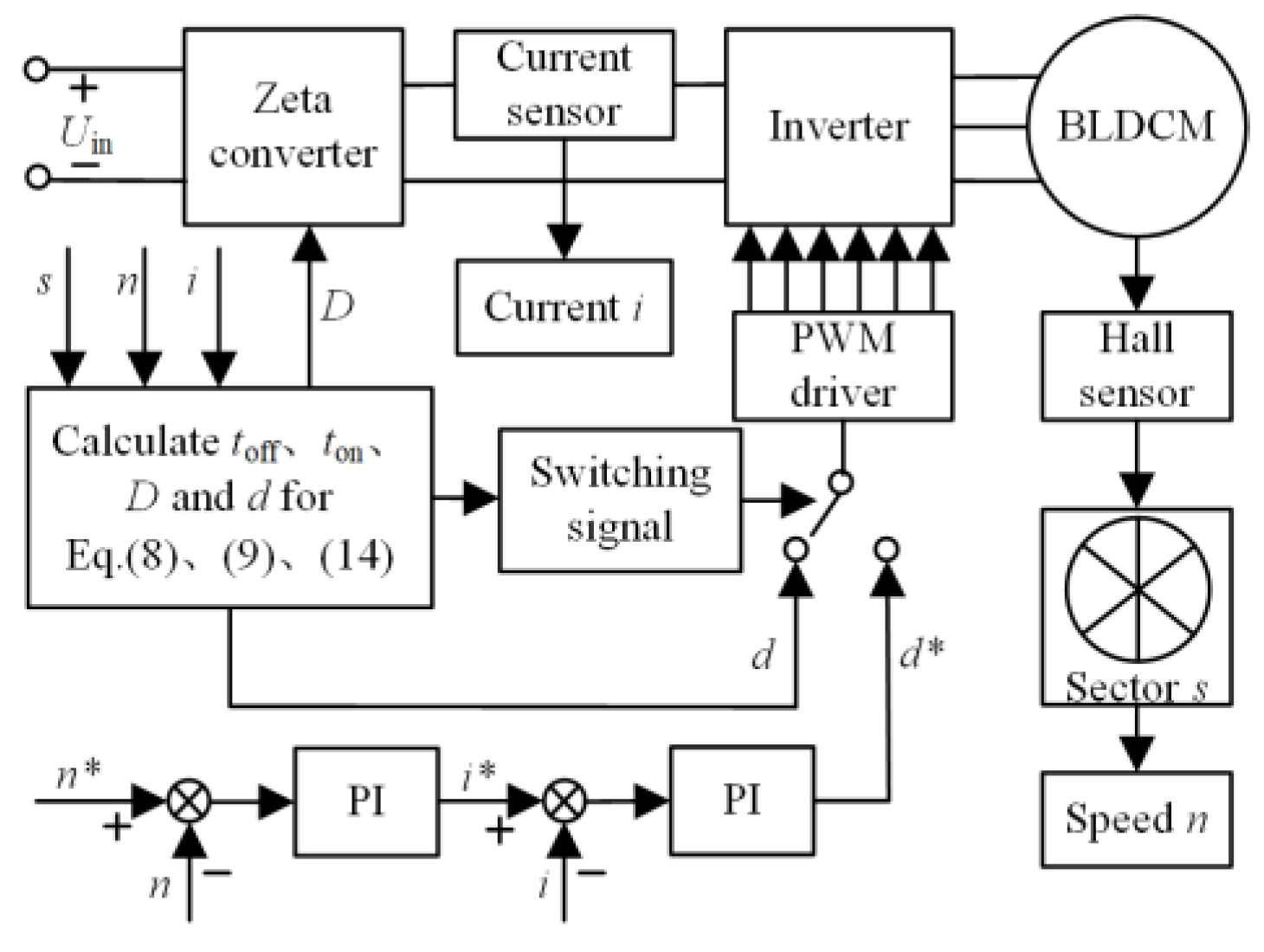
3.4. Implementation of Commutation Torque Ripple Suppression Strategy
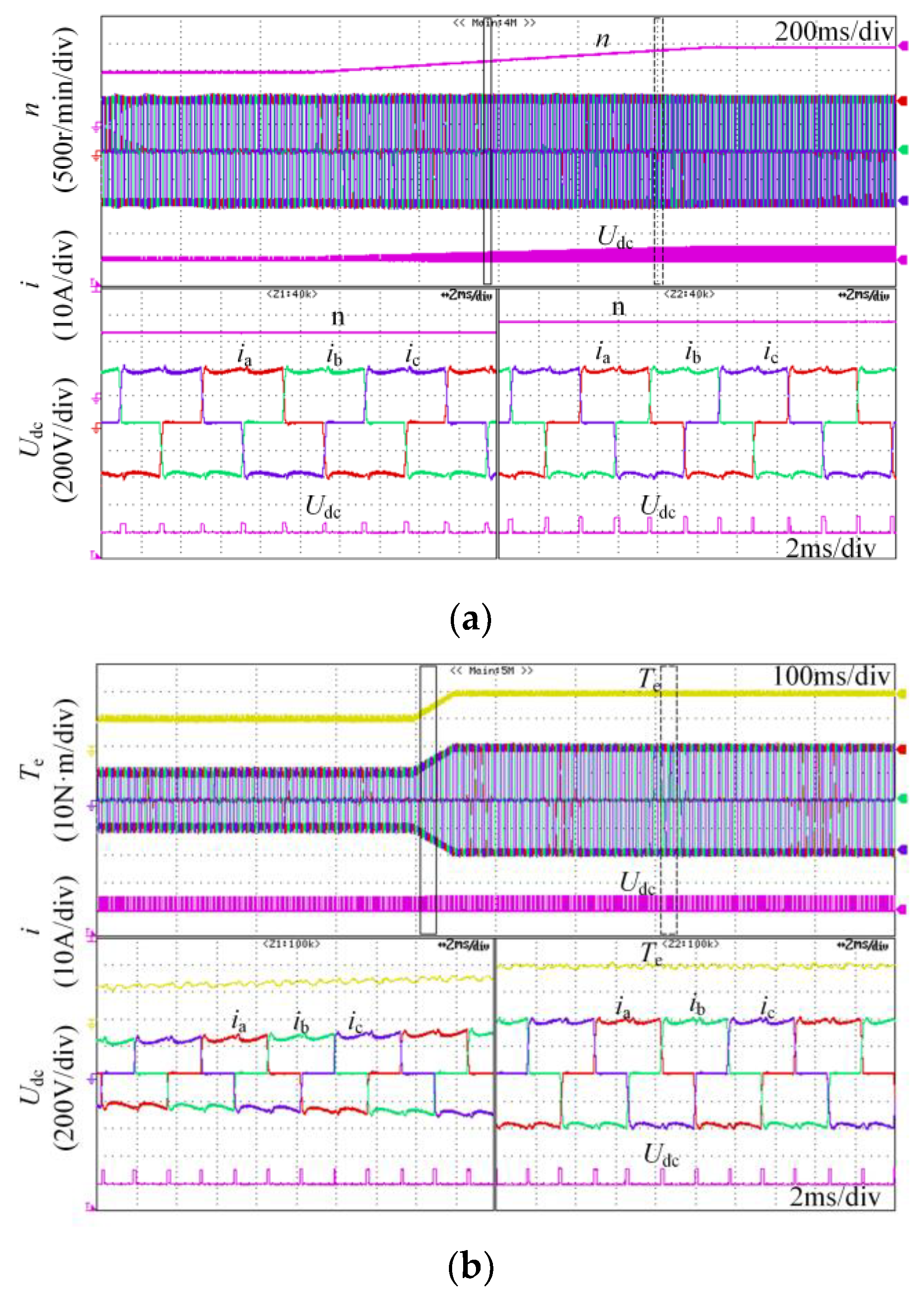
4. Simulation and Experimental Results
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Wei, J.; Liu, P.; Tao, W. An Incorporated Control Strategy of Commutation and Conduction Torque Ripples Mitigation for BLDCM Drives in Household Appliances. IEEE Access 2019, 7, 108137–108151. [Google Scholar] [CrossRef]
- Zheng, B.; Cao, Y.; Li, X.; Shi, T. An Improved DC-Link Series IGBT Chopping Strategy for Brushless DC Motor Drive with Small DC-Link Capacitance. IEEE Trans. Energy Convers. 2021, 36, 242–252. [Google Scholar] [CrossRef]
- Cai, J.; Zhang, X.; Zhang, W.; Zeng, Y. An Integrated Power Converter-Based Brushless DC Motor Drive System. IEEE Trans. Power Electron. 2022, 37, 8322–8332. [Google Scholar] [CrossRef]
- Zhou, H.; Wang, X.; Gu, C. Analysis and Suppression of DC Bus Voltage Ripple of Cascade Brushless DC Motor Drive System. In Proceedings of the 2019 22nd International Conference on Electrical Machines and Systems (ICEMS), Harbin, China, 11–14 August 2019; pp. 1–5. [Google Scholar] [CrossRef]
- Kumar, A.; Singh, B. High-Performance Brushless Direct-Current Motor Drive for Ceiling Fan. IEEE Trans. Ind. Electron. 2024, 71, 6819–6828. [Google Scholar] [CrossRef]
- Thrishana, B.B.; Nalinakshan, S.; Venkatesh, A.; Nishana, B.B. Controlling BLDC Motor with Bidirectional Converters with Cyber-Physical Systems. In Proceedings of the 2023 IEEE International Conference on Electronics, Computing and Communication Technologies (CONECCT), Bangalore, India, 14–16 July 2023; pp. 1–6. [Google Scholar] [CrossRef]
- Kim, S.-K.; Lim, S.; Ahn, C.K. Observer-Based Order-Reduction Speed Control for Converter-Fed BLDC Motors with Current-Loop Adaptation. IEEE Trans. Circuits Syst. II Express Briefs 2023, 70, 2934–2938. [Google Scholar] [CrossRef]
- Bahari, A.S.; Choulabi, E.F.; Shahalami, S.H. Reducing Switching Losses in Brushless DC Motor Drive System by a Novel Soft Switching Inverter. In Proceedings of the 2023 3rd International Conference on Electrical Machines and Drives (ICEMD), Tehran, Iran, 20–21 December 2023; pp. 1–6. [Google Scholar] [CrossRef]
- Lai, J.; Masisi, L.; Akuru, U.B. Regenerative Braking Torque Ripple Minimization of a Brushless DC Machine Using Fuzzy-PI Controller. In Proceedings of the 2023 IEEE Energy Conversion Congress and Exposition (ECCE), Nashville, TN, USA, 29 October–2 November 2023; pp. 6538–6540. [Google Scholar] [CrossRef]
- Zhang, H.; Dou, M.; Yan, L. Effects of Stator Iron Loss and Current-Loop Delay on Copper-Loss-Minimizing Torque Control of BLDCM: Analysis and Improvements. IEEE Trans. Power Electron. 2019, 34, 5620–5631. [Google Scholar] [CrossRef]
- Khazaee, A.; Yazdani, A.; Mosaddegh Hesar, H.; Wu, B. Efficient MPPT for BLDCM-Driven PV Pumping System Based on Ripple Correlation Control. IEEE Trans. Power Electron. 2023, 38, 8022–8026. [Google Scholar] [CrossRef]
- Santra, S.B.; Chatterjee, A.; Chatterjee, D.; Padmanaban, S.; Bhattacharya, K. High Efficiency Operation of Brushless DC Motor Drive Using Optimized Harmonic Minimization Based Switching Technique. IEEE Trans. Ind. Appl. 2022, 58, 2122–2133. [Google Scholar] [CrossRef]
- Zhou, Q.; Shu, J.; Cai, Z.; Liu, Q.; Du, G. Improved PWM-OFF-PWM to Reduce Commutation Torque Ripple of Brushless DC Motor Under Braking Conditions. IEEE Access 2020, 8, 204020–204030. [Google Scholar] [CrossRef]
- Cao, Y.; Shi, T.; Li, X.; Chen, W.; Xia, C. A Commutation Torque Ripple Suppression Strategy for Brushless DC Motor Based on Diode-Assisted Buck–Boost Inverter. IEEE Trans. Power Electron. 2019, 34, 5594–5605. [Google Scholar] [CrossRef]
- Kalla, U.K.; Bhati, N.; Agarwal, K.L.; Shankar, G.; Singh, B. Analysis of Canonical Switching Inverse Buck-Boost Converter Based Electric Vehicle Drive System. In Proceedings of the 2020 IEEE International Conference on Power Electronics, Drives and Energy Systems (PEDES), Jaipur, India, 16–19 December 2020; pp. 1–6. [Google Scholar] [CrossRef]
- Viswanathan, V.; Seenithangom, J. Commutation Torque Ripple Reduction in the BLDC Motor Using Modified SEPIC and Three-Level NPC Inverter. IEEE Trans. Power Electron. 2018, 33, 535–546. [Google Scholar] [CrossRef]
- Yao, X.; Zhao, J.; Wang, J.; Huang, S.; Jiang, Y. Commutation Torque Ripple Reduction for Brushless DC Motor Based on an Auxiliary Step-Up Circuit. IEEE Access 2019, 7, 138721–138731. [Google Scholar] [CrossRef]
- Wang, S.; Gou, J. BLDCM Torque Ripple Suppression Method Based on Improved Quasi-Z Source Network. In Proceedings of the 2022 3rd International Conference on Advanced Electrical and Energy Systems (AEES), Lanzhou, China, 23–25 September 2022; pp. 446–451. [Google Scholar] [CrossRef]
- Balamurugan, K.; Nandalal, V.; Suresh, G.; Shankar, B.M.; Srirevathi, B. Comparative Analysis of CUK, SEPIC, Buck-Boost and ZETA Converters to Reduce Commutation Torque Ripple in BLDC Motor. In Proceedings of the 2022 International Conference on Advanced Computing Technologies and Applications (ICACTA), Coimbatore, India, 4–5 March 2022; pp. 1–7. [Google Scholar] [CrossRef]
- Singh, B.; Singh, S. Isolated Zeta PFC converter based voltage controlled PMBLDCM drive for air-conditioning application. In Proceedings of the India International Conference on Power Electronics 2010 (IICPE2010), New Delhi, India, 28–30 January 2011; pp. 1–5. [Google Scholar] [CrossRef]
- Kumar, A.; Singh, B. Modified Ćuk Converter For Power Factor Correction in BLDC Motor Driven Ceiling-Fan. IEEE J. Emerg. Sel. Top. Ind. Electron. 2024, 1–10. [Google Scholar] [CrossRef]
- Zhou, L.; Shi, T.; Deng, J.; Chen, W.; Cao, Y.; Li, X. Torque Ripple Reduction Strategy for Brushless DC Motor Based on Output Mode Reconstruction of Cuk Converter. IEEE J. Emerg. Sel. Top. Power Electron. 2023, 11, 5244–5255. [Google Scholar] [CrossRef]
- Shchur, I. Bidirectional Single-Stage Zeta-SEPIC DC-AC Converter for Traction BLDC Motors. In Proceedings of the 2022 IEEE 3rd KhPI Week on Advanced Technology (KhPIWeek), Kharkiv, Ukraine, 3–7 October 2022; pp. 1–6. [Google Scholar] [CrossRef]
- Patil Rahul, D.; Saravana Prakash, P. A Bridge-less IPOS PFC Zeta Converter Fed BLDC Motor Drive for Home Appliances. In Proceedings of the 2022 IEEE International Conference on Power Electronics, Drives and Energy Systems (PEDES), Jaipur, India, 14–17 December 2022; pp. 1–6. [Google Scholar] [CrossRef]
- Xia, C.; Li, P.; Li, X.; Shi, T. Series IGBT Chopping Strategy to Reduce DC-Link Capacitance for Brushless DC Motor Drive System. IEEE J. Emerg. Sel. Top. Power Electron. 2017, 5, 1192–1204. [Google Scholar] [CrossRef]
- Jiang, G.; Xia, C.; Chen, W.; Shi, T.; Li, X.; Cao, Y. Commutation Torque Ripple Suppression Strategy for Brushless DC Motors with a Novel Noninductive Boost Front End. IEEE Trans. Power Electron. 2018, 33, 4274–4284. [Google Scholar] [CrossRef]




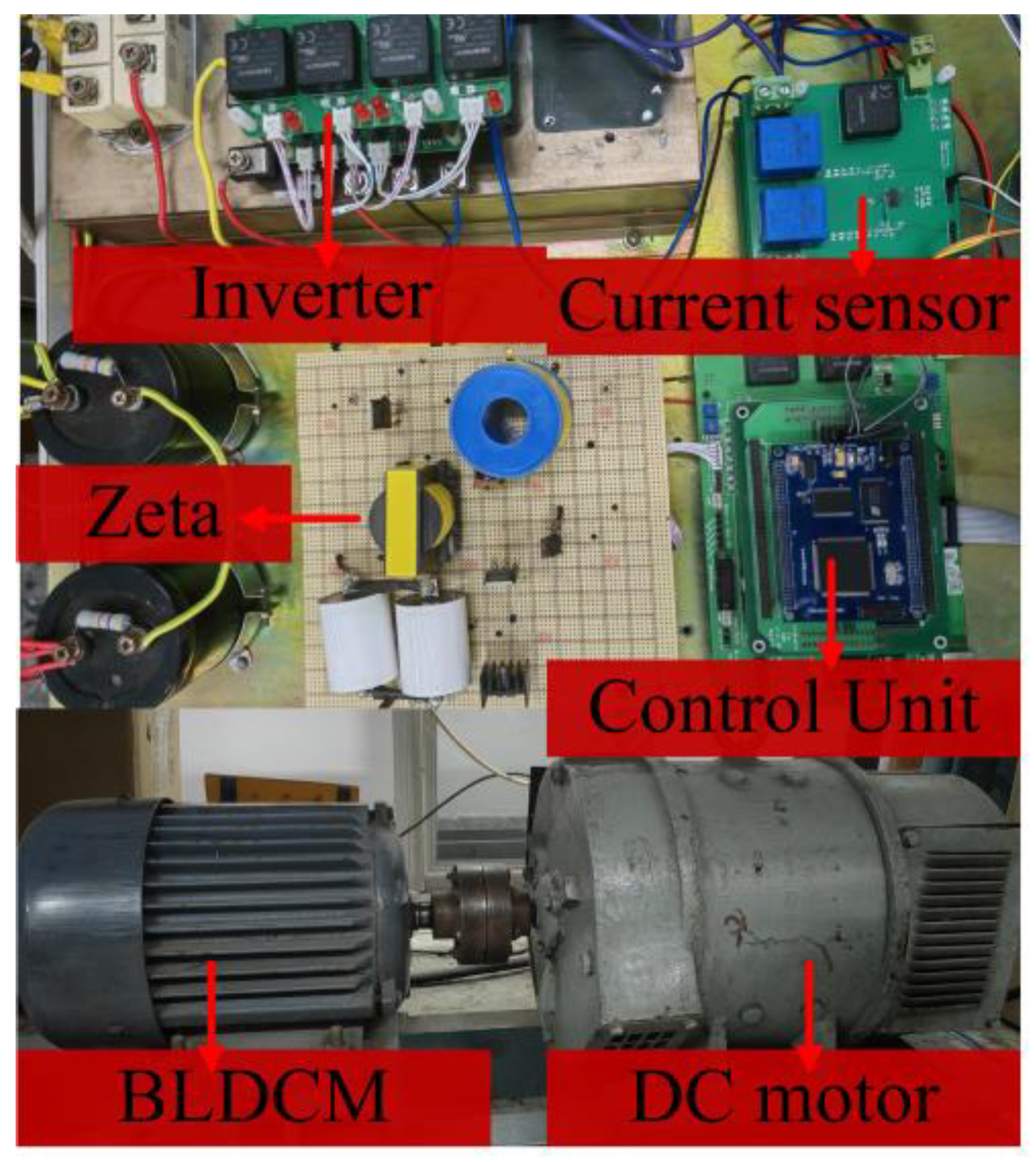





| Parameters | Symbol | Value | |
|---|---|---|---|
| BLDCM | Rated voltage (V) | UN | 200 |
| Rated power (kW) | PN | 3.5 | |
| Pole pairs | np | 4 | |
| Phase resistance (Ω) | R | 0.684 | |
| Phase inductance (mH) | L | 1.234 | |
| Back-EMF coefficient (V/(rad/s)) | k | 0.528 | |
| Zeta | Inductance of Zeta converter (mH) | LA and LB | 1 |
| Capacitance of Zeta converter (μF) | CA and CB | 1000 |
| Speed (r/min) | Torque (Nm) | Traditional Control Strategy | Proposed Method | ||
|---|---|---|---|---|---|
| Torque Ripple (Kr) | Standard Deviation (σT) | Torque Ripple (Kr) | Standard Deviation (σT) | ||
| 1000 | 10 | 30% | 2.7 Nm | 15% | 1.5 Nm |
| 1500 | 20 | 45% | 3.9 Nm | 20% | 2 Nm |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yin, T.; Yang, W.; Zhang, W.; Wu, M.; Yu, X.; Han, X. Research on Suppressing Commutation Torque Ripple of BLDCM Based on Zeta Converter. Machines 2024, 12, 592. https://doi.org/10.3390/machines12090592
Yin T, Yang W, Zhang W, Wu M, Yu X, Han X. Research on Suppressing Commutation Torque Ripple of BLDCM Based on Zeta Converter. Machines. 2024; 12(9):592. https://doi.org/10.3390/machines12090592
Chicago/Turabian StyleYin, Tao, Wanli Yang, Wenxian Zhang, Meng Wu, Xiugang Yu, and Xingchang Han. 2024. "Research on Suppressing Commutation Torque Ripple of BLDCM Based on Zeta Converter" Machines 12, no. 9: 592. https://doi.org/10.3390/machines12090592
APA StyleYin, T., Yang, W., Zhang, W., Wu, M., Yu, X., & Han, X. (2024). Research on Suppressing Commutation Torque Ripple of BLDCM Based on Zeta Converter. Machines, 12(9), 592. https://doi.org/10.3390/machines12090592






