Performance Analysis of New One-Piece Iron Roughneck and Its Spinning Mechanism
Abstract
1. Introduction
2. Structural Design and Theoretical Analysis
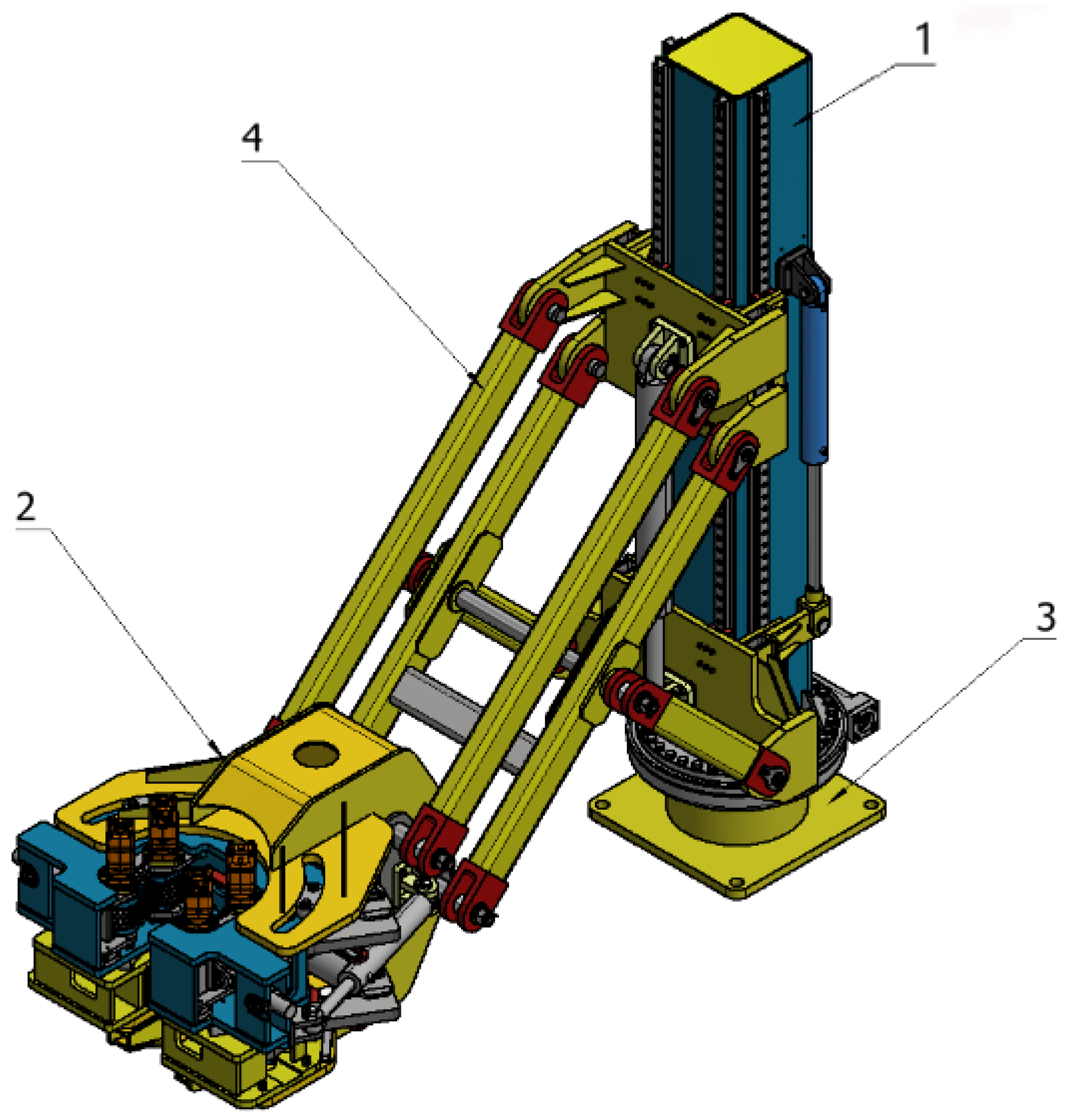
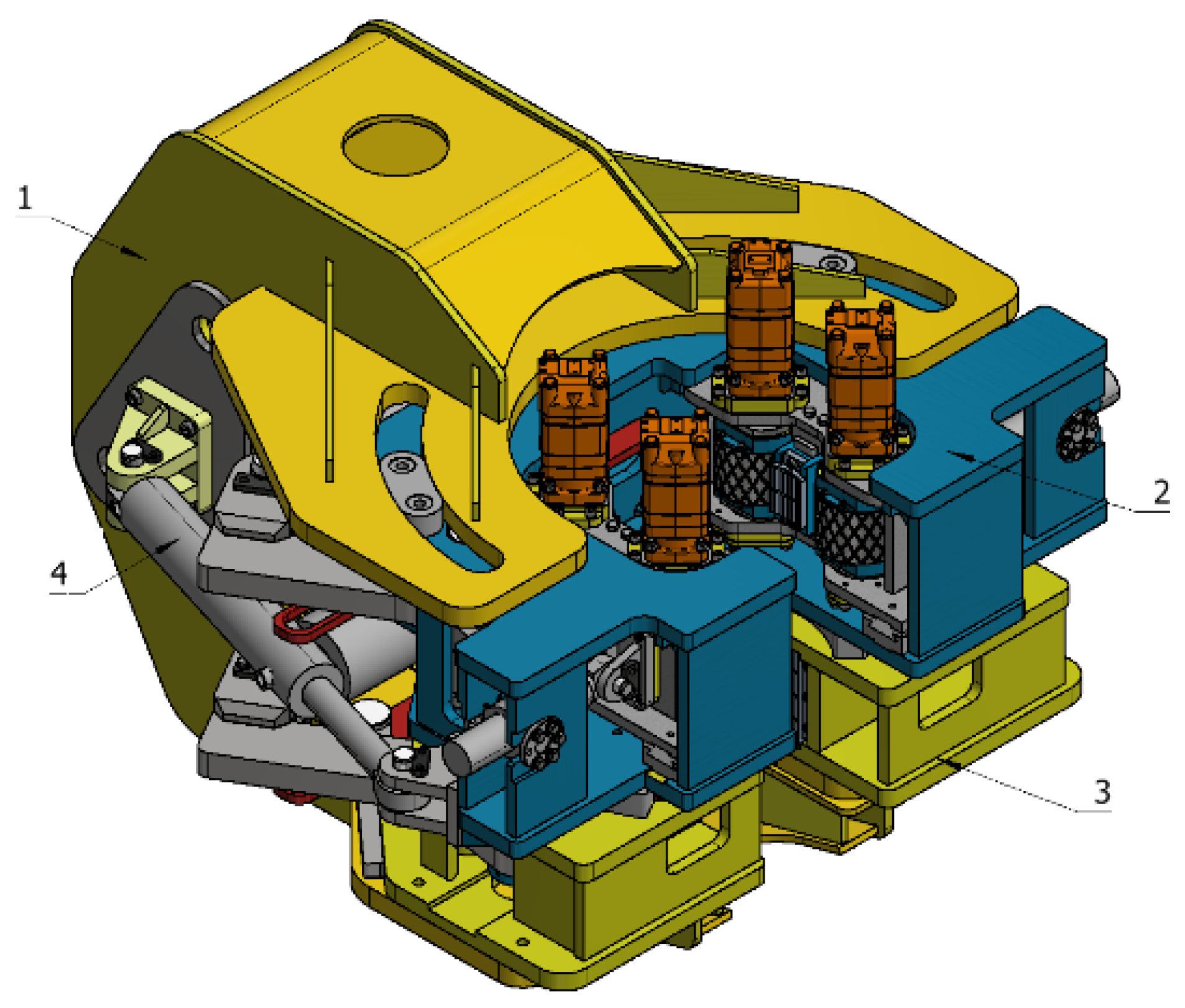
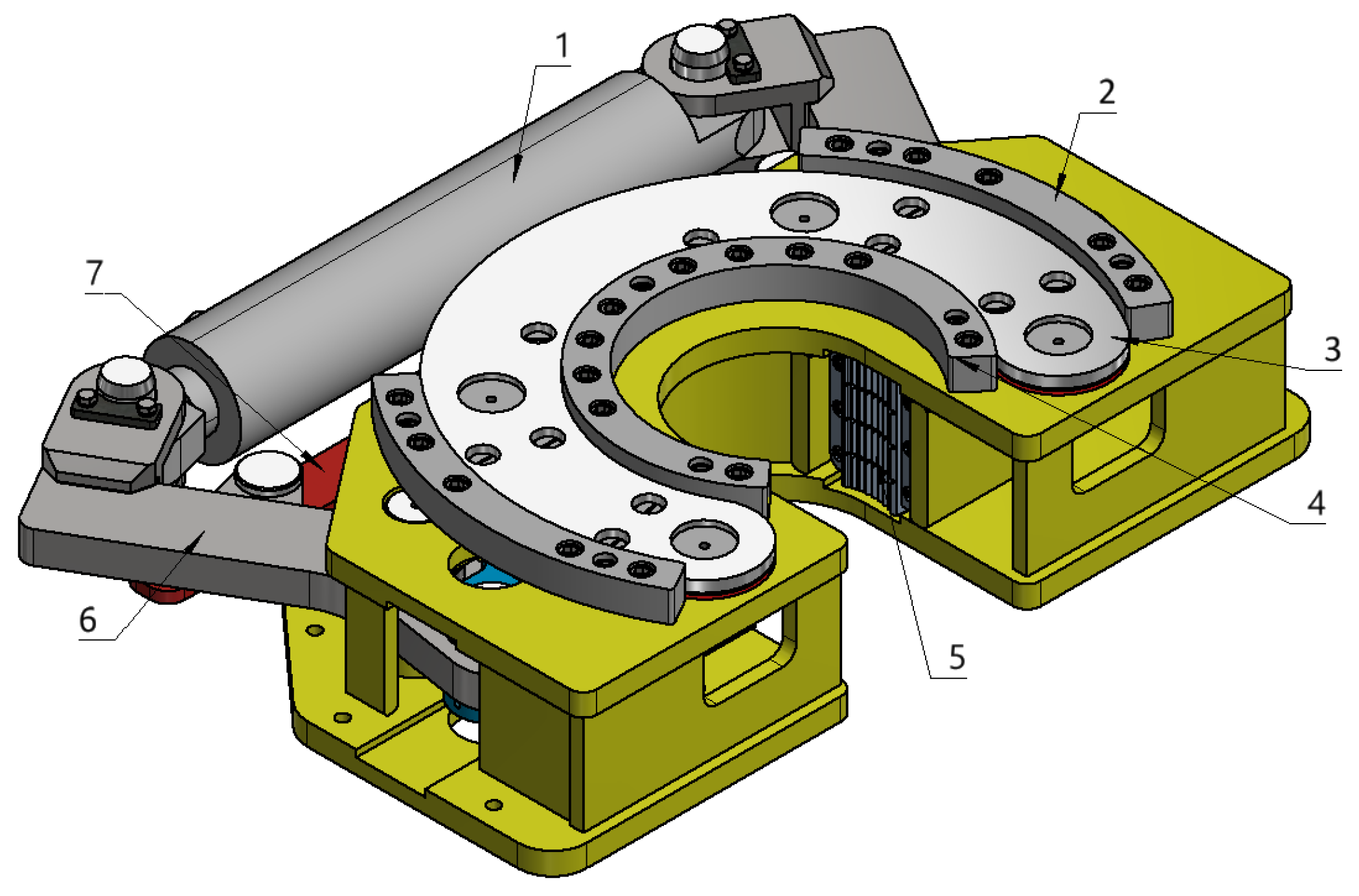
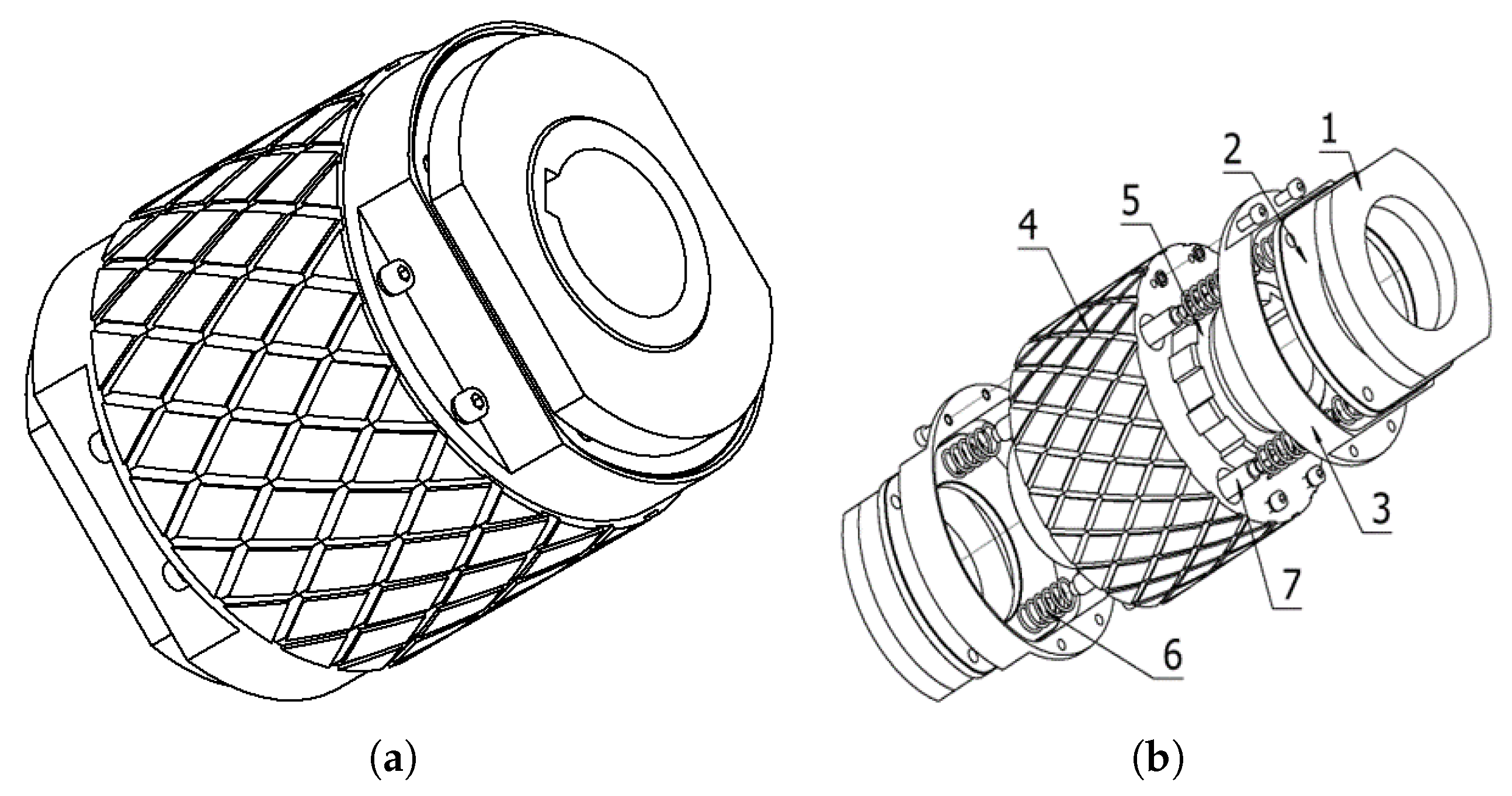
2.1. The Overall Structure of the Iron Roughneck
2.2. Main Performance Parameters of Iron Roughneck
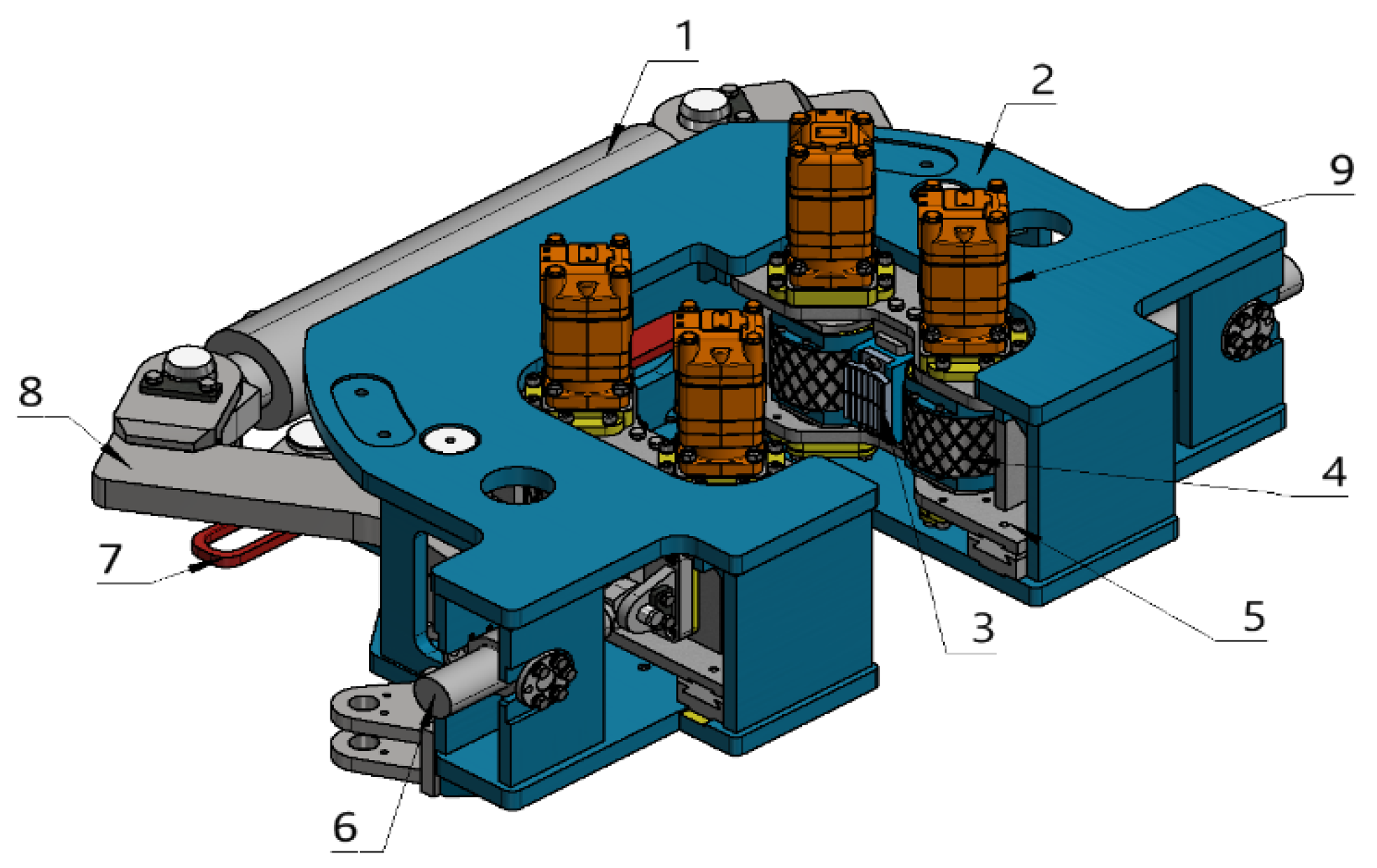
2.3. Clamp Body Structure Design and Research
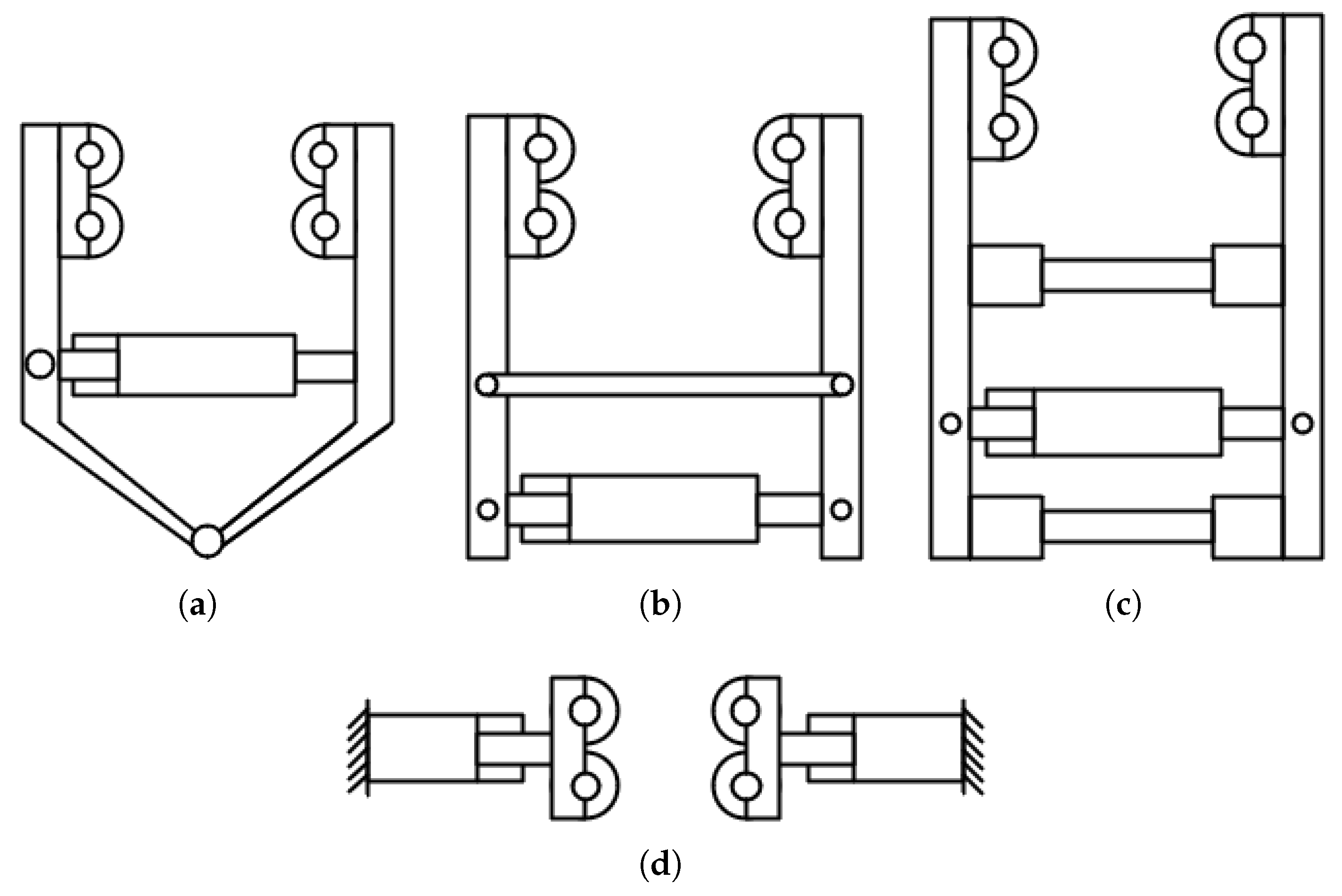
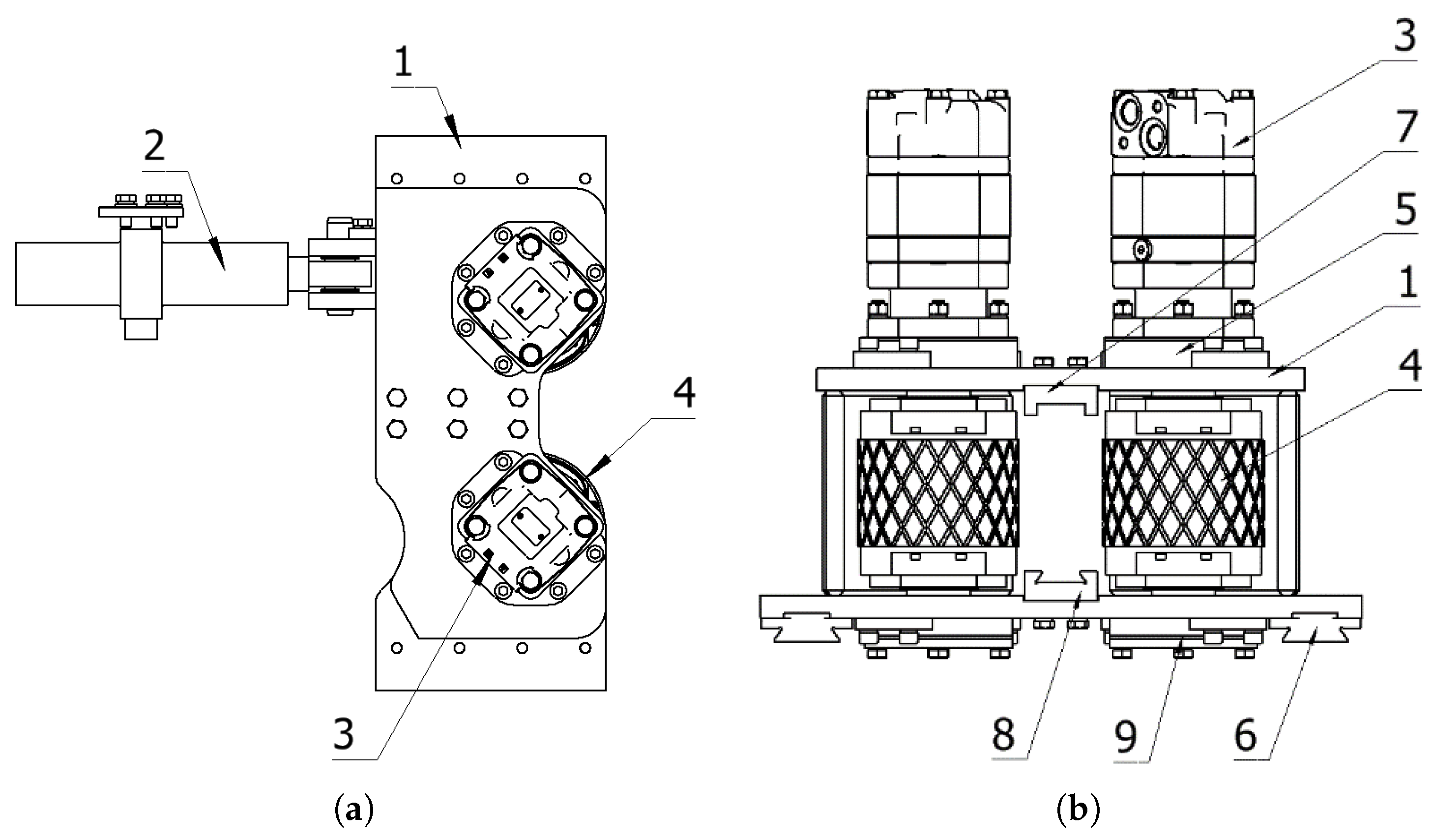
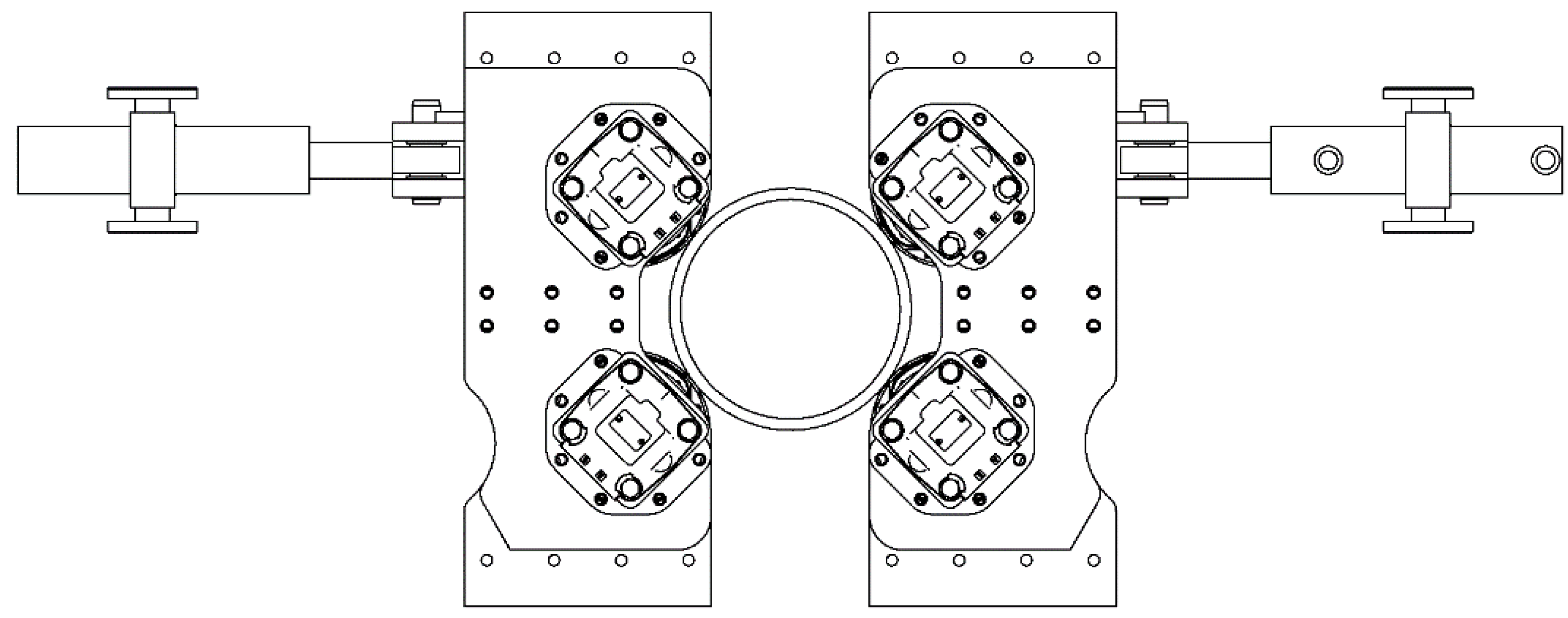
2.3.1. Design and Research of Spin Buckle Mechanism
2.3.2. Clamping Mechanism Design and Research
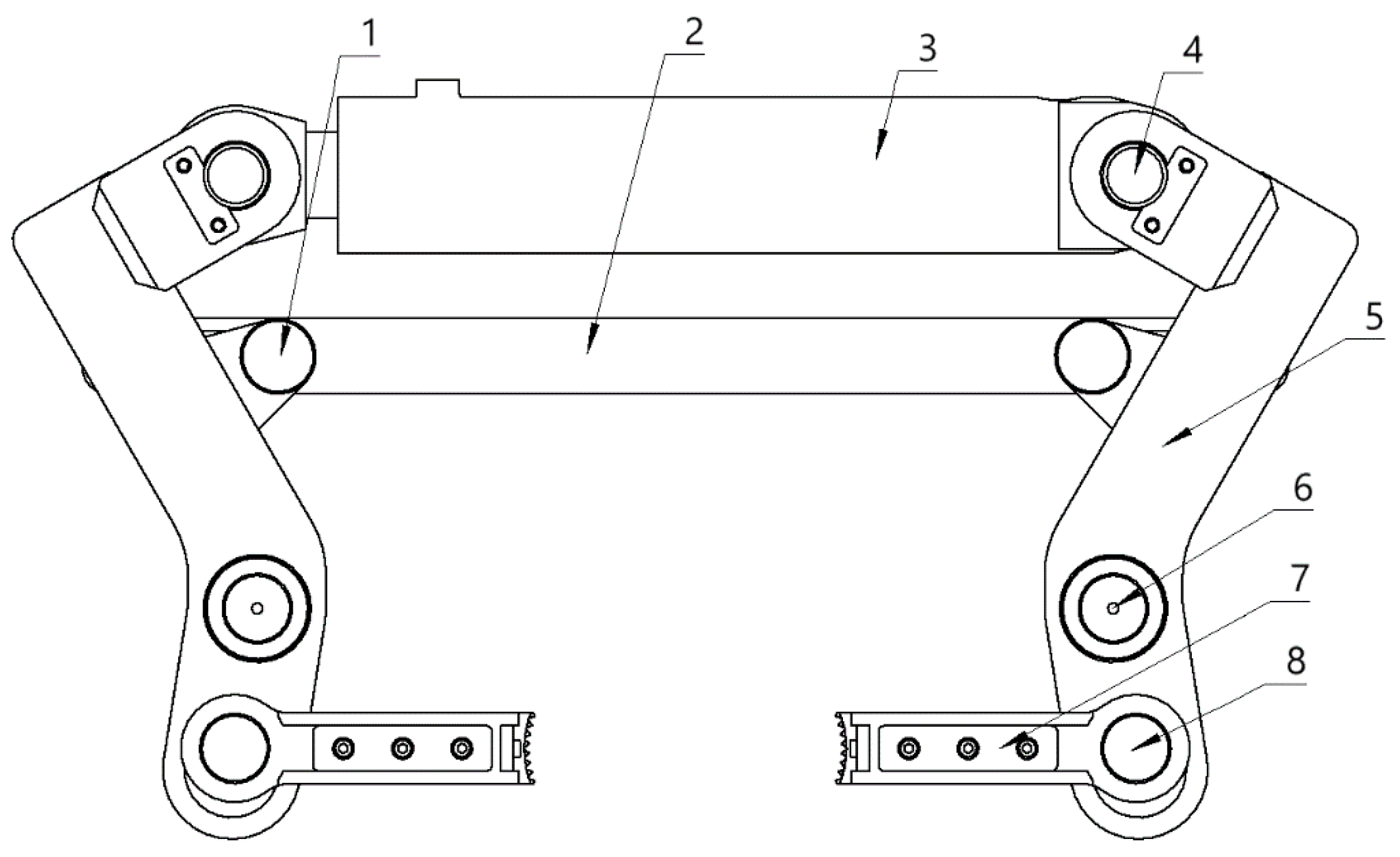
2.3.3. Clamp Body Structure Design and Research
2.4. Theoretical Analysis of Spinning Roller
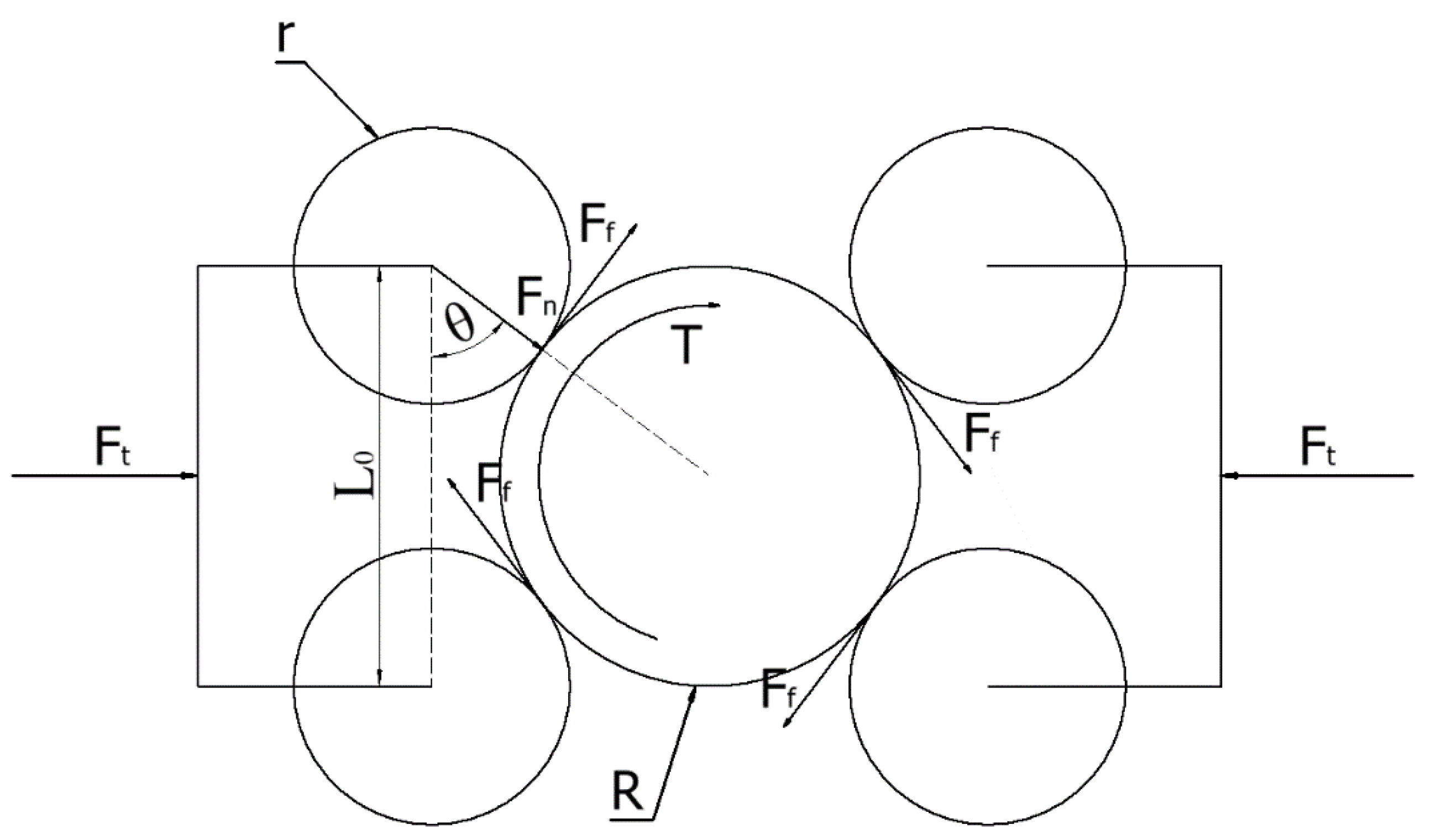
2.4.1. Force Analysis of Spin Buckle Mechanism
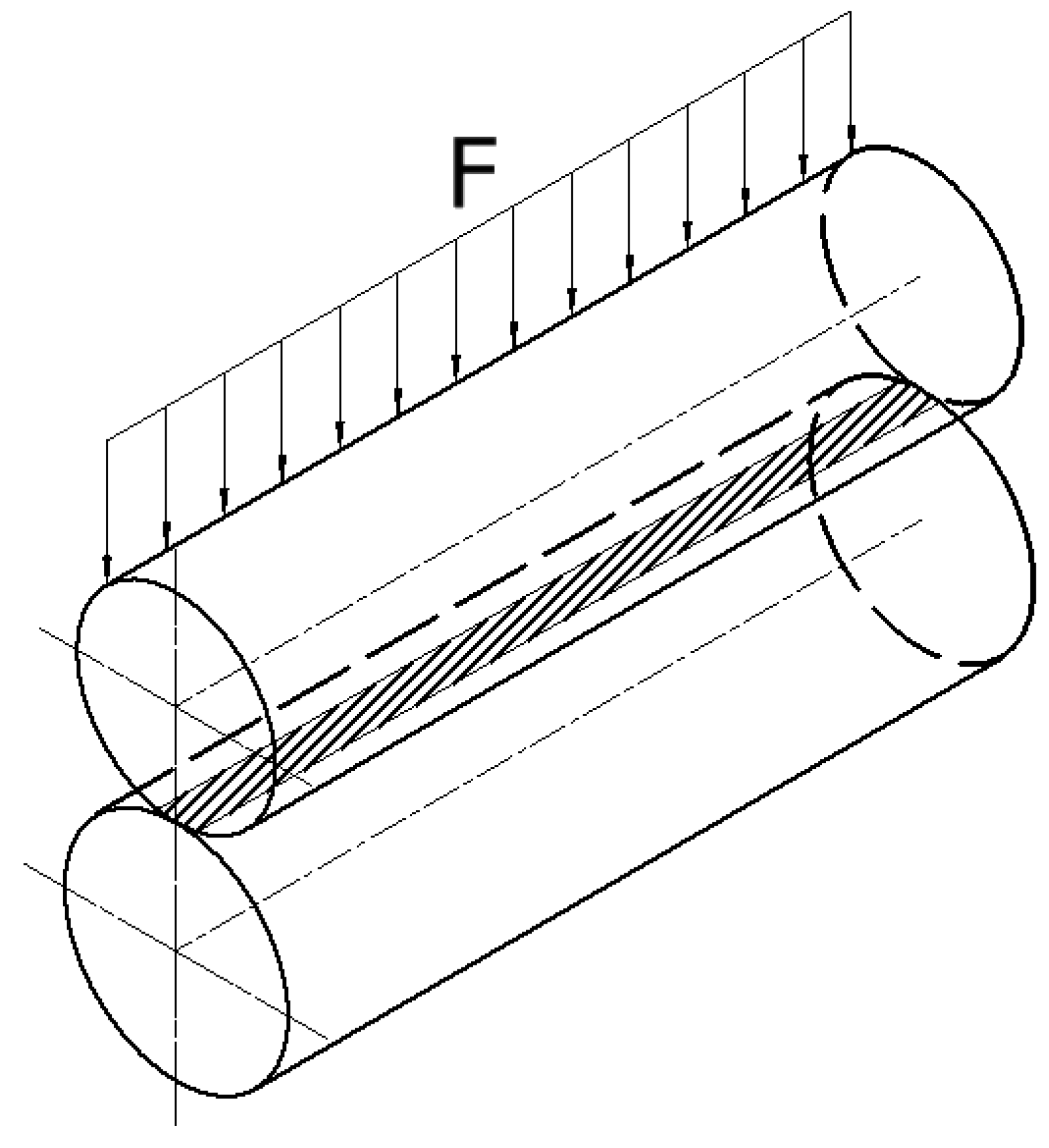
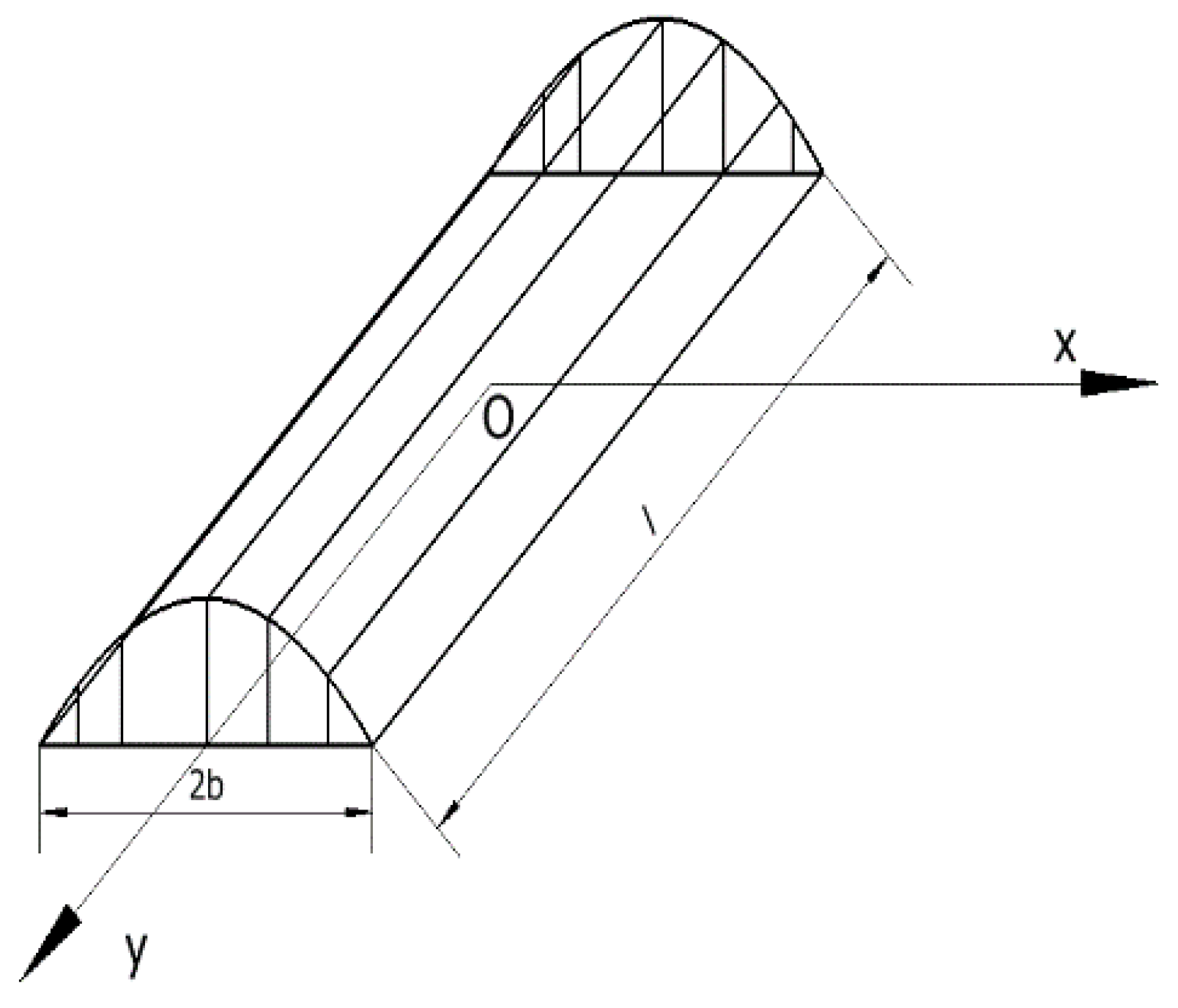
2.4.2. Theoretical Analysis of Spinning Roller–Drilling Tool Contact
- The contact body is elastic and has a uniform material distribution.
- The surface of the contact region is an ideally smooth quadratic surface without considering friction.
- The size of the contact surface is very small compared to the radius of curvature of the contacting body surface.
- The contact stress distribution is adapted to the shape of the contact area and the contact surface.
2.4.3. Rolling Friction Analysis between Spinning Roller and Drilling Tools
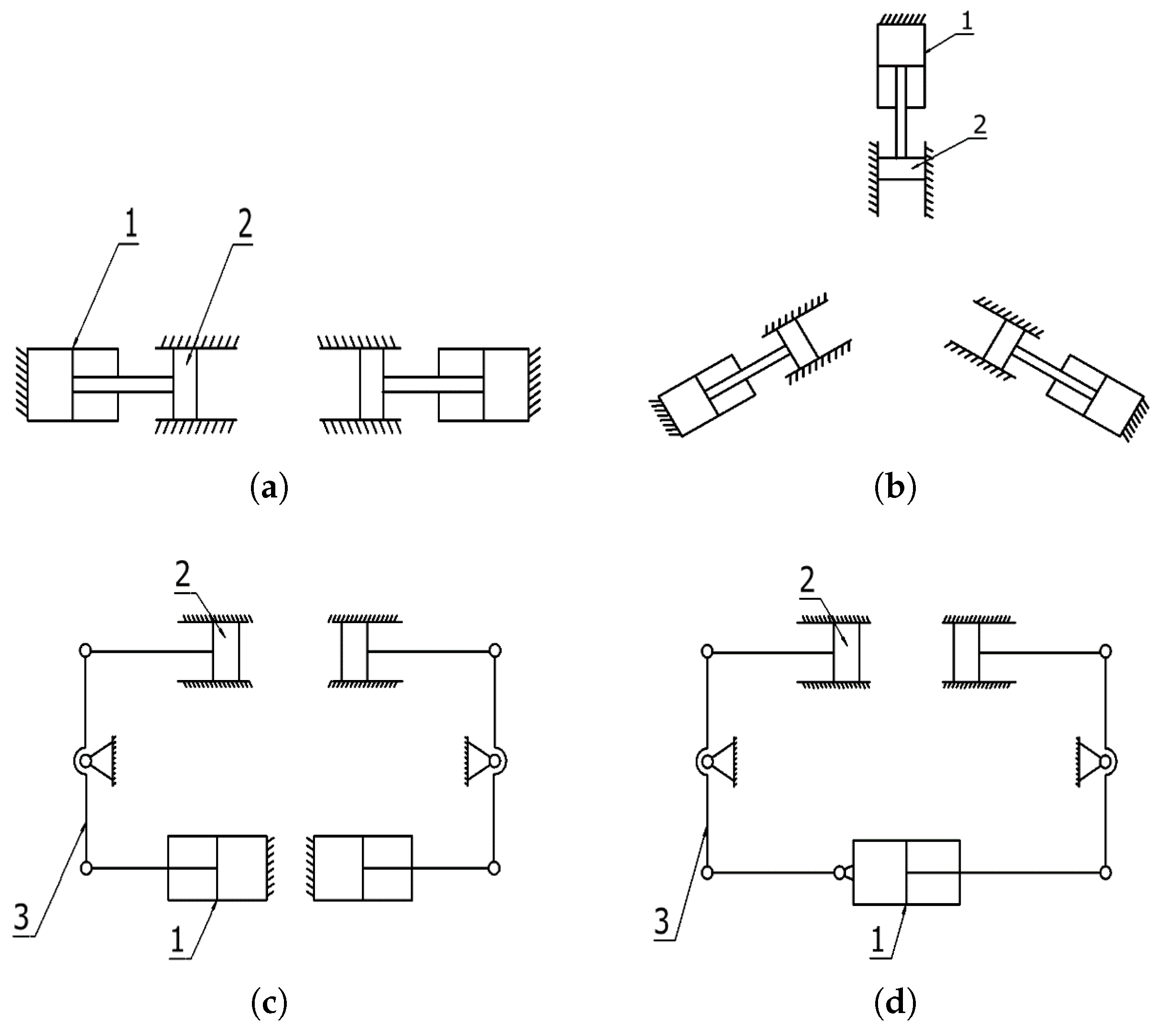
2.4.4. Spin Buckle Roller Axial Floating Device and Analysis
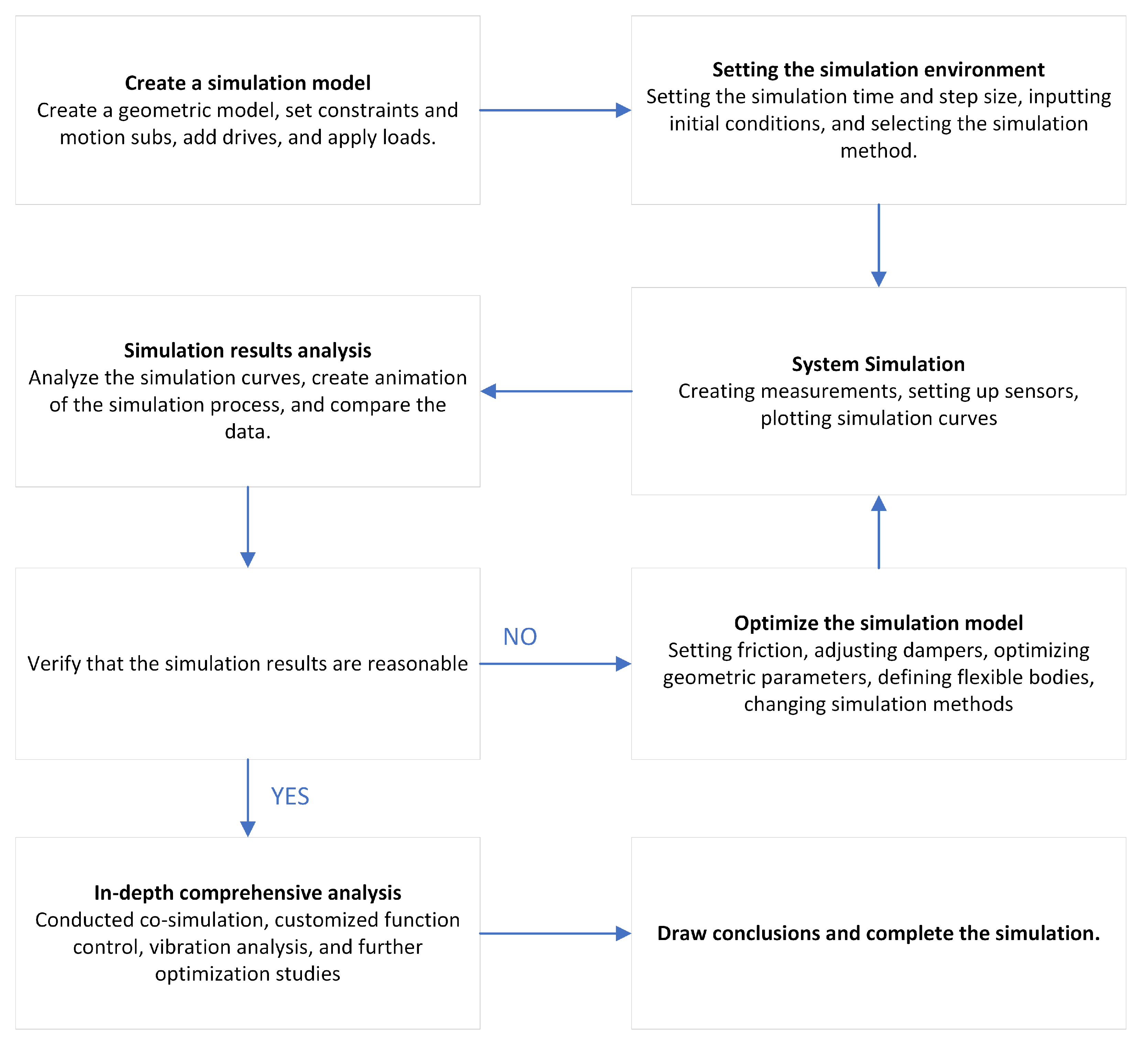
2.5. Simulation Experiment
2.5.1. Simulation Process
2.5.2. Driver Addition and Simulation Environment Setup
3. Results and Discussion
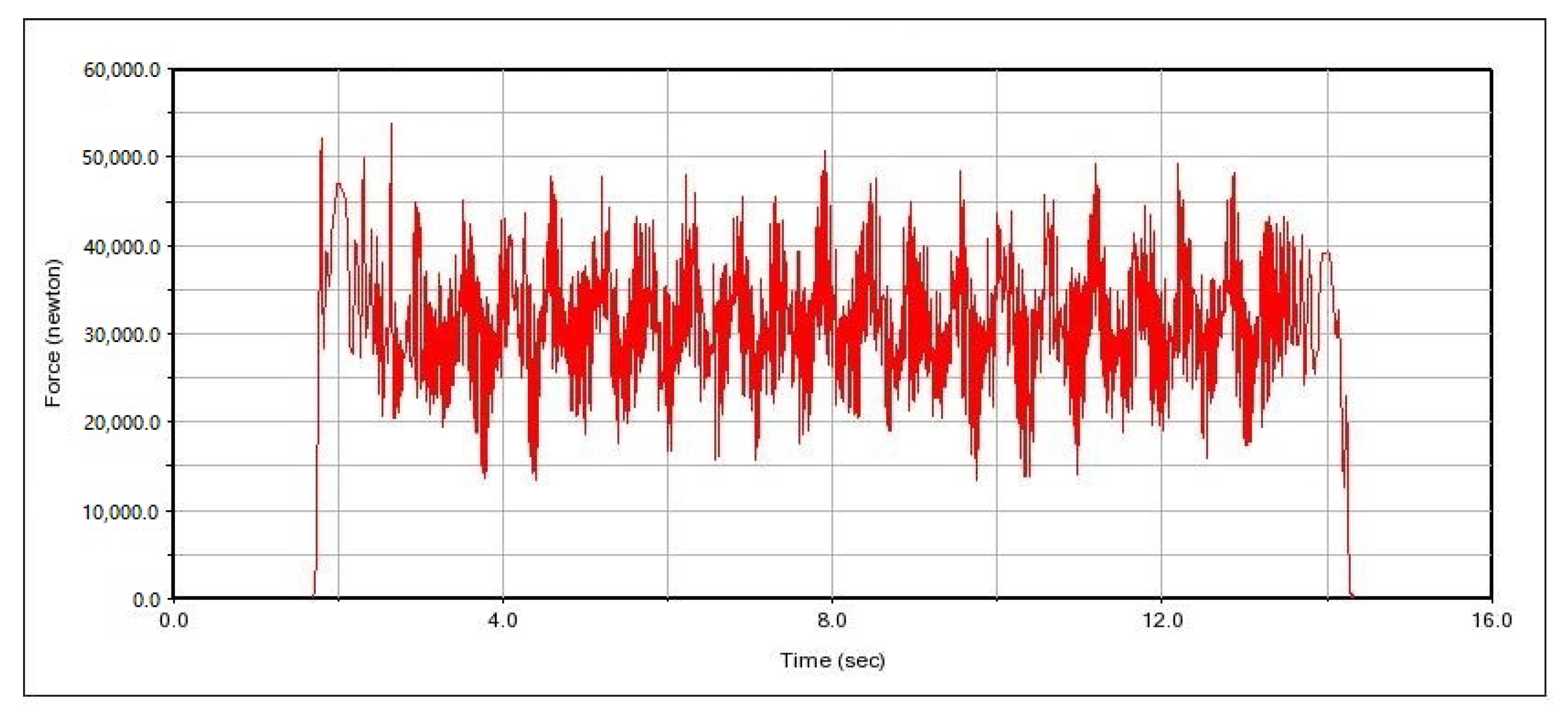
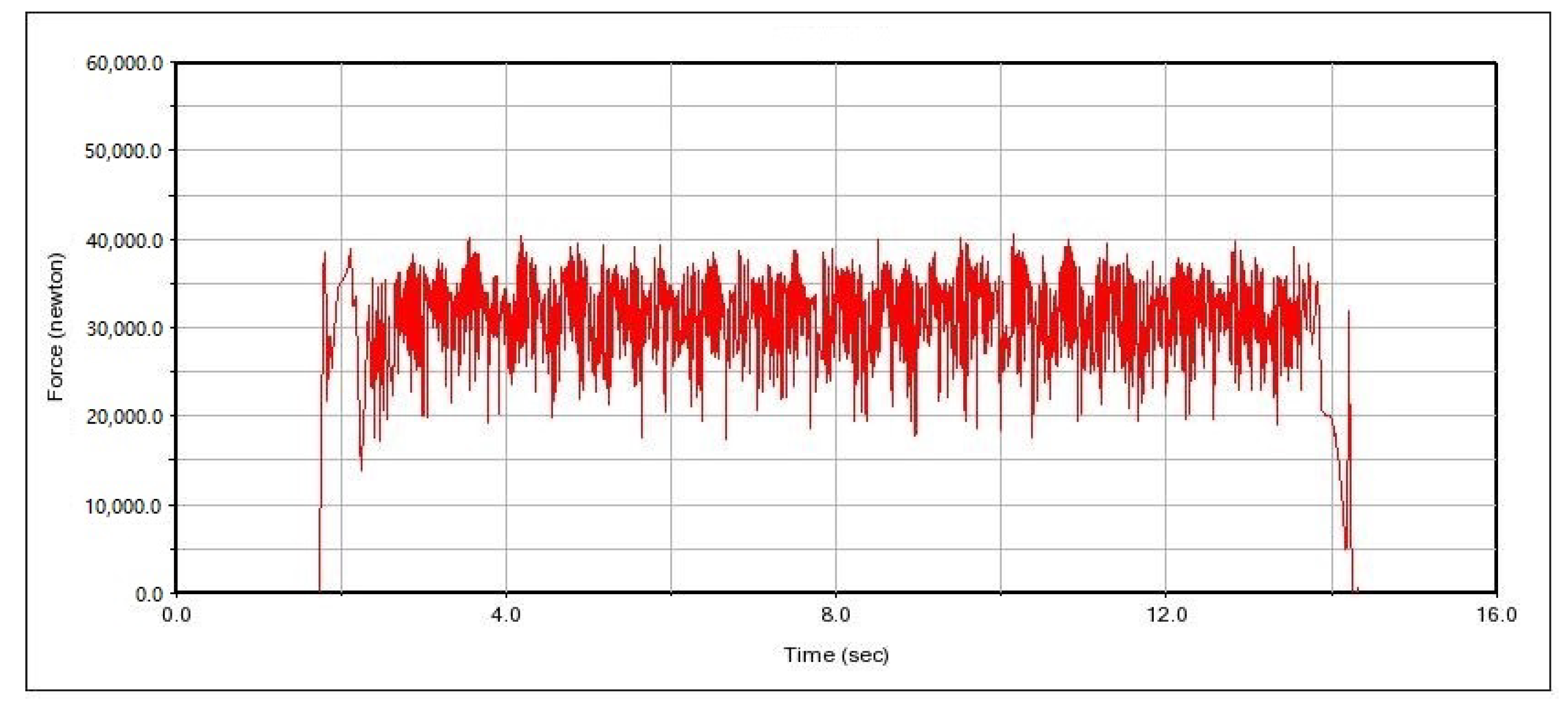
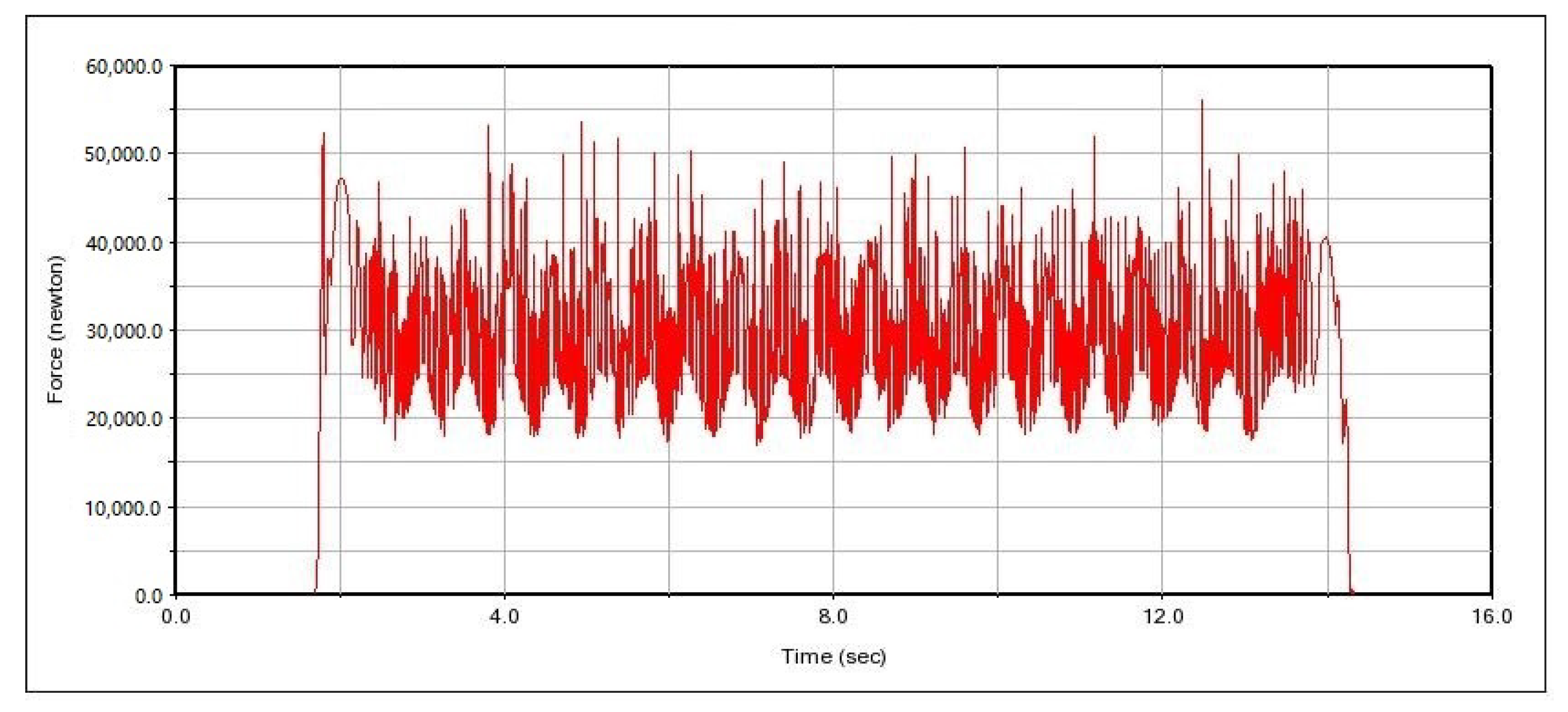
3.1. Simulation and Experimental Analysis of the Contact Force between the Spinning Roller and Drilling Tools
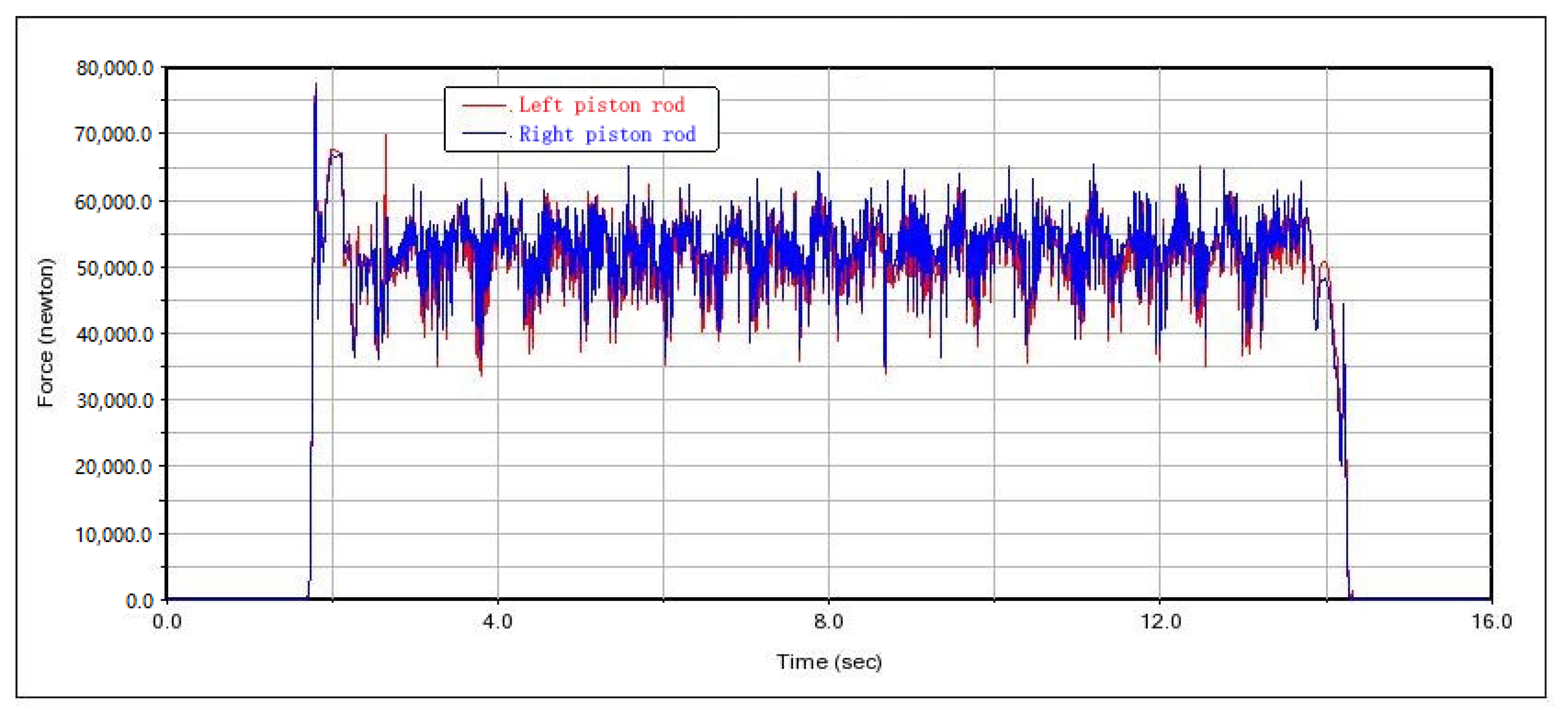
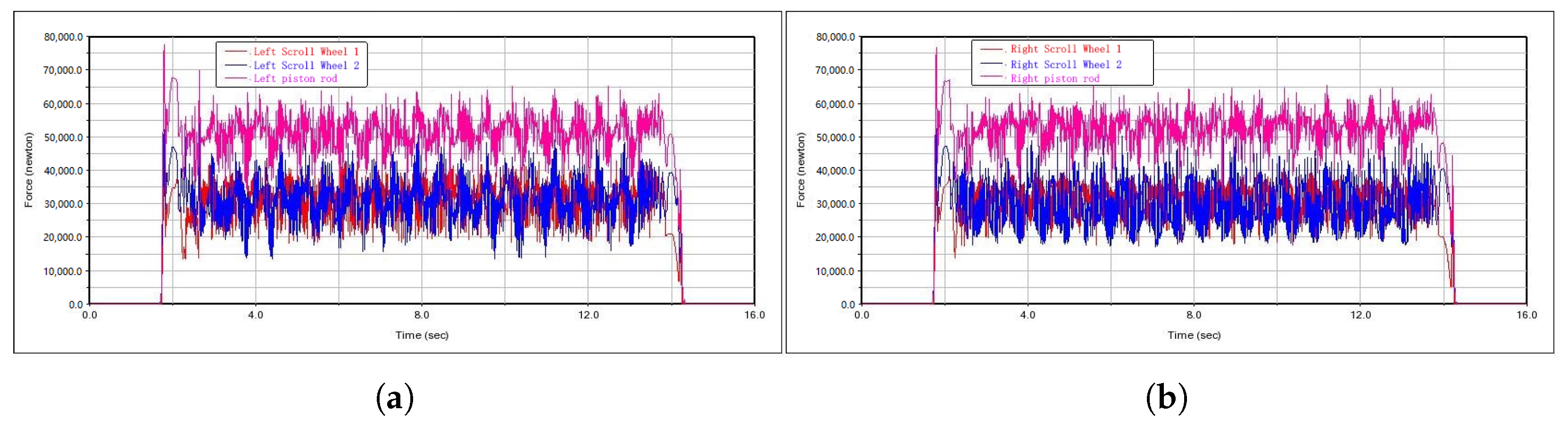
3.2. Comparative Simulation and Experimental Analysis of Force on Piston Rod of Clamping Cylinder
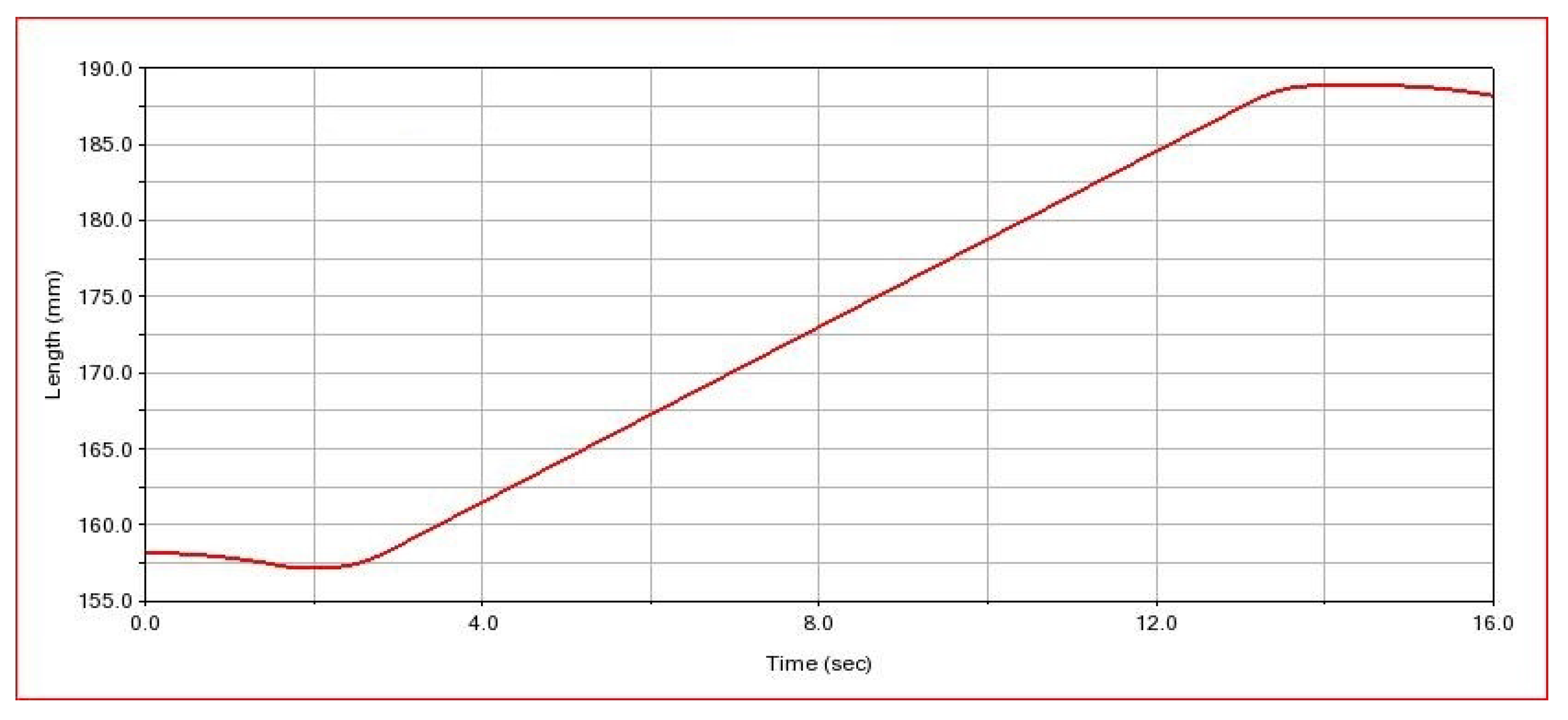
3.3. Experimental Analysis of Axial Floating Simulation of Spinning Buckle Roller
4. Conclusions
- Aiming at the current problems of poor centering performance of iron roughneck clamp body, low efficiency of spin buckle, complex floating mechanism, and poor floating effect, we have researched and designed a new one-piece iron roughneck.
- The contact and friction between the spin buckle roller and the drilling tool are analyzed from the contact mechanics point of view, and the spin buckle roller with axial floating adaptive function is designed, and the axial maximum floating of the spin buckle roller is made to be 31.68 mm.
- A new synchronized alignment mechanism is designed so that the four spin buckle rollers can synchronize the contact force output of about 30 kN to reduce machine damage.
- The kinematics and dynamics of the spin buckle mechanism are simulated and analyzed with the help of ADAMS simulation software. The dynamic simulation model of the spin buckle mechanism is established, the simulation analysis of the spin buckle mechanism is carried out, and the relevant parameter curves of the spin buckle mechanism are obtained. The performance of the spin buckle mechanism is analyzed by combining with the simulation results and comparing with the theoretical analysis results, which verifies the correctness of the theoretical analysis and the reasonableness of the simulation results.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Hongsheng, Z.; Hao, Y.; Jinfeng, Z. Current Status and Prospects of Iron Drillers. Oil Mine Mach. 2008, 37, 12–16. [Google Scholar]
- Yong, Z.; Qingyou, L.; Xiaowei, C. Development of a new type of lightweight iron driller. Oil Mine Mach. 2007, 36, 73–75. [Google Scholar]
- Mingqian, L.; Jiqing, H. Development and Application of Torque Spinning Machine for Oil Drilling Tools. Pet. Mach. 2005, 33, 27–28. [Google Scholar]
- Zhang, K.; Liu, Y.; Jia, H.; Yan, F.; Xue, G. Research on a Three-Dimensional Fuzzy Active Disturbance Rejection Controller for the Mechanical Arm of an Iron Roughneck. Processes 2023, 11, 1409. [Google Scholar] [CrossRef]
- Shuai, J.Z. A Study of Trajectory Planning for Electric Iron Roughnecks. Master’s Thesis, Xi’an Petroleum University, Xi’an, China, 2023. [Google Scholar]
- Fengqing, Z. ST-80C iron roughneck hydraulic system modification. China Pet. Chem. Stand. Qual. 2024, 44, 148–149+152. [Google Scholar]
- Tangyi, Y. Simulation and Design of Hydraulic System for TZG/G200 Rail Type Iron Roughneck. Master’s Thesis, Northeast Petroleum University, Daqing, China, 2016. [Google Scholar]
- Shengjie, H. Design and Realization of Electric Iron Roughneck Controller. Master’s Thesis, Xi’an Petroleum University, Xi’an, China, 2023. [Google Scholar]
- Bin, H.K. Mechanical Study of TZG216-110 Iron Roughneck Punching Buckle Pliers. Master’s Thesis, Lanzhou University of Science and Technology, Lanzhou, China, 2009. [Google Scholar]
- Sun, Y.; Zhang, F.; Wang, Q.; Gao, K. Application of “Crust 1” 10k ultra-deep scientific drilling rig in Songliao Basin Drilling Project (CCSD-SKII). J. Pet. Sci. Eng. 2016, 145, 222–229. [Google Scholar]
- Man, X.; Yongbai, S.; Xiaoli, L. Design of iron driller’s spinning mechanism. In Proceedings of the 18th Annual National Academic Exchange Conference on Prospecting Engineering (Geotechnical Drilling and Excavation Engineering), Harbin, China, 19–22 October 2015; China Geological Publishing House: Beijing, China, 2015; pp. 142–145. [Google Scholar]
- Xiaotong, X. Design and Analysis of New Iron Driller. Master’s Thesis, Dalian University of Technology, Dalian, China, 2018. [Google Scholar]
- Sha, Y.; Li, Q.; Zhao, X. Study on the Position Deviation between the Iron Roughneck’s Spin-Rollers and the Drilling Tool. Appl. Sci. 2022, 12, 5827. [Google Scholar] [CrossRef]
- Li, Z.; Chen, L.; Zhong, Y.; Wang, L. Study on Sinusoidal Post-Buckling Deformation of Coiled Tubing in Horizontal Wells Based on the Separation Constant Method. Machines 2023, 11, 563. [Google Scholar] [CrossRef]
- Wang, P.; Xu, H.; Ma, H.; Han, H.; Yang, Y. Effects of three types of bearing misalignments on dynamic characteristics of planetary gear set-rotor system. Mech. Syst. Signal Process. 2022, 169, 108736. [Google Scholar]
- Guo, Y.; Mi, H.; Habibi, M. Electromechanical energy absorption, resonance frequency, and low-velocity impact analysis of the piezoelectric doubly curved system. Mech. Syst. Signal Process. 2021, 157, 107723. [Google Scholar]
- Johnson, K. Contact mechanics and the wear of metals. Wear 1995, 190, 162–170. [Google Scholar]
- Sánchez, E.; Cosimo, A.; Brüls, O.; Cardona, A.; Cavalieri, F.J. Non-smooth numerical solution for Coulomb friction, rolling and spinning resistance of spheres applied to flexible multibody system dynamics. Multibody Syst. Dyn. 2023, 59, 69–103. [Google Scholar]
- Xiaojun, L. Comparative Study of Fatigue Life of Roll Materials under Frictional Wear Conditions. Master’s Thesis, Wuhan Institute of Technology, Wuhan, China, 2006. [Google Scholar]
- Ozaki, S.; Tsuda, K.; Tominaga, J. Analyses of static and dynamic behavior of coned disk springs: Effects of friction boundaries. Thin-Walled Struct. 2012, 59, 132–143. [Google Scholar]
- Tahvilzadeh, M.; Aliyari-Shoorehdeli, M.; Razi-Kazemi, A.A. Model-Aided Approach for Intelligent Fault Detection System for SF 6 High-Voltage Circuit Breaker with Spring Operating Mechanism. IEEE Trans. Power Deliv. 2023, 38, 3356–3365. [Google Scholar]
- Wang, K.; Chen, G.; Wan, S.; Tian, P. Dynamic simulation analysis of CT26 operating mechanism of high-voltage circuit breaker based on ADAMS. In Proceedings of the 2016 International Conference on Advanced Electronic Science and Technology (AEST 2016), Shenzhen, China, 19–21 August 2016; Atlantis Press: Amsterdam, The Netherlands, 2016; pp. 834–843. [Google Scholar]
- Jin, M.; Yang, H.; Xie, Z.; Sun, K.; Liu, H. Ground simulation experiment verification of space robot with ADAMS and Simulink co-simulation. In Proceedings of the 2013 IEEE International Conference on Robotics and Biomimetics (ROBIO), Shenzhen, China, 12–14 December 2013; IEEE: Shenzhen, China, 2014; pp. 2529–2534. [Google Scholar]
- Kanchwala, H.; Chatterjee, A. ADAMS model validation for an all-terrain vehicle using test track data. Adv. Mech. Eng. 2019, 11, 1687814019859784. [Google Scholar]
- Yixuan, L.; Qing, X.; Yingmou, Z.; Zhuoyu, H. The Application of ADAMS Software to Vehicle Handling Stability: A Review. In Proceedings of the IncoME-VI and TEPEN 2021: Performance Engineering and Maintenance Engineering; Mechanisms and Machine Science. Springer: Cham, Switzerland, 2022; pp. 785–795. [Google Scholar]
- Zhao, X.Y. Simulation Analyzing for Spinner Institutions of Iron Roughneck Based on ADAMS. Appl. Mech. Mater. 2014, 488, 1264–1267. [Google Scholar]







| Designation | Performance and Parameters |
|---|---|
| Clamp body form | Integrated clamp |
| Clamping method | Parallel pairs of clamps |
| Pipe diameter range | 31/–81/ |
| Maximum screwing speed | 100 rpm |
| Spinning torque | 2400 N.m |
| Buckling torque | 108,500 N.m |
| Removal torque | 108,500 N.m |
| Rated pressure of hydraulic system | 25 MPa |
| Clamp body lift stroke | 550 mm |
| Punching angle range | −20°~20° |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sha, Y.; Han, D.; Chen, D.; Liu, C. Performance Analysis of New One-Piece Iron Roughneck and Its Spinning Mechanism. Machines 2024, 12, 575. https://doi.org/10.3390/machines12080575
Sha Y, Han D, Chen D, Liu C. Performance Analysis of New One-Piece Iron Roughneck and Its Spinning Mechanism. Machines. 2024; 12(8):575. https://doi.org/10.3390/machines12080575
Chicago/Turabian StyleSha, Yongbai, Donghe Han, Donghu Chen, and Congzhi Liu. 2024. "Performance Analysis of New One-Piece Iron Roughneck and Its Spinning Mechanism" Machines 12, no. 8: 575. https://doi.org/10.3390/machines12080575
APA StyleSha, Y., Han, D., Chen, D., & Liu, C. (2024). Performance Analysis of New One-Piece Iron Roughneck and Its Spinning Mechanism. Machines, 12(8), 575. https://doi.org/10.3390/machines12080575






