Study on Rubbing-Induced Vibration Characteristics Considering the Flexibility of Coated Casings and Blades
Abstract
1. Introduction
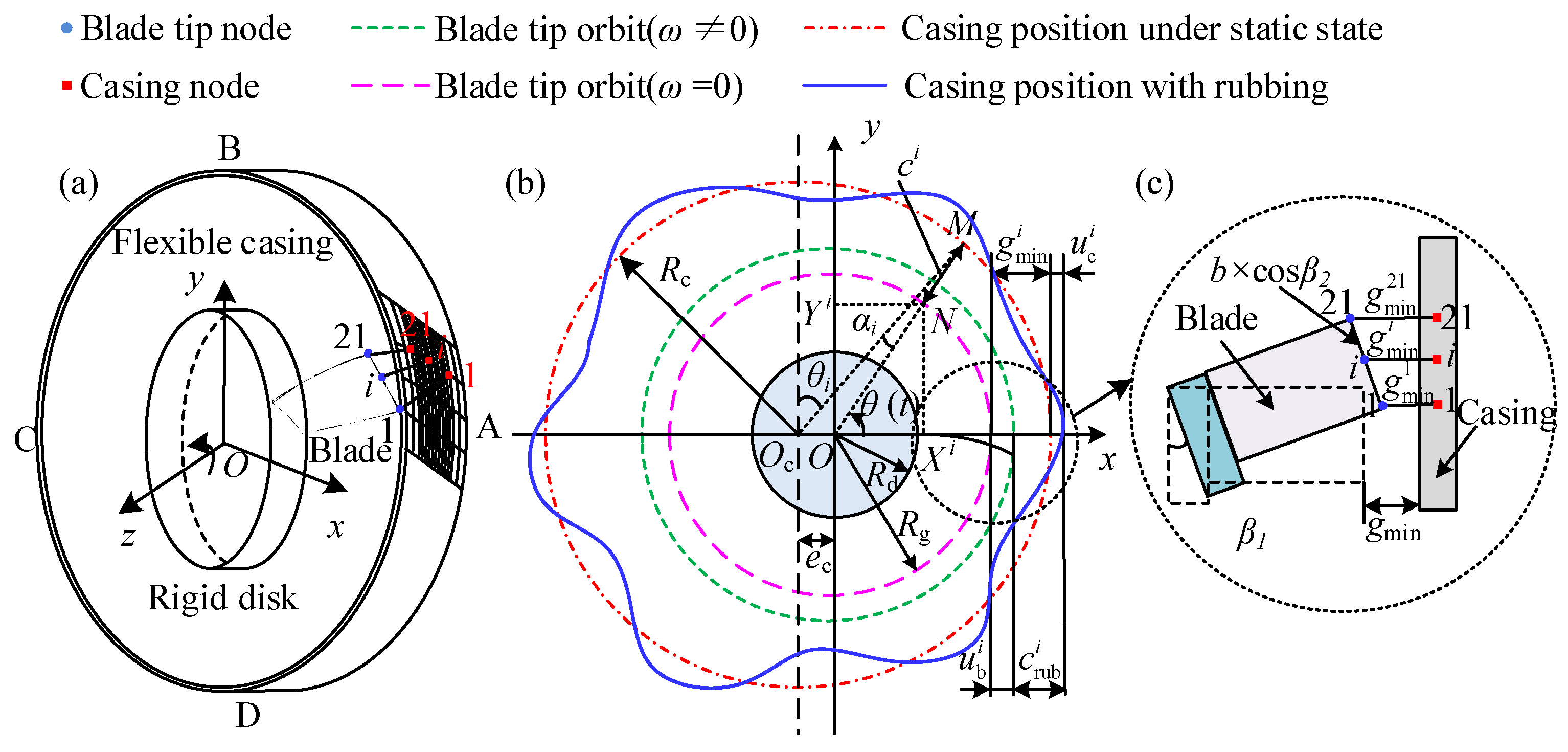
2. Dynamic Modeling of the Blade-Coated Casing System with Rubbing
- (1)
- Similar to Ref. [59], coating wear and temperature effects are not considered when rubbing occurs.
- (2)
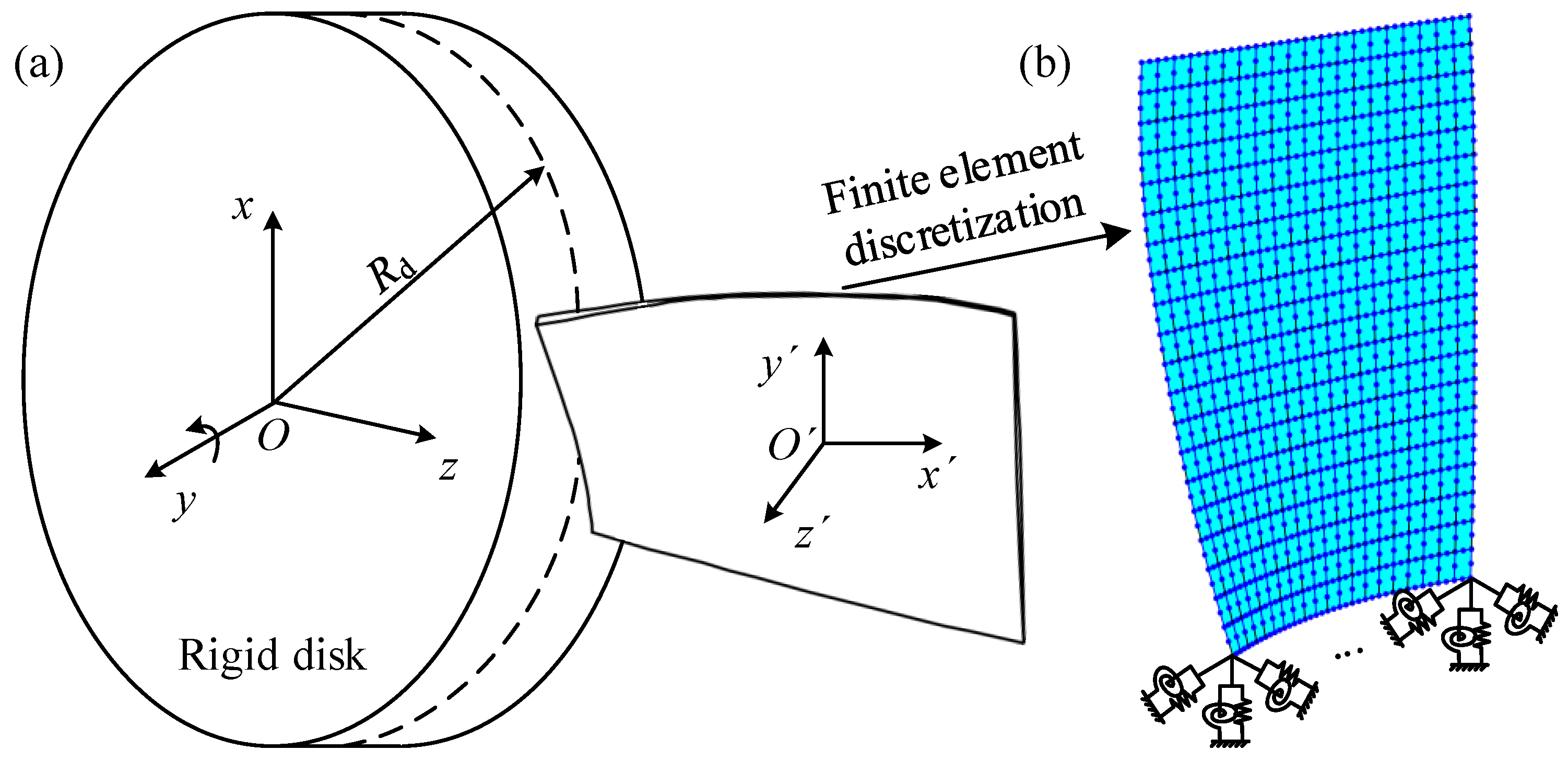
- The origin of the coordinate system is located at the center of mass of the rigid disk.

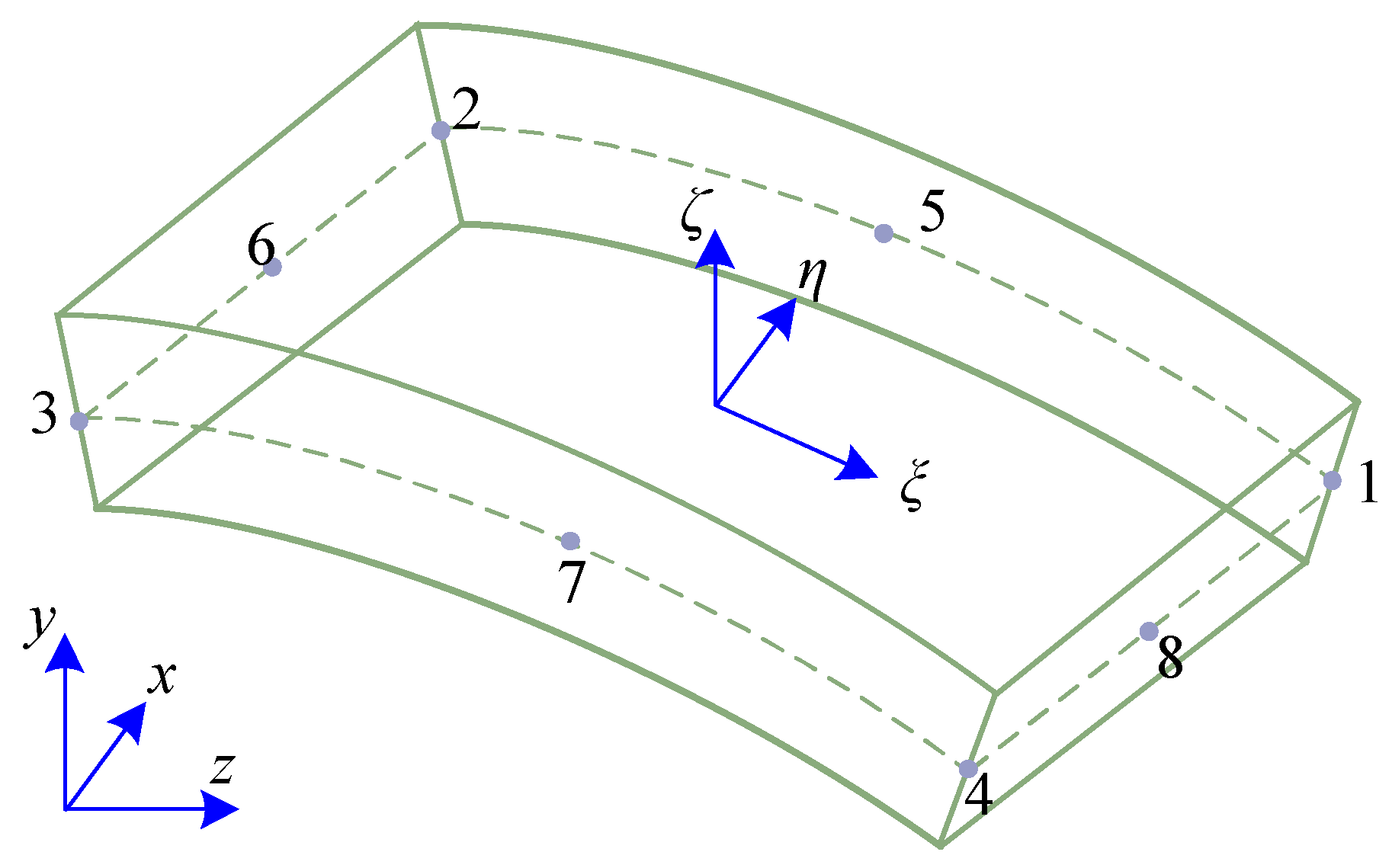
2.1. Dynamic Model of the Blade
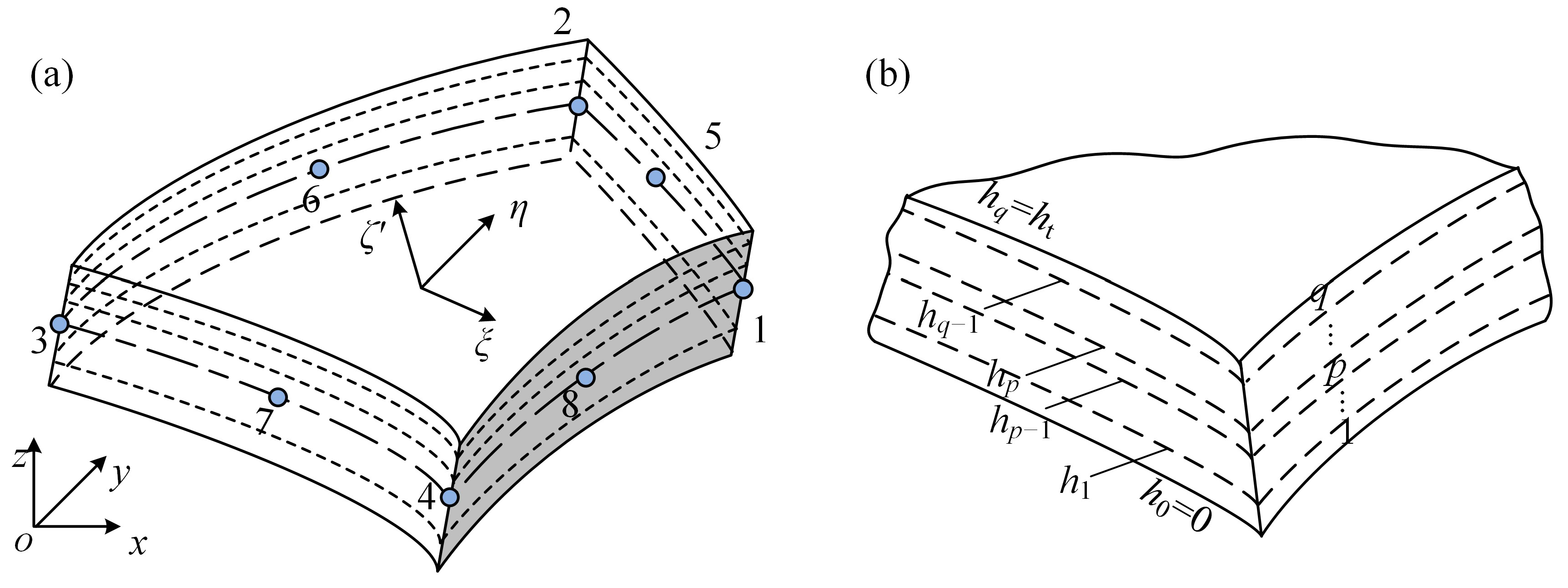
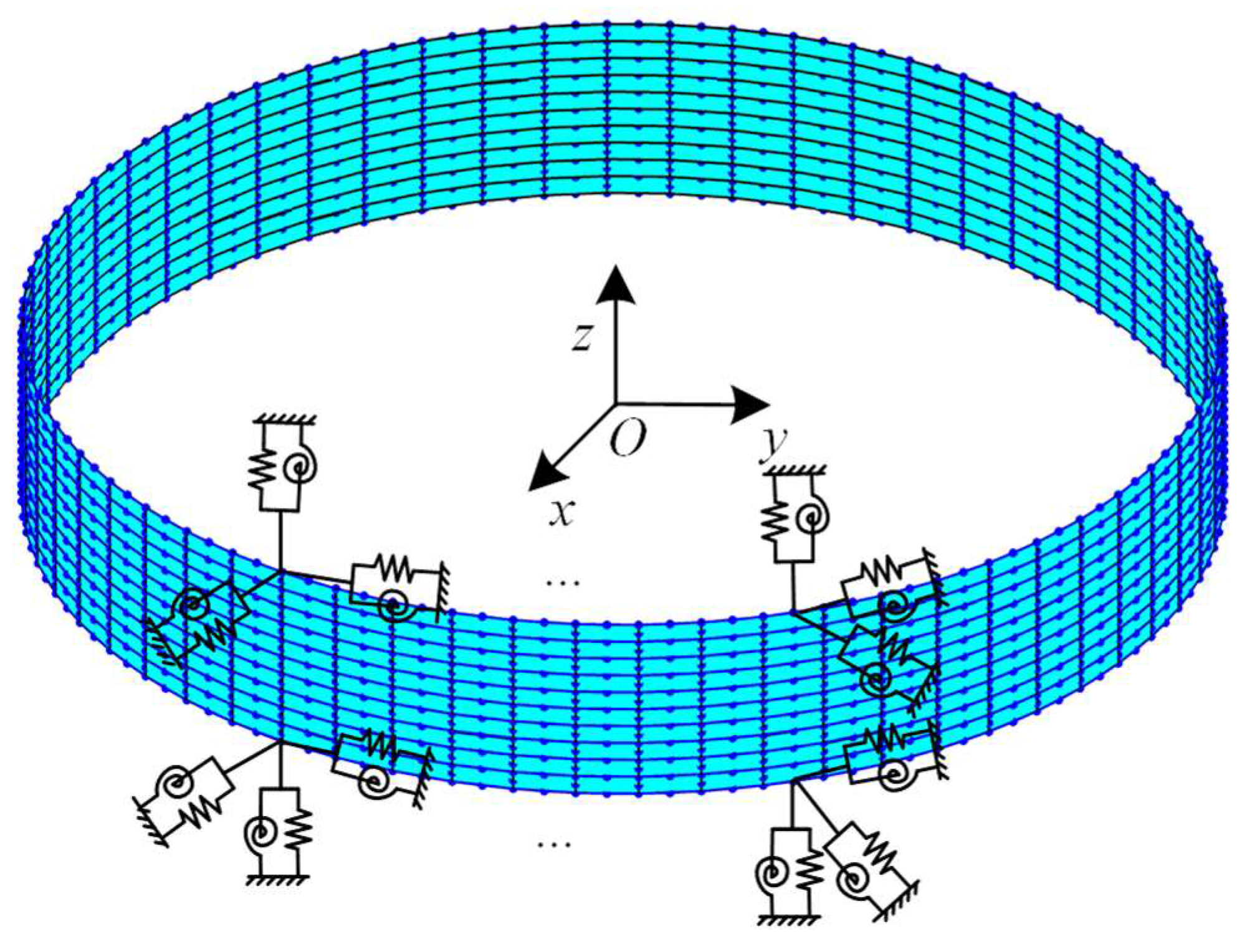
2.2. Dynamic Model for the Coated Casing
2.3. Dynamic Model of the Blade-Coated Casing System
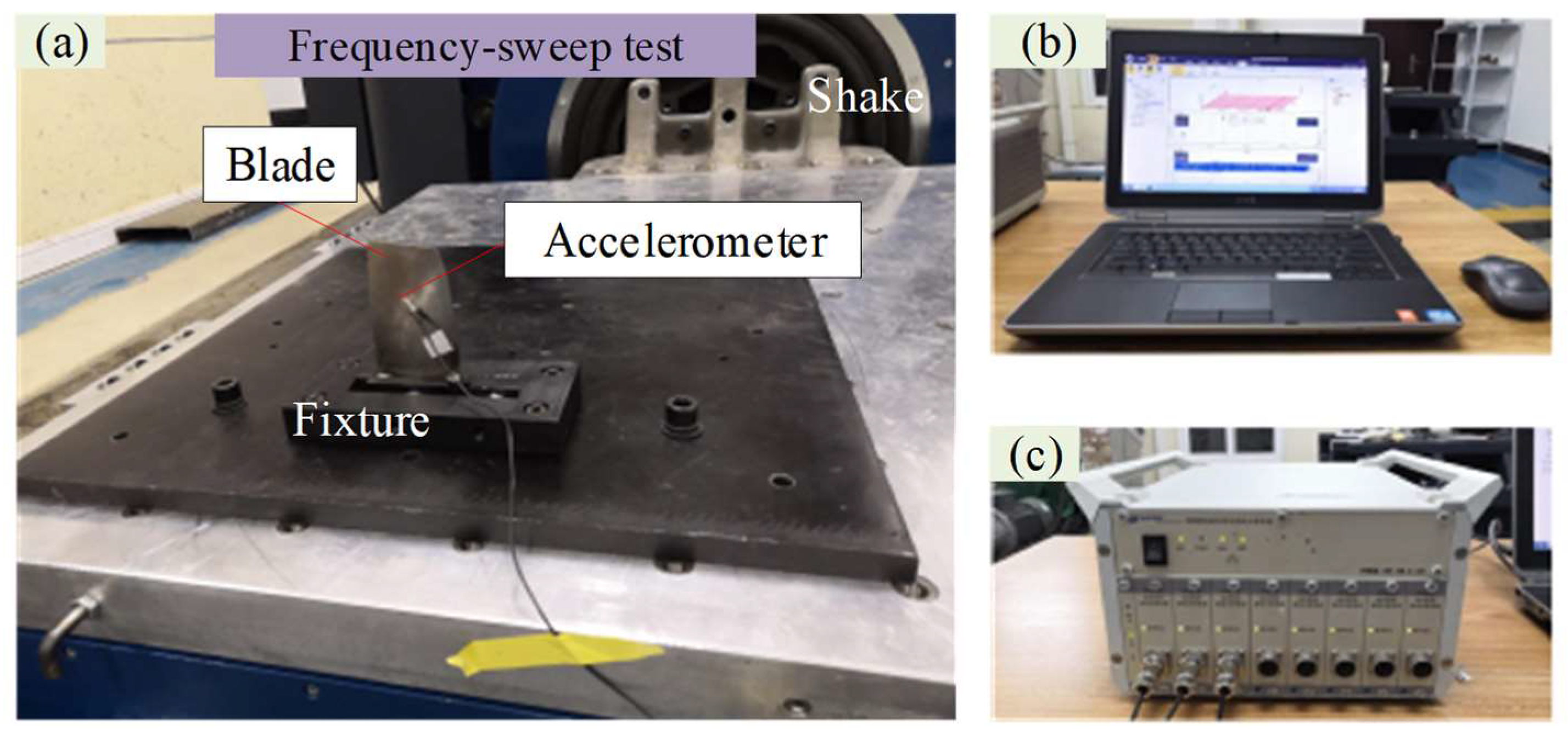
3. Numerical Analysis
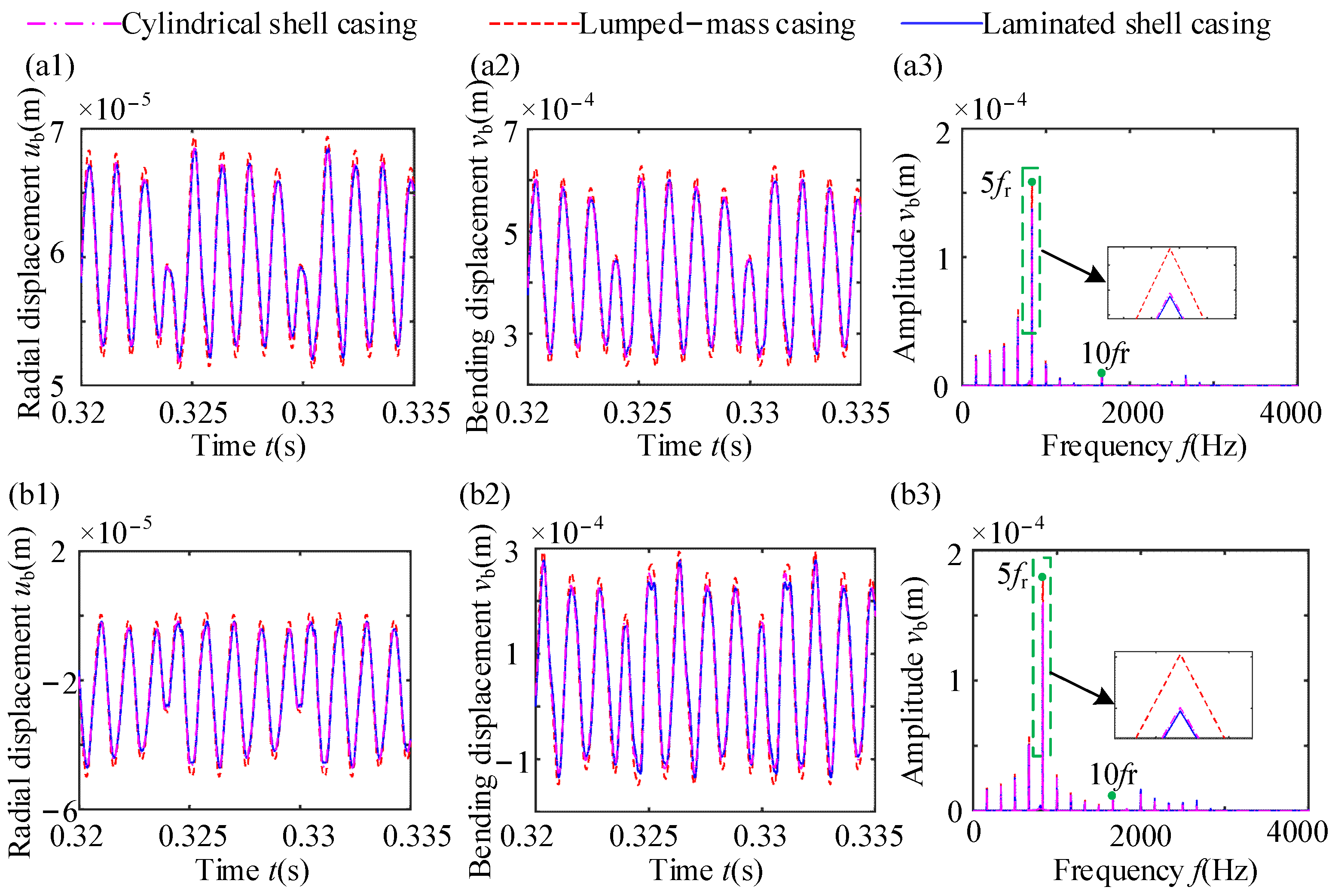
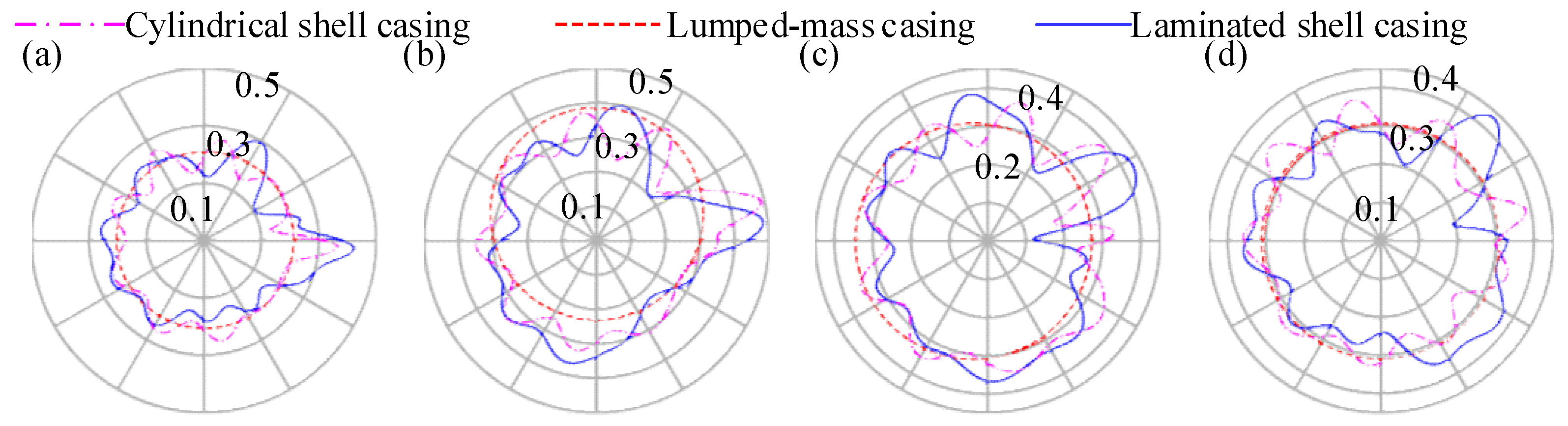
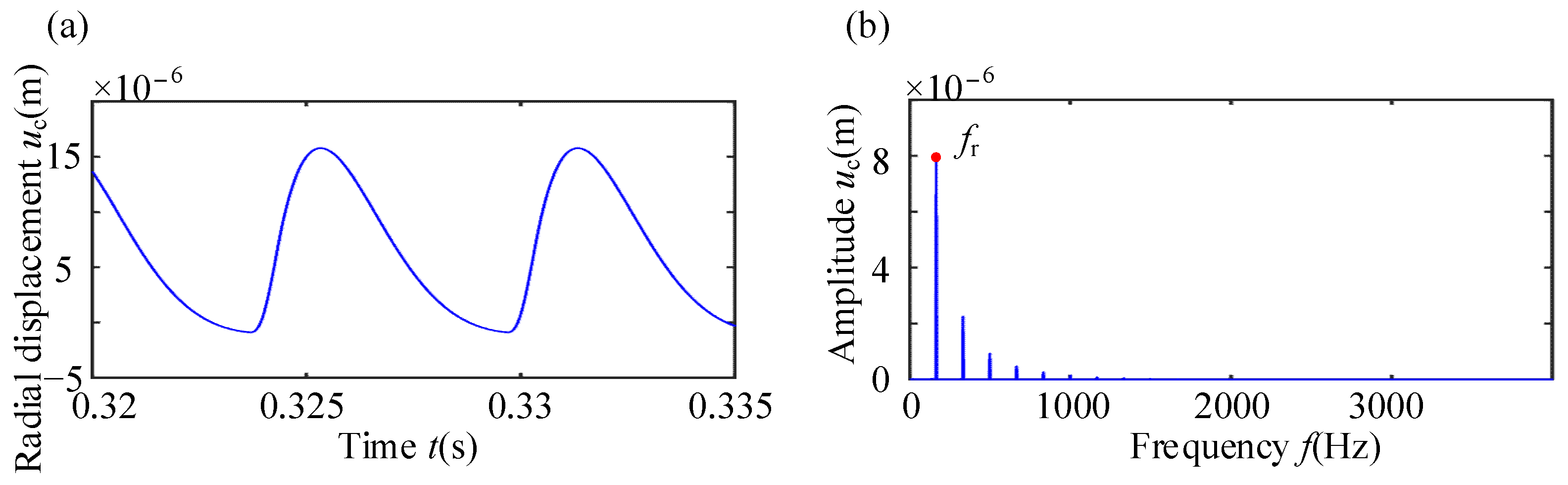
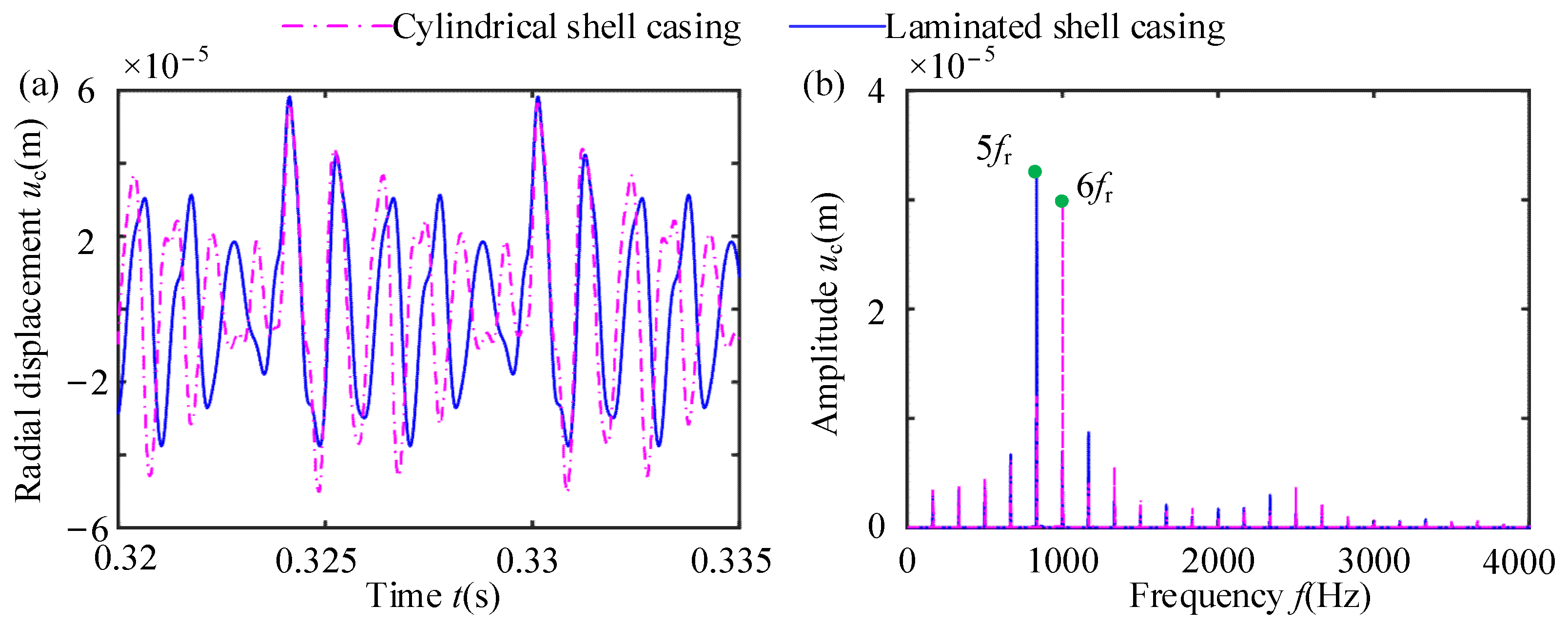
3.1. Influence of the Casing Model
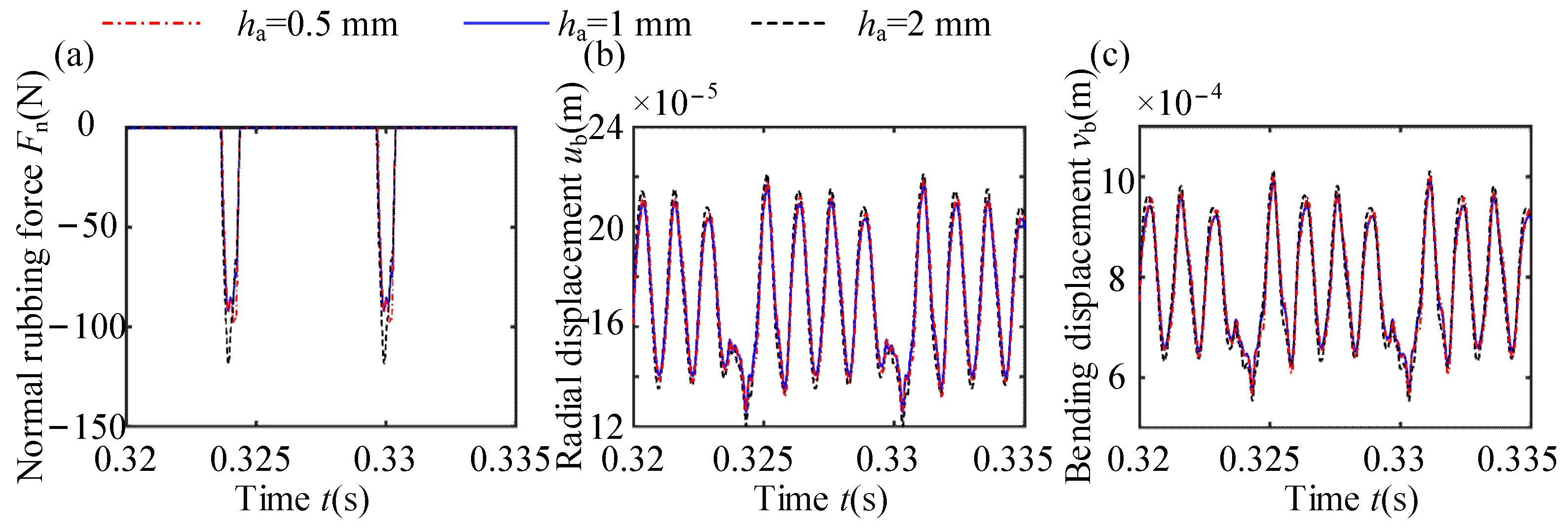
3.2. Influence of Coating Thickness
3.3. Influence of Coating Material
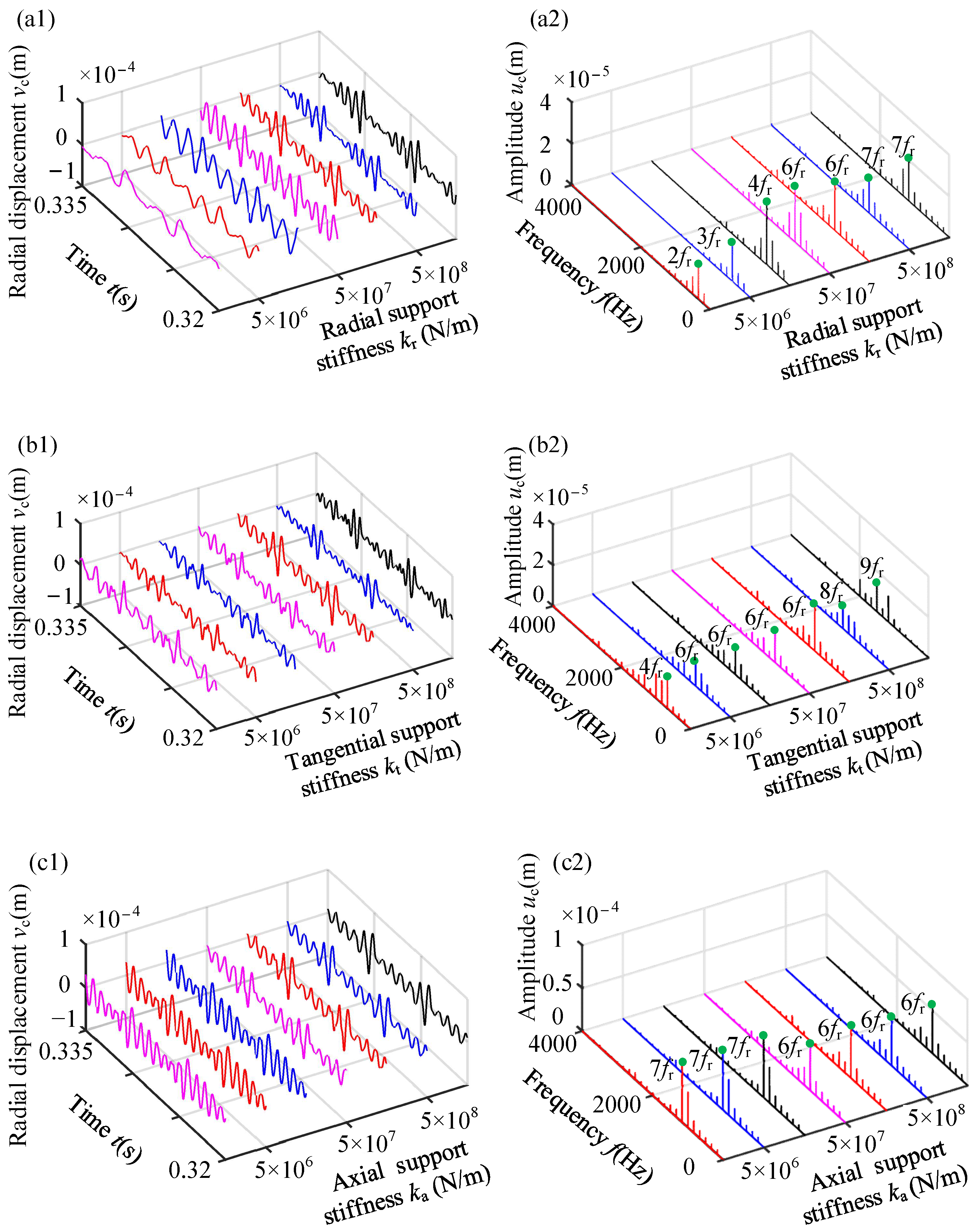
3.4. Influence of the Support Stiffness of the Coated Casing
3.5. Influence of the Length of the Coated Casing
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviation
| Nomenclature | |
| b | Blade width |
| B′p | Strain matrix in the local coordinate system ξηζ’ of the shell |
| ci | Initial clearance between the blades and the casing |
| Dynamic clearance between blade tip node i and casing | |
| Clearance between blade tip node i and casing at the jth and j-1th load steps | |
| ce | Collision recovery coefficient |
| C | Damping matrix of the blade |
| Cc | Damping matrix of the coated casing |
| Damping matrix of the reduced coated casing | |
| Dp | Elastic matrix in the local coordinate system ξηζ’ of the shell |
| ec | Misalignment distance |
| Ea | Elastic modulus of the coating |
| Eb | Elastic modulus of the blade |
| Ec | Elastic modulus of the casing |
| fn1c, fn2c | First and second NFs of the casing |
| Fe1 | Aerodynamic amplitude |
| Normal rubbing force of the ith node at the blade tip | |
| External force vector of the reduced coated casing | |
| Frb, Frc | Rubbing force vector of the blade and casing |
| Minimum clearance between the first node of the blade tip and the casing | |
| gmin | Minimum clearance between the blade tip and the casing at β1 = 0° |
| G | Coriolis force matrix of the blade |
| ht | Total thickness of the laminated shell |
| hp-1, hp | Upper and lower boundaries of the p-th layer |
| ha | Thickness of the coating |
| hc | Thickness of the casing |
| kh | Hertz contact stiffness |
| kh* | Contact stiffness between the blade and casing |
| kb, kc | Structure stiffness of the blade and casing |
| ks | Structural stiffness of the blade in series with the casing |
| kx, ky, kz, kθx, kθy, kθz | Root constraint stiffness of the blade |
| kr, kt, ka, kθr, kθt | Root constraint stiffness of the casing |
| Equivalent rubbing stiffness | |
| Ke, Kce, Kco, Ks | Structure stiffness, centrifugal stiffening, elastic support stiffness, and spin softening matrixes of the blade |
| Ke | Stiffness matrix of the shell element |
| Kc | Stiffness matrix of the coated casing |
| Stiffness matrix of the reduced coated casing | |
| Lc | Length of casing |
| mc | Mass of the casing |
| M | Mass matrix of the blade |
| Me | Mass matrix of the shell element |
| Mc | Mass matrix of the coated casing |
| Mass matrix of the reduced coated casing | |
| q | Total number of floors |
| Displacement, velocity, and acceleration vectors of the reduced casing | |
| Qp | Aerodynamic load |
| Rd | Radius of the disk |
| Rc | Inner diameter of casing |
| Rg | Orbit radius of the blade tip in a stationary state |
| Zi, Xi | Initial coordinate of the blade tip in the x and z directions |
| , | Radial displacement of the i-th node of the casing and the i-th node of the blade tip |
| Displacement, velocity, and acceleration vectors of the casing | |
| Displacement, velocity, and acceleration vectors of the blade | |
| Greek symbols | |
| β1 | Misalignment angle |
| β2 | Stagger angle of the blade tip |
| Relative initial collision velocity and the relative collision velocity at any moment | |
| Local deformation of the coating | |
| Overall penetration deformation between the blade and the casing | |
| ξ1, ξ2 | Modal damping ratio |
| ζp−1, ζp | Corresponding to −1 and 1 in the local coordinate system ξηζ′ |
| ω | Rotating speed of the rotating blade |
| Material density of the p-th layer | |
| ρa | Density of coating |
| ρc | Density of casing |
| v | Poisson’s ratio |
| vb, va | Poisson’s ratio of the blade and coating |
| Abbreviation | |
| FE | Finite element |
| NDVCs | Nodal diameter vibrations of the casings |
| NRF | Normal rubbing force |
| NFs | Natural frequencies |
| TDW | Time-domain waveform |
Appendix A
Appendix B
Appendix C
References
- Wang, N.F.; Liu, C.; Jiang, D.X. Prediction of transient vibration response of dual-rotor-blade-casing system with blade off. Proc. Inst. Mech. Eng. Part G J. Aerosp. Eng. 2019, 8, 351–364. [Google Scholar] [CrossRef]
- Yu, M.Y.; Feng, Z.G.; Huang, J.J.; Zhu, L.L. A new characteristic analysis method for aero-engine rotor–stator rubbing. Proc. Inst. Mech. Eng. Part G J. Aerosp. Eng. 2017, 232, 1120–1133. [Google Scholar] [CrossRef]
- Yang, Y.; Tang, J.; Chen, G.; Yang, Y.R.; Cao, D.Q. Rub-impact investigation of a single-rotor system considering coating effect and coating hardness. J. Vib. Eng. Technol. 2021, 9, 491–505. [Google Scholar] [CrossRef]
- Millecamps, A.; Batailly, A.; Legrand, M.; Garcin, F. Snecma’s viewpoint on the numerical and experimental simulation of blade-tip/casing unilateral contacts. In Proceedings of the ASME Turbo Expo 2015: Turbine Technical Conference and Exposition, Montreal, QC, Canada, 15–19 June 2015. [Google Scholar]
- Ma, H.; Yin, F.L.; Guo, Y.Z.; Tai, X.Y.; Wen, B.C. A review on dynamic characteristics of blade–casing rubbing. Nonlinear Dyn. 2016, 84, 437–472. [Google Scholar] [CrossRef]
- Hamdi, H.; Farah, K. Beam finite element model of a vibrate wind blade in large elastic deformation. Wind. Struct. 2018, 26, 25–34. [Google Scholar] [CrossRef]
- Arumugam, A.B.; Rajamohan, V.; Pandey, A.; Sudhagar, P. Finite element vibration analysis of rotating laminated composite beam with varying cross-section using HSDT. Int. J. Interact. Des. Manuf. 2017, 11, 703–712. [Google Scholar] [CrossRef]
- Stoykov, S.; Ribeiro, P. Vibration analysis of rotating 3D beams by the p-version finite element method. Finite Elem. Anal. Des. 2013, 65, 76–88. [Google Scholar] [CrossRef]
- Gunda, J.B.; Ganguli, R. New rational interpolation functions for finite element analysis of rotating beams. Int. J. Mech. Sci. 2007, 50, 578–588. [Google Scholar] [CrossRef]
- Carassale, L.; Maurici, M.; Traversone, L. Reduced-Order Modeling of Compressor Blades by 1D Finite Elements. In Proceedings of the ASME Turbo Expo: Turbine Technical Conference and Exposition, Montréal, QC, Canada, 15–19 June 2015. [Google Scholar] [CrossRef]
- Lee, S.L. Equivalent beam modeling scheme based on three-dimensional structure with blade element concept. Adv. Compos. Mater. 2020, 29, 191–203. [Google Scholar] [CrossRef]
- Sakar, G.; Sabuncu, M. Dynamic stability of a rotating asymmetric cross-section blade subjected to an axial periodic force. Int. J. Mech. Sci. 2003, 45, 1467–1482. [Google Scholar] [CrossRef]
- Guan, H.; Ni, K.X.; Ma, H.; Xiong, Q.; Wang, W.W.; Wang, H.J. Dynamic modeling and verification of rotating compressor blade with crack based on beam element. Appl. Math. Model. 2024, 133, 367–393. [Google Scholar] [CrossRef]
- Sabuncu, M.; Evran, K. The dynamic stability of a rotating pre-twisted asymmetric cross-section blade subjected to lateral parametric excitation. Finite Elem. Anal. Des. 2006, 42, 1113–1122. [Google Scholar] [CrossRef]
- Sabuncu, M.; Evran, K. Dynamic stability of a rotating pre-twisted asymmetric cross-section Timoshenko beam subjected to an axial periodic force. Int. J. Mech. Sci. 2006, 48, 579–590. [Google Scholar] [CrossRef]
- Oh, Y.; Yoo, H.H. Vibration analysis of a rotating pre-twisted blade considering the coupling effects of stretching, bending, and torsion. J. Sound Vib. 2018, 431, 20–39. [Google Scholar] [CrossRef]
- Kou, H.J.; Du, J.J.; Liang, M.X.; Zhu, L.; Zeng, L.; Zhu, Z.D.; Zhang, F. Nonlinear characteristics of contact-induced vibrations of the rotating variable thickness plate under large deformations. Eur. J. Mech./A Solids 2019, 77, 103801. [Google Scholar] [CrossRef]
- Yao, M.H.; Ma, L.; Zhang, W. Nonlinear dynamics of the high-speed rotating plate. Int. J. Aerosp. Eng. 2018, 2018, 5610915. [Google Scholar] [CrossRef]
- Sun, J.; Kari, L.; Arteaga, I.L. A dynamic rotating blade model at an arbitrary stagger angle based on classical plate theory and the Hamilton’s principle. J. Sound Vib. 2013, 332, 1355–1371. [Google Scholar] [CrossRef]
- Rostami, H.; Ranji, A.R.; Bakhtiari-Nejad, F. Vibration characteristics of rotating orthotropic cantilever plates using analytical approaches: A comprehensive parametric study. Arch. Appl. Mech. 2018, 88, 481–502. [Google Scholar] [CrossRef]
- Luo, Z.Z.; Zhu, Y.P.; Liu, H.P.; Wang, D.Y. Dynamic similitude design method of the distorted model on variable thickness cantilever plates. Appl. Sci. 2016, 6, 228. [Google Scholar] [CrossRef]
- Chen, Y.Z.; Zhang, D.G.; Li, L. Dynamics analysis of a rotating plate with a setting angle by using the absolute nodal coordinate formulation. Eur. J. Mech./A Solids 2019, 74, 257–271. [Google Scholar] [CrossRef]
- Sinha, S.K.; Zylka, R.P. Vibration analysis of composite airfoil blade using orthotropic thin shell bending theory. Int. J. Mech. Sci. 2017, 121, 90–105. [Google Scholar] [CrossRef]
- Sun, J.; Arteaga, I.L.; Kari, L. General shell model for a rotating pretwisted blade. J. Sound Vib. 2013, 332, 5804–5820. [Google Scholar] [CrossRef]
- Júnior, C.J.F.; Cardozo, A.C.P.; Júnior, V.M.; Neto, A.G. Modeling wind turbine blades by geometrically-exact beam and shell elements: A comparative approach. Eng. Struct. 2019, 180, 357–378. [Google Scholar] [CrossRef]
- Kee, Y.J.; Shin, S.J. Structural dynamic modeling for rotating blades using three dimensional finite elements. J. Mech. Sci. Technol. 2015, 29, 1607–1618. [Google Scholar] [CrossRef]
- Yangui, M.; Bouaziz, S.; Taktak, M.; Haddar, M.; El-Sabbagh, A. Nonlinear analysis of twisted wind turbine blade. J. Mech. 2016, 34, 269–278. [Google Scholar] [CrossRef]
- Sun, Q.; Ma, H.; Zhu, Y.P.; Han, Q.K.; Wen, B.C. Comparison of rubbing induced vibration responses using varying-thicknessp-twisted shell and solid-element blade models. Mech. Syst. Signal Process. 2018, 108, 1–20. [Google Scholar] [CrossRef]
- Rafiee, R.; Tahani, M.; Moradi, M. Simulation of aeroelastic behavior in a composite wind turbine blade. J. Wind. Eng. Ind. Aerodyn. 2016, 151, 60–69. [Google Scholar] [CrossRef]
- Kee, Y.J.; Kim, J.H. Vibration characteristics of initially twisted rotating shell type composite blades. Compos. Struct. 2003, 64, 151–159. [Google Scholar] [CrossRef]
- Xiong, Q.; Guan, H.; Ma, H.; Wu, Z.Y.; Guo, X.M.; Wang, W.W. Dynamic characteristic analysis of rotating blade with breathing crack. Mech. Syst. Signal Process. 2023, 196, 110325. [Google Scholar] [CrossRef]
- Zhao, Q.; Liu, Z.L.; He, Y.H.; Yao, H.L.; Wen, B.C. Dynamic stress prediction method for rubbing blades. J. Vibroengineering 2016, 18, 1–12. Available online: https://www.extrica.com/article/16087 (accessed on 7 April 2024).
- Guan, H.; Xiong, Q.; Ma, H.; Wang, W.W.; Ni, K.X.; Wu, Z.Y.; Yin, X.M.; Zhao, S.T.; Zhang, X.X. Comparison of nonlinear vibration responses induced by edge crack and surface crack of compressor blades. Mech. Syst. Signal Process. 2024, 216, 111465. [Google Scholar] [CrossRef]
- Wang, W.W.; Ma, H.; Zhao, C.G.; Wu, Z.Y.; Wang, H.J. Dynamic contact characteristics of a rotating twisted variable-section blade with breathing crack. J. Cent. South Univ. 2024, 31, 858–877. [Google Scholar] [CrossRef]
- Ma, H.; Wang, D.; Tai, X.Y.; Wen, B.C. Vibration response analysis of blade-disk dovetail structure under blade tip rubbing condition. J. Vib. Control 2017, 23, 252–271. [Google Scholar] [CrossRef]
- Jones, S.; Legrand, M. Forced vibrations of a turbine blade undergoing regularized unilateral contact conditions through the wavelet balance method. Int. J. Numer. Methods Eng. 2015, 101, 351–374. [Google Scholar] [CrossRef]
- Sinha, S.K. Combined torsional-bending-axial dynamics of a twisted rotating cantilever Timoshenko beam with contact-impact loads at the free end. J. Appl. Mech. 2007, 74, 505–522. [Google Scholar] [CrossRef]
- Ma, X.X.; Ma, H.; Zeng, J.; Piao, Y.H. Rubbing-induced vibration response analysis of dual-rotor-casing system. Trans. Nanjing Univ. Aeronaut. Astronaut. 2018, 35, 101–108. [Google Scholar] [CrossRef]
- Zeng, J.; Ma, H.; Yu, K.; Guo, X.M.; Wen, B.C. Rubbing response comparisons between single blade and flexible ring using different rubbing force models. Int. J. Mech. Sci. 2019, 164, 105164. [Google Scholar] [CrossRef]
- Lesaffre, N.; Sinou, J.J.; Thouverez, F. Stability analysis of rotating beams rubbing on an elastic circular structure. J. Sound Vib. 2006, 299, 1005–1032. [Google Scholar] [CrossRef]
- Guo, X.M.; Zeng, J.; Ma, H.; Zhao, C.G.; Yu, X. A dynamic model for simulating rubbing between blade and flexible casing. J. Sound Vib. 2020, 466, 115036. [Google Scholar] [CrossRef]
- Zeng, J.; Ma, H.; Ma, X.X.; Wu, Z.Y.; Qin, Z.Y. Blade-loss-caused rubbing dynamic characteristics of rotor-bladed disk-casing system. Trans. Nanjing Univ. Aeronaut. Astronaut. 2018, 35, 116–125. [Google Scholar] [CrossRef]
- Batailly, A.; Legrand, M. Unilateral contact induced blade/casing vibratory interactions in impellers: Analysis for flexible casings with friction and abradable coating. J. Sound Vib. 2015, 348, 344–364. [Google Scholar] [CrossRef]
- Zhong, R.; Tang, J.Y.; Wang, A.L.; Shuai, C.J.; Wang, Q.S. An exact solution for free vibration of cross-ply laminated composite cylindrical shells with elastic restraint ends. Comput. Math. Appl. 2019, 77, 641–661. [Google Scholar] [CrossRef]
- Sun, W.W.; Zhu, M.W.; Wang, Z. Free vibration analysis of a hard-coating cantilever cylindrical shell with elastic constraints. Aerosp. Sci. Technol. 2017, 63, 232–244. [Google Scholar] [CrossRef]
- Li, C.F.; Zhong, B.F.; Shen, Z.C.; Zhang, J.R. Modeling and nonlinear vibration characteristics analysis of symmetrically 3-layer composite thin circular cylindrical shells with arbitrary boundary conditions. Thin-Walled Struct. 2019, 142, 311–321. [Google Scholar] [CrossRef]
- Dey, T.; Ramachandra, L.S. Non-linear vibration analysis of laminated composite circular cylindrical shells. Compos. Struct. 2017, 163, 89–100. [Google Scholar] [CrossRef]
- Song, X.Y.; Han, Q.K.; Zhai, J.Y. Vibration analyses of symmetrically laminated composite cylindrical shells with arbitrary boundaries conditions via Rayleigh-Ritz method. Compos. Struct. 2015, 134, 820–830. [Google Scholar] [CrossRef]
- Zhang, Y.; Sun, W.; Yang, J.; Han, Q.K. Nonlinear vibration analysis of a hard-coating cylindrical shell with elastic constraints by finite element method. Nonlinear Dyn. 2017, 90, 2879–2891. [Google Scholar] [CrossRef]
- Zhang, Y.; Sun, W.; Yang, J. A new finite element formulation for nonlinear vibration analysis of the hard-coating cylindrical shell. Coatings 2017, 7, 70. [Google Scholar] [CrossRef]
- Khan, K.; Patel, B.P.; Nath, Y. Free and forced vibration characteristics of bimodular composite laminated circular cylindrical shells. Compos. Struct. 2015, 126, 386–397. [Google Scholar] [CrossRef]
- Ma, H.; Tai, X.Y.; Han, Q.K.; Wu, Z.Y.; Wang, D.; Wen, B.C. A revised model for rubbing between rotating blade and elastic casing. J. Sound Vib. 2015, 337, 301–320. [Google Scholar] [CrossRef]
- Ma, H.; Yin, F.L.; Tai, X.Y.; Wang, D.; Wen, B.C. Vibration response analysis caused by rubbing between rotating blade and casing. J. Mech. Sci. Technol. 2016, 30, 1983–1995. [Google Scholar] [CrossRef]
- Zhou, T.; Jia, Y.F.; Zou, L.M.; Jiang, Z.N.; Wang, W.M.; Hu, M.H. Vibration characteristics of blade-casing rubbing fault considering rotor–stator coupling. Mech. Syst. Signal Process. 2024, 218, 111589. [Google Scholar] [CrossRef]
- Hou, Y.H.; Cao, S.Q.; Kang, Y.H. Study on the frequency modulation phenomenon in the rotor system with blade-casing rub-impact fault. Int. J. Non-Linear Mech. 2024, 159, 104626. [Google Scholar] [CrossRef]
- Wu, Z.Y.; Zhao, L.C.; Yan, H.; Yan, G.; Chen, A.; Zhang, W.M. Multi-blade rubbing characteristics of the shaft-disk-blade-casing system with large rotation. Appl. Math. Mech. 2024, 45, 111–136. [Google Scholar] [CrossRef]
- Shao, J.; Wu, J.G.; Yang, K.; Zhang, Y. Dynamic characteristic analysis of a twin-spool rotor–casing system with looseness and intershaft rubbing coupling faults. J. Mech. Sci. Technol. 2024, 38, 101–120. [Google Scholar] [CrossRef]
- Li, B.Q.; Ma, H.; Zeng, J.; Guo, X.M.; Wen, B.C. Rotating blade-casing rubbing simulation considering casing flexibility. Int. J. Mech. Sci. 2018, 148, 118–134. [Google Scholar] [CrossRef]
- Yang, Y.; Cao, D.Q.; Wang, D.Y. Investigation of dynamic characteristics of a rotor system with surface coatings. Mech. Syst. Signal Process. 2017, 84, 469–484. [Google Scholar] [CrossRef]
- Yang, Y.; Ouyang, H.J.; Wu, X.L.; Jin, Y.L.; Yang, Y.R.; Cao, D.Q. Bending-torsional coupled vibration of a rotor-bearing-system due to blade-casing rub in presence of non-uniform initial gap. Mech. Mach. Theory 2019, 140, 170–193. [Google Scholar] [CrossRef]
- Cao, D.Q.; Yang, Y.; Chen, H.T.; Wang, D.Y.; Jiang, G.Y.; Li, C.G.; Wei, J.; Zhao, K. A novel contact force model for the impact analysis of structures with coating and its experimental verification. Mech. Syst. Signal Process. 2016, 70, 1056–1072. [Google Scholar] [CrossRef]
- Zeng, J.; Zhao, C.G.; Ma, H.; Yu, K.; Wen, B.C. Rubbing dynamic characteristics of the blisk-casing system with elastic supports. Aerosp. Sci. Technol. 2019, 95, 105481. [Google Scholar] [CrossRef]
- Kang, Y.H.; Cao, S.Q.; Gao, T.; You, Z.Z. Development and validation of a rotating blade-casing rubbing model by considering the blade deformation and abradable coating. J. Sound Vib. 2023, 563, 117853. [Google Scholar] [CrossRef]
- Kang, Y.H.; Cao, S.Q.; Hou, Y.H.; Chen, N.; Li, B. Dynamics research on the rubbing process and rubbing forms of rotor–blade–casing systems. Int. J. Non-Linear Mech. 2022, 147, 104242. [Google Scholar] [CrossRef]
- Jin, M.; Wang, A.L.; Wang, Q.S.; Wang, L.K. Rub-impact dynamic analysis of the central tie rod rotor-blade-casing coupling system with the Hirth couplings connection. J. Vib. Eng. Technol. 2024, 12, 1479–1503. [Google Scholar] [CrossRef]
- Tang, T.; Wang, Y.; Chen, Z.Y.; Wang, S.; Zhang, M.Q. Study on rubbing characteristics of blade-casing model considering transverse cracks. J. Sound Vib. 2023, 567, 117928. [Google Scholar] [CrossRef]
- Wang, N.F.; Liu, C.; Jiang, D.X.; Kamran, B. Casing vibration response prediction of dual-rotor-blade-casing system with blade-casing rubbing. Mech. Syst. Signal Process. 2019, 118, 61–77. [Google Scholar] [CrossRef]
- Wang, N.F.; Liu, C.; Jiang, D.X. Experimental analysis of dual-rotor-support-casing system with blade-casing rubbing. Eng. Fail. Anal. 2021, 123, 105306. [Google Scholar] [CrossRef]
- Zhang, J.H.; Lu, X.; Lin, J.W.; Ma, L.; Dai, H.W. Dynamic characteristics analysis of blade-casing rubbing faults with abradable coatings. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2021, 235, 975–987. [Google Scholar] [CrossRef]
- Jin, Y.L.; Liu, Z.W.; Yang, Y.; Li, F.S.; Chen, Y.S. Nonlinear vibrations of a dual-rotor-bearing-coupling misalignment system with blade-casing rubbing. J. Sound Vib. 2021, 497, 115948. [Google Scholar] [CrossRef]
- Salvat, N.; Batailly, A.; Legrand, M. Modeling of abradable coating removal in aircraft engines through delay differential equations. J. Eng. Gas Turbines Power 2013, 135, 102102. [Google Scholar] [CrossRef]
- Kammer, A.S.; Olgac, N. Blade/Casing rub interaction in turbomachinery: Structural parameters’ influence on stability. J. Propuls. Power 2016, 32, 928–938. [Google Scholar] [CrossRef]
- Berthoul, B.; Batailly, A.; Stainier, L.; Legrand, M.; Cartraud, P. Phenomenological modeling of abradable wear in turbomachines. Mech. Syst. Signal Process. 2018, 98, 770–785. [Google Scholar] [CrossRef]
- Legrand, M.; Batailly, A.; Pierre, C. Numerical investigation of abradable coating removal in aircraft engines through plastic constitutive law. J. Comput. Nonlinear Dyn. 2012, 7, 011010. [Google Scholar] [CrossRef]
- Batailly, A.; Legrand, M.; Millecamps, A.; Garcin, F. Numerical-experimental comparison in the simulation of rotor/stator interaction through blade-tip/abradable coating contact. J. Eng. Gas Turbines Power 2012, 134, 082504. [Google Scholar] [CrossRef]
- Batailly, A.; Cuny, M.; Legrand, M.; Philippon, S. Numerical-experimental confrontation in the simulation of tool/abradable material interaction. J. Eng. Gas Turbines Power 2013, 135, 062102. [Google Scholar] [CrossRef]
- Almeida, P.; Gibert, C.; Thouverez, F.; Leblanc, X.; Ousty, L.P. Numerical analysis of bladed disk–casing contact with friction and wear. J. Eng. Gas Turbines Power 2016, 138, 122802. [Google Scholar] [CrossRef]
- Almeida, P.; Gibert, C.; Thouverez, F. On Some Physical Phenomena Involved in Blade-Casing Contact. In Proceedings of the Eurodyn-International Conference on Structural Dynamics, Porto, Portugal, 30 June—2 July 2014; Available online: https://hal.science/hal-02121521/ (accessed on 7 April 2024).
- Ma, H.; Guan, H.; Qu, L.; Yang, T.R.; Zeng, Y.; Chen, Y.Y.; Zhu, Z.M.; Wang, H.J. Blade-coating-casing rubbing induced vibration responses and wear characteristics. Tribol. Int. 2024, 194, 109571. [Google Scholar] [CrossRef]
- Guan, H.; Ma, H.; Qu, X.C.; Wu, Z.; Zeng, J.; Xiong, Q.; Wang, H. Dynamic stress analysis of cracked rectangular blade: Simulation and experiment. Int. J. Mech. Sci. 2024, 267, 109015. [Google Scholar] [CrossRef]
- Zhao, T.Y.; Li, K.; Ma, H. Study on dynamic characteristics of a rotating cylindrical shell with uncertain parameters. Anal. Math. Phys. 2022, 12, 97. [Google Scholar] [CrossRef]


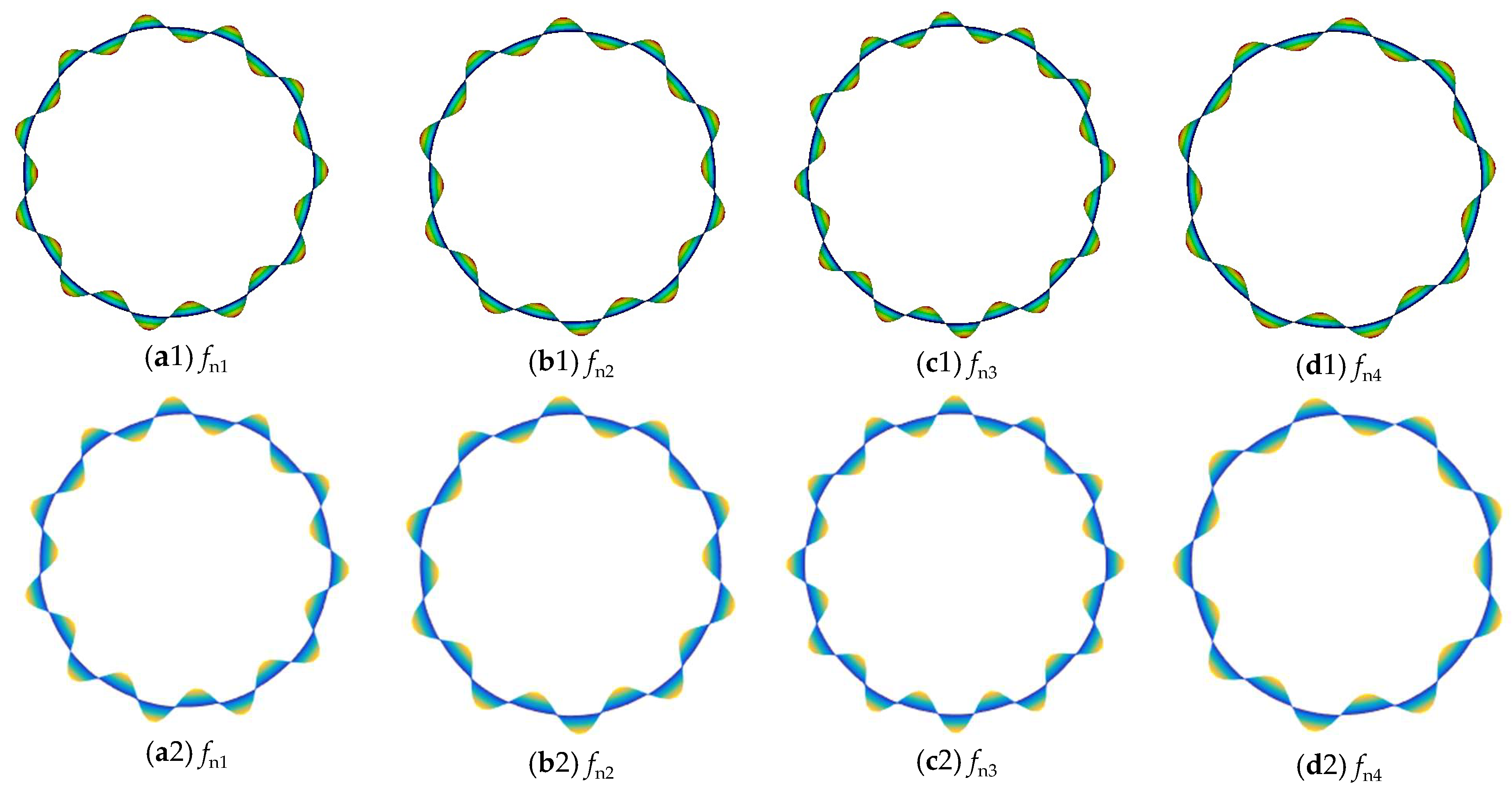




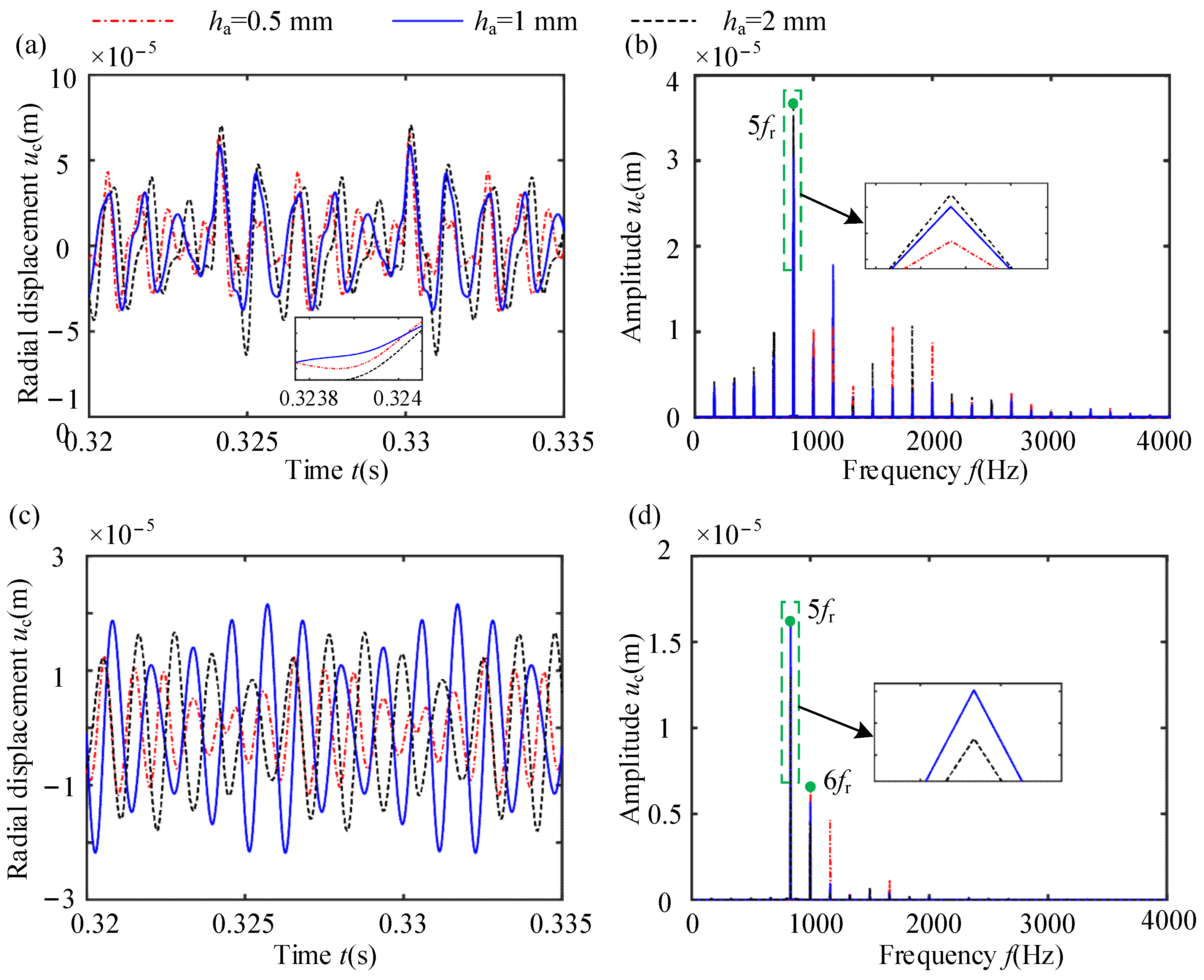


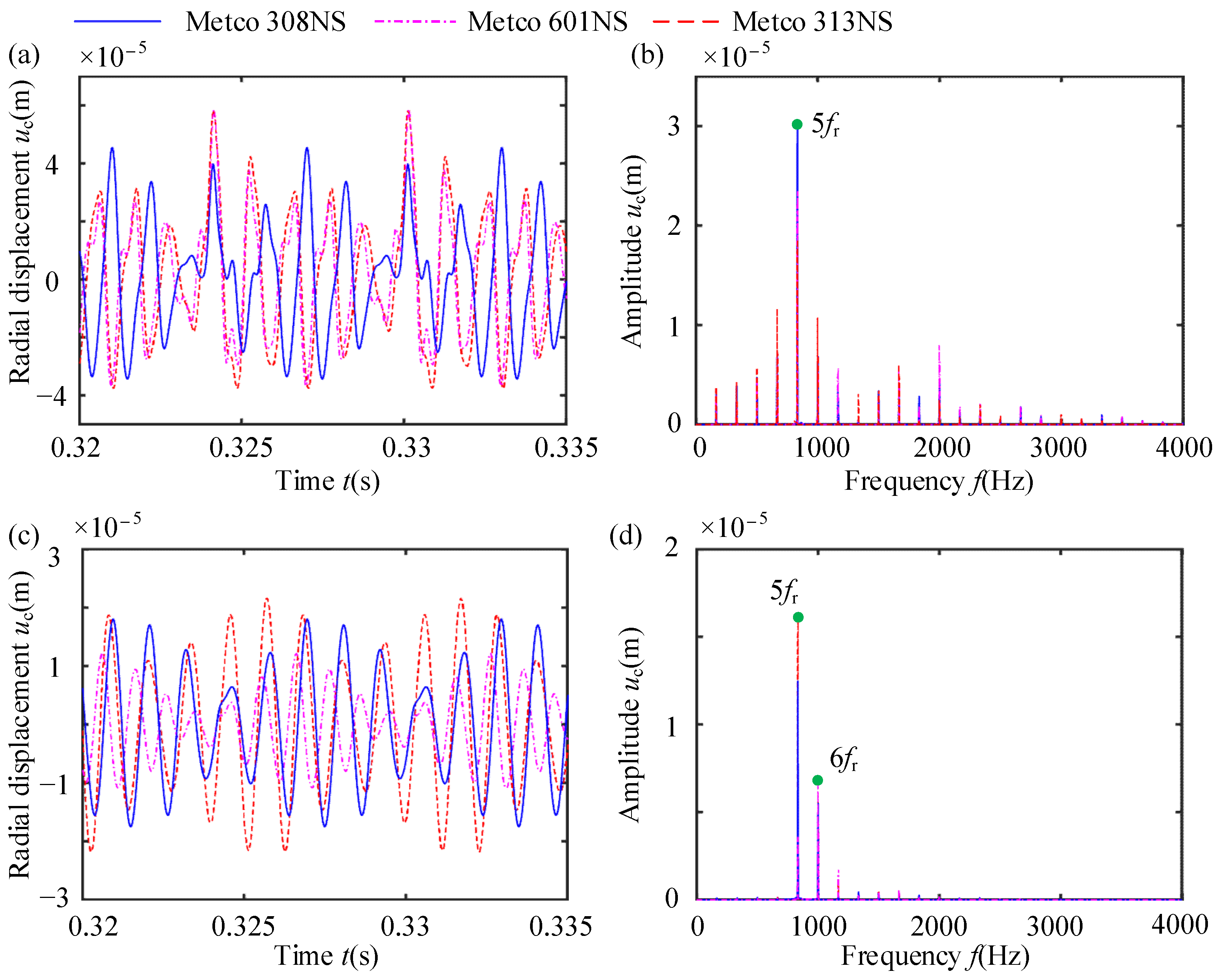
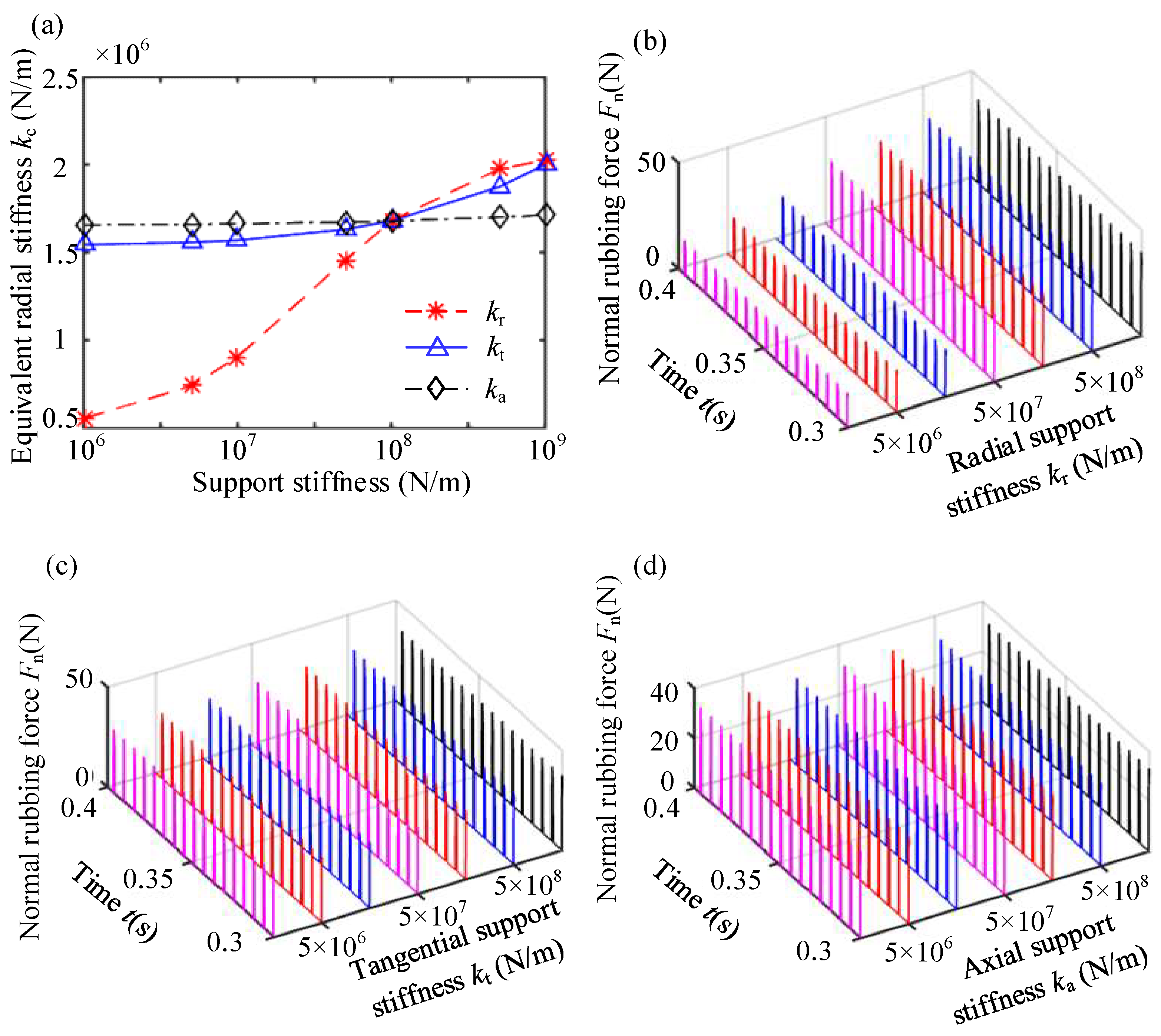
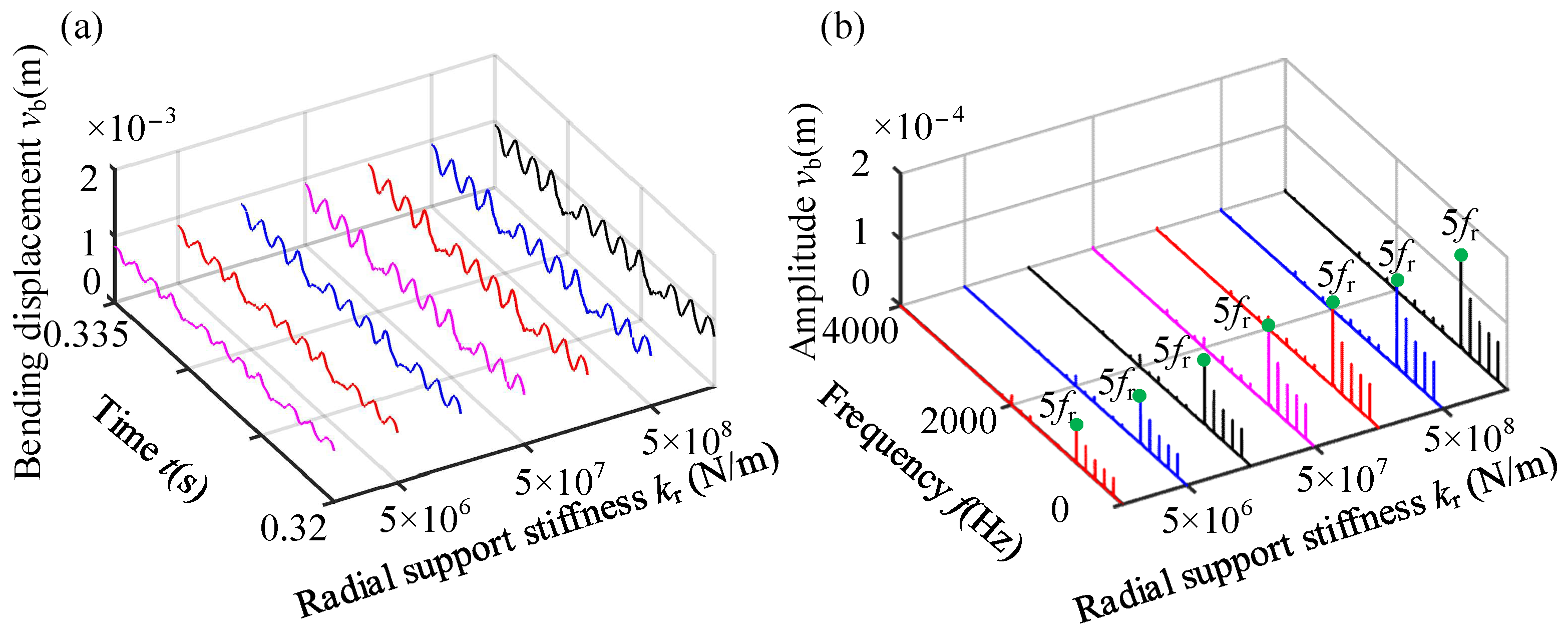

| Orders | Experiment [31] | ANSYS Model | Proposed Model | Errors between the Experiment and Proposed Model (%) | Errors between ANSYS Model and Proposed Model (%) |
|---|---|---|---|---|---|
| fn1b (Hz) | 657.5 | 680.1 | 679.0 | 3.27 | −0.15 |
| fn2b (Hz) | 2008.0 | 1993.8 | 1976.1 | −1.60 | −0.89 |
| fn3b (Hz) | 2409.0 | 2531.4 | 2500.0 | 3.77 | −1.22 |
| Parameters | Value | Parameters | Value | Parameters | Value |
|---|---|---|---|---|---|
| Thickness of coating ha (mm) | 1 | Thickness of casing hc (mm) | 2 | Length of casing Lc (m) | 0.1 |
| Density of coating ρa (kg/m3) | 2000 | Density of casing ρc (kg/m3) | 4370 | Inner diameter of casing Rc (m) | 0.30682 |
| Elastic modulus of coating Ea (GPa) | 3.11 | Elastic modulus of casing Ec (GPa) | 125 | Poisson ratio v | 0.3 |
| Orders | fn1c (Hz) | fn2c (Hz) | fn3c (Hz) | fn4c (Hz) |
|---|---|---|---|---|
| Proposed model | 1929.1 | 1930.3 | 1950.7 | 1954.9 |
| ANSYS model | 1929.8 | 1930.8 | 1951.4 | 1955.4 |
| Errors (%) | −0.036 | −0.026 | −0.036 | −0.026 |
| Orders | fn1c (Hz) | fn2c (Hz) | fn3c (Hz) | fn4c (Hz) | fn5c (Hz) | fn6c (Hz) |
|---|---|---|---|---|---|---|
| Proposed model | 823.54 | 826.63 | 827.93 | 839.19 | 842.85 | 858.30 |
| ANSYS model | 823.41 | 826.47 | 827.83 | 839.12 | 842.65 | 858.29 |
| Errors (%) | 0.0157 | 0.0193 | 0.0120 | 0.0080 | 0.0230 | 0.0012 |
| Orders | fn1c (Hz) | fn2c (Hz) | fn3c (Hz) | fn4c (Hz) | fn5c (Hz) |
|---|---|---|---|---|---|
| Unreduced model | 823.54 | 826.63 | 827.93 | 839.19 | 842.85 |
| Reduced model | 824.23 | 827.32 | 830.21 | 840.39 | 845.44 |
| Errors (%) | 0.084 | 0.084 | 0.275 | 0.140 | 0.307 |
| Parameters | Value | Parameters | Value |
|---|---|---|---|
| Frictional coefficient μ | 0.3 | Rotating speed ω (r/min) | 10,000 |
| Static parallel misalignment ec (mm) | 1.6 | Minimum clearance gmin (mm) | 0.1 |
| Misalignment angle β1 (°) | 0.15 | Blade tip staggered angle β2 (°) | 54.68 |
| Radius of the blade tip Rd (mm) | 216.52 | Inner diameter of casing Rc (mm) | 306.82 |
| Models | Parameters |
|---|---|
| Lumped-blade mass casing model | mc = 1.69 kg, kc = 1.34 × 106 N/m |
| Cylindrical-blade shell casing model | Lc=0.1 m, hc = 2 mm, Ec = 125 GPa, ρc = 4370 kg/m3 |
| Laminated-blade casing model | Lc = 0.1 m, hc = 2 mm, ha = 1 mm, Ea = 3.11 Gpa, Ec = 125 GPa, ρa = 2000 kg/m3, ρc = 4370 kg/m3 |
| Materials | Ingredient | Density ρa (kg/m3) | Elasticity Modulus Ea (GPa) | Natural Frequency f (Hz) | Equivalent Radial stiffness (N/m) |
|---|---|---|---|---|---|
| Metco 308NS | Ni-graphite | 4900 | 3.95 | 841.32 | 1.376 × 106 |
| Metco 601NS | Al-Si polymer | 1500 | 2.08 | 731.76 | 1.412 × 106 |
| Metco 313NS | Al-Si-graphite | 2000 | 3.11 | 823.55 | 1.396 × 106 |
| Varying Parameters | Ranges | Constant Parameters |
|---|---|---|
| Radial support stiffness kr | [1 × 106, 1 × 109] N/m | kt = 1 × 108 N/m, ka = 1 × 108 N/m, Lc = 0.1 m, Ec = 125 GPa, ρc = 4370 kg/m3, hc = 2 mm, Ea = 3.11 GPa, ρa = 2000 kg/m3, ha = 1 mm |
| Tangential support stiffness kt | [1 × 106, 1 × 109] N/m | kr =1 × 108 N/m, ka = 1 × 108 N/m, Lc = 0.1 m, Ec = 125 GPa, ρc = 4370 kg/m3, hc = 2 mm, Ea = 3.11 GPa, ρa = 2000 kg/m3, ha = 1 mm, |
| Axial support stiffness ka | [1 × 106, 1 × 109] N/m | kr =1 × 108 N/m, kt = 1 × 108 N/m, Lc = 0.1 m, Ec = 125 GPa, ρc = 4370 kg/m3, hc = 2 mm, Ea = 3.11 GPa, ρa = 2000 kg/m3, ha = 1 mm, |
| Casing Length Lc(m) | 0.06 | 0.08 | 0.1 | 0.12 | 0.14 | 0.16 | 0.18 | 0.2 |
| Equivalent radial stiffness kc (106 N/m) | 1.543 | 1.558 | 1.396 | 1.308 | 1.270 | 1.248 | 1.228 | 1.208 |
| 1st natural frequency fn1c (Hz) | 1146.20 | 939.93 | 860.64 | 844.81 | 684.28 | 633.77 | 591.70 | 555.67 |
| Maximum normal rubbing force Fn (N) | 33.27 | 31.36 | 33.21 | 31.09 | 28.22 | 29.16 | 28.63 | 28.87 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, Y.; Yang, S.; Tai, X.; Ma, H.; Guan, H.; Mu, Q.; Qu, L.; Ding, X. Study on Rubbing-Induced Vibration Characteristics Considering the Flexibility of Coated Casings and Blades. Machines 2024, 12, 481. https://doi.org/10.3390/machines12070481
Zhang Y, Yang S, Tai X, Ma H, Guan H, Mu Q, Qu L, Ding X. Study on Rubbing-Induced Vibration Characteristics Considering the Flexibility of Coated Casings and Blades. Machines. 2024; 12(7):481. https://doi.org/10.3390/machines12070481
Chicago/Turabian StyleZhang, Yong, Shuhua Yang, Xingyu Tai, Hui Ma, Hong Guan, Qinqin Mu, Lin Qu, and Xiangfu Ding. 2024. "Study on Rubbing-Induced Vibration Characteristics Considering the Flexibility of Coated Casings and Blades" Machines 12, no. 7: 481. https://doi.org/10.3390/machines12070481
APA StyleZhang, Y., Yang, S., Tai, X., Ma, H., Guan, H., Mu, Q., Qu, L., & Ding, X. (2024). Study on Rubbing-Induced Vibration Characteristics Considering the Flexibility of Coated Casings and Blades. Machines, 12(7), 481. https://doi.org/10.3390/machines12070481








