Preliminary Verification of the PHITS Code Applicability to Conversion Efficiency Calculation of Direct Charge Nuclear Battery
Abstract
1. Introduction
2. Methods
2.1. The Definition of DCNB Steady State Conversion Efficiency
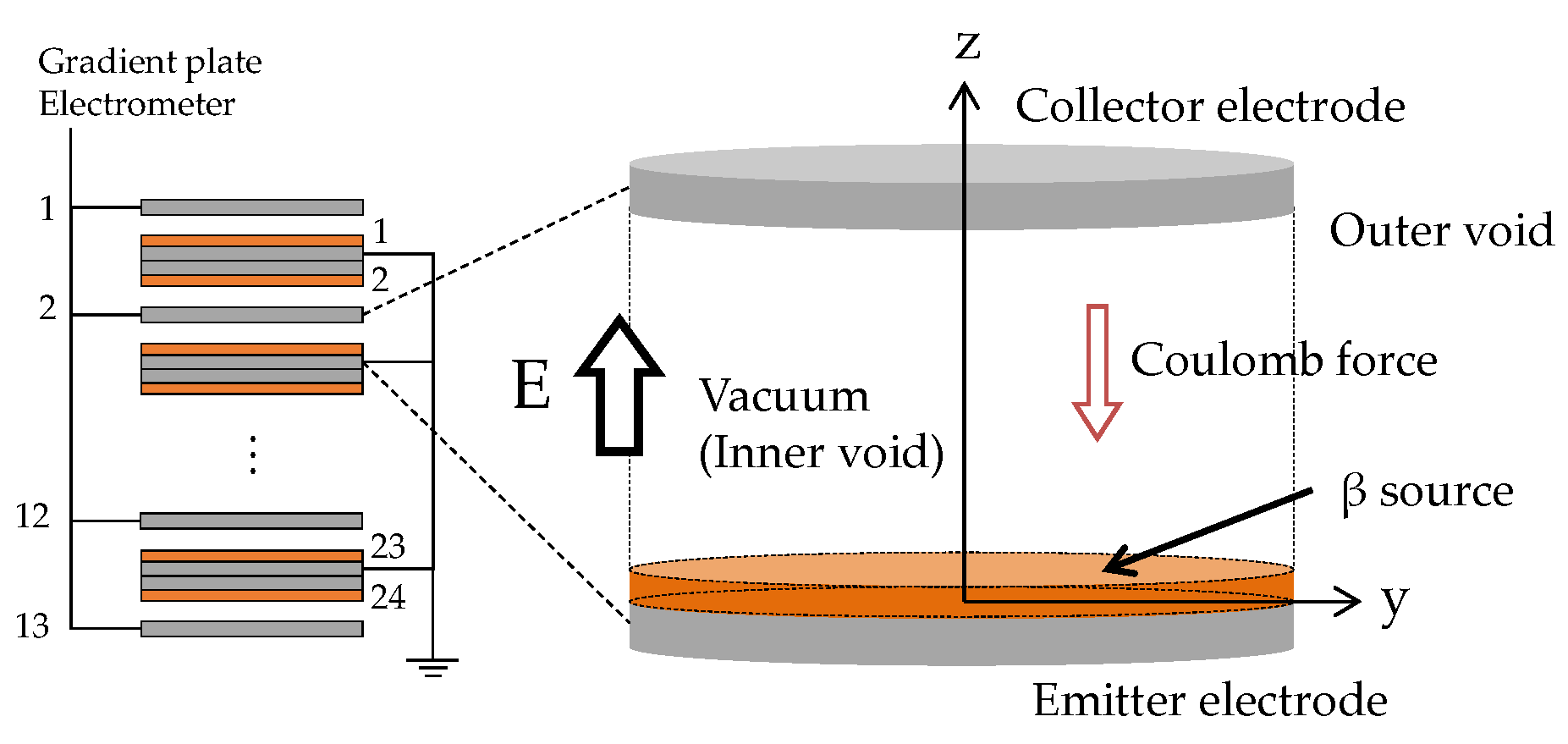
2.2. Calculation Model
2.3. Calculation Methodology
2.4. The Reference Experiment Methodology
2.4.1. Calculation of the Probability
2.4.2. Estimation of the Optimum Conversion Efficiency
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Nishihara, K.; Nakayama, S.; Morita, Y.; Oigawa, H.; Iwasaki, T. Impact of Partitioning and Transmutation on LWR High-Level Waste Disposal. J. Nucl. Sci. Technol. 2008, 45, 84–97. [Google Scholar] [CrossRef]
- Wakabayashi, T.; Tachi, Y.; Takahashi, M.; Chiba, S.; Takaki, N. Study on method to achieve high transmutation of LLFP using fast reactor. Sci. Rep. 2019, 9, 19156. [Google Scholar] [CrossRef] [PubMed]
- Olsen, L.C.; Cabauy, P.; Elkind, B.J. Betavoltaic power sources. Phys. Today 2012, 65, 35–38. [Google Scholar] [CrossRef]
- Terranova, M. Nuclear batteries: Current context and near-term expectations. Int. J. Energy Res. 2022, 46, 19368–19393. [Google Scholar] [CrossRef]
- Prelas, M.A.; Weaver, C.L.; Watermann, M.L.; Lukosi, E.D.; Schott, R.J.; Wisniewski, D.A. A review of nuclear batteries. Prog. Nucl. Energy 2014, 75, 117–148. [Google Scholar] [CrossRef]
- Prelas, M.; Boraas, M.; De La Torre Aguilar, F.; Seelig, J.-D.; Tchouaso, M.T.; Wisniewski, D. Nuclear Batteries and Radioisotopes; Springer: Cham, Switzerland, 2016. [Google Scholar]
- Moseley, H. The Attainment of High Potentials by the Use of Radium. Proc. R. Soc. Lond. 1913, A88, 471–476. [Google Scholar]
- Miley, G. Direct Conversion of Nuclear Radiation Energy; American Nuclear Society: La Grange Park, IL, USA, 1970. [Google Scholar]
- Yukabova, G.N. Nuclear Batteries with Tritium and Promethium-147 Radioactive Sources. Ph.D. Thesis, University of Illinois at Urbana-Champaign, Urbana, IL, USA, 2010. [Google Scholar]
- Kavetskiy, A.; Yakubova, G.; Yousaf, S.; Bower, K.; Robertson, J.; Garnov, A. Efficiency of Pm-147 direct charge radioisotope battery. Appl. Radiat. Isot. 2011, 69, 744–748. [Google Scholar] [CrossRef] [PubMed]
- Agostinelli, S.; Allison, J.; Amako, K.; Apostolakis, J.; Araujo, H.; Arce, P.; Asai, M.; Axen, D.; Banerjee, S.; Barrand, G.; et al. Geant4—A Simulation Toolkit. Nucl. Instrum. Methods Phys. Res. Sect. A 2003, 506, 250–303. [Google Scholar] [CrossRef]
- Wu, Y.; Song, J.; Zheng, H.; Sun, G.; Hao, L.; Long, P.; Hu, L. CAD-based Monte Carlo program for integrated simulation of nuclear system SuperMC. Ann. Nucl. Energy 2015, 82, 161–168. [Google Scholar] [CrossRef]
- Wang, X.; Han, Y.; Zhang, J.; Li, Z.; Li, T.; Zhao, X.; Wu, Y. The design of a direct charge nuclear battery with high energy conversion efficiency. Appl. Radiat. Isot. 2019, 148, 147–151. [Google Scholar] [CrossRef] [PubMed]
- Haim, Y.; Marciano, Y.; Debotton, G. Tunable direct beta-radiation harvester at the nanowatt scale. Sens. Actuators A Phys. 2018, 283, 228–234. [Google Scholar] [CrossRef]
- Haim, Y.; Debotton, G. Experimental Study of the Direct-Charge Beta Radiation Method for Energy Harvesting. IEEE Trans. Electron Devices 2020, 67, 5076–5081. [Google Scholar] [CrossRef]
- Haim, Y.; Debotton, G. Performance Analysis of the Direct-Charge β-Radiation Energy-Harvesting Method. IEEE Trans. Electron Devices 2021, 68, 2917–2925. [Google Scholar] [CrossRef]
- Iwamoto, Y.; Hashimoto, S.; Sato, T.; Matsuda, N.; Kunieda, S.; Çelik, Y.; Furutachi, N.; Niita, K. Benchmark study of particle and heavy-ion transport code system using shielding integral benchmark archive and database for accelerator-shielding experiments. J. Nucl. Sci. Technol. 2022, 59, 665–675. [Google Scholar] [CrossRef]
- Takezawa, H.; Kikuchi, T.; Otsu, N.; Nomura, A.; Uchida, Y.; Tamura, F. Preliminary Study on Conversion Efficiency of Direct Charge Nuclear Batteries Using Beta Sources for Low-Power Long-Life Battery Applications. In Proceedings of the 1st KOSEN Research International Symposium (KRIS2023), Tokyo, Japan, 1–2 March 2023. [Google Scholar]
- Live Chart of Nuclides. Available online: https://www-nds.iaea.org/relnsd/vcharthtml/VChartHTML.html (accessed on 19 January 2024).
- Hirayama, H.; Namito, Y.; Nelson, W.R.; Bielajew, A.F.; Wilderman, S.J.; Michigan, U. The EGS5 Code System. SLAC-R-730 and KEK Report 2005-8. 2005. Available online: https://rcwww.kek.jp/research/egs/egs5_manual/slac730-160113.pdf (accessed on 12 May 2024).
- Kavetsky, A.G.; Nekhoroshkov, S.N.; Meleshkov, S.P.; Kaminski, Y.L.; Akulov, G.P. Radioactive Materials, Ionizing Radiation Sources, and Radioluminescent Light Sources for Nuclear Batteries. In Polymers, Phosphors, and Voltaics for Radioisotope Microbatteries, 1st ed.; Bower, K.E., Barbanel, Y.A., Shreter, Y.G., Bohnert, G.W., Eds.; CRC Press: Boca Raton, FL, USA, 2002; pp. 39–108. [Google Scholar]
- Bochkarev, V.; Radzievsky, G.; Timofeev, L.; Demianov, N. Distribution of absorbed energy from a point beta source in a tissue-equivalent medium. Int. J. Appl. Radiat. Isot. 1972, 23, 493–497. [Google Scholar] [CrossRef] [PubMed]
- Walton, R.; Anthony, C.; Ward, M.; Metje, N.; Chapman, D. Radioisotopic battery and capacitor system for powering Wireless Sensor Networks. Sens. Actuators A Phys. 2013, 203, 405–412. [Google Scholar] [CrossRef]


| Isotope | Decay | Average Energy | Half-Life |
|---|---|---|---|
| T | β | 5.7 keV | 12.3 years |
| Ni-63 | β | 17.4 keV | 100.1 years |
| Pm-147 | β | 62 keV | 2.62 years |
| Sr-90 | β | 198 keV | 28.9 years |
| Y-90 | β | 930 keV | 64.6 hours |
| Cs-137 | β | 157 keV | 30.2 years |
| Pu-238 | α | 5.59 MeV | 87.7 years |
| UIUC Condition | Reproduction Calculation | |
|---|---|---|
| β source isotope | T | ← |
| Material of the source | ScT2 | ← |
| Dimensions of the source | 10 cm in diameter 300 nm in thickness | ← |
| Mass density of ScT2 | 2.9 g/cm3 | ← |
| Material of the electrodes | Stainless steel | SUS-304 |
| Mass density of the electrodes | N/A | 8.03 g/cm3 |
| Dimensions of the electrodes | 10 cm in diameter 0.5 mm in thickness | ← |
| Coating on collector electrode | Polyimide | Ignored |
| Electrode gap | 5.0 mm | ← |
| Gap pressure | Less than 10−5 Torr | Inner void |
| Voltage between electrodes | N/A | 0.0–20.0 kV |
| PHITS version | N/A | 3.24 |
| Number of particles | N/A | 100,000 |
| Number of batches | N/A | 50 |
| Direction of source β particles | N/A | Isotropic |
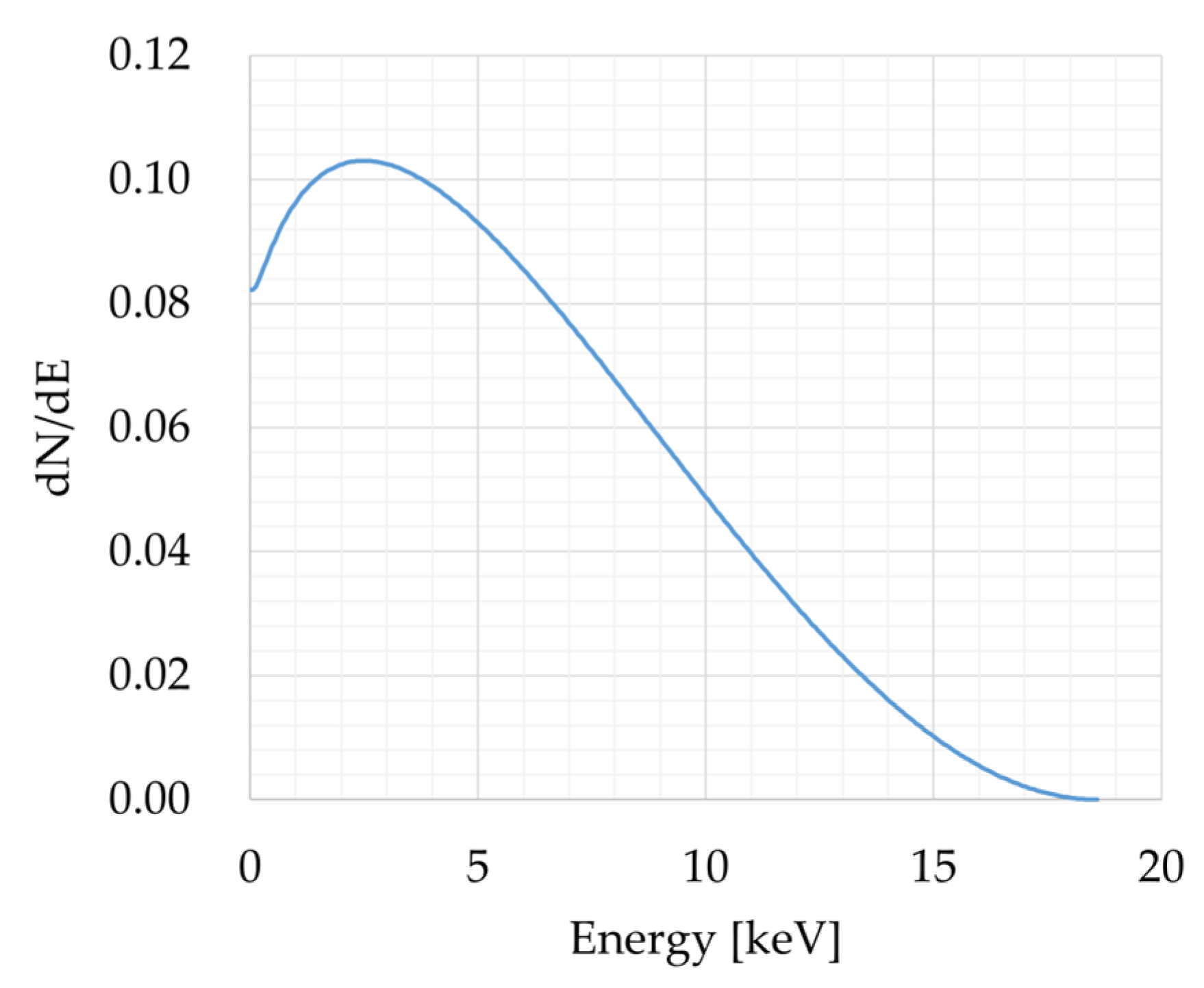
| Energy of source β particles | N/A | Figure 3 |
| Distribution of source β particles | N/A | Uniform in β source layer |
| N/A | 20.0 keV |
| SUS-304 Emitter Electrode | Reproduction Calculation | UIUC Condition |
|---|---|---|
| Included | 0.247 (σ: 0.35%) | N/A |
| Not included | 0.235 (σ: 0.34%) | 0.23 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Takezawa, H.; Kigeuchi, R.; Umeda, H.; Tamura, F.; Uchida, Y.; Kikuchi, T. Preliminary Verification of the PHITS Code Applicability to Conversion Efficiency Calculation of Direct Charge Nuclear Battery. Machines 2024, 12, 395. https://doi.org/10.3390/machines12060395
Takezawa H, Kigeuchi R, Umeda H, Tamura F, Uchida Y, Kikuchi T. Preliminary Verification of the PHITS Code Applicability to Conversion Efficiency Calculation of Direct Charge Nuclear Battery. Machines. 2024; 12(6):395. https://doi.org/10.3390/machines12060395
Chicago/Turabian StyleTakezawa, Hiroki, Ryuma Kigeuchi, Hibiki Umeda, Fumihiro Tamura, Yuki Uchida, and Takashi Kikuchi. 2024. "Preliminary Verification of the PHITS Code Applicability to Conversion Efficiency Calculation of Direct Charge Nuclear Battery" Machines 12, no. 6: 395. https://doi.org/10.3390/machines12060395
APA StyleTakezawa, H., Kigeuchi, R., Umeda, H., Tamura, F., Uchida, Y., & Kikuchi, T. (2024). Preliminary Verification of the PHITS Code Applicability to Conversion Efficiency Calculation of Direct Charge Nuclear Battery. Machines, 12(6), 395. https://doi.org/10.3390/machines12060395





