Analytical Modeling of Eddy Current Losses and Thermal Analysis of Non-Uniform-Air-Gap Combined-Pole Permanent Magnet Motors for Electric Vehicles
Abstract
1. Introduction
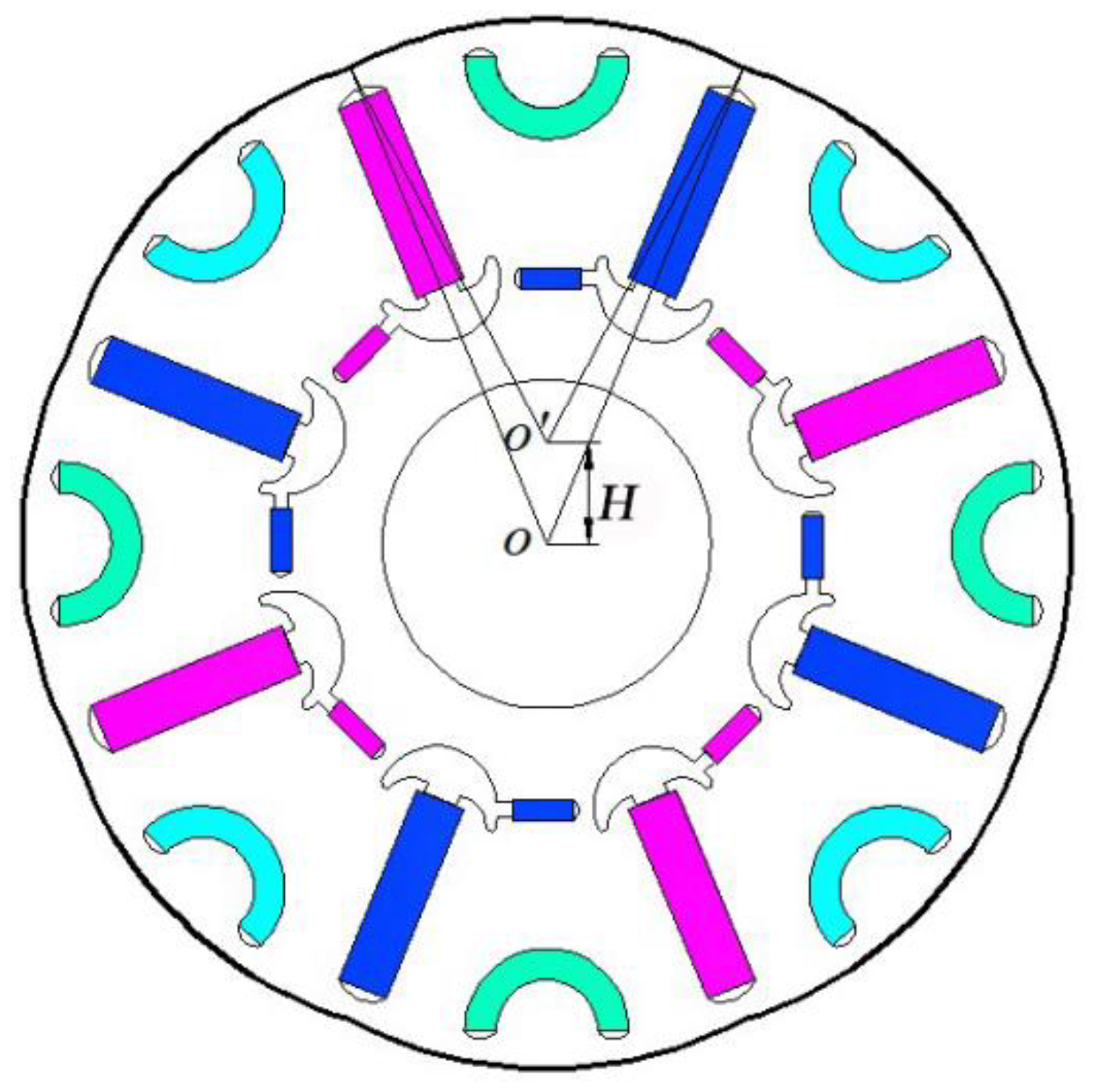
2. Structural Parameters of Permanent Magnet Motors
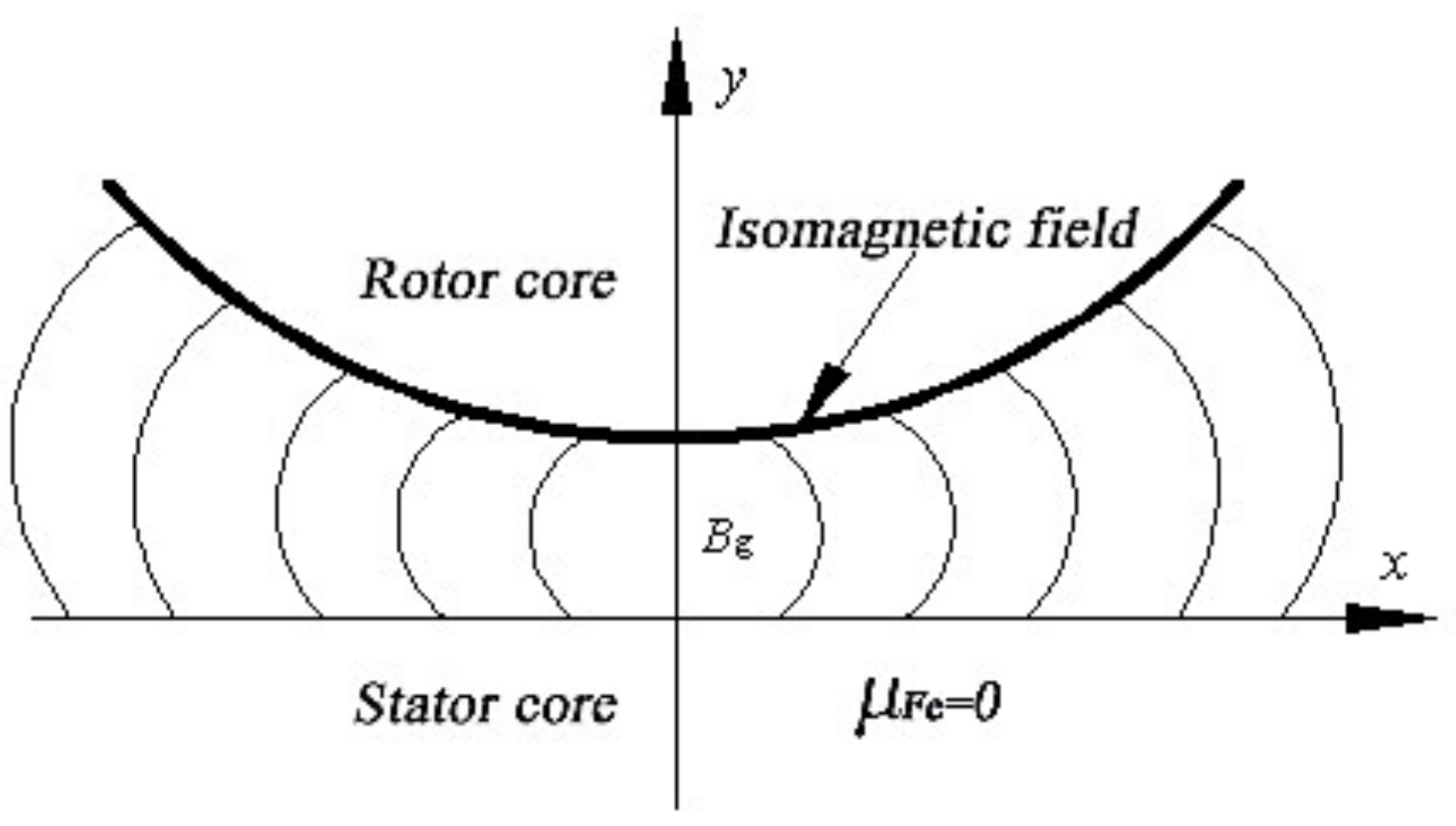
3. Establishment of a Subdomain Model of the Sinusoidal Distribution of a Non-Uniform Air Gap
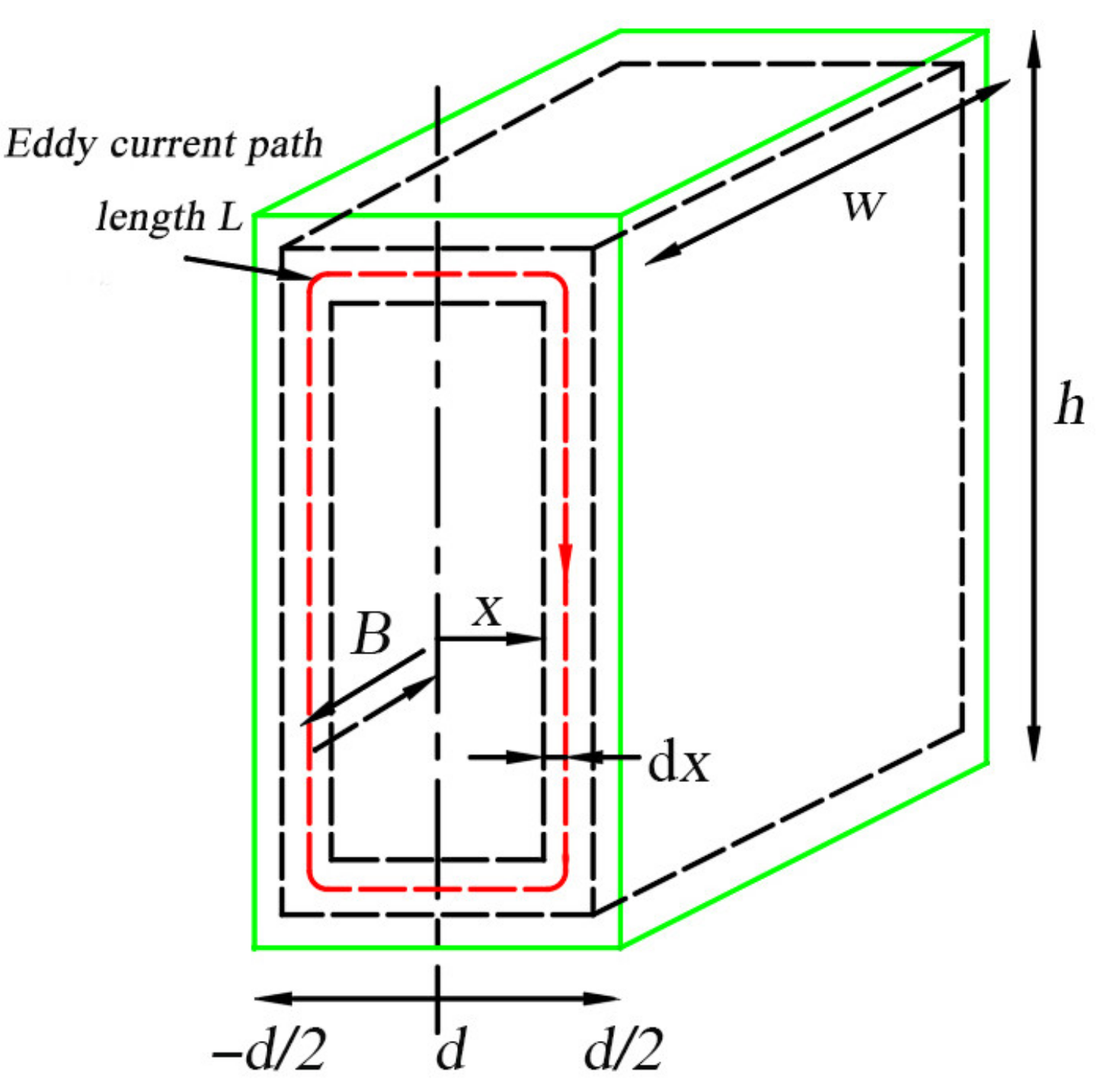
4. Establishment of an Analytical Model for Eddy Current Losses in the Rotor Core
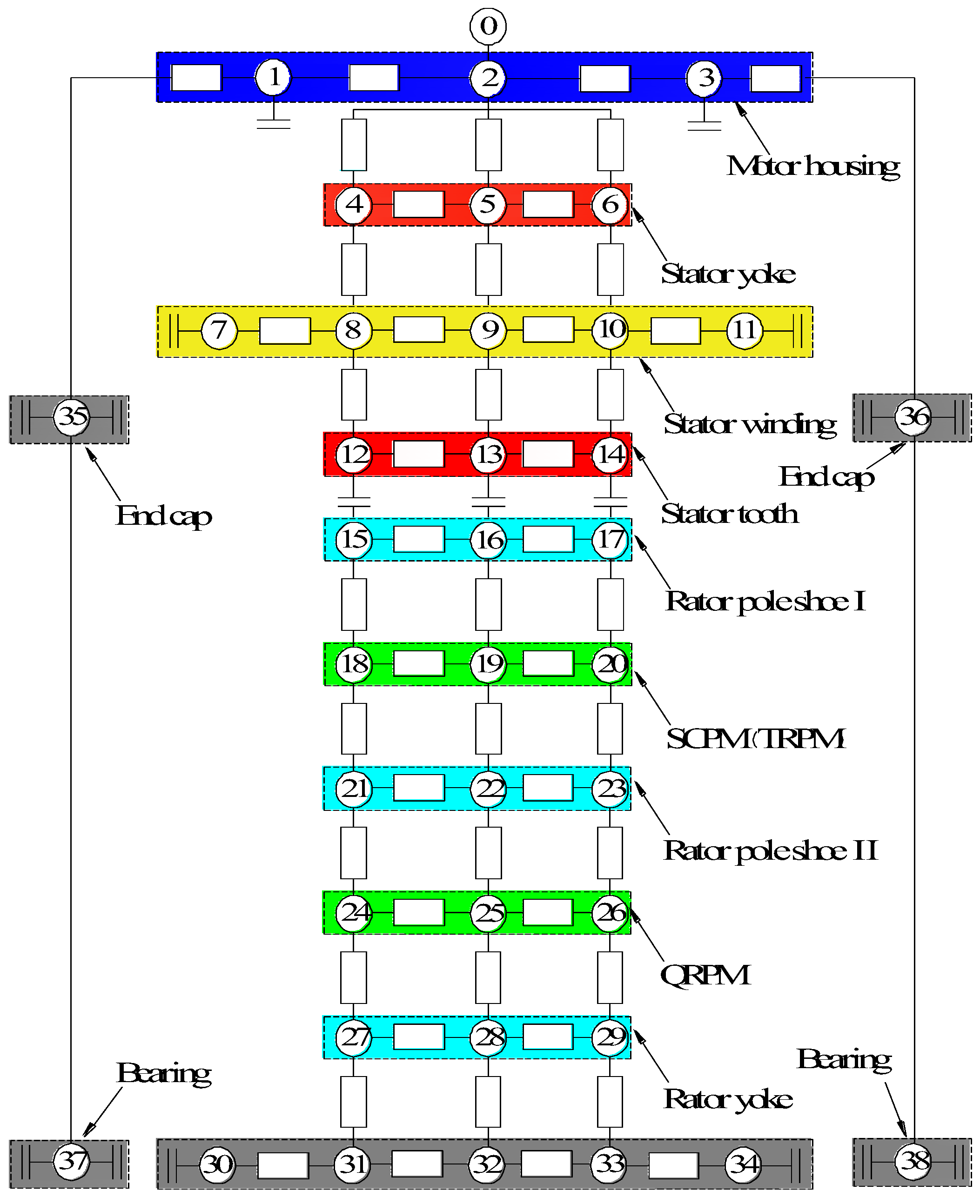
5. Establishment of an Equivalent Thermal Network Model
5.1. Equivalent Thermal Network Model
5.2. Equivalent Model of Armature Winding
5.3. Calculation Results of the Equivalent Thermal Network Model
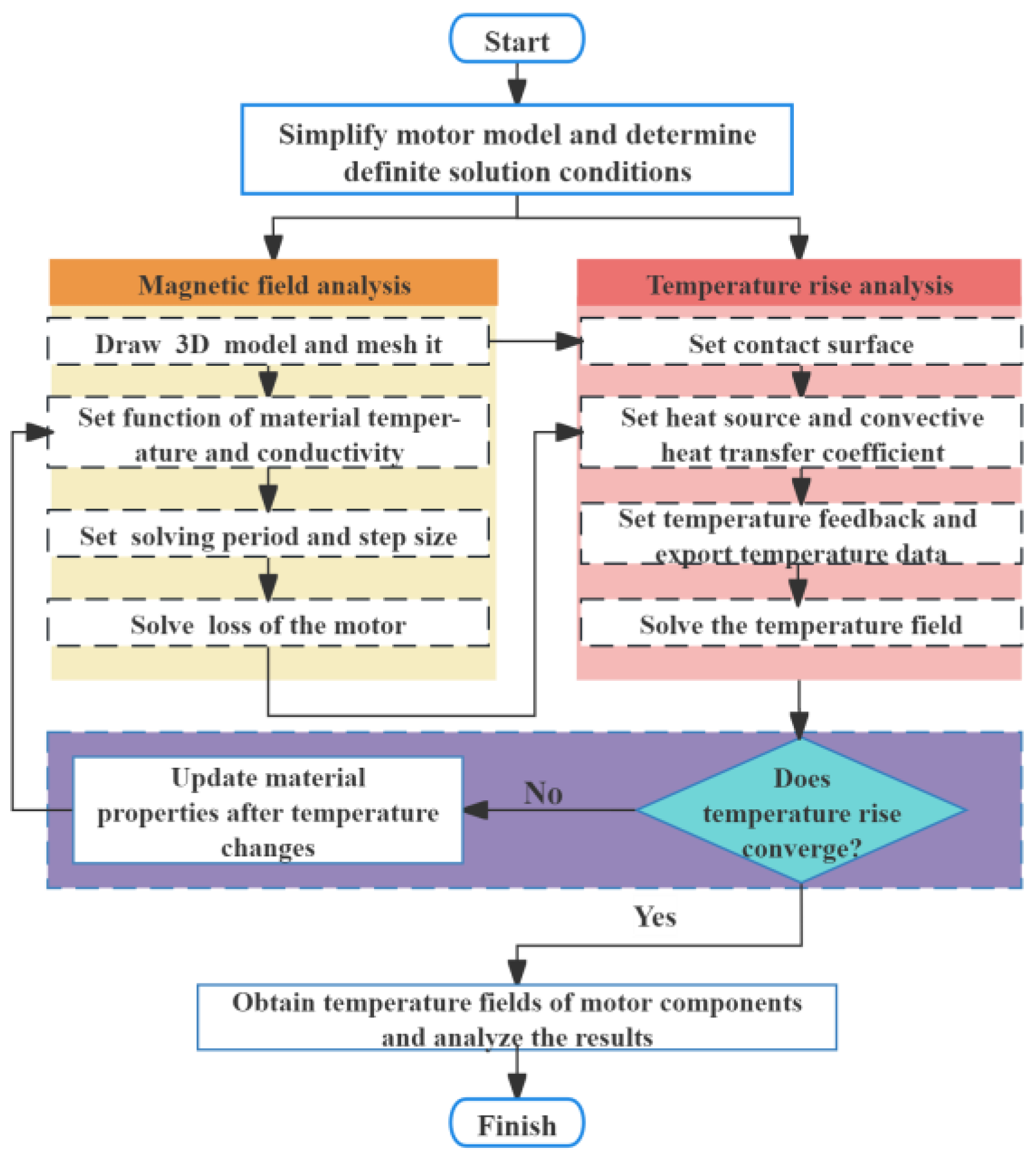
6. Magnetothermal Bidirectional Coupling Finite Element Simulation Analysis
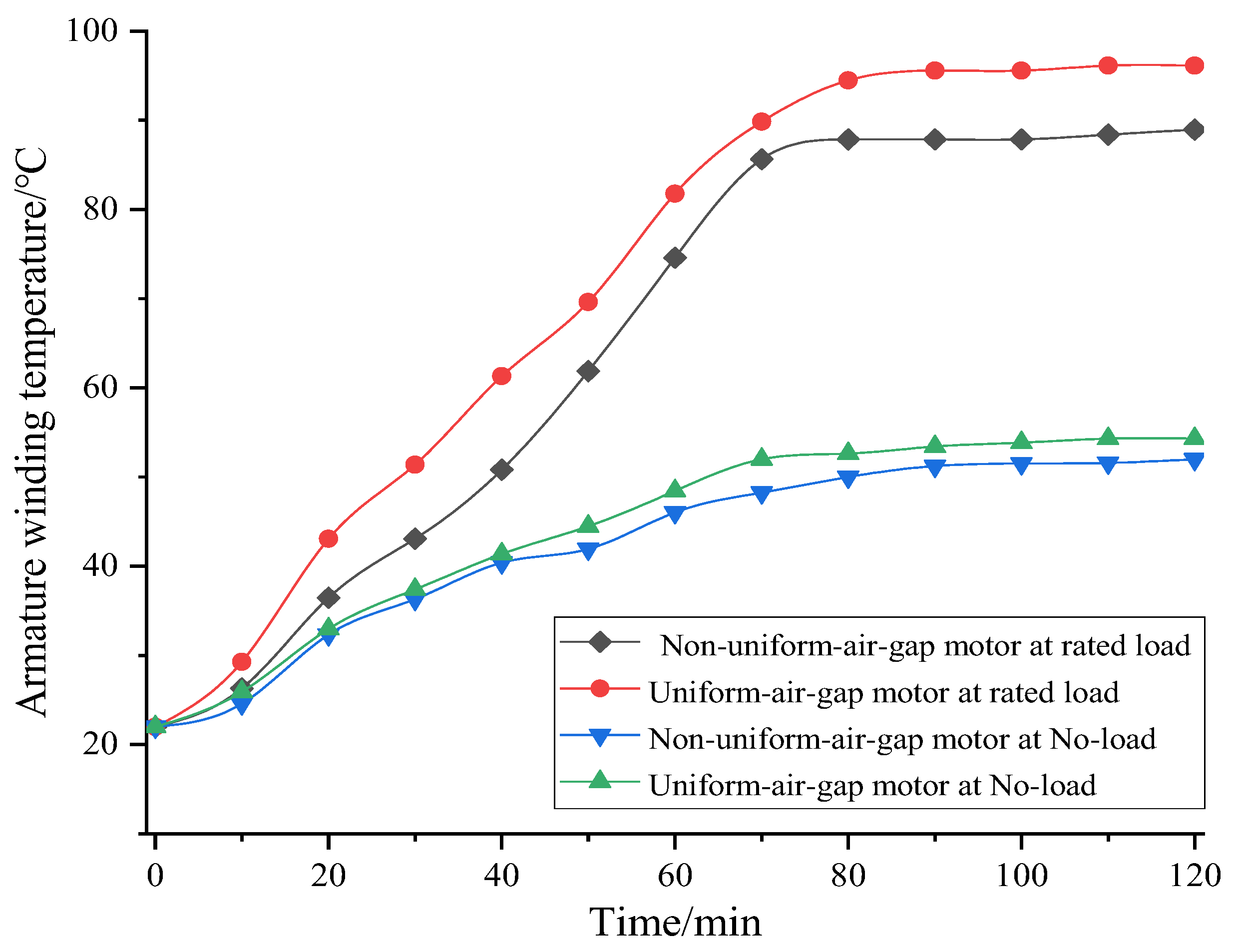
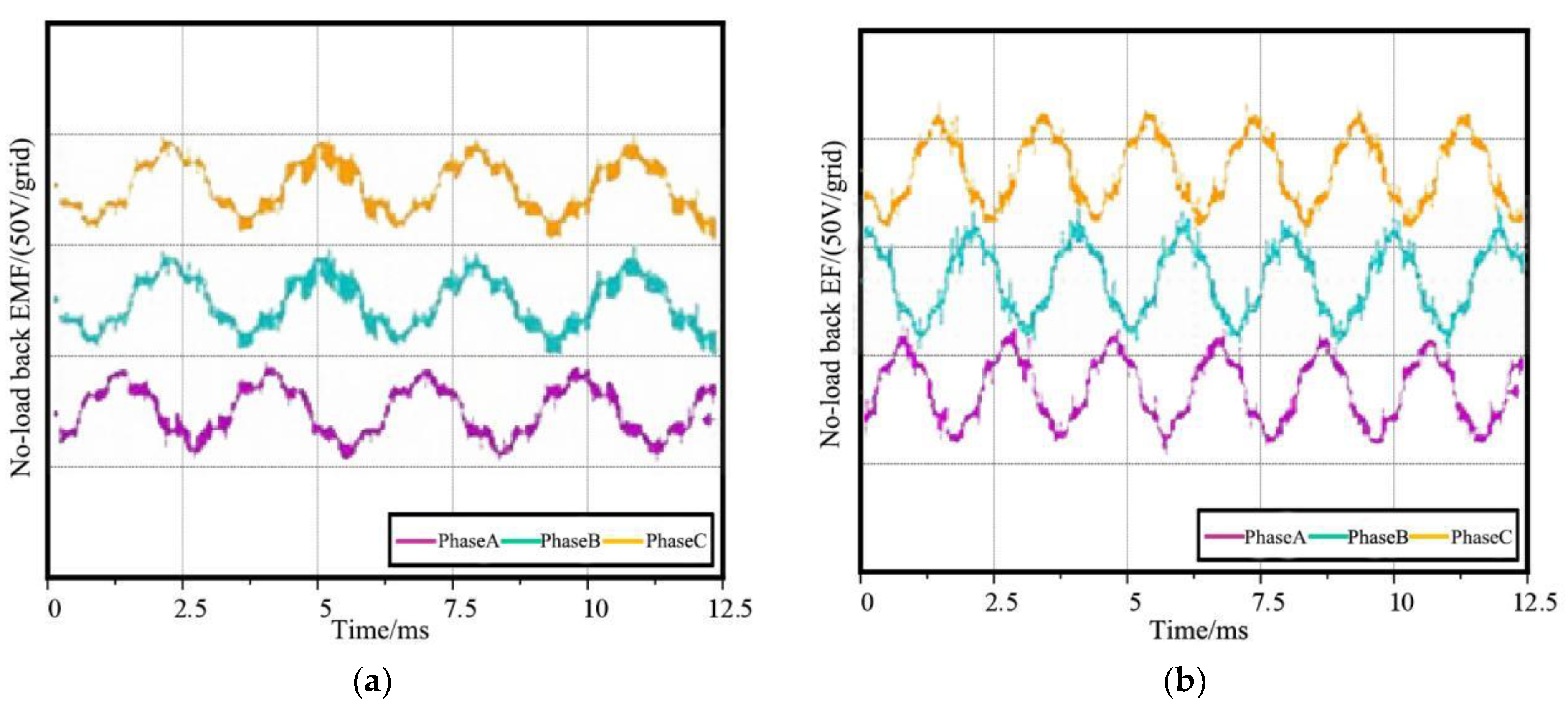
7. Experimental Validation
8. Conclusions
- (1)
- We propose a new type of interior combined-pole permanent magnet motor for electric vehicles. Through the establishment of a sinusoidal subdomain model of the non-uniform-air-gap distribution and eddy current loss model, the analytical expression between rotor eccentricity and eddy current loss was derived. It was determined that when the ITRPMM rotor eccentricity is 4 mm, the waveform of the back EMF is more similar to the sine law. The content of higher harmonics in the air gap and the eddy current losses are the least.
- (2)
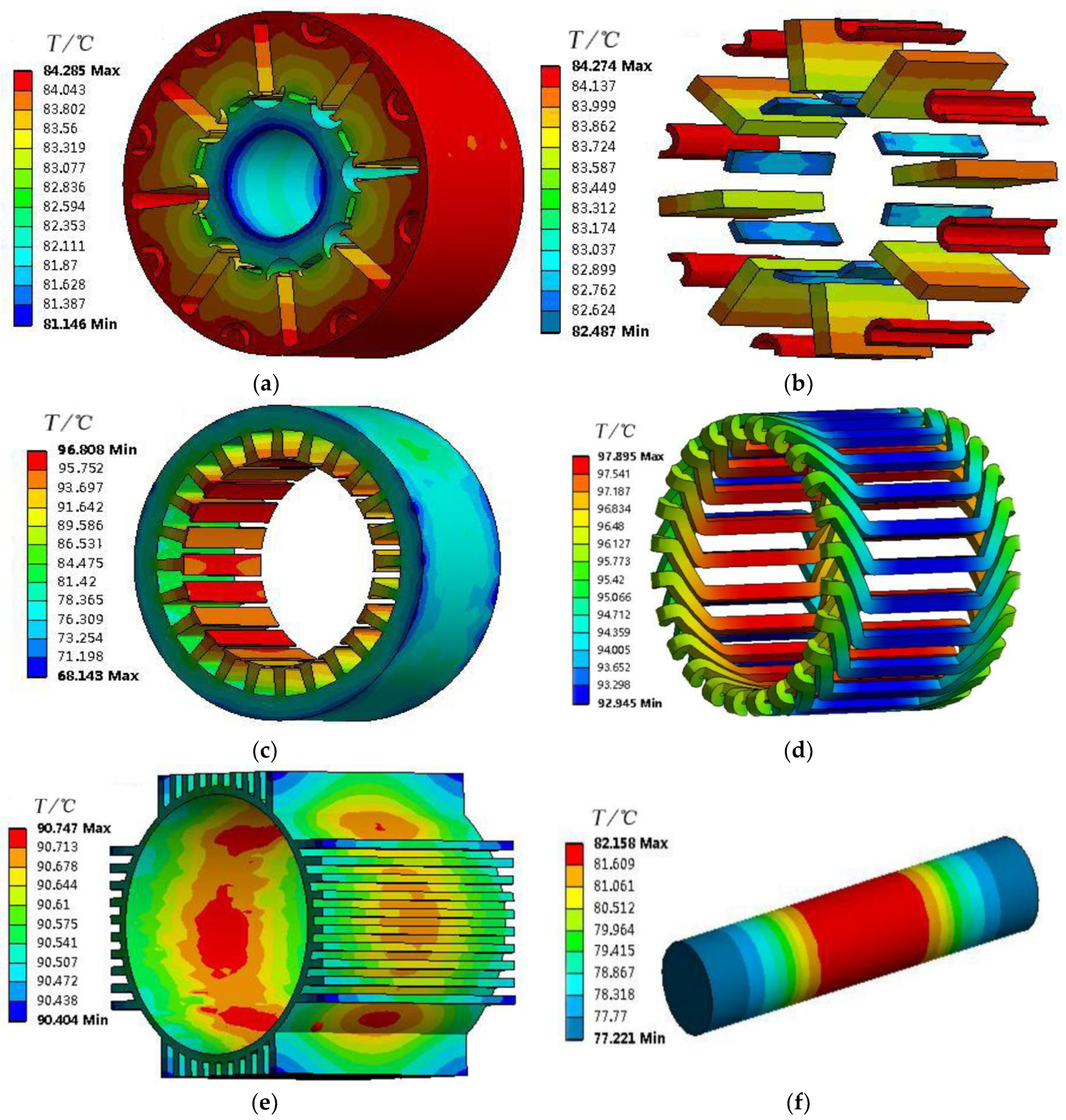
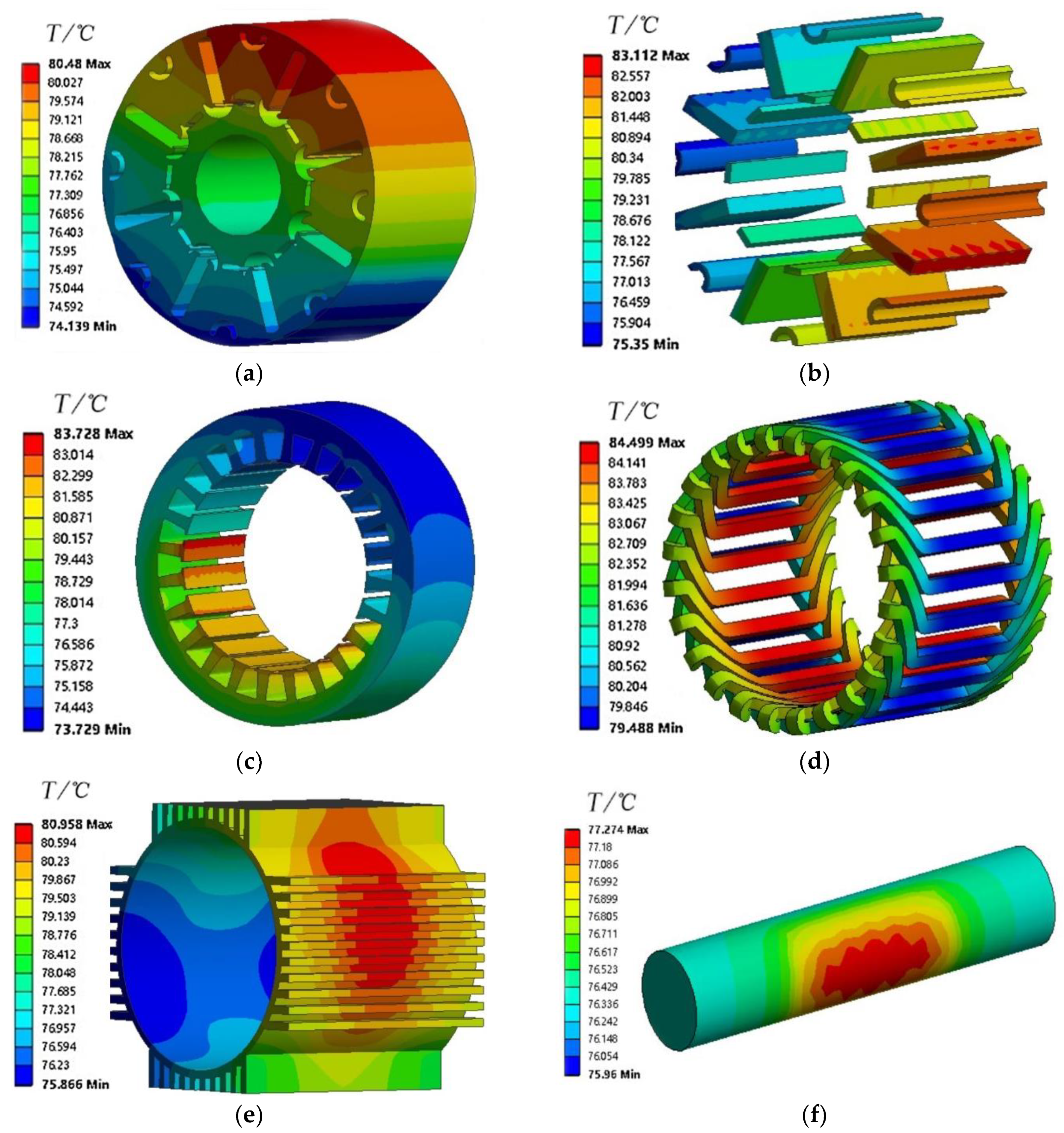
- The equivalent thermal network model of the ITRPMM was established, the temperature rise matrix expression of the ITRPMM was obtained, and the temperature rise of each node of the motor was calculated. Finite element simulations of the uniform-air-gap and non-uniform-air-gap permanent magnet motors were verified using the magnetothermal bidirectional coupling method. When the permanent magnet motor adopts a non-uniform air gap structure, the temperature rise of each component significantly reduces. The calculation results of the equivalent thermal network method are between the simulation results of the uniform-air-gap and non-uniform-air-gap permanent magnet motors, and the armature winding is the component with the largest error in the calculation results of the analytical and finite element methods.
- (3)
- The prototype experiment showed that the permanent magnet motor with a non-uniform air gap structure can effectively reduce the rotor eddy current losses, and the no-load back EMF waveform distortion rate of the non-uniform-air-gap motor is 15.9%, while the no-load back EMF waveform distortion rate of the uniform-air-gap motor is 21.8%. Meanwhile, the non-uniform-air-gap motor can reduce the motor temperature rise and increase the output torque of the motor. When the two motors adopt the same control strategy, the maximum output torque of the non-uniform-air-gap motor is 34 N·m, while that of the uniform-air-gap motor is 33.4 N·m, which is conducive to improving the power density of the motor and is more suitable for permanent magnet motors for electric vehicles.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Liu, X.; Chen, H.; Zhao, J.; Belahcen, A. Research on the performances and parameters of interior PMSM used for electric vehicles. IEEE Trans. Ind. Electron. 2016, 63, 3533–3545. [Google Scholar] [CrossRef]
- Kou, B.; Zhao, X.; Zhang, H.; Wang, M. Review and analysis of electromagnetic structure and magnetic field regulation technology of the permanent magnet synchronous motor. Proc. CSEE 2021, 41, 7126–7141. [Google Scholar]
- Li, X.; Kan, C.; Ren, T.; Chen, F.; Guo, P.; Yao, Z. Thermal network modeling and thermal stress evaluation for a high-power linear ultrasonic motor. IEEE Trans. Ind. Appl. 2022, 58, 7181–7191. [Google Scholar] [CrossRef]
- Lin, M.; Le, W.; Lin, K.; Jia, L.; Wang, S.; Yang, A.; Tu, Y. Overview on research and development of thermal design methods of axial flux permanent magnet machines. Proc. CSEE 2021, 41, 1914–1929. [Google Scholar]
- Dajaku, G.; Xie, W.; Gerling, D. Reduction of low space harmonics for the fractional slot concentrated windings using a novel stator design. IEEE Trans. Magn. 2014, 50, 8201012. [Google Scholar] [CrossRef]
- Hwang, S.-W.; Ryu, J.-Y.; Chin, J.-W.; Park, S.-H.; Kim, D.-K.; Lim, M.-S. Coupled electromagnetic-thermal analysis for predicting traction motor characteristics according to electric vehicle driving cycle. IEEE Trans. Veh. Technol. 2021, 70, 4262–4272. [Google Scholar] [CrossRef]
- Liu, X.; Zhu, Z.; Chen, D.; Shi, Z.; Sun, G. Analysis and experimental study of eddy current loss in axial flux permanent magnet motor based on rotor permanent magnet segment optimisation. J. Electrotechnol. 2024, 239632. [Google Scholar] [CrossRef]
- Chen, Q.; Liang, D.; Jia, S.; Wan, X. Analysis of multi-phase and multi-layer factional-slot concentrated-winding on pm eddy current loss considering axial segmentation and load operation. IEEE Trans. Magn. 2018, 54, 8107506. [Google Scholar] [CrossRef]
- Dajaku, G.; Gerling, D. Eddy current loss minimization in rotor magnets of PM machines using high-efficiency 12-teeth/10-slots winding topology. In Proceedings of the 2011 International Conference on Electrical Machines and Systems, Beijing, China, 20–23 August 2011; pp. 1–6. [Google Scholar]
- Chen, Z.; Xing, N.; Ma, H.; Li, Z.; Zhang, H. Modelling and analysis of harmonic eddy current losses in permanent magnets of fractional slot permanent magnet motors. J. Electrotechnol. 2022, 37, 3514–3527. [Google Scholar] [CrossRef]
- Benlamine, R.; Dubas, F.; Randi, S.-A.; Lhotellier, D.; Espanet, C. 3-D numerical hybrid method for PM eddy-current losses calculation: Application to axial-flux PMSMs. IEEE Trans. Magn. 2015, 51, 8106110. [Google Scholar] [CrossRef]
- Tong, W.; Hou, M.; Sun, L.; Hou, M.; Wu, S. Analysis Method of Rotor Eddy Current Loss of High Speed permanent magnet Motor with retaining sleeveed rotor based on precise subdomain Model. Trans. China Electrotech. Soc. 2022, 37, 4047–4059. [Google Scholar]
- Song, S.; Hu, M.; Du, Y.; Zuo, H.; Zhang, F. Temperature field investigation of remanufacturing motor with mixed stator core. Electr. Mach. Control 2020, 24, 33–42. [Google Scholar]
- Wu, S.; Hao, D.; Tong, W. Thermal calculation of high speed permanent magnet synchronous motor based on equivalent thermal network and CFD method. Electr. Mach. Control 2022, 26, 29–36. [Google Scholar]
- Tang, W.; Liang, Y. Analysis and Calculation of Electromagnetic Field of Motor; China Machine Press: Beijing, China, 2010; p. 43. [Google Scholar]
- Chen, S. Motor Design; China Machine Press: Beijing, China, 2010; p. 77. [Google Scholar]
- Shi, Y.; Wang, J.; Wang, B. Transient 3-D lumped parameter and 3-D FE thermal models of a PMASynRM under fault conditions with asymmetric temperature distribution. IEEE Trans. Ind. Electron. 2021, 68, 4623–4633. [Google Scholar] [CrossRef]
- Ding, S.; Jiang, X.; Zhu, M.; Liu, W. Starting and steady temperature rise investigation for permanent magnet synchronous motor based on lumped-parameter thermal-network. Electr. Mach. Control 2020, 24, 143–150. [Google Scholar]
- Zhang, J.; Zhu, X.; Zhang, Z. Thermal Network Modeling and Thermal Characteristics Analysis of Doubly Salient Brushless DC Generator With Stator Field Winding. Proceeding CSEE 2023, 43, 318–329. [Google Scholar]
- Wang, X.; Gao, P. Application of equivalent thermal network method and finite element method in temperature calculation of in-wheel motor. Trans. China Electrotech. Soc. 2016, 31, 26–33. [Google Scholar]


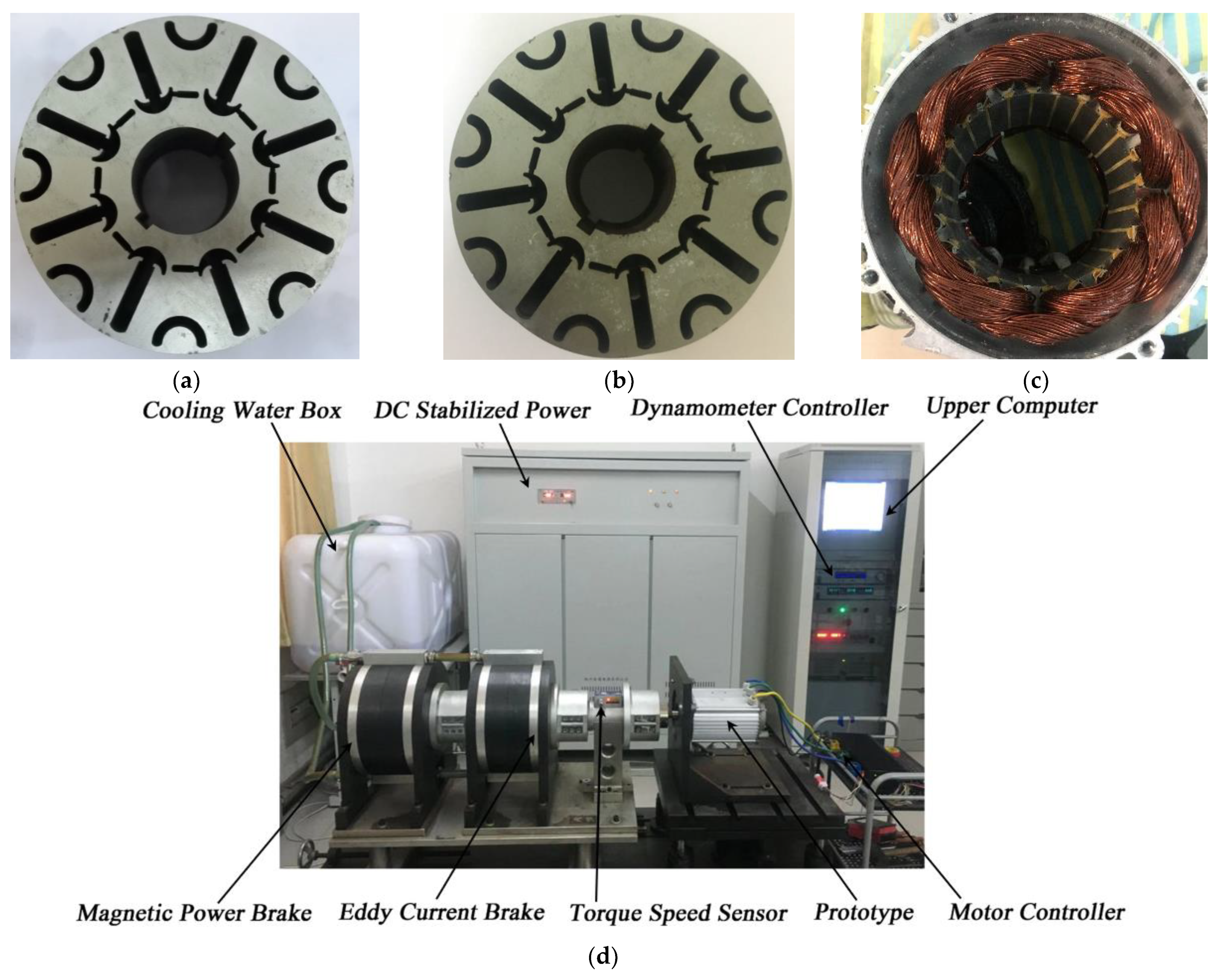


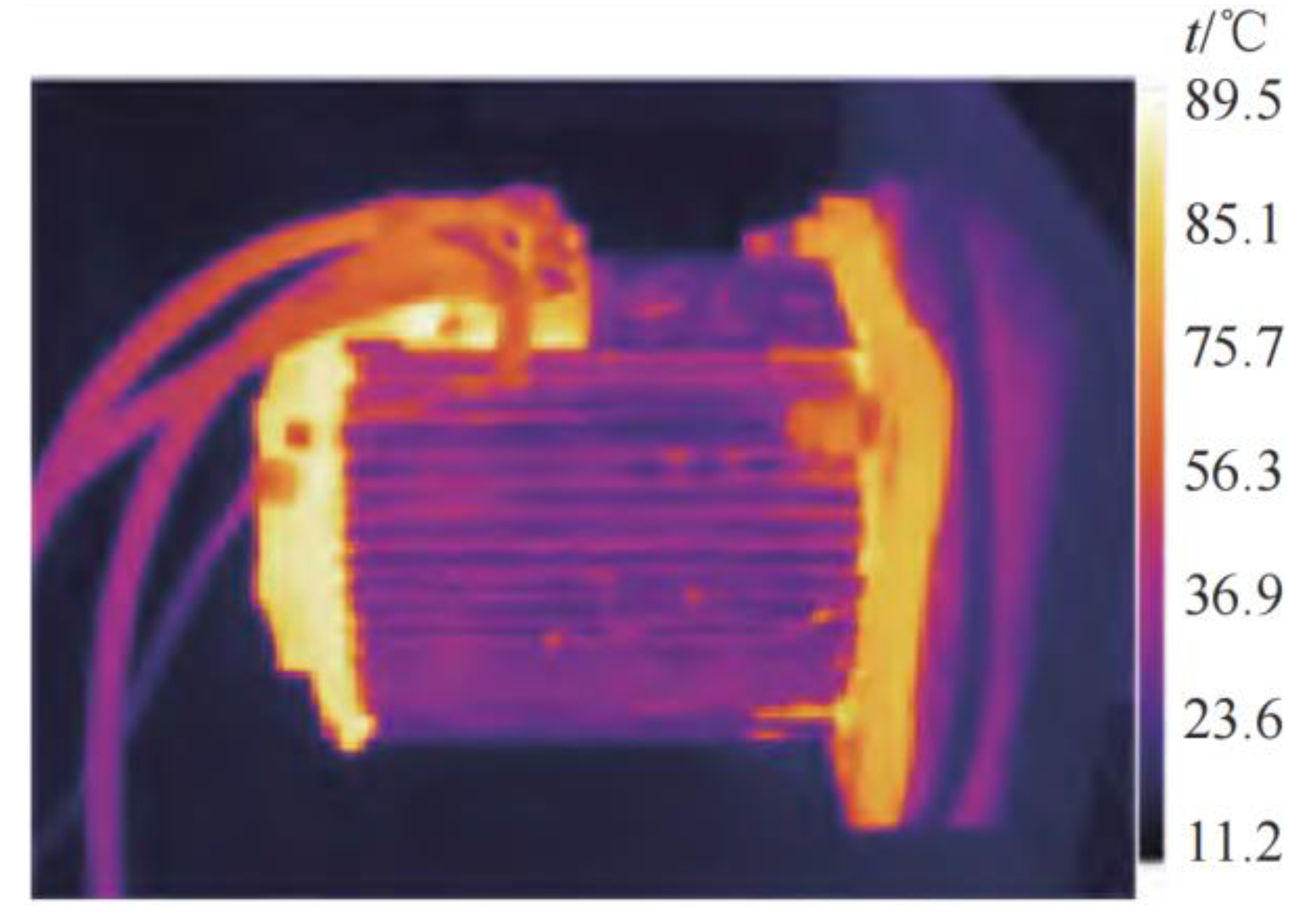


| Parameter | Value | Parameter | Value |
|---|---|---|---|
| Rated voltage/(V) | 60 | Rated speed/(r·min) | 3000 |
| Rated power/(kW) | 3 | Outer diameter of rotor/(mm) | 89 |
| Number of pole pairs | 4 | Interior diameter of rotor/(mm) | 30 |
| Slots | 36 | Axial length/(mm) | 60 |
| Inner diameter of stator/(mm) | 90 | Number of turns per slot | 11 |
| Outer diameter of stator/(mm) | 145 | Volume of permanent magnet/(mm3) | 72,000 |
| Component (Material) | λ /(W/m·K) | c /(J/kg·K) | /(kg·m−3) |
|---|---|---|---|
| Stator winding (copper) | 379 | 383 | 8954 |
| Stator and rotor (silicon steel) | 40.6 | 426 | 7700 |
| Permanent magnet (N35UH) | 7.6 | 4600 | 7500 |
| Shaft (steel) | 46 | 4800 | 7850 |
| Motor housing (aluminum) | 230 | 8800 | 2700 |
| Air gap (air) | 0.026 | 1.4 | 1293 |
| Component Name | Equivalent Thermal Network Method/ (°C) | Simulation Value of Uniform Air Gap/ (°C) | Simulation Value of Non-Uniform Air Gap/ (°C) |
|---|---|---|---|
| Motor housing | 84.6 | 90.5 | 82.5 |
| Stator yoke | 86.5 | 88.42 | 81.3 |
| Armature winding | 90.3 | 96.65 | 83.5 |
| Stator teeth | 91.8 | 93.75 | 80.5 |
| SCPM | 85.6 | 84.17 | 79.23 |
| TRPM | 83.5 | 84.27 | 77.32 |
| Rotor core | 82.1 | 83.71 | 76.85 |
| RRPM | 81.8 | 82.76 | 79.78 |
| Shaft | 77.8 | 79.68 | 76.56 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ma, S.; Ma, J.; Chen, K.; Li, C. Analytical Modeling of Eddy Current Losses and Thermal Analysis of Non-Uniform-Air-Gap Combined-Pole Permanent Magnet Motors for Electric Vehicles. Machines 2024, 12, 377. https://doi.org/10.3390/machines12060377
Ma S, Ma J, Chen K, Li C. Analytical Modeling of Eddy Current Losses and Thermal Analysis of Non-Uniform-Air-Gap Combined-Pole Permanent Magnet Motors for Electric Vehicles. Machines. 2024; 12(6):377. https://doi.org/10.3390/machines12060377
Chicago/Turabian StyleMa, Shilun, Jianwei Ma, Keqi Chen, and Changwei Li. 2024. "Analytical Modeling of Eddy Current Losses and Thermal Analysis of Non-Uniform-Air-Gap Combined-Pole Permanent Magnet Motors for Electric Vehicles" Machines 12, no. 6: 377. https://doi.org/10.3390/machines12060377
APA StyleMa, S., Ma, J., Chen, K., & Li, C. (2024). Analytical Modeling of Eddy Current Losses and Thermal Analysis of Non-Uniform-Air-Gap Combined-Pole Permanent Magnet Motors for Electric Vehicles. Machines, 12(6), 377. https://doi.org/10.3390/machines12060377






