Figure 1.
Rotor coordinate system and finite element method illustration.
Figure 1.
Rotor coordinate system and finite element method illustration.
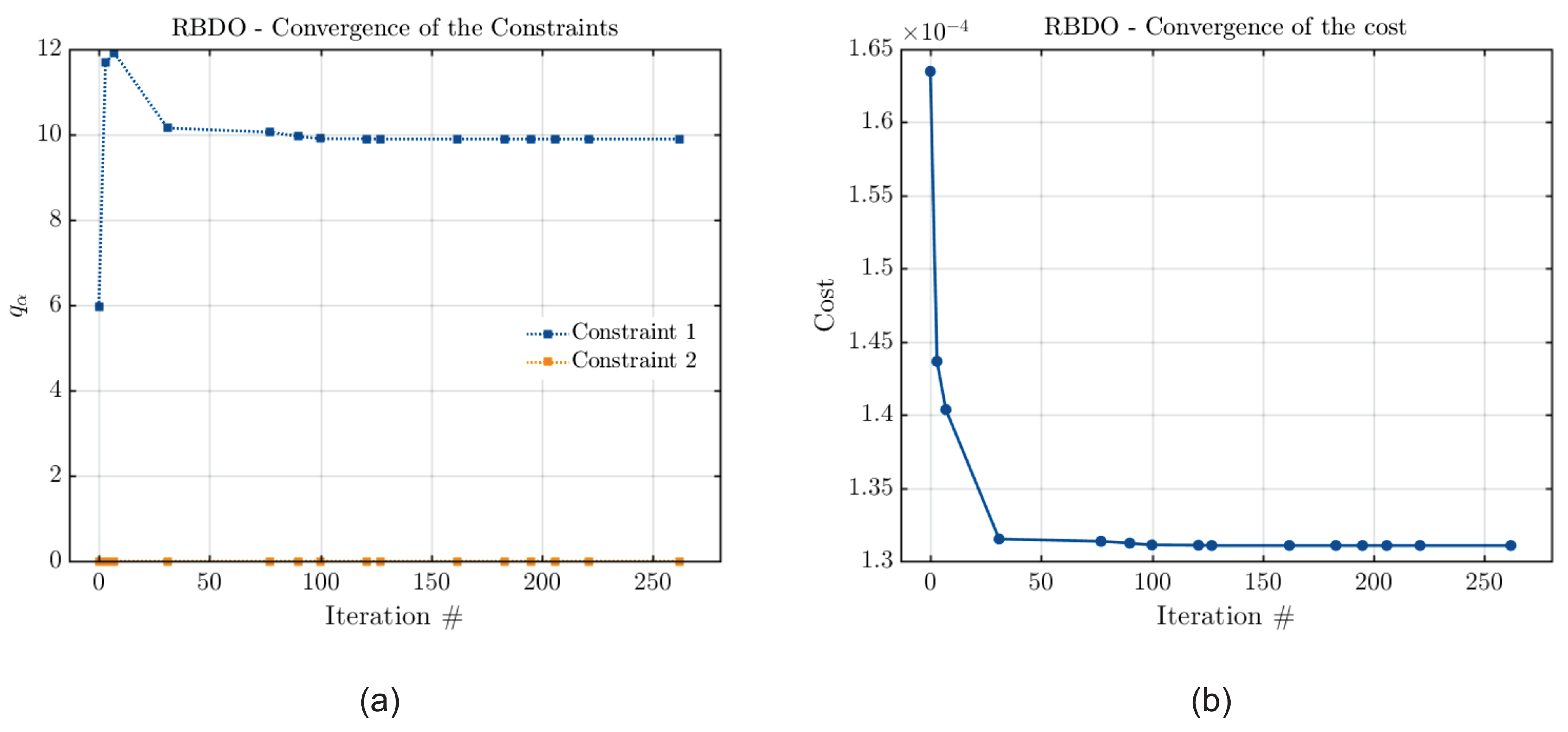
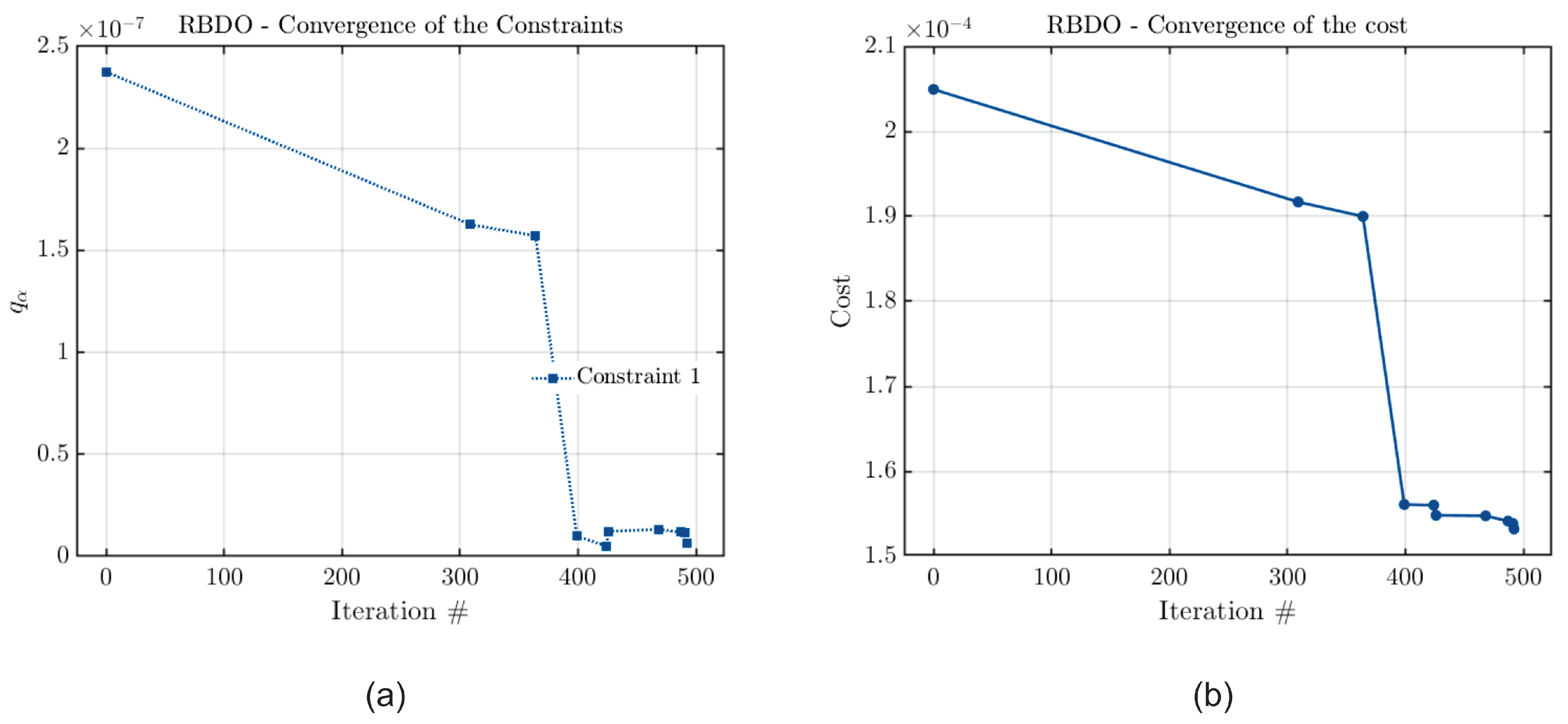
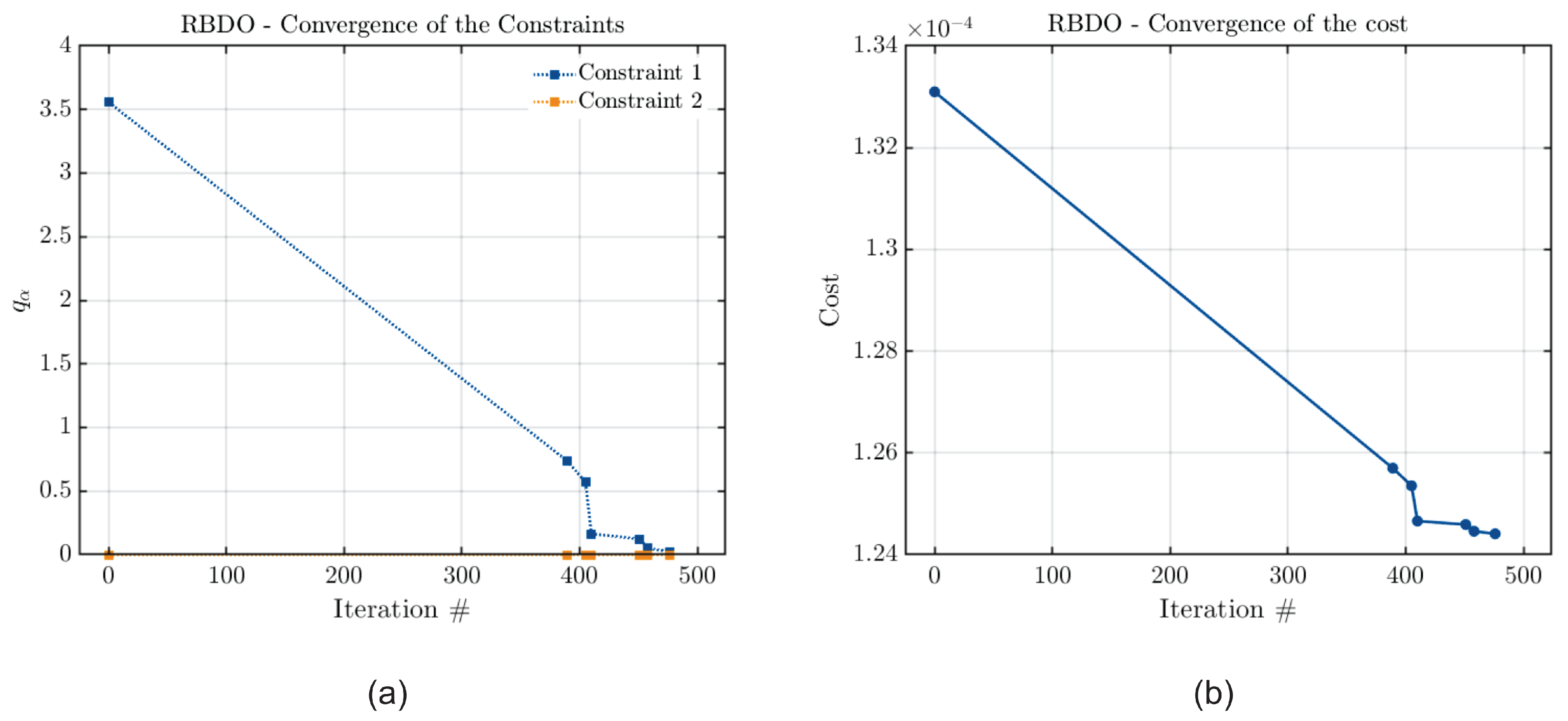
Figure 2.
Convergence curves of (a) the constraint and (b) the cost functions considering Kriging, with the rotor shaft diameter optimization and the vibration amplitude limit as a constraint.
Figure 2.
Convergence curves of (a) the constraint and (b) the cost functions considering Kriging, with the rotor shaft diameter optimization and the vibration amplitude limit as a constraint.
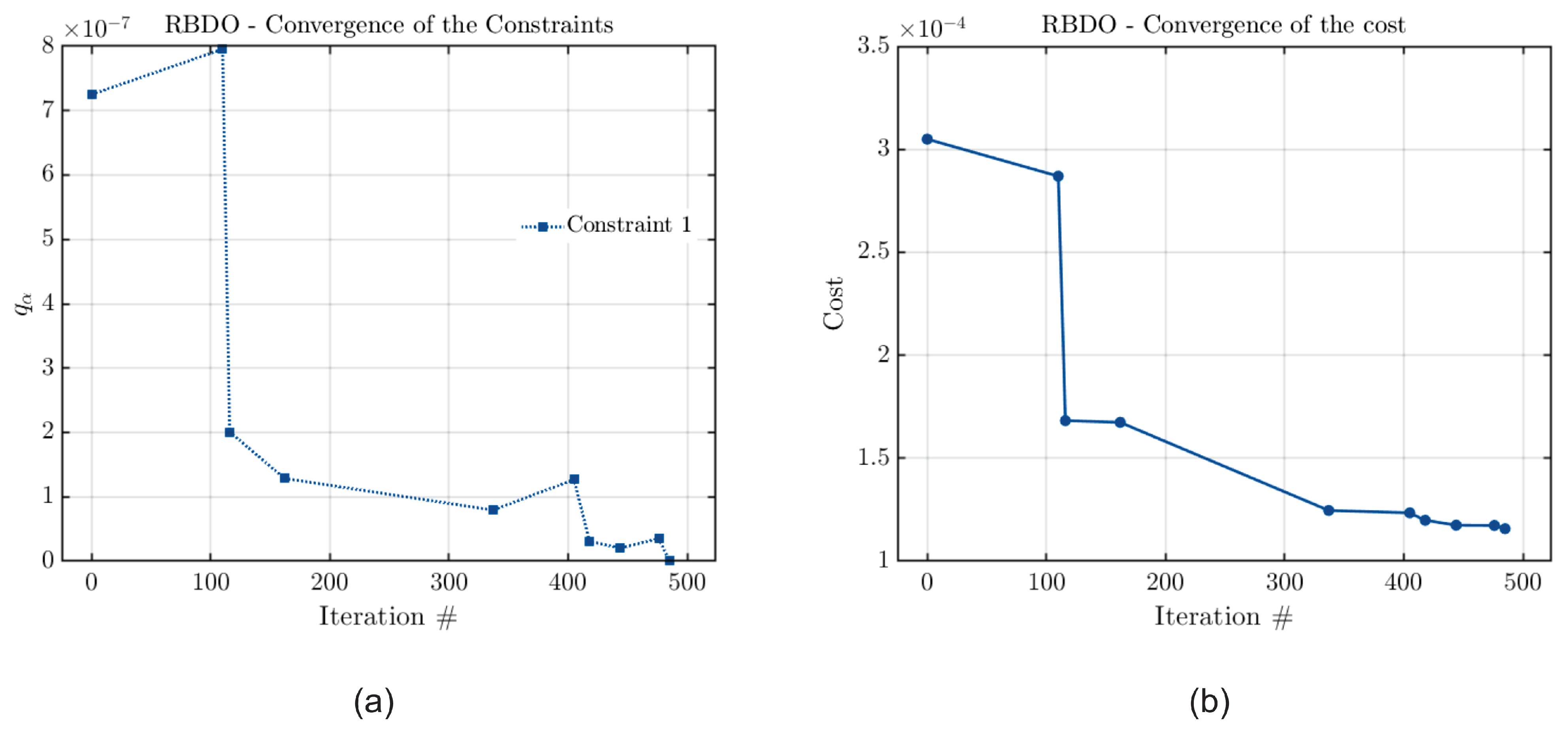
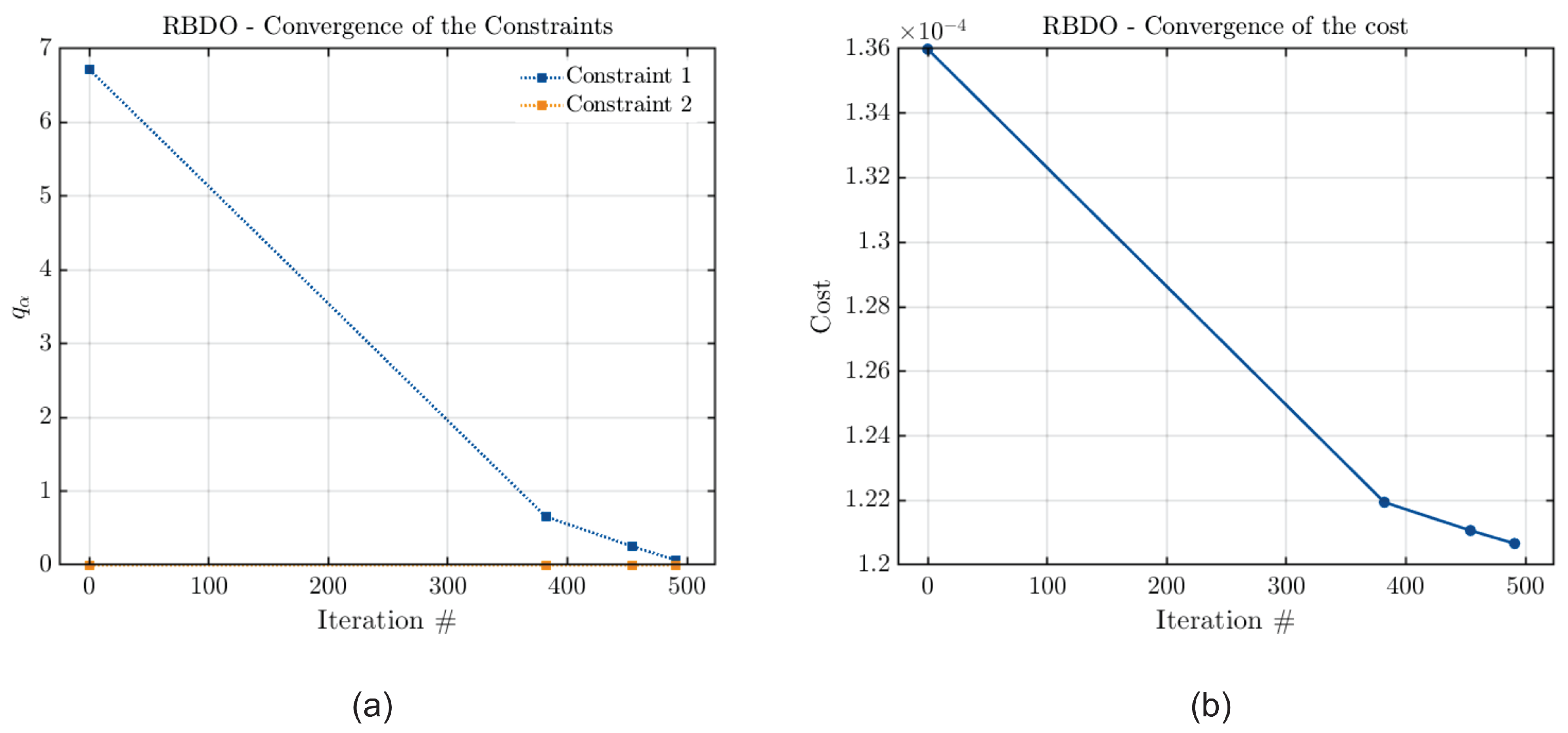
Figure 3.
Convergence curves of (a) the constraint and (b) the cost functions considering PCE, with the rotor shaft diameter optimization and the vibration amplitude limit as a constraint.
Figure 3.
Convergence curves of (a) the constraint and (b) the cost functions considering PCE, with the rotor shaft diameter optimization and the vibration amplitude limit as a constraint.
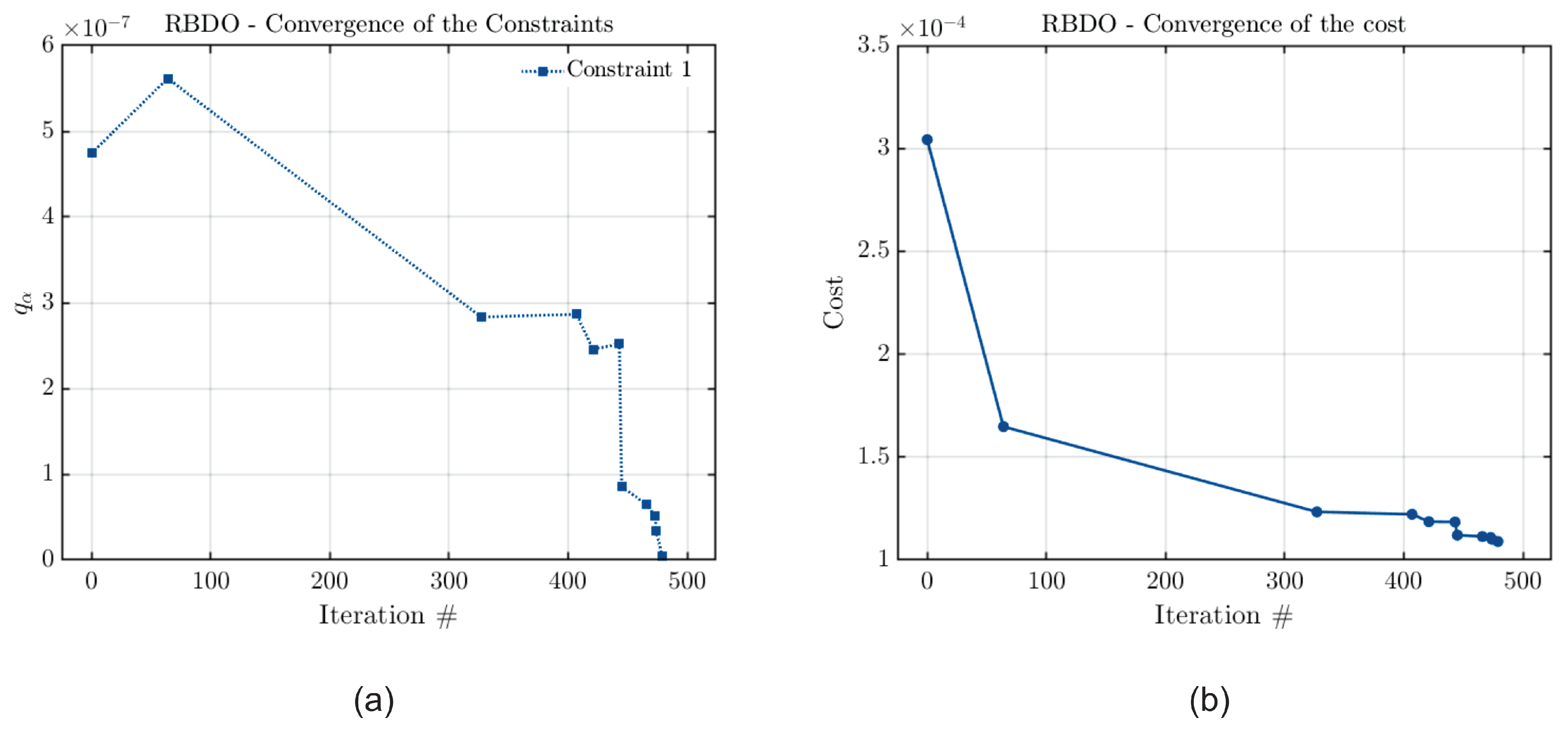
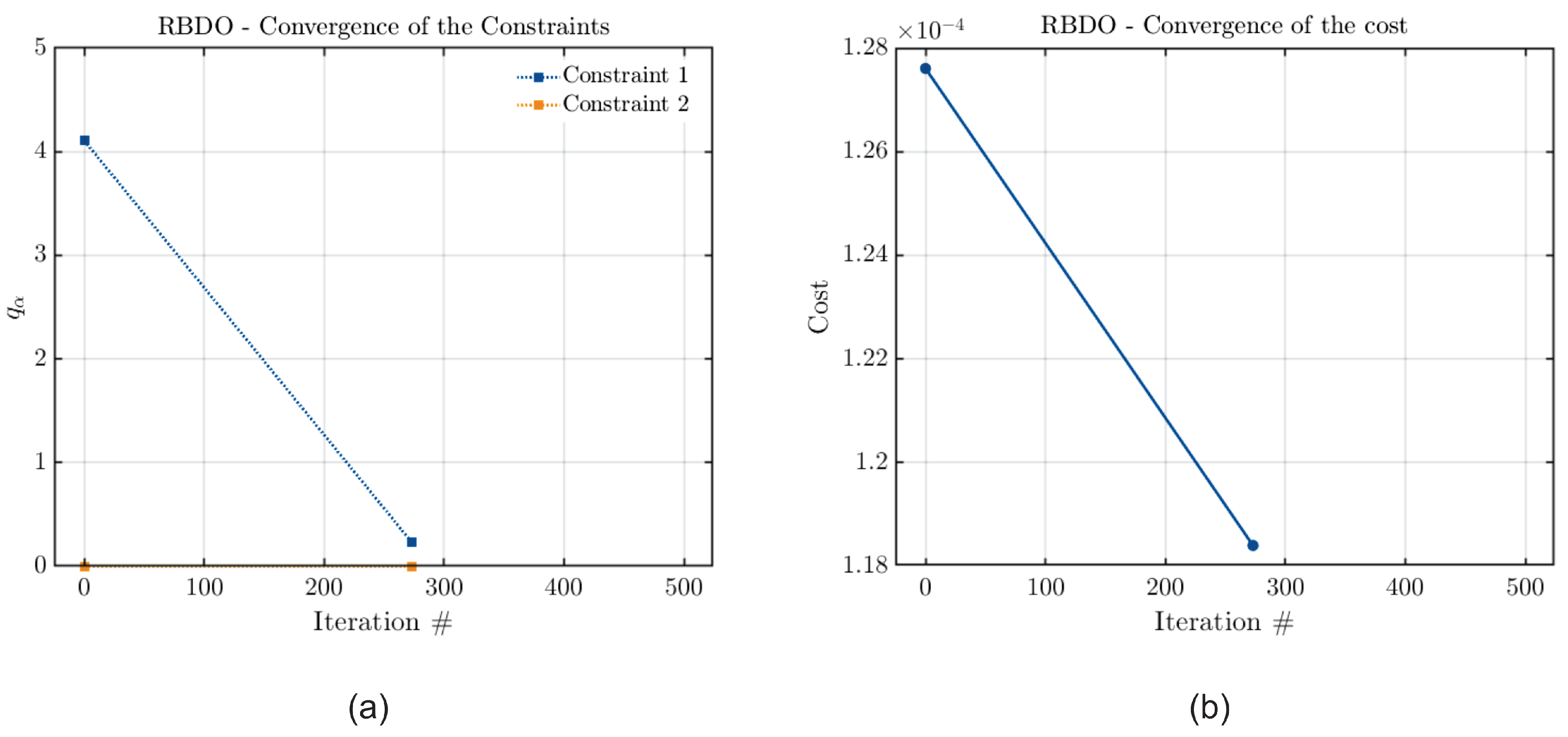
Figure 4.
Convergence curves of (a) the constraint and (b) the cost functions considering PCK, with the rotor shaft diameter optimization and the vibration amplitude limit as a constraint.
Figure 4.
Convergence curves of (a) the constraint and (b) the cost functions considering PCK, with the rotor shaft diameter optimization and the vibration amplitude limit as a constraint.
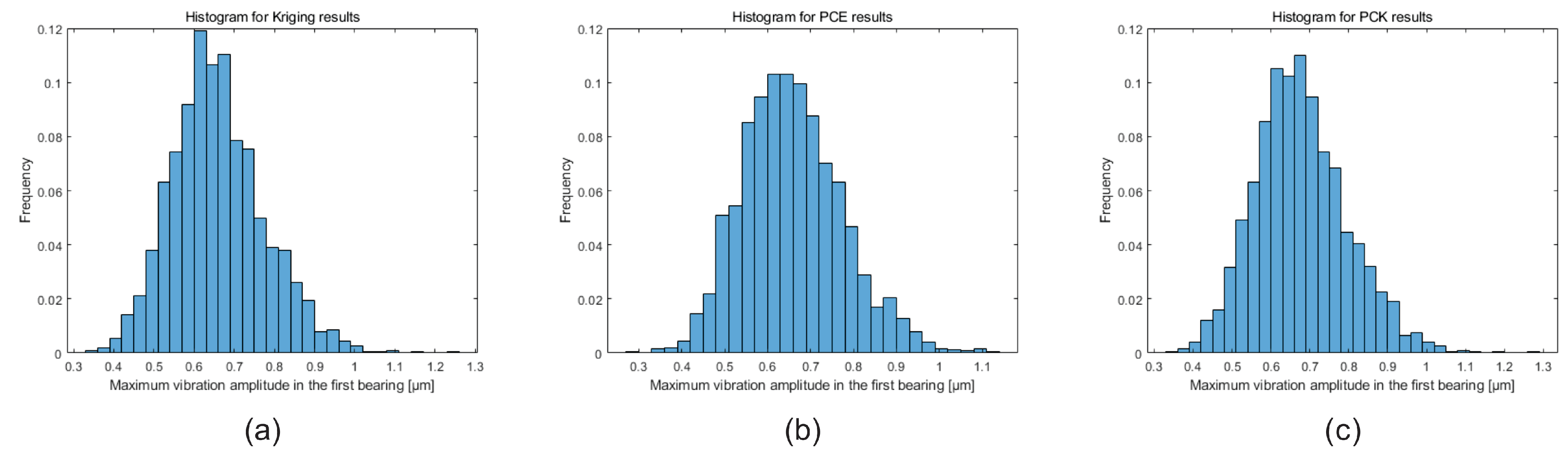
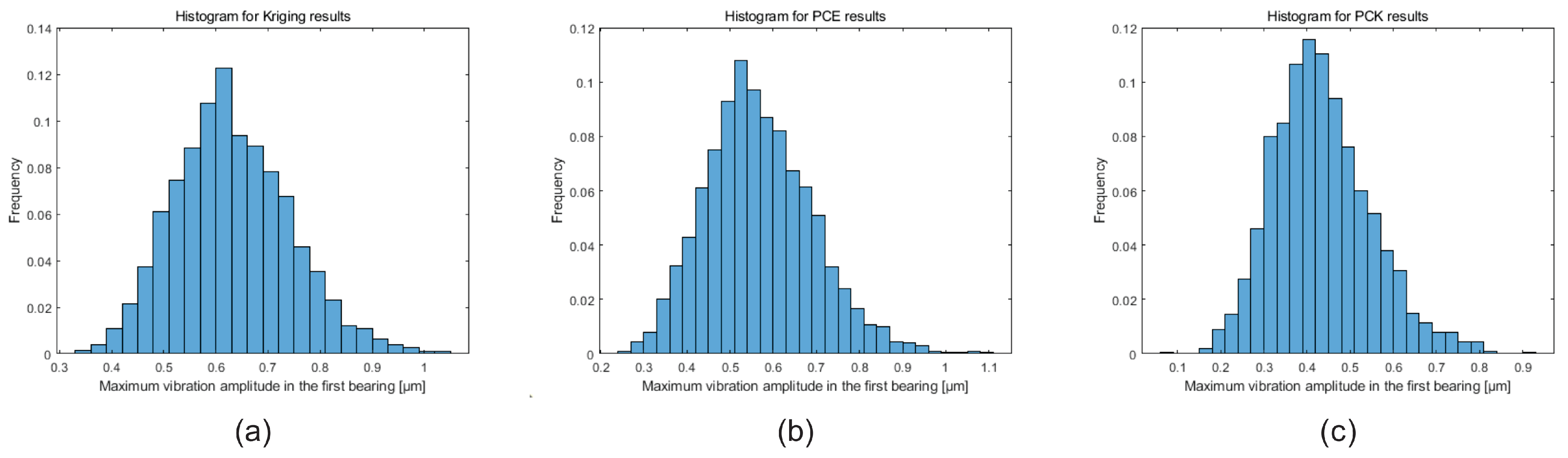
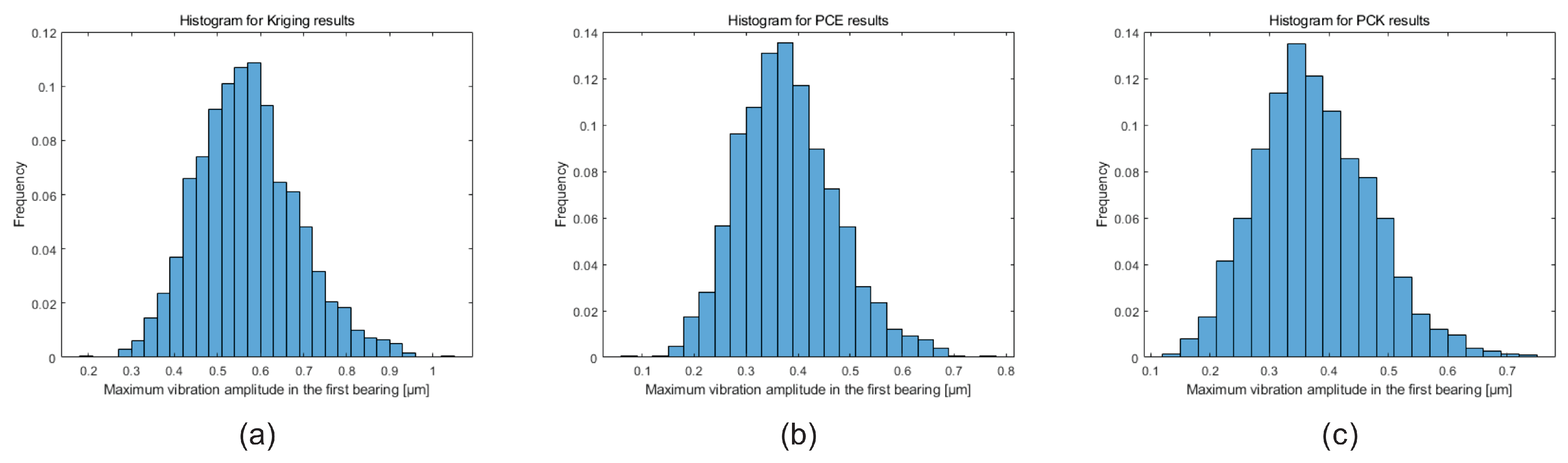
Figure 5.
Histogram of the maximum rotor vibration amplitude considering the rotor shaft diameter optimization and the vibration amplitude limit as a constraint. Results were obtained using (a) Kriging, (b) PCE, and (c) PCK.
Figure 5.
Histogram of the maximum rotor vibration amplitude considering the rotor shaft diameter optimization and the vibration amplitude limit as a constraint. Results were obtained using (a) Kriging, (b) PCE, and (c) PCK.
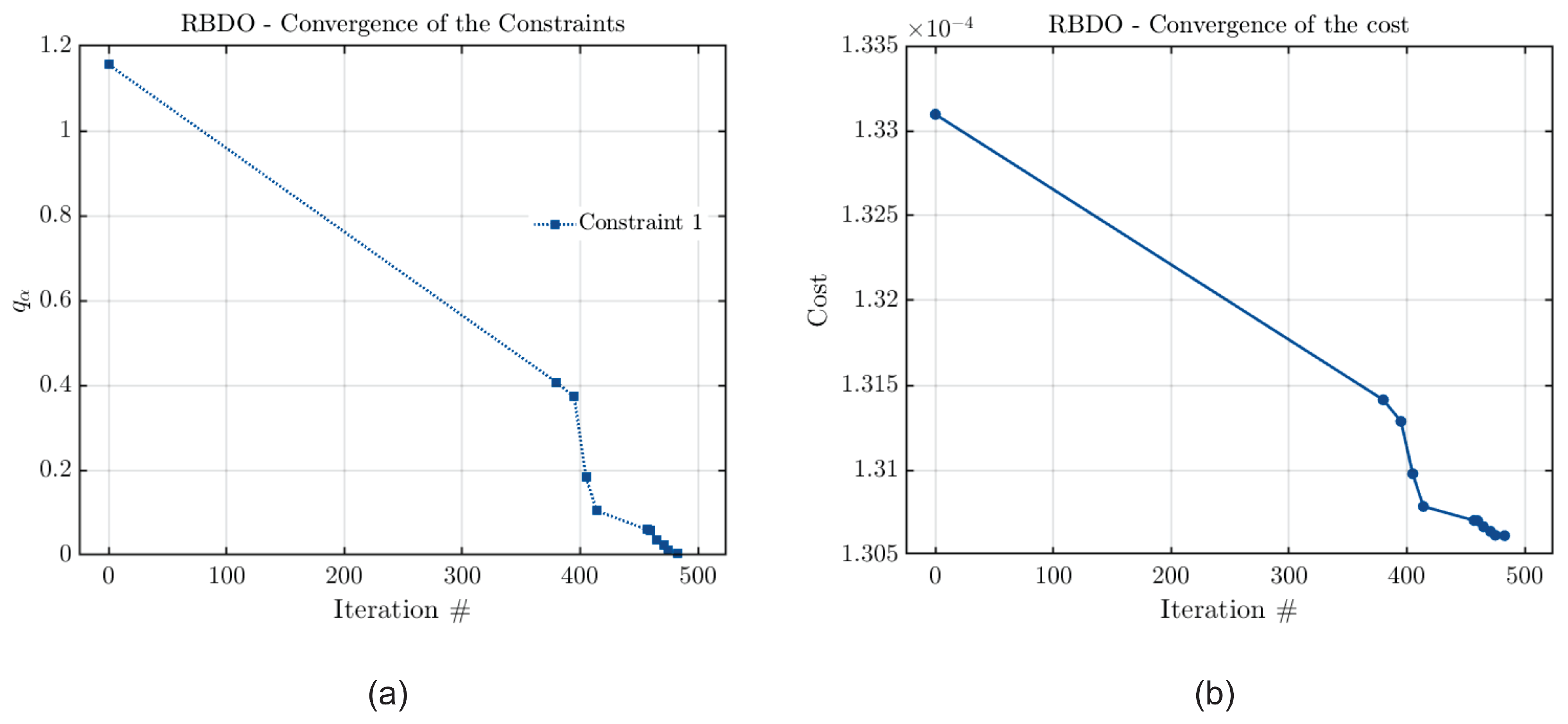
Figure 6.
Convergence curves of (a) the constraint and (b) the cost functions considering Kriging, with the rotor shaft diameter optimization and the rotor’s stability threshold frequency as a constraint.
Figure 6.
Convergence curves of (a) the constraint and (b) the cost functions considering Kriging, with the rotor shaft diameter optimization and the rotor’s stability threshold frequency as a constraint.
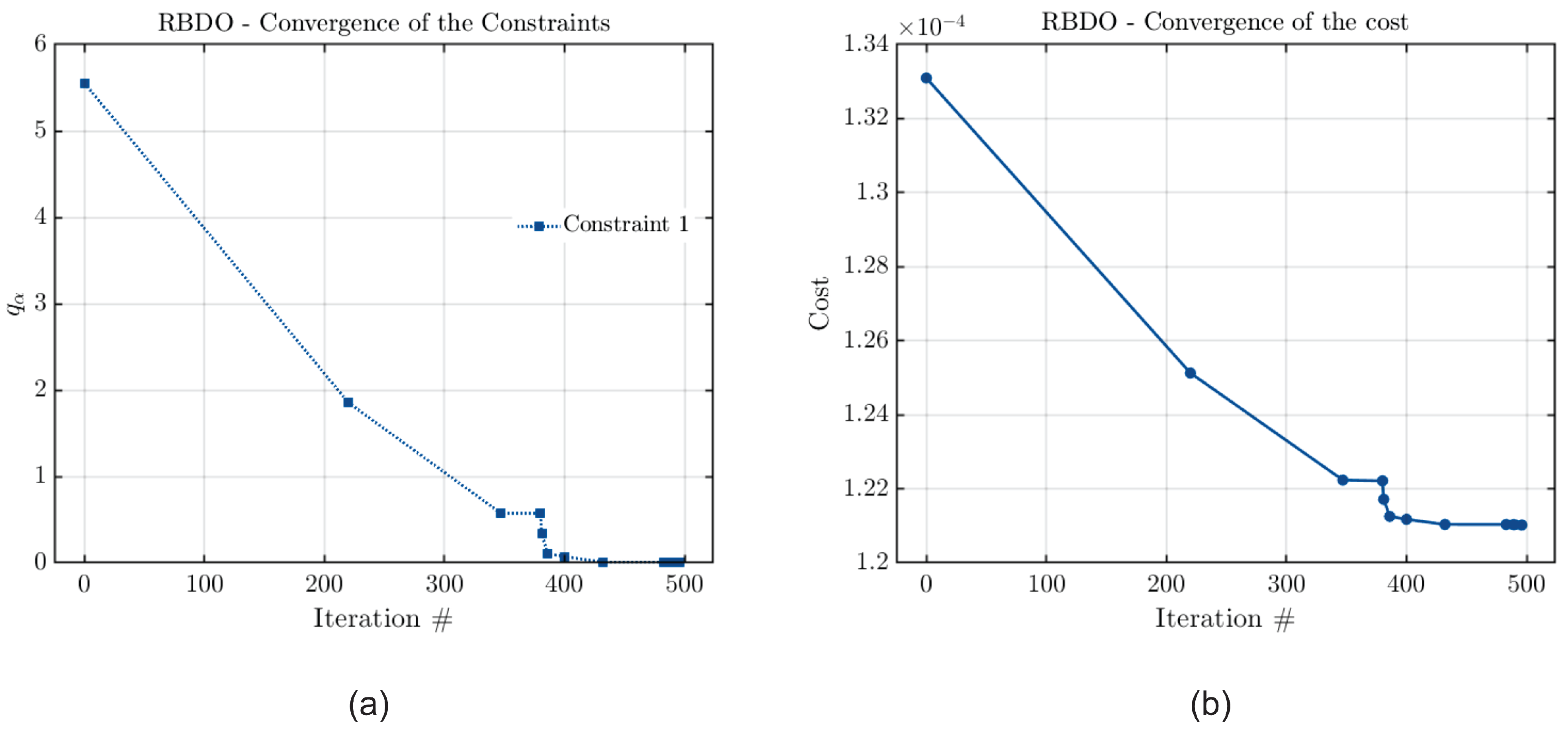
Figure 7.
Convergence curves of (a) the constraint and (b) the cost functions considering PCE, with the rotor shaft diameter optimization and the rotor’s stability threshold frequency as a constraint.
Figure 7.
Convergence curves of (a) the constraint and (b) the cost functions considering PCE, with the rotor shaft diameter optimization and the rotor’s stability threshold frequency as a constraint.
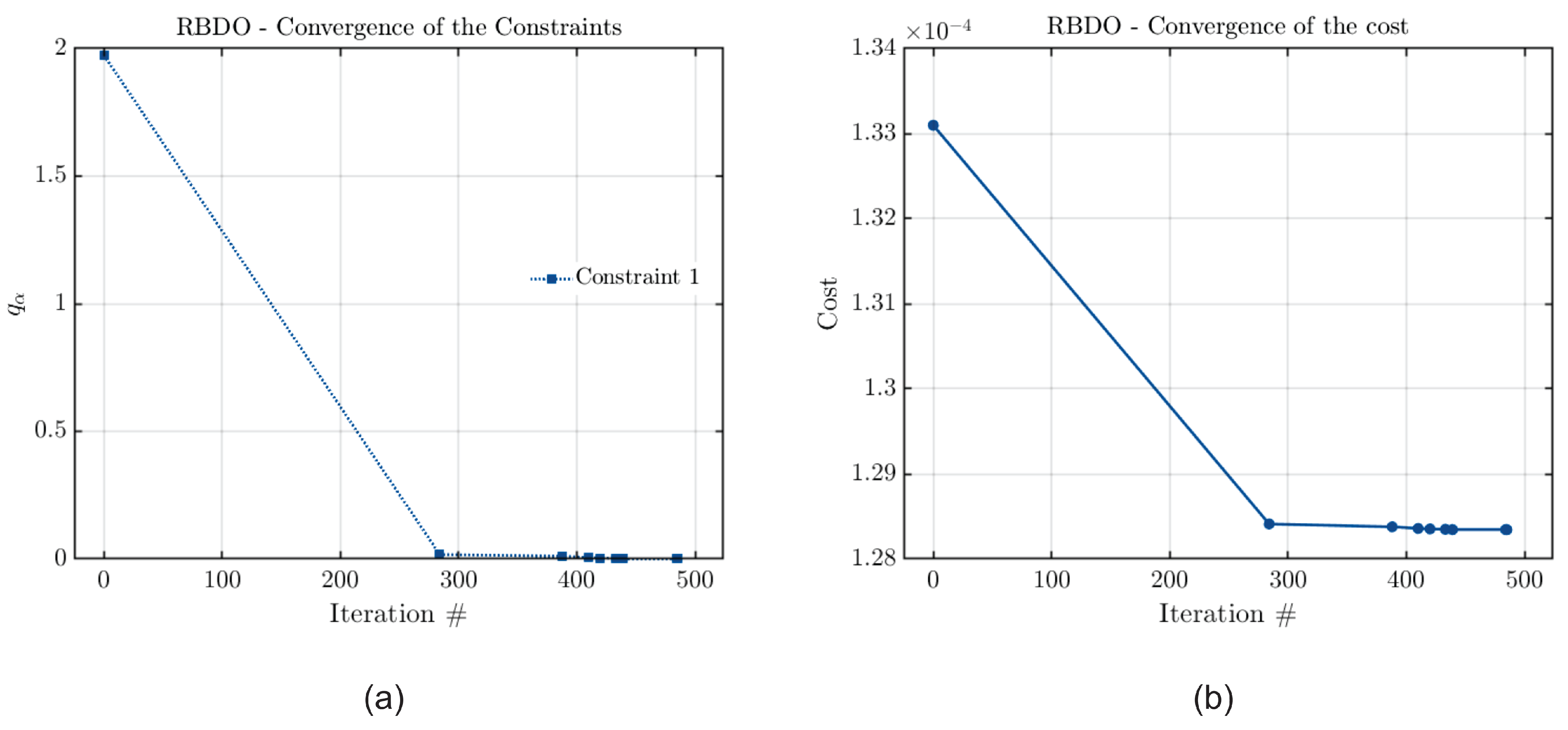
Figure 8.
Convergence curves of (a) the constraint and (b) the cost functions considering PCK, with the rotor shaft diameter optimization and the rotor’s stability threshold frequency as a constraint.
Figure 8.
Convergence curves of (a) the constraint and (b) the cost functions considering PCK, with the rotor shaft diameter optimization and the rotor’s stability threshold frequency as a constraint.
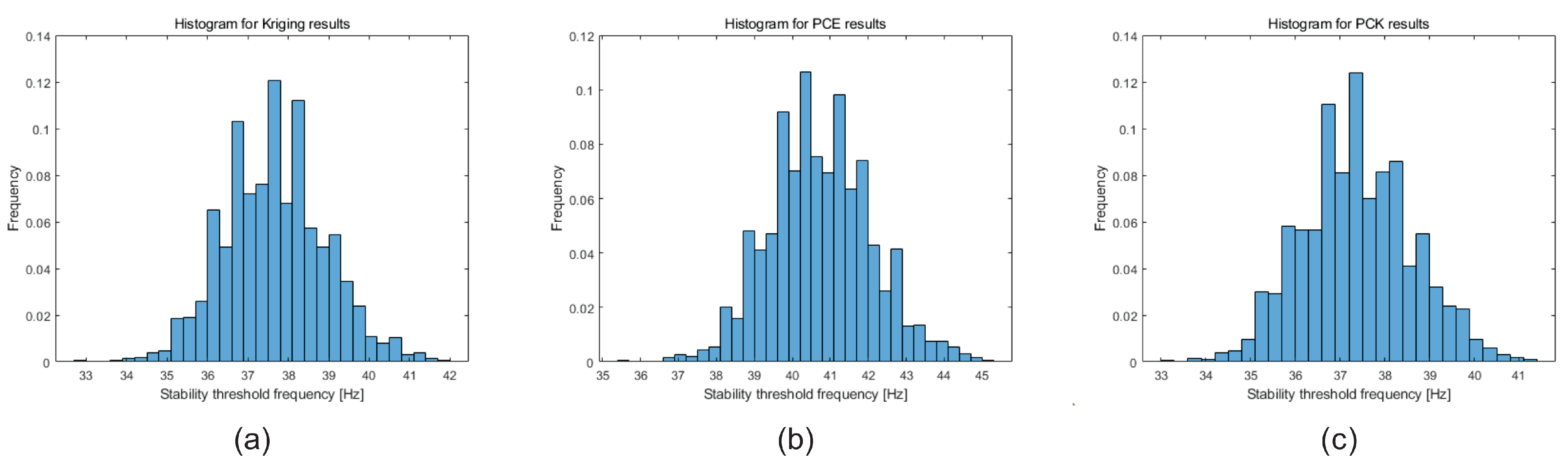
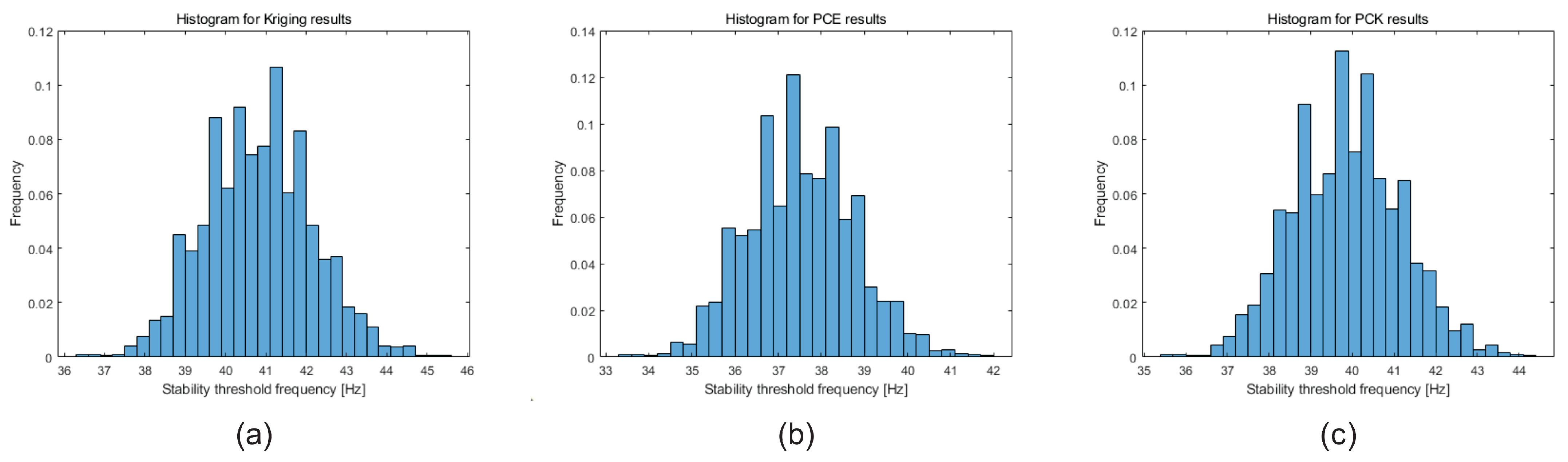
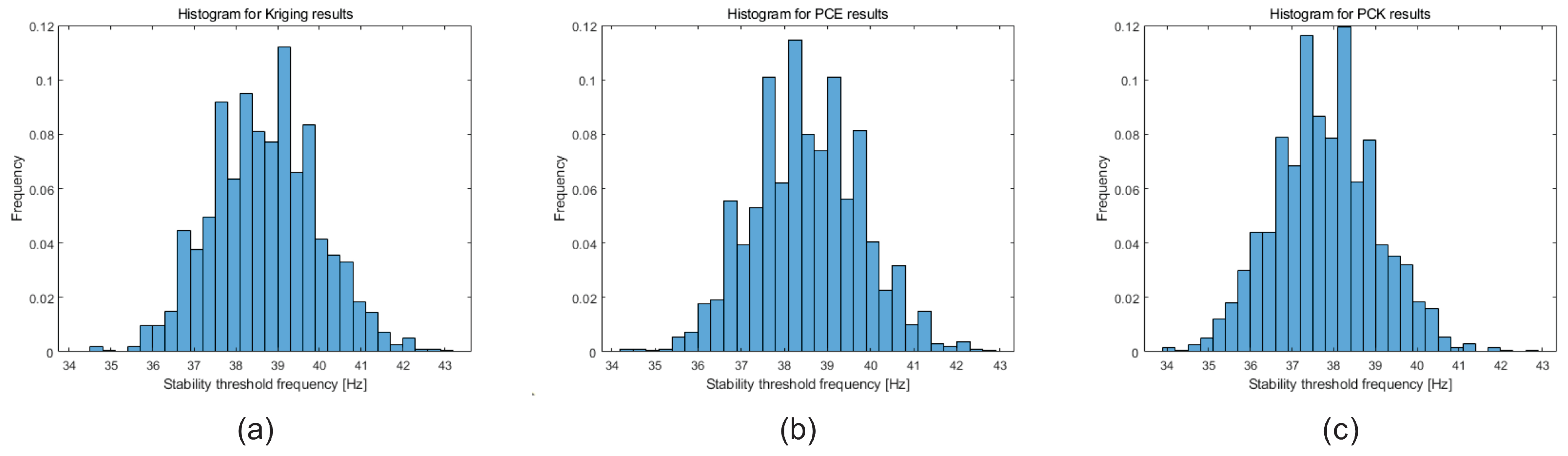
Figure 9.
Histogram of the stability threshold frequency considering the rotor shaft diameter optimization and the stability threshold frequency as a constraint. Results were obtained using (a) Kriging, (b) PCE, and (c) PCK.
Figure 9.
Histogram of the stability threshold frequency considering the rotor shaft diameter optimization and the stability threshold frequency as a constraint. Results were obtained using (a) Kriging, (b) PCE, and (c) PCK.
Figure 10.
Convergence curves of (a) the constraint and (b) the cost functions considering Kriging, with the rotor shaft diameter optimization and both parameters as constraints.
Figure 10.
Convergence curves of (a) the constraint and (b) the cost functions considering Kriging, with the rotor shaft diameter optimization and both parameters as constraints.
Figure 11.
Convergence curves of (a) the constraint and (b) the cost functions considering PCE, with the rotor shaft diameter optimization and both parameters as constraints.
Figure 11.
Convergence curves of (a) the constraint and (b) the cost functions considering PCE, with the rotor shaft diameter optimization and both parameters as constraints.
Figure 12.
Convergence curves of (a) the constraint and (b) the cost functions considering PCK, with the rotor shaft diameter optimization and both parameters as constraints.
Figure 12.
Convergence curves of (a) the constraint and (b) the cost functions considering PCK, with the rotor shaft diameter optimization and both parameters as constraints.
Figure 13.
Histogram of the maximum rotor vibration amplitude considering the rotor shaft diameter optimization and both parameters as constraints. Results were obtained using (a) Kriging, (b) PCE, and (c) PCK.
Figure 13.
Histogram of the maximum rotor vibration amplitude considering the rotor shaft diameter optimization and both parameters as constraints. Results were obtained using (a) Kriging, (b) PCE, and (c) PCK.
Figure 14.
Histogram of the stability threshold frequency considering the rotor shaft diameter optimization and both parameters as constraints. Results were obtained using (a) Kriging, (b) PCE, and (c) PCK.
Figure 14.
Histogram of the stability threshold frequency considering the rotor shaft diameter optimization and both parameters as constraints. Results were obtained using (a) Kriging, (b) PCE, and (c) PCK.
Figure 15.
Convergence curves of (a) the constraint and (b) the cost functions considering Kriging, with the joint optimization and the vibration amplitude limit as a constraint.
Figure 15.
Convergence curves of (a) the constraint and (b) the cost functions considering Kriging, with the joint optimization and the vibration amplitude limit as a constraint.
Figure 16.
Convergence curves of (a) the constraint and (b) the cost functions considering PCE, with the joint optimization and the vibration amplitude limit as a constraint.
Figure 16.
Convergence curves of (a) the constraint and (b) the cost functions considering PCE, with the joint optimization and the vibration amplitude limit as a constraint.
Figure 17.
Convergence curves of (a) the constraint and (b) the cost functions considering PCK, with the joint optimization and the vibration amplitude limit as a constraint.
Figure 17.
Convergence curves of (a) the constraint and (b) the cost functions considering PCK, with the joint optimization and the vibration amplitude limit as a constraint.
Figure 18.
Histogram of the maximum rotor vibration amplitude considering the joint optimization and the vibration amplitude limit as a constraint. Results were obtained using (a) Kriging, (b) PCE, and (c) PCK.
Figure 18.
Histogram of the maximum rotor vibration amplitude considering the joint optimization and the vibration amplitude limit as a constraint. Results were obtained using (a) Kriging, (b) PCE, and (c) PCK.
Figure 19.
Convergence curves of (a) the constraint and (b) the cost functions considering Kriging, with the joint optimization and the rotor’s stability threshold frequency as a constraint.
Figure 19.
Convergence curves of (a) the constraint and (b) the cost functions considering Kriging, with the joint optimization and the rotor’s stability threshold frequency as a constraint.
Figure 20.
Convergence curves of (a) the constraint and (b) the cost functions considering PCE, with the joint optimization and the rotor’s stability threshold frequency as a constraint.
Figure 20.
Convergence curves of (a) the constraint and (b) the cost functions considering PCE, with the joint optimization and the rotor’s stability threshold frequency as a constraint.
Figure 21.
Convergence curves of (a) the constraint and (b) the cost functions considering PCK, with the joint optimization and the rotor’s stability threshold frequency as a constraint.
Figure 21.
Convergence curves of (a) the constraint and (b) the cost functions considering PCK, with the joint optimization and the rotor’s stability threshold frequency as a constraint.
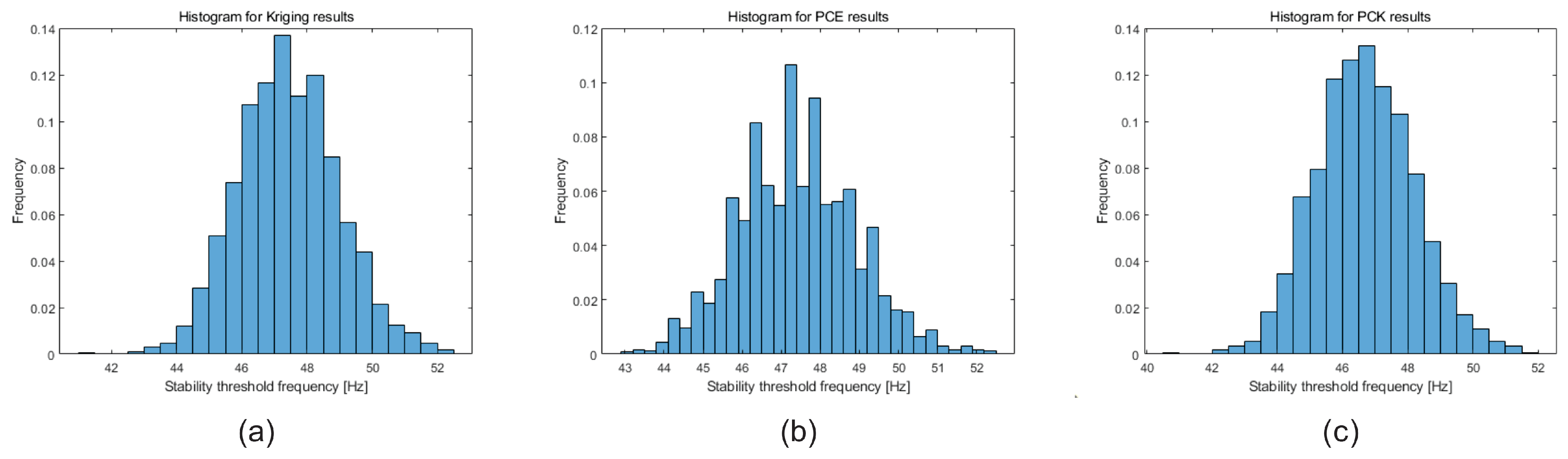
Figure 22.
Histogram of the stability threshold frequency considering the joint optimization and the stability threshold frequency as a constraint. Results were obtained using (a) Kriging, (b) PCE, and (c) PCK.
Figure 22.
Histogram of the stability threshold frequency considering the joint optimization and the stability threshold frequency as a constraint. Results were obtained using (a) Kriging, (b) PCE, and (c) PCK.
Figure 23.
Convergence curves of (a) the constraint and (b) the cost functions considering Kriging, with the joint optimization and both parameters as constraints.
Figure 23.
Convergence curves of (a) the constraint and (b) the cost functions considering Kriging, with the joint optimization and both parameters as constraints.
Figure 24.
Convergence curves of (a) the constraint and (b) the cost functions considering PCE, with the joint optimization and both parameters as constraints.
Figure 24.
Convergence curves of (a) the constraint and (b) the cost functions considering PCE, with the joint optimization and both parameters as constraints.
Figure 25.
Convergence curves of (a) the constraint and (b) the cost functions considering PCK, with the joint optimization and both parameters as constraints.
Figure 25.
Convergence curves of (a) the constraint and (b) the cost functions considering PCK, with the joint optimization and both parameters as constraints.
Figure 26.
Histogram of the maximum rotor vibration amplitude considering the joint optimization and both parameters as constraints. Results were obtained using (a) Kriging, (b) PCE, and (c) PCK.
Figure 26.
Histogram of the maximum rotor vibration amplitude considering the joint optimization and both parameters as constraints. Results were obtained using (a) Kriging, (b) PCE, and (c) PCK.
Figure 27.
Histogram of the stability threshold frequency considering the joint optimization and both parameters as constraints. Results were obtained using (a) Kriging, (b) PCE, and (c) PCK.
Figure 27.
Histogram of the stability threshold frequency considering the joint optimization and both parameters as constraints. Results were obtained using (a) Kriging, (b) PCE, and (c) PCK.
Table 1.
Deterministic input data for the rotor model.
Table 1.
Deterministic input data for the rotor model.
| Parameter | Value |
|---|
| Number of nodes | 11 |
| Location node of the disc | 6 |
| Location nodes of the bearings | 3 and 9 |
| Shaft length, mm | 820 |
| Shaft diameter inside bearings, mm | 30 |
| Disc element length, mm | 47 |
| External disc diameter, mm | 90 |
| Disc mass, kg | 2.3188 |
| Disc diametral moment of inertia, kg·m2 | 0.0016 |
| Disc polar moment of inertia, kg·m2 | 0.0024 |
| Steel shear modulus (shaft and disc material), GPa | 79.6 |
| Gravity, m/s2 | 9.81 |
| Proportional damping coefficient of the shaft | |
Table 2.
Distribution of stochastic design parameters for the rotor model considering its shaft diameter optimization.
Table 2.
Distribution of stochastic design parameters for the rotor model considering its shaft diameter optimization.
| Parameter | Distribution | Mean Value | Standard Deviation |
|---|
| Elastic modulus of the rotor material E, GPa | gamma | 210 | 10.5 |
| Density of the rotor material , kg/m3 | gamma | 7860 | 393 |
| Lubricating fluid temperature T, °C | gamma | 25 | 1.25 |
| Bearing radial clearance , m | gamma | 90 | 4.5 |
Table 3.
Distribution of stochastic design parameters for the rotor model considering the joint optimization.
Table 3.
Distribution of stochastic design parameters for the rotor model considering the joint optimization.
| Parameter | Distribution | Mean Value | Standard Deviation |
|---|
| Elastic modulus of the rotor material E, GPa | gamma | 210 | 10.5 |
| Density of the rotor material , kg/m3 | gamma | 7860 | 393 |
| Bearing radial clearance , m | gamma | 90 | 4.5 |
Table 4.
RBDO results for the rotor model considering the rotor shaft diameter optimization and the vibration amplitude limit as a constraint.
Table 4.
RBDO results for the rotor model considering the rotor shaft diameter optimization and the vibration amplitude limit as a constraint.
| Metamodel | Diameter, mm | Evaluations | Stopping Criterion |
|---|
| Kriging | 12.945 | 2,720,000 | Value of global step size below tolerance |
| PCE | 12.644 | 2,960,000 | Value of global step size below tolerance |
| PCK | 12.763 | 2,540,000 | Value of global step size below tolerance |
Table 5.
RBDO results for the rotor model considering the rotor shaft diameter optimization and the rotor’s stability threshold frequency as a constraint.
Table 5.
RBDO results for the rotor model considering the rotor shaft diameter optimization and the rotor’s stability threshold frequency as a constraint.
| Metamodel | Diameter, mm | Evaluations | Stopping Criterion |
|---|
| Kriging | 11.347 | 5,000,000 | Maximum number of evaluations reached |
| PCE | 11.858 | 2,160,000 | Value of global step size below tolerance |
| PCK | 11.310 | 2,480,000 | Value of global step size below tolerance |
Table 6.
RBDO results for the rotor model considering the rotor shaft diameter optimization and both parameters as constraints.
Table 6.
RBDO results for the rotor model considering the rotor shaft diameter optimization and both parameters as constraints.
| Metamodel | Diameter, mm | Evaluations | Stopping Criterion |
|---|
| Kriging | 12.918 | 2,700,000 | Value of global step size below tolerance |
| PCE | 12.940 | 2,860,000 | Value of global step size below tolerance |
| PCK | 12.805 | 2,260,000 | Value of global step size below tolerance |
Table 7.
RBDO results for the rotor model considering the joint optimization and the vibration amplitude limit as a constraint.
Table 7.
RBDO results for the rotor model considering the joint optimization and the vibration amplitude limit as a constraint.
| Metamodel | Diameter, mm | Temperature, °C | Evaluations | Stopping Criterion |
|---|
| Kriging | 12.824 | 24.058 | 5,080,000 | Maximum number of generations reached |
| PCE | 11.093 | 19.123 | 5,020,000 | Maximum number of generations reached |
| PCK | 10.838 | 16.682 | 5,040,000 | Maximum number of generations reached |
Table 8.
RBDO results for the rotor model considering the joint optimization and the rotor’s stability threshold frequency as a constraint.
Table 8.
RBDO results for the rotor model considering the joint optimization and the rotor’s stability threshold frequency as a constraint.
| Metamodel | Diameter, mm | Temperature, °C | Evaluations | Stopping Criterion |
|---|
| Kriging | 11.885 | 19.680 | 5,000,000 | Maximum number of generations |
| PCE | 11.341 | 20.011 | 5,000,000 | Maximum number of generations |
| PCK | 11.728 | 20.323 | 5,000,000 | Maximum number of generations reached |
Table 9.
RBDO results for the rotor model considering the joint optimization and both parameters as constraints.
Table 9.
RBDO results for the rotor model considering the joint optimization and both parameters as constraints.
| Metamodel | Diameter, mm | Temperature, °C | Evaluations | Stopping Criterion |
|---|
| Kriging | 11.532 | 19.966 | 5,000,000 | Maximum number of generations reached |
| PCE | 11.503 | 16.733 | 5,060,000 | Maximum number of generations reached |
| PCK | 11.391 | 16.494 | 5,840,000 | Maximum number of generations reached |