Research on Running Performance Optimization of Four-Wheel-Driving Ackerman Chassis by the Combining Method of Quantitative Experiment with Dynamic Simulation
Abstract
1. Introduction
- (1)
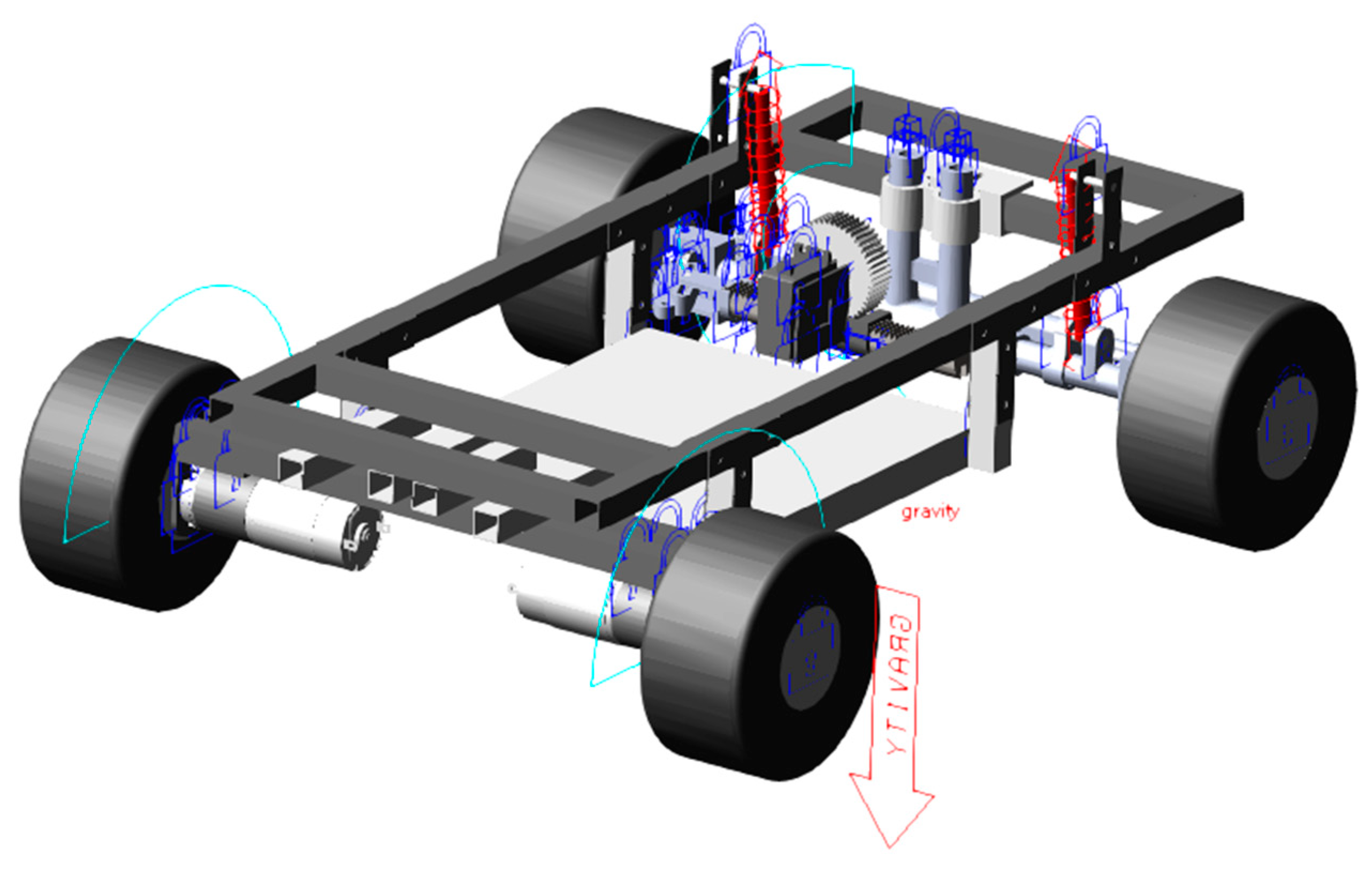
- The four-wheel-driving Ackerman carrier chassis composed of an Ackerman steering mechanism and a strong suspension and shock absorption structure is designed to achieve more stable steering and convenient control of the chassis.
- (2)
- The chassis multi-source and multi-point dynamic information testing system based on chassis performance indicators and structural characteristics is proposed and constructed, providing reliable monitoring data for the comprehensive performance evaluation of the chassis.
- (3)
- The verifying and optimizing research method for the rationality of chassis design by combining quantitative experiments with dynamic simulation is proposed.
- (4)
- The slip and offset characteristics of the initial designed chassis are analyzed, and the influence law of road conditions and shock absorption springs on the operating characteristics of the chassis is obtained.
- (5)
- The influence law of chassis structure and performance parameters such as chassis wheelbase, guide rod structure and parameters, wheel friction coefficient, and assembly error on the dynamic characteristics of the chassis is obtained, and the optimal chassis structure size and parameters are determined.
2. Design of Four-Wheel-Driving Ackerman Carrier Chassis
2.1. Design Principle and Parameter Analysis of Ackerman Carrier Chassis
2.2. Kinematic Model Construction of the Ackerman Carrier Chassis
3. Establishment of the Test System for the Four-Wheel-Driving Ackerman Carrier Chassis
3.1. Analysis of the Important Indicators
3.2. Construction of the Test System Based on Structural Characteristics and Indicator Analysis
4. Research on the Performance of Four-Wheel-Driving Ackerman Chassis Based on Quantitative Experiments
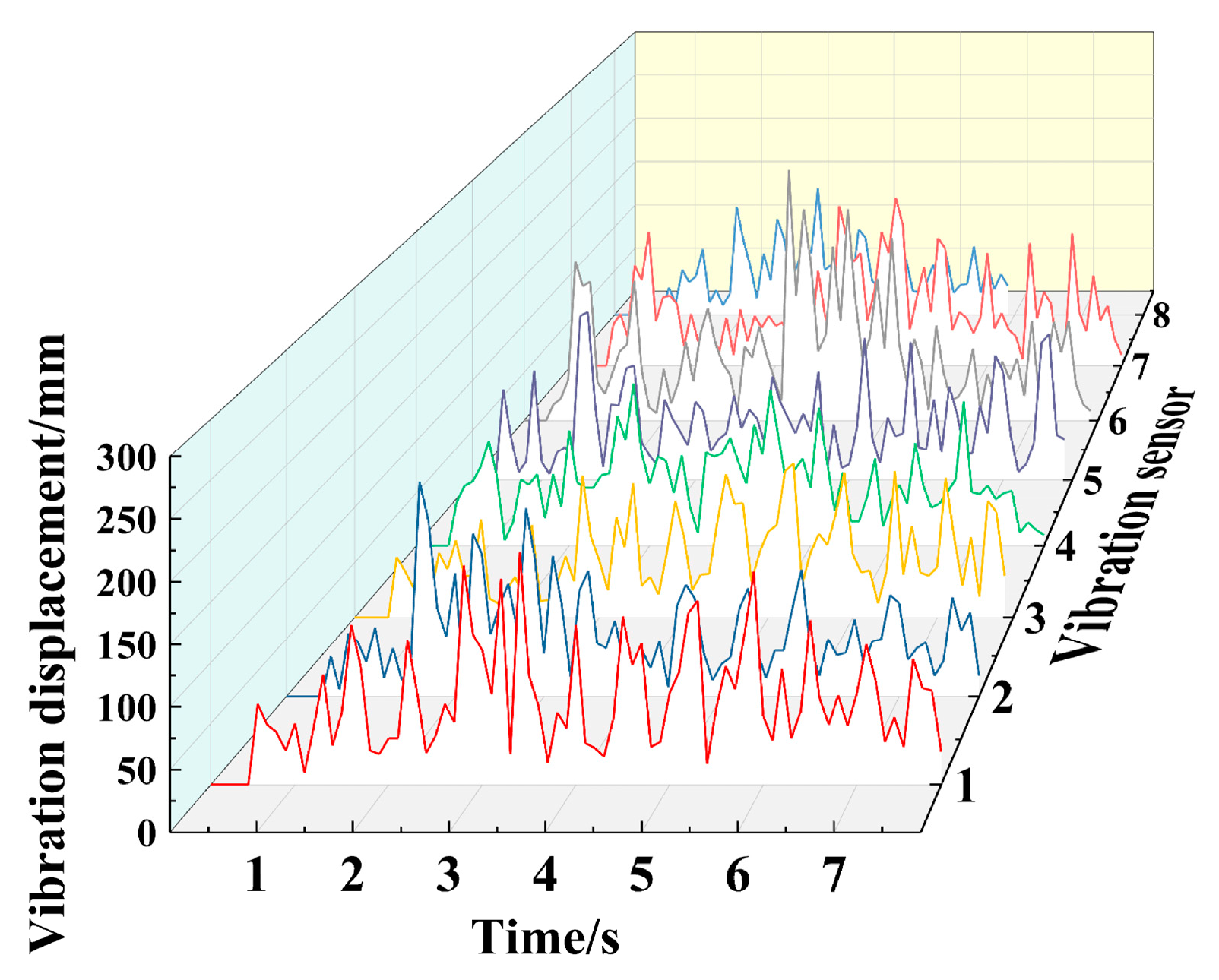
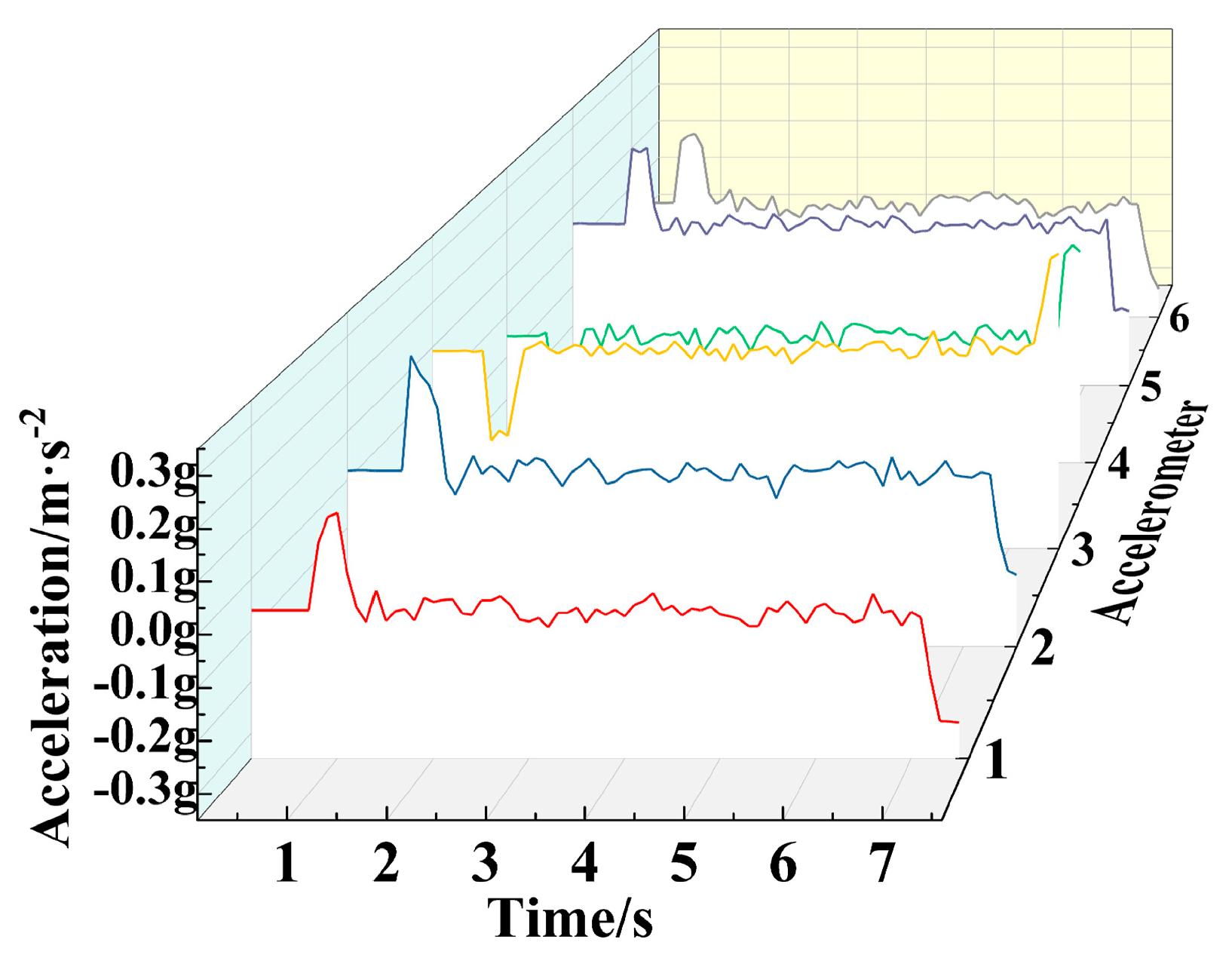
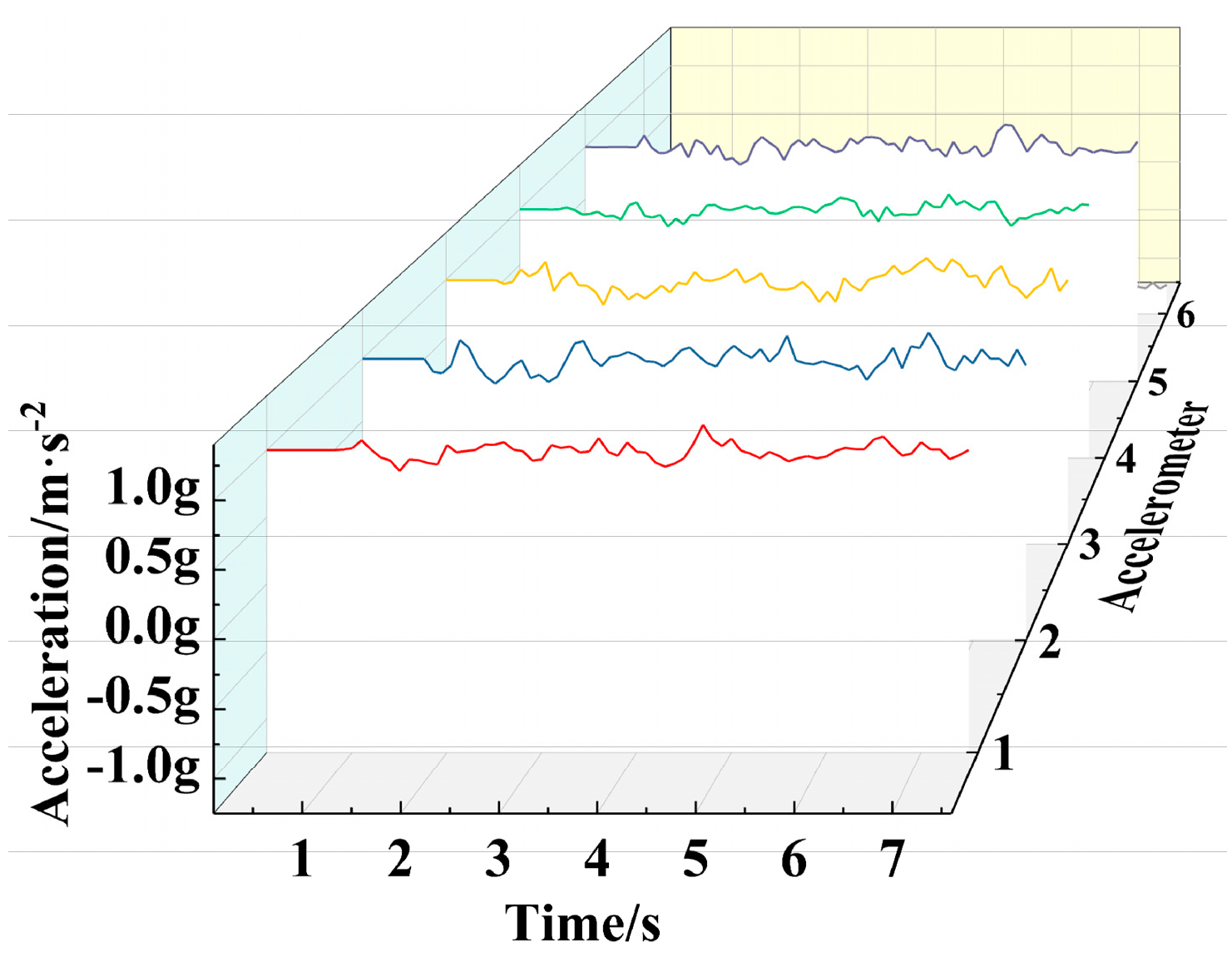
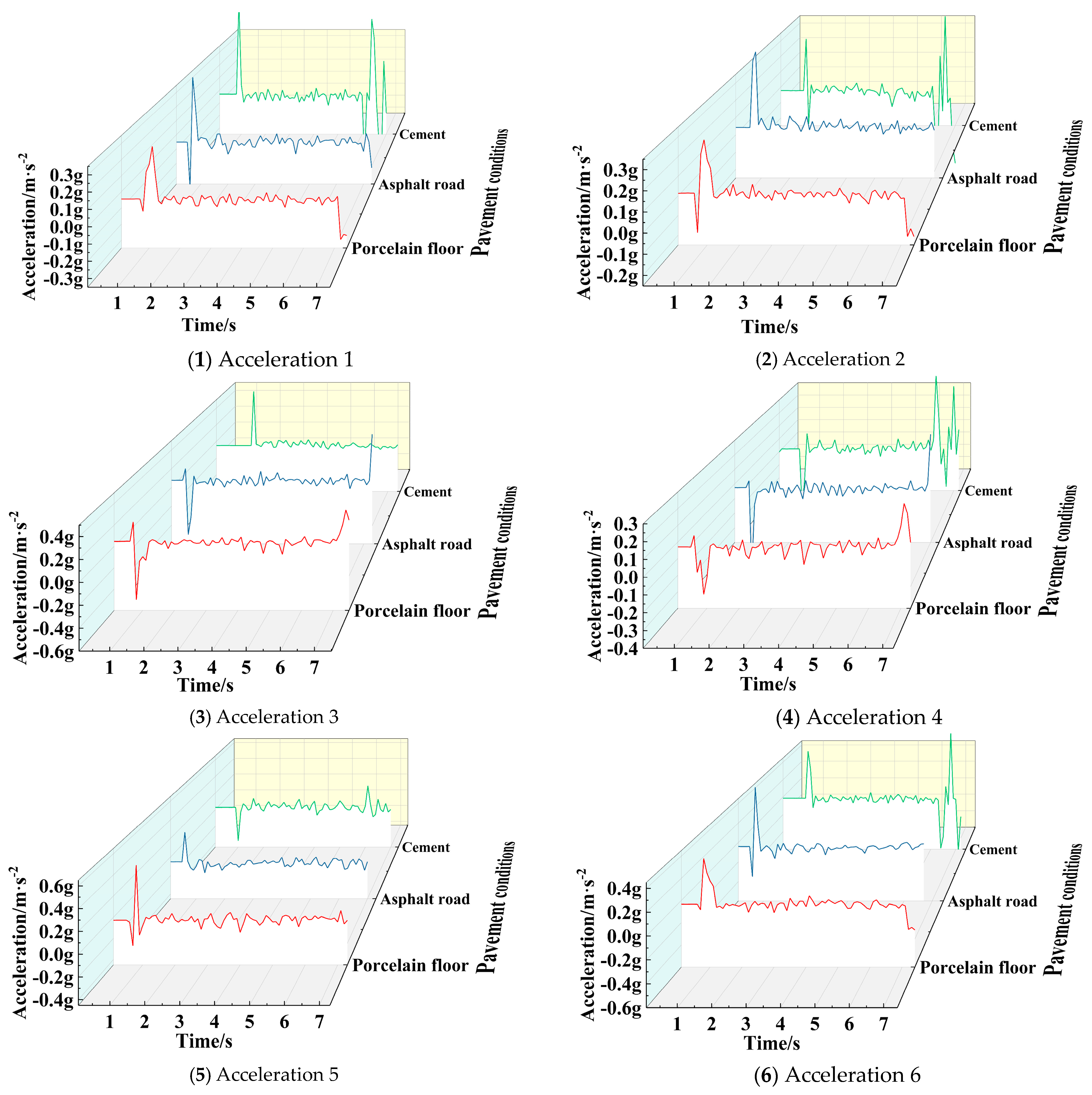
4.1. Analysis of Vibration and Acceleration Characteristics at Different Positions of the Chassis
4.2. Analysis of Chassis Slip Amount
4.2.1. Experimental Design of Chassis Slip Amount
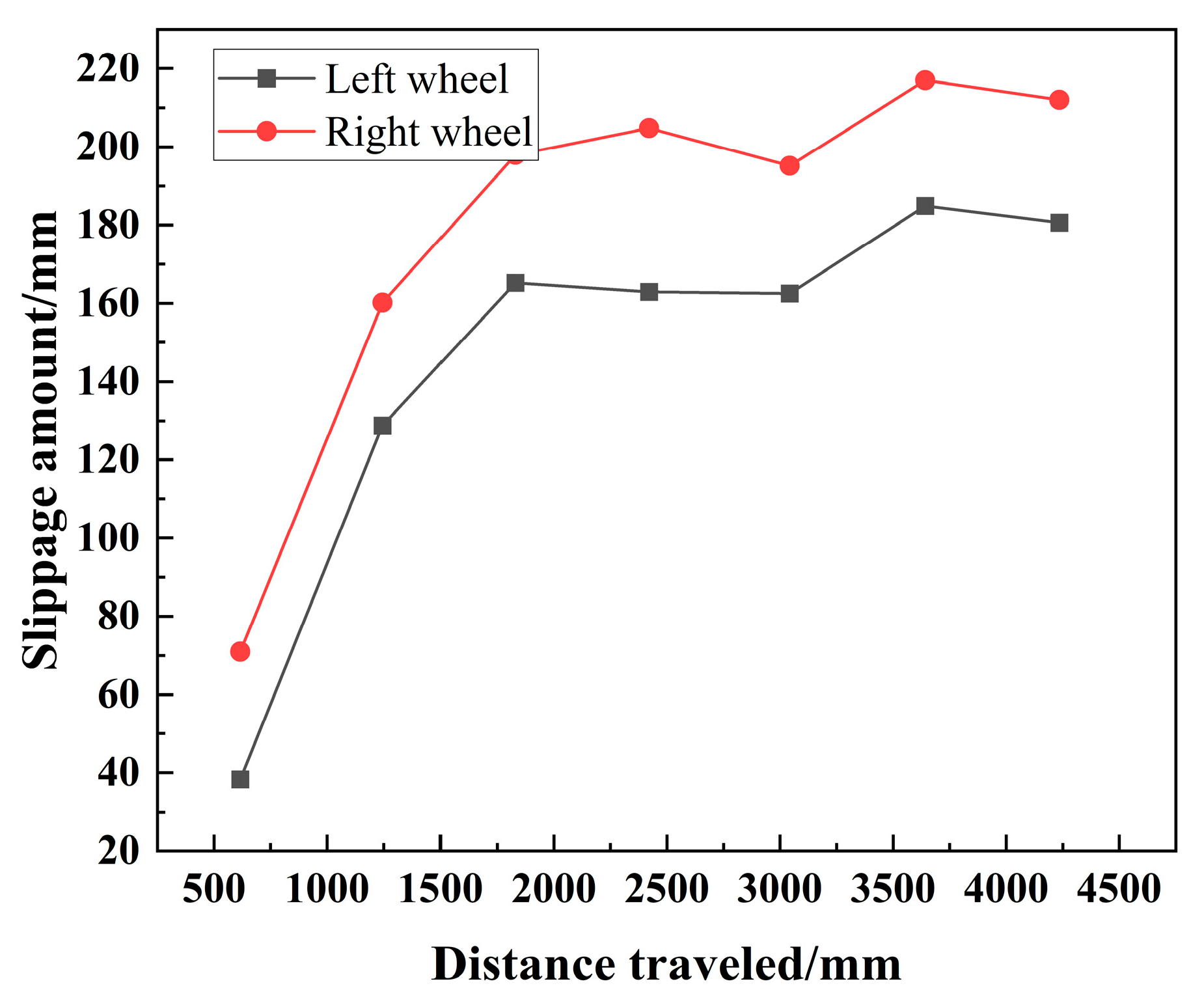
4.2.2. Chassis Slip Characteristics
4.3. Analysis of Chassis Straightness
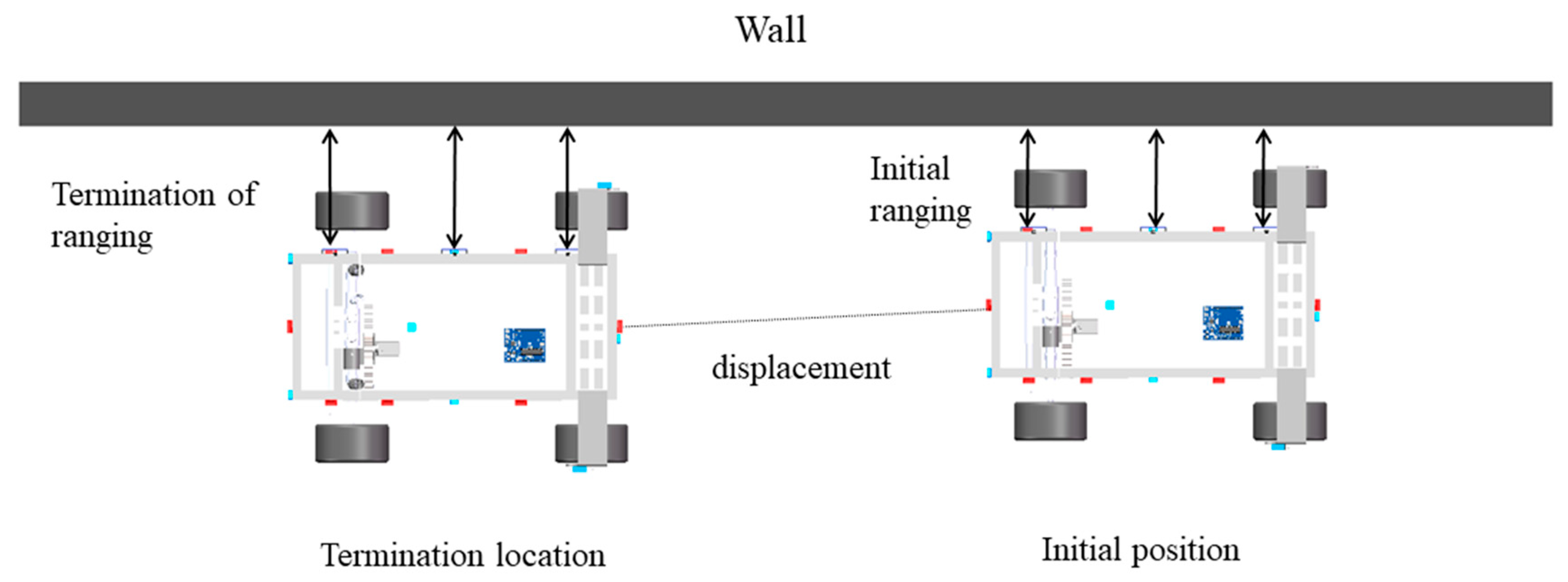
4.3.1. Experimental Design of Chassis Straightness
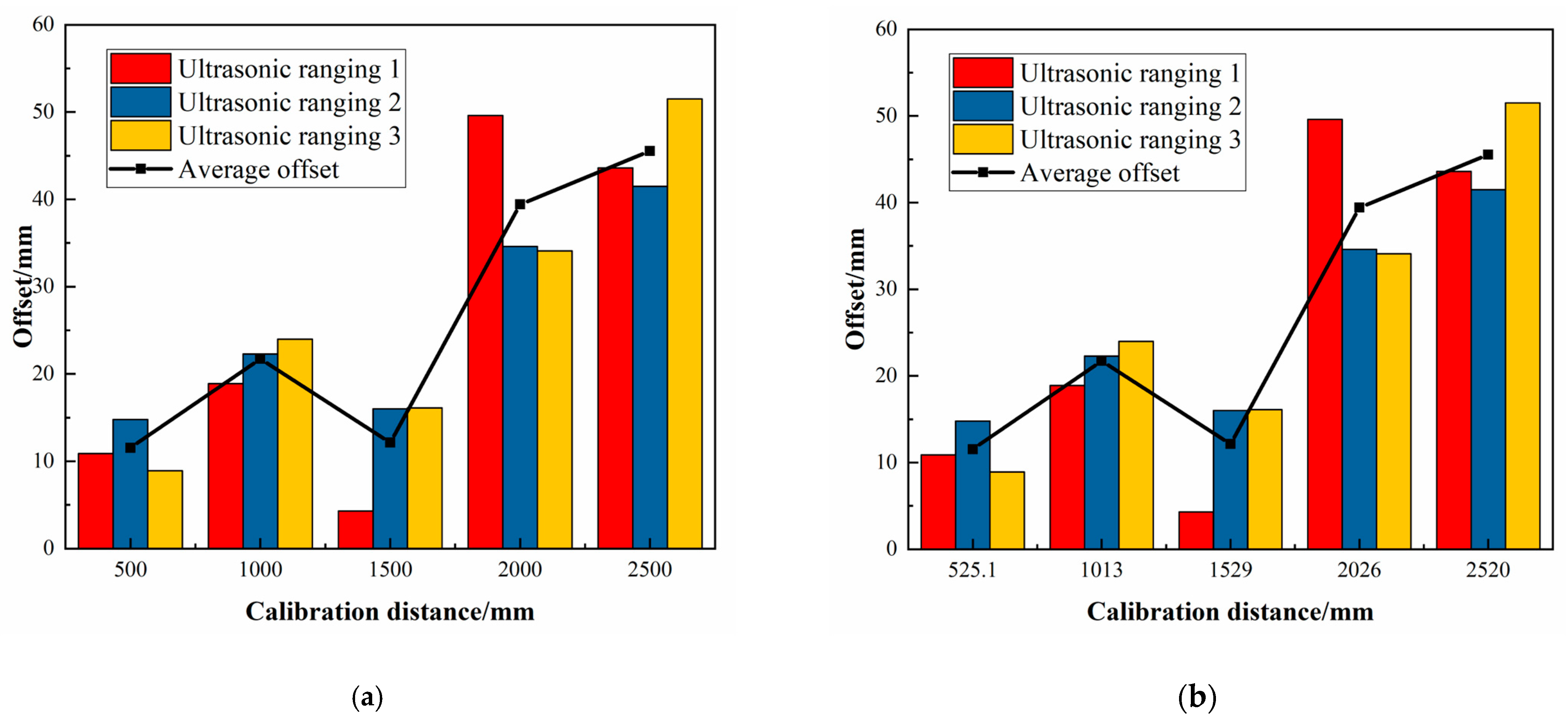
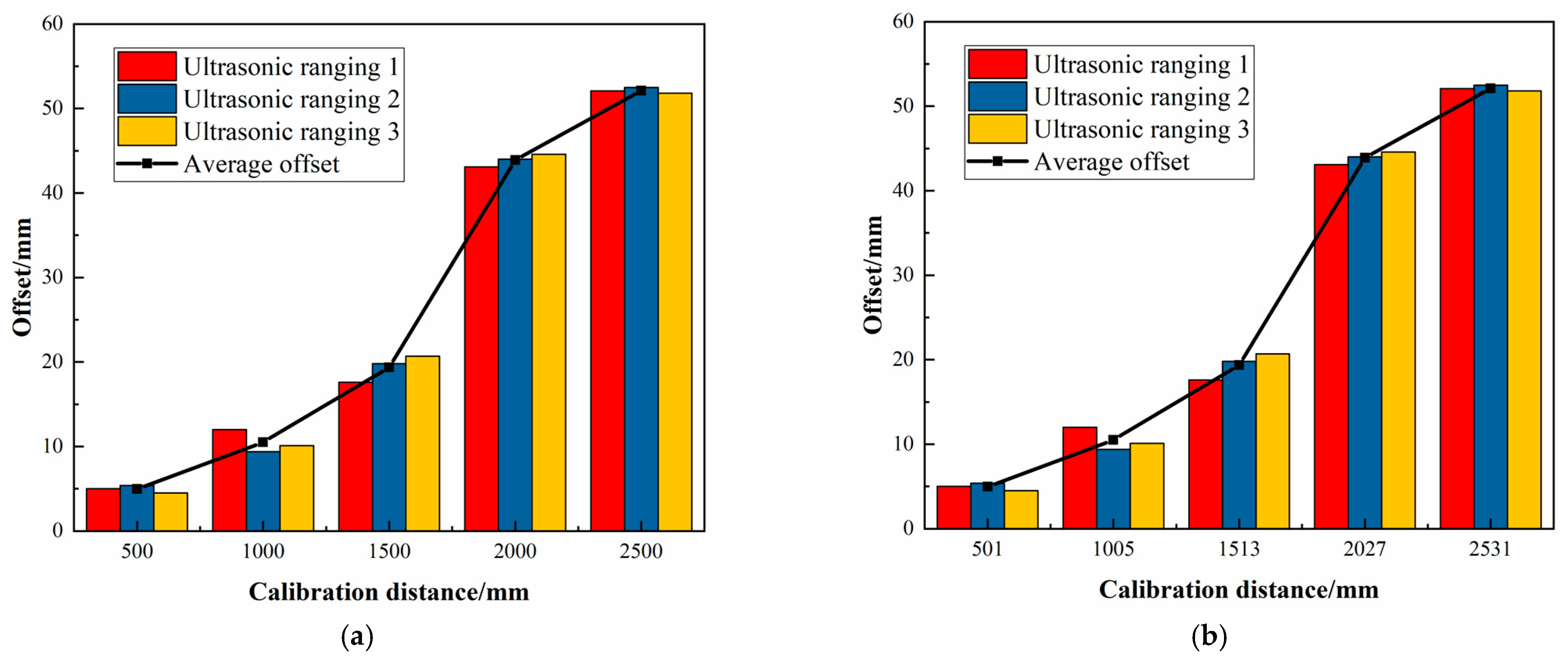
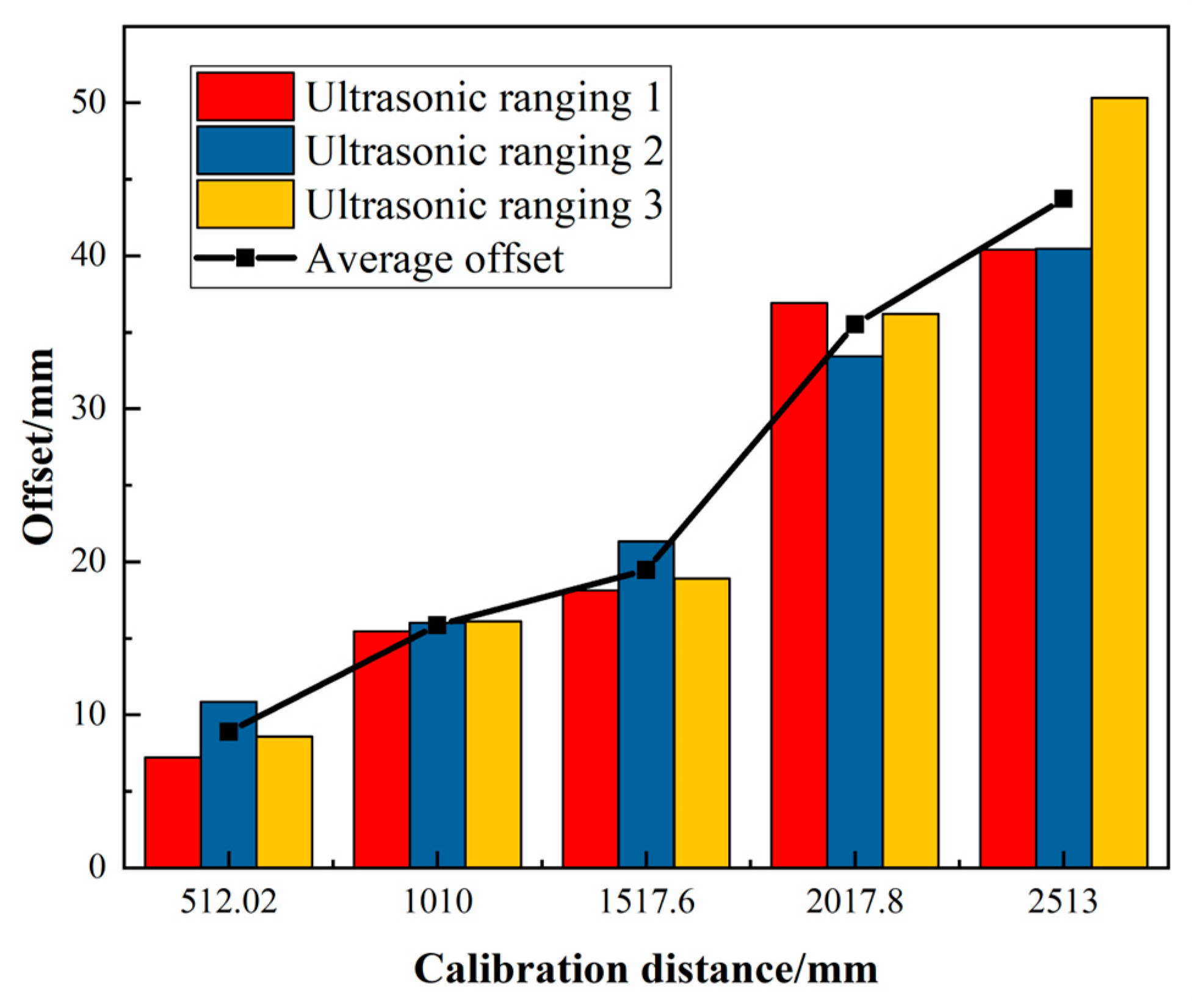
4.3.2. Chassis Offset Characteristics
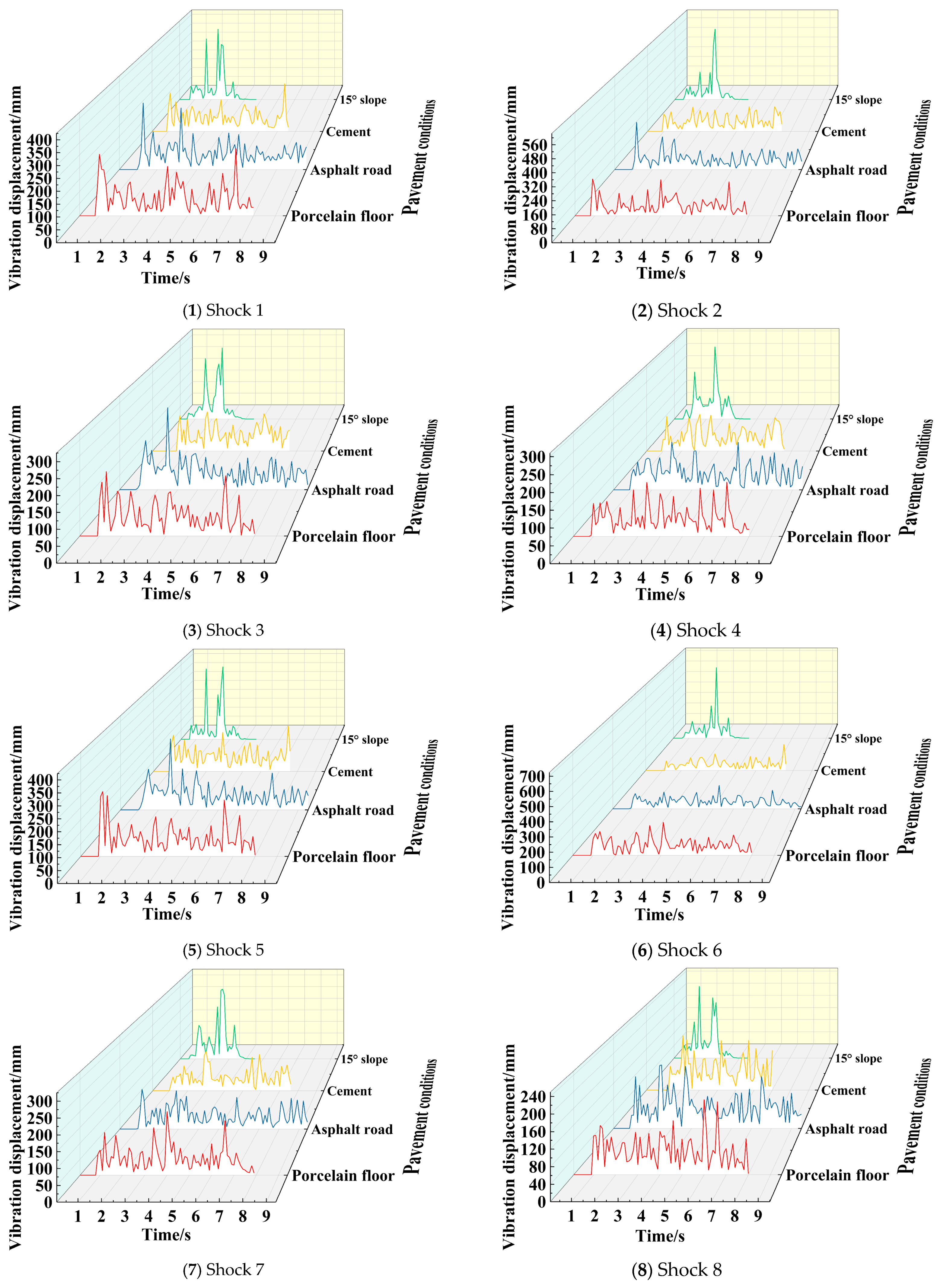
4.4. The Impact of Road Conditions on the Operating Characteristics of the Chassis
4.4.1. Experimental Design for Different Road Conditions
4.4.2. Chassis Operating Characteristics under Different Road Conditions
4.5. The Influence of Damping Spring on the Operating Characteristics of the Chassis
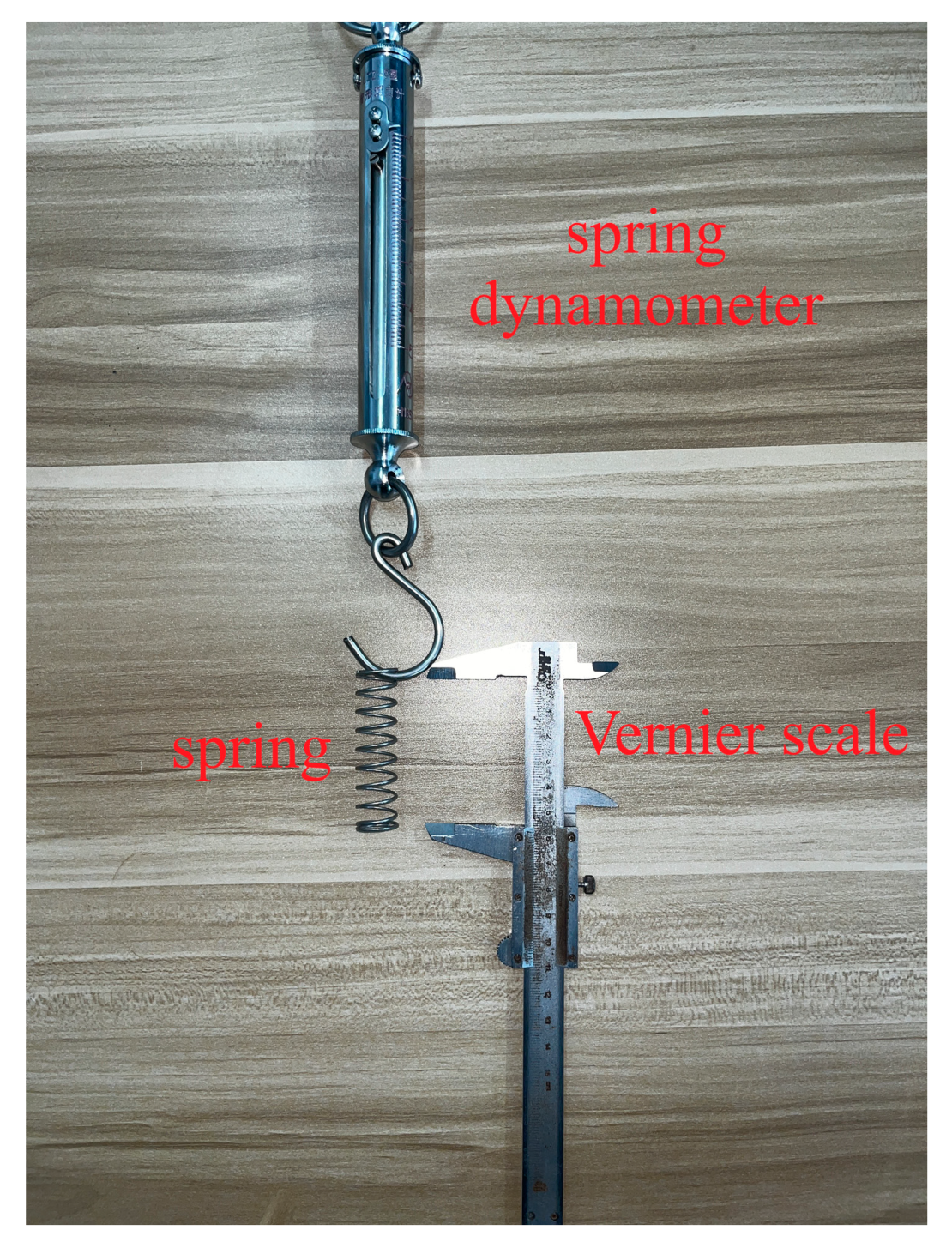
4.5.1. Calculation and Test of Spring Stiffness
4.5.2. Experimental Design under Different Shock-Absorbing Spring
4.5.3. The Operating Characteristics under Different Shock-Absorbing Spring Chassis
5. Performance Optimization of Four-Wheel-Driving Ackerman Chassis Based on Dynamic Simulation
5.1. Simulation Model Construction
5.2. Analysis of the Influence of Structure and Material Parameters on the Dynamic Characteristics of Four-Wheel-Driving Ackerman Chassis
5.2.1. The Influence of the Wheelbase on the Dynamic Characteristics
5.2.2. The Influence of the Guide Rod Structure on the Dynamic Characteristics
5.2.3. The Influence of the Friction Coefficient of the Guide Rod on the Dynamic Characteristics
5.2.4. The Influence of Wheel Friction Coefficient on the Slip Amount
5.2.5. Chassis Straightness Optimization
5.3. Determination of the Optimized Structure of the Four-Wheel-Driving Ackerman Chassis
6. Conclusions
- (1)
- Based on the Ackerman steering mechanism, a four-wheel-driving Ackerman chassis composed of Ackerman steering mechanism, guide rod, suspension shock-absorbing structure, aluminum square tube frame, drive motor, main control board, and loaded wheels is constructed.
- (2)
- The vibration characteristics, migration performance, slippage amount, and travel straightness are determined as the important performance evaluation factors of the carrier chassis. Based on this, a distributed sensing test system for chassis performance is established, which is composed of eight groups of vibration displacement sensors, six groups of travel acceleration sensors, 3three groups of ultrasonic ranging sensors, and two groups of photoelectric gate counting sensors, and the measurement methods of vibration, acceleration, slippage amount, and straightness are proposed.
- (3)
- The single-factor variable analysis method was used to carry out the quantitative operation test of four-wheel-driving Ackerman chassis under different conditions, the results show that in the initial designed four-wheel-driving Ackerman chassis, compared to the tail, the shock-absorbing mechanism in the front of the chassis body can reduce the vibration peak by 35.88%, which has an obvious shock-absorbing effect. The vibration amplitude of the chassis in motion can be controlled below 0.262 mm, and the starting or stopping acceleration mutation can be controlled below 2.94 m/s2, so it has good seismic performance and good motion stability. Although the slippage ratio of the chassis is within the normal range, the slippage amount and the slippage ratio are relatively high, and there is a large optimization space. The offset ratio of the chassis can be controlled within 2%, which meets the requirements of most carrier workshops, but there is still some room for optimization. Under the four different working conditions of porcelain floor, asphalt road, cement road, and 15° slope, the maximum vibration displacement of the chassis is only 0.649 mm, the maximum average vibration displacement is only 0.090697 mm, and the maximum average acceleration is only 0.0708 g. It shows good shock resistance and stability, so the chassis has strong generalization application ability.
- (4)
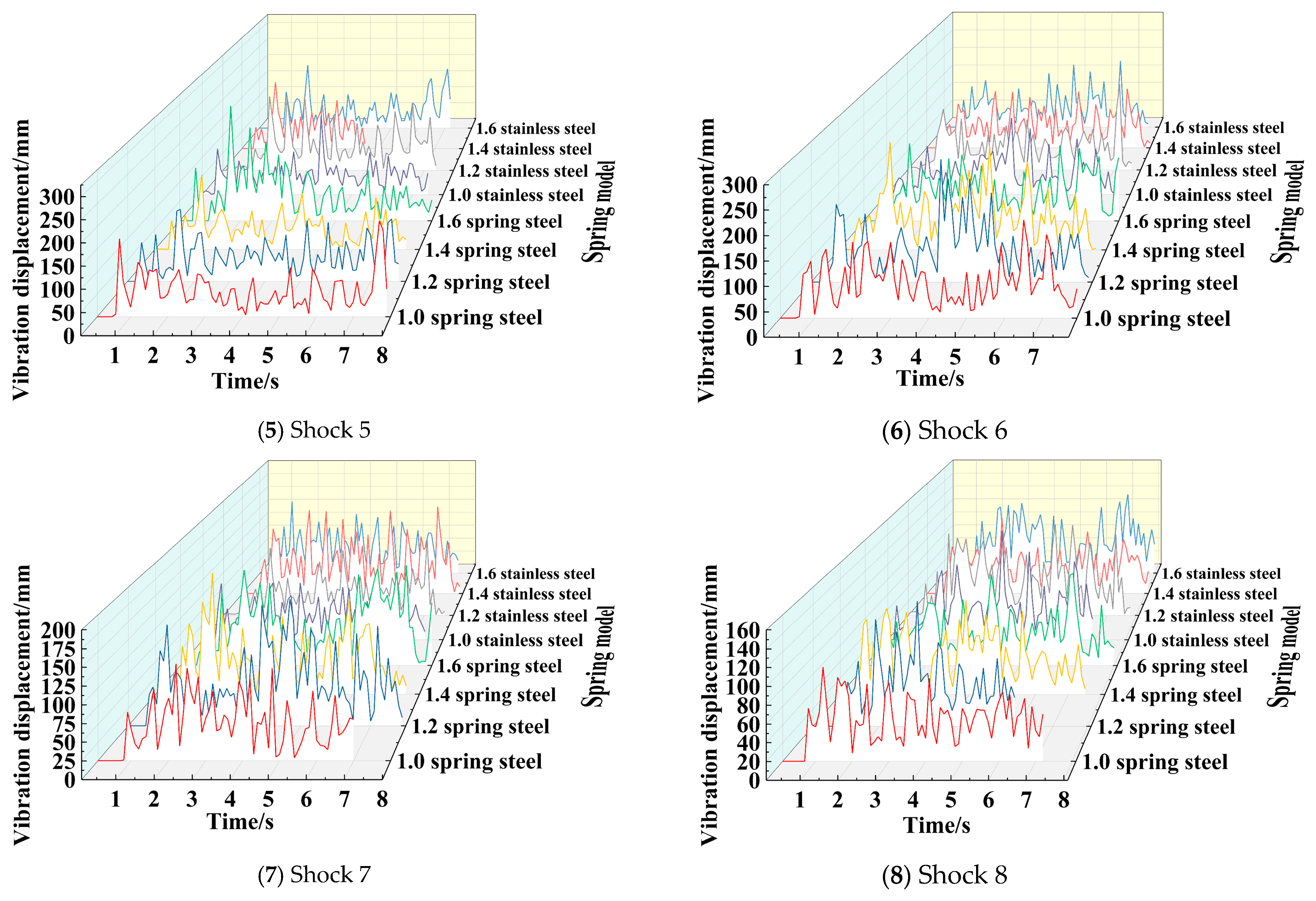
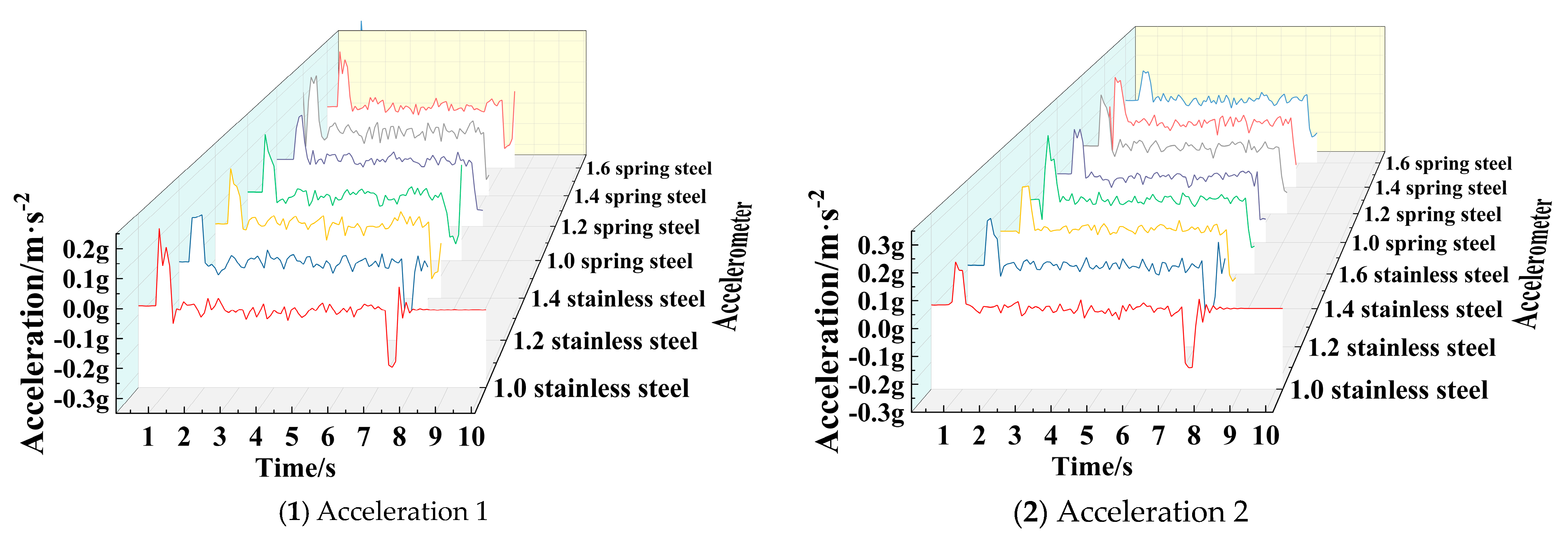
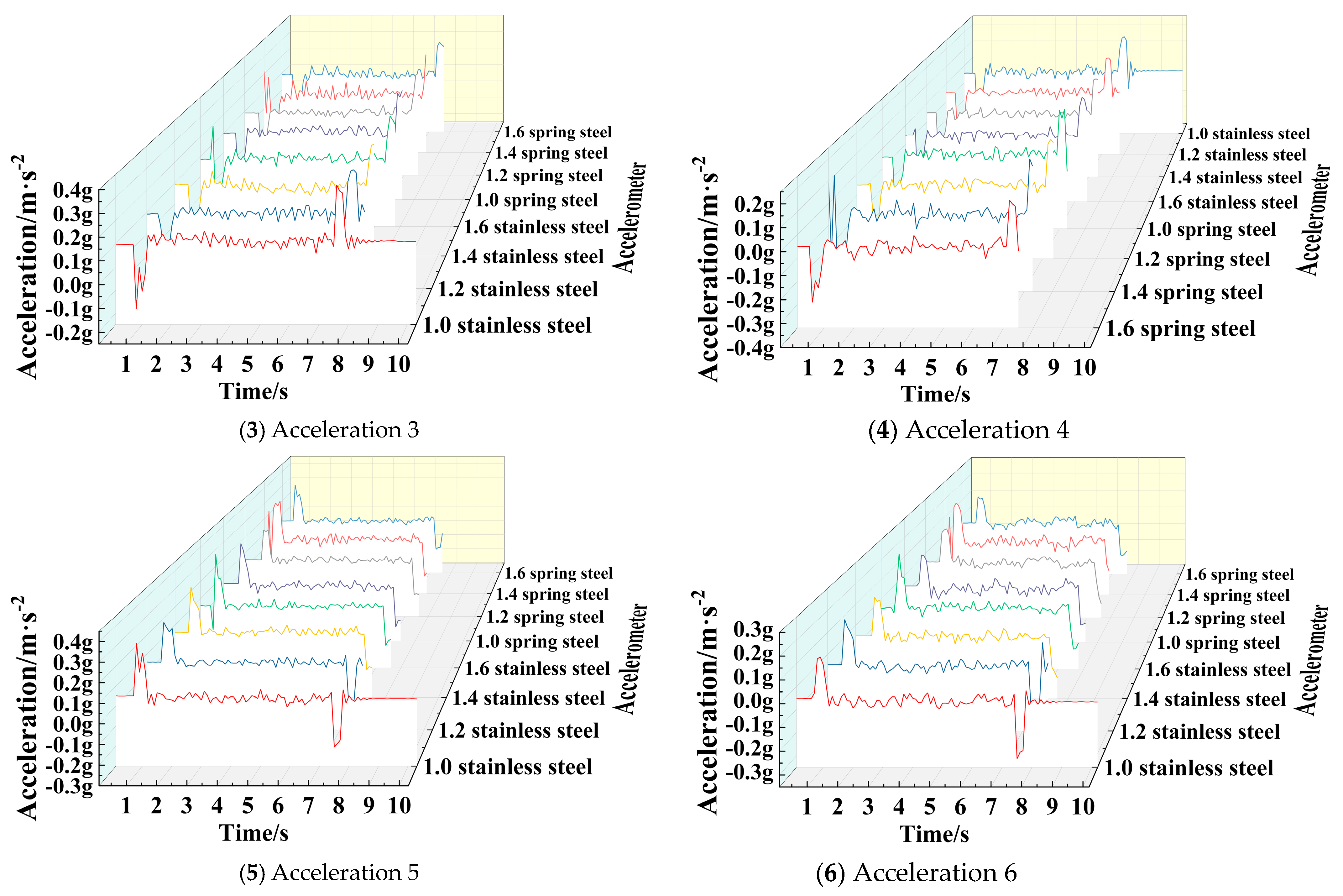
- According to the chassis running experimental results with the support springs of different diameters and materials as the shock-absorbing mechanism, it can be seen that the vibration performance and acceleration performance of the chassis are the best when the 1.4 mm wire diameter stainless steel spring is used as the shock-absorbing-mechanism. Therefore, the 1.4 mm wire diameter stainless steel spring is determined as the shock-absorbing mechanism.
- (5)
- The multi-body dynamics simulation model of the four-wheel-driving Ackerman chassis is established. The operating characteristics of the chassis under the conditions of wheelbase size change, guide rod structure and friction coefficient change, wheel friction coefficient change, and chassis installation error change are analyzed. When the wheelbase of the chassis changes, the original wheelbase+40 mm can effectively improve the overall vibration performance of the chassis. The vibration peak value and vibration mean value of the chassis with the chute guide rod are better than those of the cylindrical guide rod. When the friction coefficient between the guide rod and the guide rod sleeve is 0.3, the advantages of the mean value and peak value of the chassis vibration are very obvious. When the wheel friction coefficient is 0.8, the slippage amount and slippage ratio of the chassis are the smallest and the practicability to the ground is the highest. When the chassis installation error is eliminated and the wheel friction coefficient is 0.8, the chassis offset is significantly reduced and the straightness is effectively improved.
- (6)
- Through the operation simulation of the optimized four-wheel-driving Ackerman chassis and the comparison with the corresponding simulation results of the chassis before optimization, it can be obtained that the average vibration value of the optimized chassis decreases by 27.63%, and the average vibration peak decreases by 2.59%. After optimization, the average acceleration is reduced by 56.76%, and the peak acceleration is reduced by 31.24% when starting or braking. Under the same operating distance, the optimized chassis slippage amount decreased by 48.14% and the offset decreased by 74.63%. Therefore, the vibration performance and stability of the optimized chassis are significantly improved, and the slippage amount and straightness are effectively improved. After optimization, the straightness of the chassis is reduced to 0.399%, which is suitable for the chassis’s accurate carrier applications.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Zheng, Y.; Zheng, X.; Xiong, Y.; Yang, C.; Hou, L. Modeling and motion simulation of four wheel motor driven chassis. Electron. Meas. Technol. 2020, 43, 170–174. [Google Scholar]
- Lv, Y.; Li, Z.; Zhang, J. Innovation and optimization design on steering mechanism of front axle swing steering four-wheel chassis. J. Chin. Agric. Mech. 2016, 37, 107–110. [Google Scholar]
- Zheng, X. Study on Structure Analysis and Control of Omnidirectional Electric Chassis. Master’s Thesis, Changchun University of Science and Technology, Changchun, China, 2018. [Google Scholar]
- Tian, T. Design and Experiment Research of Four Wheel Independent Drive Chassis. Master’s Thesis, Chinese Academy of Agricultural Mechanization Sciences, Beijing, China, 2012. [Google Scholar]
- Yang, F. Research on Control of Handling and Stability for Four-Wheel Steering and Four-Wheel Independent Driving Vehicle. Master’s Thesis, Wuhan University of Technology, Wuhan, China, 2021. [Google Scholar]
- Zhu, Z.; Lv, Q.; Wang, Y.; Ding, H. Four Wheel Differential Steering Model for Omnidirectional Electric Chassis Applied in Off-road Vehicles. J. Chang. Univ. Sci. Technol. (Nat. Sci. Ed.) 2015, 38, 48–52. [Google Scholar]
- Wang, G. Structure Design and Control Research of Four Wheels Intelligent Vehicle. Master’s Thesis, ShiJiazhuang Tiedao University, Shijiazhuang, China, 2018. [Google Scholar]
- Peng, G.; Lu, Z.; Tan, Z.; He, D.; Li, X. A novel algorithm based on nonlinear optimization for parameters calibration of wheeled robot mobile chasses. Appl. Math. Model. 2021, 95, 396–408. [Google Scholar] [CrossRef]
- Wang, R.; Chen, Y.; Feng, D.; Huang, X.; Wang, J. Development and performance characterization of an electric ground vehicle with independently actuated in-wheel motors. J. Power Sources 2011, 196, 3962–3971. [Google Scholar] [CrossRef]
- Joa, E.; Yi, K.; Sohn, K.; Bae, H. Four-wheel independent brake control to limit tire slip under unknown road conditions. Control. Eng. Pract. 2018, 76, 79–95. [Google Scholar] [CrossRef]
- Wang, Z.; Yang, J.; Liu, P.; Long, X.; Li, H.; Wei, W. Development of an agricultural vehicle levelling system based on rapid active levelling. Biosyst. Eng. 2019, 186, 337–348. [Google Scholar] [CrossRef]
- Hang, P.; Chen, X. Integrated chassis control algorithm design for path tracking based on four-wheel steering and direct yaw-moment control. Proc. Inst. Mech. Eng. 2019, 233, 625–641. [Google Scholar] [CrossRef]
- Chi, C.; Xu, Y.; Xu, G.; Cheng, B.; Shen, J. Modular design and analysis of the x-by-wire center point steering independent suspension for in-wheel electric vehicle. Adv. Mech. Eng. 2018, 10, 1–12. [Google Scholar] [CrossRef]
- Zhu, Y.; Kan, J.; Li, W.; Kang, F. Strategies of traversing obstacles and the simulation for a forestry chassis. Int. J. Adv. Robot. Syst. 2018, 15, 1–13. [Google Scholar] [CrossRef]
- Zhang, Z.; Zhang, X.; Pan, H.; Salman, W.; Rasim, Y.; Liu, X.; Wang, C.; Li, X. A Novel Steering System for a Space-Saving 4WS4WD Electric Vehicle: Design, Modeling, and Road Tests. IEEE Trans. Intell. Transp. Syst. 2017, 18, 114–127. [Google Scholar] [CrossRef]
- Xu, Q.; Li, H.; Wang, Q.; Wang, C. Wheel Deflection Control of Agricultural Vehicles with Four-Wheel Independent Omnidirectional Steering. Actuators 2021, 10, 334. [Google Scholar] [CrossRef]
- McGinn, C.; Cullinan, M.F.; Otubela, M.; Kelly, K. Design of a terrain adaptive wheeled robot for human-orientated environments. Auton. Robot. 2019, 43, 63–78. [Google Scholar] [CrossRef]
- Li, J.; Xie, R.; Zhou, Y.; Tang, R. Driving Dynamics Modeling and Simulation of Wheeled Off-Road Vehicle. Syst. Simul. Technol. 2017, 13, 304–309. [Google Scholar]
- Cao, W. Research on Trajectory Tracking Control of Wheeled Mobile Robot Chassis. Master’s Thesis, Xiamen University of Technology, Xiamen, China, 2022. [Google Scholar]
- Sun, X. Vehicle Body Design and Obstacle Surmounting Performance Analysis of Wheeled Household Monitoring Robot. Master’s Thesis, Harbin University of Science and Technology, Harbin, China, 2015. [Google Scholar]
- Qu, J.; Li, H.; Zhang, R.; Guo, K.; Ding, Y.; Wang, B. Research on steering motion control and experiments of agricultural wheeled robot chassis. J. Chin. Agric. Mech. 2023, 44, 140–147+160. [Google Scholar]
- Qin, Q.; Cheng, M.; Qiu, D.; Jin, L. Research on Chassis Pass Ability of Multi-suspension Wheeled Robot. J. Jiamusi Univ. (Nat. Sci. Ed.) 2023, 41, 101–105+139. [Google Scholar]
- Gao, L.; Qin, J.; Huang, M.; Liu, X. Design and simulation of a new type wheeled robot chassis with zero turning radius. China Sci. Pap. 2021, 16, 911–918. [Google Scholar]
- Wang, C.; Wang, D.; Chen, Y.; Liu, Z.; Xiang, C. Obstacle negotiation performance analysis and simulation for six wheel all-terrain mobile robot. Manuf. Autom. 2016, 38, 72–77. [Google Scholar]
- Zhu, J.; Huang, Y.; Zhang, H. Design and Motion Control of Four-wheel Automotive Chassis Inspection Robot. J. Syst. Simul. 2015, 27, 1548–1555. [Google Scholar]
- Yim, S. Comparison among Active Front, Front Independent, 4-Wheel and 4-Wheel Independent Steering Systems for Vehicle Stability Control. Electronics 2020, 9, 798. [Google Scholar] [CrossRef]
- Obertino, M. Upgrade of the EE-Architecture of an Electric Test Vehicle with Drive-by-Wire Component. Master’s Thesis, Politecnico di Torino, Turin, Italy, 2023. [Google Scholar]
- Kolekar, M.A.; Mulani, M.S.; Nerkar, M.A.; Borchate, S. Review on Steering Mechanism. Int. J. Sci. Adv. Res. Technol. 2017, 3, 1155–1160. [Google Scholar] [CrossRef]
- Klier, W.; Reimann, G.; Reinelt, W. Concept and Functionality of the Active Front Steering System; SAE Technical Paper: Warrendale, PA, USA, 2004; 2004-21-0073; Available online: https://www.sae.org/publications/technical-papers/content/2004-21-0073/ (accessed on 20 February 2024).
- Hang, P.; Chen, X.; Fang, S.; Luo, F. Robust control for four-wheel-independent-steering electric vehicle with steer-by-wire system. Int. J. Automot. Technol. 2017, 18, 785–797. [Google Scholar] [CrossRef]
- Whitehead, J.C. Rear Wheel Steering Dynamics Compared to Front Steering. J. Dyn. Syst. Meas. Control 1990, 112, 88–93. [Google Scholar] [CrossRef]
- Zhihu. 2021 China Automotive Steering System Industry Analysis Report—Industry Scale and Development Trend Analysis. Available online: https://zhuanlan.zhihu.com/p/407711855 (accessed on 7 September 2021).
- China Economic Information Network. The Development Trend of China’s Automotive Steering System Industry in 2023, and the Domestic Industry Penetration Rate Is High, But There Is Still Room for Progress. Available online: https://www.huaon.com/channel/trend/905805.html (accessed on 25 June 2023).
- Zhihu. Bosch China/NSK/ZF Won the Top Three EPS Chinese Market Last Year, and the Domestic Market Entered the Breakthrough Cycle. Available online: https://zhuanlan.zhihu.com/p/471673039 (accessed on 24 February 2022).
- Zhihu. Automobile Active Steering Technology Analysis and Steering System Development Review. Available online: https://zhuanlan.zhihu.com/p/393699574 (accessed on 8 April 2022).
- Four-Wheel Steering System Industry Market Size and Brand Competition Analysis Report (Including Industry Key Enterprises Market Performance and Competitive Strategy Analysis). Available online: https://www.globalmarketmonitor.com.cn/reports/2218093-four-wheel-steering-system-market-report.html (accessed on 10 July 2023).
- Liang, L.; Liu, H.; Li, X.; Zhu, X.; Lan, B.; Liu, Y.; Wang, X. Model-based coordinated trajectory tracking control of skid-steer mobile robot with timing-belt servo system. Electronics 2023, 12, 699. [Google Scholar] [CrossRef]
- Choi, M.W.; Park, J.S.; Lee, B.S.; Lee, M.H. The performance of independent wheels steering vehicle(4WS) applied Ackerman geometry. In Proceedings of the 2008 International Conference on Control, Automation and System, Seoul, Republic of Korea, 14–17 October 2008. [Google Scholar]
- Zhao, J.S.; Liu ZJ, F.X.; Dai, J.S. Design of an Ackermann-type steering mechanism. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2013, 227, 2549–2562. [Google Scholar] [CrossRef]
- Gutiérrez, J.; Apostolopoulos, D.; Gordillo, J.L. Numerical comparison of steering geometries for robotic vehicles by modeling positioning error. Auton. Robot. 2007, 23, 147–159. [Google Scholar] [CrossRef]

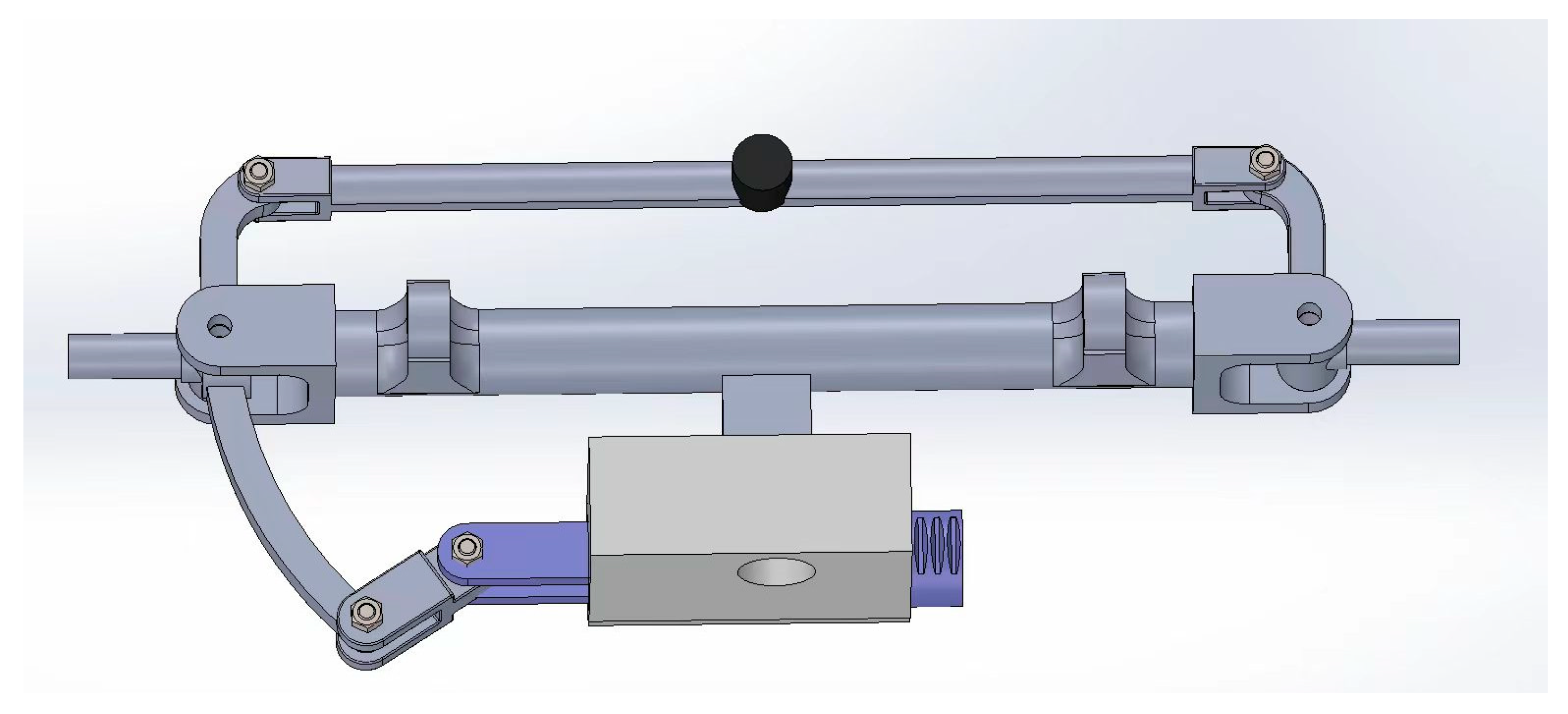
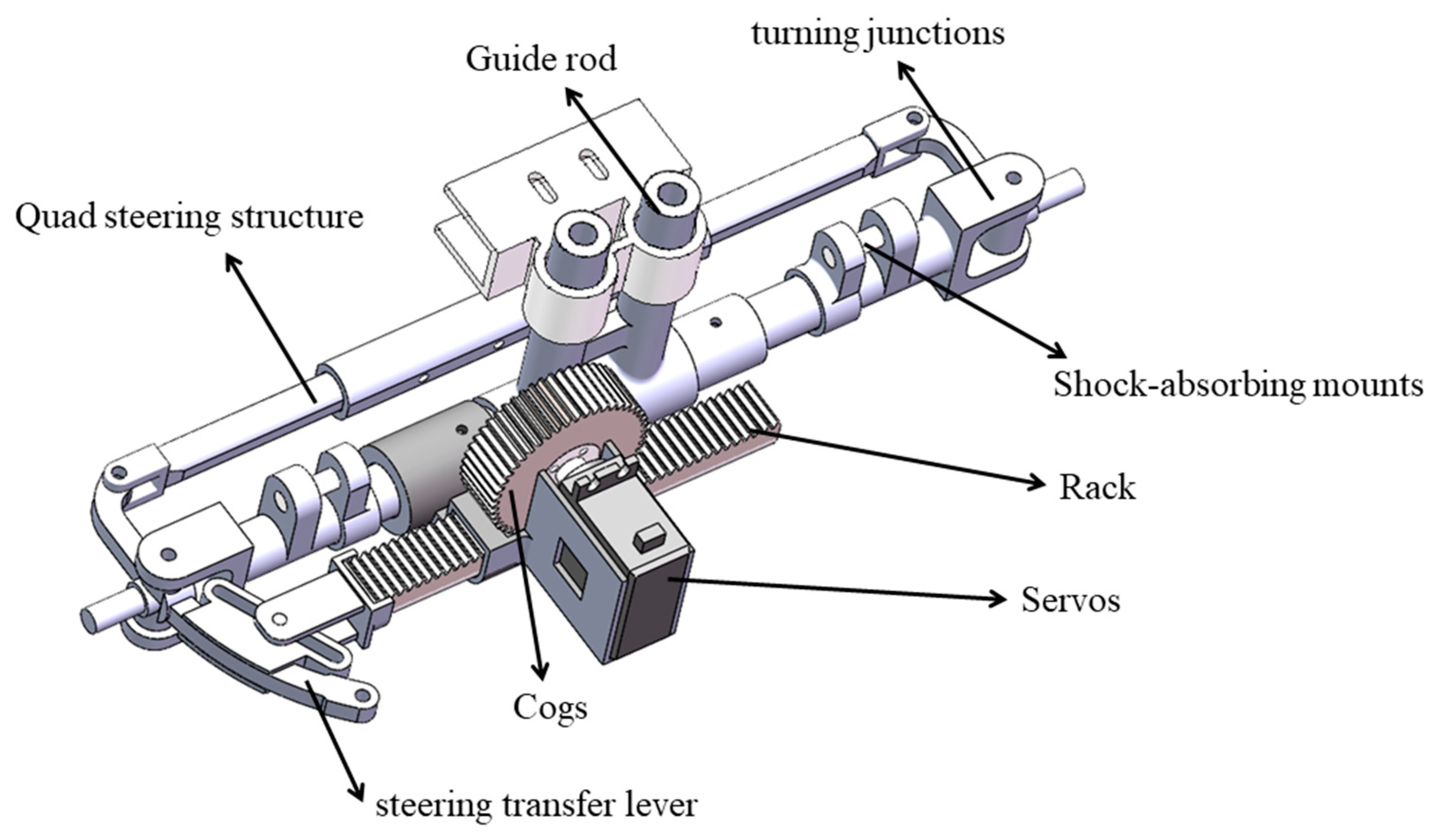
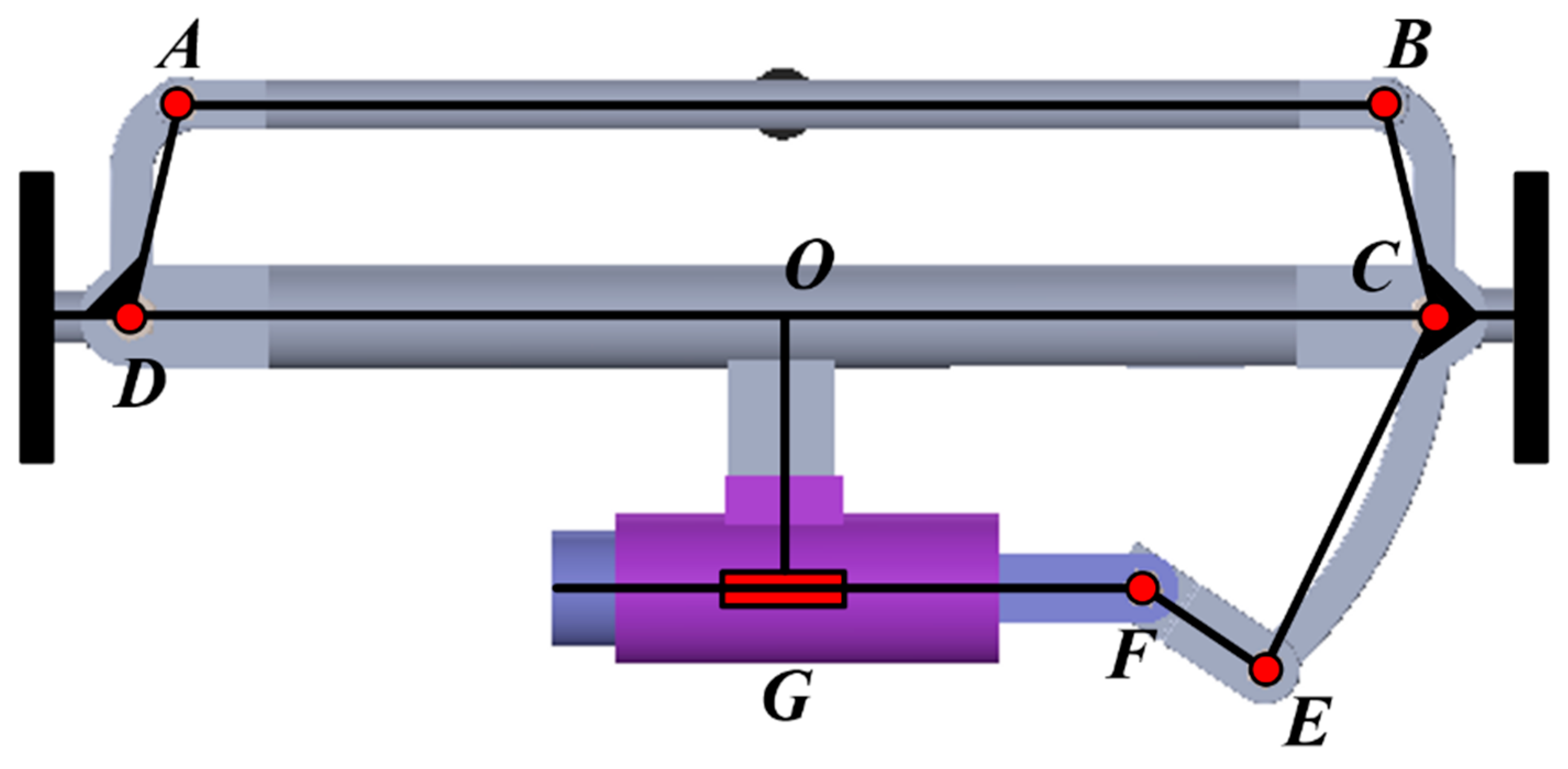


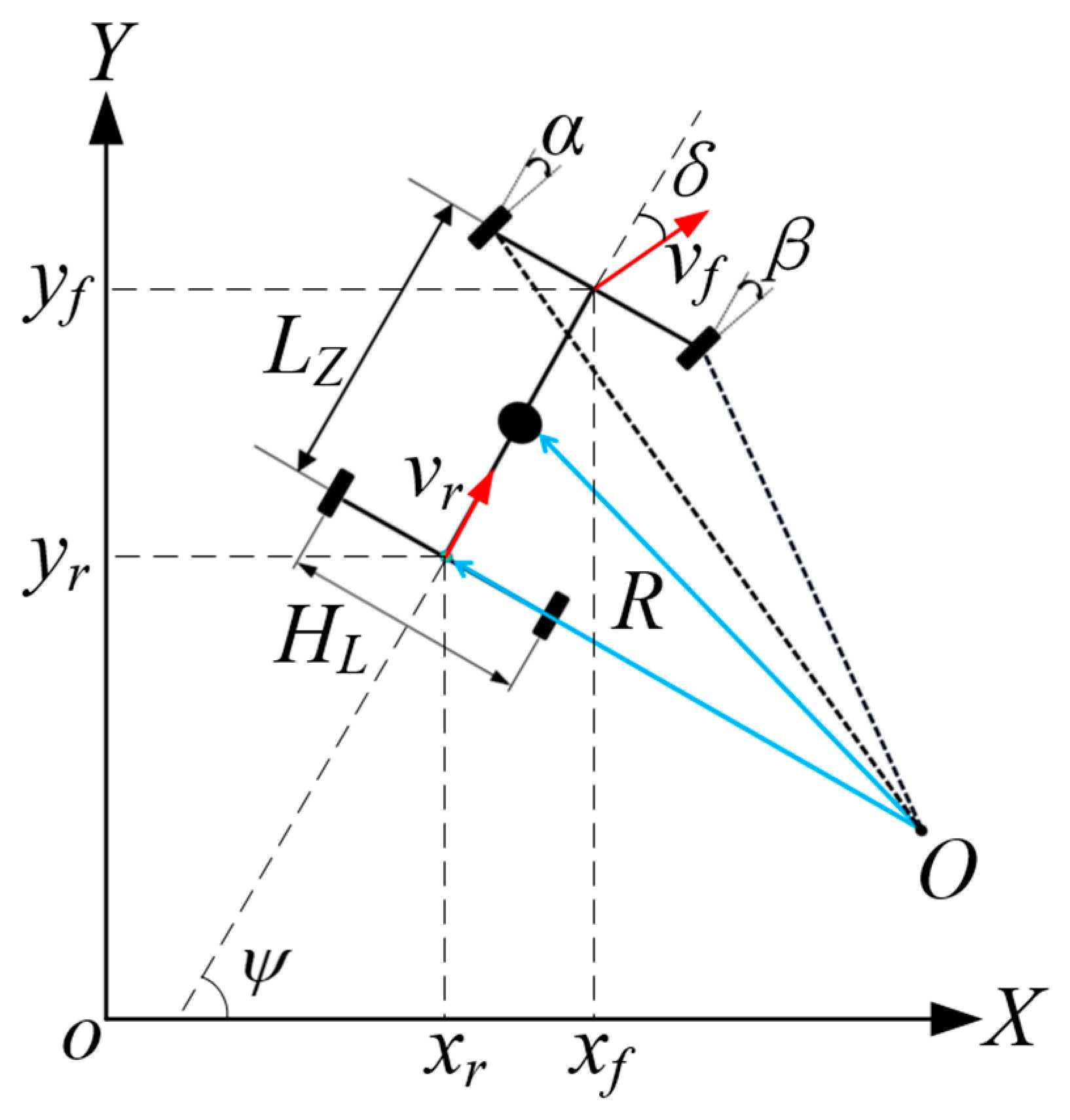













| Steering Mode | Advantage | Disadvantage |
|---|---|---|
| Conventional steering (CSS) system | Simple structure and low cost | Difficulty in steering control at high speeds and inability to control steering response characteristics |
| Four-wheel steering (4WS) system | Good maneuverability and small turning radius | Complex structure, high cost, high maintenance difficulty, and problems with low-speed steering |
| Active front steering (AFS) system | Enhance maneuverability and stability | The system is complex, the cost is high, and the maintenance is difficult. |
| Active front independent steering (AFIS) system | Provide better maneuverability | The newly proposed conceptual turn has not yet been commercially applied. |
| Front independent steering (FWIS) system | It has better handling and stability, vehicle stability control is better than AFS and 4WS, and tire wear is optimized. | Reliability degradation in extreme cases |
| Four-wheel independent steering (4WIS) system | Low speed maneuverability, high speed stability, reduce tire wear | There are difficulties in low-speed steering, cost and maintenance problems, and technical complexity. |
| Rear independent steering (RWIS) system | Improve handling and flexibility | Unstable at high speeds |
| Connecting Rod | AD | AB | BC | CD | CE | EF | GF | OG |
|---|---|---|---|---|---|---|---|---|
| Length (mm) | 61 | 335 | 61 | 363 | 109 | 41 | 100 | 76 |
| Type | Working Current | Range | Resolution Ratio | Accuracy |
|---|---|---|---|---|
| WT9011DCL-BT50 | 14 mA ± 16 g | 0.5 mg/LSB | Acceleration: 0.01 g | Angular velocity 0.2°/s |
| Output content | Output frequency | Working time | transmission distance | Attitude measurement stable angle |
| Acceleration | 0.2 Hz~200 Hz | 8 h | 90 m | 0.05° |
| Data interface | Operating voltage | Volume (mm) | Data output frequency | |
| Baud rate 115,200 | 5 V | 23.5 × 32.5 × 11.4 | 0.2 Hz~200 Hz |
| Type | Range | Detection Cycle | Transmission Distance | Working Temperature |
|---|---|---|---|---|
| WTVBO1-BT50 | 0~30,000 µm | 1~100 Hz | 90 m | −20~60 °C |
| Operating voltage | Working current | Working time | Serial port rate | Start-up time |
| 3.7 V | 15 mA | 6~8 h | 115200 bps | 1000 ms |
| Type | Operating Voltage | Collector Current Range | The Forward Current of the Diode |
|---|---|---|---|
| tcrt5000 | 5 V | 0.2 mm–15 mm | 60 mA |
| Triode collector current | Range of working temperature | Detection reflection distance | Output form |
| 100 mA | −25 °C~+85 °C | 1 mm~25 mm | Digital switching output (0 and 1) |
| Type | Operating Voltage | Working Current | Working Frequency | Maximum Range |
|---|---|---|---|---|
| HC SR04 | DC5V | 15 mA | 40 kHz | 4 m |
| Nearest range | Measuring angle | Input trigger signal | Output echo signal | Specification and size |
| 2 cm | 15° | 10uS’s TTL pulse | Output TTL level signal | 45 × 20 × 15 mm |
| Type | Operating Voltage | Working Current | Working Frequency |
|---|---|---|---|
| HC-05 | DC 1.8–3.6 V | 7.3 mA | 2.4 GHZ |
| Maximum range | Working temperature | Received power | Specification and size |
| 30 m | −40 °C~80 °C | −97 dbm | 19.6 × 14.91 × 1.8 mm |
| Vibration 1 | Vibration 2 | Vibration 3 | Vibration 4 | Vibration 5 | Vibration 6 | Vibration 7 | Vibration 8 | |
|---|---|---|---|---|---|---|---|---|
| Maximum value | 191 | 186 | 140 | 155 | 168 | 262 | 183 | 144 |
| Average value | 73.027 | 58.865 | 60.014 | 64.392 | 59.757 | 75.257 | 68.110 | 50.706 |
| Vibration 1 | Vibration 2 | Vibration 3 | Vibration 4 | Vibration 5 | Vibration 6 | ||
|---|---|---|---|---|---|---|---|
| X | Maximum value | −0.28 | 0.259 | 0.3 | −0.226 | 0.249 | −0.276 |
| Average value | −0.0651 | 0.0205 | 0.0899 | −0.0472 | 0.0588 | −0.0438 | |
| Y | Maximum value | 0.126 | −0.171 | 0.144 | −0.099 | 0.064 | 0.053 |
| Average value | 0.0568 | −0.0564 | 0.0752 | −0.0628 | 0.0223 | 0.0092 | |
| Z | Maximum value | 1.184 | 1.208 | 1.183 | 1.133 | 1.211 | −1.113 |
| Average value | 0.9891 | 0.9891 | 0.9853 | 0.9882 | 1.0016 | −0.9715 |
| Calibrated Operating Distances | 600 mm | 1200 mm | 1800 mm | 2400 mm | 3000 mm | 3600 mm | 4200 mm |
| Actual operating distance | 616 | 1245 | 1830 | 2421 | 3043 | 3642 | 4235 |
| Photoelectric counting 1 | 21 | 43 | 62 | 80 | 99 | 118 | 136 |
| Photoelectric counting 2 | 20 | 42 | 61 | 79 | 98 | 117 | 135 |
| Calibrated Distances | 600 mm | 1200 mm | 1800 mm | 2400 mm | 3000 mm | 3600 mm | 4200 mm |
| Right wheel | 686.9 | 1405.2 | 2027.9 | 2616.8 | 3238.1 | 3859.5 | 4447.025 |
| Left wheel | 654.3 | 1373.8 | 1995.2 | 2583.9 | 3205.5 | 3826.9 | 4415.6 |
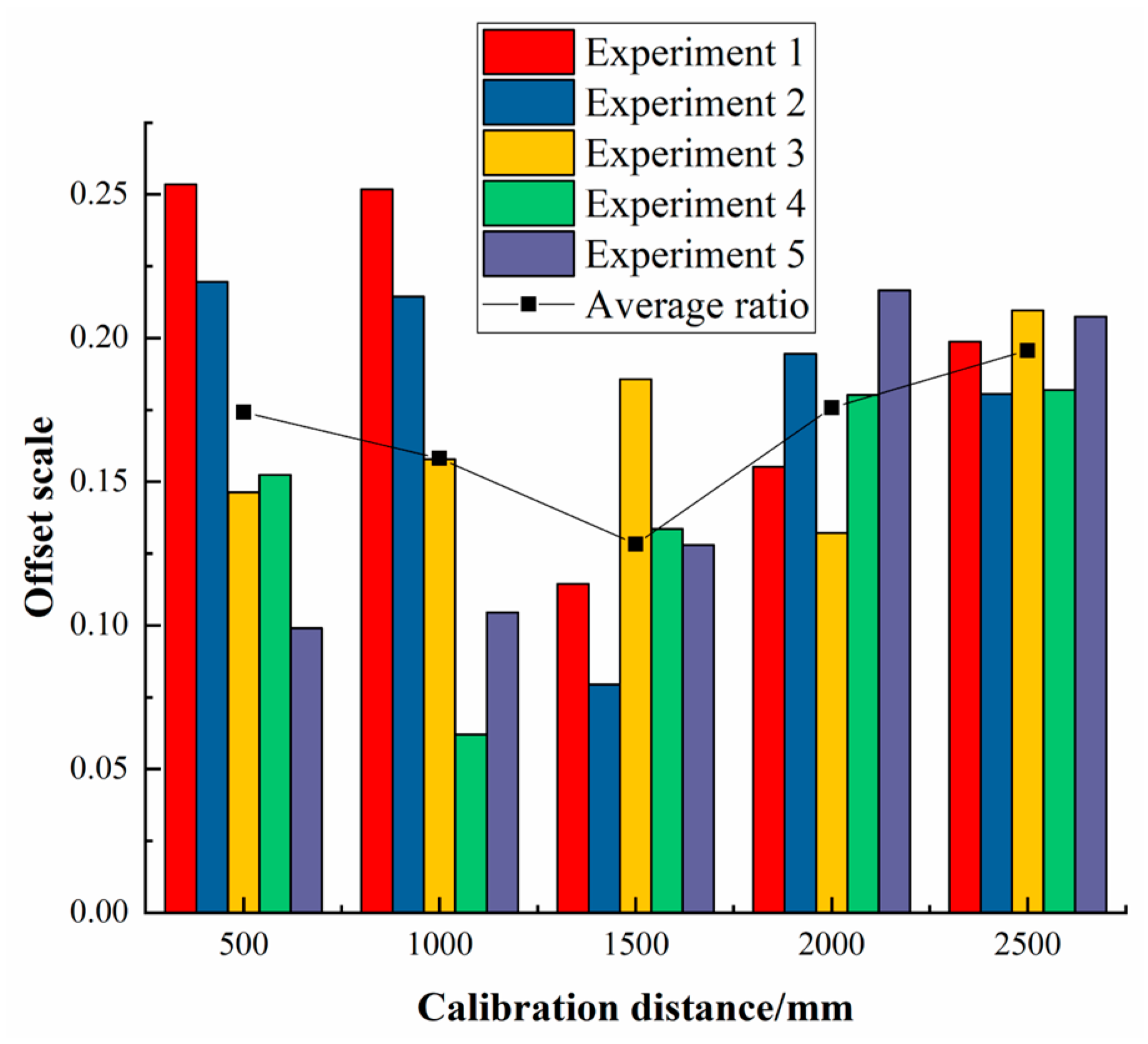
| Calibrated Distance | 500 mm | 1000 mm | 1500 mm | 2000 mm | 2500 mm | |
|---|---|---|---|---|---|---|
| Actual distance | Experimental group 1 | 480 | 978 | 1514 | 2031 | 2553 |
| Experimental group 2 | 525.1 | 1013 | 1529 | 2026 | 2520 | |
| Experimental group 3 | 540 | 1012 | 1518 | 1972 | 2466 | |
| Experimental group 4 | 514 | 1042 | 1514 | 2033 | 2495 | |
| Experimental group 5 | 501 | 1005 | 1513 | 2027 | 2531 | |
| Calibrated Distance | 500 mm | 1000 mm | 1500 mm | 2000 mm | 2500 mm |
| The average actual running distance of the five experiments | 512.02 | 1010 | 1517.6 | 2017.8 | 2513 |
| The average offset of the five experiments | 8.88 | 15.86 | 19.4533 | 35.52 | 43.7233 |
| The average deviation proportion in five groups of experiments | 0.017418 | 0.015812 | 0.012824 | 0.017578 | 0.01957 |
| Vibration 1 | Vibration 2 | Vibration 3 | Vibration 4 | Vibration 5 | Vibration 6 | Vibration 7 | Vibration 8 | ||
|---|---|---|---|---|---|---|---|---|---|
| Peak values | Porcelain floor | 276 | 223 | 202 | 162 | 265 | 231 | 200 | 181 |
| Asphalt road | 303 | 317 | 283 | 157 | 321 | 181 | 138 | 169 | |
| Cement road | 238 | 182 | 151 | 133 | 227 | 220 | 150 | 159 | |
| 15° slope | 381 | 561 | 294 | 283 | 391 | 649 | 288 | 228 | |
| Average values | Porcelain floor | 78.528 | 65.465 | 64.803 | 55.056 | 78.915 | 75.803 | 54.493 | 58.859 |
| Asphalt road | 78.765 | 72.315 | 64.443 | 58.202 | 72.034 | 57.655 | 48.576 | 58.830 | |
| Cement road | 73.209 | 79.106 | 67.143 | 57.910 | 79.227 | 56.448 | 54.439 | 62.175 | |
| 15° slope | 58.810 | 88.406 | 66.906 | 70.267 | 80.4 | 90.697 | 78.313 | 53.433 | |
| Acceleration 1 | Acceleration 2 | Acceleration 3 | Acceleration 4 | Acceleration 5 | Acceleration 6 | ||
|---|---|---|---|---|---|---|---|
| Peak values | Porcelain floor | −0.296 | 0.285 | −0.499 | −0.317 | 0.249 | 0.377 |
| Asphalt road | 0.407 | 0.48 | 0.568 | −0.611 | 0.392 | 0.567 | |
| Cement road | 0.704 | −0.548 | 0.599 | −0.536 | 0.709 | 0.739 | |
| Average values | Porcelain floor | −0.0479 | 0.00712 | 0.0336 | −0.0314 | −0.0152 | −0.0306 |
| Asphalt road | −0.0484 | 0.0602 | 0.0663 | −0.0375 | 0.0119 | −0.0448 | |
| Cement road | −0.0378 | −0.0425 | −0.0343 | −0.0655 | 0.0708 | −0.0115 |
| Material | Wire Diameter | 2 N | 3 N | 4 N | 5 N | 6 N | 7 N | 8 N | 9 N | 10 N | 11 N | 12 N | 13 N |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Spring steel springs | 1.0 mm | 8.5 | 13 | 16 | 19.5 | 23.5 | - | - | - | - | - | - | - |
| 1.2 mm | 5 | 7 | 7.5 | 8 | 8.5 | - | - | - | - | - | - | ||
| 1.4 mm | - | - | 4.5 | 5 | 7 | 8.5 | 9 | - | - | - | - | - | |
| 1.6 mm | - | - | - | 2.5 | - | 4 | - | 5 | - | 5.5 | - | 7.5 | |
| Stainless steel | 1.0 mm | 7.5 | 12.5 | 17.5 | 20.5 | 26 | - | - | - | - | - | - | - |
| 1.2 mm | 3.5 | 7 | 9.5 | 8.5 | 11 | - | - | - | - | - | - | - | |
| 1.4 mm | - | 2 | 3 | 3.5 | 5 | 6.5 | - | - | - | - | - | - | |
| 1.6 mm | - | - | 1.5 | - | 3 | - | 3.5 | - | 5 | - | 7 | - |
| Material | Wire Diameter | 2 N | 3 N | 4 N | 5 N | 6 N | 7 N | 8 N | 9 N | 10 N | 11 N | 12 N | 13 N | Stiffness |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Spring steel springs | 1.0 mm | 24 | 23.55 | 25.51 | 26.16 | 26.05 | - | - | - | - | - | - | - | 25.054 |
| 1.2 mm | - | 61.22 | 58.31 | 68.02 | 63.15 | 84.033 | - | - | - | - | - | - | 66.9466 | |
| 1.4 mm | - | - | 90.7 | 102.04 | 87.46 | 84.03 | 96.03 | - | - | - | - | - | 92.052 | |
| 1.6 mm | - | - | - | - | - | 178.57 | - | 183.67 | - | 204.08 | - | 176.87 | 189.454 | |
| Stainless steel | 1.0 mm | 27.21 | 24.49 | 24.06 | 24.89 | 23.55 | - | - | - | - | - | - | - | 24.84 |
| 1.2 mm | 58.31 | 45.11 | 42.96 | 60.02 | 55.66 | - | - | - | - | - | - | - | 52.412 | |
| 1.4 mm | - | 153.06 | 136.05 | 145.77 | 122.45 | 113.36 | - | - | - | - | - | - | 134.138 | |
| 1.6 mm | - | - | 272.11 | - | 204.08 | - | 233.23 | - | 204.08 | - | 174.93 | - | 217.686 |
| Spring Type | Shock 1 | Shock 2 | Shock 3 | Shock 4 | Shock 5 | Shock 6 | Shock 7 | Shock 8 | |
|---|---|---|---|---|---|---|---|---|---|
| Maximum value | 1.0 mm Stainless steel | 188 | 127 | 137 | 151 | 214 | 188 | 94 | 145 |
| 1.2 mm Stainless steel | 216 | 180 | 146 | 148 | 210 | 220 | 135 | 127 | |
| 1.4 mm Stainless steel | 149 | 197 | 150 | 144 | 195 | 158 | 124 | 109 | |
| 1.6 mm Stainless steel | 224 | 191 | 151 | 137 | 193 | 185 | 136 | 119 | |
| 1.0 mm Spring steel | 196 | 187 | 121 | 177 | 212 | 198 | 133 | 103 | |
| 1.2 mm Spring steel | 191 | 186 | 140 | 155 | 168 | 262 | 183 | 144 | |
| 1.4 mm Spring steel | 173 | 115 | 137 | 171 | 184 | 242 | 184 | 114 | |
| 1.6 mm Spring steel | 180 | 164 | 152 | 149 | 296 | 182 | 160 | 117 | |
| Average value | 1.0 mm Stainless steel | 56.133 | 43.840 | 45.103 | 46.878 | 51.867 | 54.319 | 39.108 | 42.833 |
| 1.2 mm Stainless steel | 69.026 | 58.468 | 45.938 | 48.260 | 69.722 | 60.816 | 39.429 | 49.883 | |
| 1.4 mm Stainless steel | 57.845 | 55.147 | 58.581 | 48.770 | 65.400 | 61.069 | 47.712 | 41.514 | |
| 1.6 mm Stainless steel | 64.188 | 63.714 | 57.814 | 53.043 | 61.226 | 60.309 | 50.217 | 54.412 | |
| 1.0 mm Spring steel | 61.288 | 61.452 | 45.162 | 55.473 | 59.784 | 73.219 | 54.968 | 48.585 | |
| 1.2 mm Spring steel | 73.027 | 58.865 | 60.014 | 64.392 | 59.757 | 75.257 | 68.110 | 50.706 | |
| 1.4 mm Spring steel | 68.437 | 49.408 | 54.169 | 60.958 | 55.606 | 75.688 | 58.686 | 49.188 | |
| 1.6 mm Spring steel | 65.403 | 58.520 | 53.684 | 50.117 | 53.329 | 76.264 | 66.108 | 45.736 |
| Spring Type | Acceleration 1 | Acceleration 2 | Acceleration 3 | Acceleration 4 | Acceleration 5 | Acceleration 6 | |
|---|---|---|---|---|---|---|---|
| Maximum value | 1.0 mm Stainless steel | −0.281 | −0.221 | −0.183 | −0.292 | 0.313 | −0.313 |
| 1.2 mm Stainless steel | −0.263 | 0.2 | 0.292 | −0.287 | 0.266 | −0.256 | |
| 1.4 mm Stainless steel | −0.276 | 0.202 | 0.196 | −0.303 | 0.311 | −0.251 | |
| 1.6 mm Stainless steel | −0.274 | 0.296 | 0.255 | −0.353 | 0.358 | −0.25 | |
| 1.0 mm Spring steel | −0.283 | 0.21 | 0.31 | −0.292 | 0.29 | −0.261 | |
| 1.2 mm Spring steel | −0.28 | 0.259 | 0.3 | −0.226 | 0.249 | −0.276 | |
| 1.4 mm Spring steel | −0.278 | 0.239 | 0.215 | −0.267 | 0.313 | −0.214 | |
| 1.6 mm Spring steel | −0.259 | 0.17 | 0.285 | 0.148 | 0.306 | −0.241 | |
| Average value | 1.0 mm Stainless steel | −0.0788 | −0.0047 | 0.1102 | −0.0341 | 0.0406 | −0.0639 |
| 1.2 mm Stainless steel | −0.1434 | −0.0635 | 0.0165 | −0.1278 | −0.0206 | −0.1307 | |
| 1.4 mm Stainless steel | −0.0624 | 0.0307 | 0.0769 | −0.0549 | 0.0657 | −0.0461 | |
| 1.6 mm Stainless steel | −0.0617 | 0.0245 | 0.0876 | −0.0495 | 0.0601 | −0.0351 | |
| 1.0 mm Spring steel | −0.0838 | −0.0017 | 0.1053 | −0.0307 | 0.0354 | −0.0662 | |
| 1.2 mm Spring steel | −0.0651 | 0.0205 | 0.0899 | −0.0472 | 0.0588 | −0.0438 | |
| 1.4 mm Spring steel | −0.0515 | 0.0231 | 0.0757 | −0.0601 | 0.0705 | −0.0406 | |
| 1.6 mm Spring steel | −0.0568 | 0.0250 | 0.0872 | −0.0533 | 0.0622 | −0.0417 |
| 1.4 mm Stainless Steel | 1.6 mm Stainless Steel | 1.2 mm Spring Steel | 1.4 mm Spring Steel | 1.6 mm Spring Steel | |
|---|---|---|---|---|---|
| Acceleration peak value | 0.311 | 0.358 | 0.3 | 0.313 | 0.306 |
| Average value of the acceleration peak value | 0.2565 | 0.2977 | 0.265 | 0.2543 | 0.2348 |
| Standard deviation of the acceleration peak value | 0.235 | 0.243 | 0.252 | 0.250 | 0.266 |
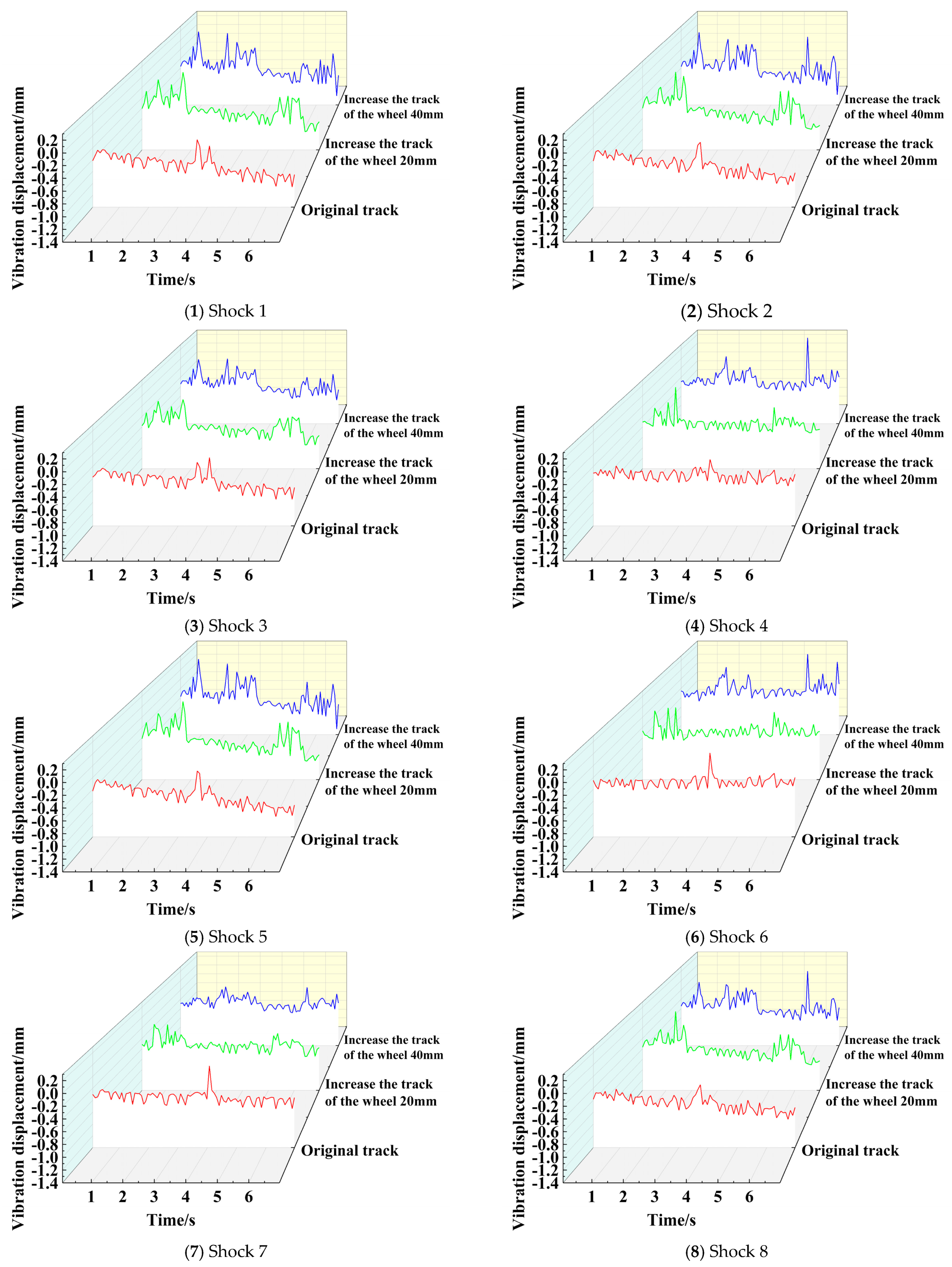
| Different Wheelbase | Shock 1 | Shock 2 | Shock 3 | Shock 4 | Shock 5 | Shock 6 | Shock 7 | Shock 8 | |
|---|---|---|---|---|---|---|---|---|---|
| Maximum value | Original wheelbase | −1.0600 | −1.0239 | −0.9490 | −0.7249 | −1.0583 | −0.6160 | −0.7458 | −0.9214 |
| Original wheelbase +20 mm | −1.0500 | −1.0040 | −0.9452 | −0.7130 | −1.0439 | −0.6491 | −0.7482 | −0.9032 | |
| Original wheelbase +40 mm | −1.2000 | −1.1757 | −0.8890 | −0.6975 | −1.2793 | −0.6753 | −0.6973 | −0.8633 | |
| Average value | Original wheelbase | −0.6899 | −0.6904 | −0.6397 | −0.6397 | −0.7045 | −0.4741 | −0.5433 | −0.6402 |
| Original wheelbase +20 mm | −0.6181 | −0.6310 | −0.5816 | −0.5248 | −0.6349 | −0.4680 | −0.5119 | −0.5946 | |
| Original wheelbase +40 mm | −0.5616 | −0.5562 | −0.5282 | −0.4587 | −0.5685 | −0.4151 | −0.4641 | −0.5228 | |
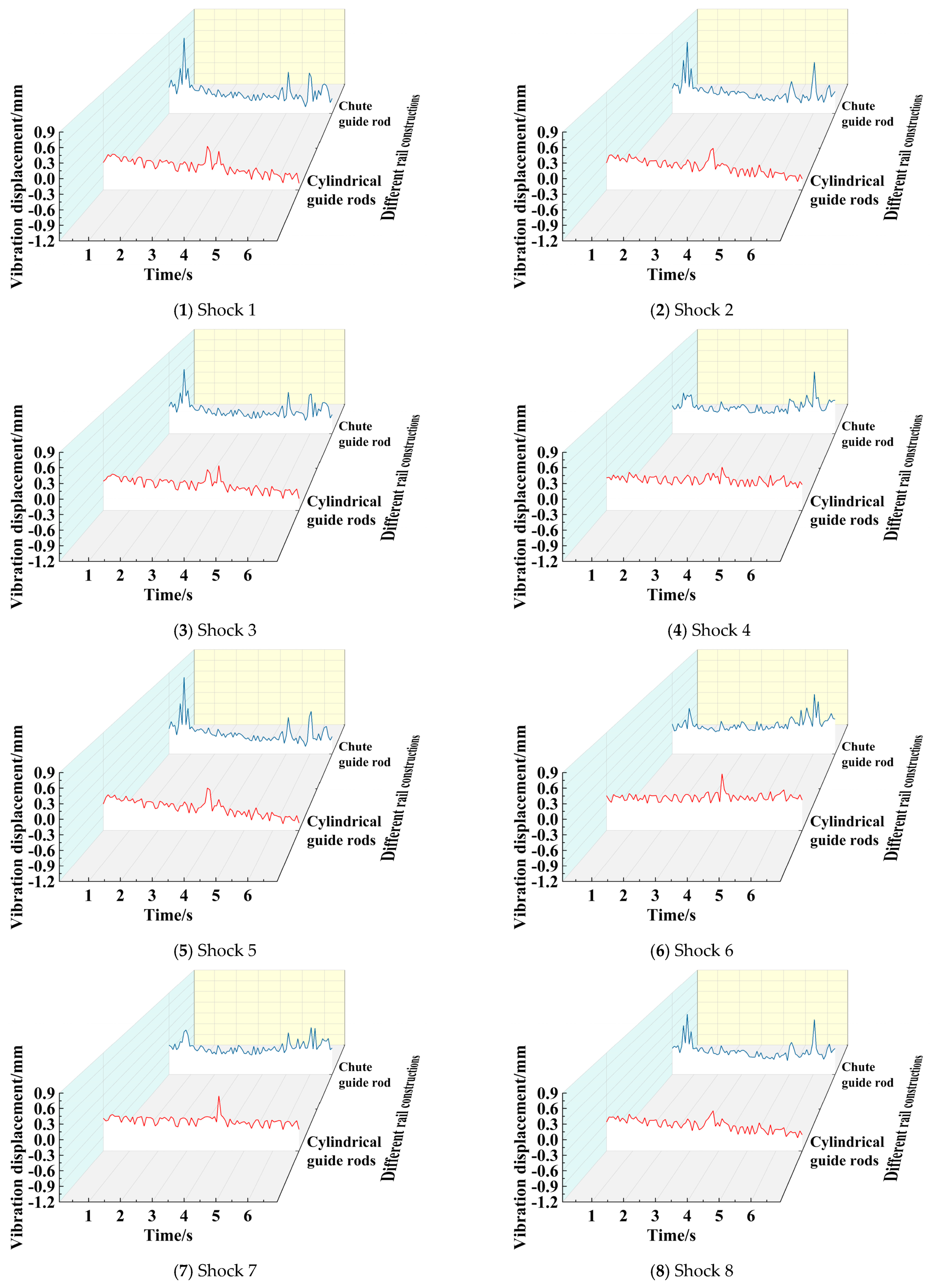
| Different Guide Rod Structure | Shock 1 | Shock 2 | Shock 3 | Shock 4 | Shock 5 | Shock 6 | Shock 7 | Shock 8 | |
|---|---|---|---|---|---|---|---|---|---|
| Maximum value | Cylindrical guide rod | −1.0600 | −1.0239 | −0.9487 | −0.7249 | −1.0583 | −0.6160 | −0.7458 | −0.9214 |
| Chute guide rod | −1.0328 | −0.9319 | −0.8589 | −0.6933 | −1.0016 | −0.6239 | −0.7005 | −0.8453 | |
| Average value | Cylindrical guide rod | −0.6880 | −0.6889 | −0.6384 | −0.5441 | −0.7026 | −0.4749 | −0.5432 | −0.6392 |
| Chute guide rod | −0.6324 | −0.6326 | −0.5842 | −0.4920 | −0.6463 | −0.4252 | −0.4918 | −0.5844 | |
| Different Guide Rod Friction Coefficient | Shock 1 | Shock 2 | Shock 3 | Shock 4 | Shock 5 | Shock 6 | Shock 7 | Shock 8 | |
|---|---|---|---|---|---|---|---|---|---|
| Maximum value | 0.05 | −1.0328 | −0.9319 | −0.8589 | −0.6933 | −1.0016 | −0.6239 | −0.7005 | −0.8453 |
| 0.1 | −0.9020 | −0.8762 | −0.8019 | −0.6908 | −0.9714 | −0.6272 | −0.6996 | −0.8000 | |
| 0.3 | −0.6480 | −0.6019 | −0.6316 | −0.6138 | −0.6187 | −0.7437 | −0.7437 | −0.6060 | |
| 0.5 | −1.2485 | −1.2021 | −1.0969 | −0.7622 | −1.2684 | −0.6276 | −0.8062 | −1.0505 | |
| Average value | 0.05 | −0.6349 | −0.6352 | −0.5845 | −0.4958 | −0.6504 | −0.4190 | −0.4877 | −0.5866 |
| 0.1 | −0.5812 | −0.5894 | −0.5520 | −0.4994 | −0.5897 | −0.4560 | −0.4961 | −0.5524 | |
| 0.3 | −0.1955 | −0.1923 | −0.2313 | −0.2946 | −0.1821 | −0.3462 | −0.3462 | −0.2248 | |
| 0.5 | −0.8120 | −0.7907 | −0.7377 | −0.5550 | −0.8242 | −0.4835 | −0.5953 | −0.7197 | |
| Friction Coefficient | 0.59 (The Original Friction Coefficient of the Wheel) | 0.7 | 0.8 |
|---|---|---|---|
| Max value | 16.14390584 | 15.8970683 | 15.20096503 |
| Slippage ratio | 0.004612545 | 0.00454202 | 0.004343133 |
| Actual Experiment Offset | Simulation Offset | |
|---|---|---|
| Actual operating distance/mm | 2513 | 2513 |
| Offset/mm | 43.7233 | 27.595 |
| Offset ratio | 0.01957 | 0.01098 |
| Friction Coefficient | 0.6 | 0.7 | 0.8 |
| Motion distance/mm | 3500 | 3500 | 3500 |
| Offset/mm | 55.0189 | 33.7013 | 16.6153 |
| Offset ratio | 0.01571938 | 0.009628899 | 0.004746765 |
| Spring Form | Wheelbase | Structure of the Guide Rod | Friction Coefficient of the Guide Rod | Wheel Friction Coefficient | Installation Error |
|---|---|---|---|---|---|
| Stainless steel spring with the wire diameter of 1.4 mm | Original wheelbase +40 mm | Chute guide rod | 0.3 | 0.8 | Optimized |
| Simulation Environment | Shock 1 | Shock 2 | Shock 3 | Shock 4 | Shock 5 | Shock 6 | Shock 7 | Shock 8 | Average Value | |
|---|---|---|---|---|---|---|---|---|---|---|
| Mean value | Optimal simulation | 0.5162 | 0.5151 | 0.4748 | 0.3943 | 0.5274 | 0.3376 | 0.3954 | 0.4737 | 0.4543 |
| Original simulation | 0.6899 | 0.6904 | 0.6397 | 0.6397 | 0.7045 | 0.4741 | 0.5433 | 0.6402 | 0.6277 | |
| Peak value | Optimal simulation | 1.0300 | 1.0078 | 0.8693 | 0.7211 | 1.0343 | 0.6911 | 0.6871 | 0.8742 | 0.8644 |
| Original simulation | 1.0600 | 1.0239 | 0.9490 | 0.7249 | 1.0583 | 0.6160 | 0.7458 | 0.9214 | 0.8874 |
| Simulation Environment | Acceleration 1 | Acceleration 2 | Acceleration 3 | Acceleration 4 | Acceleration 5 | Acceleration 6 | Average Value | |
|---|---|---|---|---|---|---|---|---|
| Mean value | Optimal simulation | 0.0055 | 0.0073 | 0.0026 | 0.0012 | 0.0073 | 0.0062 | 0.0050 |
| Original simulation | 0.0162 | 0.0165 | 0.0126 | 0.0061 | 0.0174 | 0.0009 | 0.0116 | |
| Peak value | Optimal simulation | 2.1633 | 2.1327 | 1.5423 | 1.3219 | 2.3245 | 2.2952 | 1.9633 |
| Original simulation | 3.7449 | 3.0408 | 2.9490 | 2.3061 | 3.3061 | 1.7857 | 2.8554 |
| Original Simulation | Optimal Simulation | |
|---|---|---|
| Motion distance/mm | 3500 | 3500 |
| Slippage amount/mm | 16.12 | 8.36 |
| Slippage ratio | 0.004605714 | 0.002388571 |
| Original Simulation | Optimal Simulation | |
|---|---|---|
| Motion distance/mm | 3500 | 3500 |
| Offset/mm | 55.0189 | 13.96 |
| Offset ratio | 0.01571938 | 0.003988571 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, X.; Xie, B.; Yang, Y.; Liu, Y.; Jiang, P. Research on Running Performance Optimization of Four-Wheel-Driving Ackerman Chassis by the Combining Method of Quantitative Experiment with Dynamic Simulation. Machines 2024, 12, 198. https://doi.org/10.3390/machines12030198
Zhang X, Xie B, Yang Y, Liu Y, Jiang P. Research on Running Performance Optimization of Four-Wheel-Driving Ackerman Chassis by the Combining Method of Quantitative Experiment with Dynamic Simulation. Machines. 2024; 12(3):198. https://doi.org/10.3390/machines12030198
Chicago/Turabian StyleZhang, Xiangyu, Bowen Xie, Yang Yang, Yongbin Liu, and Pan Jiang. 2024. "Research on Running Performance Optimization of Four-Wheel-Driving Ackerman Chassis by the Combining Method of Quantitative Experiment with Dynamic Simulation" Machines 12, no. 3: 198. https://doi.org/10.3390/machines12030198
APA StyleZhang, X., Xie, B., Yang, Y., Liu, Y., & Jiang, P. (2024). Research on Running Performance Optimization of Four-Wheel-Driving Ackerman Chassis by the Combining Method of Quantitative Experiment with Dynamic Simulation. Machines, 12(3), 198. https://doi.org/10.3390/machines12030198





