Equivalent Continuum Modeling for Flexible Slender Quadrilateral Truss Structure
Abstract
1. Introduction
2. Equivalent Method for Timoshenko Beam
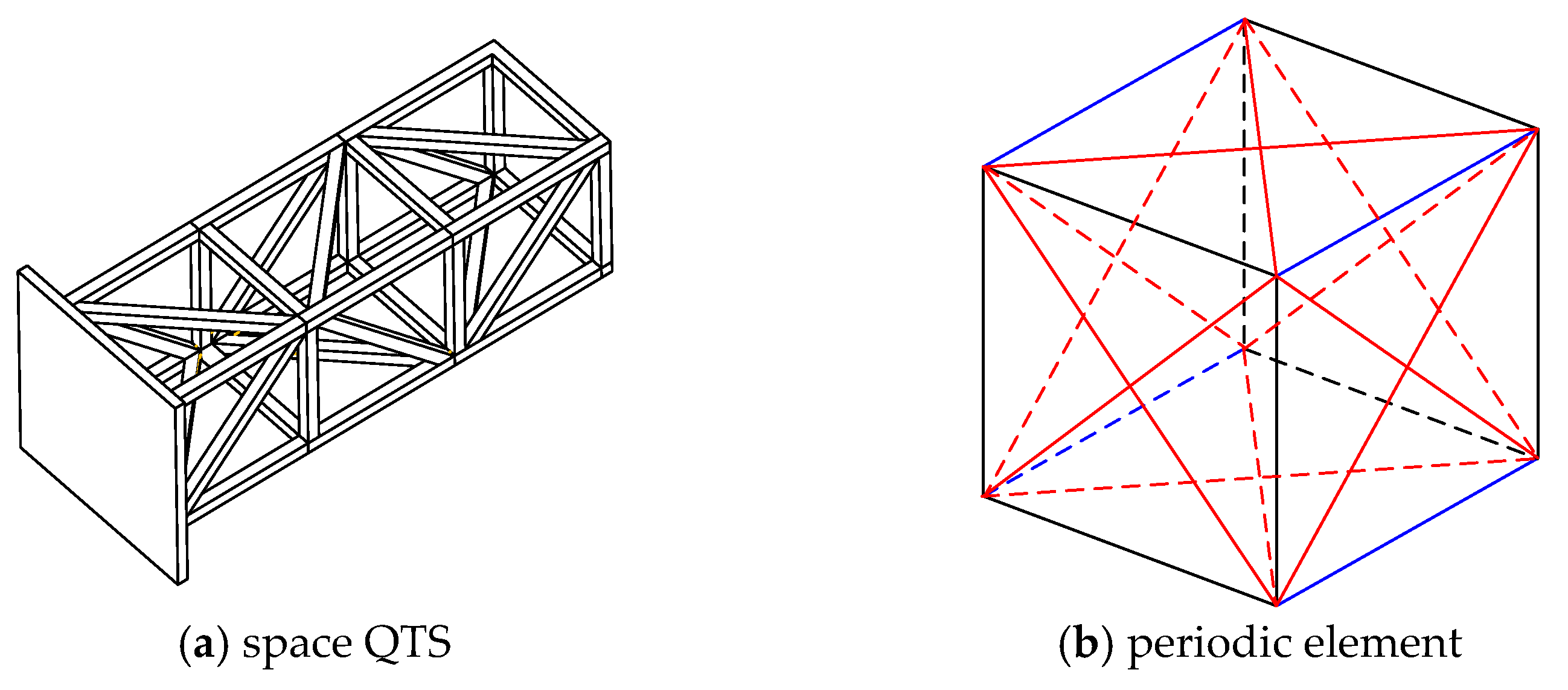
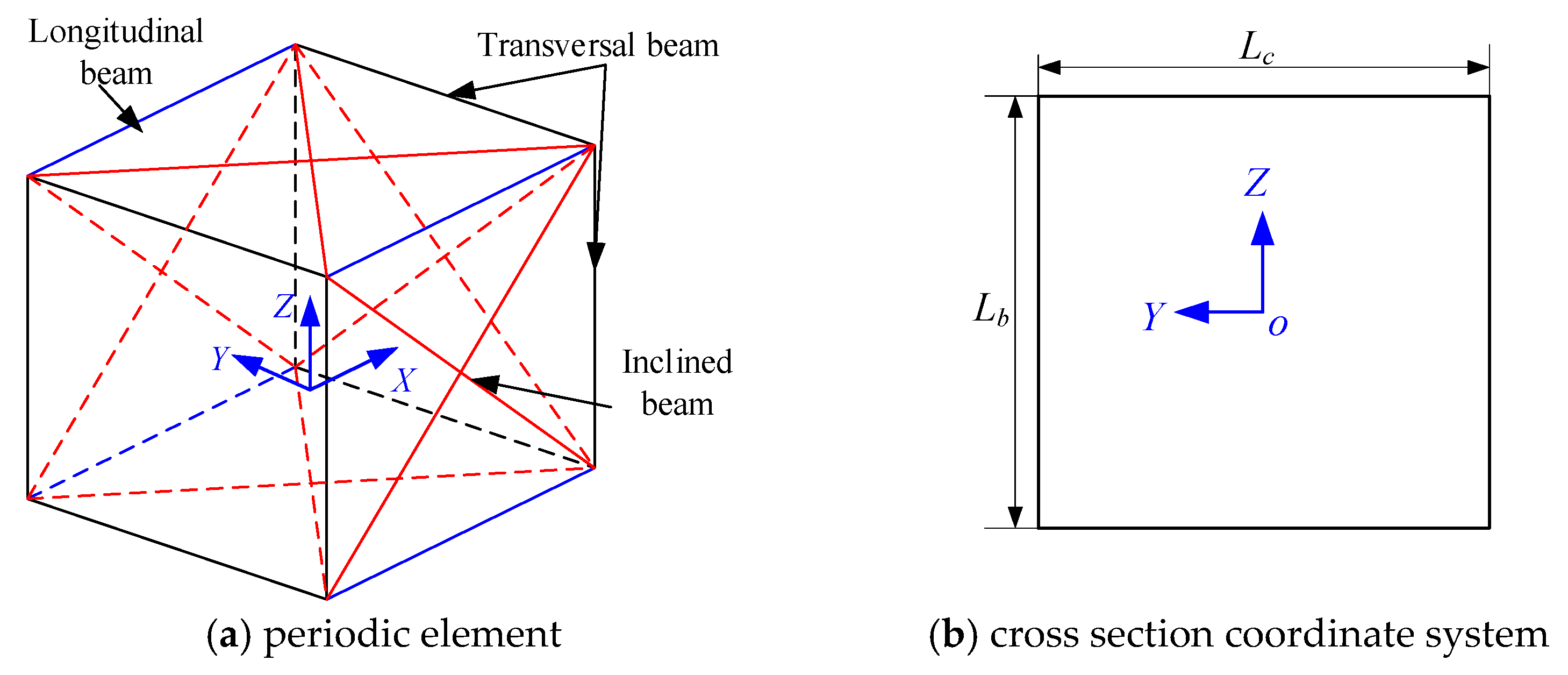
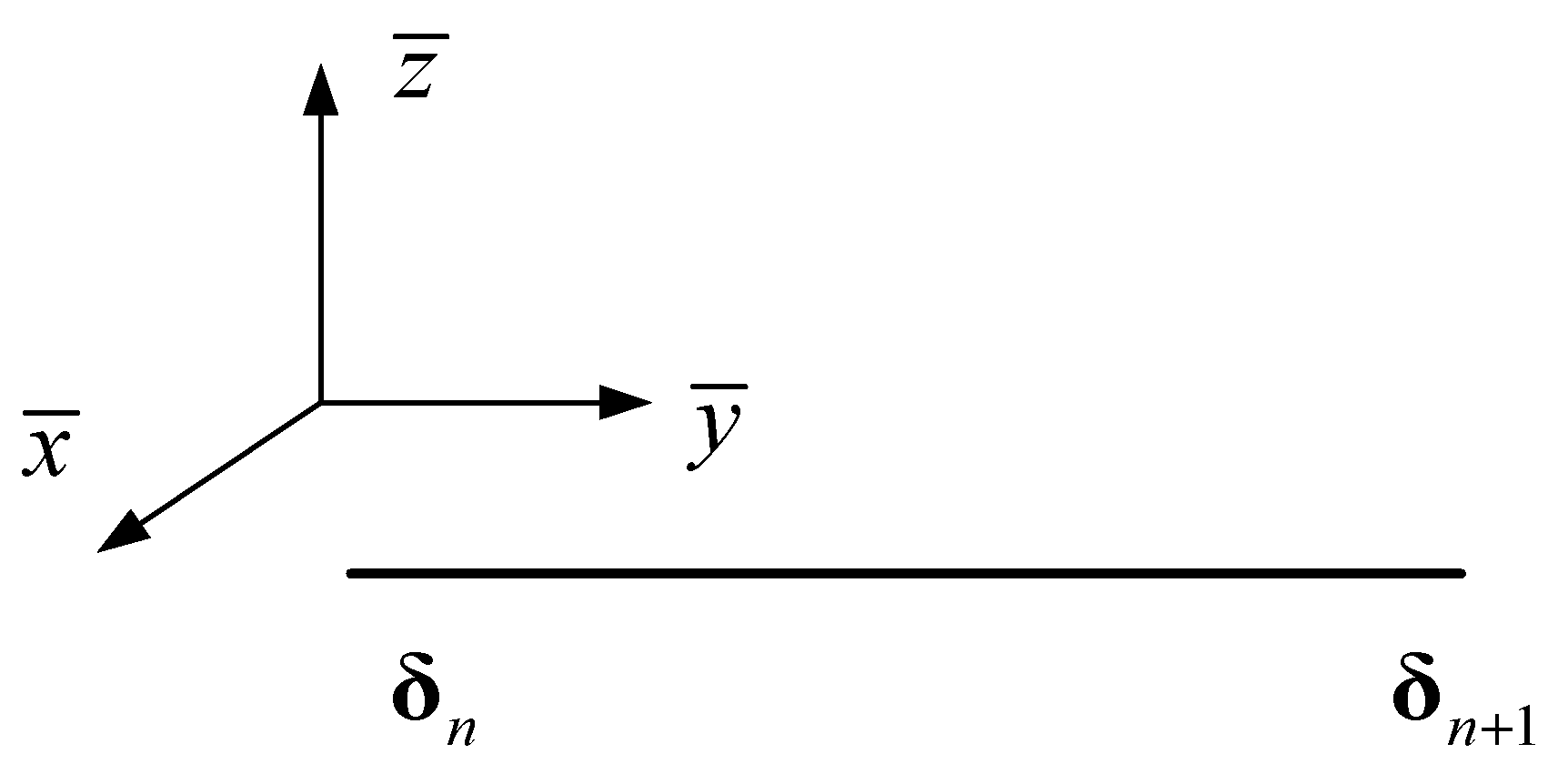
2.1. Displacement Mode of the Periodic Element
2.2. Elastic Potential and Kinetic Energy of the Periodic Element
2.3. Elastic Potential Energy and Kinetic Energy of an Equivalent Beam
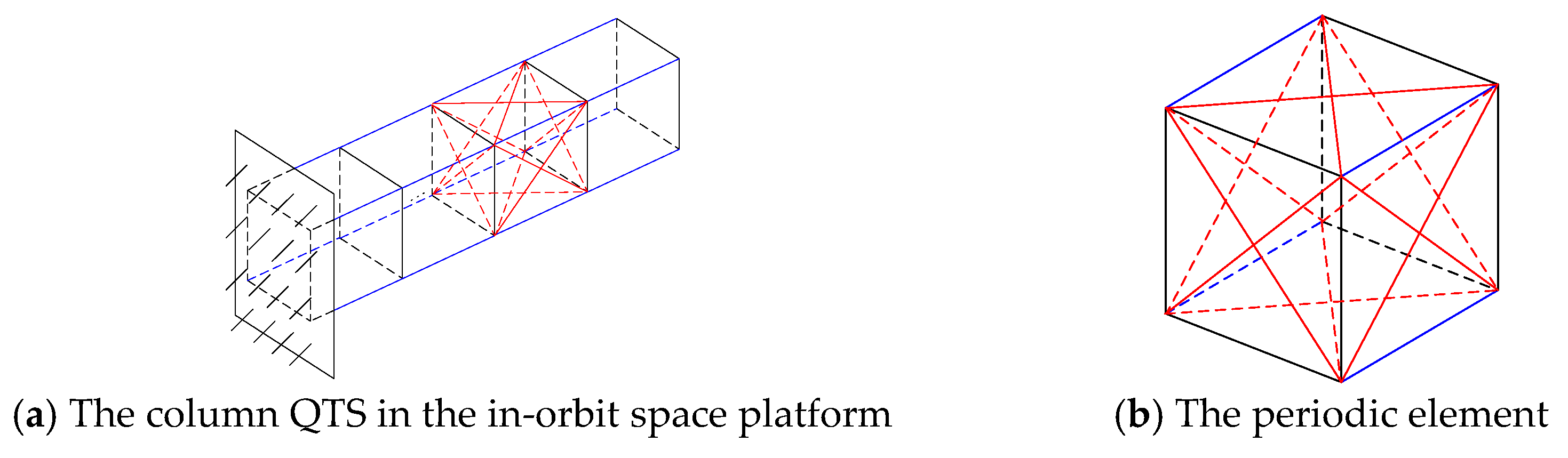
3. Equivalent Beam Model of QTS
3.1. Equivalent Beam Model Parameters of QTS
3.2. FE Discretization of Equivalent Beam
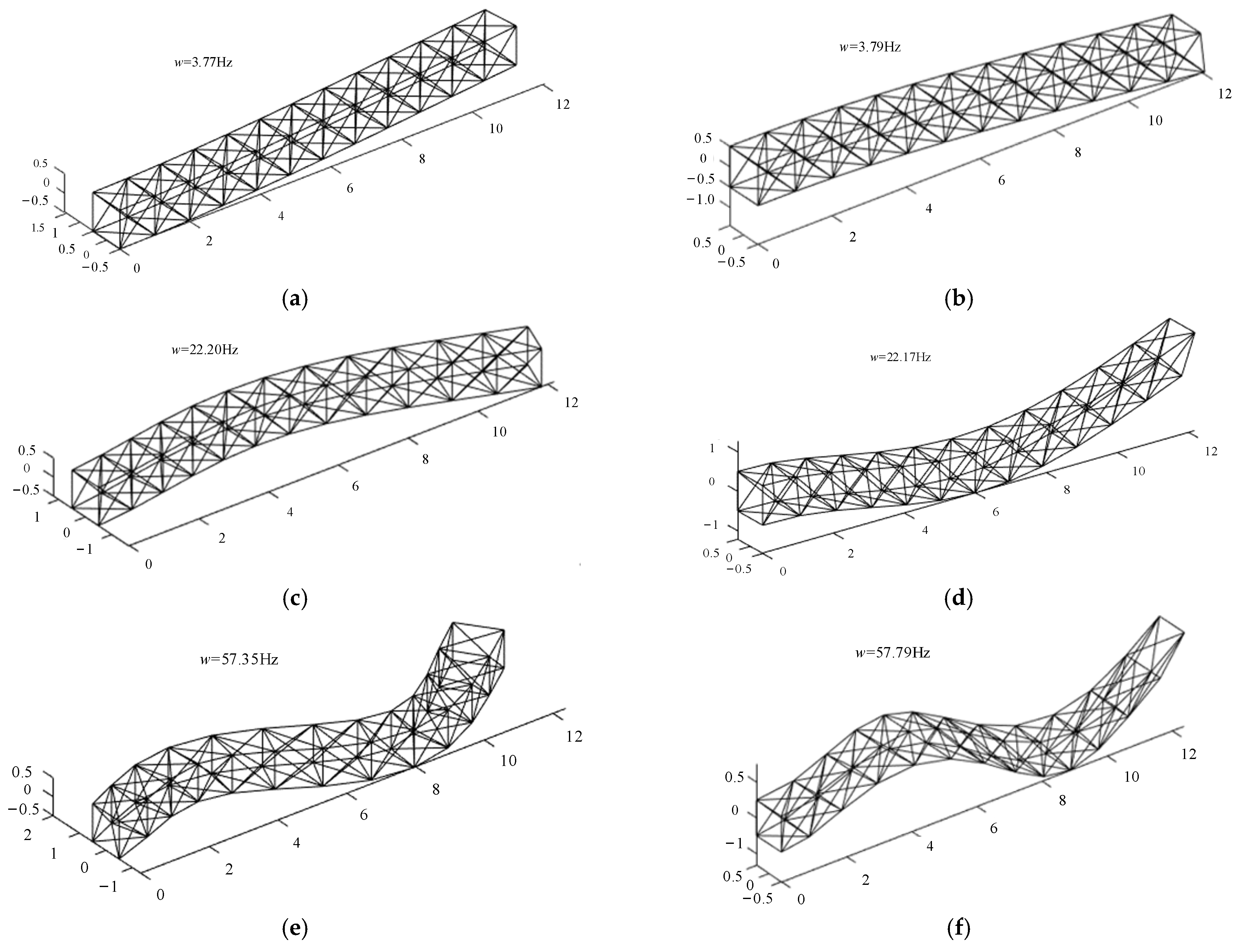
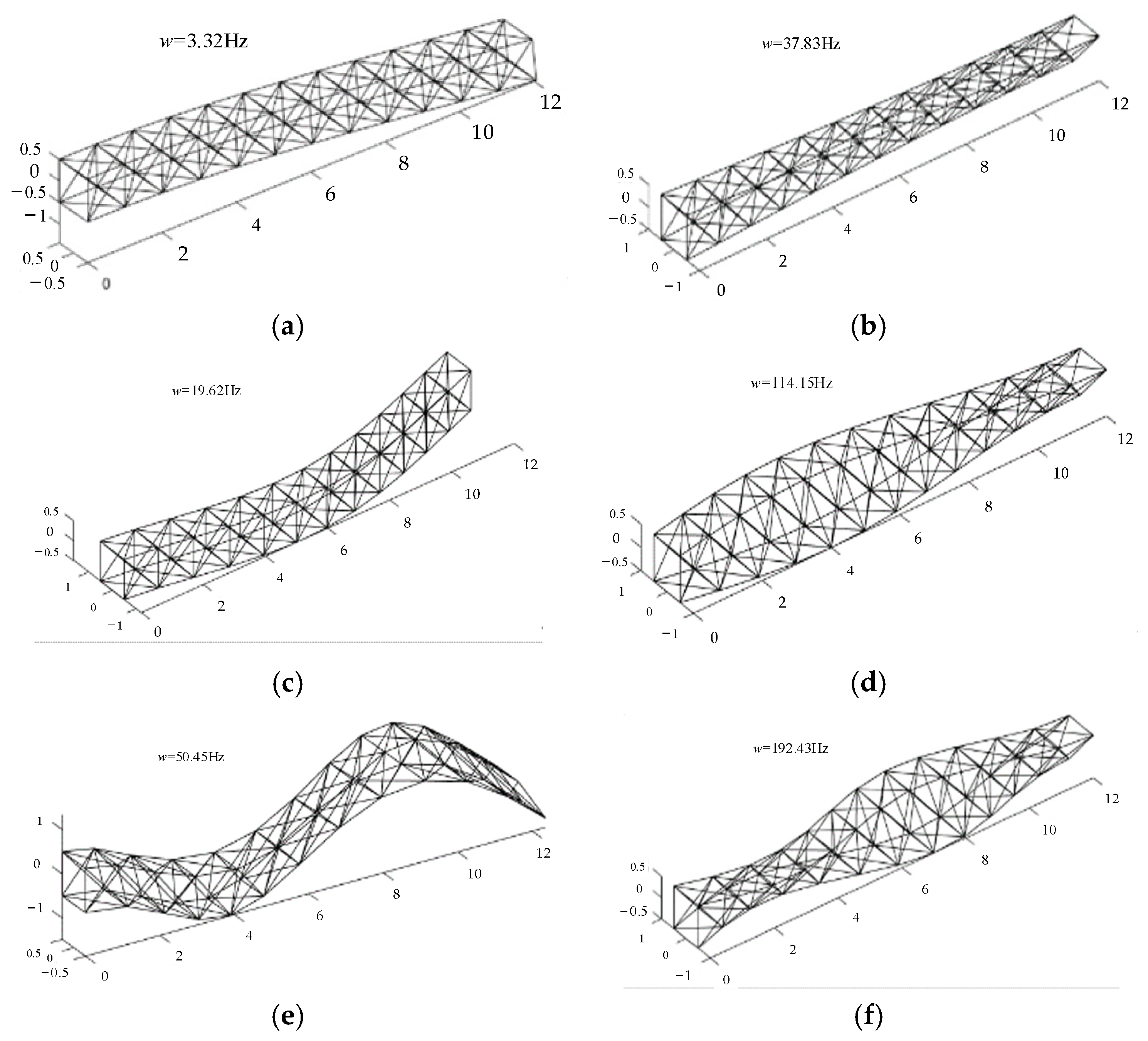
3.3. Modal Analysis Based on the FE Method
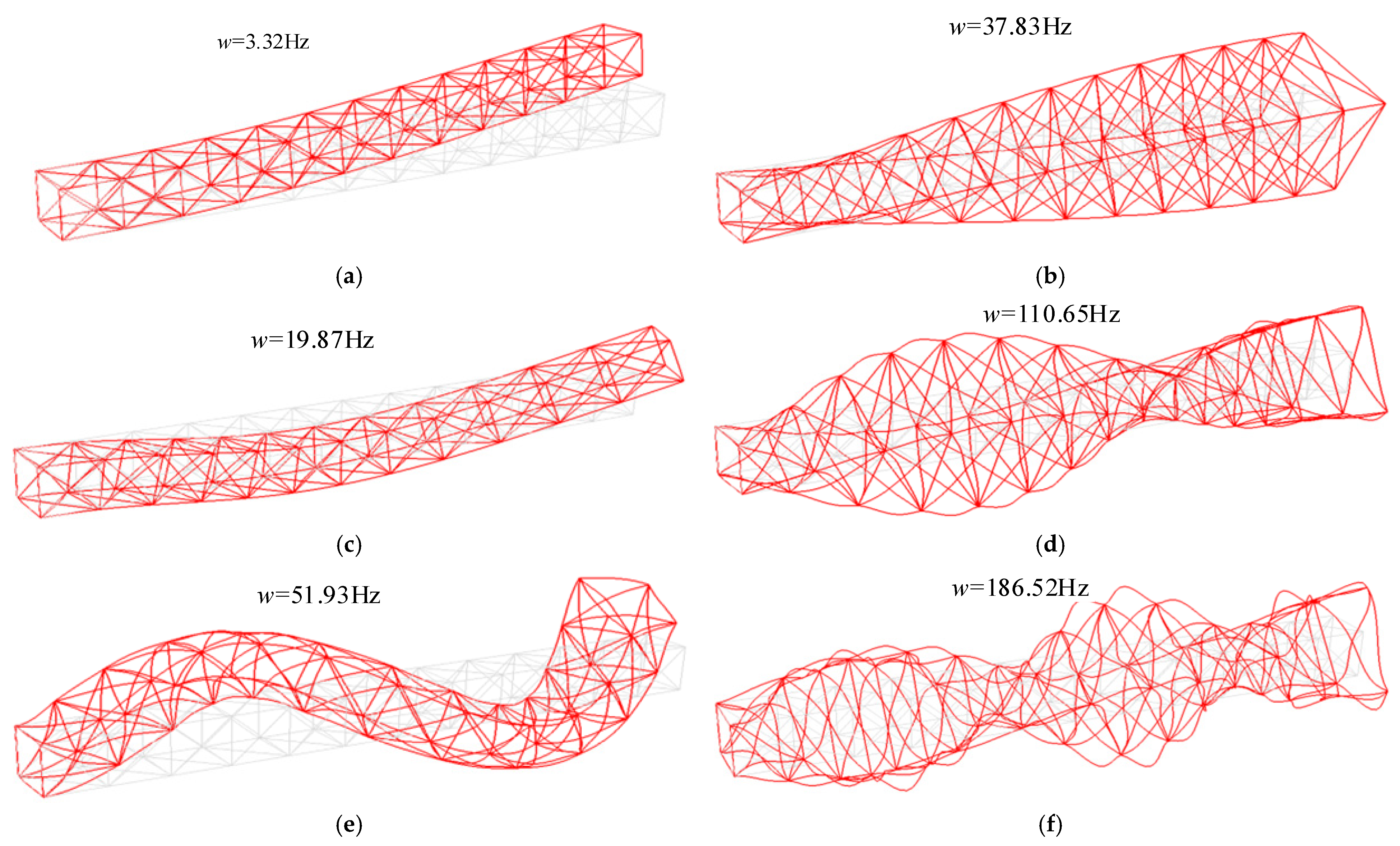
3.4. Modification of the Equivalent Beam Model
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Torabi, J.; Niiranen, J. Nonlinear finite element free and forced vibrations of cellular plates having lattice-type metamaterial cores: A strain gradient plate model approach. Mech. Syst. Signal Process. 2023, 192, 110224. [Google Scholar] [CrossRef]
- Arndt, M.; Machado, R.D.; Scremin, A. An adaptive generalized finite element method applied to free vibration analysis of straight bars and trusses. J. Sound Vib. 2010, 329, 659–672. [Google Scholar] [CrossRef]
- Chung, J.; Yoo, H.H. Dynamic analysis of a rotating cantilever beam by using the finite element method. J. Sound Vib. 2002, 249, 147–164. [Google Scholar] [CrossRef]
- Stoltani, Z.; Santer, M. The determination and enhancement of compliant modes for high-amplitude actuation in lattices. Int. J. Solids Struct. 2020, 206, 124–136. [Google Scholar] [CrossRef]
- Noor, A.K.; Nemeth, M.P. Analysis of spatial beam like lattices with rigid joints. Comput. Methods Appl. Mech. Eng. 1980, 24, 35–59. [Google Scholar] [CrossRef]
- Noor, A.K.; Nemeth, M.P. Micropolar beam models for lattice grids with rigid joints. Comput. Methods Appl. Mech. Eng. 1980, 21, 249–263. [Google Scholar] [CrossRef]
- Noor, A.K.; Anderson, M.S.; Greene, W.H. Continuum models for beam–and platelike lattice structures. AIAA J. 1978, 16, 1219–1228. [Google Scholar] [CrossRef]
- Penta, F.; Monaco, M.; Pucillo, G.P.; Gesualdo, A. Periodic beam–like structures homogenization by transfer matrix eigen–analysis: A direct approach. Mech. Res. Commun. 2017, 85, 81–88. [Google Scholar] [CrossRef]
- Weeger, O.; Boddeti, N.; Yeung, S.K.; Kaijima, S.; Dunn, M.L. Digital design and nonlinear simulation for additive manufacturing of soft lattice structures. Addit. Manuf. 2019, 25, 39–49. [Google Scholar] [CrossRef]
- Salehian, A.; Lbrahim, M.; Seigler, T.M. Damping in periodic structures: A continuum modeling approach. AIAA J. 2014, 52, 569–590. [Google Scholar] [CrossRef]
- Liu, F.; Wang, L.; Jin, D.; Liu, X.; Lu, P. Equivalent beam model for spatial repetitive lattice structures with hysteretic nonlinear joints. Int. J. Mech. Sci. 2021, 200, 106449. [Google Scholar] [CrossRef]
- Yildiz, K.; Lesieutre, G.A. Deployment of n-strut cylindrical tensegrity booms. J. Struct. Eng. 2020, 146, 04020247. [Google Scholar] [CrossRef]
- Koohestani, K. Novel topological and geometrical modeling of N-frequency geodesic icosahedron tensegrities. Eng. Comput. 2022, 38, 5733–5745. [Google Scholar] [CrossRef]
- Hrabacka, M.; Bulin, R.; Hajzman, M. New actuation planning method for the analysis and design of active tensegrity structures. Eng. Struct. 2023, 293, 116597. [Google Scholar] [CrossRef]
- Penta, F.; Esposito, F.; Pucillo, G.P.; Rosiello, V.; Gesualdo, A. On the homogenization of periodic beam-like structures. Procedia Struct. Integr. 2018, 8, 399–409. [Google Scholar] [CrossRef]
- Karttunen, A.T.; Reddy, J.N.; Romanoff, J. Two-scale constitutive modeling of a lattice core sandwich beam. Compos. Part B Eng. 2019, 160, 66–75. [Google Scholar] [CrossRef]
- Finozzi, A.; Sanfedino, F.; Alazard, D. Parametric sub-structuring models of large space truss structures for structure/control co-design. Mech. Syst. Signal Process. 2022, 180, 109427. [Google Scholar] [CrossRef]
- Sanfedino, F.; Alazard, D.; Pommier-Budinger, V.; Falcoz, A.; Boquet, F. Finite element based N-port model for preliminary design of multibody systems. J. Sound Vib. 2018, 415, 128–146. [Google Scholar] [CrossRef]
- Yang, H.; Feng, J.; Wang, Y.; Xiao, H.; Liu, Y.B. Equivalent dynamic model for large parabolic cylindrical deployable mechanism. AIAA J. 2022, 60, 6739–6752. [Google Scholar] [CrossRef]
- Zhang, G.W.; Liu, F.S. Equivalent dynamic modeling for the relative rotation of bolted joint interface using valanis model of hysteresis. Machines 2023, 11, 342. [Google Scholar] [CrossRef]
- Liu, F.S.; Wang, L.B.; Jin, D.P.; Liu, X.; Lu, P. Equivalent micropolar beam for spatial vibration analysis of planar repetitive truss structure with flexible joints. Int. J. Mech. Sci. 2020, 165, 105202. [Google Scholar] [CrossRef]


| Material Parameter | Value |
|---|---|
| Yong modulus E/Gpa | 207 |
| Density ρ kg/m3 | 7830 |
| Poisson’s ratio v | 0.3 |
| Mode | Order | Frequencies /Hz | RE1 % | |
|---|---|---|---|---|
| The FE Model | The Equivalent Beam Model | |||
| Transverse bend | 1 | 3.31 | 3.77 | 14.03 |
| 2 | 19.87 | 22.17 | 11.58 | |
| 3 | 51.93 | 56.79 | 9.37 | |
| Vertical bend | 1 | 3.31 | 3.79 | 14.78% |
| 2 | 19.87 | 22.20 | 11.73% | |
| 3 | 51.93 | 57.35 | 10.43% | |
| Torsional | 1 | 37.83 | 36.67 | 3.06 |
| 2 | 112.45 | 110.65 | 1.60 | |
| 3 | 183.76 | 186.52 | 1.50 | |
| Axial tension and compression | 1 | 43.13 | 42.94 | 0.43 |
| Mode | Order | Frequency/Hz | RE1 % | The Modified Equivalent Beam Model | RE2 % | |
|---|---|---|---|---|---|---|
| The FE Model | The Initial Equivalent Beam Model | |||||
| Bend | 1 | 3.32 | 3.77 | 14.03 | 3.32 | 0.32 |
| 2 | 19.87 | 22.17 | 11.58 | 19.54 | 1.67 | |
| 3 | 51.93 | 56.79 | 9.37 | 50.44 | 2.87 | |
| Twist | 1 | 37.83 | 36.67 | 3.06 | 37.83 | 0.01 |
| 2 | 112.45 | 110.65 | 1.60 | 114.14 | 1.50 | |
| 3 | 183.76 | 186.52 | 1.50 | 192.40 | 4.70 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, B.; Wang, Y.; Liu, Y.; Tao, J.; Ren, H.; Yang, H. Equivalent Continuum Modeling for Flexible Slender Quadrilateral Truss Structure. Machines 2024, 12, 111. https://doi.org/10.3390/machines12020111
Li B, Wang Y, Liu Y, Tao J, Ren H, Yang H. Equivalent Continuum Modeling for Flexible Slender Quadrilateral Truss Structure. Machines. 2024; 12(2):111. https://doi.org/10.3390/machines12020111
Chicago/Turabian StyleLi, Bo, Yan Wang, Yipeng Liu, Jianguo Tao, Hui Ren, and Hui Yang. 2024. "Equivalent Continuum Modeling for Flexible Slender Quadrilateral Truss Structure" Machines 12, no. 2: 111. https://doi.org/10.3390/machines12020111
APA StyleLi, B., Wang, Y., Liu, Y., Tao, J., Ren, H., & Yang, H. (2024). Equivalent Continuum Modeling for Flexible Slender Quadrilateral Truss Structure. Machines, 12(2), 111. https://doi.org/10.3390/machines12020111






