Analysis of the Nonlinear Complex Response of Cracked Blades at Variable Rotational Speeds
Abstract
1. Introduction
2. Dynamic Modeling of Variable Speed Cracked Blade
2.1. FE Modeling of Variable Speed Turbine Blades with Edge Penetration Crack
2.2. CMS–Solid Method for Cracked Blade at Time-Varying Rotational Speeds
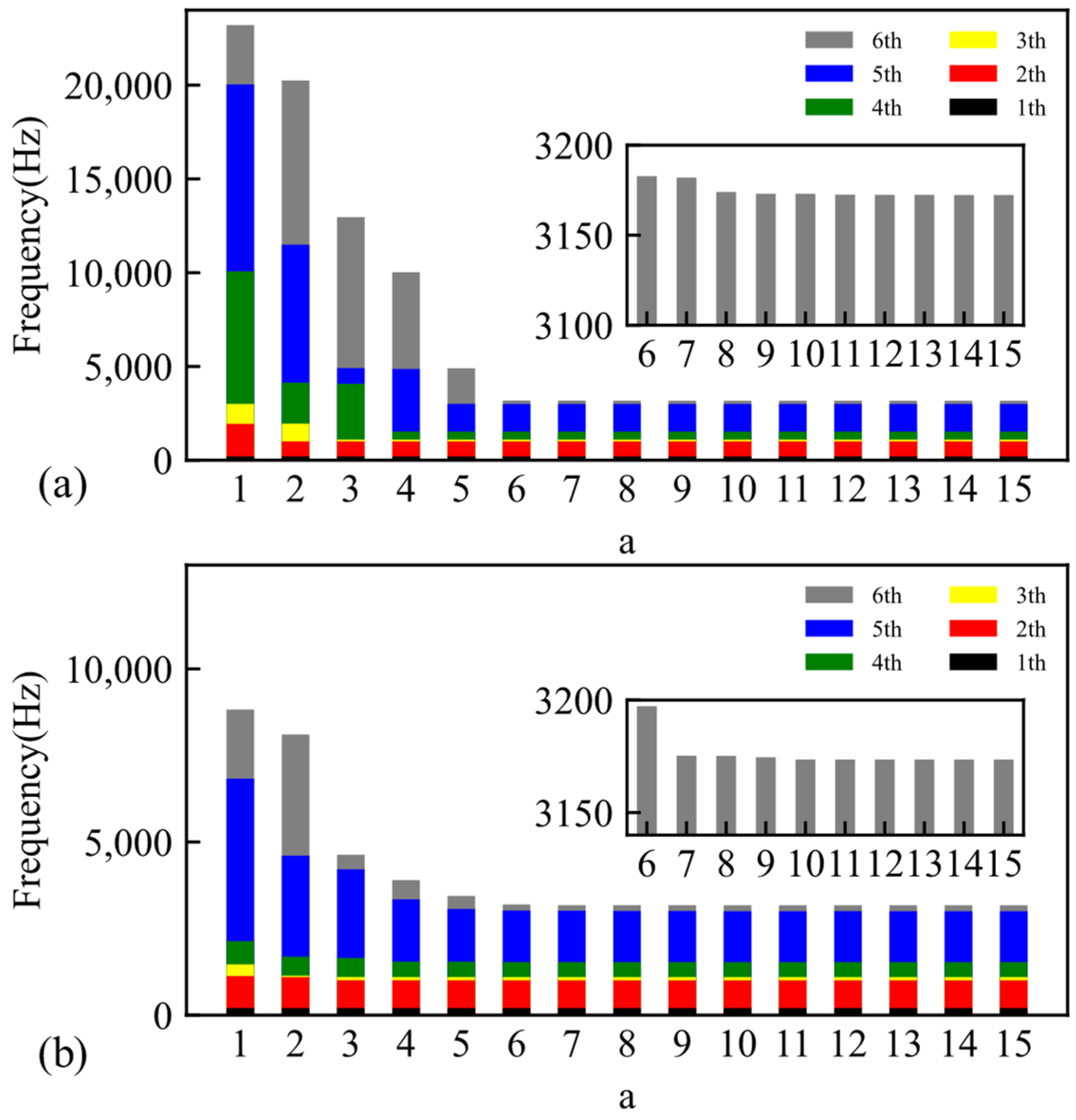
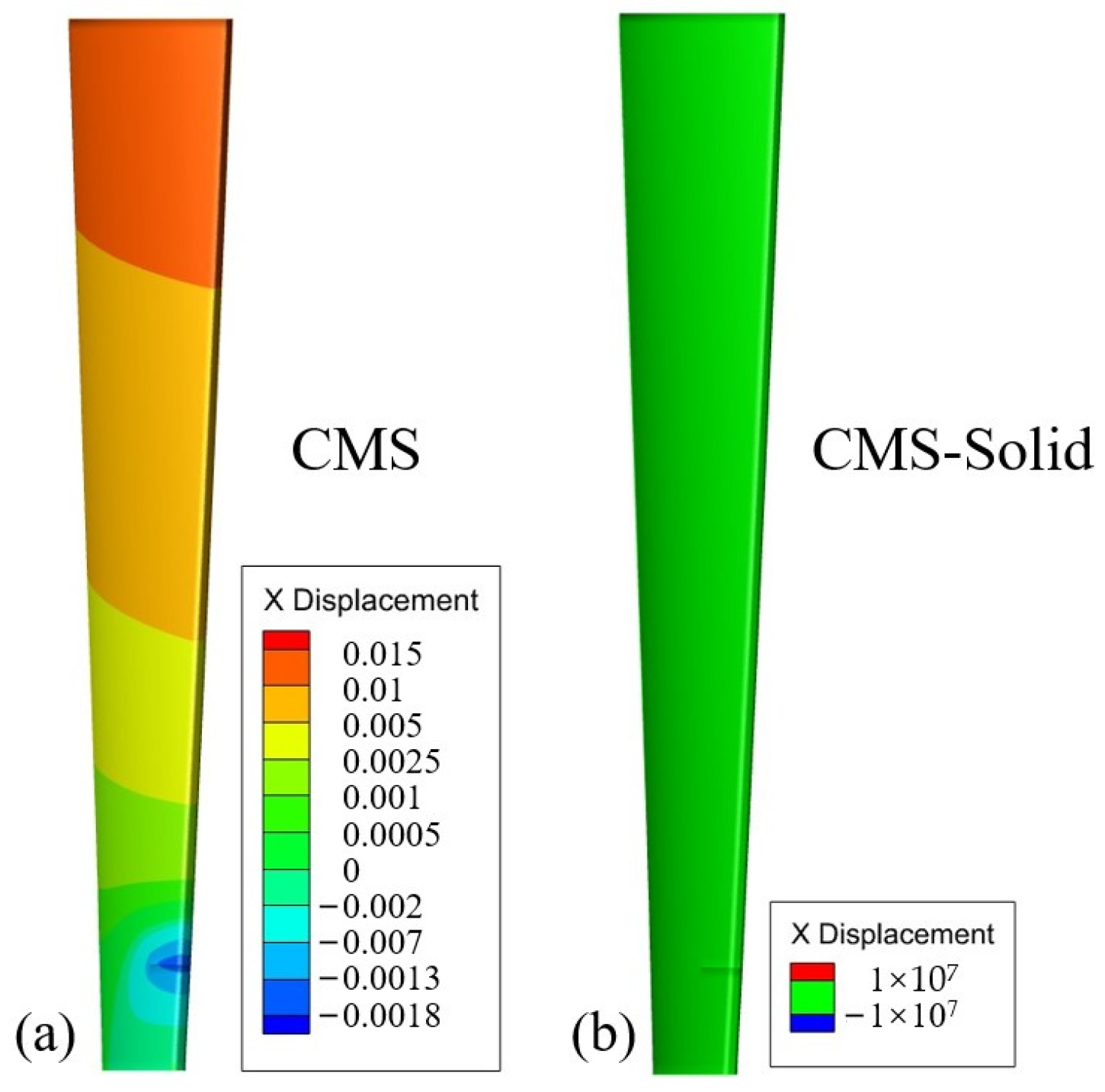
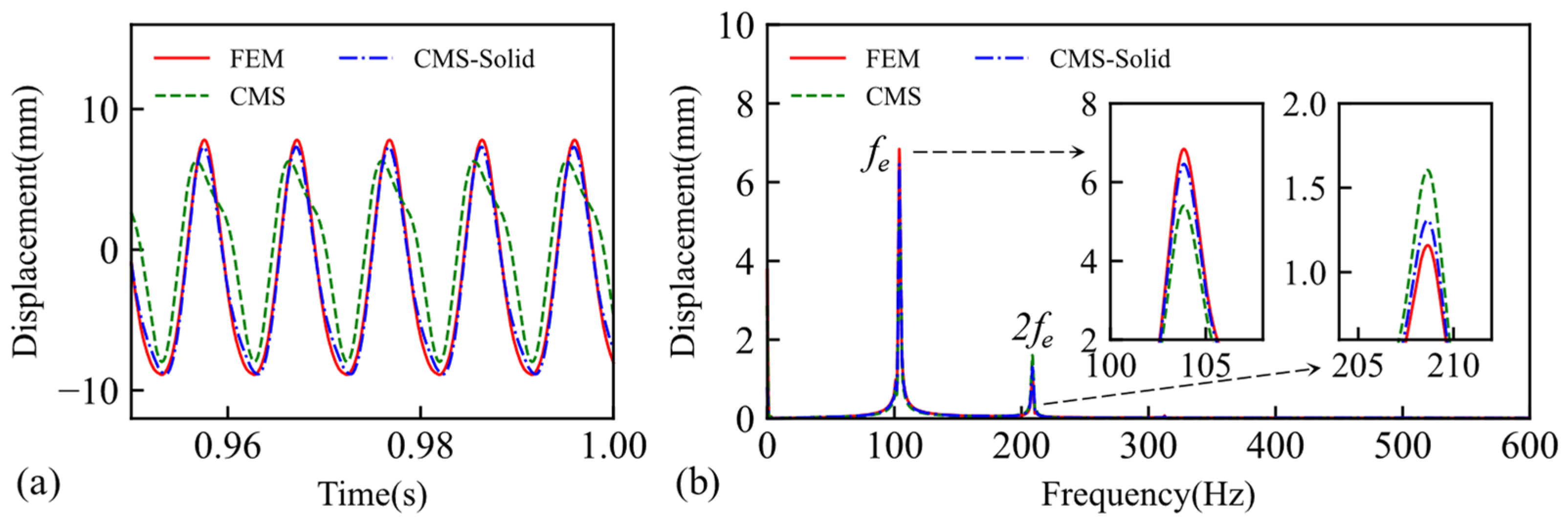
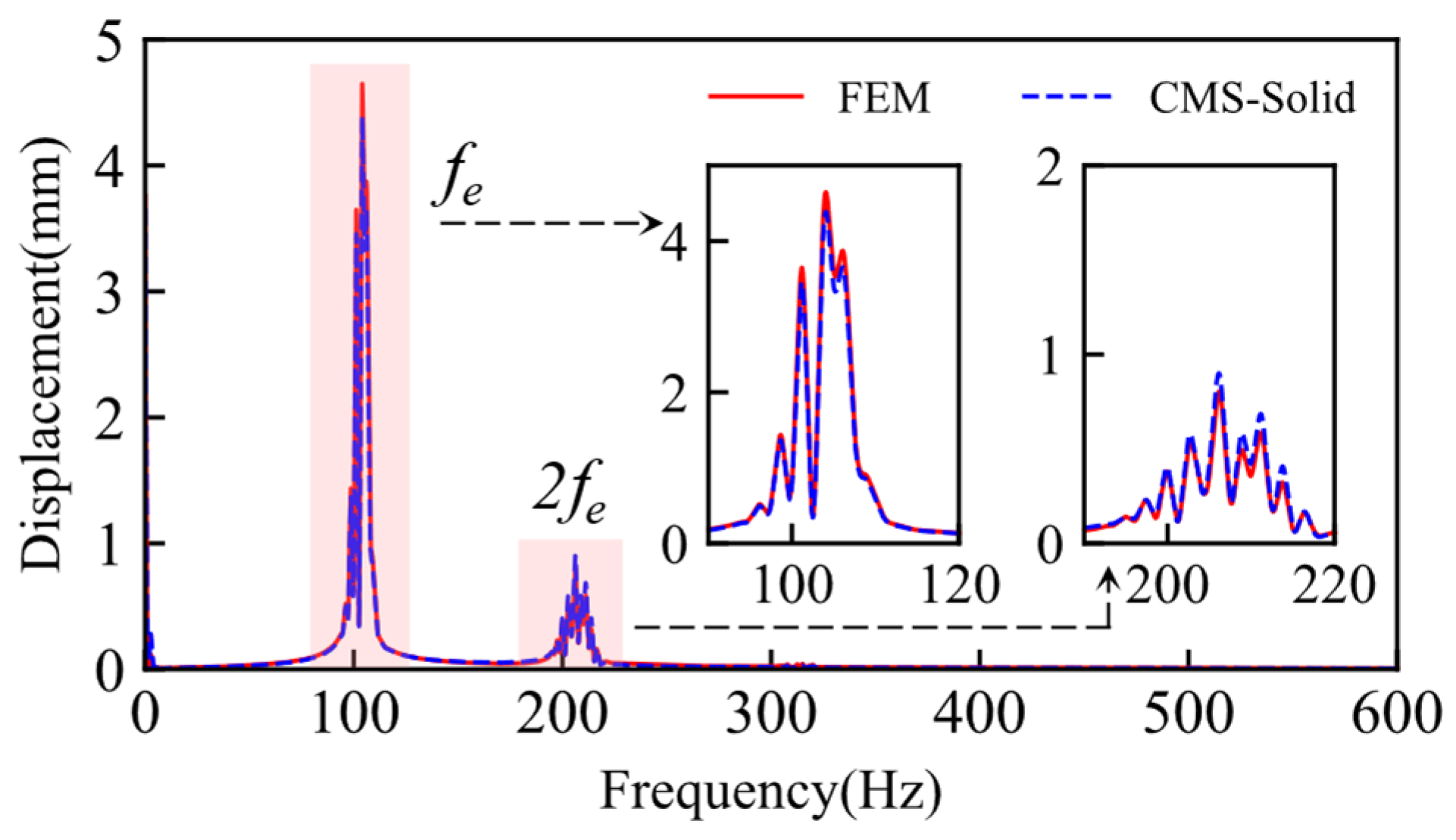
2.3. Model Verification
3. Characterisation of the Variable Speed Cracked Blade Vibrations
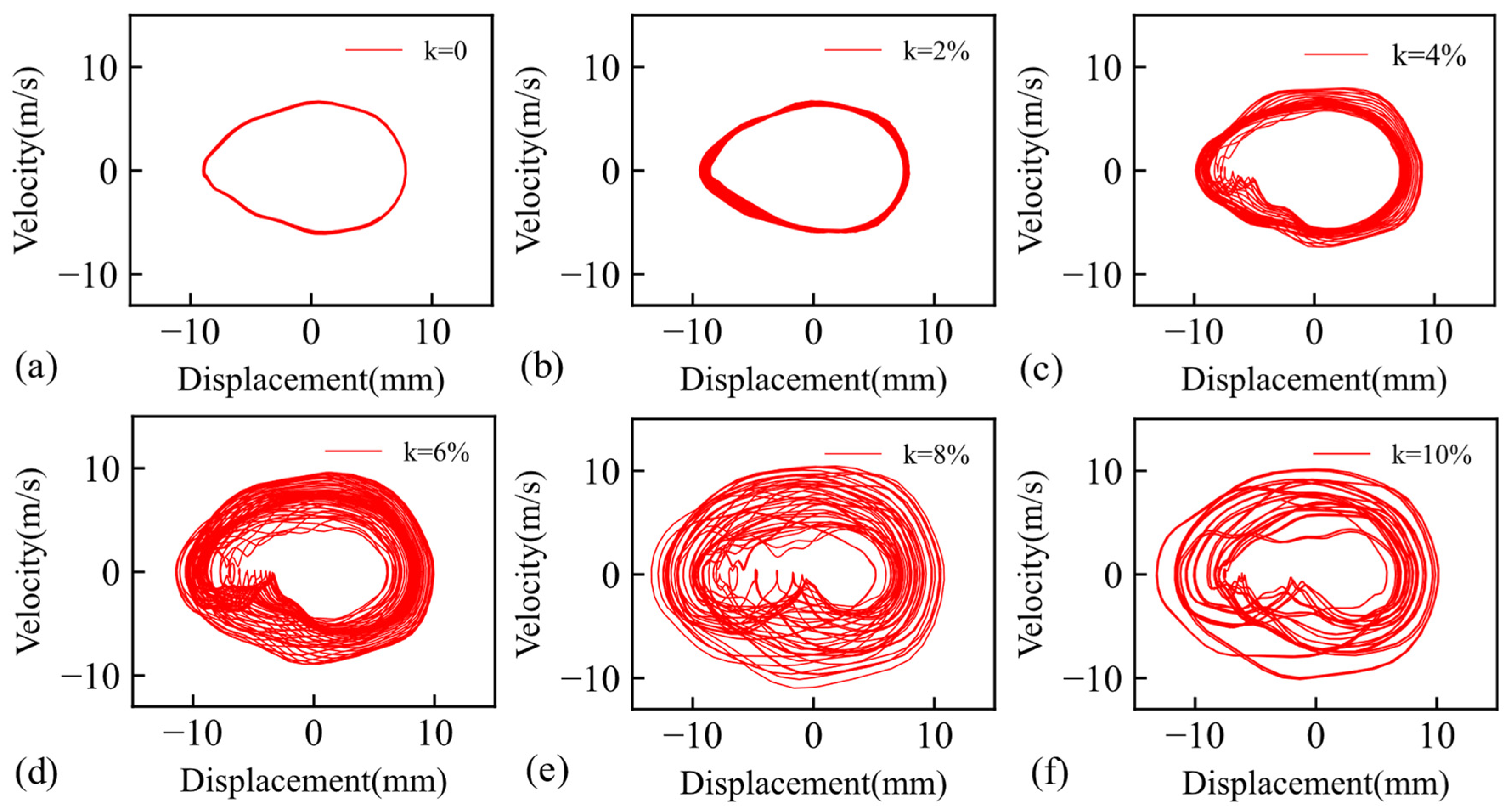
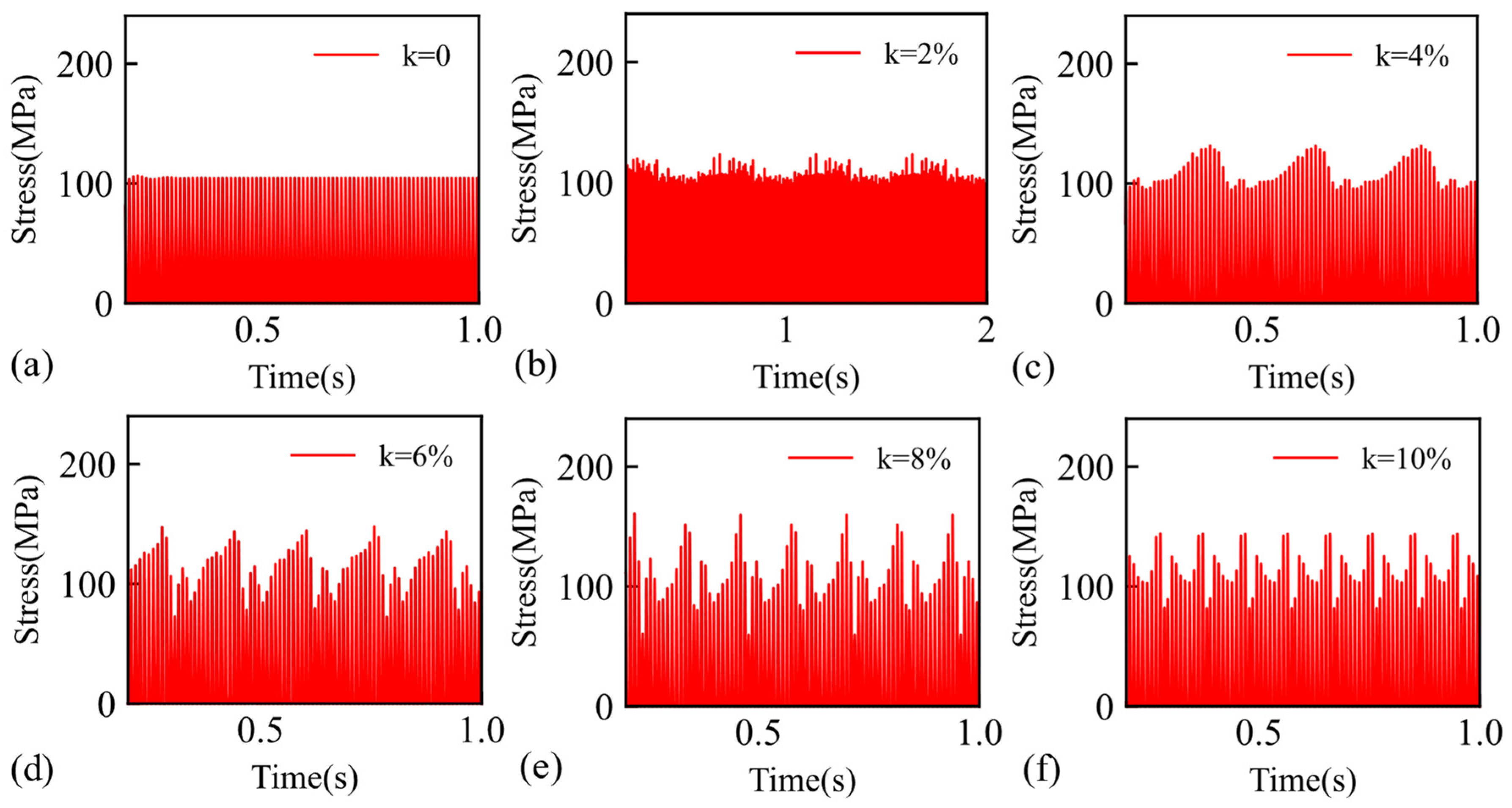
3.1. Effects of Closed-Loop Speed Perturbations
3.2. Effects of Speed Disturbances Due to Disc Shaft Failures
4. Conclusions
- For cracked blades subjected to closed-loop rotational speed perturbation control, a competition exists between the effects of rotational speed changes and unbalanced excitation on crack nonlinearity. In scenarios with low perturbations, the variation in rotational speed is the dominant factor. Conversely, at higher perturbation levels, aerodynamic excitation becomes the primary influence. When the cracked blade experiences control by fault speed disturbance, the response is predominantly characterised by the effects of crack super-harmonic nonlinearity under aerodynamic excitation.
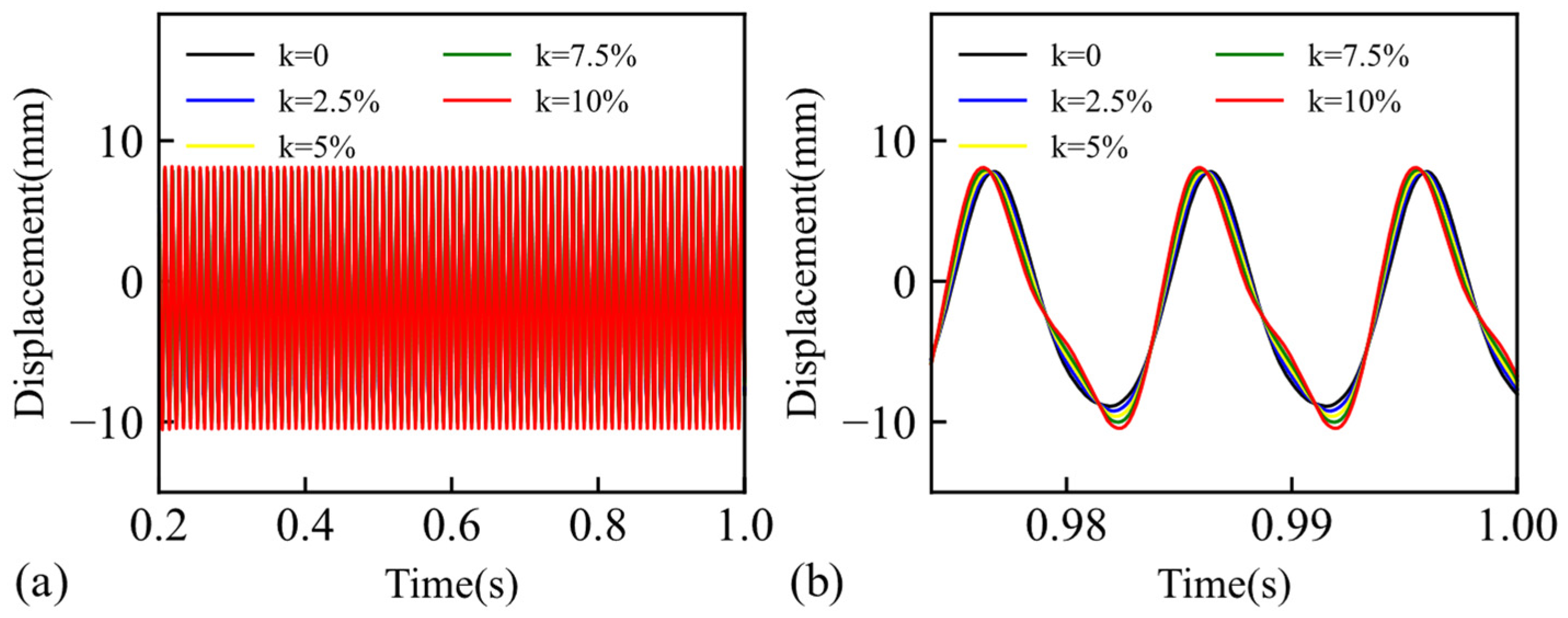
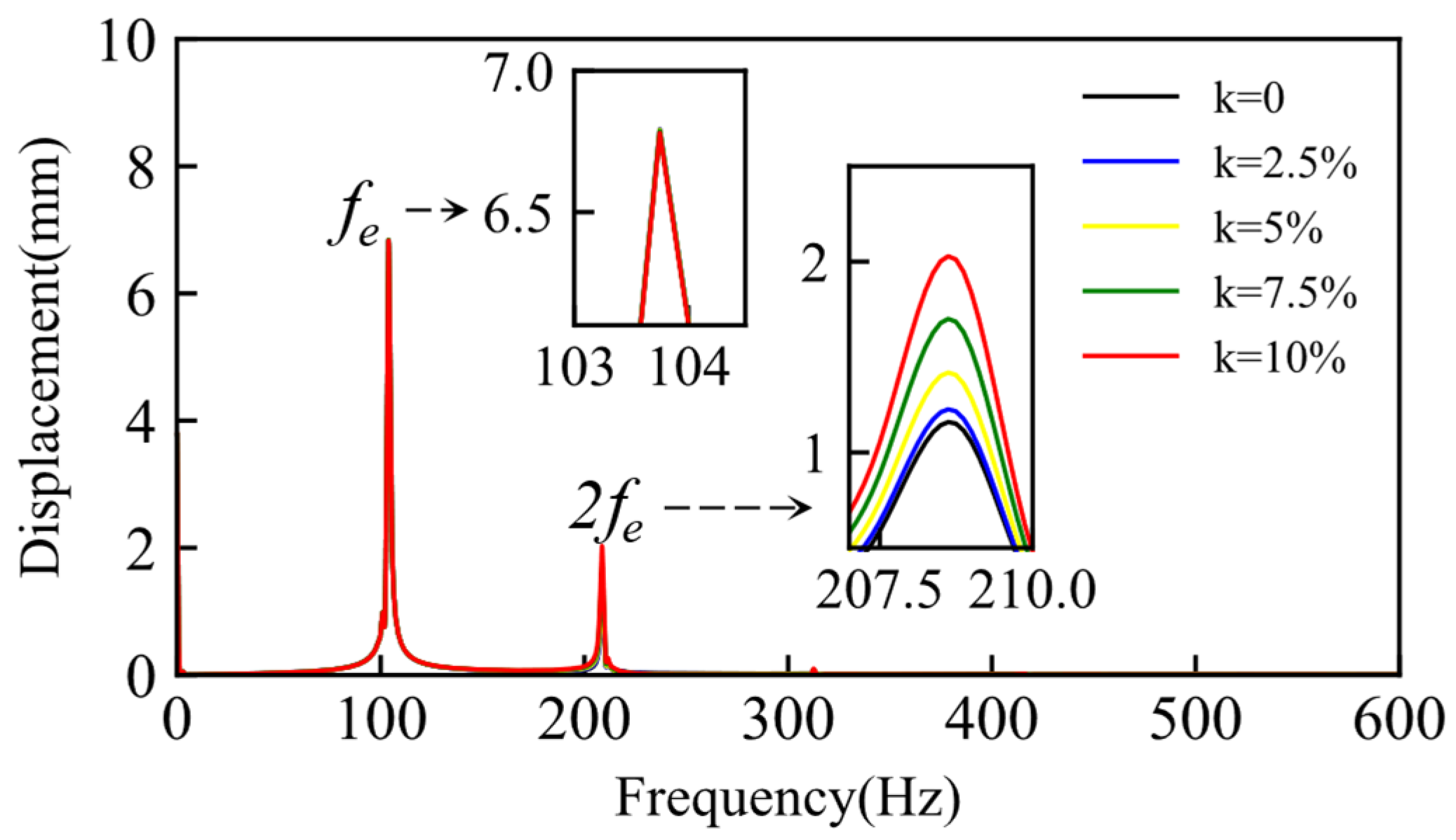
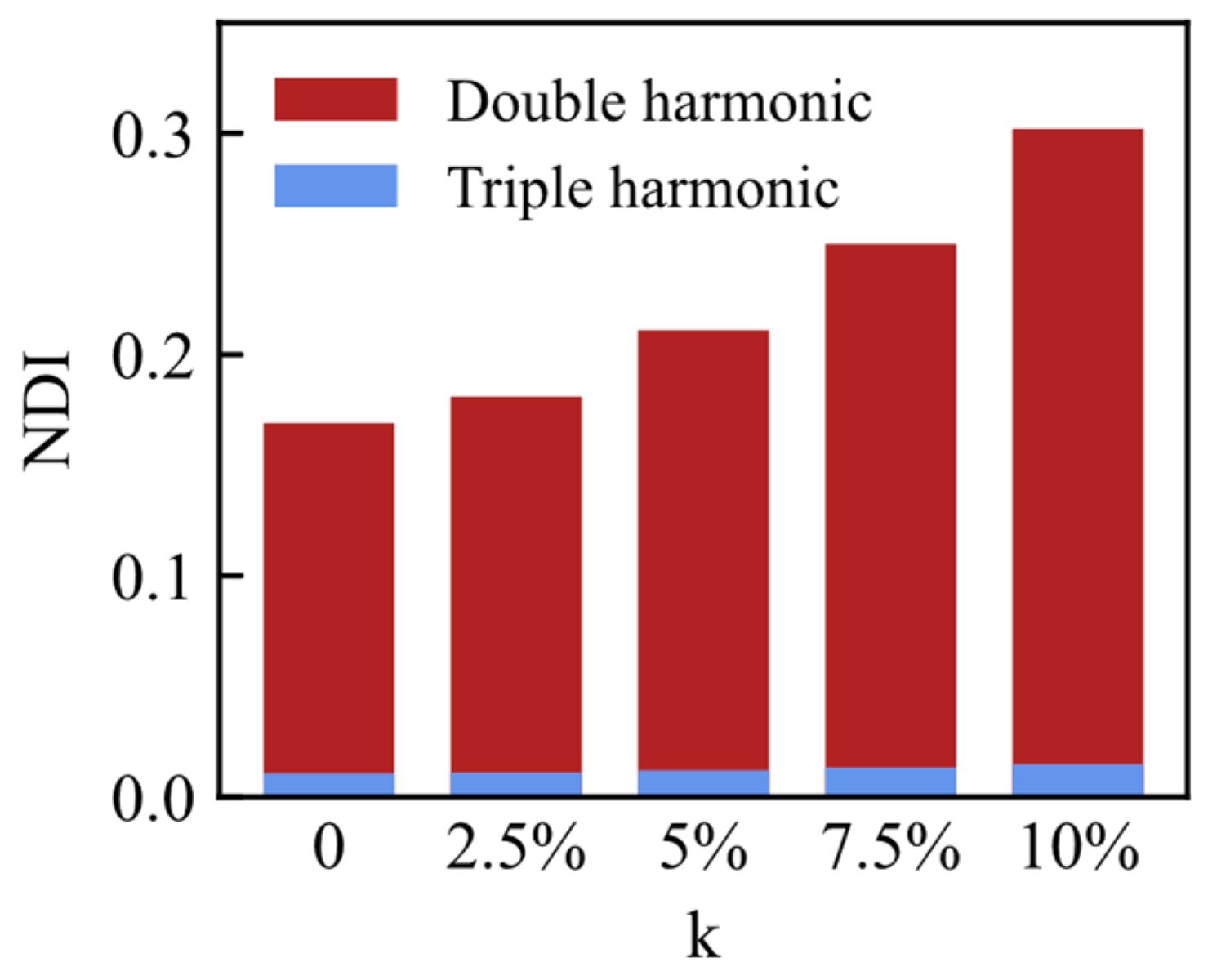
- An increase in the rotational speed perturbation coefficient results in a higher proportion of second harmonic components and a reinforcement of the super-harmonic nonlinearity associated with crack generation. The rotational speed perturbation significantly affects the proportion of second harmonic components while exerting a relatively weaker influence on lower harmonic energy and higher harmonic components.
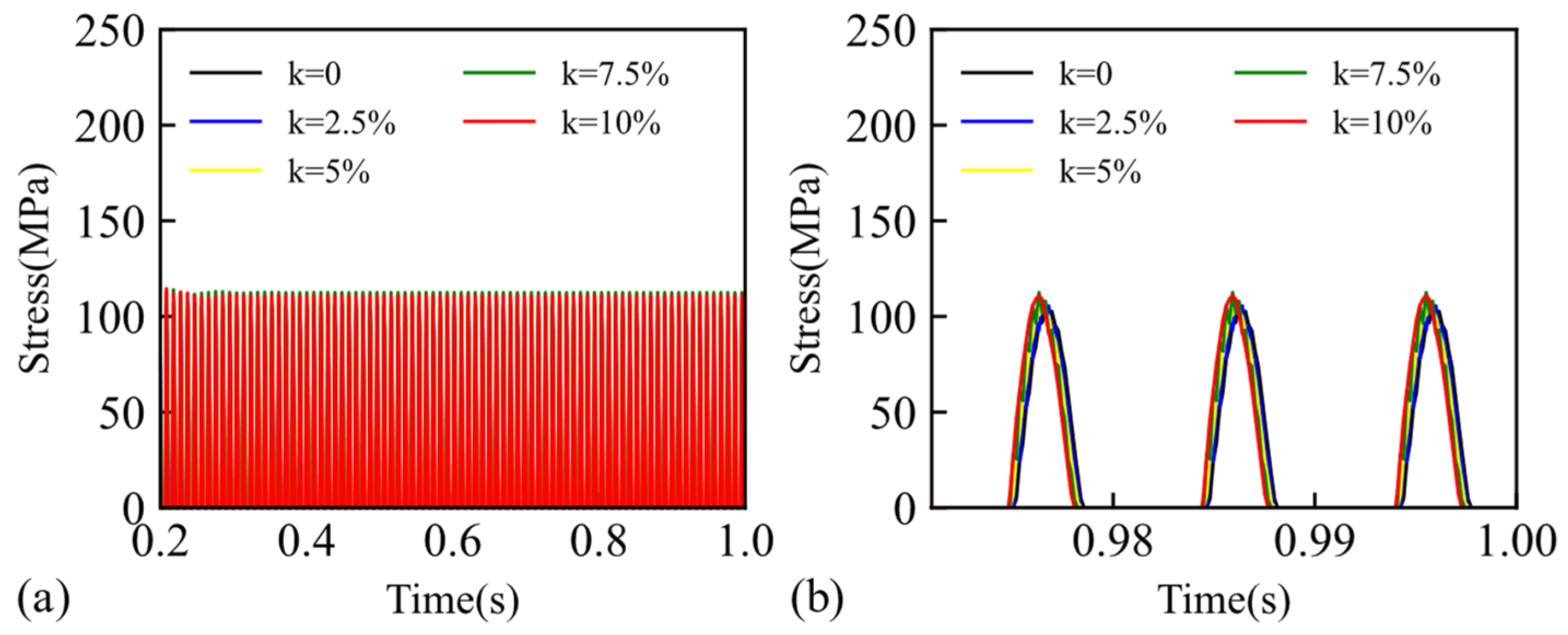
- Non-constant rotational speed has a more substantial effect on the nonlinear vibration of cracked blades. In comparison to a steady rotational speed, a non-constant rotational speed leads to an increased vibration range of the blade, enhanced super-harmonic nonlinearity due to cracks, a higher nonlinear damage index, and an intensified breathing effect of the cracks. These factors consequently raise safety concerns, including the potential for contact between the cracked blade and the magazine and an accelerated fatigue fracture of the cracked blade. Therefore, the impact of rotational speed fluctuations on cracked blades must be considered in investigations involving such blades.
- Non-linear intensity of crack breathing can be efficiently assessed with the NDI. The shape of the phase diagram can reflect the state of crack breathing, with cracks closing where the phase track lines overlap and opening where the phase track lines are dispersed. The degree of inward depression of the phase track lines can reflect the magnitude of the RPM disturbance. The greater the speed disturbance, the greater the extent of the phase track lines.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Shen, M.H.H.; Chu, Y.C. Vibrations of beams with a fatigue crack. Comput. Struct. 1992, 45, 79–93. [Google Scholar] [CrossRef]
- Pugno, N.; Surace, C.; Ruotolo, R. Evaluation of the non-linear dynamic response to harmonic excitation of a beam with several breathing cracks. J. Sound Vib. 2000, 235, 749–762. [Google Scholar] [CrossRef]
- Chondros, T.G.; Dimarogonas, A.D.; Yao, J. Vibration of a beam with a breathing crack. J. Sound Vib. 2001, 239, 57–67. [Google Scholar] [CrossRef]
- Bovsunovsky, A.P.; Surace, C. Consideration regarding superharmonic vibrations of a cracked beam and the variation in damping caused by the presence of the crack. J. Sound Vib. 2005, 288, 865–886. [Google Scholar] [CrossRef]
- Loutridis, S.; Douka, E.; Hadjileontiadis, L.J. Forced vibration behavior and crack detection of cracked beams using instantaneous frequency. NDT E Int. 2005, 38, 411–419. [Google Scholar] [CrossRef]
- Andreaus, U.; Casini, P.; Vestroni, F. Nonlinear dynamics of a cracked cantilever beam under harmonic excitation. Int. J. Non-Linear Mech. 2007, 42, 566–575. [Google Scholar] [CrossRef]
- Andreaus, U.; Baragatti, P. Cracked beam identification by numerically analysing the nonlinear behaviour of the harmonically forced response. J. Sound Vib. 2011, 330, 721–742. [Google Scholar] [CrossRef]
- Liu, C.; Jiang, D. Crack modeling of rotating blades with cracked hexahedral finite element method. Mech. Syst. Signal Process. 2014, 46, 406–423. [Google Scholar] [CrossRef]
- Liu, C.; Jiang, D.; Chu, F. Influence of alternating loads on nonlinear vibration characteristics of cracked blade in rotor system. J. Sound Vib. 2015, 353, 205–219. [Google Scholar] [CrossRef]
- Zhao, C.G.; Zeng, J.; Ma, H.; Ni, K.X.; Wen, B.C. Dynamic analysis of cracked rotating blade using cracked beam element. Results Phys. 2018, 19, 103360. [Google Scholar] [CrossRef]
- Guan, H.; Xiong, Q.; Ma, H.; Wang, W.; Ni, K.X.; Wu, Z.Y.; Yin, X.M.; Zhao, S.T.; Zhang, X.X. Comparison of nonlinear vibration responses induced by edge crack and surface crack of compressor blades. Mech. Syst. Signal Process. 2024, 216, 111465. [Google Scholar] [CrossRef]
- Eroglu, U.; Tufekci, E. Crack modeling and identification in curved beams using differential evolution. Int. J. Mech. Sci. 2017, 131, 435–450. [Google Scholar] [CrossRef]
- Zeng, J.; Ma, H.; Zhang, W.S.; Wen, B.C. Dynamic characteristic analysis of cracked cantilever beams under different crack types. Eng. Fail. Anal. 2017, 74, 80–94. [Google Scholar] [CrossRef]
- Shen, G.J.; Gu, F.S.; Yang, Y.M.; Hu, H.F.; Guan, F.J. Theoretical and experimental harmonic analysis of cracked blade vibration. Measurement 2023, 222, 113681. [Google Scholar] [CrossRef]
- Seo, H.W.; Han, J.S. Deep learning approach for predicting crack initiation position and size in a steam turbine blade using frequency response and model order reduction. J. Mech. Sci. Technol. 2024, 38, 1971–1984. [Google Scholar] [CrossRef]
- Wang, W.W.; Ma, H.; Zhao, C.G.; Wu, Z.Y.; Wang, H.J. Dynamic contact characteristics of a rotating twisted variable-section blade with breathing crack. J. Cent. South Univ. 2024, 31, 858–877. [Google Scholar] [CrossRef]
- Yang, L.H.; Sun, Y.; Yang, Z.B.; Mao, Z.; Chen, X.F. Coupling vibration mechanism of multistage blisk-rotor system with blade crack. Mech. Mach. Theory 2024, 202, 105767. [Google Scholar] [CrossRef]
- Zhang, X.D.; Xiong, Y.W.; Fan, B.C.; Zhao, Z.; Huang, X.; Dai, F. Research on dynamic characteristics of three-dimensional tip clearance with regard to blade crack depth and location of rotary blade-disk-shaft coupling system. Nonlinear Dyn. 2024, 112, 4273–4297. [Google Scholar] [CrossRef]
- Wang, J.; Guo, T.Y.; Wang, Z.W.; Liu, W.Y.; Yu, T.; Zhang, Y.H. Simulation and experimental research on vibration response of microcracked compressor blades under variable working conditions. Appl. Acoust. 2024, 216, 109766. [Google Scholar] [CrossRef]
- Saito, A.; Castanier, M.P.; Pierre, C.; Poudou, O. Efficient nonlinear vibration analysis of the forced response of rotating cracked blades. J. Comput. Nonlinear Dyn. 2009, 4, 53–63. [Google Scholar] [CrossRef]
- Xu, H.L.; Chen, Z.S.; Xiong, Y.P.; Yang, Y.M.; Tao, L.M. Nonlinear Dynamic Behaviors of Rotated Blades with Small Breathing Cracks Based on Vibration Power Flow Analysis. Shock Vib. 2016, 2016, 4197203. [Google Scholar] [CrossRef]
- Yang, L.H.; Mao, Z.; Wu, S.M.; Chen, X.F.; Yan, R.Q. Nonlinear dynamic behavior of rotating blade with breathing crack. Front. Mech. Eng. 2021, 16, 196–220. [Google Scholar] [CrossRef]
- Zhang, X.D.; Xiong, Y.W.; Huang, X.; Wang, J.H.; Fan, B.C.; Zhao, Z.; Zhu, J.H. Dynamic modeling of rotary blade crack with regard to three-dimensional tip clearance. J. Sound Vib. 2023, 544, 117414. [Google Scholar] [CrossRef]
- Lin, J.W.; Wei, Z.L.; Wu, B.; Zhang, J.H.; Dai, H.W. Nonlinear characteristics and radial-bending-torsional vibration of a blade with breathing crack. J. Sound Vib. 2025, 595, 118734. [Google Scholar] [CrossRef]
- Kammer, D.C.; Schlack, A.L. Effects of nonconstant spin rate on the vibration of a rotating beam. J. Appl. Mech.-Trans. ASME 1987, 54, 305–310. [Google Scholar] [CrossRef]
- Young, T.H. Dynamic-response of a pretwisted, tapered beam with nonconstant rotating speed. J. Sound Vib. 1991, 150, 435–446. [Google Scholar] [CrossRef]
- Yang, S.M.; Tsao, S.M. Dynamics of a pretwisted blade under nonconstant rotating speed. Comput. Struct. 1997, 62, 643–651. [Google Scholar] [CrossRef]
- Yao, M.H.; Chen, Y.P.; Zhang, W. Nonlinear vibrations of blade with varying rotating speed. Nonlinear Dyn. 2012, 68, 487–504. [Google Scholar] [CrossRef]
- Yao, M.H.; Zhang, W.; Chen, Y.P. Analysis on nonlinear oscillations and resonant responses of a compressor blade. ACTA Mech. 2014, 225, 3483–3510. [Google Scholar] [CrossRef]
- Georgiades, F.; Latalski, J.; Warminski, J. Equations of motion of rotating composite beam with a nonconstant rotation speed and an arbitrary preset angle. Mecc. J. Ital. Assoc. Theor. Appl. Mech. 2014, 49, 1833–1858. [Google Scholar] [CrossRef]
- Georgiades, F. Nonlinear dynamics of a spinning shaft with non-constant rotating speed. Nonlinear Dyn. 2018, 93, 89–118. [Google Scholar] [CrossRef]
- Arvin, H. On parametrically excited vibration and stability of beams with varying rotating speed. Iran. J. Sci. Technol.-Trans. Mech. Eng. 2019, 43, 177–185. [Google Scholar] [CrossRef]
- Zeng, J.; Chen, K.K.; Ma, H.; Duan, T.T.; Wen, B.C. Vibration response analysis of a cracked rotating compressor blade during run-up process. Mech. Syst. Signal Process. 2019, 118, 568–583. [Google Scholar] [CrossRef]
- Liu, G.; Chen, G.; Cui, F.; Xi, A. Nonlinear vibration analysis of composite blade with variable rotating speed using Chebyshev polynomials. Eur. J. Mech.-A/Solids 2020, 82, 103976. [Google Scholar] [CrossRef]
- Eftejkhari, M.; Owhadi, S. Nonlinear dynamics of the rotating beam with time varying speed under aerodynamic loads. Int. J. Dyn. Control 2022, 10, 49–68. [Google Scholar] [CrossRef]
- Lotfan, S.; Anamagh, M.R.; Bediz, B.; Cigeroglu, E. Nonlinear resonances of axially functionally graded beams rotating with varying speed including Coriolis effects. Nonlinear Dyn. 2022, 107, 533–558. [Google Scholar] [CrossRef]
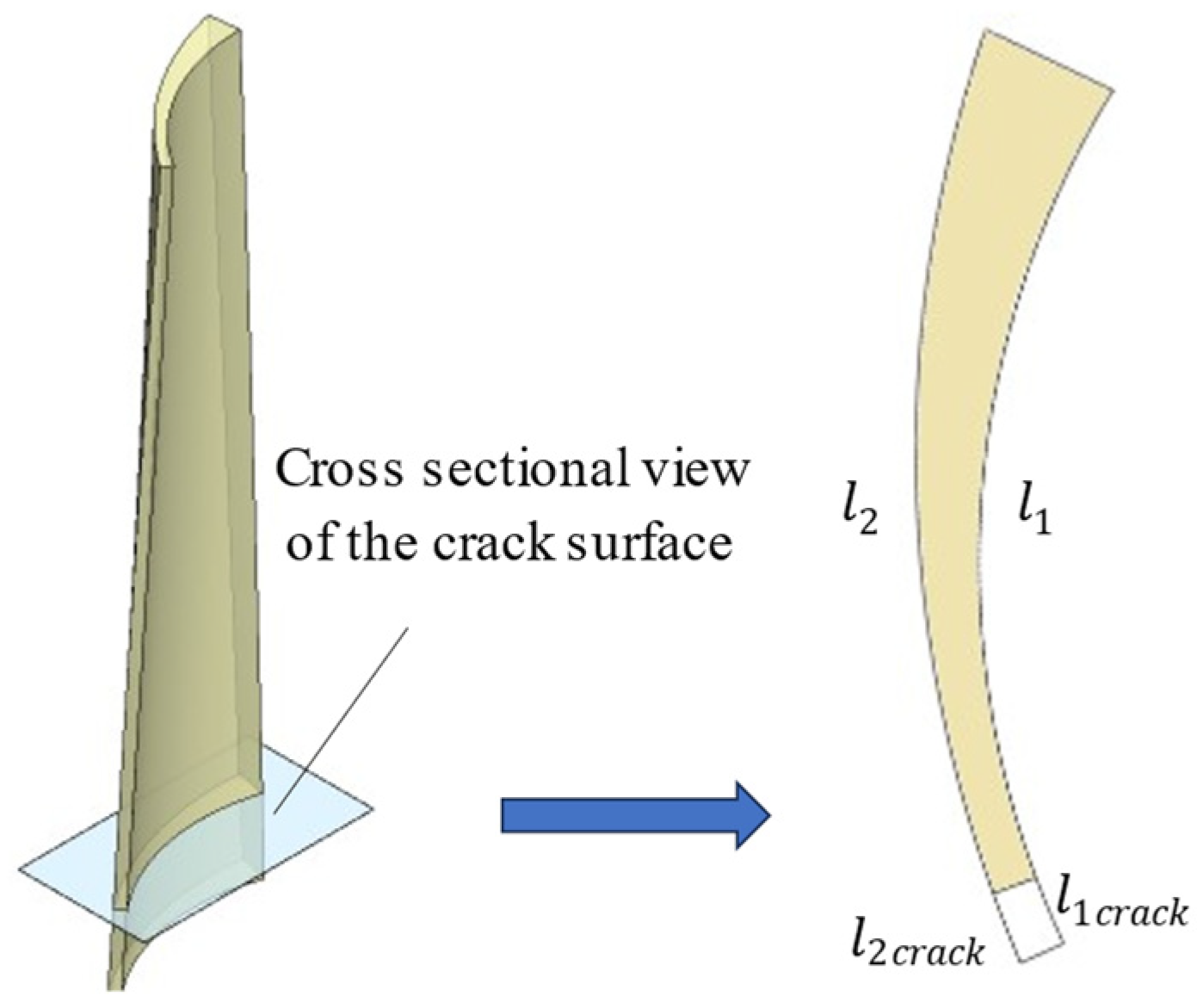

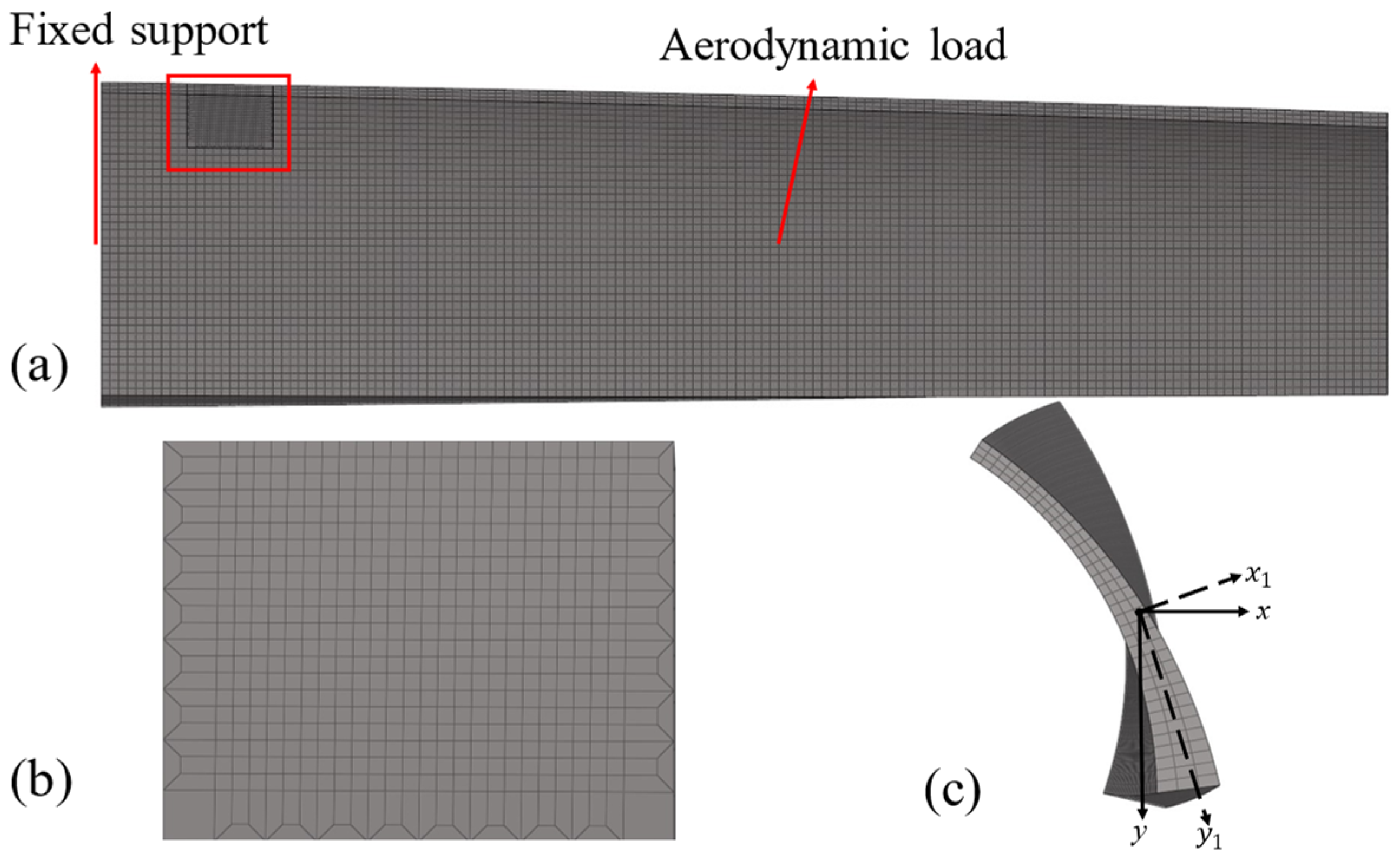








| Parameter | Value | Parameter | Value |
|---|---|---|---|
| Young’s modulus E | 70 GPa | Width l1 | 40.16 mm |
| Poisson’s ratio ν | 0.33 | Width l2 | 37.43 mm |
| Density ρ | 2650 kg/m3 | Dimensionless crack depth η1 | 0.2 |
| Rayleigh damping coefficient α | 30 | Blade length | 150 mm |
| Rayleigh damping coefficient β | 3 × 10−6 |
| FEM | CMS | CMS–Solid | |
|---|---|---|---|
| Nodes | 17,202 | 156 | 165 |
| DOF | 51,606 | 478 | 505 |
| FEM (Hz) | CMS (Hz) | Error (%) | CMS–Solid (Hz) | Error (%) | |
|---|---|---|---|---|---|
| 1 | 205.07 | 205.09 | 0.010 | 205.06 | 0.005 |
| 2 | 1004.3 | 1004.4 | 0.010 | 1004.3 | 0.000 |
| 3 | 1100.4 | 1100.5 | 0.009 | 1100.3 | 0.009 |
| 4 | 1529.5 | 1529.7 | 0.013 | 1529.5 | 0.000 |
| 5 | 3000.4 | 3000.8 | 0.013 | 3000.8 | 0.013 |
| 6 | 3170.0 | 3173.0 | 0.095 | 3173.6 | 0.114 |
| FEM | CMS | CMS–Solid | |
|---|---|---|---|
| x (mm) | 5.3153 | 5.3014 | 5.3153 |
| Error (%) | — | 0.26 | 0 |
| FEM (Hz) | CMS (Hz) | Error (%) | CMS–Solid (Hz) | Error (%) | |
|---|---|---|---|---|---|
| 1 | 208.87 | 208.87 | 0.000 | 208.92 | 0.024 |
| 2 | 962.26 | 962.35 | 0.009 | 962.31 | 0.005 |
| 3 | 1075.6 | 1075.6 | 0.000 | 1075.5 | 0.009 |
| 4 | 1500.6 | 1500.7 | 0.007 | 1500.8 | 0.013 |
| 5 | 3015.2 | 3015.7 | 0.017 | 3015.1 | 0.003 |
| 6 | 3215.4 | 3218.4 | 0.093 | 3219.9 | 0.140 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shao, B.; Fan, C.; Fu, S.; Zeng, J. Analysis of the Nonlinear Complex Response of Cracked Blades at Variable Rotational Speeds. Machines 2024, 12, 725. https://doi.org/10.3390/machines12100725
Shao B, Fan C, Fu S, Zeng J. Analysis of the Nonlinear Complex Response of Cracked Blades at Variable Rotational Speeds. Machines. 2024; 12(10):725. https://doi.org/10.3390/machines12100725
Chicago/Turabian StyleShao, Bo, Chenguang Fan, Shunguo Fu, and Jin Zeng. 2024. "Analysis of the Nonlinear Complex Response of Cracked Blades at Variable Rotational Speeds" Machines 12, no. 10: 725. https://doi.org/10.3390/machines12100725
APA StyleShao, B., Fan, C., Fu, S., & Zeng, J. (2024). Analysis of the Nonlinear Complex Response of Cracked Blades at Variable Rotational Speeds. Machines, 12(10), 725. https://doi.org/10.3390/machines12100725






