Development and Testing of a Dual-Driven Piezoelectric Microgripper with High Amplification Ratio for Cell Micromanipulation
Abstract
1. Introduction
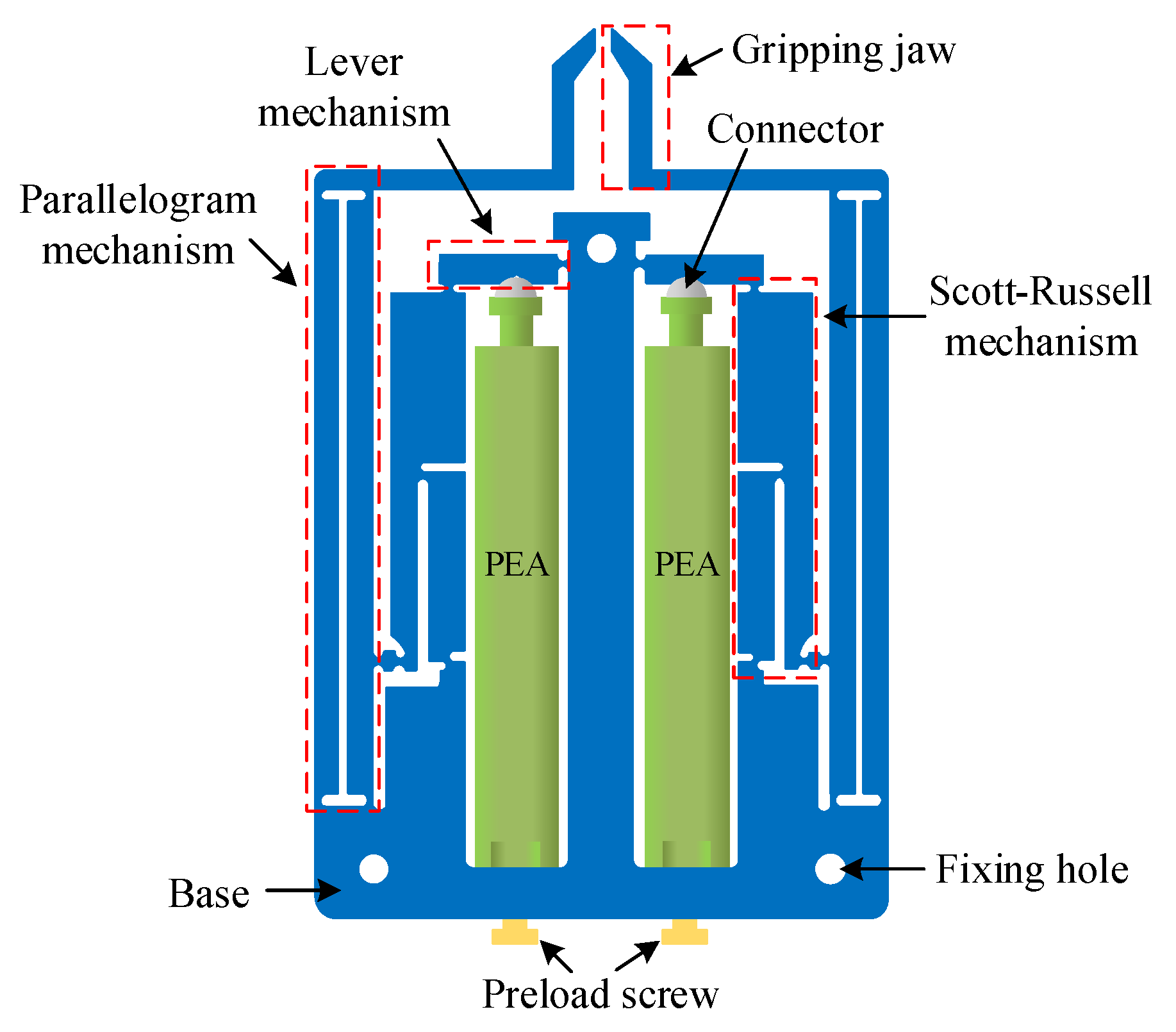
2. Structural Design of the Microgripper
3. Modeling and Parameter Optimization
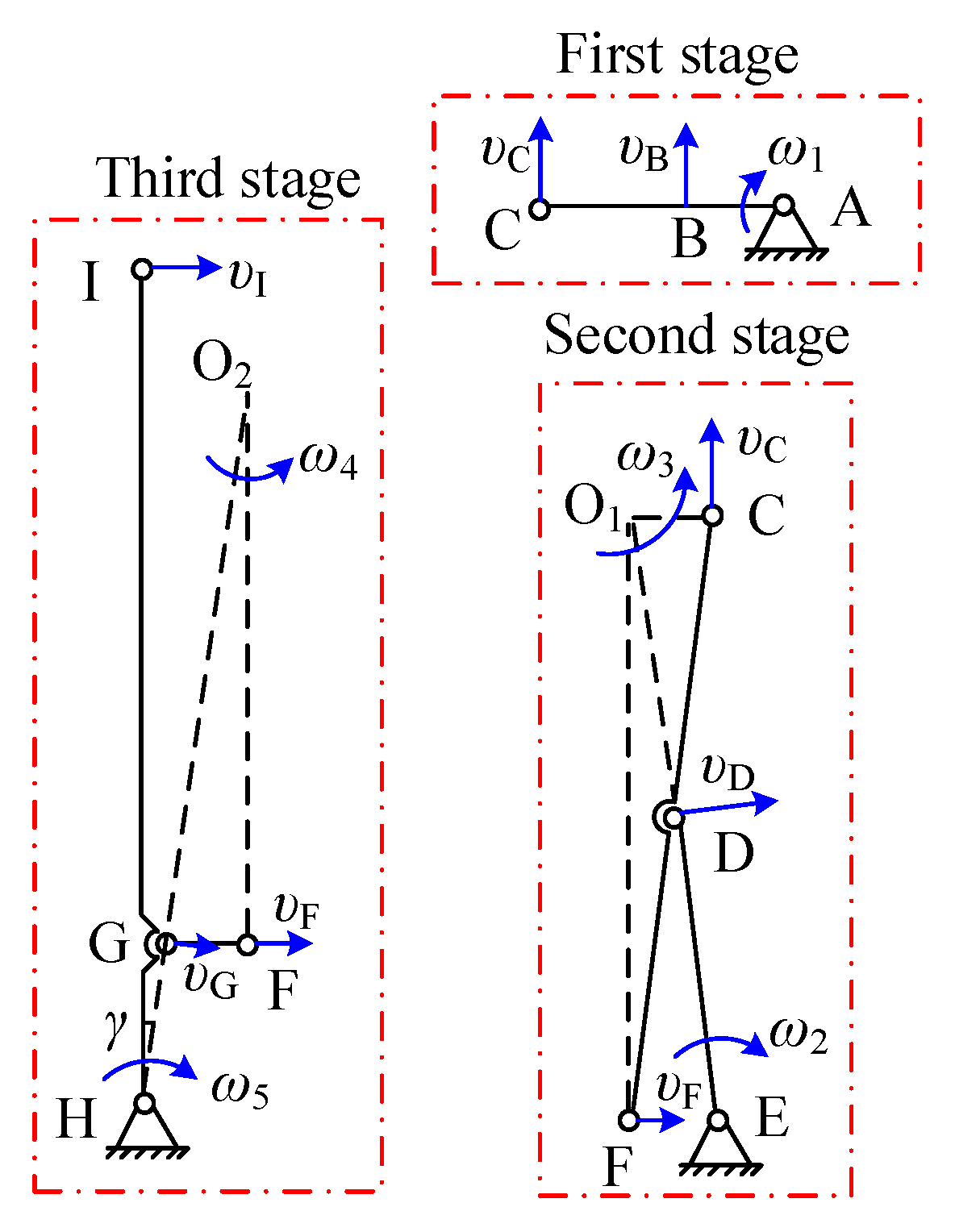
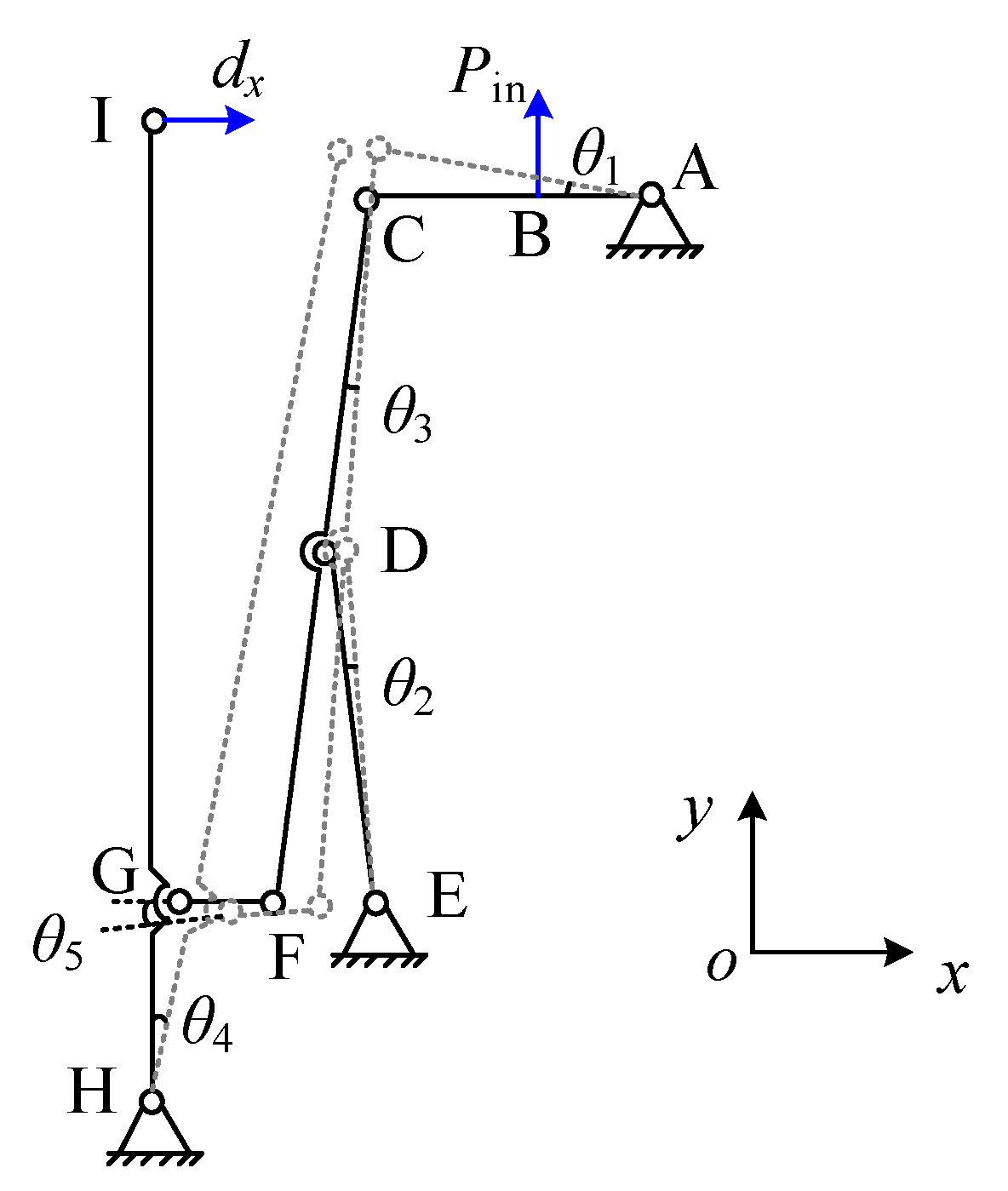
3.1. Kinematics Modeling
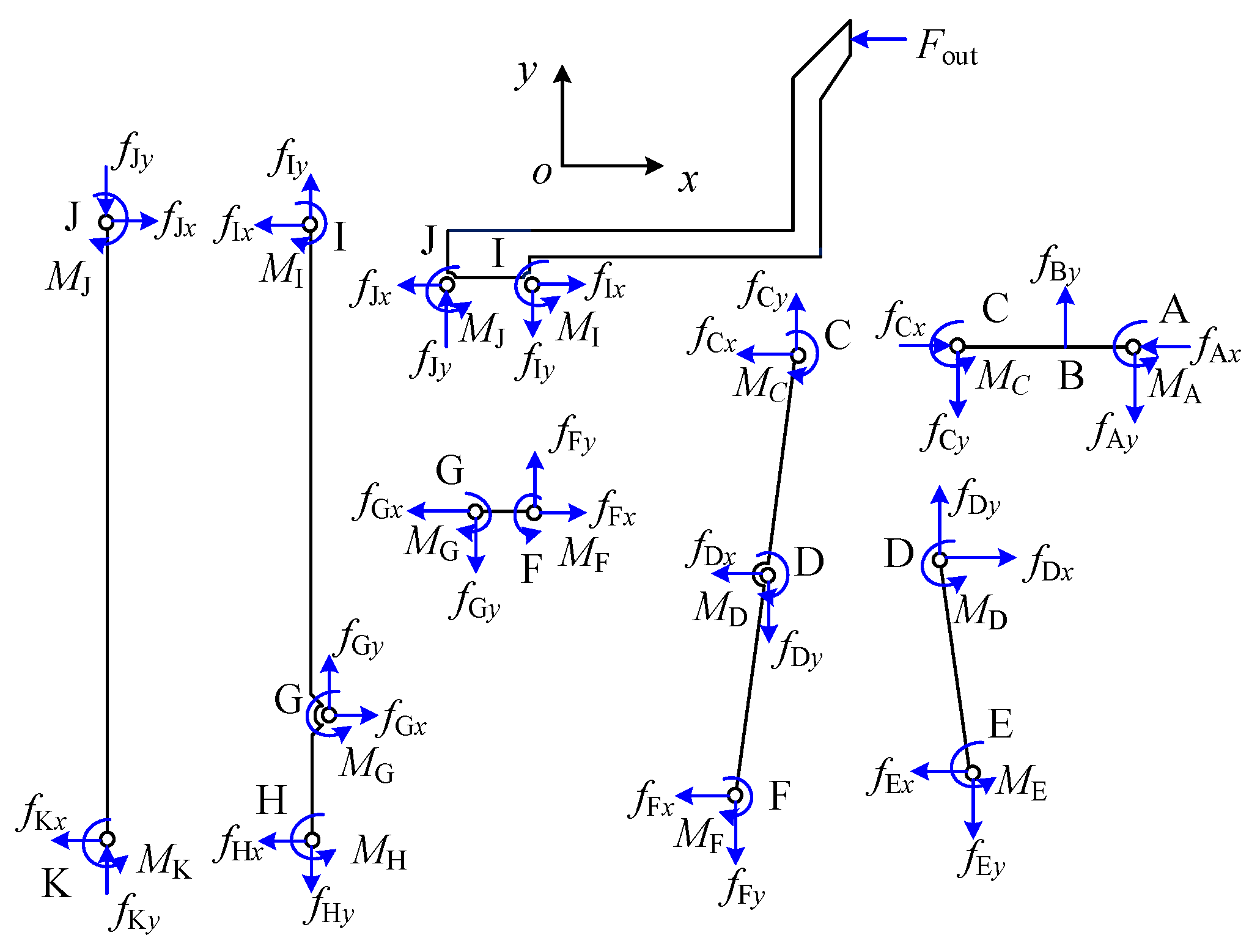
3.2. Statics Modeling
3.3. Dynamics Modeling
3.4. Parameter Optimization
- Set as a vector to be optimized, where the variables and correspond to the radius and thickness of double-notched SCFHs (i.e., A, C, D, E, F, G); and correspond to the radius and thickness of single-notched SCFHs (i.e., H, I, J, K).
- The optimization goal is to maximize the first-order natural frequency, i.e., .
- The following kinds of constraints are prescribed:
- (a)
- Size constraints: , .
- (b)
- Stiffness constraint: , where is the stiffness of PEAs. Too large input stiffness will decrease the output displacement of PEA, leading to the reduction of motion range of the microgripper.
- (c)
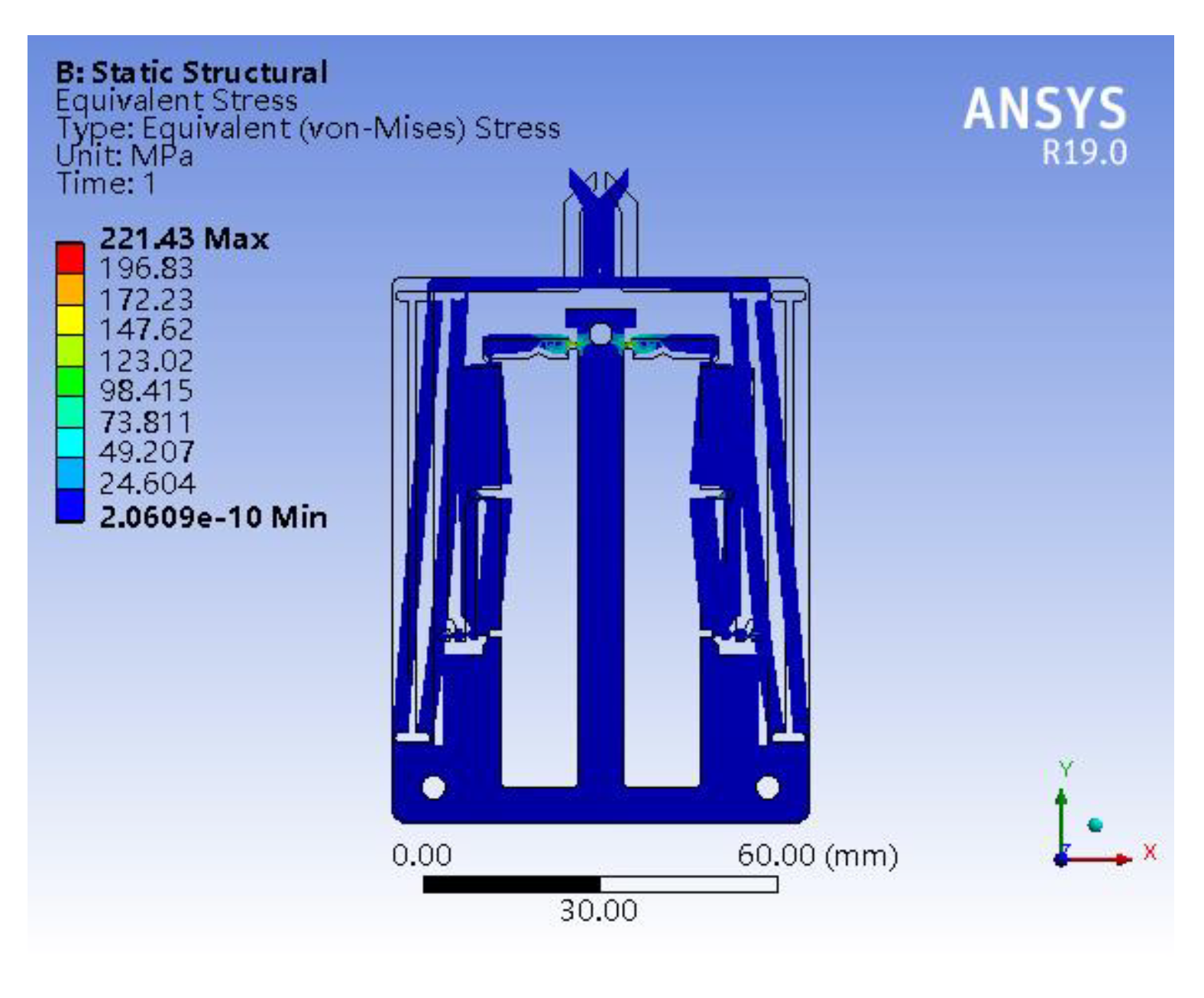
- Strength constraint: the maximum stress during the movement of the microgripper should be less than the maximum allowable stress, i.e., .
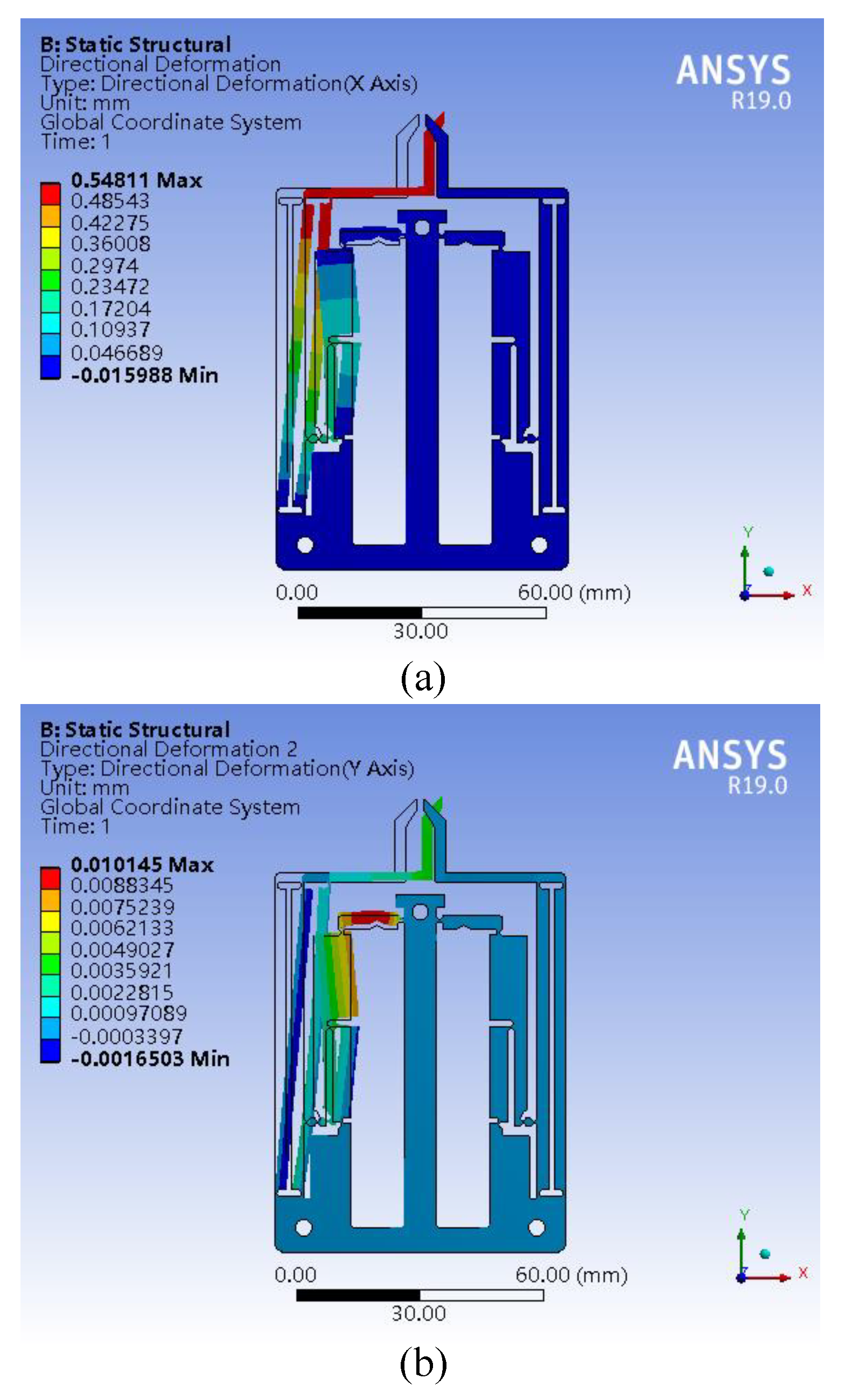
4. Finite Element Simulation Analysis
5. Experimental Verification
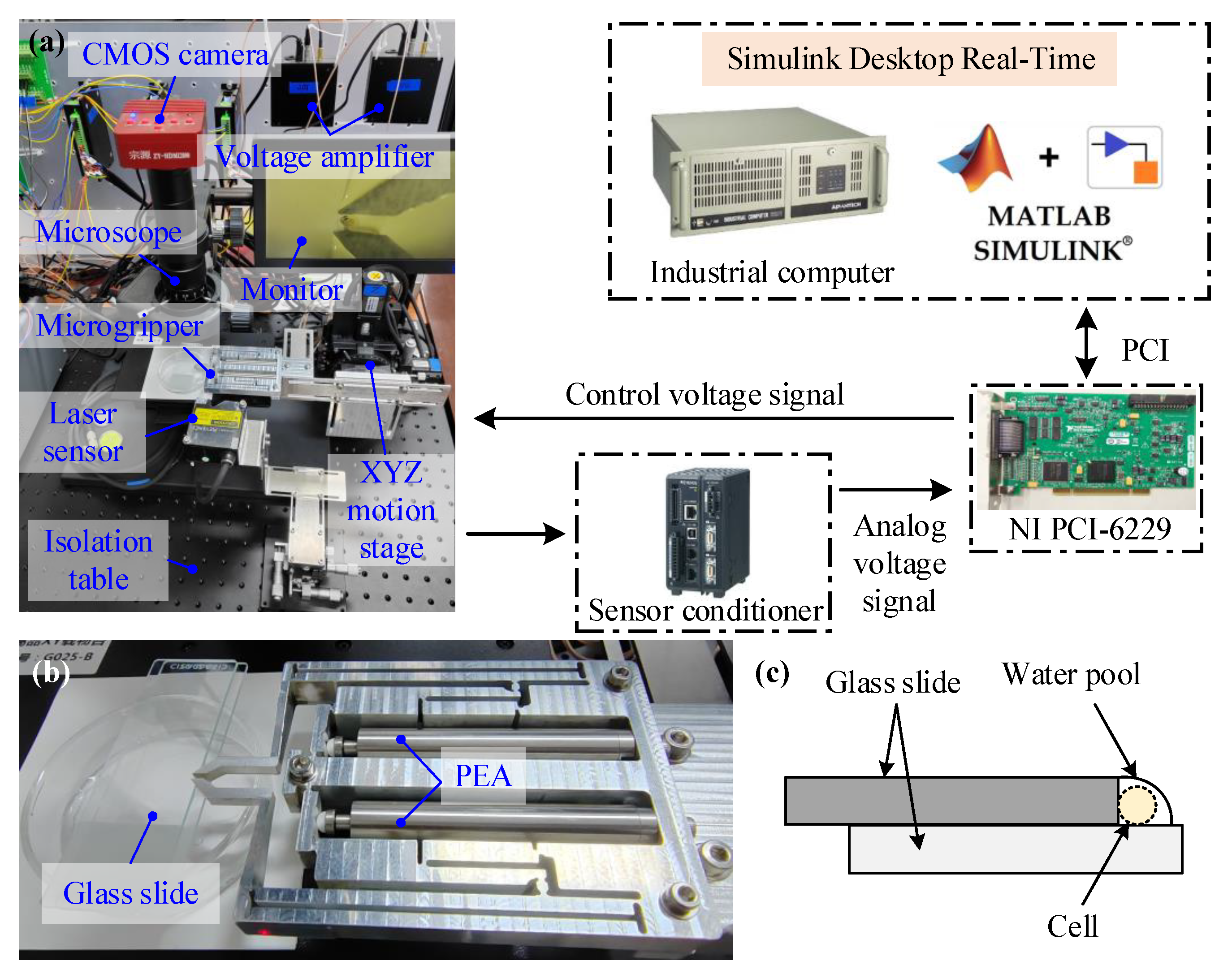
5.1. Experimental Setup
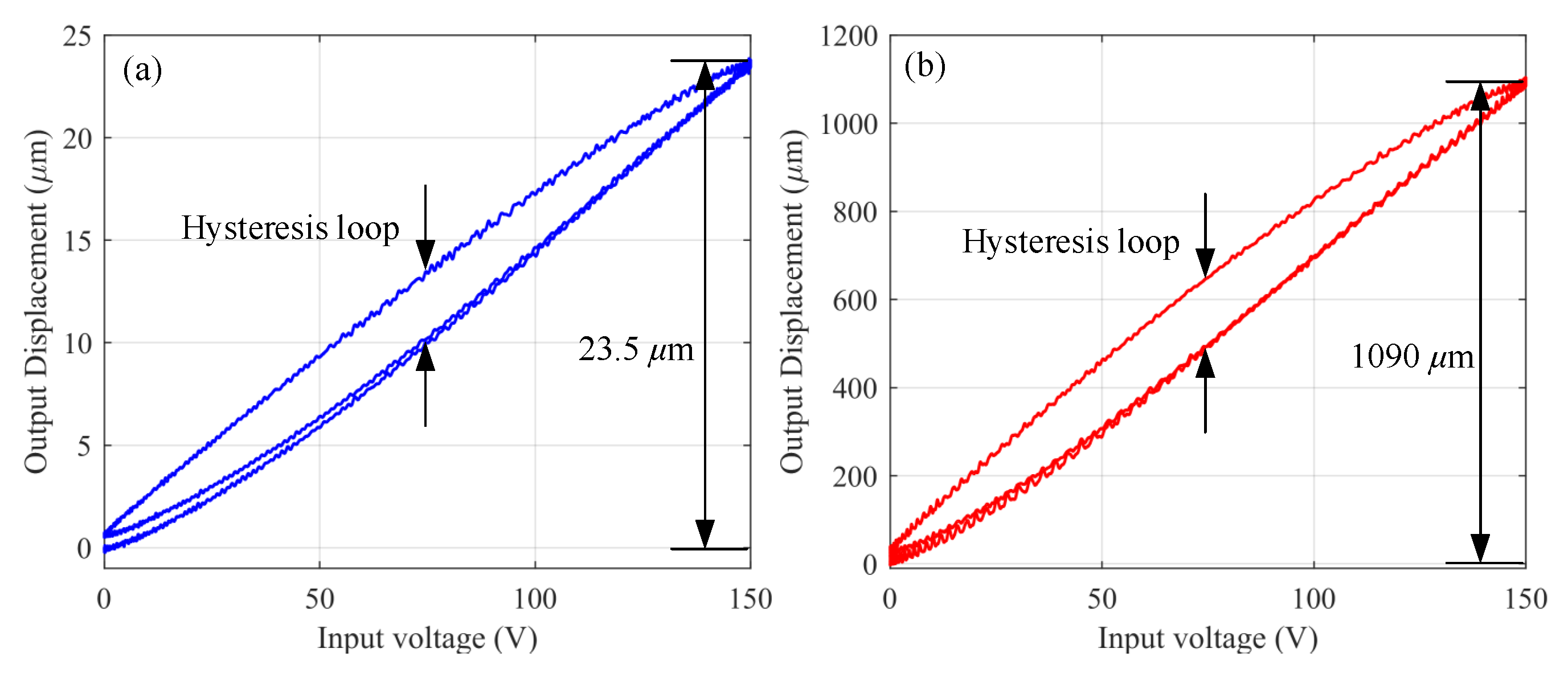
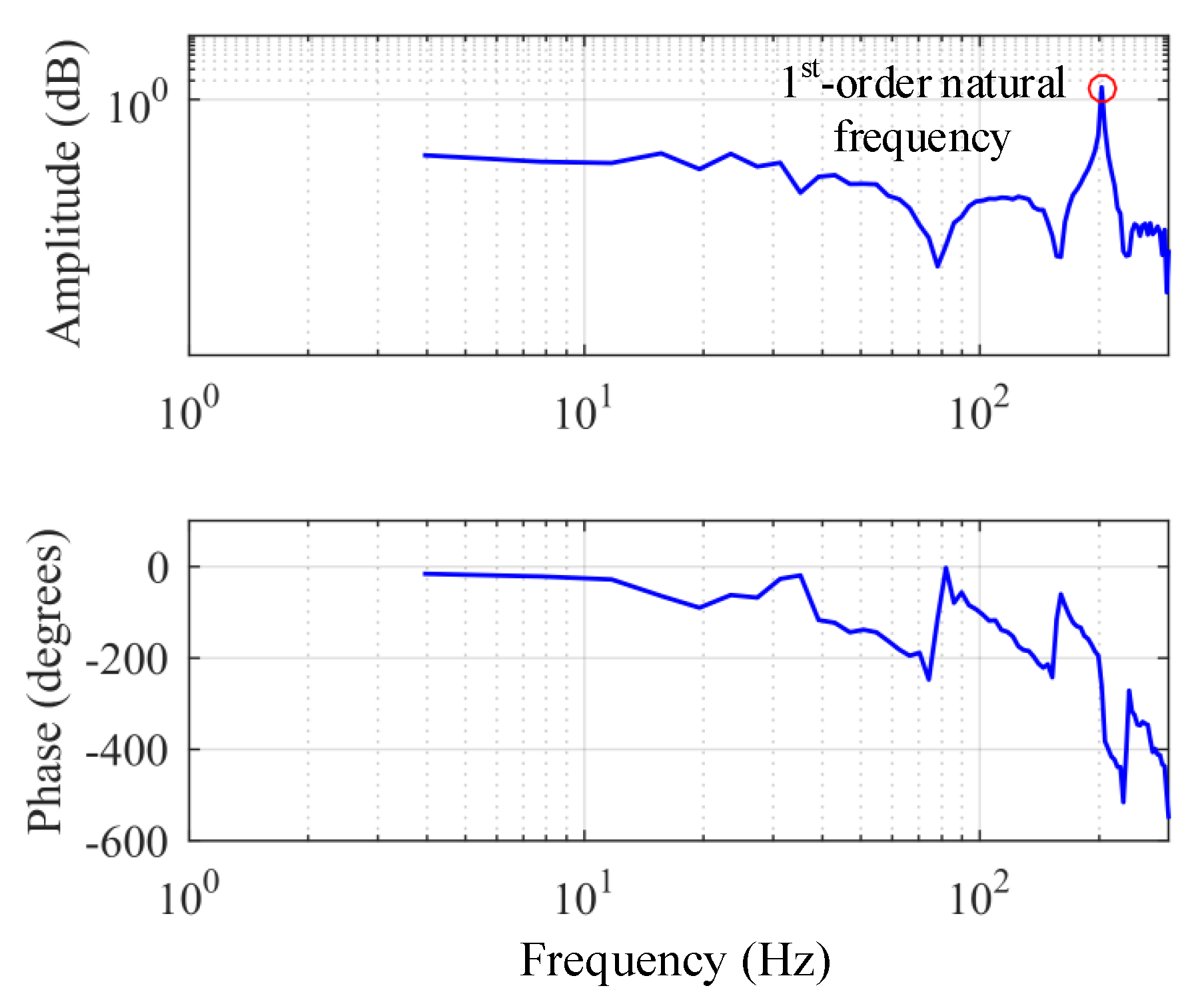
5.2. Open-Loop Tests of the Microgripper
5.3. Closed-Loop Tests of the Microgripper
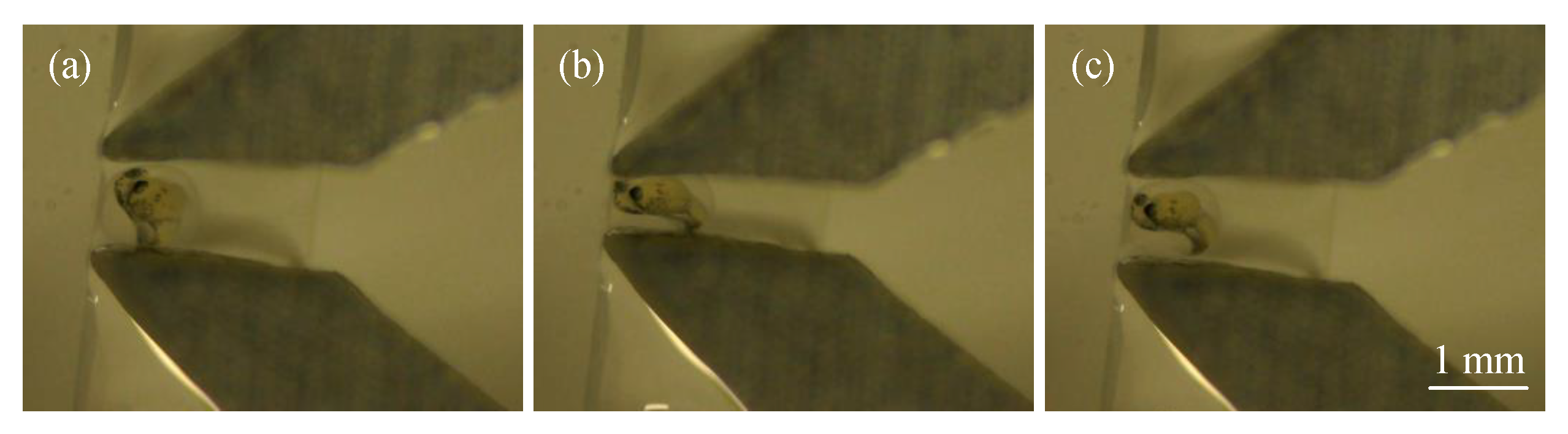
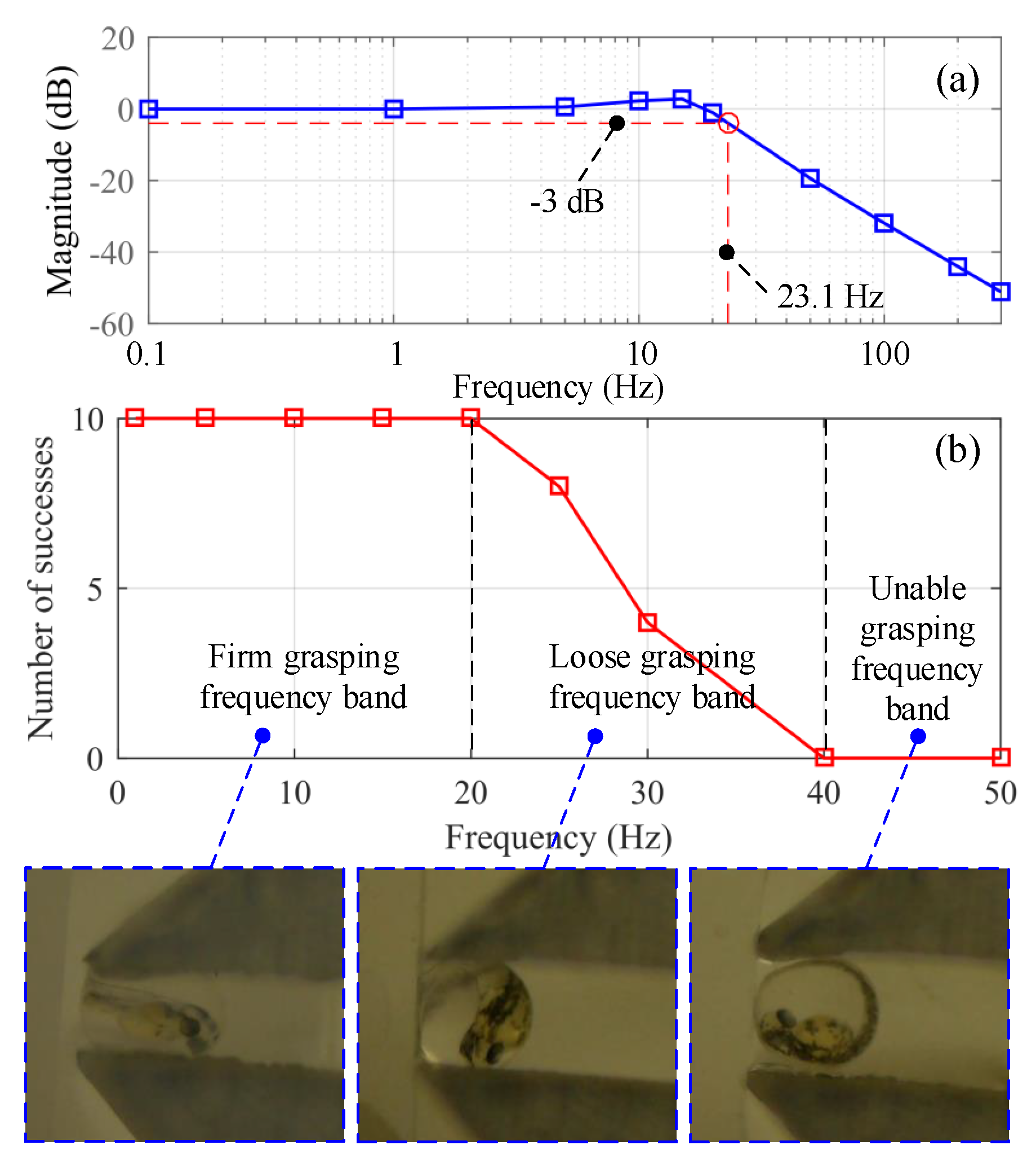
5.4. Applications to Cell Micromanipulation
5.5. Discussion
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Kodera, S.; Watanabe, T.; Yokoyama, Y.; Hayakawa, T. Microgripper using soft microactuators for manipulation of living cells. Micromachines 2022, 13, 794. [Google Scholar] [CrossRef] [PubMed]
- Kang, S.; Song, Z.; Yang, X.; Li, Y.; Wu, H.; Li, T. A rate-dependent cell microinjection model based on membrane theory. J. Biomech. Eng. 2023, 145, 091007. [Google Scholar] [CrossRef] [PubMed]
- Dai, C.; Zhuang, S.; Shan, G.; Liu, H.; Wang, Y.; Ru, C.; Sun, Y. Automated piezo-assisted sperm immobilization. IEEE Trans. Autom. Sci. Eng. 2023, 21, 3. [Google Scholar] [CrossRef]
- Shan, G.; Dai, C.; Liu, H.; Wang, X.; Dou, W.; Ru, C.; Sun, Y. Robotic blastocyst biopsy. IEEE/ASME Trans. Mechatronics 2022, 28, 1372–1383. [Google Scholar] [CrossRef]
- Guo, X.; Zhang, Y.; Jin, D.; Zhou, M. A review of single-cell pose adjustment and puncture. Adv. Intell. Syst. 2022, 4, 2200096. [Google Scholar] [CrossRef]
- Zhang, Z.; Wang, X.; Liu, J.; Dai, C.; Sun, Y. Robotic micromanipulation: Fundamentals and applications. Annu. Rev. Control Robot. Auton. Syst. 2019, 2, 181–203. [Google Scholar] [CrossRef]
- Shakoor, A.; Gao, W.; Zhao, L.; Jiang, Z.; Sun, D. Advanced tools and methods for single-cell surgery. Microsyst. Nanoeng. 2022, 8, 47. [Google Scholar] [CrossRef]
- Kang, S.; Yang, X.; Li, Y.; Wu, H.; Li, T. A fractional viscoelastic mechanical model for speed optimization of robotic cell microinjection. IEEE/ASME Trans. Mechatronics, 2024; early access. [Google Scholar]
- Zhang, J.; Lu, K.; Chen, W.; Jiang, J.; Chen, W. Monolithically integrated two-axis microgripper for polarization maintaining in optical fiber assembly. Rev. Sci. Instruments 2015, 86, 025105. [Google Scholar] [CrossRef]
- Lyu, Z.; Xu, Q. Design of a new bio-inspired dual-axis compliant micromanipulator with millimeter strokes. IEEE Trans. Robot. 2022, 39, 470–484. [Google Scholar] [CrossRef]
- Komati, B.; Clévy, C.; Lutz, P. High bandwidth microgripper with integrated force sensors and position estimation for the grasp of multistiffness microcomponents. IEEE/ASME Trans. Mechatronics 2016, 21, 2039–2049. [Google Scholar] [CrossRef]
- Shi, Q.; Yu, Z.; Wang, H.; Sun, T.; Huang, Q.; Fukuda, T. Development of a highly compact microgripper capable of online calibration for multisized microobject manipulation. IEEE Trans. Nanotechnol. 2018, 17, 657–661. [Google Scholar] [CrossRef]
- Verotti, M.; Dochshanov, A.; Belfiore, N.P. A comprehensive survey on microgrippers design: Mechanical structure. J. Mech. Des. 2017, 139, 060801. [Google Scholar] [CrossRef]
- Sabarian, D.V.; Karthikeyan, P.; Muthuramalingam, T. A review on control strategies for compensation of hysteresis and creep on piezoelectric actuators based micro systems. Mech. Syst. Signal Process. 2020, 140, 106634. [Google Scholar] [CrossRef]
- Kang, S.; Wu, H.; Li, Y.; Yang, X.; Yao, J. A fractional-order normalized bouc–wen model for piezoelectric hysteresis nonlinearity. IEEE/ASME Trans. Mechatronics 2022, 27, 126–136. [Google Scholar] [CrossRef]
- Ding, B.; Li, X.; Li, C.; Li, Y.; Chen, S.C. A survey on the mechanical design for piezo-actuated compliant micro-positioning stages. Rev. Sci. Instruments 2023, 94, 101502. [Google Scholar] [CrossRef] [PubMed]
- Dinh, T.X.; Ahn, K.K. Adaptive-gain fast nonsingular terminal sliding mode for position control of a piezo positioning stage. J. Syst. Control Eng. 2018, 232, 994–1014. [Google Scholar] [CrossRef]
- Kang, S.; Wu, H.; Yang, X.; Li, Y.; Chen, B.; Yao, J. Nonlinearities compensation of a parallel piezostage using discrete-time sliding mode predictive control with decoupling and damping properties. Mech. Syst. Signal Process. 2023, 192, 110206. [Google Scholar] [CrossRef]
- Kang, S.; Wu, H.; Yang, X.; Li, Y.; Pan, L.; Chen, B. Fractional robust adaptive decoupled control for attenuating creep, hysteresis and cross coupling in a parallel piezostage. Mech. Syst. Signal Process. 2021, 159, 107764. [Google Scholar] [CrossRef]
- Li, L.; Kang, S.; Bai, D.; Wu, H.; Yu, J. Robust high-precision tracking control for a class of nonlinear piezoelectric micropositioning systems with time-varying uncertainties. Meas. Control 2023, 56, 1396–1409. [Google Scholar] [CrossRef]
- Yu, S.; Ma, J.; Wu, H.; Kang, S. Robust precision motion control of piezoelectric actuators using fast nonsingular terminal sliding mode with time delay estimation. Meas. Control 2019, 52, 11–19. [Google Scholar] [CrossRef]
- Wu, Z.; Wang, Y.; Chen, M.; Ding, B. Design, analysis, and experimental investigations of an asymmetrical under-actuated micro-gripper. J. Intell. Mater. Syst. Struct. 2024, 35, 960–970. [Google Scholar] [CrossRef]
- Sun, X.; Chen, W.; Tian, Y.; Fatikow, S.; Zhou, R.; Zhang, J.; Mikczinski, M. A novel flexure-based microgripper with double amplification mechanisms for micro/nano manipulation. Rev. Sci. Instruments 2013, 84, 085002. [Google Scholar] [CrossRef] [PubMed]
- Wang, F.; Liang, C.; Tian, Y.; Zhao, X.; Zhang, D. Design of a piezoelectric-actuated microgripper with a three-stage flexure-based amplification. IEEE/ASME Trans. Mechatronics 2014, 20, 2205–2213. [Google Scholar] [CrossRef]
- Yang, Y.L.; Wei, Y.D.; Lou, J.Q.; Xie, F.R.; Fu, L. Development and precision position/force control of a new flexure-based microgripper. J. Micromech. Microeng. 2015, 26, 015005. [Google Scholar] [CrossRef]
- Guo, Z.; Lyu, Z.; Xu, Q. Design of a piezoelectric-driven microgripper with three working modes. IEEE/ASME Trans. Mechatronics 2023, 29, 260–270. [Google Scholar] [CrossRef]
- Hong, Y.; Wu, Y.; Jin, S.; Liu, D.; Chi, B. Design and analysis of a microgripper with three-stage amplification mechanism for micromanipulation. Micromachines 2022, 13, 366. [Google Scholar] [CrossRef]
- Tang, H.; Li, J.; Jia, Y.; Gao, J.; Li, Y. Development and testing of a large-stroke nanopositioning stage with linear active disturbance rejection controller. IEEE Trans. Autom. Sci. Eng. 2021, 19, 2461–2470. [Google Scholar] [CrossRef]
- Liang, C.; Wang, F.; Shi, B.; Huo, Z.; Zhou, K.; Tian, Y.; Zhang, D. Design and control of a novel asymmetrical piezoelectric actuated microgripper for micromanipulation. Sens. Actuators Phys. 2018, 269, 227–237. [Google Scholar] [CrossRef]
- Verotti, M. A pseudo-rigid body model based on finite displacements and strain energy. Mech. Mach. Theory 2020, 149, 103811. [Google Scholar] [CrossRef]
- Wu, H.; Lai, L.; Zhang, L.; Zhu, L. A novel compliant XY micro-positioning stage using bridge-type displacement amplifier embedded with Scott–Russell mechanism. Precis. Eng. 2022, 73, 284–295. [Google Scholar] [CrossRef]
- Ling, M.; Howell, L.L.; Cao, J.; Chen, G. Kinetostatic and dynamic modeling of flexure-based compliant mechanisms: A survey. Appl. Mech. Rev. 2020, 72, 030802. [Google Scholar] [CrossRef]
- Ling, M.; Zhang, X. Coupled dynamic modeling of piezo-actuated compliant mechanisms subjected to external loads. Mech. Mach. Theory 2021, 160, 104283. [Google Scholar] [CrossRef]
- Das, T.K.; Shirinzadeh, B.; Al-Jodah, A.; Ghafarian, M.; Pinskier, J. Computational parametric analysis and experimental investigations of a compact flexure-based microgripper. Precis. Eng. 2020, 66, 363–373. [Google Scholar] [CrossRef]
- Wei, Y.; Xu, Q. Design and testing of a new force-sensing cell microinjector based on small-stiffness compliant mechanism. IEEE/ASME Trans. Mechatronics 2020, 26, 818–829. [Google Scholar] [CrossRef]
- Shan, Y.; Ding, B.; Zhong, J.; Li, Y. Design and optimization of a decoupled serial constant force microgripper for force sensitive objects manipulation. Robotica 2023, 41, 2064–2078. [Google Scholar] [CrossRef]
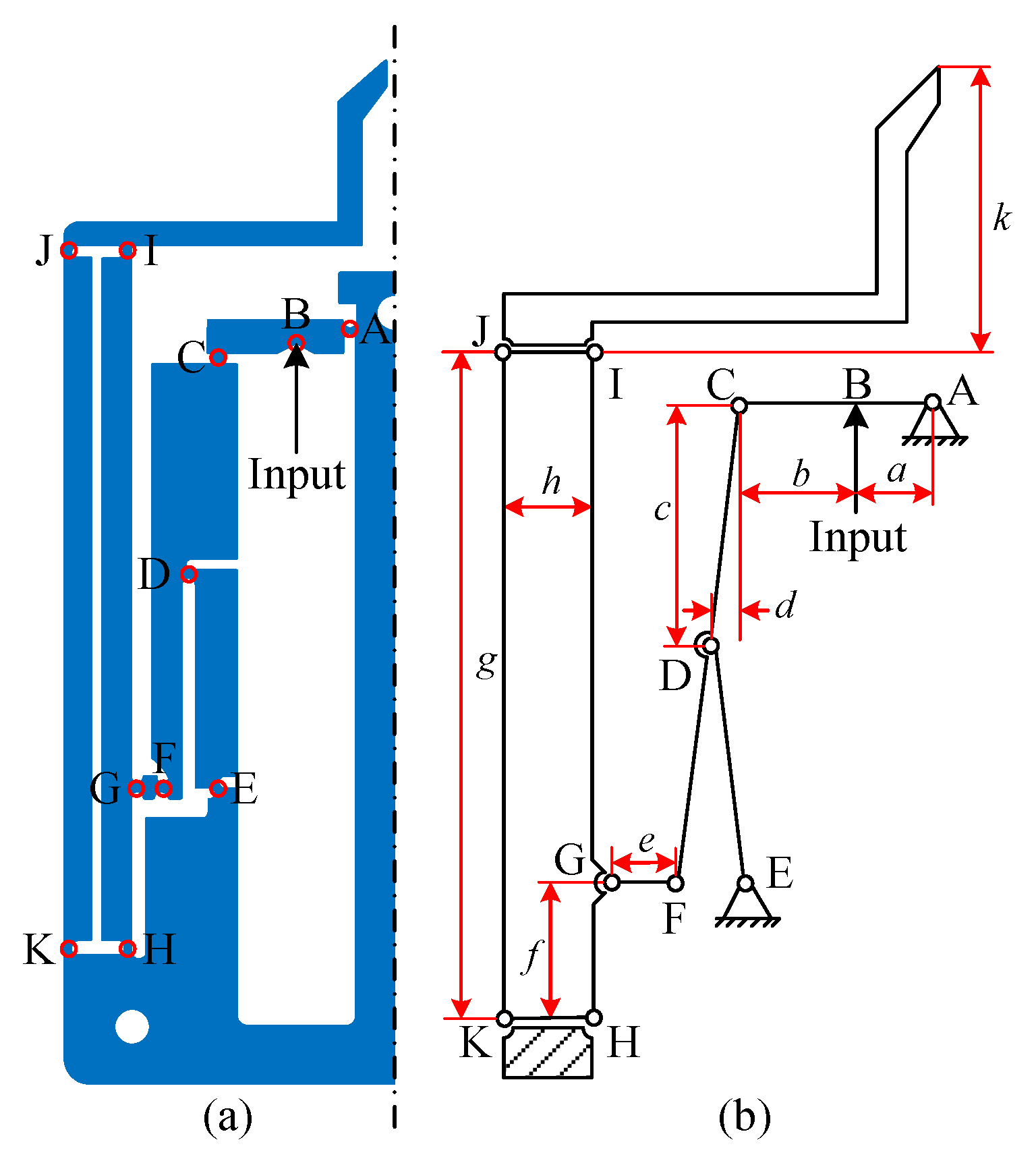


| Parameters | Symbols | Values |
|---|---|---|
| Length of link AB | a | 5.8 mm |
| Length of link BC | b | 8.5 mm |
| Vertical distance of link CD | c | 20.3 mm |
| Horizontal distance of link CD | d | 3.2 mm |
| Length of link GF | e | 2.7 mm |
| Vertical distance of link GH | f | 18.2 mm |
| Length of link JK | g | 64.6 mm |
| Length of link IJ | h | 6.4 mm |
| Vertical distance of gripping jaw | k | 21.1 mm |
| Width of the flexure hinges | n | 10.0 mm |
| Stiffness of PEA | 8.0 N/m | |
| Moment of inertia of link AC | 89.9 g·mm2 | |
| Moment of inertia of link DE | 494.6 g·mm2 | |
| Moment of inertia of link CF | 5.1e3 g·mm2 | |
| Moment of inertia of link FG | 0.3 g·mm2 | |
| Moment of inertia of link HI | 1.0e4 g·mm2 | |
| Mass of gripping jaw | 2.0 g |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lu, B.; Kang, S.; Zhou, L.; Hua, D.; Yang, C.; Zhu, Z. Development and Testing of a Dual-Driven Piezoelectric Microgripper with High Amplification Ratio for Cell Micromanipulation. Machines 2024, 12, 722. https://doi.org/10.3390/machines12100722
Lu B, Kang S, Zhou L, Hua D, Yang C, Zhu Z. Development and Testing of a Dual-Driven Piezoelectric Microgripper with High Amplification Ratio for Cell Micromanipulation. Machines. 2024; 12(10):722. https://doi.org/10.3390/machines12100722
Chicago/Turabian StyleLu, Boyan, Shengzheng Kang, Luyang Zhou, Dewen Hua, Chengdu Yang, and Zimeng Zhu. 2024. "Development and Testing of a Dual-Driven Piezoelectric Microgripper with High Amplification Ratio for Cell Micromanipulation" Machines 12, no. 10: 722. https://doi.org/10.3390/machines12100722
APA StyleLu, B., Kang, S., Zhou, L., Hua, D., Yang, C., & Zhu, Z. (2024). Development and Testing of a Dual-Driven Piezoelectric Microgripper with High Amplification Ratio for Cell Micromanipulation. Machines, 12(10), 722. https://doi.org/10.3390/machines12100722






