Study on Crashworthiness of Shrink Tube Anti-Creep Device
Abstract
1. Introduction
2. Methodology
2.1. Geometry Description
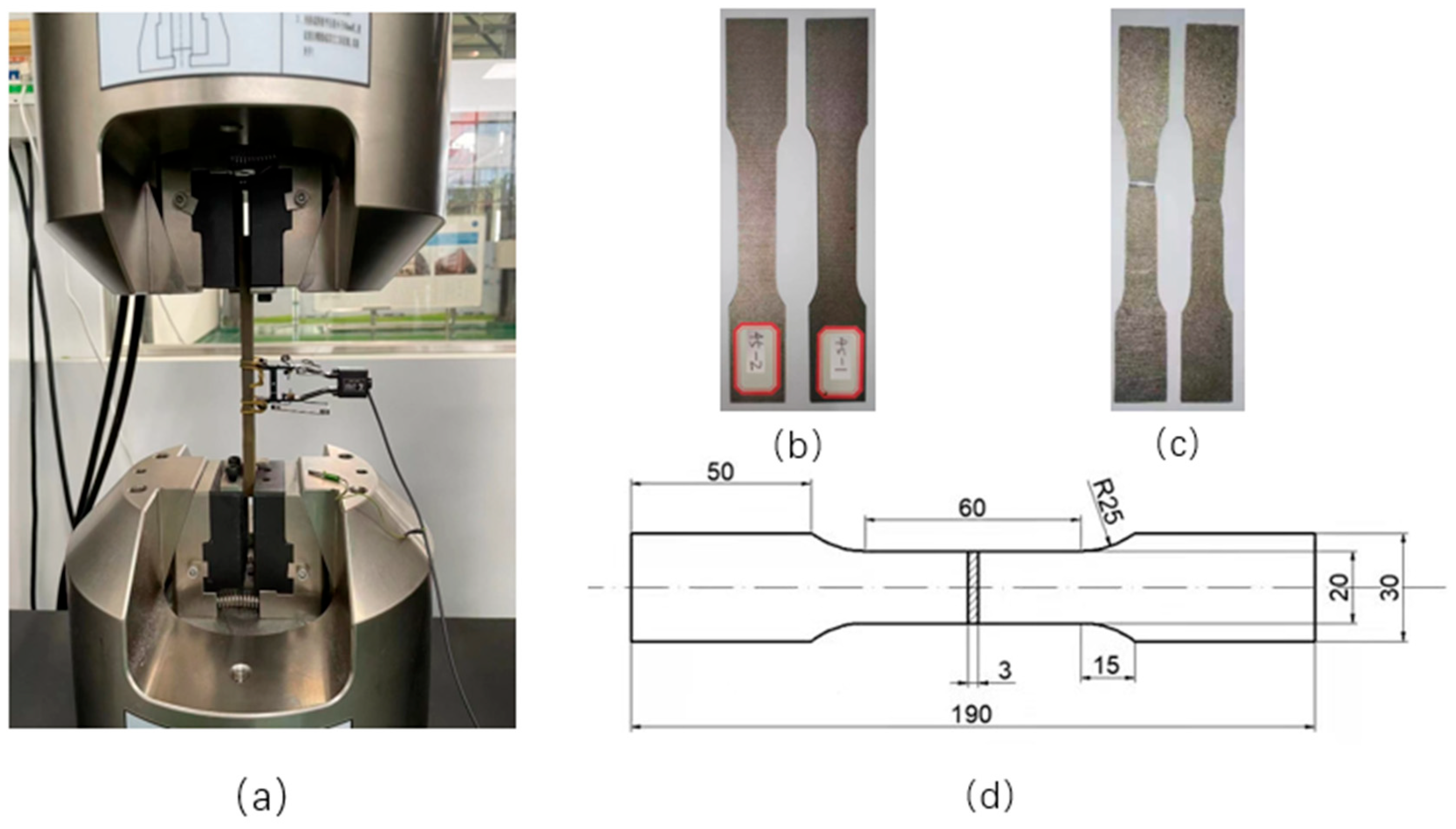
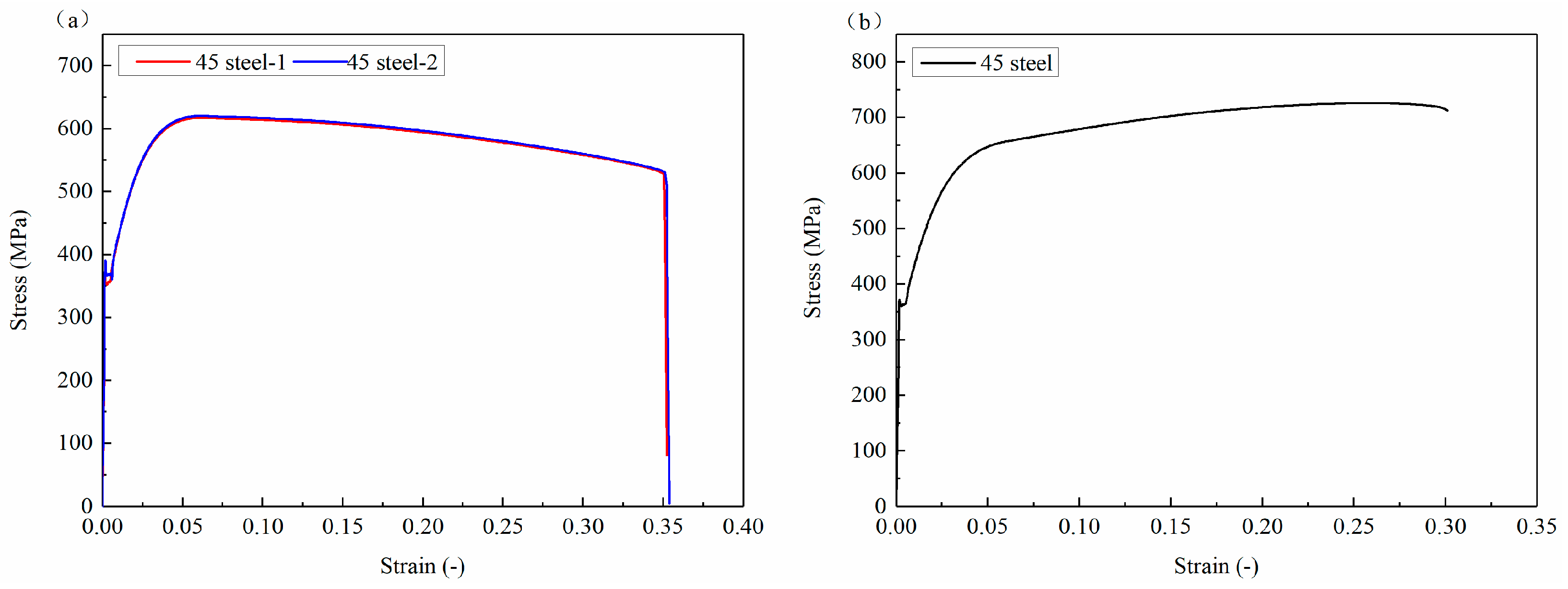
2.2. Material Properties
2.3. Crashworthiness Index
3. Trolley Test and Finite Element Modeling
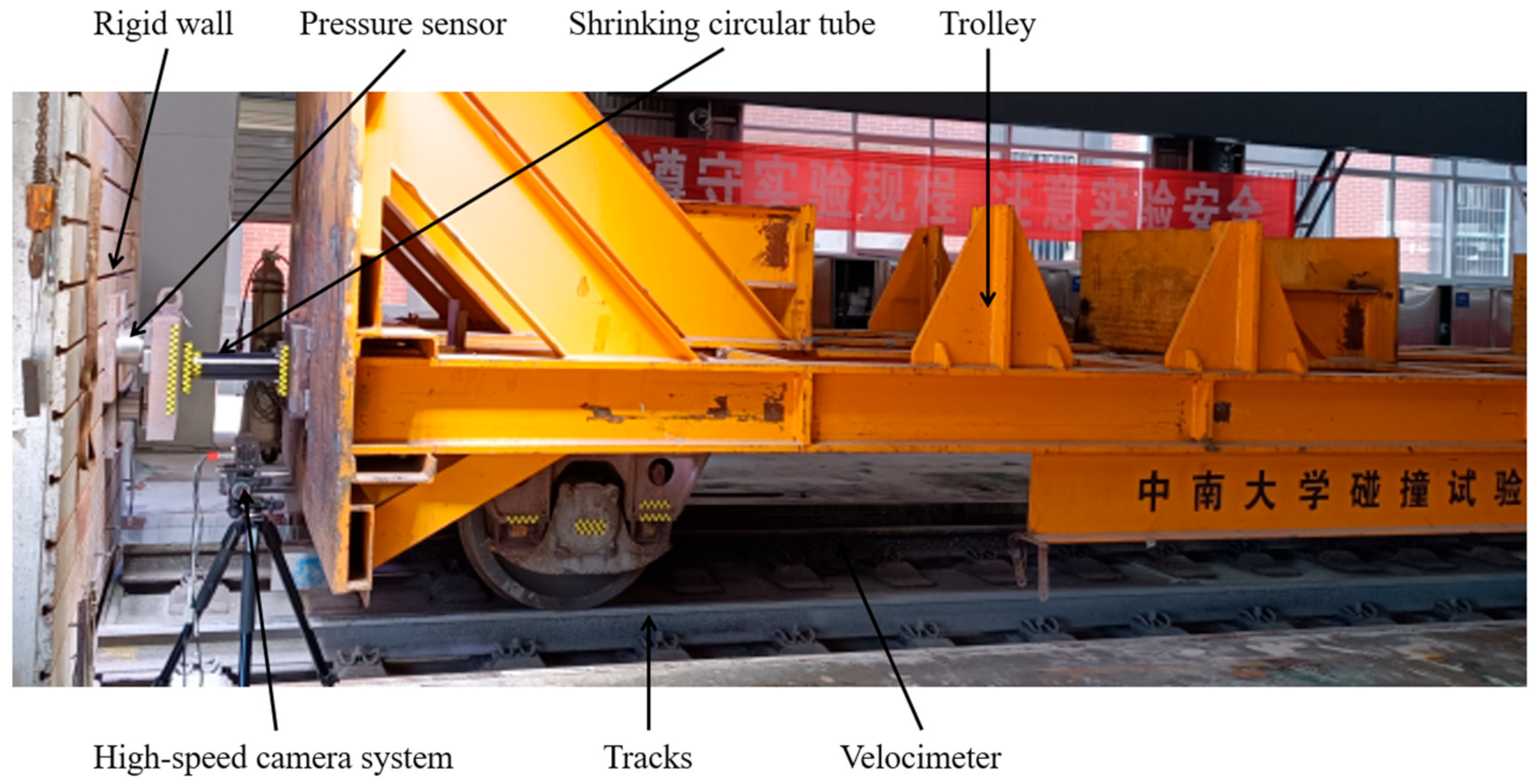
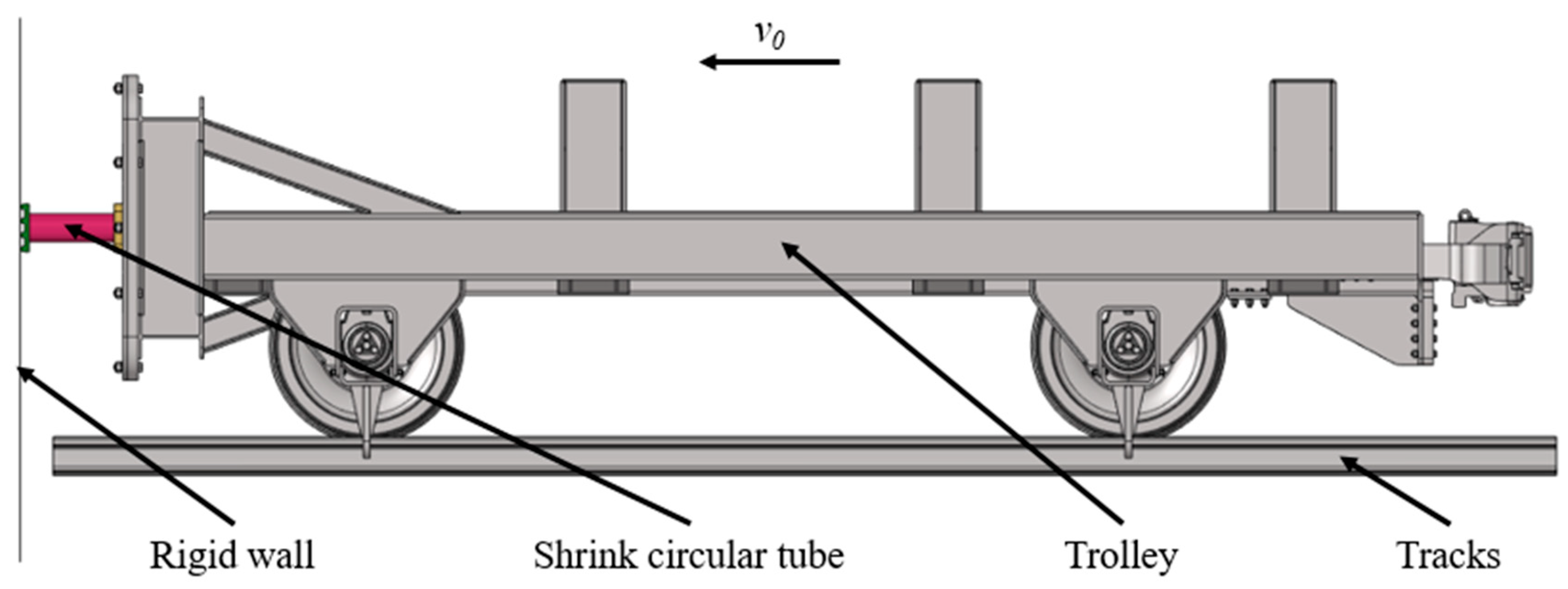
3.1. Test Scenario
3.2. Finite Element Modeling
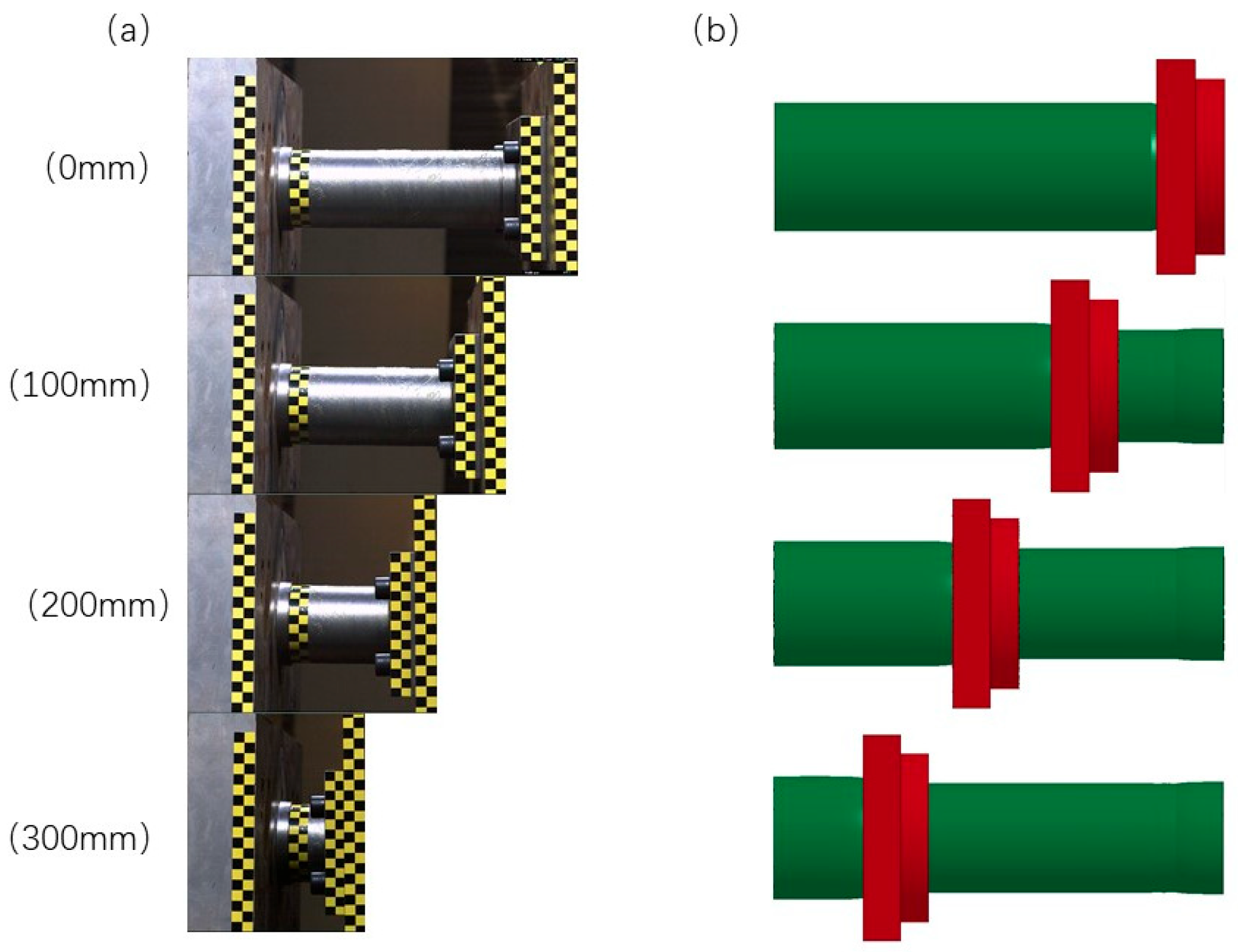
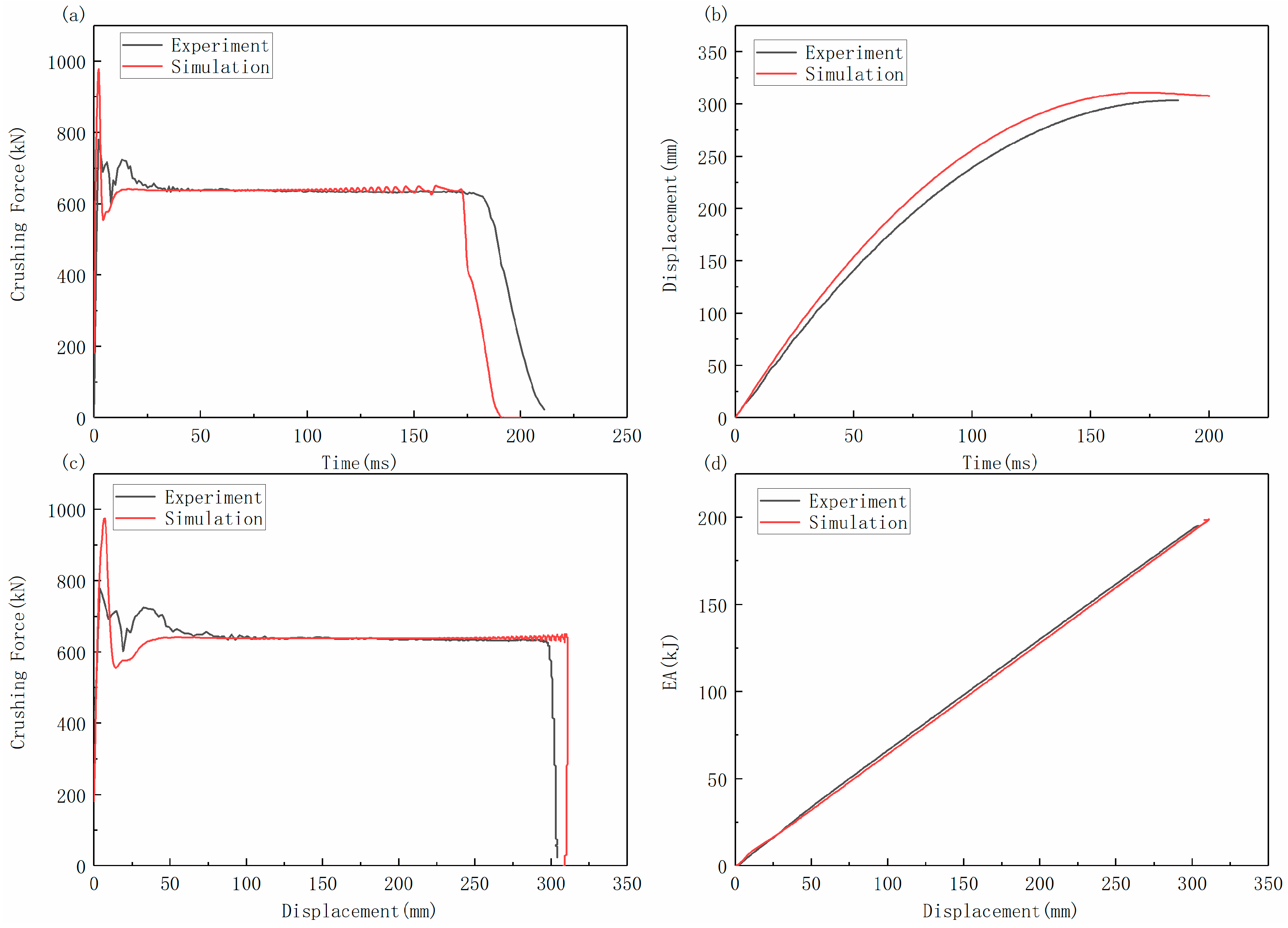
3.3. Verification of the Numerical Simulation Model
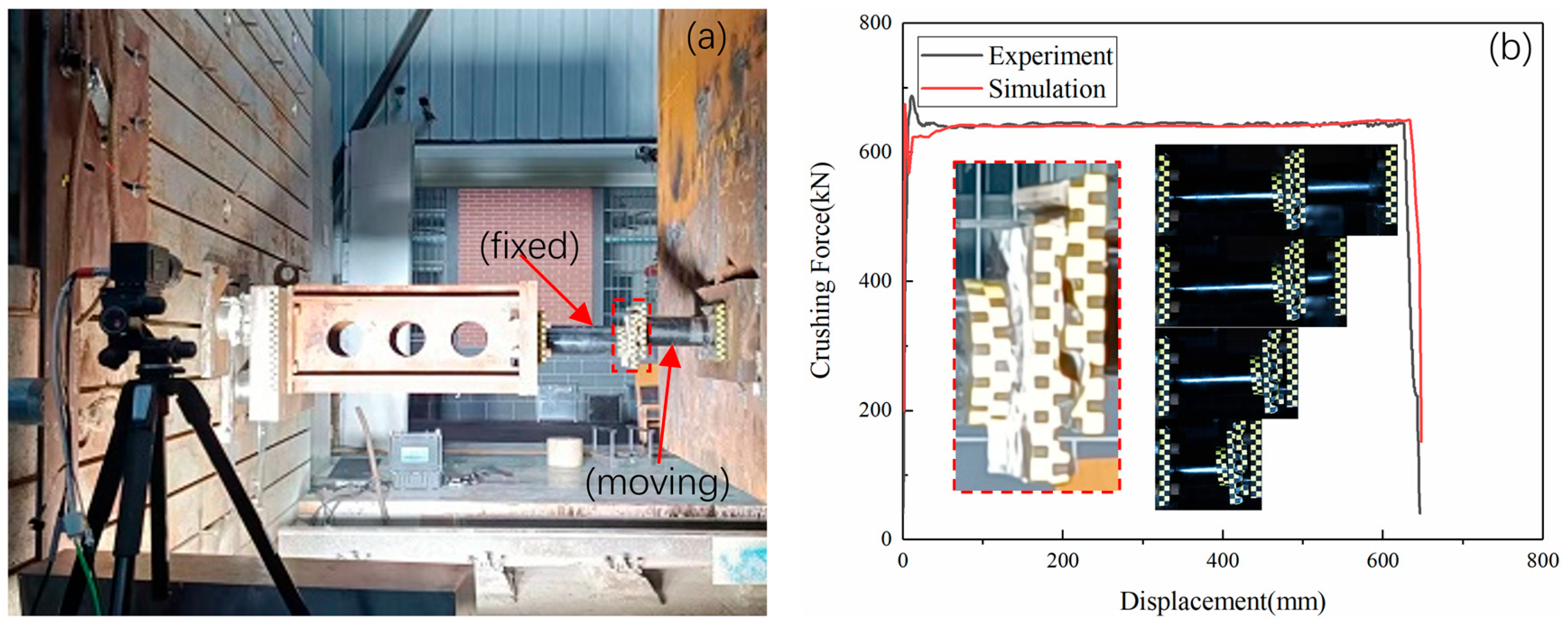
3.4. Verification of Anti-Climber Device in Collision Condition
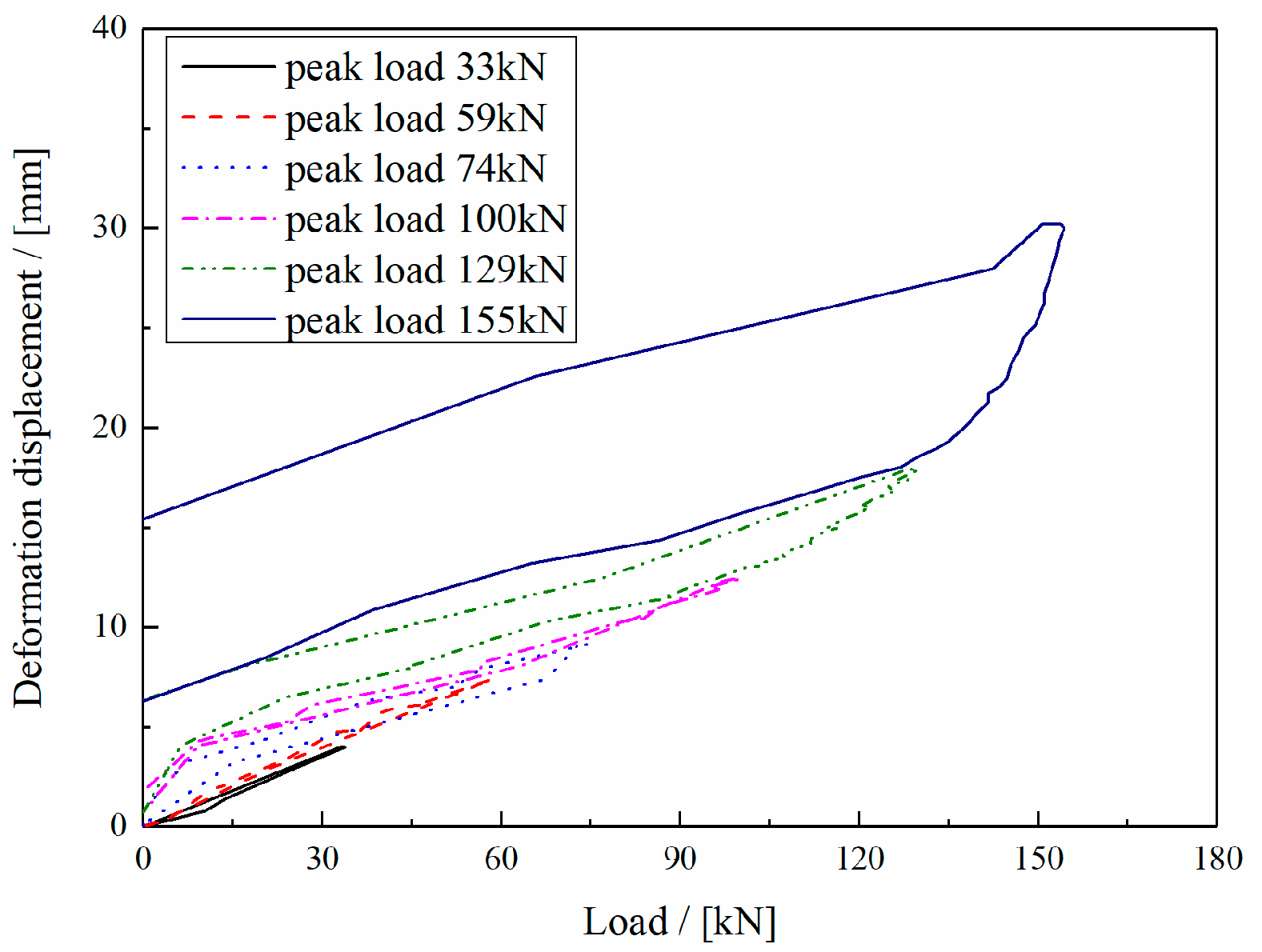
3.5. Verification of Vertical Bearing Capacity of Anti-Climber Device
4. Analysis of Influencing Factors of Crashworthiness
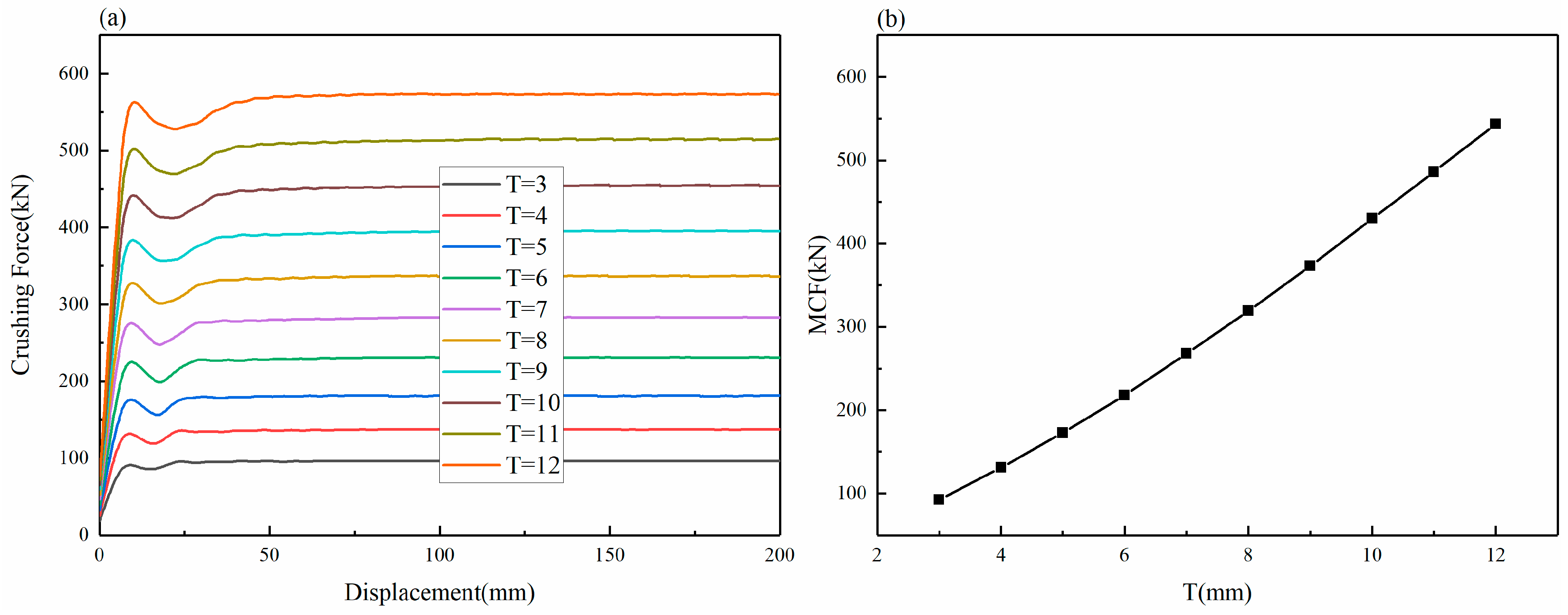
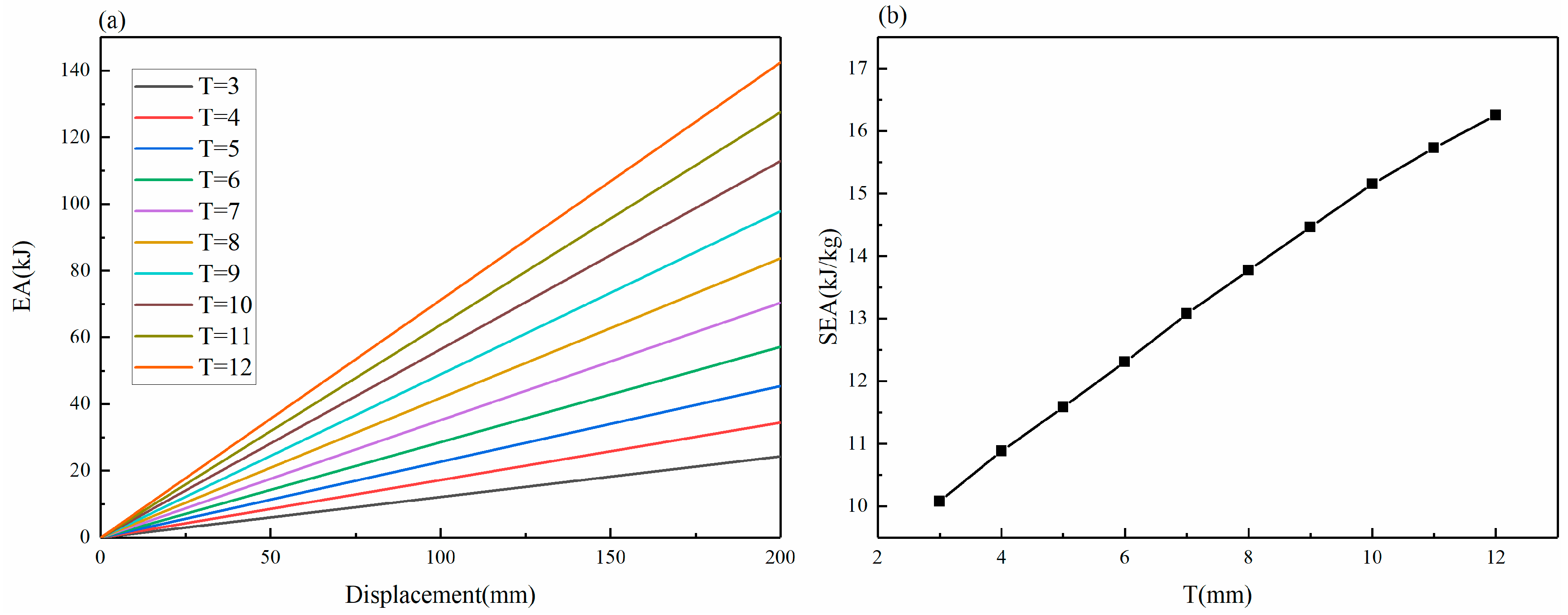
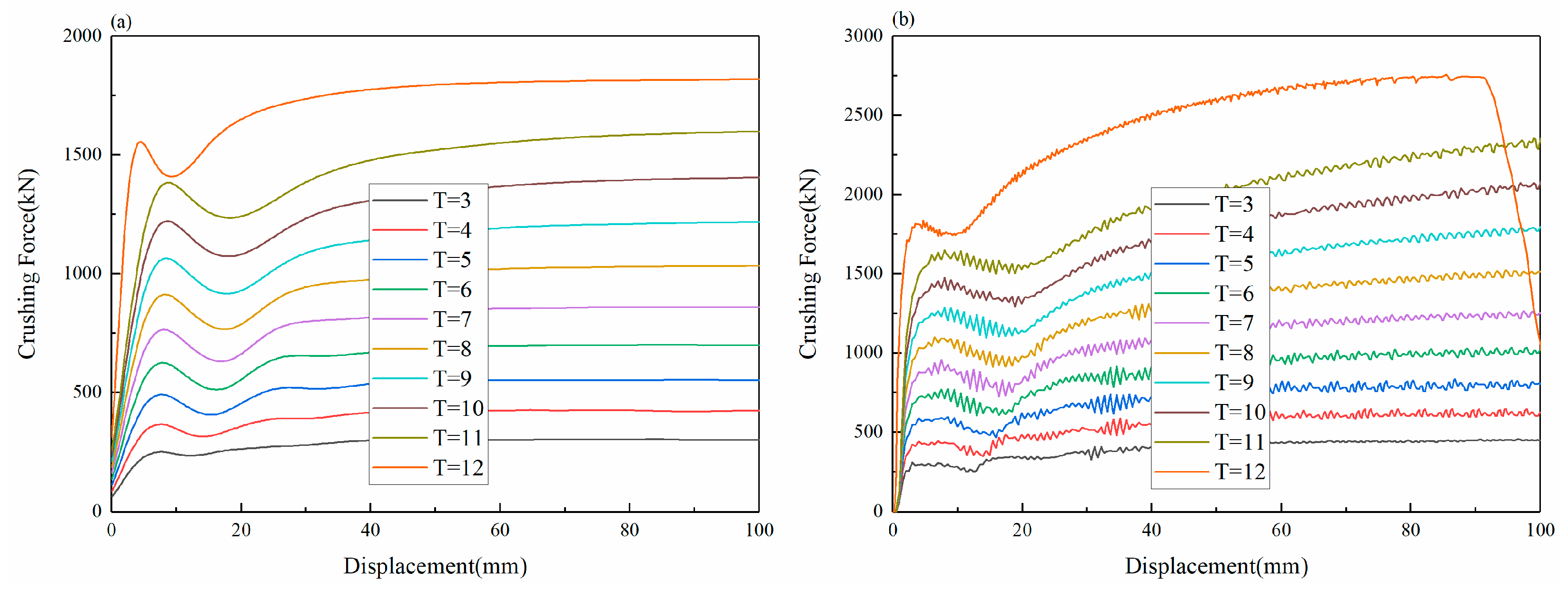
4.1. Effect of the Wall Thickness
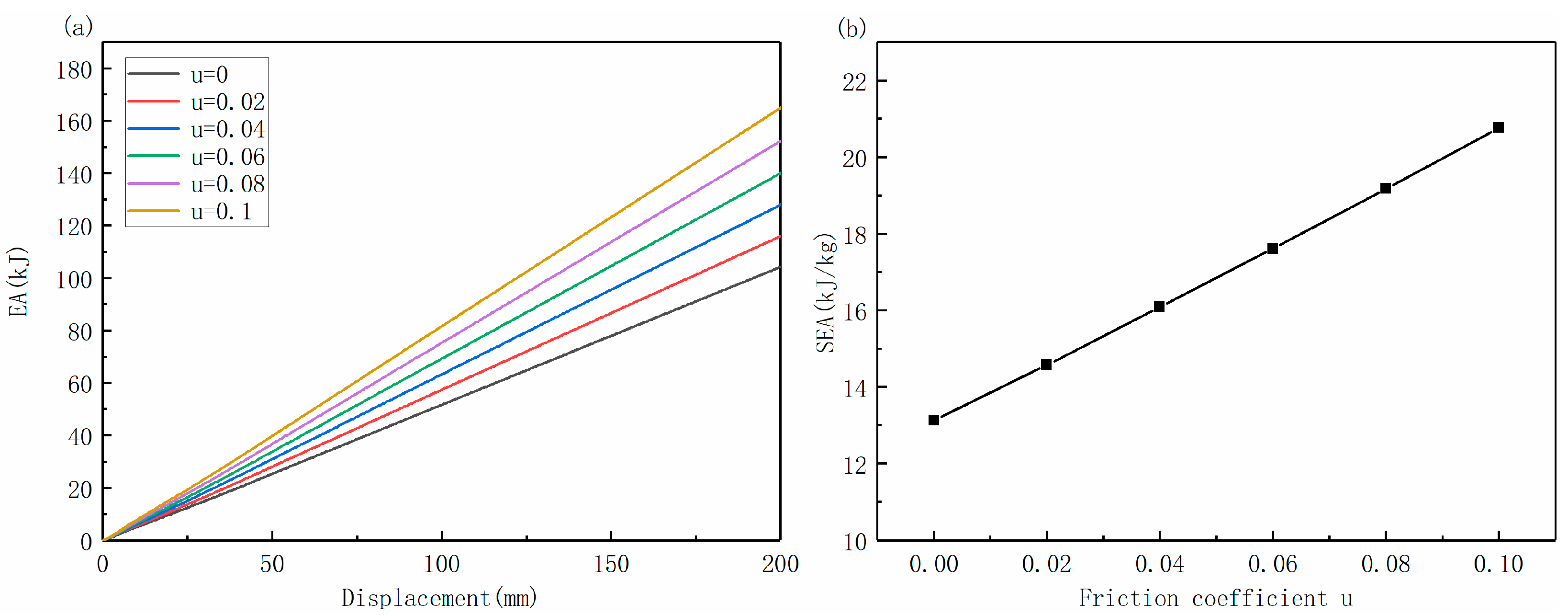
4.2. Effect of the Friction Coefficient
4.3. Effect of Axial Length of Friction Cone
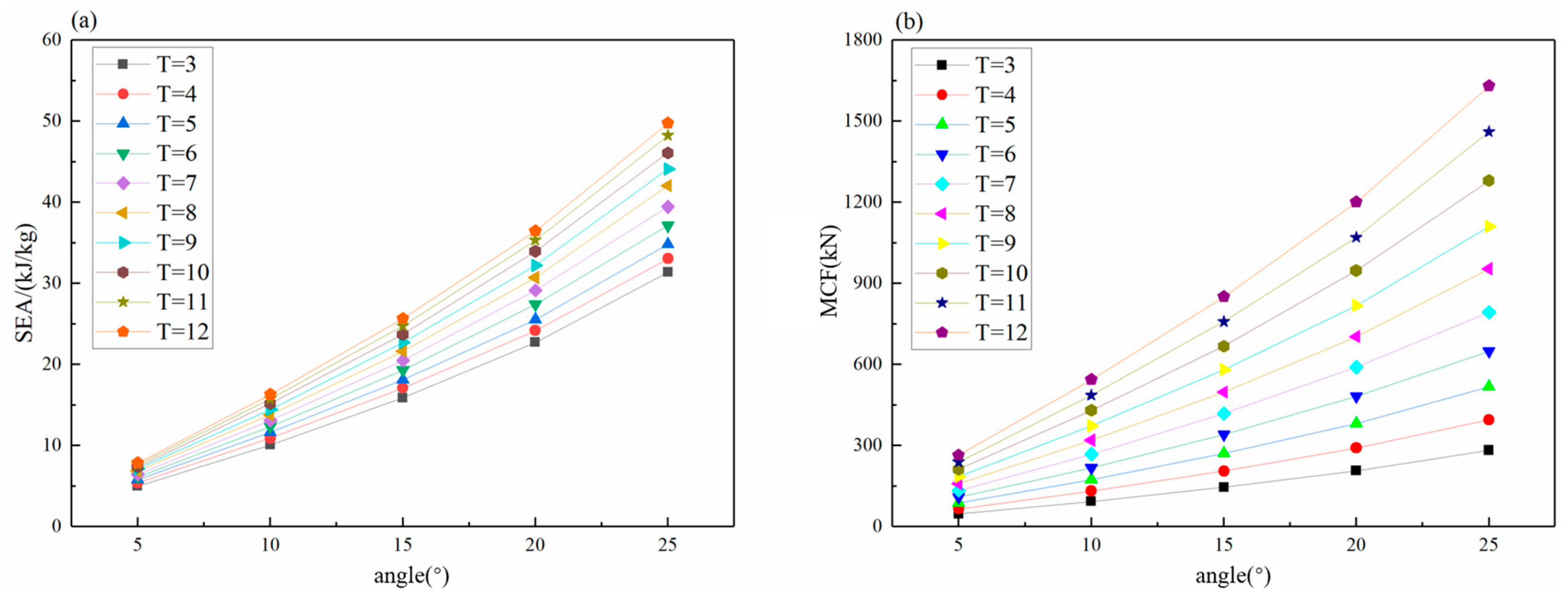
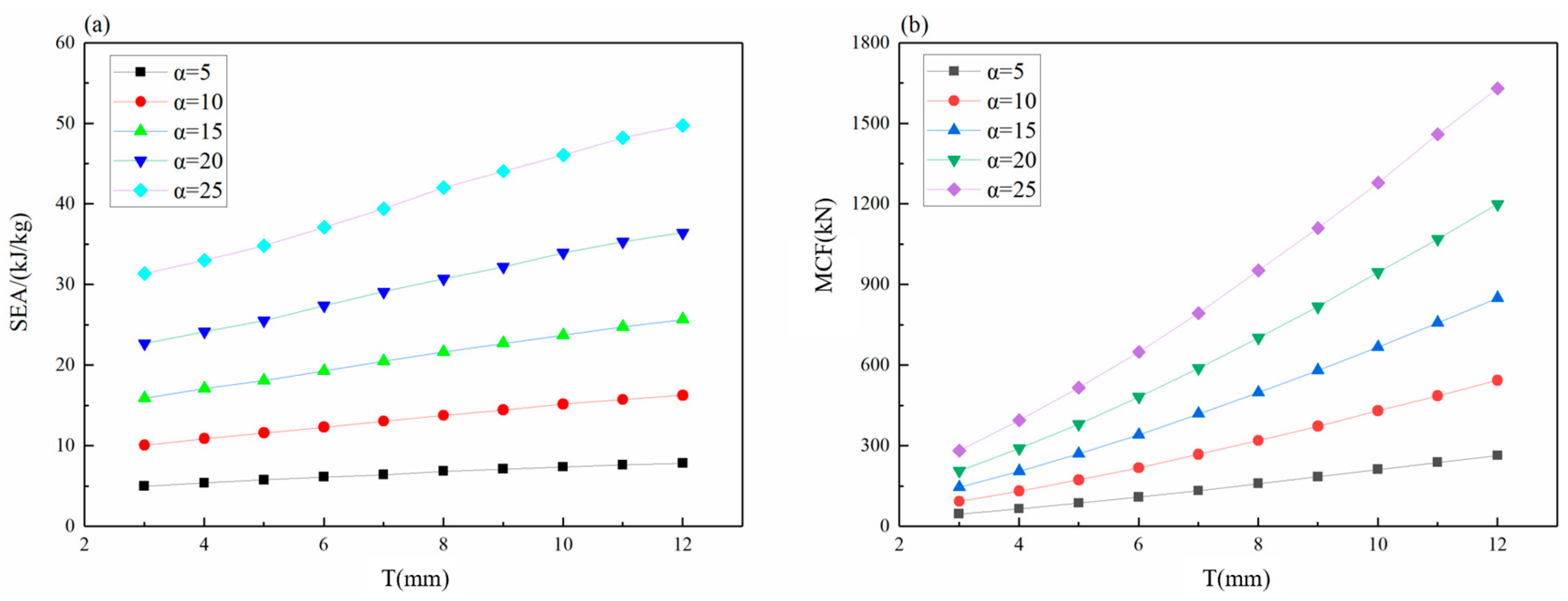
4.4. Effect of Cone Angle
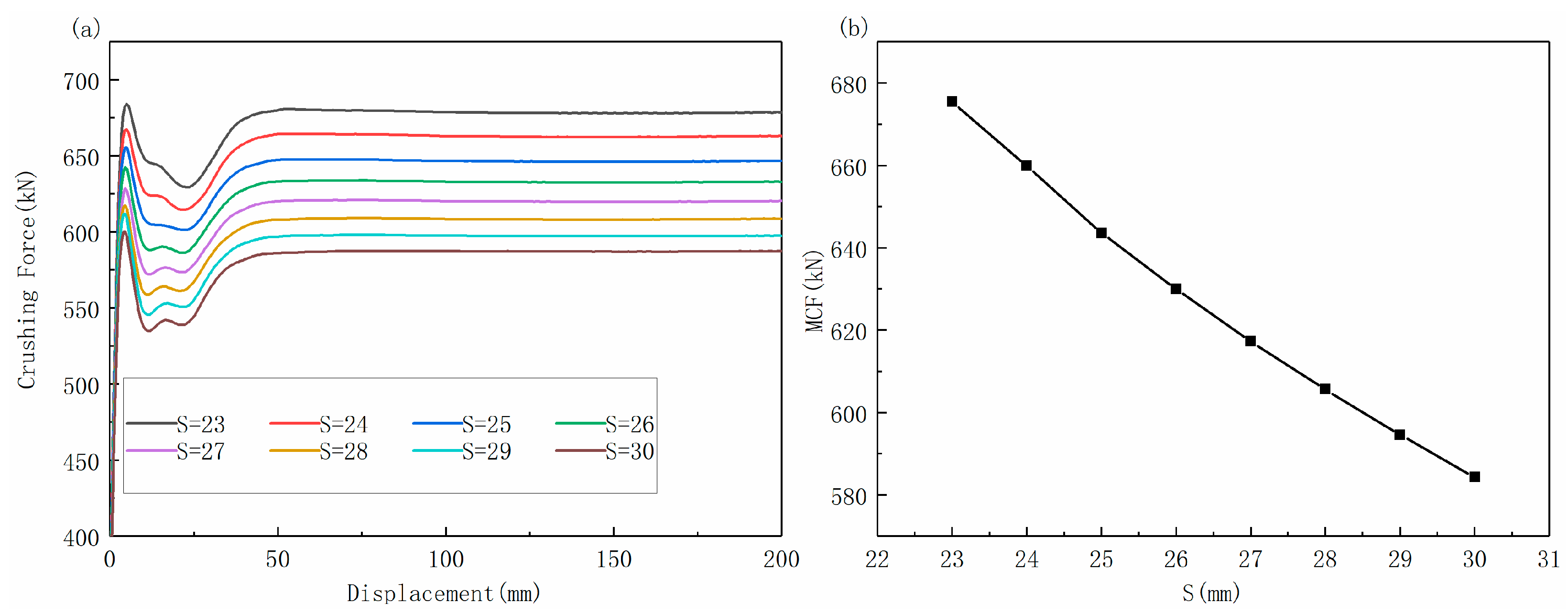
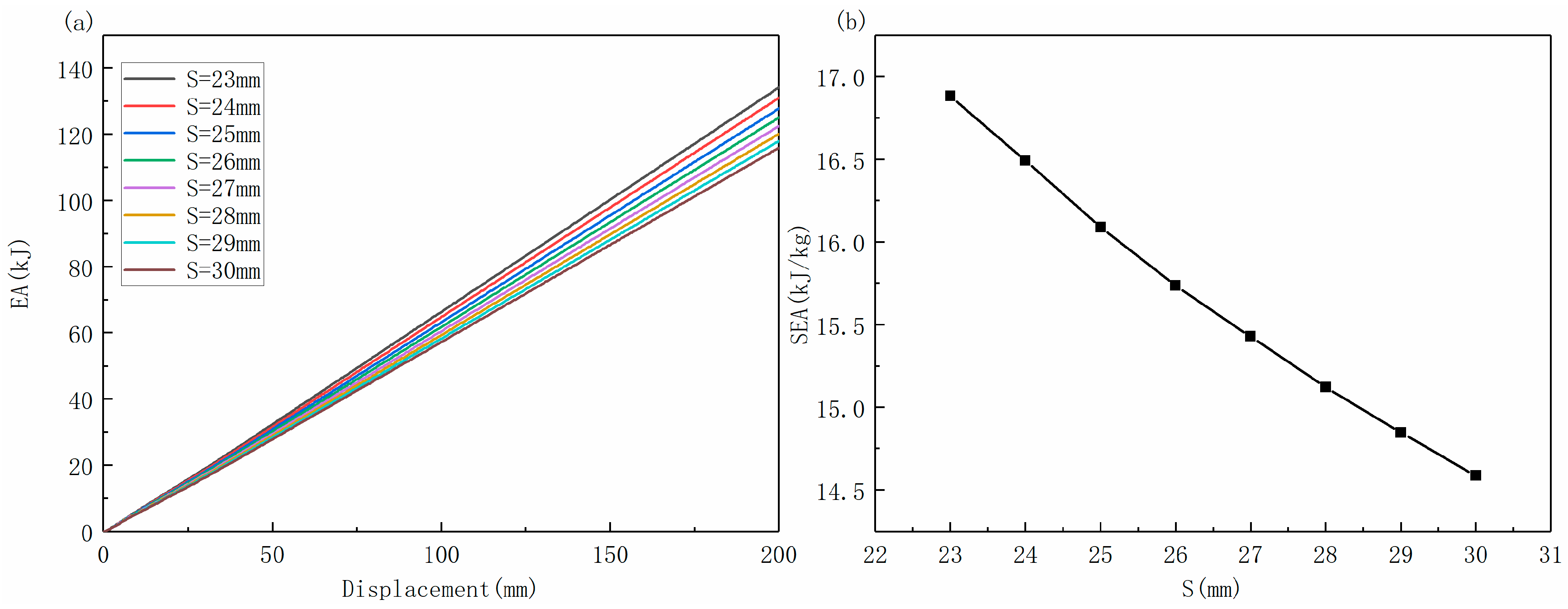
4.5. Effect of Shrinking Tube Radius
5. Discussion
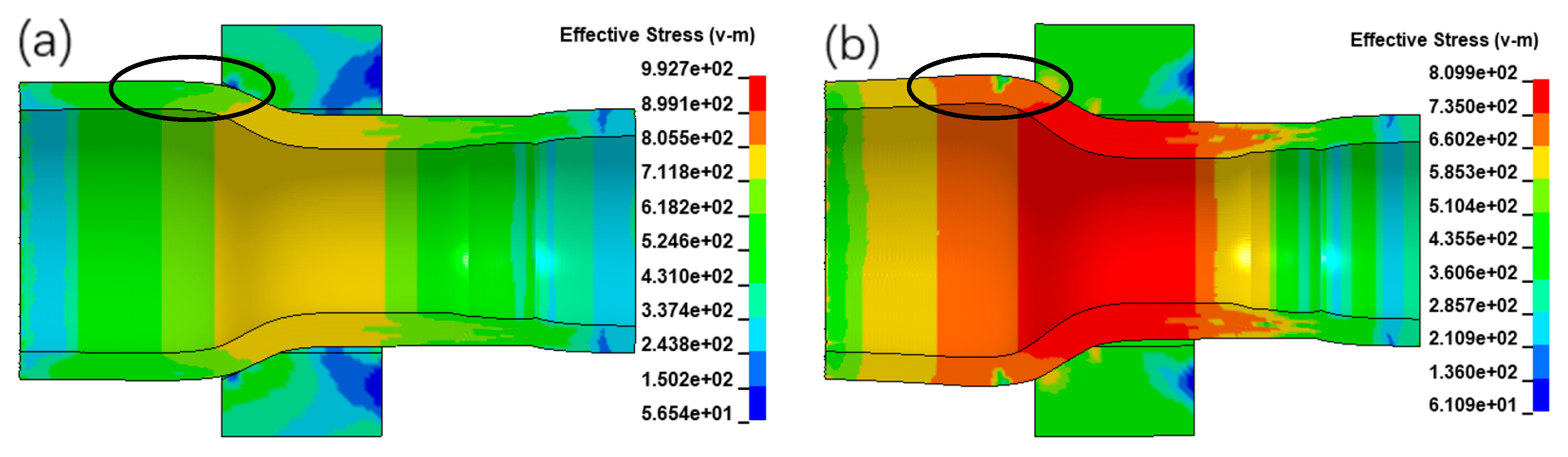
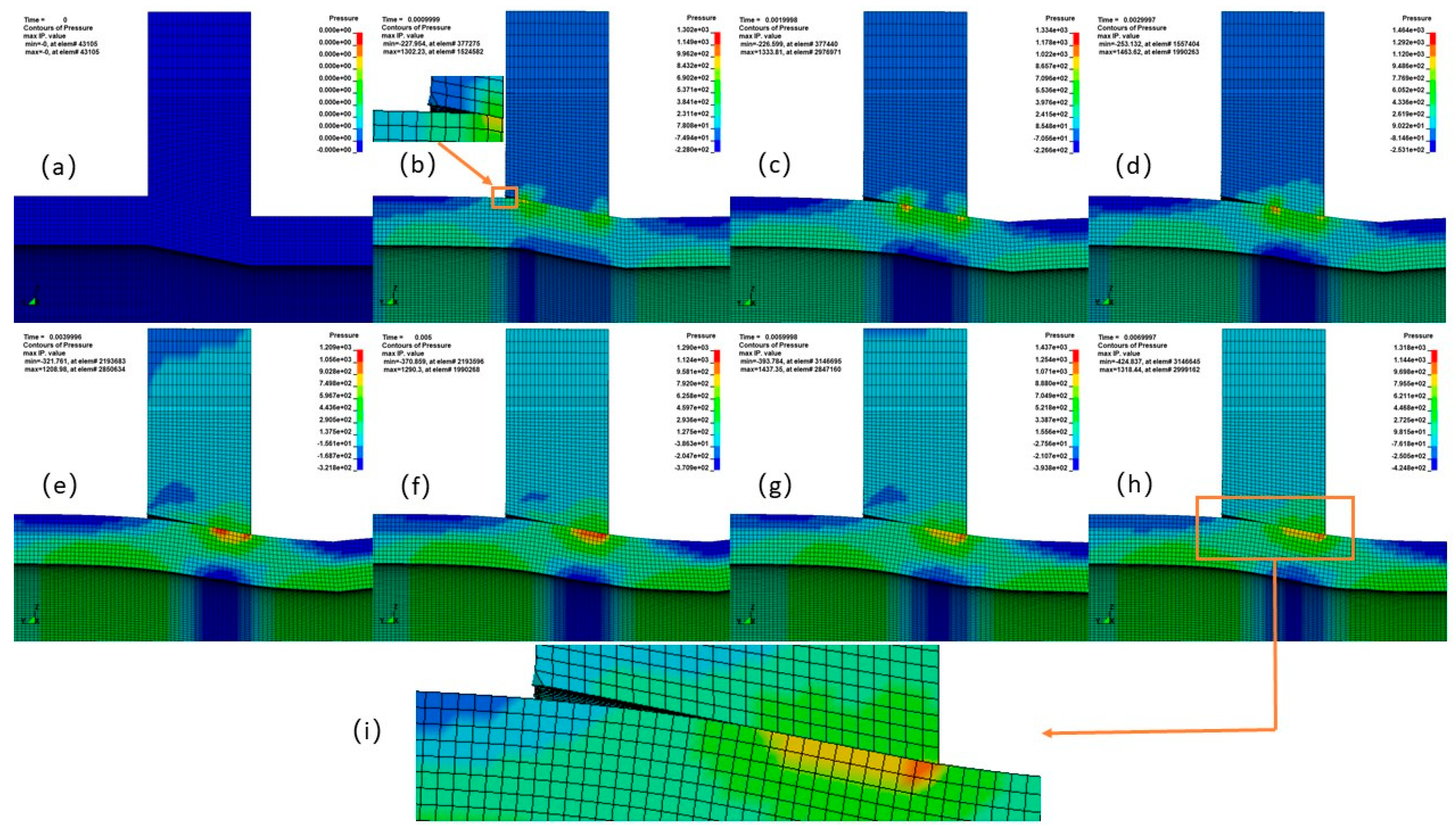
5.1. Conical Contact Length Analysis
5.2. Contact Length Change Rule
5.3. Analysis of Energy Composition
6. Conclusions
- (1)
- The MCF of the trolley test and the MCF of the numerical simulation are 642.08 kN and 644.94 kN, respectively, and they are almost consistent. The EA values of the trolley test and finite element simulation are 194.96 kJ and 198.55 kJ, respectively, and the relative error is 1.84%, which verifies the reliability of the finite element model.
- (2)
- For every 1 mm increase in the wall thickness, the MCF increases by about 45.1 kN, and the SEA increases by about 0.618 kJ/kg. When the friction coefficient increases by 0.01, the MCF increases by 30.5 kN, and the SEA increases by 0.765 kJ/kg. When the axial length of the friction cone increases by 1 mm, the MCF decreases by 13.5 kN, and the SEA decreases nonlinearly. The MCF is greatly affected by the wall thickness and friction coefficient, and it is a linear increase relationship. In contrast, the axial length of the friction cone has the smallest effect and has no linear relationship.
- (3)
- When the cone angle increases from α = 5° to α = 25°, the increase in the MCF with different thicknesses is about 600%, the increase in the SEA is more than 600%, and the MCF and SEA with the same thickness increase faster with the increase in the angle range. In the process of increasing the radius from 40 mm to 75 mm, the MCF is almost unchanged; the decrease in SEA is first faster and then slower. When the radius is increased from 40 mm to 60 mm, the decline is faster, the SEA is reduced from 33.66 kJ/kg to 21.07 kJ/kg, and the decline is slower when the radius is increased from 60 mm to 75 mm. The SEA only decreased from 21.07 kJ/kg to 17.08 kJ/kg.
- (4)
- The change of the contact length of the conical contact surface during shrinkage is analyzed, and it is found that the actual contact length of the conical surface decreases in most of the shrinkage process. The change of contact length of the friction cone under different structural parameters is compared. The contact length decreases with the increase in wall thickness, and the contact length increases with the increase in angle.
- (5)
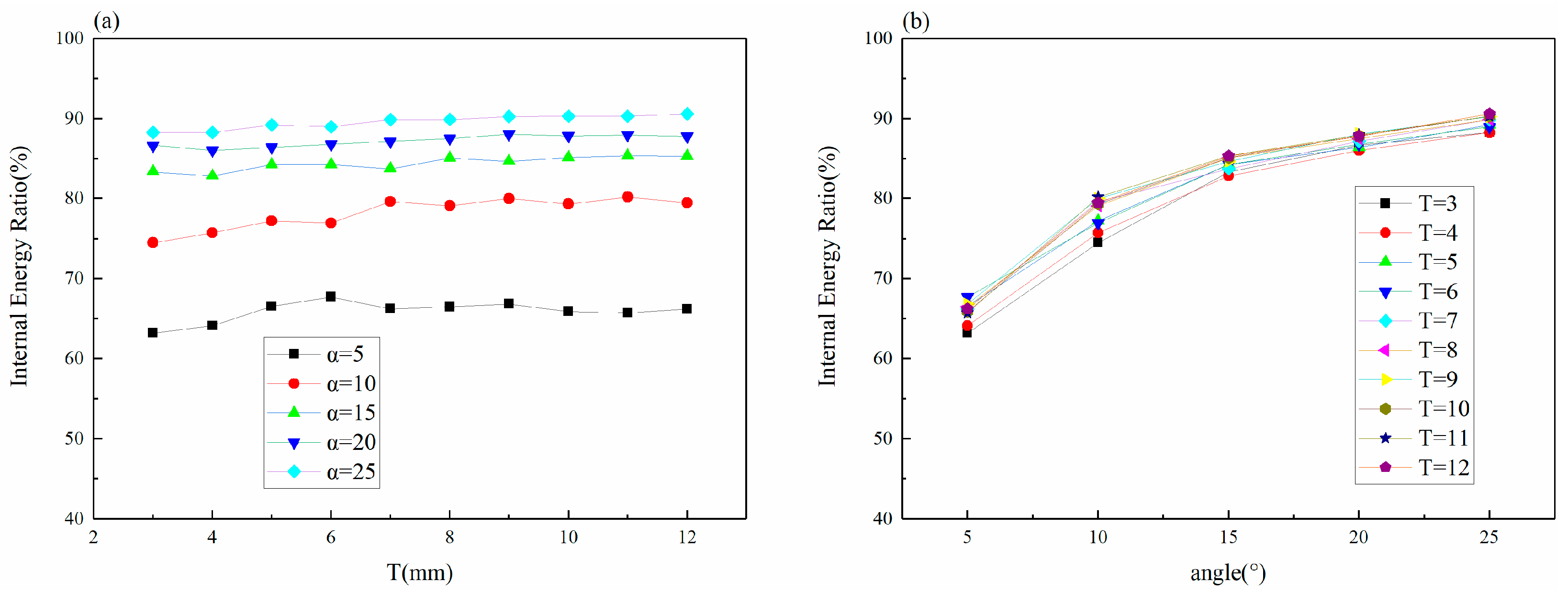
- The internal energy accounts for most of the absorption energy of the tube, and the internal energy proportion has little effect with the change of thickness but rises rapidly with the increase in the cone angle, which can be divided into two stages. When the cone angle increases from 5° to 15°, the proportion of internal energy increases greatly, from more than 60% to more than 80%. When the cone angle increases from 15° to 25°, the proportion of internal energy increases slowly, and the maximum increase is less than 6%.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Chen, Z.; Zhai, W.; Cai, C.; Sun, Y. Safety threshold of high-speed railway pier settlement based on train-track-bridge dynamic interaction. Sci. China-Technol. Sci. 2015, 58, 202–210. [Google Scholar] [CrossRef]
- Fang, J.; Gao, Y.; Sun, G.; Zhang, Y.; Li, Q. Crashworthiness design of foam-filled bitubal structures with uncertainty. Int. J. Non-Linear Mech. 2014, 67, 120–132. [Google Scholar] [CrossRef]
- Xiao, Z.; Fang, J.; Sun, G.; Li, Q. Crashworthiness design for functionally graded foam-filled bumper beam. Adv. Eng. Softw. 2015, 85, 81–95. [Google Scholar] [CrossRef]
- He, Q.; Ma, D.; Zhang, Z.; Yao, L. Mean compressive stress constitutive equation and crashworthiness optimization design of three novel honeycombs under axial compression. Int. J. Mech. Sci. 2015, 99, 274–287. [Google Scholar] [CrossRef]
- Ko, Y.; Ahn, K.; Huh, H.; Choi, W.; Jung, H.; Kwon, T. Evaluation of Crash Energy Absorption Capacity of a Tearing Tube. Exp. Appl. Mech. 2011, 6, 647–654. [Google Scholar]
- Lu, G.; Yu, T.X. Energy Absorption of Structures and Materials; Woodhead Publishing: Sawston, UK, 2003. [Google Scholar]
- Yan, J.; Yao, S.; Xu, P.; Peng, Y.; Shao, H.; Zhao, S. Theoretical prediction and numerical studies of expanding circular tubes as energy absorbers. Int. J. Mech. Sci. 2016, 105, 206–214. [Google Scholar] [CrossRef]
- Yao, S.; Yan, K.; Lu, S.; Xu, P. Prediction and application of energy absorption characteristics of thin-walled circular tubes based on dimensional analysis. Thin-Walled Struct. 2018, 130, 505–519. [Google Scholar] [CrossRef]
- Yao, S.; Li, Z.; Ma, W.; Xu, P. Crashworthiness analysis of a straight-tapered shrink tube. Int. J. Mech. Sci. 2019, 157–158, 512–527. [Google Scholar] [CrossRef]
- Liu, Y.; Qiu, X. A theoretical study of the expansion metal tubes. Int. J. Mech. Sci. 2016, 114, 157–165. [Google Scholar] [CrossRef]
- Liu, Y.; Qiu, X.; Wang, W.; Yu, T.X. An improved two-arcs deformational theoretical model of the expansion tubes. Int. J. Mech. Sci. 2017, 133, 240–250. [Google Scholar] [CrossRef]
- Yang, J.; Luo, M.; Hua, Y.; Lu, G. Energy absorption of expansion tubes using a conical–cylindrical die: Experiments and numerical simulation. Int. J. Mech. Sci. 2010, 52, 716–725. [Google Scholar] [CrossRef]
- Daxner, T.; Rammerstorfer, F.G.; Fischer, F.D. Instability phenomena during the conical expansion of circular cylindrical shells. Comput. Methods Appl. Mech. Eng. 2005, 194, 2591–2603. [Google Scholar] [CrossRef]
- Fischer, F.D.; Rammerstorfer, F.G.; Daxner, T. Flaring—An analytical approach. Int. J. Mech. Sci. 2006, 48, 1246–1255. [Google Scholar] [CrossRef]
- Shakeri, M.; Salehghaffari, S.; Mirzaeifar, R. Expansion of circular tubes by rigid tubes as impact energy absorbers: Experimental and theoretical investigation. Int. J. Crashworthiness 2007, 12, 493–501. [Google Scholar] [CrossRef]
- Seibi, A.C.; Al-Hiddabi, S.; Pervez, T. Structural Behavior of a Solid Tubular under Large Radial Plastic Expansion. J. Energy Resour. Technol. 2005, 127, 323–327. [Google Scholar] [CrossRef]
- Seibi, A.C.; Barsoum, I.; Molki, A. Experimental and Numerical Study of Expanded Aluminum and Steel Tubes. Procedia Eng. 2011, 10, 3049–3055. [Google Scholar] [CrossRef]
- Karrech, A.; Seibi, A. Analytical model for the expansion of tubes under tension. J. Mater. Process. Technol. 2010, 210, 356–362. [Google Scholar] [CrossRef]
- Liu, Y.; Qiu, X.; Yu, T.X. A theoretical model of the inversion tube over a conical die. Thin-Walled Struct. 2018, 127, 31–39. [Google Scholar] [CrossRef]
- Niknejad, A.; Moeinifard, M. Theoretical and experimental studies of the external inversion process in the circular metal tubes. Mater. Des. 2012, 40, 324–330. [Google Scholar] [CrossRef]
- Rosa, P.; Baptista, R.; Rodrigues, J.; Martins, P. An investigation on the external inversion of thin-walled tubes using a die. Int. J. Plast. 2004, 20, 1931–1946. [Google Scholar] [CrossRef]
- Rosa, P.A.R.; Rodrigues, J.M.C.; Martins, P.A.F. External inversion of thin-walled tubes using a die: Experimental and theoretical investigation. Int. J. Mach. Tools Manuf. 2003, 43, 787–796. [Google Scholar] [CrossRef]
- Yu, X.; Qiu, X.; Yu, T.X. Analysis of the free external inversion of circular tubes based on deformation theory. Int. J. Mech. Sci. 2015, 100, 262–268. [Google Scholar] [CrossRef]
- Yu, X.; Qiu, X.; Yu, T.X. Theoretical model of a metal tube under inversion over circular dies. Int. J. Mech. Sci. 2016, 108–109, 23–28. [Google Scholar] [CrossRef]
- Huang, X.; Lu, G.; Yu, T.X. On the axial splitting and curling of circular metal tubes. Int. J. Mech. Sci. 2002, 44, 2369–2391. [Google Scholar] [CrossRef]
- Moreno, C.; Beaumont, R.; Hughes, D.J.; Williams, T.; Dashwood, R. Quasi-static and dynamic testing of splitting, expansion and expansion-splitting hybrid tubes under oblique loading. Int. J. Impact Eng. 2017, 100, 117–130. [Google Scholar] [CrossRef]
- Moreno, C.; Williams, T.; Beaumont, R.; Hughes, D.J.; Dashwood, R. Testing, simulation and evaluation of a novel hybrid energy absorber. Int. J. Impact Eng. 2016, 93, 11–27. [Google Scholar] [CrossRef]
- Niknejad, A.; Rezaei, B.; Liaghat, G.H. Empty circular metal tubes in the splitting process—Theoretical and experimental studies. Thin-Walled Struct. 2013, 72, 48–60. [Google Scholar] [CrossRef]
- Rouzegar, J.; Karimi, M. Numerical and experimental study of axial splitting of circular tubular structures. Thin-Walled Struct. 2016, 105, 57–70. [Google Scholar] [CrossRef]
- Li, J.; Gao, G.; Guan, W.; Wang, S.; Yu, Y. Experimental and numerical investigations on the energy absorption of shrink circular tube under quasi-static loading. Int. J. Mech. Sci. 2018, 137, 284–294. [Google Scholar] [CrossRef]
- Almeida, B.P.P.; Alves, M.L.; Rosa, P.A.R.; Brito, A.G.; Martins, P.A.F. Expansion and reduction of thin-walled tubes using a die: Experimental and theoretical investigation. Int. J. Mach. Tools Manuf. 2006, 46, 1643–1652. [Google Scholar] [CrossRef]
- Alves, M.L.; Almeida, B.P.P.; Rosa, P.A.R.; Martins, P.A.F. End forming of thin-walled tubes. J. Mater. Process. Technol. 2006, 177, 183–187. [Google Scholar] [CrossRef]
- Tanaskovic, J.; Milkovic, D.; Lucanin, V.; Vasic Franklin, G. Experimental investigations of the shrinking–splitting tube collision energy absorber. Thin-Walled Struct. 2015, 86, 142–147. [Google Scholar] [CrossRef]
- Liu, Y.; Qiu, X. A theoretical model of the shrinking metal tubes. Int. J. Mech. Sci. 2018, 144, 564–575. [Google Scholar] [CrossRef]
- Guan, W.; Gao, G.; Yu, Y. Crushing analysis and multiobjective crashworthiness optimization of combined shrinking circular tubes under impact loading. Struct. Multidiscip. Optim. 2021, 64, 1649–1667. [Google Scholar] [CrossRef]
- Moreno, C.; Winnett, J.; Williams, T. On the effect of anisotropy on the performance and simulation of shrinking tubes used as energy absorbers for railway vehicles. Thin-Walled Struct. 2021, 161, 107513. [Google Scholar] [CrossRef]
- Fang, J.; Sun, G.; Qiu, N.; Kim, N.H.; Li, Q. On design optimization for structural crashworthiness and its state of the art. Struct. Multidiscip. Optim. 2017, 55, 1091–1119. [Google Scholar] [CrossRef]
- Gao, G.; Guan, W.; Li, J.; Dong, H.; Zou, X.; Chen, W. Experimental investigation of an active-passive integration energy absorber for railway vehicles. Thin-Walled Struct. 2017, 117, 89–97. [Google Scholar] [CrossRef]
- Xie, S.; Zhou, H. Analysis and optimisation of parameters influencing the out-of-plane energy absorption of an aluminium honeycomb. Thin-Walled Struct. 2015, 89, 169–177. [Google Scholar] [CrossRef]
- Aye, C.M.; Pholdee, N.; Yildiz, A.R.; Bureerat, S.; Sait, S.M. Multi-surrogate-assisted metaheuristics for crashworthiness optimisation. Int. J. Veh. Des. 2019, 80, 223–240. [Google Scholar] [CrossRef]
- EN 15227:2020; Railway Applications—Crashworthiness Requirements for Railway Vehicle. iTeh, Inc.: Newark, DE, USA, 2011.
- Guan, W.; Gao, G. Crashworthiness analysis of shrink circular tube energy absorbers with anti-climbers under multiple loading cases. Mech. Adv. Mater. Struct. 2022, 30, 1453–1469. [Google Scholar] [CrossRef]






| Mesh-Size (mm) | MCF (kN) | Relative Error (%) |
|---|---|---|
| 5 × 5 × 5 | 700 | 22.16 |
| 4 × 4 × 4 | 587 | 2.44 |
| 3 × 3 × 3 | 573 | - |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zou, F.; Yao, S.; Zheng, X.; Xie, M.; Yang, L. Study on Crashworthiness of Shrink Tube Anti-Creep Device. Machines 2024, 12, 720. https://doi.org/10.3390/machines12100720
Zou F, Yao S, Zheng X, Xie M, Yang L. Study on Crashworthiness of Shrink Tube Anti-Creep Device. Machines. 2024; 12(10):720. https://doi.org/10.3390/machines12100720
Chicago/Turabian StyleZou, Fan, Shuguang Yao, Xin Zheng, Minhan Xie, and Lei Yang. 2024. "Study on Crashworthiness of Shrink Tube Anti-Creep Device" Machines 12, no. 10: 720. https://doi.org/10.3390/machines12100720
APA StyleZou, F., Yao, S., Zheng, X., Xie, M., & Yang, L. (2024). Study on Crashworthiness of Shrink Tube Anti-Creep Device. Machines, 12(10), 720. https://doi.org/10.3390/machines12100720





