Synthesis and Prototyping of a Sit-to-Stand Assisting Device †
Abstract
1. Introduction
2. Problem Formulation
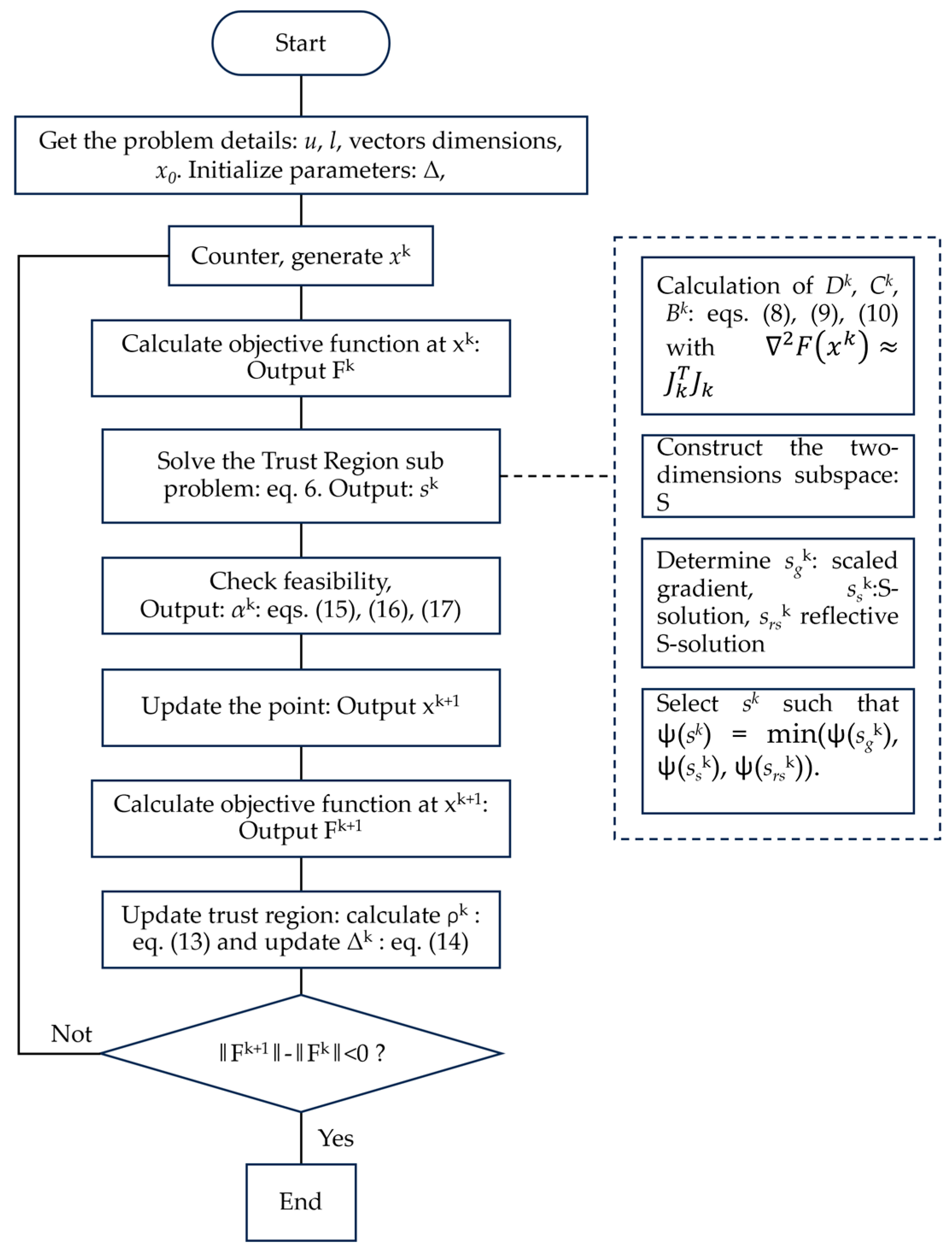
Numerical Algorithm
- Generate iterate xk such that xk ϵ int(F), int(F) = {x ϵ Rn+m|l < x < u};
- Solve the Trust-region sub problem such to obtain sk;
- Calculate ψ(sk);
- Truncate the trail step sk by αk such that xk+1 = xk + αk sk was strictly feasible.
- Calculate and accordingly update Δk;
- Accept the iterate if ‖Fk+1‖ < ‖Fk‖ with Δk unchanged, otherwise go to the first step with an updated Δk according to ρk calculation of step 5.
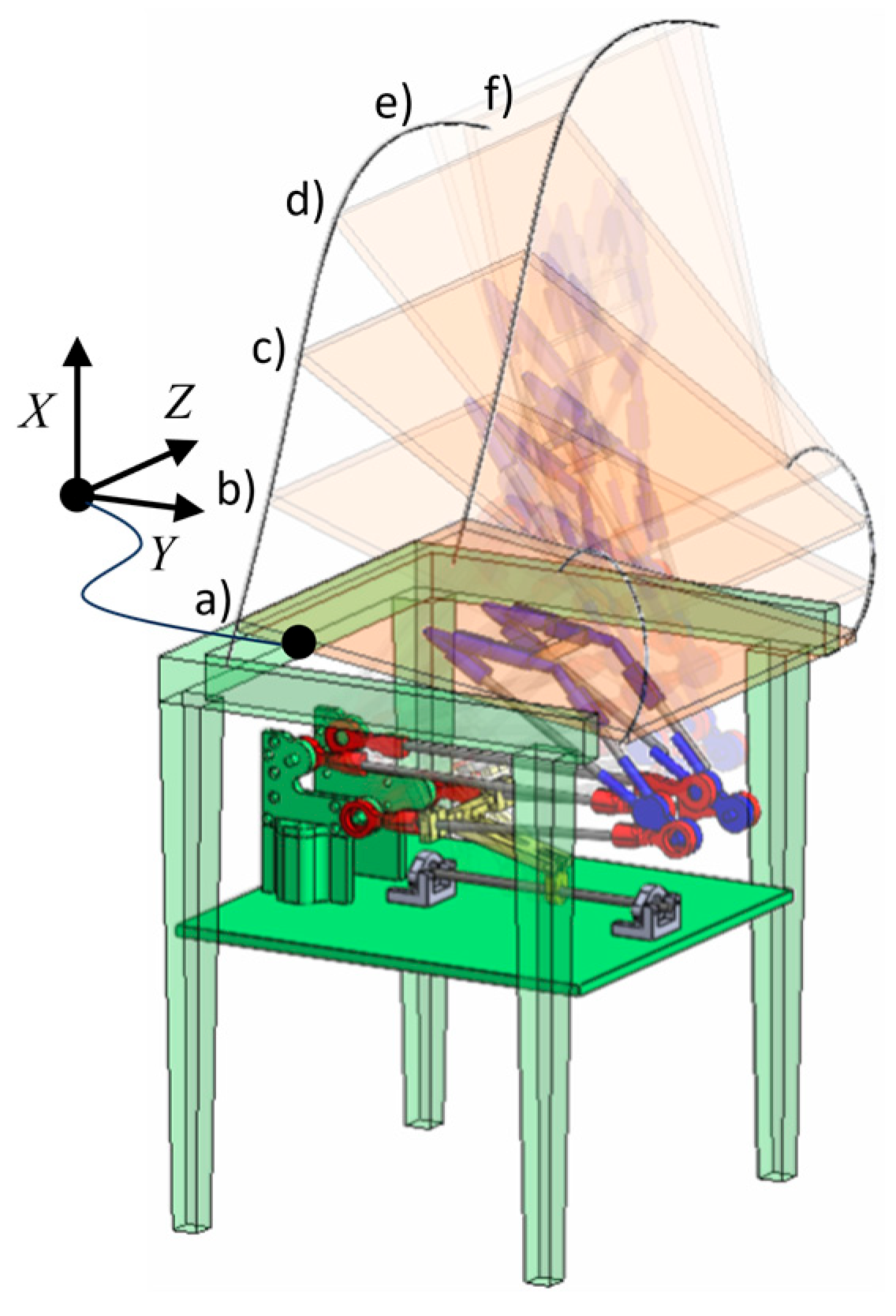
3. The STS Assisting Device
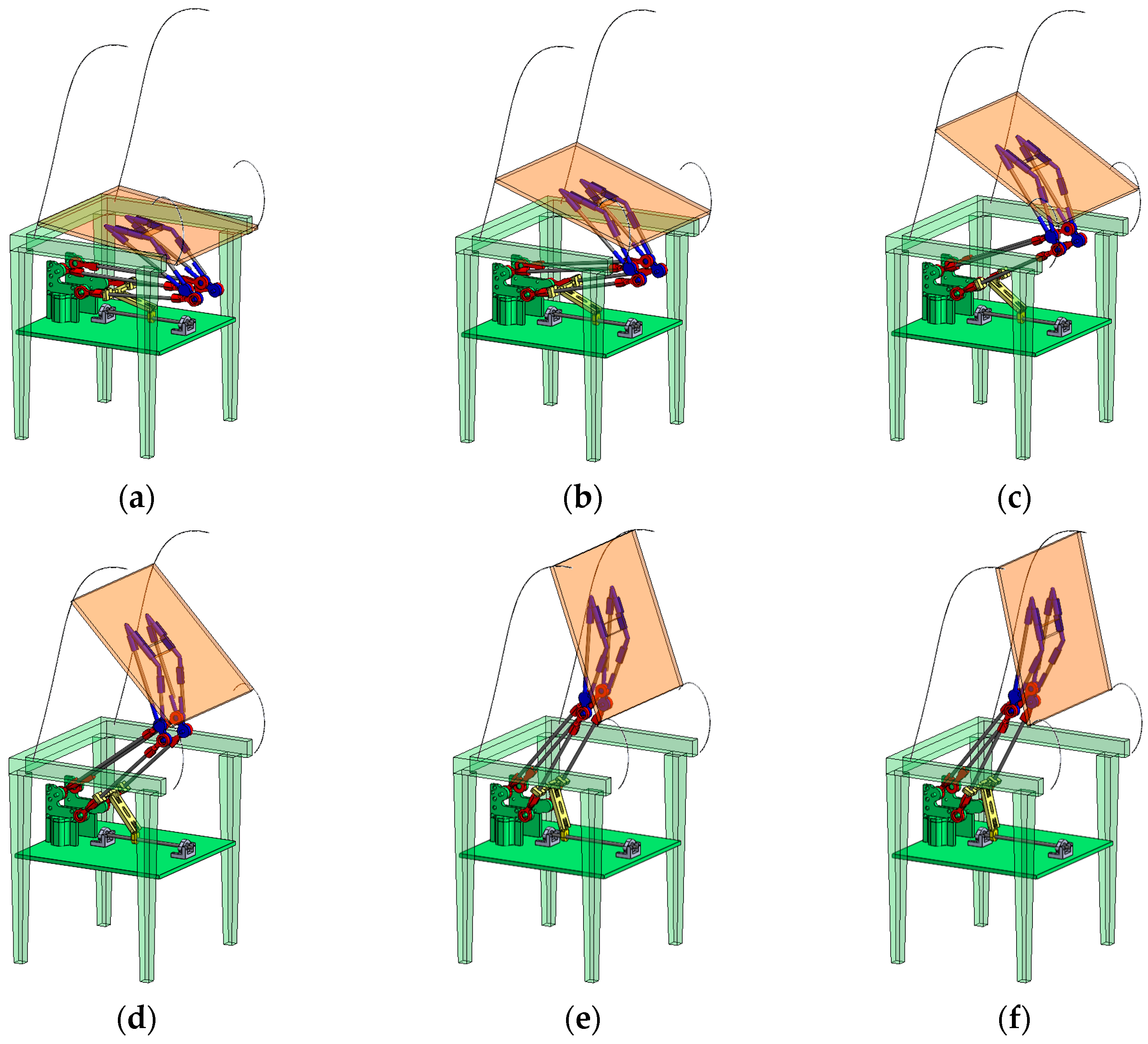
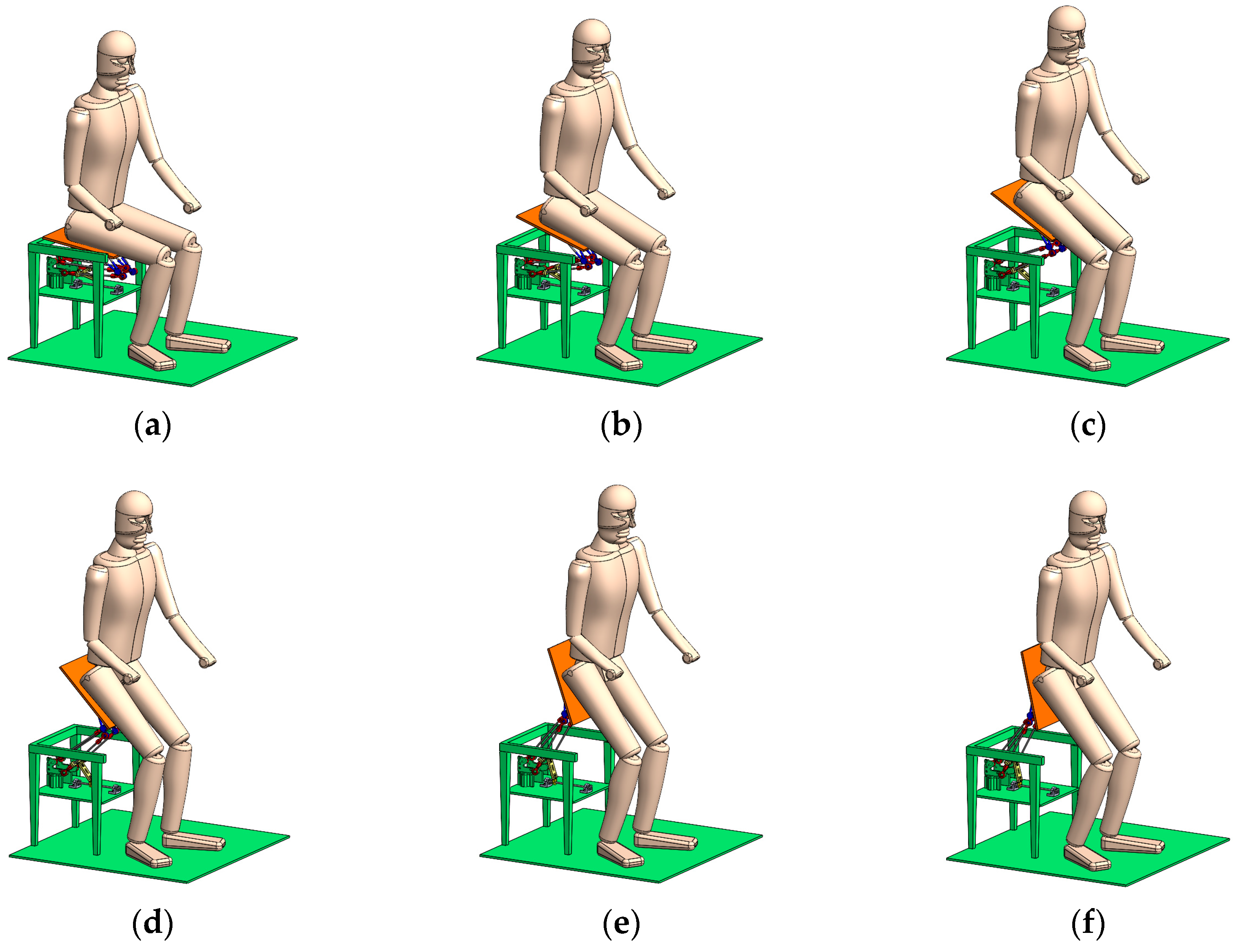
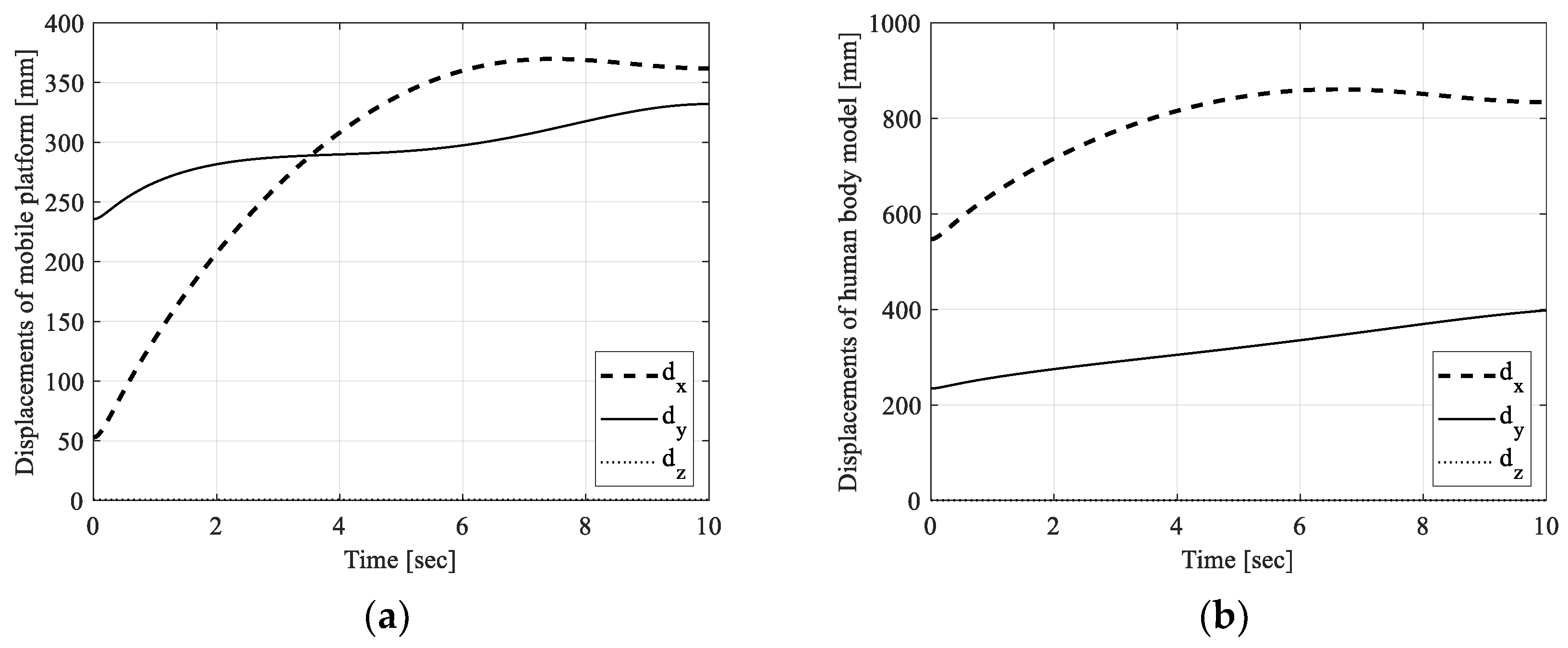
Simulation of the Device
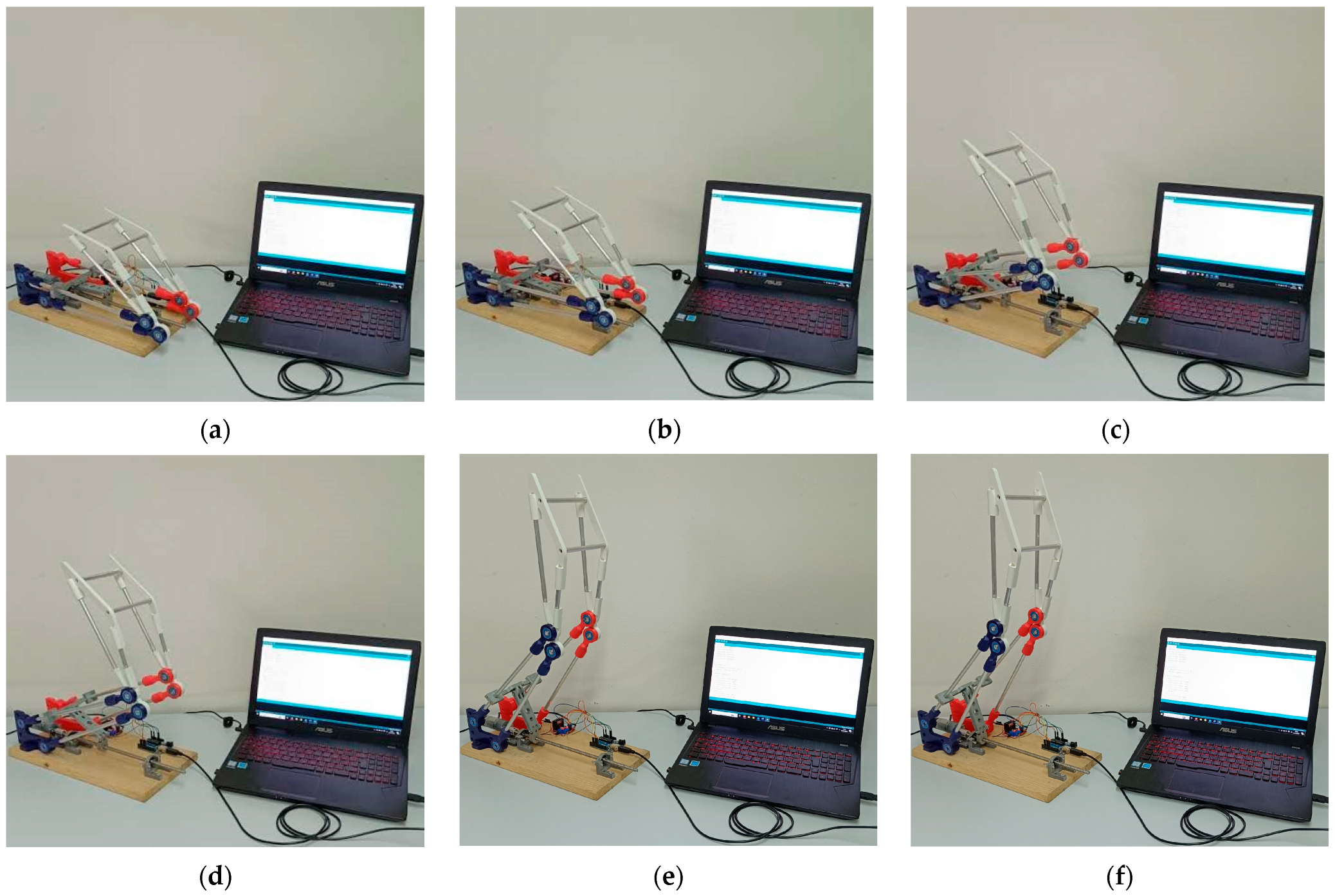
4. Prototyping and Mechatronic Design
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Ioannidis, J.P.A.; Axfors, C.; Contopoulos-Ioannidis, D.G. Population-level COVID-19 mortality risk for non-elderly individuals overall and for non-elderly individuals without underlying diseases in pandemic epicenters. Environ. Res. 2020, 188, 109890. [Google Scholar] [CrossRef]
- De Pue, S.; Gillebert, C.; Dierckx, E.; Vanderhasselt, M.A.; De Raedt, R.; Van den Bussche, E. The impact of the COVID-19 pandemic on wellbeing and cognitive functioning of older adults. Sci. Rep. 2021, 11, 4636. [Google Scholar] [CrossRef] [PubMed]
- World Health Organization. Assistive Product Specifications and How to Use Them; Electronic Version; World Health Organization: Geneva, Switzerland, 2023; ISBN 978-92-4-002028-3. [Google Scholar]
- Sorli, M.; Figliolini, G.; Pastorelli, S.; Rea, P. Experimental identification and validation of a pneumatic positioning servo-system. In Power Transmission and Motion Control, PTMC 2005; John Wiley & Sons: Hoboken, NJ, USA, 2005; pp. 365–378. [Google Scholar]
- Ceccarelli, M.; Ottaviano, E.; Galvagno, M. A 3-DOF Parallel Manipulator as Earthquake Motion Simulator. In Proceedings of the 7th International Conference on Control, Automation, Robotics and Vision, ICARCV 2002, Singapore, 2–5 December 2002; pp. 944–949. [Google Scholar]
- Kapsalyamov, A.; Jamwal, P.K.; Hussain, S.; Ghayesh, M.H. State of the Art Lower Limb Robotic Exoskeletons for Elderly Assistance. IEEE Access 2019, 7, 95075–95086. [Google Scholar] [CrossRef]
- Yan, T.; Cempini, M.; Oddo, C.M.; Vitiello, N. Review of assistive strategies in powered lower-limb orthoses and exoskeletons. Robot. Auton. Syst. 2015, 64, 120–136. [Google Scholar] [CrossRef]
- Nakamura, K.; Saga, N. Current Status and Consideration of Support/Care Robots for Stand-Up Motion. Appl. Sci. 2021, 11, 1711. [Google Scholar] [CrossRef]
- Ruggiu, M. Kinematic and dynamic analysis of a two-degree-of-freedom spherical wrist. J. Mech. Robot. 2010, 2, 031006. [Google Scholar] [CrossRef]
- Deidda, R.; Mariani, A.; Ruggiu, M. On the kinematics of the 3-RRUR spherical parallel manipulator. Robotica 2010, 28, 821–832. [Google Scholar] [CrossRef]
- Imamura, Y.; Endo, Y.; Yoshida, E. Simulation–based Design of Transfer Support Robot and Experimental Verification. In Proceedings of the 2019 2nd IEEE International Conference on Soft Robotics (RoboSoft), Seoul, Republic of Korea, 14–18 April 2019. [Google Scholar]
- Santos, A.V.F.; Licursi, L.A.; Amaral, M.F.; Cavalcanti, A.; Silveira, Z.C. User-centered design of a customized assistive device to support feeding. Procedia CIRP 2019, 84, 743–748. [Google Scholar]
- Scaletta, T.; Komada, S.; Oboe, R. Development of a Human Assistive Robot to Support Hip Joint Movement during Sit-to-stand Using Non-linear Springs. IEEJ J. Ind. Appl. 2016, 5, 261–266. [Google Scholar] [CrossRef]
- Roebroeck, M.E.; Doorenbosch, C.A.; Harlaar, J.; Jacobs, R.; Lankhorst, G.J. Biomechanics and muscular activity during sit-to-stand transfer. Clin. Biomech. 1994, 9, 235–244. [Google Scholar] [CrossRef]
- Graham Lift. Available online: https://www.vitalitymedical.com/lumex-stand-assist-patient-transport-lift-graham-field-lf1600.html (accessed on 10 November 2023).
- QuickMove. Webpage. Available online: https://www.handicare.com/en/product/quickmove/ (accessed on 10 November 2023).
- Molift Quick Raiser 2, Webpage. Available online: https://www.etac.com/products/patient-handling/sit-to-stand-aids/molift-quick-raiser-2/ (accessed on 10 November 2023).
- Zhou, B.; Xue, Q.; Yang, S.; Zhang, H.; Wang, T. Design and control of a sit-to-stand assistive device based on analysis of kinematics and dynamics. Automatika 2021, 62, 353–364. [Google Scholar] [CrossRef]
- Hojjati Najafabadi, A.; Amini, S.; Farahmand, F. Mechanical Design and Simulation of a Saddle-Assistive Device for Sit-to-Stand Transfer in Healthy Subjects. Int. J. Adv. Des. Manuf. Technol. 2017, 10, 37–44. [Google Scholar]
- Liang, X.; Chen, F. A Study on Design of Adaptive Assistant Devices for the Sit-To-Stand. In Proceedings of the 2021 IEEE International Conference on Power, Intelligent Computing and Systems (ICPICS), Shenyang, China, 29–31 July 2021; pp. 196–201. [Google Scholar] [CrossRef]
- Biomechanics Dataset of Healthy Human Walking at Various Conditions. Available online: https://github.com/timvanderzee/human-walking-biomechanics (accessed on 10 October 2023).
- Nandikolla, V.K.; Bochen, R.; Meza, S.; Garcia, A. Experimental Gait Analysis to Study Stress Distribution of the Human Foot. J. Med. Eng. 2017, 2017, 3432074. [Google Scholar] [CrossRef] [PubMed]
- Ottaviano, E.; Ceccarelli, M.; Palmucci, F. An application of CaTraSys, a cable-based parallel measuring system for an experimental characterization of human walking. Robotica 2010, 28, 119–133. [Google Scholar] [CrossRef]
- Rea, P.; Ottaviano, E. Functional Design for Customizing Sit-To-Stand Assisting Devices. J. Bionic Eng. 2018, 15, 83–93. [Google Scholar] [CrossRef]
- Pasinetti, S.; Nuzzi, C.; Covre, N.; Luchetti, A.; Maule, L.; Serpelloni, M.; Lancini, M. Validation of Marker-Less System for the Assessment of Upper Joints Reaction Forces in Exoskeleton Users. Sensors 2020, 20, 3899. [Google Scholar] [CrossRef] [PubMed]
- Lam, W.W.T.; Tang, Y.M.; Fong, K.N.K. A systematic review of the applications of markerless motion capture (MMC) technology for clinical measurement in rehabilitation. J. NeuroEng. Rehabil. 2023, 20, 57. [Google Scholar] [CrossRef]
- Rahul, M. Review on motion capture technology. Glob. J. Comput. Sci. Technol. 2018, 18, 3–6. [Google Scholar]
- Corazza, S.; Mündermann, L.; Gambaretto, E.; Ferrigno, G.; Andriacchi, T.P. Markerless motion capture through visual hull, articulated ICP and subject specific model generation. Int. J. Comput. Vis. 2010, 87, 156–169. [Google Scholar] [CrossRef]
- Alves, J.; Lima, T.M.; Gaspar, P.D. Novel Design of Assistive Technologies Based on the Interconnection of Motion Capture and Virtual Reality Systems to Foster Task Performance of the Ageing Workforce. Designs 2023, 7, 23. [Google Scholar] [CrossRef]
- Muñoz, D.; De Marchis, C.; Gizzi, L.; Severini, G. Predictive simulation of sit-to-stand based on reflexive-controllers. PLoS ONE 2022, 17, e0279300. [Google Scholar] [CrossRef] [PubMed]
- Raisin, S.N.; Jamaludin, J.; Jamal, M.; Farah, A.; Hazwani, N.; Naeem, B. Cyber-Physical System (CPS) Application—A Review. REKA ELKOMIKA J. Pengabdi. Kpd. Masy. 2020, 1, 52–65. [Google Scholar] [CrossRef]
- Arafsha, F.; Laamarti, F.; El Saddik, A. Cyber-Physical System Framework for Measurement and Analysis of Physical Activities. Electronics 2019, 8, 248. [Google Scholar] [CrossRef]
- El Saddik, A. Digital Twins: The Convergence of Multimedia Technologies. IEEE Multimed. 2018, 25, 87–92. [Google Scholar] [CrossRef]
- Lu, Y.; Zheng, H.; Chand, S.; Xia, W.; Liu, Z.; Xu, X.; Wang, L.; Qin, Z.; Bao, J. Outlook on human-centric manufacturing towards Industry 5.0. J. Manuf. Syst. 2022, 62, 612–627. [Google Scholar] [CrossRef]
- Norheim, K.L.; Samani, A.; Madeleine, P. The effects of age on response time, accuracy, and shoulder/arm kinematics during hammering. Appl. Ergon. 2020, 90, 103157. [Google Scholar] [CrossRef] [PubMed]
- Kačerová, I.; Kubr, J.; Hořejší, P.; Kleinová, J. Ergonomic Design of a Workplace Using Virtual Reality and a Motion Capture Suit. Appl. Sci. 2022, 12, 2150. [Google Scholar] [CrossRef]
- Babadi, S.Y.; Daneshmandi, H. Effects of virtual reality versus conventional balance training on balance of the elderly. Exp. Gerontol. 2021, 153, 111498. [Google Scholar] [CrossRef]
- Liagkou, V.; Salmas, D.; Stylios, C. Stylios, Realizing Virtual Reality Learning Environment for Industry 4.0. Procedia CIRP 2019, 79, 712–717. [Google Scholar] [CrossRef]
- Abuwarda, Z.; Mostafa, K.; Oetomo, A.; Hegazy, T.; Morita, P. Wearable devices: Cross benefits from healthcare to construction. Autom. Constr. 2022, 142, 104501. [Google Scholar] [CrossRef]
- Lemos, J.; Gaspar, P.D.; Lima, T.M. Individual Environmental Risk Assessment and Management in Industry 4.0: An IoT-Based Model. Appl. Syst. Innov. 2022, 5, 88. [Google Scholar] [CrossRef]
- McDevitt, S.; Hernandez, H.; Hicks, J.; Lowell, R.; Bentahaikt, H.; Burch, R.; Ball, J.; Chander, H.; Freeman, C.; Taylor, C.; et al. Wearables for Biomechanical Performance Optimization and Risk Assessment in Industrial and Sports Applications. Bioengineering 2022, 9, 33. [Google Scholar] [CrossRef] [PubMed]
- Nikolakis, N.; Maratos, V.; Makris, S. A cyber physical system (CPS) approach for safe human-robot collaboration in a shared workplace. Robot. Comput.-Integr. Manuf. 2019, 56, 233–243. [Google Scholar] [CrossRef]
- Balan, L.; Bone, G.M. Real-time 3D Collision Avoidance Method for Safe Human and Robot Coexistence. In Proceedings of the 2006 IEEE/RSJ International Conference on Intelligent Robots and Systems, Beijing, China, 9–15 October 2006; pp. 276–282. [Google Scholar] [CrossRef]
- Flacco, F.; Kröger, T.; De Luca, A.; Khatib, O. A depth space approach to human-robot collision avoidance. In Proceedings of the 2012 IEEE International Conference on Robotics and Automation, Saint Paul, MN, USA, 14–18 May 2012; pp. 338–345. [Google Scholar] [CrossRef]
- Burmester, L. Lehrbuch der Kinematik; A. Felix: Leipzig, Germany, 1888. [Google Scholar]
- Sandor, G.N.; Erdman, A. Advanced Mechanism Design: Analysis and Synthesis; Prentice-Hall, Inc.: Hoboken, NJ, USA, 1984; Volume 2. [Google Scholar]
- Ravani, B.; Roth, B. Motion synthesis using kinematic mappings. ASME J. Mech. Transm. Autom. Des. 1983, 105, 460–467. [Google Scholar] [CrossRef]
- Hayes, M.; Zsombor-Murray, P. Solving the Burmester problem using kinematic mapping. In Proceedings of the ASME 2002 International Design Engineering Technical Conferences and Computers and Information in Engineering Conference, Proc. ASME DETC’2002, Montreal, QC, Canada, 29 September–2 October 2002. #MECH-34378. [Google Scholar]
- Brunnthaler, K.; Pfurner, M.; Husty, M. Synthesis of Planar Four-Bar Mechanisms. Trans. CSME 2006, 30, 297–313. [Google Scholar] [CrossRef]
- Bottema, O.; Roth, B. Theoretical Kinematics; North-Holland Pub. Co.: New York, NY, USA, 1979. [Google Scholar]
- Hunt, K.H. Kinematic Geometry of Mechanisms; Oxford University Press: New York, NY, USA, 1978. [Google Scholar]
- McCarthy, J.M. Geometric Design of Linkages; Springer: New York, NY, USA, 2000. [Google Scholar]
- Yao, J.; Angeles, J. Computation of all optimum dyads in the approximate synthesis of planar linkages for rigid-body guidance. Mech. Mach. Theory 2000, 35, 1065–1078. [Google Scholar] [CrossRef]
- Coleman, T.F.; Li, Y. An Interior, Trust Region Approach for Nonlinear Minimization Subject to Bounds. SIAM J. Optim. 1996, 6, 418–445. [Google Scholar] [CrossRef]
- Reimer, S.M.F.; Abdul-Sater, K.; Lueth, T.C. Bio-Kinematic Design of Individualized Lift-Assist Devices. New Trends Med. Serv. Robot. Mech. Mach. Sci. 2018, 48, 59–72. [Google Scholar]
- Rea, P.; Ruggiu, M.; Ottaviano, E. A Sit-to-Stand Assisting Device for Accomplishing Daily-Life Activities. In Advances in Mechanism and Machine Science, Proceedings of the 16th IFToMM World Congress 2023, Tokyo, Japan, 5–10 November 2023; Mechanisms and Machine Science; Okada, M., Ed.; Springer: Cham, Switzerland, 2023; Volume 147. [Google Scholar] [CrossRef]
- Figliolini, G.; Rea, P. Overall design of Ca.U.M.Ha. robotic hand for harvesting horticulture products. Robotica 2006, 24, 329–331. [Google Scholar] [CrossRef]



| j | |||
|---|---|---|---|
| 1 | 0.0101 | 0.0010 | 0.1120 |
| 2 | 0.0143 | 0.0026 | 0.3113 |
| 3 | 0.0072 | 0.0040 | 0.2175 |
| 4 | 0.0146 | 0.0098 | 0.4528 |
| 5 | 0.0033 | 0.0049 | 0.0591 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rea, P.; Ruggiu, M.; Ottaviano, E. Synthesis and Prototyping of a Sit-to-Stand Assisting Device. Machines 2024, 12, 33. https://doi.org/10.3390/machines12010033
Rea P, Ruggiu M, Ottaviano E. Synthesis and Prototyping of a Sit-to-Stand Assisting Device. Machines. 2024; 12(1):33. https://doi.org/10.3390/machines12010033
Chicago/Turabian StyleRea, Pierluigi, Maurizio Ruggiu, and Erika Ottaviano. 2024. "Synthesis and Prototyping of a Sit-to-Stand Assisting Device" Machines 12, no. 1: 33. https://doi.org/10.3390/machines12010033
APA StyleRea, P., Ruggiu, M., & Ottaviano, E. (2024). Synthesis and Prototyping of a Sit-to-Stand Assisting Device. Machines, 12(1), 33. https://doi.org/10.3390/machines12010033









