Design of Z Profile Roller Tooling and Research on Roller Clearance
Abstract
1. Introduction
2. Materials and Methods
2.1. Roll-Bending Principle
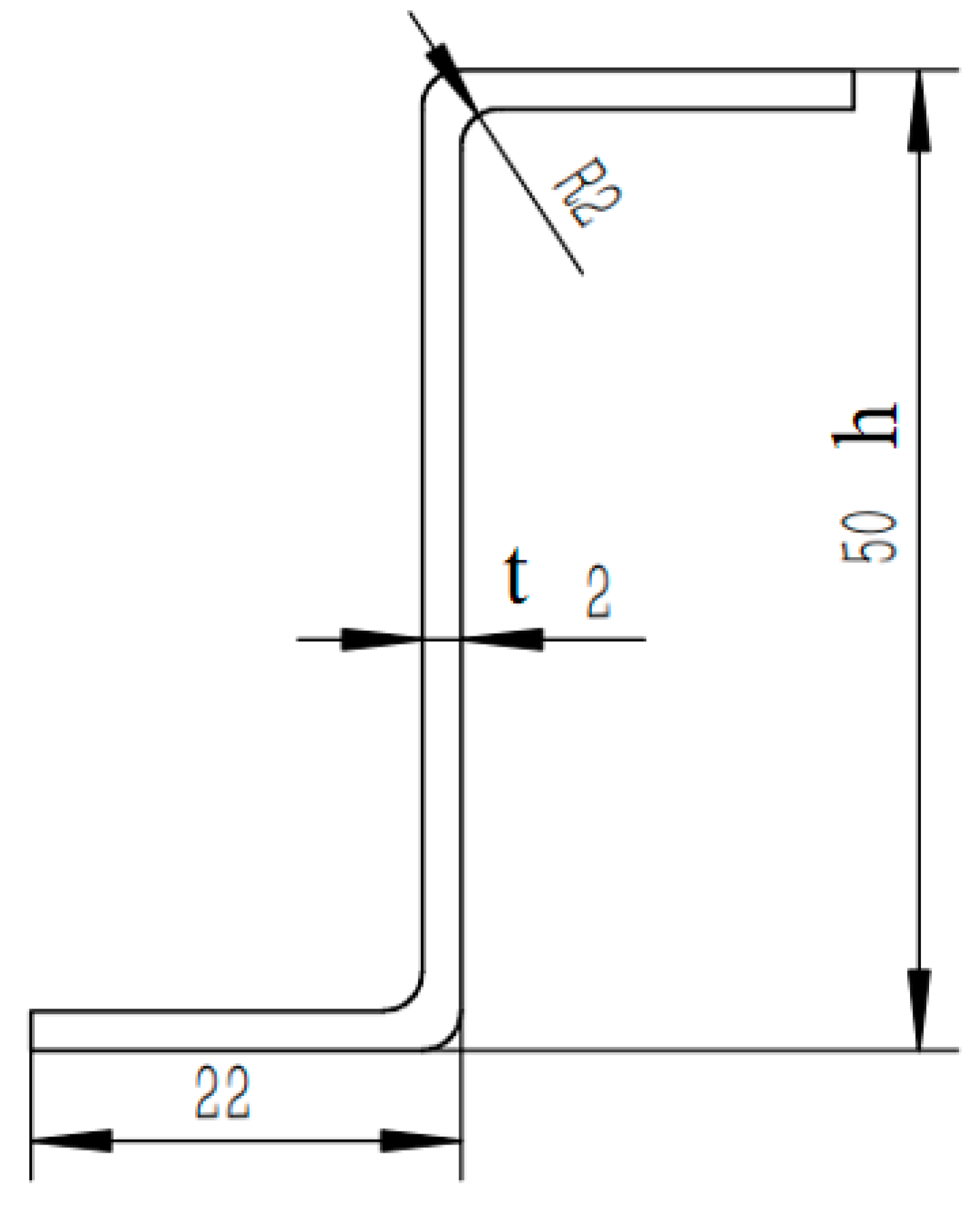
2.2. Tooling Design and Improvement
2.3. Establishment of Finite Element Model for Four-Roll Bending
2.3.1. Mechanical Property Testing of 6063-T5 Aluminum Alloy
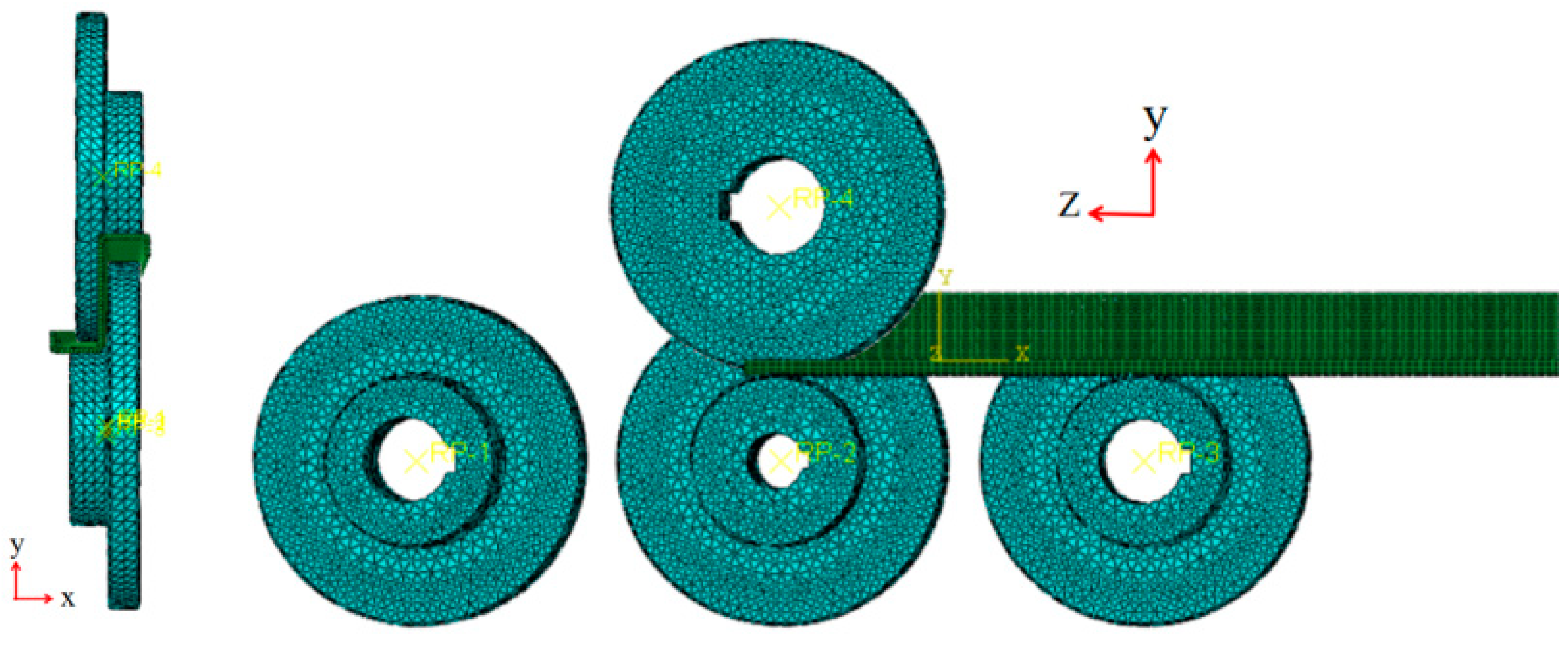
2.3.2. Build Geometric Model
2.3.3. Material Properties
2.3.4. Contact Definition and Constraint
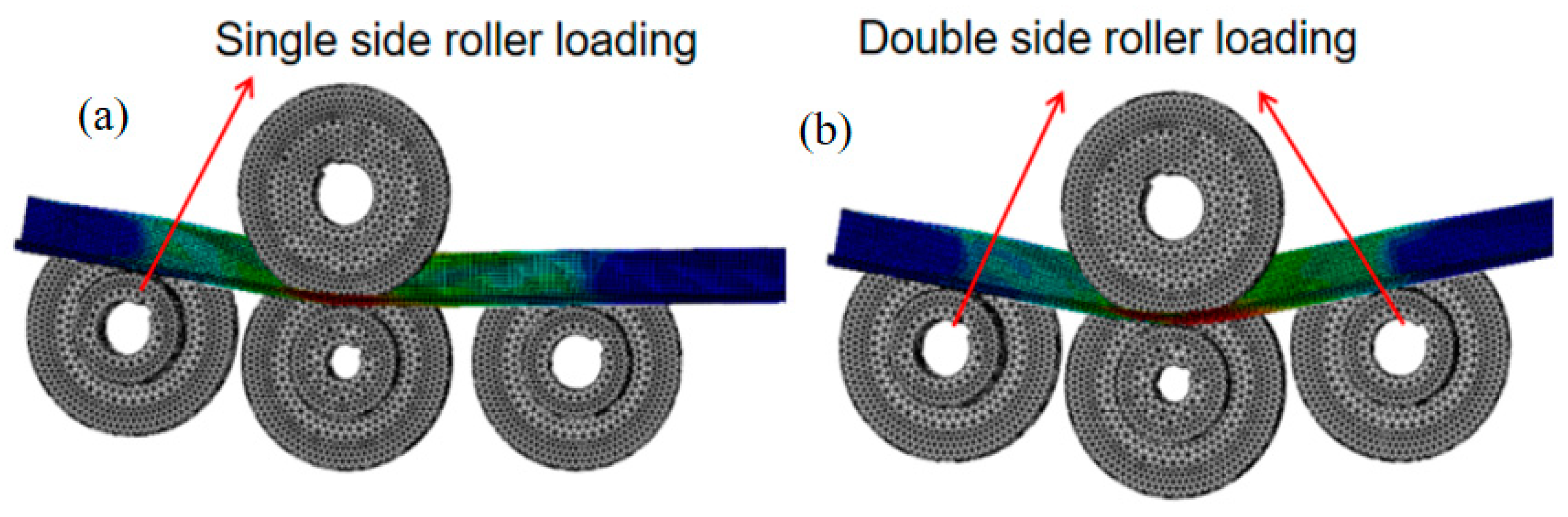
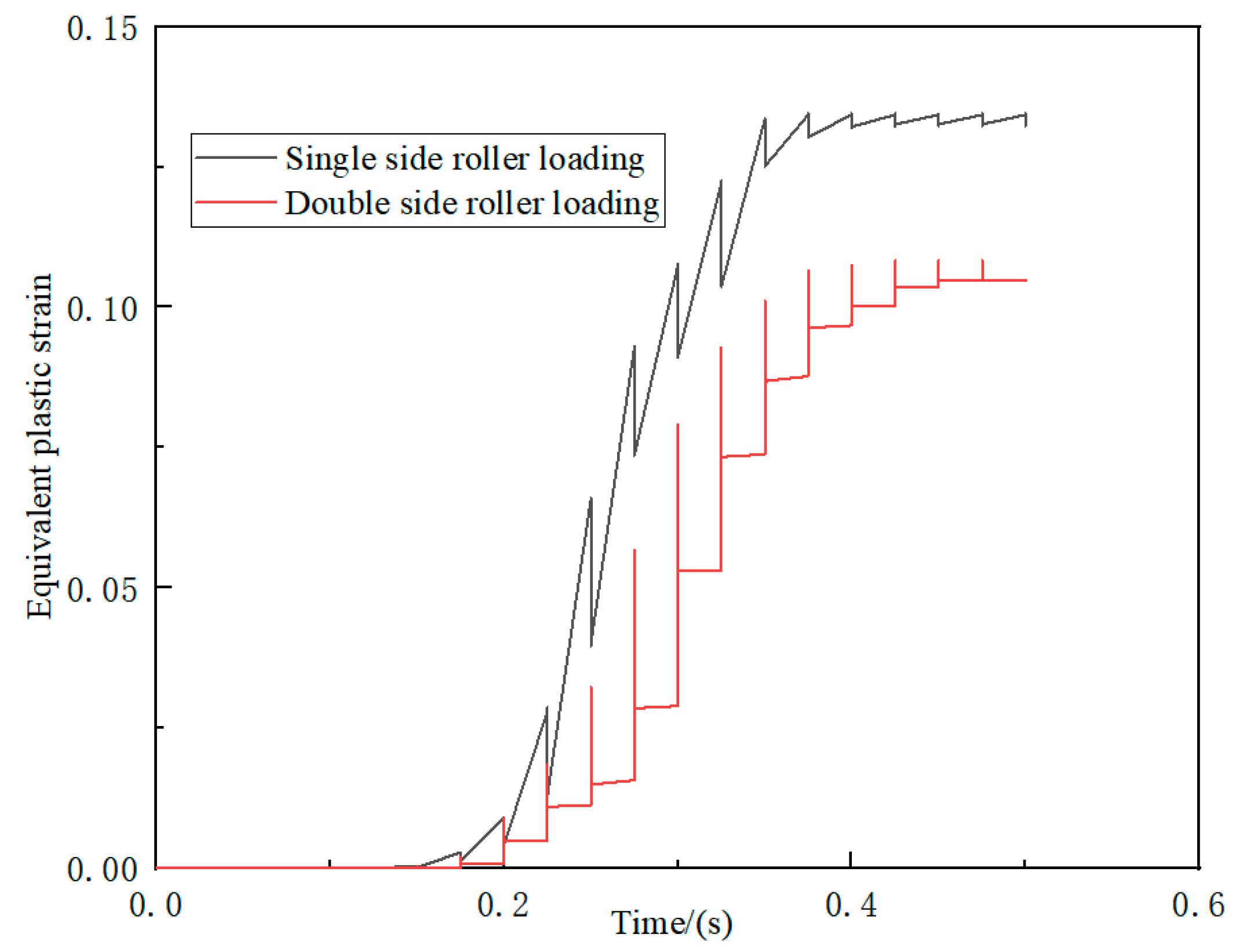
2.3.5. Effects of Different Loading Methods
2.3.6. Model Accuracy Verification
2.4. Orthogonal Experiment
3. Results and Discussion
3.1. Result Analysis
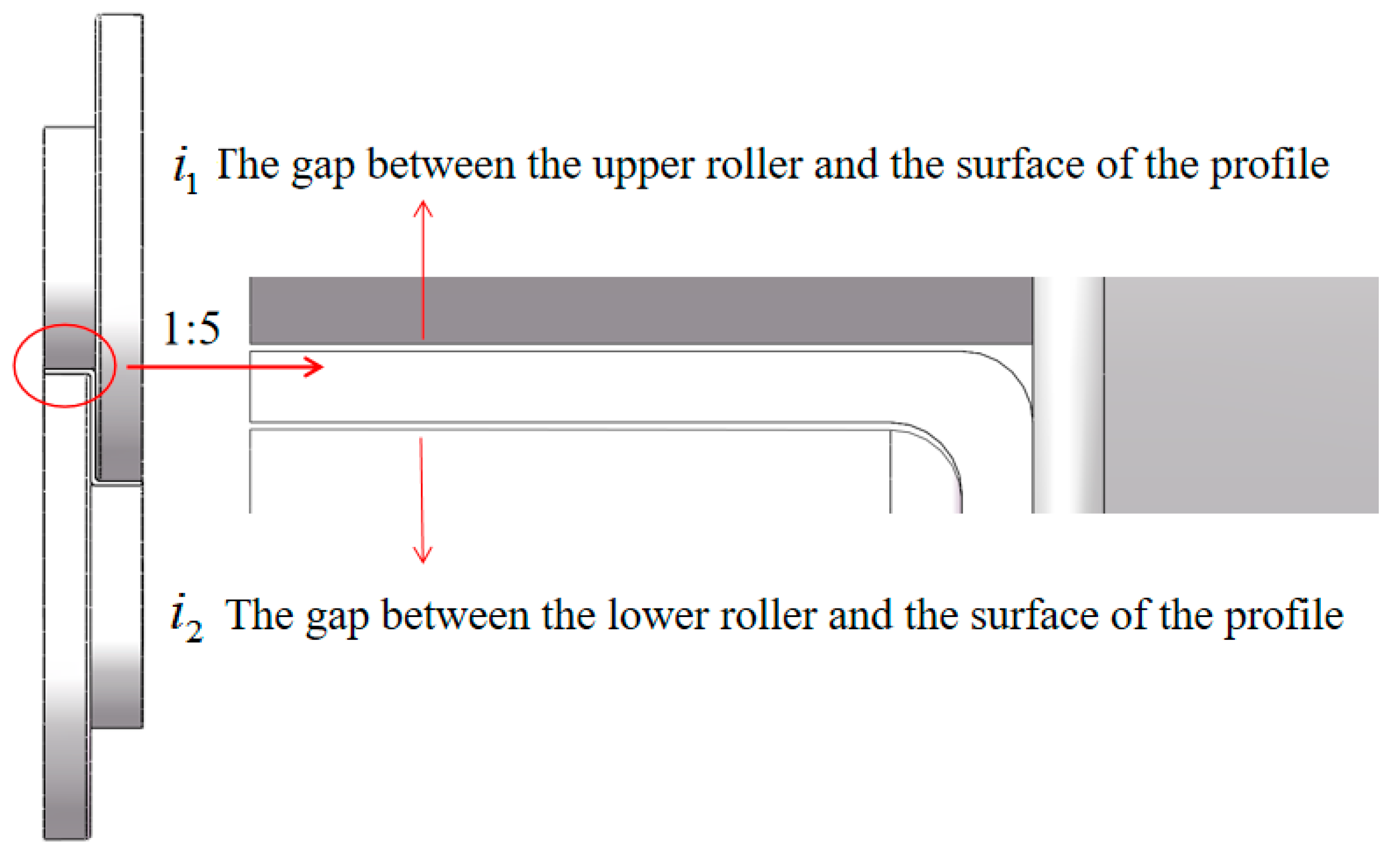
- Influence of Clearance Level on Stresses: The upper roller clearance has a more significant impact on stresses than the lower roller clearance. Stresses demonstrate an increasing and then decreasing pattern with increasing upper roller clearance.
- The Effect of Gap Level on Equivalent Plastic Strain: The effect of the upper roller gap on equivalent plastic strain is greater than that of the lower roller gap. The equivalent plastic strain tends to decrease first and then increase as the gap between the upper roller and the roller increases, and as the gap between the lower rollers increases, it keeps increasing.
3.2. Parameter Optimization
3.3. Discussion
4. Conclusions
- This study analyzed and addressed issues such as increased scratching and poor quality on the surface of the profile during bending using regular rollers. It was determined that the inconsistent linear velocities between the upper and lower surfaces of the profile were the main cause of poor surface quality. The proposed solution involved maintaining clearances at the contact interface between the roller and profile.
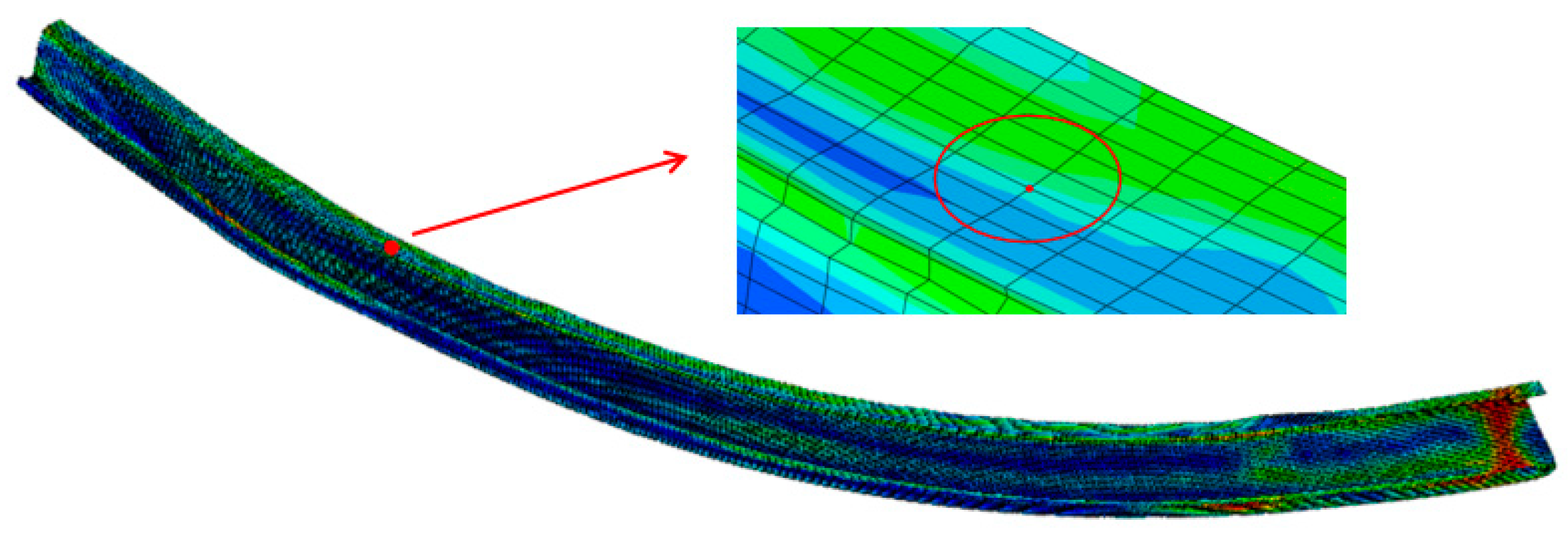
- Finite element simulation was used to simulate the different experiments, and a range analysis was performed on the simulation results. The gap between the upper roller and the surface is 0.4 mm, and the gap between the lower roller and the surface is 0.3 mm. Compared with no gap left, the maximum stress decreases from 225.6 to 204.1 MPa, and the equivalent plastic strain decreases from 0.032 to 0.019. It can be seen that the surface quality of the profile processed with a gap has been improved. It has been proven that leaving gaps between the roller and the upper and lower surfaces can reduce the equivalent plastic strain on the surface of the profile, thereby reducing damage to the surface of the profile.
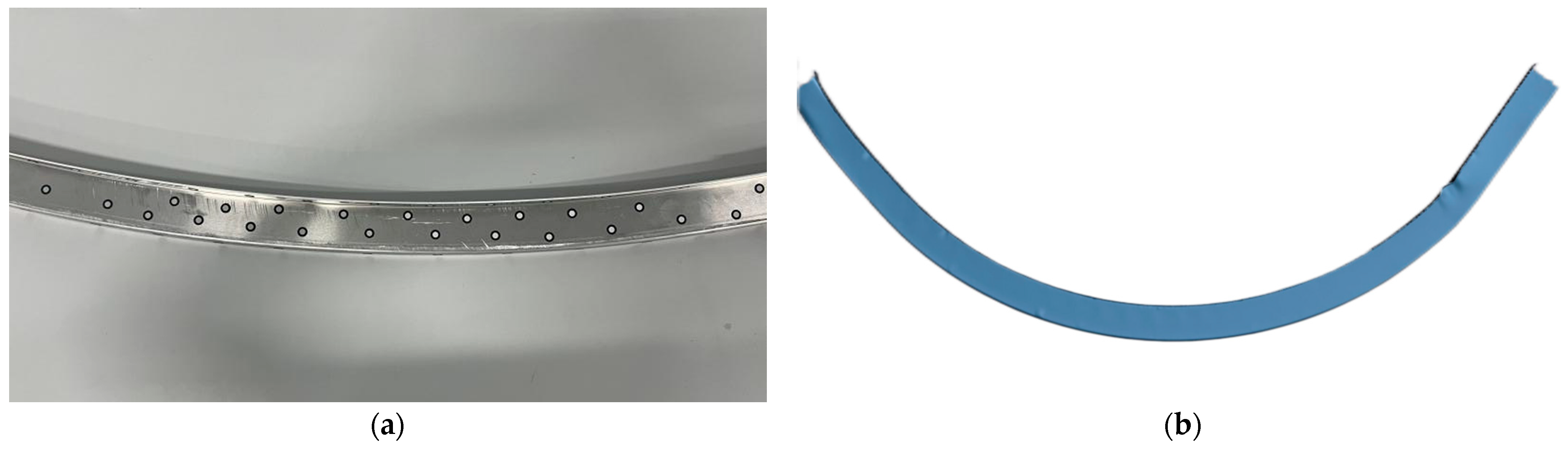
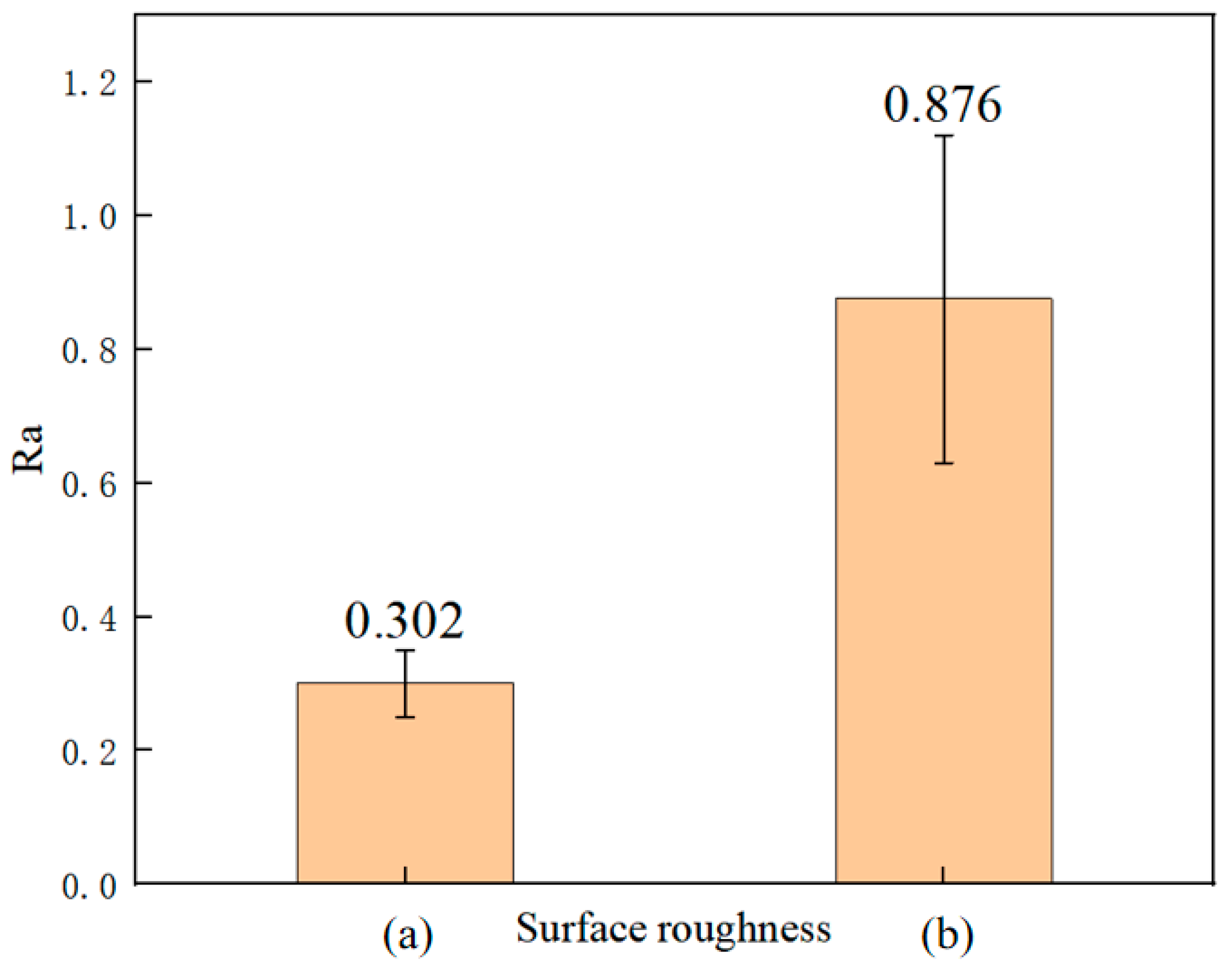
- Based on the optimal clearances, a new fixture design for rollers was implemented. A VMA251 optical measuring instrument was used to observe the surface of the profile after processing with improved rollers. The quality of the profile processed with improved rollers was significantly better than that of the profile processed without improvements. The results indicate that using a finite element simulation to determine roller clearances is a scientifically sound method. Compared with traditional experimental methods, it reduces material waste, improves clearance optimization, and enhances processing efficiency.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Mercuri, A.; Fanelli, P.; Giorgetti, F.; Rubino, G.; Stefanini, C. Experimental and numerical analysis of roll bending process of thick metal sheets. In Proceedings of the IOP Conference Series: Materials Science and Engineering, Sanya, China, 12–14 November 2021; IOP Publishing: Bristol, UK, 2021; Volume 1038, p. 012067. [Google Scholar]
- Fu, Z.; Mo, J.; Zhang, W. Study on multiple-step incremental air-bending forming of sheet metal with springback model and FEM simulation. Int. J. Adv. Manuf. Technol. 2009, 45, 448–458. [Google Scholar] [CrossRef]
- Paralikas, J.; Salonitis, K.; Chryssolouris, G. Investigation of the effect of roll forming pass design on main redundant deformations on profiles from AHSS. Int. J. Adv. Manuf. Technol. 2011, 56, 475–491. [Google Scholar] [CrossRef]
- Feng, Z.; Champliaud, H. Comparison between numerical simulation and experimentation of asymmetrical three-roll bending process. In Proceedings of the ASME International Mechanical Engineering Congress and Exposition, Vancouver, BC, Canada, 12–18 November 2010; Volume 44274, pp. 33–37. [Google Scholar]
- Yu, J.; Zhao, G.; Chen, L. Investigation of interface evolution, microstructure and mechanical properties of solid-state bonding seams in hot extrusion process of aluminum alloy profiles. J. Mater. Process. Technol. 2016, 230, 153–166. [Google Scholar] [CrossRef]
- Lou, S.; Zhao, G.; Wang, R.; Wu, X. Modeling of aluminum alloy profile extrusion process using finite volume method. J. Mater. Process. Technol. 2008, 206, 481–490. [Google Scholar] [CrossRef]
- Hua, M.; Baines, K.; Cole, I.M. Bending mechanisms, experimental techniques and preliminary tests for the continuous four-roll plate bending process. J. Mater. Process. Technol. 1995, 48, 159–172. [Google Scholar] [CrossRef]
- Cai, Z.Y.; Li, M.Z.; Lan, Y.W. Three-dimensional sheet metal continuous forming process based on flexible roll bending: Principle and experiments. J. Mater. Process. Technol. 2012, 212, 120–127. [Google Scholar] [CrossRef]
- Baek, G.Y.; Shim, D.S. FE Simulation-based process design for manufacturing structural members of commercial vehicle using pipes with rectangular Cross-Sections. Int. J. Precis. Eng. Manuf. 2015, 16, 723–733. [Google Scholar] [CrossRef]
- Wan, L.; Liu, R.; Deng, Y.; Tang, J.; Zhang, Y. The effect of aging process on the crushing performance of 6061 aluminum alloy profiles for automobiles. Mater. Introd. 2021, 35, 8099–8102. [Google Scholar]
- Dong, L.; Xie, F.; Chen, L.; Liu, Z.; Meng, X.; Li, Q.; Li, P. The effect of trace elements on the bending performance of aluminum alloy anti-collision beams. Non Ferr. Mater. Eng. 2021, 42, 37–41. [Google Scholar]
- Li, C.; Zhou, W.; Deng, Y.; Li, J. Bending and Forming Manufacturing Technology of Aluminum Alloy Shapes for Rail Transit and Automobiles. In Proceedings of the 2018 China Aluminum Processing Industry Annual Conference, Foshan, China, 26–27 June 2018; China Nonferrous Metal Processing Industry Association: Beijing, China, 2018. [Google Scholar]
- Hua, M.; Baines, K.; Cole, I.M. Continuous four-roll plate bending: A production process for the manufacture of single seamed tubes of large and medium diameters. Int. J. Mach. Tools Manuf. 1999, 39, 905–935. [Google Scholar] [CrossRef]
- Hua, M.; Lin, Y.H. Large deflection analysis of elastoplastic plate in steady continuous four-roll bending process. Int. J. Mech. Sci. 1999, 41, 1461–1483. [Google Scholar] [CrossRef]
- Zhou, Y. Improvement of the roller structure for profile rolling and bending processing. Technol. Inf. Acad. Res. 2007, 36, 424–426. [Google Scholar]
- Luo, Z.; Wen, S.; Hu, Y.; Chen, Z.; Wu, J. Roll bending forming and roller design of aluminum lithium alloy I-shaped profiles. Mold Manuf. 2015, 15, 77–80. [Google Scholar] [CrossRef]
- Hu, Z.; Chen, M.; Xie, L. Skew bending control of 4-axis rolling bending of aluminum lithium alloy profiles in 1999-T83. Rare Met. Mater. Eng. 2017, 46, 2957–2962. [Google Scholar]
- Chen, P.; Xue, H.; Wang, J.; Zhang, X.; Liu, P. Uniform-curvature arc approximation algorithm and its implementation of CNC rollbending for Z-shaped variable curvature profiles. Mater. Sci. Technol. 2014, 22, 68–73. [Google Scholar]
- Li, F. Study on Mathematical Model for Roll-Bending Plastic Forming of Sheet Metal; Fudan University: Shanghai, China, 2002. [Google Scholar]
- Zeng, G.; Lai, X.M.; Yu, Z.Q.; Lin, Z.Q. Numerical simulation and sensitivity analysis of parameters for multi-stand roll forming of channel section with outer edge. J. Iron Steel Res. Int. 2009, 16, 32–37. [Google Scholar] [CrossRef]
- Bettaieb, M.B.; Lemoine, X.; Duchêne, L.; Habraken, A.M. Simulation of the bending process of hardening metallic sheets using damage model. Part I: Theoretical development and numerical implementation. Mater. Sci. Eng. A 2010, 528, 434–441. [Google Scholar] [CrossRef]
- Chen, G.; Wang, Y. Simulation of profile roll-bending and analysis of stress strain. J. Shaanxi Univ. Sci. Technol. Nat. Sci. Ed. 2012, 30, 94–96. [Google Scholar]
- Wang, A.; Xue, H.; Bayraktar, E.; Yang, Y.; Saud, S.; Chen, P. Analysis and control of twist defects of aluminum profiles with large z-section in roll bending process. Metals 2019, 10, 31. [Google Scholar] [CrossRef]
- Zhan, M.; Yang, H.; Huang, L.; Gu, R. Springback analysis of numerical control bending of thin-walled tube using numerical-analytic method. J. Mater. Process. Technol. 2006, 177, 197–201. [Google Scholar] [CrossRef]
- Panthi, S.K.; Ramakrishnan, N.; Pathak, K.K.; Chouhan, J.S. An analysis of springback in sheet metal bending using finite element method (FEM). J. Mater. Process. Technol. 2007, 186, 120–124. [Google Scholar] [CrossRef]
- Yu, H.Q.; Chen, J.D. Principles of Metal Plastic Forming; China Machine Press: Beijing, China, 1999. [Google Scholar]
- Wang, Y.; Hu, J.; Xu, G.; Li, S.; Xiong, W. Numerical simulation and experimental verification of three roll asymmetric rolling forming. China Mech. Eng. 2016, 27, 2085–2090. [Google Scholar]
- Wang, Y.; Hu, J.; Xu, G.; Wang, J.; Li, S. Mechanism and experimental analysis of four roll pre bending and continuous roll bending forming. J. Plast. Eng. 2016, 23, 69–75. [Google Scholar]
- Zhou, C.; Sun, Y.; Liu, Q.; Chang, X.; Wu, K.; Sheng, Y. Calculation and Numerical Simulation of Side Roll Displacement in Four Roll Continuous Rolling Bending. Forg. Technol. 2017, 42, 36–42. [Google Scholar] [CrossRef]
- Tian, Y.; Zhu, L.; Luo, J.; Hui, W.; Que, Y.; Wu, G.; Zhou, H. Study on stress and strain characteristics of composite scratch dent zone of X80 pipeline. J. Plast. Eng. 2021, 28, 177–182. [Google Scholar]
- Zhang, C. Experimental Design and Data Processing; Chemical Industry Press: Beijing, China, 2009. [Google Scholar]

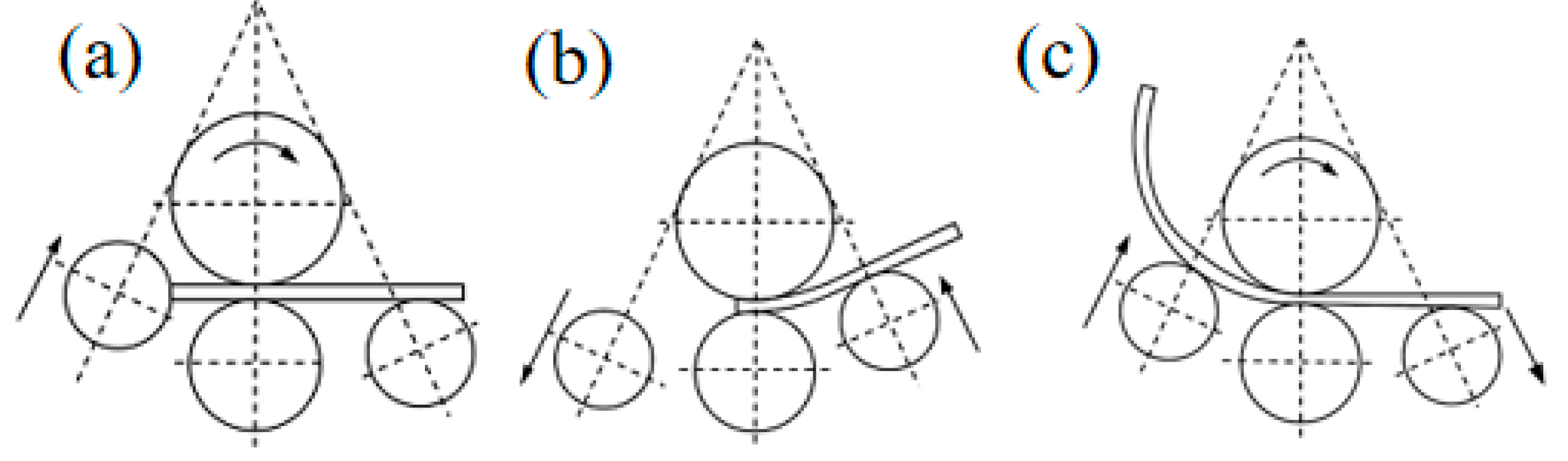




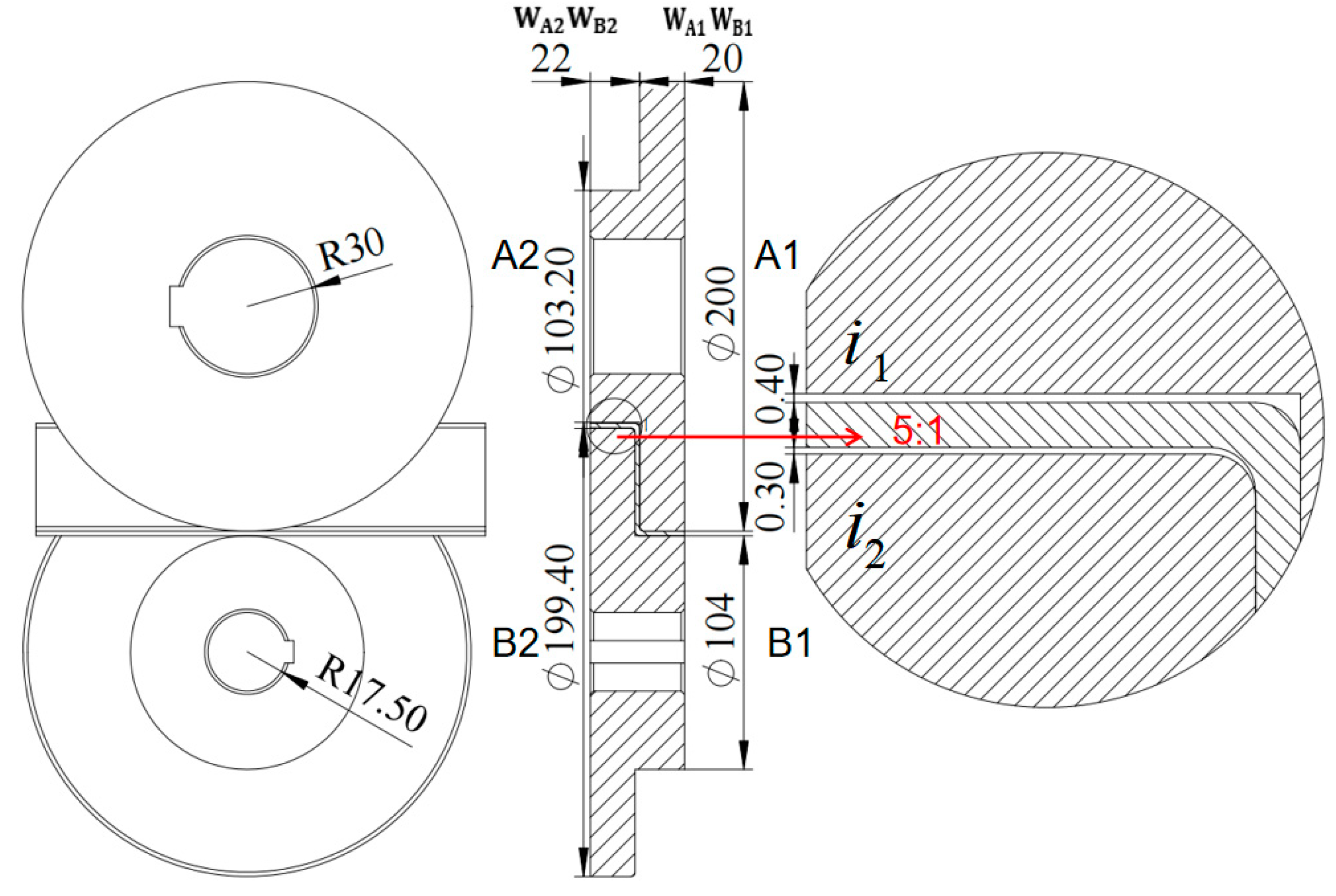


| Code Name | Roller Radius (mm) | Roller Width (mm) | ||||
|---|---|---|---|---|---|---|
| Numeric value | 100 | 100 | 52 | 52 | 20 | 22 |
| 156 | 70,000 | 0.33 | 2700 | 9 |
| Constraint Type | Upper Roller | Lower Roller | Left Roller | Right Roller |
|---|---|---|---|---|
| Move constraint | X, Y, Z | X, Y, Z | X | X |
| Rotational constraint | Y, Z | Y, Z | Y, Z | Y, Z |
| Side Roll Displacement (mm) | Experimental Result Curvature of Profile Forming (mm) | Simulation Result Curvature of Profile Forming (mm) | Error |
|---|---|---|---|
| 20 | 17,873 | 17,464 | 2.2% |
| 30 | 10,021 | 9776 | 2.4% |
| 40 | 7341 | 6982 | 4.8% |
| 50 | 5105 | 4888 | 4.2% |
| Factor | 1 Level (mm) | 2 Level (mm) | 3 Level (mm) | 4 Level (mm) |
|---|---|---|---|---|
| (A) Upper roller clearance | 0.1 | 0.2 | 0.3 | 0.4 |
| (B) Lower roller clearance | 0.1 | 0.2 | 0.3 | 0.4 |
| Experiment No | Factors and Levels | Maximum Stress (MPa) | Equivalent Plastic Strain | |
|---|---|---|---|---|
| 1 | 1 | 1 | 223.27 | 0.0168 |
| 2 | 1 | 2 | 222.68 | 0.0174 |
| 3 | 1 | 3 | 221.44 | 0.0177 |
| 4 | 1 | 4 | 222.71 | 0.0183 |
| 5 | 2 | 1 | 221.75 | 0.0152 |
| 6 | 2 | 2 | 223.50 | 0.0180 |
| 7 | 2 | 3 | 223.20 | 0.0182 |
| 8 | 2 | 4 | 223.02 | 0.0180 |
| 9 | 3 | 1 | 222.75 | 0.0171 |
| 10 | 3 | 2 | 224.02 | 0.0172 |
| 11 | 3 | 3 | 224.03 | 0.0202 |
| 12 | 3 | 4 | 223.16 | 0.0185 |
| 13 | 4 | 1 | 222.57 | 0.0190 |
| 14 | 4 | 2 | 223.85 | 0.0192 |
| 15 | 4 | 3 | 206.11 | 0.0189 |
| 16 | 4 | 4 | 217.07 | 0.0240 |
| Order Number | A | B | |
|---|---|---|---|
| Stress | K11 | 222.52 | 222.58 |
| K12 | 222.87 | 223.51 | |
| K13 | 223.49 | 218.69 | |
| K14 | 217.4 | 221.49 | |
| R1 | 6.09 | 4.82 | |
| Equivalent plastic strain | K21 | 0.0176 | 0.0170 |
| K22 | 0.0174 | 0.0180 | |
| K23 | 0.0183 | 0.0188 | |
| K24 | 0.0203 | 0.0197 | |
| R2 | 0.0029 | 0.0027 | |
| Code Name | Roller Radius (mm) | Roller Width (mm) | ||||
|---|---|---|---|---|---|---|
| Numeric value | 100 | 99.6 | 52 | 51.7 | 20 | 22 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jing, Y.; Sun, Q.; Zhao, Y. Design of Z Profile Roller Tooling and Research on Roller Clearance. Machines 2023, 11, 767. https://doi.org/10.3390/machines11070767
Jing Y, Sun Q, Zhao Y. Design of Z Profile Roller Tooling and Research on Roller Clearance. Machines. 2023; 11(7):767. https://doi.org/10.3390/machines11070767
Chicago/Turabian StyleJing, Yigang, Qun Sun, and Ying Zhao. 2023. "Design of Z Profile Roller Tooling and Research on Roller Clearance" Machines 11, no. 7: 767. https://doi.org/10.3390/machines11070767
APA StyleJing, Y., Sun, Q., & Zhao, Y. (2023). Design of Z Profile Roller Tooling and Research on Roller Clearance. Machines, 11(7), 767. https://doi.org/10.3390/machines11070767






