Tendon-Driven Crawling Robot with Programmable Anisotropic Friction by Adjusting Out-of-Plane Curvature
Abstract
1. Introduction
2. Design of the Origami Crawling Robot
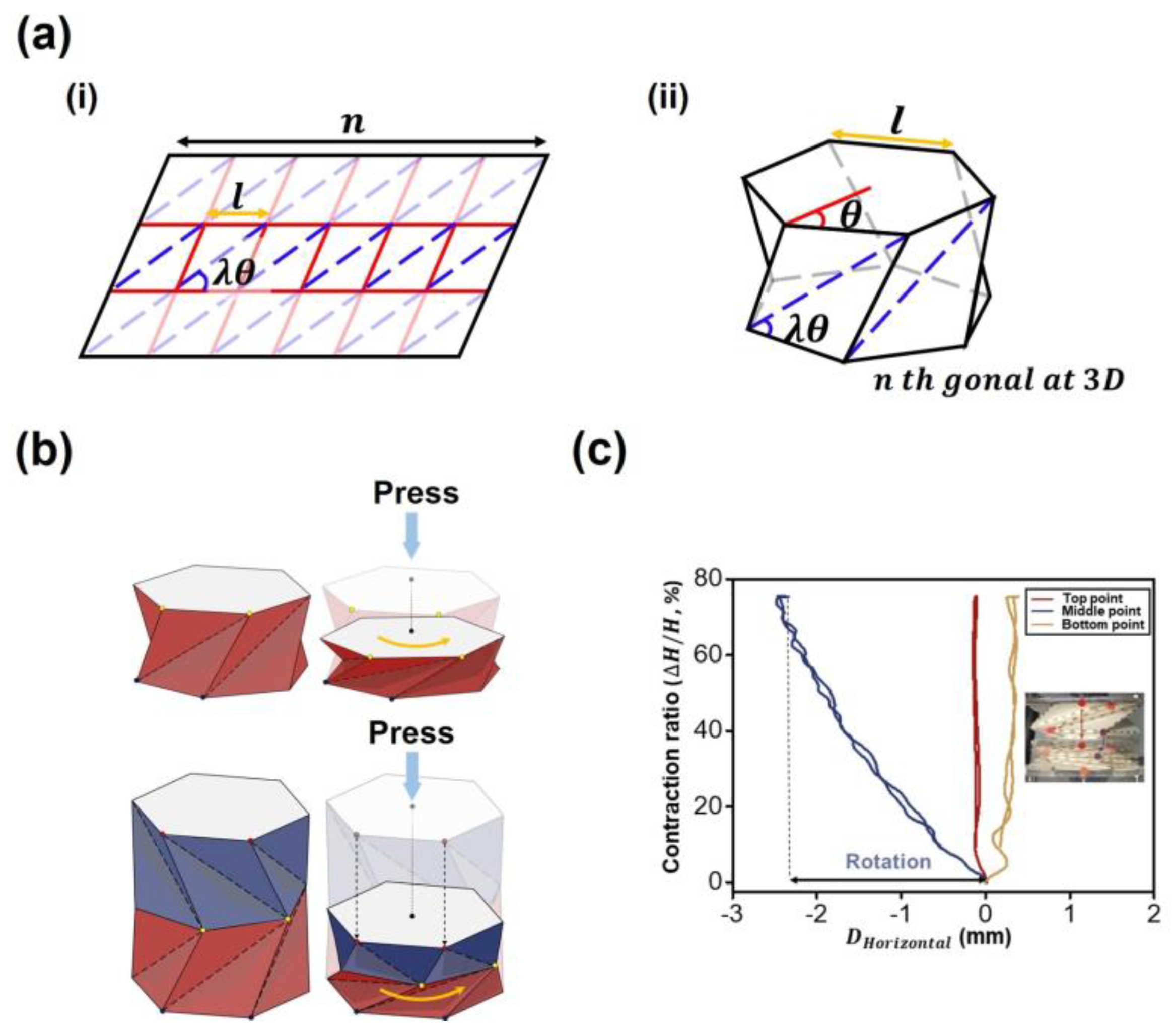
2.1. Characteristics of the Origami Structure
2.2. Origami Crawler Mechanism
2.2.1. The Relationship between the Anisotropic Friction and Out-of-Plane Curvature
2.2.2. The Out-of-Plane Curvature for Stride-Efficient Crawler
2.3. The Crawling Mechanism of the Robot
2.4. Fabrication of the Origami Crawler
3. Result and Discussion
3.1. Experiment Setup
3.2. Experiment Results
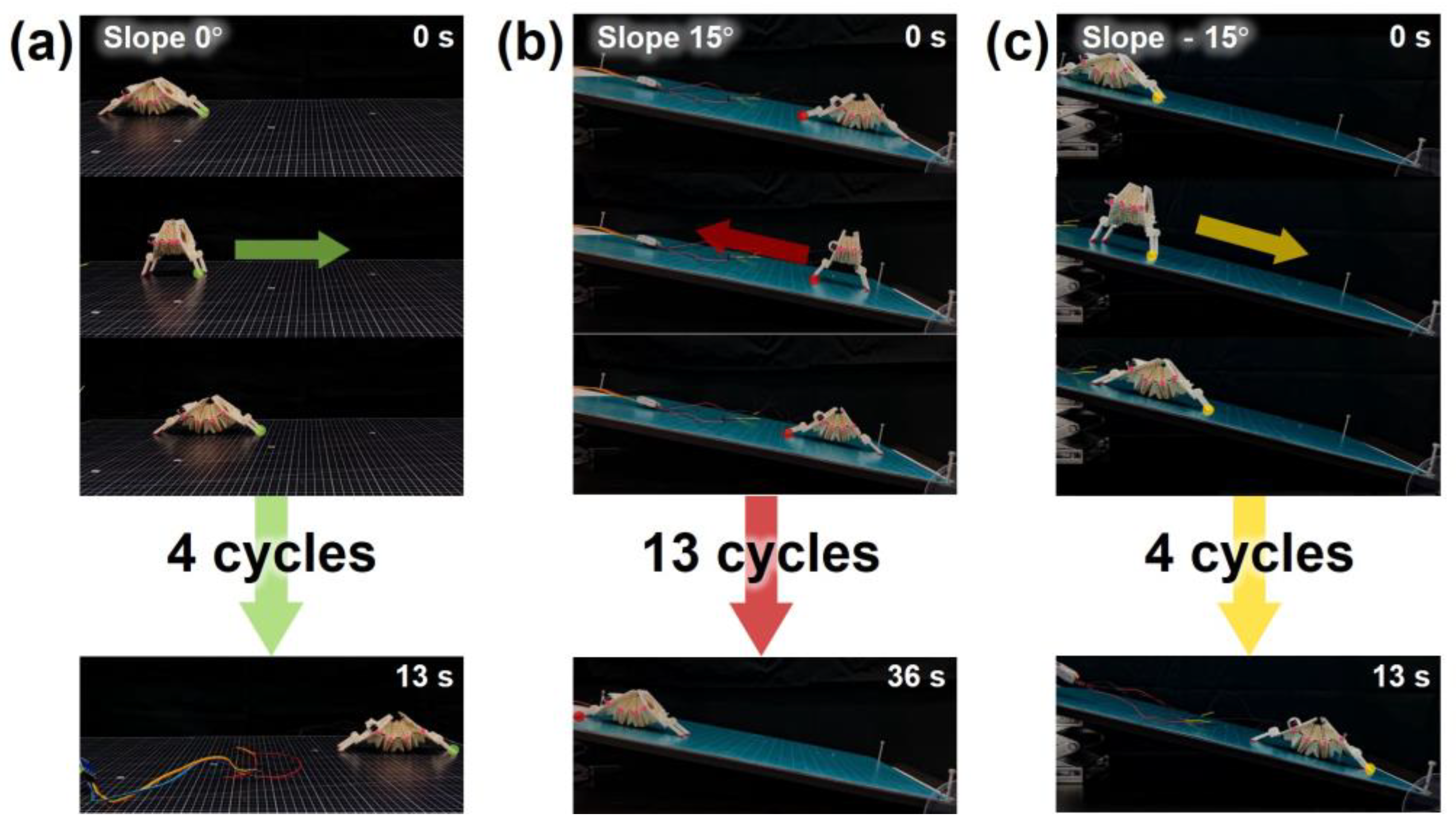
3.3. Crawling on the Sloped Surface
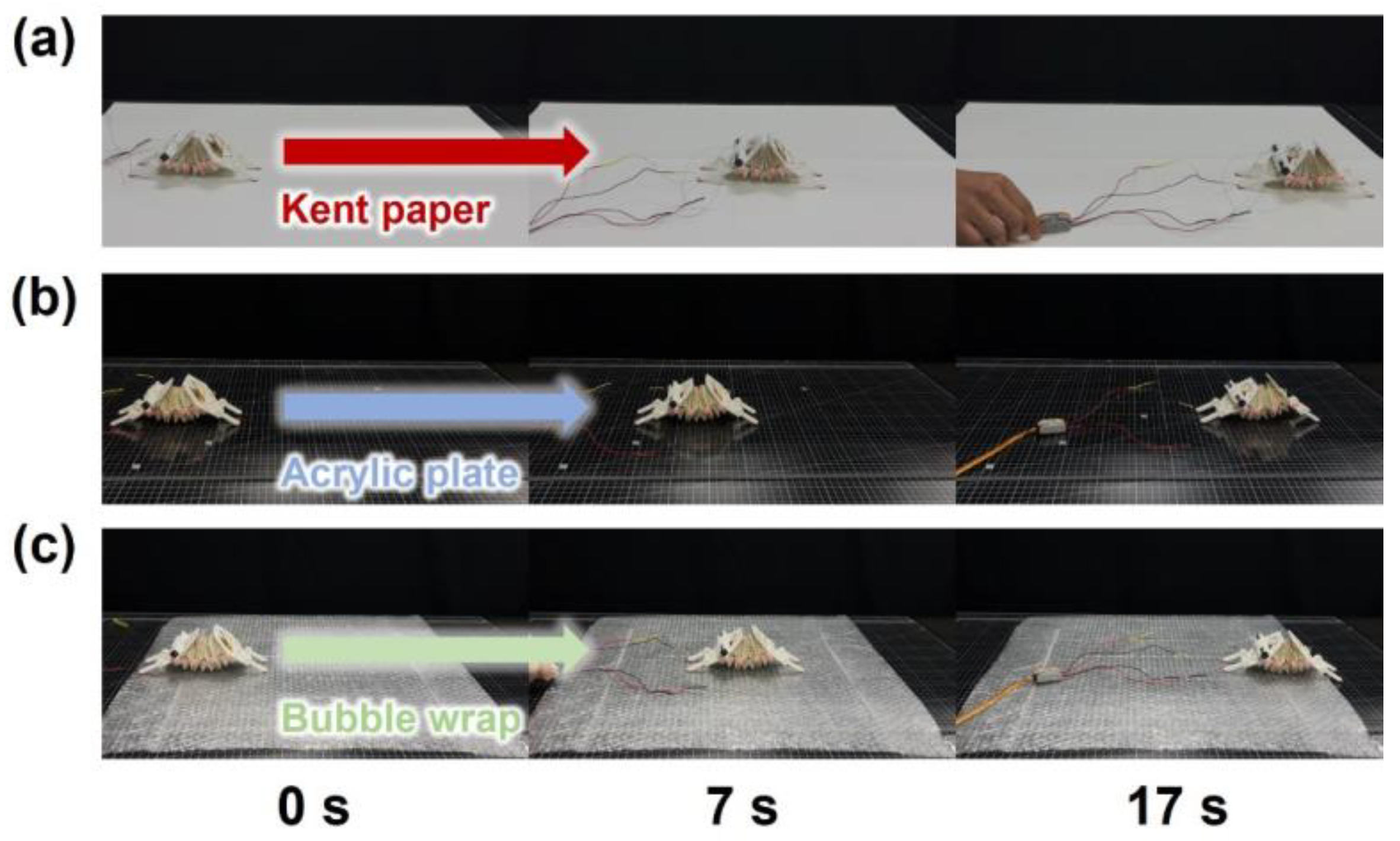
3.4. Motion Implementation on Various Surfaces
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Rus, D.; Sung, C. Spotlight on Origami Robots. Sci. Robot. 2018, 3, eaat0938. [Google Scholar] [CrossRef]
- Zhao, Z.; Kuang, X.; Wu, J.; Zhang, Q.; Paulino, G.H.; Qi, H.J.; Fang, D. 3D Printing of Complex Origami Assemblages for Reconfigurable Structures. Soft Matter 2018, 14, 8051–8059. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Z.; Fan, W.; Chen, G.; Luo, J.; Lu, Q.; Wang, H. A 3D Printable Origami Vacuum Pneumatic Artificial Muscle with Fast and Powerful Motion. In Proceedings of the 2021 IEEE 4th International Conference on Soft Robotics (RoboSoft), New Haven, CT, USA, 12–16 April 2021; IEEE: Piscataway, NJ, USA, 2021; pp. 551–554. [Google Scholar]
- Melancon, D.; Forte, A.E.; Kamp, L.M.; Gorissen, B.; Bertoldi, K. Inflatable Origami: Multimodal Deformation via Multistability. Adv. Funct. Mater. 2022, 32, 2201891. [Google Scholar] [CrossRef]
- Wu, S.; Ze, Q.; Dai, J.; Udipi, N.; Paulino, G.H.; Zhao, R. Stretchable Origami Robotic Arm with Omnidirectional Bending and Twisting. Proc. Natl. Acad. Sci. USA 2021, 118, e2110023118. [Google Scholar] [CrossRef]
- Namiki, A.; Yokosawa, S. Origami Folding by Multifingered Hands with Motion Primitives. Cyborg Bionic Syst. 2021, 2021, 9851834. [Google Scholar] [CrossRef] [PubMed]
- Rothemund, P.W.K. Folding DNA to Create Nanoscale Shapes and Patterns. Nature 2006, 440, 297–302. [Google Scholar] [CrossRef] [PubMed]
- Liu, F.; Liu, X.; Huang, Q.; Arai, T. Recent Progress of Magnetically Actuated DNA Micro/Nanorobots. Cyborg Bionic Syst. 2022, 2022, 9758460. [Google Scholar] [CrossRef]
- Saccà, B.; Niemeyer, C.M. DNA Origami: The Art of Folding DNA. Angew. Chem. Int. Ed. 2012, 51, 58–66. [Google Scholar] [CrossRef] [PubMed]
- Kim, M.; Lee, C.; Jeon, K.; Lee, J.Y.; Kim, Y.-J.; Lee, J.G.; Kim, H.; Cho, M.; Kim, D.-N. Harnessing a Paper-Folding Mechanism for Reconfigurable DNA Origami. Nature 2023, 619, 78–86. [Google Scholar] [CrossRef]
- Cho, H.; Kim, D.-N. Controlling the Stiffness of Bistable Kirigami Surfaces via Spatially Varying Hinges. Mater. Des. 2023, 231, 112053. [Google Scholar] [CrossRef]
- Zhang, J.; Fang, Q.; Xiang, P.; Sun, D.; Xue, Y.; Jin, R.; Qiu, K.; Xiong, R.; Wang, Y.; Lu, H. A Survey on Design, Actuation, Modeling, and Control of Continuum Robot. Cyborg Bionic Syst. 2022, 2022, 9754697. [Google Scholar] [CrossRef]
- Xu, Y.; Peyron, Q.; Kim, J.; Burgner-Kahrs, J. Design of Lightweight and Extensible Tendon-Driven Continuum Robots Using Origami Patterns. In Proceedings of the 2021 IEEE 4th International Conference on Soft Robotics (RoboSoft), New Haven, CT, USA, 12–14 April 2021; IEEE: Piscataway, NJ, USA, 2021; pp. 308–314. [Google Scholar]
- Kim, J.; Lee, D.-Y.; Kim, S.-R.; Cho, K.-J. A Self-Deployable Origami Structure with Locking Mechanism Induced by Buckling Effect. In Proceedings of the 2015 IEEE International Conference on Robotics and Automation (ICRA), Seattle, WA, USA, 26–30 May 2015; IEEE: Piscataway, NJ, USA, 2015; Volume 2015, pp. 3166–3171. [Google Scholar]
- Kim, S.-J.; Lee, D.-Y.; Jung, G.-P.; Cho, K.-J. An Origami-Inspired, Self-Locking Robotic Arm That Can Be Folded Flat. Sci. Robot. 2018, 3, eaar2915. [Google Scholar] [CrossRef] [PubMed]
- Bhovad, P.; Kaufmann, J.; Li, S. Peristaltic Locomotion without Digital Controllers: Exploiting Multi-Stability in Origami to Coordinate Robotic Motion. Extreme Mech. Lett. 2019, 32, 100552. [Google Scholar] [CrossRef]
- Hu, Q.; Li, J.; Dong, E.; Sun, D. Soft Scalable Crawling Robots Enabled by Programmable Origami and Electrostatic Adhesion. IEEE Robot. Autom. Lett. 2023, 8, 2365–2372. [Google Scholar] [CrossRef]
- Tramsen, H.T.; Gorb, S.N.; Zhang, H.; Manoonpong, P.; Dai, Z.; Heepe, L. Inversion of Friction Anisotropy in a Bio-Inspired Asymmetrically Structured Surface. J. R. Soc. Interface 2018, 15, 20170629. [Google Scholar] [CrossRef]
- Ta, T.D.; Umedachi, T.; Suzuki, M.; Kawahara, Y. A Printable Soft-Bodied Wriggle Robot with Frictional 2D-Anisotropy Surface. J. Inf. Process. 2022, 30, 201–208. [Google Scholar] [CrossRef]
- Sheng, X.; Xu, H.; Zhang, N.; Ding, N.; Zhu, X.; Gu, G. Multi-Material 3D Printing of Caterpillar-Inspired Soft Crawling Robots with the Pneumatically Bellow-Type Body and Anisotropic Friction Feet. Sens. Actuators A Phys. 2020, 316, 112398. [Google Scholar] [CrossRef]
- Ta, T.D.; Umedachi, T.; Kawahara, Y. Design of Frictional 2D-Anisotropy Surface for Wriggle Locomotion of Printable Soft-Bodied Robots. In Proceedings of the 2018 IEEE International Conference on Robotics and Automation (ICRA), Brisbane, Australia, 21–25 May 2018; IEEE: Piscataway, NJ, USA, 2018; pp. 6779–6785. [Google Scholar]
- Shepherd, R.F.; Ilievski, F.; Choi, W.; Morin, S.A.; Stokes, A.A.; Mazzeo, A.D.; Chen, X.; Wang, M.; Whitesides, G.M. Multigait Soft Robot. Proc. Natl. Acad. Sci. USA 2011, 108, 20400–20403. [Google Scholar] [CrossRef]
- Yu, M.; Yang, W.; Yu, Y.; Cheng, X.; Jiao, Z. A Crawling Soft Robot Driven by Pneumatic Foldable Actuators Based on Miura-Ori. Actuators 2020, 9, 26. [Google Scholar] [CrossRef]
- Ze, Q.; Wu, S.; Nishikawa, J.; Dai, J.; Sun, Y.; Leanza, S.; Zemelka, C.; Novelino, L.S.; Paulino, G.H.; Zhao, R.R. Soft Robotic Origami Crawler. Sci. Adv. 2022, 8, 7834. [Google Scholar] [CrossRef]
- Pagano, A.; Yan, T.; Chien, B.; Wissa, A.; Tawfick, S. A Crawling Robot Driven by Multi-Stable Origami. Smart Mater. Struct. 2017, 26, 94007. [Google Scholar] [CrossRef]
- Yeow, B.S.; Yang, H.; Sivaperuman Kalairaj, M.; Gao, H.; Cai, C.J.; Xu, S.; Chen, P.; Ren, H. Magnetically Steerable Serial and Parallel Structures by Mold-Free Origami Templating and Domain Setting. Adv. Mater. Technol. 2022, 7, 2101140. [Google Scholar] [CrossRef]
- Jin, T.; Li, L.; Wang, T.; Wang, G.; Cai, J.; Tian, Y.; Zhang, Q. Origami-Inspired Soft Actuators for Stimulus Perception and Crawling Robot Applications. IEEE Trans. Robot. 2022, 38, 748–764. [Google Scholar] [CrossRef]
- Joyee, E.B.; Pan, Y. A Fully Three-Dimensional Printed Inchworm-Inspired Soft Robot with Magnetic Actuation. Soft Robot. 2019, 6, 333–345. [Google Scholar] [CrossRef]
- Liu, M.; Xu, Z.; Ong, J.J.; Zhu, J.; Lu, W.F. An Earthworm-like Soft Robot with Integration of Single Pneumatic Actuator and Cellular Structures for Peristaltic Motion. In Proceedings of the 2020 IEEE/RSJ International Conference on Intelligent Robots and Systems (IROS), Las Vegas, NV, USA, 25–29 October 2020; IEEE: Piscataway, NJ, USA, 2020; pp. 7840–7845. [Google Scholar]
- Wang, W.; Lee, J.-Y.; Rodrigue, H.; Song, S.-H.; Chu, W.-S.; Ahn, S.-H. Locomotion of Inchworm-Inspired Robot Made of Smart Soft Composite (SSC). Bioinspir Biomim. 2014, 9, 46006. [Google Scholar] [CrossRef]
- Onal, C.D.; Wood, R.J.; Rus, D. An Origami-Inspired Approach to Worm Robots. IEEE/ASME Trans. Mechatron. 2013, 18, 430–438. [Google Scholar] [CrossRef]
- Li, J.; Godaba, H.; Zhang, Z.Q.; Foo, C.C.; Zhu, J. A Soft Active Origami Robot. Extreme Mech. Lett. 2018, 24, 30–37. [Google Scholar] [CrossRef]
- Wang, H.; Yamamoto, A.; Higuchi, T. A Crawler Climbing Robot Integrating Electroadhesion and Electrostatic Actuation. Int. J. Adv. Robot. Syst. 2014, 11, 191. [Google Scholar] [CrossRef]
- Li, Q.; Zhang, F.; Jing, Z.; Yu, F.; Chen, Y. A Hybrid Territorial Aquatic Bionic Soft Robot with Controllable Transition Capability. J. Bionic Eng. 2023, 20, 568–583. [Google Scholar] [CrossRef]
- Sriratanasak, N.; Axinte, D.; Dong, X.; Mohammad, A.; Russo, M.; Raimondi, L. Tasering Twin Soft Robot: A Multimodal Soft Robot Capable of Passive Flight and Wall Climbing. Adv. Intell. Syst. 2022, 4, 2200223. [Google Scholar] [CrossRef]
- Kim, Y.; Cha, Y. Soft Pneumatic Gripper With a Tendon-Driven Soft Origami Pump. Front. Bioeng. Biotechnol. 2020, 8, 461. [Google Scholar] [CrossRef] [PubMed]
- Banerjee, H.; Pusalkar, N.; Ren, H. Single-Motor Controlled Tendon-Driven Peristaltic Soft Origami Robot. J. Mech. Robot. 2018, 10, 64501. [Google Scholar] [CrossRef]
- Luo, M.; Yan, R.; Wan, Z.; Qin, Y.; Santoso, J.; Skorina, E.H.; Onal, C.D. OriSnake: Design, Fabrication, and Experimental Analysis of a 3-D Origami Snake Robot. IEEE Robot. Autom. Lett. 2018, 3, 1993–1999. [Google Scholar] [CrossRef]
- Pagano, A.; Leung, B.; Chien, B.; Yan, T.; Wissa, A.; Tawfick, S. Multi-Stable Origami Structure for Crawling Locomotion. In Proceedings of the Volume 2: Modeling, Simulation and Control, Bio-Inspired Smart Materials and Systems; Energy Harvesting, Stowe, VT, USA, 28–30 September 2016; American Society of Mechanical Engineers: New York, NY, USA, 2016. [Google Scholar]
- Hawkes, E.W.; Jiang, H.; Christensen, D.L.; Han, A.K.; Cutkosky, M.R. Grasping Without Squeezing: Design and Modeling of Shear-Activated Grippers. IEEE Trans. Robot. 2018, 34, 303–316. [Google Scholar] [CrossRef]
- Han, A.K.; Hajj-Ahmad, A.; Cutkosky, M.R. Hybrid Electrostatic and Gecko-Inspired Gripping Pads for Manipulating Bulky, Non-Smooth Items. Smart Mater. Struct. 2021, 30, 25010. [Google Scholar] [CrossRef]


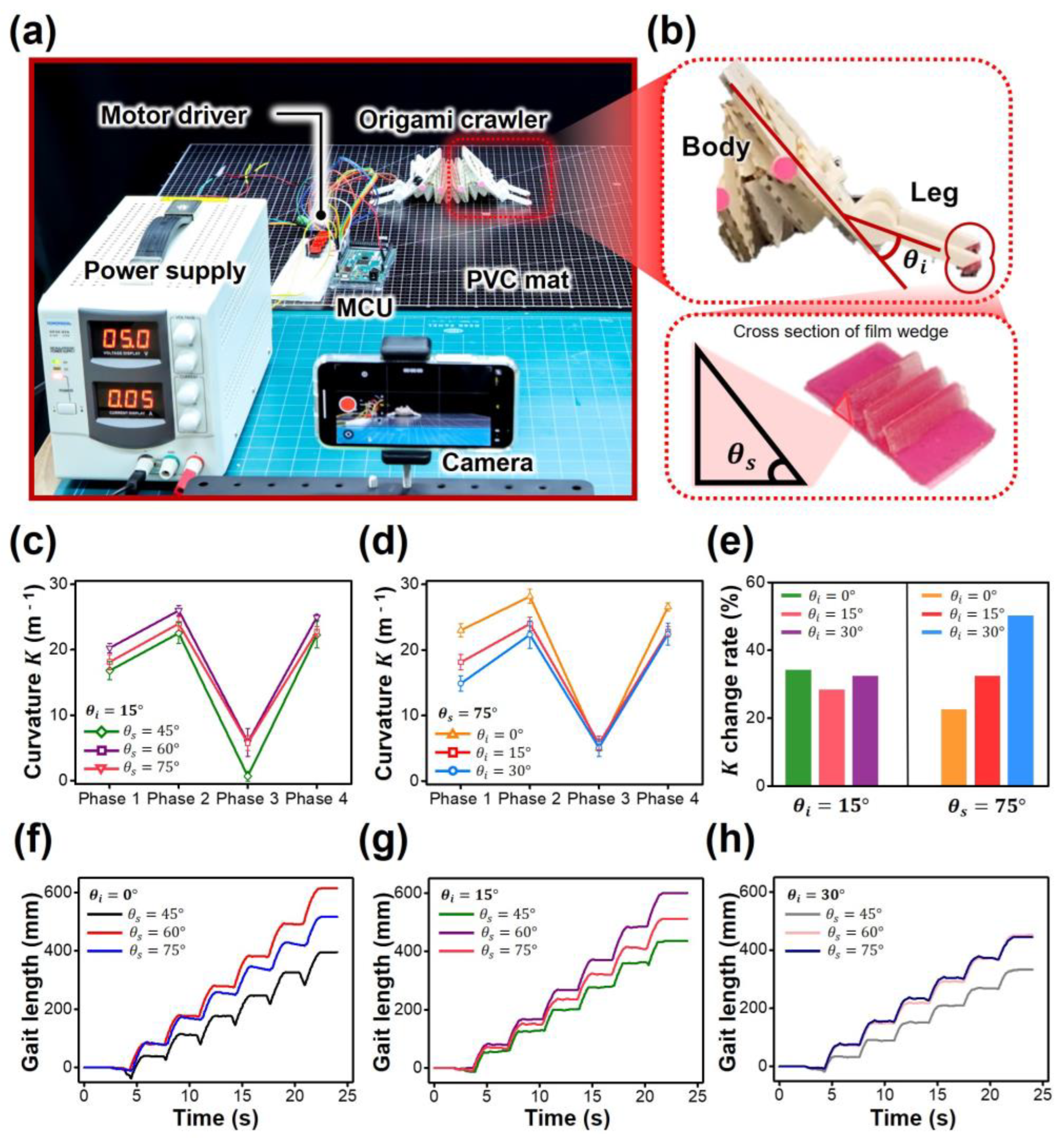
| Curvature Change (Phase 1–2, %) | ||
|---|---|---|
| 75 (fixed) | 0 | 22.47 |
| 15 | 32.33 | |
| 30 | 50.03 | |
| 45 | 15 (fixed) | 34.03 |
| 60 | 28.16 | |
| 75 | 32.33 |
| Total Gait Length (6 Cycles, mm) | Gait Loss (Mean, mm) | Gait Loss Ratio (%) | ||
|---|---|---|---|---|
| 0 | 45 | 395.15 | 29.68 | 31.41 |
| 60 | 615.17 | 3.77 | 3.59 | |
| 75 | 516.43 | 8.86 | 9.39 | |
| 15 | 45 | 435.42 | 4.33 | 8.94 |
| 60 | 600.58 | 1.94 | 1.96 | |
| 75 | 511.24 | 4.36 | 4.92 | |
| 30 | 45 | 332.48 | 4.39 | 7.49 |
| 60 | 451.58 | 2.25 | 2.96 | |
| 75 | 444.71 | 6.74 | 8.37 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kim, H.; Cho, S.; Kam, D.; Lee, S.J.; Park, S.; Choi, D.; Kim, J. Tendon-Driven Crawling Robot with Programmable Anisotropic Friction by Adjusting Out-of-Plane Curvature. Machines 2023, 11, 763. https://doi.org/10.3390/machines11070763
Kim H, Cho S, Kam D, Lee SJ, Park S, Choi D, Kim J. Tendon-Driven Crawling Robot with Programmable Anisotropic Friction by Adjusting Out-of-Plane Curvature. Machines. 2023; 11(7):763. https://doi.org/10.3390/machines11070763
Chicago/Turabian StyleKim, Hyeonsu, Sumin Cho, Dongik Kam, Seong Jin Lee, Seongjae Park, Dongwhi Choi, and Jongwoo Kim. 2023. "Tendon-Driven Crawling Robot with Programmable Anisotropic Friction by Adjusting Out-of-Plane Curvature" Machines 11, no. 7: 763. https://doi.org/10.3390/machines11070763
APA StyleKim, H., Cho, S., Kam, D., Lee, S. J., Park, S., Choi, D., & Kim, J. (2023). Tendon-Driven Crawling Robot with Programmable Anisotropic Friction by Adjusting Out-of-Plane Curvature. Machines, 11(7), 763. https://doi.org/10.3390/machines11070763







