Loss Analysis of a Transonic Rotor with a Differential Approach to Entropy Generation
Abstract
1. Introduction
1.1. Review of Differential Entropy Generation
1.2. Research into Loss in Turbomachinery
1.3. Motivation for Present Research
1.4. Structure of This Paper
2. Case Studies
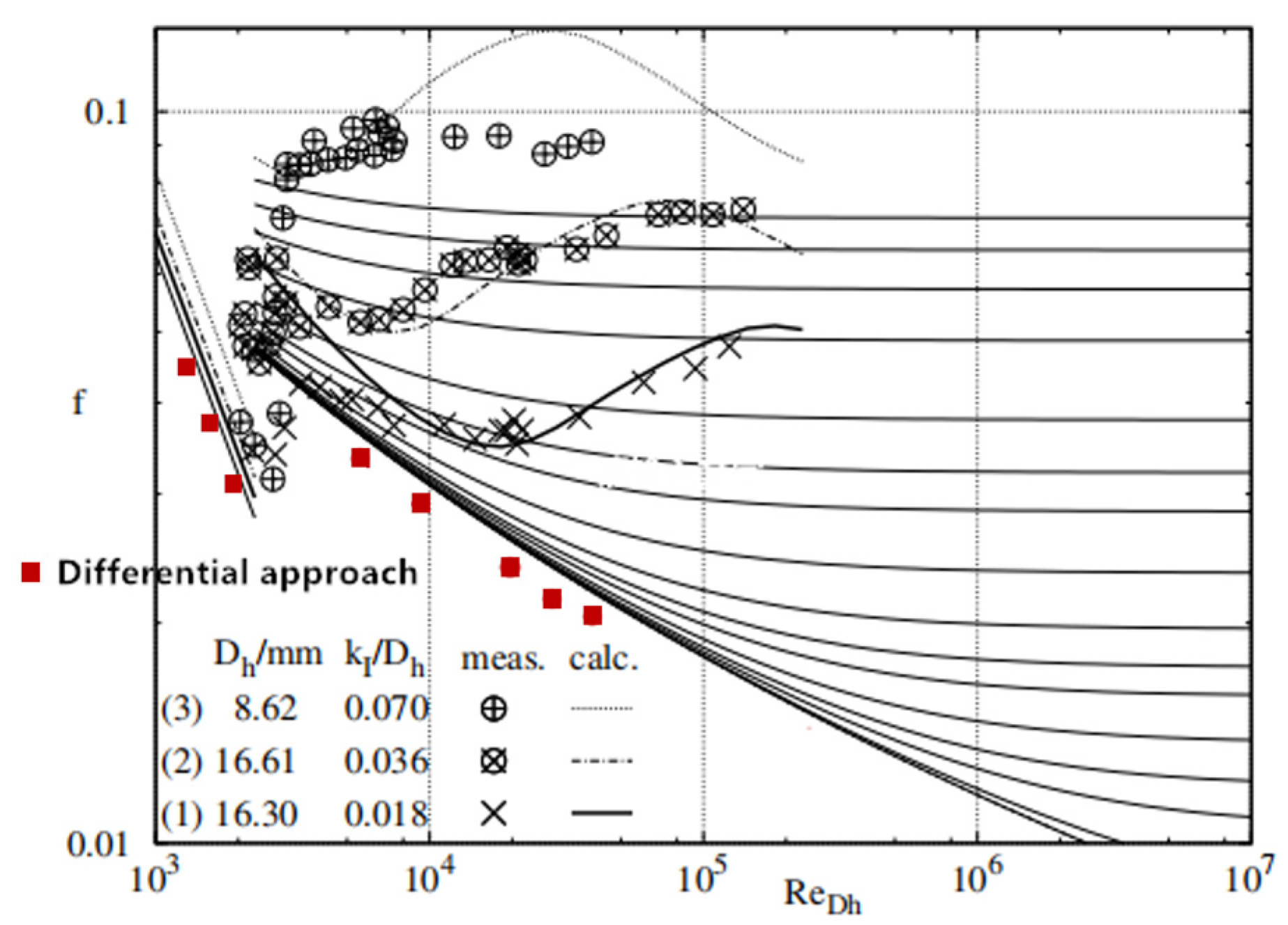
2.1. Case One: Laminar and Turbulent Incompressible Flows in Straight Circular Ducts
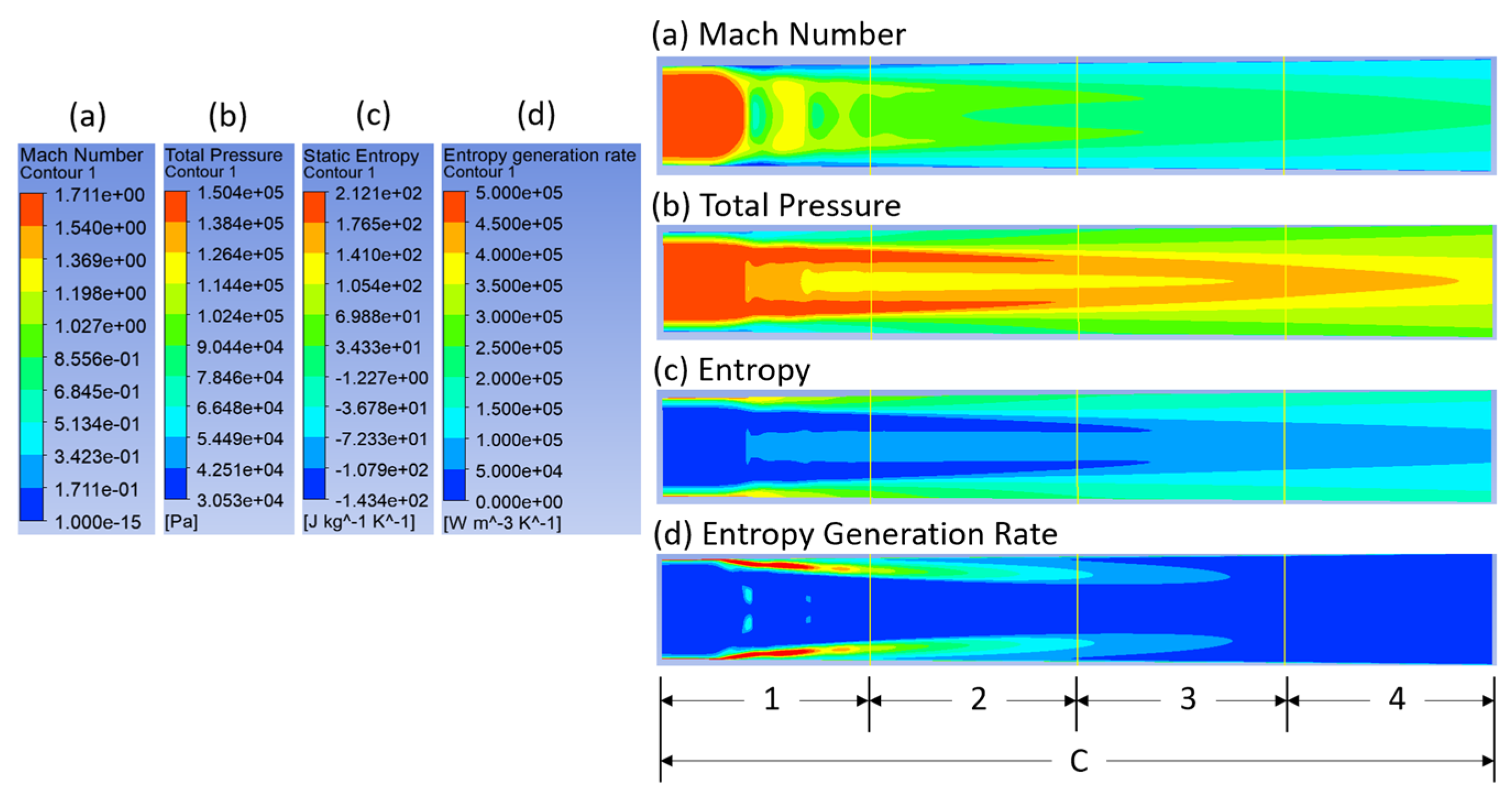
2.2. Case Two: Turbulent Compressible Flows in a Convergent-and-Divergent Nozzle (Laval Nozzle)
3. Identification of Loss Sources in an Axial Compressor Rotor
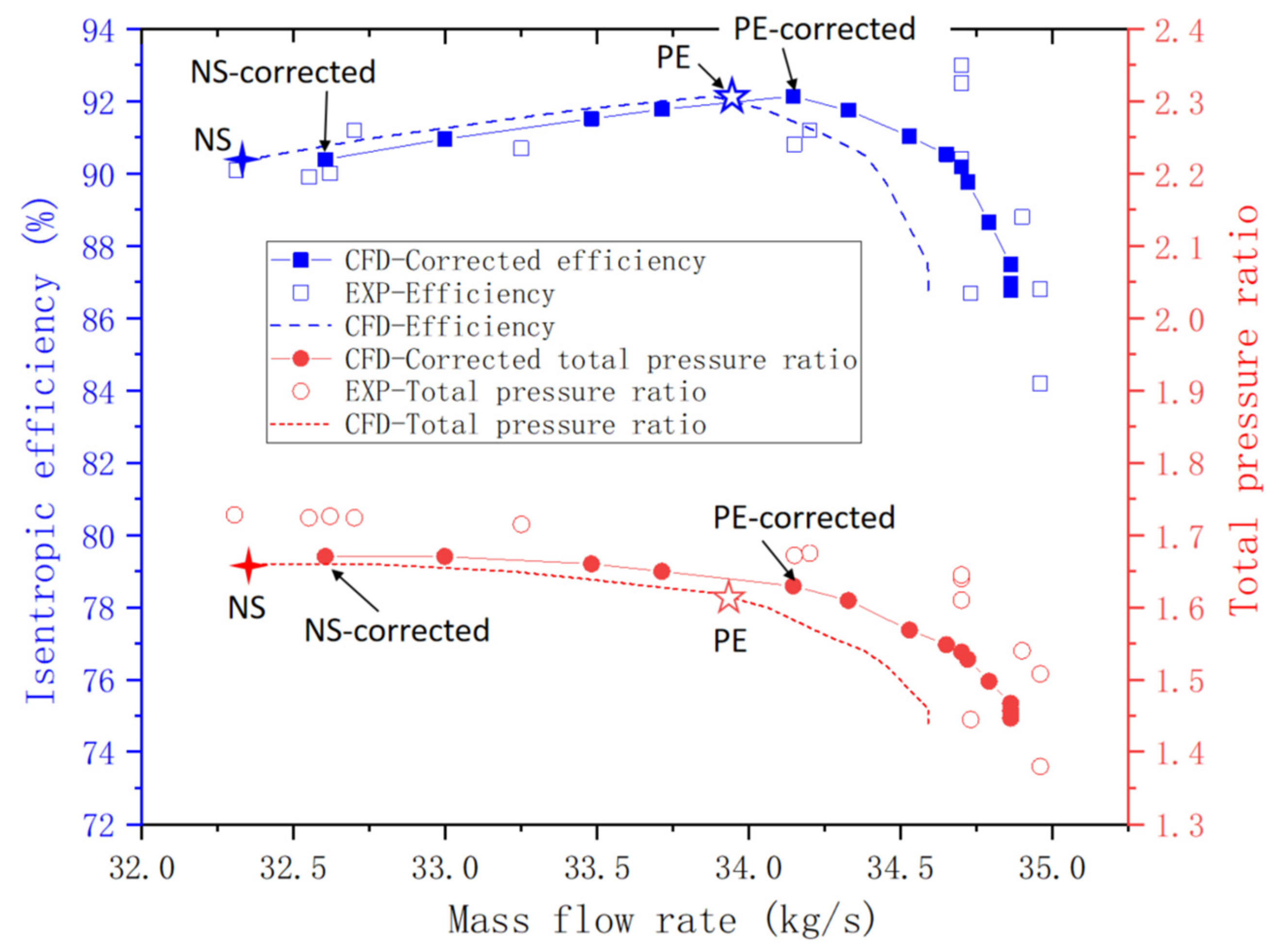
3.1. Validation of Numerical Simulation
3.2. Flow Structures and Loss Sources
- (1)
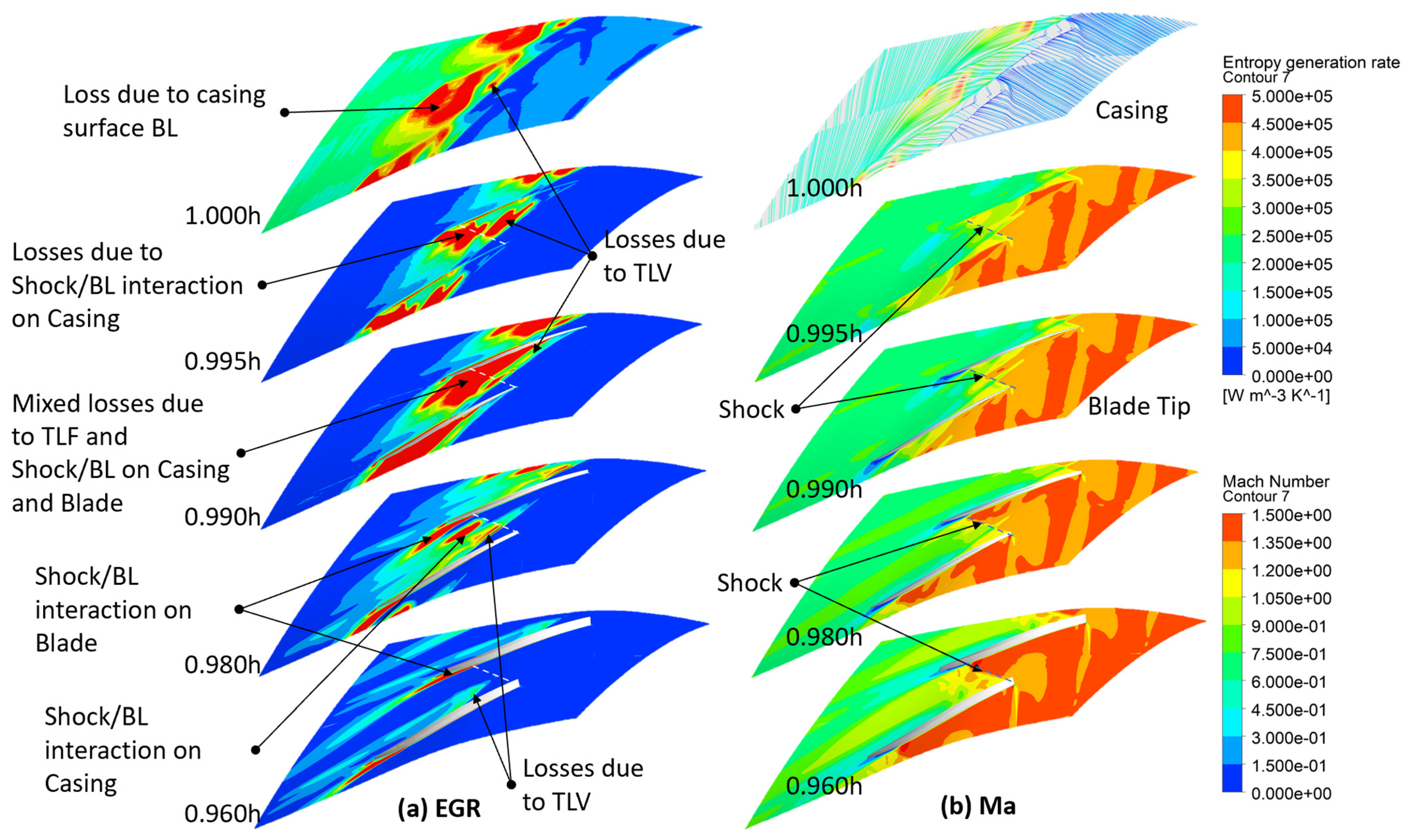
- Tip section: Tip leakage flow, wherein a tip leakage vortex is initiated near the leading edge [19,20,21,22,23]; shock, and shock–boundary-layer interaction on the casing surface and on the blade’s suction surface. Some of the aforementioned flow structures cannot be directly observed from the limiting streamlines on the blade surface. However, they have been demonstrated in many studies because R67 has been widely studied worldwide ever since its data were made accessible to the public over 30 years ago.
- (2)
- (3)
- Through-flow section: most simple and clean flow with only a weak shock.
- (4)
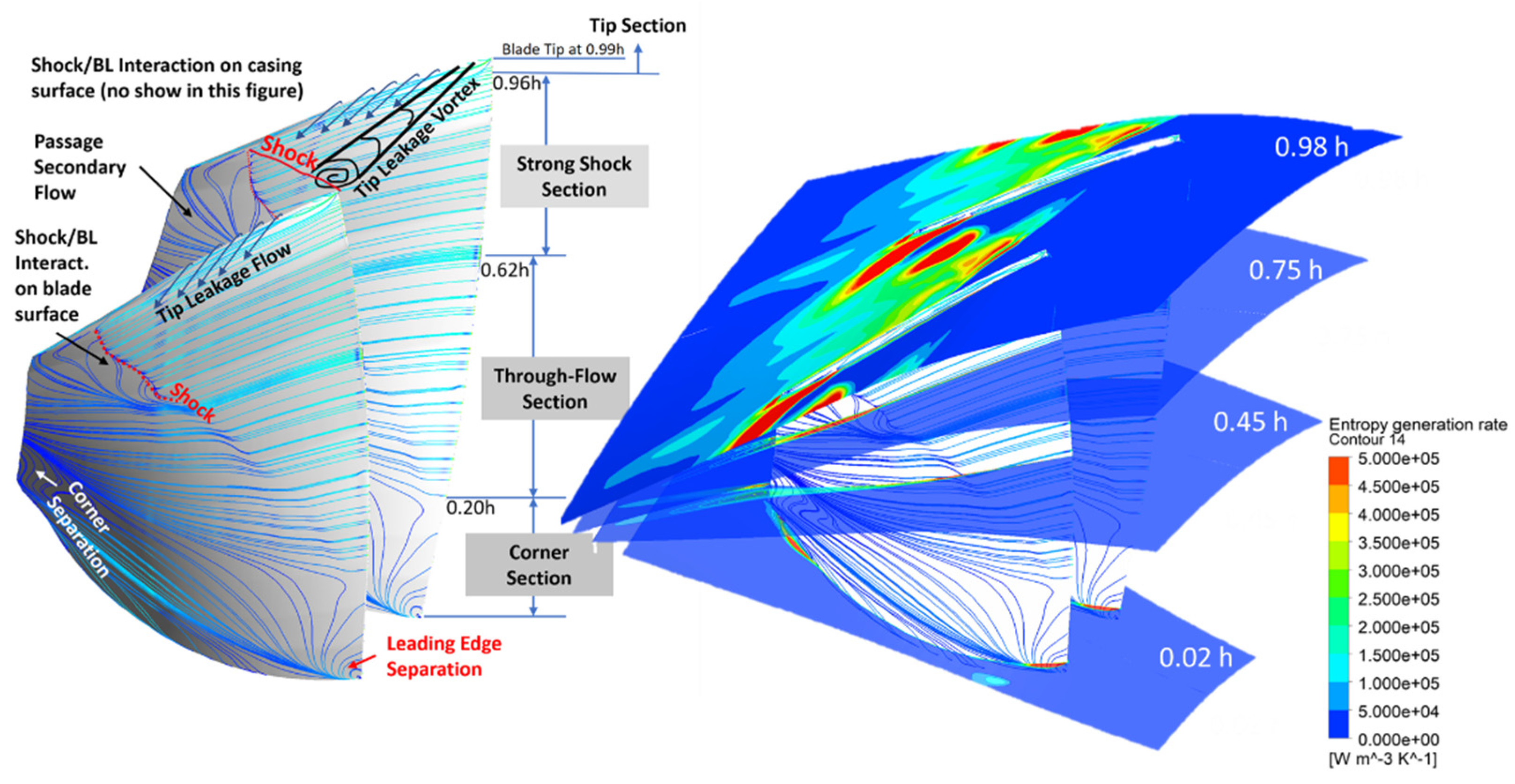
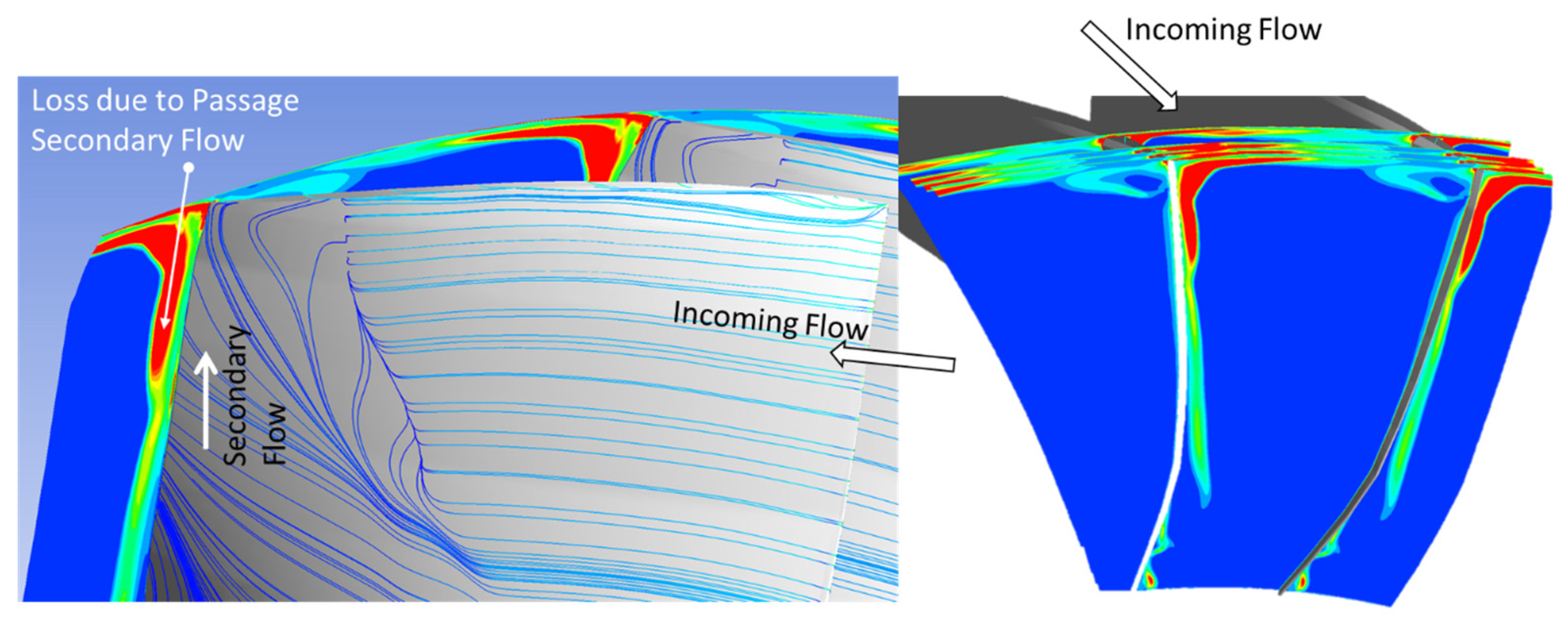
3.2.1. Details in the Tip Section
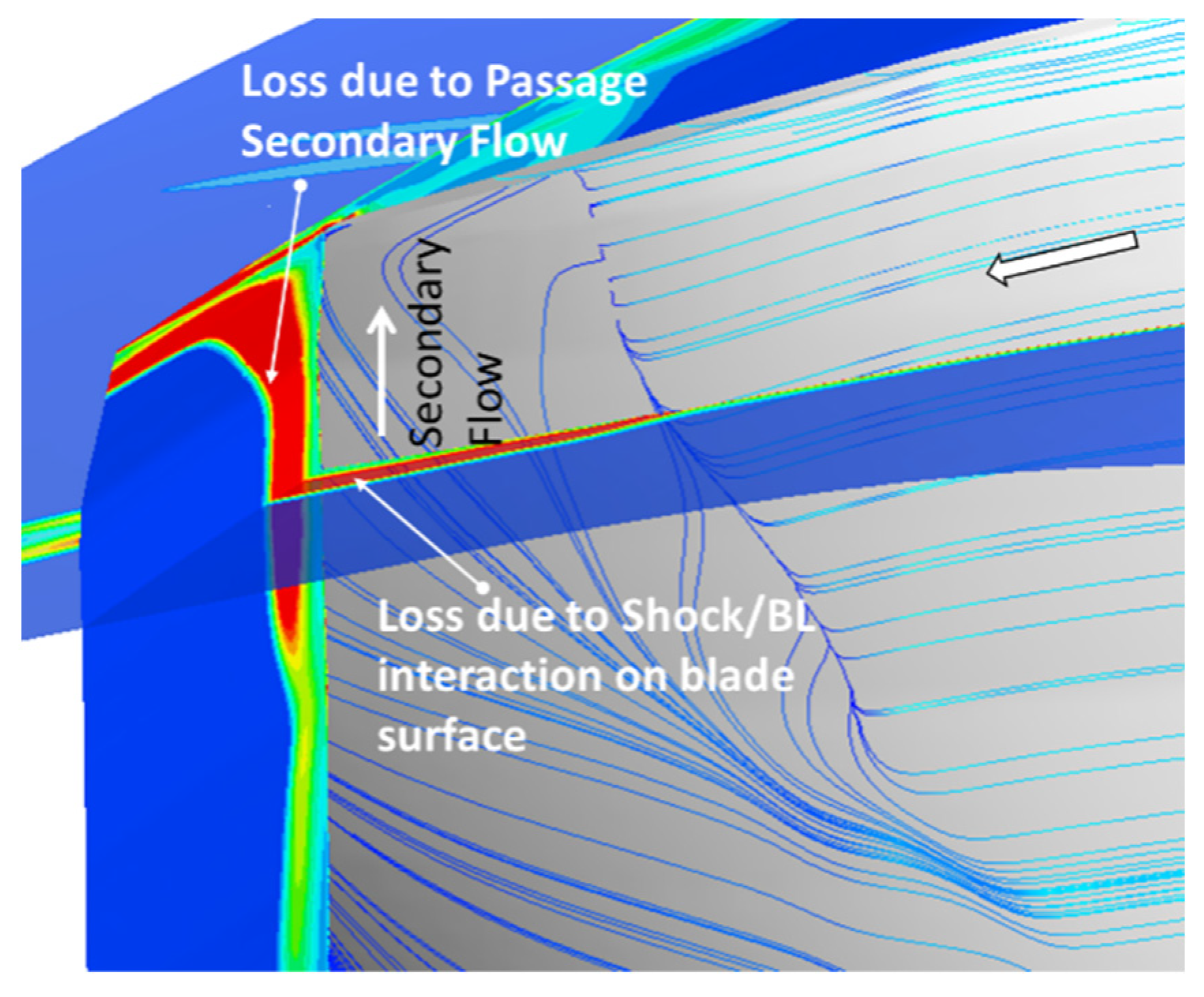
3.2.2. Details in the Strong-Shock Section
4. Summary and Conclusions
- (1)
- Grid Independence. Grid independence should not only be based on the common indicators but additionally on the overall entropy generation. The required number of grids (and the quality of the grids) is far greater than the normal number.
- (2)
- Test Case 1—Straight Ducts. The results of straight ducts show that the differential approach results are qualitatively consistent with the experimental data (the Moody chart) for both laminar and turbulent flows (only with a smooth wall). Therefore, the differential approach should be used as a tool for qualitative comparison rather than for quantitative design.
- (3)
- Test Case 2—Laval nozzle. The EGR due to the shock itself is much lower than that of the separation induced by the shock–boundary-layer interaction. In other words, the turbulence caused by the separation produces far more loss than the irreversibility across the shock waves. This is a key feature for identifying the loss sources within the transonic rotor R67.
- (4)
- R67 at near Peak Efficiency.
- a.
- About 63% of the total loss stems from the upper 0.38h of the blade span (0.62h–1.00h), which is mainly due to the shock–boundary-layer interaction on the casing and on the blade’s suction surface, tip leakage flow, tip leakage vortex, and passage secondary flow. The remaining 37% loss is produced by the main blade span (0.2h–0.62h). The corner section (0h–0.2h), which includes the corner separation, accounts for only 13% of the loss, which is surprising because this is quite different from the results of the cascade research. Therefore, the above loss-source identification indicates that the casing boundary layer makes an important contribution to the overall entropy generation. Loss-source identification indicates that the casing boundary layer makes an important contribution to the overall entropy generation. It interacts with the shock, the tip leakage flow, and the tip leakage vortex, all of which are major sources of loss.
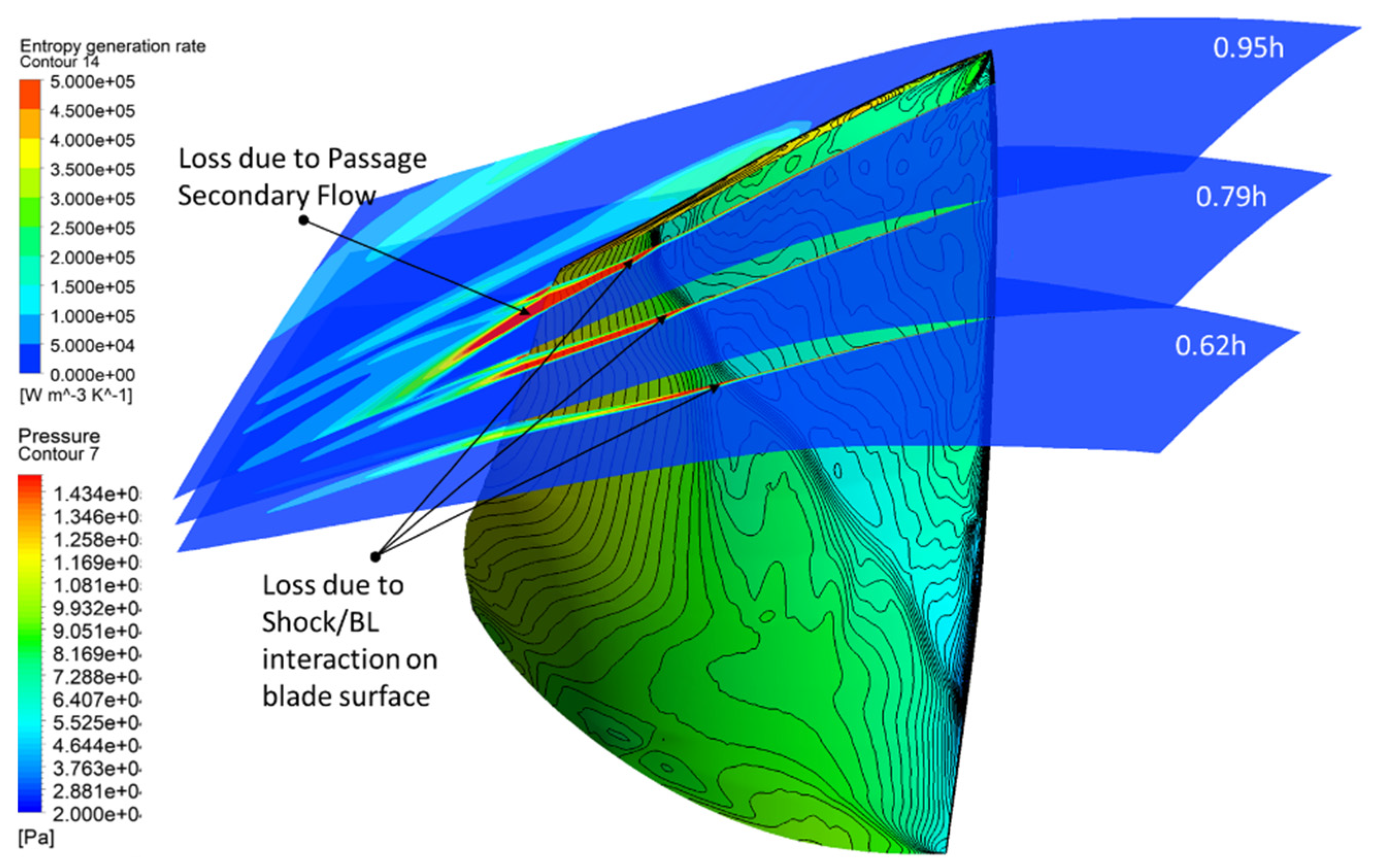
- b.
- Passage secondary flow is a very important contributor to the total loss. Its loss is generated in the portion of the blade close to the trailing edge, which smoothly merges with the shock/boundary layer on the blade suction surface. Therefore, these two loss sources are barely separable. However, with the help of the differential approach, both these loss sources are determined to be equally important. This recalls a debate in 1991, where Li and Cumpsty [37] presented their measurements and argued that the circumferential mixing due to passage secondary vortex contributes the most loss in a large low-speed compressor stage. In contrast, Leylek and Wisler [38] demonstrated that both secondary flows and turbulent diffusion play important roles in the mixing process and contribute to both spanwise and cross-passage mixing. The relative importance of each of these two mechanisms depends on configuration and loading. Using the current CFD tool, the details of flow can be visualized and EGR contours can be plotted, providing a means to resolve the debate, as shown in this study.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature and Abbreviations
| Nomenclature | |
| thermal diffusivity, (m2/s) | |
| t | thermal diffusivity of the fluctuating temperature, (m2/s) |
| Local dissipation rate of turbulent kinetic energy component, W kg−1 | |
| dimensionless temperature, K | |
| thermal conductivity, J s−1 m−1 K−1 | |
| dynamic viscosity, kg m−1 s−1 | |
| Density, kg m−3 | |
| local shear stress component, kg m−1 s2−1 | |
| entropy production term, (WK/m3) | |
| characteristic frequency, MHz | |
| Dh | hydraulic diameter, m |
| energy, J kg−1 | |
| local specific internal energy, J kg−1 | |
| local relative total energy, J kg−1 | |
| local specific enthalpy, J kg−1 | |
| sum of specific enthalpy, J kg−1 | |
| local specific exergy of a gas in an open system, J kg−1 | |
| head loss coefficient | |
| k | turbulent kinetic energy, (m2/s2) |
| L | length of the pipe or channel, m |
| mass flow rate, kg s−1 | |
| rotation speed, rpm | |
| local pressure, N m−2 | |
| heat transfer rates, W/(m2·K) | |
| mass flow, kg s−1 | |
| Entropy, J K−1 m−3 s−1 | |
| entropy generation rate density in the flow field, J K−1 m−3 s−1 | |
| entropy generation rate density in the temperature field, J K−1 m−3 s−1 | |
| total entropy generation rate, (J/(kg K)) | |
| entropy production rate by turbulent dissipation, (W/(m3 K)) | |
| entropy production rate by viscous dissipation, (W/(m3 K)) | |
| entropy production rate by heat transfer with gradients of the fluctuating temperature, (W/(m3 K)) | |
| entropy production rate by heat transfer with mean temperature gradients, (W/(m3 K)) | |
| s | entropy per unit mass, (J/(kg K)) |
| Local entropy per unit mass component, (J/(kg K)) | |
| bulk temperature, K | |
| temperature, K | |
| temperature reservoirs, K | |
| t | time, (s) |
| local velocity component, m s−1 | |
| local velocity component, m s−1 | |
| local frame velocity component, m s−1 | |
| Power, W | |
| reversible power, W | |
| coordinate vector component, m | |
| Abbreviations | |
| EG | Entropy generation |
| EGR | Entropy generation rate |
| EXP | Experimental data |
| NS | Near stall |
| NS-corrected | Corrected near stall |
| PE | Peak efficiency |
| PE-corrected | Corrected peak efficiency |
| R67 | NASA Rotor 67 |
| Subscripts | |
| gen | generation rate |
| turbulent dissipation | |
| viscous dissipation | |
| heat transfer with gradients of the fluctuating temperature | |
| heat transfer with mean temperature gradients | |
| reversible | |
| t | relative total |
References
- Bejan, A. Entropy generation minimization: The new thermodynamics of finite size devices and finite time processes. J. Appl. Phys. 1996, 79, 1191–1218. [Google Scholar] [CrossRef]
- Kock, F.; Herwig, H. Local entropy production in turbulent shear flows: A high-Reynolds number model with wall functions. Int. J. Heat Mass Transf. 2004, 47, 2205–2215. [Google Scholar] [CrossRef]
- Herwig, H.; Schmandt, B. How to determine losses in a flow field: A paradigm shift towards the second law analysis. Entropy 2014, 16, 2959–2989. [Google Scholar] [CrossRef]
- Schmandt, B.; Herwig, H. Losses due to conduit components: An optimization strategy and its application. J. Fluid Mech. 2016, 138, 031204. [Google Scholar] [CrossRef]
- Jin, Y.; Du, J.; Li, Z.Y.; Zhang, H.W. Second-law analysis of irreversible losses in gas turbines. Entropy 2017, 19, 470. [Google Scholar] [CrossRef]
- Geng, L.P.; Jin, Y.; Herwig, H. Can pulsation unsteadiness increase the convective heat transfer in a pipe flow? A systematic study. Numer. Heat Transf. Part B Fundam. 2020, 78, 160–174. [Google Scholar] [CrossRef]
- Greitzer, E.M.; Tan, C.S.; Graf, M.B. Internal Flow; Cambridge University Press: New York, NY, USA, 2004. [Google Scholar]
- Denton, J.; Pullan, G. A numerical investigation into the sources of endwall loss in axial flow turbines. In Proceedings of the ASME Turbo Expo 2012, Copenhagen, Denmark, 11–15 June 2012. [Google Scholar] [CrossRef]
- Zlatinov, M.B.; Tan, C.S.; Montgomery, M.; Islam, T.; Harris, M. Turbine hub and shroud sealing flow loss mechanisms. J. Turbomach. 2012, 134, 061027. [Google Scholar] [CrossRef]
- Lin, D.; Yuan, X.; Su, X.R. Local entropy generation in compressible flow through a high pressure turbine with delayed detached eddy simulation. Entropy 2017, 19, 29. [Google Scholar] [CrossRef]
- Zhang, L.; Lang, J.H.; Jiang, K.; Wang, S.L. Simulation of entropy generation under stall conditions in a centrifugal fan. Entropy 2014, 16, 3573–3589. [Google Scholar] [CrossRef]
- Wang, H.; Lin, D.; Su, X.R.; Yuan, X. Entropy analysis of the interaction between the corner separation and wakes in a compressor cascade. Entropy 2017, 19, 324. [Google Scholar] [CrossRef]
- Li, Z.Y.; Du, J.; Jemcov, A.; Ottavy, X.; Lin, F. A study of loss mechanism in a linear compressor cascade at the corner stall condition. In Proceedings of the ASME Turbo Expo 2017: Turbine Technical Conference and Exposition, Charlotte, NC, USA, 26–30 June 2017. [Google Scholar] [CrossRef]
- Zhang, Q.F.; Du, J.; Li, Z.H.; Li, J.C.; Zhang, H.W. Entropy generation analysis in a mixed-flow compressor with casing treatment. J. Therm. Sci. 2019, 28, 915–928. [Google Scholar] [CrossRef]
- Ma, W.; Ottavy, X.; Lu, L.P.; Leboeuf, F.; Gao, F. Experimental investigations of corner stall in a linear compressor cascade. In Proceedings of the ASME 2011 Turbo Expo: Turbine Technical Conference and Exposition, Vancouver, BC, Canada, 6–10 June 2011. [Google Scholar] [CrossRef]
- You, D.; Wang, M.; Moin, P.; Mittal, R. Large-eddy simulation analysis of mechanisms for viscous losses in a turbomachinery tip-clearance flow. J. Fluid Mech. 2007, 586, 177–204. [Google Scholar] [CrossRef]
- Katiyar, S.; Sarkar, S. Flow transition on the suction surface of a controlled-diffusion compressor blade using a large-eddy simulation. Phys. Fluids 2022, 34, 094108. [Google Scholar] [CrossRef]
- Zhu, H.L.; Zhou, L.; Meng, T.T.; Ji, L.C. Corner stall control in linear compressor cascade by blended blade and endwall technique based on large eddy simulation. Phys. Fluids 2021, 33, 115124. [Google Scholar] [CrossRef]
- Du, H.; Yu, X.; Zhang, Z.; Liu, B. Relationship between the flow blockage of TLV and its evolutionary procedures inside the rotor passage of a subsonic axial compressor. J. Therm. Sci. 2013, 22, 522–531. [Google Scholar] [CrossRef]
- Gao, Y.; Liu, Y.; Zhong, L.; Hou, J.; Lu, L. Study of the standard k-ε model for tip leakage flow in an axial compressor rotor. Int. J. Turbo Jet-Engines 2016, 33, 353–360. [Google Scholar] [CrossRef]
- Smith, M.H.; Pullan, G.; Grimshaw, S.D.; Greitzer, E.M.; Spakovszky, Z.S. The role of tip leakage flow in spike-type rotating stall inception. J. Turbomach. 2019, 141, 061010. [Google Scholar] [CrossRef]
- Zhang, B.T.; Liu, B.; Mao, X.C.; Wang, H.J.; Yang, Z.H.; Li, Z.Y. Interaction mechanism between the tip leakage flow and inlet boundary layer in a highly loaded compressor cascade based on scale-adaptive simulation. Phys. Fluids 2022, 34, 116112. [Google Scholar] [CrossRef]
- Ventosa-Molina, J.; Lange, M.; Mailach, R.; Fröhlich, J. Study of relative endwall motion effects in a compressor cascade through direct numerical simulations. J. Turbomach. 2021, 143, 011005. [Google Scholar] [CrossRef]
- Niu, H.; Chen, J.; Xiang, H.; Du, G. Investigation of incoming boundary layer effects on the flow field of transonic compressor rotor. J. Eng. Therm. Energy Power 2021, 36, 60–68. [Google Scholar]
- Hou, J.X.; Liu, Y.W.; Zhong, L.Y.; Zhong, W.B.; Tang, Y.M. Effect of vorticity transport on flow structure in the tip region of axial compressors. Phys. Fluids 2022, 34, 055102. [Google Scholar] [CrossRef]
- Liu, Y.; Yan, H.; Liu, Y.; Lu, L.; Li, Q. Numerical study of corner separation in a linear compressor cascade using various turbulence models. Chin. J. Aeronaut. 2016, 29, 639–652. [Google Scholar] [CrossRef]
- Wang, Z.; Geng, S.; Zhang, H. Effects of inlet boundary layer on corner flow in a linear compressor cascade. J. Propul. Technol. 2017, 38, 54–60. [Google Scholar]
- Meng, T.; Li, X.; Zhou, L.; Zhu, H.; Li, J.; Ji, L. Large eddy simulation and combined control of corner separation in a compressor cascade. Phys. Fluids 2022, 34, 075113. [Google Scholar] [CrossRef]
- Hou, J.; Zhou, C. Loss mechanism of low-pressure turbine secondary flows due to different incoming boundary layers. J. Eng. Gas Turbines Power 2020, 142, 101004. [Google Scholar] [CrossRef]
- Gmelin, C.; Thiele, F.; Liesner, K.; Meyer, R. Investigations of Secondary Flow Suction in a High Speed Compressor Cascade. In Proceedings of the ASME 2011 Turbo Expo: Turbine Technical Conference and Exposition, Vancouver, BC, Canada, 6–10 June 2011; pp. 405–415. [Google Scholar]
- Liesner, K.; Meyer, R.; Lemke, M.; Gmelin, C.; Thiele, F. On the efficiency of secondary flow suction in a compressor cascade. In Proceedings of the ASME Turbo Expo 2010: Power for Land, Sea, and Air, Glasgow, UK, 14–18 June 2010; pp. 151–160. [Google Scholar]
- Krug, A.; Busse, P.; Vogeler, K. Experimental investigation into the effects of the steady wake-tip clearance vortex interaction in a compressor cascade. J. Turbomach. 2015, 137, 061006. [Google Scholar] [CrossRef]
- Babu, S.; Chatterjee, P.; Pradeep, A.M. Transient nature of secondary vortices in an axial compressor stage with a tandem rotor. Phys. Fluids 2022, 34, 065125. [Google Scholar] [CrossRef]
- White, F.M. Fluid Mechanics, 6th ed.; McGraw-Hill College: New York, NY, USA, 2006. [Google Scholar]
- Liepmann, H.W.; Roshko, A. Elements of Gas Dynamics; John Wiley & Sons: New York, NY, USA, 1957. [Google Scholar]
- Strazisar, A.J.; Wood, J.R.; Hathaway, M.D.; Suder, K.L. Laser Anemometer Measurements in a Transonic Axial-Flow Fan Rotor; NASA Technical Paper; NASA: Washington, DC, USA, 1989; p. 2879. [Google Scholar]
- Li, Y.S.; Cumpsty, N.A. Mixing in axial flow compressors: Part I-test facilities and measurements in a four-stage compressor. J. Turbomach. 1991, 113, 161–165. [Google Scholar] [CrossRef]
- Leylek, J.H.; Wisler, D.C. Mixing in axial-flow compressors: Conclusions drawn from three-dimensional Navier-Stokes analyses and experiments. J. Turbomach. 1991, 113, 139–143. [Google Scholar] [CrossRef]

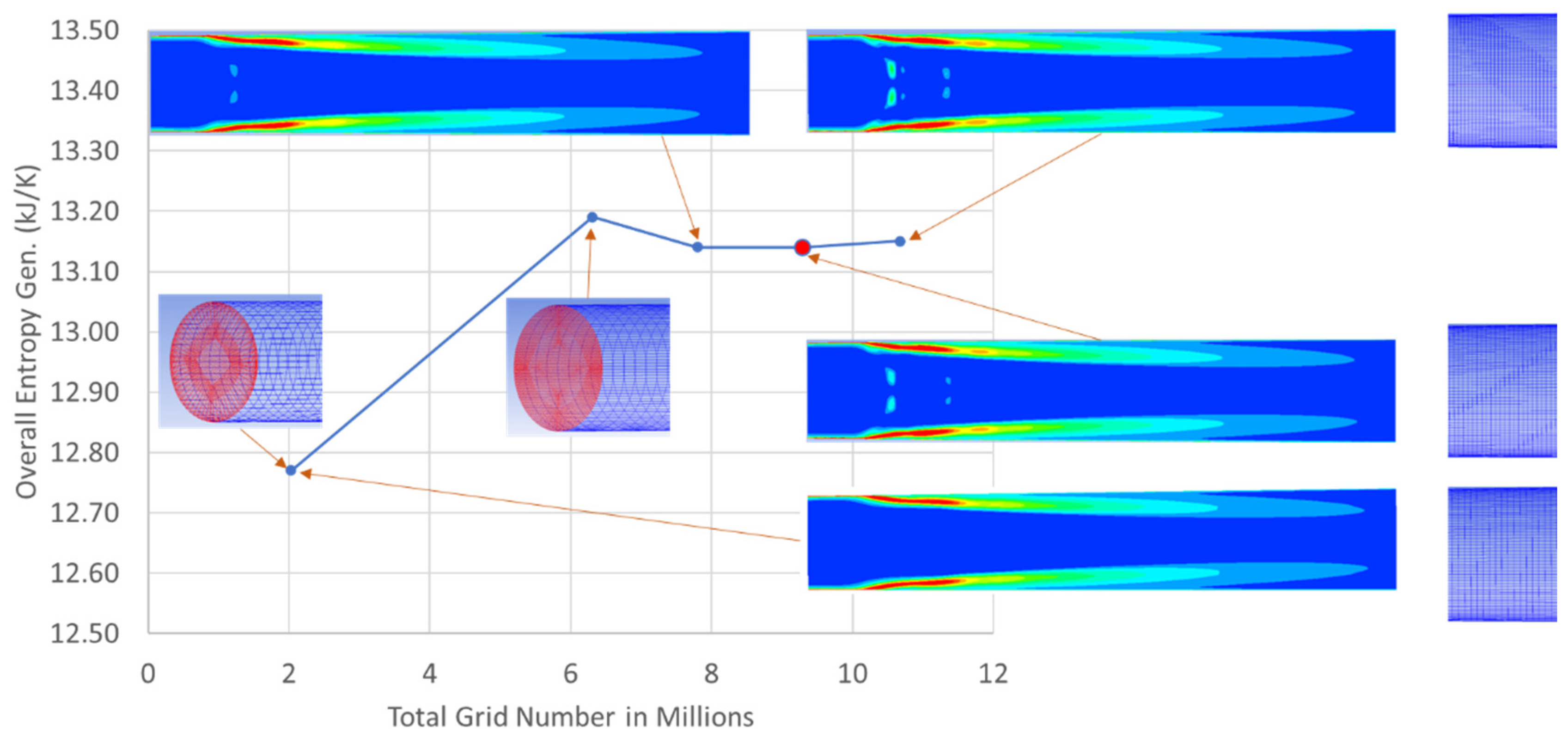



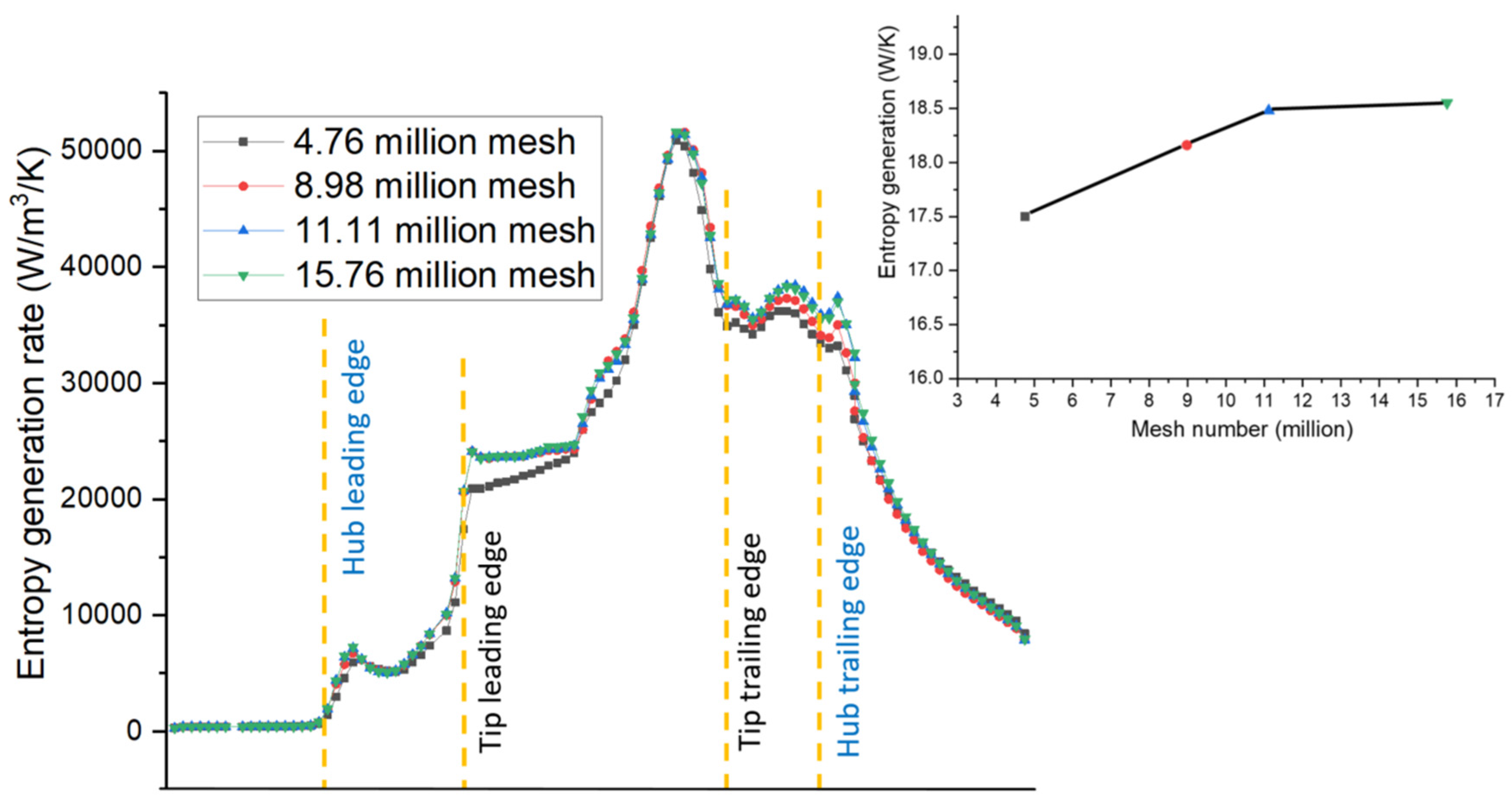
| Mesh Number (Million) | Mass Flow (kg/s) | Efficiency (%) | Total Pressure Ratio | Entropy Generation of Rotor Passage (W/K) | Percentage of Entropy Generation Variance (%) |
|---|---|---|---|---|---|
| 4.76 | 34.31 | 90.6 | 1.54 | 17.5 | −5.3 |
| 8.98 | 34.39 | 90.6 | 1.54 | 18.16 | −1.73 |
| 11.11 | 34.38 | 90.6 | 1.54 | 18.48 | base |
| 15.76 | 34.38 | 90.6 | 1.54 | 18.55 | 0.38 |
| Section | Entropy Generation (W/K) | Percentage |
|---|---|---|
| Tip (0.631.00h) | 4.71 | 27% |
| Strong Shock (0.62–0.96h) | 6.26 | 36% |
| Through-Flow (0.20–0.62h) | 4.3 | 24% |
| Corner (0.00–0.20h) | 2.34 | 13% |
| TOTAL | 17.61 | 100% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ma, J.; Lin, F. Loss Analysis of a Transonic Rotor with a Differential Approach to Entropy Generation. Machines 2023, 11, 472. https://doi.org/10.3390/machines11040472
Ma J, Lin F. Loss Analysis of a Transonic Rotor with a Differential Approach to Entropy Generation. Machines. 2023; 11(4):472. https://doi.org/10.3390/machines11040472
Chicago/Turabian StyleMa, Jingyuan, and Feng Lin. 2023. "Loss Analysis of a Transonic Rotor with a Differential Approach to Entropy Generation" Machines 11, no. 4: 472. https://doi.org/10.3390/machines11040472
APA StyleMa, J., & Lin, F. (2023). Loss Analysis of a Transonic Rotor with a Differential Approach to Entropy Generation. Machines, 11(4), 472. https://doi.org/10.3390/machines11040472






