Study on the 2D Equivalent Simulation Model of Bearing and Spindle for Precision Machine Tools
Abstract
1. Introduction
2. Bearing and Spindle
2.1. Angular Contact Ball Bearing—7008C
2.2. Mechanical Spindle—BT30
3. Methodology
3.1. Equivalent 2D Model of Bearing
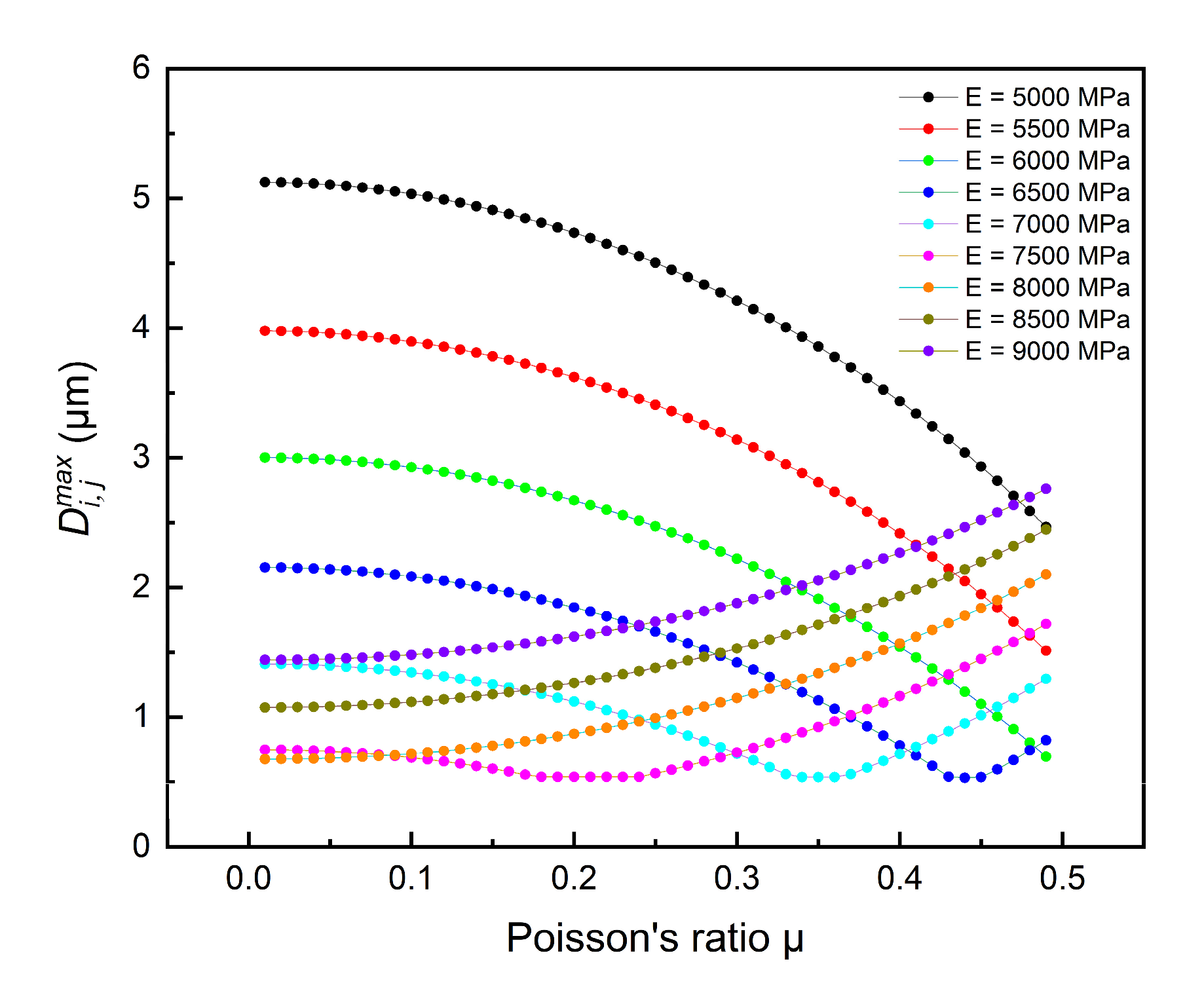
3.2. Parametric Inversed Method
3.3. Hertz Point/Line Contact Theory
3.4. Equivalent 2D Model of Spindle
4. Results and Discussion
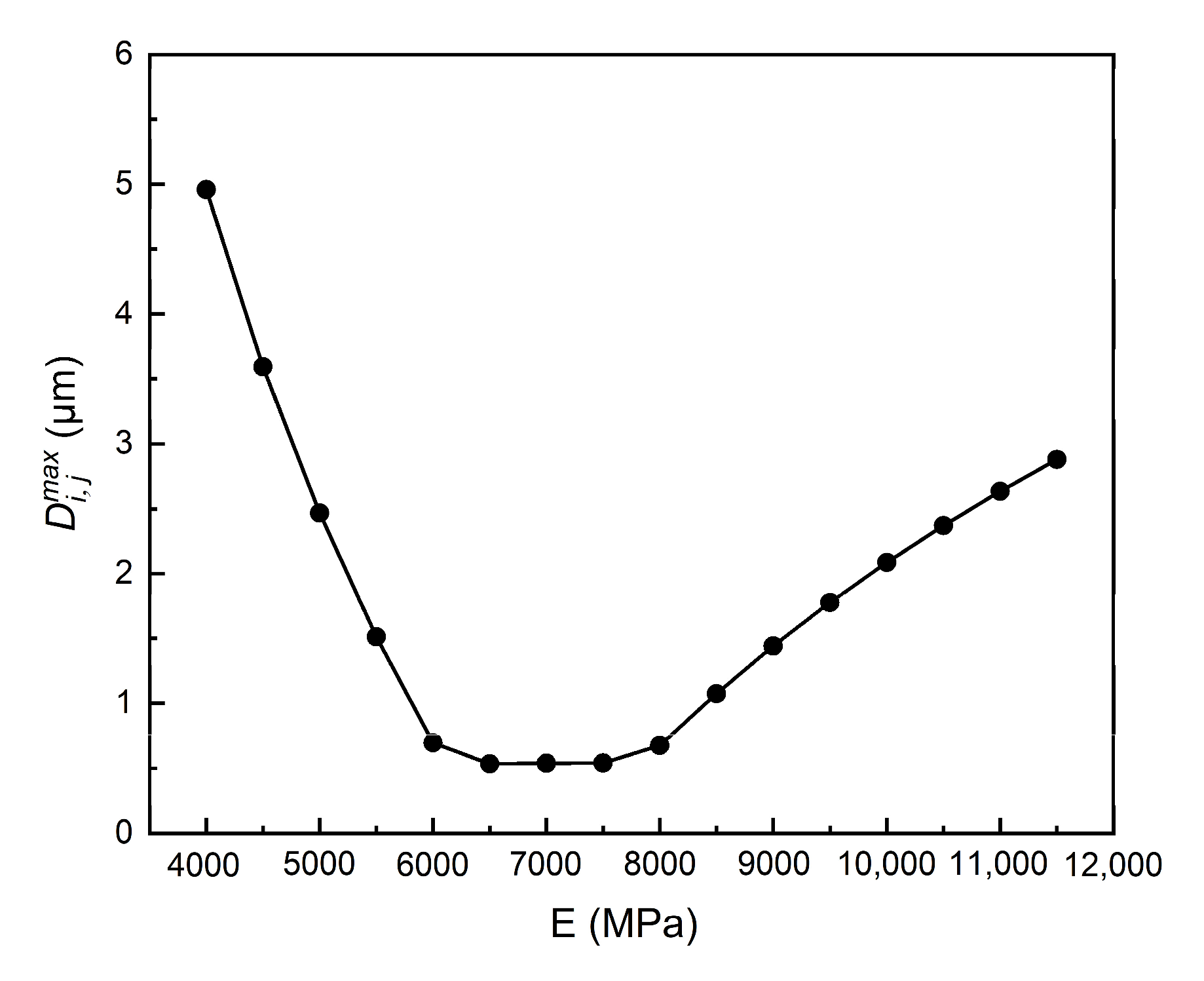
4.1. Parameter Reversion of 2D Virtual Bearing Ball
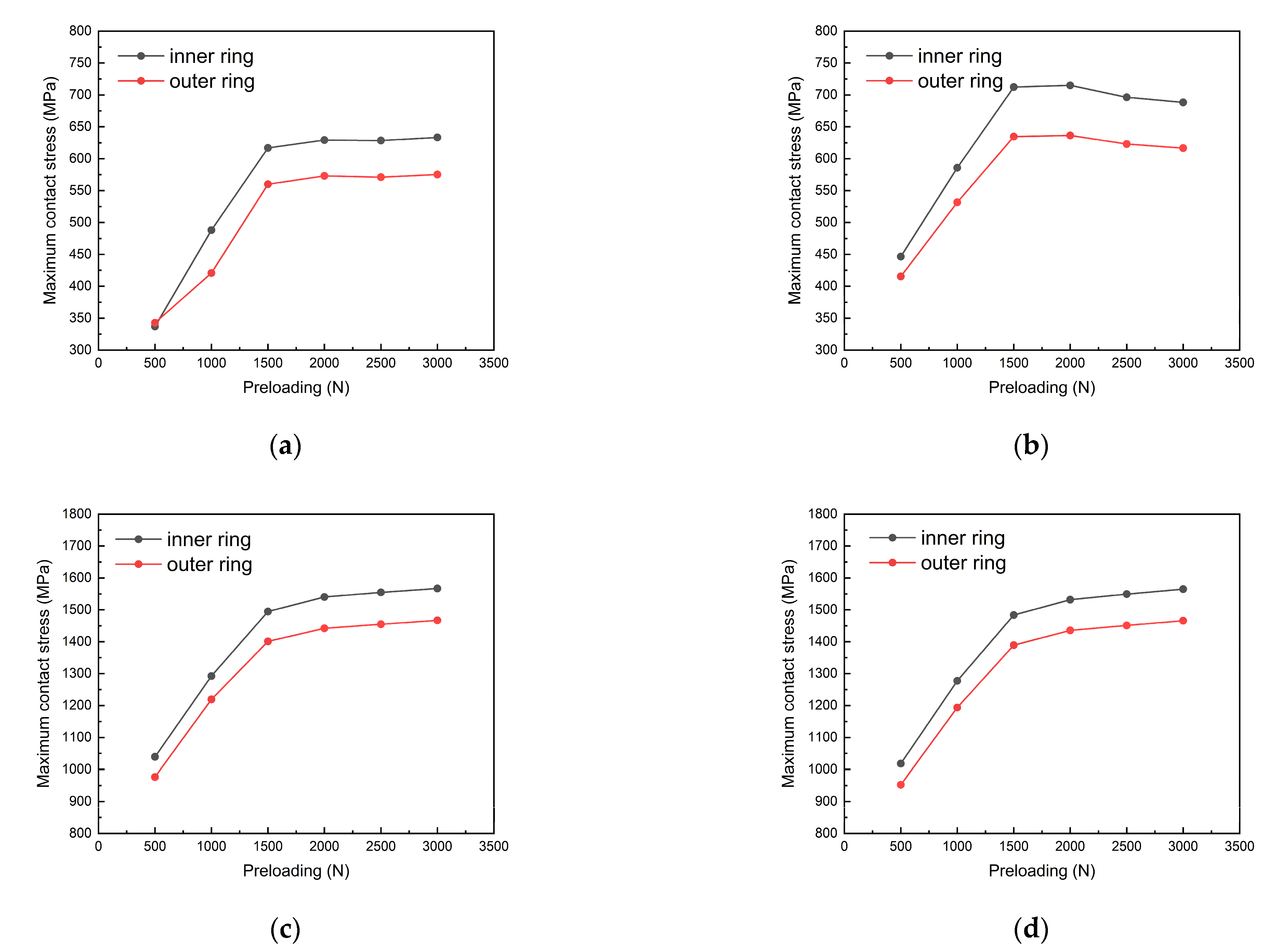
4.2. Contact Stress Calculation of 2D Bearing Model
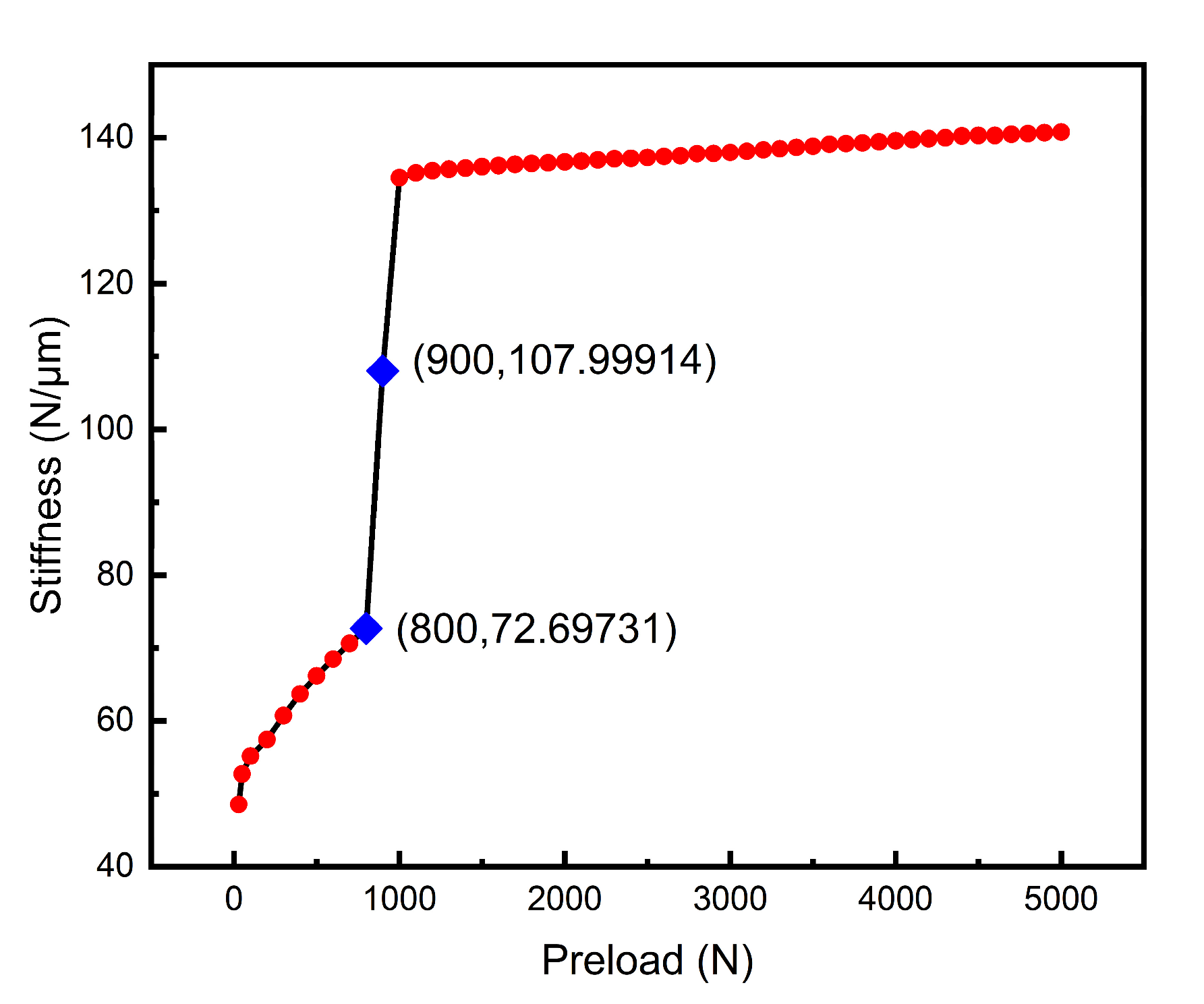
4.3. Effect of Preload on Mechanical Properties of Spindle
5. Conclusions
- (1)
- The new 2D axisymmetric simulation models of bearing and spindle were established by introducing a virtual rotating structure. The corresponding equivalent material parameters of virtual rotating structure were obtained via a parametric inversed method and the stickout measured result of bearing.
- (2)
- The relationship of the actual maximum contact stress between the roller and the inner/outer ring between the 2D and the 3D bearing model was deduced via the Hertz point and line contact theory of bearing; it can be used to calculate the contact stress of the bearing under different spindle structure and assembly.
- (3)
- The 2D simulation model established in this paper can be used to calculate the stiffness of the spindle and the contact stress of the bearing in the spindle under different bearing structure, spindle structure and spindle assembly parameters.
- (4)
- As the preload of the spindle increases, the axial stiffness of the spindle increases slowly at first, then increases rapidly in the case of the bearing ring being in contact with the spindle spacer sleeve and finally increases very slowly when the contact between the bearing ring and the spindle spacer sleeve is stable.
- (5)
- The maximum contact stress of the bearings’ inner and outer ring is positively correlated with the preload of the spindle. The preload shall be reduced as much as possible under the premise of ensuring that the spindle has a great rigidity to extend its service life.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Palmgren, A. Ball and Roller Bearing Engineering, 3rd ed.; Burbank Press: Philadelphia, PA, USA, 1959; pp. 1–12. [Google Scholar]
- Jones, A.B. Ball motion and sliding friction in ball bearings. J. Basic Eng. 1959, 81, 1–12. [Google Scholar] [CrossRef]
- Jones, A.B. A general theory for elastically constrained ball and radial roller bearings under arbitrary load and speed conditions. J. Basic Eng. 1960, 82, 309–320. [Google Scholar] [CrossRef]
- Wang, W.Z.; Hu, L.; Zhang, S.G.; Zhao, Z.Q.; Ai, S.Y. Modeling angular contact ball bearing without raceway control hypothesis. Mech. Mach. Theory 2014, 82, 154–172. [Google Scholar] [CrossRef]
- Gupta, P.K. Dynamics of rolling-element bearings—Part III: Ball bearing analysis. J. Lubr. Technol. 1979, 101, 312–318. [Google Scholar] [CrossRef]
- Gupta, P.K. Dynamics of rolling-element bearings—Part IV: Ball bearing results. J. Lubr. Technol. 1979, 101, 319–326. [Google Scholar] [CrossRef]
- Xi, S.T.; Cao, H.R.; Chen, X.F. Dynamic modeling of spindle bearing system and vibration response investigation. Mech. Syst. Signal Pr. 2019, 114, 486–511. [Google Scholar] [CrossRef]
- Cao, H.; Li, B.; Li, Y.; Kang, T.; Chen, X.F. Model-based error motion prediction and fit clearance optimization for machine tool spindles. Mech. Syst. Signal Pr. 2019, 133, 106252. [Google Scholar] [CrossRef]
- Liu, J.L.; Ma, C.; Wang, S.L. Thermal-structure interaction characteristics of a high-speed spindle bearing system. Int. J. Mach. Tool. Manu. 2019, 137, 42–57. [Google Scholar] [CrossRef]
- Yu, X.K.; Zhao, C.J.; Ge, S.D.; Huang, Q.X. Finite element analysis of axial loaded high speed angular contact ball bearings. Mech. Eng. Auto. 2011, 5, 51–55. [Google Scholar]
- Wang, H.; Dai, S. Study on contact finite element simulation of the high-angular contact thrust ball bearing. Comput. Simul. 2013, 30, 305–309. [Google Scholar]
- Zhang, J.H.; Fang, B.; Zhu, Y.S.; Hong, J. A comparative study and stiffness analysis of angular contact ball bearings under different preload mechanisms. Mech. Mach. Theory 2017, 115, 1–17. [Google Scholar] [CrossRef]
- Jiang, S.Y.; Mao, H.B. Investigation of variable optimum preload for a machine tool spindle. Int. J. Mach. Tool. Manu. 2010, 50, 19–28. [Google Scholar] [CrossRef]
- Li, B.; Zhang, J.B.; Jiang, L.S. A research of shaft modal simulation method based on the preloaded angular contact ball bearing. Appl. Mech. Mater. 2013, 389, 364–370. [Google Scholar] [CrossRef]
- Bian, Q.; Zeng, G.; Zhao, C.J.; Liu, B.Y.; Xiao, Z.G. Kinematics analysis of high-speed angular contact ball bearings based on finite element. J. Mech. Str. 2021, 43, 966–971. [Google Scholar]
- Liu, Y.Q.; Chen, Z.G.; Wang, K.Y.; Zhai, W.M. Non-uniform roller-race contact performance of bearings along width in the rotor-bearing system under dynamic loads. J. Sound Vib. 2022, 538, 117251. [Google Scholar] [CrossRef]
- Li, X.H.; Li, H.F.; Zhang, Y.F.; Hong, J. Investigation of non-uniform preload on the static and rotational performances for spindle bearing system. Int. J. Mach. Tool. Manu. 2016, 106, 11–21. [Google Scholar]
- Ozturk, E.; Kumar, U.; Schmitz, T. Investigation of spindle bearing preload on dynamics and stability limit in milling. CIRP Ann. Manuf. Techn. 2012, 61, 343–346. [Google Scholar] [CrossRef]
- Christian, B.; Stephan, N.; Marcus, G.; Leonardo, C.; Feliciano, G.; Guillermo, M.E.; Lang, D.F. New bearing steel for high-speed applications. MM Sci. J. 2021, 12, 5334–5339. [Google Scholar]
- Hertz, H. On the contact of elastic solids. J. Reine Angew. Math. 1881, 92, 156–171. [Google Scholar]
- Luo, J.W. Analysis and development of rolling bearing. Bearing 2001, 9, 28–31. [Google Scholar]
- Brewe, D.; Hamrock, B. Simplified solution for elliptical-contact deformation between two elastic solids. ASME Tran. J. Lub. Tech. 1977, 101, 231–239. [Google Scholar] [CrossRef]
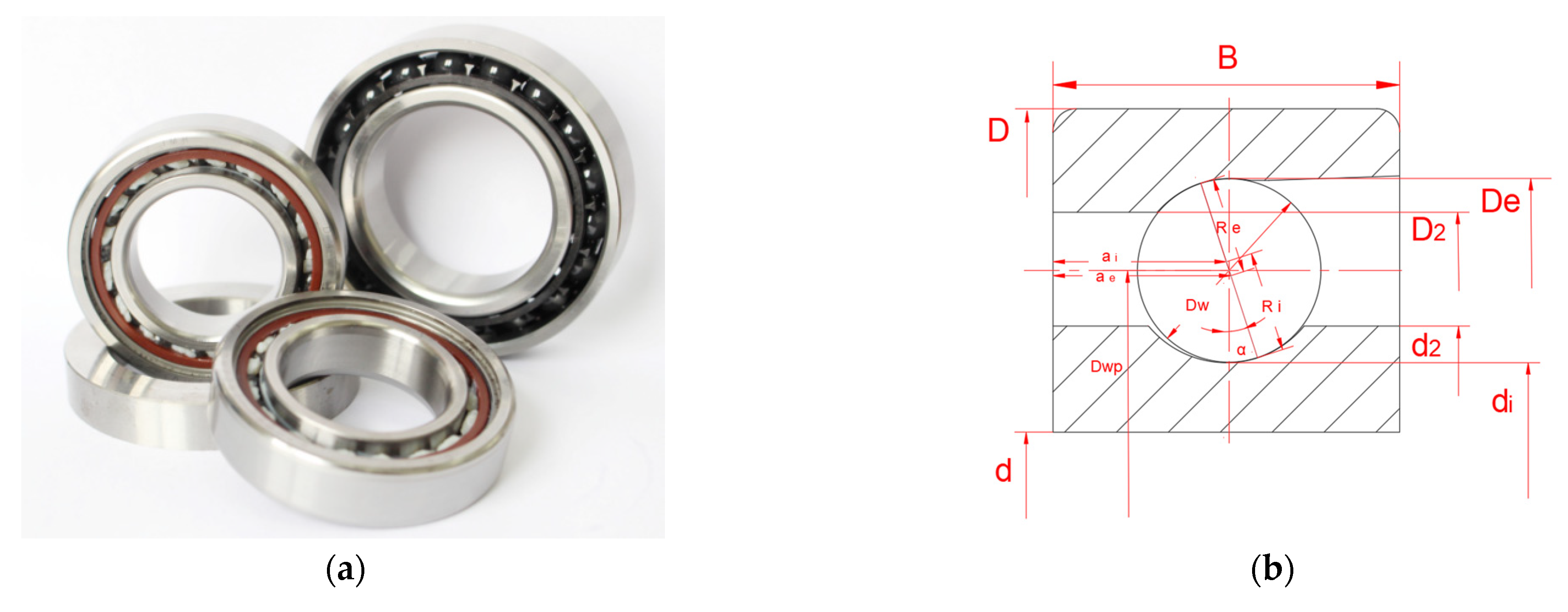


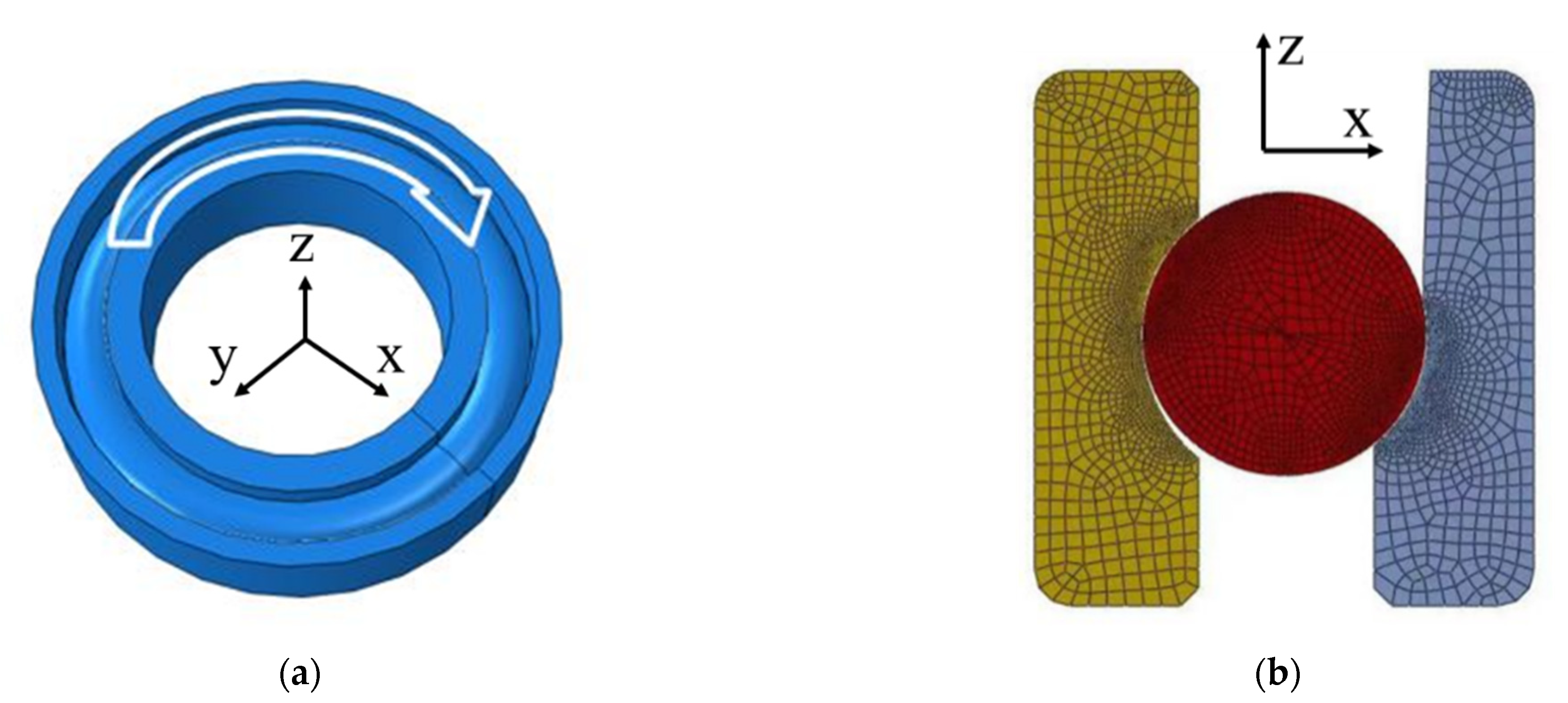
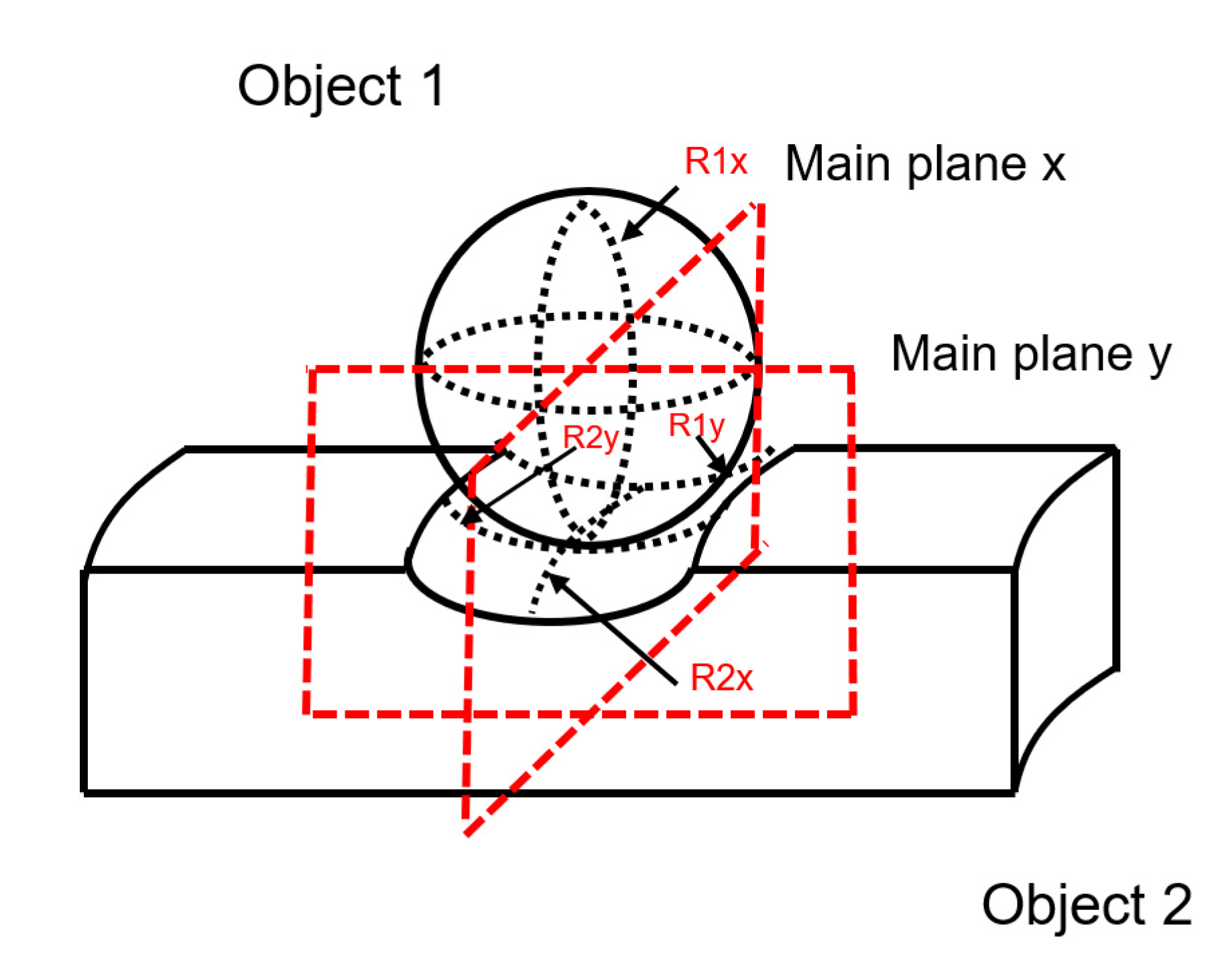



| Notation | Specification | Value |
|---|---|---|
| D | External diameter | 68 mm |
| d | Internal diameter | 40 mm |
| B | Width | 15 mm |
| Z | Number of rolling elements | 18 |
| Dw | Diameter of rolling elements | 7.938 mm |
| α | Contact angle | 18° |
| Dwp | Diameter of pitch circle | 54 mm |
| fe | External groove curvature | 0.53 |
| fi | Internal groove curvature | 0.55 |
| ai | External groove position | 7.696 mm |
| ae | Internal groove position | 7.5 mm |
| D2 | Diameter of the outer ring’s flange | 59.04 mm |
| d2 | Diameter of the inner ring’s flange | 49.19 mm |
| De | Diameter of the outer ring’s groove bottom circle | 61.961 mm |
| di | Diameter of the inner ring’s groove bottom circle | 46.023 mm |
| t | Height of the outer ring’s locking | 0.084 mm |
| Material | Elastic Modulus | Poisson’s Ratio | |
|---|---|---|---|
| Bearing ring | GCr15 | 208 GPa | 0.3 |
| Rolling element | Si3N4 | 310 GPa | 0.26 |
| Preload (N) | Number of the Test Bearing Samples | |||||||
|---|---|---|---|---|---|---|---|---|
| 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | |
| 30 | 19 | 18 | 22 | 23 | 15 | 12 | 30 | 28 |
| 60 | 22 | 20 | 23 | 24 | 16 | 13 | 31 | 30 |
| 90 | 23 | 21 | 24 | 24 | 17 | 14 | 32 | 31 |
| 120 | 24 | 22 | 25 | 25 | 18 | 16 | 34 | 32 |
| 150 | 25 | 23 | 25 | 26 | 19 | 17 | 35 | 34 |
| 180 | 26 | 24 | 26 | 28 | 20 | 18 | 36 | 35 |
| 210 | 27 | 25 | 28 | 29 | 21 | 19 | 37 | 36 |
| 270 | 29 | 27 | 30 | 31 | 24 | 22 | 39 | 38 |
| 330 | 30 | 29 | 31 | 32 | 25 | 23 | 40 | 39 |
| 360 | 31 | 29 | 32 | 33 | 26 | 24 | 41 | 39 |
| 390 | 32 | 30 | 32 | 34 | 27 | 24 | 42 | 40 |
| 420 | 32 | 30 | 33 | 34 | 27 | 24 | 43 | 41 |
| Component | Height | Thickness | Diameter |
|---|---|---|---|
| External sleeve | 330.98 mm | 5.5 mm | 40 mm (external) |
| Mandrel | 162.5 mm | 13.5 mm | 68 mm (internal) |
| Inner spacer sleeve | 90.5 mm | 5.5 mm | 40 mm (internal) |
| Outer spacer sleeve | 90.5 mm | 5.5 mm | 68 mm (external) |
| Lock nut | 24 mm | 5.5 mm | 40 mm (internal) |
| Type of Model | 3D Model | 2D Model |
|---|---|---|
| Number of nodes | 332,683 | 2085 |
| Number of elements | 228,831 | 1906 |
| Minimum size of meshes | 0.5 mm | 0.05 mm |
| Type of elements | C3D8R | CAX4R |
| Shape of elements | Tet | Quad-dominated |
| Technique | Free | Free |
| Algorithm | Default algorithm | Advancing front (default) |
| Component | Number of Mesh Elements | Type of Mesh | Minimum Mesh Size |
|---|---|---|---|
| External sleeve | 4403 | CAX4R | 0.2 mm |
| Mandrel | 2131 | CAX4RT | 1 mm |
| Inner spacer sleeve | 2157 | CAX4R | 0.2 mm |
| Outer spacer sleeve | 2242 | CAX4R | 0.2 mm |
| Lock nut | 305 | CAX4R | 0.2 mm |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xiu, S.; Li, J.; Chen, X.; Xia, Y.; Wang, P. Study on the 2D Equivalent Simulation Model of Bearing and Spindle for Precision Machine Tools. Machines 2023, 11, 461. https://doi.org/10.3390/machines11040461
Xiu S, Li J, Chen X, Xia Y, Wang P. Study on the 2D Equivalent Simulation Model of Bearing and Spindle for Precision Machine Tools. Machines. 2023; 11(4):461. https://doi.org/10.3390/machines11040461
Chicago/Turabian StyleXiu, Shijun, Jibo Li, Xiangjun Chen, Yifan Xia, and Pei Wang. 2023. "Study on the 2D Equivalent Simulation Model of Bearing and Spindle for Precision Machine Tools" Machines 11, no. 4: 461. https://doi.org/10.3390/machines11040461
APA StyleXiu, S., Li, J., Chen, X., Xia, Y., & Wang, P. (2023). Study on the 2D Equivalent Simulation Model of Bearing and Spindle for Precision Machine Tools. Machines, 11(4), 461. https://doi.org/10.3390/machines11040461





