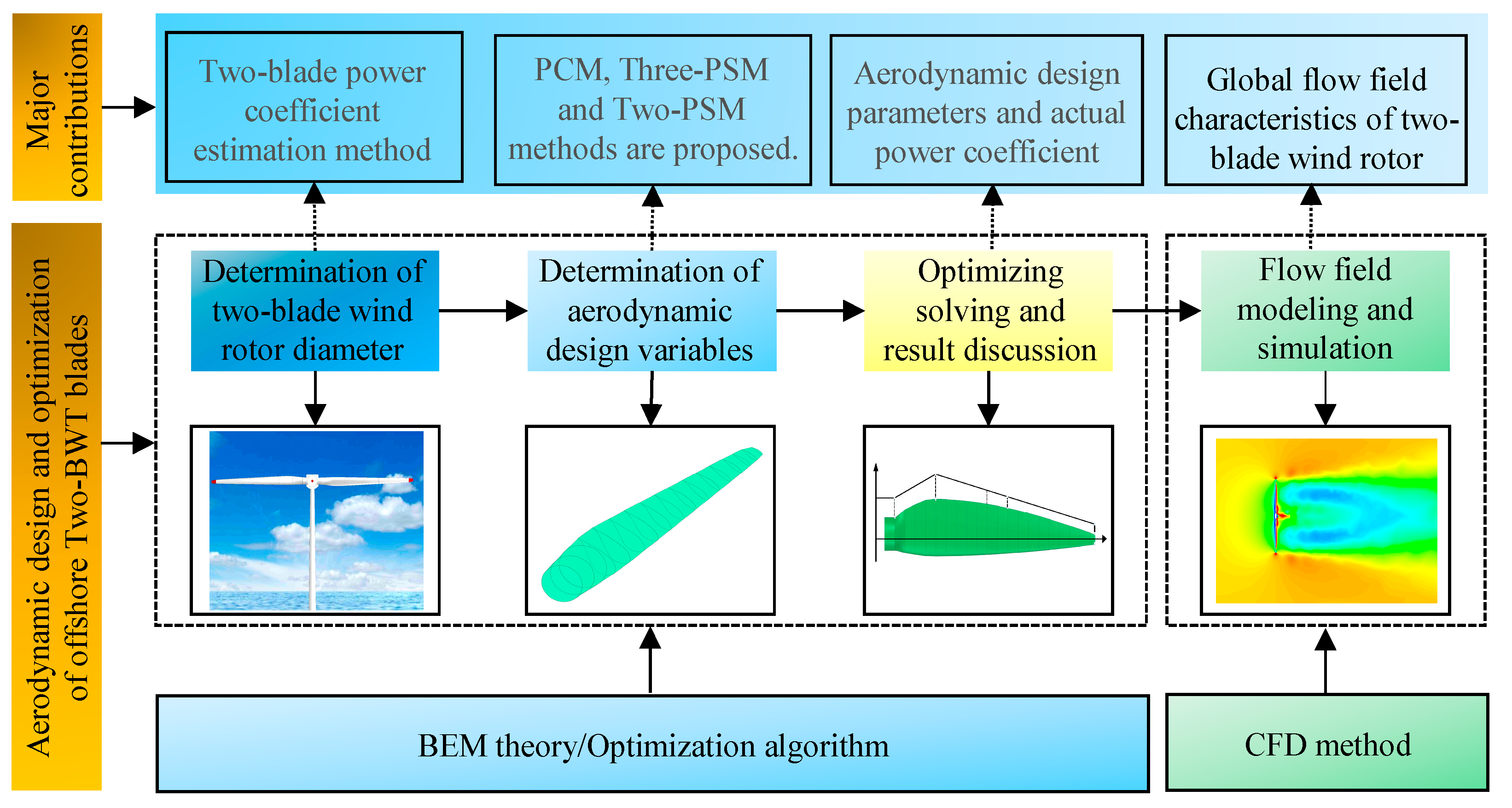
New Two-BWT Blade Aerodynamic Design and CFD Simulation
Abstract
1. Introduction
- An aerodynamic design method for novel offshore Two-BWT blades is presented.
- The design variable control models (PCM, Three-PSM, and Two-PSM) are adopted.
- The wind speed distribution and blade pressure distribution in the Two-BWT flow field are investigated.
2. Determination of Two-Blade Wind Rotor Diameter
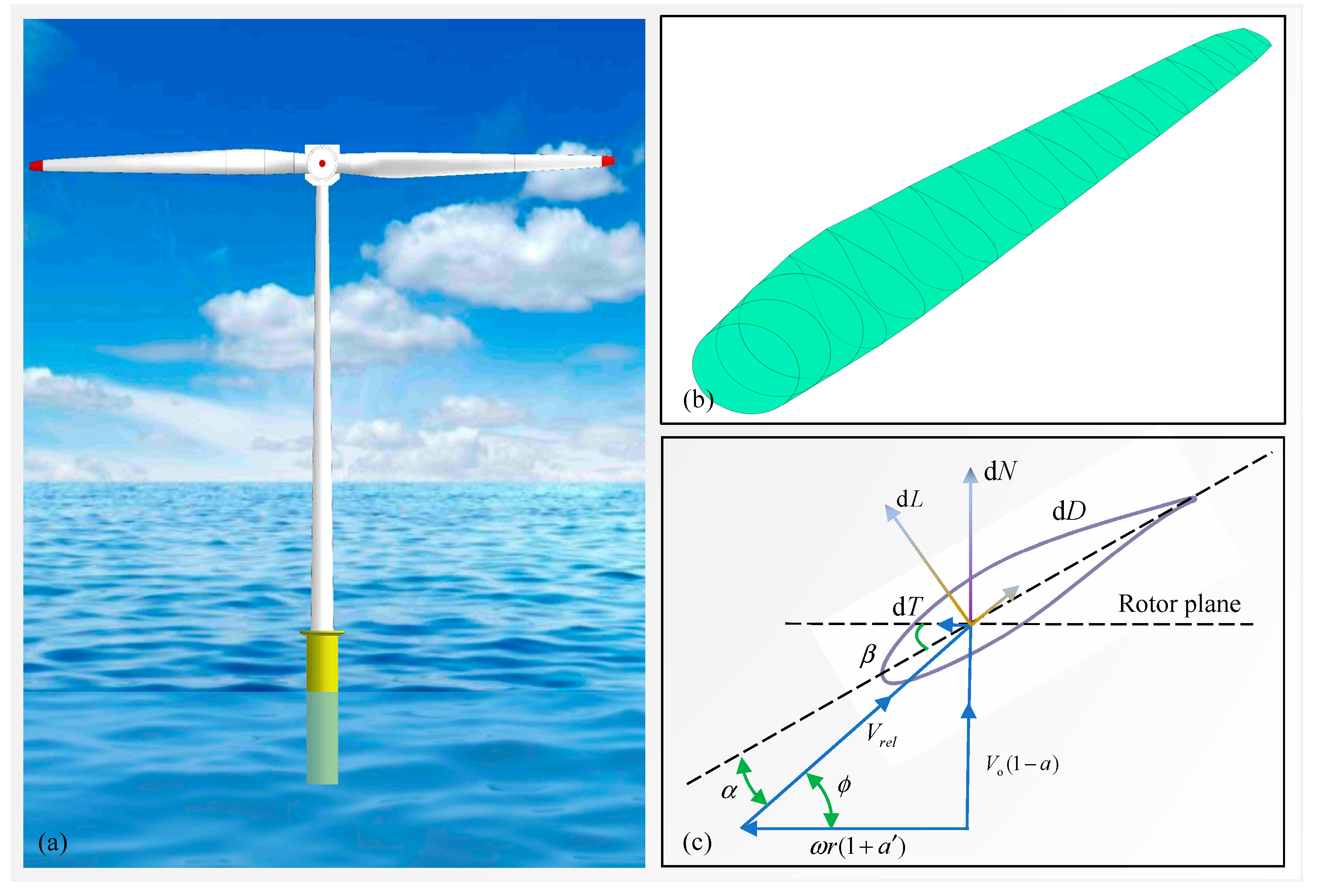
2.1. Blade Aerodynamic Modeling
2.2. Estimation of the Two-BWT Rotor Diameter
3. Determination of Aerodynamic Design Variables
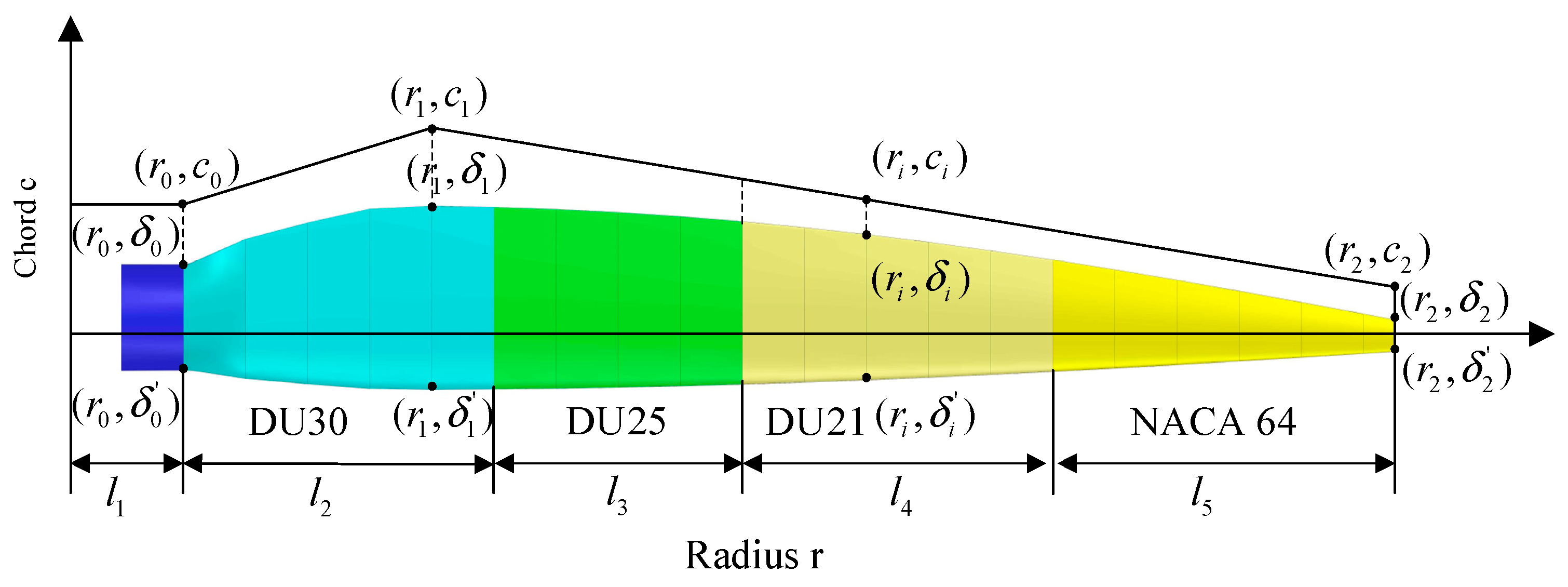
3.1. Airfoil Distribution Variables
3.2. Chord Length Distribution Variables
3.3. Twist Angle Distribution Variables
4. Optimizing Solving and Result Discussion
4.1. Design Objective and Solutions
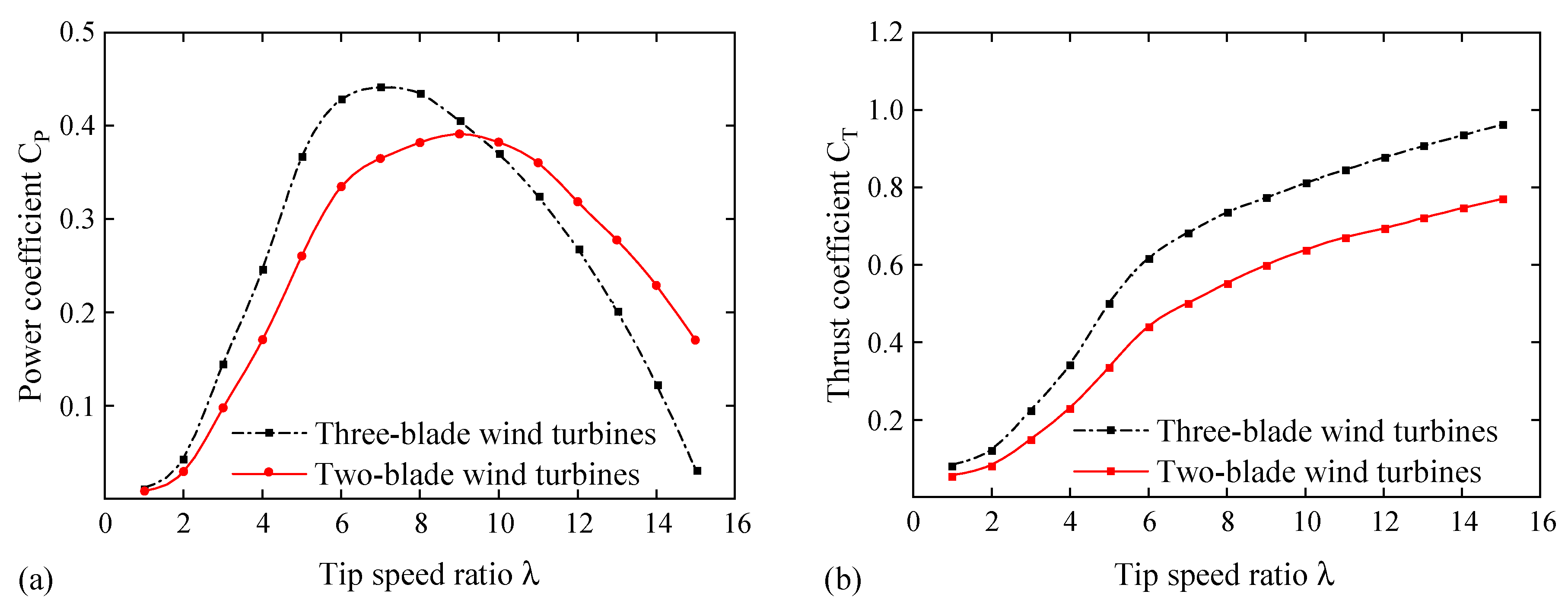
4.2. Result Discussion
5. Flow Field Modeling and Simulation
5.1. Modeling and Flow Field Setup
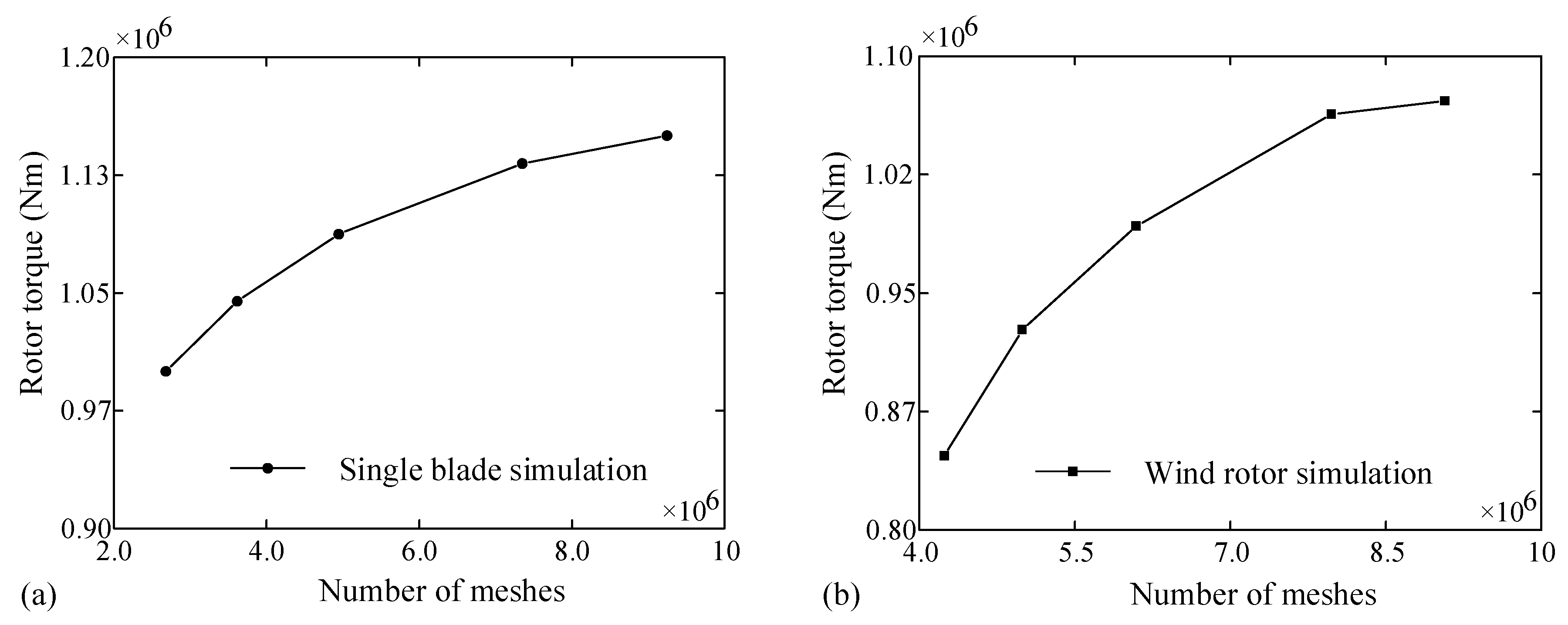
5.2. Mesh Sensitivity Analysis
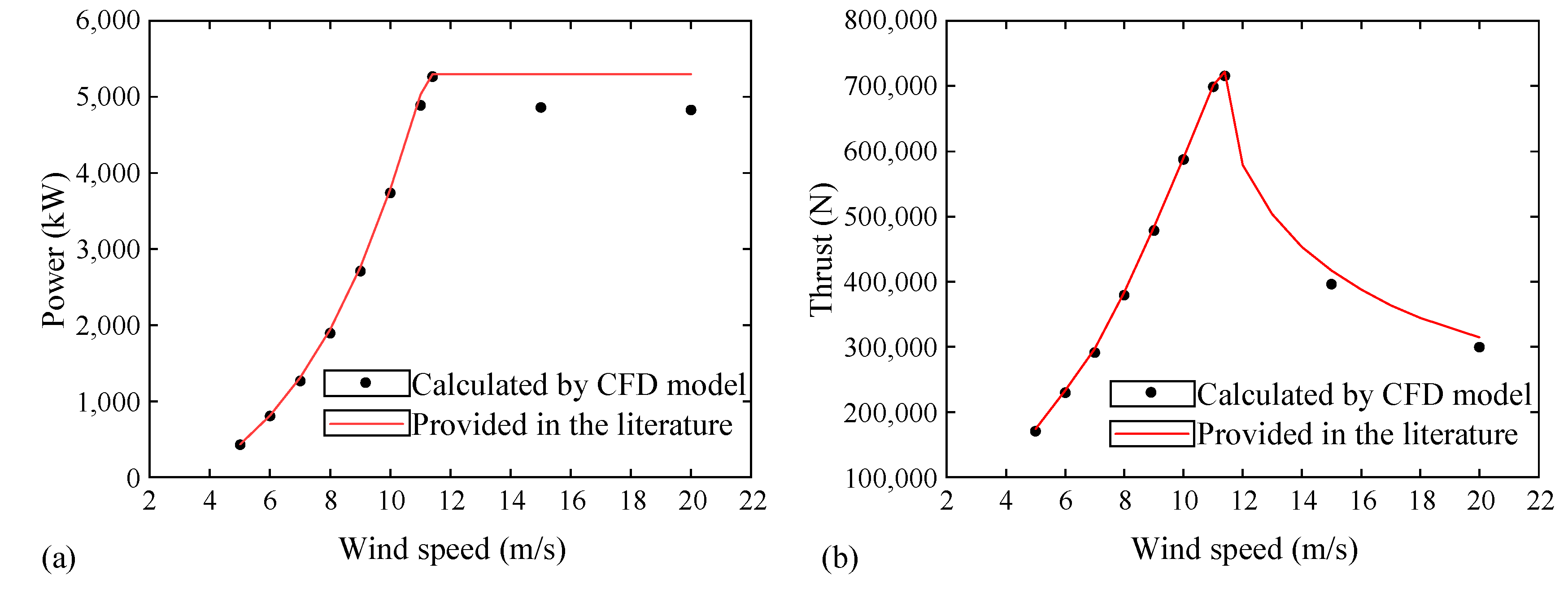
5.3. Model Feasibility Verification
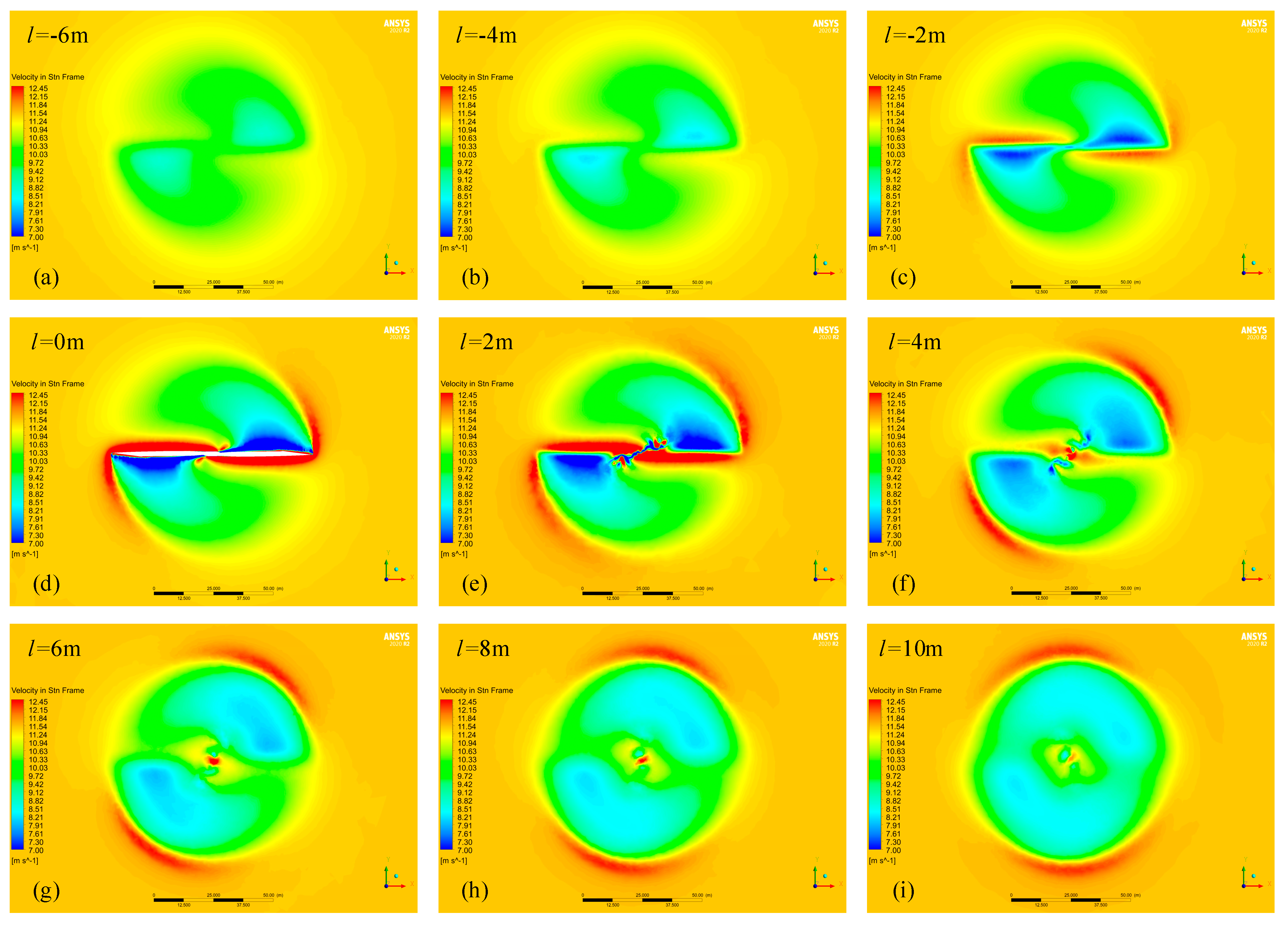
5.4. Simulation Results and Discussion
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Salvador, C.B.; Arzaghi, E.; Yazdi, M.; Jahromi, H.A.F.; Abbassi, R. A multi-criteria decision-making framework for site selection of offshore wind farms in Australia. Ocean Coast. Manag. 2022, 224, 106196. [Google Scholar] [CrossRef]
- Dai, J.; Yang, X.; Wen, L. Development of wind power industry in China: A comprehensive assessment. Renew. Sustain. Energy Rev. 2018, 97, 156–164. [Google Scholar] [CrossRef]
- Dai, J.; Hu, W.; Yang, X.; Yang, S. Modeling and investigation of load and motion characteristics of offshore floating wind turbines. Ocean Eng. 2018, 159, 187–200. [Google Scholar] [CrossRef]
- Çiftci, C.; Erdoğan, A.; Genç, M.S. Investigation of the mechanical behavior of a new generation wind turbine blade technology. Energies 2023, 16, 1961. [Google Scholar] [CrossRef]
- Chudzik, S. Wind Microturbine with Adjustable Blade Pitch Angle. Energies 2023, 16, 945. [Google Scholar] [CrossRef]
- Martynowicz, P. Experimental study on the optimal-based vibration control of a wind turbine tower using a small-scale electric drive with MR damper support. Energies 2022, 15, 9530. [Google Scholar] [CrossRef]
- Hou, Z.; Lv, X.; Zhuang, S. Optimized extreme learning machine-based main bearing temperature monitoring considering ambient conditions’ effects. Energies 2021, 14, 7529. [Google Scholar] [CrossRef]
- Qin, Z.; Qiang, S.; Zhang, M.; Rong, X.; Liao, C.; Wang, J.; Xu, J. Design and structural responses of a 38-meter sectional wind turbine blade under extreme static loads. Compos. Struct. 2022, 290, 115487. [Google Scholar] [CrossRef]
- Serafeim, G.P.; Manolas, D.I.; Riziotis, V.A.; Chaviaropoulos, P.K.; Saravanos, D.A. Optimized blade mass reduction of a 10MW-scale wind turbine via combined application of passive control techniques based on flap-edge and bend-twist coupling effects. J. Wind Eng. Ind. Aerodyn. 2022, 225, 105002. [Google Scholar] [CrossRef]
- Bagherpoor, T.; Xuemin, L. Structural optimization design of 2MW composite wind turbine blade. Energy Procedia 2017, 105, 1226–1233. [Google Scholar] [CrossRef]
- Vesel, R.W.; McNamara, J.J. Performance enhancement and load reduction of a 5 MW wind turbine blade. Renew. Energy 2014, 66, 391–401. [Google Scholar] [CrossRef]
- Damiano, M.; Russo, A.; Sellitto, A.; Vecchio, E.; Stellato, T.; Riccio, A. Design of a composite wind turbine blade manufactured with the ONE SHOT BLADE® technology. Mater. Today: Proc. 2021, 34, 103–105. [Google Scholar] [CrossRef]
- Juchuan, D.; Shanghong, Z.; Xiyun, Y.; Deshun, L.; Zejun, W. Design and optimizaiton of aerodynamic shape and operating characteristics of large scale wind turbine blade. J. Mech. Eng. 2015, 51, 138–145. [Google Scholar]
- Pinto, R.L.U.d.F.; Gonçalves, B.P.F. A revised theoretical analysis of aerodynamic optimization of horizontal-axis wind turbines based on BEM theory. Renew. Energy 2017, 105, 625–636. [Google Scholar] [CrossRef]
- Bavanish, B.; Thyagarajan, K. Optimization of power coefficient on a horizontal axis wind turbine using bem theory. Renew. Sustain. Energy Rev. 2013, 26, 169–182. [Google Scholar] [CrossRef]
- Laalej, S.; Bouatem, A.; AlMers, A.; El Maani, R. Wind turbine performances prediction using BEM approach with Jonkman-Buhl brake state model coupled to CFD method. Mater. Today Proc. 2022, 65, 3829–3838. [Google Scholar] [CrossRef]
- Esfahanian, V.; Salavati Pour, A.; Harsini, I.; Haghani, A.; Pasandeh, R.; Shahbazi, A.; Ahmadi, G. Numerical analysis of flow field around NREL Phase II wind turbine by a hybrid CFD/BEM method. J. Wind Eng. Ind. Aerodyn. 2013, 120, 29–36. [Google Scholar] [CrossRef]
- Avvad, M.; Vishwanath, K.C.; Kaladgi, A.R.; Muneer, R.; Kareemullah, M.; Navaneeth, I.M. Performance analysis of aerofoil blades at different pitch angles and wind speeds. Mater. Today Proc. 2021, 47, 6249–6256. [Google Scholar] [CrossRef]
- Huang, S.; Qiu, H.; Wang, Y. Aerodynamic performance of horizontal axis wind turbine with application of dolphin head-shape and lever movement of skeleton bionic airfoils. Energy Convers. Manag. 2022, 267, 115803. [Google Scholar] [CrossRef]
- Yen, S.-C.; Liu, W.-S.; San, K.-C.; Wang, W.-F. Design of wind-turbine blades for improving aerodynamic performance using hybrid blades. Ocean Eng. 2021, 227, 108889. [Google Scholar] [CrossRef]
- Alkhabbaz, A.; Yang, H.-S.; Weerakoon, A.H.S.; Lee, Y.-H. A novel linearization approach of chord and twist angle distribution for 10 kW horizontal axis wind turbine. Renew. Energy 2021, 178, 1398–1420. [Google Scholar] [CrossRef]
- Jia, L.; Hao, J.; Hall, J.; Nejadkhaki, H.K.; Wang, G.; Yan, Y.; Sun, M. A reinforcement learning based blade twist angle distribution searching method for optimizing wind turbine energy power. Energy 2021, 215, 119148. [Google Scholar] [CrossRef]
- Tahani, M.; Kavari, G.; Masdari, M.; Mirhosseini, M. Aerodynamic design of horizontal axis wind turbine with innovative local linearization of chord and twist distributions. Energy 2017, 131, 78–91. [Google Scholar] [CrossRef]
- Tahani, M.; Kavari, G.; Mirhosseini, M.; Ghiyasi, S. Different functionalized chord and twist distributions in aerodynamic design of HAWTs. Environ. Prog. Sustain. Energy 2019, 38, 13108. [Google Scholar] [CrossRef]
- Rahgozar, S.; Pourrajabian, A.; Kazmi, S.A.A.; Kazmi, S.M.R. Performance analysis of a small horizontal axis wind turbine under the use of linear/nonlinear distributions for the chord and twist angle. Energy Sustain. Dev. 2020, 58, 42–49. [Google Scholar] [CrossRef]
- Pourrajabian, A.; Dehghan, M.; Rahgozar, S. Genetic algorithms for the design and optimization of horizontal axis wind turbine (HAWT) blades: A continuous approach or a binary one? Sustain. Energy Technol. Assess. 2021, 44, 101022. [Google Scholar] [CrossRef]
- Sessarego, M.; Feng, J.; Ramos-García, N.; Horcas, S.G. Design optimization of a curved wind turbine blade using neural networks and an aero-elastic vortex method under turbulent inflow. Renew. Energy 2020, 146, 1524–1535. [Google Scholar] [CrossRef]
- Lanzafame, R.; Messina, M. Fluid dynamics wind turbine design: Critical analysis, optimization and application of BEM theory. Renew. Energy 2007, 32, 2291–2305. [Google Scholar] [CrossRef]
- Dai, J.C.; Hu, Y.P.; Liu, D.S.; Long, X. Aerodynamic loads calculation and analysis for large scale wind turbine based on combining BEM modified theory with dynamic stall model. Renew. Energy 2011, 36, 1095–1104. [Google Scholar] [CrossRef]
- Jonkman, J.; Butterfield, S.; Musial, W.; Scott, G. Definition of a 5-MW Reference Wind Turbine for Offshore System Development; National Renewable Energy Lab.(NREL): Golden, CO, USA, 2009.
- Moriarty, P.; Hansen, A. Aerodyn Theory Manual; NREL/TP-500-36881; National Renewable Energy Laboratory: Golden, CO, USA, 2005.
- Wang, L.; Quant, R.; Kolios, A. Fluid structure interaction modelling of horizontal-axis wind turbine blades based on CFD and FEA. J. Wind Eng. Ind. Aerodyn. 2016, 158, 11–25. [Google Scholar] [CrossRef]
- Ji, B.; Zhong, K.; Xiong, Q.; Qiu, P.; Zhang, X.; Wang, L. CFD simulations of aerodynamic characteristics for the three-blade NREL Phase VI wind turbine model. Energy 2022, 249, 123670. [Google Scholar] [CrossRef]
- Eltayesh, A.; Castellani, F.; Burlando, M.; Hanna, M.B.; Huzayyin, A.; El-Batsh, H.M.; Becchetti, M. Experimental and numerical investigation of the effect of blade number on the aerodynamic performance of a small-scale horizontal axis wind turbine. Alex. Eng. J. 2021, 60, 3931–3944. [Google Scholar] [CrossRef]

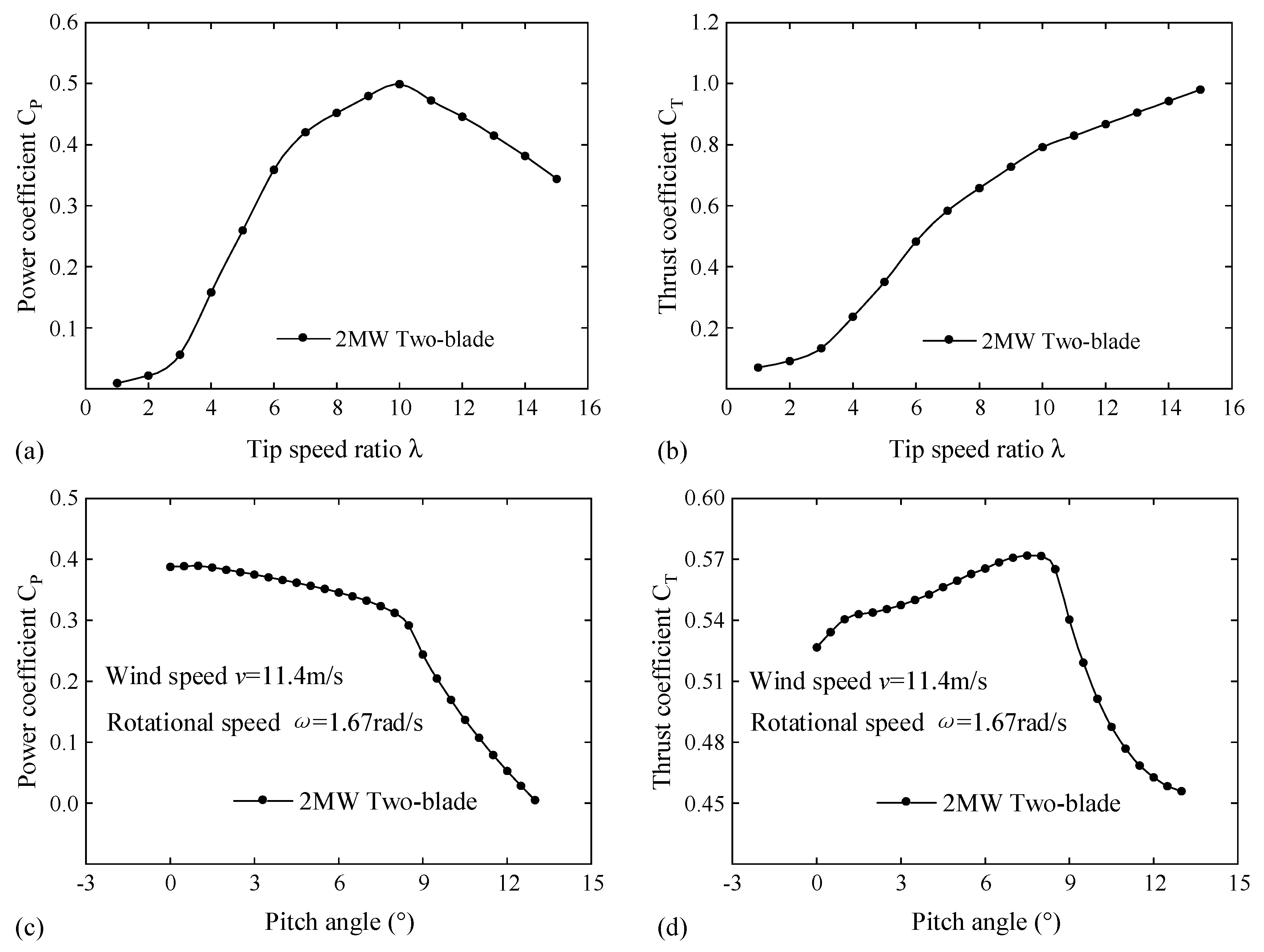

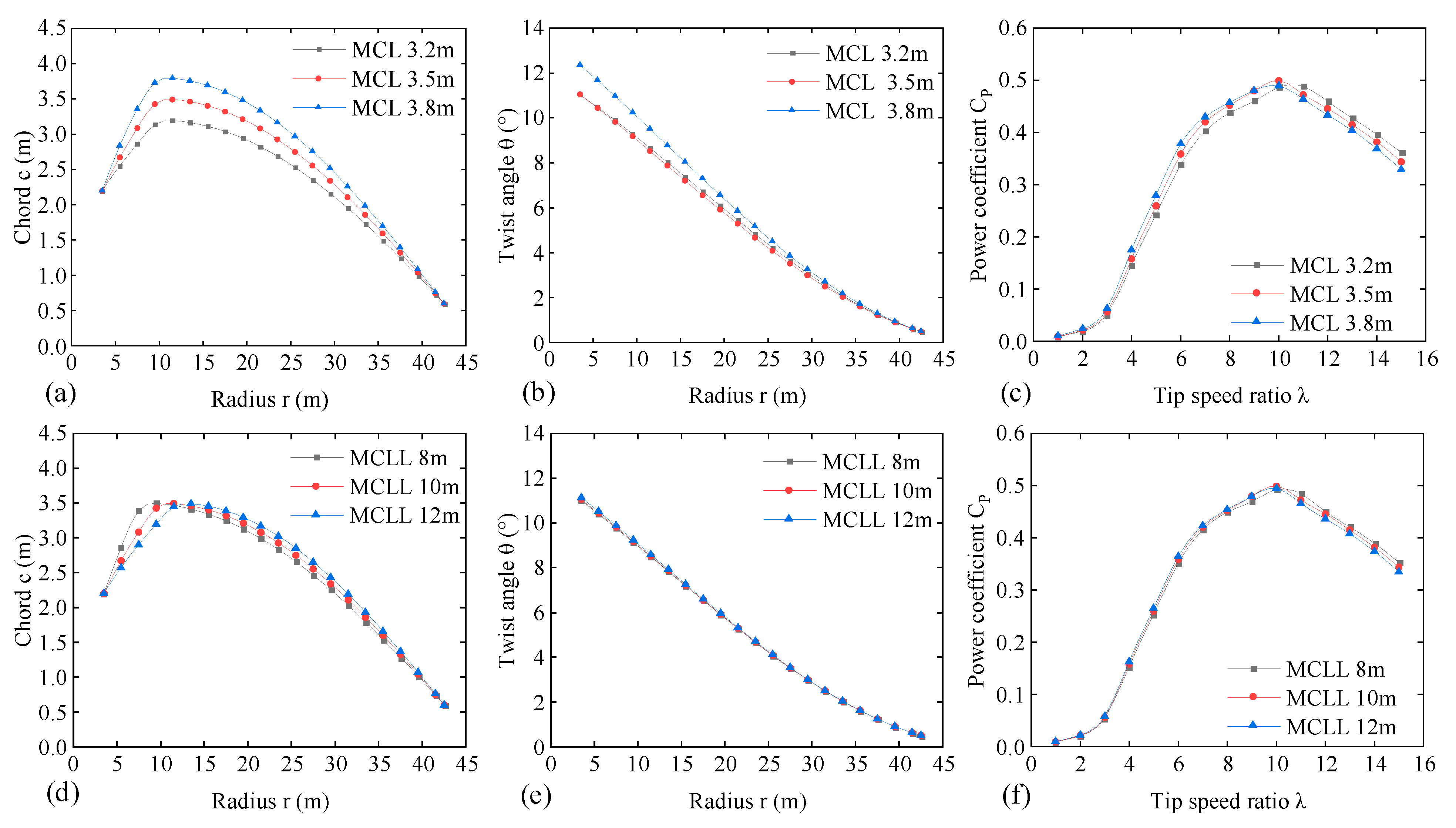
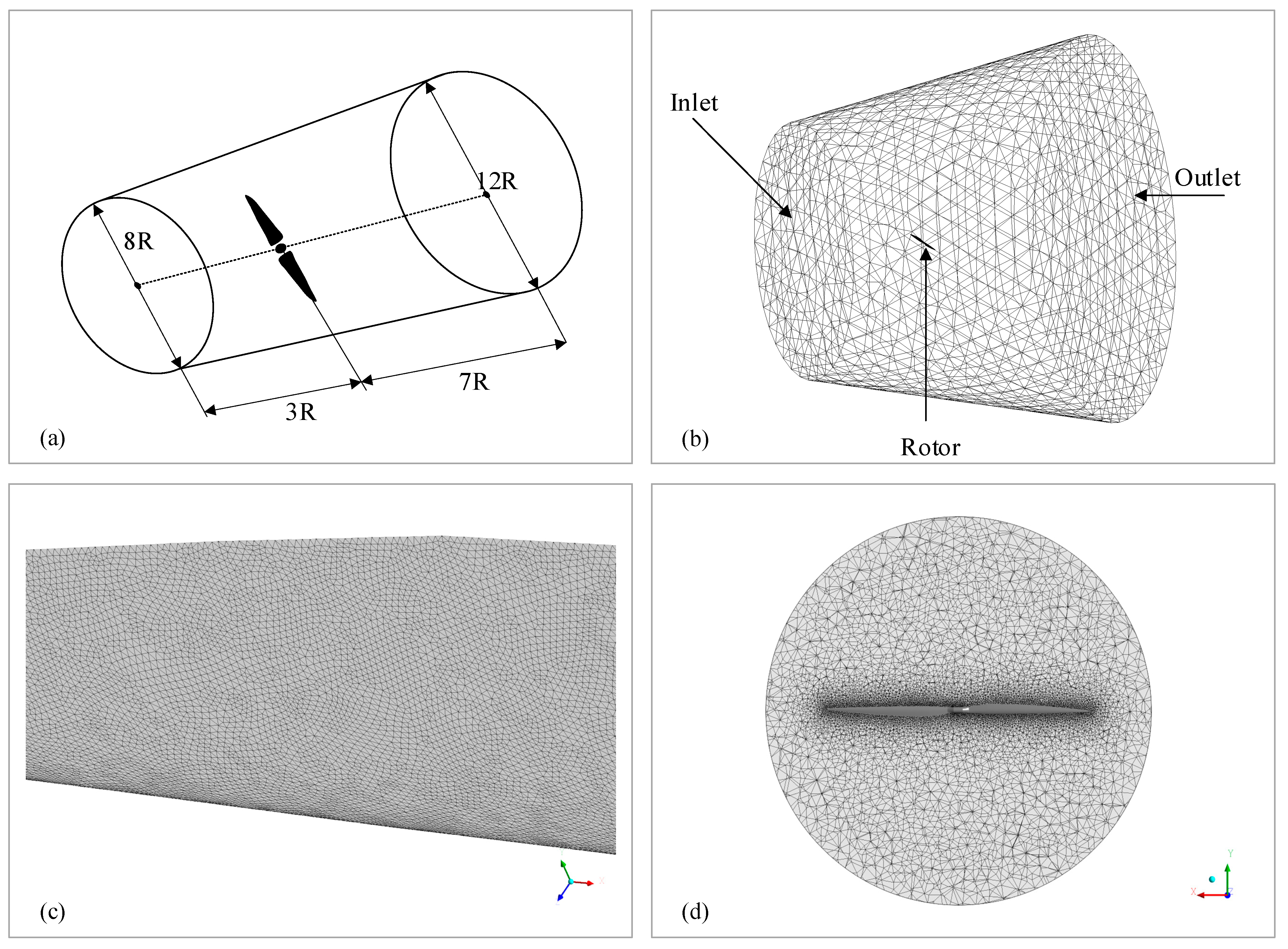
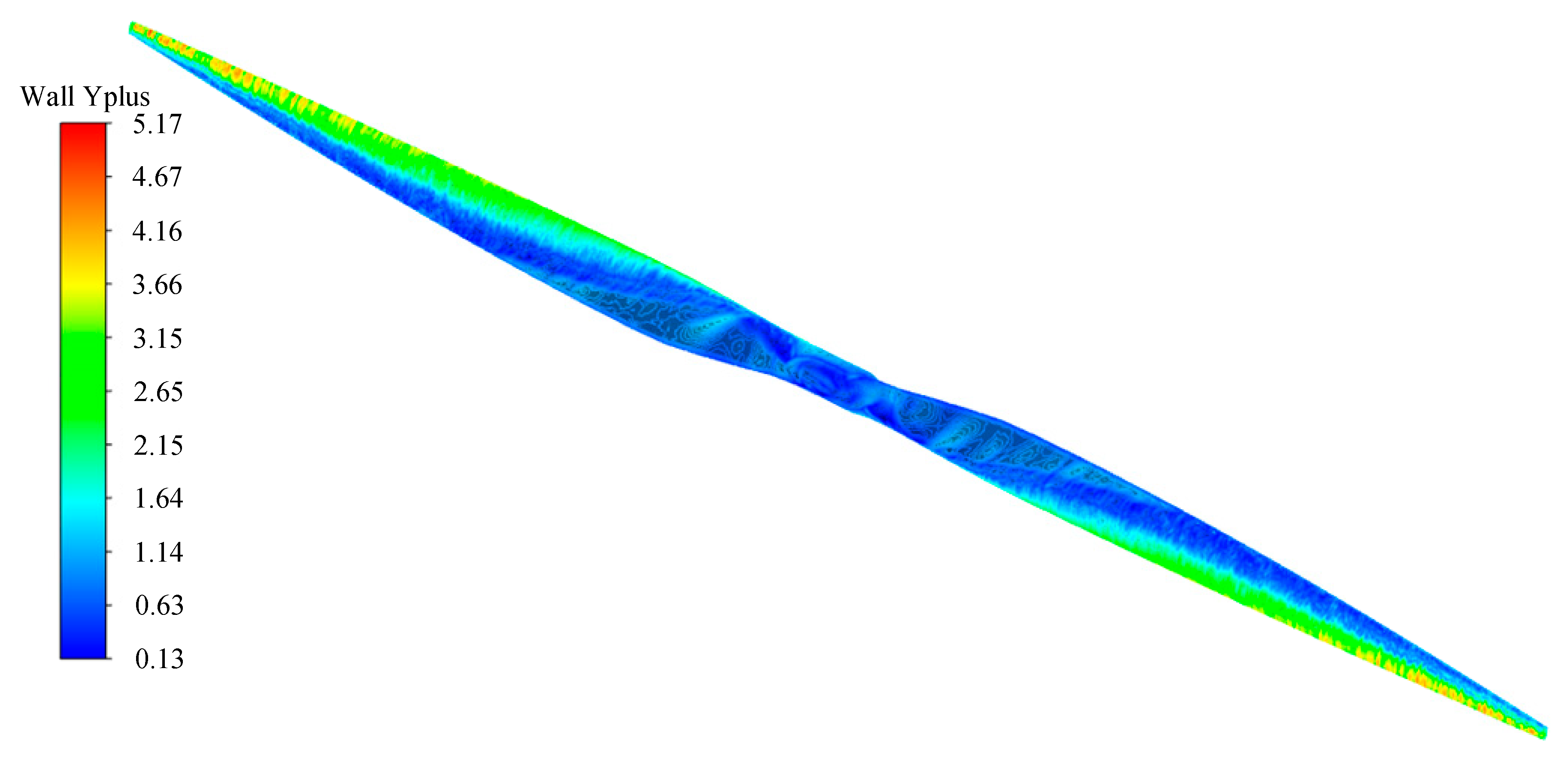


| Node | RNodes/m | Twist Angle/° | Chord/m | Airfoil |
|---|---|---|---|---|
| 1 | 2.8667 | 13.308 | 3.542 | Cylinder |
| 2 | 5.6000 | 13.308 | 3.854 | Cylinder |
| 3 | 8.3333 | 13.308 | 4.167 | Cylinder |
| 4 | 11.7500 | 13.308 | 4.557 | DU40_A17 |
| 5 | 15.8500 | 11.480 | 4.652 | DU35_A17 |
| 6 | 19.9500 | 10.162 | 4.458 | DU35_A17 |
| 7 | 24.0500 | 9.011 | 4.249 | DU30_A17 |
| 8 | 28.1500 | 7.795 | 4.007 | DU25_A17 |
| 9 | 32.2500 | 6.544 | 3.748 | DU25_A17 |
| 10 | 36.3500 | 5.361 | 3.502 | DU21_A17 |
| 11 | 40.4500 | 4.188 | 3.256 | DU21_A17 |
| 12 | 44.5500 | 3.125 | 3.010 | NACA64_A17 |
| … | … | … | … | … |
| 17 | 61.6333 | 0.106 | 1.419 | NACA64_A17 |
| Spanwise Length/m | Twist Angle/° | Chord Length/m | Airfoil |
|---|---|---|---|
| 3.500 | 11.06 | 2.200 | DU30 |
| 5.500 | 10.45 | 2.674 | DU25 |
| 7.500 | 9.82 | 3.086 | DU25 |
| 9.500 | 9.18 | 3.427 | DU21 |
| 11.500 | 8.53 | 3.493 | DU21 |
| 13.500 | 7.87 | 3.460 | DU21 |
| 15.500 | 7.22 | 3.402 | DU21 |
| 17.500 | 6.56 | 3.319 | DU21 |
| 19.500 | 5.92 | 3.212 | DU21 |
| 21.500 | 5.29 | 3.080 | DU21 |
| 23.500 | 4.68 | 2.926 | NACA64 |
| 25.500 | 4.09 | 2.750 | NACA64 |
| 27.500 | 3.53 | 2.554 | NACA64 |
| 29.500 | 2.99 | 2.338 | NACA64 |
| 31.500 | 2.49 | 2.106 | NACA64 |
| 33.500 | 2.03 | 1.858 | NACA64 |
| 35.500 | 1.61 | 1.596 | NACA64 |
| 37.500 | 1.23 | 1.322 | NACA64 |
| 39.500 | 0.90 | 1.039 | NACA64 |
| 41.500 | 0.62 | 0.748 | NACA64 |
| 42.500 | 0.50 | 0.600 | NACA64 |
| (m) | (m) | (°) | (rad/s) | (m) | (m) | (m) | |||
|---|---|---|---|---|---|---|---|---|---|
| 10 | 3.2 | 3.800 | 3.2 | 7.000 | 11.04 | 1.75 | 2 | 4 | 19.95 |
| 3.5 | 3.966 | 3.5 | 7.820 | 11.06 | 1.67 | ||||
| 3.8 | 4.000 | 3.8 | 8.600 | 12.36 | 1.61 | ||||
| 8 | 3.5 | 3.966 | 3.5 | 7.787 | 11.01 | 1.70 | |||
| 10 | 3.966 | 3.5 | 7.820 | 11.04 | 1.67 | ||||
| 12 | 3.966 | 3.5 | 7.872 | 11.13 | 1.64 |
| Mesh Size | Blade Simulation | Wind Rotor Simulation | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| 0.035 m | 0.04 m | 0.05 m | 0.06 m | 0.07 m | 0.055 m | 0.06 m | 0.07 m | 0.08 m | 0.09 m | |
| Rotor torque (Nm) | 1,150,238 | 1,132,342 | 1,087,524 | 1,044,600 | 1,000,030 | 1,071,540 | 1,063,252 | 992,447 | 926,710 | 846,790 |
| Number of meshes | 9,246,797 | 7,349,406 | 4,944,918 | 3,611,421 | 2,677,254 | 9,069,465 | 7,974,692 | 6,090,860 | 4,991,385 | 4,243,162 |
| Wind Speed (m/s) | Rotational Speed of the Wind Rotor (rad/s) | Power (kW) | Deviation (%) | |
|---|---|---|---|---|
| Theoretically Calculated Value Based on BEM | Values Based on CFD Simulations | |||
| 10.2 | 1.67 | 1565 | 1515 | 3.2 |
| 10.6 | 1.67 | 1710 | 1633 | 4.5 |
| 11.0 | 1.67 | 1855 | 1762 | 5.0 |
| 11.4 | 1.67 | 2000 | 1891 | 5.5 |
| 11.8 | 1.67 | 2120 | 2012 | 5.1 |
| 12.2 | 1.67 | 2235 | 2129 | 4.7 |
| 12.6 | 1.67 | 2336 | 2256 | 3.4 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, G.; Dai, J.; Zhang, F.; Zuo, C. New Two-BWT Blade Aerodynamic Design and CFD Simulation. Machines 2023, 11, 399. https://doi.org/10.3390/machines11030399
Li G, Dai J, Zhang F, Zuo C. New Two-BWT Blade Aerodynamic Design and CFD Simulation. Machines. 2023; 11(3):399. https://doi.org/10.3390/machines11030399
Chicago/Turabian StyleLi, Guo, Juchuan Dai, Fan Zhang, and Chengming Zuo. 2023. "New Two-BWT Blade Aerodynamic Design and CFD Simulation" Machines 11, no. 3: 399. https://doi.org/10.3390/machines11030399
APA StyleLi, G., Dai, J., Zhang, F., & Zuo, C. (2023). New Two-BWT Blade Aerodynamic Design and CFD Simulation. Machines, 11(3), 399. https://doi.org/10.3390/machines11030399





