Structural Design and Theoretical Analysis of Jetting Spin Type Lotus Root Digging Machine Based on CFD
Abstract
:1. Introduction
2. Materials and Methods
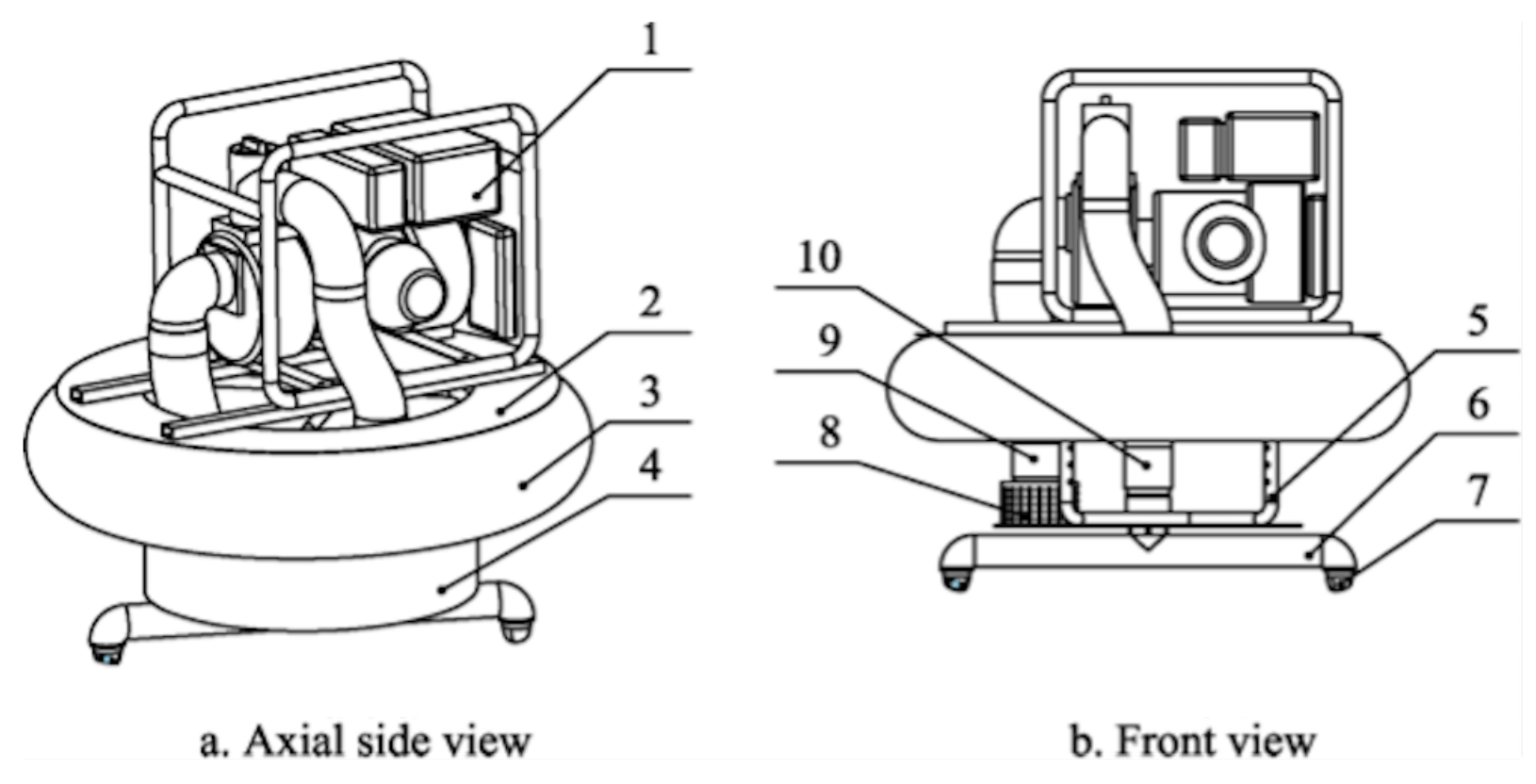
2.1. Machine Structure Design
2.2. Rotating Pipe Structure
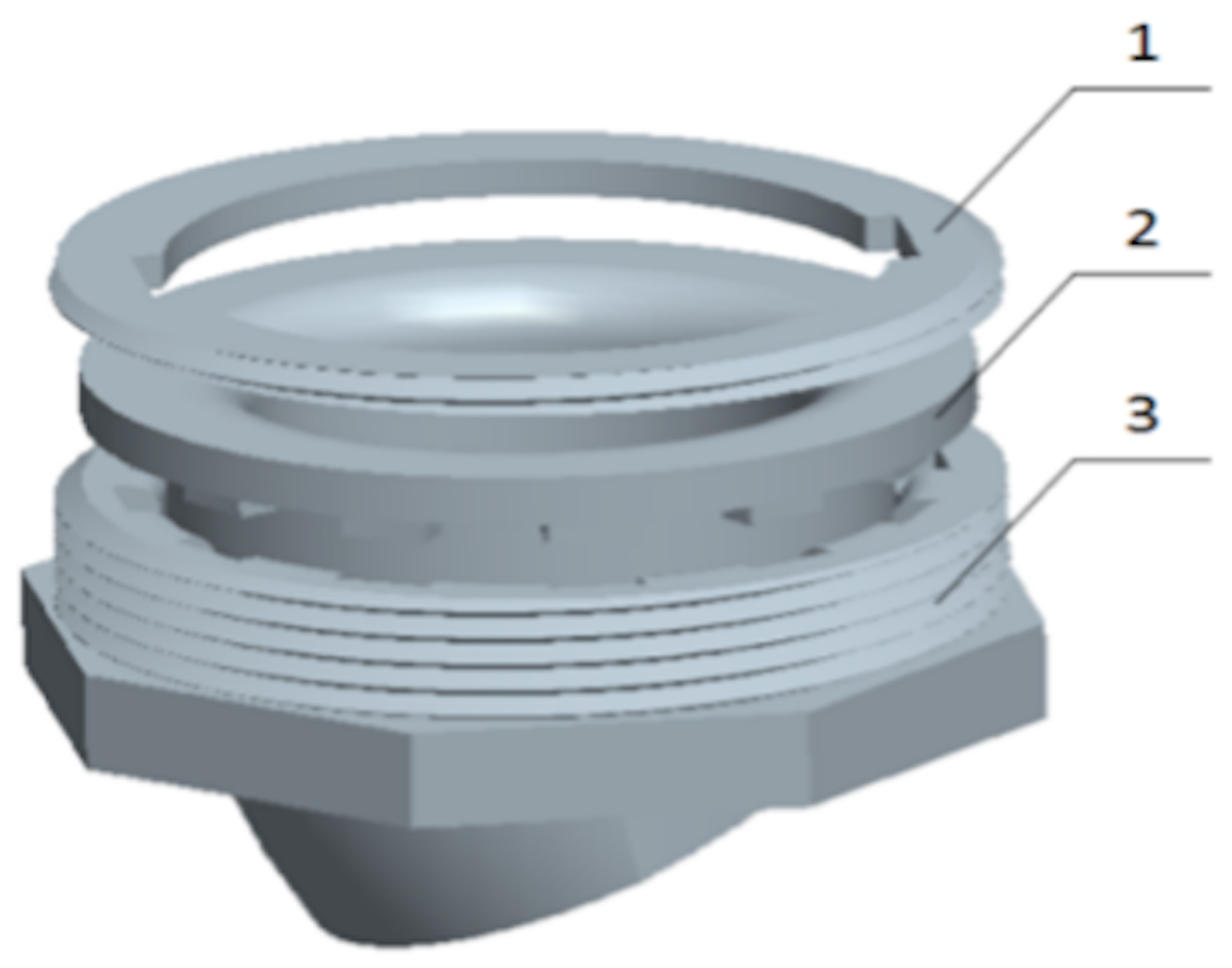
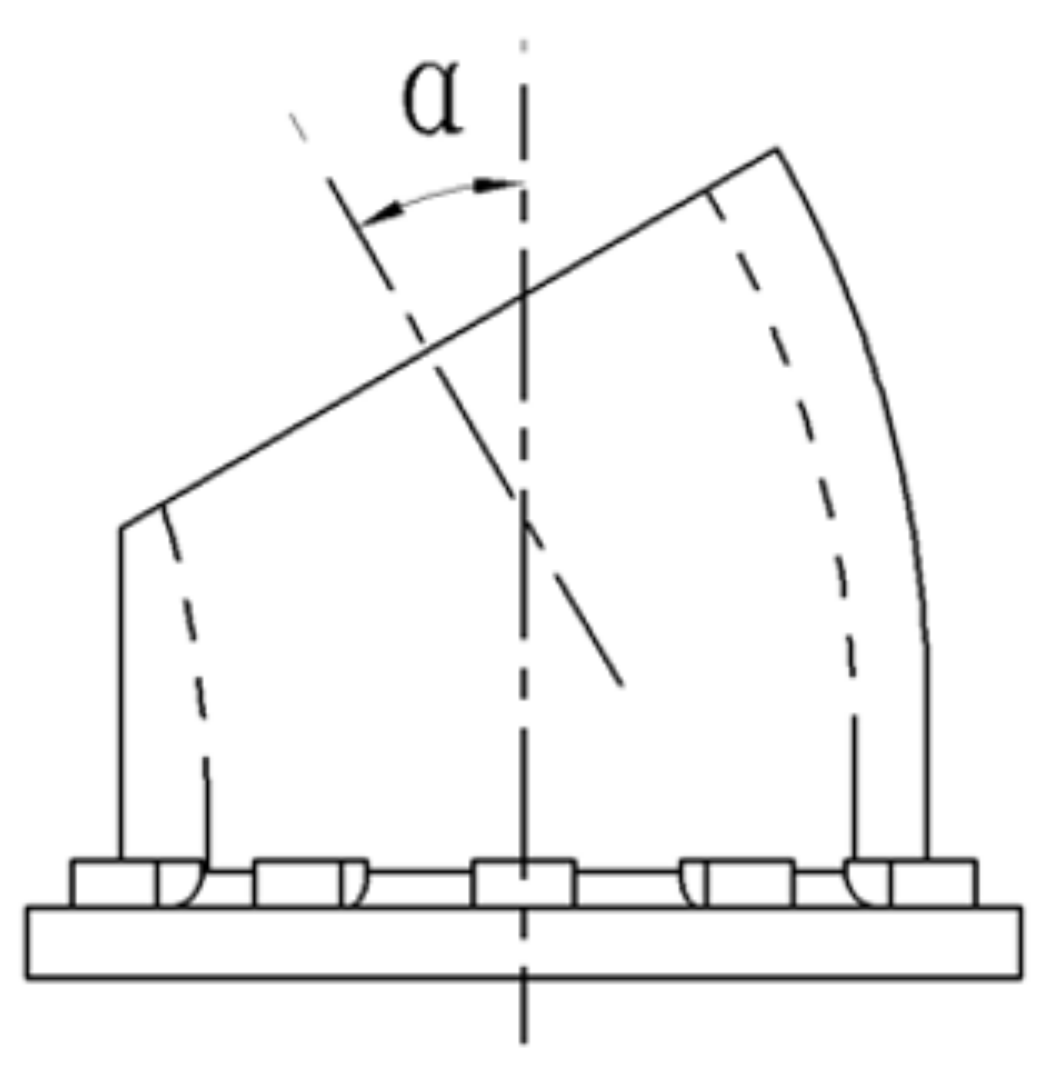
2.3. Nozzle Structure
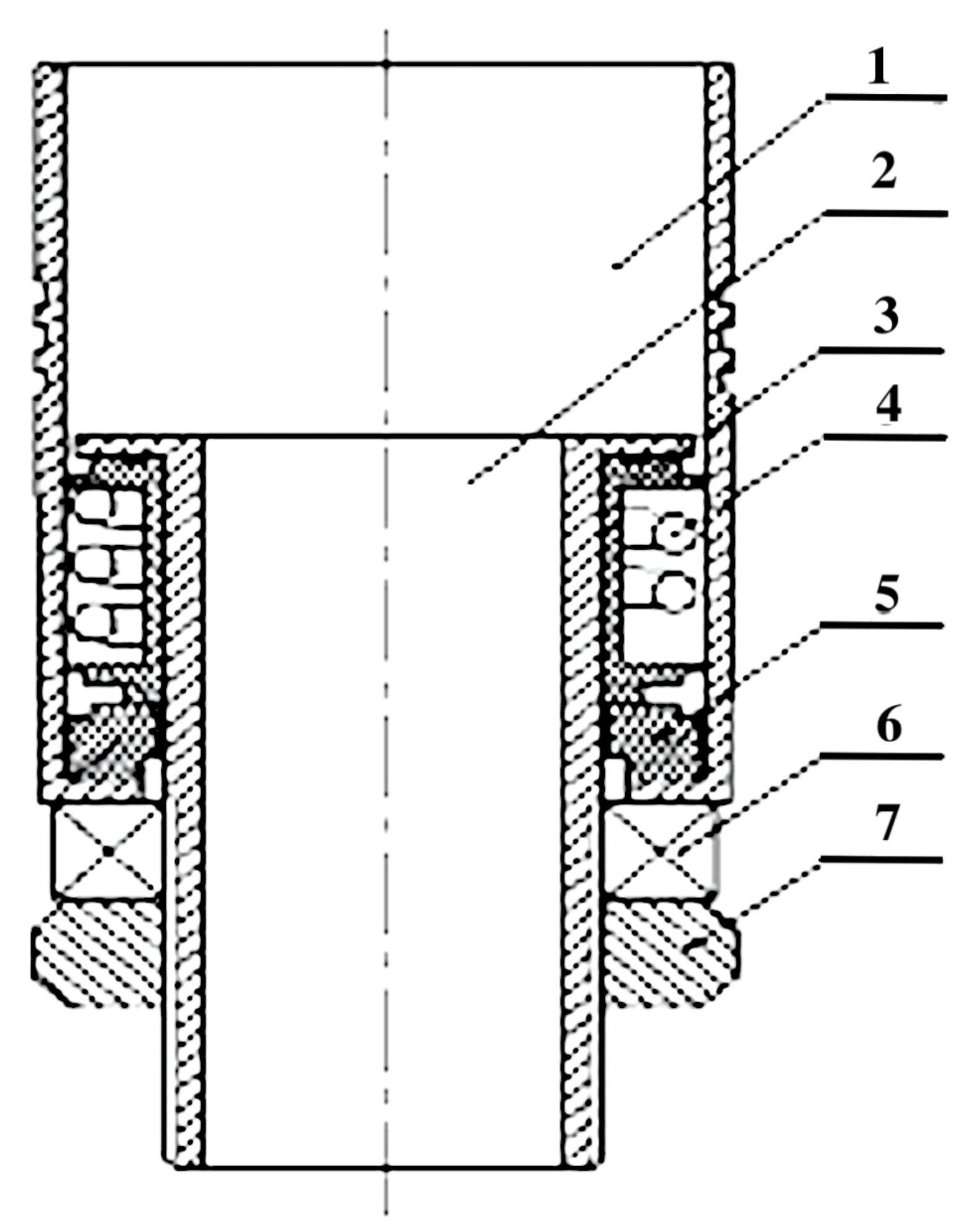
2.4. Swivel Joint Structure
3. Key Components Based on Mathematical Model Analysis
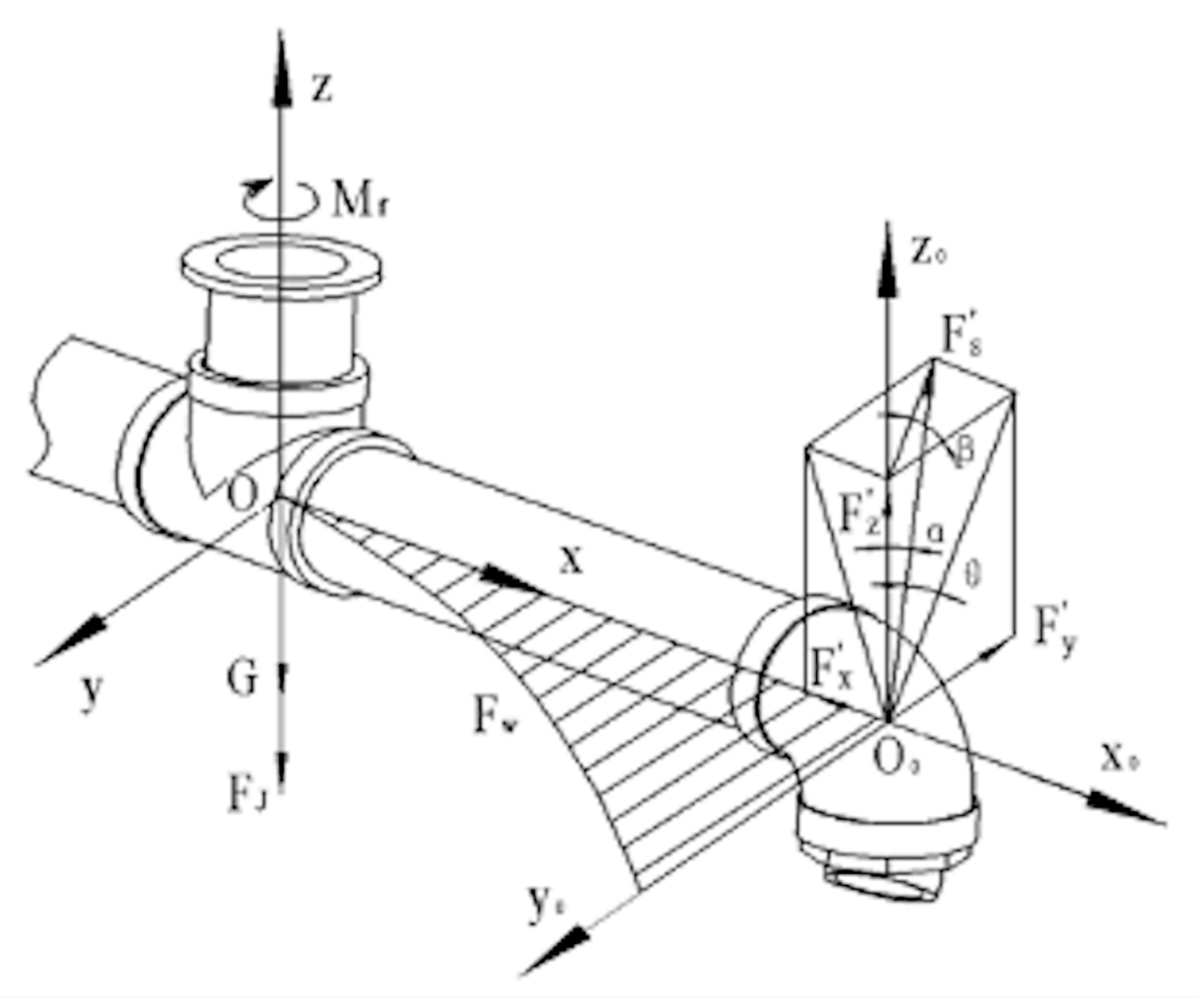
3.1. Force Analysis for Parameter Settings
3.1.1. Nozzle
3.1.2. Rotating Pipe
3.1.3. Friction Ring Torque
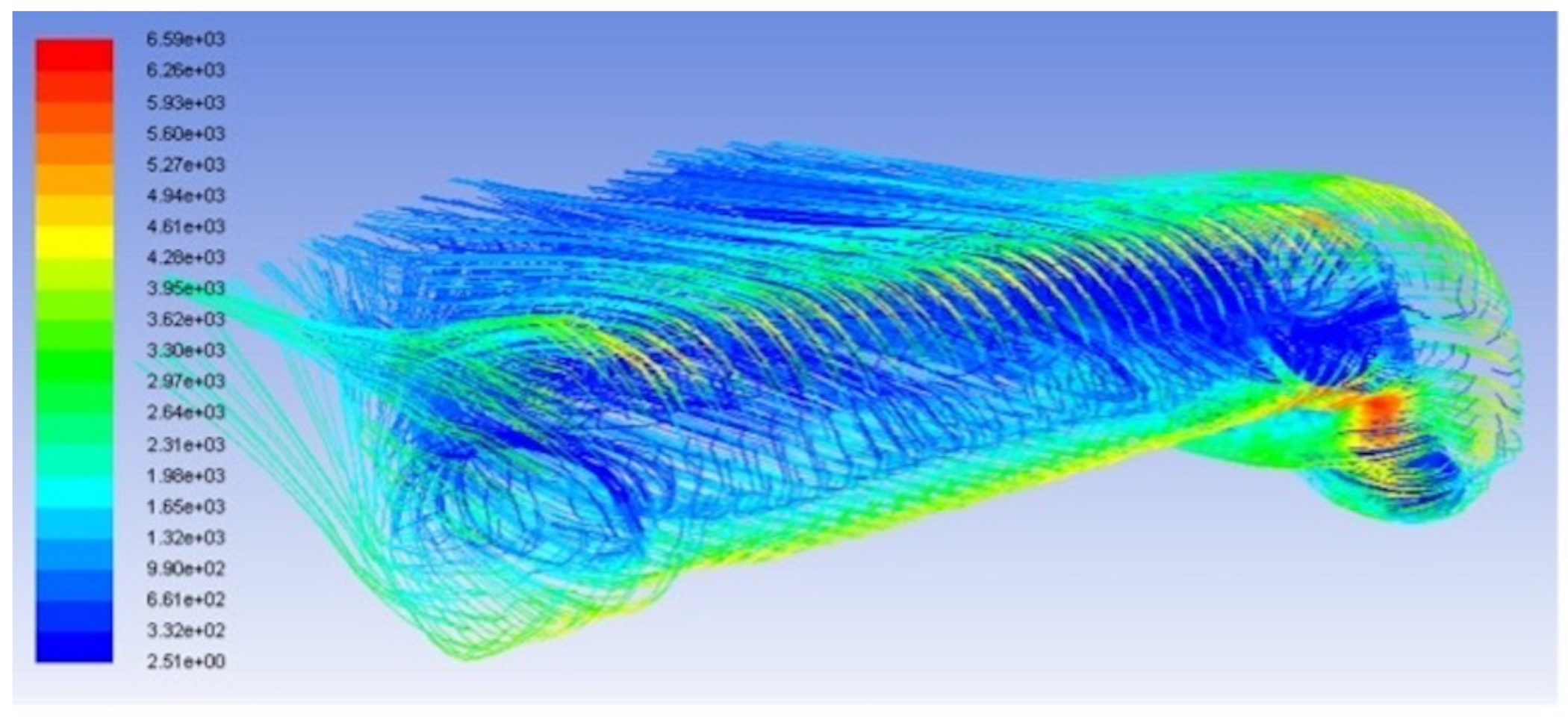
3.1.4. Moment of Resistance of Water Flow
3.2. Parametric Analysis Based on Kinematic Analysis
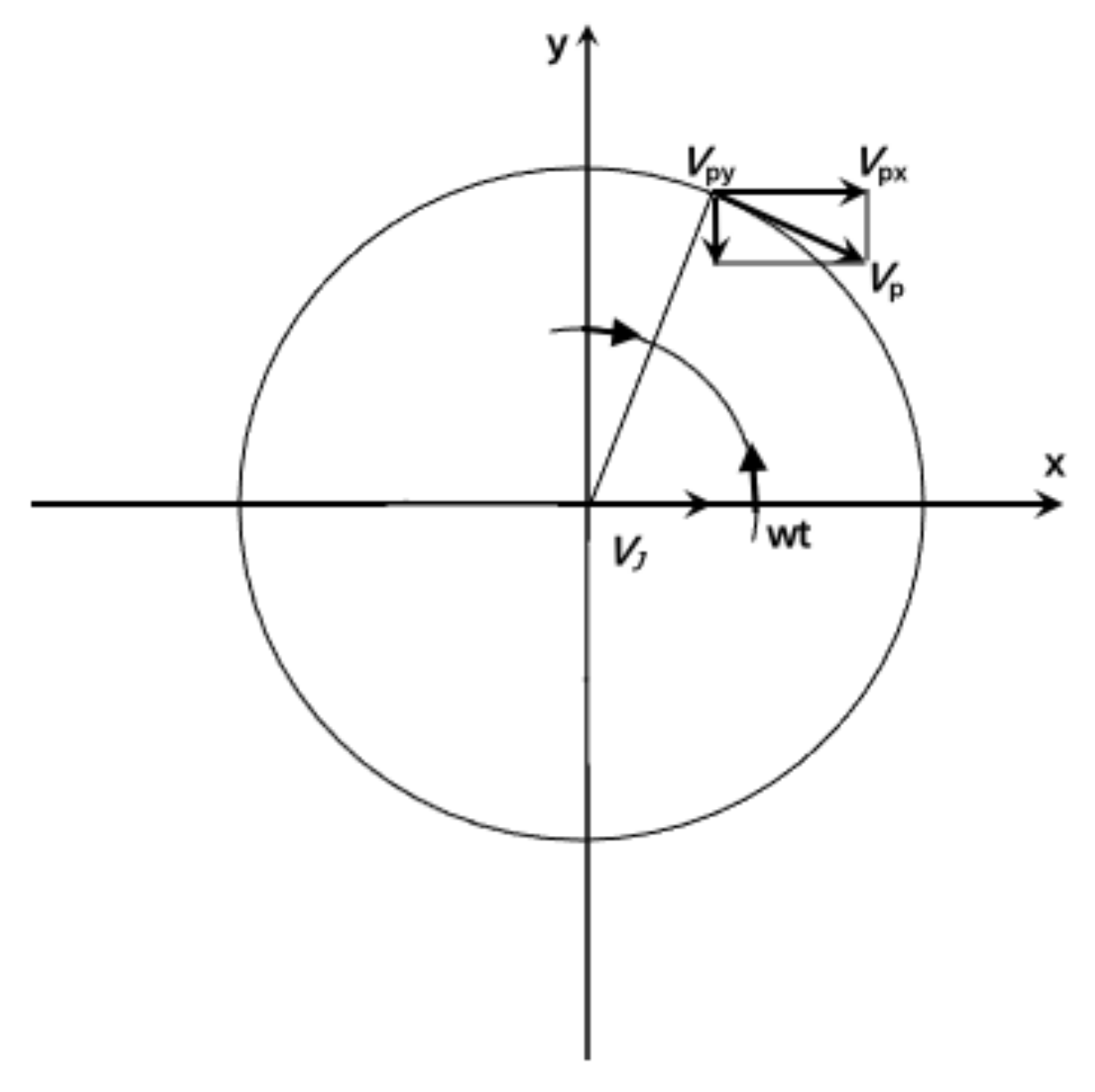
3.2.1. Rotating Pipe Speed
3.2.2. Nozzle
3.2.3. Minimum Number of Impacts
3.2.4. Single Impact Time
3.3. Kinetic Analysis for Parameter Optimization
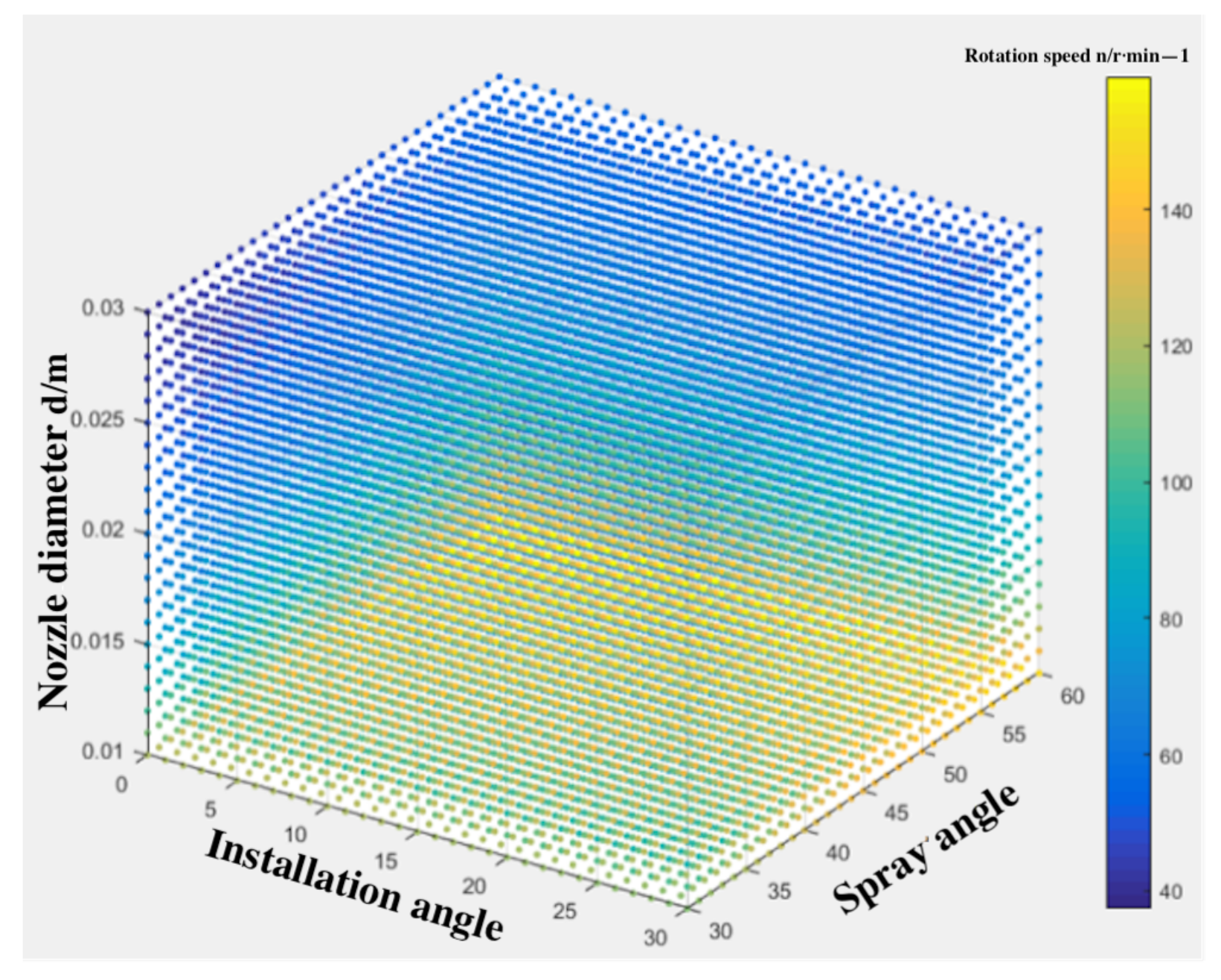
3.3.1. Nozzle Diameter and Jet Angle
3.3.2. Optimal Parameter Analysis
4. Discussion and Conclusions
- This paper provides a detailed description of the structural design of the lotus root digger and explains its operating principles.
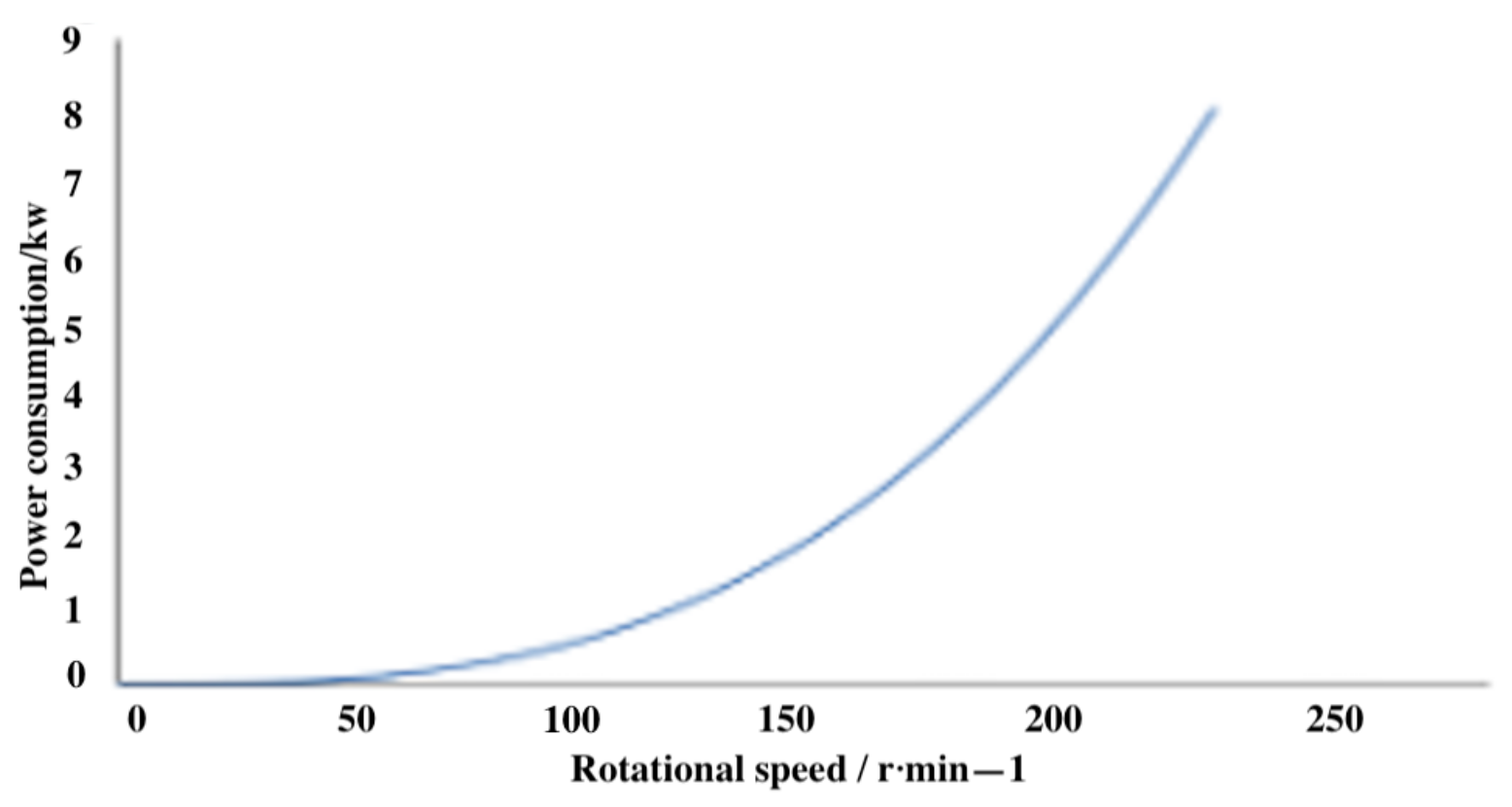
- To build mathematical analysis models for better optimisation of machine parameters, this paper obtains the water flow resistance coefficient during the linear motion of the rotating pipeline combined with CFD simulation analysis through the mechanical analysis of the nozzle and rotating pipe. Then, the mathematical model of rotating pipe speed and nozzle diameter, jet angle, and installation angle were established, and the spatial four-dimensional three-dimensional mathematical model diagram was drawn. The kinematic analysis of the nozzle was used to derive the trajectory of the nozzle. After plotting the trajectory graphs using MATLAB and combining the CFD simulation data for analysis, mathematical models of the maximum number of impacts, and the minimum time of a single impact with the radius of the effective range of the jet impact, the rotating pipe speed and the forward speed of the machine were developed, respectively. Through the analysis of the dynamics of the rotating pipeline, the mathematical model between the power consumed by the rotation of the rotating pipe and the rotational speed is established, and it is concluded that, when the rotational speed is larger, more power is consumed, the adjustable range of the impact frequency is smaller, and its positive effect on the dredging efficiency is adjusted less compared to the negative effect of the energy loss generated, ignoring the effect of the impact frequency caused by the rotating pipe on the dredging effect. The mathematical models of the forward speed of the root digger and the time required for a single point of soil impact, the rotating pipe speed, and the radius of the effective range of the jet impact were derived from a comprehensive analysis, providing a theoretical basis for the adjustment of the operating parameters of the jetting spin type lotus root digging machine.
- The optimum jet angle, nozzle diameter, nozzle installation angle, the time required to excavate the depth of the soil at a certain point, and the radius of the effective range of the jet impact are derived from the prototype tests, and then the mathematical model obtained from the theoretical analysis can be used to determine the forward speed of the jet spin dredge and the power consumed by the water flow. When the jet velocity is 20 m/s, the jet angle = 45°, and the nozzle diameter is 17 mm°, the excavation depth can reach 4 m, which is greater than the growth depth of most lotus roots. According to the results of data processing, the linear motion resistance coefficient of the rotary pipeline is = 14.95 kg/m. When the rotation speed is 100 r/min, the impact frequency can be calculated to be 3.33 Hz, but the power consumption is 1.07 KW. Thus, as the soil structure and physical characteristics of the lotus root field vary greatly from region to region, appropriate parameters need to be adjusted for different regions of the lotus root field.For joint harvesting of lotus root and large-scale lotus root field harvesting, the following work can be further carried out: Seek new theoretical analysis and simulation research methods to obtain the action mechanism of the moving jet on the soil during the nozzle movement process.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Xia, J.F.; Xu, Q.C.; Huang, H.D. Research on accelerating the mechanical research of lotus root mining. Agric. Mach. 2005, 5, 79. [Google Scholar]
- Haung, H.D.; Zhang, G.Z.; Xia, J.F. Research and development of 4CWO-3.2 type ship dredging machine. Hubei Agric. Mech. 2008, 14, 24–25. [Google Scholar]
- Wang, W. The Development of 4SWO-1.2 Type Hydraulic Excavator; Nanjing Agricultural University: Nanjing, China, 2009. [Google Scholar]
- Zhou, L. 4OZ-3 self-propelled hydraulic lotus root extractor. Agric. Mach. 2002, 8, 58. [Google Scholar]
- Liu, Y.; Zhou, Y.; Lv, W.; Huang, H.; Zhang, G.; Tu, M.; Huang, L. Design and experiment of hydraulic scouring system of wide-width lotus root digging machine. Agriculture 2021, 11, 1110. [Google Scholar] [CrossRef]
- Guo, Y.M. Design and Research of Lotus Root Mining in Paddy Field; Huazhong Agricultural University: Wuhan, China, 2015. [Google Scholar]
- Shao, L.Z.; Ma, J.C. Investigation on the development and use of the 4CW-2.6 ship type dredger. Shandong Agric. Mech. 2009, 12, 23. [Google Scholar]
- Jia, L. Ship type automatic root digging machine. Agric. Mach. 2000, 9, 61. [Google Scholar]
- Tu, M.; Lin, Y.; Cao, T.; Zhang, X.; Jiao, J.; Zhou, Y.; Zhang, G. Design and Test of a Riding Pontoon Hydraulic Lotus Root Digging Machine. Agriculture 2022, 12, 1229. [Google Scholar] [CrossRef]
- Huang, L.; Zhou, Y.; Zhang, G.Z.; Tu, Y.; Wang, H.B.; Wu, Z.D. Design and experiment of walking hydraulic scour type lotus root digging machine. J. Huazhong Agric. Univ. 2021, 40, 5. [Google Scholar]
- Fu, Z.; Li, K.; Pang, Y.; Ma, L.; Wang, Z.; Jiang, B. Study on Water Jet Characteristics of Square Nozzle Based on CFD and Particle Image Velocimetry. Symmetry 2022, 14, 2392. [Google Scholar] [CrossRef]
- Wang, J.F.; Ma, L.X.; Shao, D.W. Design and experiment of maize root stubble harvester. Chin. Soc. Agric. Mach. 2012, 43, 68–72. [Google Scholar]
- Du, J.; Xia, J.; Wu, H.; Xu, W. An Investigation of the Performance of Waterjet for Lotus Root Digging Device: Simulation and Experiment. Int. J. Fluid Mach. Syst. 2020, 13, 160–166. [Google Scholar] [CrossRef]
- Zeng, R.; Lin, Y.; Wan, Z.; Tu, M.; Jiao, J.; Zhang, G. An Investigation of Pull-Out Force of Semi-Buried Lotus Roots after Hydraulic Scouring. Agriculture 2021, 11, 706. [Google Scholar] [CrossRef]
- Feng, C.C.; Zhou, Y.; Tu, M.; Ke, H.B.; Chen, H.; Wu, H.; Jiao, J. Design and experiment of screw-propelled type lotus root diggingmachine. Gansu Agric. Univ. 2020, 11, 191–199. [Google Scholar]
- Zhang, Y.H.; Jiang, Z.X. Development of a jet-type lotus root digging machine. Cereal Oils Pro. 1982, 8, 33–38. [Google Scholar]
- Wang, L.; Ding, X.M. Experimental investigation of washing vegetables with submerged jets of water. Trans. Chin. Soc. Agric. Eng. 2007, 12, 124–130. [Google Scholar]
- Wu, H. Working Mechanism and Experimental Study of Jet Spinning Lotus Root Digging Machine. J. Huazhong Agric. Univ. 2018, 4, 79. [Google Scholar]
- Wu, H.; Xia, J.; Zhang, G.Z.; Wang, P.; Lao, S.F.; Zhang, X.M. Design and experiment of spin-jet flow type lotus root digging machine based on EDEM-Fluent. Trans. Chin. Soc. Agric. Eng. 2018, 34, 9–14. [Google Scholar]
- Xiao, K.X. The Design and Experiment of Harvest Device for Paddy-Field Lotus Root; Huazhong Agricultural University: Wuhan, China, 2016. [Google Scholar]
- Guo, Y.M.; Xia, J.F.; Xiao, K.X.; Gui, P. Determination and analysis on the yield strength and compressive strength of lotus roots. J. Huazhong Agric. Univ. 2015, 34, 148–151. [Google Scholar]




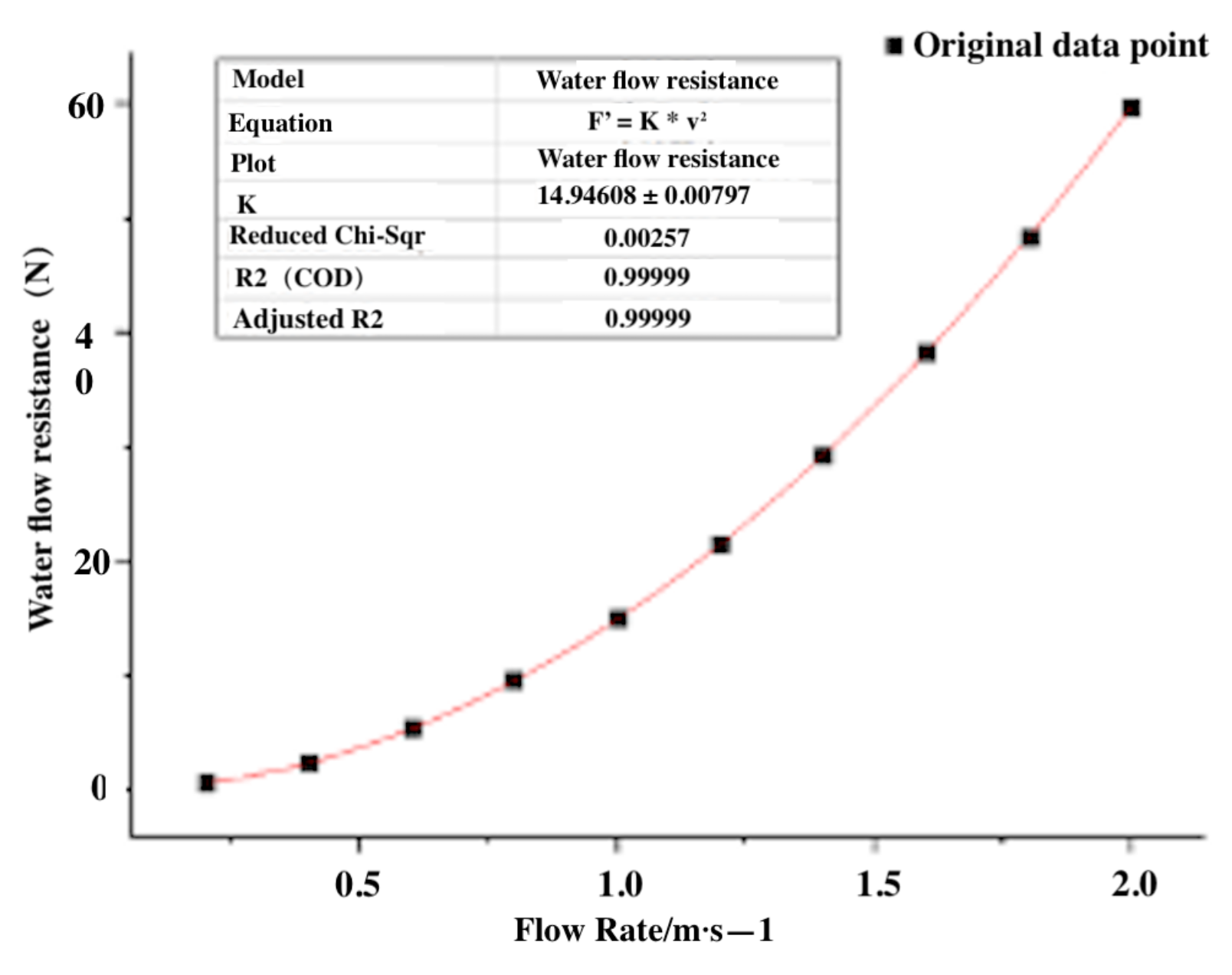


| Flow Rate | Water Flow Resistance |
|---|---|
| 0.2 | 0.61 |
| 0.4 | 2.42 |
| 0.6 | 5.43 |
| 0.8 | 9.63 |
| 1.0 | 15.01 |
| 1.2 | 21.58 |
| 1.4 | 29.34 |
| 1.6 | 38.28 |
| 1.8 | 48.41 |
| 2.0 | 59.71 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, Y.; Wu, H.; Jiang, Y.; Hu, Z. Structural Design and Theoretical Analysis of Jetting Spin Type Lotus Root Digging Machine Based on CFD. Machines 2023, 11, 269. https://doi.org/10.3390/machines11020269
Wang Y, Wu H, Jiang Y, Hu Z. Structural Design and Theoretical Analysis of Jetting Spin Type Lotus Root Digging Machine Based on CFD. Machines. 2023; 11(2):269. https://doi.org/10.3390/machines11020269
Chicago/Turabian StyleWang, Yingjie, Hao Wu, Yajun Jiang, and Zhigang Hu. 2023. "Structural Design and Theoretical Analysis of Jetting Spin Type Lotus Root Digging Machine Based on CFD" Machines 11, no. 2: 269. https://doi.org/10.3390/machines11020269
APA StyleWang, Y., Wu, H., Jiang, Y., & Hu, Z. (2023). Structural Design and Theoretical Analysis of Jetting Spin Type Lotus Root Digging Machine Based on CFD. Machines, 11(2), 269. https://doi.org/10.3390/machines11020269






