A Novel Tooth Modification Methodology for Improving the Load-Bearing Capacity of Non-Orthogonal Helical Face Gears

Abstract

This study proposes a double-crown tooth surface modification technology that improves the load-carrying capacity of non-orthogonal helical tooth surface gears. The tooth modification is determined by a modified rack-cutter, and its feed motion is related to an intentionally designed transmission error. The novelty of the tooth modification design is that the transmission error can be pre-designed. First, changing the tooth profile of the tool enables preliminary modification along the tooth profile direction; second, by modifying the interaction between the tool and the machined gear, subsequent fine adjustments are made to the contact path. This two-stage tooth modification strategy not only retains the advantages of the traditional method but also significantly improves the balance of the load distribution on the tooth surface through an original contact path modification strategy. Through systematic tooth contact analysis (TCA) and loaded tooth contact analysis (LTCA), it was verified that the new method reduces contact stress and tooth root bending stress and improves the gear’s resistance to misalignment errors. This research provides the basis and motivation for further exploring and improving this tooth profile modification technology to solve the challenges faced by more complex gear systems.

1. Introduction

Transmission systems, which are integral to mechanical devices, benefit significantly from the enhancement of gear performance, as it directly affects the overall quality of the equipment. The modification of gear teeth is a prevalent method for enhancing the performance of gear transmission [1,2,3,4,5,6]. By implementing this technology, the typical line contact found on unmodified gear teeth transitions to point contact after modification. This shift notably diminishes the susceptibility to errors, resulting in a concurrent reduction in both vibration and noise while enhancing the meshing of gears [7]. The process of tooth modification stands out for both its effectiveness and cost-efficiency. It involves precise, micron-level alterations to the gear teeth, leaving the macro structure and overall dimensions of the gear system intact; importantly, it also does not contribute additional weight to the system. Its broad application across diverse gear-driven mechanisms underscores its utility [8,9,10,11,12].

As an evolved transmission variant, face gear systems are pivotal in the realm of machinery, notably within helicopter main reducers, where they have been utilized with considerable success. These systems, due to their rapid operational speeds and substantial load requirements, necessitate tooth modifications [13,14,15,16,17]. The chief aim of teeth modification in this context is to redistribute the forces acting across the teeth surfaces and stabilize the contact patterns in the central tooth zone. Techniques for gear tooth adjustments may include profile, longitudinal, and three-dimensional modifications, collectively referenced here as conventional tooth modification methods. Although these adjustments can lead to diminished vibration and noise, they can inadvertently cause the load to concentrate in the central region of the teeth, thereby posing a risk for stress concentration. This is particularly concerning in the current landscape where machine loads are progressively increasing, leading to immediate loading of the middle tooth region subsequent to the engagement of the top and root areas. The resulting spike in contact stress can not only accelerate surface wear and reduce the operational lifespan but also decrease the efficiency of gear drives [18,19,20,21,22,23,24].

However, there are some limitations in the current tooth surface modification methods, such as the transmission error correction of helical bevel gears and the high-order transmission error (HTE) correction of helical involute gears. These aspects still require further research and improvement. Some researchers have begun to study new methods of tooth surface modification. Su et al. [25] introduced a method to correct transmission errors up to the seventh order in spiral bevel gears. Their research demonstrated that following this correction, the bending stresses on the gear teeth were reduced compared to those observed on parabolic tooth surfaces, with the contact stress remaining largely unaffected. Jiang et al. [26] designed a high-order transmission error correction for helical involute gears through particle swarm optimization (PSO), but there is a lack of correction during the correction process with full consideration of the polynomial coefficients. In addition, Jia et al. [27] proposed a new method based on compensated conjugation to reduce the peak-to-peak transfer error in the design of cylindrical gear tooth surface modification. Yu et al. [28] and Yang et al. [29] proposed an improved tooth surface modification strategy based on spiral theory and the curvature synthesis method. Further research can be carried out on the optimization of transmission performance and surface shape. Mu et al. [30] proposed a functional design-oriented gear design method, emphasizing the importance of gear transmission performance with high-order transmission errors. Samani et al. [31] conducted a nonlinear vibration study on a spiral bevel gear pair with high-order transmission errors and parabolic transmission errors. Korta and Mundo [32] conducted a gear optimization study using the response surface method. Lu et al. [33] developed a tooth profile generation algorithm for non-bevel gears, which is used to correct the pitch points of high-order Archimedes spiral bevel gears and quadratic bevel gears. Finally, Zhao et al. [34] proposed an automatic green onion seedling raising mechanism using an asymmetric transmission high-order non-circular planetary gear train to improve the efficiency of automatic green onion transplanting.

In the above literature, we found that with the continuous improvement of the aviation industry, the new generation of aircraft has put forward higher requirements for face gear transmission systems. Therefore, further research on face gear modification technology still has important practical significance. Traditional modification methods can effectively reduce contact stress, but there is a risk of stress concentration. In order to improve the load-bearing capacity of non-orthogonal helical tooth surface gears, we propose a novel tooth profile modification method, namely the double crown modification method. This method performs corrections along the direction of the tooth profile and the direction of the contact path. By comparing the results of traditional tooth modifications with standard teeth, with and without misalignment errors, the effect of this new tooth modification method is verified.

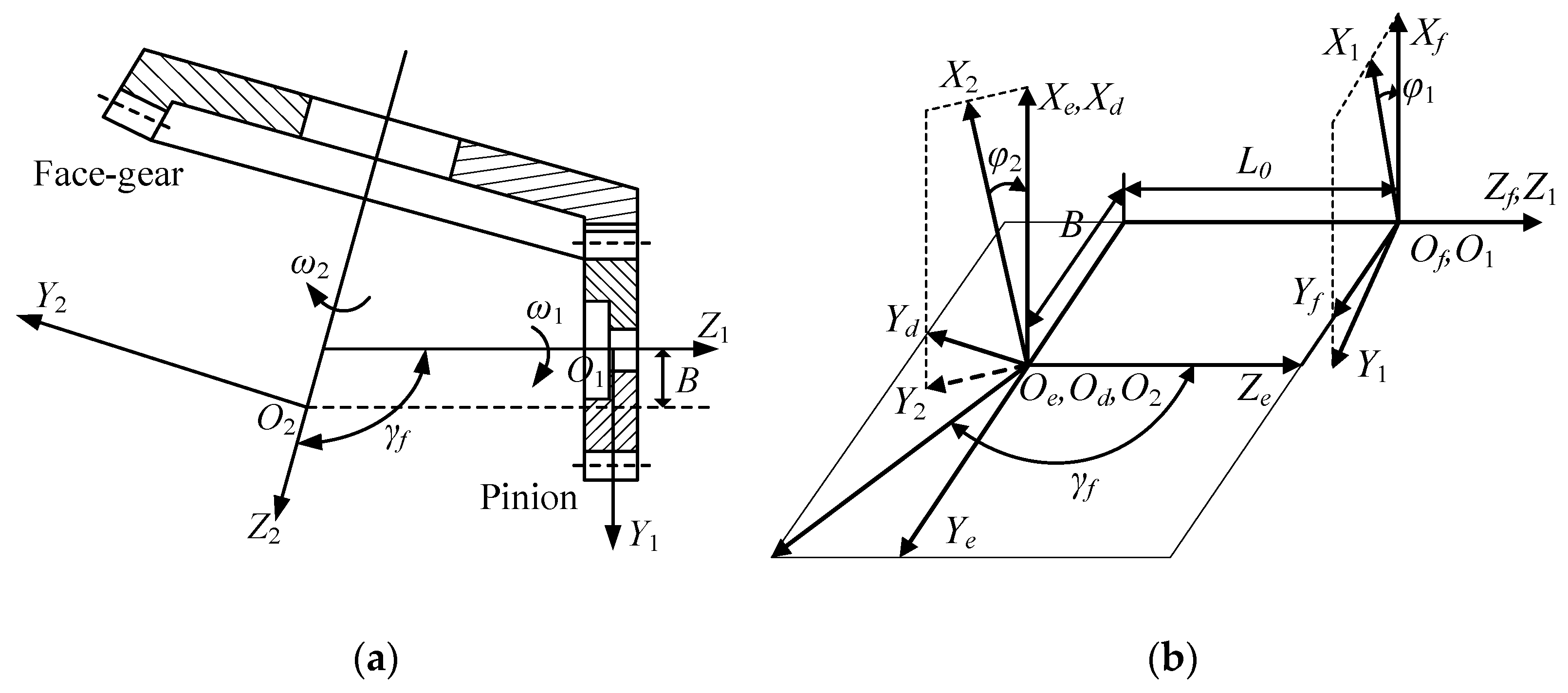
2. Generation of Non-Orthogonal Helical Face Gear Pair

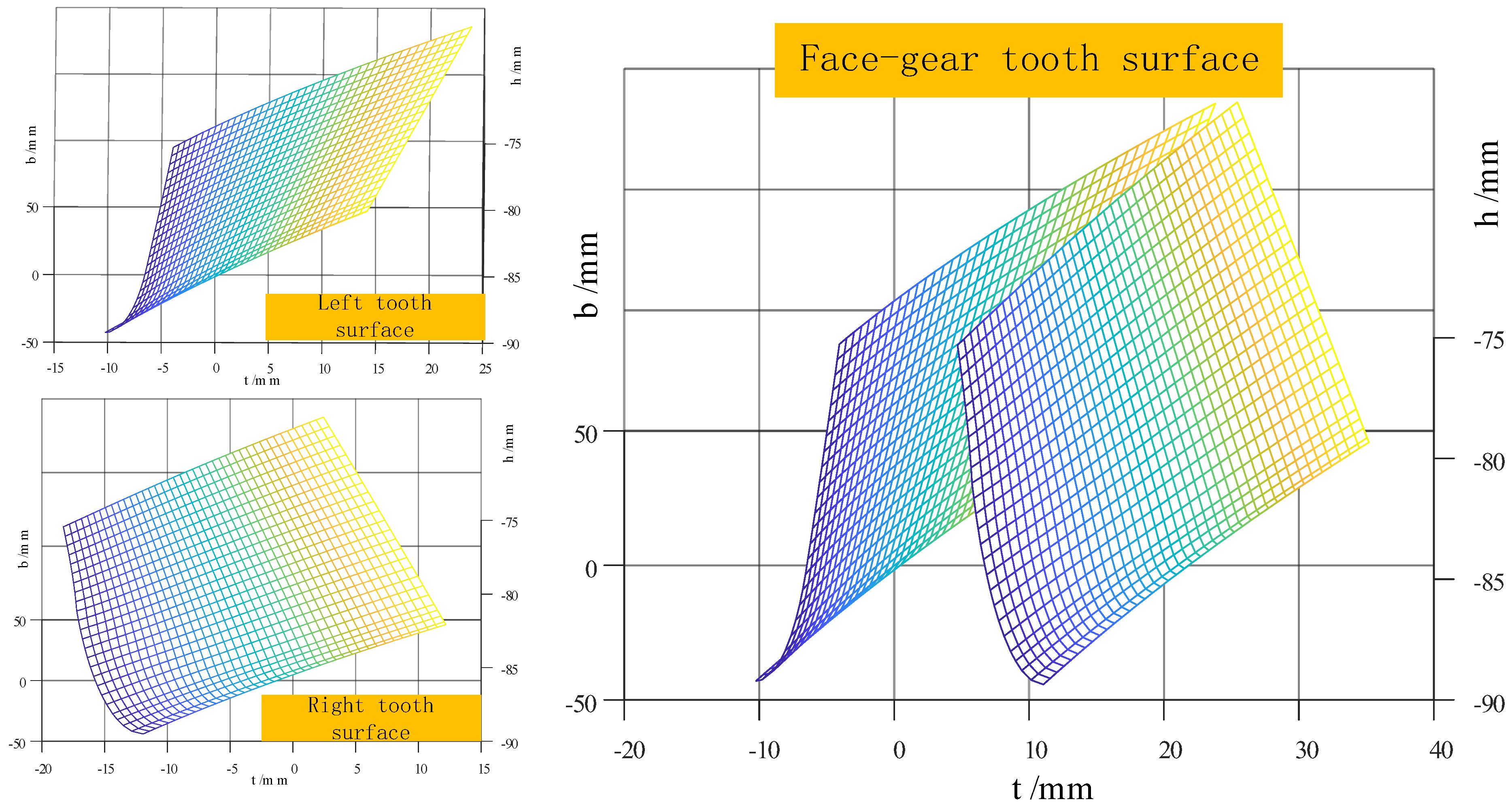
2.1. Generation of Tooth Surface of Face Gear

As shown in Figure 1, the face gear machining process is carried out by using a grinding wheel to simulate a single-tooth gear cutter. The grinding wheel is regarded as a single tooth of the gear cutter, which moves in the direction of the tooth face while rotating and cutting itself, where S₂, S_g, and S_S are rigidly connected to the face gear, the disk grinding wheel and the virtual cutter, and Z₂, Z_g, and Zs are the corresponding rotation axes, respectively.

The distance between the origin O₂ and Os of the two coordinate systems S₂ and S_S is L₀, and the angle γ_m is the shaft angle. The center distance between the disk grinding wheel and the virtual cutter is E_g, and E_g is defined as $E_g=r_g-r_{ps}$, where r_ps is the radius of the pitch circle of the virtual cutter, and r_g is the radius of the pitch circle of the disk grinding wheel; l_g is the moving distance of the center of the disk grinding wheel. There are three kinds of motion during the grinding process: the disc-shaped grinding wheel swings around the rotation axis Z_S of the virtual gear shaping cutter at an angular velocity ω_s, the face gear rotates around its own rotation axis Z₂ at an angular velocity ω₂, and the grinding wheel rotates around its own rotation axis X_g at a high speed ω_g to form a cutting motion.

Through the movement of the tool and the face gear, the position vector of the virtual tool is converted from the coordinate system S_S to the coordinate system S₂ [35]. Then, the tooth surface of the face gear is generated using the meshing equation.

What is clearly shown in Figure 2 above is a detailed tooth profile illustration of the rack-cutter specifically designed for the purpose of machining the theoretical tooth surfaces of the disk grinding wheel. According to the knowledge of the fundamental principle of gear meshing theory, the tooth surface of the disk grinding wheel depicted in Figure 1 is meticulously shaped through the process of enveloping the toothed surface of the rack-cutter.

The tooth profile of the rack-cutter is determined via Equations (1) and (2).

$${\stackrel{\rightharpoonup }{r}}_{a2}={[-a_{c2}{u}_2{}^2, u_2, l_g,1]}^T$$

(1)

$${\stackrel{\rightharpoonup }{n}}_{a2}=\frac{1}{\sqrt{{(-2a_{c2}{u}_2)}^2+1^2}}\left[\begin{array}{c}-2a_{c2}{u}_2\\ 1\\ 0\end{array}\right]$$

(2)

In the context of tooth profile modification for the cutter, a_c2 represents the modification parameter. The parameters u₂ and l_g are associated with the flank characteristics of the cutting tool.

The profile of the tooth surface for the hypothetical cutter is defined by Equations (3) and (4).

$${\stackrel{\rightharpoonup }{r}}_s(u_2,l_g,{\theta }_s)=M_{sa}{\stackrel{\rightharpoonup }{r}}_{a2}(u_2,l_g)$$

(3)

$${\stackrel{\rightharpoonup }{n}}_s(u_2,{\theta }_s)=L_{sa}{\overrightarrow{n}}_{a2}(u_2)$$

(4)

where $M_{sa}$ is a 4 × 4 coordinate transformation matrix from S_a to S_si, and $L_{sa}$ is a 3 × 3 submatrix of $M_{sa}$. $M_{sa}$ is determined via Equation (5).

$$M_{sa}=M_{s,ns}{M}_{ns,c}{M}_{cb}{M}_{ba}$$

(5)

$$M_{ba}=\left[\begin{array}{cccc}\cos \alpha & \sin \alpha & 0& -0.5s_0{\cos }^2\alpha \\ -\sin \alpha & \cos \alpha & 0& 0.5s_0\sin \alpha \cos \alpha \\ 0& 0& 1& 0\\ 0& 0& 0& 1\end{array}\right]$$

(6)

$$M_{cb}=\left[\begin{array}{cccc}\cos {\beta }_2& 0& \sin {\beta }_2& 0\\ 0& 1& 0& 0\\ -\sin {\beta }_2& 0& \cos {\beta }_2& 0\\ 0& 0& 0& 1\end{array}\right]$$

(7)

$$M_{ns,c}=\left[\begin{array}{cccc}1& 0& 0& r_{ps}{\theta }_s\\ 0& 1& 0& -r_{ps}\\ 0& 0& 1& 0\\ 0& 0& 0& 1\end{array}\right]$$

(8)

$$M_{s,ns}=\left[\begin{array}{cccc}\cos {\theta }_s& -\sin {\theta }_s& 0& 0\\ \sin {\theta }_s& \cos {\theta }_s& 0& 0\\ 0& 0& 1& 0\\ 0& 0& 0& 1\end{array}\right]$$

(9)

In Figure 3, the disk grinding wheel rotates around axis X_g at a high speed ω_g to form a cutting motion.

The tooth surface of the disk grinding wheel is determined by Equations (10) and (11).

$${\stackrel{\rightharpoonup }{r}}_g(u_2,{\theta }_g)=M_{gs}{\stackrel{\rightharpoonup }{r}}_s(u_2)$$

(10)

$${\stackrel{\rightharpoonup }{n}}_g(u_2,{\theta }_g)=L_{gs}{\stackrel{\rightharpoonup }{n}}_s(u_2)$$

(11)

where θ_g is the rotation angle of the face gear; M_gs is a 4 × 4 coordinate transformation matrix from S_s to S_g; and L_gs is a 3 × 3 submatrix of M_gs. M_gs is determined via Equation (12).

$$M_{gs}=M_{gt}{M}_{ts}$$

(12)

$$M_{ts}=\left[\begin{array}{cccc}1& 0& 0& 0\\ 0& 1& 0& -E_g\\ 0& 0& 1& 0\\ 0& 0& 0& 1\end{array}\right]$$

(13)

$$M_{gt}=\left[\begin{array}{cccc}1& 0& 0& 0\\ 0& \cos {\theta }_g& \sin {\theta }_g& 0\\ 0& -\sin {\theta }_g& \cos {\theta }_g& 0\\ 0& 0& 0& 1\end{array}\right]$$

(14)

The tooth surface of the face gear is determined by Equation (15).

$$\left\{\begin{array}{l}{\stackrel{\rightharpoonup }{r}}_2(u_2,l_g,{\phi }_g,{\theta }_g)=M_{2g}{\stackrel{\rightharpoonup }{r}}_g(u_2,{\theta }_g)\\ f_1={\stackrel{\rightharpoonup }{n}}_g(u_2,{\theta }_g){\stackrel{\rightharpoonup }{v}}_{g1}(u_2,l_g,{\phi }_g,{\theta }_g)=0\\ f_2={\stackrel{\rightharpoonup }{n}}_g(u_2,{\theta }_g){\stackrel{\rightharpoonup }{v}}_{g2}(u_2,l_g,{\phi }_g,{\theta }_g)=0\end{array}\right.$$

(15)

$${\overrightarrow{n}}_2(u_2,l_g,{\phi }_g,{\theta }_g)=L_{2g}{\overrightarrow{n}}_g(u_2,{\theta }_g)$$

(16)

where $M_{2g}$ is a 4 × 4 coordinate transformation matrix from S_g to S₂, and $L_{2g}$ is a 3 × 3 submatrix of $M_{2g}$. $f_1$, $f_1$ are the meshing equations of face gears in the coordinate system S_s. ${\stackrel{\rightharpoonup }{v}}_{g1}$ is the grinding wheel’s center speed, while ${\stackrel{\rightharpoonup }{v}}_{g2}$ is the non-orthogonal helical face gears’ relative speed to the grinding wheel. $M_{2g}$ is determined via Equation (17).

$$M_{2g}=M_{2p}{M}_{ps}{M}_{sm}{M}_{mg}$$

(17)

$$M_{mg}=\left[\begin{array}{cccc}1& 0& 0& 0\\ 0& 1& 0& E_g\\ 0& 0& 1& l_g\\ 0& 0& 0& 1\end{array}\right]$$

(18)

$$M_{sm}=\left[\begin{array}{cccc}\cos {\phi }_g& -\sin {\phi }_g& 0& 0\\ \sin {\phi }_g& \cos {\phi }_g& 0& 0\\ 0& 0& 1& 0\\ 0& 0& 0& 1\end{array}\right]$$

(19)

$$M_{ps}=\left[\begin{array}{cccc}1& 0& 0& 0\\ 0& \cos {\gamma }_m& -\sin {\gamma }_m& -L_0\sin {\gamma }_m\\ 0& \sin {\gamma }_m& \cos {\gamma }_m& L_0\cos {\gamma }_m\\ 0& 0& 0& 1\end{array}\right]$$

(20)

$$M_{2p}=\left[\begin{array}{cccc}\cos {\theta }_2& \sin {\theta }_2& 0& 0\\ -\sin {\theta }_2& \cos {\theta }_2& 0& 0\\ 0& 0& 1& 0\\ 0& 0& 0& 1\end{array}\right]$$

(21)

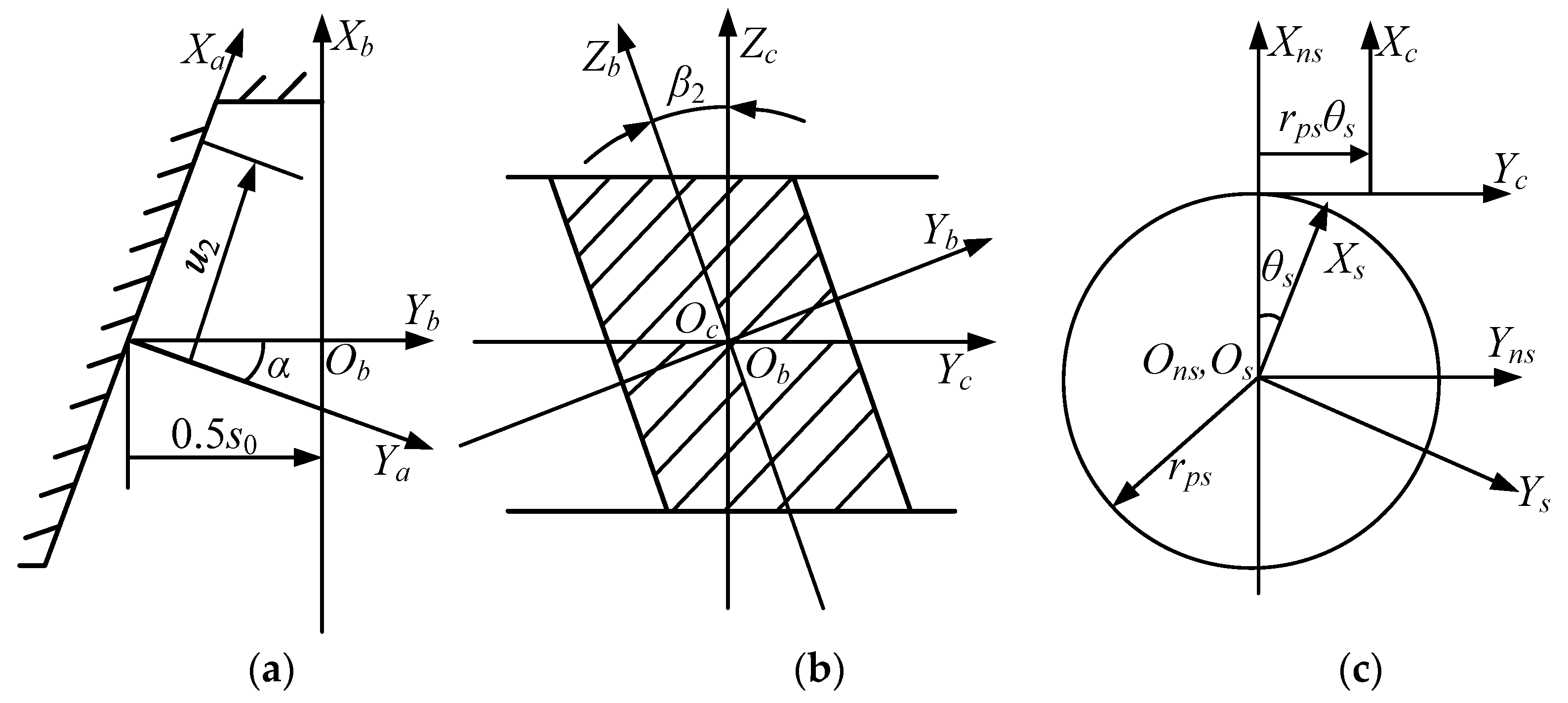
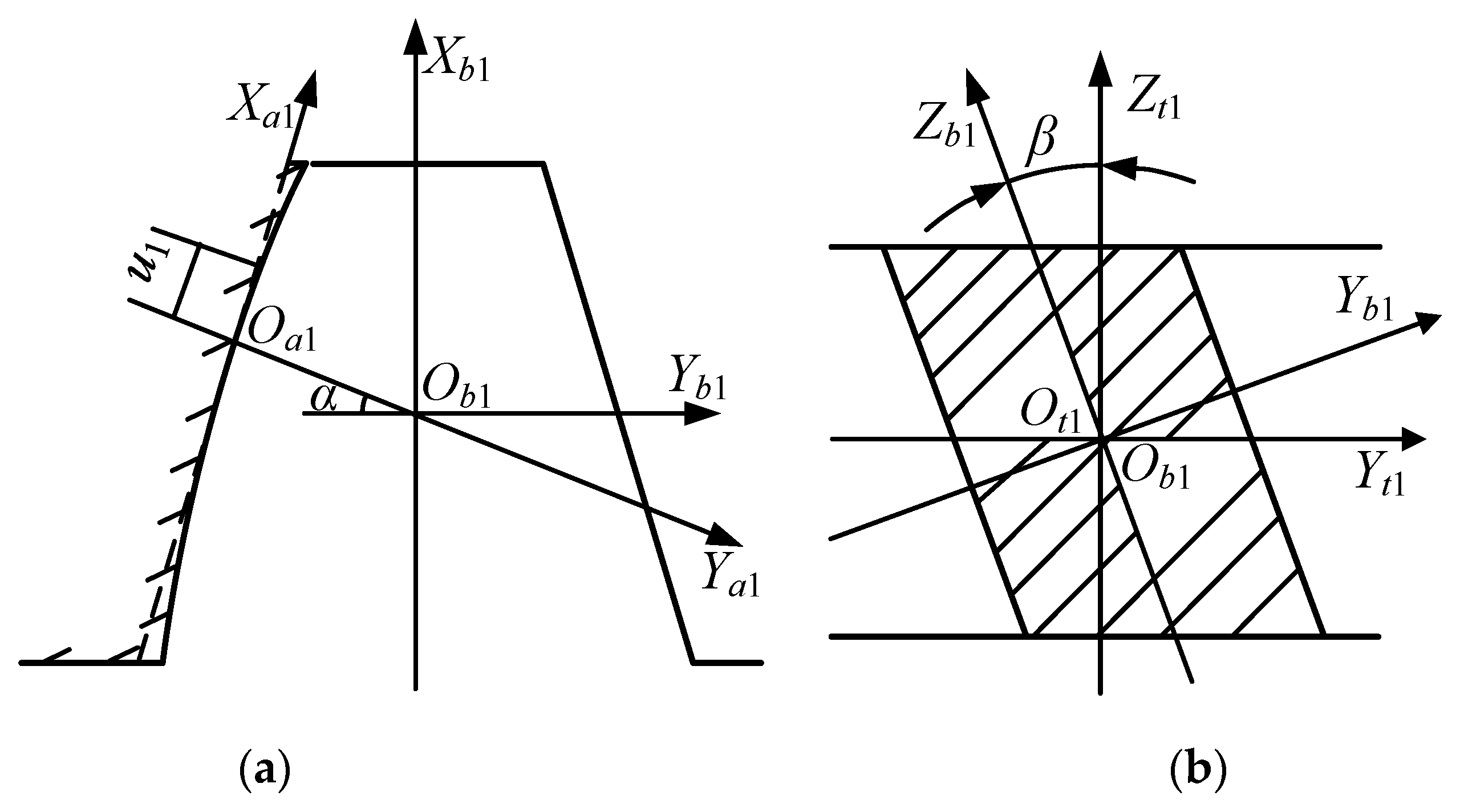
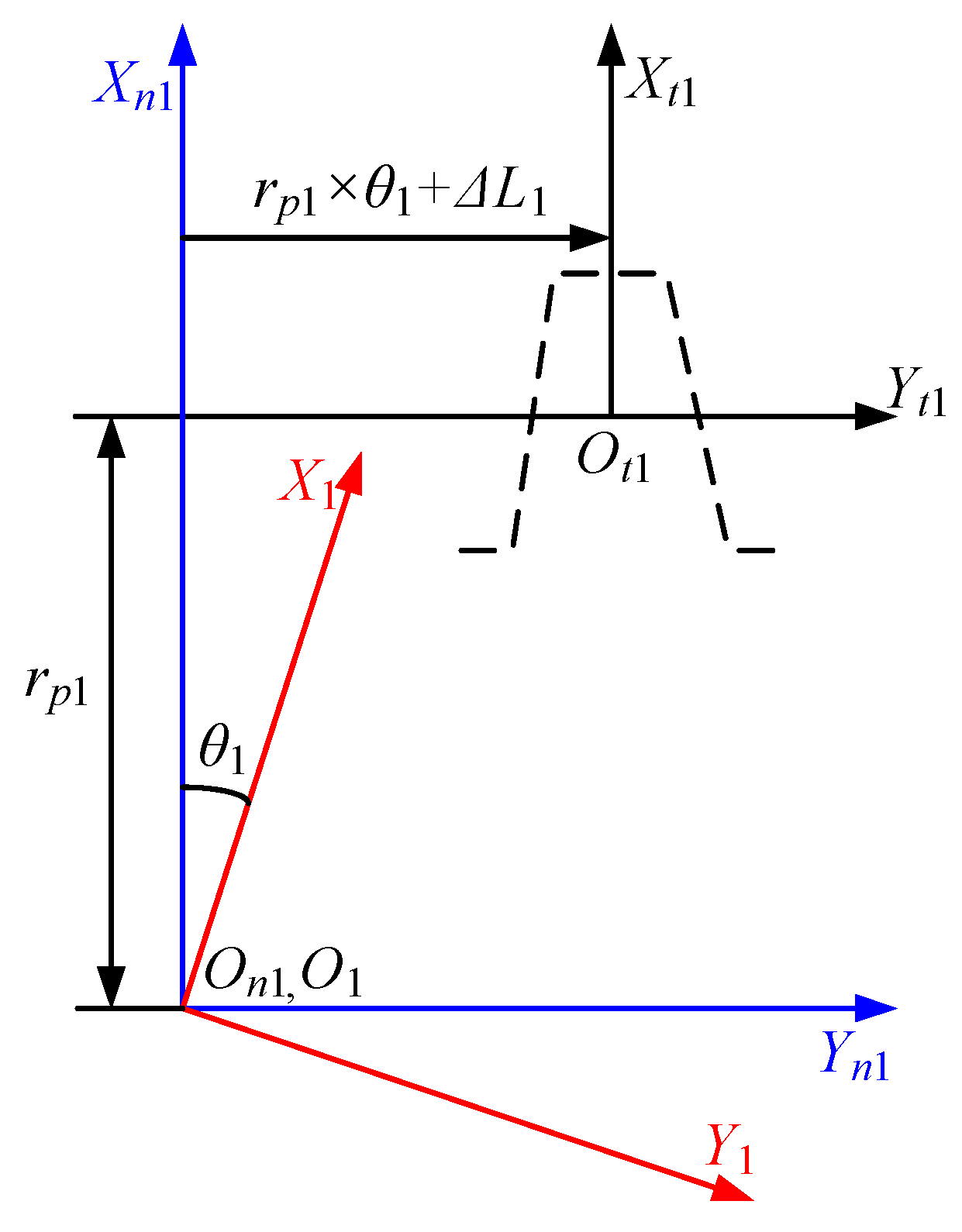
2.2. Generation of Novel Double-Crowned Tooth Surface of Pinion

This research focuses exclusively on the alteration of the pinion tooth. The innovative tooth modification approach is established through the utilization of a modified rack-cutter, alongside its feeding motion, which correlates with a deliberately engineered higher-order transmission error. By meshing with the modified rack, Figure 4 can clearly see the tooth profile of the pinion before and after modification. The amount of practice in the middle is less, and the amount of shaping in and out is larger.

As shown in Figure 5, the coordinate systems S_a1, S_b1, and S_t1 is connected to the rack-cutter. The tooth modification along the tooth profile direction can be realized based on the tooth modification of the rack-cutter.

In Figure 5, the tooth profile of the rack-cutter is a second-order parabola.

As shown in Figure 6, the pinion is generated by the modified rack-cutter. The motion of the rack-cutter and the produced pinion can be changed to achieve a different direction of tooth modification. The produced pinion’s rotating motion is represented by θ₁. ΔL₁ is the rack-cutter’s extra translation motion parameter, which is related to the intentional designed high-order transmission error. S_n1 is the fixed coordinate system. r_p1 is the pitch radius of the pinion. S_t1 and S₁ are the coordinate systems of the rack-cutter and generated pinion, respectively.

The double-crowned modified pinion surface can be represented by Equation (22).

$$\left\{\begin{array}{l} \\ {\stackrel{\rightharpoonup }{r}}_1(u_1,l_1,\Delta L_1,{\theta }_1)={[M]}_{1,t1}{\stackrel{\rightharpoonup }{r}}_{t1}^{}(u_1,l_1)\\ {\stackrel{\rightharpoonup }{n}}_1={[L]}_{1,t1}{\stackrel{\rightharpoonup }{n}}_{t1}^{}\\ f(u_1,l_1,\Delta L_1,{\theta }_1)=\left(\frac{\partial {\stackrel{\rightharpoonup }{r}}_1}{\partial u_1}\times \frac{\partial {\stackrel{\rightharpoonup }{r}}_1}{\partial l_1}\right)\cdot \frac{\partial {\stackrel{\rightharpoonup }{r}}_1}{\partial {\theta }_1}=0\end{array}\right.$$

(22)

where ${\left[M\right]}_{1,t1}$ is a 4 × 4 coordinate transformation matrix from S_t1 to S₁ and ${\left[L\right]}_{1,t1}$ is a 3 × 3 submatrix of ${\left[M\right]}_{1,t1}$; ${\stackrel{\rightharpoonup }{r}}_{t1}$ and ${\stackrel{\rightharpoonup }{n}}_{t1}$ are vectors in the coordinate system S_t1, both obtained via Equation (23).

$$\left\{\begin{array}{l}{\stackrel{\rightharpoonup }{r}}_{t1}(u_1,l_1)={[M]}_{t1,a1}{[u_1, a_1{u}_1^2, l_1,1]}^T\\ {\stackrel{\rightharpoonup }{n}}_{t1}=\frac{\partial {\stackrel{\rightharpoonup }{r}}_{t1}}{\partial u_1}\times \frac{\partial {\stackrel{\rightharpoonup }{r}}_{t1}}{\partial l_1}/\left|\frac{\partial {\stackrel{\rightharpoonup }{r}}_{t1}}{\partial u_1}\times \frac{\partial {\stackrel{\rightharpoonup }{r}}_{t1}}{\partial l_1}\right|\end{array}\right.$$

(23)

where a₁ is the modification parameter for the tooth profile modification of the cutter; u₁ and l₁ are the related tooth flank parameters of the cutter.

3. Analysis of Meshing Performance of Face Gears

3.1. Tooth Contact Analysis

The tooth touch analysis (TCA) approach was designed to more effectively assess the real state of the tooth flanks while they are touching, and it is a numerical method to program the mesh process of the tooth flanks Σ1 and Σ2 with the use of coordinate transformations in order to obtain the contact imprints and the transmission errors. The structure of the face gear sub-structure is shown in Figure 7, with S₁ being the fixed coordinate system of the cylindrical gear and S₂ being the fixed coordinate system of the face gear. In the figure, B = r_ps − r_p1 and γ_f = γ_m + Δγ, where γ_m is the shaft angle, and Δγ is the shaft angle error.

The coordinate transformation method is used to describe the equations for the tooth surface of the pinion and face gears in the coordinate system Sf. Equations (24)–(27) can be used to model the tooth surface.

$${\stackrel{\rightharpoonup }{r}}_2{}^{\left(f\right)}\left(u_2,l_g,{\phi }_g,{\theta }_g,{\phi }_2\right)=M_{f2}{\stackrel{\rightharpoonup }{r}}_2\left(u_2,l_g,{\phi }_g,{\theta }_g\right)$$

(24)

$${\stackrel{\rightharpoonup }{r}}_1{}^{\left(f\right)}\left(u_1,l_1,{\phi }_1\right)=M_{f1}{\stackrel{\rightharpoonup }{r}}_1\left(u_1,l_1\right)$$

(25)

$${\stackrel{\rightharpoonup }{n}}_2{}^{\left(f\right)}\left(u_2,l_g,{\phi }_g,{\theta }_g,{\phi }_2\right)=L_{f2}{\stackrel{\rightharpoonup }{n}}_2\left(u_2,l_g,{\phi }_g,{\theta }_g\right)$$

(26)

$${\stackrel{\rightharpoonup }{n}}_1{}^{\left(f\right)}\left(u_1,l_1,{\phi }_1\right)=L_{f1}{\stackrel{\rightharpoonup }{n}}_1\left(u_1,l_1\right)$$

(27)

where ${\stackrel{\rightharpoonup }{r}}_1^{(f)}$ and ${\stackrel{\rightharpoonup }{r}}_2^{(f)}$ are position vectors for the pinion’s tooth surface∑₁ and face gear ∑₂; ${\stackrel{\rightharpoonup }{n}}_1^{(f)}$ and ${\stackrel{\rightharpoonup }{n}}_2^{(f)}$ are unit normal vectors for the pinion’s tooth surface ${\stackrel{\rightharpoonup }{r}}_1^{(f)}$ and the face gear ${\stackrel{\rightharpoonup }{n}}_2^{(f)}$, respectively; $M_{fi}$ (i = 1, 2) is a 4 × 4 matrix; $L_{fi}$ (i = 1, 2) is a 3 × 3 submatrix of $M_{fi}$ (i = 1, 2).

According to the principle of gear meshing, two gears will have a common contact point, which should satisfy that in the same coordinate system, the position vector of this meshing point is the same and the unit normal vector is also the same on both tooth faces. The above conditions can be represented by Equation (28).

$$\left\{\begin{array}{l}{f}_2(u_2,l_g,{\phi }_g,{\theta }_g)=0\\ {\stackrel{\rightharpoonup }{r}}_2^{(f)}\left(u_2,l_g,{\phi }_g,{\theta }_g,{\phi }_2\right)={\stackrel{\rightharpoonup }{r}}_1^{(f)}\left(u_1,l_1,{\phi }_1\right)\\ {\stackrel{\rightharpoonup }{n}}_2^{(f)}\left(u_2,l_g,{\phi }_g,{\theta }_g,{\phi }_2\right)={\stackrel{\rightharpoonup }{n}}_1^{(f)}\left(u_1,l_1,{\phi }_1\right)\end{array}\right.$$

(28)

where φ₂ is the rotation angle of the face gear; φ₁ is the rotation angle of the pinion.

Considering edge contact, when one of the pinion teeth exits meshing, the contact range is truncated from the original ellipse to a semi-ellipse, which is obviously smaller than the contact area in normal meshing, resulting in stress concentration. Currently, the coordinate system in which the two tooth surfaces are located is Sf, the distance between the tooth contact point and the coordinate origin is the same, and the tangent vector of the pinion’s tooth top at the contact point is perpendicular to the normal vector of the tooth surface of the face gear. When the pinion enters into meshing, there is also a truncation of the contact area, resulting in edge contact. By placing the two tooth surfaces in the same coordinate system S_f, the distance from the tooth contact point to the coordinate origin is the same, and the top edge tangent vector of the face gear is perpendicular to the tooth normal vector of the pinion.

Both cases can be represented by Equations (29) and (30).

$$\frac{\partial {\stackrel{\rightharpoonup }{r}}_1^{(f)}(u_1,l_1,{\phi }_1)}{\partial l_1}\cdot {\stackrel{\rightharpoonup }{n}}_2^{(f)}=0$$

(29)

$$\frac{\partial {\stackrel{\rightharpoonup }{r}}_2^{(f)}(u_2,l_g,{\phi }_g,{\theta }_g,{\phi }_2)}{\partial l_g}\cdot {\stackrel{\rightharpoonup }{n}}_1^{(f)}=0$$

(30)

where $\frac{\partial {\stackrel{\rightharpoonup }{r}}_1^{(f)}(u_1,l_1,{\phi }_1)}{\partial l_1}$ is the tangent vector of the edge of the crest of the pinion; $\frac{\partial {\stackrel{\rightharpoonup }{r}}_2^{(f)}(u_2,l_g,{\phi }_g,{\theta }_g,{\phi }_2)}{\partial l_g}$ is the tangent vector of the edge of the crest of the face gear; ${\stackrel{\rightharpoonup }{n}}_1^{(f)}$ is the normal vector of the tooth surface of pinion; ${\stackrel{\rightharpoonup }{n}}_2^{(f)}$ is the normal vector of the tooth surface of the face gear.

By solving the above equations, the contact point of the face gear can be determined.

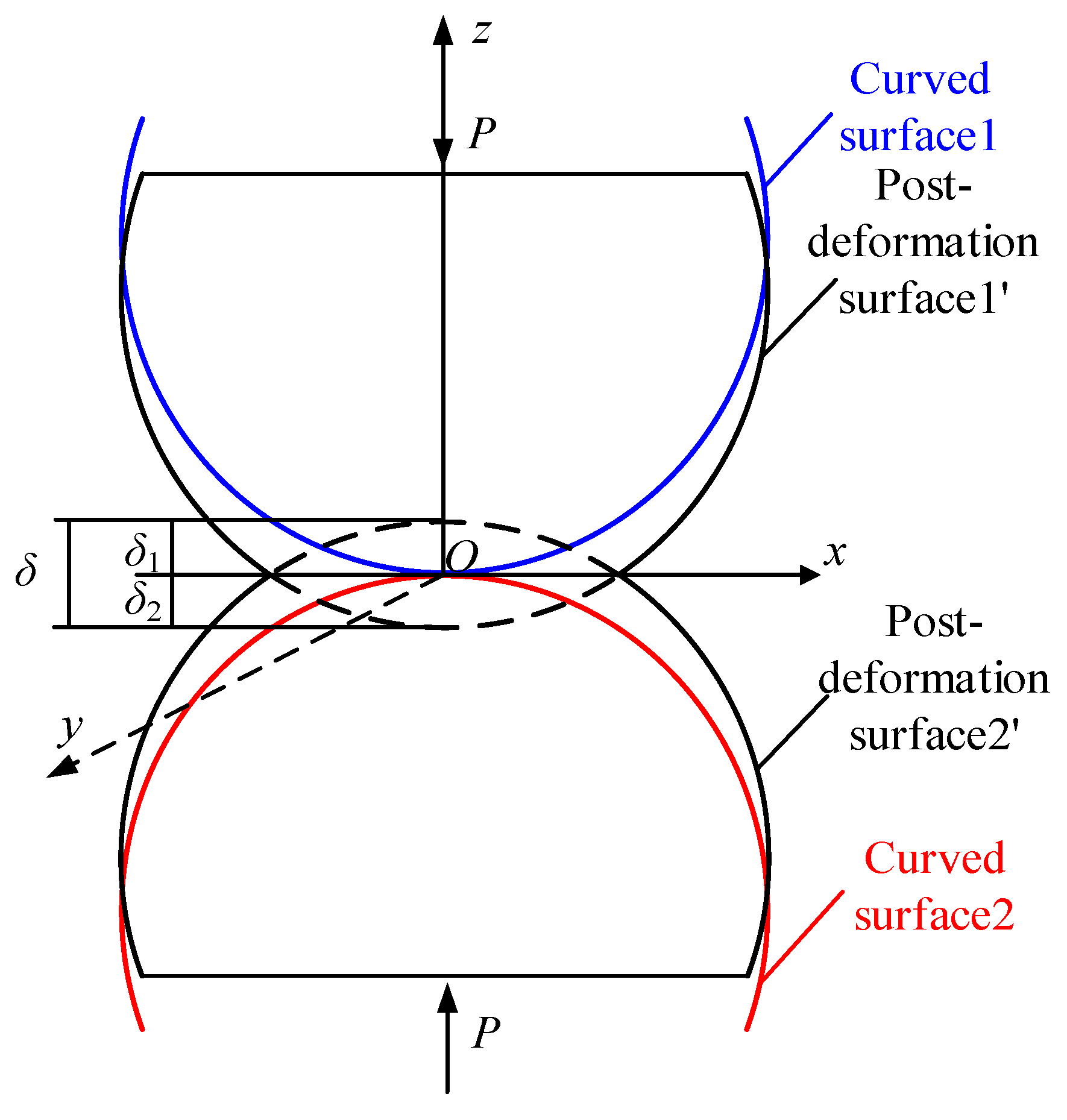
3.2. Calculation of Hertzian Contact Stress

According to the theory of contact mechanics, the contact location is deformed by the load, and the contact area expands outward into an ellipse with the contact point as the center. Among these, the ellipse’s center experiences the most deformation and contact stress, which gradually diminishes as it moves outward. The curvature of the position of the contact point affects the size of the elliptical contact region, and a corresponding digitizing program can be written to calculate the corresponding curvature.

The two gears in contact are deformed by the normal load and expand into an elliptical contact area at the original contact point O, according to the Hertzian elastic contact theory [36,37]. The contact deformation is shown in Figure 8, where δ₁ and δ₂ are the shape variables of surface 1 and surface 2, respectively.

$$\frac{B}{A}=\frac{\left({\mathrm{a}}^2/b^2\right)E\left(e\right)-K\left(e\right)}{K\left(e\right)-E\left(e\right)}$$

(31)

$$F_n=\frac{2}{3}{p}_0\pi ab$$

(32)

$$e={\left(1-\frac{b^2}{a^2}\right)}^{1/2}$$

(33)

$$\frac{1}{E^{*}}=\frac{1-v_1^2}{E_1}+\frac{1-v_2^2}{E_2}$$

(34)

$$p_0=\frac{1.5F_n}{\pi ab}$$

(35)

where F_n is the contact point’s normal load, a is the ellipse’s semi-professional axis; b is its semi-minor axis; e is the eccentricity of the ellipse, and K(e) and E(e) are the elliptic integrals of the first and second kinds, respectively; p₀ is the maximum contact stress.

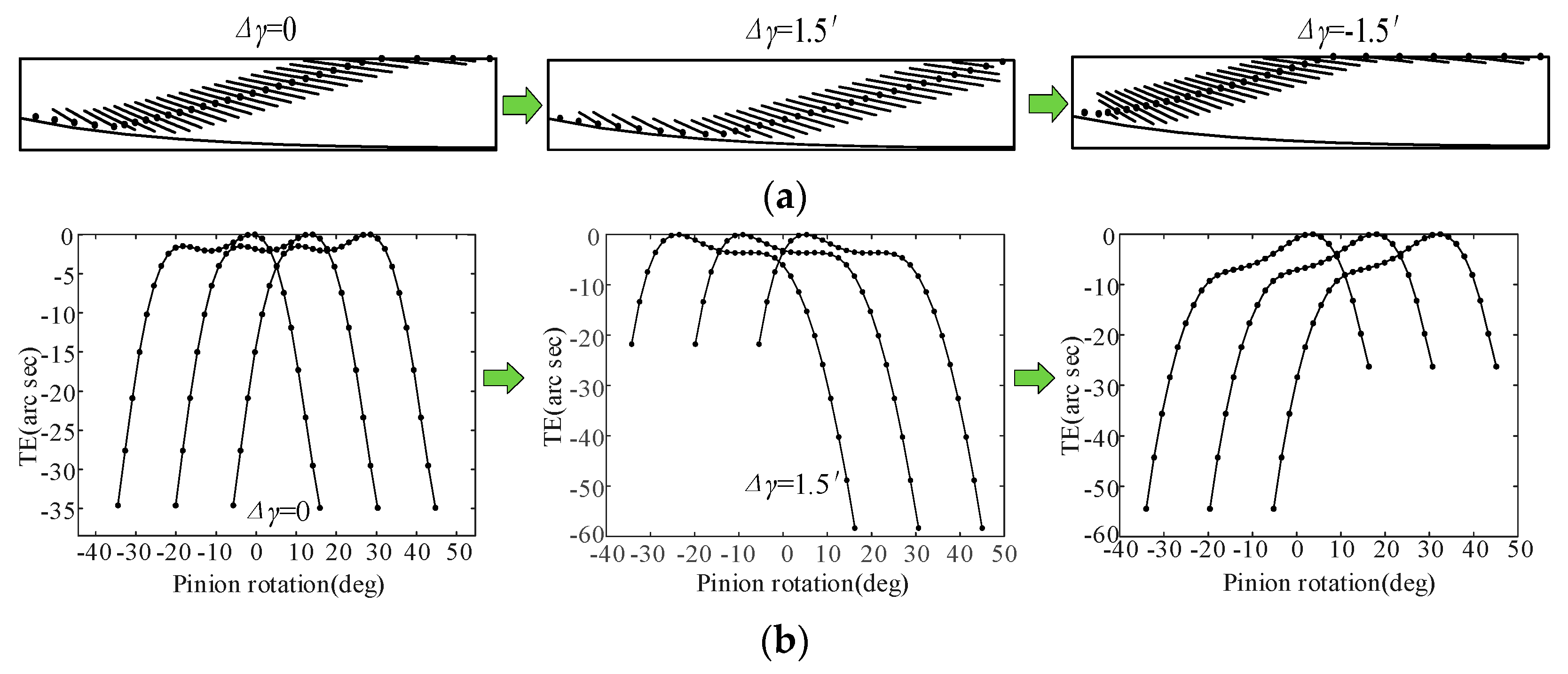
4. Designation of Intentional High-Order Transmission Error

In Figure 9, the transmission error (TE) is pre-designed as a sixth-order parabolic function. The x-coordinate and y-coordinate of the five given points are the rotation angle of the pinion and the transmission error of the face gear, respectively. The points P_A and P_E denote the positions of the gears entering and exiting meshing, respectively. The points P_B and P_D are the two peaks of the curve. The point P_C is the transition point in the middle, and the positional relationship between P_B, P_C, and P_D is determined by the two parameters of λ_B and λ_D. T_m denotes the meshing period of the gears.

The TE function of Figure 8 can be represented as Equation (35).

$$\left\{\begin{array}{l}\delta {\phi }_2=a_0+a_1{\phi }_1+a_2{\phi }_1^2+a_3{\phi }_1^3+a_4{\phi }_1^4+a_5{\phi }_1^5+a_6{\phi }_1^6=X{Y}^T\\ X=\left[a_0 a_1 a_2 a_3 a_4 a_5 a_6\right]\\ Y=\left[1 {\phi }_1 {\phi }_1^2 {\phi }_1^3 {\phi }_1^4 {\phi }_1^5 {\phi }_1^6\right]\end{array}\right.$$

(36)

where φ₁ is the rotation angle of the pinion; δφ₂ is the transmission error; a₀~a₆ are the coefficients of the HTE function.

Based on the coordinates of the five pre-designed points, the restriction equation of Equation (35) can be written as Equation (36).

$$\left\{\begin{array}{l}x={\phi }_{1A}, y=\delta {\phi }_{2A}\\ x={\phi }_{1B}, y=\delta {\phi }_{2B}\\ x={\phi }_{1B}, dy/dx=0\\ x={\phi }_{1C}, y=\delta {\phi }_{2C}\\ x={\phi }_{1D}, y=\delta {\phi }_{2D}\\ x={\phi }_{1D}, dy/dx=0\\ x={\phi }_{1E}, y=\delta {\phi }_{2E}\end{array}\right.$$

(37)

where

$$\left\{\begin{array}{l}{\phi }_{1C}=\frac{{\phi }_{1A}+{\phi }_{1E}}{2}\\ {\phi }_{1B}={\phi }_{1C}-{\lambda }_B{T}_m\\ {\phi }_{1D}={\phi }_{1C}+{\lambda }_D{T}_m\\ T_m=\frac{2\pi }{Z_1}\end{array}\right.$$

(38)

Equation (18) can be written in a matrix form via Equation (38).

$$AX=B$$

(39)

where

$$\left\{\begin{array}{l}A=\left[\begin{array}{l}1 {\phi }_{1A} {\left({\phi }_{1A}\right)}^2 {\left({\phi }_{1A}\right)}^3 {\left({\phi }_{1A}\right)}^4 {\left({\phi }_{1A}\right)}^5 {\left({\phi }_{1A}\right)}^6\\ 1 {\phi }_{1B} {\left({\phi }_{1B}\right)}^2 {\left({\phi }_{1B}\right)}^3 {\left({\phi }_{1B}\right)}^4 {\left({\phi }_{1B}\right)}^5 {\left({\phi }_{1B}\right)}^6\\ 0 1 2{\phi }_{1B} 3{\left({\phi }_{1B}\right)}^2 4{\left({\phi }_{1B}\right)}^3 5{\left({\phi }_{1B}\right)}^4 6{\left({\phi }_{1B}\right)}^5\\ 1 {\phi }_{1C} {\left({\phi }_{1C}\right)}^2 {\left({\phi }_{1C}\right)}^3 {\left({\phi }_{1C}\right)}^4 {\left({\phi }_{1C}\right)}^5 {\left({\phi }_{1C}\right)}^6\\ 1 {\phi }_{1D} {\left({\phi }_{1D}\right)}^2 {\left({\phi }_{1D}\right)}^3 {\left({\phi }_{1D}\right)}^4 {\left({\phi }_{1D}\right)}^5 {\left({\phi }_{1D}\right)}^6\\ 0 1 2{\phi }_{1D} 3{\left({\phi }_{1D}\right)}^2 4{\left({\phi }_{1D}\right)}^3 5{\left({\phi }_{1D}\right)}^4 6{\left({\phi }_{1D}\right)}^5\\ 1 {\phi }_{1E} {\left({\phi }_{1E}\right)}^2 {\left({\phi }_{1E}\right)}^3 {\left({\phi }_{1E}\right)}^4 {\left({\phi }_{1E}\right)}^5 {\left({\phi }_{1E}\right)}^6\end{array}\right]\\ B={\left[\delta {\phi }_{2A} \delta {\phi }_{2B} 0 \delta {\phi }_{2C} \delta {\phi }_{2D} 0 \delta {\phi }_{2E}\right]}^T\end{array}\right.$$

(40)

Then, coefficient vector X is calculated via Equation (40).

$$X=A^{-1}B$$

(41)

Figure 10 shows the traditional second-order parabolic transmission error. The TE function can be determined by substituting Equation (41) into Equation (40).

$$\left\{\begin{array}{l}A=\left[\begin{array}{l}1 {\phi }_{1A} {\left({\phi }_{1A}\right)}^2\\ 1 {\phi }_{1B} {\left({\phi }_{1B}\right)}^2\\ 1 {\phi }_{1C} {\left({\phi }_{1C}\right)}^2\end{array}\right]\\ B={\left[\delta {\phi }_{2A} \delta {\phi }_{2B} \delta {\phi }_{2C}\right]}^T\end{array}\right.$$

(42)

where

$${\phi }_{1B}=\frac{{\phi }_{1A}+{\phi }_{1C}}{2}$$

(43)

5. Numerical Examples and Discussions

Only the pinion is modified, herein. The design parameters of the examples of face gears are shown in

Table 1. Basic design parameters of a non-orthogonal helical face gear.

Parameter	Value
Pinion tooth number	25
Cutter tooth number	28
Face gear tooth number	160
Normal module (mm)	6.35
Pressure angle (degree)	25
Helix angle (degree)	15
Shaft angle (degree)	100
Inner radius (mm)	510
External radius (mm)	600

. In the following, “non-mod” (non-modification) indicates the results of a tooth surface without modification; “tra-mod” (traditional modification) indicates the results of a tooth surface using the traditional tooth modification method; “nov-mod” (novel tooth modification) indicates the results of a tooth surface using the novel modification method. The input torque was 105 Nm, which was applied to the pinion.

Figure 11 and Figure 12 shows the face gear tooth and pinion tooth models. Gear tooth face establishment is divided into left tooth face and right tooth face. The gear tooth profile parameters can be substituted into the tooth face equation to obtain the left tooth face, and the rack displacement direction and tooth profile parameters to obtain the opposite number can be processed to obtain the right tooth face of the gear. The steps for generating the tooth surface are as follows:

(1)

Parameter calculation. According to the tooth height of the gear shaping cutter, the tooth height parameter z₂ of the face gear in the coordinate system S₂ is derived;

(2)

Calculate the tooth width. Find the minimum inner diameter R₁ of the gear undercut and the maximum outer diameter R₂ without tooth tip sharpening, and select an appropriate tooth width within the range of R₁ and R₂ as the known quantity y₂;

(3)

Discrete y₂ and z₂. Through discretization, i discrete values of y_2i (y₂₁, y₂₂, y₂₃, …, y_2i) and j discrete values of z_2j (z₂₁, z₂₂, z₂₃, …, z_2j) are obtained; based on the y_2i and z_2j, which are used as the input values and substituted into the tooth surface equation, we can obtain i × j values of θ_Sij (θ_Si1, θ_Si2, θ_Si3, …, θ_Sij) and i × j values of φ_Sij (φ_Si1, φ_Si2, φ_Si3, …, φ_Sij);

(4)

Visualization of the working tooth surface. Back-substitute the i × j group (θ_Sij, φ_Sij) into the non-orthogonal asymmetric surface gear tooth surface equation to obtain i × j discrete coordinate points (x_ij, y_ij, z_ij) on the corresponding tooth surface, and apply MatLab instructions to generate work surfaces for gears with non-orthogonal faces.

Similar to the generation method of face gear tooth surfaces, cylindrical gears are also divided into left tooth surfaces and right tooth surfaces. Substitute the tooth shape parameters of the cylindrical gear into the equation, and then use the mesh statement of MATLAB software (2018b) to output the cylindrical gear mesh model, as shown in Figure 12.

5.1. Tooth Modification

Changes in the tooth flanks can often be reflected in the amount of tooth flank modification to study the changes in gear tooth flanks, first analyze the changes in the amount of tooth flank modification.

Figure 13 shows the modification amount of the cylindrical gear tooth surface under different modification methods. As shown in Figure 13a, the cylindrical gear using second-order modification has a medium convex shape, with a small amount of modification in the middle position, and a large amount of modification near the meshing in and meshing out positions. The overall modification amount transitions smoothly, but the uneven distribution of the modification amount on the tooth surface may have adverse effects. In Figure 13b, the cylindrical gear is modified according to the preset HTE. Different from the traditional convex TE, the HTE is concave, and the corresponding tooth surface modification amount is also concave. It can be clearly seen from the modification amount that the high-order modification design ensures a higher modification amount in the middle area, which can make the distribution of the modification amount on the tooth surface more reasonable. At the same time, it ensures that the stress distribution in the middle area of the gear surface is more uniform during meshing, avoiding stress concentration.

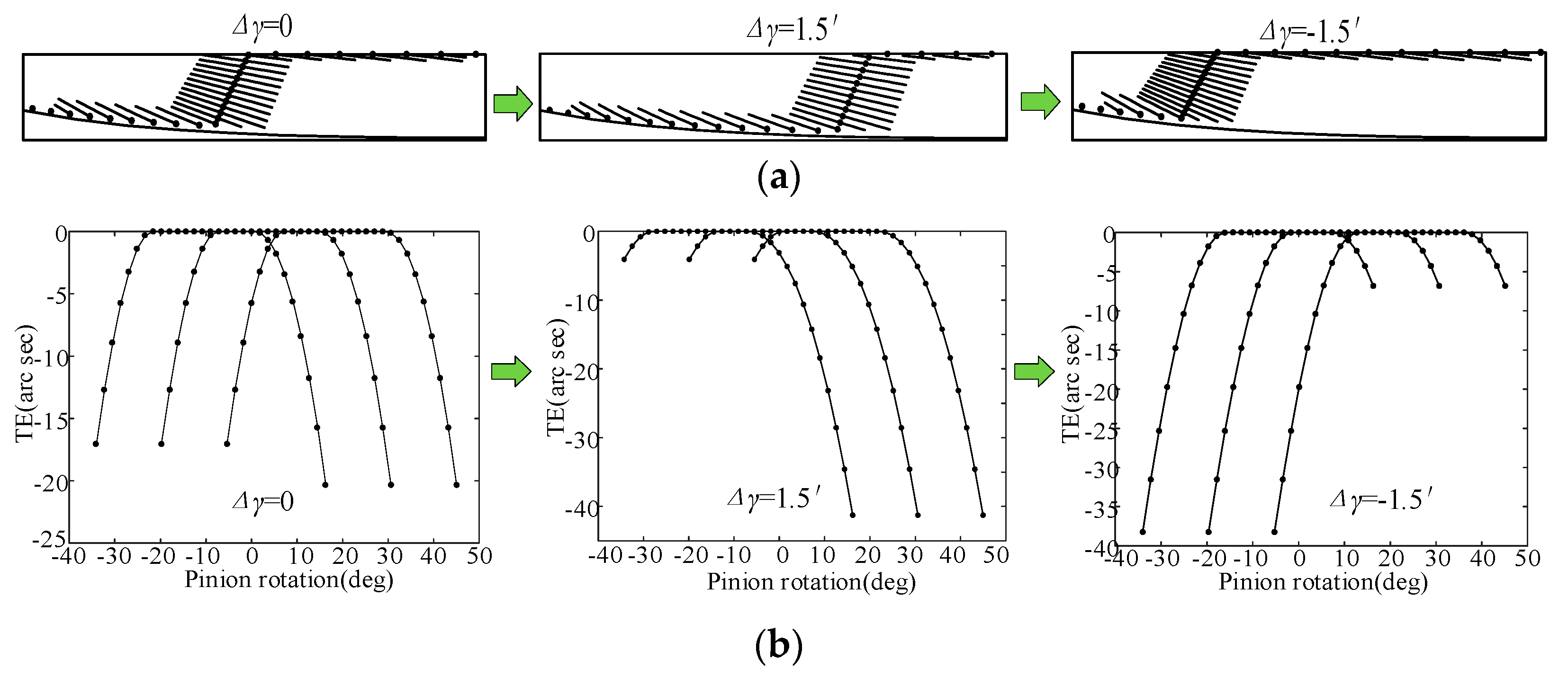
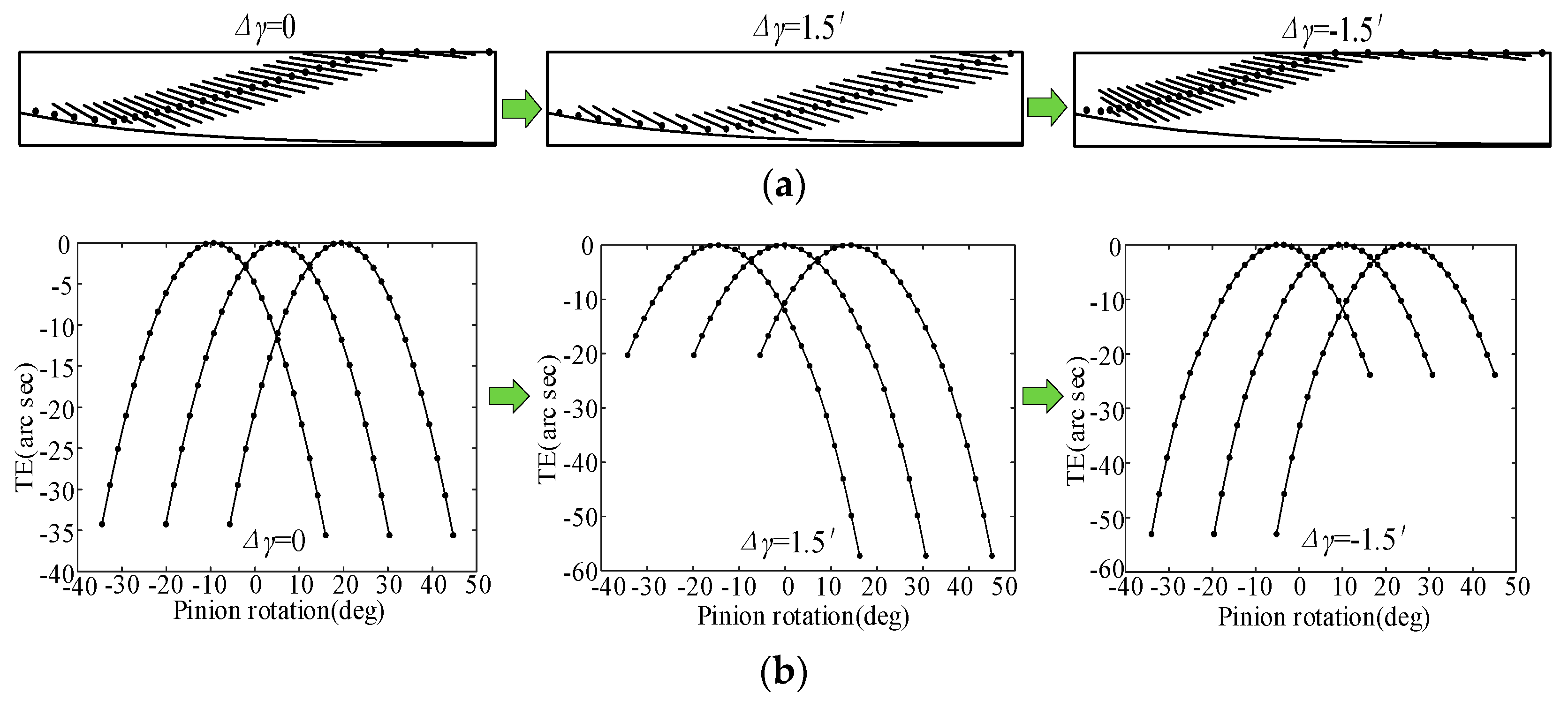
5.2. Tooth Contact Analysis

The contact pattern and the transmission error can be obtained from the TCA calculation. The contact pattern is the main characteristic target of the process of designing, machining, testing, and other related processes due to its intuitiveness, and it is also the key index to measure the transmission and meshing performance of the face gears.

As shown in Figure 14, the TCA calculation results of non-mod, tra-mod, and nov-mod without misalignment error are given. It can be found that the transmission error increases after tooth modification. Furthermore, there are more contact points on the tooth surface, which means that the contact ratio of the face gears increases after the tooth modification. In addition, after tooth modification, the original tooth surface meshing line contact is changed to point contact, so the error sensitivity is reduced. Figure 15 show simplified face gear and pinion transmission models, where Δq is the axial mounting error, ΔE is the offset mounting error, and Δγ is the misalignment error. The misalignment error has the most significant effect on the face gear TCA results; therefore, this paper focuses on the misalignment error. Figure 16, Figure 17 and Figure 18 show the variations in the contact pattern and transmission error with the misalignment error for non-mod, tra-mod, and nov-mod, respectively. Compared to the standard installation situation (Δγ = 0), when Δγ = 1.5′, the position of the tooth surface contact point of the face gear continues to shift toward the outer end position, the tooth top edge contact points decrease, the tooth root edge contact points increase, and the meshing position increases. The transmission error also increases significantly. When Δγ = −1.5′, the position of the tooth surface contact point continues to shift toward the inner end position, the tooth root edge contact points decrease, the tooth top edge contact points increase, and the transmission error at the meshing position also increases significantly.

However, judging from the calculation results of TCA alone, the advantages of the nov-mod are not shown. Therefore, further LTCA of face gears is required to further analyze the loaded meshing performance of the nov-mod.

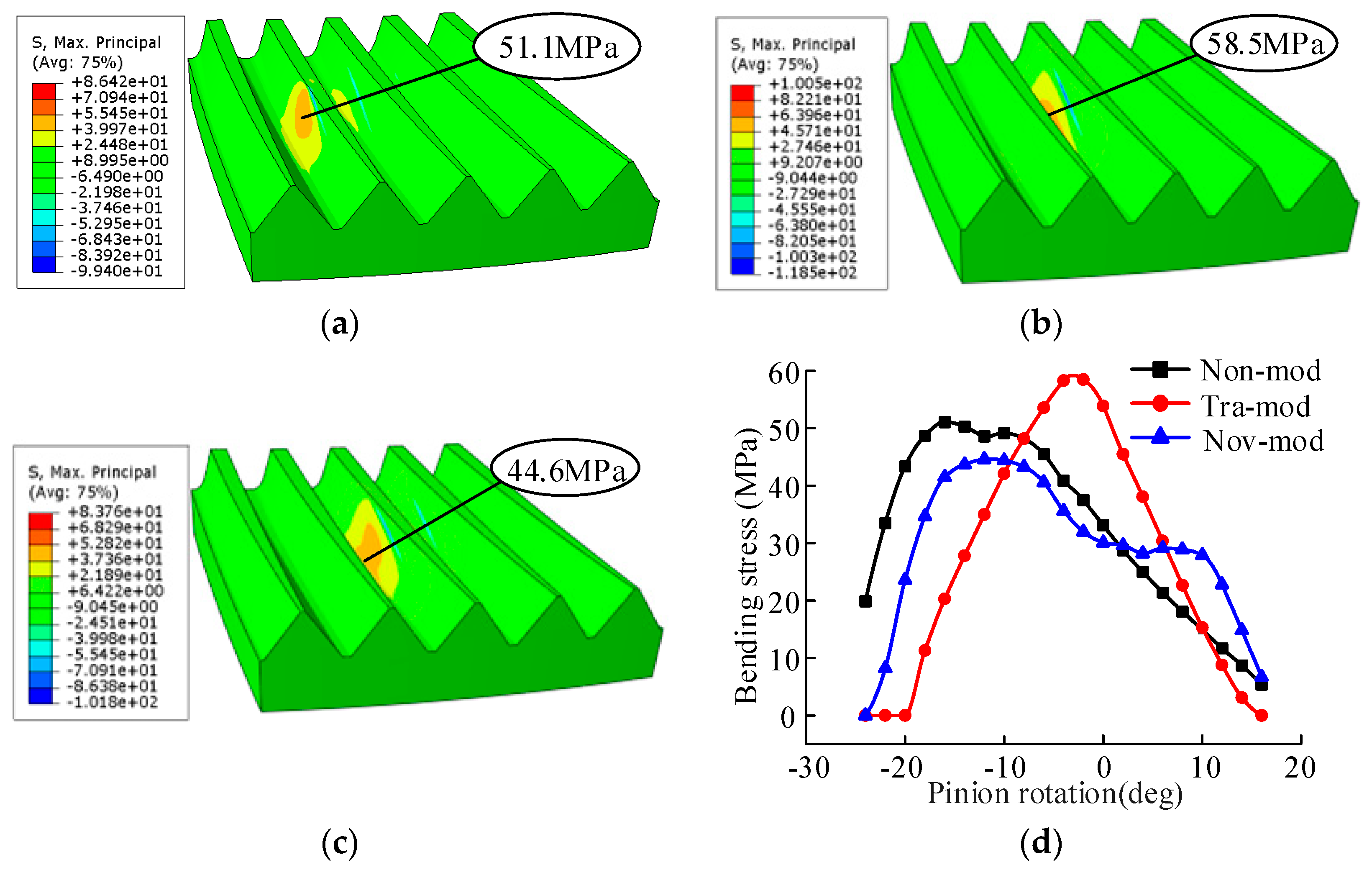
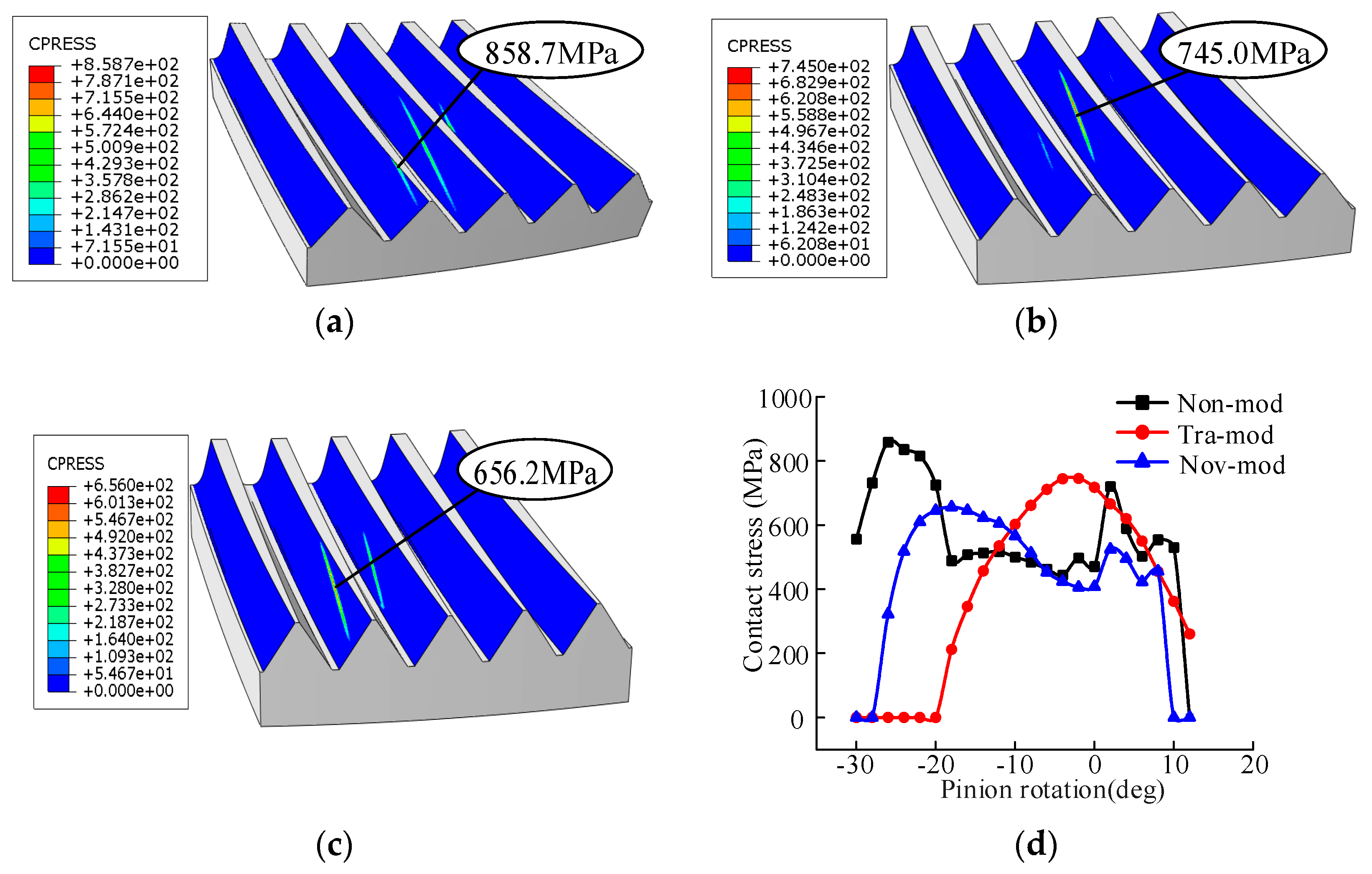
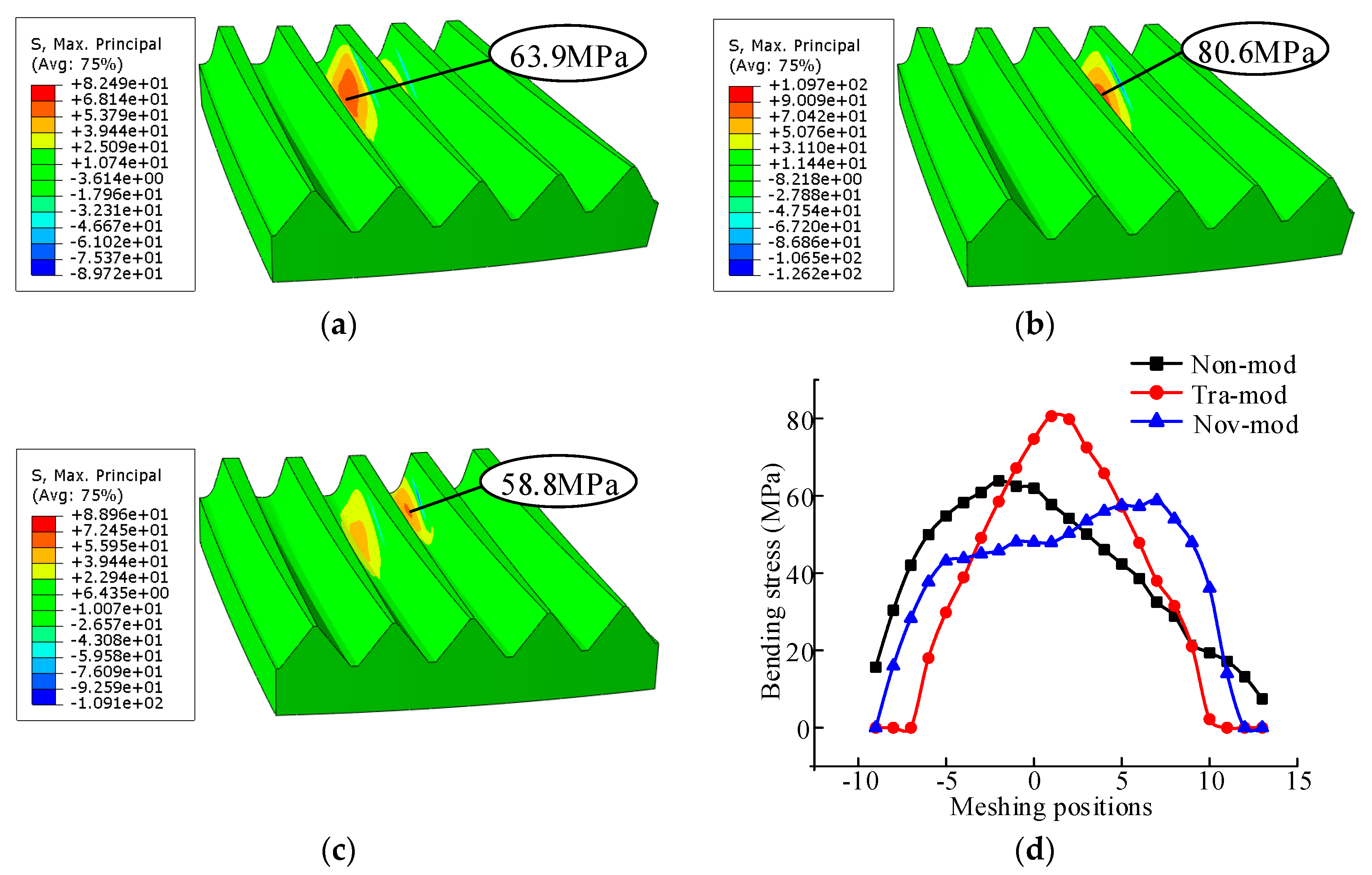
5.3. Loaded Tooth Contact Analysis

Using ABAQUS software (2021), we conducted a finite element simulation analysis on the non-orthogonal helical tooth surface gear transmission to obtain the contact stress and bending stress when the gear teeth are in contact, thereby judging the performance of the new method of cultivating the subsequent gear transmission. The magnitude of the force applied in this analysis was 1600N∙m.

Figure 19 shows the contact stress comparison of non-mod, tra-mod, and nov-mod without misalignment error. Figure 19a–c shows the contact stress nephograms of non-mod, tra-mod, and nov-mod at the position of the pitch circle. It can be seen from the stress nephogram that the contact stress increases after the tooth modification. This is because before tooth modification, the gears are in line contact, but after tooth modification, the gears become point contact, so the contact stress increases. However, it can also be seen that the contact stress of the nov-mod is smaller than that of the tra-mod. This is because the nov-mod makes the load on the tooth surface more consistent and improves the load distribution.

Figure 19d shows the contact stress comparison of the three types of tooth surfaces during the whole process from the gear teeth entering the mesh to exiting the mesh. It can be seen from the figure that when entering and exiting the mesh, the contact stress of the non-mod is relatively large because the contact line of the gears is relatively short in these two areas. The maximum contact stress of the non-mod is 890.7 MPa. After tooth modification, the center of the tooth surface experiences an increase in contact stress while the border of the tooth surface experiences a decrease in contact stress. Therefore, tooth modification is beneficial to gear transmission. However, it can also be seen in Figure 19d that the contact stress of the tra-mod in the middle area of the tooth surface increases sharply. The maximum contact stress of the tra-mod is 739.7 MPa. By comparing the contact stress of the nov-mod with that of the tra-mod, the advantages of the nov-mod are shown. The maximum contact stress of the nov-mod is 580.4 MPa. Compared to the tra-mod, the maximum contact stress of the nov-mod is reduced by 34.83%.

Figure 20 illustrates the bending stress comparison of non-mod, tra-mod, and nov-mod without misalignment error. Figure 20a–c shows the bending stress nephograms of non-mod, tra-mod, and nov-mod at the position of the pitch circle. It can be seen in the figures that the tooth modification also has a great influence on the bending stress of the gears. Tooth modification changes the load distribution on the tooth surface and then affects the bending stress of the gears. Figure 20d shows the contact stress comparison of the three types of tooth surfaces during the whole process from the gear teeth entering the mesh to exiting the mesh. In the figure, the maximum bending stresses of the non-mod, tra-mod, and nov-mod are 51.1 MPa, 58.5 MPa, and 44.6 MPa, respectively. Similar to the contact stress, additionally, the nov-mod’s bending stress is lower than the tra-mod’s, with a decrease of up to 12.72%. The maximum contact stress and maximum bending stress without misalignment errors are shown in

Table 2. Comparison of the maximum contact stress and maximum bending stresses without misalignment error.

Items	Contact Stress		Bending Stress
	Results (MPa)	Variation	Results (MPa)	Variation
Non-mod	890.7	—	51.1	—
Tra-mod	739.7	−16.95%	58.5	+14.48%
Nov-mod	580.4	−34.83%	44.6	−12.72%

.

It is well-known that errors in assembly and manufacturing are unavoidable in actual conditions. Therefore, the influence of the misalignment error on the meshing performance of face gears are studied below.

Figure 21 shows the contact stress comparison of non-mod, tra-mod, and nov-mod with misalignment error Δγ = 1.5′. Figure 21d shows the contact stress comparison of the three types of tooth surfaces during the whole process, from the gear teeth entering the mesh to exiting the mesh. The maximum contact stress of non-mod is 858.7 MPa. After tooth modification, the center of the tooth surface experiences an increase in contact stress, while the border of the tooth surface experiences a decrease in contact stress. Therefore, tooth modification is beneficial to gear transmission. However, it can also be seen from Figure 21d that the contact stress of the tra-mod in the middle area of the tooth surface increases sharply. The maximum contact stress of tra-mod is 745.0MPa. By comparing the contact stress of the nov-mod with that of the tra-mod, the advantages of the nov-mod are shown. The maximum contact stress of the nov-mod is 656.2 MPa. Compared to the tra-mod, the maximum contact stress of the nov-mod is reduced by 23.58%.

Figure 22 illustrates the bending stress comparison of non-mod, tra-mod, and nov-mod with misalignment error Δγ = 1.5′. Figure 22d shows the contact stress comparison of the three types of tooth surfaces during the whole process, from the gear teeth entering the mesh to exiting the mesh. In the figure, the maximum bending stresses of non-mod, tra-mod, and nov-mod are 56.4 MPa, 58.5 MPa, and 47.7 MPa, respectively. Similar to the contact stress, with a decrease of up to 15.42%, the bending stress of the nov-mod is also lower than that of the tra-mod. The maximum contact stress and maximum bending stress with misalignment error Δγ = 1.5′ are shown in

Table 3. Comparison of the maximum contact stress and maximum bending stresses with misalignment error, Δγ = 1.5′.

Items	Contact Stress		Bending Stress
	Results (MPa)	Variation	Results (MPa)	Variation
Non-mod	940.9	—	63.9	—
Tra-mod	774.2	−17.73%	80.6	+26.13%
Nov-mod	694.5	−26.24%	58.8	−7.98%

.

6. Conclusions

This paper proposes a new gear modification method to improve the load-bearing capacity of non-orthogonal helical gears and draws the following conclusions from the current research:

(1)

This paper proposes a new bidirectional gear modification method. The tooth modification is determined by the modified rack-cutter, and its feed motion is related to an intentionally designed transmission error. The novelty of the tooth modification design is that the transmission error can be predesigned.

(2)

The performance of the introduced novel tooth modification is studied through TCA and LTCA. Under the non-misalignment error working conditions, the contact stress and bending stress of the novel tooth modification decrease by as much as 34.83% and 12.72%, which shows better meshing performance compared to the traditional tooth modification.

(3)

Under the misalignment error working conditions, the contact stress and bending stress of the novel tooth modification decrease by as much as 26.24% and 7.98%. The introduced new gear modification method has lower tooth profile contact stress and tooth root bending stress both with and without misalignment errors.

(4)

The introduced novel tooth modification in this paper is universal, and not limited to face gears but can be extended to other types of gears.