Modal Balancing of Warped Rotors without Trial Runs Using the Numerical Assembly Technique
Abstract
1. Introduction
- Investigation of the optimal adaption of the model-based modal balancing procedure for warped rotors.
- Adaption of the procedure to measurements outside of critical speeds.
- Experimental verification of the proposed procedure.
2. Materials and Methods
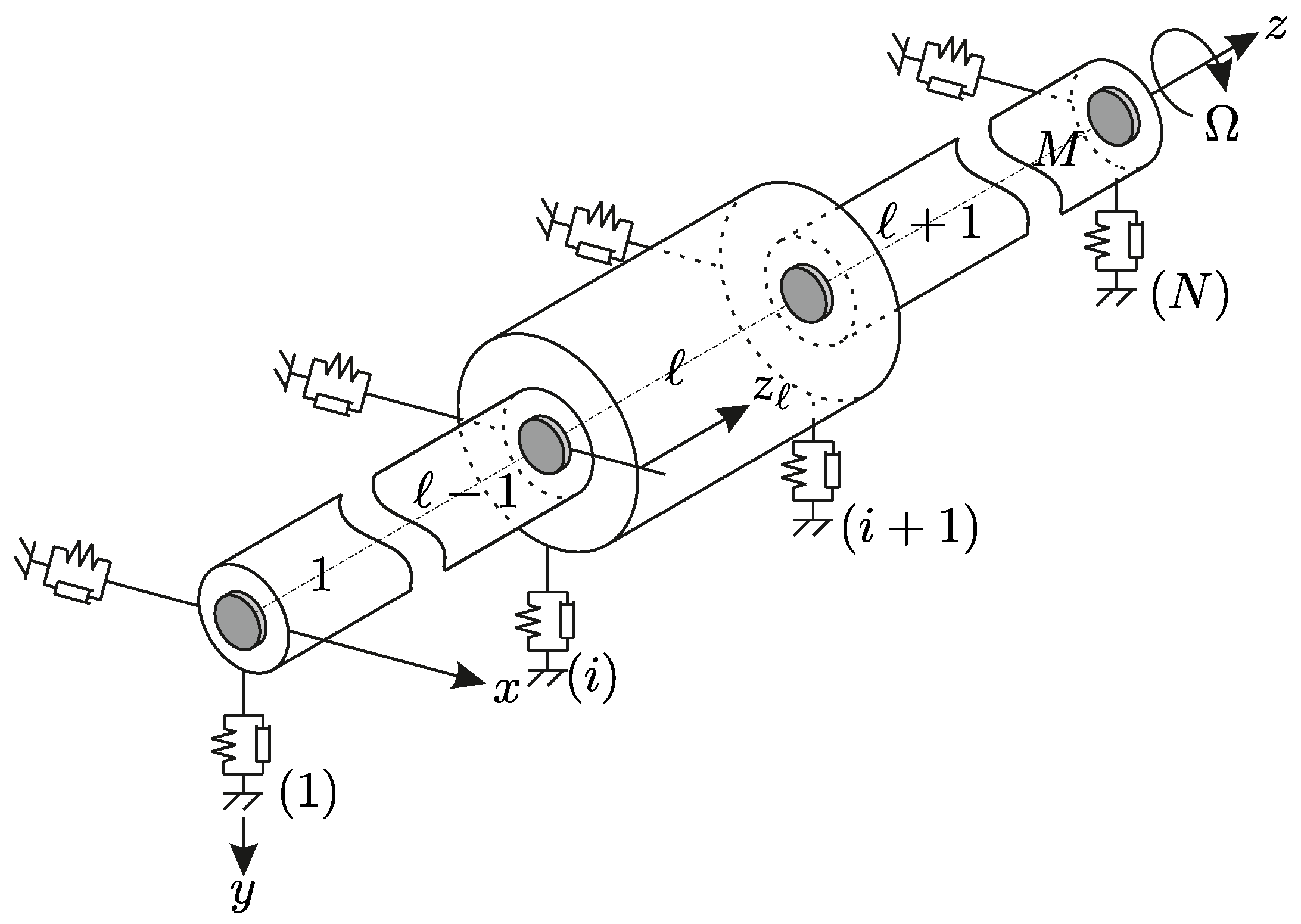
2.1. Numerical Assembly Technique
2.1.1. Characteristic Equations
2.1.2. Homogeneous Solution
2.1.3. Boundary and Interface Conditions
2.1.4. Particular Solution
2.1.5. Assembly and Solution Procedure
2.2. Modal Balancing Method
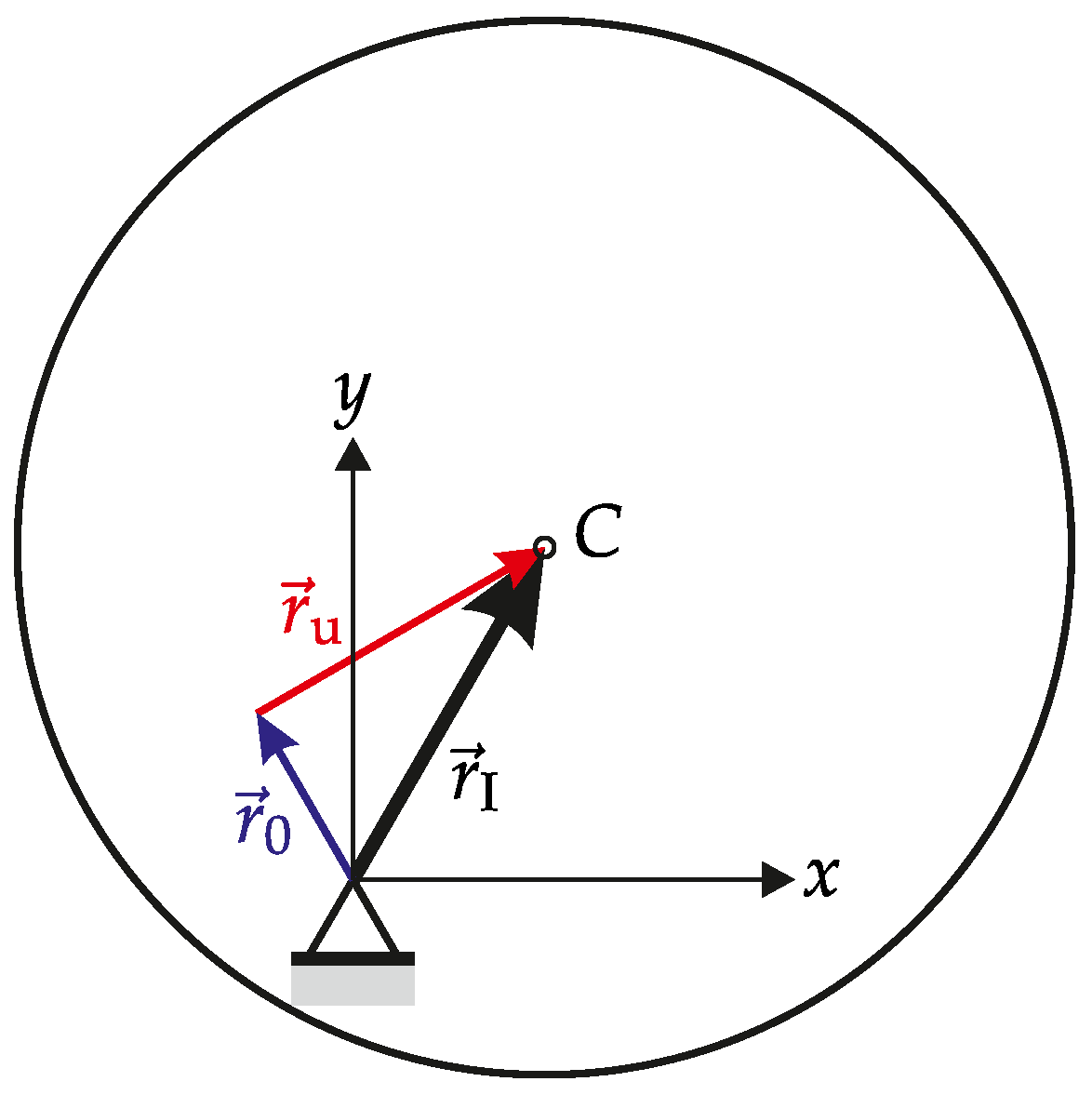
2.3. Theory of Bow Compensation
3. Results and Discussion
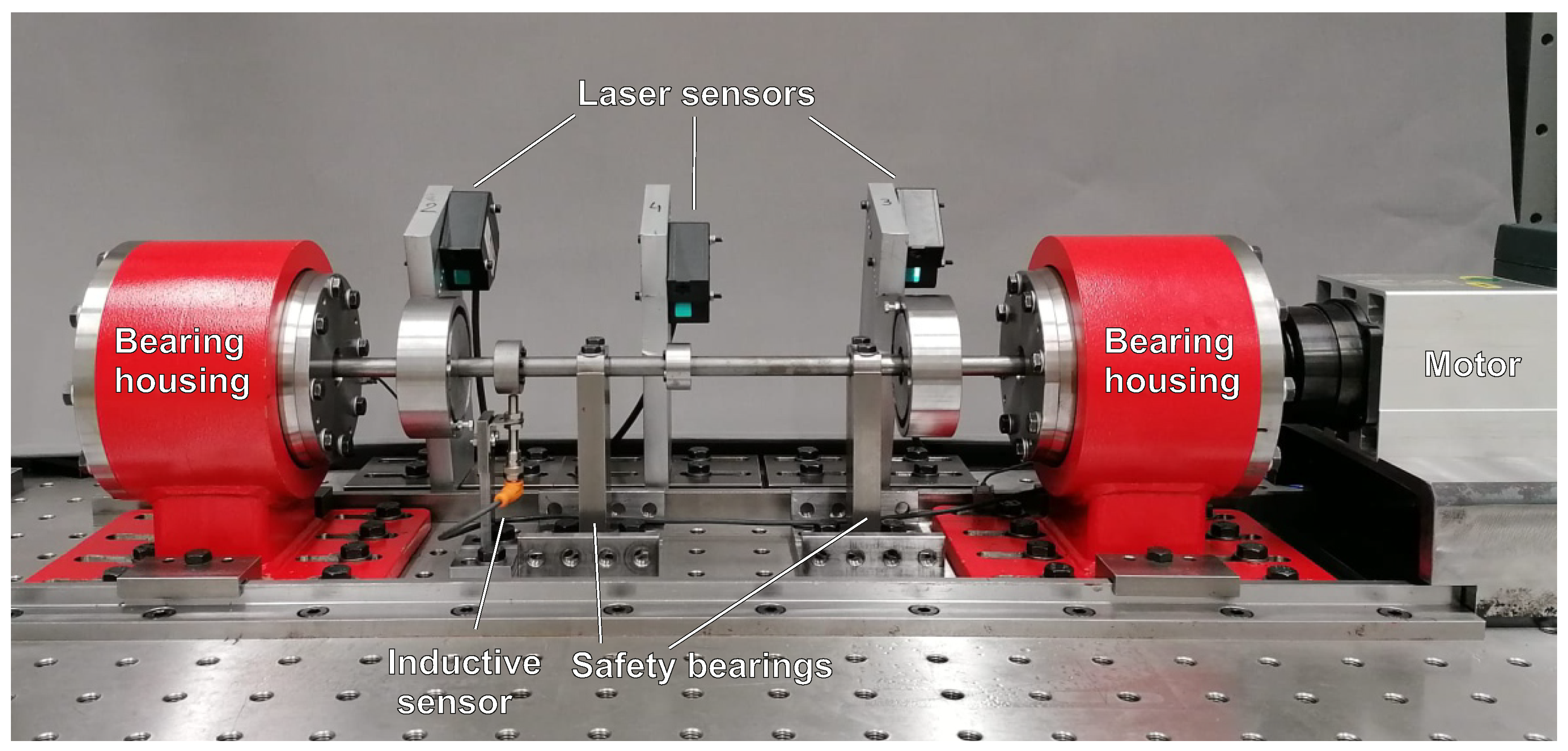
3.1. Test Bed
3.2. Rotor Model
- Density: 7700 ;
- Shear modulus: ;
- Shear correction factor: 0.89;
- External damping coefficient: 45 .
- : ;
- : ;
- : .
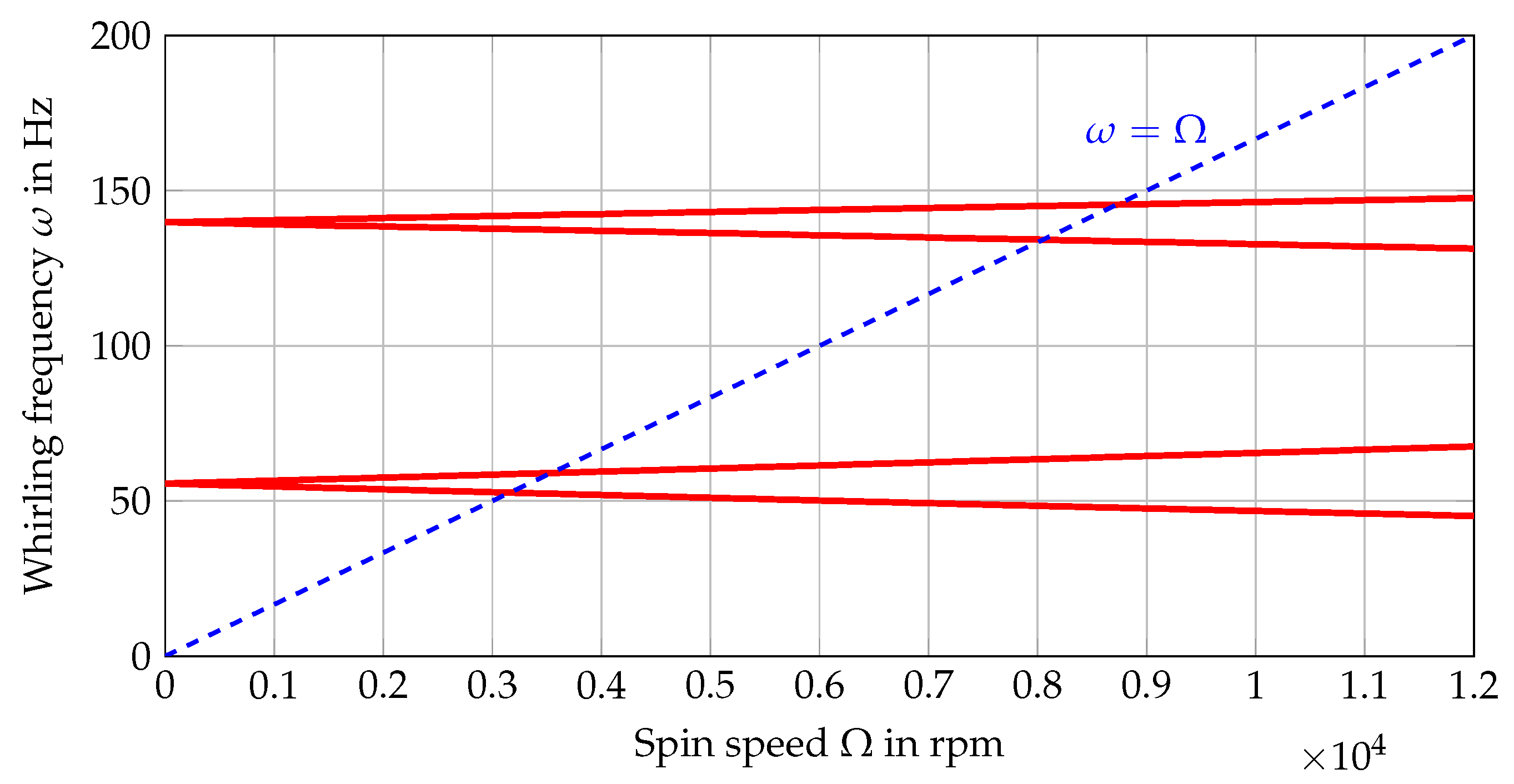
3.3. Numerical Analysis
- Unknown parameters, especially the influence of the foundation and the coupling.
- Warped shafts.
- Measurement and mounting errors.
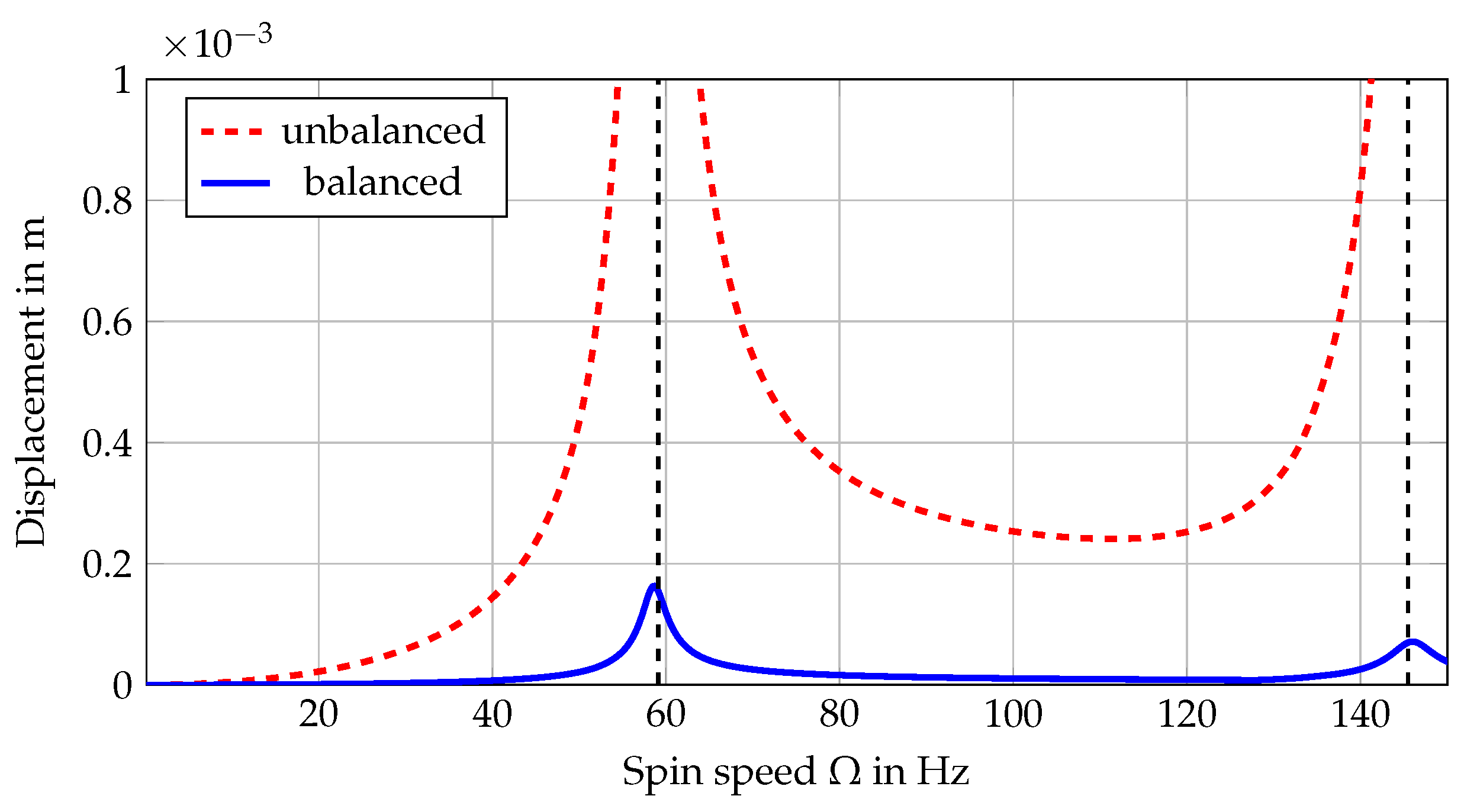
3.4. Investigation of Optimal Bow Compensation
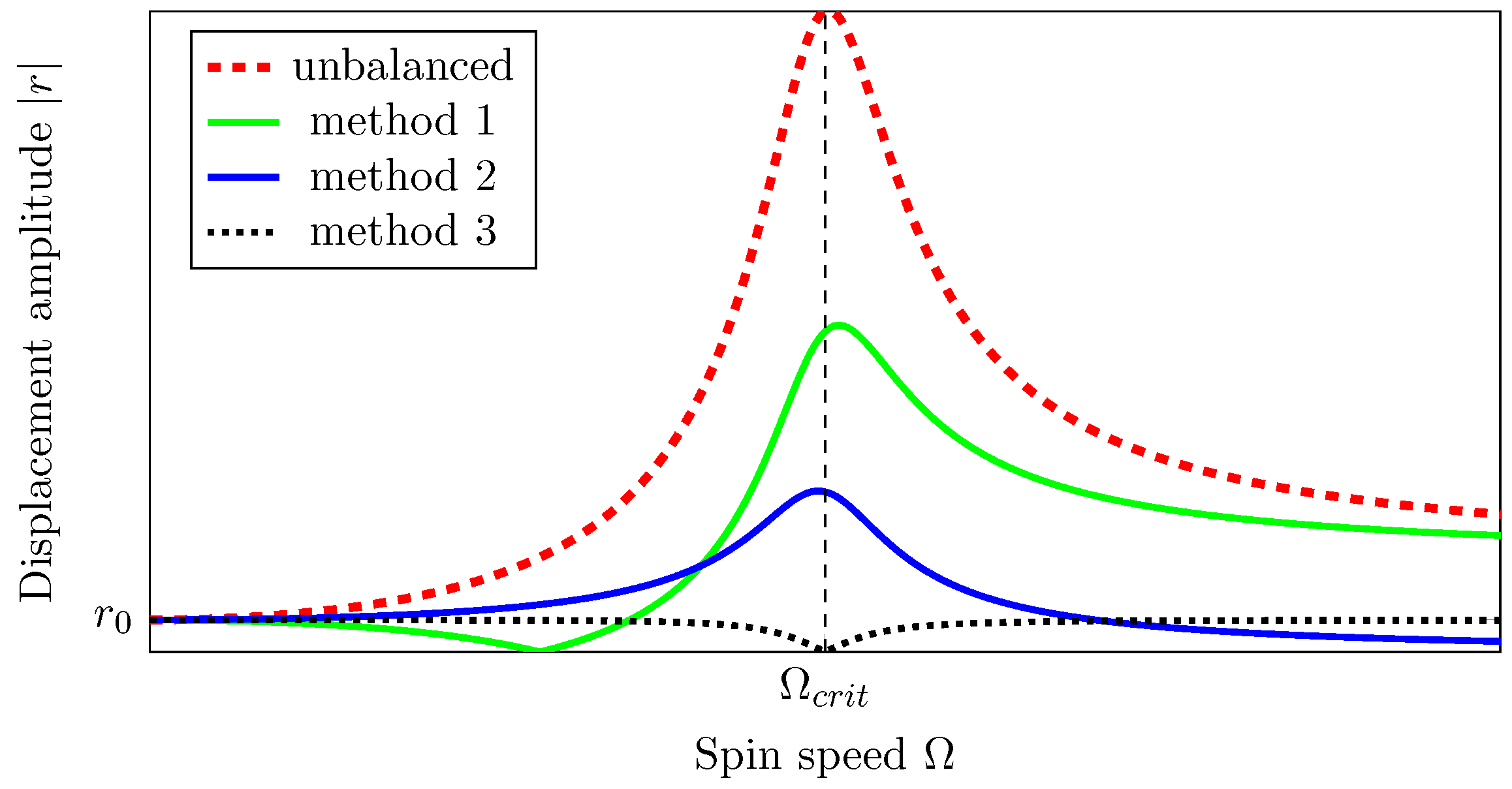
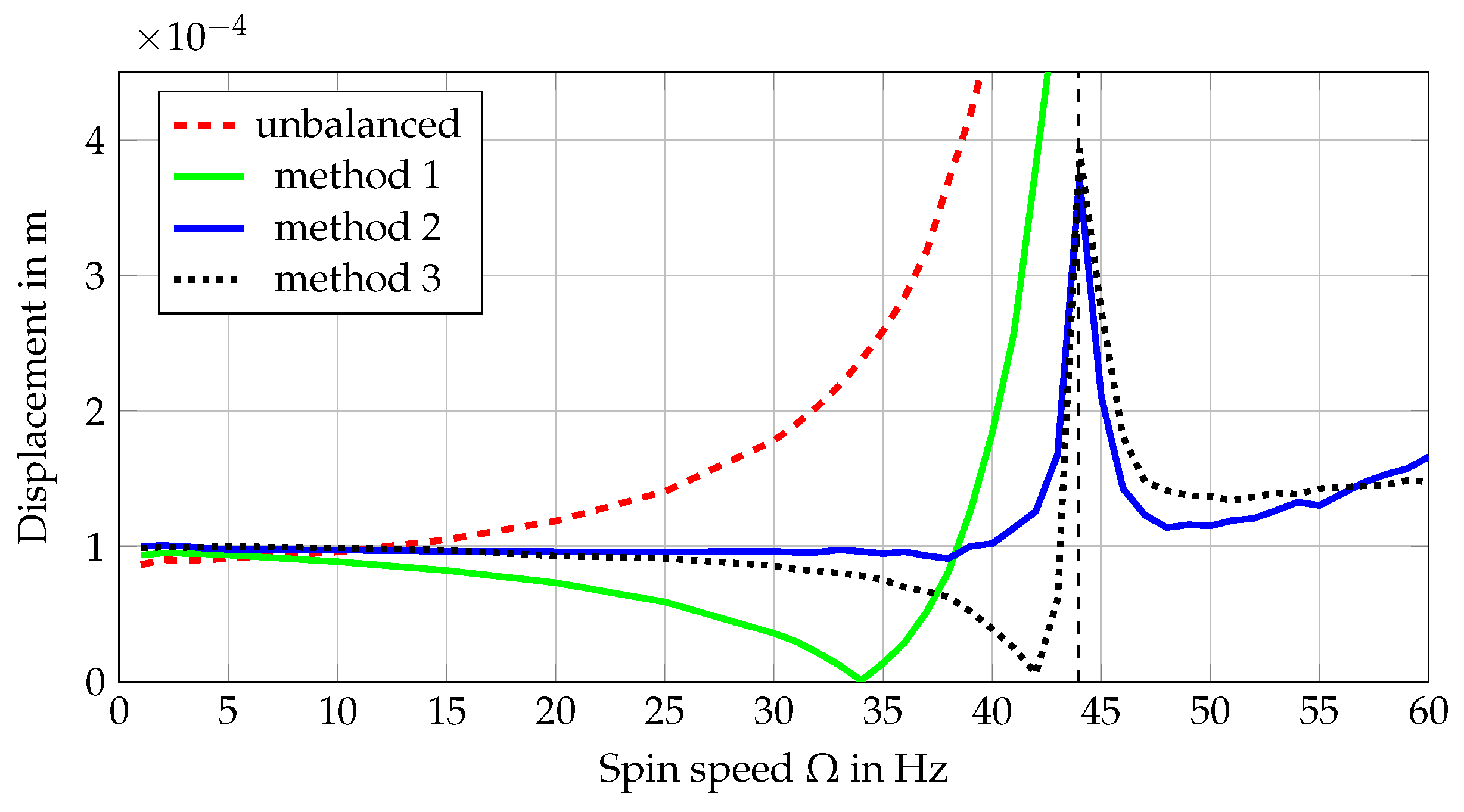
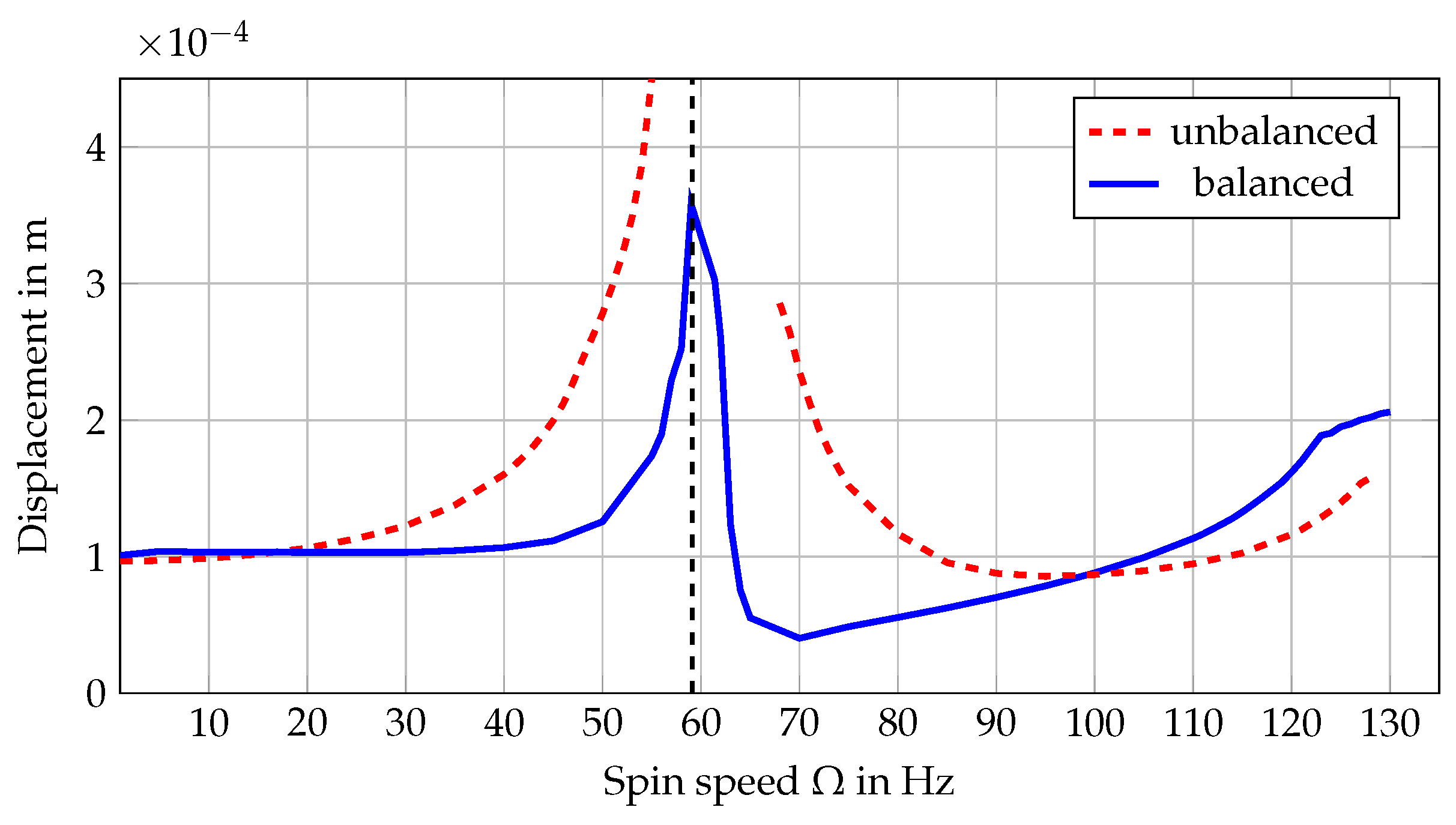
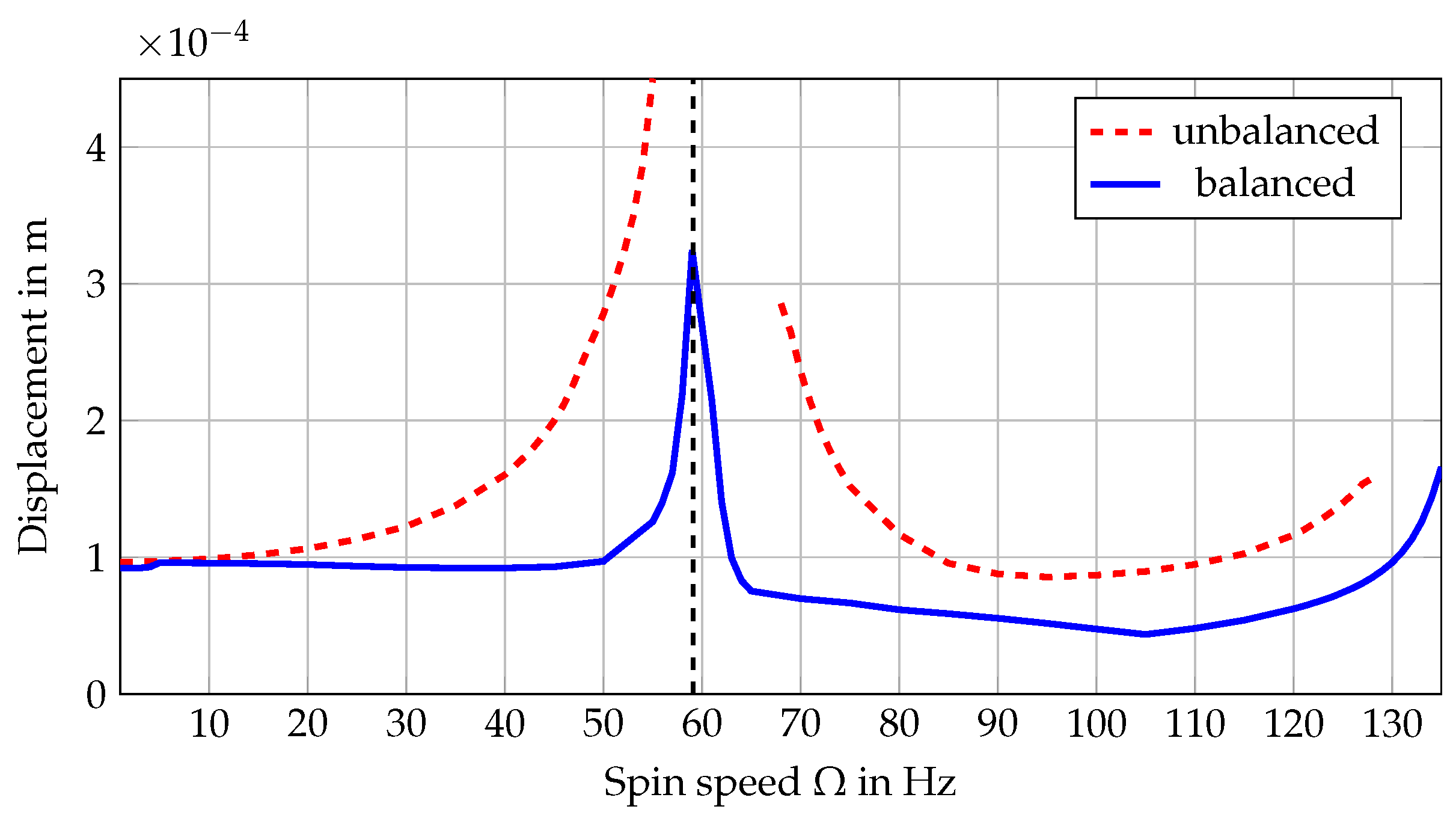
3.5. Balancing of the First Mode of the Multi-Disk System
3.6. Balancing of the First and Second Mode of the Multi-Disk System
3.7. Discussion
- Despite the unknown foundation parameters, the eigenfrequencies were accurately predicted. In this test case, the shaft was substantially more flexible than the bearings and bearing foundations, reducing their influence. Further research could extend NAT with detailed foundation behaviour and investigate systems on elastic supports. Also, the balancing on rotors supported on fluid film bearings using NAT has only been theoretically presented [33], but has not yet been experimentally verified.
- The fractional time derivative damping model depicted the behaviour of the shaft correctly. Since the shaft is made out of steel, which shows very low viscoelastic properties, a less sophisticated material model would suffice. The applied material model in combination with NAT has already been used to calculate eigenfrequencies of strongly viscoelastic materials like Polyvinylchlorid [37], suggesting that the balancing of rotors made out of these materials could also be possible. This should be verified experimentally.
- Although NAT assumes a straight shaft, the vibration amplitudes of the first two modes of a warped rotor were successfully reduced using the presented procedure. Since the pre-bend of the test bed is significantly higher than the usually accepted values for high-speed machinery, it is inferred that a slight bow of the shaft poses no problem for the method.
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| FEM | Finite element method |
| NAT | Numerical assembly technique |
| rpm | Rotations per minute |
References
- Bishop, R.E.D.; Gladwell, G.M.L. The Vibration and Balancing of an Unbalanced Flexible Rotor. J. Mech. Eng. Soc. 1959, 1, 66–77. [Google Scholar] [CrossRef]
- Thearle, E.L. Dynamic Balancing of Rotating Machinery in the Field. Trans. ASME 1934, 56, 745–753. [Google Scholar] [CrossRef]
- Tessarzik, J.M.; Badgley, R.H.; Anderson, W.J. Flexible Rotor Balancing by the Exact Point-Speed Influence Coefficient Method. J. Eng. Ind. 1972, 94, 145–158. [Google Scholar] [CrossRef]
- Nicholas, J.C.; Gunter, E.J.; Allaire, P.E. Effect of Residual Shaft Bow on Unbalance Response and Balancing of a Single Mass Flexible Rotor—Part II: Balancing. J. Eng. Power 1976, 98, 171–181. [Google Scholar] [CrossRef]
- Gnielka, P. Modal balancing of flexible rotors without test runs: An experimental investigation. J. Vib. 1982, 90, 152–170. [Google Scholar] [CrossRef]
- Federn, K. Grundlagen Einer Systematischen Schwingungsentstorung Wellenelastischer Rotoren. VDI-Bericht 1957, 24, 9. [Google Scholar]
- Parkinson, A.G.; Bishop, R.E.D. Residual vibration in modal balancing. J. Mech. Eng. Sci. 1965, 7, 33–39. [Google Scholar] [CrossRef]
- Kellenberger, W. Das Wuchten elastischer Rotoren auf zwei allgemeinelastischen Lagern. Brown Boveri Mitteilungen 1967, 54, 603–617. [Google Scholar]
- Darlow, M.; Smalley, A.; Parkinson, A. Demonstration of a unified approach to the balancing of flexible rotors. J. Eng. Power 1981, 103, 101–107. [Google Scholar] [CrossRef]
- Zhao, S.; Ren, W.; Deng, W.; Lu, K.; Yang, Y.; Fu, C. A transient characteristic-based balancing method of rotor system without trail weights. Mech. Syst. Signal Process. 2021, 1487, 107117. [Google Scholar] [CrossRef]
- Goldman, P.; Muszynska, A. Application of full spectrum to rotating machinery diagnostics. Orbit 1999, 20, 17–21. [Google Scholar]
- Deepthikumar, M.B.; Sekhar, A.S.; Srikanthan, M.R. Balancing of flexible rotor with bow using transfer matrix method. J. Vib. Control 2014, 20, 225–240. [Google Scholar] [CrossRef]
- Green, K.; Champneys, A. Bifurcation analysis of an automatic dynamic balancing mechanism for eccentric rotors. J. Sound Vib. 2006, 291, 861–881. [Google Scholar] [CrossRef]
- Nauclér, P.; Söderström, T. Unbalance estimation using linear and nonlinear regression. Automatica 2010, 46, 1752–1761. [Google Scholar] [CrossRef]
- Yun, X.; Mei, X.; Jiang, G.; Hu, Z.; Zhang, Z. Investigation on a no trial weight spray online dynamic balancer. Shock Vib. 2018, 2018, 7021215. [Google Scholar] [CrossRef]
- Carvalho, V.N.; Dourado, A.D.; Rende, B.R.; Cavalini, J.A.A.; Steffen, J.V. Experimental validation of a robust model-based balancing approach. J. Vib. Control 2019, 25, 423–434. [Google Scholar] [CrossRef]
- Nordmann, R.; Knopf, E.; Abrate, B. Numerical analysis of influence coefficients for on-site balancing of flexible rotors. In Proceedings of the 10th International Conference on Rotor Dynamics, Rio de Janeiro, Brazil, 23–27 September 2018; pp. 157–172. [Google Scholar]
- Nordmann, R.; Knopf, E.; Krueger, T. Balancing of flexible rotors by means of calculated influence coefficients. In Proceedings of the SIRM 2021 International Conference on Dynamics of Rotating Machinery, Gdansk, Poland, 17–19 February 2021; pp. 1–13. [Google Scholar]
- Wu, J.-S.; Chou, H.M. A new approach for determining the natural frequency of mode shapes of a uniform beam carrying any number of sprung masses. J. Sound Vib. 1999, 220, 451–468. [Google Scholar] [CrossRef]
- Wu, J.-S.; Chen, D.-W. Free vibration analysis of a Timoshenko beam carrying multiple spring masses by using the numerical assembly technique. Int. J. Numer. Methods Eng. 2001, 50, 1039–1058. [Google Scholar] [CrossRef]
- Chen, D.-W. The exact solutions for the natural frequencies and mode shapes of non-uniform multi-span beamscarrying multiple various concentrated elements. Struct. Eng. Mech. Int. J. 2003, 16, 153–176. [Google Scholar] [CrossRef]
- Chen, D.-W. The exact solutions for free vibration of uniform beams carrying multiple two-degree-of-freedom spring-mass systems. J. Sound Vib. 2006, 295, 342–361. [Google Scholar] [CrossRef]
- Lin, H.-Y.; Tsai, Y.-C. On the natural frequencies and mode shapes of a multi-step beam carrying a number of intermediate lumped masses and rotary inertias. Struct. Eng. Mech. Int. J. 2006, 22, 701–717. [Google Scholar] [CrossRef]
- Lin, H.-Y.; Tsai, Y.-T. Free vibration analysis of a uniform multi-span beam carrying multiple spring-mass systems. J. Sound Vib. 2007, 302, 442–456. [Google Scholar] [CrossRef]
- Wang, J.-R.; Liu, T.-L.; Chen, D.-W. Free vibration analysis of a Timoshenko beam carrying multiple sping mass systems with the effect of shear deformation and rotary inertia. Struct. Eng. Mech. Int. J. 2007, 26, 1–14. [Google Scholar] [CrossRef]
- Yesilce, Y.; Demirdag, O. Effect of axial force on free vibration of Timoshenko multi-span beam carrying multiple spring-mass systems. Int. J. Mech. Sci. 2008, 50, 995–1003. [Google Scholar] [CrossRef]
- Yesilce, Y. Free Vibrations of a Reddy-Bickford Multi-span Beam Carrying Multiple Spring-mass Systems. J. Shock Vib. 2011, 18, 709–726. [Google Scholar] [CrossRef][Green Version]
- Wu, J.-S.; Lin, F.-T.; Shaw, H.-J. Analytical Solution for Whirling Speeds and Mode Shapes of a Distributed-Mass Shaft with Arbitrary Rigid Disks. J. Appl. Mech. 2014, 81, 034503. [Google Scholar] [CrossRef] [PubMed]
- Farghaly, S.H.; El-Sayed, T.A. Exact free vibration of a multi-step Timoshenko beam system with several attachments. Mech. Syst. Signal Process. 2016, 72, 525–546. [Google Scholar] [CrossRef]
- Vaz, J.D.C.; de Lima, J.J., Jr. Vibration anaysis of Euler–Bernoulli beams in multiple steps and different shapes of cross section. J. Vib. Control 2016, 22, 193–204. [Google Scholar] [CrossRef]
- Klanner, M.; Prem, M.S.; Ellermann, K. Steady-state harmonic vibrations of a linear rotor- bearing system with a discontinuous shaft and arbitrarily distributed mass unbalance. In Proceedings of the ISMA2020 International Conference on Noise and Vibration Engineering and USD2020 International Conference on Uncertainty in Structural Dynamics, Leuven, Belgium, 7–9 September 2020; pp. 1257–1272. [Google Scholar]
- Quinz, G.; Prem, M.S.; Klanner, M.; Ellermann, K. Balancing of a linear elastic rotor-bearing system with arbitrarily distributed unbalance using the Numerical Assembly Technique. Bull. Pol. Acad. Sci. Tech. Sci. 2021, 69, e138237. [Google Scholar]
- Quinz, G.; Klanner, M.; Ellermann, K. Balancing of Flexible Rotors Supported on Fluid Film Bearings by Means of Influence Coefficients Calculated by the Numerical Assembly Technique. Energies 2022, 15, 2009. [Google Scholar] [CrossRef]
- Quinz, G.; Überwimmern, G.; Klanner, M.; Ellermann, K. Field balancing of flexible rotors without trial runs using the Numerical Assembly Technique. In Proceedings of the 15th International Conference on Dynamics of Rotating Machinery, Darmstadt, Germany, 22–24 February 2023. [Google Scholar]
- Bauchau, O.A.; Craig, J.I. Structural Analysis—With Applications to Aerospace Structures; Springer: Berlin/Heidelberg, Germany, 2009. [Google Scholar]
- Pritz, T. Analysis of four-parameter fractional derivative model of real solid materials. J. Sound Vib. 1996, 195, 103–115. [Google Scholar] [CrossRef]
- Prem, M.S.; Klanner, M.; Ellermann, K. Model parameter estimation of ball bearings using generalized Polynomial Chaos Expansion. In Proceedings of the SIRM 2021 International Conference on Dynamics of Rotating Machinery, Gdansk, Poland, 17–19 February 2021; pp. 331–340. [Google Scholar]
- Davies, B. Integral Transforms and Their Applications; Springer Science and Business Media: Berlin/Heidelberg, Germany, 2002; Volume 41. [Google Scholar]
- Hayek, S.I. Advanced Mathematical Methods in Science and Engineering; CRC Press: Boca Raton, FL, USA, 2000. [Google Scholar]
- Mitrinovic, D.S.; Keckic, J.D. The Cauchy Method of Residues: Theory and Applications; Springer Science and Business Media: Berlin/Heidelberg, Germany, 1984; Volume 9. [Google Scholar]
- Rao, J.S. Dynamic analysis of bowed rotors. In Proceedings of the VETOMAC-1 Conference, Bangalore, India, 25–27 October 2000. [Google Scholar]
- Deepthikumar, M.B.; Sekhar, A.S.; Srikanthan, M.R. Modal balancing of flexible rotors with bow and distributed unbalance. J. Sound Vib. 2013, 332, 6216–6233. [Google Scholar] [CrossRef]
- Sanches, F.D.; Dalmazzo, F. Simultaneous identification of unbalance and shaft bow in a two-disk rotor based on correlation analysis and the SEREP model order reduction method. J. Sound Vib. 2018, 433, 230–247. [Google Scholar] [CrossRef]
- Ehrich, F.F. Handbook of Rotordynamics; Krieger Publishing Company: Malabar, FL, USA, 1999. [Google Scholar]
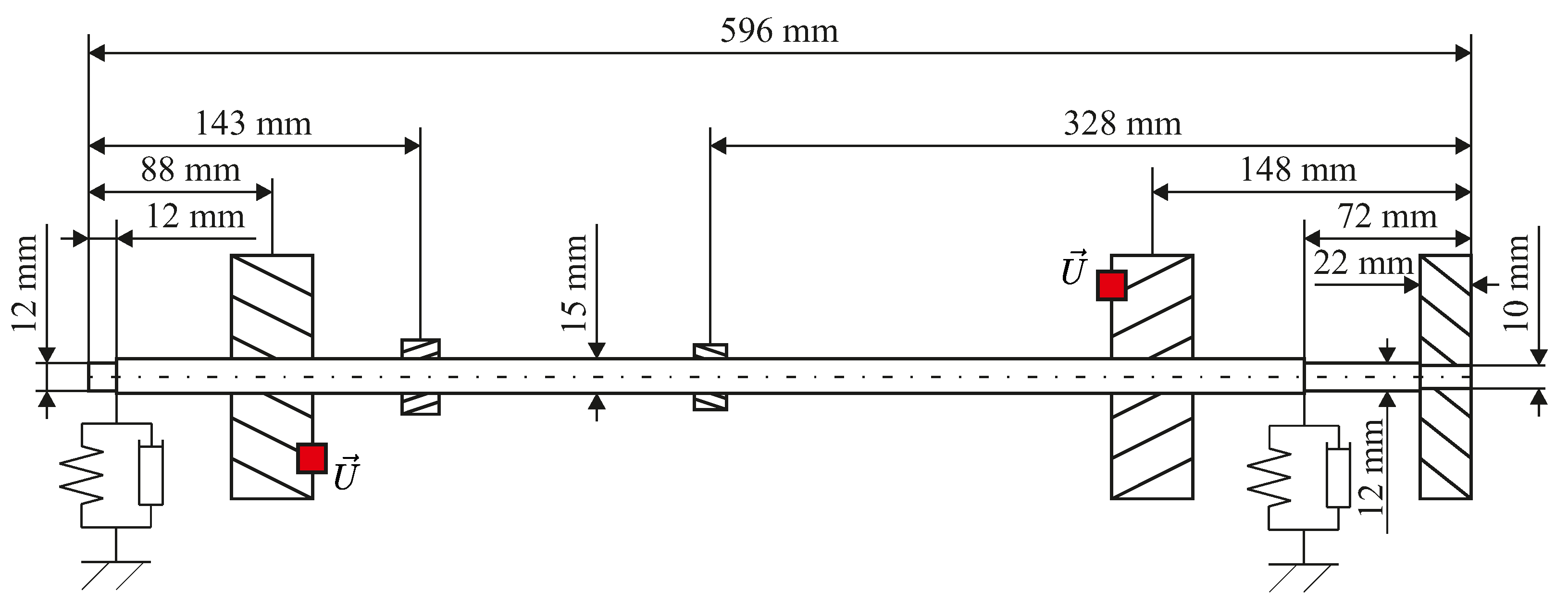



| Left Disk | Right Disk | |
|---|---|---|
| Axial position | 88 mm | 448 mm |
| Bow | 0.08694 mm | 0.11108 mm |
| Angular position |
| z | m | d | ||||
|---|---|---|---|---|---|---|
| m | kg | kg m2 | kg m2 | N/m | N/m | Ns/m |
| 0 | 0 | 0 | 0 | 0 | 0 | 0 |
| 0.008 | 0 | 0 | 0 | 282,540,000 | 129,968,400 | 80 |
| 0.012 | 0 | 0 | 0 | 0 | 0 | 0 |
| 0.088 | 2.0200 | 0.00150 | 0.0028 | 0 | 0 | 0 |
| 0.143 | 0.1300 | 0 | 0 | 0 | ||
| 0.268 | 0.0926 | 0 | 0 | 0 | ||
| 0.448 | 2.0200 | 0.00150 | 0.0028 | 0 | 0 | 0 |
| 0.524 | 0 | 0 | 0 | 0 | 0 | 0 |
| 0.528 | 0 | 0 | 0 | 282,540,000 | 129,968,400 | 80 |
| 0.574 | 0.6471 | 0.0020322 | 0.00034134 | 0 | 0 | 0 |
| 0.596 | 0 | 0 | 0 | 0 | 0 | 0 |
| Axial Position | Amount | Direction |
|---|---|---|
| 0.088 m | 3.7233 m | 4.6251 |
| 0.143 m | 1.600 m | 3.1400 |
| 0.268 m | 1.600 m | 4.800 |
| 0.448 m | 3.7233 m | 5.0789 |
| Left Disk | Right Disk | |
|---|---|---|
| Amount | 948 g mm | 1297 g mm |
| Position |
| Method 1 | Method 2 | Method 3 | |
|---|---|---|---|
| Amount | 8.71 g | 5.80 g | 7.63 g |
| Position |
| Left Disk | Right Disk | |
|---|---|---|
| Amount | 1.4808 g | 9.0608 g |
| Position |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Quinz, G.; Überwimmer, G.; Klanner, M.; Ellermann, K. Modal Balancing of Warped Rotors without Trial Runs Using the Numerical Assembly Technique. Machines 2023, 11, 1073. https://doi.org/10.3390/machines11121073
Quinz G, Überwimmer G, Klanner M, Ellermann K. Modal Balancing of Warped Rotors without Trial Runs Using the Numerical Assembly Technique. Machines. 2023; 11(12):1073. https://doi.org/10.3390/machines11121073
Chicago/Turabian StyleQuinz, Georg, Gregor Überwimmer, Michael Klanner, and Katrin Ellermann. 2023. "Modal Balancing of Warped Rotors without Trial Runs Using the Numerical Assembly Technique" Machines 11, no. 12: 1073. https://doi.org/10.3390/machines11121073
APA StyleQuinz, G., Überwimmer, G., Klanner, M., & Ellermann, K. (2023). Modal Balancing of Warped Rotors without Trial Runs Using the Numerical Assembly Technique. Machines, 11(12), 1073. https://doi.org/10.3390/machines11121073






