Analysis of Hydraulic Losses in Vortex Rope Inside the Draft Tube of Francis Pump-Turbine Based on Entropy Production Theory
Abstract
:1. Introduction
2. Numerical Simulation Methodology
2.1. Governing Equations and Turbulence Model
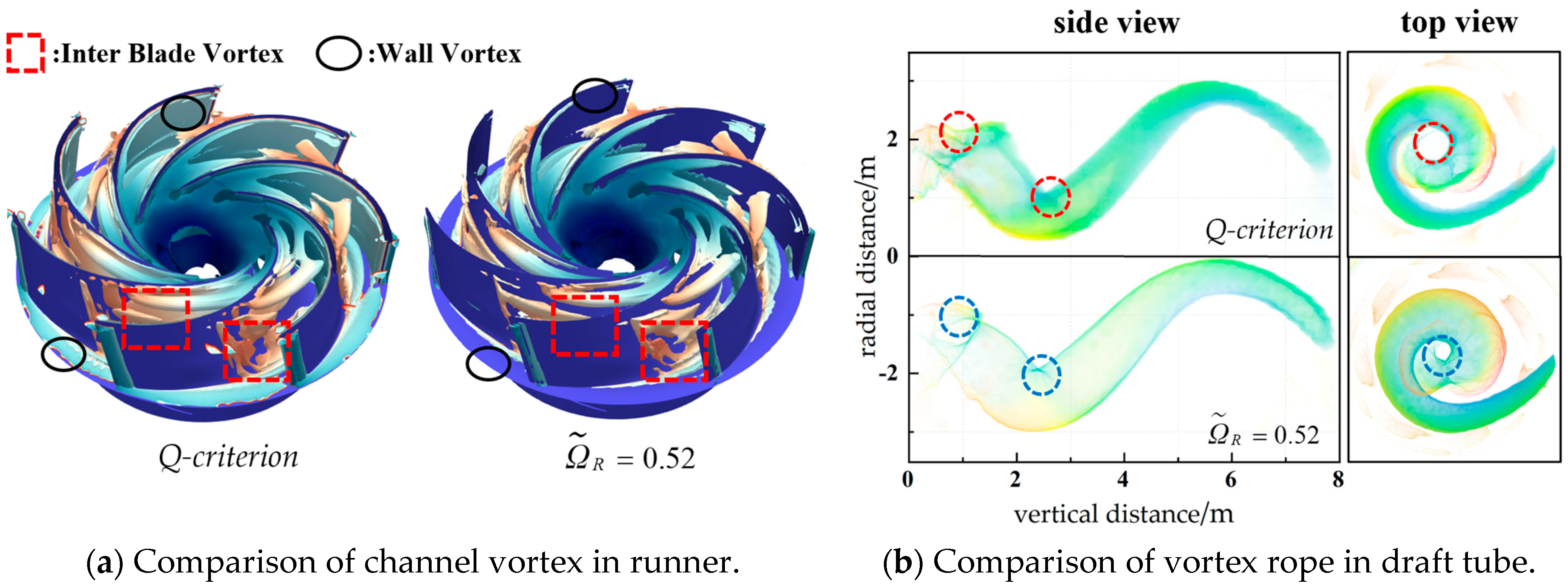
2.2. Vortex Identification Method
2.3. Entropy Production Theory Model
3. Numerical Simulation Subject
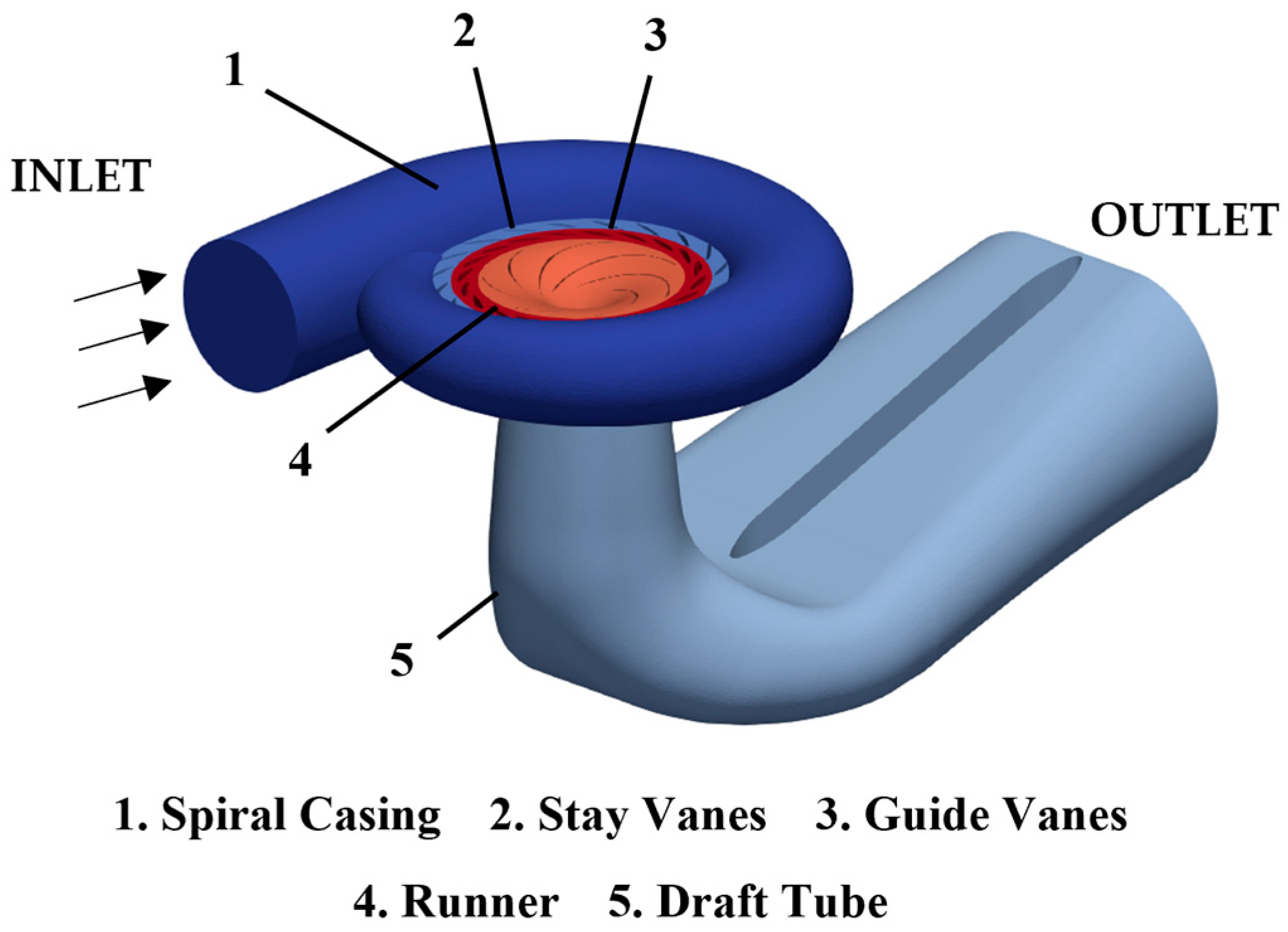
3.1. Computational Domain Model
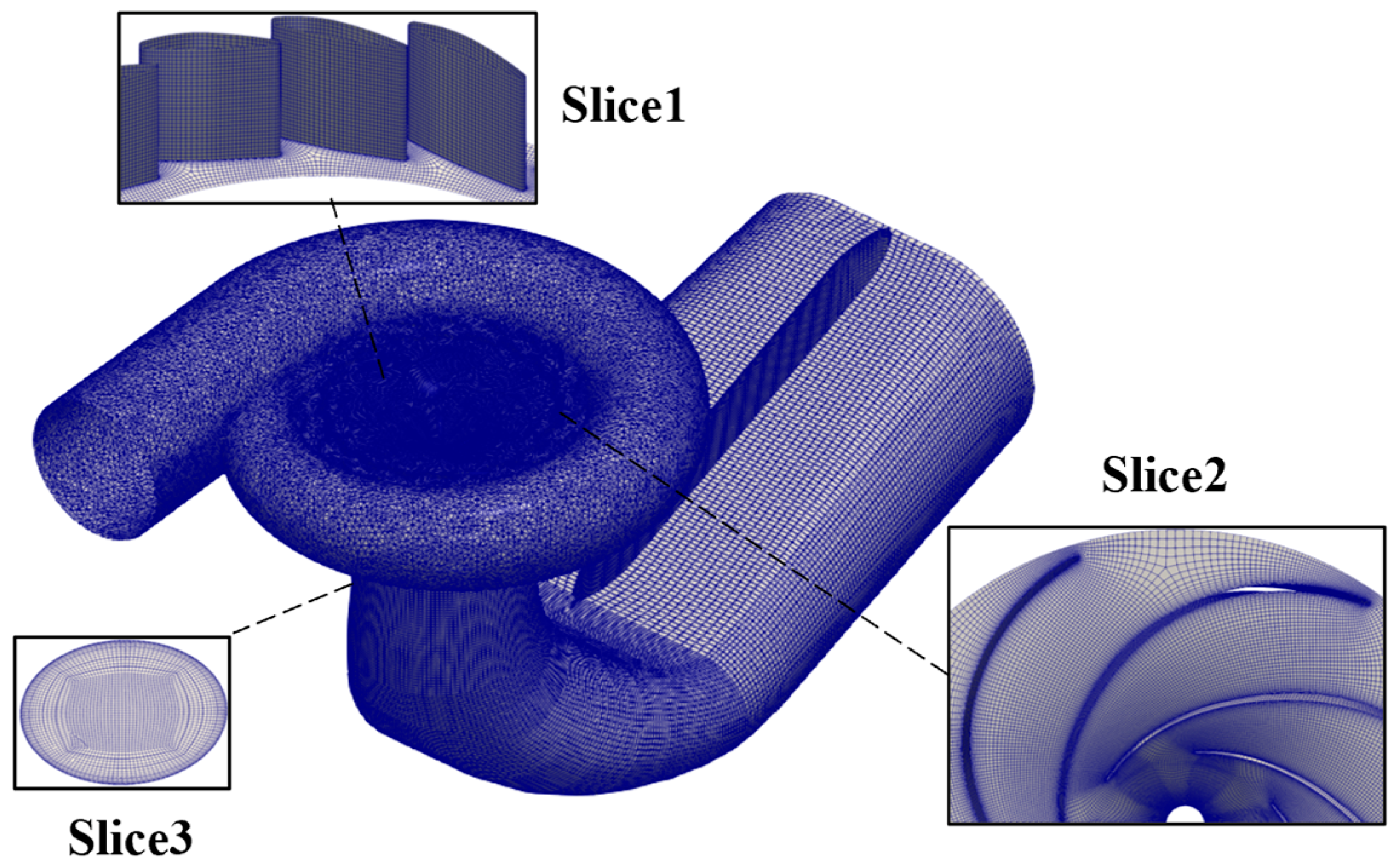
3.2. Mesh Generation and Convergence Assessment
3.3. Calculation Condition Setting
4. Analysis of Numerical Simulation Results
4.1. Verification of Computational Accuracy
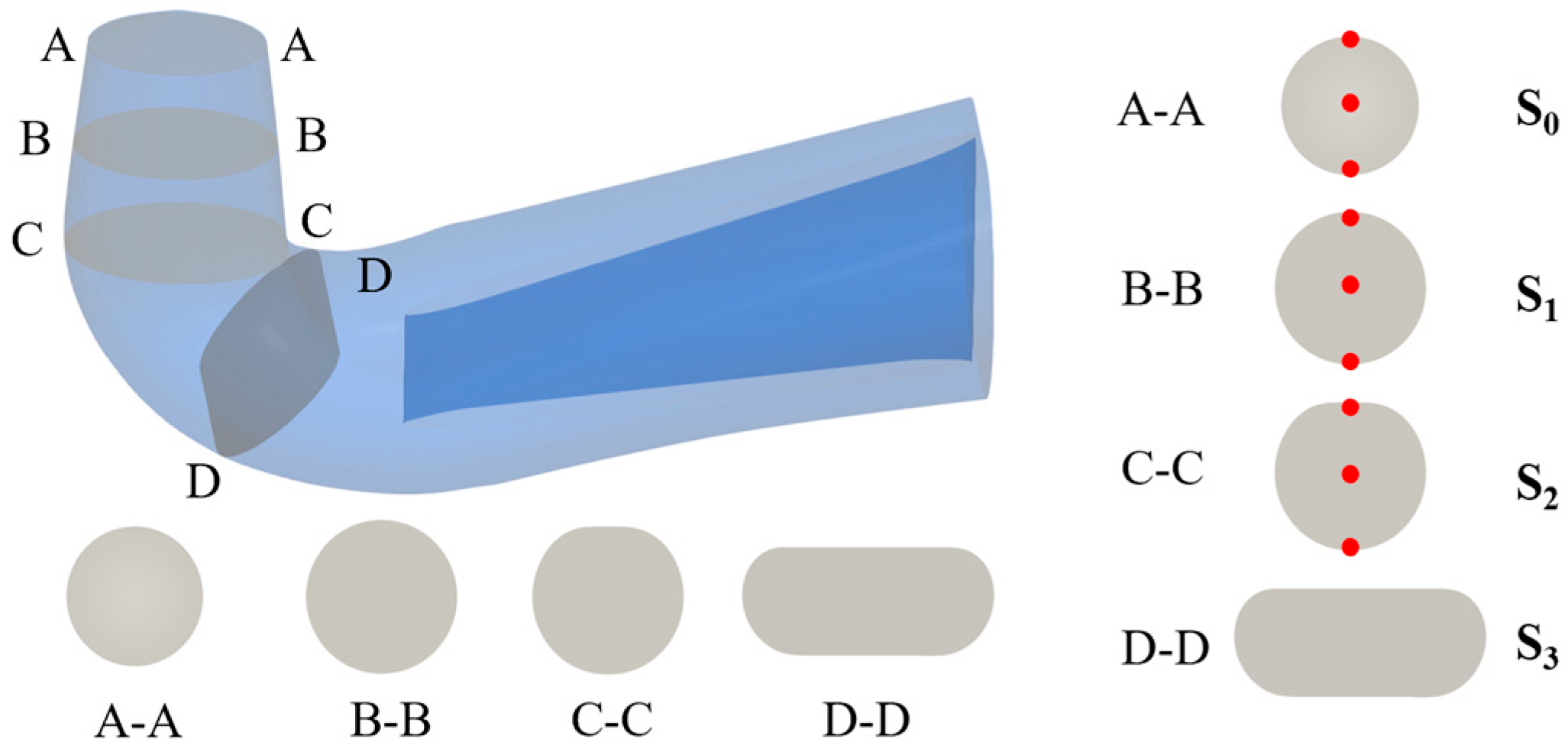
4.2. Monitoring Points and Cross-Sectional Planes
4.3. Analysis of Internal Flow Field of the Draft Tube
4.4. Analysis of Entropy Production Results
5. Conclusions
- (1)
- By comparing different vortex identification methods applied in the runner and draft tube, the method can precisely identify different types of strong and weak vortex structures and reduce the interference of wall shear stress on the accuracy of vortex identification to obtain distinct and thorough vortex rope outlines.
- (2)
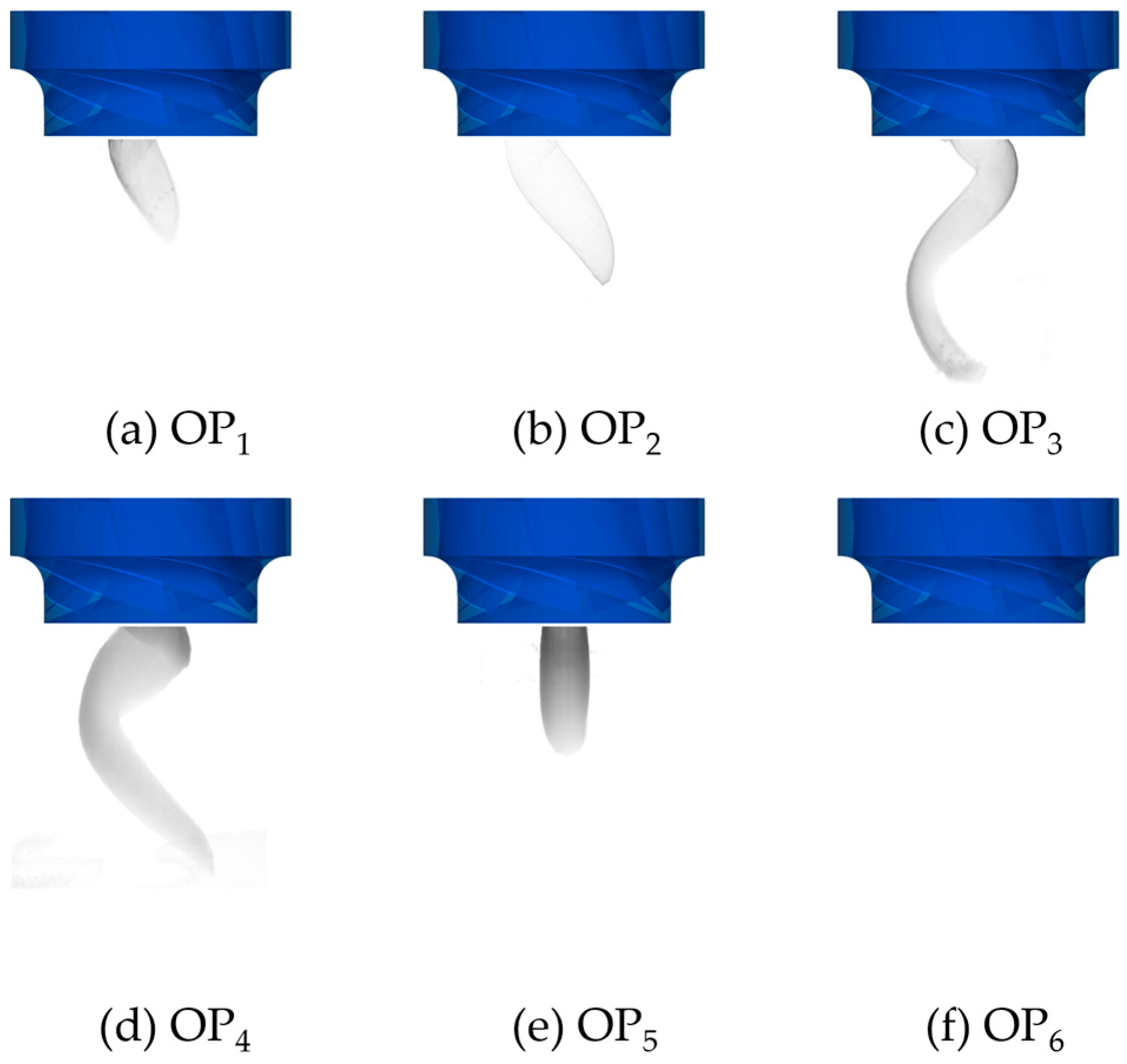
- The spiral vortex rope inside the draft tube is primarily caused by the circumferential velocity component at the runner outlet. Furthermore, the low-frequency pulsation phenomenon of 0.168fn at each cross-section can be observed, and the motion of the rotating vortex rope induces significant amplitude fluctuations of both tangential and axial velocity. However, as the flow rate increases, the flow pattern within the draft tube gradually improves. The vortex rope developed is less disruptive to the flow field.
- (3)
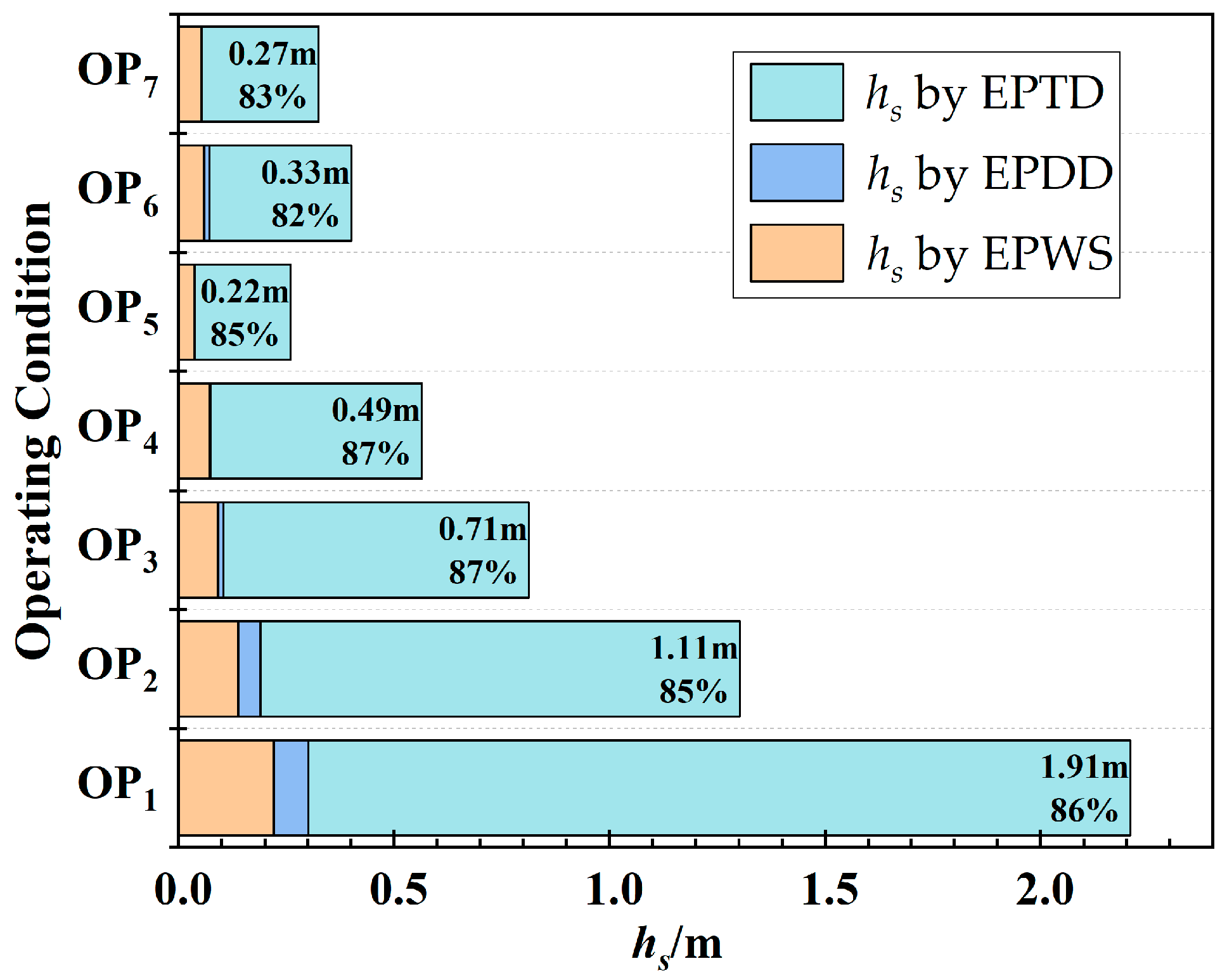
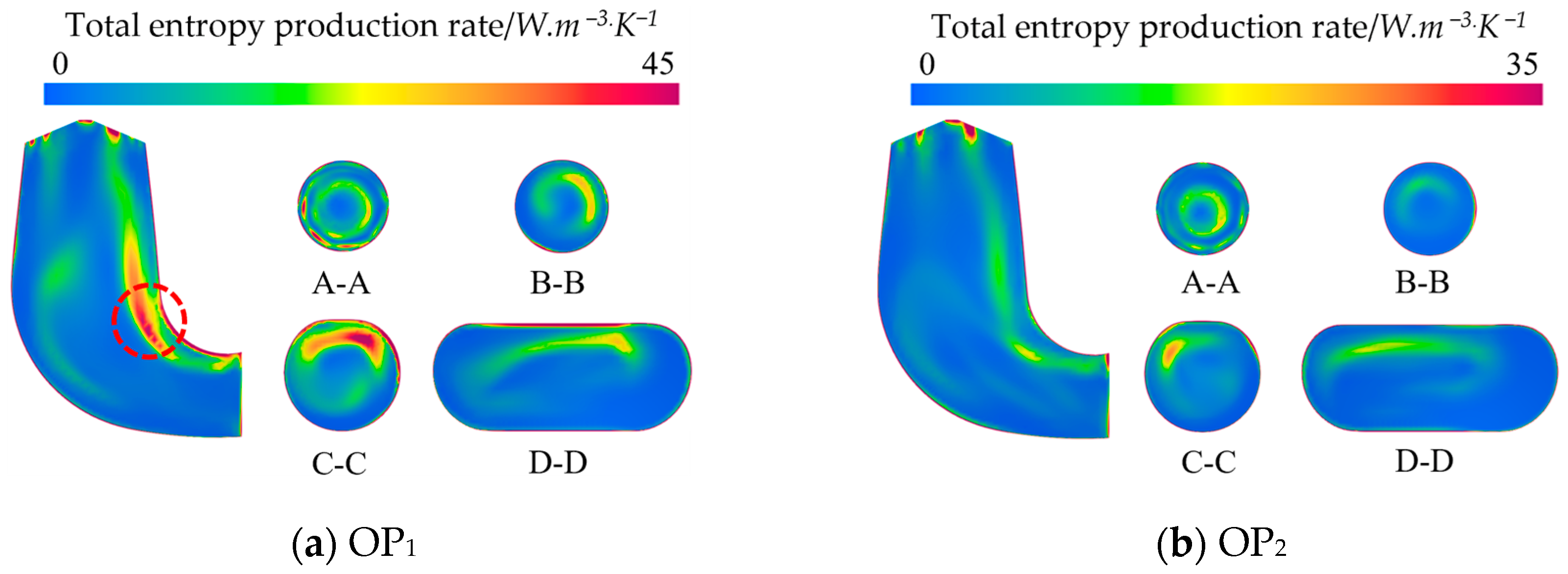
- The dispersion of the vortex ropes substantially correlates with the local entropy production rate (LEPR) inside the draft tube. Since the vortex rope would drastically change the internal flow field distribution during movements, it may cause hydraulic excitation phenomena including large-scale vortex and backflow, which would result in more hydraulic losses. Nevertheless, the hydraulic loss in the flow field inside the draft tube steadily decreases towards the QN conditions, and TEPR is mostly spread along the wall of the draft tube.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Mao, X.L.; Chen, X.K.; Lu, J.H.; Liu, P.; Zhang, Z. Improving internal fluid stability of pump turbine in load rejection process by co-adjusting inlet valve and guide vane. J. Energy Storage 2022, 50, 104623. [Google Scholar] [CrossRef]
- Skripkin, S.; Zuo, Z.; Tsoy, M.; Kuibin, P.; Liu, S. Oscillation of Cavitating Vortices in Draft Tubes of a Simplified Model Turbine and a Model Pump–Turbine. Energies 2022, 15, 2965. [Google Scholar] [CrossRef]
- Lei, W.; Jiayi, C.; Lingfeng, S.; Denghui, J.; Chun, X.; Linwei, L.; Peijian, Z. Research on the Vortex Rope Control Techniques in Draft Tube of Francis Turbines. Energies 2022, 15, 9280. [Google Scholar]
- Mao, X.L.; Chen, D.Y.; Wang, Y.C.; Mao, G.J.; Zheng, Y. Investigation on optimization of self-adaptive closure law for load rejection to a reversible pump turbine based on CFD. J. Clean. Prod. 2021, 283, 124739. [Google Scholar] [CrossRef]
- Li, X.Y.; Cao, J.W.; Zhuang, J.L.; Wu, T.M.; Zheng, H.Y.; Wang, Y.F.; Zheng, W.Q.; Lin, G.Q.; Wang, Z.W. Effect of Operating Head on Dynamic Behavior of a Pump-Turbine Runner in Turbine Mode. Energies 2022, 15, 4004. [Google Scholar] [CrossRef]
- Martínez-Lucas, G.; Pérez-Díaz, J.I.; Chazarra, M.; Sarasúa, J.I.; Cavazzini, G.; Pavesi, G.; Ardizzon, G. Risk of penstock fatigue in pumped-storage power plants operating with variable speed in pumping mode. Renew. Energy 2019, 133, 636–646. [Google Scholar] [CrossRef]
- Wanquan, D.; Lianchen, X.; Zhen, L.; Wen, T.; Xiaolong, W.; Linmin, S.; Demin, L.; Xiaobing, L. Stability Analysis of Vaneless Space in High-Head Pump-Turbine under Turbine Mode: Computational Fluid Dynamics Simulation and Particle Imaging Velocimetry Measurement. Machines 2022, 10, 143. [Google Scholar]
- Zhang, F.F.; Xiao, R.F.; Zhu, D.; Liu, W.C.; Tao, R. Pressure pulsation reduction in the draft tube of pump turbine in turbine mode based on optimization design of runner blade trailing edge profile. J. Energy Storage 2023, 59, 106541. [Google Scholar] [CrossRef]
- Zhang, X.X.; Zeng, W.; Cheng, Y.G.; Yang, Z.Y.; Chen, Q.H.; Yang, J.D. Mechanism of Fast Transition of Pressure Pulsations in the Vaneless Space of a Model Pump-Turbine During Runaway. J. Fluids Eng.-Trans. ASME 2019, 141, 121104. [Google Scholar] [CrossRef]
- Liu, C.Q.; Gao, Y.S.; Dong, X.R.; Wang, Y.Q.; Liu, J.M.; Zhang, Y.N.; Cai, X.S.; Gui, N. Third generation of vortex identification methods: Omega and Liutex/Rortex based systems. J. Hydrodyn. 2019, 31, 205–223. [Google Scholar] [CrossRef]
- Zang, W.; Zheng, Y.; Zhang, Y.; Lin, X.; Li, Y.; Fernandez-Rodriguez, E. Numerical Investigation on a Diffuser-Augmented Horizontal Axis Tidal Stream Turbine with the Entropy Production Theory. Mathematics 2023, 11, 116. [Google Scholar] [CrossRef]
- Wang, Y.Q.; Gao, Y.S.; Xu, H.Y.; Dong, X.R.; Liu, J.M.; Xu, W.Q.; Chen, M.L.; Liu, C.Q. Liutex theoretical system and six core elements of vortex identification. J. Hydrodyn. 2020, 32, 197–211. [Google Scholar] [CrossRef]
- Zhao, W.W.; Wang, J.H.; Wan, D.C. Vortex identification methods in marine hydrodynamics. J. Hydrodyn. 2020, 32, 286–295. [Google Scholar] [CrossRef]
- Gao, Y.S.; Yu, Y.F.; Liu, J.M.; Liu, C.Q. Explicit expressions for Rortex tensor and velocity gradient tensor decomposition. Phys. Fluids 2019, 31, 081704. [Google Scholar] [CrossRef]
- Gao, Y.S.; Liu, C.Q. Rortex based velocity gradient tensor decomposition. Phys. Fluids 2019, 31, 011704. [Google Scholar] [CrossRef]
- Gao, Y.S.; Liu, C.Q. Rortex and comparison with eigenvalue-based vortex identification criteria. Phys. Fluids 2018, 30, 085107. [Google Scholar] [CrossRef]
- Zhang, Y.N.; Liu, K.H.; Li, J.W.; Xian, H.Z.; Du, X.Z. Analysis of the vortices in the inner flow of reversible pump turbine with the new omega vortex identification method. J. Hydrodyn. 2018, 30, 463–469. [Google Scholar] [CrossRef]
- Zhan, J.M.; Li, Y.T.; Wai, W.H.O.; Hu, W.Q. Comparison between the Q criterion and Rortex in the application of an in-stream structure. Phys. Fluids 2019, 31, 121701. [Google Scholar] [CrossRef]
- Zhao, M.S.; Zhao, W.W.; Wan, D.C.; Wang, Y.Q. Applications of Liutex-based force field models for cavitation simulation. J. Hydrodyn. 2021, 33, 488–493. [Google Scholar] [CrossRef]
- Su, W.T.; Binama, M.; Li, Y.; Zhao, Y. Study on the method of reducing the pressure fluctuation of hydraulic turbine by optimizing the draft tube pressure distribution. Renew. Energy 2020, 162, 550–560. [Google Scholar] [CrossRef]
- Zhou, X.; Shi, C.Z.; Miyagawa, K.; Wu, H.G. Effect of modified draft tube with inclined conical diffuser on flow instabilities in Francis turbine. Renew. Energy 2021, 172, 606–617. [Google Scholar] [CrossRef]
- Zhang, Y.; Zang, W.; Zheng, J.; Cappietti, L.; Zhang, J.; Zheng, Y.; Fernandez-Rodriguez, E. The influence of waves propagating with the current on the wake of a tidal stream turbine. Appl. Energy 2021, 290, 116729. [Google Scholar] [CrossRef]
- Kan, K.; Zhang, Q.Y.; Huang, J.C. Turbulence characteristics over a tubular pump using immersed boundary method based large-eddy simulation. J. Drain. Irrig. Mach. Eng. 2022, 40, 144–149. (In Chinese) [Google Scholar]
- Zhou, X.; Wu, H.G.; Cheng, L.; Huang, Q.S.; Shi, C.Z. A new draft tube shape optimisation methodology of introducing inclined conical diffuser in hydraulic turbine. Energy 2023, 265, 126374. [Google Scholar] [CrossRef]
- Zheng, X.H.; Zhang, S.Q.; Zhang, Y.N.; Li, J.W.; Zhang, Y.N. Dynamic characteristic analysis of pressure pulsations of a pump turbine in turbine mode utilizing variational mode decomposition combined with Hilbert transform. Energy 2023, 280, 128148. [Google Scholar] [CrossRef]
- Xu, Z.; Zheng, Y.; Kan, K.; Chen, H.X. Flow instability and energy performance of a coastal axial-flow pump as turbine under the influence of upstream waves. Energy 2023, 272, 127121. [Google Scholar] [CrossRef]
- Gong, R.Z.; Wang, H.J.; Chen, L.X.; Li, D.Y.; Zhang, H.C.; Wei, X.Z. Application of entropy production theory to hydro-turbine hydraulic analysis. Sci. China-Technol. Sci. 2013, 56, 1636–1643. [Google Scholar] [CrossRef]
- Yu, A.; Tang, Q.; Chen, H.; Zhou, D. Investigations of the thermodynamic entropy evaluation in a hydraulic turbine under various operating conditions. Renew. Energy 2021, 180, 1026–1043. [Google Scholar] [CrossRef]
- Xiaotong, Y.; Kan, K.; Zheng, Y.; Chen, H.; Maxime, B. Entropy Production Evaluation within a Prototype Pump-Turbine Operated in Pump Mode for a Wide Range of Flow Conditions. Processes 2022, 10, 2058. [Google Scholar]
- Qin, Y.L.; Li, D.Y.; Wang, H.J.; Liu, Z.S.; Wei, X.Z.; Wang, X.H. Multi-objective optimization design on high pressure side of a pump-turbine runner with high efficiency. Renew. Energy 2022, 190, 103–120. [Google Scholar] [CrossRef]
- Kan, K.; Zhao, F.; Xu, H.; Feng, J.G.; Chen, H.X.; Liu, W.D. Energy performance evaluation of an axial-flow pump as turbine under conventional and reverse operating modes based on an energy loss intensity model. Phys. Fluids 2023, 35, 015125. [Google Scholar] [CrossRef]
- Kock, F.; Herwig, H. Local entropy production in turbulent shear flows: A high-Reynolds number model with wall functions. Int. J. Heat Mass Transf. 2004, 47, 2205–2215. [Google Scholar] [CrossRef]
- Kock, F.; Herwig, H. Entropy production calculation for turbulent shear flows and their implementation in cfd codes. Int. J. Heat Mass Transf. 2005, 26, 672–680. [Google Scholar] [CrossRef]
- Duan, L.; Wu, X.L.; Ji, Z.L.; Fang, Q.X. Entropy generation analysis on cyclone separators with different exit pipe diameters and inlet dimensions. Chem. Eng. Sci. 2015, 138, 622–633. [Google Scholar] [CrossRef]
- Roache, P.J. Perspective: A Method for Uniform Reporting of Grid Refinement Studies. J. Fluids Eng.-Trans. ASME 1994, 116, 405–413. [Google Scholar] [CrossRef]
- Meana-Fernández, A.; Fernández Oro, J.M.; Argüelles Díaz, K.M.; Galdo-Vega, M.; Velarde-Suárez, S. Application of Richardson extrapolation method to the CFD simulation of vertical-axis wind turbines and analysis of the flow field. Eng. Appl. Comp. Fluid Mech. 2019, 13, 359–376. [Google Scholar] [CrossRef]
- Caretto, L.S.; Gosman, A.D.; Patankar, S.V.; Spalding, D.B. Two calculation procedures for steady, three-dimensional flows with recirculation. In Proceedings of the Third International Conference on Numerical Methods in Fluid Mechanics; Springer: Berlin/Heidelberg, Germany, 1973. [Google Scholar]
- Issa, R.I.; Ahmadi-Befrui, B.; Beshay, K.R.; Gosman, A.D. Solution of the implicitly discretised reacting flow equations by operator-splitting. J. Comput. Phys. 1991, 93, 388–410. [Google Scholar] [CrossRef]






| Parameter | Value |
|---|---|
| Rated head Hr (m) | 105.8 |
| Rated flow Qr (m3/s) | 148.7 |
| Rated rotate speed n (rpm) | 200 |
| Number of runner blades Nr | 7 |
| Rated power of turbine Pr (MW) | 139 |
| Runner inlet diameter D1 (m) | 5.22 |
| Number of guide/stay vanes Nv | 20 |
| N (Million) | Q (m3/s) | H (%) | h | r | e1 | e2 | ||
|---|---|---|---|---|---|---|---|---|
| 7.864 | 148.92 | 91.5 | 0.0091 | 1.297 | 0.4% | 0.33% | 0.5% | 1.12% |
| 4.651 | 148.62 | 91.2 | 0.0118 | |||||
| 2.766 | 148.04 | 90.8 | 0.0153 | 1.300 | 0.34% | 0.44% | ||
| Operating Condition | Q11/L·s−1 | N11/kW | η/% | |
|---|---|---|---|---|
| 0.43QN | OP1 | 226.87 | 1.47 | 66.2 |
| 0.54QN | OP2 | 287.96 | 2.29 | 81.2 |
| 0.66QN | OP3 | 351.39 | 3.04 | 88.3 |
| 0.75QN | OP4 | 395.96 | 3.49 | 89.9 |
| 0.8QN (QBEP) | OP5 | 429.31 | 3.89 | 92.3 |
| 0.92QN | OP6 | 489.92 | 4.41 | 91.7 |
| QN | OP7 | 536.87 | 4.72 | 89.7 |
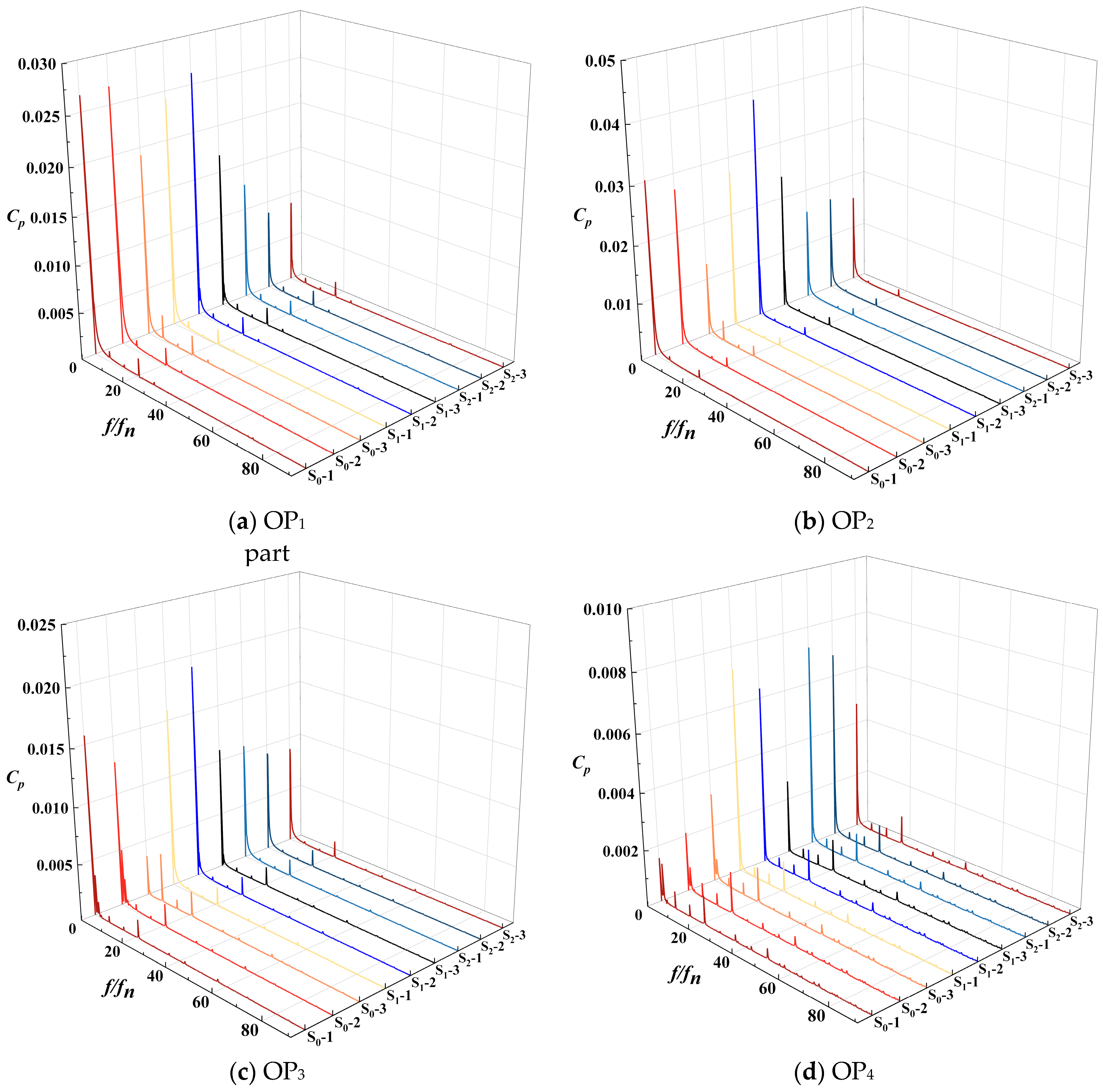
| Operating Condition | f | S0-1 | S0-2 | S0-3 | S1-1 | S1-2 | S1-3 | S2-1 | S2-2 | S2-3 |
|---|---|---|---|---|---|---|---|---|---|---|
| OP1 | 0.168fn | 0.0267 | 0.0268 | 0.0193 | 0.0242 | 0.0262 | 0.0169 | 0.0128 | 0.0075 | 0.0072 |
| OP2 | 0.168fn | 0.03 | 0.0274 | 0.0128 | 0.0276 | 0.0387 | 0.0239 | 0.016 | 0.0169 | 0.0157 |
| OP3 | 0.168fn | 0.0128 | 0.0128 | 0.0036 | 0.0159 | 0.019 | 0.011 | 0.0106 | 0.0092 | 0.009 |
| OP4 | 0.168fn | 0.0016 | 0.0016 | 0.0032 | 0.0072 | 0.0063 | 0.0027 | 0.0073 | 0.0068 | 0.0047 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, H.; Zhou, D.; Guo, J.; Xu, L. Analysis of Hydraulic Losses in Vortex Rope Inside the Draft Tube of Francis Pump-Turbine Based on Entropy Production Theory. Machines 2023, 11, 965. https://doi.org/10.3390/machines11100965
Wang H, Zhou D, Guo J, Xu L. Analysis of Hydraulic Losses in Vortex Rope Inside the Draft Tube of Francis Pump-Turbine Based on Entropy Production Theory. Machines. 2023; 11(10):965. https://doi.org/10.3390/machines11100965
Chicago/Turabian StyleWang, Haobo, Daqing Zhou, Junxun Guo, and Lianchen Xu. 2023. "Analysis of Hydraulic Losses in Vortex Rope Inside the Draft Tube of Francis Pump-Turbine Based on Entropy Production Theory" Machines 11, no. 10: 965. https://doi.org/10.3390/machines11100965
APA StyleWang, H., Zhou, D., Guo, J., & Xu, L. (2023). Analysis of Hydraulic Losses in Vortex Rope Inside the Draft Tube of Francis Pump-Turbine Based on Entropy Production Theory. Machines, 11(10), 965. https://doi.org/10.3390/machines11100965





