Effect of Machined Tooth Surface Mixed Lubrication Sliding Wear on Gear Dynamic Characteristics
Abstract
1. Introduction
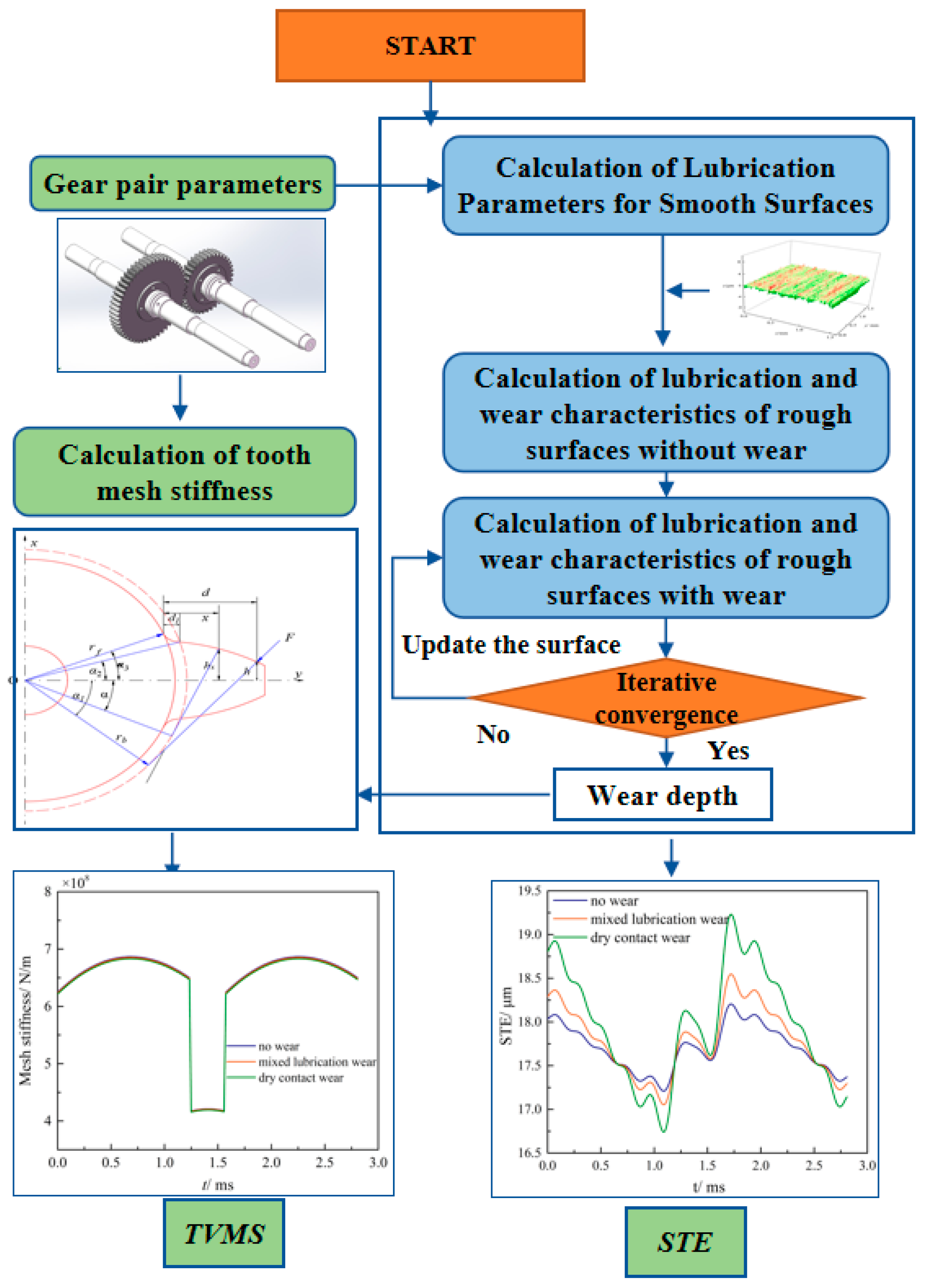
2. Theoretical Background
2.1. Gear System Dynamic Model
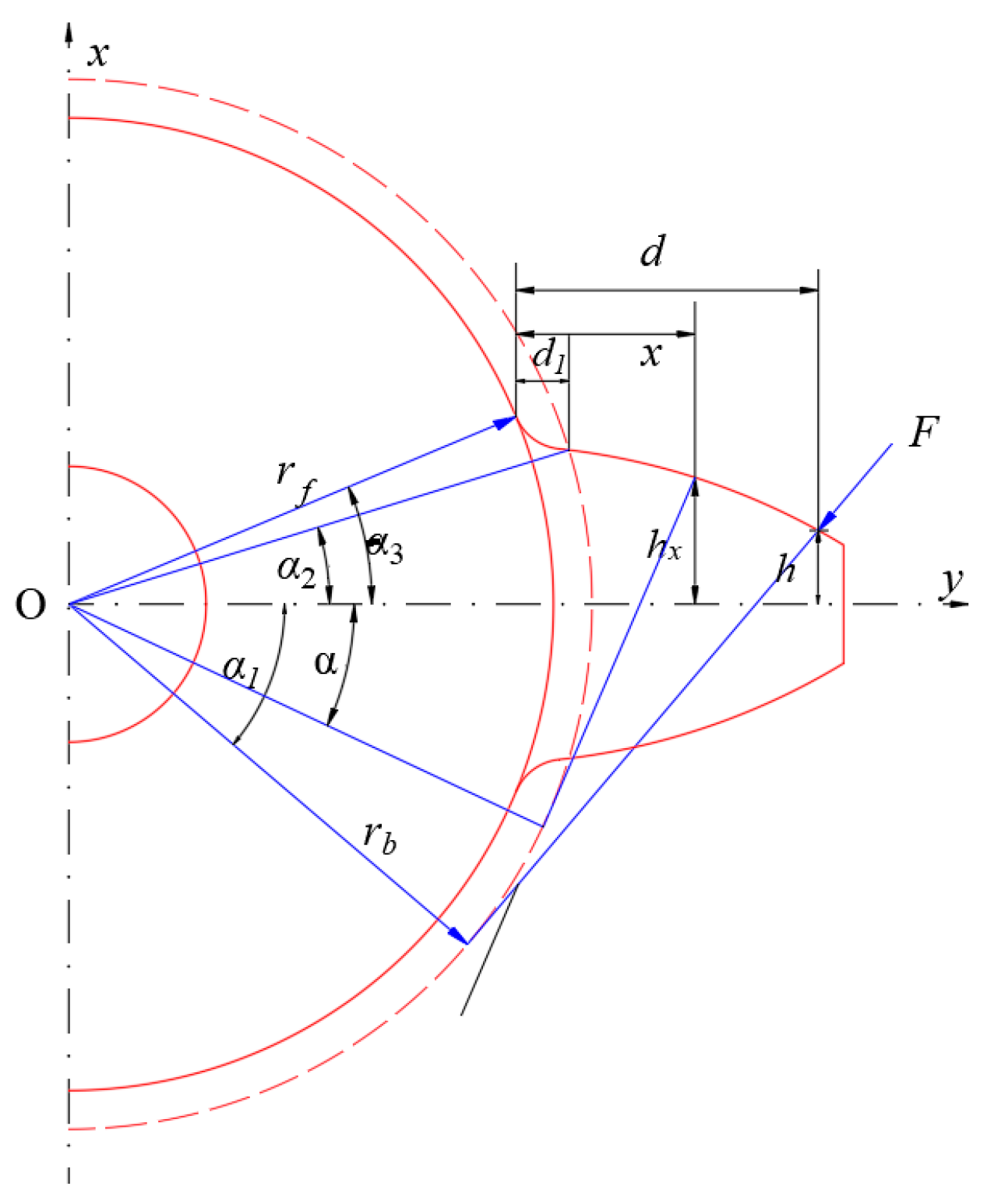
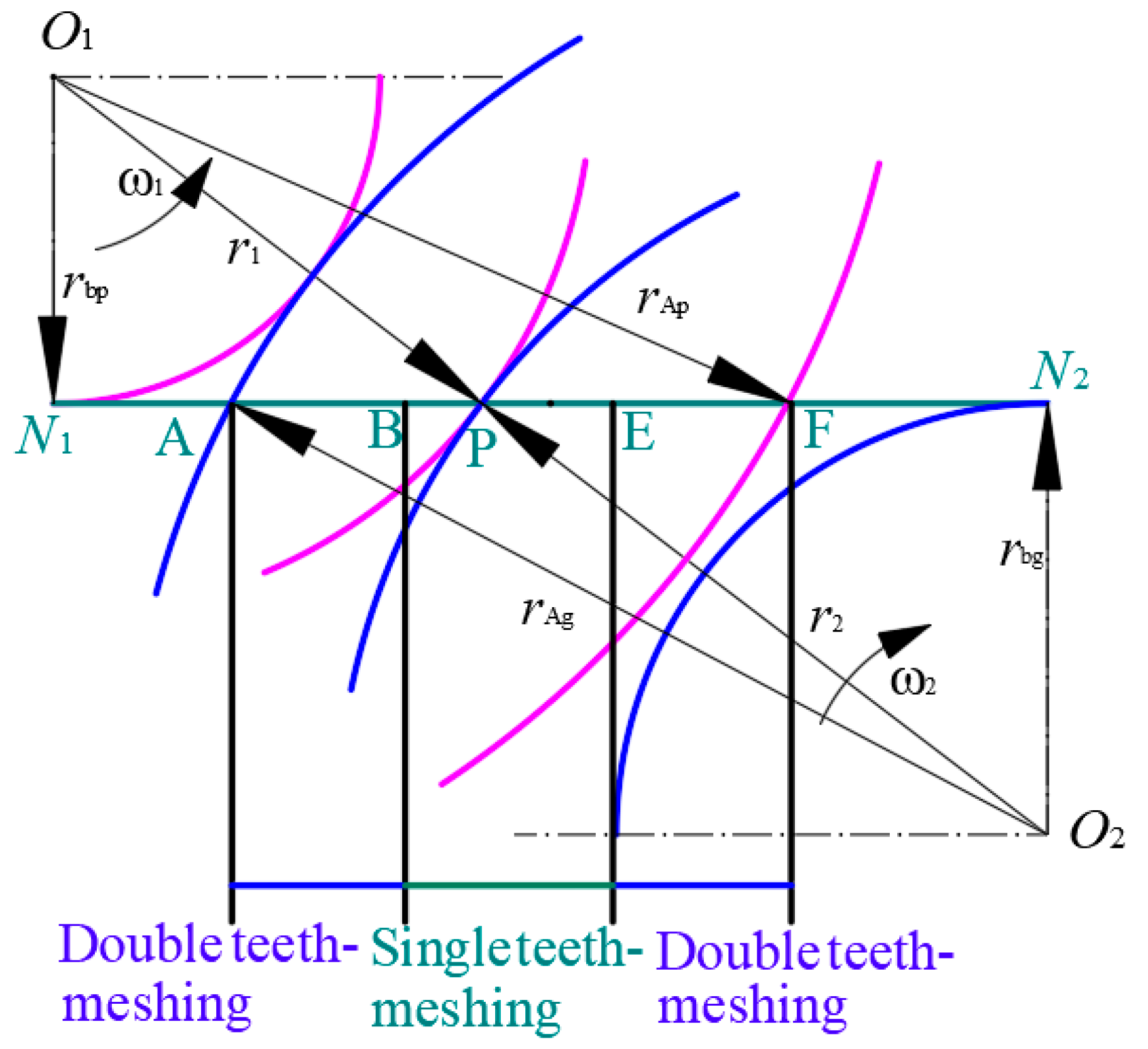
2.2. TVMS and STE Calculation Considering Wear
2.3. Wear Depth of Tooth Surface under Mixed Lubrication
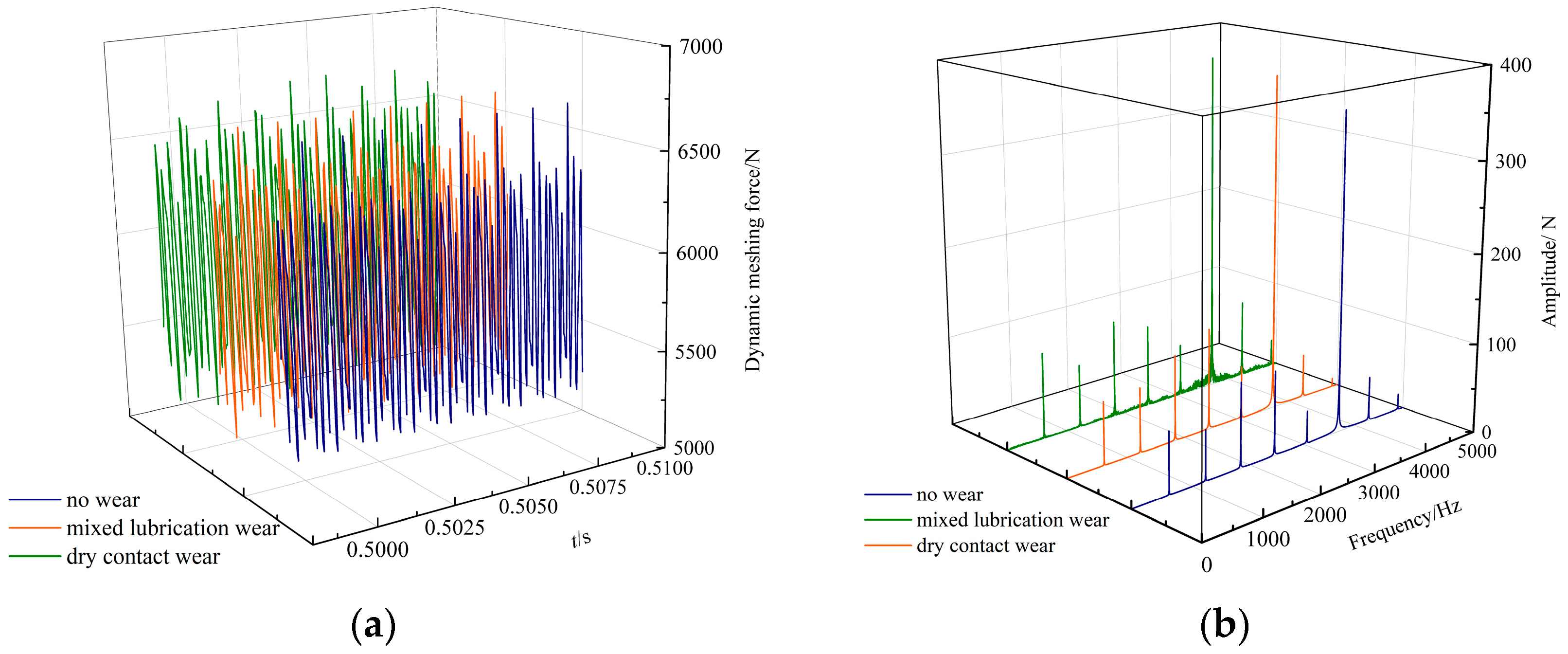
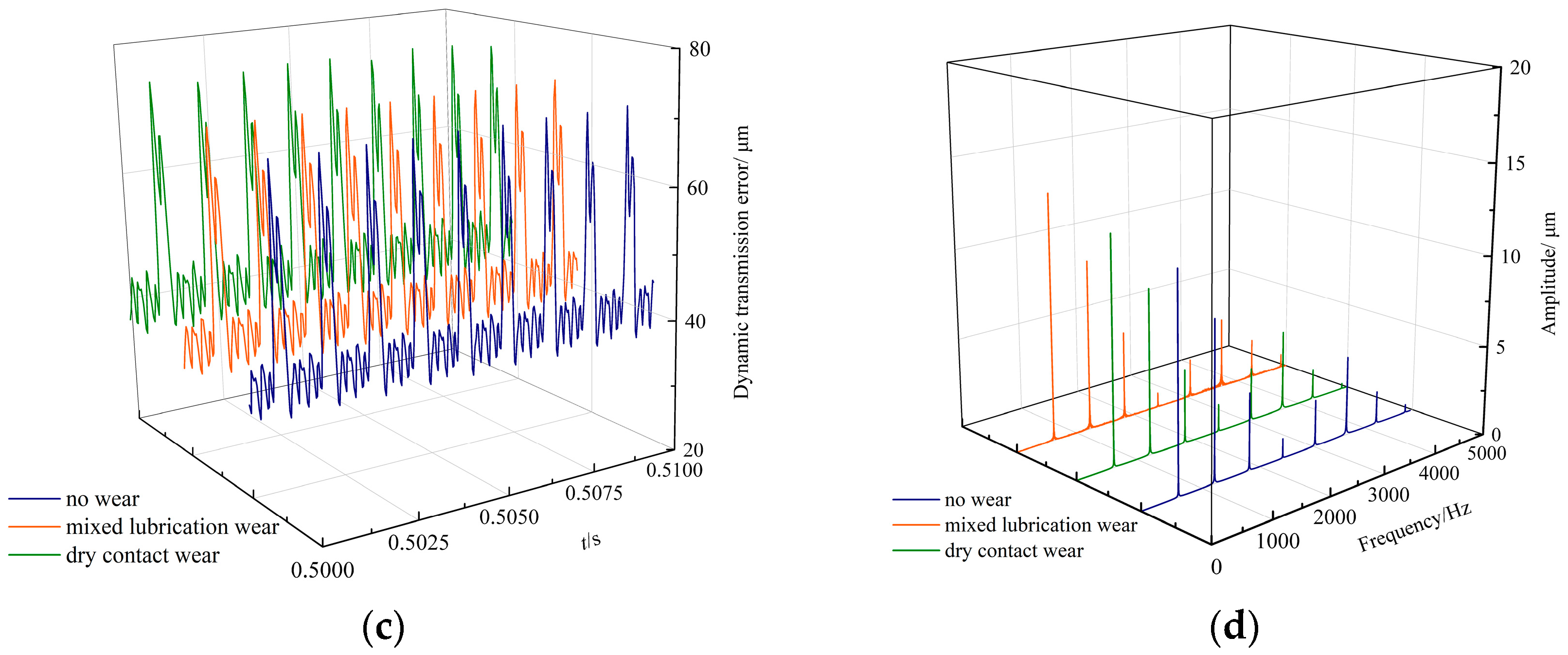
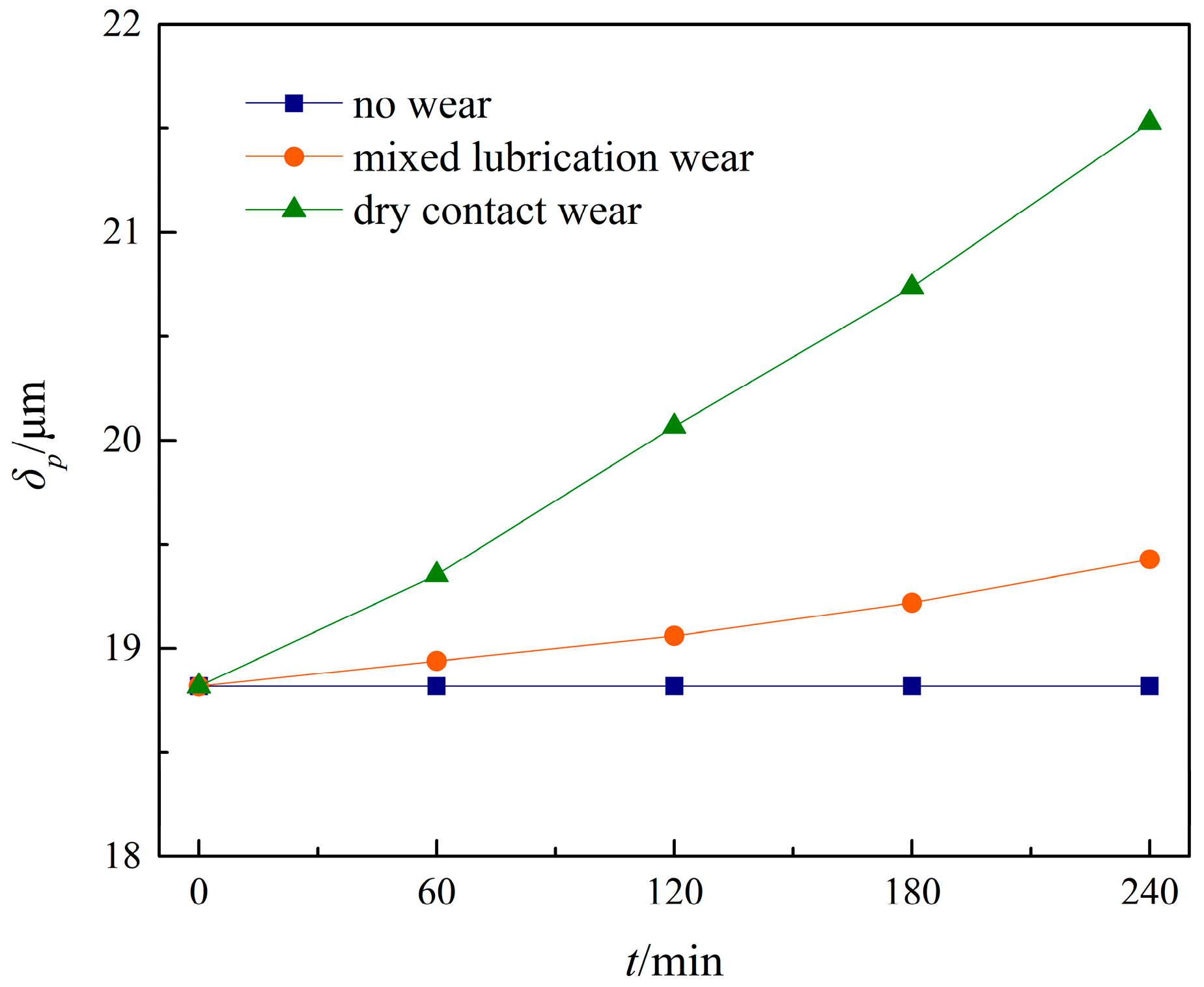
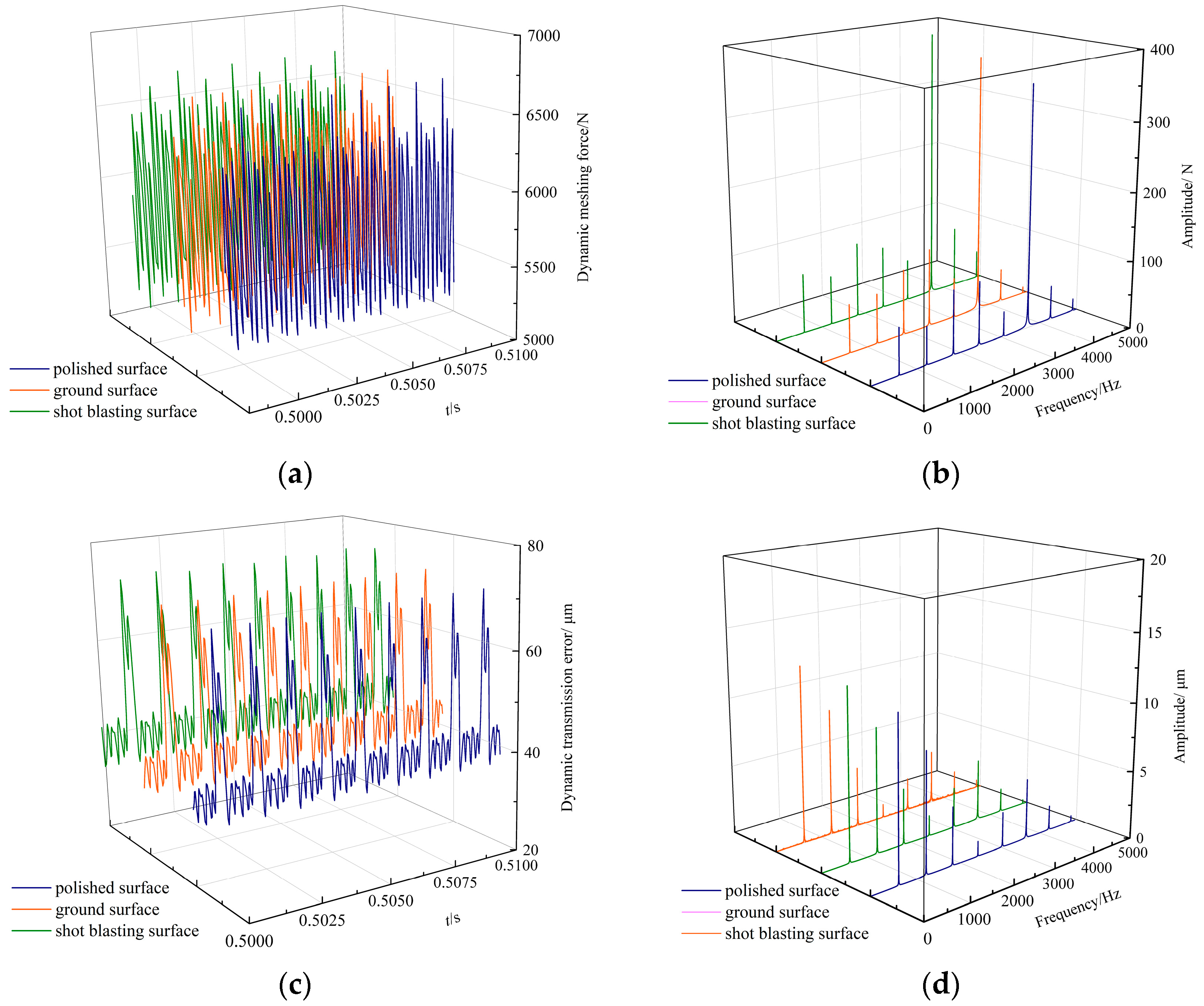
3. Results and Discussion
3.1. Dynamic Characteristics Table 3. UP-Dule Mode Performance Indicators of Gears under Dry Wear and Mixed Lubrication Wear
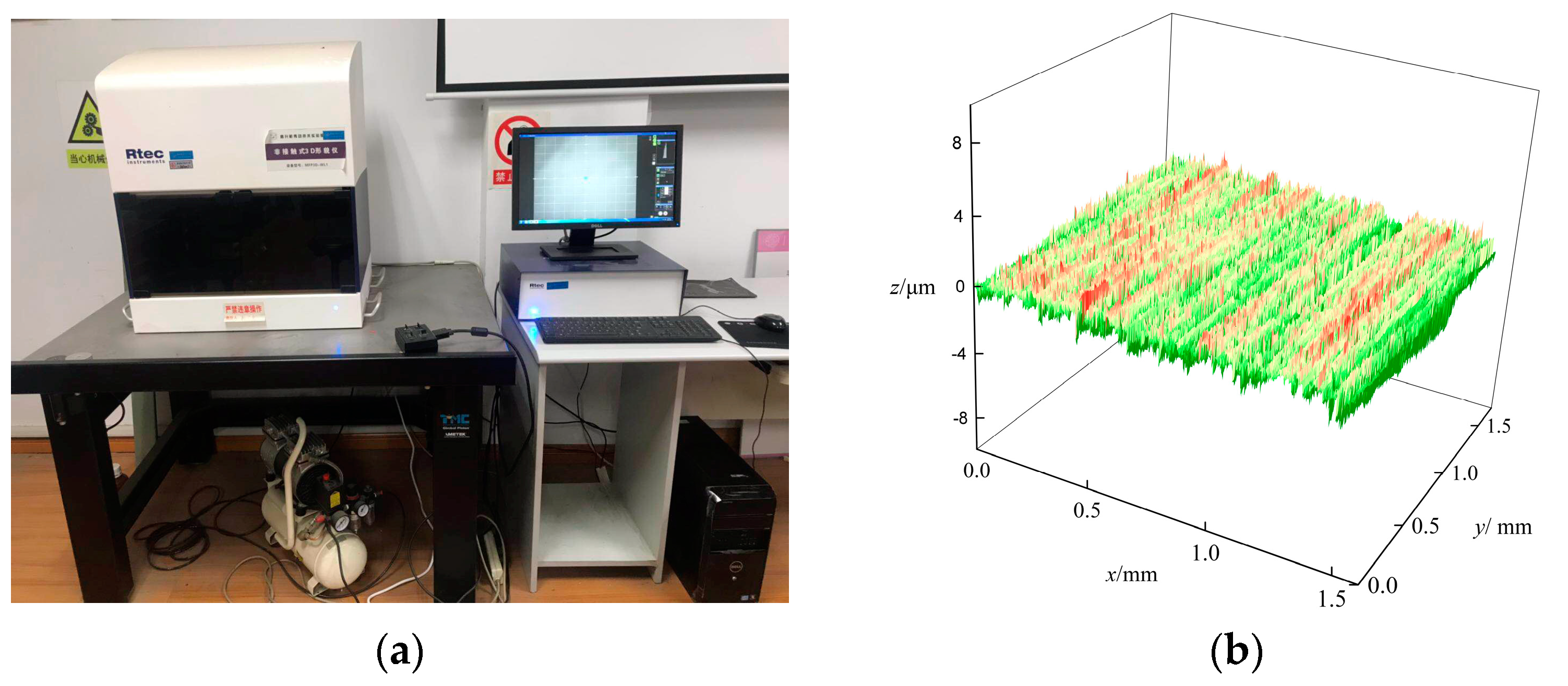
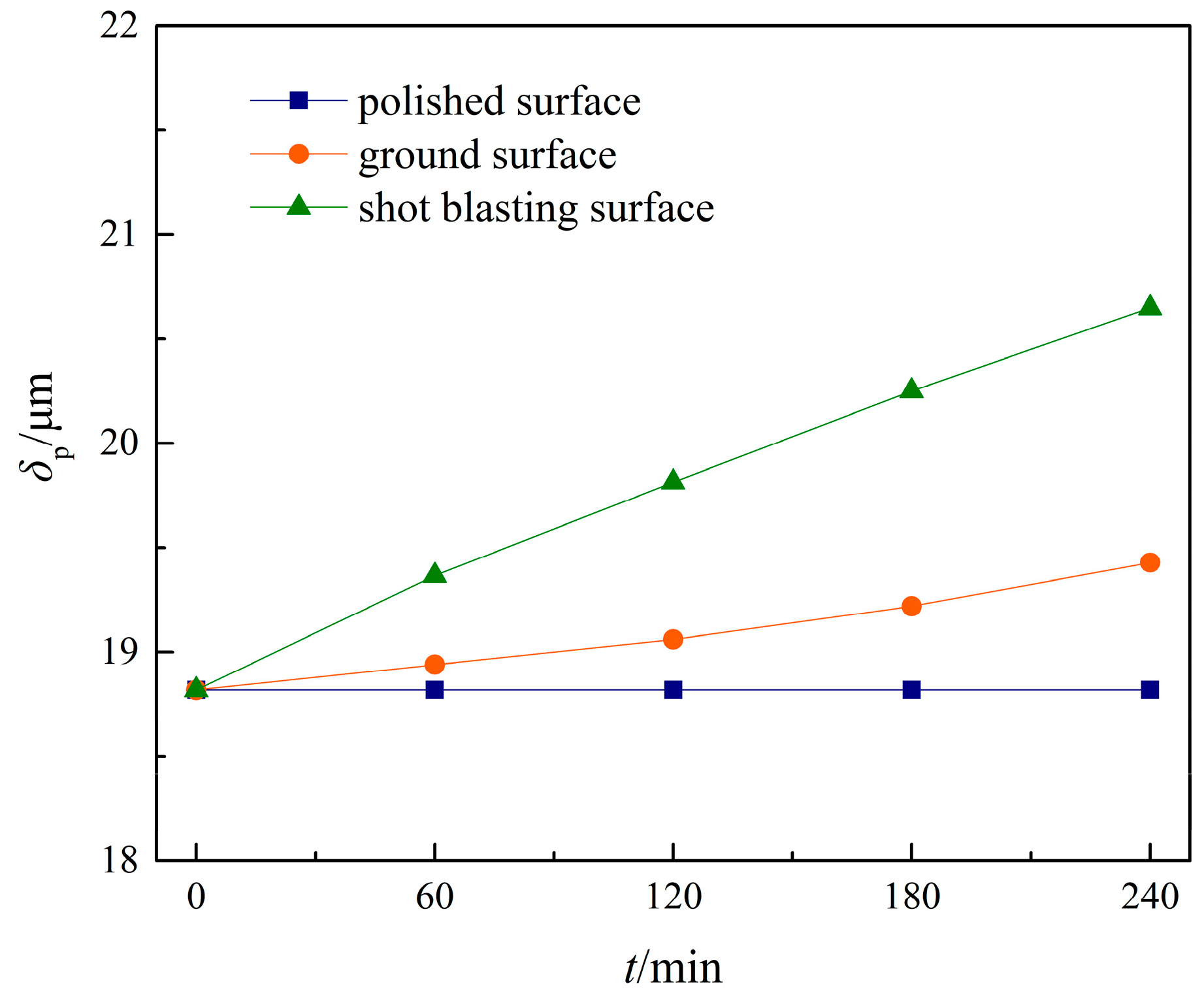
3.2. Effect of Tooth Surface Topography on Dynamic Characteristics in Mixed Lubrication
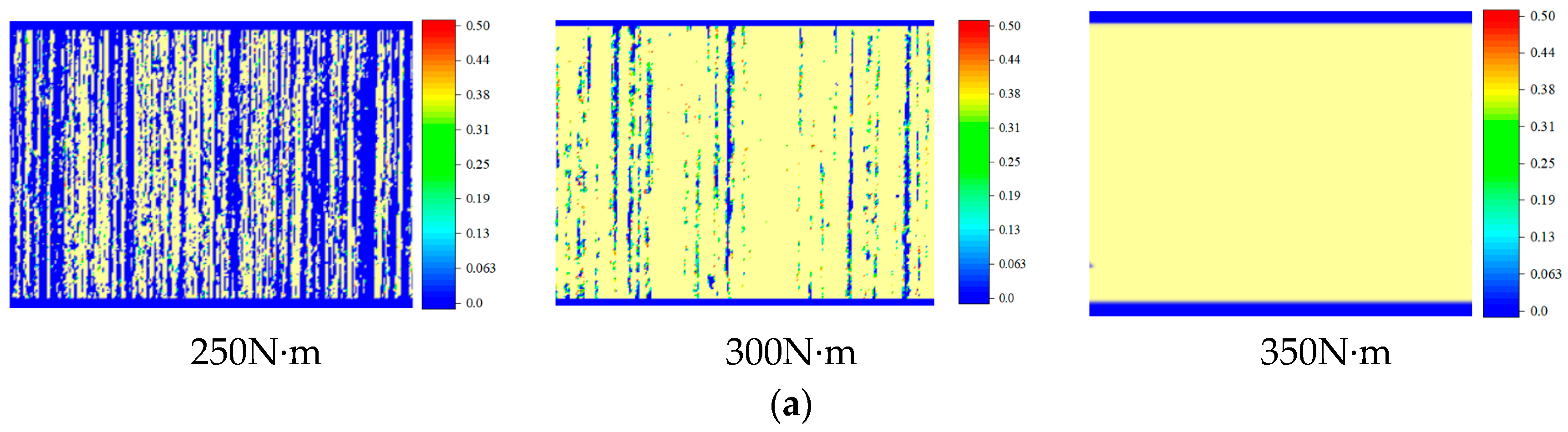
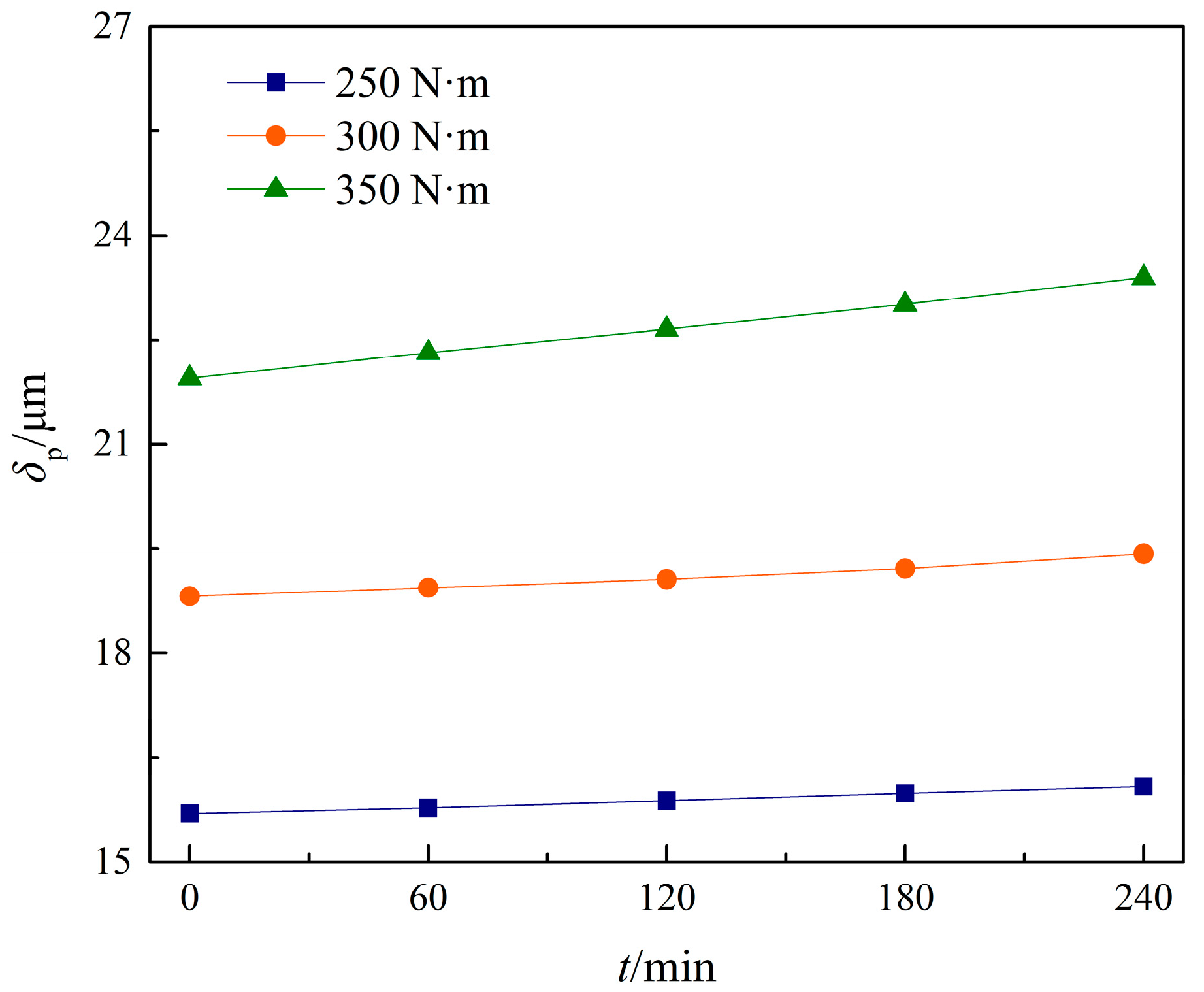
3.3. Effect of Torques on Dynamic Characteristics in Mixed Lubrication Sliding Wear
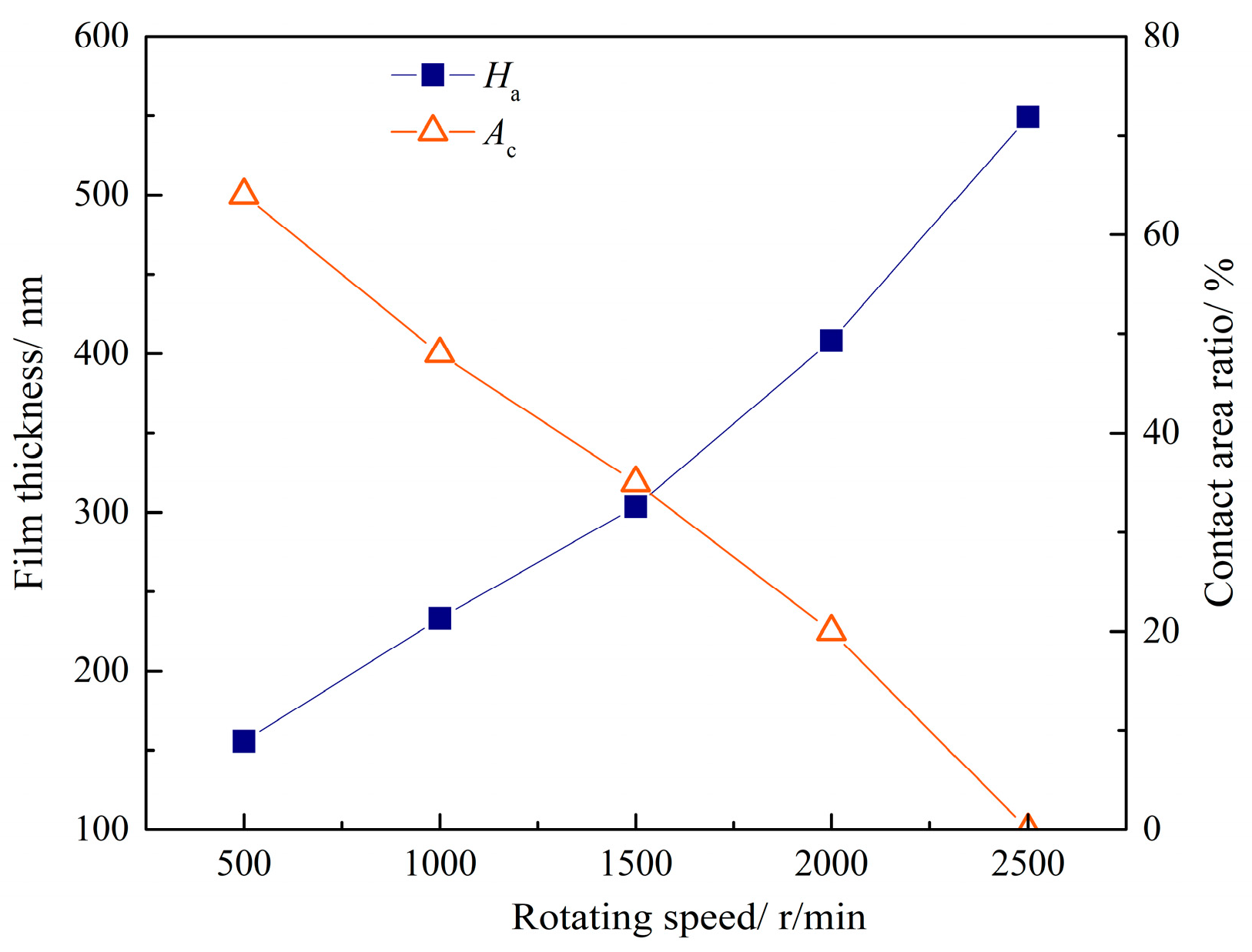
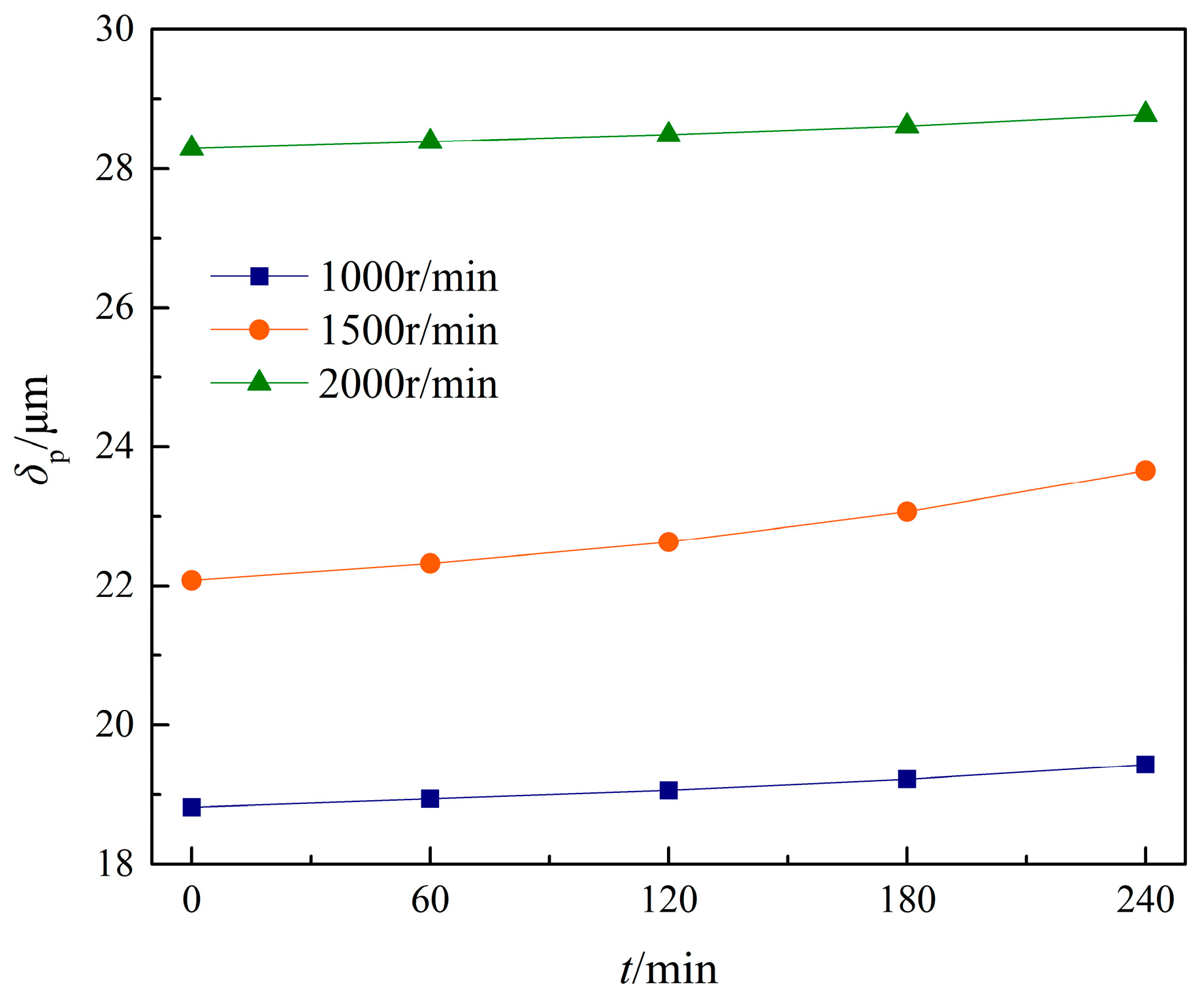
3.4. Effect of Rotating Speed on Dynamic Characteristics in Mixed Lubrication Sliding Wear
4. Conclusions
- (1)
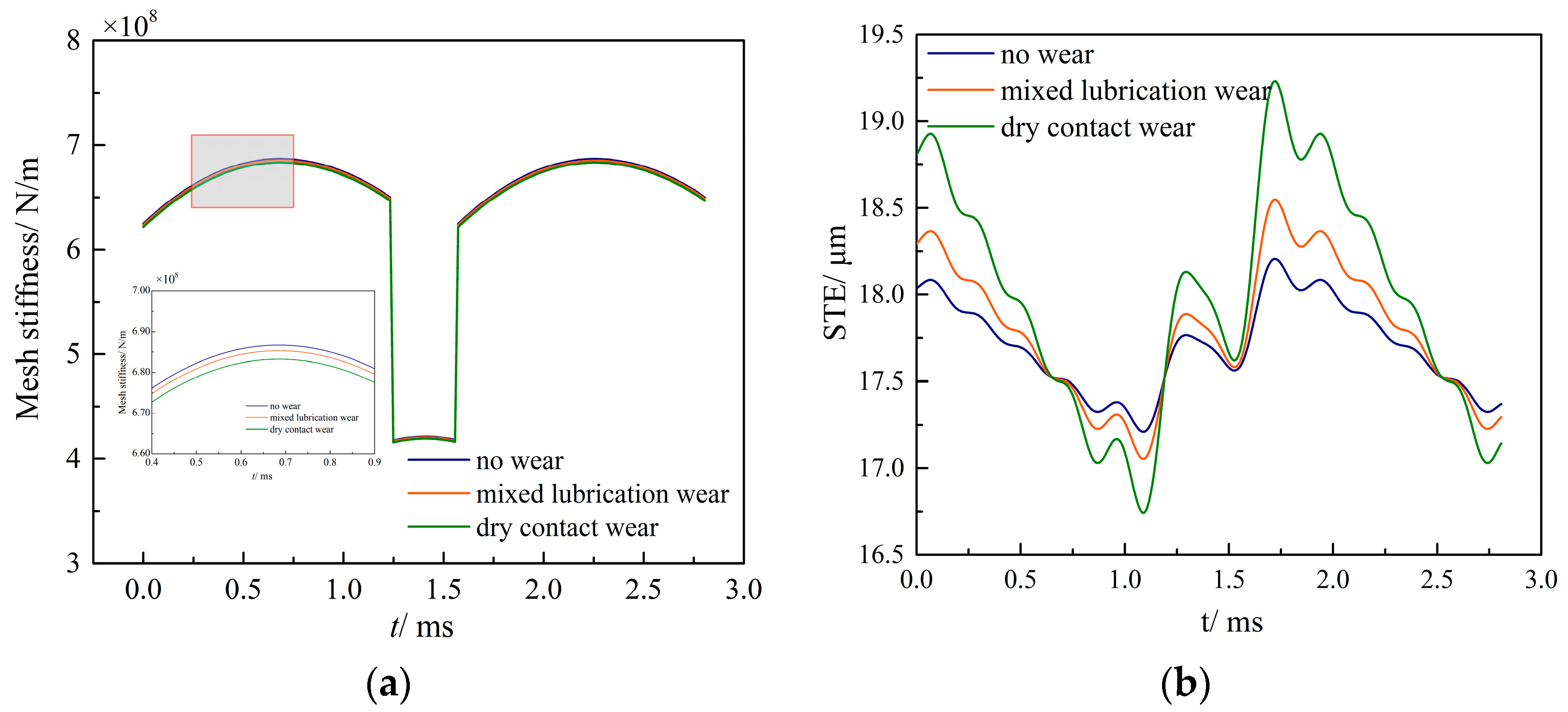
- For the same time period, the wear depth of mixed lubrication sliding wear is 40%-60% less than that of dry contact wear. Meanwhile, the variation in gear meshing stiffness and transmission error caused by lubrication wear is less than that caused by dry contact wear.
- (2)
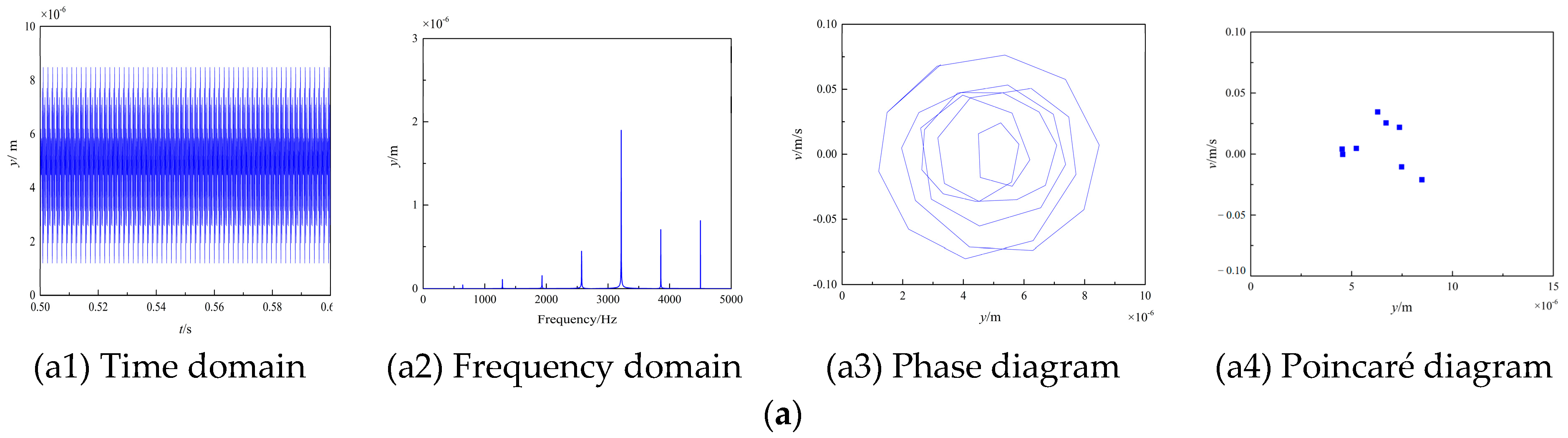
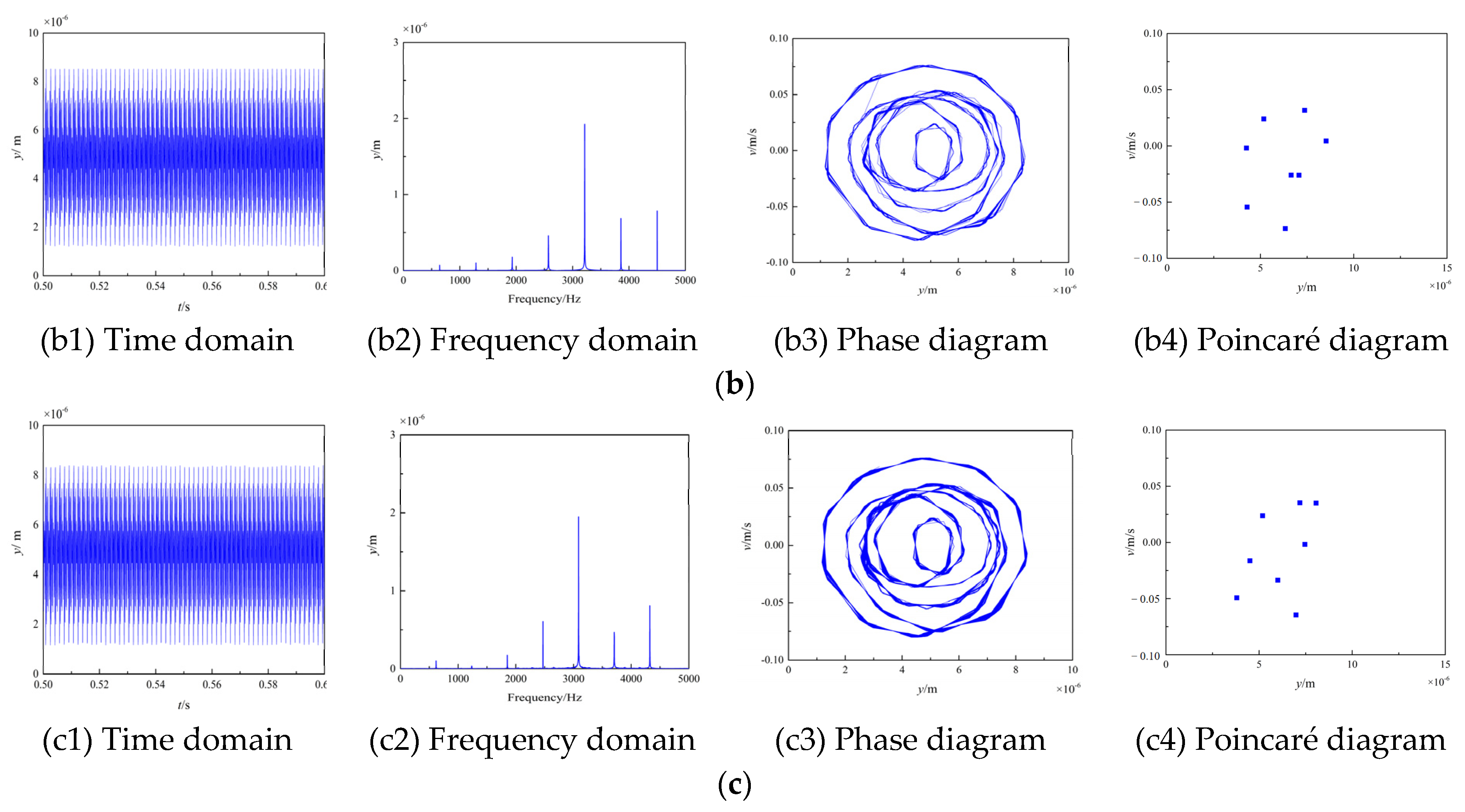
- Tooth surface wear increases the relative vibration displacement of the gear pair in each frequency band, with the amplitude of the system’s main frequency increasing the most.
- (3)
- As torque increases, film thickness decreases by about 31% and wear depth increases by 80–95%. The peak-to-peak value of gear dynamic transmission error rises as torque rises. With increasing rotating speeds, the wear degree increases first and then decreases, and the peak-to-peak increase rate of gear dynamic transmission error increases first and then decreases.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| a | Hertzian half width (mm) | rbp, rbg | Base circle radius (mm) |
| Ax | The area of the section | S | Sliding distance (mm) |
| The head clearance coefficient | SR | Slide-roll ratio | |
| E | Effective Young’s modulus (GPa) | Sf | The arc length of the tooth root (mm) |
| e | Static transmission error (μm) | s | The distance from the meshing point to the pitch point (mm) |
| Fm | Meshing force (N) | T | Torque (N·m) |
| Ff | Friction force (N) | U | Entrainment speed (m/s) |
| f | The friction coefficient of solid-to-solid contact | u | Relative sliding velocity (m/s) |
| fpt | Pitch error (μm) | U1, U2 | The velocity of the pinion and gear at contact point (m/s) |
| ff | Tooth profile error (μm) | W1, W2 | wear depth of surface 1 and surface 2 |
| fb | Tooth direction error (μm) | Wc | contact load ratio |
| fa | Center distance installation error (μm) | w | contact load (N/m) |
| H | Material hardness (GPa) | xβ | the distance from the meshing point to the tooth centerline (mm) |
| Ha | Central average film thickness (nm) | yβ | the horizontal distance from the meshing point to the origin (mm) |
| h | Film thickness (nm) | α | Pressure-viscosity coefficient |
| The addendum height coefficient | ν | Poisson’s ratio | |
| hwear | Wear depth (mm) | θ | Torsional angle |
| I | Inertia moment | υ | Elastic deformation |
| Ix | the section moment | The pressure angle of the pitch cycle (°) | |
| k | wear coefficient | ρ | The lubricant density (kg/m3) |
| k1, k2 | The meshing stiffness of the pinion and gear (N/m) | , | The angular velocities of the pinion and gear (rad/s) |
| km and cm | meshing stiffness (N/m) and meshing damping (Ns·m) | η | lubricant viscosity (Pa·s) |
| ka | Axial compression stiffness (N/m) | δ | Relative displacement (μm) |
| kb | Bending stiffness (N/m) | δ1, δ2 | The roughness amplitudes of surface 1 and surface 2 (mm) |
| kf | Tooth base stiffness (N/m) | r | reference radius (mm) |
| kh | Hertz contact stiffness (N/m) | rρ | Fillet radius at the top of the tool (mm) |
| ks | Shear stiffness (N/m) | ||
| kz and cz | Support stiffness (N/m) and support damping (Ns·m) | ||
| L | Tooth width (mm) | ||
| l | Friction arm (mm) | ||
| M | Module (mm) | ||
| m | Quality (kg) | ||
| n1, n2 | Rotational velocity of the pinion and gear (mm) | ||
| p | film pressure (Pa) | ||
| pc | Direct rough contact load (Pa) | ||
| Hertzian contact pressure (Pa) | |||
| R | The equivalent radius of curvature (mm) |
References
- Liu, H.; Liu, H.; Zhu, C.; Tang, J. Study on gear contact fatigue failure competition mechanism considering tooth wear evolution. Tribol. Int. 2020, 147, 106277. [Google Scholar] [CrossRef]
- Liu, H.; Liu, H.; Zhu, C.; Parker, R. Effects of lubrication on gear performance: A review. Mech. Mach. Theory 2020, 145, 82–90. [Google Scholar] [CrossRef]
- Chen, Z.; Ji, P. Research on the variation of mesh stiffness and transmission error for spur gear with tooth profile modification and wear fault. Eng. Fail. Anal. 2021, 122, 105184. [Google Scholar] [CrossRef]
- Wang, H.; Zhou, C.; Lei, Y.; Liu, Z. An adhesive wear model for helical gears in line-contact mixed elastohydrodynamic lubrication. Wear 2019, 426–427, 896–909. [Google Scholar] [CrossRef]
- Zhou, C.; Xing, M.; Hu, B.; Zhaoyao, S. A Modified Wear Model Considering Contact Temperature for Spur Gears in Mixed Elastohydrodynamic Lubrication. Tribol. Lett. 2020, 68, 110. [Google Scholar] [CrossRef]
- Karpat, F.; Ekwaro-Osire, S. Influence of Tip Relief Modification on the Wear of Spur Gears with Asymmetric Teeth. Tribol. Trans. 2008, 51, 581–588. [Google Scholar] [CrossRef]
- Huangfu, Y.; Chen, K.; Ma, H.; Li, X.; Yu, X.; Zhao, B.; Wen, B. Investigation on meshing and dynamic characteristics of spur gears with tip relief under wear fault. Sci. China Technol. Sci. 2019, 62, 1948–1960. [Google Scholar] [CrossRef]
- Liu, G.; Parker, R.G. Impact of tooth friction and its bending effect on gear dynamics. J. Sound Vib. 2009, 320, 1039–1063. [Google Scholar] [CrossRef]
- Feng, K.; Borghesani, P.; Smith, W.A.; Randall, R.B.; Chin, Z.Y.; Ren, J.; Peng, Z. Vibration-based updating of wear prediction for spur gears. Wear 2019, 426–427, 1410–1415. [Google Scholar] [CrossRef]
- Liu, X.; Yang, Y.; Zhang, J. Investigation on coupling effects between surface wear and dynamics in a spur gear system. Tribol. Int. 2016, 101, 383–394. [Google Scholar] [CrossRef]
- Yesilyurt, I.; Gu, F.; Ball, A. Gear tooth stiffness reduction measurement using modal analysis and its use in wear fault severity assessment of spur gears. NDT & E Int. 2003, 36, 357–372. [Google Scholar]
- Ding, H.; Kahraman, A. Interactions between nonlinear spur gear dynamics and surface wear. J. Sound Vib. 2007, 307, 662–679. [Google Scholar] [CrossRef]
- Osman, T.; Velex, P. Static and dynamic simulations of mild abrasive wear in wide-faced solid spur and helical gears. Mech. Mach. Theory 2010, 45, 911–924. [Google Scholar] [CrossRef]
- Yuksel, C.; Kahraman, A. Dynamic tooth loads of planetary gear sets having tooth profile wear. Mech. Mach. Theory 2004, 39, 695–715. [Google Scholar] [CrossRef]
- Wang, X.S.; Wu, S.J.; Zhou, X.H.; Hu, J.C. Nonlinear dynamics analysis of gear transmission system with wear fault. J. Vib. Shock 2013, 32, 37–43. [Google Scholar]
- Shen, Z.; Qiao, B.; Yang, L.; Luo, W.; Yan, R.; Chen, X. Dynamic modeling of planetary gear set with tooth surface wear. Procedia Manuf. 2020, 49, 49–54. [Google Scholar] [CrossRef]
- Zhang, Z.; Zheng, C.; Wen, M.; Yang, S.; Li, H.; Du, Q. A Wear Prediction Model for Spur Gears Based on the Dynamic Meshing Force and Tooth Profile Reconstruction. In Proceedings of the International Conference on Computer Engineering, Information Science & Application Technology, Wuhan, China, 8–9 July 2017; Atlantis Press: Amsterdam, The Netherlands, 2017. [Google Scholar]
- Zhou, C.; Xiao, Z.; Chen, S.; Han, X. Normal and tangential oil film stiffness of modified spur gear with non-Newtonian elastohydrodynamic lubrication. Tribol. Int. 2016, 109, 319–327. [Google Scholar] [CrossRef]
- Shi, X.; Sun, W.; Lu, X.; Ma, X.; Zhu, D.; Zhao, B.; He, T. Three-dimensional mixed lubrication analysis of spur gears with machined roughness. Tribol. Int. 2019, 140, 105864. [Google Scholar] [CrossRef]
- Zhao, J.; Sheng, W.; Li, Z.; Zhang, H.; Zhu, R. Effect of lubricant selection on the wear characteristics of spur gear under oil-air mixed lubrication. Tribol. Int. 2022, 167, 107382. [Google Scholar] [CrossRef]
- Shi, J.L.; Ma, X.G.; Xu, C.L.; Zang, S.J. Meshing Stiffness Analysis of Gear Using the Ishikawa Method. Appl. Mech. Mater. 2013, 401–403, 203–206. [Google Scholar] [CrossRef]
- Wang, H.; Li, Z.; Zhang, B.; Zhu, R. Calculation of static transmission errors associated with thermo-elastic coupling contacts of spur gears. Vibroeng. Procedia 2018, 20, 237–241. [Google Scholar] [CrossRef]
- Wang, H. Research on Key Incentive Calculation Method for Cylindrical Gear Dynamics; Nanjing University of Aeronautics and Astronautics: Nanjing, China, 2019. [Google Scholar]
- Ren, N.; Zhu, D.; Chen, W.; Liu, Y.; Wang, Q. A Three-Dimensional Deterministic Model for Rough Surface Line-Contact EHL Problems. J. Tribol. 2009, 131, 011501. [Google Scholar] [CrossRef]
- Hu, Y.Z.; Wang, H.; Wang, W.Z.; Zhu, D. A computer model of mixed lubrication in point contacts. Tribol. Int. 2001, 34, 65–73. [Google Scholar] [CrossRef]
- Hu, Y.Z.; Zhu, D. A Full Numerical Solution to the Mixed Lubrication in Point Contacts. J. Tribol. 1999, 122, 1–9. [Google Scholar] [CrossRef]
- Liu, S.; Wang, Q.; Liu, G. A versatile method of discrete convolution and FFT (DC-FFT) for contact analyses. Wear 2000, 243, 101–111. [Google Scholar] [CrossRef]
- Zhu, D.; Martini, A.; Wang, W.; Hu, Y.; Lisowsky, B.; Wang, Q.J. Simulation of Sliding Wear in Mixed Lubrication. J. Tribol. 2007, 129, 544–552. [Google Scholar] [CrossRef]
- Zhu, D.; Liu, Y.; Wang, Q. On the Numerical Accuracy of Rough Surface EHL Solution. Tribol. Trans. 2014, 57, 570–580. [Google Scholar] [CrossRef]
- Zhao, J.; Li, Z.; Zhang, H.; Zhu, R. Effect of micro-textures on lubrication characteristics of spur gears under 3D line-contact EHL model. Ind. Lubr. Tribol. 2021, 73, 1132–1145. [Google Scholar] [CrossRef]
- Pu, W. Effect of surface topography associated with arbitrary velocity direction on the lubrication film thickness in elliptical contacts. Ind. Lubr. Tribol. 2018, 70, 444–452. [Google Scholar] [CrossRef]
- Yan, X.-L.; Wang, X.-L.; Zhang, Y.-Y. A Parametric Study on Fatigue Life for Mixed Elastohydrodynamic Lubrication Point Contacts. J. Tribol. 2013, 135, 04150. [Google Scholar] [CrossRef]
- Zhou, S.; Song, G.; Sun, M.; Ren, Z. Nonlinear dynamic response analysis on gear-rotor-bearing transmission system. J. Vib. Control 2016, 24, 1632–1651. [Google Scholar] [CrossRef]







| Pinion | Gear | |
|---|---|---|
| Tooth number Z | 37 | 53 |
| Pressure angle/° | 20 | |
| Module/mm | 3 | |
| Tooth width/mm | 12 | 12 |
| Quality/kg | 0.907 | 1.863 |
| Moment of inertia | 0.0014 | 0.0058 |
| Pitch error fpt/μm | 4.5 | |
| Tooth profile error ff/μm | 5 | |
| Tooth direction error fb/μm | 5.5 | |
| Center distance installation error fa/μm | 6 | |
| Support damping cz/(Ns·m) | 1.8 × 105 | |
| Support stiffness kz/N/m) | 5 × 109 | |
| Meshing stiffness km/(N/m) | 5.2 × 108 | |
| Mesh damping cm/(Ns·m) | 2765 | |
| Name | |
|---|---|
| Density (kg/m3) | 7860 |
| Hardness (GPa) | 2.7 |
| Poisson’s ratio | 0.29 |
| Young’s modulus (GPa) | 210 |
| wear coefficient | 0.018 |
| Indicators | |
|---|---|
| Measurement Mode | VSI, PSI, VXI |
| Vertical measurement range | 0.1 nm–10 mm |
| Vertical Resolution | ≤0.1 nm |
| Maximum travel in z-direction | 150 mm |
| Sample table moving stroke | 150 mm × 150 mm |
| Scanning speed | >47 um/s |
| Sample stage tilt range | ±6° |
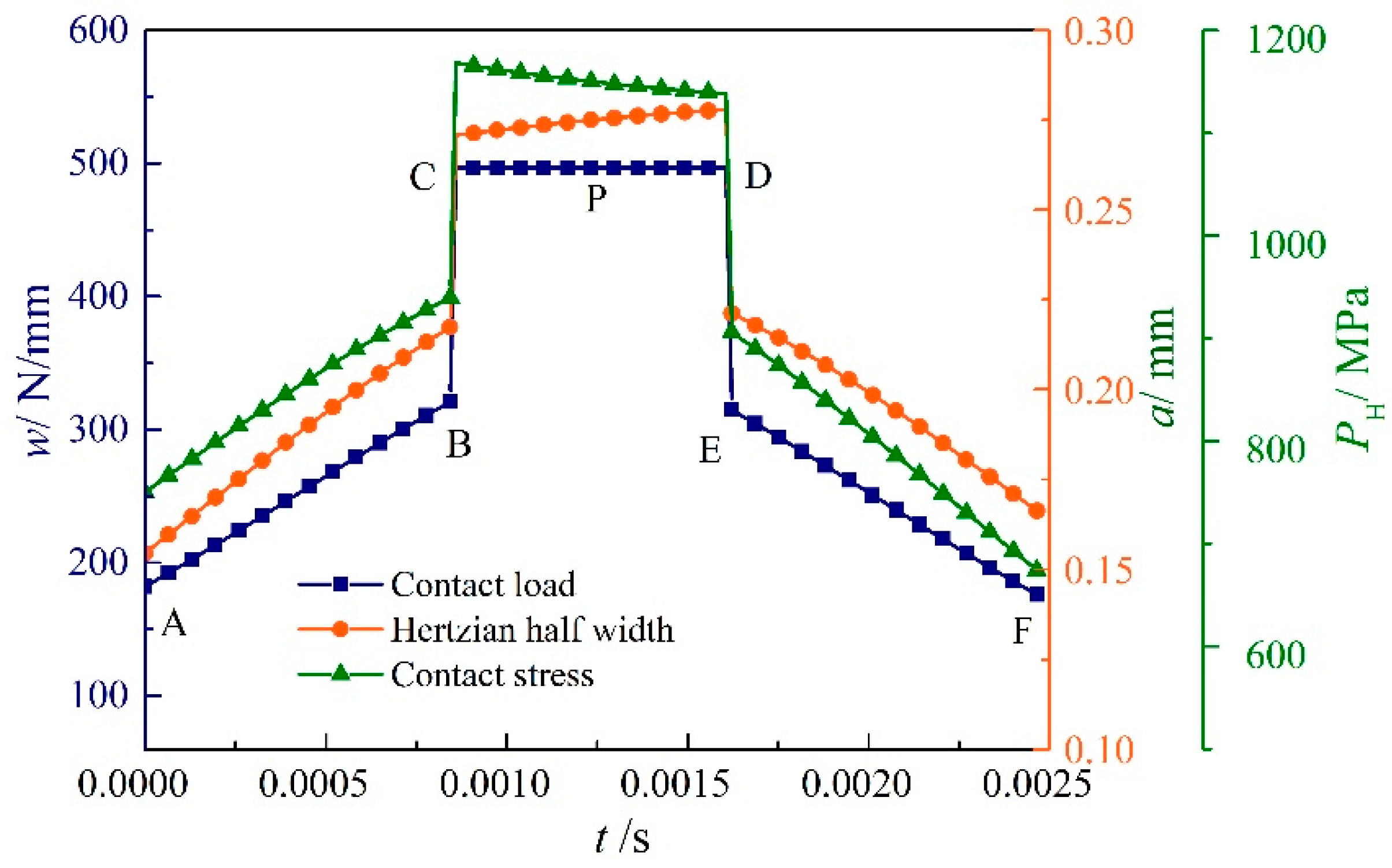
| A | B | C | P | D | E | F | |
|---|---|---|---|---|---|---|---|
| Contact load (N/mm) | 187.836 | 317.735 | 500 | 500 | 500 | 352.164 | 222.265 |
| Relative sliding speed (m/s) | 1.112 | 0.192 | 0.179 | 0 | 0.149 | 0.162 | 1.069 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhao, J.; Yu, X.; Sheng, W.; Li, Z.; Zhang, H.; Zhu, R. Effect of Machined Tooth Surface Mixed Lubrication Sliding Wear on Gear Dynamic Characteristics. Machines 2023, 11, 25. https://doi.org/10.3390/machines11010025
Zhao J, Yu X, Sheng W, Li Z, Zhang H, Zhu R. Effect of Machined Tooth Surface Mixed Lubrication Sliding Wear on Gear Dynamic Characteristics. Machines. 2023; 11(1):25. https://doi.org/10.3390/machines11010025
Chicago/Turabian StyleZhao, Jiang, Xiaofeng Yu, Wei Sheng, Zhengminqing Li, Hong Zhang, and Rupeng Zhu. 2023. "Effect of Machined Tooth Surface Mixed Lubrication Sliding Wear on Gear Dynamic Characteristics" Machines 11, no. 1: 25. https://doi.org/10.3390/machines11010025
APA StyleZhao, J., Yu, X., Sheng, W., Li, Z., Zhang, H., & Zhu, R. (2023). Effect of Machined Tooth Surface Mixed Lubrication Sliding Wear on Gear Dynamic Characteristics. Machines, 11(1), 25. https://doi.org/10.3390/machines11010025






