Effect of the Inclined Part Length of an Inclined Blade on the Cavitation Characteristics of Vortex Pumps
Abstract
1. Introduction
2. Numerical Calculation Method
2.1. Model of the Fluid Domain
2.2. Mesh Partitioning
2.3. Cavitation Model and Parameter Setting
3. Cavitation Flow Analysis of the Vortex Pumps
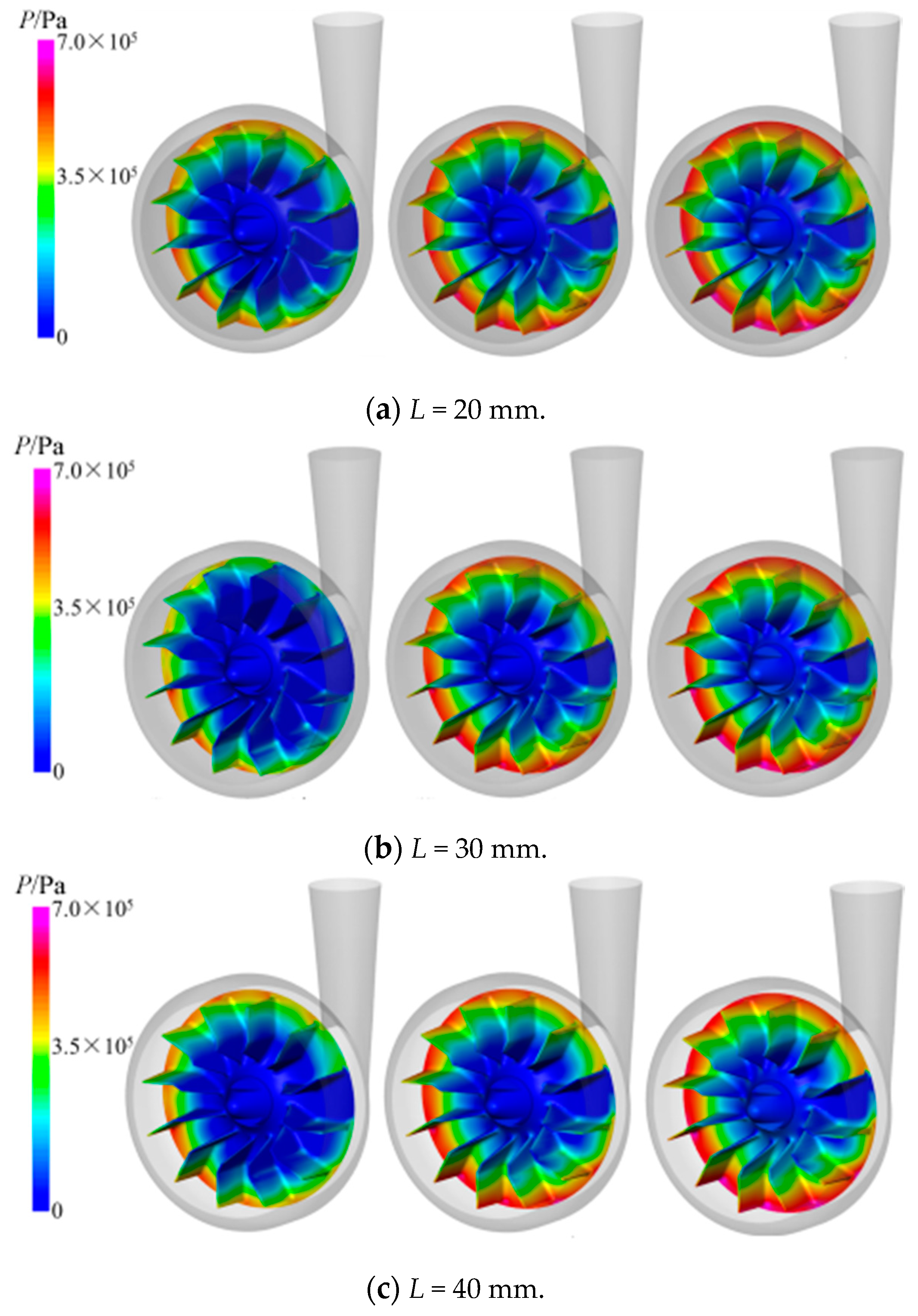
3.1. Analysis of the Static Pressure Distribution of the Vortex Pump under Different Inlet Total Pressures
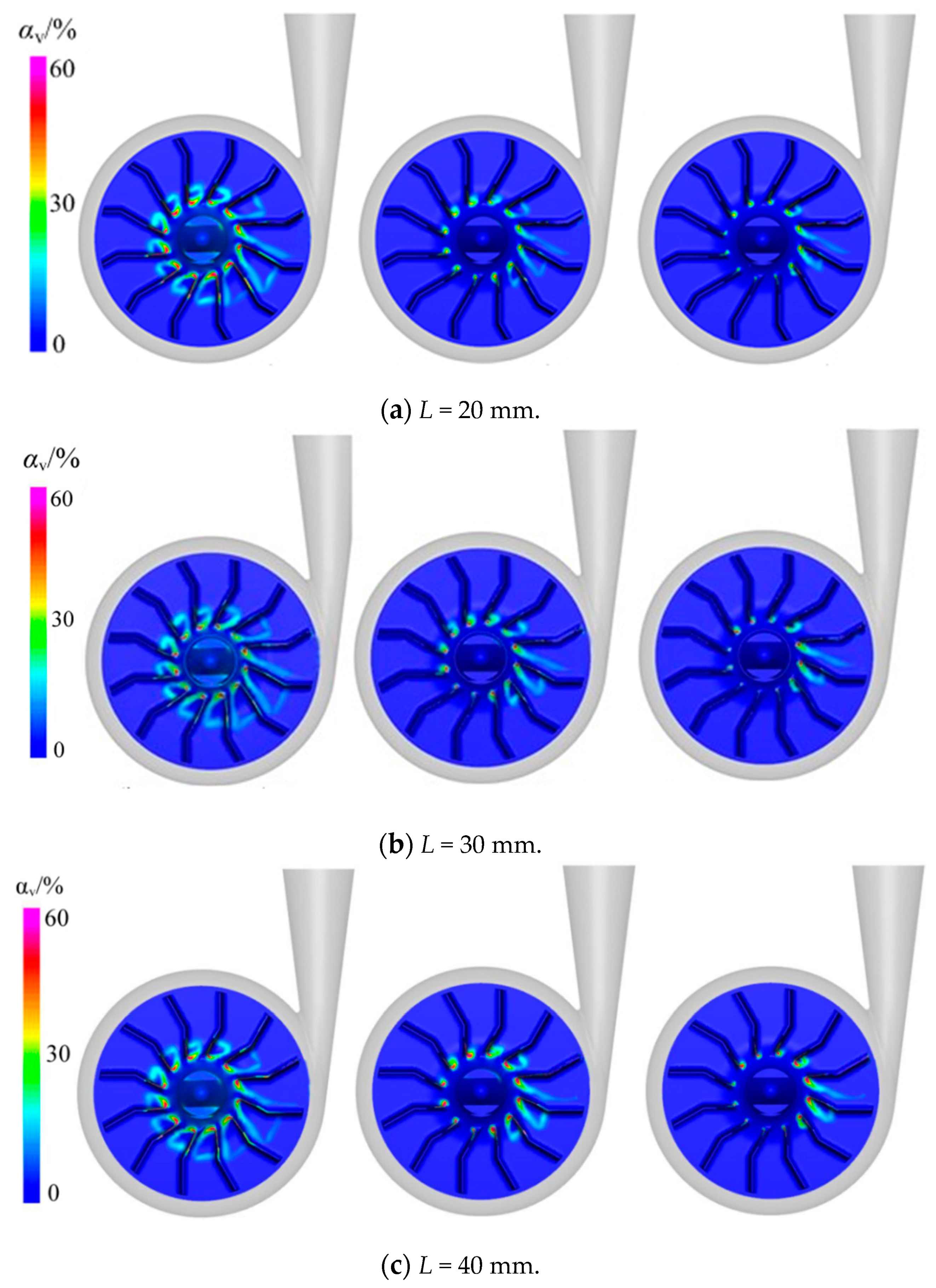
3.2. Analysis of the Bubble Volume Fraction Distribution of the Vortex Pump Impeller under Different Inlet Total Pressures
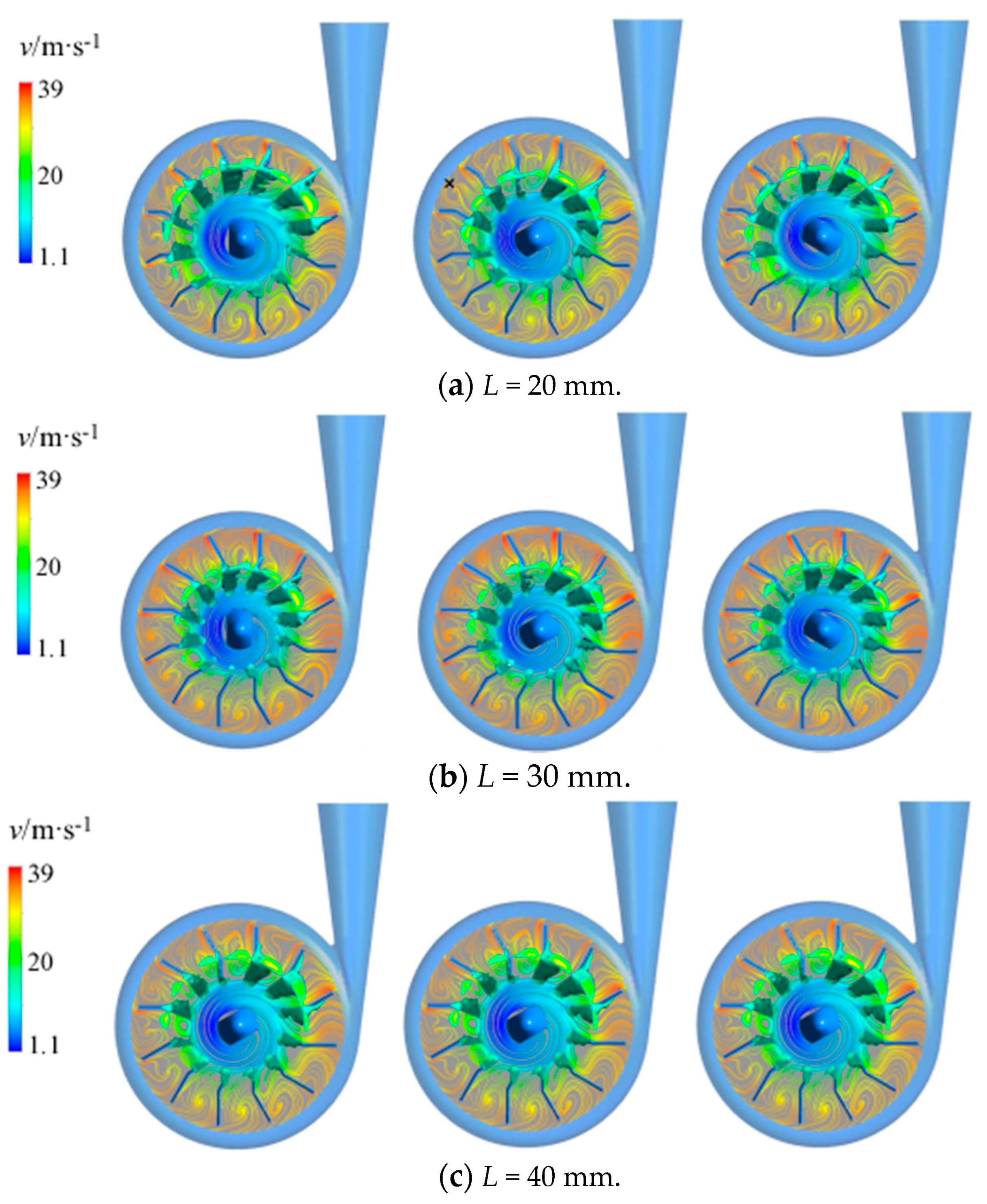
3.3. Analysis of the Flow Streamline and Bubble Distribution of the Vortex Pump Impeller under Different Instants
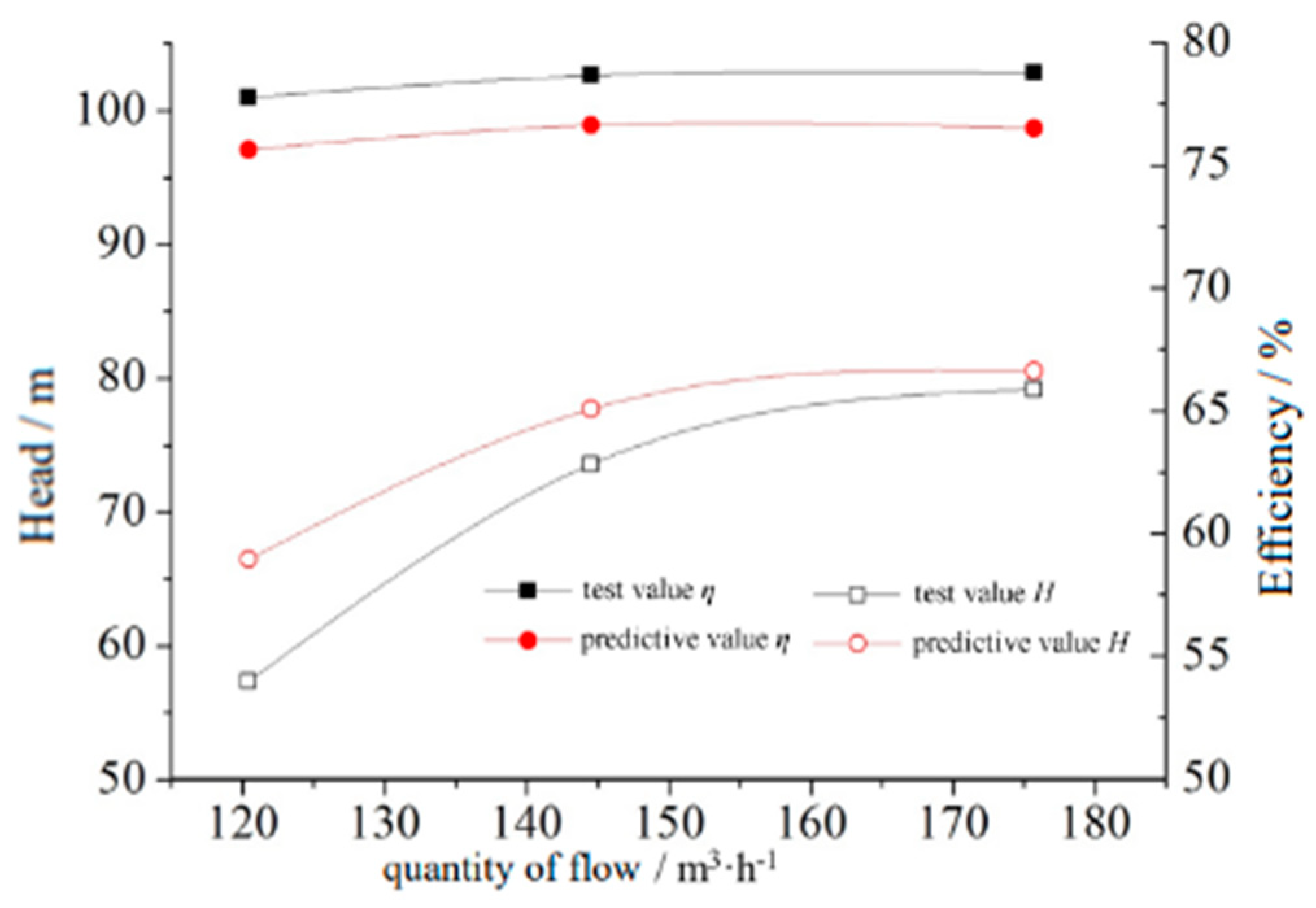
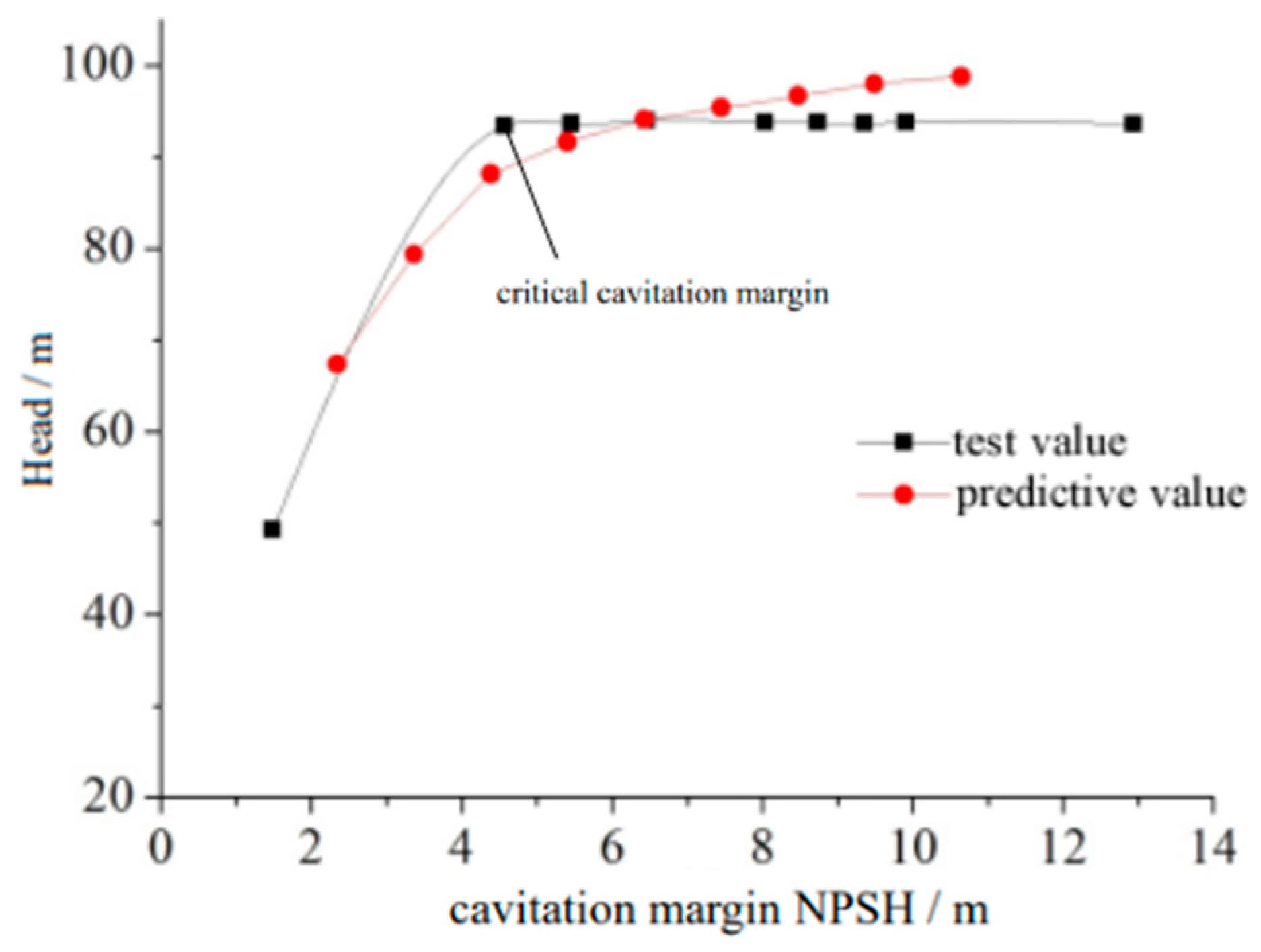
4. Test Verification
5. Conclusions
- (1)
- For the vortex pump with the same inlet total pressure, the longer the second inclined part length of the inclined blade, the larger the low-pressure region inside the flow passage, and this led to more fluid vaporization. When the vaporization bubble reached the high-pressure region, the volume decreased sharply and resulted in a burst, causing severe cavitation; therefore, a shorter second inclined part length could improve the pressure distribution inside the vortex pump flow passage to reduce the possibility of cavitation.
- (2)
- With the increase in the length of the second inclined part of the inclined blade, the bubble volume fraction in the flow passage of the vortex pump increased under the same inlet total pressure inside. The shorter the second inclined part length, the smaller the bubble volume fraction in the flow passage, resulting in a better cavitation performance of the vortex pump. When the inlet total pressure was too low, the change in the length of the second inclined part could not restrain the occurrence of cavitation, leading to severe cavitation.
- (3)
- When the impeller inlet total pressure was low, resulting in a large number of vortices in the flow passage, the hole generated in the low-pressure region of the vortex led to severe impact and vortex cavitation, which was the reason for the serious cavitation of the vortex pump. With the increase in the length of the second inclined part of the inclined blade, the increase in the rate of change of the fluid velocity and the worse flow separation led to more intense cavitation.
- (4)
- The results of the test and numerical calculation were consistent; before the critical cavitation margin, with the decrease in the cavitation margin, the pump head slowly decreased, while after reaching the critical cavitation margin, the head declined sharply.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Quan, H.; Li, Y.; Kang, L.; Yu, X.; Song, K.; Wu, Y. Influence of Blade Type on the Flow Structure of a Vortex Pump for Solid-Liquid Two-Phase Flow. Machines 2021, 9, 353. [Google Scholar] [CrossRef]
- Peng, G.; Chen, Q.; Zhou, L.; Pan, B.; Zhu, Y. Effect of blade outlet angle on the flow field and preventing overload in a centrifugal pump. Micromachines 2020, 11, 811. [Google Scholar] [CrossRef] [PubMed]
- Xu, Y.; Tan, L.; Liu, Y.; Cao, S. Pressure fluctuation and flow pattern of a mixed-flow pump with different blade tip clearances under cavitation condition. Adv. Mech. Eng. 2017, 9, 1687814017696227. [Google Scholar] [CrossRef]
- Li, W. Vortex Pump as Turbine for Energy Recovery in Viscous Fluid Flows With Reynolds Number Effect. J. Fluids Eng. 2022, 144, 021207. [Google Scholar] [CrossRef]
- He, C.; Gu, Y.; Zhang, J.; Ma, L.; Yan, M.; Mou, J.; Ren, Y. Preparation and modification technology analysis of ionic polymer-metal composites (IPMCs). Int. J. Mol. Sci. 2022, 23, 3522. [Google Scholar] [CrossRef] [PubMed]
- Gu, Y.; Yu, L.; Mou, J.; Wu, D.; Xu, M.; Zhou, P.; Ren, Y. Research strategies to develop environmentally friendly marine antifouling coatings. Mar. Drugs 2020, 18, 371. [Google Scholar] [CrossRef]
- Svoboda, D.G.; Zharkovskii, A.A.; Ivanov, E.A. Influence of the Geometric Parameters of the Impeller of a Free-Vortex Pump on the Energy and Cavitation Characteristics of the Pump. Chem. Pet. Eng. 2019, 54, 673–680. [Google Scholar] [CrossRef]
- Gao, X.; Zhao, T.; Shi, W.; Zhang, D.; Shi, Y.; Zhou, L.; Chang, H. Numerical investigation of an open-design vortex pump with different blade wrap angles of impeller. Processes 2020, 8, 1601. [Google Scholar] [CrossRef]
- Huan, Y.; Liu, Y.; Li, X.; Zhu, Z.-C.; Qu, J.-T.; Zhe, L.; Han, A.-D. Experimental and numerical investigations of cavitation evolution in a high-speed centrifugal pump with inducer. J. Hydrodyn. 2021, 33, 140–149. [Google Scholar] [CrossRef]
- Kondić, Ž.; Medić, S.; Kondić, V. Experimental and Numerical Investigation of Centrifugal Vortex Pump Operating Benefits for Energy Efficient Systems. Teh. Vjesn. 2020, 27, 1519–1523. [Google Scholar]
- Cheng, X.; Chang, Z.; Jiang, Y. Study on the influence of the specific area of balance hole on cavitation performance of high-speed centrifugal pump. J. Mech. Sci. Technol. 2020, 34, 3325–3334. [Google Scholar] [CrossRef]
- Ye, D.; Li, H.; Ma, Q.; Han, Q.; Sun, X. Numerical Investigation of Performance Improvement and Erosion Characteristics of Vortex Pump Using Particle Model. Shock. Vib. 2020, 2020, 5103261. [Google Scholar] [CrossRef]
- Ju, Y.; Liu, S.; Zhang, C. Effect of blade shape on hydraulic performance and vortex structure of vortex pumps. J. Hydrodyn. 2018, 30, 499–506. [Google Scholar] [CrossRef]
- Quan, H.; Guo, Y.; Li, R.; Su, Q.; Chai, Y. Optimization design and experimental study of vortex pump based on orthogonal test. Sci. Prog. 2020, 103, 0036850419881883. [Google Scholar] [CrossRef]
- Gu, Y.; Zhang, J.; Yu, S.; Mou, C.; Li, Z.; He, C.; Wu, D.; Mou, J.; Ren, Y. Unsteady numerical simulation method of hydrofoil surface cavitation. Int. J. Mech. Sci. 2022, 228, 107490. [Google Scholar] [CrossRef]
- Guo, C.; Gao, M.; Wang, J.; Shi, Y.; He, S. The effect of blade outlet angle on the acoustic field distribution characteristics of a centrifugal pump based on Powell vortex sound theory. Appl. Acoust. 2019, 155, 297–308. [Google Scholar] [CrossRef]
- Yan, D.; Kovacevic, A.; Tang, Q.; Rane, S. Numerical investigation of cavitation in twin-screw pumps. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2018, 232, 3733–3750. [Google Scholar] [CrossRef]
- Han, C.; Xu, S.; Cheng, H.; Ji, B.; Zhang, Z.-Y. LES method of the tip clearance vortex cavitation in a propelling pump with special emphasis on the cavitation-vortex interaction. J. Hydrodyn. 2020, 32, 1212–1216. [Google Scholar] [CrossRef]
- Li, J.; Tang, L.; Zhang, Y. The influence of blade angle on the performance of plastic centrifugal pump. Adv. Mater. Sci. Eng. 2020, 2020, 7205717. [Google Scholar] [CrossRef]
- Cheng, H.; Ji, B.; Long, X.; Huai, W.-X.; Farhat, M. A review of cavitation in tip-leakage flow and its control. J. Hydrodyn. 2021, 33, 226–242. [Google Scholar] [CrossRef]
- Quan, H.; Wu, Y.; Guo, Y.; Mastroroberto, P.; Speziale, G.; Nasso, G. Multiobjective Hydraulic Design and Performance Analysis of a Vortex Pump Based on Orthogonal Tests. Shock Vib. 2021, 2021, 6687856. [Google Scholar] [CrossRef]
- Condello, I.; Santarpino, G.; Serraino, G.F.; Mastroroberto, P.; Speziale, G.; Nasso, G. Magnetic levitation pump versus constrained vortex pump: A pilot study on the hemolysis effect during minimal invasive extracorporeal circulation. J. Cardiothorac. Surg. 2021, 16, 1–4. [Google Scholar] [CrossRef] [PubMed]
- Rosanov, N.N.; Fedorov, S.V. Topology of energy fluxes in vortex dissipative soliton structures. J. Opt. 2016, 18, 074005. [Google Scholar] [CrossRef]
- Sookhak, M.; Gani, A.; Talebian, H.; Akhunzada, A.; Khan, S.U.; Buyya, R.; Zomaya, A.Y. Remote Data Auditing in Cloud Computing Environments: A Survey, Taxonomy, and Open Issues. Acm Comput. Surv. 2015, 47, 65. [Google Scholar] [CrossRef]
- Dratman, E.; Matera, G. Newton’s method and a mesh-independence principle for certain semilinear boundary-value problems. J. Comput. Appl. Math. 2016, 292, 188–212. [Google Scholar] [CrossRef]
- Zhao, Y.; Wang, G.; Huang, B. A cavitation model for computations of unsteady cavitating flows. Acta Mech. Sin. 2016, 32, 273–283. [Google Scholar] [CrossRef]
- Chen, G.H.; Wang, G.Y.; Huang, B.; Hu, C.L.; Wang, Z.Y.; Wang, J. Numerical investigation of dynamics of unsteady sheet/cloud cavitating flow using a compressible fluid model. Mod. Phys. Lett. B 2015, 29, 1450269. [Google Scholar] [CrossRef]
- Ye, Y.; Li, G. Modeling of hydrodynamic cavitating flows considering the bubble-bubble interaction. Int. J. Multiph. Flow 2016, 84, 155–164. [Google Scholar] [CrossRef]
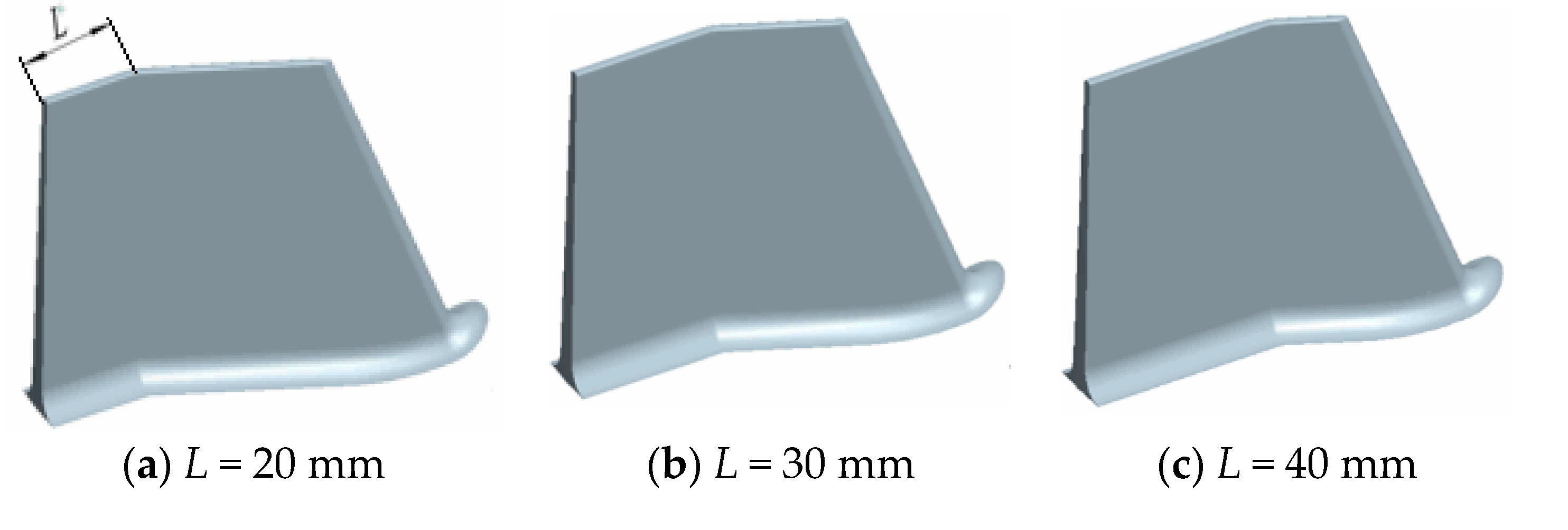
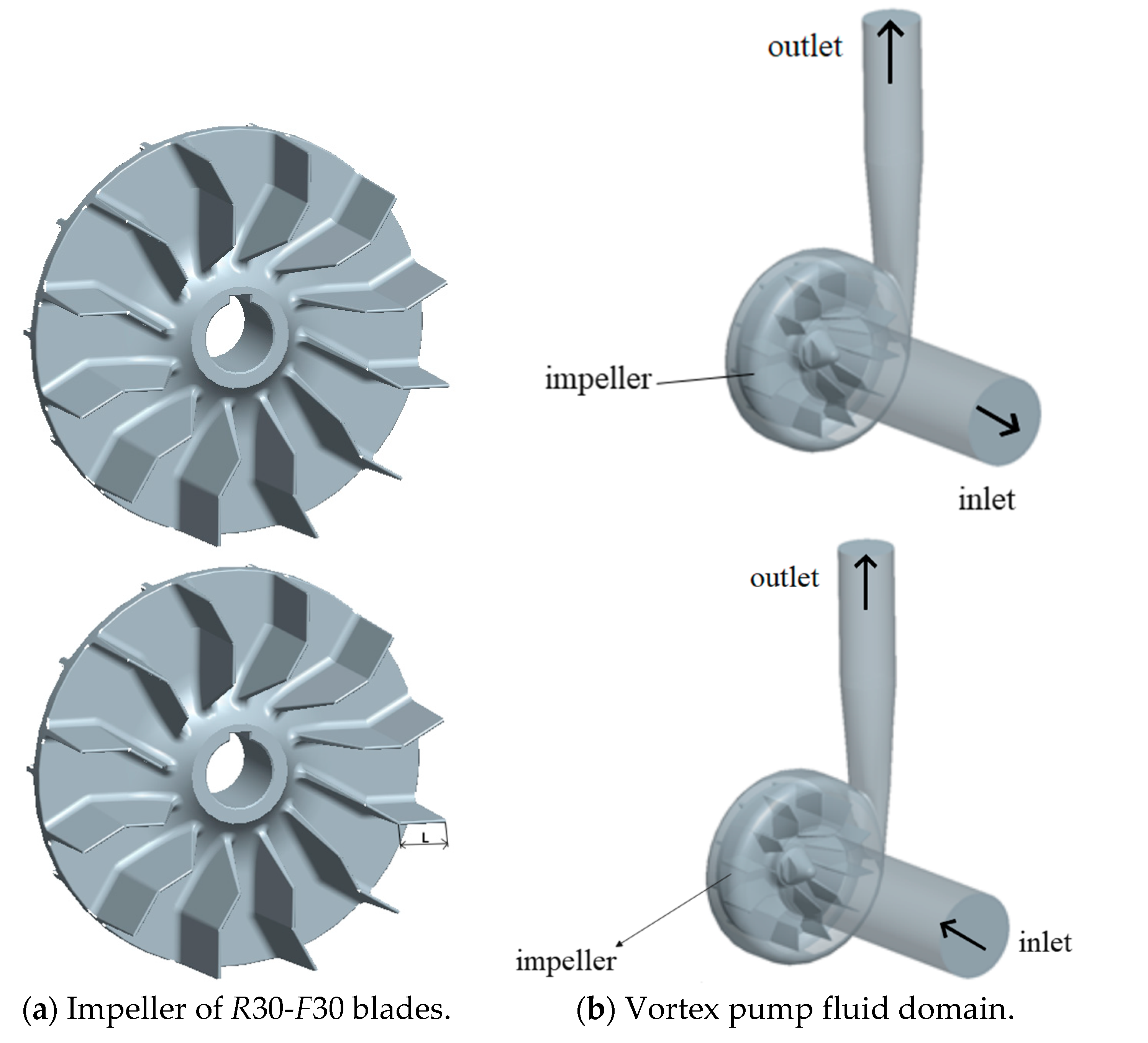
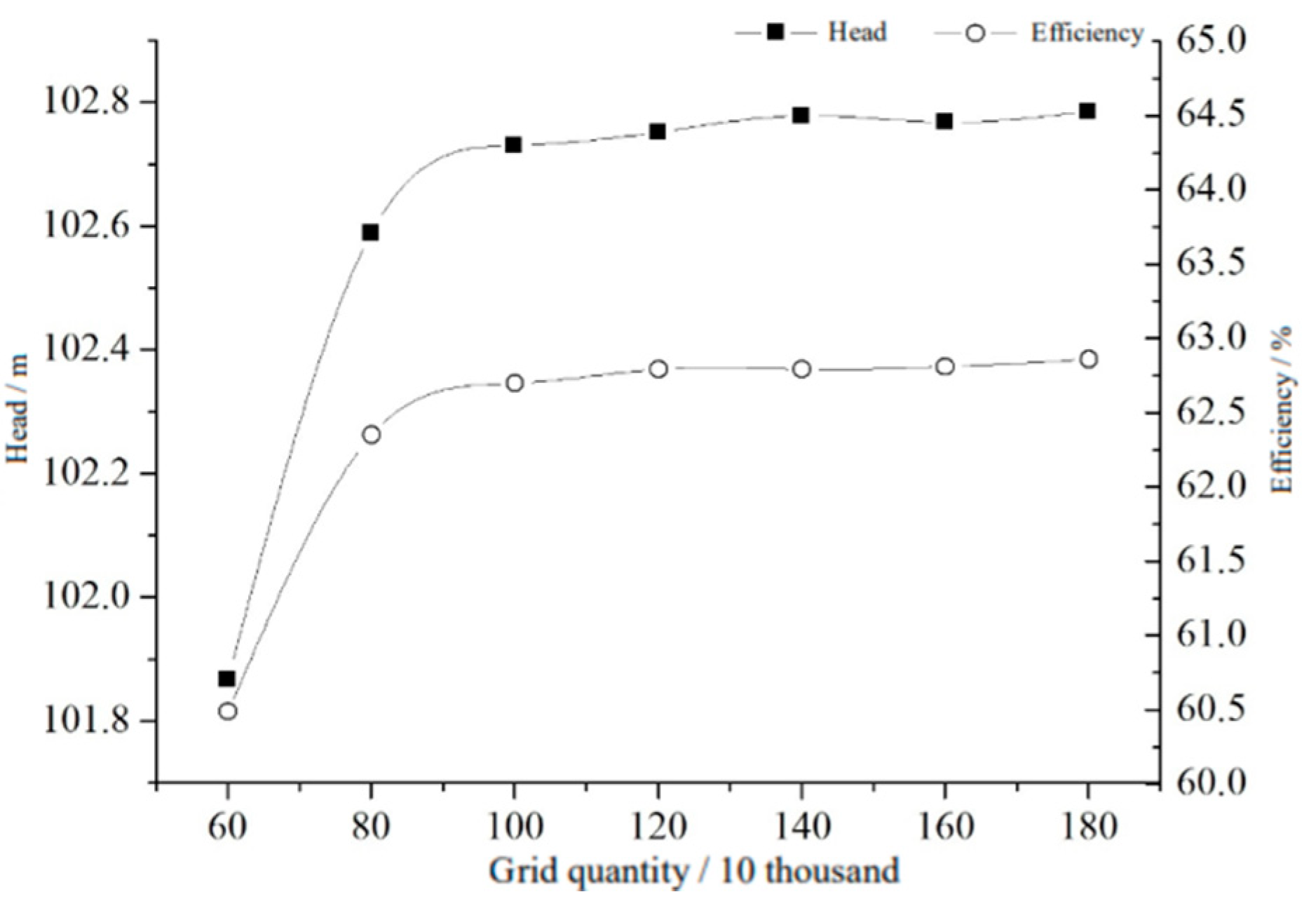

| Inlet Diameter | Outlet Diameter | Impeller Diameter | Flow (Q) | Head (H) | Cavitation Margin (NPSH) | Power (P) |
|---|---|---|---|---|---|---|
| 125 mm | 80 mm | 230 mm | 144 m3/s | 80 m | 3.6 m | 75 kW |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yin, Z.; Gu, Y.; Fan, T.; Li, Z.; Wang, W.; Wu, D.; Mou, J.; Zheng, S. Effect of the Inclined Part Length of an Inclined Blade on the Cavitation Characteristics of Vortex Pumps. Machines 2023, 11, 21. https://doi.org/10.3390/machines11010021
Yin Z, Gu Y, Fan T, Li Z, Wang W, Wu D, Mou J, Zheng S. Effect of the Inclined Part Length of an Inclined Blade on the Cavitation Characteristics of Vortex Pumps. Machines. 2023; 11(1):21. https://doi.org/10.3390/machines11010021
Chicago/Turabian StyleYin, Zhuofan, Yunqing Gu, Tianxing Fan, Zhou Li, Wenting Wang, Denghao Wu, Jiegang Mou, and Shuihua Zheng. 2023. "Effect of the Inclined Part Length of an Inclined Blade on the Cavitation Characteristics of Vortex Pumps" Machines 11, no. 1: 21. https://doi.org/10.3390/machines11010021
APA StyleYin, Z., Gu, Y., Fan, T., Li, Z., Wang, W., Wu, D., Mou, J., & Zheng, S. (2023). Effect of the Inclined Part Length of an Inclined Blade on the Cavitation Characteristics of Vortex Pumps. Machines, 11(1), 21. https://doi.org/10.3390/machines11010021







