A Simplified Method for Inverse Kinematics of a Flexible Panel Continuum Robot for Real-Time Shape Morphing
Abstract
:1. Introduction
2. Kinematic Analysis Based on Large Deformation Beam Theory
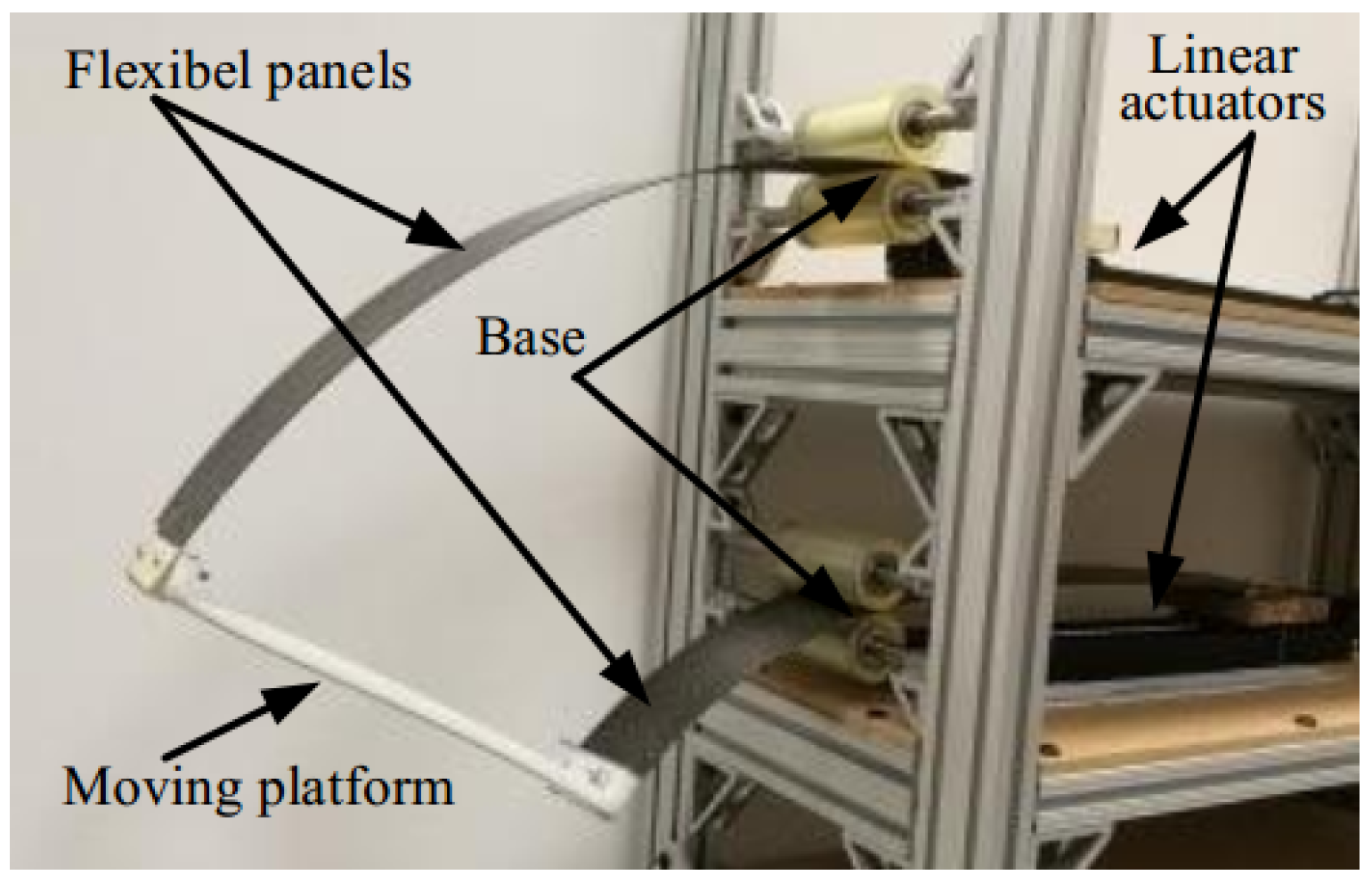
2.1. System Description
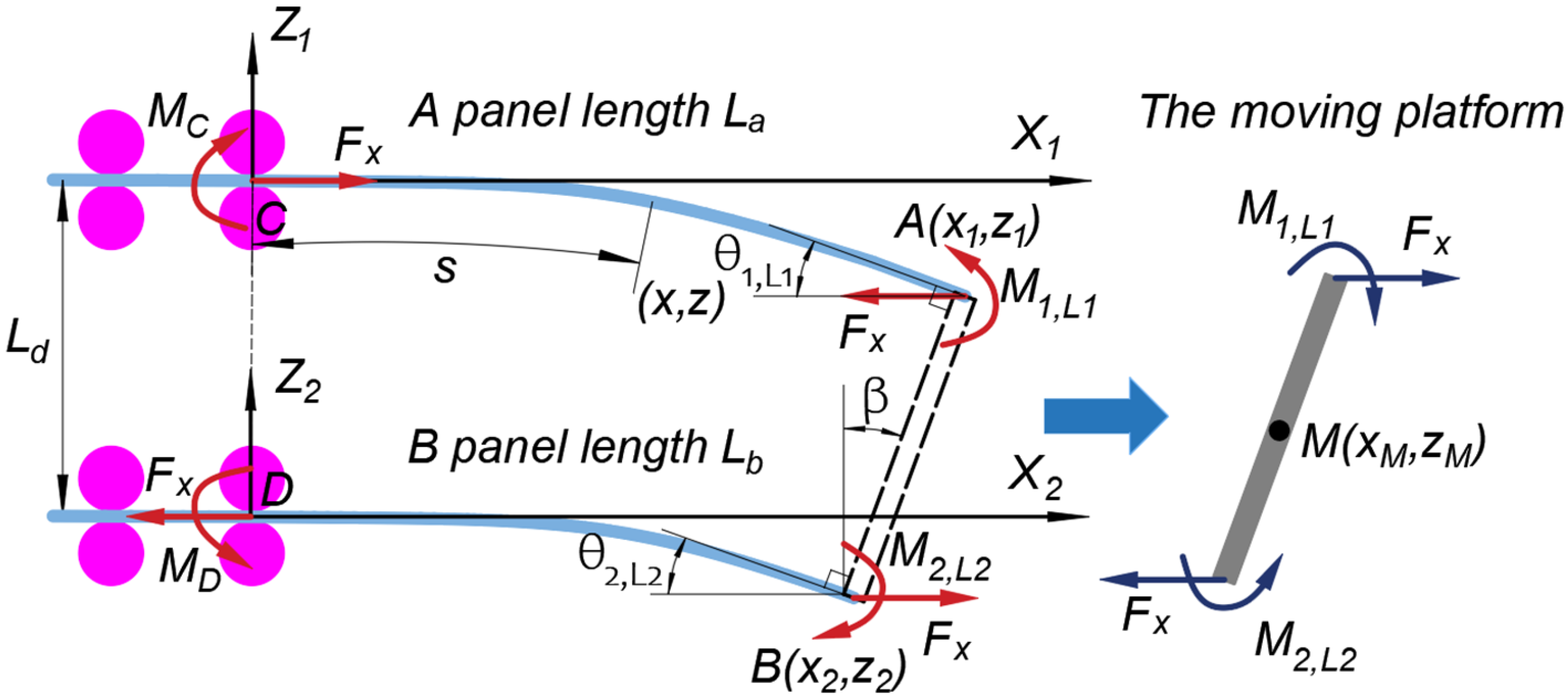
2.2. Review of Forward Kinematic Modeling
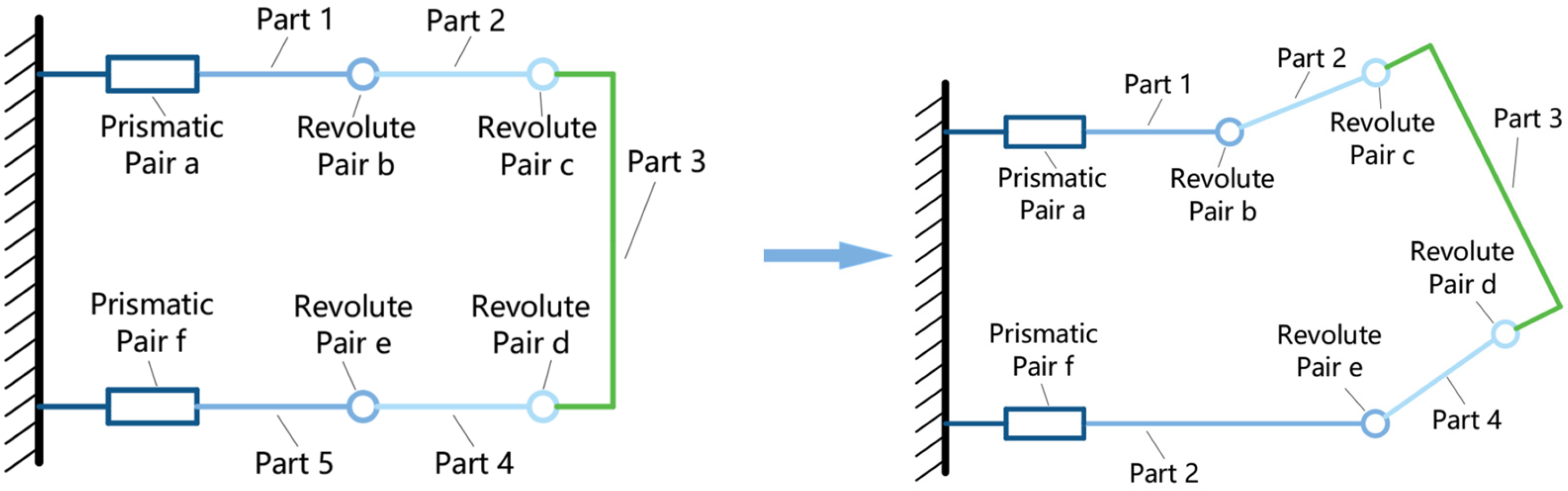
3. Mobility Analysis of the Continuum Robot
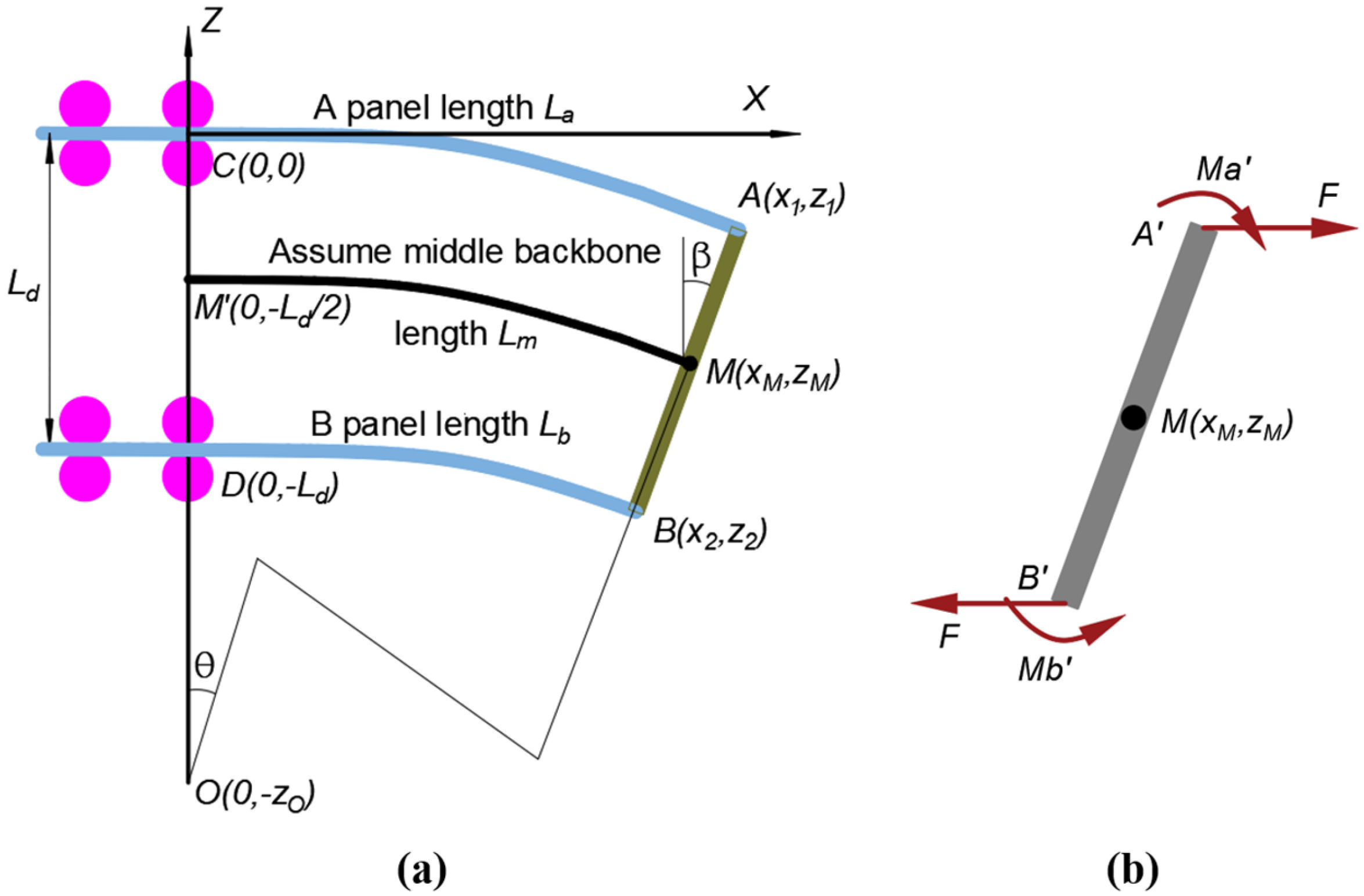
4. A Quasi-Rigid Model for Inverse Kinematics
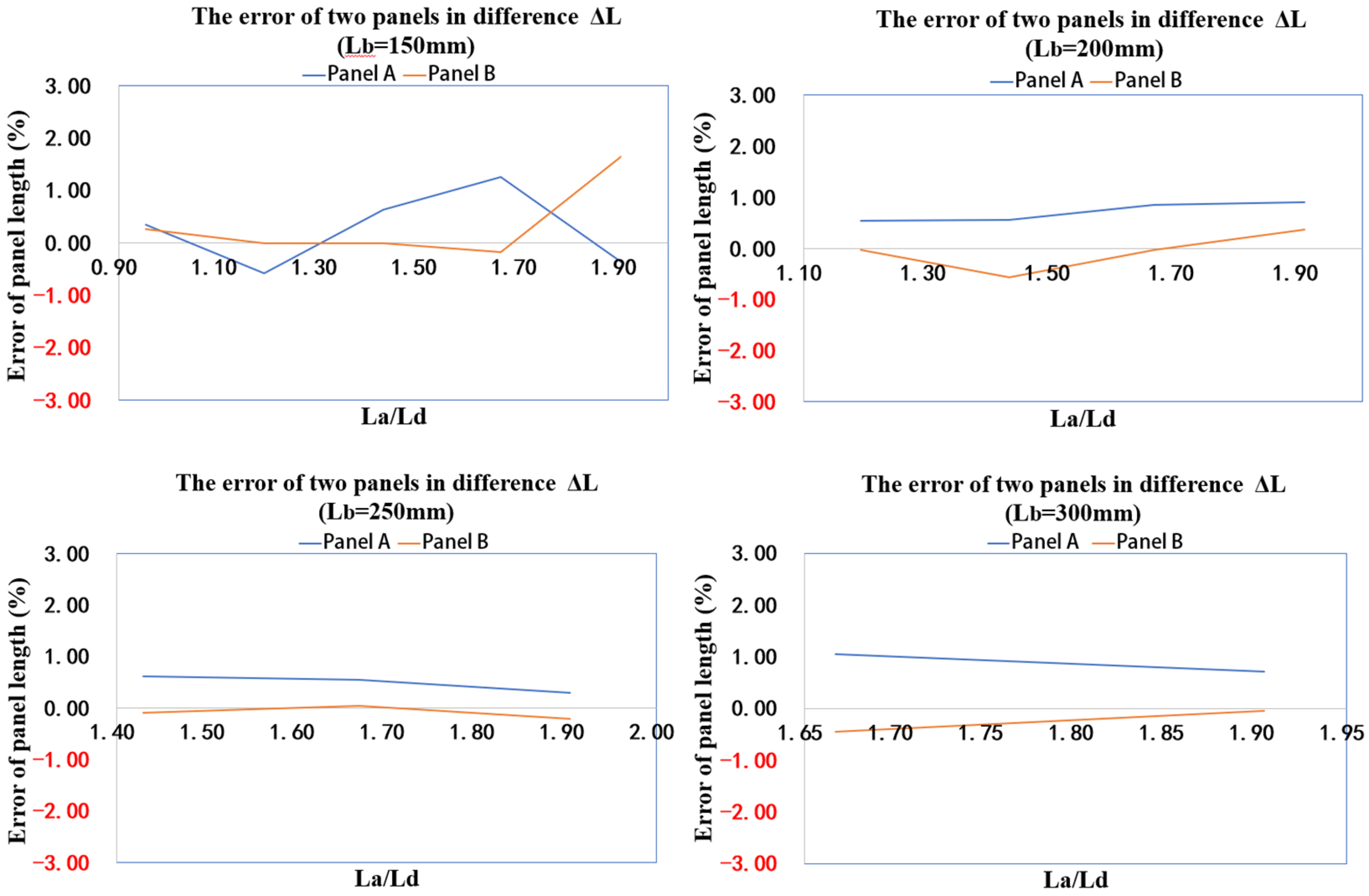
5. Accuracy Analysis of The Method
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Hirose, S.; Mori, M. Biologically inspired snake-like robots. In Proceedings of the IEEE International Conference on Robotics and Biomimetics (ROBIO), Shenyang, China, 22–26 August 2004. [Google Scholar]
- Hannan, M.W.; Walker, I.D. Kinematics and the implementation of an elephant’s trunk manipulator and other continuum style robots. J. Robot. Syst. 2003, 20, 45–63. [Google Scholar] [CrossRef] [PubMed]
- Laschi, C.; Mazzolai, B.; Mattoli, V.; Cianchetti, M.; Dario, P. Design of a biomimetic robotic octopus arm. Bioinspiration Biomim. 2009, 4, 015006. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Walker, I.D.; Dawson, D.M.; Flash, T.; Grasso, F.W.; Hanlon, R.T.; Hochner, B.; Kier, W.M.; Pagano, C.C.; Rahn, C.D.; Zhang, Q.M. Continuum robot arms inspired by cephalopods. In Proceedings SPIE, Defense and Security; SPIE: Orlando, FL, USA, 2005. [Google Scholar]
- Jabbari, M.; Zakeri, M. Motion planning of a continuum robot in medical surgeries. In Proceedings of the 2nd Al Farabi International Congress on Applied Sciences, Nakhchivan, Azerbaijan, 2–4 May 2021. [Google Scholar]
- Robotics, O.C. Snake-arm robots access the inaccessible. Nucl. Technol. 2008, 1, 92–94. [Google Scholar]
- Walker, I.D. Use of continuum robots for remote inspection operations. In Proceedings of the 2017 Computing Conference, London, UK, 18–20 July 2017.
- Xiang, L.Q.; Feng, J.U.; Fei, Q.; Wang, Y.M.; Hua, D.W.; Chen, B. Kinematics research of continuum robot for in-situ detection of aero-engine. J. Mech. Electr. Eng. 2019, 36, 464–469. [Google Scholar]
- Jones, B.A.; Walker, I.D. Practical kinematics for real time implementation of continuum robots. IEEE Trans. Robot. 2006, 22, 1087–1099. [Google Scholar] [CrossRef] [Green Version]
- Jones, B.A.; Walker, I.D. Kinematics for multi-section continuum robots. IEEE Trans. Robot. 2006, 22, 43–55. [Google Scholar] [CrossRef]
- Simaan, N.; Taylor, R.; Flint, P. A dexterous system for laryngeal surgery. In Proceedings of the IEEE International Conference on Robotics and Automation (ICRA), New Orleans, LA, USA, 26 April–1 May 2004. [Google Scholar]
- Godage, I.S.; Guglielmino, E.; Branson, D.T.; Medrano-Cerda, G.A.; Caldwell, D.G. Novel modal approach for kinematics of multisection continuum arms. In Proceedings of the 2011 IEEE/RSJ International Conference on Intelligent Robots and Systems, San Francisco, CA, USA, 25–30 September 2011. [Google Scholar]
- Godage, I.S.; Branson, D.T.; Guglielmino, E.; Medrano-Cerda, G.A.; Caldwell, D.G. Shape function-based kinematics and dynamics for variable length continuum robotic arms. In Proceedings of the IEEE International Conference on Robotics & Automation, Shanghai, China, 9–13 May 2011. [Google Scholar]
- Rolf, M.; Steil, J.J. Constant curvature continuum kinematics as fast approximate model for the bionic handling assistant. In Proceedings of the 2012 IEEE/RSJ International Conference on Intelligent Robots and Systems, Vilamoura-Algarve, Portugal, 7–12 October 2012. [Google Scholar]
- Escande, C.; Merzouki, R.; Pathak, P.M.; Coelen, V. Geometric modelling of multisection bionic manipulator: Experimental validation on robotinoxt. In Proceedings of the 2012 IEEE International Conference on Robotics and Biomimetics (ROBIO), Guangzhou, China, 11–14 December 2012. [Google Scholar]
- Hollerbach, J.M.; Wampler, C.W. The calibration index and taxonomy for robot kinematic calibration methods. Int. J. Robot. Res. 1996, 15, 573–591. [Google Scholar] [CrossRef]
- AGoldenberg; Benhabib, B.; Fenton, R.G. A complete generalized solution to the inverse kinematics of robots. IEEE J. Robot. Autom. 1985, 1, 14–20. [Google Scholar] [CrossRef]
- Giorelli, M.; Renda, F.; Ferri, G.; Laschi, C. A feed-forward neural network learning the inverse kinetics of a flexible cable-driven manipulator moving in three-dimensional space. In Proceedings of the EEE/RSJ International Conference on Intelligent Robots and Systems. IEEE/RSJ International Conference on Intelligent Robots and Systems, Tokyo, Japan, 23–27 October 2013. [Google Scholar]
- Rolf, M.; Steil, J.J. Efficient exploratory learning of inverse kinematics on a bionic elephant trunk. IEEE Trans. Neural Netw. Learn. Syst. 2014, 25, 1147–1160. [Google Scholar]
- Mahl, T.; Hildebrandt, A.; Sawodny, O. Forward kinematics of a compliant pneumatically actuated redundant manipulator. In Proceedings of the 2012 7th IEEE Conference on Industrial Electronics and Applications (ICIEA), Singapore, 18–20 June 2012. [Google Scholar]
- Wang, W.; Xi, F.; Tian, Y.; Zhao, Y. Modeling and Analysis of a planar flexible panel continuum mechanism. ASME J. Mech. Robot. 2020, 12, 044503. [Google Scholar] [CrossRef]
- Howell, L.; Midha, A. Parametric deflection approximations for end loaded large deflection beams in compliant mechanisms. ASME Trans. J. Mech. Des. 1995, 117, 156–165. [Google Scholar] [CrossRef]
- Howell, L.; Midha, A. Evaluation of equivalent spring stiffness for use in a pseudo-rigid-body model of large deflection compliant mechanisms. ASME Trans. J. Mech. Des. 1996, 118, 126–131. [Google Scholar] [CrossRef] [Green Version]
- Midha, A.; Howell, L. A method for the design of compliant mechanism with small-length flexural pivots. ASME Trans. J. Mech. Des. 1994, 116, 280–290. [Google Scholar]
- Zhonglei, F.; Yueqing, Y.; Wenjing, W. 2R Pseudo-rigid-body Model of Compliant Mechanisms with Compliant Links to Simulate Tip Characteristic. J. Mech. Eng. 2011, 47, 37–42. [Google Scholar]
- Su, H.-J. A pseudo-rigid-body 3R model for determining large deflection of cantilever beams subject to tip loads. ASME J. Mech. Robot. 2009, 1, 021008. [Google Scholar] [CrossRef]
- Yu, Y. New PR pseudo-rigid-body model of compliant mechanisms subject to combined loads. J. Mech. Eng. 2013, 49, 9–14. [Google Scholar] [CrossRef]
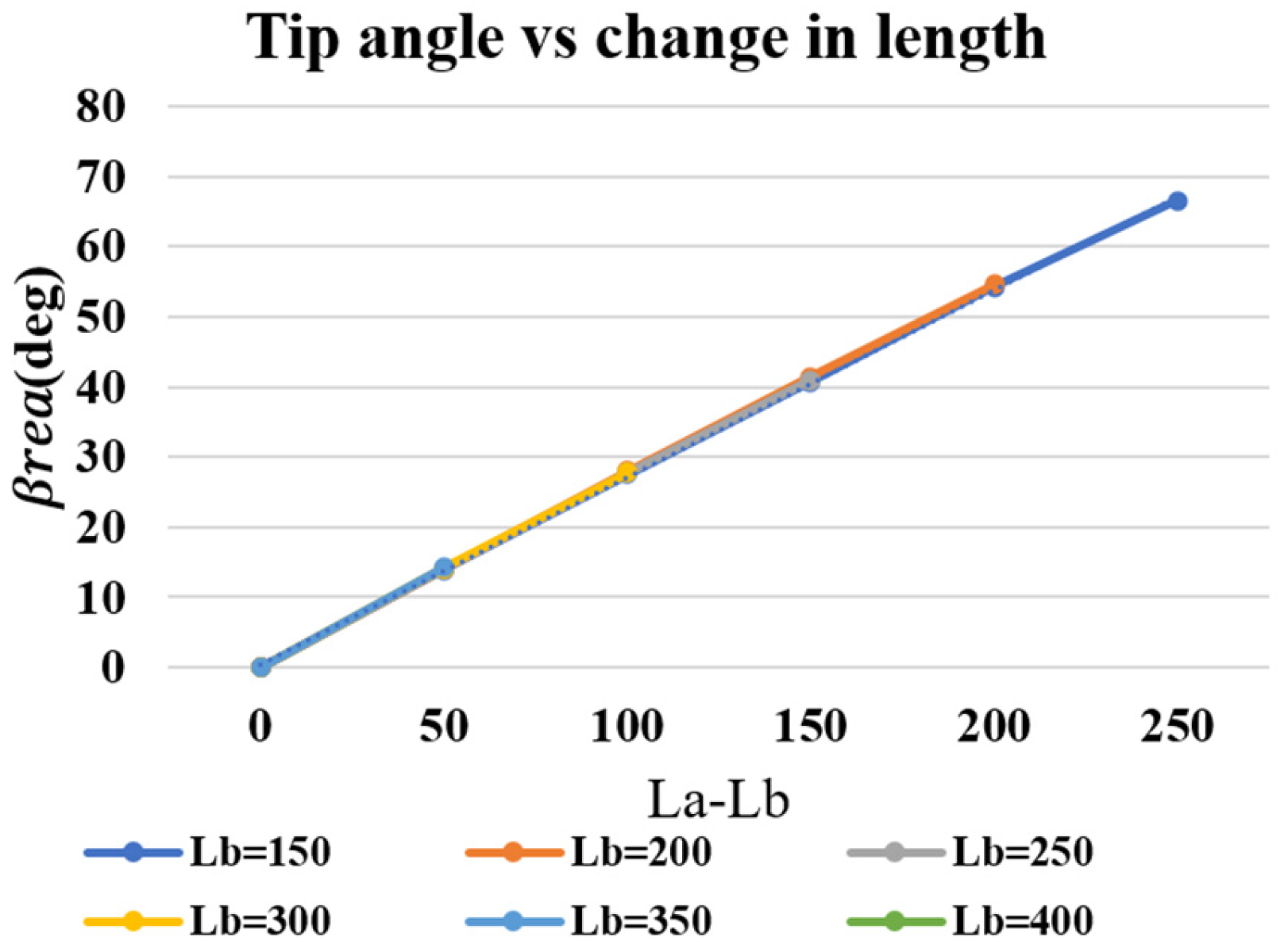
| Series1: Lb = 150; | ||||
| La = 200; | 174.06 | −120.79 | 13.38 | 3.737 |
| La = 250; | 193.98 | −145.74 | 27.55 | 3.630 |
| La = 300; | 210.10 | −175.54 | 40.71 | 3.685 |
| La = 350; | 219.17 | −208.68 | 54.26 | 3.686 |
| La = 400; | 219.87 | −239.81 | 66.56 | 3.756 |
| Series2: Lb = 200; | ||||
| La = 250; | 222.91 | −128.43 | 13.95 | 3.584 |
| La = 300; | 243.26 | −155.72 | 28.06 | 3.560 |
| La = 350; | 255.21 | −191.32 | 41.41 | 3.622 |
| La = 400; | 262.07 | −227.22 | 54.62 | 3.662 |
| Series3: Lb = 250; | ||||
| La = 300; | 271.85 | −135.51 | 14.01 | 3.569 |
| La = 350; | 289.41 | −171.09 | 27.71 | 3.609 |
| La = 400; | 300.26 | −209.69 | 40.88 | 3.670 |
| Series4: Lb = 300; | ||||
| La = 350; | 319.96 | −142.68 | 14.06 | 3.556 |
| La = 400; | 336.88 | −183.78 | 27.82 | 3.595 |
| Series5: Lb = 350; | ||||
| La = 400; | 370.91 | −150.14 | 14.25 | 3.509 |
| 0.05 | 0.1741 | −0.1208 | 0.1750 | 0.1750 | 0.000 |
| 0.10 | 0.1940 | −0.1457 | 0.1996 | 0.2000 | −0.0004 |
| 0.15 | 0.2101 | −0.1755 | 0.2255 | 0.2250 | 0.0005 |
| 0.20 | 0.2201 | −0.2087 | 0.2513 | 0.2500 | 0.0013 |
| 0.25 | 0.2199 | −0.2398 | 0.2714 | 0.2725 | −0.0011 |
| 0.05 | 0.2229 | −0.1284 | 0.2245 | 0.2250 | −0.0005 |
| 0.10 | 0.2433 | −0.1557 | 0.2503 | 0.2500 | 0.0003 |
| 0.15 | 0.2552 | −0.1913 | 0.2742 | 0.2750 | −0.0008 |
| 0.20 | 0.2621 | −0.2272 | 0.2983 | 0.3000 | −0.0017 |
| 0.05 | 0.2718 | −0.1355 | 0.2741 | 0.2750 | −0.0005 |
| 0.10 | 0.2894 | −0.1710 | 0.2994 | 0.3000 | 0.0003 |
| 0.15 | 0.3003 | −0.2097 | 0.3242 | 0.3250 | −0.0009 |
| 0.05 | 0.3200 | −0.1427 | 0.3226 | 0.3250 | −0.0024 |
| 0.10 | 0.3369 | −0.1838 | 0.3488 | 0.3500 | −0.0012 |
| 0.05 | 0.3709 | −0.1501 | 0.3745 | 0.3750 | −0.0005 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, W.; Yu, X.; Zhao, Y.; Li, L.; Li, Y.; Tian, Y.; Xi, F. A Simplified Method for Inverse Kinematics of a Flexible Panel Continuum Robot for Real-Time Shape Morphing. Machines 2023, 11, 104. https://doi.org/10.3390/machines11010104
Wang W, Yu X, Zhao Y, Li L, Li Y, Tian Y, Xi F. A Simplified Method for Inverse Kinematics of a Flexible Panel Continuum Robot for Real-Time Shape Morphing. Machines. 2023; 11(1):104. https://doi.org/10.3390/machines11010104
Chicago/Turabian StyleWang, Wenbin, Xiangping Yu, Yinjun Zhao, Long Li, Yuwen Li, Yingzhong Tian, and Fengfeng Xi. 2023. "A Simplified Method for Inverse Kinematics of a Flexible Panel Continuum Robot for Real-Time Shape Morphing" Machines 11, no. 1: 104. https://doi.org/10.3390/machines11010104
APA StyleWang, W., Yu, X., Zhao, Y., Li, L., Li, Y., Tian, Y., & Xi, F. (2023). A Simplified Method for Inverse Kinematics of a Flexible Panel Continuum Robot for Real-Time Shape Morphing. Machines, 11(1), 104. https://doi.org/10.3390/machines11010104








