Dynamic Tangential Contact Stiffness and Damping Model of the Solid–Liquid Interface
Abstract
:1. Introduction
2. Calculation Model for Tangential Contact Stiffness and Damping of the Solid–Liquid Interface Fluid
2.1. Average Flow Equation Considering the Roughness Lubrication Effect
2.2. Calculation of the Bearing Capacity of the Solid–Liquid Interface
2.3. Calculation of Viscous Shear Force of the Oil Film on the Solid–Liquid Interface
2.4. Calculation of the Friction Coefficient of the Solid–Liquid Interface
2.5. Difference Model for Calculating Partial Tangential Stiffness and Damping of the Solid–Liquid Interface
3. Calculation Model of Tangential Contact Stiffness and Damping of the Solid–Liquid Interface
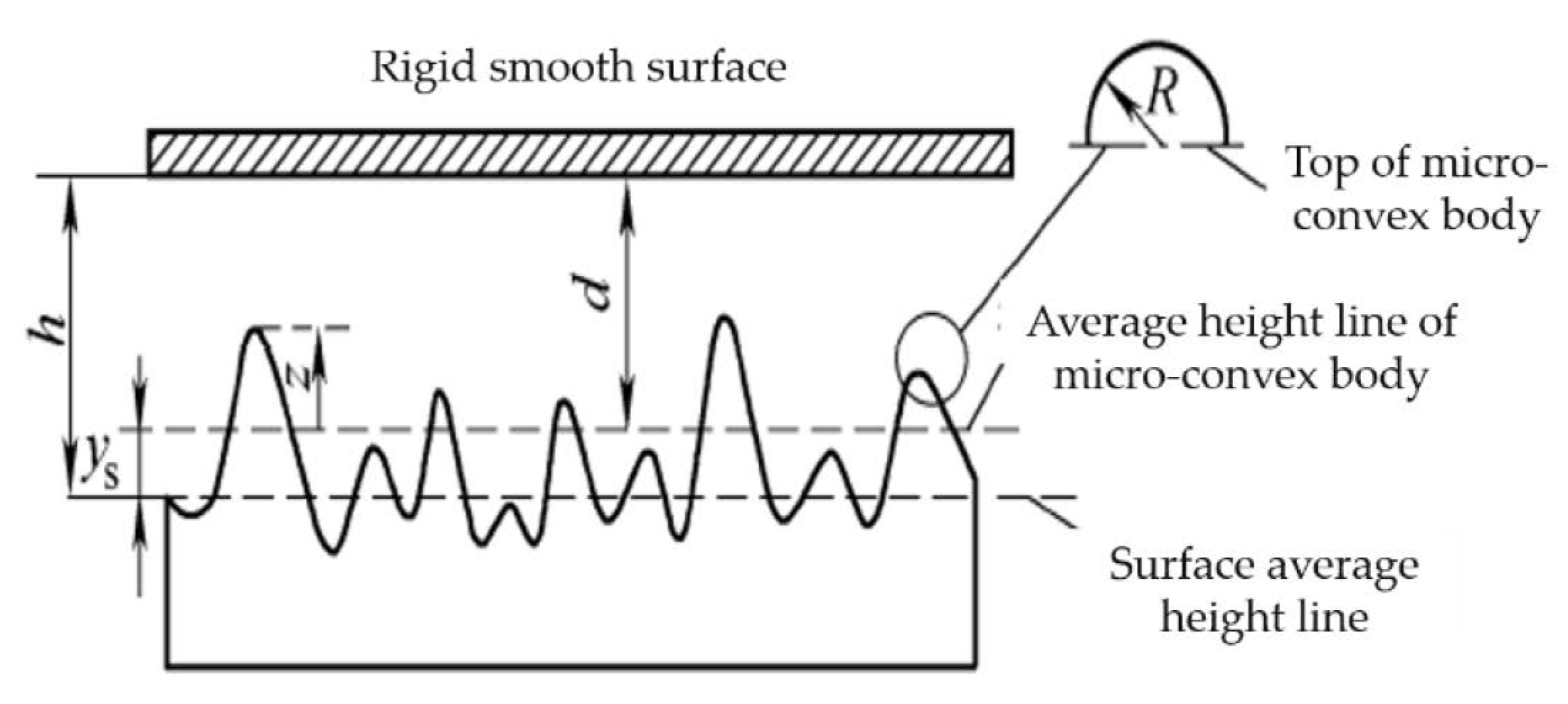
3.1. Relationship between a Normal Load and Deformation of the Microconvex Body
3.2. Tangential Contact Stiffness and Damping Model of the Microconvex Body
3.2.1. Elastic Stage
3.2.2. Elastic–Plastic Stage
3.2.3. Plastic Stage
3.3. Dynamic Statistical Model of the Tangential Contact of Solids in the Solid–Iquid Interface
3.4. Calculation Model of Tangential Contact Stiffness and Damping of the Solid–Liquid Interface
4. Simulation Analysis of Dynamic Tangential Stiffness and Damping of the Solid–Liquid Interface
4.1. Simulation Analysis of Dynamic Tangential Stiffness of the Solid–Liquid Interface
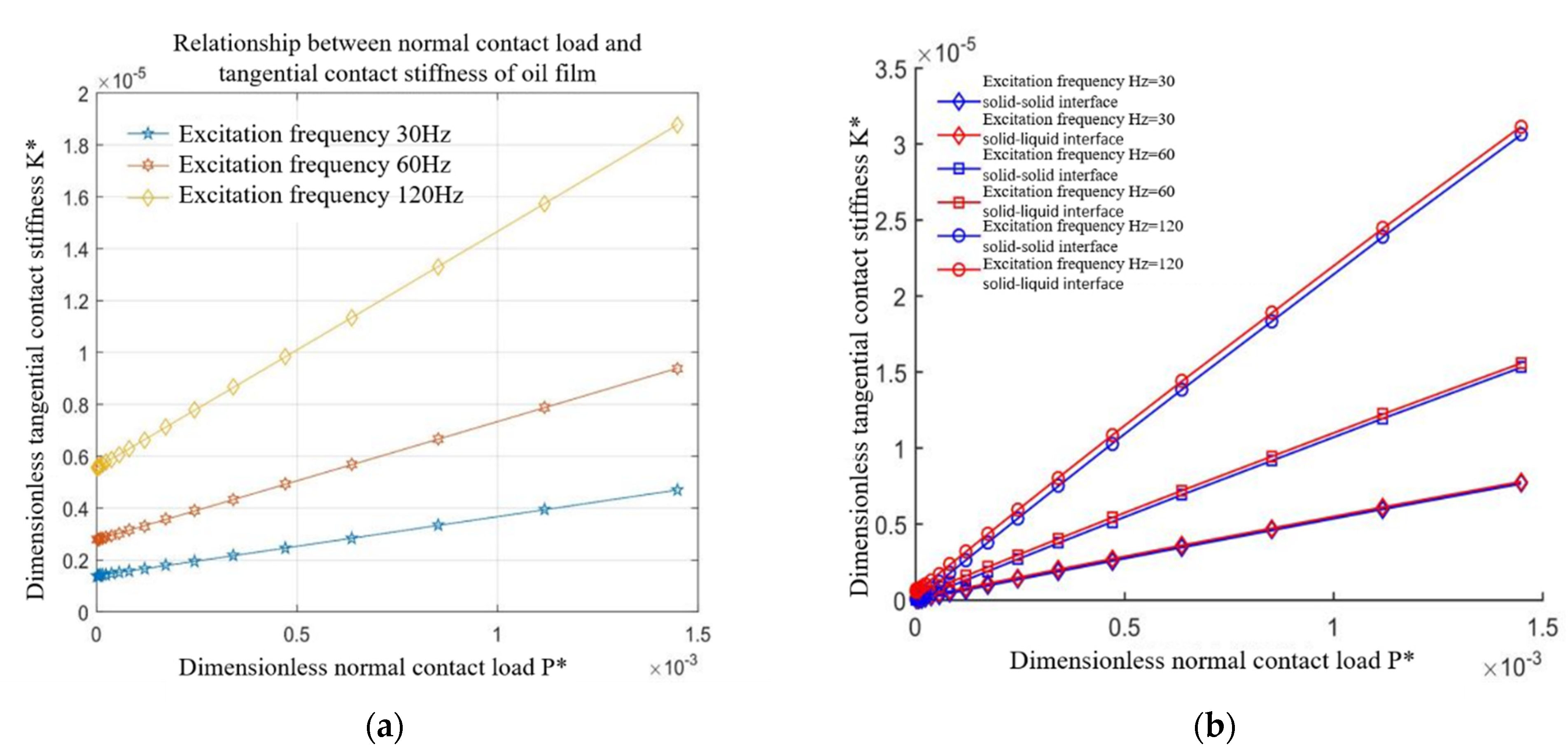
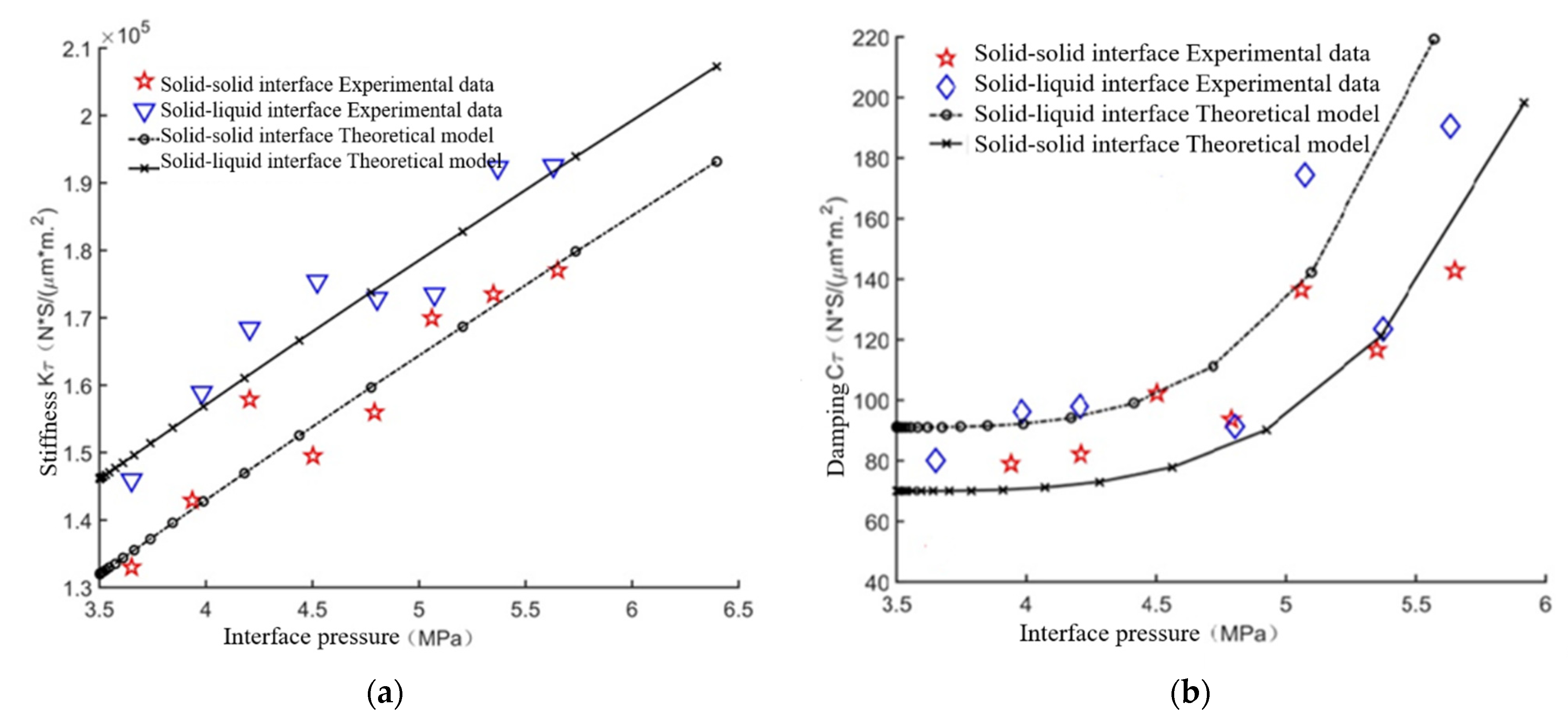
4.1.1. Effect of a Normal Contact Load on the Dynamic Tangential Contact Stiffness
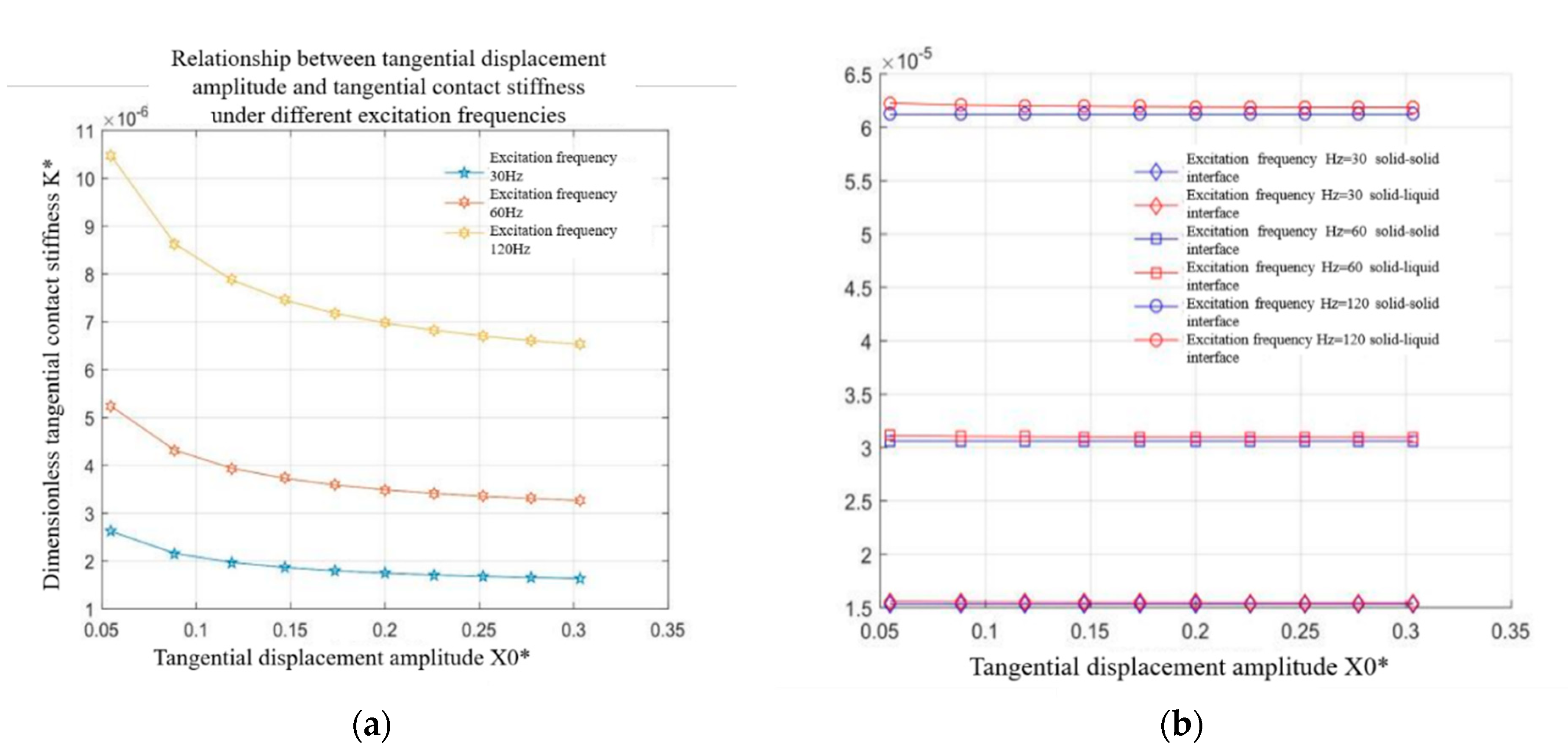
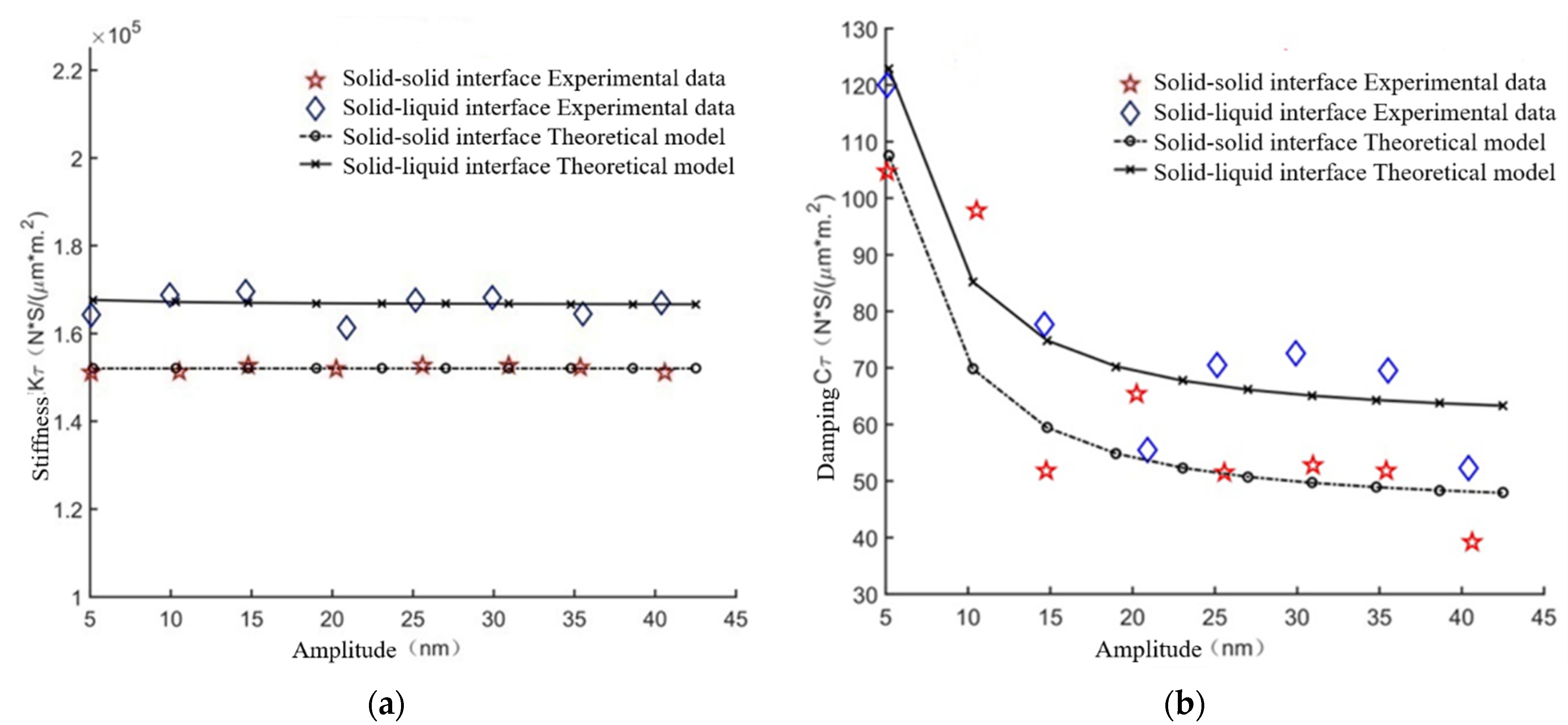
4.1.2. Influence of the Tangential Displacement Amplitude on the Tangential Contact Stiffness
4.1.3. Effect of Excitation Frequency on Tangential Contact Stiffness
4.2. Simulation Analysis of Dynamic Tangential Damping of the Solid–Liquid Interface
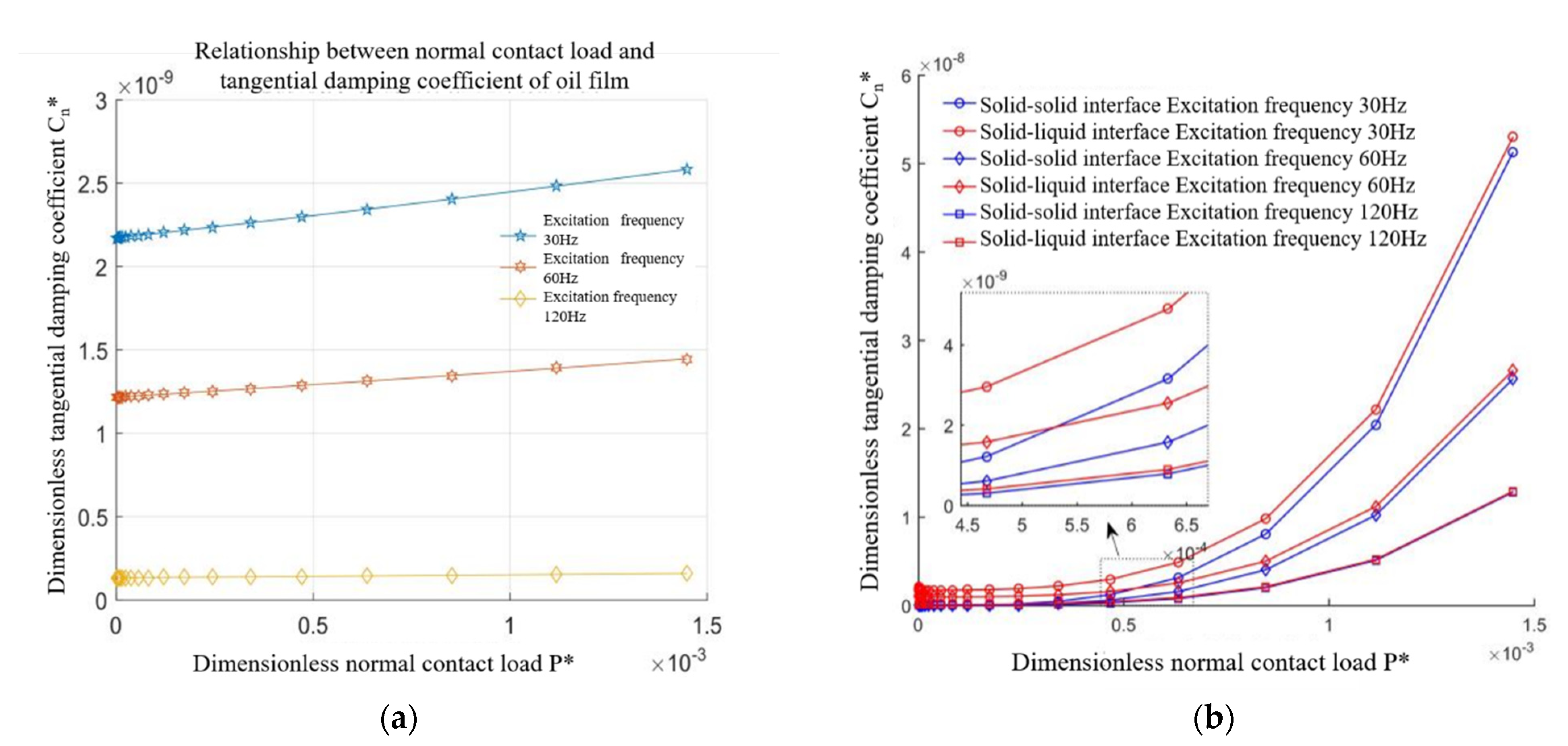
4.2.1. Effect of a Normal Contact Load on Tangential Contact Damping
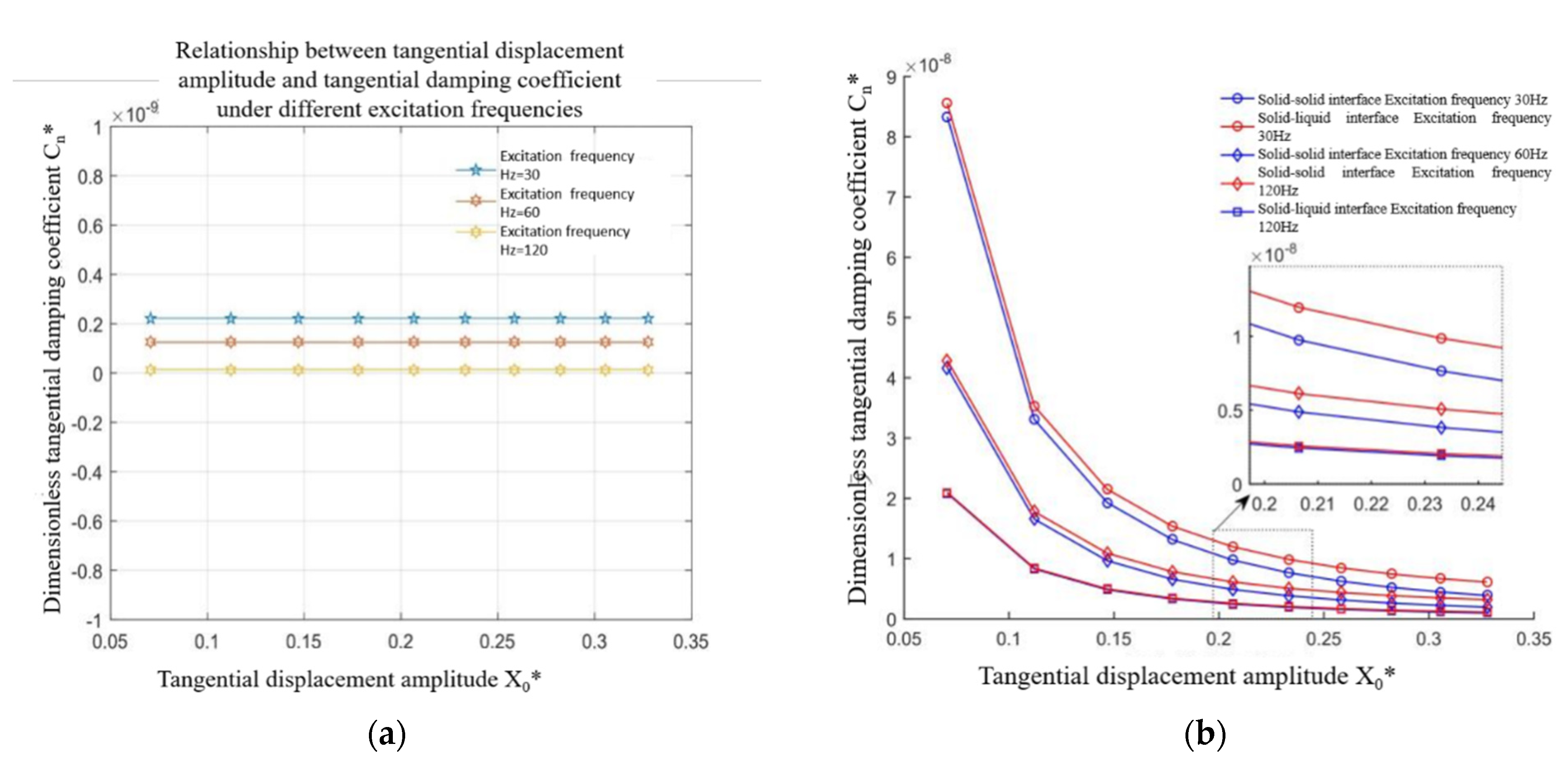
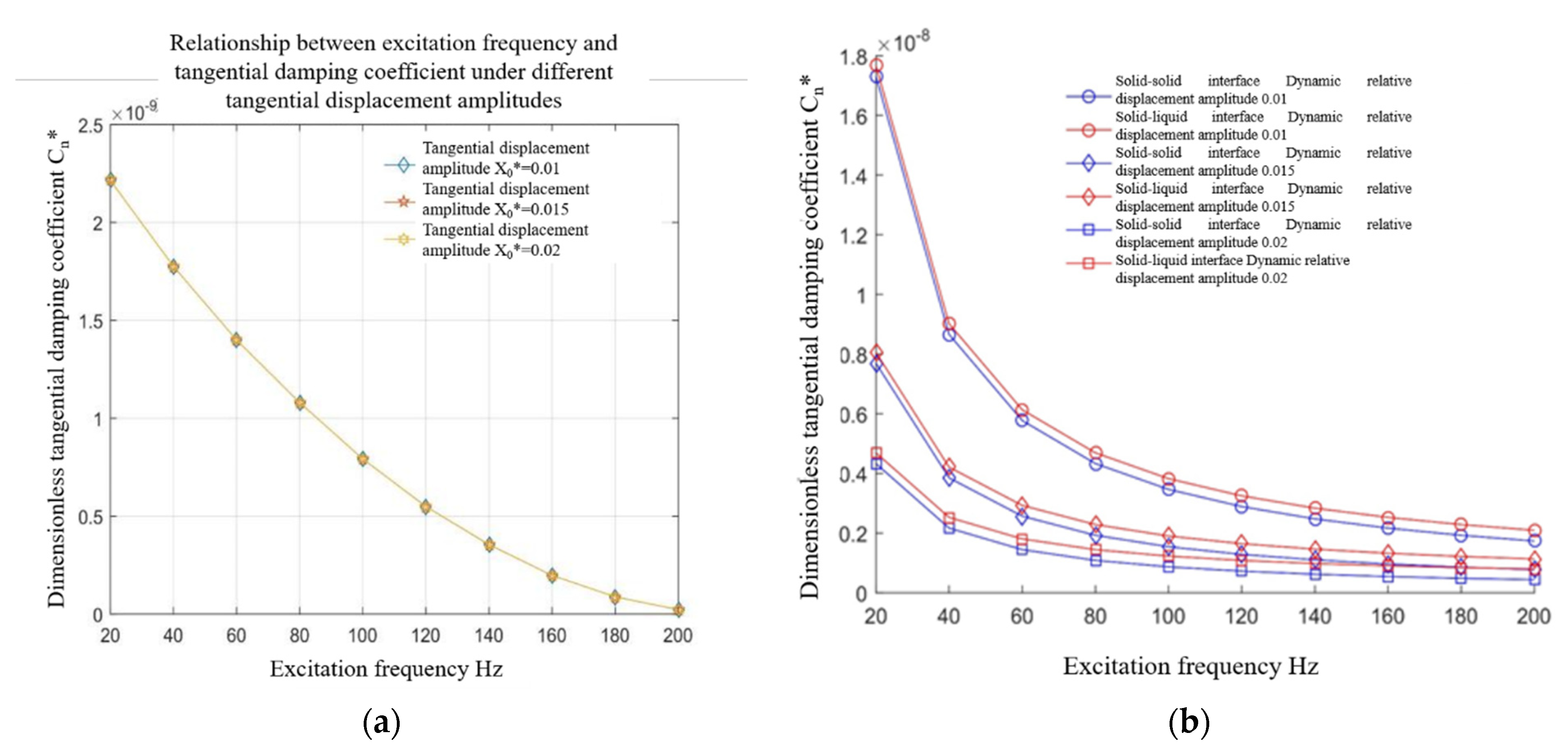
4.2.2. Effect of Tangential Displacement Amplitude on Tangential Contact Damping
4.2.3. Effect of Excitation Frequency on Tangential Damping
5. Experimental Verification
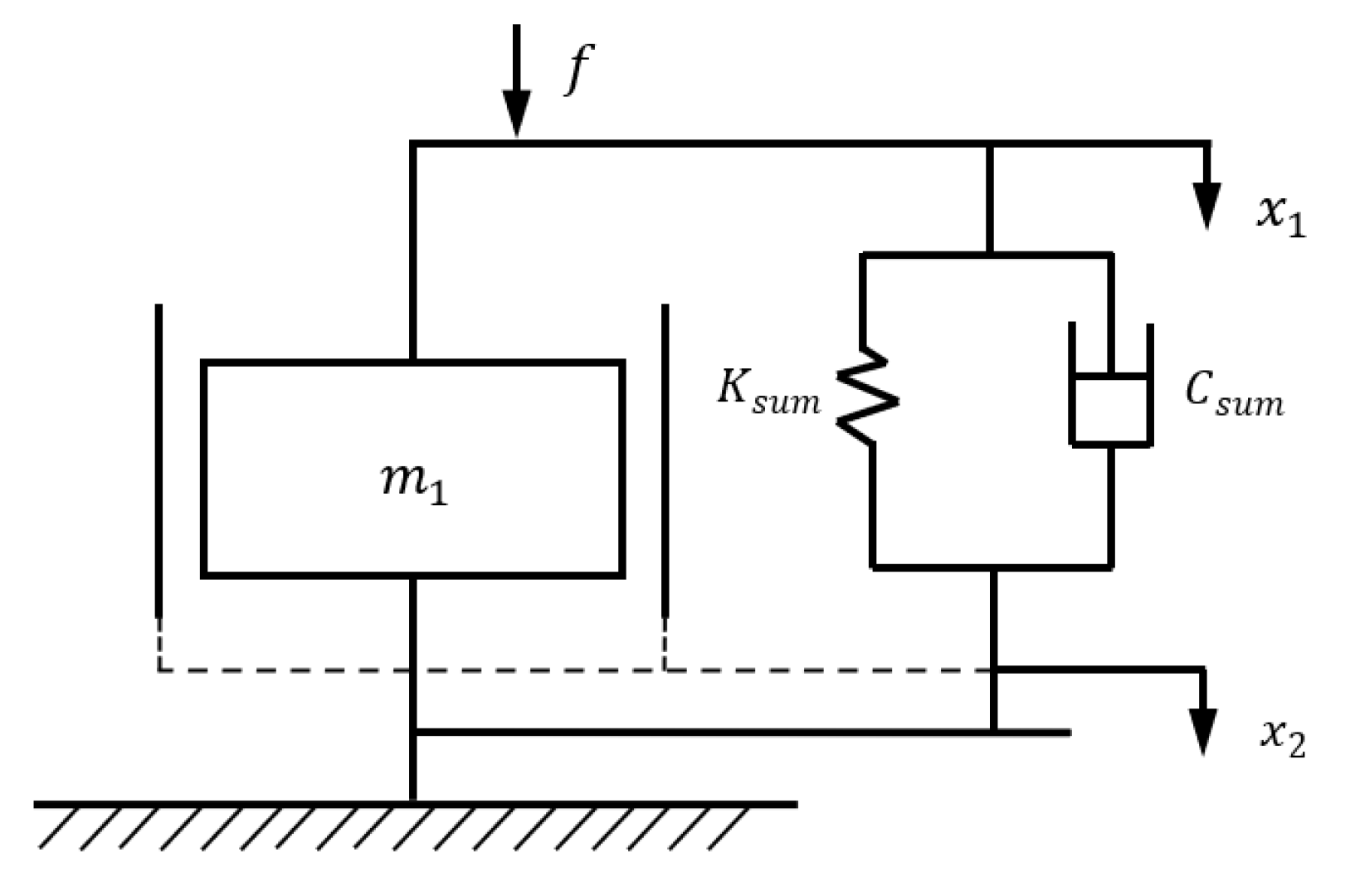
5.1. The Principle of the Experiment
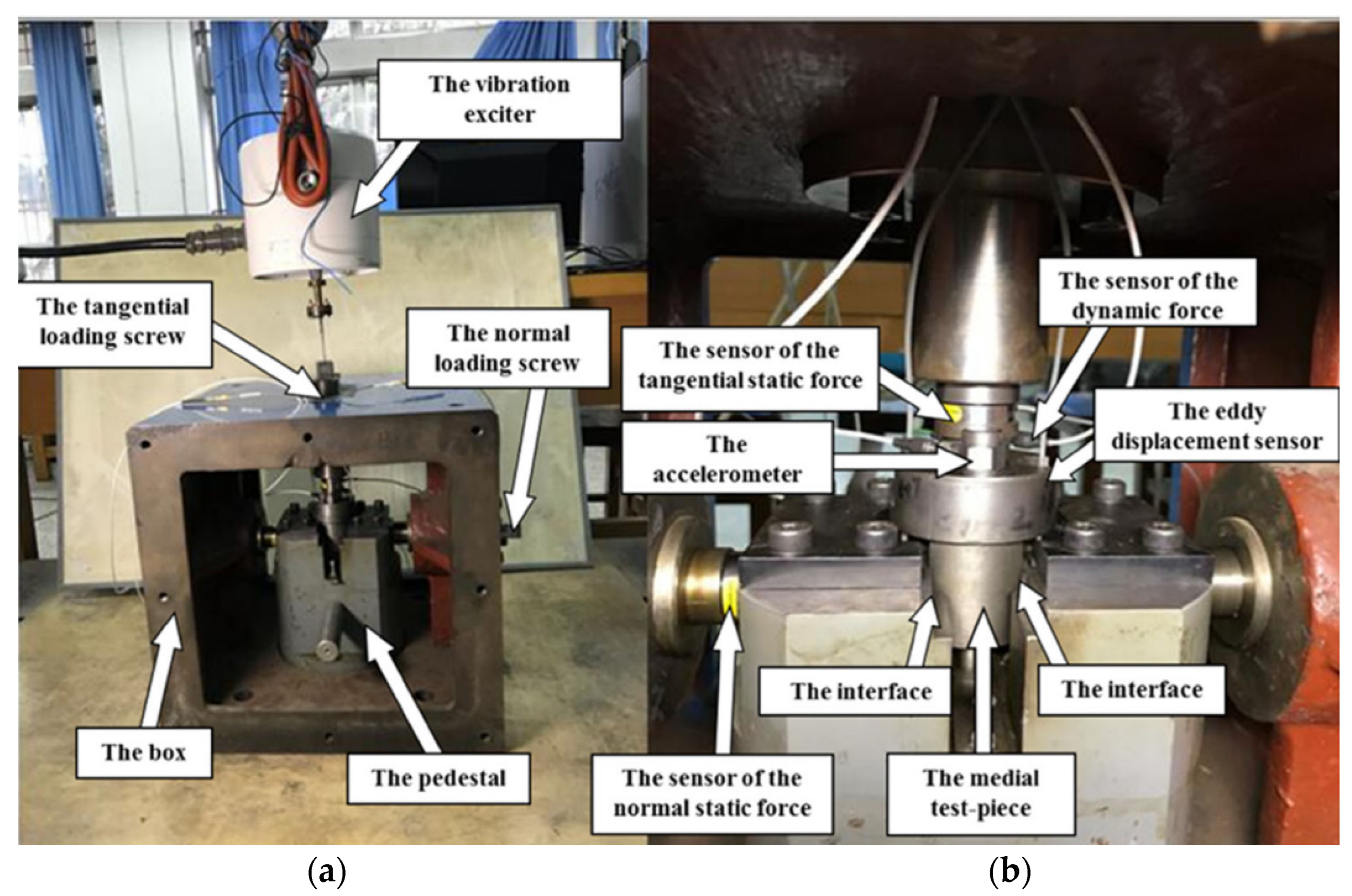
5.2. Experimental Device
5.3. The Comparison between Theoretical Model and Experiment Results
6. Conclusions
Author Contributions
Funding
Informed Consent Statement
Conflicts of Interest
References
- Guozhong, D.; Hui, Z.; Yue, L.; Guangneng, D. Modeling and tribological characteristics of hybrid elastohydrodynamic lubrication system. J. Xi’an Jiaotong Univ. 2018, 52, 107–114. [Google Scholar]
- Wang, X.; Zhao, X.; Xu, X.; Li, X.; He, K.; Tian, H. Mixed lubrication of a linear motion rolling guideway pair. J. Vib. Shock. 2020, 39, 260–266. [Google Scholar]
- Carrella, A.; Brennan, M.J.; Waters, T.P.; Lopes, V., Jr. Force and displacement transmissibility of a nonlinear isolator with high-static-low-dynamic-stiffness. Int. J. Mech. Sci. 2012, 55, 22–29. [Google Scholar] [CrossRef]
- Zou, H.; Wang, B. Investigation of the contact stiffness variation of linear rolling guides due to the effects of friction and wear during operation. Tribol. Int. 2015, 92, 472–484. [Google Scholar] [CrossRef]
- Li, X.P.; Pan, W.J.; Gao, J.Z.; Li, S.J.; Zhao, G.H.; Wen, B.C. Influence of surface topography characteristics on mode coupling instability system. J. Mech. Eng. 2017, 53, 116–127. [Google Scholar] [CrossRef]
- Oh, K.-J.; Cao, L.; Chung, S.-C. Explicit modeling and investigation of friction torques in double-nut ball screws for the precision design of ball screw feed drives. Tribol. Int. 2020, 141, 105841. [Google Scholar] [CrossRef]
- Zheng, F.Y. Theory and application of variable speed gear transmission with moving shaft. J. Mech. Eng. 2019, 55, 52–64. [Google Scholar]
- Liu, J.; Ma, C.; Wang, S. Precision loss modeling method of ball screw pair. Mechanical Syst. Signal Process. 2020, 135, 106397. [Google Scholar] [CrossRef]
- Gonzalez-Valadez, M.; Dwyer-Joyce, R.; Lewis, R. Ultrasonic reflection from mixed liquid-solid contacts and the determination of interface stiffness. In Tribology and Interface Engineering Series; Elsevier: Amsterdam, The Netherlands, 2005; Volume 48, pp. 313–320. [Google Scholar]
- Dwyer-Joyce, R.; Reddyhoff, T.; Zhu, J. Ultrasonic measurement for film thickness and solid contact in elastohydrodynamic lubrication. J. Tribol. 2011, 133, 407–411. [Google Scholar] [CrossRef]
- Shi, X.; Polycarpou, A.A. Measurement and modeling of normal contact stiffness and contact damping at the meso scale. J. Vib. Acoust. 2005, 127, 52–60. [Google Scholar] [CrossRef]
- Ren, P.; Wang, L.H.; Wang, C.F. Experimental study on normal dynamic contact stiffness of guide joint under lubrication. China Mech. Eng. 2018, 29, 811–816. [Google Scholar]
- Fu, W.P.; Lou, L.T.; Gao, Z.Q.; Wang, W.; Wu, J.B. Theoretical model of normal contact stiffness and damping of mechanical joint. J. Mech. Eng. 2017, 53, 73–82. [Google Scholar] [CrossRef]
- Ma, C.; Duan, Y.; Yu, B.; Sun, J.; Tu, Q. The comprehensive effect of surface texture and roughness under hydrodynamic and mixed lubrication conditions. Proc. Inst. Mech. Eng. Part J. J. Eng. Tribol. 2017, 231, 1307–1319. [Google Scholar] [CrossRef]
- Xiao, H.F.; Sun, Y.Y.; Xu, J.W. Study on calculation model and characteristics of normal contact stiffness of rough interface under mixed lubrication. Vib. Shock. 2018, 37, 106–114+147. [Google Scholar]
- Sun, Y.; Xiao, H.; Xu, J.; Yu, W. Study on the normal contact stiffness of the fractal rough surface in mixed lubrication. Proc. Inst. Mech. Eng. Part. J. J. Eng. Tribol. 2018, 232, 1604–1617. [Google Scholar] [CrossRef]
- Li, L.; Yun, Q.Q.; Li, Z.Q.; Cai, A.J.; Duan, Z.S. Contact characteristics of joint surfaces considering bulk substrate deformation in mixed lubrication. Vib. Test Diagn. 2019, 39, 953–959+1128–1129. [Google Scholar]
- Li, L.; Pei, X.Y.; Shi, X.H.; Li, Z.Q.; Cai, A.J. Study on normal contact stiffness of joint under mixed lubrication. Vib. Shock. 2020, 39, 16–23. [Google Scholar]
- Wen, X.Y.; Zhang, X.L.; Tan, W.B.; Zhang, W. Study on three-dimensional Fractal Model of normal contact stiffness of mixed Lubrication Joint Surface. Modul. Mach. Tool Autom. Mach. Technol. 2020, 11, 49–53. [Google Scholar]
- Zhou, C.J.; Xiao, Z.L.; Chen, S.Y.; Han, X. Normal and tangential oil film stiffness of modified spur gear with non-Newtonian elastohydrodynamic lubrication. Tribiology Int. 2016, 109, 319–327. [Google Scholar] [CrossRef]
- Zhou, C.J.; Xiao, Z.L. Stiffness and damping models for the oil film in line contact elastohydrodynamic lubrication and applications in the gear drive. Appl. Math. Model. 2018, 61, 634–649. [Google Scholar] [CrossRef]
- Jones, R.E. A Greenwood-Williamson model of small-scale friction. J. Appl. Mech. 2007, 74, 31–40. [Google Scholar] [CrossRef]
- Wang, J.; Chen, T.; Wang, X.; Xi, Y. Dynamic identification of tangential contact stiffness by using friction damping in moving contact. Tribol. Int. 2019, 131, 308–317. [Google Scholar] [CrossRef]
- Wu, J.; Yuan, R.; He, Z.; Zhang, D.; Xie, Y. Experimental study on dry friction damping characteristics of the steam turbine blade material with nonconforming contacts. Adv. Mater. Sci. Eng. 2015, 2015, 849253. [Google Scholar] [CrossRef]
- Eriten, M.; Chen, S.; Usta, A.D.; Yerrapragada, K. In Situ Investigation of Load-Dependent Nonlinearities in Tangential Stiffness and Damping of Spherical Contacts. J. Tribol. 2021, 143, 061501. [Google Scholar] [CrossRef]
- Wen, S.Z.; Huang, P. Principles of Tribology, 2nd ed.; Qinghua University Press: Beijing, China, 2002. [Google Scholar]
- Schlichting, H.; Kestin, J. Boundary Layer Theory; Springer: Berlin/Heidelberg, Germany, 2017. [Google Scholar]
- Wu, C.; Zheng, L. An average Reynolds equation for partial film lubrication with a contact factor. J. Tribol. 1989, 111, 188–191. [Google Scholar] [CrossRef]
- Li, R.H. Numerical Solution of Partial Differential Equation; Higher Education Press: Beijing, China, 2005. [Google Scholar]
- Ma, C.B. Study on Lubrication Calculation Model and Antifriction Characteristics of Textured Surface. Ph.D. Thesis, China University of Mining and Technology, Beijing, China, 2010. [Google Scholar]
- Rowe, W.; Chong, F. Computation of dynamic force coefficients for hybrid (hydrostatic/hydrodynamic) journal bearings by the finite disturbance and perturbation techniques. Tribol. Int. 1986, 19, 260–271. [Google Scholar] [CrossRef]
- Ma, C.B. Study on Lubrication calculation Model and antifriction characteristics of textured Surface. J. Mech. Eng. 2007, 3, 95–101. [Google Scholar]
- Kadin, Y.; Kligerman, Y.; Etsion, I. Unloading an elastic–plastic contact of rough surfaces. J. Mech. Phys. Solids 2006, 54, 2652–2674. [Google Scholar] [CrossRef]
- Fujimoto, T.; Kagami, J.; Kawaguchi, T.; Hatazawa, T. Micro-displacement characteristics under tangential force. Wear 2000, 241, 136–142. [Google Scholar] [CrossRef]
- Gao, Z.Q. Study on the Theoretical Model of Contact Stiffness and Damping of Mechanical Joint. Ph.D. Thesis, Xi’an University of Technology, Xi’an, China, 2018. [Google Scholar]
- Kogut, L.; Etsion, I. Elastic-plastic contact analysis of a sphere and a rigid flat. J. Appl. Mech. 2002, 69, 657–662. [Google Scholar] [CrossRef]
- Gorbatikh, L.; Popova, M. Modeling of a locking mechanism between two rough surfaces under cyclic loading. Int. J. Mech. Sci. 2006, 48, 1014–1020. [Google Scholar] [CrossRef]
- Thornton, C.; Ning, Z. A theoretical model for the stick/bounce behaviour of adhesive, elastic-plastic spheres. Powder Technol. 1998, 99, 154–162. [Google Scholar] [CrossRef]
- Thornton, C.; Ning, Z.; Chuan-yu, W.; Nasrullah, M.; Long-yuan, L. Contact mechanics and coefficients of restitution. In Granular Gases; Springer: Berlin/Heidelberg, Germany, 2001; pp. 184–194. [Google Scholar]
- Thornton, C. Coefficient of restitution for collinear collisions of elastic-perfectly plastic spheres. J. Appl. Mech. 1997, 64, 383–386. [Google Scholar] [CrossRef]
- He, S.M.; Wu, Y.; Shen, J. Microscopic displacement characteristics of elastic-plastic materials under tangential load. Eng. Mech. 2010, 27, 73–77. [Google Scholar]
- Dai, D.P. Damping Vibration and Noise Reduction Technology; Xi’an Jiaotong University: Xi’an, China, 1986. [Google Scholar]
- Fu, W.P.; Gao, Z.Q.; Wang, W.; Wu, J.B. A Model of Tangential Contact Damping Considering Asperity Interaction and Lateral Contact. Acta Mech Solida Sin. 2018, 31, 758–774. [Google Scholar] [CrossRef]
- Liang, J.W.; Feeny, B.F. Identifying Coulomb and Viscous Friction from Free-Vibration Decrements. Nonlin Dyn. 1998, 16, 337–347. [Google Scholar] [CrossRef]




Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Peng, L.; Gao, Z.; Ban, Z.; Gao, F.; Fu, W. Dynamic Tangential Contact Stiffness and Damping Model of the Solid–Liquid Interface. Machines 2022, 10, 804. https://doi.org/10.3390/machines10090804
Peng L, Gao Z, Ban Z, Gao F, Fu W. Dynamic Tangential Contact Stiffness and Damping Model of the Solid–Liquid Interface. Machines. 2022; 10(9):804. https://doi.org/10.3390/machines10090804
Chicago/Turabian StylePeng, Lixia, Zhiqiang Gao, Zhaoyang Ban, Feng Gao, and Weiping Fu. 2022. "Dynamic Tangential Contact Stiffness and Damping Model of the Solid–Liquid Interface" Machines 10, no. 9: 804. https://doi.org/10.3390/machines10090804
APA StylePeng, L., Gao, Z., Ban, Z., Gao, F., & Fu, W. (2022). Dynamic Tangential Contact Stiffness and Damping Model of the Solid–Liquid Interface. Machines, 10(9), 804. https://doi.org/10.3390/machines10090804





