Energy Harvesting Performance of a Novel Nonlinear Quad-Stable Piezoelectric Energy Harvester with Only One External Magnet
Abstract
:1. Introduction
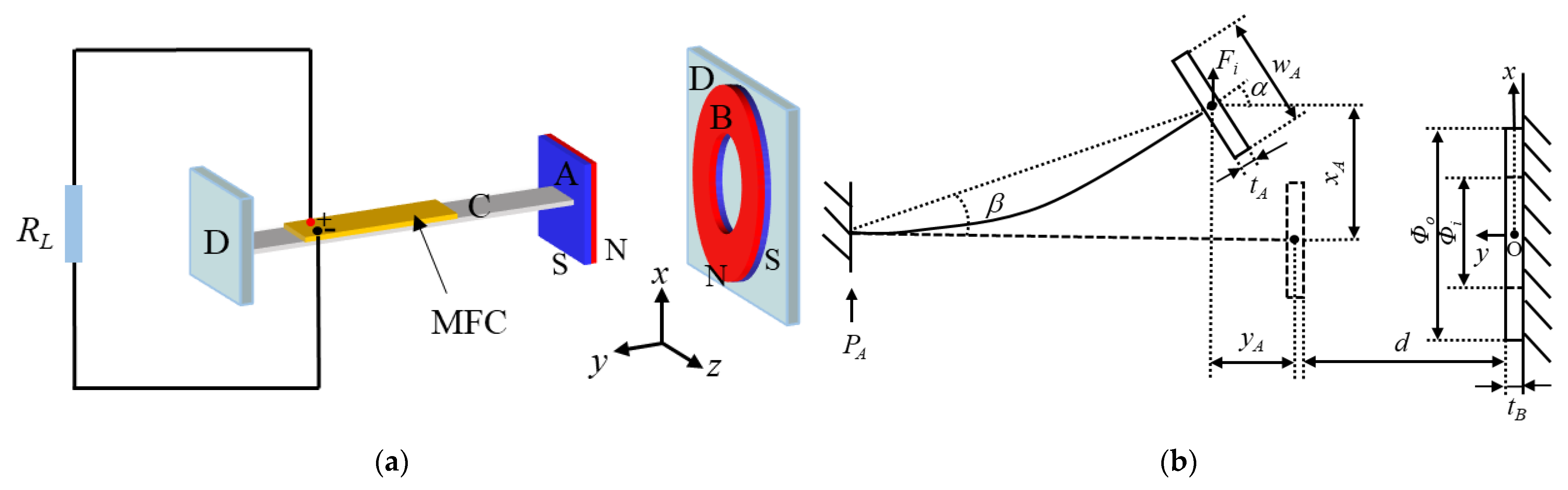
2. Configuration and Model
3. Numerical Simulation
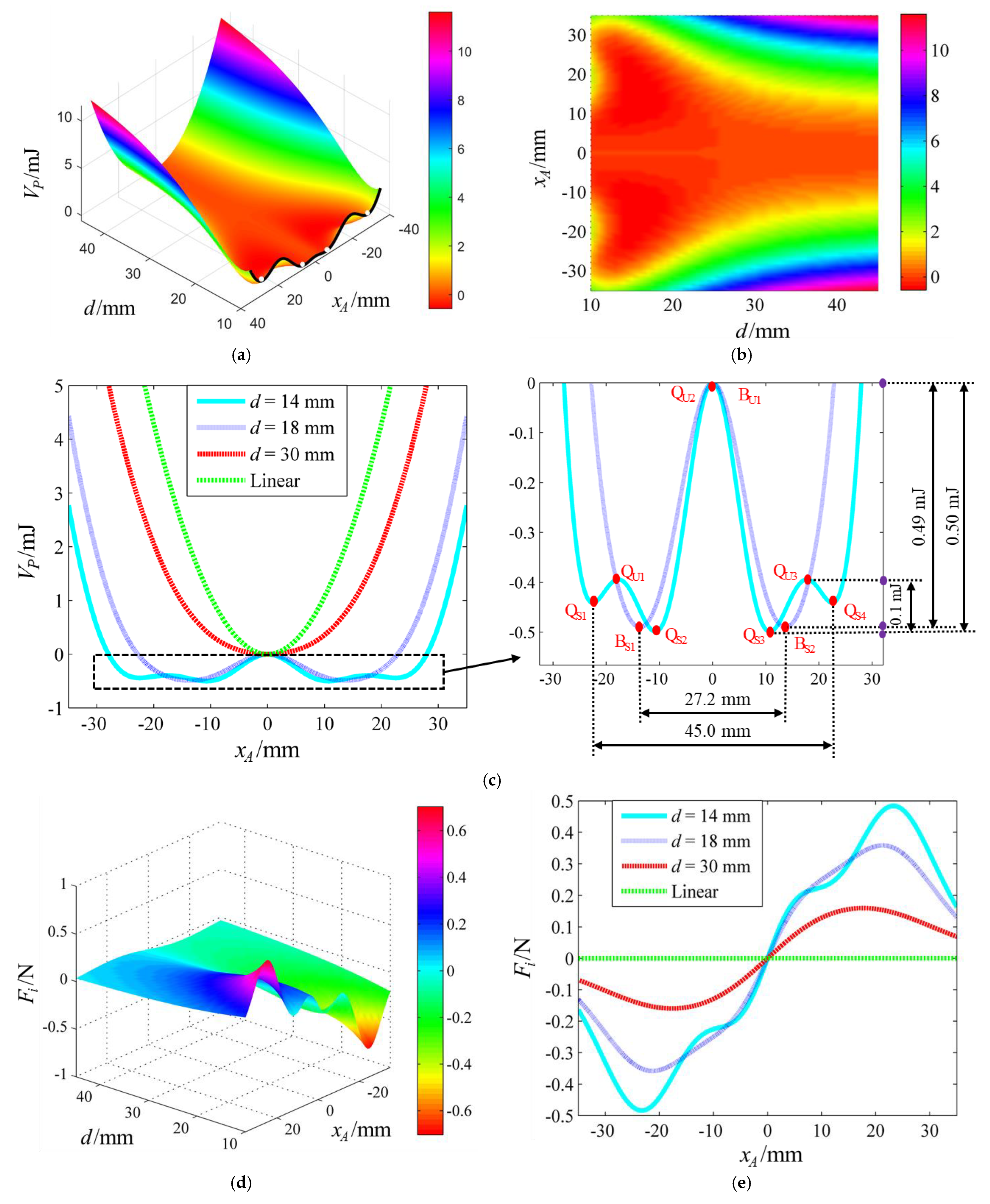
3.1. Potential Energy and Magnetic Force of the QPEH
3.2. Estimated Energy Harvesting Performance of the QPEH
4. Experimental Verification
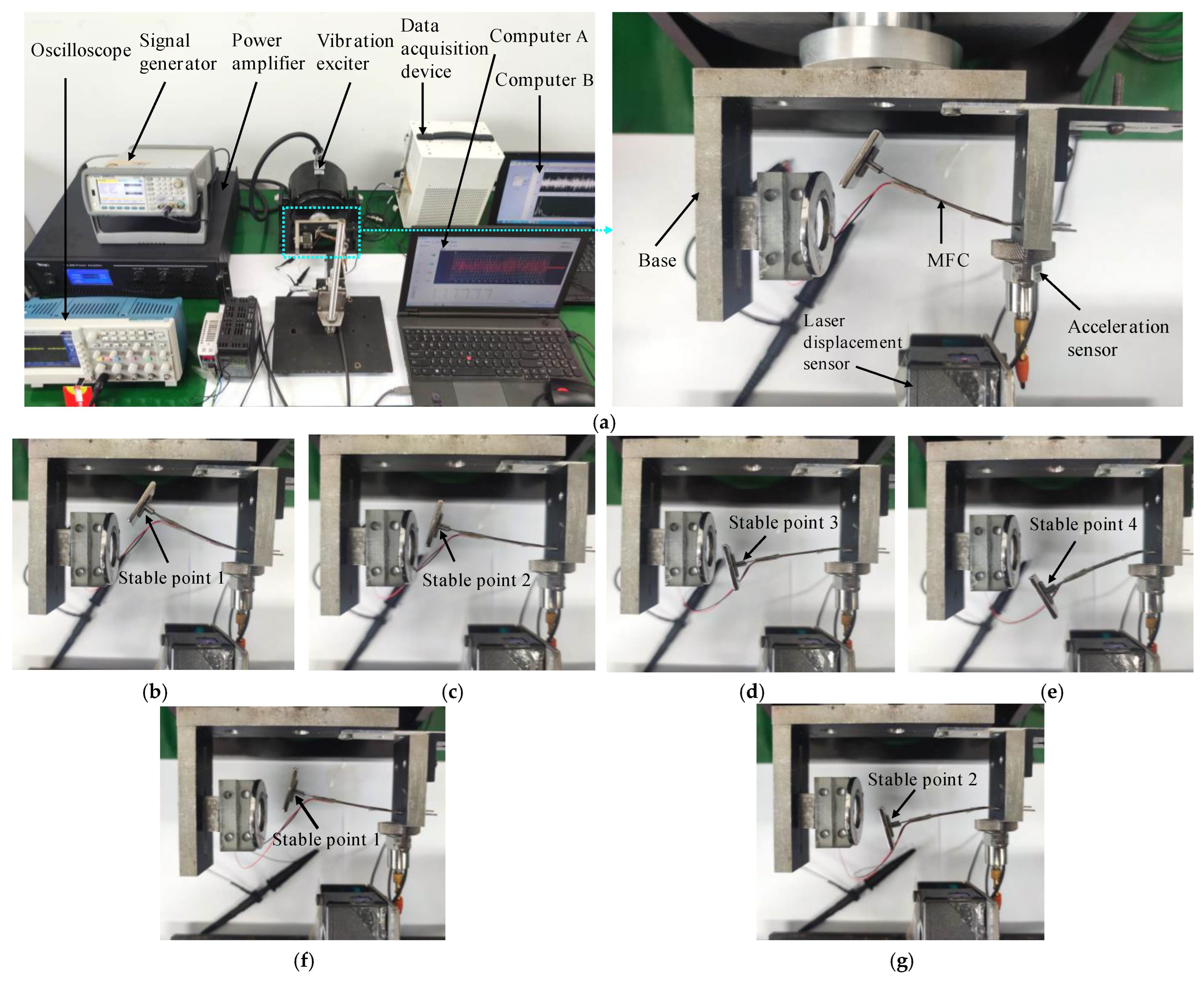
4.1. Experimental Setup
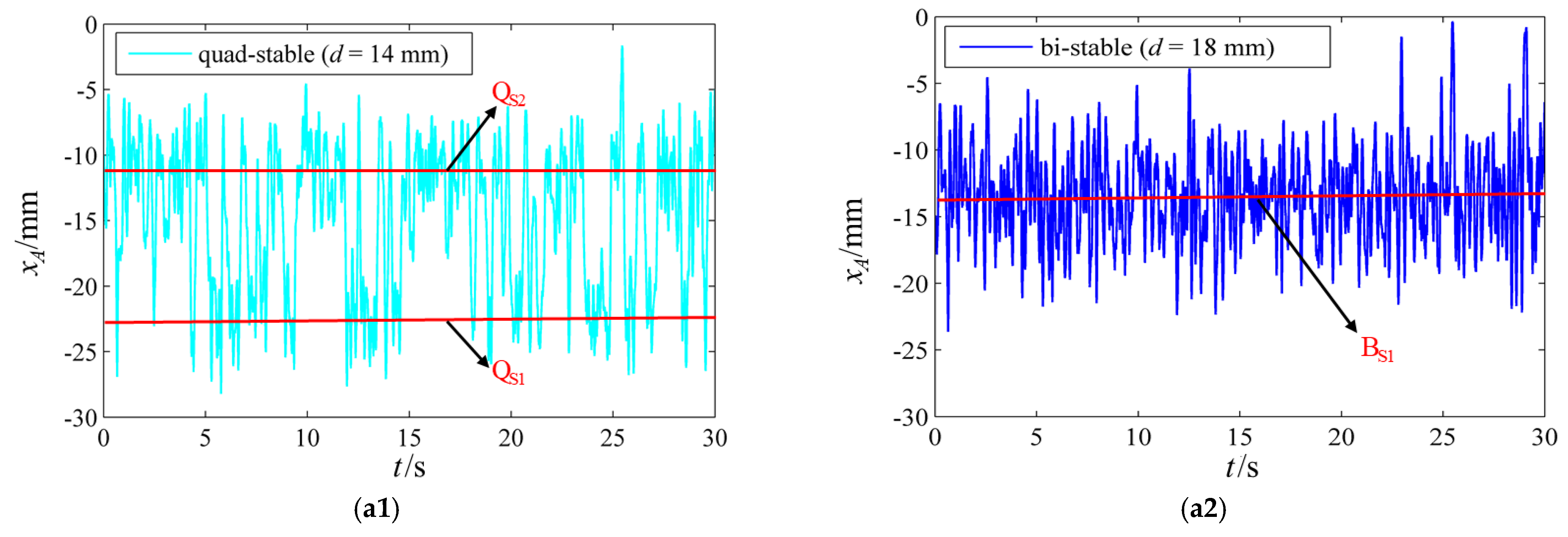
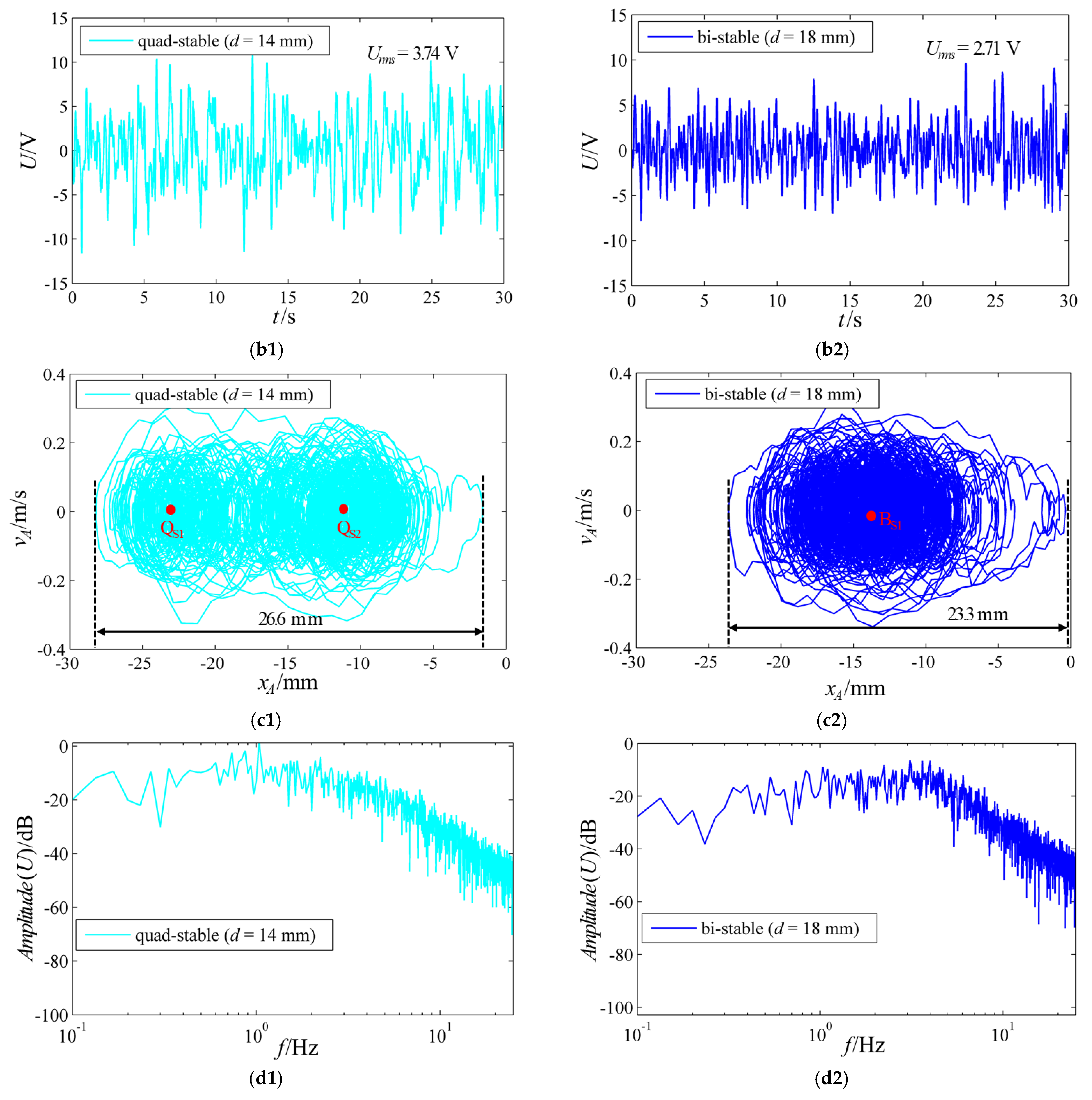
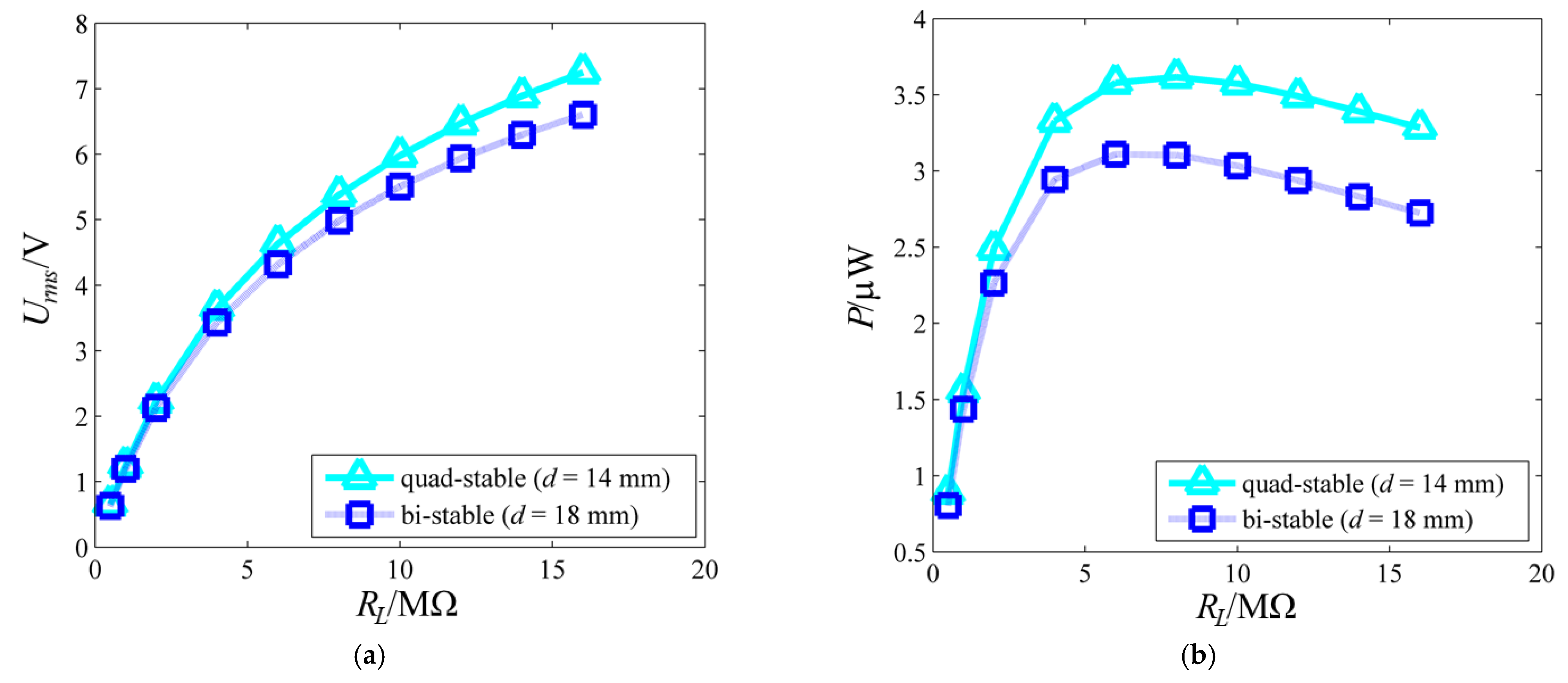
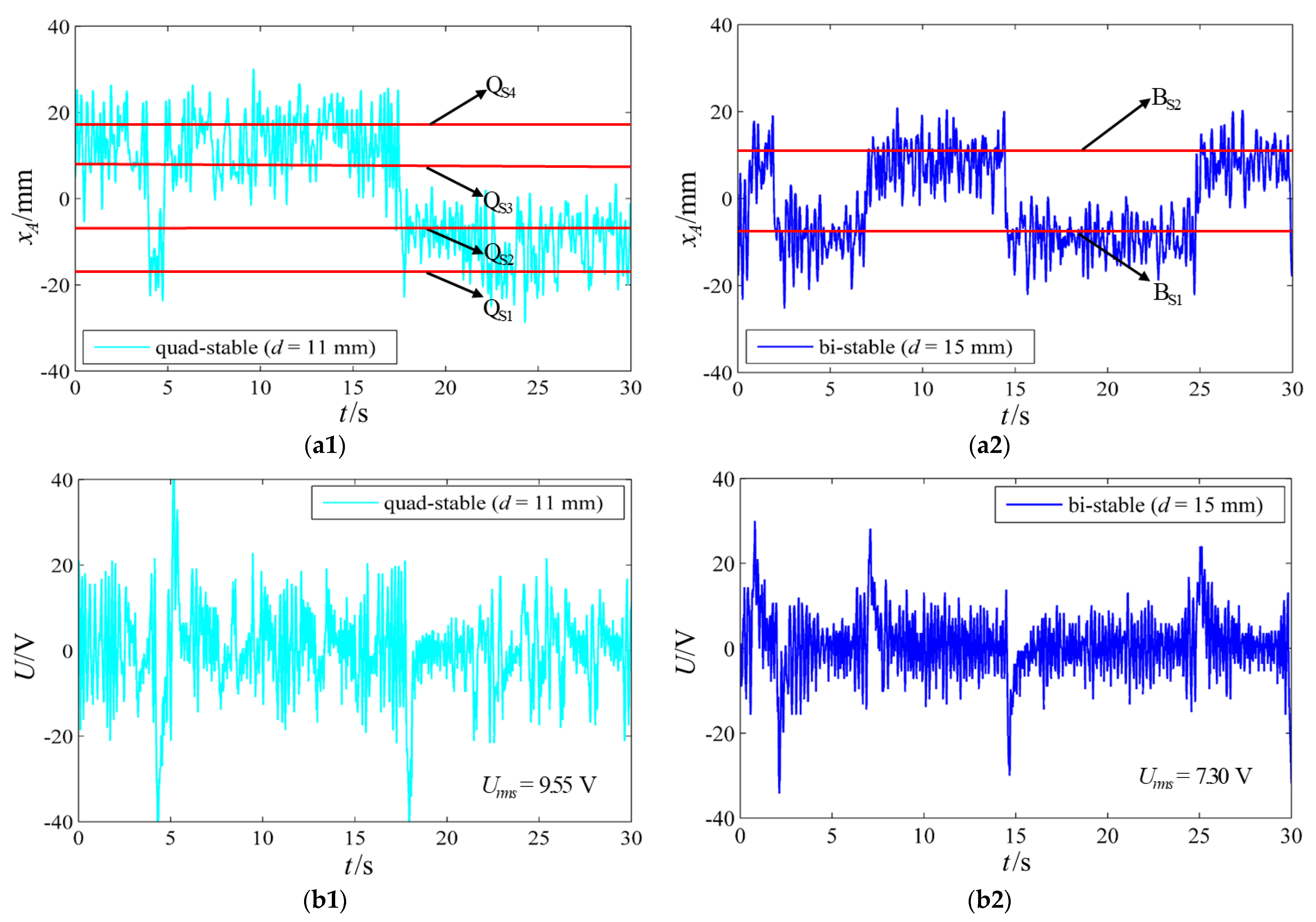
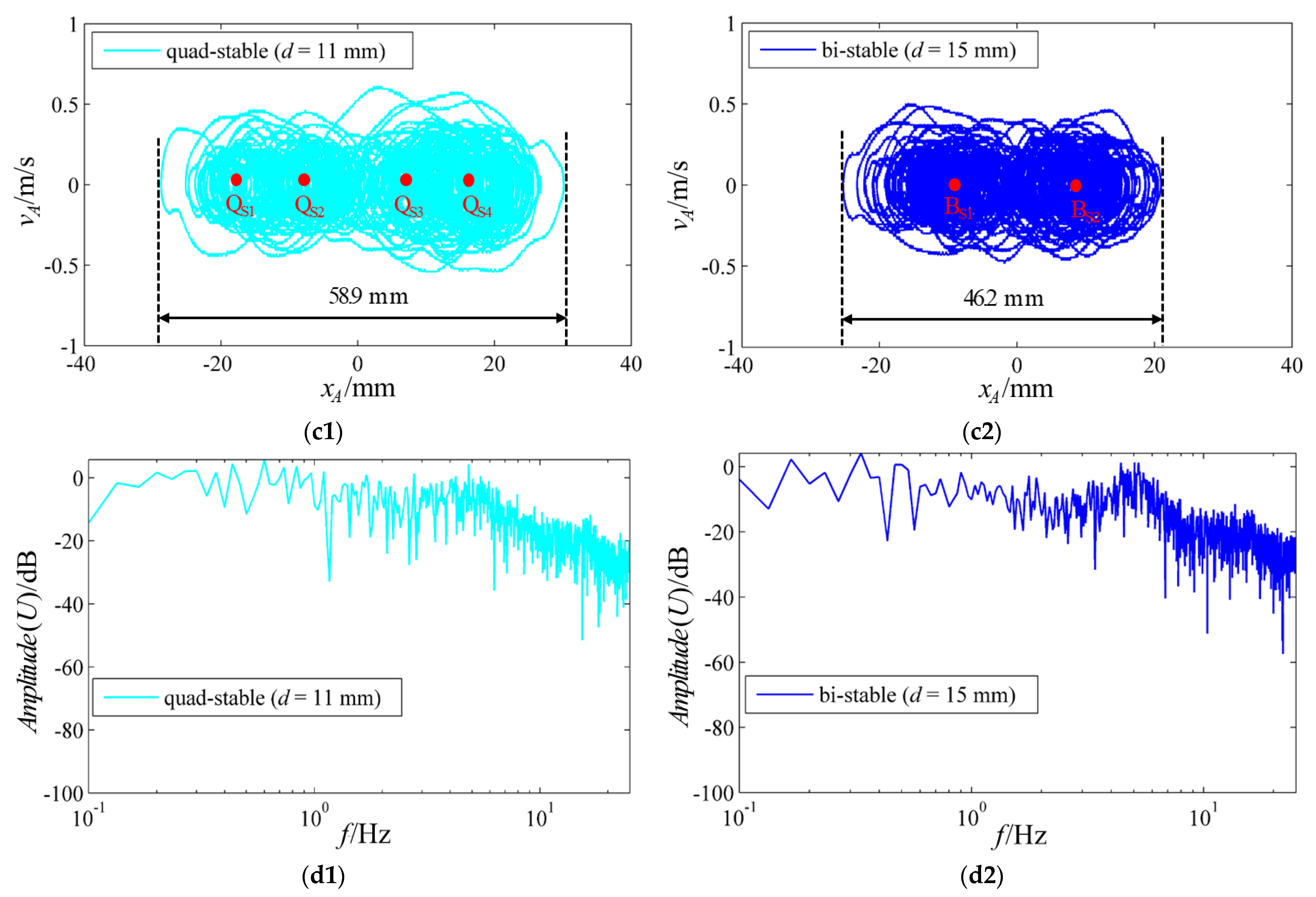
4.2. Experimental Energy Harvesting Performance of the QPEH
5. Discussion
6. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Orrego, S.; Shoele, K.; Ruas, A.; Doran, K.; Caggiano, B.; Mittal, R.; Kang, S. Harvesting ambient wind energy with an inverted piezoelectric flag. Appl. Energy 2017, 194, 212–222. [Google Scholar] [CrossRef]
- Lim, K.; Peddigari, M.; Park, C.; Lee, H.; Min, Y.; Kim, J.; Ahn, C.; Choi, J.; Hahn, B.; Choi, J.; et al. A high output magneto-mechano-triboelectric generator enabled by accelerated water-soluble nano-bullets for powering a wireless indoor positioning system. Energy Environ. Sci. 2019, 12, 666–674. [Google Scholar] [CrossRef]
- Peddigari, M.; Kwak, M.; Min, Y.; Ahn, C.; Choi, J.; Hahn, B.; Choi, C.; Hwang, G.; Yoon, W.; Jang, J. Lifetime estimation of single crystal macro-fiber composite-based piezoelectric energy harvesters using accelerated life testing. Nano Energy 2021, 88, 106279. [Google Scholar] [CrossRef]
- Hannan, M.; Mutashar, S.; Samad, S.; Hussain, A. Energy harvesting for the implantable biomedical devices: Issues and challenges. BioMed. Eng. Online 2014, 13, 79. [Google Scholar] [CrossRef]
- Hwang, G.; Park, H.; Lee, J.; Oh, S.; Park, K.; Byun, M.; Park, H.; Ahn, G.; Jeong, C.; No, K.; et al. Self-powered cardiac pacemaker enabled by flexible single crystalline PMN-PT piezoelectric energy harvester. Adv. Mater. 2014, 26, 4880–4887. [Google Scholar] [CrossRef]
- Lai, Z.; Xu, J.; Bowen, C.; Zhou, S. Self-powered and self-sensing devices based on human motion. Joule 2022, 6, 1501–1565. [Google Scholar] [CrossRef]
- Jung, I.; Choi, J.; Park, H.; Lee, T.; Nahm, S.; Song, H.; Kim, S.; Kang, C. Design principles for coupled piezoelectric and electromagnetic hybrid energy harvesters for autonomous sensor systems. Nano Energy 2020, 75, 104921. [Google Scholar] [CrossRef]
- Song, H.; Kim, S.; Kim, H.; Lee, D.; Kang, C.; Nahm, S. Piezoelectric energy harvesting design principles for materials and structures: Material figure-of-merit and self-resonance tuning. Adv. Mater. 2020, 32, 2002208. [Google Scholar] [CrossRef]
- Khazaee, M.; Rezaniakolaie, A.; Rosendahl, L. A broadband macro-fiber-composite piezoelectric energy harvester for higher energy conversion from practical wideband vibrations. Nano Energy 2020, 76, 104978. [Google Scholar] [CrossRef]
- Lin, Z.; Yang, Y.; Wu, J.; Liu, Y.; Zhang, F.; Wang, Z. BaTiO3 nanotubes-based flexible and transparent nanogenerators. J. Phys. Chem. Lett. 2012, 3, 3599–3604. [Google Scholar] [CrossRef] [Green Version]
- Foong, F.; Thein, C.; Yurchenko, D. A novel high-power density, low-frequency electromagnetic vibration energy harvester based on anti-phase motion. Energy Convers. Manag. 2021, 238, 114175. [Google Scholar] [CrossRef]
- Wang, Z.; Wang, W.; Gu, F.; Wang, C.; Zhang, Q.; Feng, G.; Ball, A. On-rotor electromagnetic energy harvester for powering a wireless condition monitoring system on bogie frames. Energy Convers. Manag. 2021, 243, 114413. [Google Scholar] [CrossRef]
- Thomson, G.; Lai, Z.; Val, D.; Yurchenko, D. Advantages of nonlinear energy harvesting with dielectric elastomers. J. Sound Vib. 2019, 442, 167–182. [Google Scholar] [CrossRef]
- Zhang, C.; Lai, Z.; Rao, X.; Zhang, J.; Yurchenko, D. Energy harvesting from a novel contact-type dielectric elastomer generator. Energy Convers. Manag. 2020, 205, 112351. [Google Scholar] [CrossRef]
- Mohammadi, S.; Esfandiari, A. Magnetostrictive vibration energy harvesting using strain energy method. Energy 2015, 81, 519–525. [Google Scholar] [CrossRef]
- Liu, H.; Zhao, L.; Chang, Y.; Cong, C. Design and characteristic analysis of magnetostrictive bistable vibration harvester with displacement amplification mechanism. Energy Convers. Manag. 2021, 243, 114361. [Google Scholar] [CrossRef]
- Han, Y.; Wang, W.; Zou, J.; Li, Z.; Cao, X.; Xu, S. Self-powered energy conversion and energy storage system based on triboelectric nanogenerator. Nano Energy 2020, 76, 105008. [Google Scholar] [CrossRef]
- Jiao, J.; Lu, Q.; Wang, Z.; Qin, Y.; Cao, X. Sandwich as a triboelectric nanogenerator. Nano Energy 2021, 79, 105411. [Google Scholar] [CrossRef]
- Fu, J.; Hou, Y.; Zheng, M.; Zhu, M. Flexible piezoelectric energy harvester with extremely high power generation capability by sandwich structure design strategy. ACS Appl. Mater. Interfaces 2020, 12, 9766–9774. [Google Scholar] [CrossRef]
- Kwak, W.; Lee, Y. Optimal design and experimental verification of piezoelectric energy harvester with fractal structure. Appl. Energy 2021, 282, 116121. [Google Scholar] [CrossRef]
- Song, H.; Kumarb, P.; Sriramdasb, R.; Leeb, H.; Sharpesc, N.; Kang, M.; Mauryab, D.; Sanghadasad, M.; Kang, H.; Ryu, J.; et al. Broadband dual phase energy harvester: Vibration and magnetic field. Appl. Energy 2018, 225, 1132–1142. [Google Scholar] [CrossRef]
- Priya, S.; Song, H.; Zhou, Y.; Varghese, R.; Chopra, A.; Kim, S.; Kanno, I.; Wu, L.; Ha, D.; Ryu, J.; et al. A review on piezoelectric energy harvesting: Materials, methods, and circuits. Energy Harvest. Syst. 2019, 4, 3–39. [Google Scholar] [CrossRef]
- Song, H.; Kumar, P.; Maurya, D.; Kang, M.; Reynolds, W.; Jeong, D.; Kang, C.; Priya, S. Ultra-low resonant piezoelectric MEMS energy harvester with high power density. J. Microelectromech. Syst. 2017, 26, 1226–1234. [Google Scholar] [CrossRef]
- Jiang, X.; Zou, H.; Zhang, W. Design and analysis of a multi-step piezoelectric energy harvester using buckled beam driven by magnetic excitation. Energy Convers. Manag. 2017, 145, 129–137. [Google Scholar] [CrossRef]
- Li, Z.; Zu, J.; Yang, Z. Introducing hinge mechanisms to one compressive-mode piezoelectric energy harvester. J. Renew. Sustain. Energy 2018, 10, 034704. [Google Scholar] [CrossRef]
- Ferrari, M.; Ferrari, V.; Guizzetti, M.; Marioli, D.; Taroni, A. Piezoelectric multifrequency energy converter for power harvesting in autonomous microsystems. Sensor Actuators A-Phys. 2018, 142, 329–335. [Google Scholar] [CrossRef]
- Liu, J.; Fang, H.; Xu, Z.; Mao, X.; Shen, X.; Chen, D.; Liao, H.; Cai, B. A MEMS-based piezoelectric power generator array for vibration energy harvesting. Microelectron. J. 2008, 39, 802–806. [Google Scholar] [CrossRef]
- Wickenheiser, A.; Garcia, E. Broadband vibration-based energy harvesting improvement through frequency up-conversion by magnetic excitation. Smart Mater. Struct. 2010, 19, 65020. [Google Scholar] [CrossRef]
- Li, P.; Xu, N.; Gao, C. A multi-mechanisms composite frequency up-conversion energy harvester. Int. J. Precis. Eng. Manuf. 2020, 21, 1781–1788. [Google Scholar] [CrossRef]
- Cottone, F.; Vocca, H.; Gammaitoni, L. Nonlinear energy harvesting. Phys. Rev. Lett. 2009, 102, 080601. [Google Scholar] [CrossRef] [Green Version]
- Arrieta, A.; Hagedorn, P.; Erturk, A.; Inman, D. A piezoelectric bistable plate for nonlinear broadband energy harvesting. Appl. Phys. Lett. 2010, 97, 104102. [Google Scholar] [CrossRef]
- Masana, R.; Daqaq, M. Relative performance of a vibratory energy harvester in mono- and bi-stable potentials. J. Sound Vib. 2011, 330, 6036–6052. [Google Scholar] [CrossRef]
- Masana, R.; Daqaq, M. Energy harvesting in the super-harmonic frequency region of a twin-well oscillator. J. Appl. Phys. 2012, 111, 044501. [Google Scholar] [CrossRef]
- Qian, F.; Zhou, S.; Zuo, L. Approximate solutions and their stability of a broadband piezoelectric energy harvester with a tunable potential function. Commun. Nonlinear Sci. Numer. Simulat. 2020, 80, 104984. [Google Scholar] [CrossRef]
- Qian, F.; Hajj, M.; Zuo, L. Bio-inspired bi-stable piezoelectric harvester for broadband vibration energy harvesting. Energy Convers. Manag. 2020, 222, 113174. [Google Scholar] [CrossRef]
- Syta, A.; Bowen, C.; Kim, H.; Rysak, A.; Litak, G. Experimental analysis of the dynamical response of energy harvesting devices based on bistable laminated plates. Meccanica 2015, 50, 1961–1970. [Google Scholar] [CrossRef] [PubMed]
- Syta, A.; Bowen, C.; Kim, H.; Rysak, A.; Litak, G. Responses of bistable piezoelectric composite energy harvester by means of recurrences. Mech. Syst. Signal Process. 2016, 76, 823–832. [Google Scholar] [CrossRef]
- Arrieta, A.; Delpero, T.; Bergamini, A.; Ermanni, P. Broadband vibration energy harvesting based on cantilevered piezoelectric bi-stable composites. Appl. Phys. Lett. 2013, 102, 173904. [Google Scholar] [CrossRef]
- Li, Y.; Zhou, S.; Yang, Z.; Guo, T.; Mei, X. High-performance low-frequency bistable vibration energy harvesting plate with tip mass blocks. Energy 2019, 180, 737–750. [Google Scholar] [CrossRef]
- Firouzian-Nejad, A.; Bowen, C.; Mustapha, S.; Ghayour, M.; Ziaei-Rad, S. Bi-stable hybrid composite laminates containing metallic strips: An experimental and numerical investigation. Smart Mater. Struct. 2019, 28, 055030. [Google Scholar] [CrossRef]
- Firouzian-Nejad, A.; Mustapha, S.; Ziaei-Rad, S.; Ghayour, M. Characterization of bistable pure and hybrid composite laminates–an experimental investigation of the static and dynamic responses. J. Compos. Mater. 2019, 53, 653–667. [Google Scholar] [CrossRef]
- Ferrari, M.; Ferrari, V.; Guizzetti, M.; Ando, B.; Baglio, S.; Trigona, C. Improved energy harvesting from wideband vibrations by nonlinear piezoelectric converters. Sensor Actuators A-Phys. 2010, 162, 425–431. [Google Scholar] [CrossRef]
- Leng, Y.; Gao, Y.; Tan, D.; Fan, S.; Lai, Z. An elastic-support model for enhanced bistable piezoelectric energy harvesting from random vibrations. J. Appl. Phys. 2015, 117, 064901. [Google Scholar] [CrossRef]
- Wang, G.; Liao, W.; Yang, B.; Wang, X.; Xu, W.; Li, X. Dynamic and energetic characteristics of a bistable piezoelectric vibration energy harvester with an elastic magnifier. Mech. Syst. Signal Process. 2018, 105, 427–446. [Google Scholar] [CrossRef]
- Zhou, S.; Cao, J.; Inman, D.; Lin, J.; Liu, S.; Wang, Z. Broadband tristable energy harvester: Modeling and experiment verification. Appl. Energy 2014, 133, 33–39. [Google Scholar] [CrossRef]
- Cao, J.; Zhou, S.; Wang, W.; Li, J. Influence of potential well depth on nonlinear tristable energy harvesting. Appl. Phys. Lett. 2015, 106, 173903. [Google Scholar] [CrossRef]
- Leng, Y.; Tan, D.; Liu, J.; Zhang, Y.; Fan, S. Magnetic force analysis and performance of a tri-stable piezoelectric energy harvester under random excitation. J. Sound Vib. 2017, 406, 146–160. [Google Scholar] [CrossRef]
- Zhou, Z.; Qin, W.; Zhu, P. Harvesting performance of quad-stable piezoelectric energy harvester: Modeling and experiment. Mech. Syst. Signal Process. 2018, 110, 260–272. [Google Scholar] [CrossRef]
- Zhou, Z.; Qin, W.; Zhu, P. A broadband quad-stable energy harvester and its advantages over bi-stable harvester: Simulation and experiment verification. Mech. Syst. Signal Process. 2017, 84, 158–168. [Google Scholar] [CrossRef]
- Mei, X.; Zhou, S.; Yang, Z.; Kaizuka, T.; Nakano, K. Enhancing energy harvesting in low-frequency rotational motion by a quad-stable energy harvester with time-varying potential wells. Mech. Syst. Signal Process. 2021, 148, 107167. [Google Scholar] [CrossRef]
- Ju, Y.; Li, Y.; Tan, J.; Zhao, Z.; Wang, G. Transition mechanism and dynamic behaviors of a multi-stable piezoelectric energy harvester with magnetic interaction. J. Sound Vib. 2021, 501, 116074. [Google Scholar] [CrossRef]
- Zou, D.; Chen, K.; Rao, Z.; Cao, J.; Liao, W. Design of a quad-stable piezoelectric energy harvester capable of programming the coordinates of equilibrium points. Nonlinear Dynam. 2022, 108, 857–871. [Google Scholar] [CrossRef]
- Wang, C.; Zhang, Q.; Wang, W.; Feng, J. A low-frequency, wideband quad-stable energy harvester using combined nonlinearity and frequency up-conversion by cantilever-surface contact. Mech. Syst. Signal Process. 2018, 112, 305–318. [Google Scholar] [CrossRef]
- Karami, M.; Inman, D. Equivalent damping and frequency change for linear and nonlinear hybrid vibrational energy harvesting systems. J. Sound Vib. 2011, 330, 5583–5597. [Google Scholar] [CrossRef]
- Erturk, A.; Inman, D. Broadband piezoelectric power generation on high-energy orbits of the bistable Duffing oscillator with electromechanical coupling. J. Sound Vib. 2011, 330, 2339–2353. [Google Scholar] [CrossRef]
- Sun, S.; Leng, Y.; Su, X.; Zhang, Y.; Chen, X.; Xu, J. Performance of a novel dual-magnet tri-stable piezoelectric energy harvester subjected to random excitation. Energy Convers. Manag. 2021, 239, 114246. [Google Scholar] [CrossRef]
- Sun, S.; Leng, Y.; Zhang, Y.; Su, X.; Fan, S. Analysis of magnetic force and potential energy function of multi-stable cantilever beam with two magnets. Acta. Phys. Sin. 2020, 69, 140502. [Google Scholar] [CrossRef]
- Sun, S.; Leng, Y. Investigation of a novel tri-stable cantilever beam with two magnets. In Proceedings of the International Design Engineering Technical Conferences and Computers and Information in Engineering Conference, Virtual, 17–19 August 2020; Volume 83969, p. V007T07A005. [Google Scholar]





| Parameter | Value |
|---|---|
| Cantilever beam substrate (silicon steel) | |
| Young’s modulus Ec | 200 GPa |
| Density ρC | 7700 kg/m3 |
| Length lC | 62 mm |
| Width wC | 10 mm |
| Thickness tC | 0.18 mm |
| Piezoelectric patch (MFC: M2807-P2) | |
| Young’s modulus EP | 30 GPa |
| Density ρP | 5440 kg/m3 |
| Length lP | 28 mm |
| Width wP | 7 mm |
| Thickness tP | 0.3 mm |
| Equivalent capacitance CP | 20 × 10−9 F |
| Piezoelectric cantilever beam | |
| Equivalent mass Meq | 0.0145 kg |
| Equivalent stiffness Keq | 21.4 N/m |
| Equivalent damping ηeq | 0.295 N⋅s/m |
| Electromechanical coupling coefficient θ | −8.25 × 10−6 N/V |
| Tip magnet (Nd2Fe14B: N35) | |
| Density ρA | 7500 kg/m3 |
| Length lA | 27.5 mm |
| Width wA | 27.5 mm |
| Thickness tA | 2.5 mm |
| Magnetization MA | 6.2 × 105 A/m |
| External magnet (Nd2Fe14B: N35) | |
| Density ρB | 7500 kg/m3 |
| Thickness tB | 3.5 mm |
| Outer diameter Φo | 40 mm |
| Inner diameter Φi | 20 mm |
| Magnetization MB | 6.2 × 105 A/m |
| Vacuum permeability μ0 | 4π × 10−7 N/A2 |
| Resistance load RL | 30 MΩ |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sun, S.; Leng, Y.; Hur, S.; Sun, F.; Su, X.; Song, H.-C.; Kang, C.-Y. Energy Harvesting Performance of a Novel Nonlinear Quad-Stable Piezoelectric Energy Harvester with Only One External Magnet. Machines 2022, 10, 803. https://doi.org/10.3390/machines10090803
Sun S, Leng Y, Hur S, Sun F, Su X, Song H-C, Kang C-Y. Energy Harvesting Performance of a Novel Nonlinear Quad-Stable Piezoelectric Energy Harvester with Only One External Magnet. Machines. 2022; 10(9):803. https://doi.org/10.3390/machines10090803
Chicago/Turabian StyleSun, Shuailing, Yonggang Leng, Sunghoon Hur, Fei Sun, Xukun Su, Hyun-Cheol Song, and Chong-Yun Kang. 2022. "Energy Harvesting Performance of a Novel Nonlinear Quad-Stable Piezoelectric Energy Harvester with Only One External Magnet" Machines 10, no. 9: 803. https://doi.org/10.3390/machines10090803
APA StyleSun, S., Leng, Y., Hur, S., Sun, F., Su, X., Song, H.-C., & Kang, C.-Y. (2022). Energy Harvesting Performance of a Novel Nonlinear Quad-Stable Piezoelectric Energy Harvester with Only One External Magnet. Machines, 10(9), 803. https://doi.org/10.3390/machines10090803






