Vibration Measurement and Numerical Modeling Analysis of Transformer Windings and Iron Cores Based on Voltage and Current Harmonics
Abstract
:1. Introduction
2. Structure and Operating Environment
2.1. Structural Composition and Operating Parameters
2.2. Types of Converter Transformers
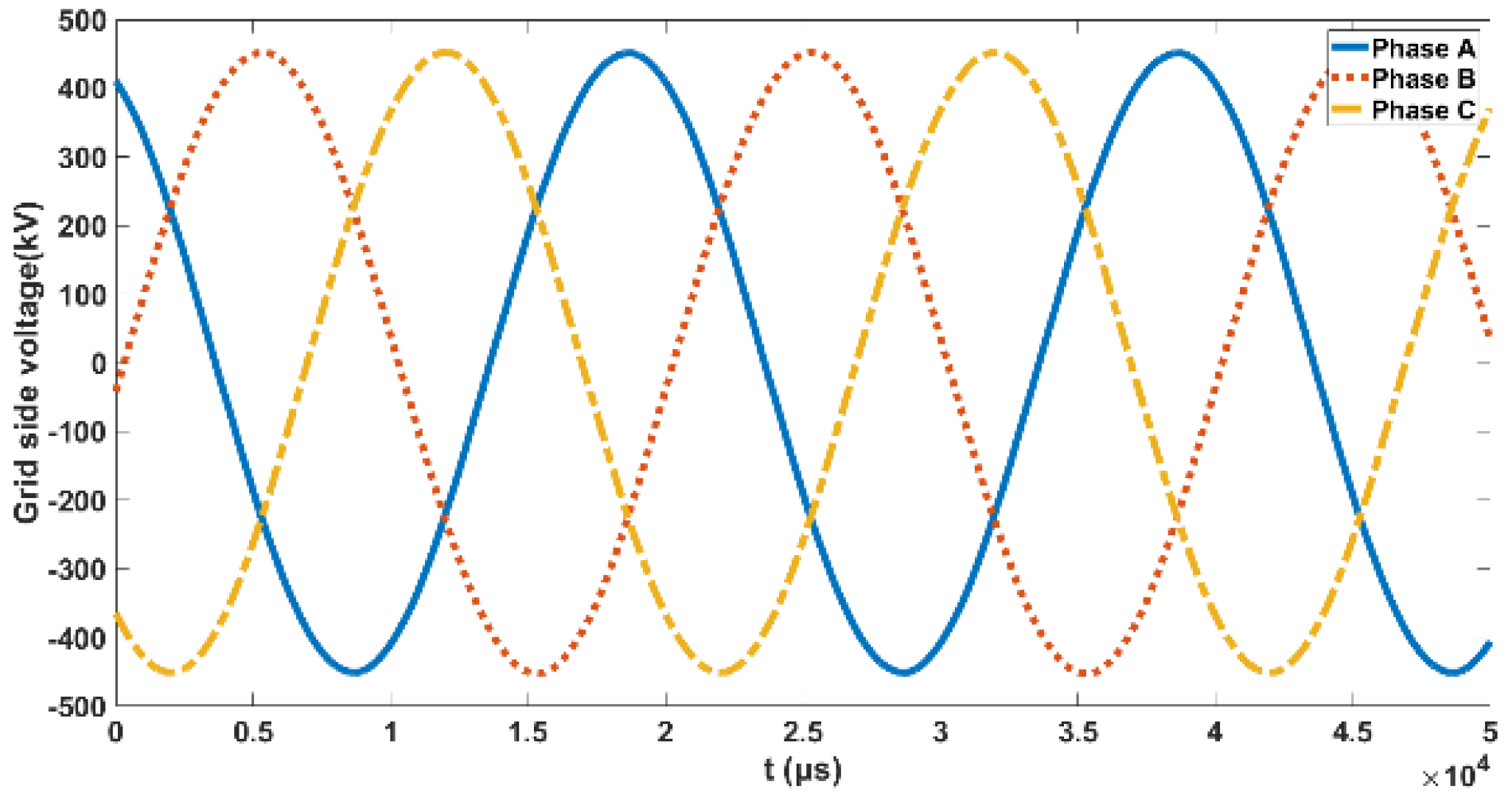
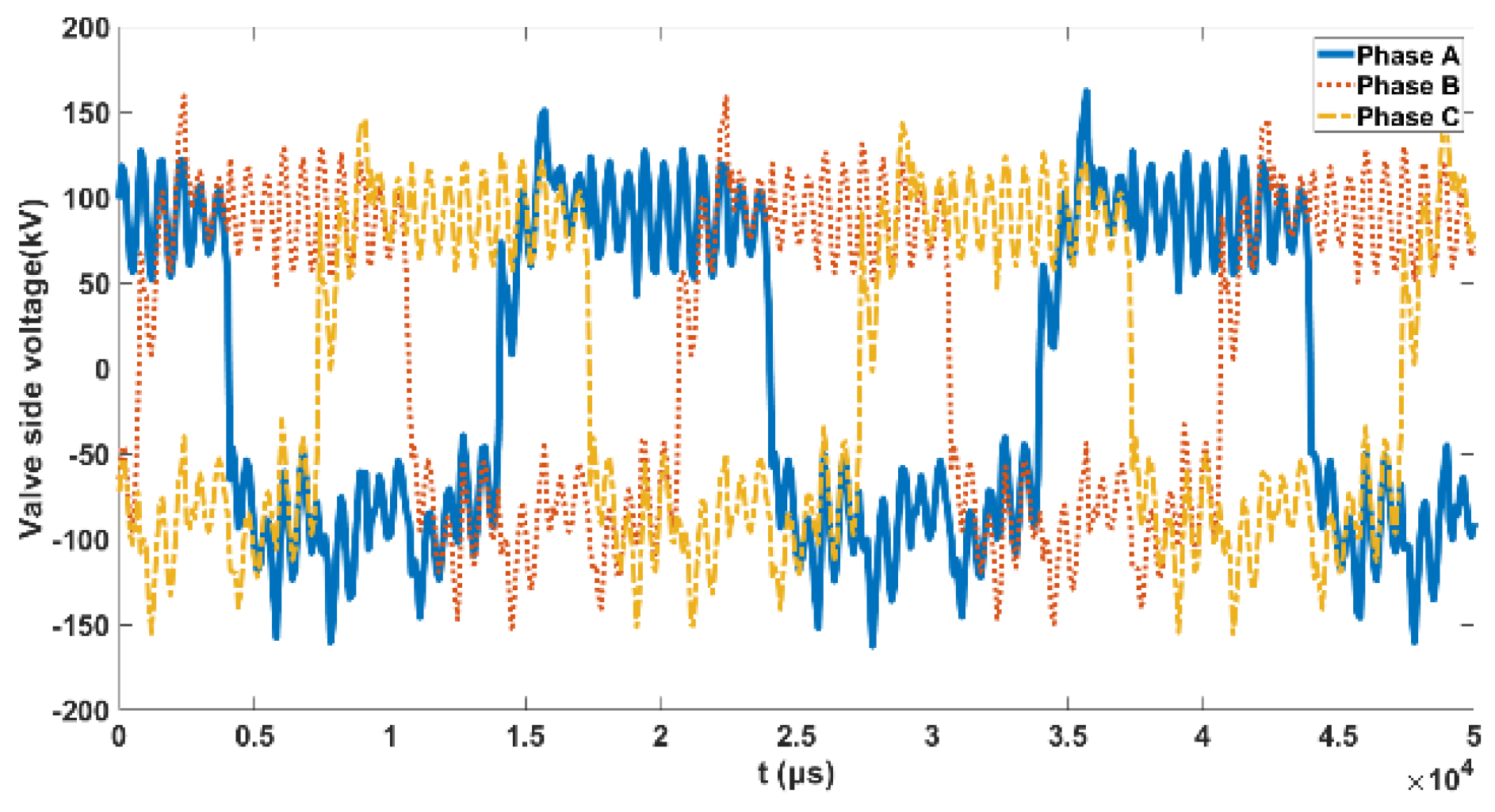
2.3. Grid Side and Valve Side Voltage
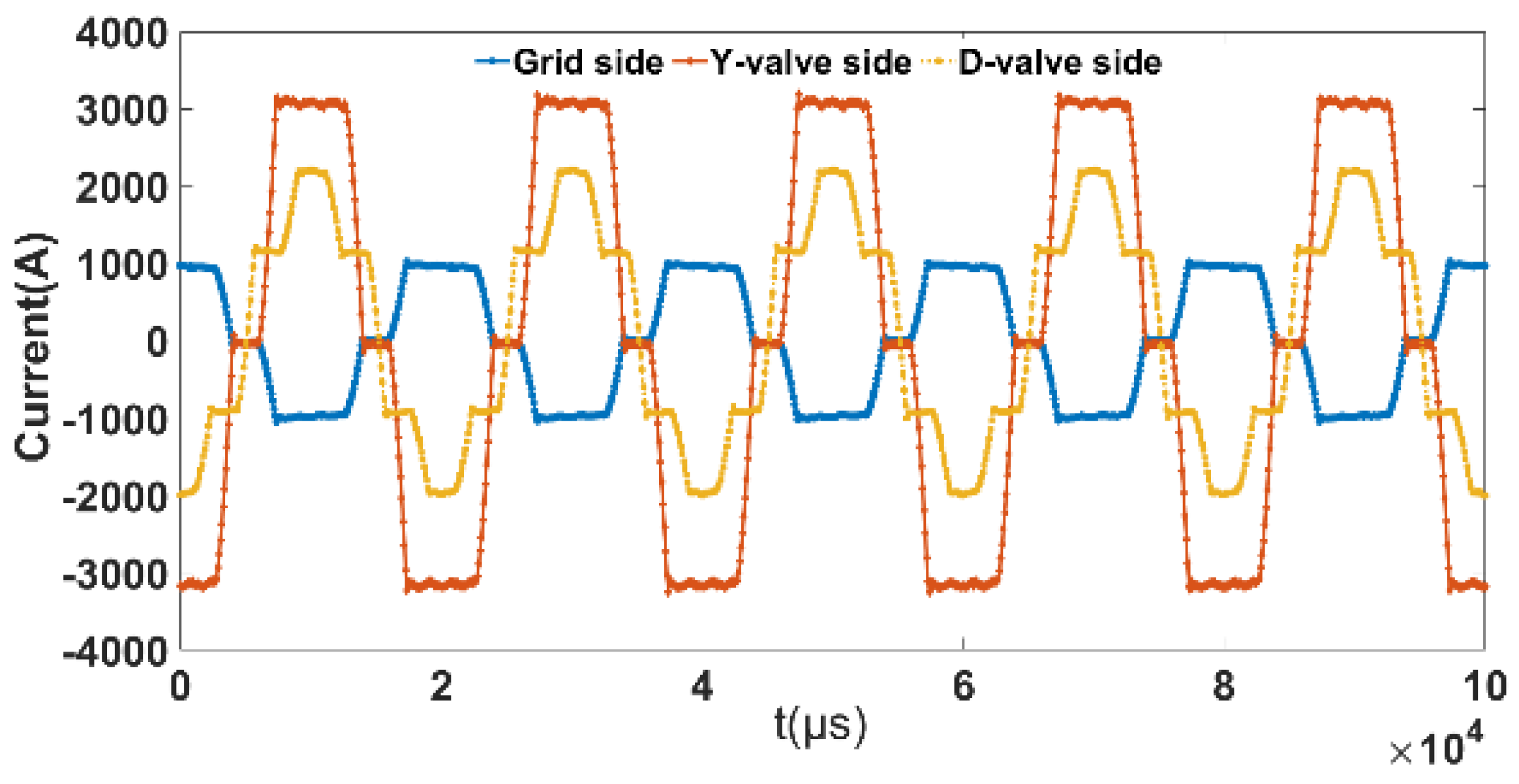
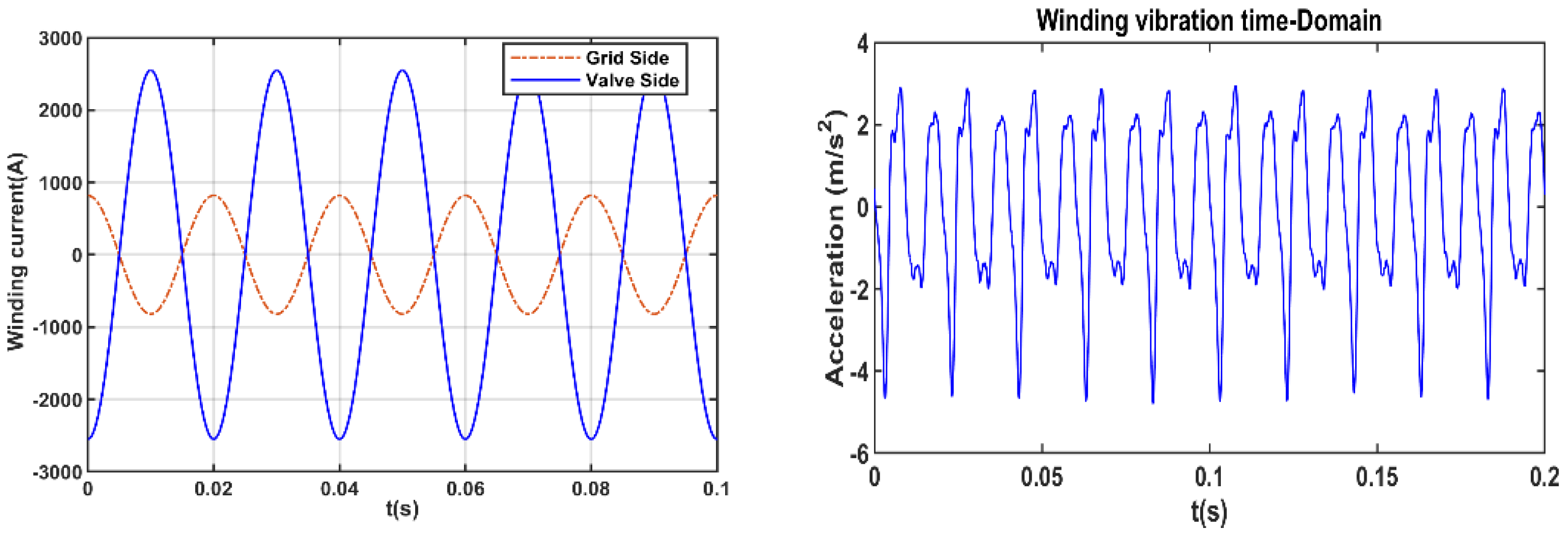
2.4. Grid-Side and Valve-Side Current
3. Numerical Model of Winding and Core Vibration Considering Harmonics
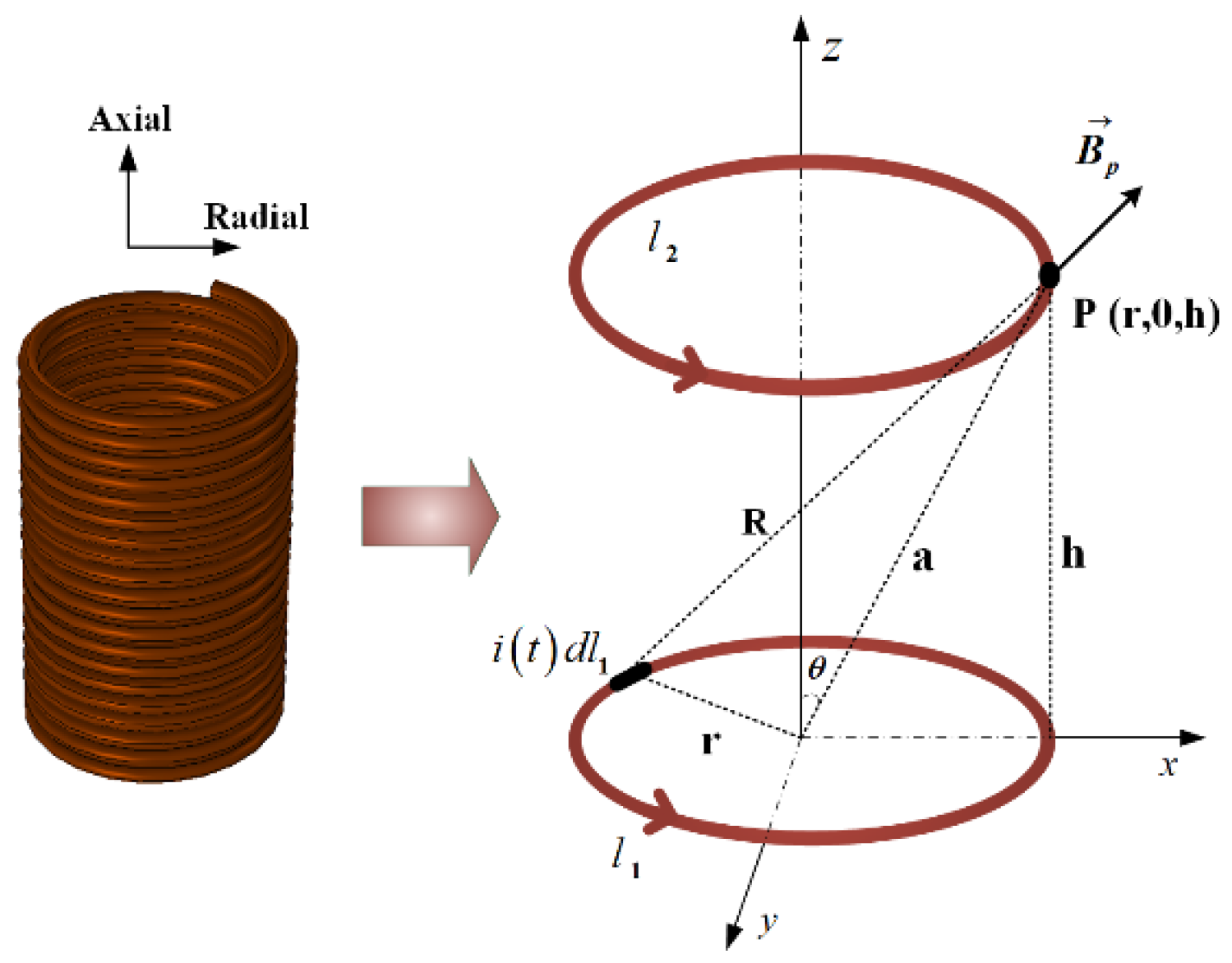
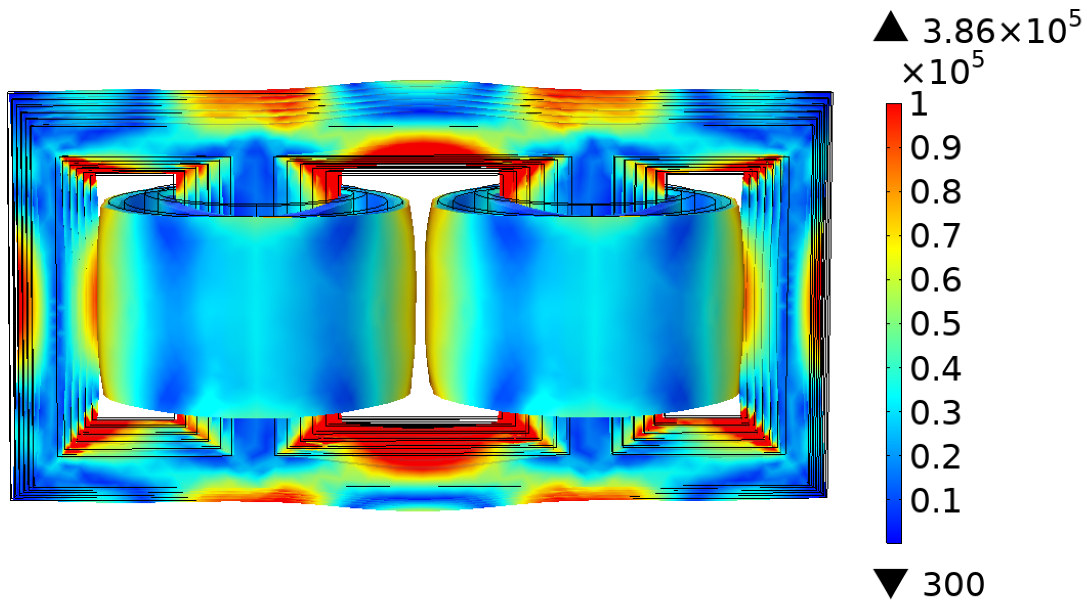
3.1. Iron Core Vibration Model under Harmonics
3.2. Winding Vibration Model under Harmonics
3.3. Theoretical Calculation of Vibration under Fundamental and Harmonics
4. Vibration Measurement Experiment Design
4.1. Vibration Measurement and Sensor Parameters
4.2. Sensors Layout
4.3. Vibration Condition Design with or without Harmonics
5. Results and Discussion
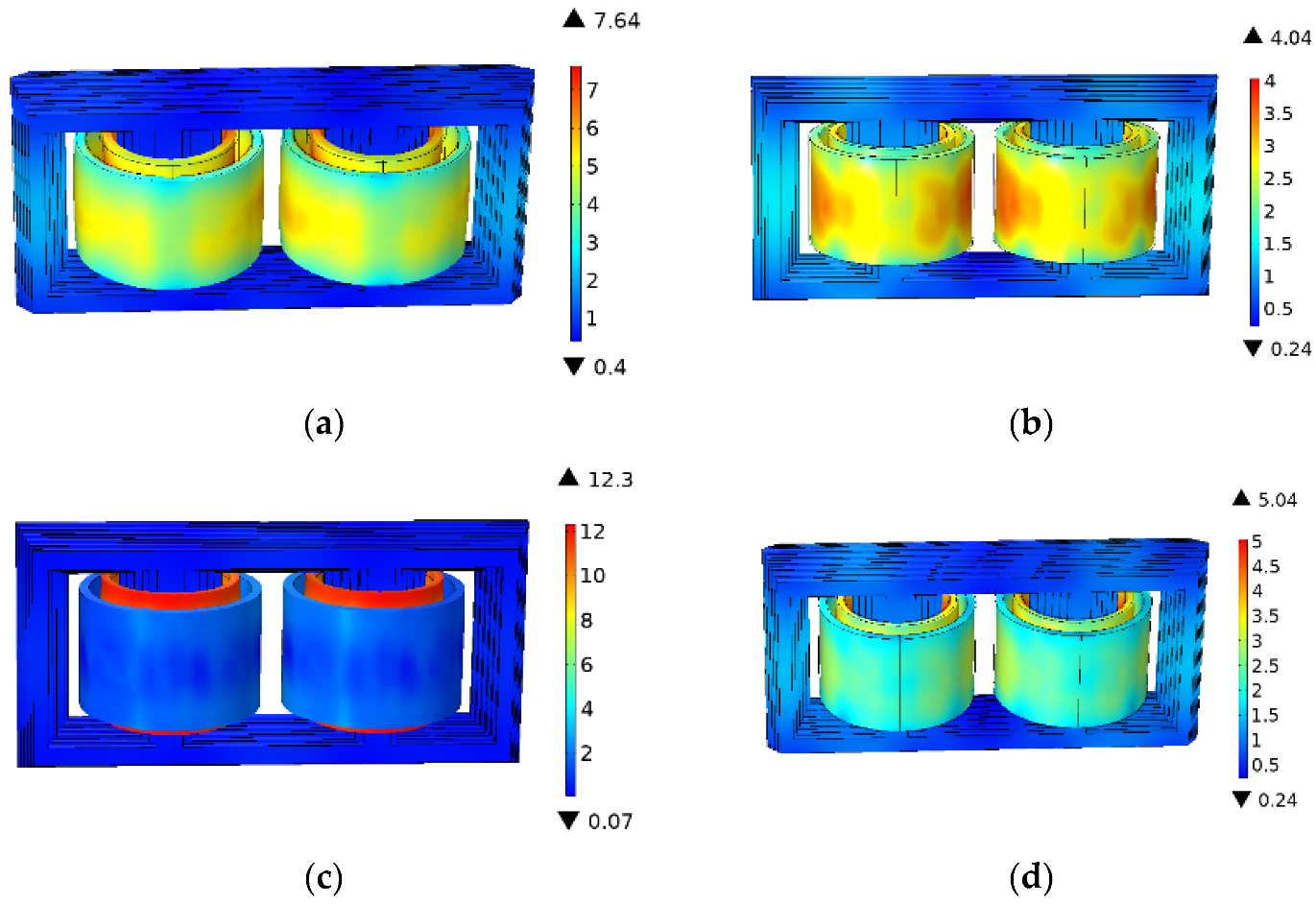
5.1. Winding and Core Vibration under Sinusoidal Excitation (No Harmonics)
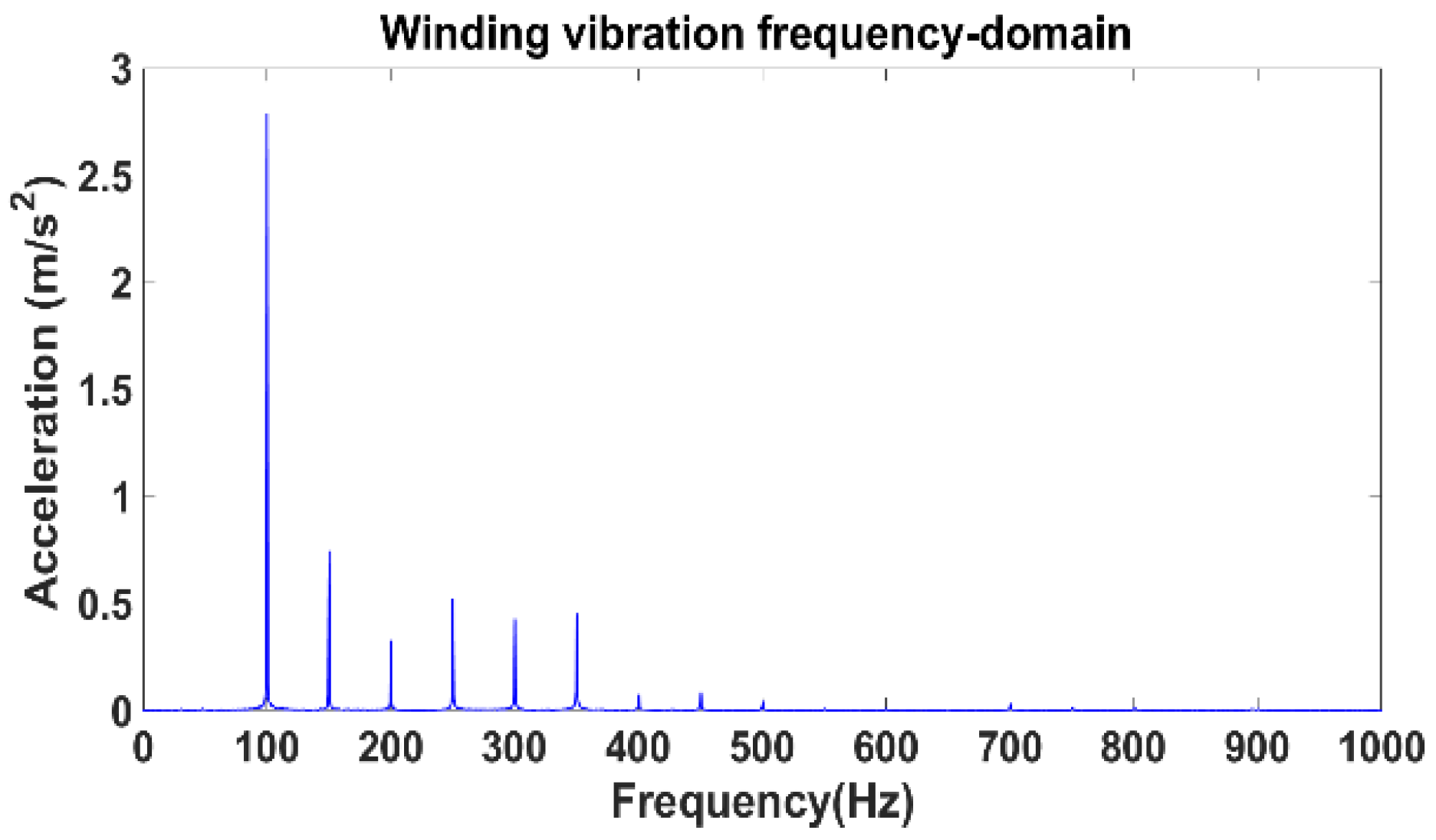
5.1.1. Winding Vibration under Sinusoidal Current
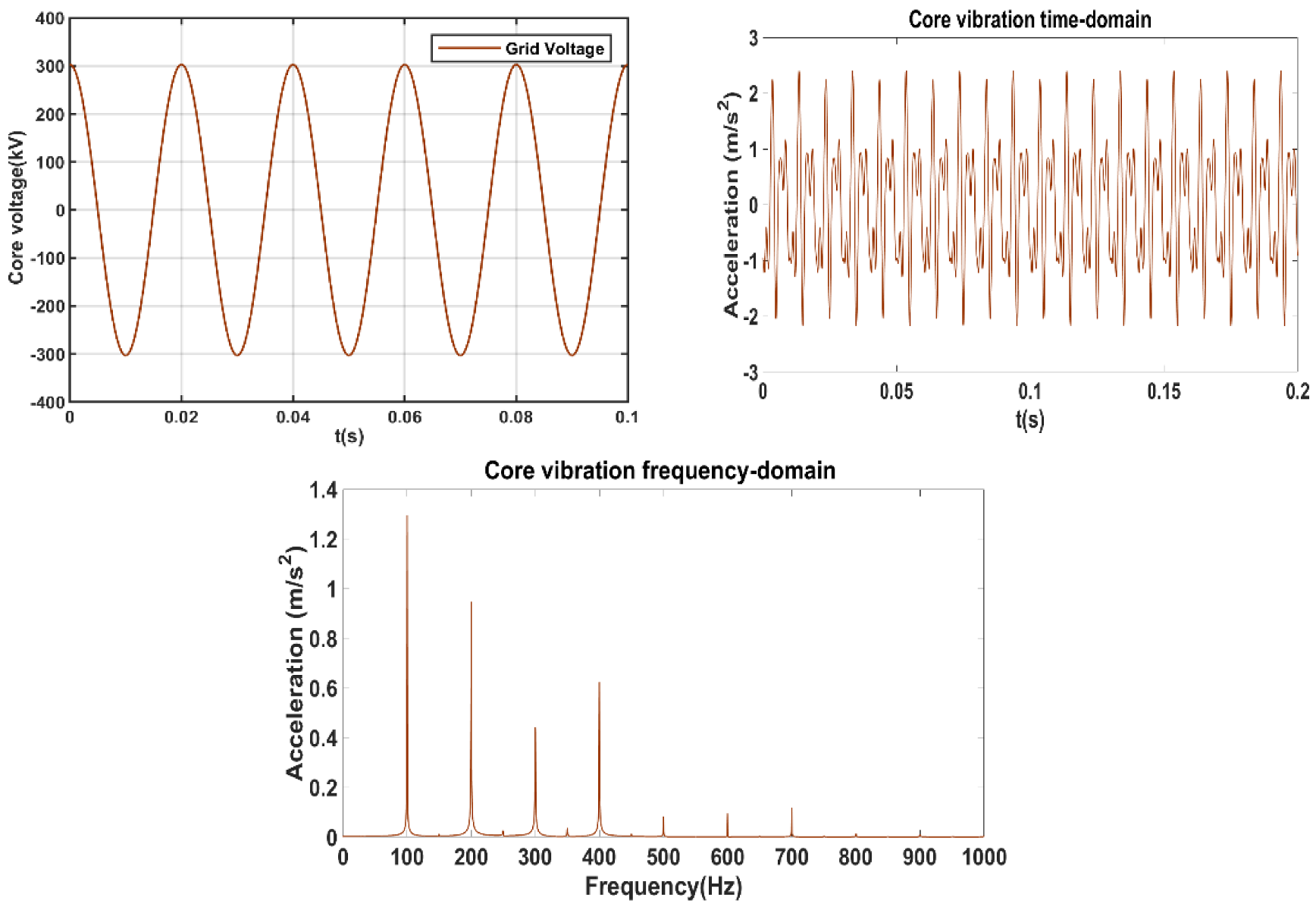
5.1.2. Core Vibration under Sinusoidal Voltage
5.2. Winding and Core Vibration under Sinusoidal Excitation (No Harmonics)
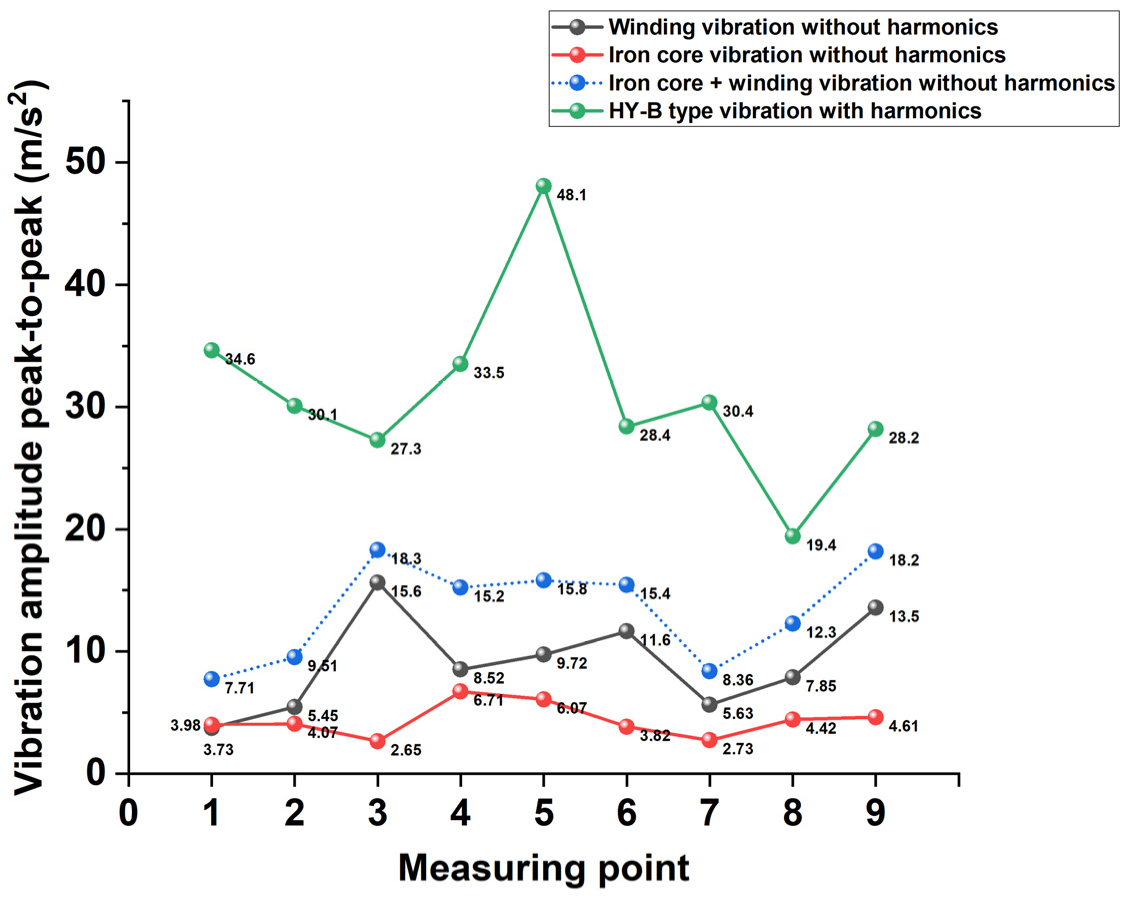
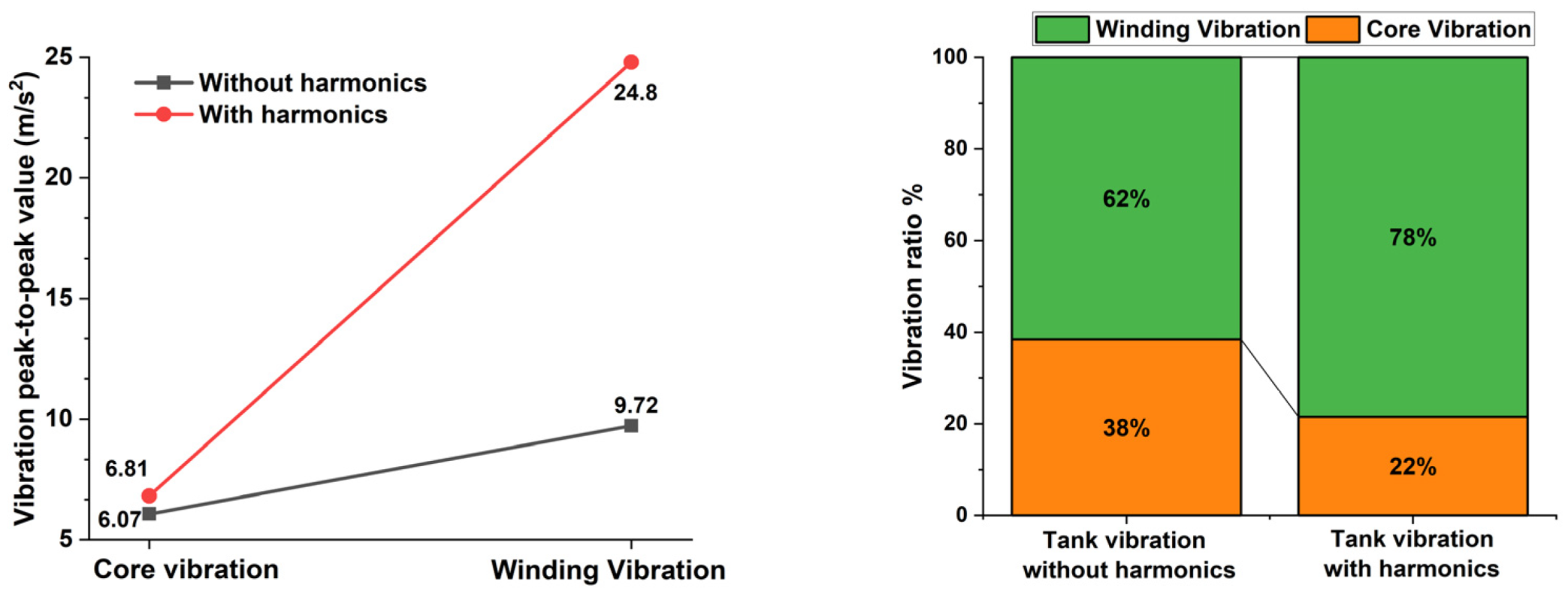
5.2.1. Vibration Peak-to-Peak Value in the Time Domain
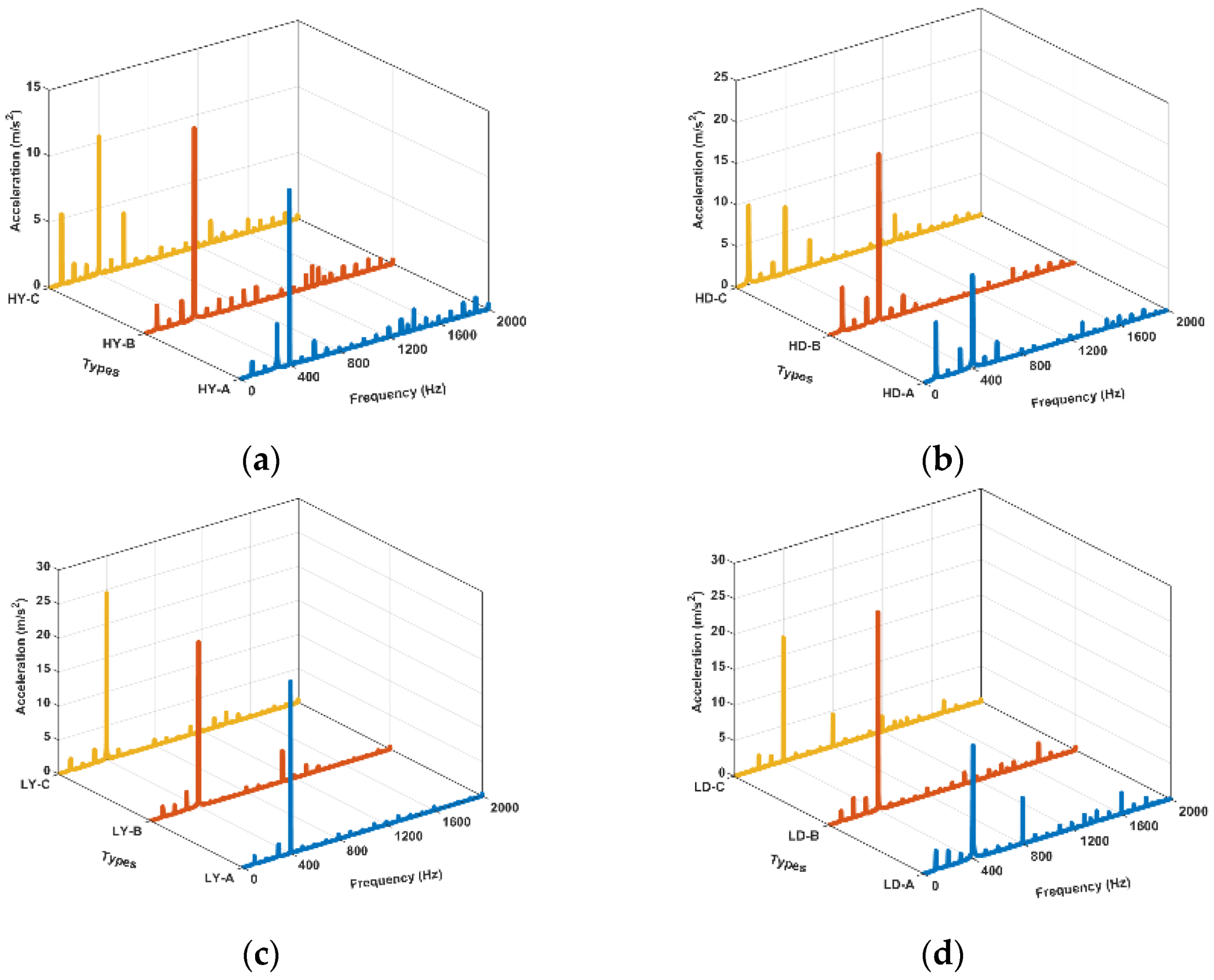
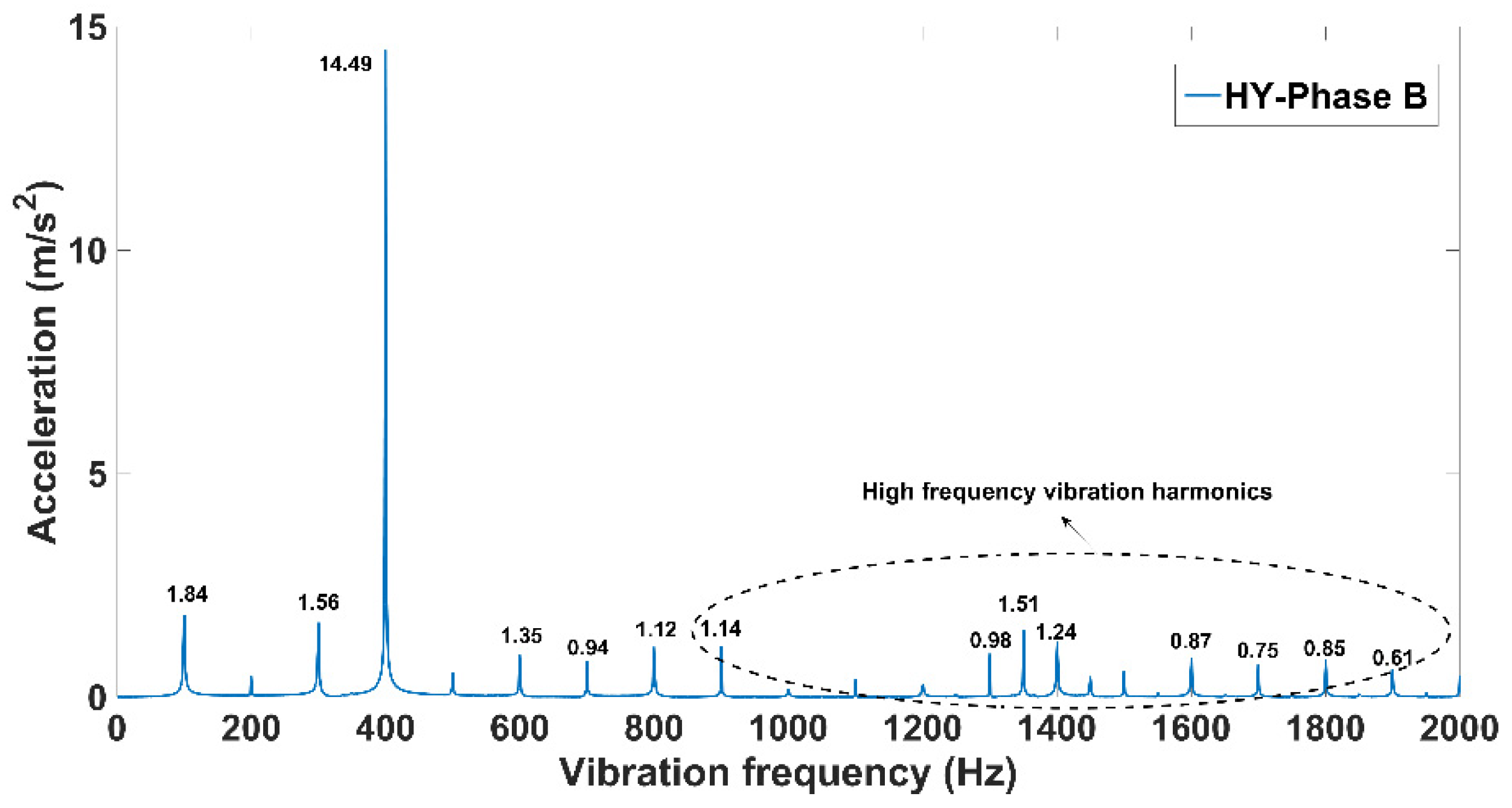
5.2.2. Harmonic Vibration Spectrum
5.2.3. Dominant Frequency under Different Working Conditions
5.3. Contribution of Winding and Iron Core Vibration under Harmonics
5.4. Contribution of Winding and Iron Core Vibration under Harmonics
6. Discussion
7. Conclusions
- (1)
- Under operating conditions, the voltage harmonic components and contents on the grid side and valve side are different, while the current harmonic contents on the grid side and valve side are the same. Among them, the grid-side voltage contains almost no harmonics and the valve-side voltage has abundant (4k ± 1) odd and 6k even harmonics. The currents are dominated by (6k ± 1) odd harmonics. Then, the electromagnetic-vibration numerical model of winding and iron core is constructed based on harmonic distribution.
- (2)
- Harmonics will increase the vibration amplitude, the dominant frequency and the frequency spectrum. The vibration amplitude is more than 3 times that of no harmonics and under the 5th, 7th and other (6k ± 1)th current harmonics, the dominant frequency is mainly 400 Hz and there are many vibration harmonics in the range of 1000–2000 Hz. This is very different from the 100 Hz dominant frequency of the AC transformer.
- (3)
- The winding vibration is related to the current and harmonics, the iron core vibration is determined by the grid-side voltage and the two have different contributions to the tank vibration. The proportion of winding vibration under harmonics increases from 62% to 78%. It can be considered that the main vibration of the tank is winding vibration at this time.
- (4)
- The winding connection method will change the vibration dominant frequency distribution of the tank and the larger current and harmonics make it easier for the dominant frequency to shift from 400 Hz to 800 Hz or 1200 Hz.
Author Contributions
Funding
Conflicts of Interest
References
- Tabrez, M.; Sadhu, P.K.; Lipu, M.S.H.; Iqbal, A.; Husain, M.A.; Ansari, S. Power conversion techniques using multi-phase transformer: Configurations, applications, issues, and recommendations. Machines 2022, 10, 13. [Google Scholar] [CrossRef]
- Shu, Y.; Chen, W. Research and application of UHV power transmission in China. High Volt. 2018, 3, 1–13. [Google Scholar] [CrossRef]
- Liu, D.; Li, X.; Cai, Z. Analysis of the Harmonic Transmission Characteristics of HVDC Transmission Based on a Unified Port Theory Model. IEEE Access 2020, 8, 8922–8934. [Google Scholar] [CrossRef]
- Zollanvari, A.; Kunanbayev, K.; Bitaghsir, S.A.; Bagheri, M. Transformer Fault Prognosis Using Deep Recurrent Neural Network over Vibration Signals. IEEE Trans. Instrum. Meas. 2021, 70, 2502011. [Google Scholar] [CrossRef]
- García, B.; Burgos, J.; Alonso, Á. Transformer tank vibration modeling as a method of detecting winding deformations—Part I: Theoretical foundation. IEEE Trans. Power Deliv. 2006, 21, 157–163. [Google Scholar] [CrossRef]
- Tanzer, T.; Pregartner, H.; Riedenbauer, M.; Labinsky, R.; Witlatschil, M.; Muetze, A.; Krischan, K. Magnetostriction of Electrical Steel and Its Relation to the No-Load Noise of Power Transformers. IEEE Trans. Ind. Appl. 2018, 54, 4306–4314. [Google Scholar] [CrossRef]
- Ni, Q.; Luo, L.; Fan, J.; Jin, Z. Harmonic loss analysis of converter transformer in LCL-HVDC system. Energy Rep. 2020, 6, 352–357. [Google Scholar] [CrossRef]
- Liang, C.; Xu, J.; Luo, L.; Li, Y.; Qi, Q.; Gao, P.; Fu, Y.; Peng, Y. Harmonic Elimination Using Parallel Delta-Connected Filtering Windings for Converter Transformers in HVDC Systems. IEEE Trans. Power Deliv. 2017, 32, 933–941. [Google Scholar] [CrossRef]
- Yamashita, K.I.; Kameda, Y.; Nishikata, S. A Harmonics Elimination Method Using a Three-Winding Transformer for HVDC Transmission Systems. IEEE Trans. Ind. Appl. 2018, 54, 1645–1651. [Google Scholar] [CrossRef]
- Kalpana, R.; Bhuvaneswari, G.; Singh, B.; Singh, S. Harmonic mitigator based on 12-pulse ac-dc converter for switched mode power supply. IET Power Electron. 2010, 3, 947–964. [Google Scholar] [CrossRef]
- Sun, W.; Yang, L.; Zare, F.; Xia, Y.W.; Cheng, L.; Zhou, K. 3D modeling of an HVDC converter transformer and its application on the electrical field of windings subject to voltage harmonics. Int. J. Electr. Power Energy Syst. 2020, 117, 105581. [Google Scholar] [CrossRef]
- Liu, X.; Yang, Y.; Huang, Y.; Jadoon, A. Vibration characteristic investigation on distribution transformer influenced by DC magnetic bias based on motion transmission model. Int. J. Electr. Power Energy Syst. 2018, 98, 389–398. [Google Scholar] [CrossRef]
- Pan, C.; Yi, S.; Su, H.; Shi, W. Excitation-vibration harmonic response research of transformer in dc biasing operation. IET Electr. Power Appl. 2019, 13, 410–417. [Google Scholar] [CrossRef]
- Yadav, S.; Mehta, R. Modelling of magnetostrictive vibration and acoustics in converter transformer. IET Electr. Power Appl. 2021, 15, 332–347. [Google Scholar] [CrossRef]
- Jiang, P.; Zhang, Z.; Dong, Z.; Wu, Y.; Xiao, R.; Deng, J.; Pan, Z. Research on distribution characteristics of vibration signals of ±500 kV HVDC converter transformer winding based on load test. Int. J. Electr. Power Energy Syst. 2021, 132, 107200. [Google Scholar] [CrossRef]
- Hu, Y.; Zheng, J.; Huang, H. Experimental research on power transformer vibration distribution under different winding defect conditions. Electronics 2019, 8, 842. [Google Scholar] [CrossRef]
- Shao, P.; Luo, L.; Li, Y.; Rehtanz, C. Electromagnetic vibration analysis of the winding of a new HVDC converter transformer. IEEE Trans. Power Deliv. 2012, 27, 123–130. [Google Scholar] [CrossRef]
- Simões, A.N.; Carvalho, D.J.; Morita, E.d.S.; Moretti, H.L.; Vendrameto, H.V.; Fu, L.; Torres, F.; Souza, A.N.d.; Bizzo, W.A.; Mazon, T. A Triboelectric Nanogenerator for Energy Harvesting from Transformers’ Vibrations. Machines 2022, 10, 215. [Google Scholar] [CrossRef]
- Cui, H. Faults diagnosis of converter transformer based on the vibration method. Adv. Manuf. Sci. Eng. 2013, 712, 2101–2106. [Google Scholar] [CrossRef]
- Smajic, J.; Hughes, J.; Steinmetz, T.; Pusch, D.; Mönig, W.; Carlen, M. Numerical computation of ohmic and eddy-current winding losses of converter transformers including higher harmonics of load current. IEEE Trans. Magn. 2012, 48, 827–830. [Google Scholar] [CrossRef]
- Pang, B.; Liang, J.X.; Liu, H. Intelligent Bearing Fault Diagnosis Based on Multivariate Symmetrized Dot Pattern and LEG Transformer. Machines 2022, 10, 550. [Google Scholar] [CrossRef]
- Radja, N.; Rachek, M.; Larbi, S.N. Non-destructive testing for winding insulation diagnosis using inter-turn transient voltage signature analysis. Machines 2018, 6, 21. [Google Scholar] [CrossRef]
- Chen, H.; Fan, M.; Su, W.; Guo, Y.; Huang, Y. Effects of particle erosion on the submersible pump in an ultra-high voltage transformer. Energy Rep. 2021, 7, 7072–7081. [Google Scholar] [CrossRef]
- García, B.; Burgos, J.C.; Alonso, Á. Transformer tank vibration modeling as a method of detecting winding deformations—Part II: Experimental verification. IEEE Trans. Power Deliv. 2006, 21, 164–169. [Google Scholar] [CrossRef]
- Ji, S.; Luo, Y.; Li, Y. Research on extraction technique of transformer core fundamental frequency vibration based on OLCM. IEEE Trans. Power Deliv. 2006, 21, 1981–1988. [Google Scholar]




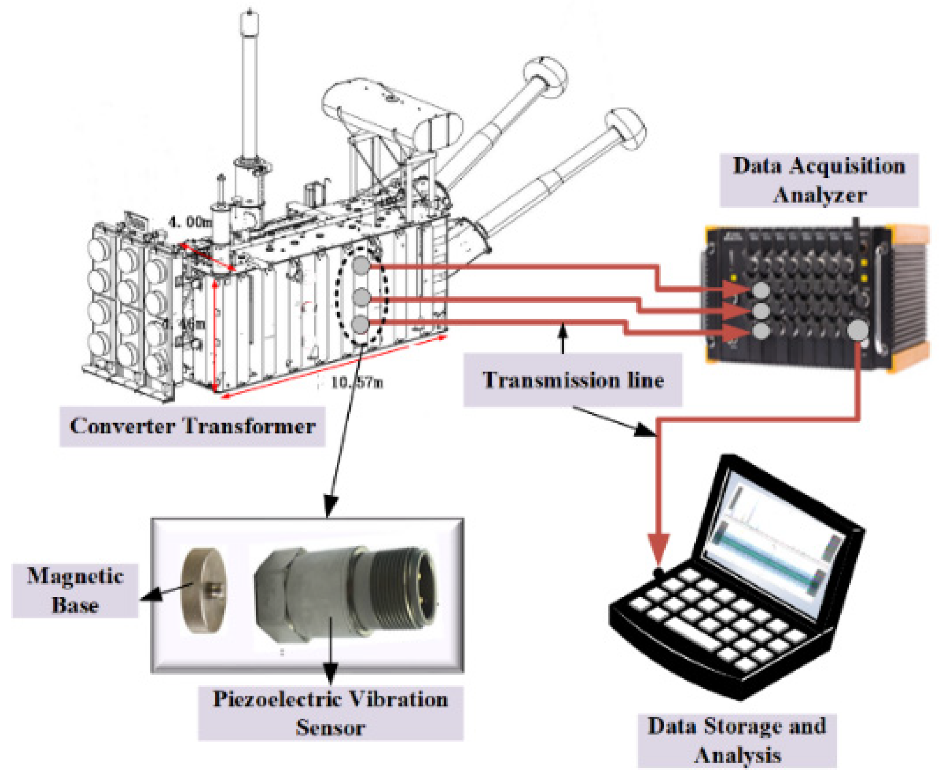
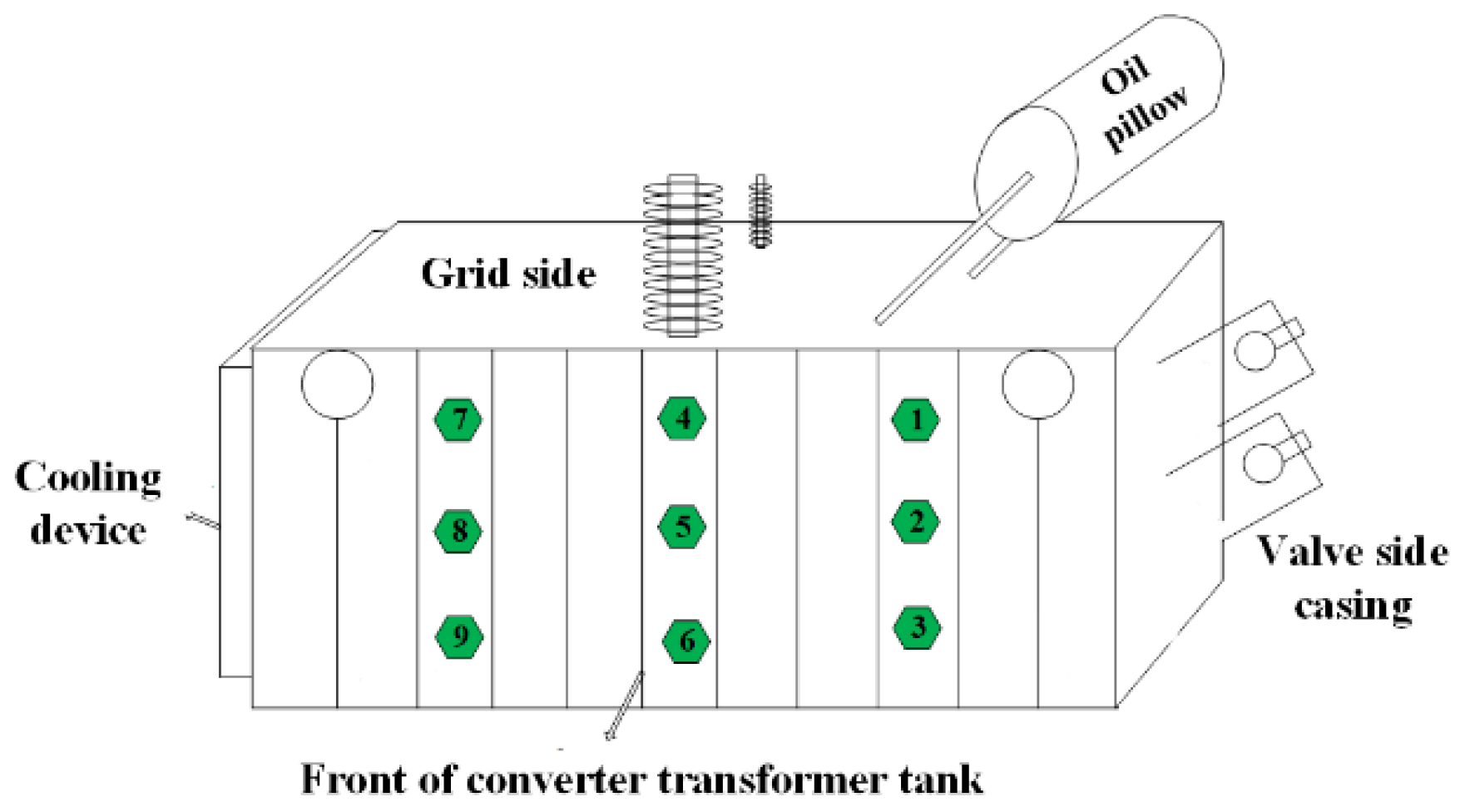
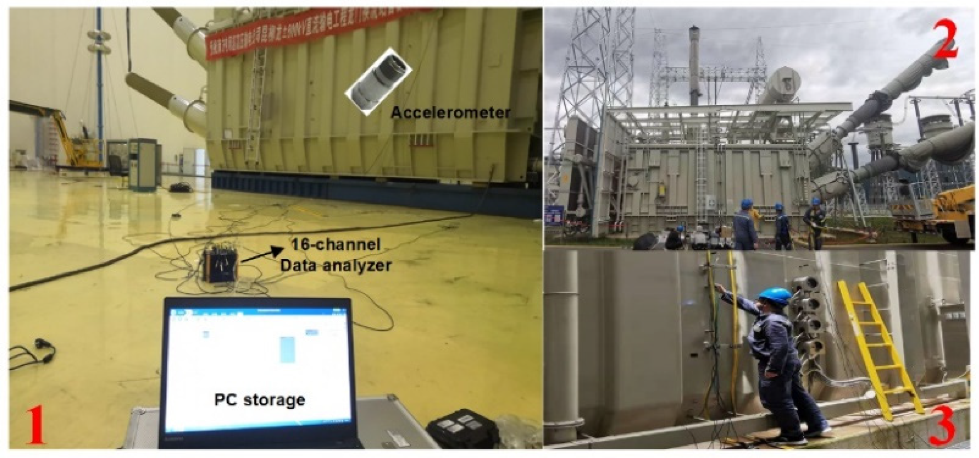



| Parameter | Value |
|---|---|
| Rated capacity (MVA) | 248.6 |
| Voltage (grid side/valve side) (kV) | |
| Rated frequency (Hz) | 50 |
| Odd | Valid Value (kV) | Content (%) | Even | Valid Value (kV) | Content (%) |
|---|---|---|---|---|---|
| 1 | 83.80 | - | 6 | 6.82 | 8.13 |
| 3 | 28.35 | 33.83 | 12 | 3.33 | 3.98 |
| 5 | 12.04 | 14.36 | 18 | 5.28 | 6.29 |
| 7 | 10.32 | 12.32 | 24 | 3.73 | 4.45 |
| 27 | 13.52 | 16.14 | 30 | 13.17 | 15.71 |
| Contents | Grid Side (%) | Valve Side (%) | |
|---|---|---|---|
| Y-Type | D-Type | ||
| Fundamental | 753A | 2411A | 1399A |
| 5 | 17.19 | 17.12 | 17.07 |
| 7 | 10.47 | 10.40 | 10.40 |
| 11 | 3.55 | 3.50 | 3.49 |
| 13 | 2.41 | 2.39 | 2.40 |
| With Harmonics | Without Harmonics | |||||||
|---|---|---|---|---|---|---|---|---|
| k | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 1 |
| 1.02 | 0.17 | 0.27 | 0.15 | 0.03 | 0.02 | 0.01 | 1 | |
| 4.07 | 2.72 | 9.72 | 9.60 | 2.89 | 2.45 | 1.96 | 4 | |
| Parameter | Range |
|---|---|
| ) | 100 |
| ) | ±50 |
| ) | 1~7000 |
| ) | −40~120 |
| ) | 2~10 |
| ) | 50,000 |
| Working Condition | Dominant Frequency (Hz) |
|---|---|
| Winding vibration without harmonics | 100 |
| Iron core vibration without harmonics | 100 |
| Theoretical tank vibration without harmonics | 100 |
| Tank vibration with harmonics | 400 |
| Dominant Frequency (Hz) | 100 | 300 | 400 | 800 | 1200 |
|---|---|---|---|---|---|
| Number of measuring points | 5 | 7 | 94 | 1 | 1 |
| Percentage (%) | 4.6 | 6.5 | 87.0 | 0.9 | 0.9 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jiang, P.; Zhang, Z.; Dong, Z.; Yang, Y. Vibration Measurement and Numerical Modeling Analysis of Transformer Windings and Iron Cores Based on Voltage and Current Harmonics. Machines 2022, 10, 786. https://doi.org/10.3390/machines10090786
Jiang P, Zhang Z, Dong Z, Yang Y. Vibration Measurement and Numerical Modeling Analysis of Transformer Windings and Iron Cores Based on Voltage and Current Harmonics. Machines. 2022; 10(9):786. https://doi.org/10.3390/machines10090786
Chicago/Turabian StyleJiang, Peiyu, Zhanlong Zhang, Zijian Dong, and Yu Yang. 2022. "Vibration Measurement and Numerical Modeling Analysis of Transformer Windings and Iron Cores Based on Voltage and Current Harmonics" Machines 10, no. 9: 786. https://doi.org/10.3390/machines10090786
APA StyleJiang, P., Zhang, Z., Dong, Z., & Yang, Y. (2022). Vibration Measurement and Numerical Modeling Analysis of Transformer Windings and Iron Cores Based on Voltage and Current Harmonics. Machines, 10(9), 786. https://doi.org/10.3390/machines10090786








