Conjugate Heat Transfer Simulation of Overall Cooling Performance for Cratered Film Cooling Holes
Abstract
:1. Introduction
2. Physical Model and Numerical Method
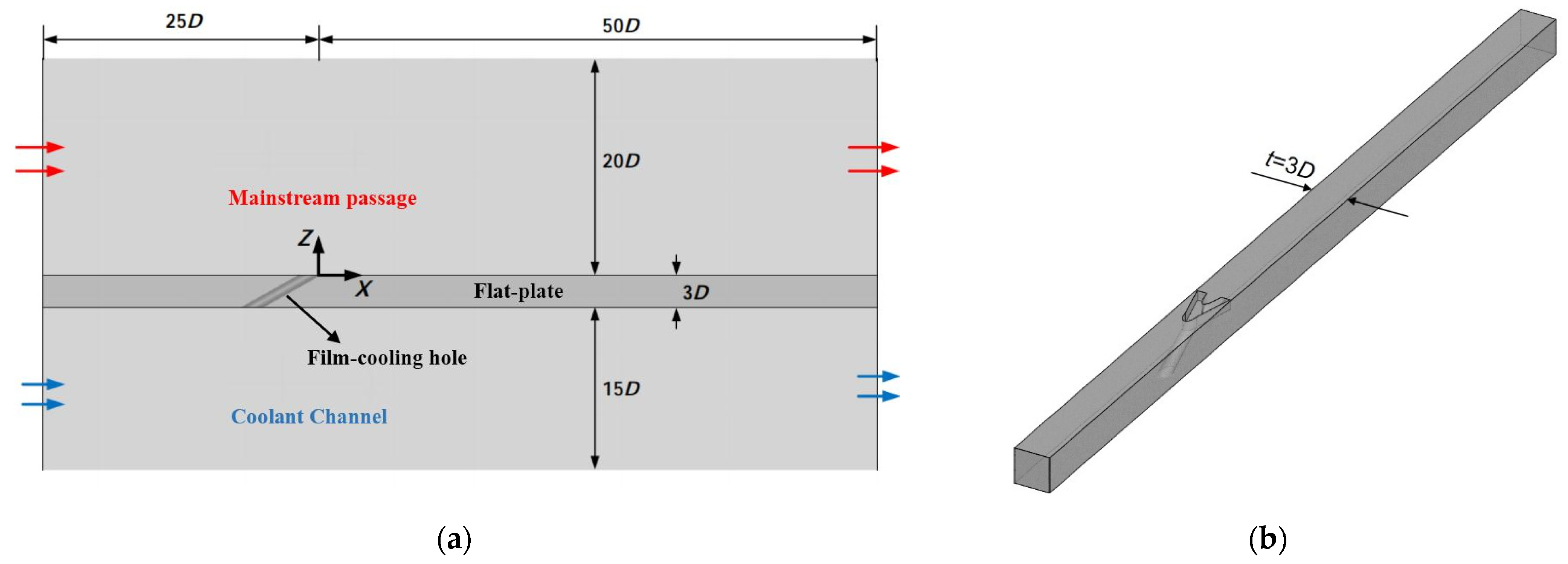
2.1. Physical Model
2.2. Boundary Condition and Data Reduction
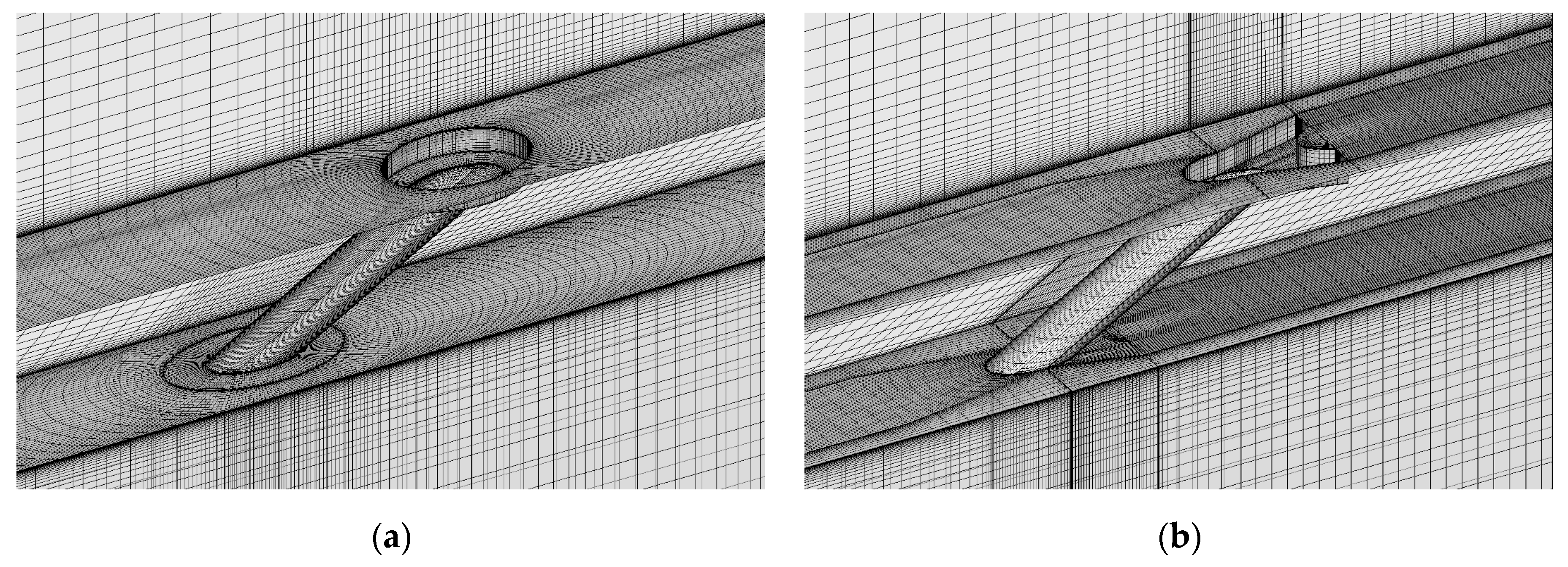
2.3. Numerical Method Validation
3. Results and Discussions
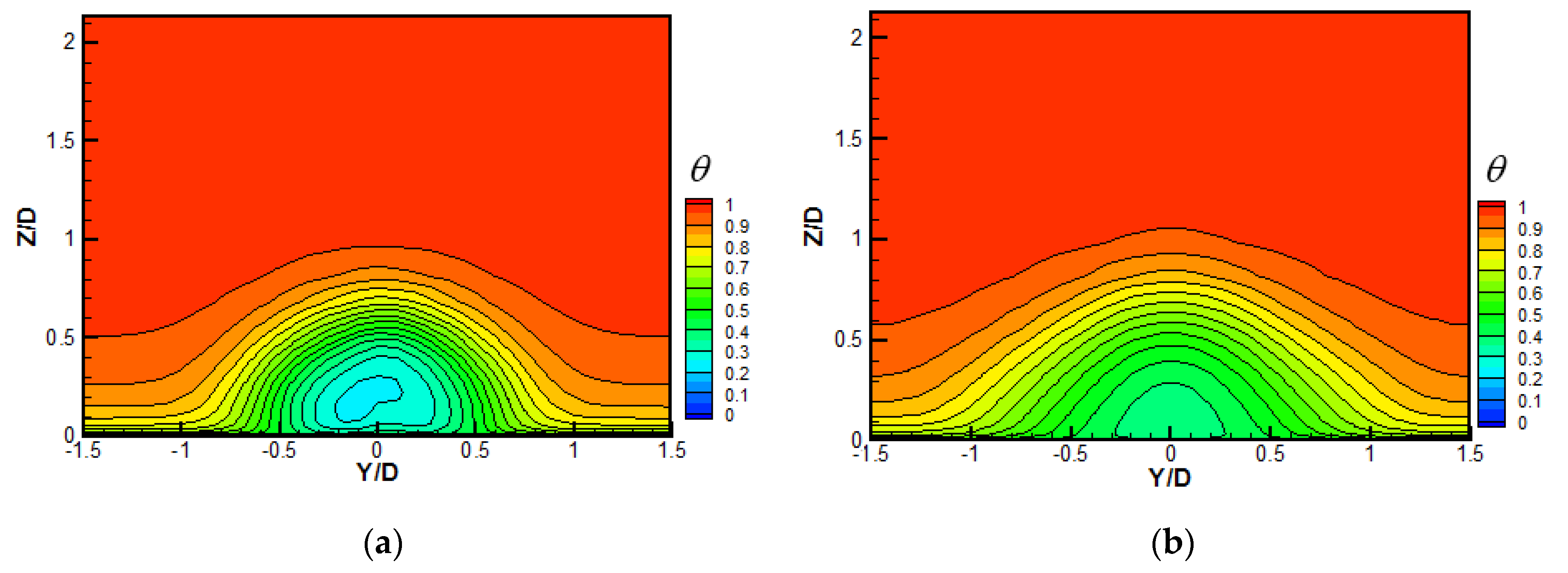
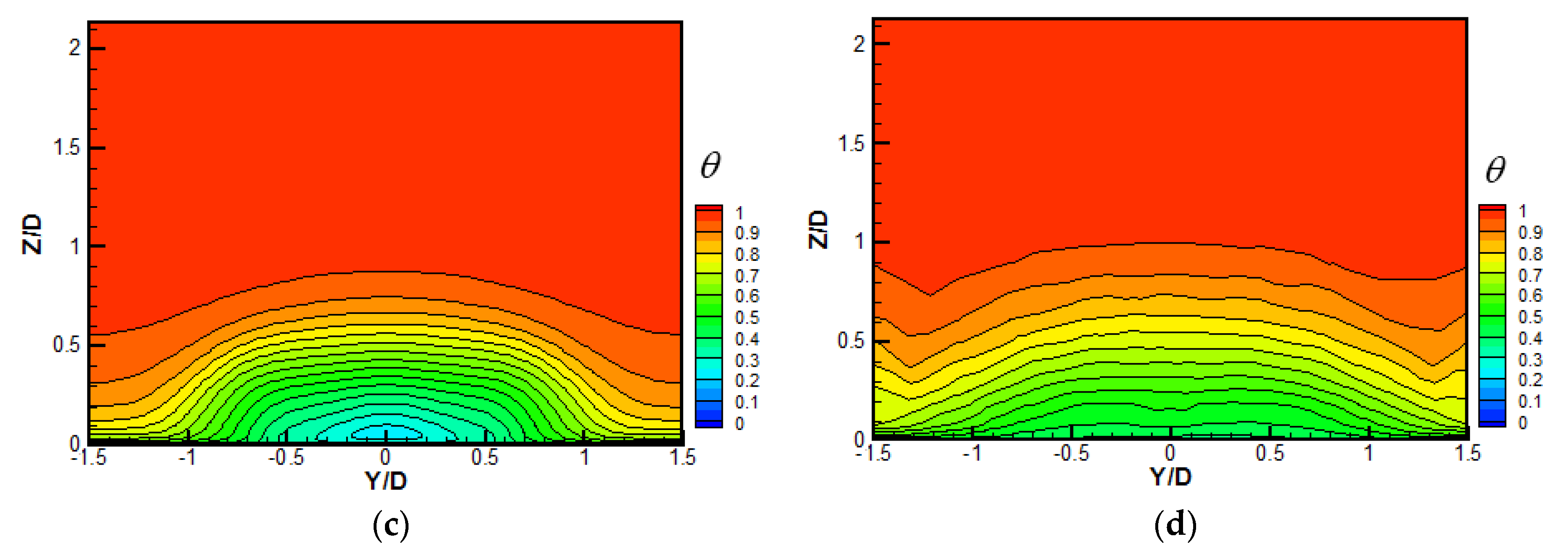
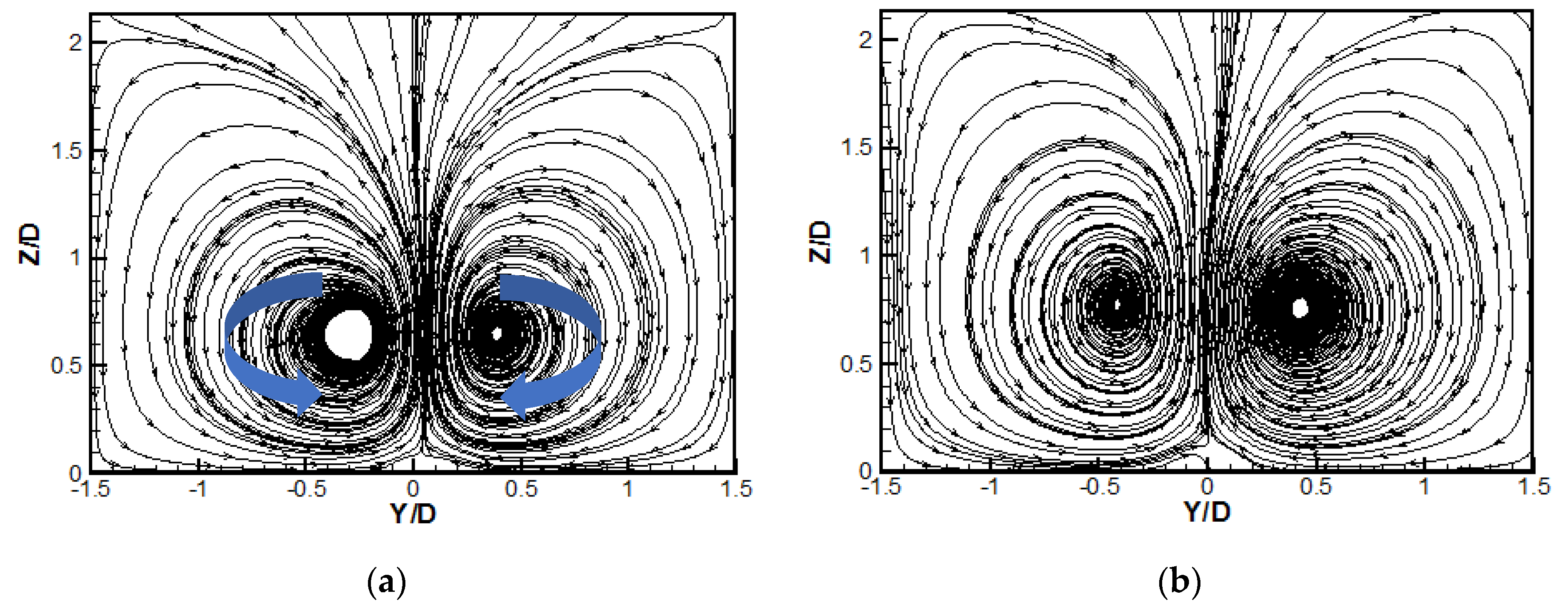
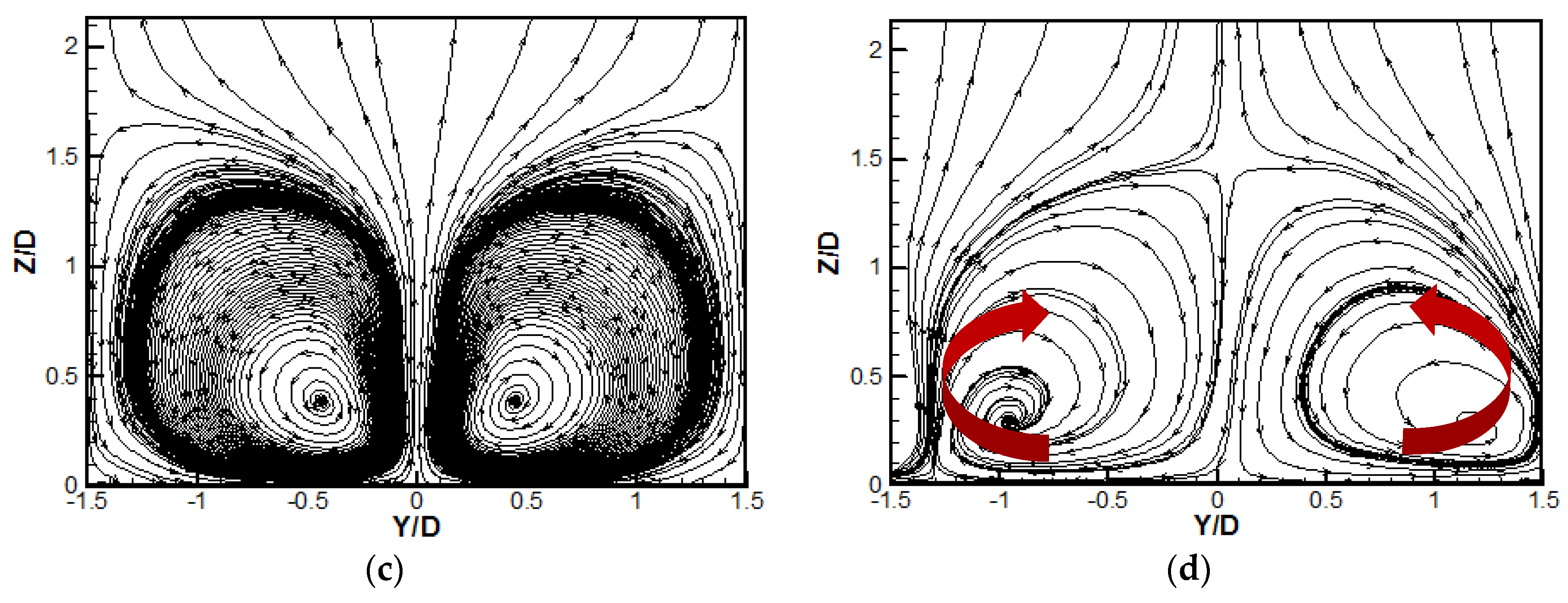
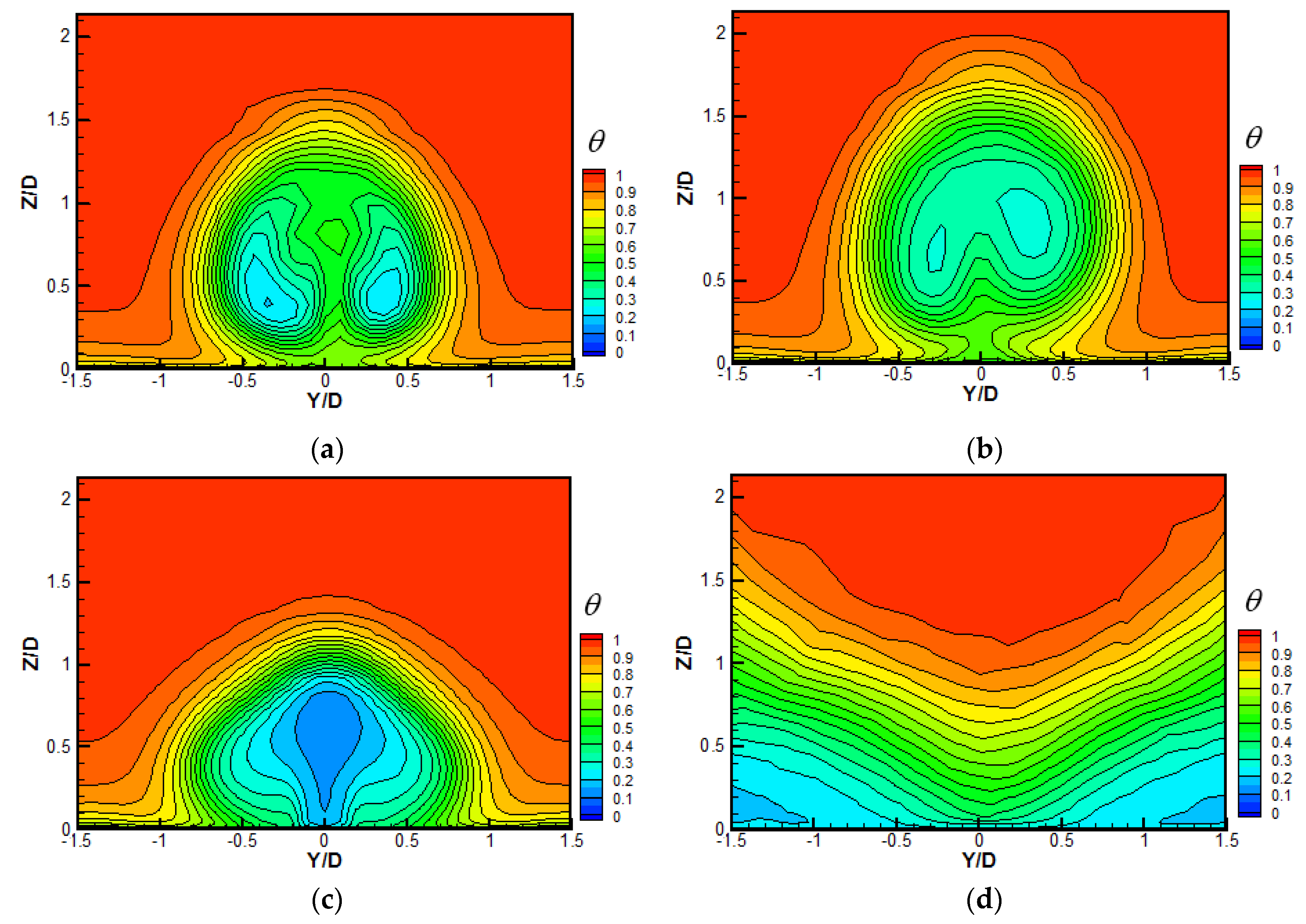
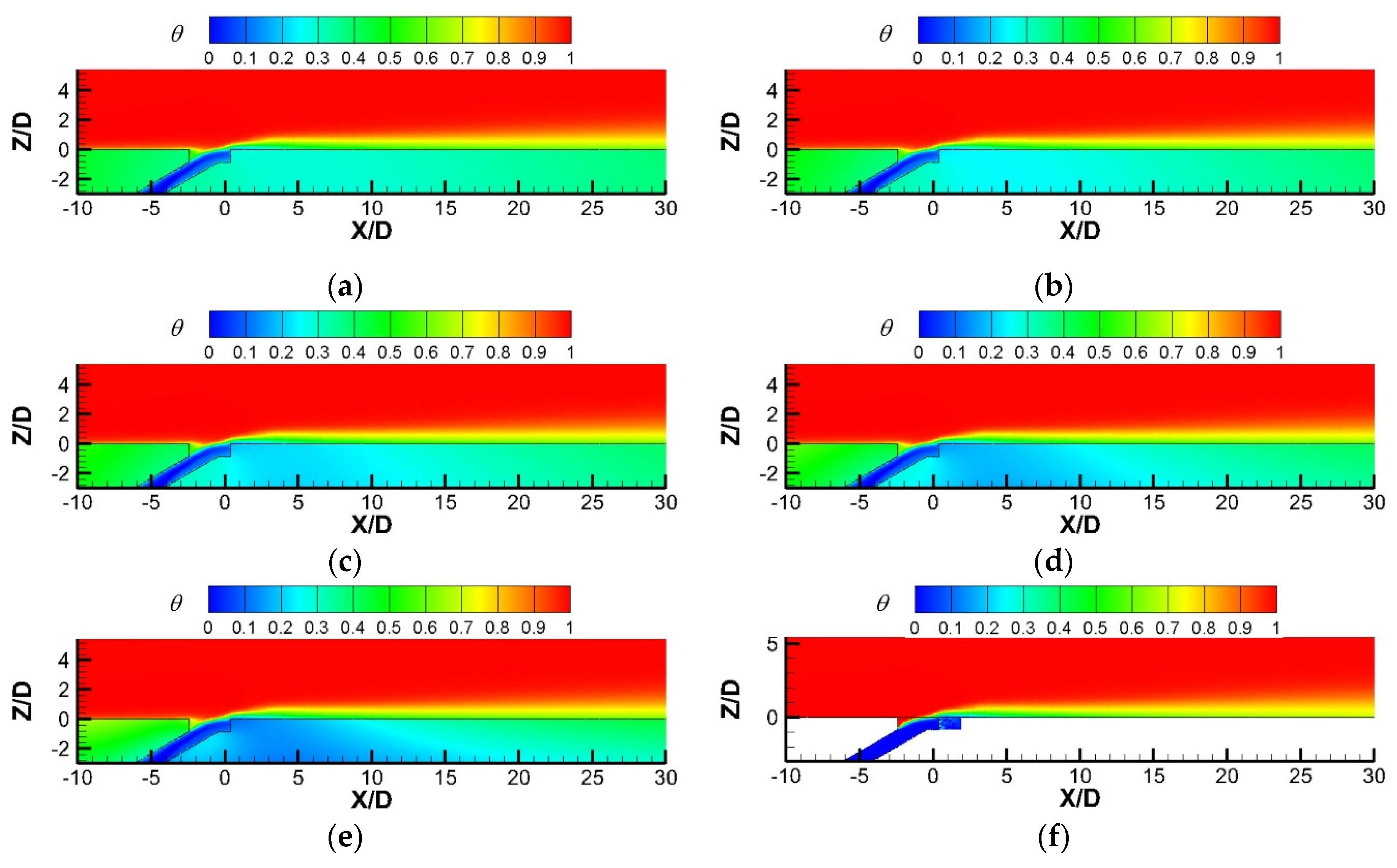
3.1. Flow Structure and Temperature Field
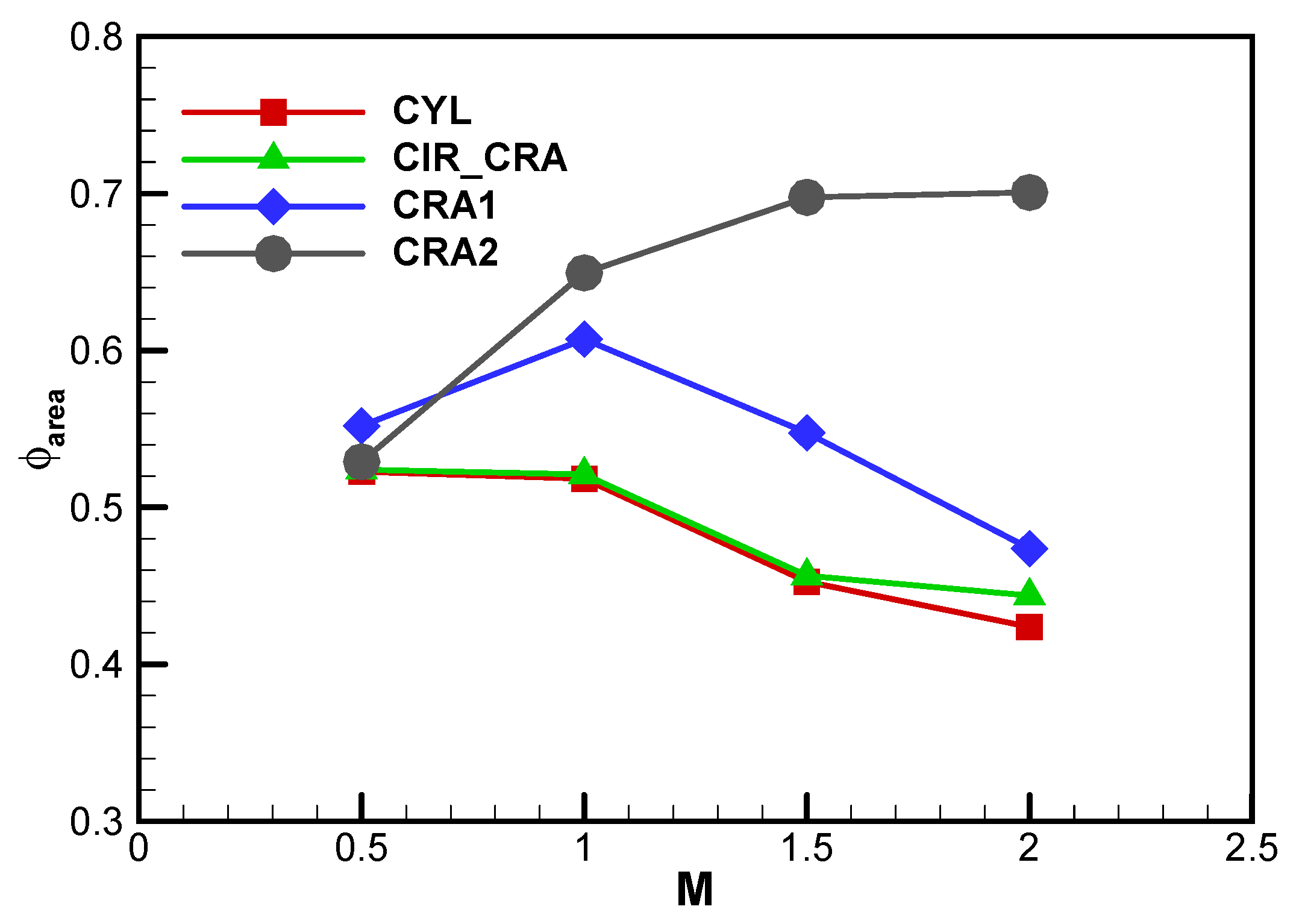
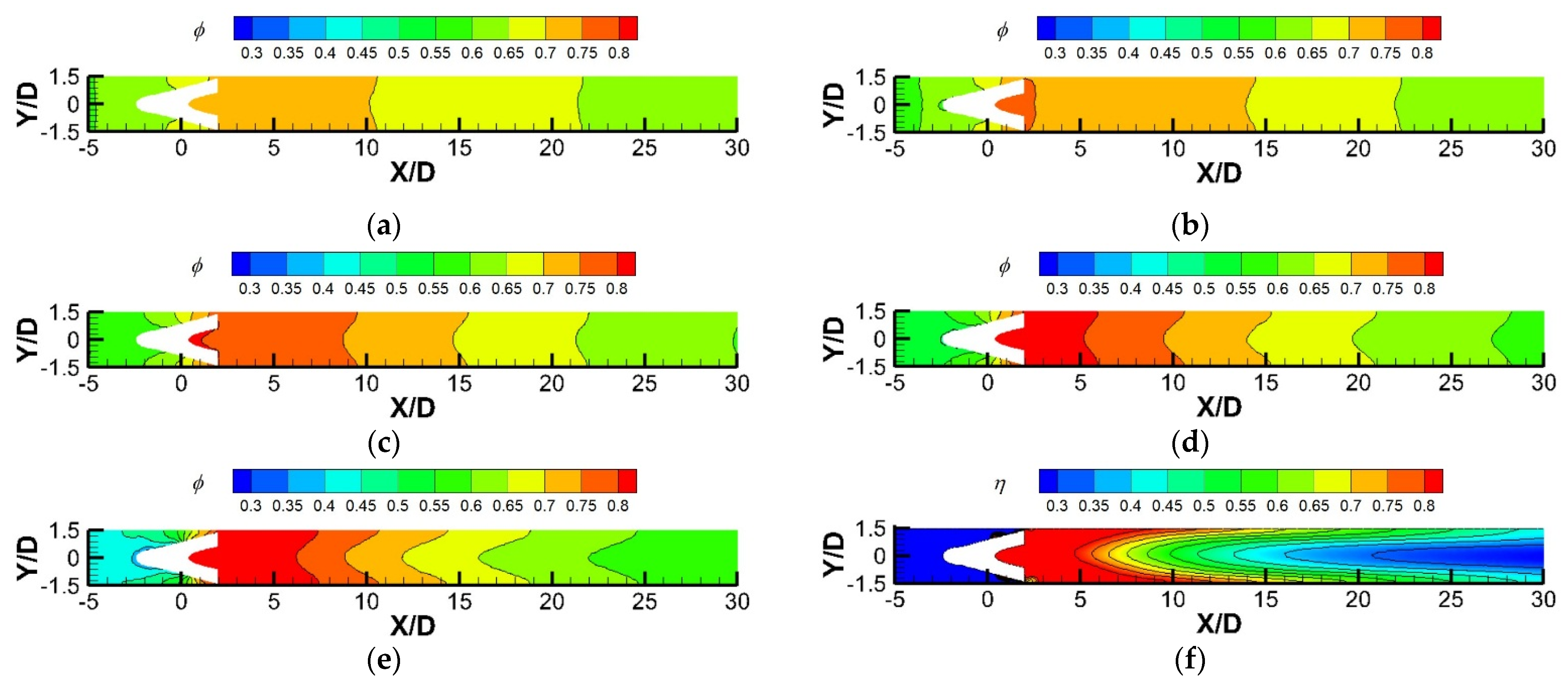
3.2. Overall Cooling Effectiveness
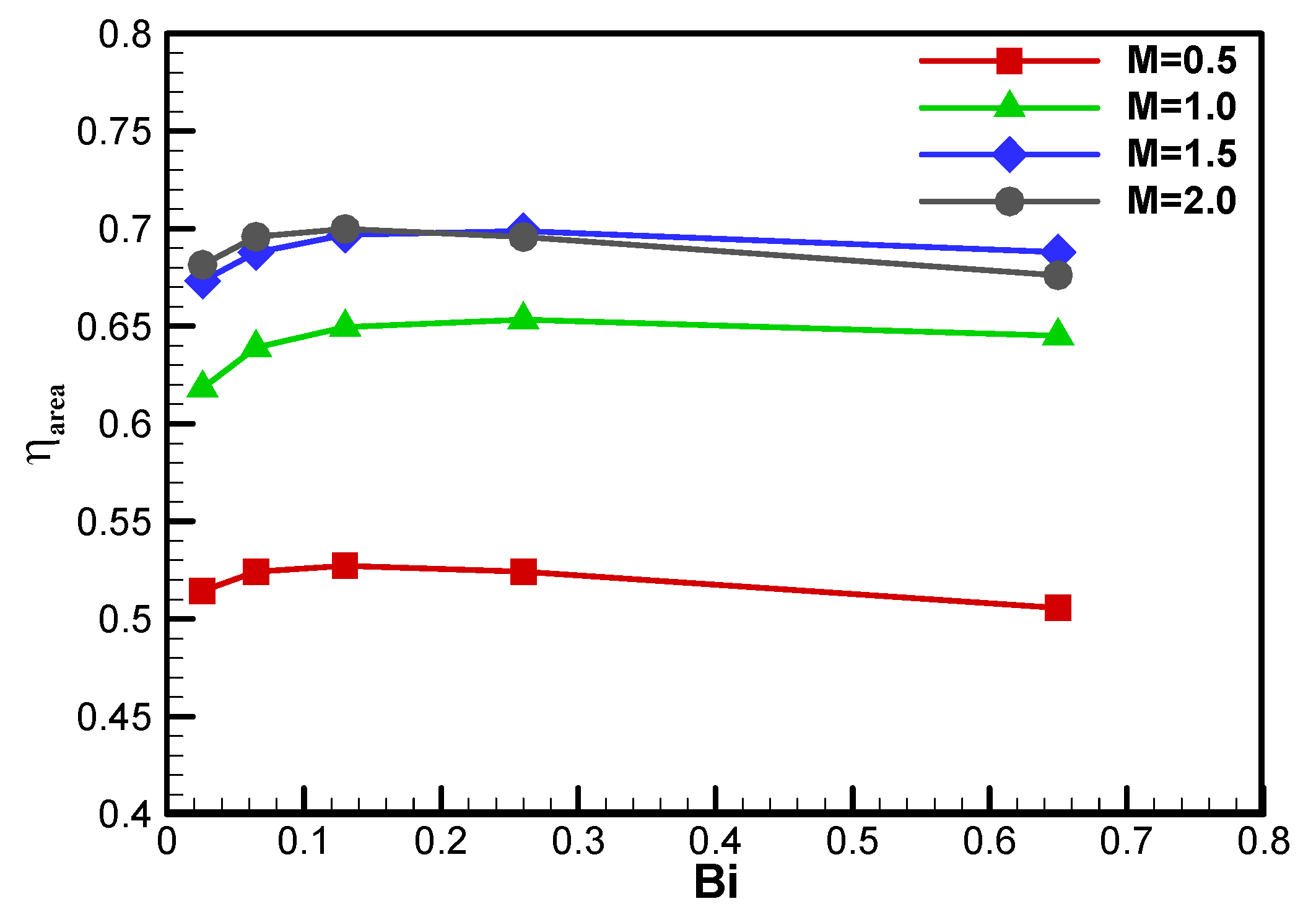
3.3. The Effect of Biot Number
4. Conclusions
- Compared with the cylindrical hole (CYL), the additional anti-kidney-shaped vortex pair for the cratered hole improves the coolant coverage and the overall cooling effectiveness over the wall surface. The overall cooling effectiveness improvement amplitude depends on the crater shape, geometry parameters, and the specific blowing ratio. The concentric circular cratered hole (CIR_CRA) gives a comparative overall cooling performance to the cylindrical hole at M = 0.5–1.5, but an improvement of 4.68% at M = 2.0. Comparatively, the contoured cratered holes provide large enhancements. The maximum area-averaged overall cooling effectiveness increases are 5.58% for the CRA1 hole at M = 0.5 and 25.28–65.30% for the CRA2 hole at M = 1.0–2.0, respectively.
- For the CRA2 hole model, the variation of Biot number influences little on the flow structure but significantly on the local overall cooling effectiveness distribution and temperature gradient in the solid domain. Furthermore, the area-averaged overall cooling effectiveness is insensitive to the Biot number, but the area-averaged overall cooling effectiveness uniformity coefficient strongly depends on the specific value of the Biot number. As the Biot number increases, the temperature gradient in the solid near the hole and the cooling uniformity both increase, raising a possible high thermal stress.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Goldstein, R.J. Film Cooling. Adv. Heat Transf. 1971, 7, 321–379. [Google Scholar]
- Sinha, A.K.; Bogard, D.G.; Crawford, M.E. Film-cooling effectiveness downstream of a single row of holes with variable density ratio. J. Turbomach. 1991, 113, 442–449. [Google Scholar] [CrossRef]
- Goldstein, R.J.; Jin, P.; Olson, R.L. Film cooling effectiveness and mass/heat transfer coefficient downstream of one row of discrete holes. J. Turbomach. 1999, 121, 225–232. [Google Scholar] [CrossRef]
- Baldauf, S.M.; Schulz, A.; Wittig, S. High-resolution measurements of local effectiveness from discrete hole film cooling. J. Turbomach. 2001, 123, 758–765. [Google Scholar] [CrossRef]
- Goldstein, R.J.; Eckert, E.; Burggraf, F. Effects of hole geometry and density on three-dimensional film cooling. Int. J. Heat Mass Transf. 1974, 17, 596–607. [Google Scholar] [CrossRef]
- Gritsch, M.; Colban, W.; Schär, H.; Döbbeling, K. Effect of hole geometry on the thermal performance of fan-shaped film cooling holes. J. Turbomach. 2005, 127, 718–725. [Google Scholar] [CrossRef]
- Lee, K.D.; Kim, K.Y. Shape optimization of a fan-shaped hole to enhance film-cooling effectiveness. Int. J. Heat Mass Transf. 2010, 53, 2996–3005. [Google Scholar] [CrossRef]
- Krishna, A.V.G.; Parammasivam, K.M. Thermal barrier coated surface modifications for gas turbine film cooling: A review. J. Therm. Anal. Calorim. 2021, 146, 545–580. [Google Scholar] [CrossRef]
- Tran, N.; Nguyen, S.; Ho, S.; Kapat, J. Prediction of adiabatic effectiveness of various cratered film hole configurations: Sensitivity analysis for the rectangle shaped mask. In Proceedings of the 48th AIAA Aerospace Sciences Meeting Including the New Horizons Forum and Aerospace Exposition, Orlando, FL, USA, 4–7 January 2010. [Google Scholar]
- Lu, Y.P.; Dhungel, A.; Ekkad, S.; Bunker, R. Film cooling measurements for cratered cylindrical inclined holes. J. Turbomach. 2009, 131, 011005. [Google Scholar] [CrossRef]
- Khalatov, A.A.; Panchenko, N.A.; Severi, S.D. Application of cylindrical, triangular and hemispherical dimples in the film cooling technology. J. Phys. Conf. Ser. 2017, 891, 012145. [Google Scholar] [CrossRef]
- Khalatov, A.A.; Shi-Ju, E.; Wang, D.Y.; Borisov, I. Film cooling evaluation of a single array of triangular craters. Int. J. Heat Mass Transf. 2020, 159, 120055. [Google Scholar] [CrossRef]
- Kalghatgi, P.; Acharya, S. Improved film cooling effectiveness with a round film cooling hole embedded in a contoured crater. J. Turbomach. 2015, 137, 101006. [Google Scholar] [CrossRef]
- Kalghatgi, P.; Acharya, S. Flow dynamics of a film cooling jet issued from a round hole embedded in contoured crater. J. Turbomach. 2019, 141, 081006. [Google Scholar] [CrossRef]
- An, B.T.; Liu, J.J.; Zhang, X.D.; Zhou, S.J.; Zhang, C. Film cooling effectiveness measurements of a near surface streamwise diffusion hole. Int. J. Heat Mass Transf. 2016, 103, 1–13. [Google Scholar] [CrossRef]
- Fu, J.L.; Bai, L.C.; Zhang, C.; Ju, P.F. Film cooling performance for cylindrical holes embedded in contoured craters: Effect of the crater depth. J. Appl. Mech. Tech. Phys. 2019, 60, 1068–1076. [Google Scholar] [CrossRef]
- Bai, L.C.; Zhang, C.; Tong, Z.T.; Ju, P.F. Optimization of geometric parameters of cylindrical film cooling hole with contoured craters to enhance film-cooling effectiveness. Thermophys. Aeromech. 2021, 28, 835–848. [Google Scholar] [CrossRef]
- Bohn, D.; Ren, J.; Kusterer, K. Conjugate heat transfer analysis for film cooling configurations with different hole geometries. In Proceedings of the ASME Turbo Expo 2003, collocated with the 2003 International Joint Power Generation Conference, Atlanta, GA, USA, 16–19 June 2003. [Google Scholar]
- Slilieti, M.; Kassab, A.; Divo, E. Film cooling effectiveness: Comparison of adiabatic and conjugate heat transfer CFD models. Int. J. Therm. Sci. 2009, 48, 2237–2248. [Google Scholar] [CrossRef]
- Ghorab, M.G. Adiabatic and conjugate cooling effectiveness of a new hybrid scheme. Int. J. Therm. Sci. 2011, 50, 965–983. [Google Scholar] [CrossRef]
- Albert, J.E.; Bogard, D.G. Measurements of adiabatic film and overall cooling effectiveness on a turbine vane pressure side with a trench. J. Turbomach. 2013, 135, 051007. [Google Scholar] [CrossRef]
- Kusterer, K.; Hagedorn, T.; Bohn, D.; Sugimoto, T.; Tanaka, R. Improvement of a film-cooled blade by application of the conjugate calculation technique. J. Turbomach. 2006, 128, 572–578. [Google Scholar] [CrossRef]
- Alizadeh, M.; Izadi, A.; Fathi, A. Sensitivity analysis on turbine blade temperature distribution using conjugate heat transfer simulation. J. Turbomach. 2014, 136, 011001. [Google Scholar] [CrossRef]
- Wang, Z.; Zhang, C.; Du, W.J.; Li, S.J. Multi-field coupling analysis on the film-cooling with transverse and arched trenches. J. Therm. Sci. Tech. 2019, 14, JTST0012. [Google Scholar] [CrossRef] [Green Version]
- Fu, J.L.; Cao, Y.; Zhang, C.; Zhu, J.Q. Investigation of the conjugate heat transfer and flow field for a flat plate with combined film and impingement cooling. J. Therm. Sci. 2020, 29, 955–971. [Google Scholar] [CrossRef]
- Bryant, C.E.; Rutledge, J.L. A computational technique to evaluate the relative influence of internal and external cooling on overall effectiveness. J. Turbomach. 2020, 142, 051008. [Google Scholar] [CrossRef]
- Zhang, C.; Liu, J.J.; Wang, Z.; An, B.T. The effects of biot number on the conjugate film Cooling effectiveness under different blowing ratios. In Proceedings of the ASME Turbo Expo 2013: Turbine Technical Conference and Exposition, San Antonio, TX, USA, 3–7 June 2013. [Google Scholar]
- Liu, X.B.; Zhang, C.; Song, L.M.; Li, J. Influence of biot number and geometric parameters on the overall cooling effectiveness of double wall structure with pins. Appl. Therm. Eng. 2021, 198, 117439. [Google Scholar] [CrossRef]
- Zhang, P. Investigation of Film Cooling and Combined Cooling Using Conjugate Heat Transfer Method. Master’s Thesis, University of Chinese Academy of Sciences, Beijing, China, 2015. [Google Scholar]
- Bai, L.C. Research on Optimization Design and Thermal Coupling Analysis of the Cratered Hole. Master’s Thesis, Tianjin University of Technology, Tianjin, China, 2021. [Google Scholar]
- Fric, T.F.; Roshko, A. Vortical structure in the wake of a transverse jet. J. Fluid Mech. 1994, 277, 1–47. [Google Scholar] [CrossRef] [Green Version]
- Javadi, K. Introducing film cooling uniformity coefficient. Heat Transf. Eng. 2018, 39, 180–193. [Google Scholar] [CrossRef]







Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, C.; Wang, W.; Wang, Z.; Tong, Z. Conjugate Heat Transfer Simulation of Overall Cooling Performance for Cratered Film Cooling Holes. Machines 2022, 10, 395. https://doi.org/10.3390/machines10050395
Zhang C, Wang W, Wang Z, Tong Z. Conjugate Heat Transfer Simulation of Overall Cooling Performance for Cratered Film Cooling Holes. Machines. 2022; 10(5):395. https://doi.org/10.3390/machines10050395
Chicago/Turabian StyleZhang, Chao, Wenzhuang Wang, Zhan Wang, and Zhiting Tong. 2022. "Conjugate Heat Transfer Simulation of Overall Cooling Performance for Cratered Film Cooling Holes" Machines 10, no. 5: 395. https://doi.org/10.3390/machines10050395
APA StyleZhang, C., Wang, W., Wang, Z., & Tong, Z. (2022). Conjugate Heat Transfer Simulation of Overall Cooling Performance for Cratered Film Cooling Holes. Machines, 10(5), 395. https://doi.org/10.3390/machines10050395





