Study of the Self-Locking Characteristics of the Swing Scraper of an Elliptical Rotor Scraper Pump
Abstract
:1. Introduction
1.1. Research Motivation
1.2. Literature Review
1.3. Scientific Contribution of the Paper
- -
- This paper proposes an elliptical rotor scraper pump (ERSP) and establishes the 3-D model and mathematical model of the ERSP.
- -
- In order to provide a set of basic criteria for the normal operation of ERSP, this paper analyzes the self-locking characteristics of ERSP.
- -
- We calculate the minimum length of the swing scraper according to the self-locking characteristics, and the volume utilization ratio of ERSP is improved.
- -
- Our research group developed a prototype that verifies the rationality of ERSP and the correctness of related research.
1.4. Organization of the Paper
2. Structure and Working Principle
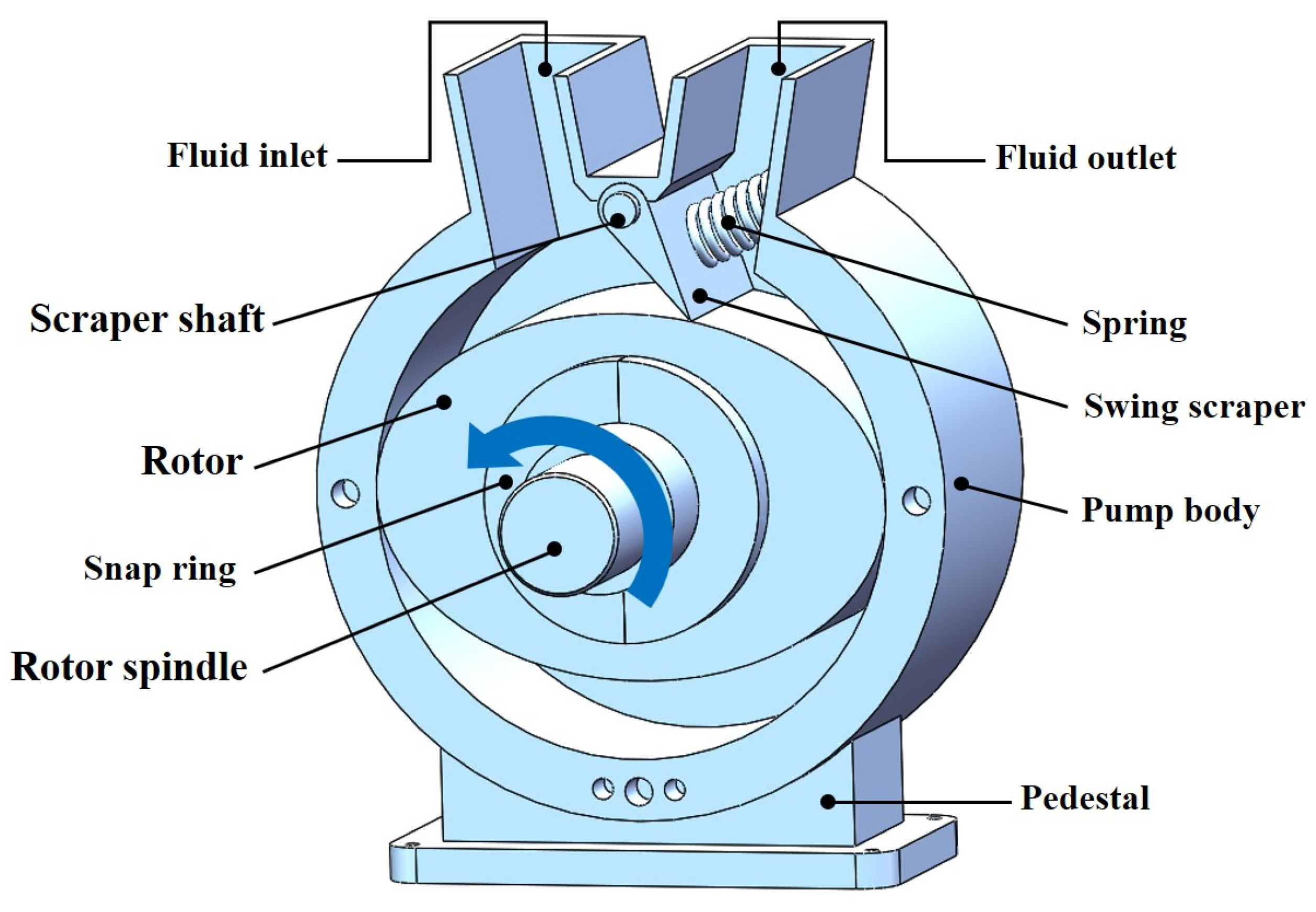
2.1. 3-D Model of the ERSP
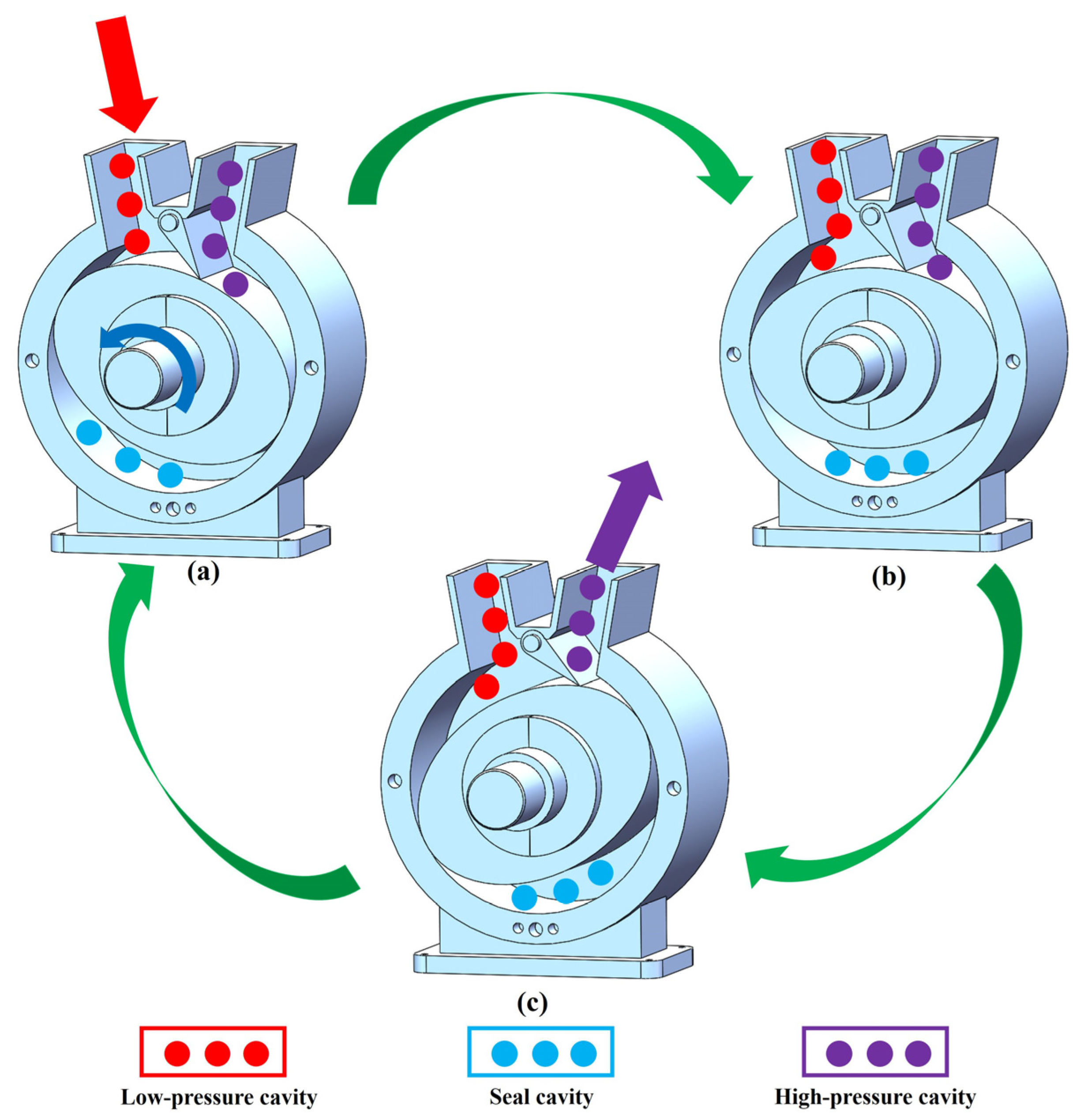
2.2. Working Principle of the ERSP
3. Mathematical Model and Kinematic Equation
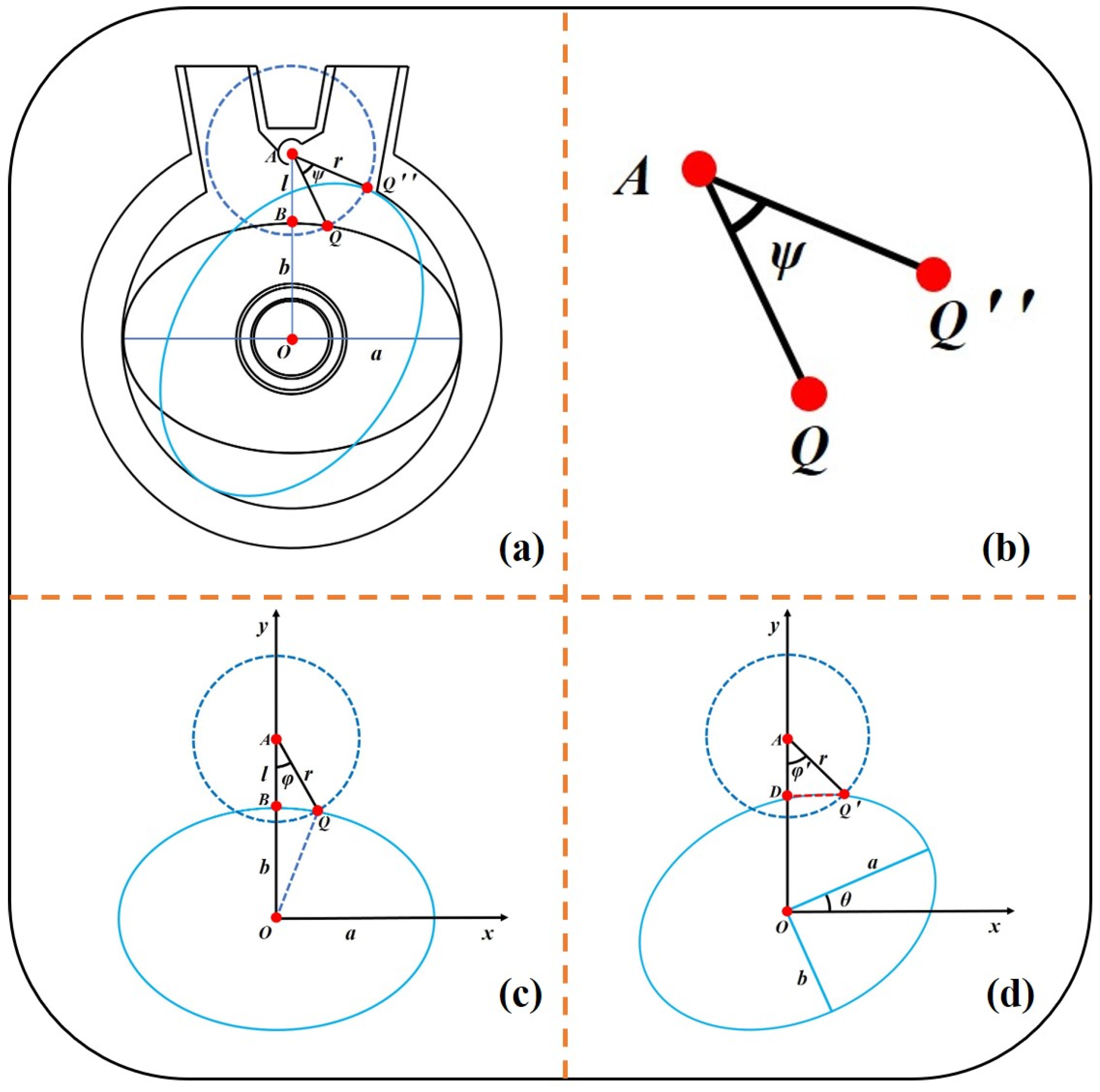
3.1. Establishment of Mathematical Model
3.2. Establishment of Scraper Kinematic Equation
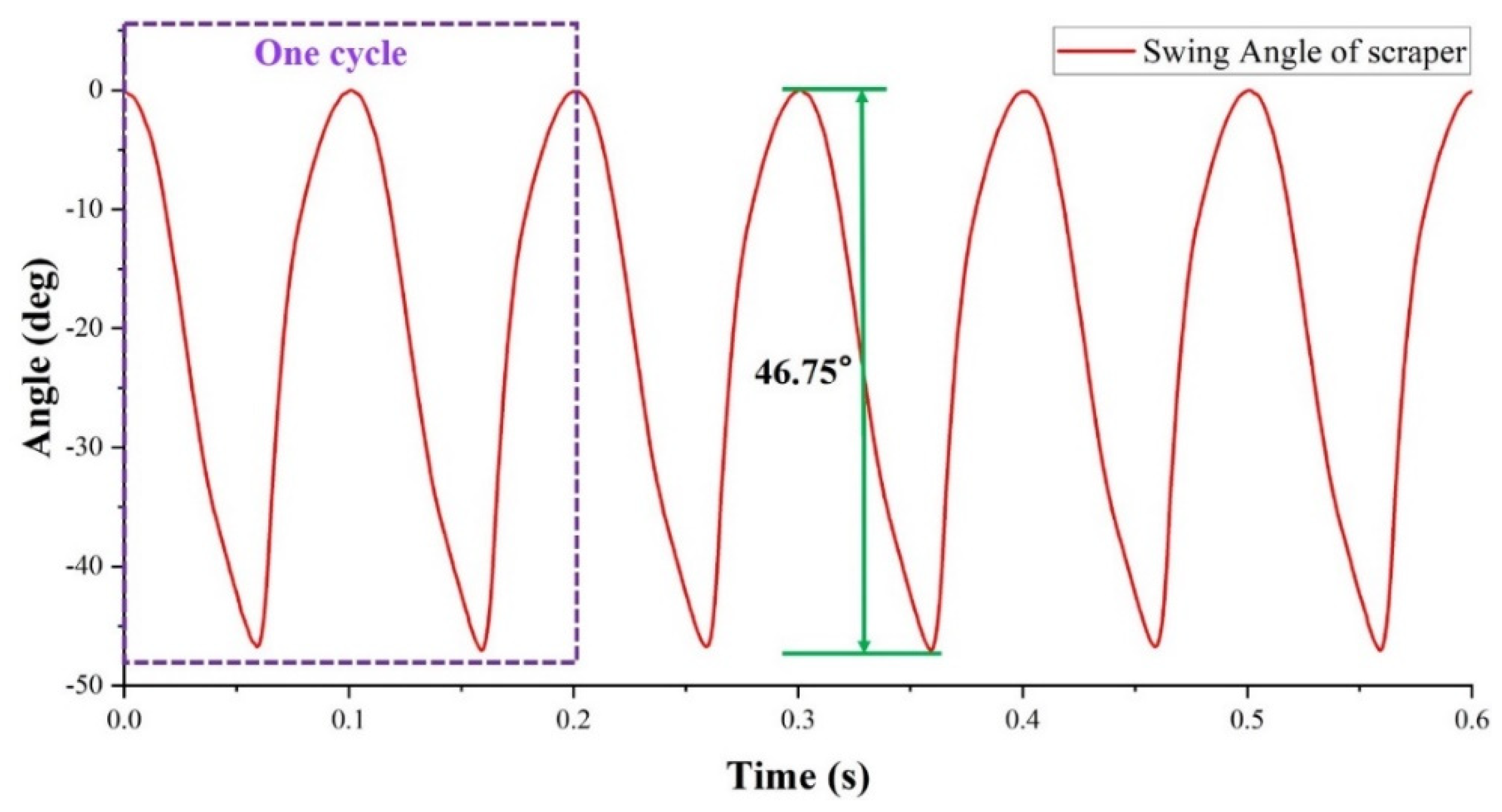
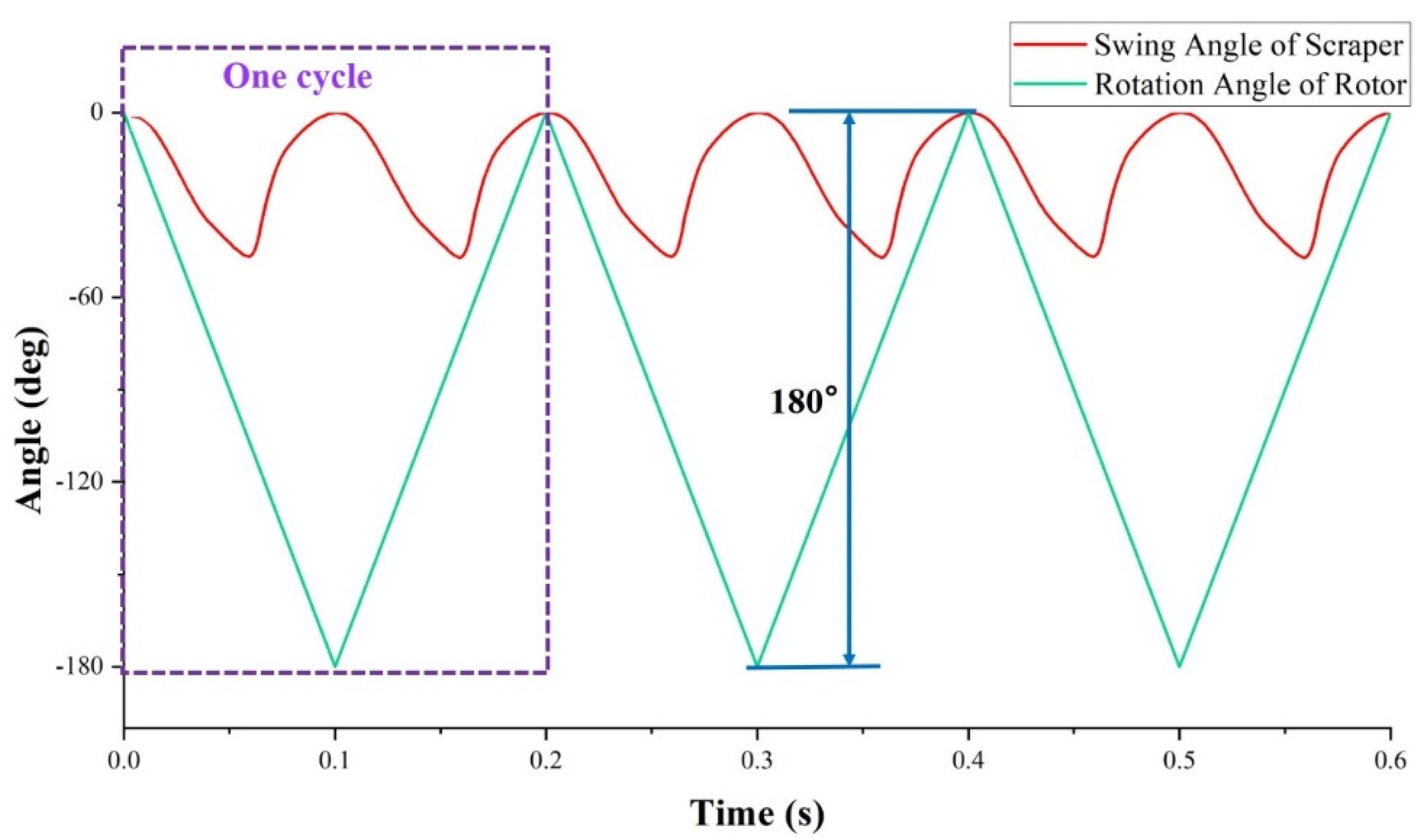
4. Simulation Analysis
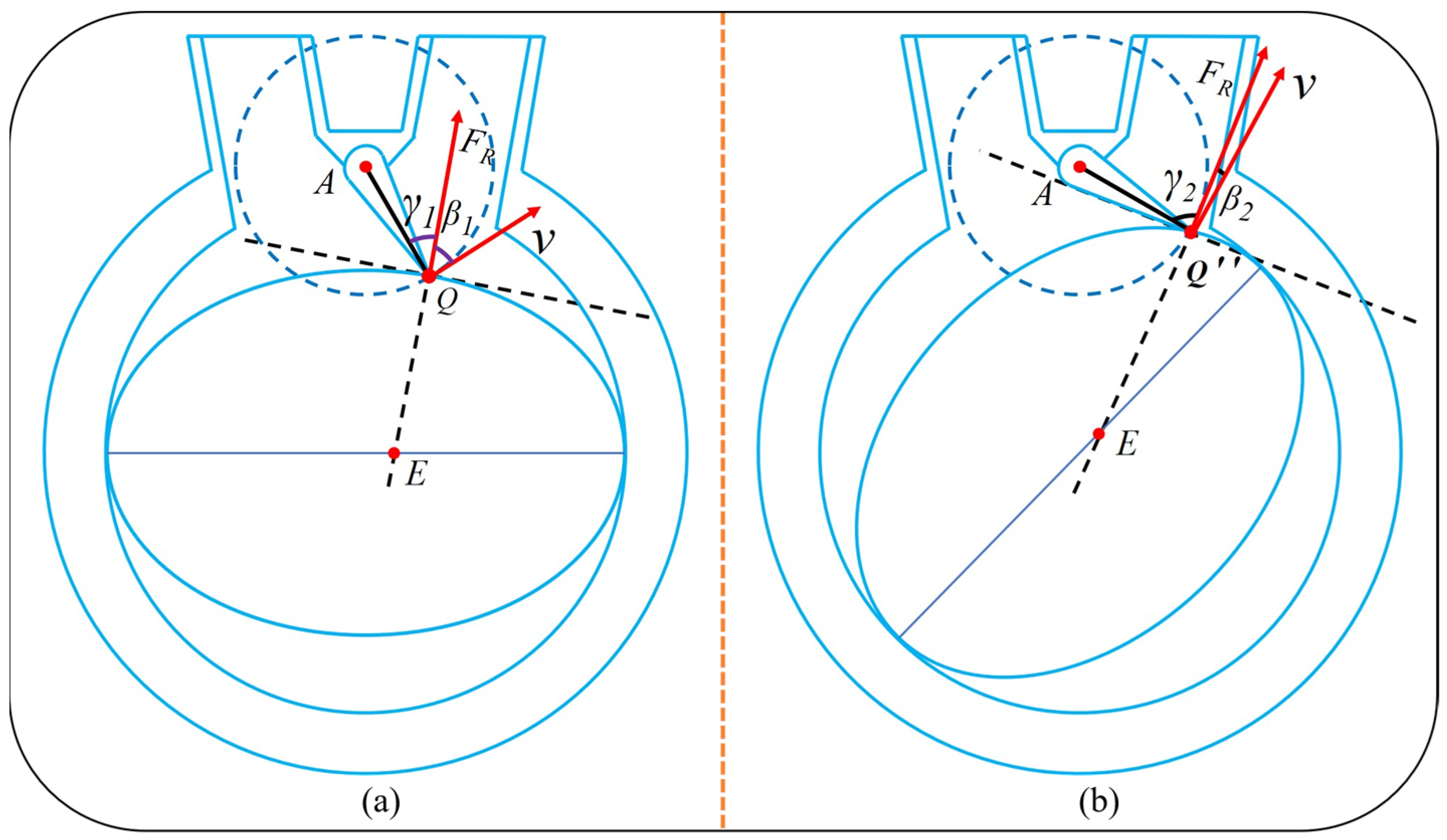
5. Self-Locking Characteristics Analysis
5.1. Theoretical Formula Derivation
5.2. Example Analysis
6. Prototype Physical Display
7. Conclusions and Prospects
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Josifovic:, A.; Corney, J.; Davies, B. Valve dynamics in multi-cylinder positive displacement pump model. In Proceedings of the IEEE International Conference on Advanced Intelligent Mechatronics, Busan, Korea, 7–11 July 2015; pp. 35–41. [Google Scholar]
- van Rijswick, R.; Talmon, A.; van Rhee, C. Fluid structure interaction (fsi) in piston diaphragm pumps. Can. J. Chem. Eng. 2016, 94, 1116–1126. [Google Scholar] [CrossRef]
- Zhao, F.; Zhang, S.-W.; Han, F.; Li, R.-D. Thermodynamics Analysis of Roots Vacuum Pump. J. Phys. Conf. Ser. 2020, 1519, 012016. [Google Scholar] [CrossRef]
- Bhandari, N.; Damodaran, M. Computational Prediction of the Performance of Positive Displacement Pumps; Springer: Delhi, India, 2017. [Google Scholar]
- Bai, J.J.; Xu, M.M. Research Status and Development Trend of Vane Pump. Appl. Mech. Mater. 2014, 556–562, 1143–1146. [Google Scholar] [CrossRef]
- Bartel, S.; Kluszczynski, K.; Pilch, Z. Concept of electromagnetic periodical duty pump with programmable liquid flow. In Proceedings of the 2019 15th Selected Issues of Electrical Engineering and Electronics (WZEE), Zakopane, Poland, 8–10 December 2019. [Google Scholar]
- Konieczny, J.; Stojek, J. Use of the K-Nearest Neighbour Classifier in Wear Condition Classification of a Positive Displacement Pump. Sensors 2021, 21, 6247. [Google Scholar] [CrossRef]
- Wu, X.; Chen, C.; Hong, C.; He, Y. Flow ripple analysis and structural parametric design of a piston pump. J. Mech. Sci. Technol. 2017, 31, 4245–4254. [Google Scholar] [CrossRef]
- Ding, H. Application of non-circular planetary gear mechanism in the gear pump. Adv. Mater. Res. 2012, 591–593, 2139–2142. [Google Scholar] [CrossRef]
- Wang, H.; Du, S.S. External Gear Pump Model and Simulation. Appl. Mech. Mater. 2011, 127, 228–232. [Google Scholar] [CrossRef]
- Zhu, H.L.; Pan, J.; Min, Z.; Wu, H.N.; Qin, X. Which will win in the gear pump technology. Trans. Can. Soc. Mech. Eng. 2013, 37, 129–134. [Google Scholar] [CrossRef]
- Maqsood, M.; Usman, A.; Bodla, M.F.; Ali, J. Evaluation of performance parameters of indigenously developed roots pumping system. IOP Conf. Ser. Mater. Sci. Eng. 2016, 146, 012047. [Google Scholar] [CrossRef] [Green Version]
- Mikhal’Chenkova, A.N.; Lagutkin, M.G.; Baranova, E.Y. Comparative Analysis of Vortex Ejector and Jet Pump Characteristics. Chem. Pet. Eng. 2020, 56, 522–528. [Google Scholar] [CrossRef]
- Al-Habahbeh, O.; Al-Saqqa, M.; Safi, M.; Khater, T.A. Review of magnetohydrodynamic pump applications. Alex. Eng. J. 2016, 55, 1347–1358. [Google Scholar] [CrossRef] [Green Version]
- Bashiri, M.; Derakhshan, S.; Shahrabi, J. Design Optimization of a Centrifugal Pump Using Particle Swarm Optimization Algorithm. Int. J. Fluid Mach. Syst. 2019, 12, 322–331. [Google Scholar] [CrossRef]
- Liu, D.; Ba, Y.; Ren, T. Flow fluctuation abatement of high-order elliptical gear pump by external noncircular gear drive. Mech. Mach. Theory 2019, 134, 338–348. [Google Scholar] [CrossRef]
- Wang, J.; Yang, S.; Sha, R.; Li, H.; Xu, C. Geometric Design and Analysis of Novel Asymmetrical Rotors for Roots Vacuum Pumps. J. Mech. Des. 2019, 142, 1–8. [Google Scholar] [CrossRef]
- Wu, Y.-R.; Tran, V.-T. Generation method for a novel Roots rotor profile to improve performance of dry multi-stage vacuum pumps. Mech. Mach. Theory 2018, 128, 475–491. [Google Scholar] [CrossRef]
- Hsieh, C.F. A new curve for application to the rotor profile of rotary lobe pumps. Mech. Mach. Theory 2015, 87, 70–81. [Google Scholar] [CrossRef]
- Wu, X.; Sun, K. Research on a new type of water hydraulic vane pump. In Proceedings of the International Conference on Fluid Power & Mechatronics, Harbin, China, 5–7 August 2015; pp. 649–653. [Google Scholar]
- Shim, S.-B.; Park, Y.-J.; Nam, J.-S.; Kim, S.-C.; Kim, J.-M.; Kim, K.-U. Development of a rotary clap mechanism for positive-displacement rotary pumps: Pump performance analysis. Int. J. Precis. Eng. Manuf. 2017, 18, 575–585. [Google Scholar] [CrossRef]
- Shim, S.-B.; Park, Y.-J.; Nam, J.-S.; Kim, S.-C. Development of a rotary clap mechanism for positive-displacement rotary pumps: Experimental verification and optimization. Int. J. Precis. Eng. Manuf. 2017, 18, 587–597. [Google Scholar] [CrossRef]
- Keisar, D.; Eilan, B.; Greenblatt, D. High Pressure Vertical Axis Wind Pump. J. Fluids Eng. 2021, 143, 051204. [Google Scholar] [CrossRef]
- Xu, W.; Zhang, H.; Shu, P.; Gao, L. Kinematics research of rotating-sleeve distributing-flow system for reciprocating plunger pump. In Proceedings of the 5th International Conference on Advanced Design and Manufacturing Engineering, Shenzhen, China, 19–20 September 2015. [Google Scholar]
- Lu, Q.; Qin, N. Research on new type of giant magnetostrictive precision flow pump. In Proceedings of the 2015 International Symposium on Material, Energy and Environment Engineering, Changsha, China, 28–29 November 2015. [Google Scholar]
- Ivanovi, L.; Stojanovi, B.; Blagojevi, J.; Bogdanovi, G.; Marinkovi, A. Analysis of the flow rate and the volumetric efficiency of the trochoidal pump by application of taguchi method. Teh. Vjesn. 2017, 24, 265–270. [Google Scholar]
- Leonesio, M.; Bianchi, G. Self-locking analysis in closed kinematic chains. Mech. Mach. Theory 2009, 44, 2038–2052. [Google Scholar] [CrossRef]
- Lin, R.; Guo, W. Type synthesis of reconfiguration parallel mechanisms transforming between trusses and mechanisms based on friction self-locking composite joints. Mech. Mach. Theory 2021, 168, 104597. [Google Scholar] [CrossRef]
- Zhang, Z.; Zhang, T.; Zhang, H.; Yang, J.; Cao, Y.; Jiang, Y.; Tian, D. Modeling, Kinematic Characteristics Analysis and Experimental Testing of an Elliptical Rotor Scraper Pump. Machines 2022, 10, 78. [Google Scholar] [CrossRef]



| Parameters | Value |
|---|---|
| Long axis of elliptical line a | 741 mm |
| Short axis of elliptical line b | 520 mm |
| Length of swing scraper r | 368 mm |
| Length of the AB l | 297 mm |
| Thickness of elliptical rotor | 228 mm |
| Suction Inlet Diameter | Rotary Speed | Flow Rate | Volume Efficiency | |
|---|---|---|---|---|
| Roots Pump | 100 mm | 1310 r/min | 4.73 m3/min | 72.4% |
| ERSP | 100 mm | 1310 r/min | 5.09 m3/min | 83.2% |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cao, Y.; Zhang, T.; Zhang, H.; Zhang, Z.; Yang, J.; Liu, B. Study of the Self-Locking Characteristics of the Swing Scraper of an Elliptical Rotor Scraper Pump. Machines 2022, 10, 370. https://doi.org/10.3390/machines10050370
Cao Y, Zhang T, Zhang H, Zhang Z, Yang J, Liu B. Study of the Self-Locking Characteristics of the Swing Scraper of an Elliptical Rotor Scraper Pump. Machines. 2022; 10(5):370. https://doi.org/10.3390/machines10050370
Chicago/Turabian StyleCao, Yang, Tiezhu Zhang, Hongxin Zhang, Zhen Zhang, Jian Yang, and Baoquan Liu. 2022. "Study of the Self-Locking Characteristics of the Swing Scraper of an Elliptical Rotor Scraper Pump" Machines 10, no. 5: 370. https://doi.org/10.3390/machines10050370
APA StyleCao, Y., Zhang, T., Zhang, H., Zhang, Z., Yang, J., & Liu, B. (2022). Study of the Self-Locking Characteristics of the Swing Scraper of an Elliptical Rotor Scraper Pump. Machines, 10(5), 370. https://doi.org/10.3390/machines10050370







