Distribution Characteristics of Screw Axes Belonging to General Three-System of Screws

Abstract

The cylindroid describes the exact distribution of the ${\infty }^1$ screw axes of the general two-system of screws. For the general three-system, this article introduces the surface on which lies the position vector endpoints of its ${\infty }^2$ screw axes, and then develops the finite distribution zone in which they most densely distribute. In particular, this paper explores the inner structure of the zone by partitioning the general three-system into ${\infty }^1$ two-subsystems, and then reveals the principle of constructing the distribution space of the general three-system by that of the general two-system in Euclidean three-space. In pursuit of a generalized decomposition method, we propose the varying-pitch ruled surface carrying the screws whose pitch value continuously varies according to any desired function rule, based on the pitch-hyperboloid carrying all the equal-pitch screws. This investigation provides some useful guides for the axode planning and then the motion planning of three degree-of-freedom parallel mechanisms.

1. Introduction

When planning the motion of lower-mobility parallel mechanisms, we may need to plan the distribution of a serial of instantaneous screw axes (ISAs) of the moving platform (i.e., to plan its axode) in the reference frame of the mechanisms for some special application scenarios. However, the rotational axes of lower-mobility parallel mechanisms cannot be chosen arbitrarily in the reference frame of the mechanisms [1]. For the above fact accounts the corresponding lower-order screw system (the order of screw system is no more than three) [2] whose screw axes distribute in a finite zone of Euclidean three-space. So, this finite zone must be taken into consideration when we plan the axode of lower-mobility parallel mechanisms.

Although Ball and Hunt [3,4,5] have revealed that the equal-pitch screws of the general three-system lie on a pitch-hyperboloid, there have been few papers providing a deeper and more comprehensive study on the finite zone and the distribution characteristics of the ${\infty }^2$ screw axes of the general three-system in Euclidean three-space. To provide some useful guides for the axode planning of three degree-of-freedom parallel mechanisms, we originally present the mathematical expressions of the finite distribution zone of the ${\infty }^2$ screw axes of the general three-system by deriving the ${\infty }^1$ striction points on a screw axis when it is infinitely close to its surrounding ${\infty }^1$ screw axes in its infinitesimal neighborhood space. Furthermore, we originally reveal the distribution regularity of the ${\infty }^2$ screw axes of the general three-system by partitioning them into ${\infty }^1$ two-subsystems [6].

For the convenience of analysis of the distribution characteristics of the general three-system, we compute and derive it in its principal frame [4,7] in which its three principal screws attain their simplest forms because they are mutually and orthogonally concurrent at the origin of the principal frame and because their screw axes are along the x-, y-, and z-axes of the principal frame, respectively. When planning the axode of lower-mobility parallel mechanisms, we need to transform the distribution zone to the reference frame of mechanisms. So, identifying the principal screws of a screw system when a set of general basis screws has been provided is a key issue in axode planning. This problem has been reduced to an eigenproblem by Parkin [8] (1990), Tsai [9] (1993), Parkin [10] (1997), Rico [11] (1998), Bandyopadhyay [12] (2004), Bandyopadhyay [13] (2009), and Zhao [14] (2009). In particular, Zhang [15] (1998) proposed an algebraic method computing the principal screws of three-systems according to the relationship between two-systems and three-systems. Fang [16] (1998) obtained the principal screws of three-systems by employing the conical section degenerating theory. Bandyopadhyay [13] pointed out that the solution of the generalized eigenproblem gives rise to the extreme values of the pitch by differentiating the expression of the pitch and arriving at the identical equation with the eigenproblem.

On the distribution of screw axes of the screw system, Huang [17] discussed the correspondence between the finite screw system and projective space. Inspired by the one-to-one mapping relationship [3] between the points on a unit circle and the screws within a two-system, Zlatanov [6] developed the one-to-one mapping between the points on a unit sphere and the screws within a three-system. Study’s map [18] clarifies there is a one-to-one correspondence between oriented straight lines in Euclidean three-space and the dual points on the surface of a dual unit sphere in dual space. Hence, a differential curve on the sphere corresponds to a ruled surface in line space.

Since the above references study the distribution of screw axes in abstract spaces, it is hard to apply them to axode planning in Euclidean three-space. To develop intuitive and visual methods of axode planning, we especially study the distribution regularity of the screw axes of the general three-system in Euclidean three-space. Furthermore, to build a bridge between axode planning and the motion planning of parallel mechanisms, we have developed a kinematic model of screw rolling between fixed and moving axodes [19,20], which solves the problem, “Given a pair of fixed and moving axodes, how to reproduce the associated continuous rigid motion?” For the distribution zone of the screw axes of higher-order screw systems, perhaps it can be decomposed into that of lower-order screw systems, as Chen [2] has decomposed the four-, five-, and six-systems into the direct sums of several two- and three-systems by the decomposition of the positive semidefinite (PSD) method.

The advances of screw theory in mechanisms are briefly listed here. Toscano [21] developed the kinetostatic model for HRs based on the screw theory. Zhao [22] proved that the time derivative of the twist is a screw and revealed that its physical meaning is the rigid-body acceleration. Sharafian [23] enhanced the joint-based method to a new version by resorting to reciprocal screws, and introduced the constraint forces and moments distribution indices. Gallardo [24] carried out the kinematic and dynamic analyses of the 2 (3-RPS) series-parallel manipulator by employing the theory of screws and the principle of virtual work. Fan [25] proposed a novel concept of a virtual screw that is formulated by a combination of virtual angular velocity and virtual linear velocity. Bu [26] developed a novel metric based on the geometric average normalized volume spanned by weighted screws to measure the distance from the current configuration to a singular configuration. Gallardo [27] obtained the input–output equations of velocity and acceleration of the 5-RPUR parallel mechanism by resorting to reciprocal screws.

The remainder of this article is organized as follows. Section 2 discusses the distribution of the position vector endpoints of the ${\infty }^2$ screw axes of the general three-system. Since the position endpoints are the particular case of the striction points on the screw axis, Section 3 studies the distribution of the ${\infty }^3$ striction points of the ${\infty }^2$ screw axes. The above two sections exactly describe the finite distribution zone of screw axes of the general three-system in Euclidean three-space. To reveal the inner structure of this finite zone, Section 4 decomposes the general three-system into ${\infty }^1$ two-subsystems and presents the detailed distribution regularity of ${\infty }^1$ cylindroids fulfilling the finite zone by three theorems. In pursuit of a generalized decomposition method, Section 5 proposes the varying-pitch ruled surface based on the pitch-hyperboloid. A numerical example of the 3-RPS pyramid mechanism is presented for the application of the concept ‘the densest distribution zone’ in Section 6.

2. Distribution Surface of Position Vector Endpoints of Screw Axes

In this section, we mainly discuss the positions of the ${\infty }^2$ screw axes of the general three-system. The general three-system of screws was firstly proposed by Hunt [4] when he classified the screw systems by the pitch values of their principal screws. In the principal frame, three principal screws of general three-systems can be expressed, respectively, as:

$${\$}_1^p=\left(1, 0, 0; h_1, 0, 0\right)\hspace{1em}{\$}_2^p=\left(0, 1, 0; 0, h_2, 0\right)\hspace{1em}{\$}_3^p=\left(0, 0, 1; 0, 0, h_3\right)$$

(1)

in the form of unit Plücker coordinates, where the superscript p represents principal screws. Without a loss of generality, we take the three principal pitches as $h_1>h_2>h_3$ because they are mutually distinct and are finite real numbers. Consequently, any screw within this screw system has the form

$${\$}_i={\$}_1^p+{\lambda }_1{\$}_2^p+{\lambda }_2{\$}_3^p=\left(1, {\lambda }_1, {\lambda }_2; h_1, {\lambda }_1{h}_2, {\lambda }_2{h}_3\right)$$

(2)

where the coefficients ${\lambda }_1\in (-\infty ,+\infty )$ and ${\lambda }_2\in (-\infty ,+\infty )$ are both real numbers.

Remark: 

The${\infty }^2$screws represented by the equation ${\$}_i=-{\$}_1^p+{\lambda }_1{\$}_2^p+{\lambda }_2{\$}_3^p$are collinear, but have opposite directions with those represented by Equation(2), respectively. Since they are mirror images, they have the same distribution characteristics in Euclidean three-space. So, we only confine attention to Equation(2) in this paper.

Setting the primary part ${\mathit{S}}_i=(1, {\lambda }_1, {\lambda }_2)$ of ${\$}_i$ and the dual part ${\mathit{S}}_i^0=(h_1, {\lambda }_1{h}_2, {\lambda }_2{h}_3)$ of ${\$}_i$, the pitch of ${\$}_i$ has the form

$$h_i=\frac{{\mathit{S}}_i\cdot {\mathit{S}}_i^0}{{\mathit{S}}_i\cdot {\mathit{S}}_i}=\frac{h_1+{\lambda }_1^2{h}_2+{\lambda }_2^2{h}_3}{1+{\lambda }_1^2+{\lambda }_2^2}$$

(3)

We make a segment $\overline{OP}$ from the origin O of the principal frame to the point P on the axis of ${\$}_i$. For the uniqueness of the position representation of the screw ${\$}_i$, P is the nearest point to the origin O among the ${\infty }^1$ points on the axis of ${\$}_i$. In other words, the segment $\overline{OP}$ is perpendicular to the axis of ${\$}_i$. The vector $\overline{OP}$ is defined as the position vector of the screw ${\$}_i$ with respect to the principal frame and has the form

$$\overline{OP}=\frac{{\mathit{S}}_i\times {\mathit{S}}_i^0}{{\mathit{S}}_i\cdot {\mathit{S}}_i}=\frac{\left({\lambda }_1{\lambda }_2(h_3-h_2), {\lambda }_2(h_1-h_3), {\lambda }_1(h_2-h_1)\right)}{1+{\lambda }_1^2+{\lambda }_2^2}$$

(4)

The perpendicular foot P is defined as the position vector endpoint of the screw ${\$}_i$, with respect to the principal frame, in which P with the coordinates $(x, y, \mathrm{z})$ has the following parametric equations in terms of the coefficients ${\lambda }_1$ and ${\lambda }_2$:

$$\{\begin{array}{l}x=\frac{{\lambda }_1{\lambda }_2(h_3-h_2)}{1+{\lambda }_1^2+{\lambda }_2^2}\\ y=\frac{{\lambda }_2(h_1-h_3)}{1+{\lambda }_1^2+{\lambda }_2^2}\in (\frac{h_3-h_1}{2},\frac{h_1-h_3}{2})\\ z=\frac{{\lambda }_1(h_2-h_1)}{1+{\lambda }_1^2+{\lambda }_2^2}\in (\frac{h_2-h_1}{2},\frac{h_1-h_2}{2})\end{array}$$

(5)

In the above equations, dividing equation x by equation y and equation z, respectively, and rearranging, yields

$${\lambda }_1=\frac{x(h_1-h_3)}{y(h_3-h_2)}\hspace{1em}{\lambda }_2=\frac{x(h_1-h_2)}{z(h_2-h_3)}$$

(6)

Substituting the above expressions of ${\lambda }_1$ and ${\lambda }_2$ into equation y of Equation (5) and rearranging yields

$${(h_3-h_2)}^2{z}^2{y}^2+{(h_1-h_3)}^2{x}^2{z}^2+(h_2-h_1)x^2{y}^2-(h_3-h_2)(h_1-h_3)(h_2-h_1)xyz=0$$

(7)

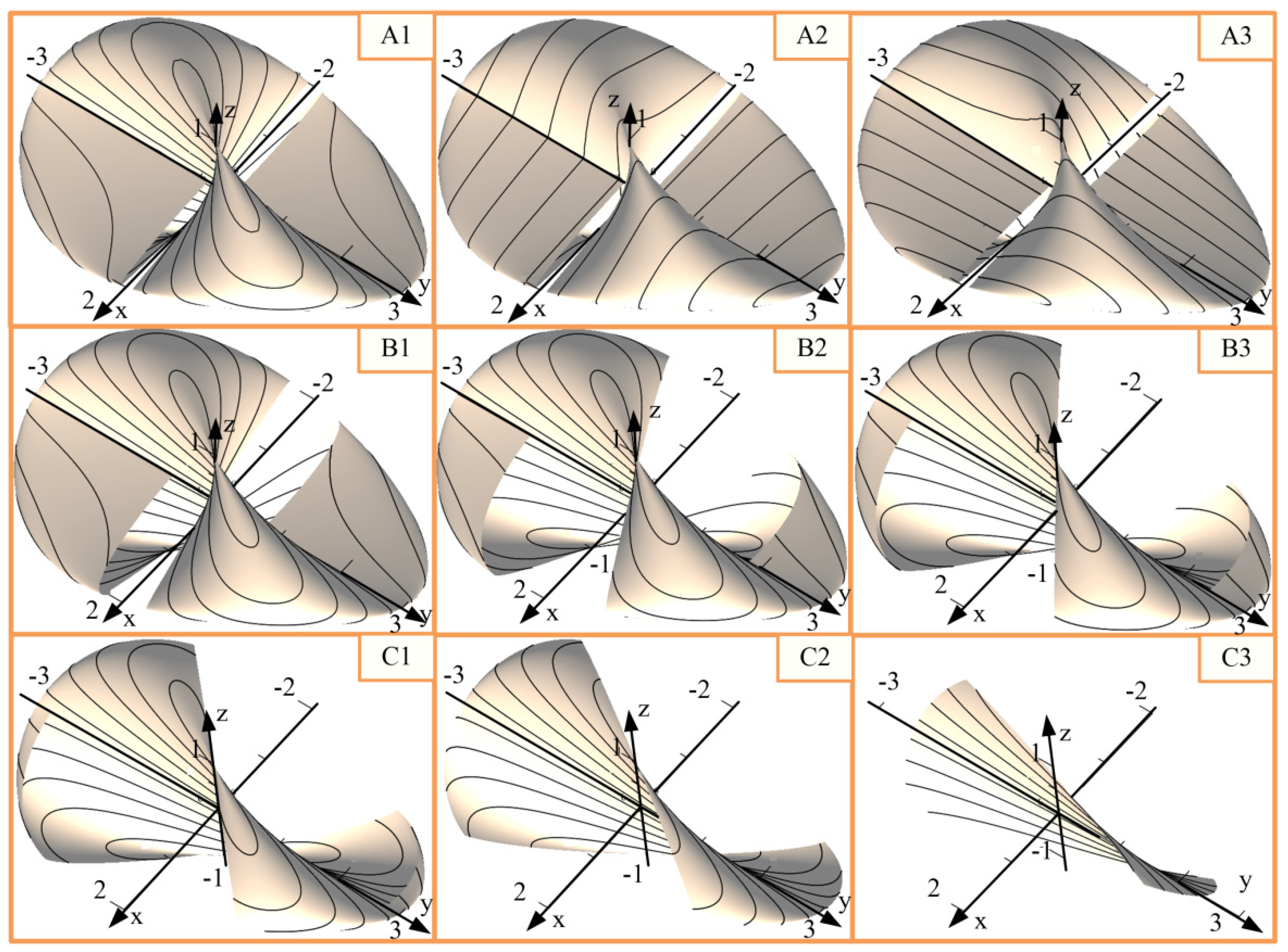
The above equation is simply the expression of the surface $S^{\perp }$ proposed by Hunt [28]. For an insight into the inner structure of this surface, it is plotted in Figure 1 by Equation (5) when we set $h_1=7$, $h_2=5$, and $h_3=1$, and set the coefficients ${\lambda }_1$ and ${\lambda }_2$ as the two primary variables.

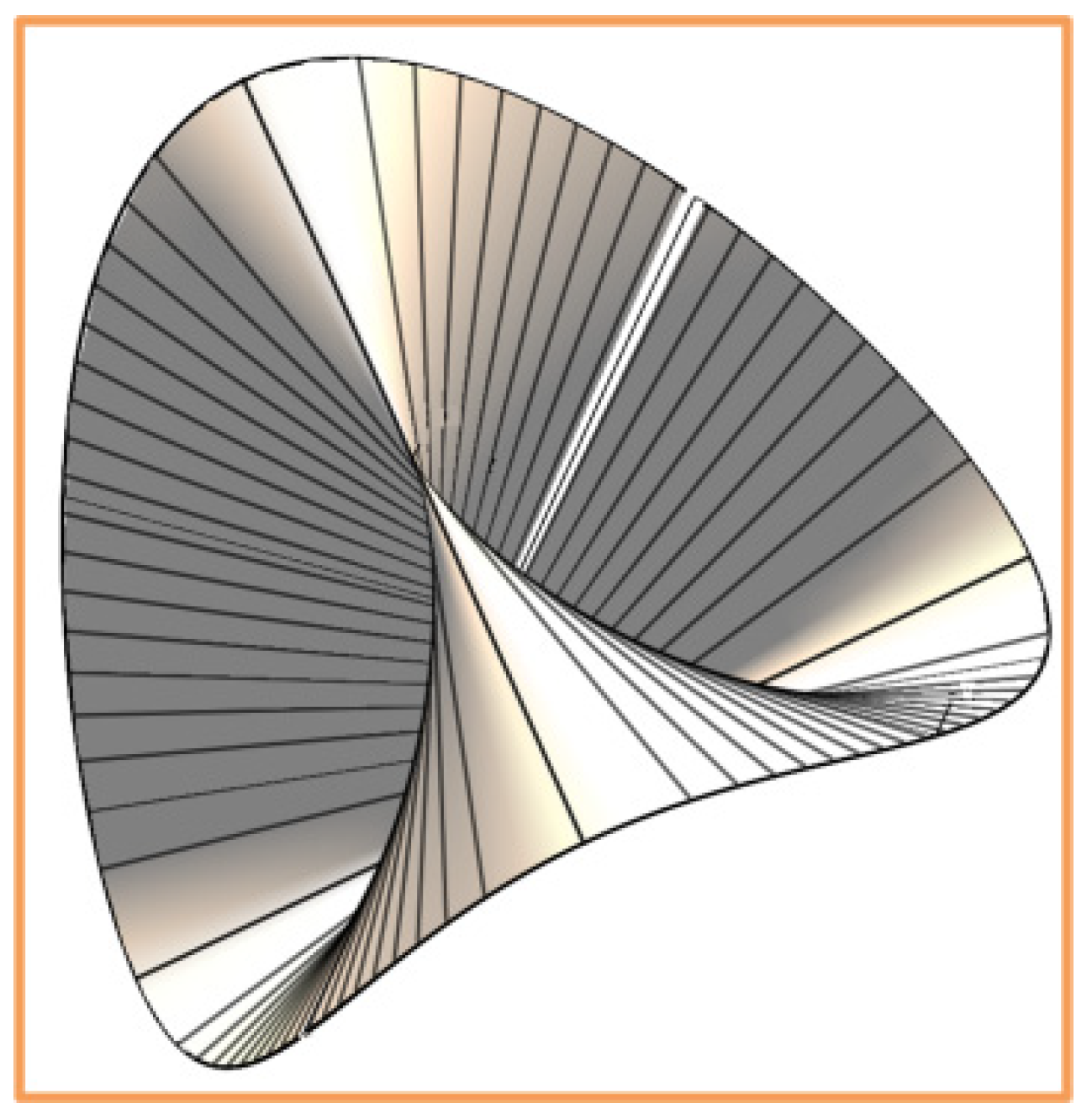
The curves in Figure 1(A1–A3) are the contour lines of the surface $S^{\perp }$ perpendicular to the z-, y-, and x-axes of the principal frame, respectively. The primary variables ${\lambda }_1$ and ${\lambda }_2$ both continuously take values in the interval $[-d, d]$. The surface $S^{\perp }$ grows up from a patch of surface to a semi-closed surface with four cavity structures, as shown in Figure 1(C3,C2,C1,B3,B2,B1,A3) when the range parameter d takes the values 0.5, 1, 1.5, 2, 3, 8, and 30 in turn, respectively. When the pair of primary variables $({\lambda }_1,{\lambda }_2)$ takes the pair of values $(0,1)$ and $(0,-1)$, the coordinate y in Equation (5) obtains its two extreme values $(h_1-h_3)/2$ and $(h_3-h_1)/2$, respectively. Similarly, coordinate z in Equation (5) obtains the two extreme values $(h_2-h_1)/2$ and $(h_1-h_2)/2$ when the pair of primary variables $({\lambda }_1,{\lambda }_2)$ takes the pair of values $(1,0)$ and $(-1,0)$, respectively. So, the cavity structures begin to appear when d = 1, as shown in the Figure 1(C2). In particular, since coordinate x in Equation (5) has no extreme values, the four cavity structures infinitely approach the x-axis of the principal frame, but will never be close even though the primary variables ${\lambda }_1$ and ${\lambda }_2$ both tend to positive or negative infinity. The surface $S^{\perp }$ describes the exact distribution of the position of the ${\infty }^2$ screw axes in Euclidean three-space.

3. Densest Distribution Zone of Screw Axes

In this section, we will derive the expression of the finite distribution zone and then reveal that the position vector endpoint is the particular case of the striction points on the axis of ${\$}_i$.

When two lines lying on a ruled surface infinitely approach each other, the two endpoints of their common perpendicular segment infinitely tend to a limit point. This limit point is called a striction point of the ruled surface. Analogously, every one of the ${\infty }^2$ screw axes of a general three-system will get ${\infty }^1$ striction points when it is infinitely close to its surrounding ${\infty }^1$ screw axes in all directions in its infinitesimal neighborhood space. So, the ${\infty }^3$ striction points of the general three-system constitute a finite striction zone. According to its definition, the ${\infty }^2$ screw axes all pass through this zone, and they most densely distribute in this zone. Therefore, we define this finite striction zone as the densest distribution zone of the general three-system. In the following lines, we will derive the parametric equation of the densest distribution zone.

Setting $\mathit{P}=\overline{OP}$ as the position vector of the screw ${\$}_i$, its axis has the following Plücker coordinates:

$${\$}_L=\left(\mathit{S}; \mathit{P}\times \mathit{S}\right)$$

(8)

where $\mathit{S}={\mathit{S}}_i/\left|{\mathit{S}}_i\right|$ is the unit vector. Without a loss of generality, the axis of another screw, ${\$}_j$, which is just in the infinitesimal neighborhood space of the screw ${\$}_i$, is denoted by:

$${\$}_{L1}=\left({\mathit{S}}_1; {\mathit{P}}_1\times {\mathit{S}}_1\right)$$

(9)

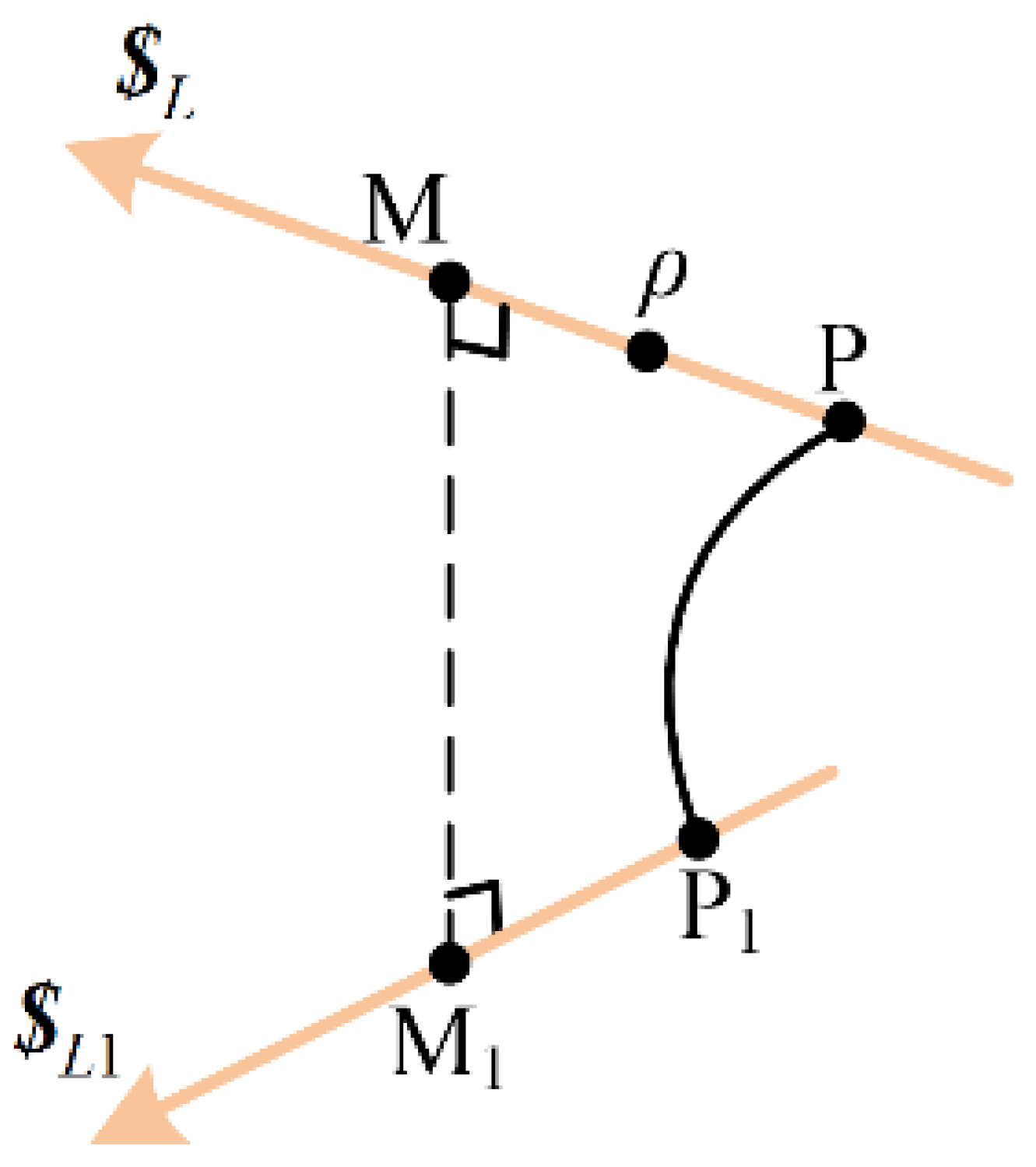
The relative location between ${\$}_i$ and ${\$}_j$ is shown in Figure 2.

As seen in Figure 2, $\overline{M{M}_1}$ is the screw axes’ common perpendicular segment. P and $P_1$ are their respective position vector endpoints, and $\mathit{\rho }$ is a striction point generated by ${\$}_L$ and ${\$}_{L1}$ when they are infinitely close to each other. According to Equations (1–31) of [29], the point ${\mathit{M}}_1$ has the form

$${\mathit{M}}_1=\frac{\mathit{S}\times (\mathit{S}\times {\mathit{S}}_1)\times ({\mathit{P}}_1\times {\mathit{S}}_1)+\left(\mathit{S}\times (\mathit{P}\times \mathit{S})\cdot \mathit{S}\times (\mathit{S}\times {\mathit{S}}_1)\right){\mathit{S}}_1}{{\mathit{S}}_1\cdot \mathit{S}\times (\mathit{S}\times {\mathit{S}}_1)}$$

(10)

Considering $\mathit{S}$, $\mathit{P}$, ${\mathit{S}}_1$, and ${\mathit{P}}_1$ are the vector functions of the two variables in ${\lambda }_1$ and ${\lambda }_2$, we set

$$\begin{array}{l}\mathit{S}=\mathit{S}({\lambda }_1, {\lambda }_2){\mathit{S}}_1=\mathit{S}({\lambda }_1+\mathrm{\Delta }{\lambda }_1, {\lambda }_2+\mathrm{\Delta }{\lambda }_2)\\ \mathit{P}=\mathit{P}({\lambda }_1, {\lambda }_2){\mathit{P}}_1=\mathit{P}({\lambda }_1+\mathrm{\Delta }{\lambda }_1, {\lambda }_2+\mathrm{\Delta }{\lambda }_2)\end{array}$$

(11)

where $\mathrm{\Delta }{\lambda }_1$ and $\mathrm{\Delta }{\lambda }_2$ are the increments of the variables ${\lambda }_1$ and ${\lambda }_2$, respectively. According to Taylor’s formula for functions of two variables, we have

$$\begin{array}{l}{\mathit{S}}_1=\mathit{S}+\mathrm{\Delta }{\lambda }_1\frac{\partial \mathit{S}}{\partial {\lambda }_1}+\mathrm{\Delta }{\lambda }_2\frac{\partial \mathit{S}}{\partial {\lambda }_2}+{\mathit{R}}_{s1}\\ {\mathit{P}}_1=\mathit{P}+\mathrm{\Delta }{\lambda }_1\frac{\partial \mathit{P}}{\partial {\lambda }_1}+\mathrm{\Delta }{\lambda }_2\frac{\partial \mathit{P}}{\partial {\lambda }_2}+{\mathit{R}}_{p1}\end{array}$$

(12)

where ${\mathit{R}}_{s1}$ and ${\mathit{R}}_{p1}$ are the remainder vectors of ${\mathit{S}}_1$ and ${\mathit{P}}_1$, respectively, which both consist of higher-order vector derivatives and mixed derivatives, and their coefficients are all the infinitesimal amounts of more higher-order than $\mathrm{\Delta }{\lambda }_1$ and $\mathrm{\Delta }{\lambda }_2$ when they infinitely tend to zero. By setting

$$\mathrm{\Delta }\mathit{S}=\mathrm{\Delta }{\lambda }_1\frac{\partial \mathit{S}}{\partial {\lambda }_1}+\mathrm{\Delta }{\lambda }_2\frac{\partial \mathit{S}}{\partial {\lambda }_2}+{\mathit{R}}_{s1}\hspace{1em}\mathrm{\Delta }\mathit{P}=\mathrm{\Delta }{\lambda }_1\frac{\partial \mathit{P}}{\partial {\lambda }_1}+\mathrm{\Delta }{\lambda }_2\frac{\partial \mathit{P}}{\partial {\lambda }_2}+{\mathit{R}}_{p1}$$

(13)

Equation (12) can be rewritten as

$${\mathit{S}}_1=\mathit{S}+\mathrm{\Delta }\mathit{S}\hspace{1em}{\mathit{P}}_1=\mathit{P}+\mathrm{\Delta }\mathit{P}$$

(14)

Substituting Equation (14) into Equation (10) yields

$${\mathit{M}}_1=\mathit{P}+\mathrm{\Delta }\mathit{P}-\frac{\left(\mathit{S}\cdot \mathrm{\Delta }\mathit{P}(\mathit{S}\cdot \mathrm{\Delta }\mathit{S})-\mathrm{\Delta }\mathit{S}\cdot \mathrm{\Delta }\mathit{P}\right)(\mathit{S}+\mathrm{\Delta }\mathit{S})}{{(\mathit{S}\cdot \mathrm{\Delta }\mathit{S})}^2-\mathrm{\Delta }\mathit{S}\cdot \mathrm{\Delta }\mathit{S}}$$

(15)

In Euclidean two-space endowed with the Cartesian coordinate system $O{\lambda }_1{\lambda }_2$, when point $({\lambda }_1+\mathrm{\Delta }{\lambda }_1,{\lambda }_2+\mathrm{\Delta }{\lambda }_2)$ infinitely tends to point $({\lambda }_1, {\lambda }_2)$, i.e., $\mathrm{\Delta }{\lambda }_1$ and $\mathrm{\Delta }{\lambda }_2$ both get near to zero, ${\mathit{M}}_1$ moves along the moving segment $\overline{M{M}_1}$ toward $\mathit{M}$ and then it moves along ${\$}_L$ toward $\mathit{\rho }$, i.e., point ${\mathit{M}}_1$ infinitely tends to point $\mathit{M}$ and then tends to striction point $\mathit{\rho }$ in the principal frame of the general three-system. Consequently, taking the limitation of Equation (15) when $\mathrm{\Delta }{\lambda }_1$ and $\mathrm{\Delta }{\lambda }_2$ both infinitely get near to zero, we have:

$$\begin{array}{ll}\mathit{\rho }& =\underset{\{\begin{array}{l}\mathrm{\Delta }{\lambda }_1\to 0\\ \mathrm{\Delta }{\lambda }_2\to 0\end{array}}{\lim }{\mathit{M}}_1=\mathit{P}-\underset{\{\begin{array}{l}\mathrm{\Delta }{\lambda }_1\to 0\\ \mathrm{\Delta }{\lambda }_2\to 0\end{array}}{\lim }\frac{\left(\frac{\mathit{S}\cdot \mathrm{\Delta }\mathit{P}(\mathit{S}\cdot \mathrm{\Delta }\mathit{S})}{{(\mathrm{\Delta }{\lambda }_1)}^2}-\frac{\mathrm{\Delta }\mathit{S}\cdot \mathrm{\Delta }\mathit{P}}{{(\mathrm{\Delta }{\lambda }_1)}^2}\right)(\mathit{S}+\mathrm{\Delta }\mathit{S})}{\frac{{(\mathit{S}\cdot \mathrm{\Delta }\mathit{S})}^2-\mathrm{\Delta }\mathit{S}\cdot \mathrm{\Delta }\mathit{S}}{{(\mathrm{\Delta }{\lambda }_1)}^2}}\\ & =\mathit{P}-\frac{\left\{\left(\mathit{S}\cdot (\frac{\partial \mathit{P}}{\partial {\lambda }_1}+k\frac{\partial \mathit{P}}{\partial {\lambda }_2})\right)\left(\mathit{S}\cdot (\frac{\partial \mathit{S}}{\partial {\lambda }_1}+k\frac{\partial \mathit{S}}{\partial {\lambda }_2})\right)-(\frac{\partial \mathit{S}}{\partial {\lambda }_1}+k\frac{\partial \mathit{S}}{\partial {\lambda }_2})\cdot (\frac{\partial \mathit{P}}{\partial {\lambda }_1}+k\frac{\partial \mathit{P}}{\partial {\lambda }_2})\right\}}{{\left(\mathit{S}\cdot (\frac{\partial \mathit{S}}{\partial {\lambda }_1}+k\frac{\partial \mathit{S}}{\partial {\lambda }_2})\right)}^2-(\frac{\partial \mathit{S}}{\partial {\lambda }_1}+k\frac{\partial \mathit{S}}{\partial {\lambda }_2})\cdot (\frac{\partial \mathit{S}}{\partial {\lambda }_1}+k\frac{\partial \mathit{S}}{\partial {\lambda }_2})}\mathit{S}\end{array}$$

(16)

provided the limit exists, where

$$k=\underset{\{\begin{array}{l}\mathrm{\Delta }{\lambda }_1\to 0\\ \mathrm{\Delta }{\lambda }_2\to 0\end{array}}{\lim }\frac{\mathrm{\Delta }{\lambda }_2}{\mathrm{\Delta }{\lambda }_1}=\frac{d{\lambda }_2}{d{\lambda }_1}=f^{\prime }({\lambda }_1)$$

(17)

In particular, we introduce the function ${\lambda }_2=f({\lambda }_1)$, which represents an arbitrary curve in ${\lambda }_1{\lambda }_2$ plane of the Cartesian coordinate system $O{\lambda }_1{\lambda }_2$, for that the point $({\lambda }_1+\mathrm{\Delta }{\lambda }_1, {\lambda }_2+\mathrm{\Delta }{\lambda }_2)$ can arbitrarily tend to the fixed point $({\lambda }_1, {\lambda }_2)$ when $\mathrm{\Delta }{\lambda }_1$ and $\mathrm{\Delta }{\lambda }_2$ both get near to zero. Correspondingly, ${\$}_j$ can be arbitrarily and infinitely close to ${\$}_i$ in its infinitesimal neighborhood space in the principal frame of the general three-system. Substituting Equation (17) into Equation (16) will degenerate it into the limit of the vector function of one variable as follows:

$$\begin{array}{ll}\mathit{\rho }& =\mathit{P}-\frac{\left\{\left(\mathit{S}\cdot (\frac{\partial \mathit{P}}{\partial {\lambda }_1}+\frac{\partial \mathit{P}}{\partial {\lambda }_2}\frac{d{\lambda }_2}{d{\lambda }_1})\right)\left(\mathit{S}\cdot (\frac{\partial \mathit{S}}{\partial {\lambda }_1}+\frac{\partial \mathit{S}}{\partial {\lambda }_2}\frac{d{\lambda }_2}{d{\lambda }_1})\right)-(\frac{\partial \mathit{S}}{\partial {\lambda }_1}+\frac{\partial \mathit{S}}{\partial {\lambda }_2}\frac{d{\lambda }_2}{d{\lambda }_1})\cdot (\frac{\partial \mathit{P}}{\partial {\lambda }_1}+\frac{\partial \mathit{P}}{\partial {\lambda }_2}\frac{d{\lambda }_2}{d{\lambda }_1})\right\}}{{\left(\mathit{S}\cdot (\frac{\partial \mathit{S}}{\partial {\lambda }_1}+\frac{\partial \mathit{S}}{\partial {\lambda }_2}\frac{d{\lambda }_2}{d{\lambda }_1})\right)}^2-(\frac{\partial \mathit{S}}{\partial {\lambda }_1}+\frac{\partial \mathit{S}}{\partial {\lambda }_2}\frac{d{\lambda }_2}{d{\lambda }_1})\cdot (\frac{\partial \mathit{S}}{\partial {\lambda }_1}+\frac{\partial \mathit{S}}{\partial {\lambda }_2}\frac{d{\lambda }_2}{d{\lambda }_1})}\mathit{S}\\ & =\mathit{P}-\frac{\left((\mathit{S}\cdot \frac{d\mathit{P}}{d{\lambda }_1})(\mathit{S}\cdot \frac{d\mathit{S}}{d{\lambda }_1})-\frac{d\mathit{S}}{d{\lambda }_1}\cdot \frac{d\mathit{P}}{d{\lambda }_1}\right)}{{(\mathit{S}\cdot \frac{d\mathit{S}}{d{\lambda }_1})}^2-\frac{d\mathit{S}}{d{\lambda }_1}\cdot \frac{d\mathit{S}}{d{\lambda }_1}}\mathit{S}\end{array}$$

(18)

Since $\mathit{S}$ is a unit vector, we have $\mathit{S}\cdot (d\mathit{S}/d{\lambda }_1)=0$. So, the above equation reduces to

$$\mathit{\rho }=\mathit{P}-\frac{\frac{d\mathit{S}}{d{\lambda }_1}\cdot \frac{d\mathit{P}}{d{\lambda }_1}}{\frac{d\mathit{S}}{d{\lambda }_1}\cdot \frac{d\mathit{S}}{d{\lambda }_1}}\mathit{S}$$

(19)

For the compactness of the following formulas, we set $h_1=h_3+e+g$, $h_2=h_3+e$, $e>0$, and $g>0$ without a loss of generality. Although Equation (19) is just the formula of the striction point of ruled surfaces, it can be used to derive the ${\infty }^3$ striction points of the general three-system. Substituting $\mathit{S}$, $\mathit{P}$, ${\lambda }_2=f({\lambda }_1)$, and k into Equation (19) and rearranging lead to

$$\mathit{\rho }=\mathit{P}-A_{sp}\mathit{S}$$

(20)

where

$$A_{sp}=\frac{g{\lambda }_1{\lambda }_2{k}^2+\left((e+g){\lambda }_1^2-g{\lambda }_2^2+e\right)k-(e+g){\lambda }_1{\lambda }_2}{\sqrt{1+{\lambda }_1^2+{\lambda }_2^2}\left((1+{\lambda }_1^2)k^2-2{\lambda }_1{\lambda }_{2}k+{\lambda }_2^2+1\right)}$$

(21)

is defined as the distribution coefficient of the ${\infty }^3$ striction points within the general three-system because it involves the three primary variables ${\lambda }_1$, ${\lambda }_2$, and k, and the two parametric variables e and g. In order to analyze the distribution range of the ${\infty }^1$ striction points on the axis of ${\$}_i$, we set ${\lambda }_1$, ${\lambda }_2$, e, and g as the parametric variables and k as the primary variable taking values in the interval $(-\infty ,+\infty )$. According to Equation (21), when the point $({\lambda }_1+\mathrm{\Delta }{\lambda }_1, {\lambda }_2+\mathrm{\Delta }{\lambda }_2)$ tends to the point $({\lambda }_1, {\lambda }_2)$ in different directions, i.e., k takes different values, we will get different striction points on the screw axis ${\$}_L({\lambda }_1, {\lambda }_2)$, i.e., the limit of Equation (16) does not exist in general cases. Setting $A_{sp}=0$ yields

$$k=\frac{g\left({\lambda }_2^2-{\lambda }_1^2\right)-e{\lambda }_1^2-e\pm \sqrt{{\left(e+g\right)}^2{\lambda }_1^4+2\left(e+g\right)\left(g{\lambda }_2^2+e\right){\lambda }_1^2+{\left(e-g{\lambda }_2^2\right)}^2}}{2g{\lambda }_1{\lambda }_2}$$

(22)

This means that there exist two directions along which the screw ${\$}_i({\lambda }_1, {\lambda }_2)$ is infinitely close to its infinitesimal neighboring screws, generating two limit points which are its position vector endpoints. In particular, when ${\lambda }_1=0$ and ${\lambda }_2=0$, substituting them into Equation (21) and setting $A_{sp}=0$ yields $k=0$. When ${\lambda }_1\ne 0$ and ${\lambda }_2=0$, substituting them into Equation (21) and setting $A_{sp}=0$ yields $k=0$. When ${\lambda }_1=0$ and ${\lambda }_2\ne 0$, substituting them into Equation (21) and setting $A_{sp}=0$ yields $k=0$ or ${\lambda }_2=\pm \sqrt{e/g}$.

Remark: 

The screws${\$}_i({\lambda }_1=0, {\lambda }_2=\pm \sqrt{e/g})$are defined as the singular screws which always receive a unique striction point (i.e., their position vector endpoint), whatever values k takes. So, the limit of Equation (16) exists for these singular screws.

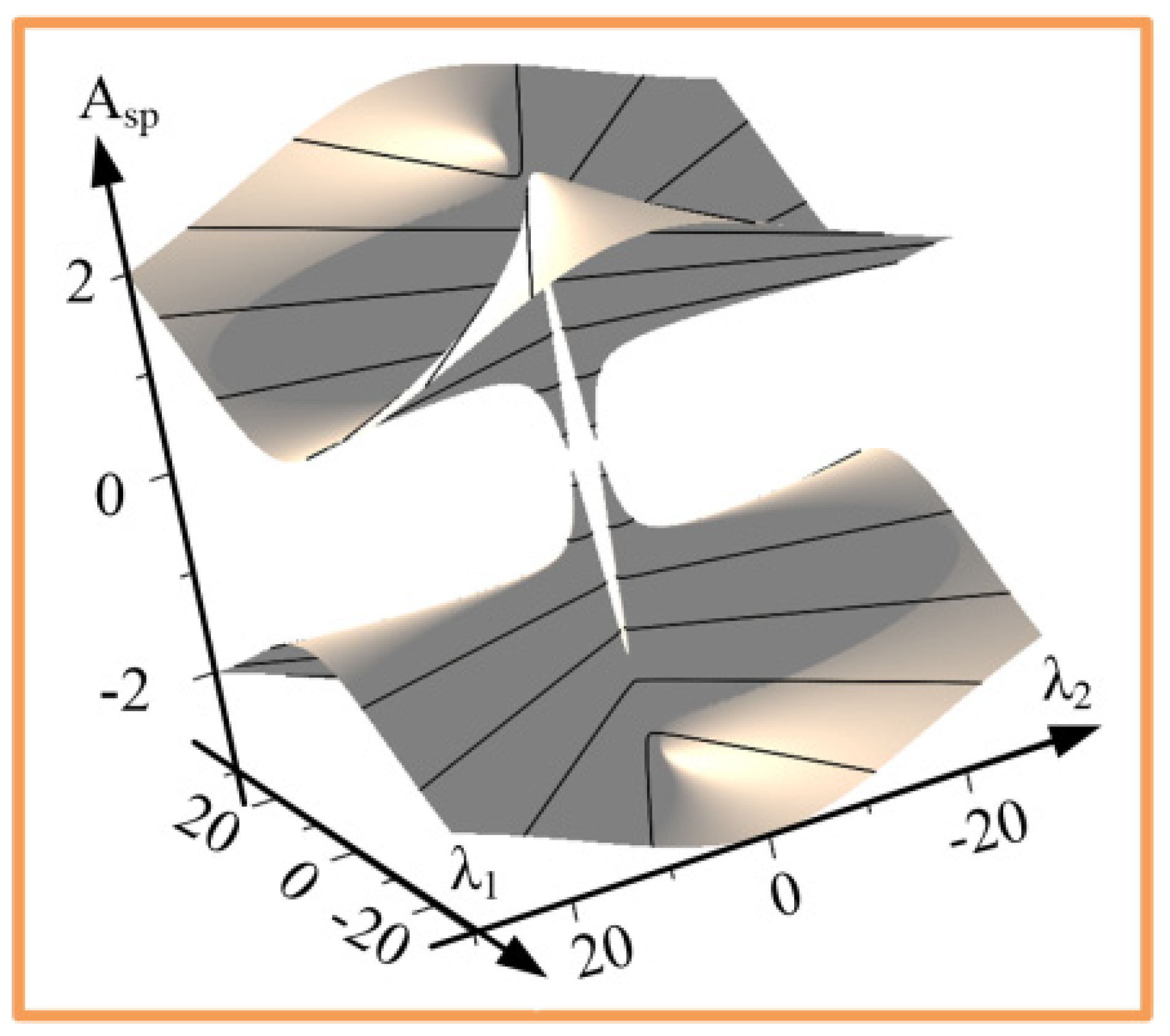
When

$$\begin{array}{ll}k& =\frac{(e+g){\lambda }_1^3{\lambda }_2+\left(g{\lambda }_2^2+e+2g\right){\lambda }_1{\lambda }_2}{(e+g){\lambda }_1^4+\left(g{\lambda }_2^2+2e+g\right){\lambda }_1^2-g{\lambda }_2^2+e}\\ & \pm \frac{\sqrt{\left({(e+g)}^2{\lambda }_1^4+2(e+g)(g{\lambda }_2^2+e){\lambda }_1^2+{(e-g{\lambda }_2^2)}^2\right)(1+{\lambda }_1^2+{\lambda }_2^2)}}{(e+g){\lambda }_1^4+\left(g{\lambda }_2^2+2e+g\right){\lambda }_1^2-g{\lambda }_2^2+e}\end{array}$$

(23)

$A_{sp}$ attains its two extreme values $A_{sp\max }$ and $A_{sp\min }$ as follows:

$$A_{sp\max , sp\min }=\frac{\pm \sqrt{{(e+g)}^2{\lambda }_1^4+2(e+g)(g{\lambda }_2^2+e){\lambda }_1^2+{(e-g{\lambda }_2^2)}^2}}{2(1+{\lambda }_1^2+{\lambda }_2^2)}$$

(24)

where $A_{sp\max , sp\min }$ stands for $A_{sp\max }$ and $A_{sp\min }$, which are associated with the symbol “$+$” and the symbol “$-$” in the symbol “$\pm$”, respectively. Setting $e=4$ and $g=2$, and setting ${\lambda }_1$ and ${\lambda }_2$ as the primary variables, we can get the surface of $A_{sp\max }$ and $A_{sp\min }$ in the Cartesian coordinate system $A_{sp}-{\lambda }_1{\lambda }_2$, as shown in Figure 3.

As seen in Figure 3, the curves on the surfaces are their contour lines. Equations (24) and (20) illustrate that when k takes values in the interval $(-\infty ,+\infty )$, ${\infty }^1$ striction points on every screw axis of the general three-system symmetrically distribute on both sides of the position vector endpoint P, as shown in Figure 4.

As seen in Figure 4, $q_1$ and $q_2$ are defined as the top- and bottom-end striction points, respectively. So far, we can formulate the densest distribution zone of the general three-system as:

$$\mathit{\rho }({\lambda }_1,{\lambda }_2,v)=\mathit{P}({\lambda }_1,{\lambda }_2)-v{A}_{sp\max }({\lambda }_1,{\lambda }_2)\mathit{S}({\lambda }_1,{\lambda }_2),v\in [-1,+1]$$

(25)

where $\mathit{\rho }({\lambda }_1,{\lambda }_2,v)$ is a three-dimensional vector function of three variables in ${\lambda }_1$, ${\lambda }_2$ and v. The variable v partitions the densest distribution zone $\mathit{\rho }({\lambda }_1,{\lambda }_2,v)$ into ${\infty }^1$ striction point surfaces. Among these surfaces, there are three important surfaces. When $v=0$, $\mathit{\rho }({\lambda }_1,{\lambda }_2,0)=\mathit{P}({\lambda }_1,{\lambda }_2)$ holds. We define the position vector endpoint P as the centric striction point. So, the parametric surface $\mathit{P}({\lambda }_1,{\lambda }_2)$ is also called the centric striction point surface (the surface $S^{\perp }$). Clearly, the position vector endpoint P is a particular case of the striction points. When $v=-1$,

$${\mathit{q}}_1({\lambda }_1,{\lambda }_2)=\mathit{\rho }({\lambda }_1,{\lambda }_2,-1)=\mathit{P}({\lambda }_1,{\lambda }_2)+A_{sp\max }({\lambda }_1,{\lambda }_2)\mathit{S}({\lambda }_1,{\lambda }_2)$$

(26)

We define the parametric surface ${\mathit{q}}_1({\lambda }_1,{\lambda }_2)$ as the top-end striction point surface. When $v=1$,

$${\mathit{q}}_2({\lambda }_1,{\lambda }_2)=\mathit{\rho }({\lambda }_1,{\lambda }_2,1)=\mathit{P}({\lambda }_1,{\lambda }_2)-A_{sp\max }({\lambda }_1,{\lambda }_2)\mathit{S}({\lambda }_1,{\lambda }_2)$$

(27)

We define the parametric surface ${\mathit{q}}_2({\lambda }_1,{\lambda }_2)$ as the bottom-end striction point surface. The above two parametric surfaces constitute the boundary surface of the densest distribution zone. Their parametric equations have the form

$${\mathit{q}}_{1,2}({\lambda }_1,{\lambda }_2)=\left(\begin{array}{l}\frac{-2e{\lambda }_1{\lambda }_2\sqrt{1+{\lambda }_1^2+{\lambda }_2^2}\pm \sqrt{{(e+g)}^2{\lambda }_1^4+2(e+g)(g{\lambda }_2^2+e){\lambda }_1^2+{(e-g{\lambda }_2^2)}^2}}{2{(1+{\lambda }_1^2+{\lambda }_2^2)}^{3/2}}\\ \frac{(e+g){\lambda }_2\sqrt{1+{\lambda }_1^2+{\lambda }_2^2}\pm {\lambda }_1\sqrt{{(e+g)}^2{\lambda }_1^4+2(e+g)(g{\lambda }_2^2+e){\lambda }_1^2+{(e-g{\lambda }_2^2)}^2}}{2{(1+{\lambda }_1^2+{\lambda }_2^2)}^{3/2}}\\ \frac{-2g{\lambda }_1\sqrt{1+{\lambda }_1^2+{\lambda }_2^2}\pm {\lambda }_2\sqrt{{(e+g)}^2{\lambda }_1^4+2(e+g)(g{\lambda }_2^2+e){\lambda }_1^2+{(e-g{\lambda }_2^2)}^2}}{2{(1+{\lambda }_1^2+{\lambda }_2^2)}^{3/2}}\end{array}\right)$$

(28)

where ${\mathit{q}}_{1,2}({\lambda }_1,{\lambda }_2)$ stands for ${\mathit{q}}_1({\lambda }_1,{\lambda }_2)$ and ${\mathit{q}}_2({\lambda }_1,{\lambda }_2)$, which are associated with the symbol “$+$” and the symbol “$-$”, respectively, in the symbol “$\pm$”.

By the procedure of the surface $S^{\perp }$ evolving into the boundary surface of the densest distribution zone, we have an insight into its inner structure. In Equation (25), setting $e=4$ and $g=2$ and setting the primary variables ${\lambda }_1$ and ${\lambda }_2$, both continuously taking values in the interval $[-24, 24]$ yield the evolving procedure as shown in the Figure 5(E1–E3,D1–D3,C1–C3,B1–B3,A1–A3), when v takes the values 0, ±0.3, ±0.5, ±0.8, and ±1, respectively. The curves on the surfaces are their contour lines.

To compare the relative location of the five evolutionary surfaces in Figure 5, we set the primary variables ${\lambda }_1$ and ${\lambda }_2$ to take values in the interval $[-d, d]$ and the range parameter d to take the values 0.3, 0.5, 0.8, 1, 1.3, 1.6, 2, 2.5, 3, 6, 12, and 24 in turn, respectively. The growing up process of the five evolutionary surfaces is shown in Figure 6.

From Figure 6, we know that the evolutionary surface associated with a bigger absolute value of v (in blue) enfolds the one associated with a smaller absolute value of v (in blue). In other words, top- and bottom-end striction point surfaces are indeed the boundary of the densest distribution zone. From the above discussion follows at once an important proposition: the striction curve of the ruled surface, which consists of arbitrary ${\infty }^1$ screw axes of the general three-system, must be located inside the densest distribution zone. Figure 6 describes the inner structure of the densest distribution zone in detail.

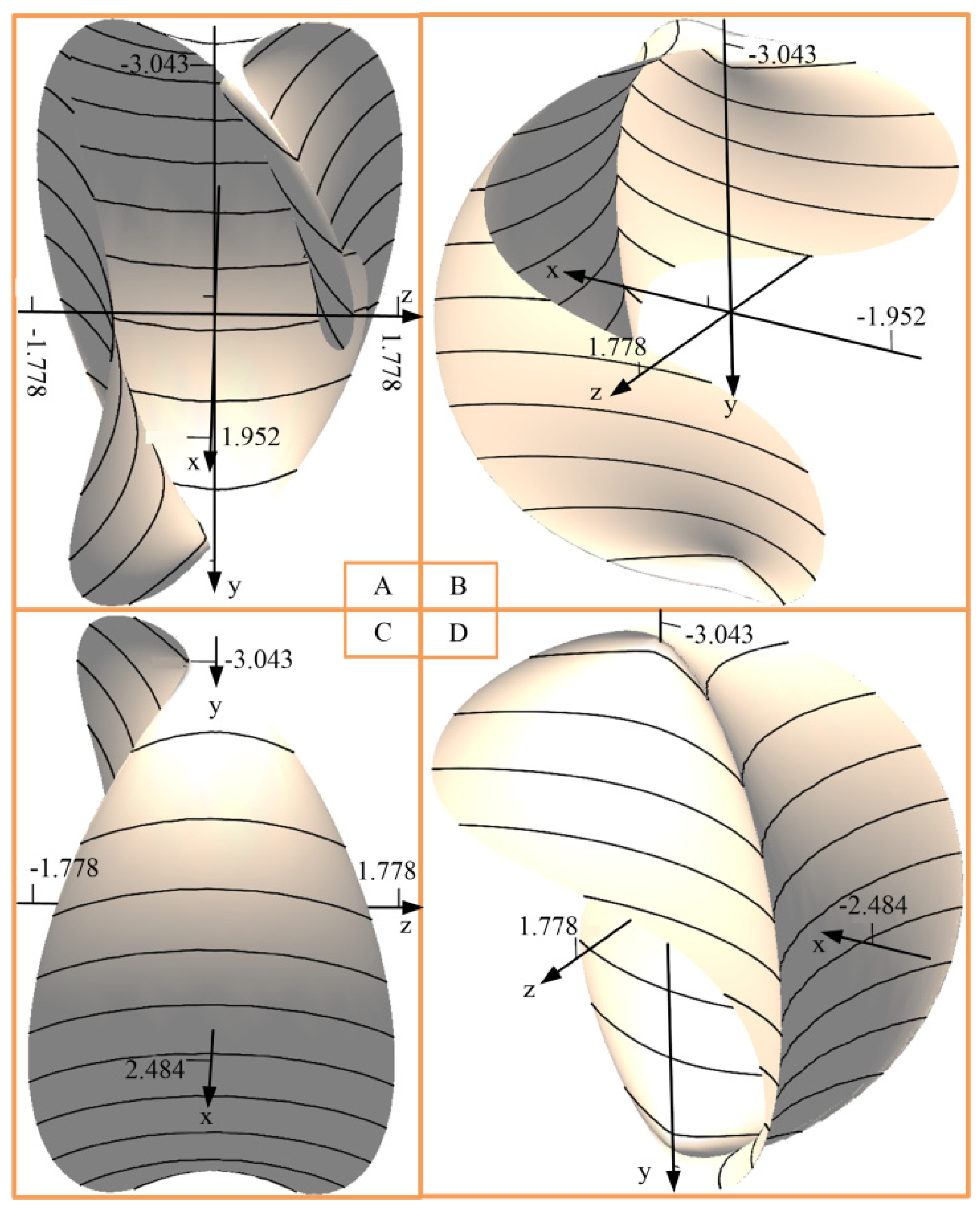
The boundary surface of the densest distribution zone of the general three-system is our original contribution. Its beauteous details are shown in Figure 7 by four close-up views. Figure 7A,B are the top-end striction point surfaces. Figure 7C,D are the bottom-end striction point surfaces.

4. Distribution Regularity of the ${\infty }^1$ Two-Subsystems of the General Three-System

The above two sections discuss the densest distribution zone of the ${\infty }^2$ screw axes of the general three-system in Euclidean three-space and decompose this zone into ${\infty }^1$ striction point surfaces by the primary variable v in Equation (25) to explore its inner structure. In this section, we attempt to establish the relationship between this zone and the cylindroids.

Equation (2) maps the ${\infty }^2$ screws within a general three-system to the ${\infty }^2$ points on the ${\lambda }_1{\lambda }_2$ plane. These points can be partitioned into ${\infty }^1$ straight lines (a family of straight lines). Clearly, every straight line corresponds to a two-subsystem [6] of the general three-system, whose ${\infty }^1$ screw axes lie on a two-subsystem cylindroid (TC), as shown in Figure 8. In other words, the distribution zone of the general three-system can be partitioned into ${\infty }^1$ cylindroids.

Remark: 

A partitioning method corresponds to a family of two-subsystems which consists of${\infty }^1$two-subsystems, and then to a family of straight lines in the${\lambda }_1{\lambda }_2$plane. Any family of straight lines in the${\lambda }_1{\lambda }_2$plane can be expressed as

$${\lambda }_2=k{\lambda }_1+c$$

(29)

where the slope k, primary variable${\lambda }_1$, and intercept c are real numbers and take values in the interval$(-\infty ,+\infty )$. So, the parameter k essentially determines the${\infty }^1$partitioning methods of the general three-system into${\infty }^1$two-subsystems. When the partition method has been given, the parameter c taking values in the interval$(-\infty ,+\infty )$will generate the two-subsystems of this family of two-subsystems. In a two-subsystem, the parameter${\lambda }_1$taking values in the interval$(-\infty ,+\infty )$will generate the${\infty }^1$screws.

In order to decompose the densest distribution zone into cylindroids, we establish the relationship between the cylindroid and the three parameters ${\lambda }_1$, c, and k in theorems 1, 2, and 3, respectively. In Theorem 1, k and c are set as the parametric variables and ${\lambda }_1$ is set as the primary variable.

Theorem 1.

On a certain cylindroid lie the${\infty }^1$screws of a general three-system associated with the${\infty }^1$points on any straight line of the${\lambda }_1{\lambda }_2$plane, while on two planar pencils lie those associated with the${\infty }^1$points on the two exceptional straight lines${\lambda }_2=\pm \sqrt{g/e}$and${\lambda }_1\in (-\infty ,+\infty )$, respectively.

Proof. 

Substituting Equation (29) into Equation (2) and rearranging lead to the unit Plücker coordinate of any screw within this two-subsystem, as follows:

$$\begin{array}{ll}{\$}_i=& (\frac{1}{\sqrt{(1+k^2){\lambda }_1^2+2ck{\lambda }_1+c^2+1}}, \frac{{\lambda }_1}{\sqrt{(1+k^2){\lambda }_1^2+2ck{\lambda }_1+c^2+1}}, \frac{k{\lambda }_1+c}{\sqrt{(1+k^2){\lambda }_1^2+2ck{\lambda }_1+c^2+1}};\\ & \frac{h_1}{\sqrt{(1+k^2){\lambda }_1^2+2ck{\lambda }_1+c^2+1}}, \frac{{\lambda }_1{h}_2}{\sqrt{(1+k^2){\lambda }_1^2+2ck{\lambda }_1+c^2+1}}, \frac{(k{\lambda }_1+c)h_3}{\sqrt{(1+k^2){\lambda }_1^2+2ck{\lambda }_1+c^2+1}})\end{array}$$

(30)

When the primary variable is ${\lambda }_1\in (-\infty ,+\infty )$, the screw axis of ${\$}_i$ traces a ruled surface whose striction curve has the following parametric equation:

$${\mathit{\rho }}_1=\left(\begin{array}{c}\frac{-(c^2+k^2+1)ek{\lambda }_1{}^2+((g-e)k^2-e{c}^2+g)c{\lambda }_1+(g{c}^2-e)k}{(c^2+k^2+1)((k^2+1){\lambda }_1{}^2+2ck{\lambda }_1+c^2+1}\\ \frac{(g{k}^2+e+g)c{\lambda }_1{}^2+((e+2g)c^2+(e+g)k^2+g)k{\lambda }_1+c(e+g)(c^2+(k^2+1))}{(c^2+k^2+1)((k^2+1){\lambda }_1{}^2+2ck{\lambda }_1+c^2+1)}\\ \frac{(g{k}^2+e+g)ck{\lambda }_1{}^2+((2g{c}^2-e-g)k^2+e{c}^2-g){\lambda }_1+(g{c}^2-e)ck}{(c^2+k^2+1)((k^2+1){\lambda }_1{}^2+2ck{\lambda }_1+c^2+1)}\end{array}\right)$$

(31)

From Equation (31), we know that when

$$\begin{array}{ll}{\lambda }_1=& {\lambda }_{1\min , 1\max }=\frac{-(e+g)c{k}^3-\left(e{c}^2+2e+g\right)ck}{(e+g)k^4+\left(e{c}^2+e+2g\right)k^2-e{c}^2+g}\\ & \frac{\pm \sqrt{\left({(e+g)}^2{k}^4+2(e+g)(e{c}^2+g)k^2+{\left(e{c}^2-g\right)}^2\right)(1+c^2+k^2)}}{(e+g)k^4+\left(e{c}^2+e+2g\right)k^2-e{c}^2+g}\end{array}$$

(32)

The striction curve ${\mathit{\rho }}_1$ attains its two extreme endpoints ${\mathit{\rho }}_{1\min }$ and ${\mathit{\rho }}_{1\max }$, respectively, in the principal frame, and ${\lambda }_{1\min , 1\max }$ stands for ${\lambda }_{1\min }$ and ${\lambda }_{1\max }$, which are associated with the symbol “$+$” and the symbol “$-$” in the symbol “$\pm$”, respectively. Setting the length of the secant vector ${\mathit{\rho }}_{1\max }-{\mathit{\rho }}_{1\min }$ of the striction curve ${\mathit{\rho }}_1$ as A, we have

$$A^2=\frac{{(e+g)}^2{k}^4+2(e+g)(e{c}^2+g)k^2+{(e{c}^2-g)}^2}{{(c^2+k^2+1)}^2}$$

(33)

Setting $A=0$ and $k=0$, and solving the above equation for c lead to $c=\pm \sqrt{g/e}$. This means the striction curves of the ruled surfaces associated with the lines ${\lambda }_2=\pm \sqrt{g/e}, {\lambda }_1\in (-\infty ,+\infty )$ degenerate into the two points $(0,\pm \sqrt{eg},0)$, respectively. Further computing finds that the screws $({\$}_1^p\pm \sqrt{g/e}{\$}_3^p)$ and the screw ${\lambda }_1{\$}_2^p$ have the same pitch $h_2$, and they constitute the basis screws of the first special two-systems [4]. In other words, the screws associated with lines ${\lambda }_2=\pm \sqrt{g/e}$ form two first special two-systems, respectively. So, the screws associated with the exceptional lines ${\lambda }_2=\pm \sqrt{g/e}$ lie on two planar pencils centered at points $(0,\pm \sqrt{eg},0)$, respectively.

Setting $A=0$ and $k\ne 0$ and solving Equation (33) for $k^2$ leads toand solving Equation (33) for

$$k^2=\frac{-e{c}^2-g+2c\sqrt{eg}}{e+g}\le \frac{-2\left|c\right|\sqrt{eg}+2c\sqrt{eg}}{e+g}=\frac{2\sqrt{eg}(c-\left|c\right|)}{e+g}\le 0$$

(34)

and

$$k^2=\frac{-e{c}^2-g-2c\sqrt{eg}}{e+g}\le \frac{-2\left|c\right|\sqrt{eg}-2c\sqrt{eg}}{e+g}=\frac{-2\sqrt{eg}(c+\left|c\right|)}{e+g}\le 0$$

(35)

The above two solutions both contradict with k² > 0. That means there are no more straight lines in the ${\lambda }_1{\lambda }_2$ plane associated with the ruled surfaces satisfying the condition $A=0$. When $A\ne 0$, the straight line determined by the unit vector ${\mathit{s}}_1=(-c,-k,1)/\sqrt{1+c^2+k^2}$ of the secant vector ${\mathit{\rho }}_{1\max }-{\mathit{\rho }}_{1\min }$ and the point ${\mathit{\rho }}_1({\lambda }_1=0)$ has the form

$$\mathit{r}\times \frac{(-c,-k,1)}{\sqrt{1+c^2+k^2}}=\left(\frac{(g{k}^2+e+g)c}{{(c^2+k^2+1)}^{3/2}},\frac{(e-g{c}^2)k}{{(c^2+k^2+1)}^{3/2}},\frac{(e+g)c^2+e{k}^2}{{(c^2+k^2+1)}^{3/2}}\right)$$

(36)

Since all points in Equation (31) satisfy the above equation, the striction curve ${\mathit{\rho }}_1({\lambda }_1)$ is a straight line. In addition, the two screws ${\$}_i({\lambda }_1={\lambda }_{1\min , 1\max })$ passing through the two extreme endpoints ${\mathit{\rho }}_{1\max }$ and ${\mathit{\rho }}_{1\min }$, respectively, are mutually perpendicular and are both perpendicular to the striction line ${\mathit{\rho }}_1({\lambda }_1)$. When

$${\lambda }_1={\lambda }_{mid1, mid2}=\frac{e(k^2-c^2)+g(1+k^2)\pm \sqrt{{(e+g)}^2{k}^4+2(e+g)(e{c}^2+g)k^2+{(e{c}^2-g)}^2}}{2eck}$$

(37)

the striction line ${\mathit{\rho }}_1({\lambda }_1)$ attains the midpoint ${\mathit{\rho }}_{1mid}$ of the two extreme endpoints ${\mathit{\rho }}_{1\max }$ and ${\mathit{\rho }}_{1\min }$ twice, and ${\lambda }_{mid1, mid2}$ stands for ${\lambda }_{mid1}$ and ${\lambda }_{mid2}$, which are associated with the symbol “$+$” and the symbol “$-$” in the symbol “$\pm$”, respectively. In particular, if k and c make the denominator $2eck$ of ${\lambda }_{mid1, mid2}$ equal to zero, then ${\lambda }_{mid1}=0$ and ${\lambda }_{mid2}=\pm \infty$. Furthermore, the two screws ${\$}_i({\lambda }_1={\lambda }_{mid1, mid2})$ and the striction line ${\mathit{\rho }}_1({\lambda }_1)$ are mutually and orthogonally concurrent at the midpoint ${\mathit{\rho }}_{1mid}$ of the striction line ${\mathit{\rho }}_1({\lambda }_1)$. Clearly, ${\$}_i({\lambda }_1={\lambda }_{mid1, mid2})$ are two principal screws and ${\$}_i({\lambda }_1={\lambda }_{1\min , 1\max })$ are two end screws [4], respectively. To summarize, the ruled surface traced by ${\$}_i$ possesses all the geometric features of a cylindroid. Therefore, Theorem 1 is proven. The cylindroid constructed in Theorem 1 is defined as the two-subsystem cylindroid (TC). □

In Theorem 2, k is set as a parametric variable and ${\lambda }_1$ and c are set as the primary variables. The ${\infty }^1$ lines with the same slope k in the ${\lambda }_1{\lambda }_2$ plane correspond to a certain family of two-subsystems.

Theorem 2.

On a certain cylindroid lie the${\infty }^1$spine lines associated with the${\infty }^1$two-subsystem cylindroids within any family of two-subsystems of a general three-system.

Proof. 

The unit Plücker coordinate of the spine line of a two-subsystem cylindroid has the form

$$\begin{array}{ll}{\$}_1^s& =({\mathit{s}}_1,{\mathit{\rho }}_1({\lambda }_1=0)\times {\mathit{s}}_1)\\ & =\frac{1}{\sqrt{c^2+k^2+1}}(-c,-k, 1; \frac{(g{k}^2+e+g)c}{c^2+k^2+1},\frac{(e-g{c}^2)k}{c^2+k^2+1},\frac{(e+g)c^2+e{k}^2}{c^2+k^2+1})\end{array}$$

(38)

where the superscript s represents the spine line. When c takes values in the interval $(-\infty ,+\infty )$, ${\$}_1^s$ traces a spine-ruled surface in the principal frame whose striction curve has the form

$${\mathit{\rho }}_2=\left(\frac{-ek}{k^2+1}, \frac{\left(g{k}^2+e+g\right)c}{\left(k^2+1\right)\left(c^2+k^2+1\right)}, \frac{\left(g{k}^2+e+g\right)ck}{\left(k^2+1\right)\left(c^2+k^2+1\right)}\right)$$

(39)

When $c=\pm \sqrt{k^2+1}$, the striction curve ${\mathit{\rho }}_2$ attains its two extreme endpoints ${\mathit{\rho }}_{2\max }$ and ${\mathit{\rho }}_{2\min }$ as follows:

$${\mathit{\rho }}_{2\max , 2\min }=\pm \left(\mp \frac{ek}{k^2+1}, \frac{g{k}^2+e+g}{2{\left(k^2+1\right)}^{3/2}}, \frac{\left(g{k}^2+e+g\right)k}{2{\left(k^2+1\right)}^{3/2}}\right)$$

(40)

where ${\mathit{\rho }}_{2\max , 2\min }$ stands for ${\mathit{\rho }}_{2\max }$ and ${\mathit{\rho }}_{2\min }$, which are associated with the symbol “$+$” and the symbol “$-$” in the symbol “$\pm$”, respectively, and are associated with the symbol “$-$” and the symbol “$+$” in the symbol “$\mp$”, respectively. Setting the length of the secant vector ${\mathit{\rho }}_{2\max }-{\mathit{\rho }}_{2\min }$ of the striction curve ${\mathit{\rho }}_2$ as A leads to

$$A^2=\frac{{\left(g{k}^2+e+g\right)}^2}{{\left(k^2+1\right)}^2}$$

(41)

Clearly, $A>0$ holds for any value of k. The straight line determined by the unit vector ${\mathit{s}}_2=(0,1,k)/\sqrt{1+k^2}$ of the secant vector ${\mathit{\rho }}_{2\max }-{\mathit{\rho }}_{2\min }$ and the point ${\mathit{\rho }}_2(c=0)$ has the form

$$\mathit{r}\times \frac{(0,1,k)}{\sqrt{1+k^2}}=\left(0,\frac{e{k}^2}{{(k^2+1)}^{3/2}},\frac{-ek}{{(k^2+1)}^{3/2}}\right)$$

(42)

Since all points in Equation (39) satisfy the above equation, the striction curve of the spine-ruled surface is a straight line. In addition, the two screws ${\$}_1^s(c=\pm \sqrt{k^2+1})$ passing through the two extreme endpoints ${\mathit{\rho }}_{2\max }$ and ${\mathit{\rho }}_{2\min }$, respectively, are mutually perpendicular and are both perpendicular to the striction line ${\mathit{\rho }}_2(c)$. The striction points ${\mathit{\rho }}_2(c=\pm \infty , 0)=(-ek/(k^2+1), 0, 0)$ are both the midpoints of the two extreme endpoints ${\mathit{\rho }}_2(c=\pm \sqrt{k^2+1})$. The line vectors ${\$}_1^s(c=\pm \infty )$ and ${\$}_1^s(c=0)$ have the direction vectors $(\pm 1, 0, 0)$ and $(0, -k/\sqrt{k^2+1}, 1/\sqrt{k^2+1})$, respectively. Moreover, these two line vectors, ${\$}_1^s(c=\pm \infty )$ and ${\$}_1^s(c=0)$, and the striction line ${\mathit{\rho }}_2(c)$ are mutually and orthogonally concurrent at the midpoint ${\mathit{\rho }}_2(c=\pm \infty , 0)$ of the striction line ${\mathit{\rho }}_2(c)$. Clearly, ${\$}_1^s(c=\pm \infty )$ and ${\$}_1^s(c=0)$ are two principal screws, and ${\$}_1^s(c=\pm \sqrt{k^2+1})$ are two end screws, respectively. To summarize, the spine-ruled surface traced by ${\$}_1^s$ possesses all the geometric features of a cylindroid. Therefore, Theorem 2 is proven. The cylindroid constructed in Theorem 2 is defined as the first-order spine cylindroid (FSC). □

In Theorem 3, k, ${\lambda }_1$, and c are set as the primary variables. We will get the ${\infty }^1$ families of two-subsystems within a general three-system.

Theorem 3.

On a certain cylindroid lie the${\infty }^1$spine lines associated with the${\infty }^1$first-order spine cylindroids within a general three-system.

Proof. 

The unit Plücker coordinate of the spine line of a first-order spine cylindroid has the form

$${\$}_2^s=({\mathit{s}}_2,{\mathit{\rho }}_2(c=0)\times {\mathit{s}}_2)=\left(0, \frac{1}{\sqrt{k^2+1}}, \frac{k}{\sqrt{k^2+1}}; 0, \frac{e{k}^2}{{(k^2+1)}^{3/2}}, \frac{-ek}{{(k^2+1)}^{3/2}}\right)$$

(43)

When k takes values in the interval $(-\infty ,+\infty )$, ${\$}_2^s$ traces another spine-ruled surface whose striction curve has the form

$${\mathit{\rho }}_3=\left(-ek/(k^2+1), 0, 0\right)$$

(44)

When $k=\pm 1$, the striction curve ${\mathit{\rho }}_3(k)$ attains its two extreme endpoints ${\mathit{\rho }}_{3\min }$ and ${\mathit{\rho }}_{3\max }$ as follows:

$${\mathit{\rho }}_{3\min , 3\max }=\mp \left(e/2, 0, 0\right)$$

(45)

where ${\mathit{\rho }}_{3\min , 3\max }$ stands for ${\mathit{\rho }}_{3\min }$ and ${\mathit{\rho }}_{3\max }$, which are associated with the symbol “$-$” and the symbol “$+$” in the symbol “$\mp$”, respectively. Clearly, the length of the secant vector ${\mathit{\rho }}_{3\max }-{\mathit{\rho }}_{3\min }$ is e, which is greater than zero forever. The straight line determined by the unit vector ${\mathit{s}}_3=(1,0,0)$ of the secant vector ${\mathit{\rho }}_{3\max }-{\mathit{\rho }}_{3\min }$ and the point ${\mathit{\rho }}_3(k=0)$ has the form

$$\mathit{r}\times (1,0,0)=(0,0,0)$$

(46)

Since all points in Equation (44) satisfy the above equation, the striction curve of the spine-ruled surface is a straight line. In addition, the two screws ${\$}_2^s(k=\pm 1)$ passing through the two extreme endpoints ${\mathit{\rho }}_{3\max }$ and ${\mathit{\rho }}_{3\min }$, respectively, are perpendicular mutually and are both perpendicular to the striction line ${\mathit{\rho }}_3(k)$. The striction points ${\mathit{\rho }}_3(k=\pm \infty , 0)=0$ are the midpoints of the two extreme endpoints ${\mathit{\rho }}_3(k=\pm 1)$. The line vectors ${\$}_2^s(k=\pm \infty )$ and ${\$}_2^s(k=0)$ have the direction vector $(0, 0, \pm 1)$ and $(0, 1, 0)$, respectively. So, the two line vectors, ${\$}_2^s(k=\pm \infty )$ and ${\$}_2^s(k=0)$, and the striction line ${\mathit{\rho }}_3(k)$ are mutually and orthogonally concurrent at the midpoint ${\mathit{\rho }}_3(k=\pm \infty , 0)=0$ of the striction line ${\mathit{\rho }}_3(k)$. Clearly, ${\$}_2^s(k=\pm \infty )$ and ${\$}_2^s(k=0)$ are the two principal screws and ${\$}_2^s(k=\pm 1)$ are the two end screws. To summarize, the spine-ruled surface traced by ${\$}_2^s$ possesses all the geometric features of a cylindroid. Therefore, Theorem 3 is proven. The cylindroid constructed in Theorem 3 is defined as the second-order spine cylindroid (SSC). □

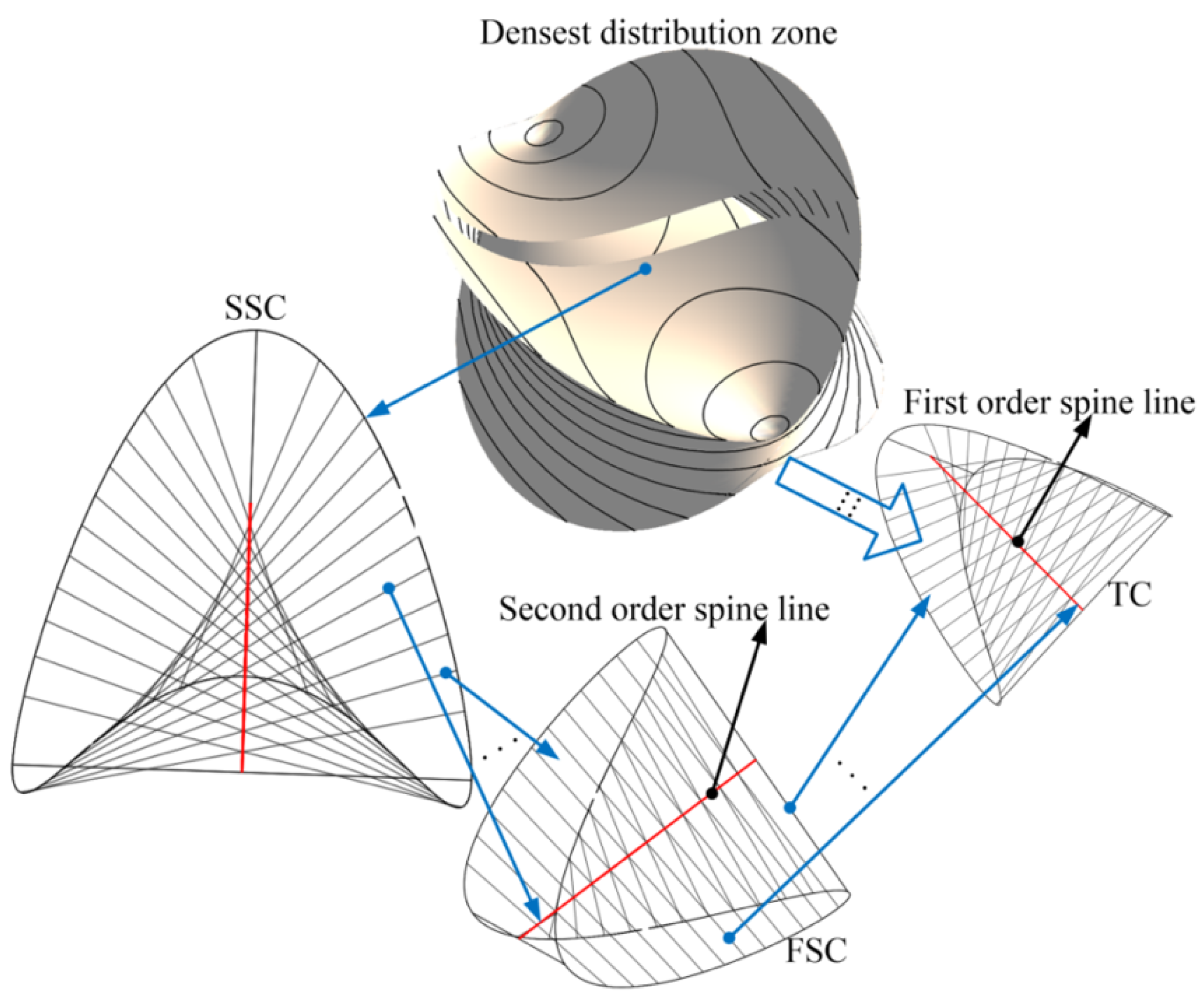
The above three theorems reveal the principle of constructing the densest distribution zone of ${\infty }^2$ screw axes of the general three-system by cylindroids of the general two-system in Euclidean three-space, as shown in Figure 9.

The densest distribution zone of the general three-system can be decomposed into ${\infty }^1$ TCs, whose spine lines constitute an FSC. There are actually ${\infty }^1$ decomposition methods corresponding to ${\infty }^1$ FSCs, whose spine lines constitute the SSC. The above decomposition procedure is shown in Figure 9 in detail.

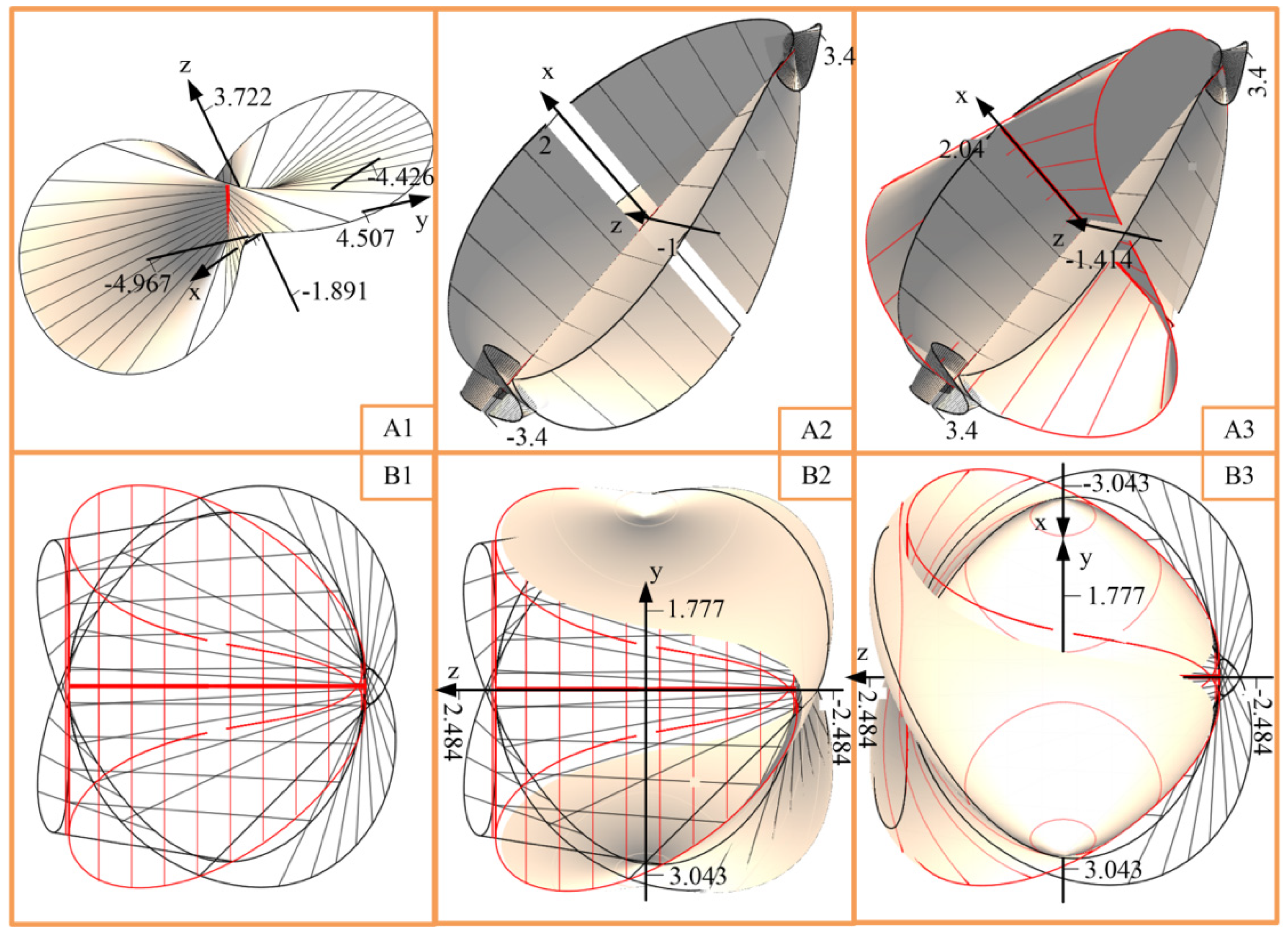
Setting $h_1=7$, $h_2=5$, and $h_3=1$, Figure 10 depicts the relative locations among TCs, FSCs, and SSC in the densest distribution zone of the general three-system.

Figure 10(A1) shows the TC corresponding to the two-subsystem associated with the line ${\lambda }_2=6{\lambda }_1+5$ in the ${\lambda }_1{\lambda }_2$ plane. The length of the segment lying on the TC represents twice the pitch of the screw passing through the line. Figure 10(A2) shows the FSC corresponding to the family of two-subsystems associated with the family of straight lines ${\lambda }_2=0\times {\lambda }_1+c$ in the ${\lambda }_1{\lambda }_2$ plane. The segments lying on the FSC are the spine lines of the TCs within the above family of two-subsystems. The spine lines of the two TCs (${\lambda }_2=0\times {\lambda }_1\pm 1$) are coincident with the two end screw axes of the FSC, respectively. Figure 10(A3) shows the SSC (in red curves and lines). The red segments lying on the SSC are the spine lines of the FSCs corresponding to all the families of two-subsystems within the general three-system ($h_1=7$, $h_2=5$, and $h_3=1$). The spine line of the FSC (${\lambda }_2=0\times {\lambda }_1+c$) is coincident with the principal screw axis of the SSC. Figure 10(B1) shows the SSC (in red curves and lines) and two FSCs (${\lambda }_2=\pm {\lambda }_1+c$) whose spine lines are coincident with the two end screw axes of the SSC, respectively. The three cylindroids are represented by boundary curves and segments lying on the surface. Figure 10(B2,B3) show that the boundary surface of the densest distribution zone completely wraps the three cylindroids shown in Figure 10(B1). So, the proposition in the end of Section 3 is verified again geometrically.

Hunt and Phillips [5,28] presented a distribution characteristic about the central plane of the two-subsystem cylindroid (see Theorem 4). In this section, we try to prove it with a simple computation.

Theorem 4.

Through the origin of the principal frame passes every central plane of TC (including degenerated planar pencils) within a general three-system.

Proof. 

Substituting the straight line ${\lambda }_2={\lambda }_{1}k+c$ into Equation (2) leads to the Plücker coordinate of any screw within a two-subsystem, as follows:

$${\$}_i=\left(1, {\lambda }_1, {\lambda }_{1}k+c; h_1, {\lambda }_1{h}_2, ({\lambda }_{1}k+c)h_3\right)$$

(47)

When $k=0$ and $c=\pm \sqrt{g/e}$, the above equation reduces to

$${\$}_i=\left(1, {\lambda }_1, \pm \sqrt{g/e}; h_1, {\lambda }_1{h}_2, \pm \sqrt{g/e}{h}_3\right)$$

(48)

Clearly, the pitch $h_i$ of ${\$}_i$ is equal to $h_2$ for any value of ${\lambda }_1$. So, the screws represented by Equation (48) belong to the first special two-system. Since these screws lie on a concentric planar pencil, the planar pencil is coincident with the central plane of this two-subsystem. Without a loss of generality, we take two screws as follows:

$$\begin{array}{l}{\$}_i({\lambda }_1=1)=\left(1, 1, \pm \sqrt{g/e}; h_1, h_2, \pm \sqrt{g/e}{h}_3\right)\\ {\$}_i({\lambda }_1=2)=\left(1, 2, \pm \sqrt{g/e}; h_1, 2h_2, \pm \sqrt{g/e}{h}_3\right)\end{array}$$

(49)

and then the normal vector of the central plane has the form

$$n=\left(\mp \sqrt{eg}/\sqrt{(2e+g)(5e+g)}, 0, e/\sqrt{(2e+g)(5e+g)}\right)$$

(50)

In addition, the equation of the central plane [29] has the form

$$r\cdot n=r_0\cdot n=0$$

(51)

where $r_0=(\mp e\sqrt{eg}/(2e+g), \pm (e+g)\sqrt{eg}/(2e+g), \mp eg/(2e+g))$ is the position vector endpoint of ${\$}_i({\lambda }_1=1)$. The origin $r=(0,0,0)$ of the principal frame satisfies the above equation of the central plane. When the screw axes of a two-subsystem lie on a cylindroid, the pitch h_i of any screw has the form

$$h_i=\frac{(k^2{h}_3+h_2){\lambda }_1^2+2ck{h}_3{\lambda }_1+c^2{h}_3+h_1}{(k^2+1){\lambda }_1^2+2ck{\lambda }_1+c^2+1}$$

(52)

Differentiating the above equation with respect to ${\lambda }_1$ leads to

$$h_i^{\text{'}}=\frac{2eck{\lambda }_1^2+2(e{c}^2-(e+g)k^2-g){\lambda }_1-2ck(e+g)}{{((k^2+1){\lambda }_1^2+2ck{\lambda }_1+c^2+1)}^2}$$

(53)

Setting ${h^{\prime }}_i=0$, we receive the two stationary points

$${\lambda }_{1\max , 1\min }=\frac{(k^2-c^2)e+g{k}^2+g\pm \sqrt{{(e+g)}^2{k}^4+2(e+g)(e{c}^2+g)k^2+{(e{c}^2-g)}^2}}{2eck}$$

(54)

where ${\lambda }_{1\max , 1\min }$ stands for ${\lambda }_{1\max }$ and ${\lambda }_{1\min }$, which are associated with the symbol “$+$” and the symbol “$-$” in the symbol “$\pm$”, respectively. Substituting them into Equation (47) yields the two principal screws ${\$}_1^p$ and ${\$}_2^p$(see the ordinary case of Appendix A). In particular, if k and c make the denominator $2eck$ of ${\lambda }_{1\max , 1\min }$ equal to zero, then ${\lambda }_{1\max }=0$ and ${\lambda }_{1\min }=\pm \infty$. For the above three particular cases, three pairs of principal screws are listed in the particular cases of Appendix A. The central plane determined by the above four pairs of principal screws has the form

$$r\cdot n=r_0\cdot n=0$$

(55)

where the normal vector $n=(-c,-k,1)/\sqrt{1+c^2+k^2}$, $r_0$ is the position vector endpoint of ${\$}_1^p$. The origin $r=(0,0,0)$ of the principal frame satisfies the above equation. Therefore, Theorem 4 is proven. □

The above four theorems exactly describe the geometrical regularity of the distribution of all two-subsystems in Euclidean three-space. They will provide exact guides for the axode planning of three degree-of-freedom parallel mechanisms in its reference frame.

5. Varying-Pitch Ruled Surface

The distribution zone of the general three-system can also be decomposed into ${\infty }^1$ pitch-hyperboloids. Furthermore, cylindroids and pitch-hyperboloids can be regarded as two particular decomposition methods of the distribution zone of the general three-system. In this section, we will propose a generalized decomposition method: varying-pitch ruled surface based on the pitch-hyperboloid.

It is well known that screws with the same pitch value lie on the same pitch-hyperboloid [4,5]. According to the pitch value, the distribution space of the ${\infty }^2$ screw axes within a general three-system can be partitioned into ${\infty }^1$ pitch-hyperboloids, two concentric planar pencils, and two coordinate axes of the principal frame. Setting the pitch $h_i$ as a parametric variable, Equation (3) can be rewritten as

$${\lambda }_1^2(h_2-h_i)+{\lambda }_2^2(h_3-h_i)=h_i-h_1$$

(56)

When $h_i=h_2$, Equation (56) degenerates into two lines ${\lambda }_2=\pm \sqrt{g/e}, {\lambda }_1\in (-\infty ,+\infty )$. Considering the proof of Theorem 1, we know that screws with pitch $h_2$ lie on two concentric planar pencils. When $h_i=h_1$, Equation (56) degenerates into the origin of the ${\lambda }_1{\lambda }_2$ plane, i.e., the screw with pitch $h_1$ lies on the x-axis of the principal frame. When $h_i=h_3$, Equation (56) does not hold, but the screw with pitch $h_3$ lies on the z-axis.

When $h_i\in (h_2,h_1)$, $(h_i-h_1)/(h_2-h_i)>0$ and $(h_i-h_1)/(h_3-h_i)>0$ hold. Equation (56) can be rewritten as the stand equation of the family of ellipses as follows:

$$\frac{{\lambda }_1^2}{\frac{h_i-h_1}{h_2-h_i}}+\frac{{\lambda }_2^2}{\frac{h_i-h_1}{h_3-h_i}}=1$$

(57)

Their function expressions have the form

$${\lambda }_2=\pm \sqrt{\frac{{\lambda }_1^2\left(h_2-h_i\right)+\left(h_1-h_i\right)}{h_i-h_3}} {\lambda }_1\in (-\sqrt{\frac{h_i-h_1}{h_2-h_i}},\sqrt{\frac{h_i-h_1}{h_2-h_i}})$$

(58)

When $h_i\in (h_3,h_2)$, $(h_i-h_1)/(h_i-h_2)>0$ and $(h_i-h_1)/(h_3-h_i)>0$ hold. Equation (56) can be rewritten as the stand equation of the family of hyperbolas as follows:

$$\frac{{\lambda }_2^2}{\frac{h_i-h_1}{h_3-h_i}}-\frac{{\lambda }_1^2}{\frac{h_i-h_1}{h_i-h_2}}=1$$

(59)

Their function expressions have the form

$${\lambda }_2=\pm \sqrt{\frac{{\lambda }_1^2\left(h_2-h_i\right)+\left(h_1-h_i\right)}{h_i-h_3}} {\lambda }_1\in (-\infty ,+\infty )$$

(60)

Setting $h_1=7$, $h_2=5$, and $h_3=1$, the curves represented by Equation (56) are plotted in the ${\lambda }_1{\lambda }_2$ plane when the parametric variable $h_i$ takes values in the interval $[h_3,h_1]$, as shown in Figure 11.

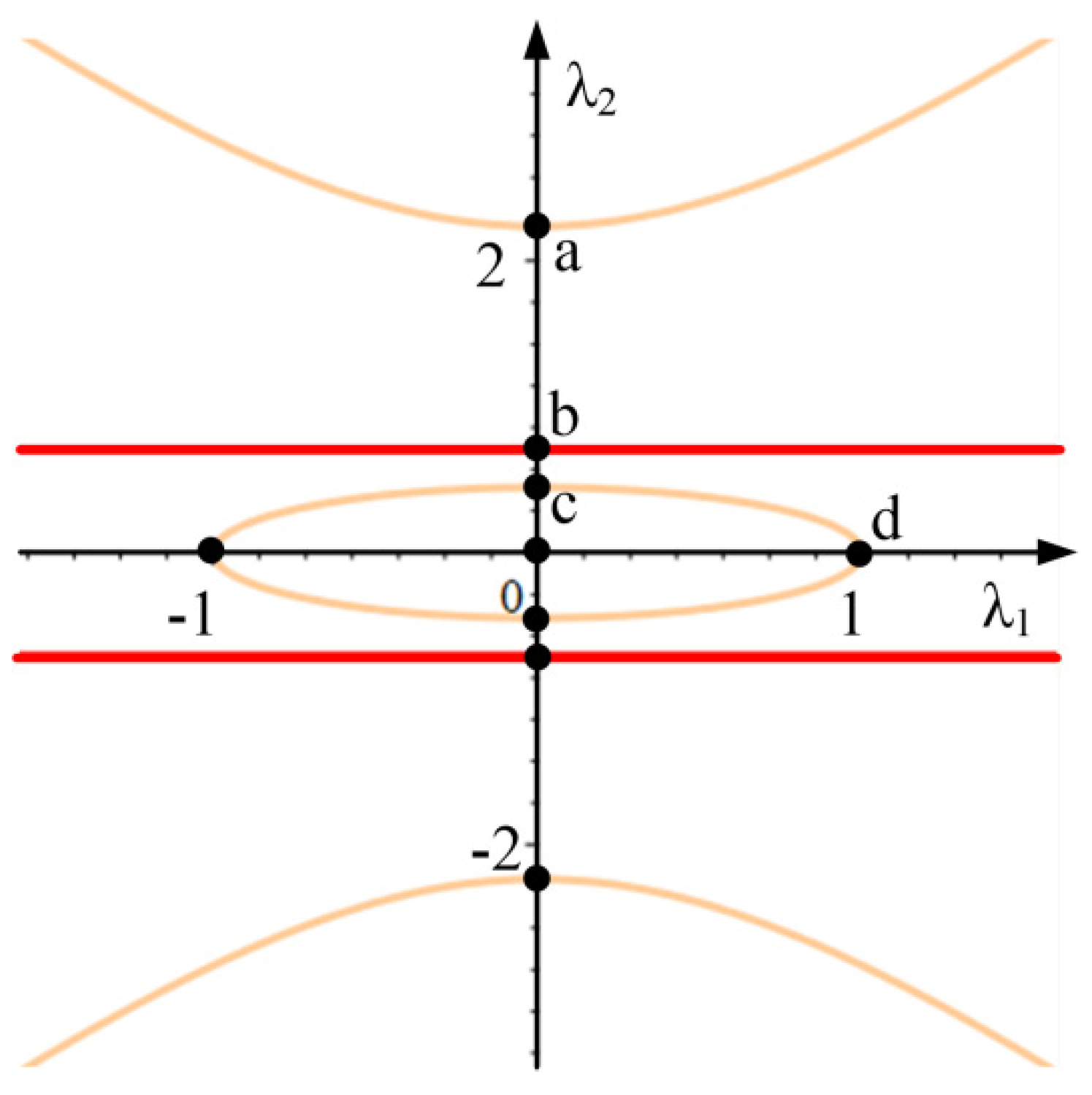
In Figure 11, the top endpoint a of the major axis of the hyperbola is at $(0, \sqrt{(h_1-h_i)/(h_i-h_3)})$. The intersection b of the straight line ${\lambda }_2=\sqrt{g/e}$ and the ${\lambda }_2$-axis is at $(0, \sqrt{g/e})$. When $h_i$ infinitely approaches $h_2$, point a infinitely tends to b, and the slope of the asymptotes of the hyperbola infinitely tends to zero. That means the top half of the hyperbola gradually becomes the line ${\lambda }_2=\sqrt{g/e}$. When $h_i$ infinitely approaches $h_3$, point a infinitely tends to the positive infinity of the ${\lambda }_2$-axis. That means the regions above the line ${\lambda }_2=\sqrt{g/e}$ and below the line ${\lambda }_2=-\sqrt{g/e}$ can be completely covered by the family of hyperbolas. The top endpoint c of the ellipse’s minor axis is at $(0, \sqrt{(h_1-h_i)/(h_i-h_3)})$. The right endpoint d of the ellipse’s major axis is at $(\sqrt{(h_i-h_1)/(h_2-h_i)}, 0)$. When $h_i$ infinitely approaches $h_1$, points c and d both infinitely tend to the origin of the ${\lambda }_1{\lambda }_2$ plane. When $h_i$ infinitely approaches $h_2$, point c infinitely tends to point b, and point d infinitely tends to the positive infinity of the ${\lambda }_1$-axis. That means the region between the two lines ${\lambda }_2=\pm \sqrt{g/e}$ can be completely covered by the family of ellipses. To summarize, the ${\infty }^2$ points of the ${\lambda }_1{\lambda }_2$ plane can be completely covered by the curves represented by Equation (56) when $h_i$ continuously takes values in the interval $[h_3,h_1]$.

Substituting Equation (58) and Equation (60) into Equation (20) yields the parametric equation of the striction curve of the ruled surface on which lie screws with the pitches $h_2<h_i<h_1$ and $h_3<h_i<h_2$, respectively, as follows:

$$\mathit{\rho }=\pm \left(\begin{array}{c}\frac{{\lambda }_1(h_2-h_3)(h_i-h_2)\sqrt{h_i-h_3}\sqrt{(h_2-h_i){\lambda }_1^2+h_1-h_i}}{(h_2-h_i)(h_2-h_3){\lambda }_1^2+(h_1-h_i)(h_1-h_3)}\\ -\frac{(h_i-h_1)(h_1-h_3)\sqrt{h_i-h_3}\sqrt{(h_2-h_i){\lambda }_1^2+h_1-h_i}}{(h_2-h_i)(h_2-h_3){\lambda }_1^2+(h_1-h_i)(h_1-h_3)}\\ \mp \frac{(h_2-h_i)(h_1-h_i)(h_1-h_2){\lambda }_1}{(h_2-h_i)(h_2-h_3){\lambda }_1^2+(h_1-h_i)(h_1-h_3)}\end{array}\right)$$

(61)

where $h_2<h_i<h_1$ and $h_3<h_i<h_2$ are associated with the symbol “$+$” and the symbol “$-$” in the symbol “$\pm$”, respectively, and are associated with the symbol “$-$” and the symbol “$+$” in the symbol “$\mp$”, respectively.

Setting $h_1=7$, $h_2=5$, and $h_3=1$, when $h_2<h_i=6<h_1$, the corresponding ruled surface, striction curve (in red), and pitch-hyperboloid are as shown in Figure 12A. When $h_3<h_i=3<h_2$, the corresponding ruled surface, striction curve (in red), and pitch-hyperboloid are as shown in Figure 12B.

Remark: 

Although the ruled surfaces lie on the pitch-hyperboloids, their central ellipses do not cover the striction curves of the ruled surfaces. In the following lines, variation of the parameter $h_i$ in Equation (56) yields the varying-pitch ruled surface.

In particular, if the parametric variable $h_i$ in Equation (56) is substituted by a continuous function of ${\lambda }_1$, i.e., $h_i=h({\lambda }_1)\in$$(h_3,h_1)$, Equation (56) can be rewritten as

$${\lambda }_1^2(h_2-h({\lambda }_1))+{\lambda }_2^2(h_3-h({\lambda }_1))=h({\lambda }_1)-h_1$$

(62)

Substituting the function expression of Equation (62) into Equation (2) leads to a serial of screws whose pitch value varies according to any desired continuous function $h({\lambda }_1)$. These screws lie on a ruled surface which is defined as the varying-pitch ruled surface. For example, setting $h({\lambda }_1)={\lambda }_1+c$, and considering $0<{\lambda }_1+c-h_3<h_1-h_3$, the function expression of Equation (62) has the form

$${\lambda }_2=\pm \sqrt{\frac{-{\lambda }_1^3+\left(h_2-c\right){\lambda }_1^2-{\lambda }_1-c+h_1}{{\lambda }_1+c-h_3}}$$

(63)

Setting $h({\lambda }_1)={\lambda }_1^2+c$, and considering $0<{\lambda }_1^2+c-h_3<h_1-h_3$, the function expression of Equation (62) has the form

$${\lambda }_2=\pm \sqrt{\frac{-{\lambda }_1^4+\left(h_2-1-c\right){\lambda }_1^2-c+h_1}{{\lambda }_1^2+c-h_3}}$$

(64)

Setting $h_1=7$, $h_2=5$, and $h_3=1$, and substituting Equation (63) and Equation (64) into Equation (2) yield two serials of screws, respectively. Their varying-pitch ruled surfaces and striction curves (in red) are plotted in the Figure 13A and Figure 13B, respectively. The parametric variable c takes the values 3 and 2, as seen in the Figure 13A and Figure 13B, respectively.

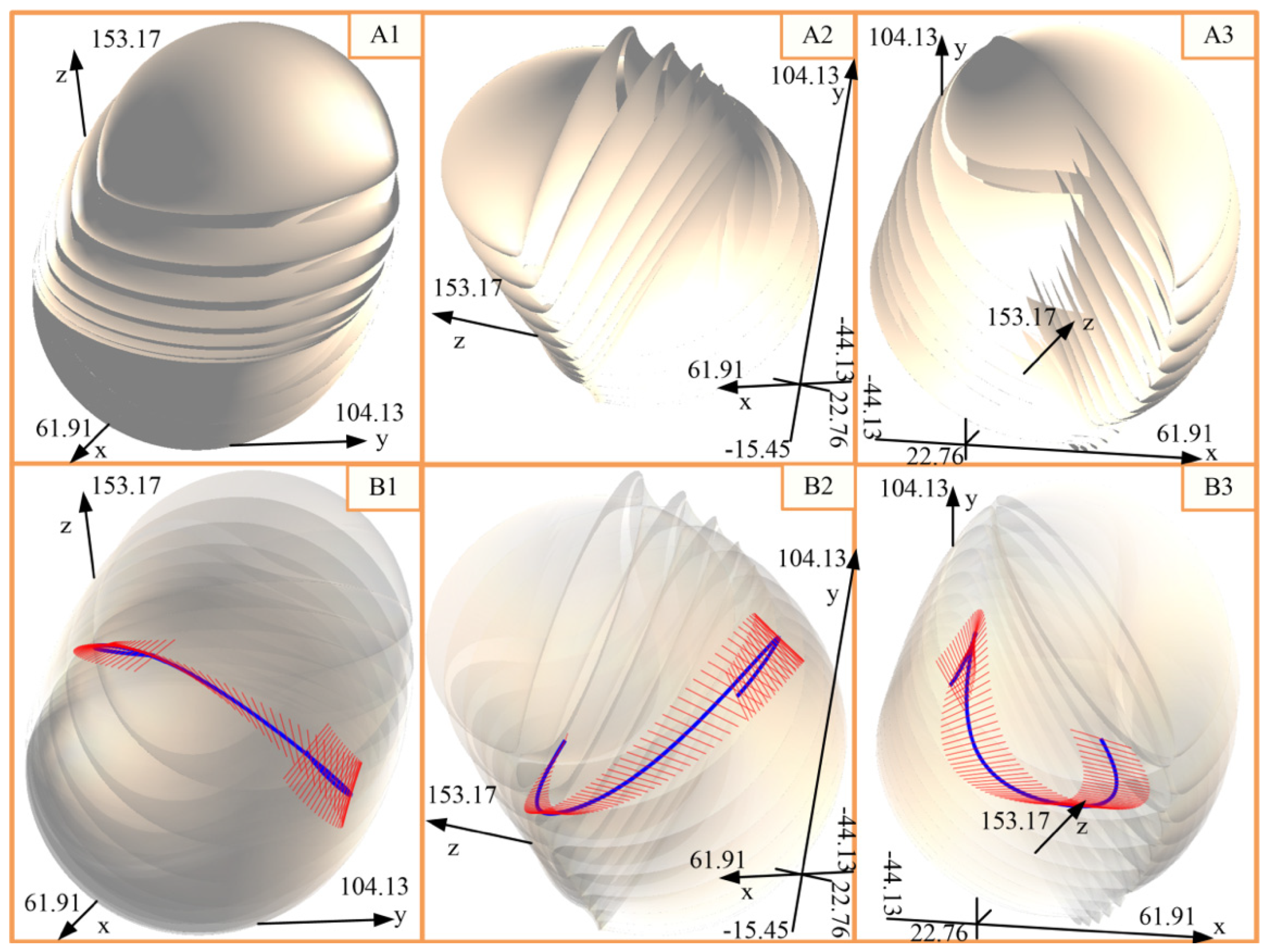
6. Numerical Examples of the 3-RPS Pyramid Mechanism

In axode planning, there is a basic problem: given an axode arbitrarily in the reference frame of a parallel mechanism, how can one visually tell whether this axode can be produced by the mechanism when it passes through a serial of configurations? The necessary condition is that its striction curve is inside the zone consisting of a serial of densest distribution zones associated with a serial of configurations, respectively.

Huang [30] pointed out that the twist screw system of the 3-RPS pyramid mechanism is just a general three-system of screws. So, in this section, we present a numerical example of a 3-RPS pyramid mechanism to demonstrate that the necessary conduction is valid. The mechanism consists of a base $O-A_1{A}_2{A}_3$, a moving platform $D-a_1{a}_2{a}_3$, and three identical-limb RPSs. Here, R represents the revolute joint, P denotes the prismatic joint, and S stands for the spherical joint. In particular, the axis of the R joint is perpendicular to that of the P joint. The reference frame $O-XYZ$ attached to the base has its origin located at the point $O$ and its three axes are along the three ones of the R joints of the base, respectively. The body frame $o-xyz$ attached to the moving platform has its origin located at the midpoint of the triangle $a_1{a}_2{a}_3$, its z-axis perpendicular to the plane $a_1{a}_2{a}_3$, its y-axis parallel to the segment $a_2{a}_3$ and in the plane $a_1{a}_2{a}_3$, and its x-axis defined according to the right-hand rule as shown in Figure 14. In the configuration shown in Figure 14, the lengths of three P joints are M, and the mechanism takes on the form of a cube.

Limb i imposes a constraint wrench screw ${\$}_i^r(i=1,2,3)$ on the moving platform. They pass through the three centers of the S joints and are parallel to the three axes of three R joints, respectively. So, they are linearly independent and constrain three translational freedoms of this mechanism, whatever configuration it is under. The reciprocal screw system of the above three constraint wrench screws is a general three-system of screws and stands for three rotational freedoms of the mechanism. The 3-RPS pyramid mechanism sets its structural parameters and input parameters as follows:

$$R_O^o=\left[\begin{array}{ccc}0.813& -0.469& 0.342\\ 0.543& 0.823& -0.163\\ -0.205& 0.318& 0.925\end{array}\right] \begin{array}{c}\begin{array}{l}{L}_1=(3t^2-2t)mm (0\le t\le 4.5s)\\ L_2=(2t^2-3t)mm (0\le t\le 4.5s)\\ L_3=(5t)mm (0\le t\le 4.5s) M=60mm\end{array}\end{array}$$

(65)

where $R_O^o$ represents the initial orientation $(t=0)$ of the body frame with respect to the reference frame. M is the length of the segment $O{A}_1$. $L_1$, $L_2$ and $L_3$ are the input parameters of the three P joints, respectively, when the mechanism performs a motion task. In this motion task, a serial of densest distribution zones and an axode can be obtained as shown in Figure 15. The Figure 15(A1–A3) display the densest distribution zones from three different views, respectively. The striction curve (in blue) of the axode is shown in the Figure 15(B1–B3) from three different views, respectively. As shown in Figure 15, the striction curve indeed is located inside the zone consisting of a serial of densest distribution zones.

7. Discussions

Section 4 partitions the general three-system according to the family of straight lines in the ${\lambda }_1{\lambda }_2$ plane, while Section 5 partitions it according to the family of ellipses and the family of hyperbolas. In particular, the varying-pitch ruled surface associated with the desired continuous function $h_i=h({\lambda }_1)$ corresponds to more general curves in the ${\lambda }_1{\lambda }_2$ plane. So, the varying-pitch ruled surface is a generalized decomposition method. In the first two decomposition methods, the family of lines and the family of ellipses and hyperbolas both completely cover the ${\infty }^2$ points in the ${\lambda }_1{\lambda }_2$ plane.

8. Conclusions

This paper has addressed the distribution regularity of the screw axes of the general three-system. The main achieved findings are that the position vector endpoints of the ${\infty }^2$ screw axes lie on the surface $S^{\perp }$. Further, these screw axes most densely distribute in the densest distribution zone bounded by two end striction point surfaces. Furthermore, the densest distribution zone of screw axes of the general three-system can be decomposed into ${\infty }^1$ two-subsystem cylindroids whose spine lines lie on a certain first-order spine cylindroid.