Mechanical Design and Performance Analysis of a Weevil-Inspired Jumping Mechanism
Abstract
:1. Introduction
2. Design of Jumping Mechanism
2.1. Biological Working Principle
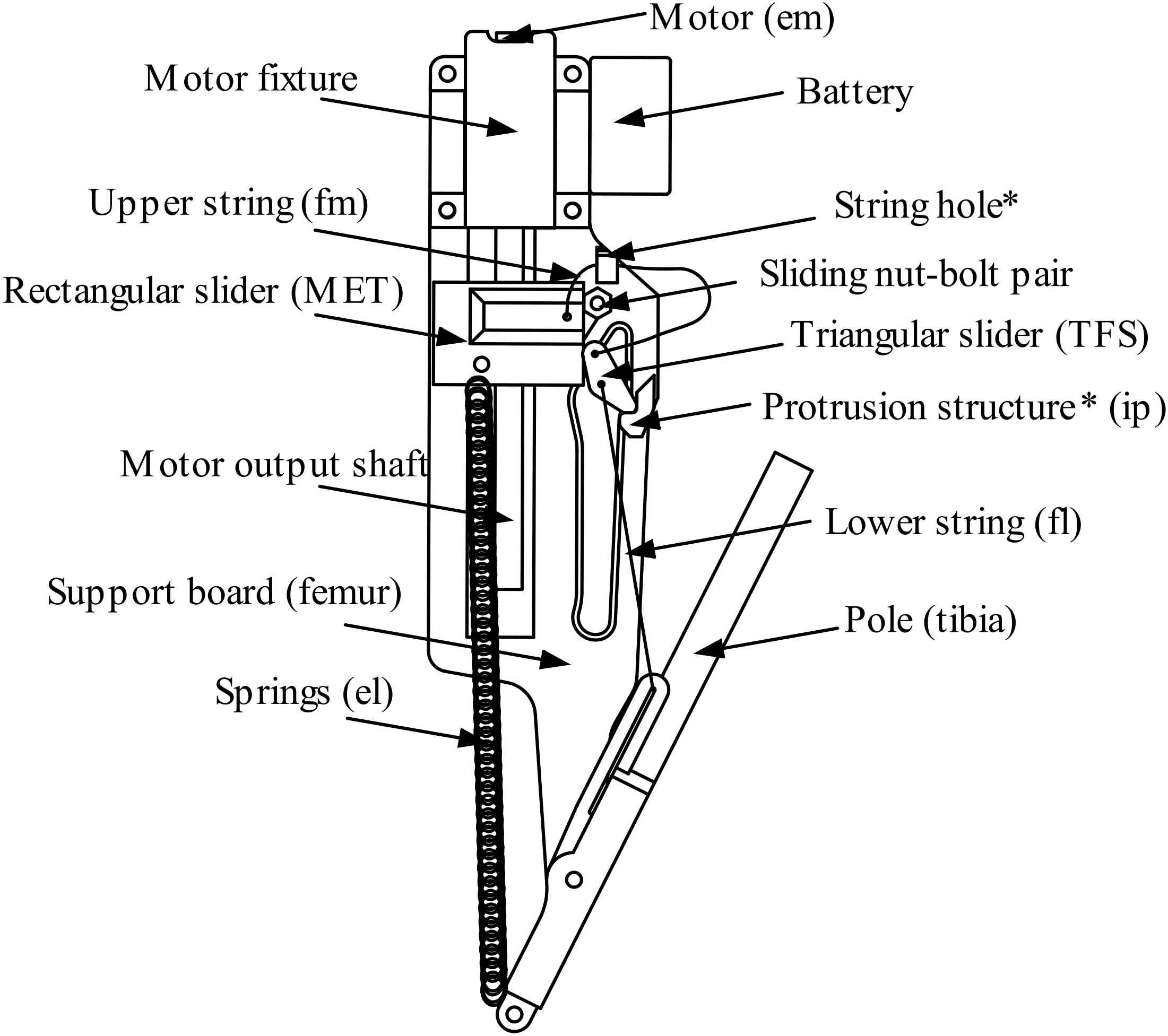
2.2. Mechanical Redesign
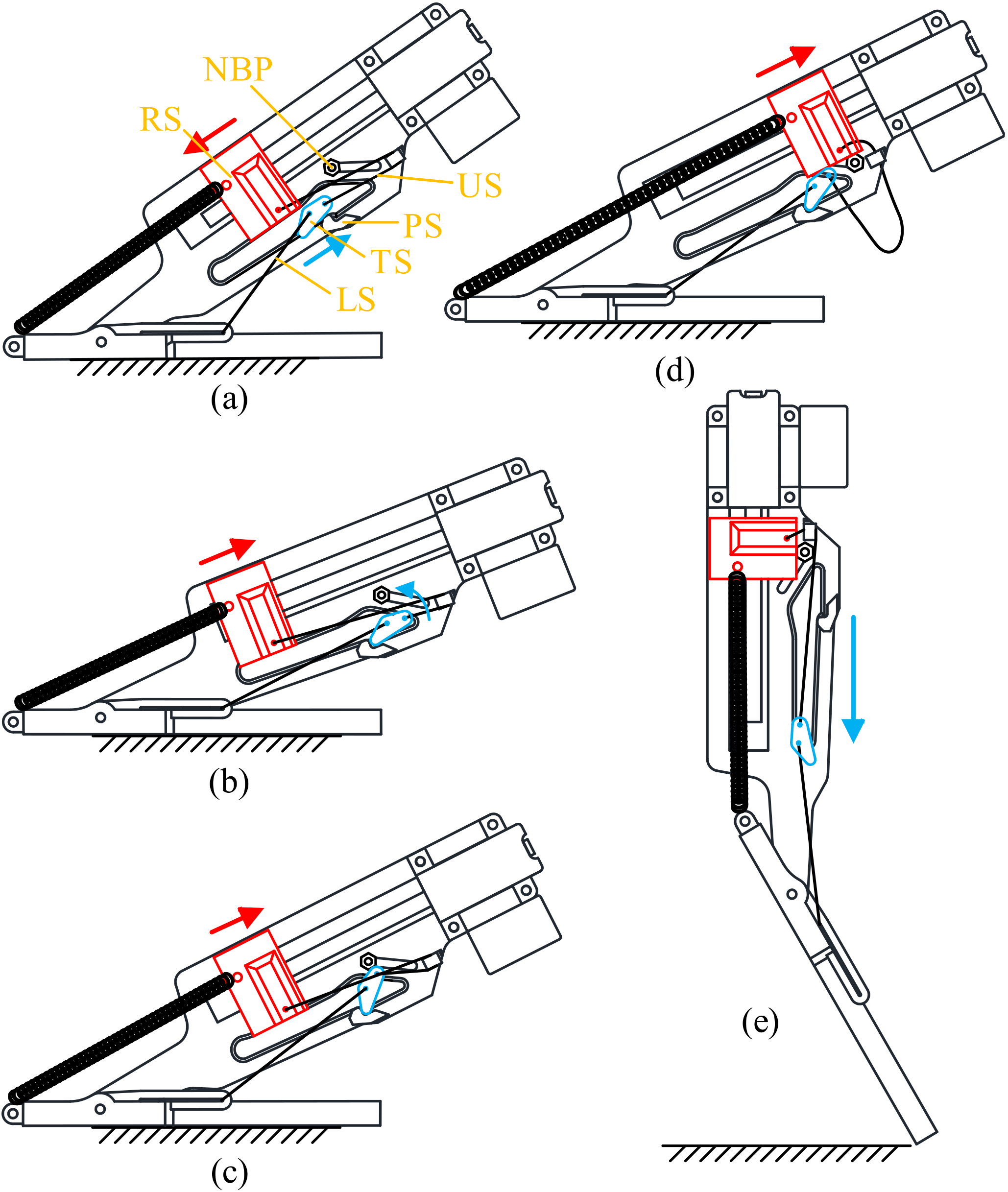
2.3. Working Cycle
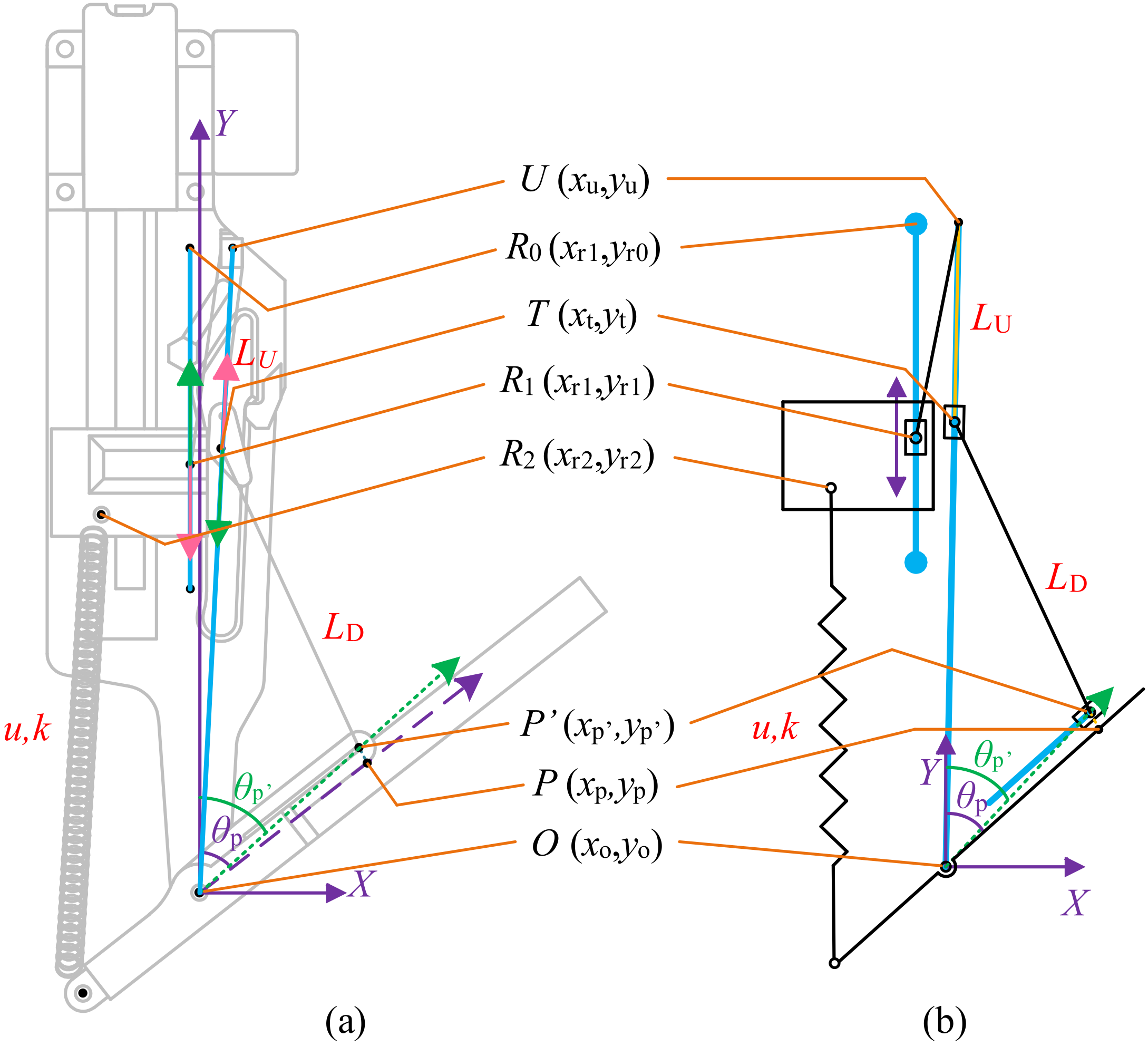
2.4. Theoretical Dynamical Analysis
3. Virtual Prototype Simulation
3.1. Introduction to Simulation
3.2. Spepated Variable Simulation
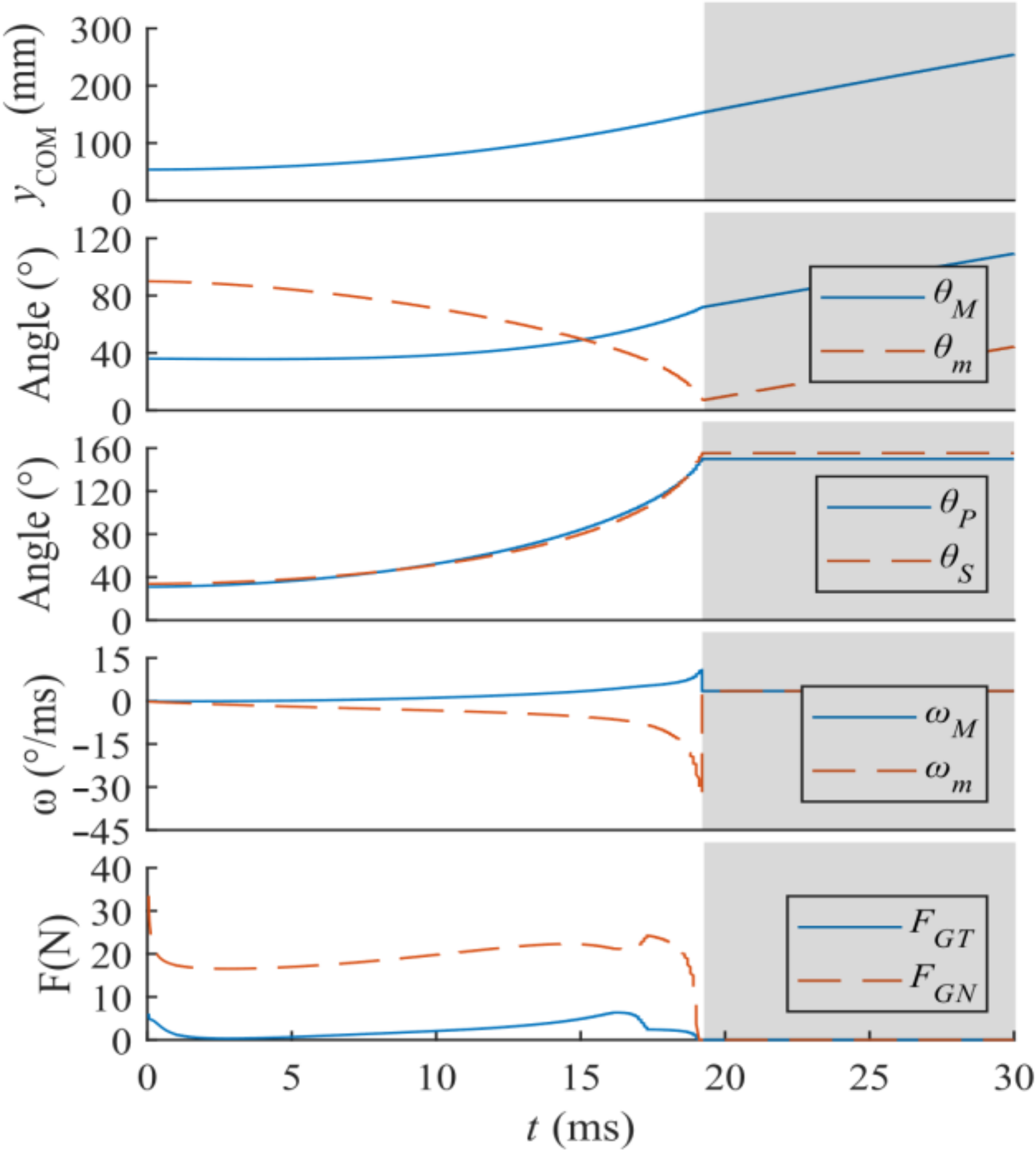
3.3. Dynamical Quantities vs. Time
4. Experimental Studies
4.1. Introduction
4.2. Orbit Test
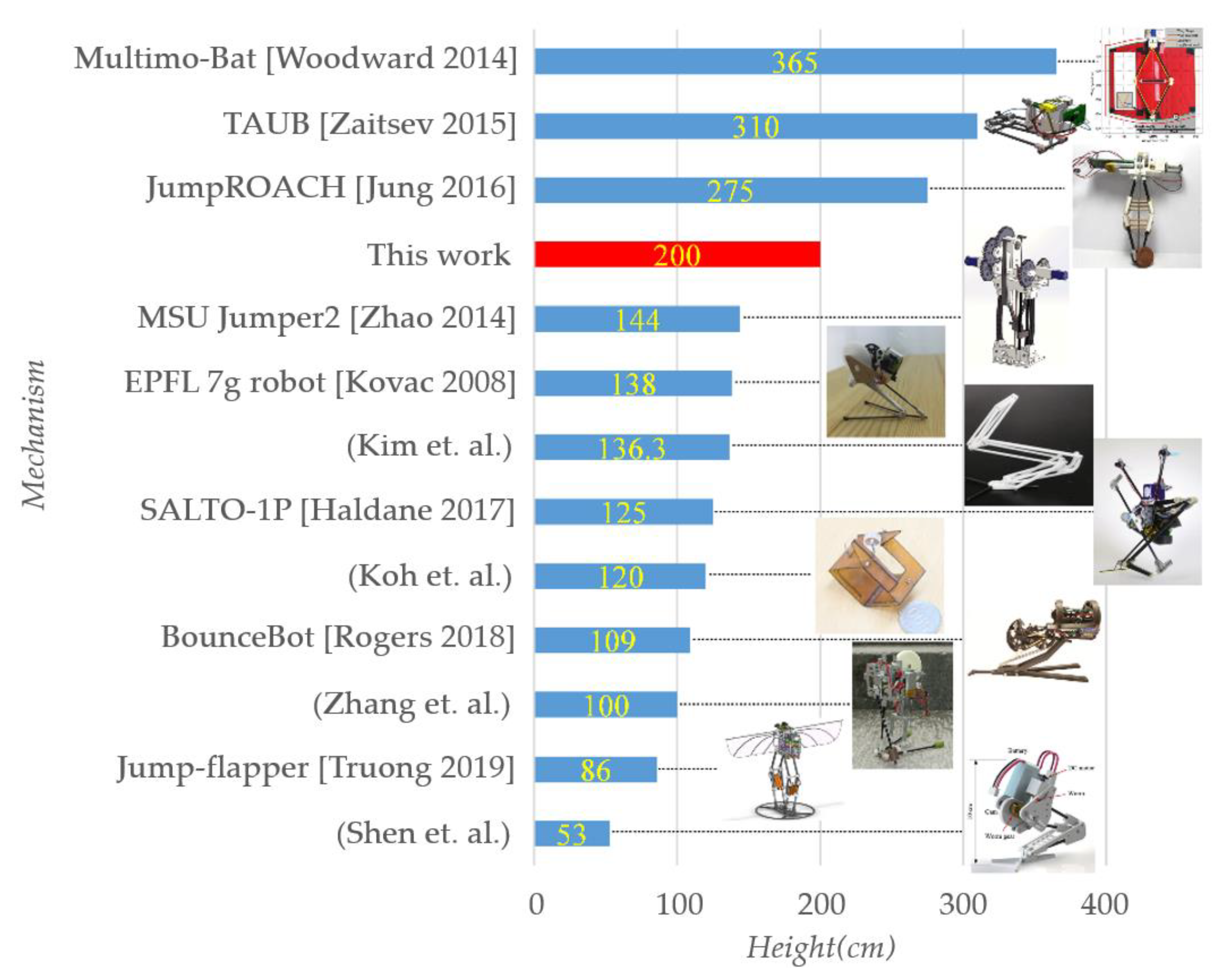
5. Discussion
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Anderson, F.C.; Pandy, M. Storage and utilization of elastic strain energy during jumping. J. Biomech. 1993, 26, 1413–1427. [Google Scholar] [CrossRef]
- Anderson, F.C.; Pandy, M.G. A Dynamic Optimization Solution for Vertical Jumping in Three Dimensions. Comput. Methods Biomech. Biomed. Eng. 1999, 2, 201–231. [Google Scholar] [CrossRef] [PubMed]
- Jung, G.-P.; Casarez, C.S.; Lee, J.-E.; Baek, S.-M.; Yim, S.-J.; Chae, S.-H.; Fearing, R.S.; Cho, K.-J.; Fearing, R.S.; Chae, S.-H. JumpRoACH: A Trajectory-Adjustable Integrated Jumping–Crawling Robot. IEEE/ASME Trans. Mechatron. 2019, 24, 947–958. [Google Scholar] [CrossRef]
- Jung, G.-P.; Casarez, C.S.; Jung, S.-P.; Fearing, R.S.; Cho, K.-J. An integrated jumping-crawling robot using height-adjustable jumping module. In Proceedings of the 2016 IEEE International Conference on Robotics and Automation (ICRA), Stockholm, Sweden, 16–21 May 2016; pp. 4680–4685. [Google Scholar] [CrossRef]
- Woodward, M.; Sitti, M. Multimo-bat: A biologically inspired integrated jumping-gliding robot. Int. J. Robot. Res. 2014, 33, 1511–1529. [Google Scholar] [CrossRef]
- Ye, C.; Wang, B.; Wei, B.; Tang, B. Modeling and Analysis of a Jumping Robot with Deforming Wheeled Mechanism. In Proceedings of the 2018 IEEE International Conference on Mechatronics and Automation (ICMA), Changchun, China, 5–8 August 2018; pp. 980–985. [Google Scholar] [CrossRef]
- Truong, N.T.; Phan, H.V.; Park, H.C. Design and demonstration of a bio-inspired flapping-wing-assisted jumping robot. Bioinspiration Biomim. 2019, 14, 036010. [Google Scholar] [CrossRef] [PubMed]
- Zhao, J.; Xu, J.; Gao, B.; Xi, N.; Cintron, F.J.; Mutka, M.W.; Xiao, L. MSU Jumper: A Single-Motor-Actuated Miniature Steerable Jumping Robot. IEEE Trans. Robot. 2013, 29, 602–614. [Google Scholar] [CrossRef]
- Zhao, J.; Zhao, T.; Xi, N.; Mutka, M.W.; Xiao, L. MSU Tailbot: Controlling Aerial Maneuver of a Miniature-Tailed Jumping Robot. IEEE/ASME Trans. Mechatron. 2015, 20, 2903–2914. [Google Scholar] [CrossRef]
- Zhao, J.; Yan, W.; Xi, N.; Mutka, M.W.; Xiao, L. A miniature 25 grams running and jumping robot. In Proceedings of the 2014 IEEE International Conference on Robotics and Automation (ICRA), Hong Kong, China, 31 May–7 June 2014; pp. 5115–5120. [Google Scholar] [CrossRef]
- Shen, Y.; Ge, W.; Mo, X.; Hou, Z. Design of a locust-inspired miniature jumping robot. In Proceedings of the 2018 IEEE International Conference on Robotics and Biomimetics (ROBIO), Kuala Lumpur, Malaysia, 12–15 December 2018; pp. 2322–2327. [Google Scholar] [CrossRef]
- Zhang, J.; Song, G.; Li, Y.; Qiao, G.; Song, A.; Wang, A. A bio-inspired jumping robot: Modeling, simulation, design, and experimental results. Mechatronics 2013, 23, 1123–1140. [Google Scholar] [CrossRef]
- Kovac, M.; Fuchs, M.; Guignard, A.; Zufferey, J.-C.; Floreano, D. A miniature 7g jumping robot. In Proceedings of the 2008 IEEE International Conference on Robotics and Automation, Pasadena, CA, USA, 19–23 May 2008; pp. 373–378. [Google Scholar]
- Plecnik, M.M.; Haldane, D.W.; Yim, J.K.; Fearing, R.S. Design Exploration and Kinematic Tuning of a Power Modulating Jumping Monopod. J. Mech. Robot. 2016, 9, 011009. [Google Scholar] [CrossRef]
- Haldane, D.W.; Plecnik, M.M.; Yim, J.K.; Fearing, R.S. Robotic vertical jumping agility via series-elastic power modulation. Sci. Robot. 2016, 1, eaag2048. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Haldane, D.W.; Yim, J.K.; Fearing, R.S. Repetitive extreme-acceleration (14-g) spatial jumping with Salto-1P. In Proceedings of the 2017 IEEE/RSJ International Conference on Intelligent Robots and Systems (IROS), Vancouver, BC, Canada, 24–28 September 2017; pp. 3345–3351. [Google Scholar]
- Zhang, J.; Song, G.; Qiao, G.; Li, Z.; Wang, W.; Song, A. A novel one-motor driven robot that jumps and walks. In Proceedings of the 2013 IEEE International Conference on Robotics and Automation, Karlsruhe, Germany, 6–10 May 2013; pp. 13–19. [Google Scholar]
- Chen, G.; Tu, J.; Ti, X.; Hu, H. A Single-legged Robot Inspired by the Jumping Mechanism of Click Beetles and Its Hopping Dynamics Analysis. J. Bionic Eng. 2020, 17, 1109–1125. [Google Scholar] [CrossRef]
- Zhang, Z.; Chang, B.; Zhao, J.; Yang, Q.; Liu, X. Design, Optimization, and Experiment on a Bioinspired Jumping Robot with a Six-Bar Leg Mechanism Based on Jumping Stability. Math. Probl. Eng. 2020, 2020, 1–23. [Google Scholar] [CrossRef]
- Noh, M.; Kim, S.-W.; An, S.; Koh, J.-S.; Cho, K.-J. Flea-Inspired Catapult Mechanism for Miniature Jumping Robots. IEEE Trans. Robot. 2012, 28, 1007–1018. [Google Scholar] [CrossRef]
- Koh, J.-S.; Jung, S.-P.; Noh, M.; Kim, S.-W.; Cho, K.-J. Flea inspired catapult mechanism with active energy storage and release for small scale jumping robot. In Proceedings of the 2013 IEEE International Conference on Robotics and Automation, Karlsruhe, Germany, 6–10 May 2013; pp. 26–31. [Google Scholar] [CrossRef]
- Nadein, K.; Betz, O. Jumping mechanisms and performance in beetles II. Weevils (Coleoptera: Curculionidae: Rhamphini). Arthropod Struct. Dev. 2018, 47, 131–143. [Google Scholar] [CrossRef] [PubMed]
- Komarsofla, A.K.; Yazdi, E.A.; Eghtesad, M. Dynamic Modeling and Control of a Novel One-Legged Hopping Robot. Robotica 2021, 39, 1692–1710. [Google Scholar] [CrossRef]
- Ding, Y.; Park, H.-W. Design and experimental implementation of a quasi-direct-drive leg for optimized jumping. In Proceedings of the 2017 IEEE/RSJ International Conference on Intelligent Robots and Systems (IROS), Vancouver, BC, Canada, 24–28 September 2017; pp. 300–305. [Google Scholar] [CrossRef]
- Zaitsev, V.; Gvirsman, O.; Ben Hanan, U.; Weiss, A.; Ayali, A.; Kosa, G. A locust-inspired miniature jumping robot. Bioinspiration Biomim. 2015, 10, 066012. [Google Scholar] [CrossRef] [PubMed]
- Beck, A.; Zaitsev, V.; Ben Hanan, U.; Kosa, G.; Ayali, A.; Weiss, A. Jump stabilization and landing control by wing-spreading of a locust-inspired jumper. Bioinspiration Biomim. 2017, 12, 066006. [Google Scholar] [CrossRef] [PubMed]
- Rogers, J.; Page-Bailey, K.; Smith, R. BounceBot: A One-Legged Jumping Robot. In Proceedings of the 19th Annual Conference, TAROS 2018, Bristol, UK, 25–27 July 2018; pp. 52–63. [Google Scholar] [CrossRef]
- Kim, M.-J.; Yun, D. Kinematic design and system implementation of jumping robot legs. In Proceedings of the 2017 IEEE International Conference on Multisensor Fusion and Integration for Intelligent Systems (MFI), Daegu, Korea, 16–18 November 2017; pp. 592–596. [Google Scholar] [CrossRef]
- Saab, W.; Rone, W.S.; Ben-Tzvi, P. Robotic tails: A state-of-the-art review. Robotica 2018, 36, 1263–1277. [Google Scholar] [CrossRef] [Green Version]




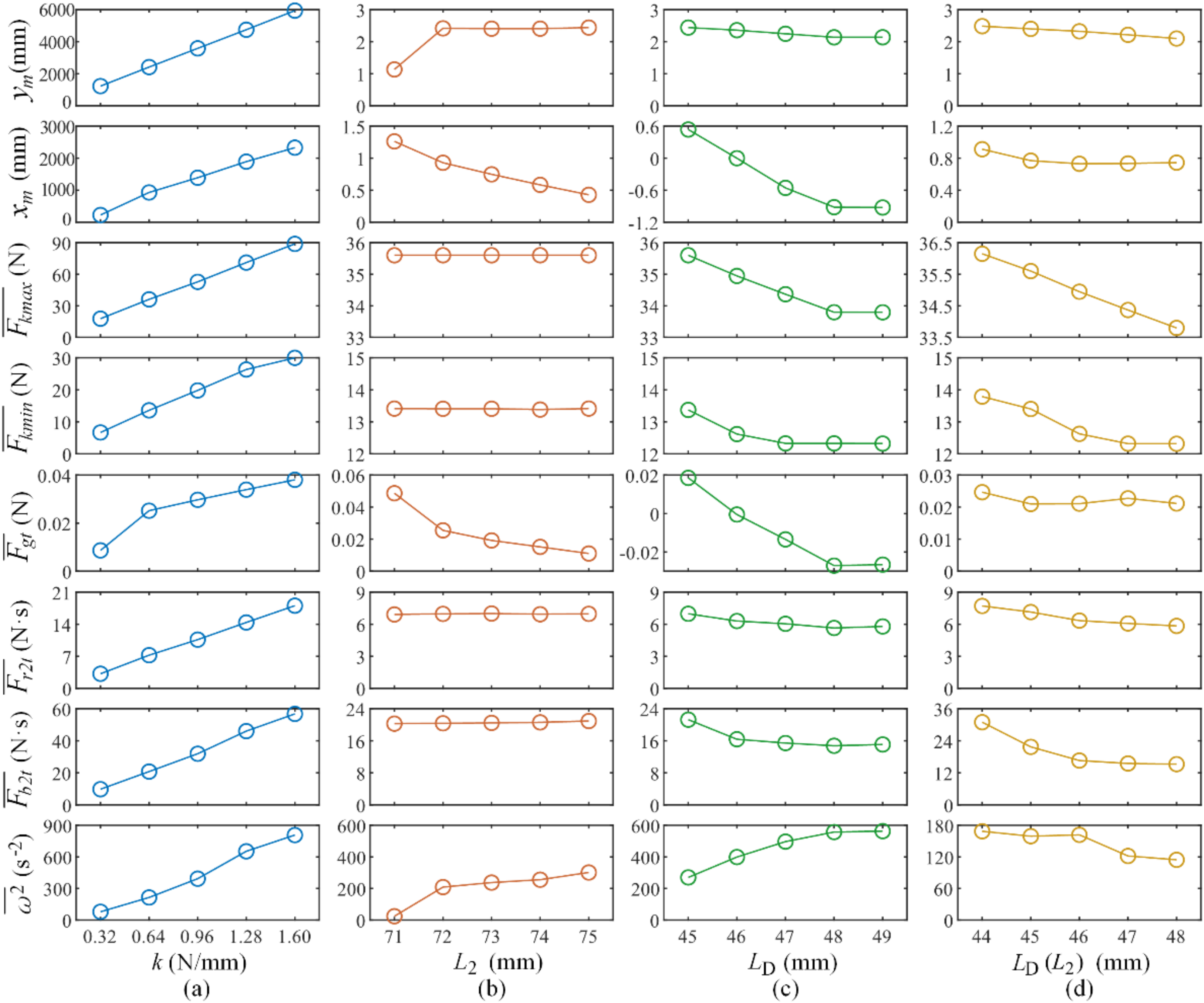



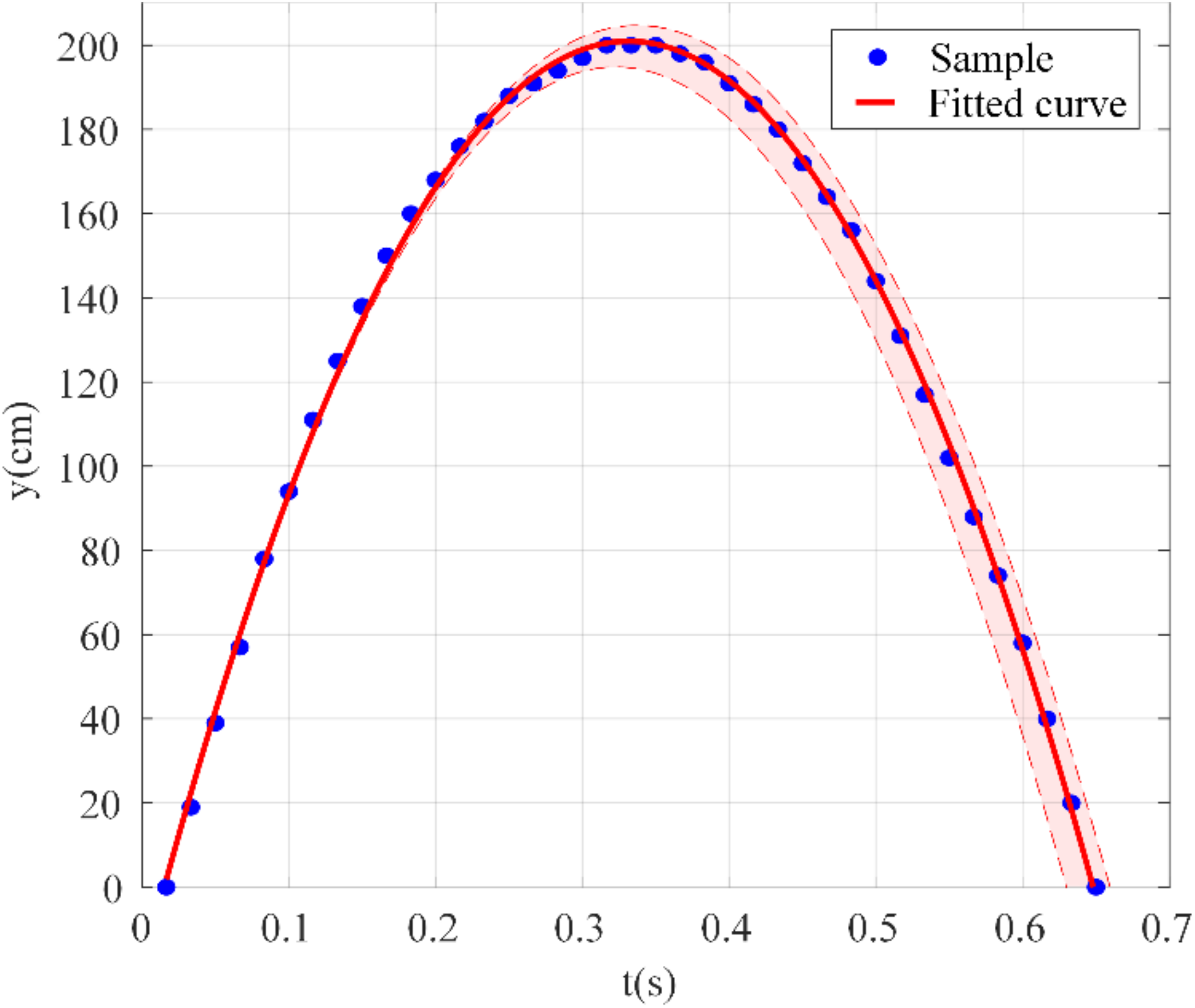
| Variable Name | Definition | Practical Significance |
|---|---|---|
| Displacement on x axis at landing | Idealized horizontal displacement | |
| Displacement on y axis at apex | Idealized jumping height | |
| Maximum spring tension force | Stored elastic energy and deformation of the spring | |
| Minimum spring tension force | Unreleased elastic energy | |
| Average of tangential component of force between ground and mechanism with time | Initial horizontal velocity | |
| Average of tangential force between SB and TS with time | Interaction forces between components | |
| Average angular velocity of SB | Measure of spinning |
| Part | Mass[g] | Portion |
|---|---|---|
| Motor | 13.7 | 30.6% |
| Battery | 5.0 | 11.2% |
| Motor fixtures | 3.0 | 6.7% |
| Pole | 6.8 | 15.2% |
| SB | 6.3 | 14.1% |
| RS | 1.9 | 4.3% |
| TS | 0.2 | 0.4% |
| Springs | 4.0 | 8.9% |
| Nuts and bolts | 3.8 | 8.5% |
| Total mechanism | 44.7 | 100% |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yu, Z.; Zeng, Y.; Guo, C. Mechanical Design and Performance Analysis of a Weevil-Inspired Jumping Mechanism. Machines 2022, 10, 161. https://doi.org/10.3390/machines10030161
Yu Z, Zeng Y, Guo C. Mechanical Design and Performance Analysis of a Weevil-Inspired Jumping Mechanism. Machines. 2022; 10(3):161. https://doi.org/10.3390/machines10030161
Chicago/Turabian StyleYu, Zhiwei, Yifan Zeng, and Ce Guo. 2022. "Mechanical Design and Performance Analysis of a Weevil-Inspired Jumping Mechanism" Machines 10, no. 3: 161. https://doi.org/10.3390/machines10030161
APA StyleYu, Z., Zeng, Y., & Guo, C. (2022). Mechanical Design and Performance Analysis of a Weevil-Inspired Jumping Mechanism. Machines, 10(3), 161. https://doi.org/10.3390/machines10030161







