Impact of Electric Vehicles on a Power Line with Photovoltaic Power Plants Connected
Abstract
:1. Introduction
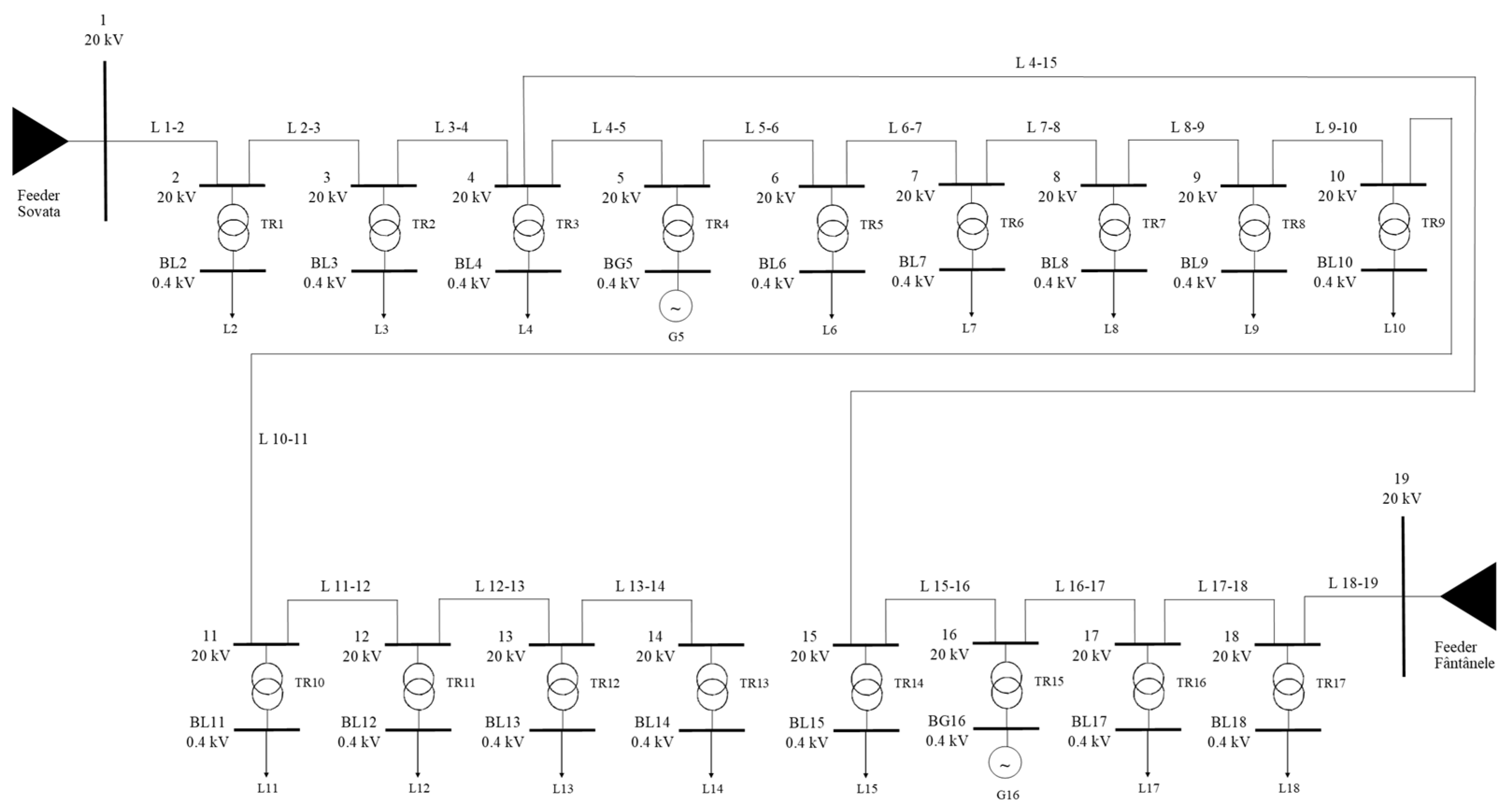
- The study will be performed for a real power line located in the Mureș County, Romania, namely the Sovata–Fântânele power line. Other researchers have considered test systems in most of their studies, while in the cases where real systems have been considered, the approach has been to manage the charging such that the power network is not affected.
- The power line has two and fifteen loads (two photovoltaic (PV) power plants connected in Ghindari and Sângeorgiu de Pădure, respectively). The power supplied by the PV power plants and the power demand of the loads determined with the power meters located at the consumer location will be taken into account in the studies performed.
- The electric vehicles (EVs) were connected in different points (buses) of the power line. Compared to the work of other researchers who considered only one supply option, three supply options for the power line were considered. In addition, two seasons (summer and winter) and different charging scenarios for the EVs were considered, while other researchers had a different approach, i.e., charging was performed in a controlled manner in their studies. The power demand of the EVs will be added to the demand of loads. The analyses will be performed with the NEPLAN software [56], and the results regarding the power losses and voltage level will be obtained.
2. Materials and Methods
- charging at a fast station starting at 2:00 (scenario 1);
- charging at a fast station starting at 5:00 (scenario 2);
- charging at a fast station starting at 9:00 (scenario 3);
- charging at a fast station starting at 12:00 (scenario 4);
- charging at a fast station starting at 15:00 (scenario 5);
- charging at a fast station starting at 18:00 (scenario 6);
- charging at a fast station starting at 22:00 (scenario 7).
3. Results and Discussion
- power line supplied from Sovata and Fântânele;
- power line supplied from Sovata;
- power line supplied from Fântânele.
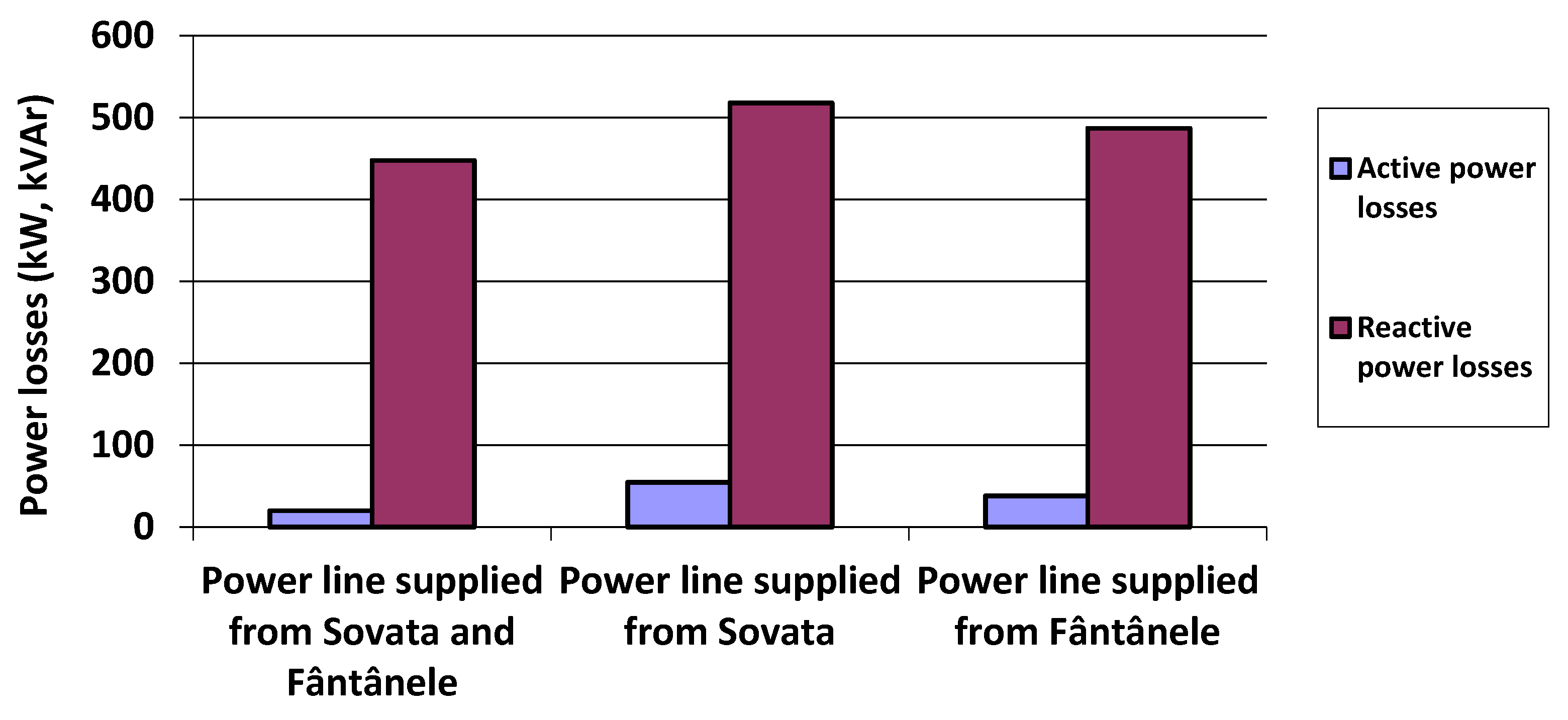
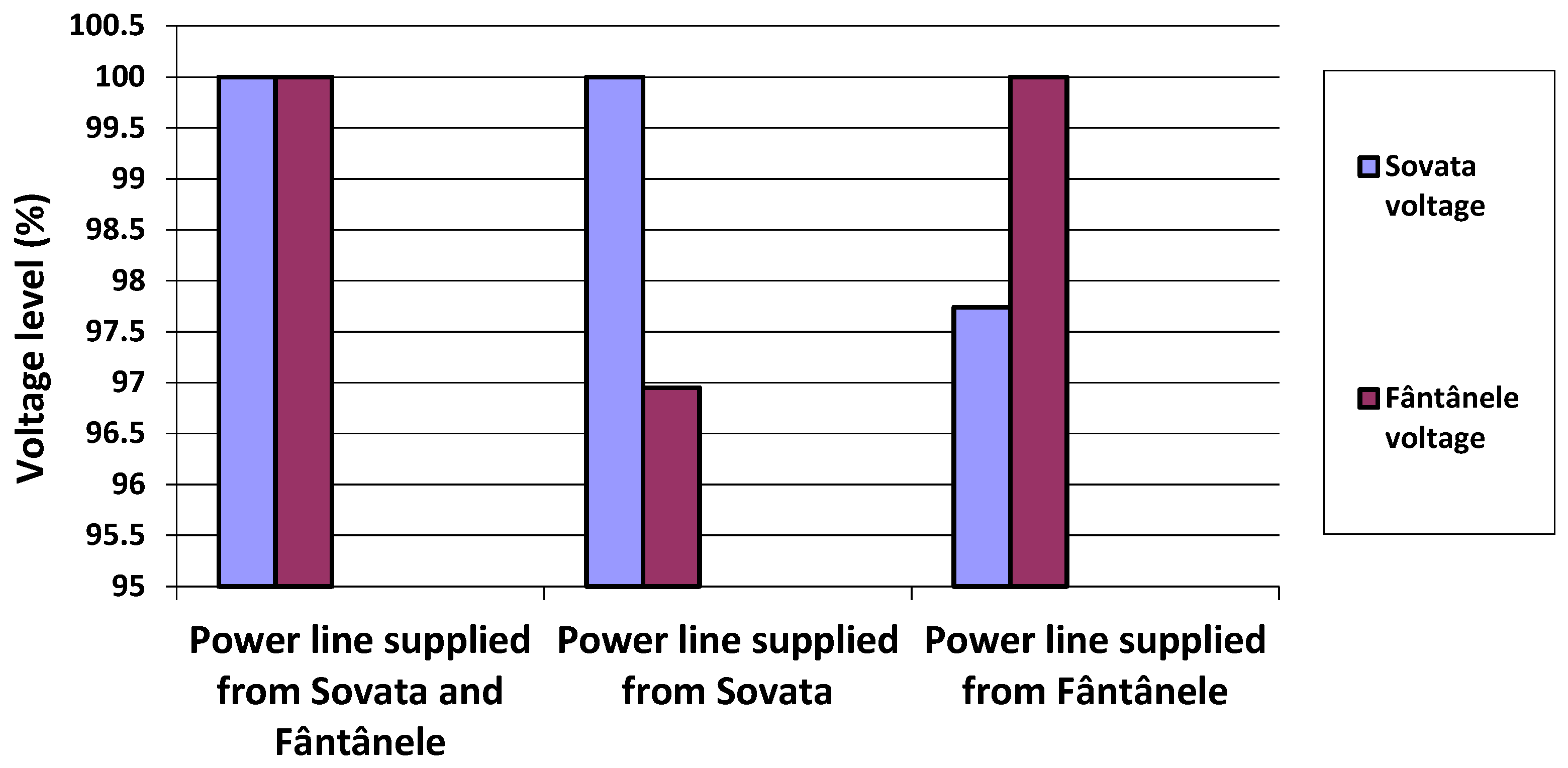
3.1. EVs Not Connected
3.2. EVs Connected and Charging (Scenario 1)
3.3. EVs Connected and Charging (Scenario 2)
3.4. EVs Connected and Charging (Scenario 3)
3.5. EVs Connected and Charging (Scenario 4)
3.6. EVs Connected and Charging (Scenario 5)
3.7. EVs Connected and Charging (Scenario 6)
3.8. EVs Connected and Charging (Scenario 7)
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- National Institute of Statistics from Romania. Available online: https://insse.ro/cms/ (accessed on 17 December 2021).
- Rahman, M.M.; Al-Ammar, E.A.; Das, S.H.; Ko, W. Comprehensive impact analysis of electric vehicle charging scheduling on load-duration curve. Comput. Electr. Eng. 2020, 85, 106673. [Google Scholar] [CrossRef]
- Huang, S.; Infield, D. The impact of domestic plug-in hybrid electric vehicles on power distribution system loads. In Proceedings of the 2010 International Conference on Power System Technology, Hangzhou, China, 24–28 October 2010; pp. 1–7. [Google Scholar] [CrossRef] [Green Version]
- Neaimeh, M.; Wardle, R.; Jenkins, A.M.; Yi, J.; Hill, G.; Lyons, P.F.; Hübner, Y.; Blythe, P.T.; Taylor, P.C. A probabilistic approach to combining smart meter and electric vehicle charging data to investigate distribution network impacts. Appl. Energy 2015, 157, 688–698. [Google Scholar] [CrossRef] [Green Version]
- Mauri, G.; Valsecchi, A. Fast charging stations for electric vehicle: The impact on the mv distribution grids of the Milan metropolitan area. In Proceedings of the 2012 IEEE International Energy Conference and Exhibition (ENERGYCON), Florence, Italy, 9–12 September 2012; pp. 1055–1059. [Google Scholar] [CrossRef]
- Vagropoulos, S.I.; Balaskas, G.A.; Bakirtzis, A.G. An investigation of plug-in electric vehicle charging impact on power systems scheduling and energy costs. IEEE Trans. Power Syst. 2017, 32, 1902–1912. [Google Scholar] [CrossRef]
- Papadopoulos, P.; Skarvelis-Kazakos, S.; Grau, I.; Cipcigan, L.M.; Jenkins, N. Predicting electric vehicle impacts on residential distribution networks with distributed generation. In Proceedings of the 2010 IEEE Vehicle Power and Propulsion Conference, Lille, France, 1–3 September 2010; pp. 1–5. [Google Scholar] [CrossRef]
- Turan, M.T.; Ates, Y.; Erdinc, O.; Gokalp, E.; Catalão, J.P.S. Effect of electric vehicle parking lots equipped with roof mounted photovoltaic panels on the distribution network. Int. J. Electr. Power Energy Syst. 2019, 109, 283–289. [Google Scholar] [CrossRef]
- Farhoodnea, M.; Mohamed, A.; Shareef, H.; Zayandehroodi, H. Power quality impacts of high-penetration electric vehicle stations and renewable energy-based generators on power distribution systems. Measurement 2013, 46, 2423–2434. [Google Scholar] [CrossRef]
- Hanemann, P.; Behnert, M.; Bruckner, T. Effects of electric vehicle charging strategies on the German power system. Appl. Energy 2017, 203, 608–622. [Google Scholar] [CrossRef]
- Lin, H.; Liu, Y.; Sun, Q.; Xiong, R.; Li, H.; Wennersten, R. The impact of electric vehicle penetration and charging patterns on the management of energy hub—A multi-agent system simulation. Appl. Energy 2018, 230, 189–206. [Google Scholar] [CrossRef]
- Hernández, J.C.; Ruiz-Rodriguez, F.J.; Jurado, F. Modelling and assessment of the combined technical impact of electric vehicles and photovoltaic generation in radial distribution systems. Energy 2017, 141, 316–332. [Google Scholar] [CrossRef]
- Calderaro, V.; Galdi, V.; Graber, G.; Graditi, G.; Lamberti, F. Impact assessment of energy storage and electric vehicles on smart grids. In Proceedings of the 2014 Electric Power Quality and Supply Reliability Conference (PQ), Rakvere, Estonia, 11–13 June 2014; pp. 15–18. [Google Scholar] [CrossRef]
- Ye, G.; Xiang, Y.; Cobben, J.F.G. Assessment of the voltage level and losses with photovoltaic and electric vehicle in low voltage network. In Proceedings of the 2014 14th International Conference on Environment and Electrical Engineering, Krakow, Poland, 10–12 May 2014; pp. 431–436. [Google Scholar] [CrossRef]
- Manríquez, F.; Sauma, E.; Aguado, J.; de la Torre, S.; Contreras, J. The impact of electric vehicle charging schemes in power system expansion planning. Appl. Energy 2020, 262, 114527. [Google Scholar] [CrossRef]
- Tovilović, D.M.; Rajaković, N.L.J. The simultaneous impact of photovoltaic systems and plug-in electric vehicles on the daily load and voltage profiles and the harmonic voltage distortions in urban distribution systems. Renew. Energy 2015, 76, 454–464. [Google Scholar] [CrossRef]
- Delgado, J.; Faria, R.; Moura, P.; de Almeida, A.T. Impacts of plug-in electric vehicles in the Portuguese electrical grid. Transp. Res. Part D Transp. Environ. 2018, 62, 372–385. [Google Scholar] [CrossRef]
- Van Roy, J.; Leemput, N.; Geth, F.; Büscher, J.; Salenbien, R.; Driesen, J. Electric vehicle charging in an office building microgrid with distributed energy resources. IEEE Trans. Sustain. Energy 2014, 5, 1389–1396. [Google Scholar] [CrossRef]
- Mohd Shariff, N.B.; Al Essa, M.; Cipcigan, L. Probabilistic analysis of electric vehicles charging load impact on residential distributions networks. In Proceedings of the 2016 IEEE International Energy Conference (ENERGYCON), Leuven, Belgium, 4–8 April 2016; pp. 1–6. [Google Scholar] [CrossRef]
- Chang, M.; Bae, S.; Yoon, G.; Park, S.; Choy, Y. impact of electric vehicle charging demand on a Jeju island radial distribution network. In Proceedings of the 2019 IEEE Power & Energy Society Innovative Smart Grid Technologies Conference (ISGT), Washington, DC, USA, 18–21 February 2019; pp. 1–5. [Google Scholar] [CrossRef]
- Abaspahić, A.; Šarić, M.; Hivziefendić, J.; Konjić, T. Impact of complementary integration of electric vehicle charging stations and photovoltaics on voltage quality and voltage stability. In Proceedings of the 2021 20th International Symposium INFOTEH-JAHORINA (INFOTEH), East Sarajevo, Bosnia and Herzegovina, 17–19 March 2021; pp. 1–6. [Google Scholar] [CrossRef]
- Osório, G.J.; Shafie-khah, M.; Coimbra, P.D.L.; Lotfi, M.; Catalão, J.P.S. Distribution system operation with electric vehicle charging schedules and renewable energy resources. Energies 2018, 11, 3117. [Google Scholar] [CrossRef] [Green Version]
- Clairand, J.-M.; Álvarez-Bel, C.; Rodríguez-García, J.; Escrivá-Escrivá, G. Impact of electric vehicle charging strategy on the long-term planning of an isolated microgrid. Energies 2020, 13, 3455. [Google Scholar] [CrossRef]
- Jones, C.B.; Lave, M.; Vining, W.; Garcia, B.M. Uncontrolled electric vehicle charging impacts on distribution electric power systems with primarily residential, commercial or industrial loads. Energies 2021, 14, 1688. [Google Scholar] [CrossRef]
- Ruiz-Rodriguez, F.J.; Hernández, J.C.; Jurado, F. Voltage behaviour in radial distribution systems under the uncertainties of photovoltaic systems and electric vehicle charging loads. Int. Trans. Electr. Energy Syst. 2017, 28, e2490. [Google Scholar] [CrossRef]
- Zaidi, A.; Sunderland, K.; Conlon, M. Impact assessment of high-power domestic EV charging proliferation of a distribution network. IET Gener. Transm. Distrib. 2020, 14, 5918–5926. [Google Scholar] [CrossRef]
- Akhtar, Z.; Opatovsky, M.; Chaudhuri, B.; Hui, S.Y.R. Comparison of point-of-load versus mid-feeder compensation in LV distribution networks with high penetration of solar photovoltaic generation and electric vehicle charging stations. IET Smart Grid 2019, 2, 283–292. [Google Scholar] [CrossRef]
- Stanev, R.; Georgiev, M.; Krusteva, A. An approach for estimation of the impact of electric vehicle charging devices on distribution networks. MATEC Web Conf. 2017, 125, 02068. [Google Scholar] [CrossRef] [Green Version]
- Flammini, M.G.; Prettico, G.; Fulli, G.; Bompard, E.; Chicco, G. Interaction of consumers, photovoltaic systems and electric vehicle energy demand in a reference network model. In Proceedings of the 2017 International Conference of Electrical and Electronic Technologies for Automotive, Turin, Italy, 15–16 June 2017; pp. 1–5. [Google Scholar] [CrossRef]
- Slednev, V.; Jochem, P.; Fichtner, W. Impacts of electric vehicles on the European high and extra high voltage power grid. J. Ind. Ecol. 2021, 2021, 1–14. [Google Scholar] [CrossRef]
- Drude, L.; Pereira Junior, L.C.; Rüther, R. Photovoltaics (PV) and electric vehicle-to-grid (V2G) strategies for peak demand reduction in urban regions in Brazil in a smart grid environment. Renew. Energy 2014, 68, 443–451. [Google Scholar] [CrossRef]
- Kikusato, H.; Mori, K.; Yoshizawa, S.; Fujimoto, Y.; Asano, H.; Hayashi, Y.; Kawashima, A.; Inagaki, S.; Suzuki, T. Electric vehicle charge–discharge management for utilization of photovoltaic by coordination between home and grid energy management systems. IEEE Trans. Smart Grid 2019, 10, 3186–3197. [Google Scholar] [CrossRef]
- Wang, L.; Nian, V.; Li, H.; Yuan, J. Impacts of electric vehicle deployment on the electricity sector in a highly urbanised environment. J. Clean. Prod. 2021, 295, 126386. [Google Scholar] [CrossRef]
- Rawat, T.; Niazi, K.R.; Gupta, N.; Sharma, S. Impact assessment of electric vehicle charging/discharging strategies on the operation management of grid accessible and remote microgrids. Int. J. Energy Res. 2019, 43, 9034–9048. [Google Scholar] [CrossRef]
- Torres-Moreno, J.L.; Gimenez-Fernandez, A.; Perez-Garcia, M.; Rodriguez, F. Energy management strategy for micro-grids with pv-battery systems and electric vehicles. Energies 2018, 11, 522. [Google Scholar] [CrossRef] [Green Version]
- Kasturi, K.; Nayak, C.K.; Nayak, M.R. Photovoltaic and electric vehicle-to-grid strategies for peak load shifting in low voltage distribution system under time of use grid pricing. Iran. J. Sci. Technol. Trans. Electr. Eng. 2021, 45, 789–801. [Google Scholar] [CrossRef]
- Hussain, M.T.; Sulaiman, N.B.; Hussain, M.S.; Jabir, M. Optimal management strategies to solve issues of grid having electric vehicles (EV): A review. J. Energy Storage 2021, 33, 102114. [Google Scholar] [CrossRef]
- Liu, T.; Tan, W.; Tang, X.; Zhang, J.; Xing, Y.; Cao, D. Driving conditions-driven energy management strategies for hybrid electric vehicles: A review. Renew. Sustain. Energy Rev. 2021, 151, 111521. [Google Scholar] [CrossRef]
- Mohamed, M.A.; Abdullah, H.M.; El-Meligy, M.A.; Sharaf, M.; Soliman, A.T.; Hajjiah, A. A novel fuzzy cloud stochastic framework for energy management of renewable microgrids based on maximum deployment of electric vehicles. Int. J. Electr. Power Energy Syst. 2021, 129, 106845. [Google Scholar] [CrossRef]
- Tang, Y.; Wang, S. Resilient residential energy management with vehicle-to-home and photovoltaic uncertainty. Int. J. Electr. Power Energy Syst. 2021, 132, 107206. [Google Scholar] [CrossRef]
- Lan, T.; Jermsittiparsert, K.; Alrashood, S.T.; Rezaei, M.; Al-Ghussain, L.; Mohamed, M.A. An advanced machine learning based energy management of renewable microgrids considering hybrid electric vehicles’ charging demand. Energies 2021, 14, 569. [Google Scholar] [CrossRef]
- Zhang, F.; Hu, X.; Langari, R.; Wang, L.; Cui, Y.; Pang, H. Adaptive energy management in automated hybrid electric vehicles with flexible torque request. Energy 2021, 214, 118873. [Google Scholar] [CrossRef]
- Guo, N.; Zhang, X.; Zou, Y.; Guo, L.; Du, G. Real-time predictive energy management of plug-in hybrid electric vehicles for coordination of fuel economy and battery degradation. Energy 2021, 214, 119070. [Google Scholar] [CrossRef]
- Behi, H.; Karimi, D.; Youssef, R.; Suresh Patil, M.; Van Mierlo, J.; Berecibar, M. Comprehensive passive thermal management systems for electric vehicles. Energies 2021, 14, 3881. [Google Scholar] [CrossRef]
- Pasetti, M.; Rinaldi, S.; Flammini, A.; Longo, M.; Foiadelli, F. Assessment of electric vehicle charging costs in presence of distributed photovoltaic generation and variable electricity tariffs. Energies 2019, 12, 499. [Google Scholar] [CrossRef] [Green Version]
- Wang, Z.; Yu, L.; Ji, T.; Zeng, Y.; Ji, Y. Research on voltage control method of distributed photovoltaic distribution network considering electric vehicle charging. IOP Conf. Ser. Earth Environ. Sci. 2021, 651, 022007. [Google Scholar] [CrossRef]
- Gschwendtner, C.; Sinsel, S.R.; Stephan, A. Vehicle-to-X (V2X) implementation: An overview of predominate trial configurations and technical, social and regulatory challenges. Renew. Sustain. Energy Rev. 2021, 145, 110977. [Google Scholar] [CrossRef]
- Chandran, V.; Patil, C.K.; Karthick, A.; Ganeshaperumal, D.; Rahim, R.; Ghosh, A. State of charge estimation of lithium-ion battery for electric vehicles using machine learning algorithms. World Electr. Veh. J. 2021, 12, 38. [Google Scholar] [CrossRef]
- Khan, M.; Sun, H.; Xiang, Y.; Shi, D. Electric vehicles participation in load frequency control based on mixed H2/H∞. Int. J. Electr. Power Energy Syst. 2021, 125, 106420. [Google Scholar] [CrossRef]
- Robledo, C.B.; Oldenbroek, V.; Abbruzzese, F.; van Wijk, A.J.M. Integrating a hydrogen fuel cell electric vehicle with vehicle-to-grid technology, photovoltaic power and a residential building. Appl. Energy 2018, 215, 615–629. [Google Scholar] [CrossRef]
- Venegas, F.G.; Petit, M.; Perez, Y. Active integration of electric vehicles into distribution grids: Barriers and frameworks for flexibility services. Renew. Sustain. Energy Rev. 2021, 145, 111060. [Google Scholar] [CrossRef]
- Wu, C.; Gao, S.; Liu, Y.; Song, T.E.; Han, H. A model predictive control approach in microgrid considering multi-uncertainty of electric vehicles. Renew. Energy 2021, 163, 1385–1396. [Google Scholar] [CrossRef]
- Venegas, F.G.; Petit, M.; Perez, Y. Plug-in behavior of electric vehicles users: Insights from a large-scale trial and impacts for grid integration studies. eTransportation 2021, 10, 100131. [Google Scholar] [CrossRef]
- Higashitani, T.; Ikegami, T.; Uemichi, A.; Akisawa, A. Evaluation of residential power supply by photovoltaics and electric vehicles. Renew. Energy 2021, 178, 745–756. [Google Scholar] [CrossRef]
- Dulău, L.I.; Bică, D. Effects of electric vehicles on power networks. Procedia Manuf. 2020, 46, 370–377. [Google Scholar] [CrossRef]
- Available online: www.neplan.ch (accessed on 17 December 2021).

| Feeder/Generator Connected at Bus | V (p.u.) | P (kW) |
|---|---|---|
| 1 | 1 | - |
| G5 | 1 | 1200 |
| G16 | 1 | 2000 |
| 19 | 1 | - |
| Load | Active Power Demand (kW) | Reactive Power Demand (kVAr) |
|---|---|---|
| L2 | 633 | 475 |
| L3 | 476 | 375 |
| L4 | 56 | 42 |
| L6 | 420 | 315 |
| L7 | 125 | 94 |
| L8 | 56 | 42 |
| L9 | 196 | 147 |
| L10 | 35 | 26 |
| L11 | 196 | 147 |
| L12 | 40 | 30 |
| L13 | 35 | 26 |
| L14 | 56 | 42 |
| L15 | 287 | 215 |
| L17 | 734 | 550 |
| L18 | 1387 | 1040 |
| Hour (h) | Load Scale Factor for Summer | Load Scale Factor for Winter |
|---|---|---|
| 1 | 0.5717 | 0.7071 |
| 2 | 0.5682 | 0.7129 |
| 3 | 0.5765 | 0.7359 |
| 4 | 0.6177 | 0.7992 |
| 5 | 0.6651 | 0.8792 |
| 6 | 0.6854 | 0.9366 |
| 7 | 0.695 | 0.962 |
| 8 | 0.6989 | 0.965 |
| 9 | 0.7078 | 0.9516 |
| 10 | 0.7164 | 0.9445 |
| 11 | 0.7089 | 0.9401 |
| 12 | 0.7054 | 0.9242 |
| 13 | 0.7059 | 0.9187 |
| 14 | 0.7065 | 0.9301 |
| 15 | 0.7041 | 0.9699 |
| 16 | 0.7056 | 0.9841 |
| 17 | 0.7159 | 0.9754 |
| 18 | 0.7437 | 0.9435 |
| 19 | 0.7103 | 0.8862 |
| 20 | 0.6533 | 0.7769 |
| 21 | 0.6089 | 0.7861 |
| 22 | 0.584 | 0.7446 |
| 23 | 0.5679 | 0.7228 |
| 24 | 0.5691 | 0.7096 |
| Power Line between Bus and Bus | Length (km) |
|---|---|
| 1–2 | 8.3 |
| 2–3 | 5.3 |
| 3–4 | 4.3 |
| 4–5 | 0.1 |
| 4–15 | 3.8 |
| 5–6 | 0.1 |
| 6–7 | 4.1 |
| 7–8 | 5.8 |
| 8–9 | 2.6 |
| 9–10 | 4.5 |
| 10–11 | 1.2 |
| 11–12 | 6 |
| 12–13 | 5.6 |
| 13–14 | 2.4 |
| 15–16 | 8.1 |
| 16–17 | 7.6 |
| 17–18 | 7.6 |
| 18–19 | 6.7 |
| Electric Vehicle | Battery Capacity (kWh) |
|---|---|
| Toyota Prius PHEV | 8.8 |
| Renault Zoe | 41 |
| Nissan Leaf | 40 |
| BMW i3 | 33.2 |
| Volkswagen e-Golf | 35.8 |
| Dacia Spring | 26.8 |
| Electric Vehicle | Charging Time at Home (2.3 kW) (h) | Charging Time at Regular 22 kW Charging Station (h) | Charging Time at Fast 50 kW Charging Station (h) |
|---|---|---|---|
| Toyota Prius PHEV | 4 | 3 | 2 |
| Renault Zoe | 19 | 2 | 0.75 |
| Nissan Leaf | 19 | 7 | 0.5 |
| BMW i3 | 14 | 3 | 0.25 |
| Volkswagen e-Golf | 16.5 | 5.25 | 0.7 |
| Dacia Spring | 13.75 | 5 | 1 |
| Bus | Bus Voltage Level for Power Line Supplied from Sovata and Fântânele (%) | Bus Voltage Level for Power Line Supplied from Sovata (%) | Bus Voltage Level for Power Line Supplied from Fântânele (%) |
|---|---|---|---|
| BL2 | 97.73 | 97.3 | 95.85 |
| BL3 | 98.06 | 97.36 | 96.46 |
| BL4 | 99.25 | 98.34 | 97.89 |
| BL6 | 98.19 | 97.27 | 96.81 |
| BL7 | 98.93 | 98.02 | 97.57 |
| BL8 | 99 | 98.09 | 97.64 |
| BL9 | 98.54 | 97.62 | 97.17 |
| BL10 | 98.94 | 98.03 | 97.58 |
| BL11 | 98.47 | 97.55 | 97.1 |
| BL12 | 98.89 | 97.97 | 97.52 |
| BL13 | 98.88 | 97.97 | 97.52 |
| BL14 | 98.82 | 97.9 | 97.45 |
| BL15 | 98.63 | 97.49 | 97.42 |
| BL17 | 97.33 | 95.2 | 96.75 |
| BL18 | 95.33 | 92.57 | 95.07 |
| Power Losses (kW, kVar) | Power Line Supplied from Sovata and Fântânele | Power Line Supplied from Sovata | Power Line Supplied from Fântânele |
|---|---|---|---|
| Active power losses with no EVs (summer) | 12.18 | 46.78 | 39.82 |
| Reactive power losses with no EVs (summer) | 83.03 | 127.34 | 117.48 |
| Active power losses with EVs (summer) | 65.37 | 338.76 | 224.98 |
| Reactive power losses with EVs (summer) | 766.81 | 1167.52 | 990.68 |
| Active power losses with no EVs (winter) | 18.79 | 114.83 | 61.86 |
| Reactive power losses with no EVs (winter) | 128.34 | 72.93 | 182.44 |
| Active power losses with EVs (winter) | 78.94 | 409.41 | 275.11 |
| Reactive power losses with EVs (winter) | 883.02 | 1376.79 | 1160.25 |
| Power Losses (kW, kVar) | Power Line Supplied from Sovata and Fântânele | Power Line Supplied from Sovata | Power Line Supplied from Fântânele |
|---|---|---|---|
| Active power losses with no EVs (summer) | 12.55 | 48.21 | 41.04 |
| Reactive power losses with no EVs (summer) | 85.53 | 131.25 | 121.05 |
| Active power losses with EVs (summer) | 13.19 | 51.43 | 43.3 |
| Reactive power losses with EVs (summer) | 92.02 | 141.15 | 129.63 |
| Active power losses with no EVs (winter) | 20.06 | 78.03 | 66.14 |
| Reactive power losses with no EVs (winter) | 137.12 | 212.59 | 195.06 |
| Active power losses with EVs (winter) | 20.86 | 82.43 | 69.01 |
| Reactive power losses with EVs (winter) | 145.21 | 226.49 | 205.86 |
| Bus | Bus Voltage Level for Power Line Supplied from Sovata and Fântânele (%) | Bus Voltage Level for Power Line Supplied from Sovata (%) | Bus Voltage Level for Power Line Supplied from Fântânele (%) |
|---|---|---|---|
| Sovata (no EVs in summer) | 100 | 100 | 97.46 |
| Fântânele (no EVs in summer) | 100 | 97.15 | 100 |
| BL2 (no EVs in summer) | 98.57 | 98.11 | 96.4 |
| BL17 (no EVs in summer) | 98.24 | 96.17 | 97.5 |
| BL18 (no EVs in summer) | 97.32 | 94.76 | 96.98 |
| Sovata (EVs in summer) | 100 | 100 | 94.64 |
| Fântânele (EVs in summer) | 100 | 93.01 | 100 |
| BL2 (EVs in summer) | 97.8 | 96.63 | 93.15 |
| BL17 (EVs in summer) | 97.15 | 91.93 | 95.53 |
| BL18 (EVs in summer) | 96.35 | 89.91 | 95.61 |
| Sovata (no EVs in winter) | 100 | 100 | 96.83 |
| Fântânele (no EVs in winter) | 100 | 96.43 | 100 |
| BL2 (no EVs in winter) | 98.22 | 97.64 | 95.5 |
| BL17 (no EVs in winter) | 97.81 | 95.21 | 96.88 |
| BL18 (no EVs in winter) | 96.65 | 93.42 | 96.22 |
| Sovata (EVs in winter) | 100 | 100 | 93.94 |
| Fântânele (EVs in winter) | 100 | 92.15 | 100 |
| BL2 (EVs in winter) | 97.42 | 96.09 | 92.13 |
| BL17 (EVs in winter) | 96.67 | 90.77 | 94.83 |
| BL18 (EVs in winter) | 95.6 | 88.28 | 94.75 |
| Bus | Bus Voltage Level for Power Line Supplied from Sovata and Fântânele (%) | Bus Voltage Level for Power Line Supplied from Sovata (%) | Bus Voltage Level for Power Line Supplied from Fântânele (%) |
|---|---|---|---|
| Sovata (no EVs in summer) | 100 | 100 | 97.42 |
| Fântânele (nos EVs in summer) | 100 | 97.11 | 100 |
| BL2 (no EVs in summer) | 98.55 | 98.08 | 96.35 |
| BL17 (no EVs in summer) | 98.22 | 96.11 | 97.46 |
| BL18 (no EVs in summer) | 97.28 | 94.68 | 96.94 |
| Sovata (EVs in summer) | 100 | 100 | 97.36 |
| Fântânele (EVs in summer) | 100 | 97.02 | 100 |
| BL2 (EVs in summer) | 98.54 | 98.05 | 96.28 |
| BL17 (EVs in summer) | 98.2 | 96.03 | 97.43 |
| BL18 (EVs in summer) | 97.26 | 94.59 | 96.91 |
| Sovata (no EVs in winter) | 100 | 100 | 96.72 |
| Fântânele (no EVs in winter) | 100 | 96.31 | 100 |
| BL2 (no EVs in winter) | 98.16 | 97.56 | 95.35 |
| BL17 (no EVs in winter) | 97.74 | 95.04 | 96.77 |
| BL18 (no EVs in winter) | 96.53 | 93.19 | 96.09 |
| Sovata (EVs in winter) | 100 | 100 | 96.66 |
| Fântânele (EVs in winter) | 100 | 96.22 | 100 |
| BL2 (EVs in winter) | 98.15 | 97.53 | 95.28 |
| BL17 (EVs in winter) | 97.72 | 94.96 | 96.74 |
| BL18 (EVs in winter) | 96.51 | 93.08 | 96.07 |
| Power Losses (kW, kVar) | Power Line Supplied from Sovata and Fântânele | Power Line Supplied from Sovata | Power Line Supplied from Fântânele |
|---|---|---|---|
| Active power losses with no EVs (summer) | 16.78 | 64.49 | 55.15 |
| Reactive power losses with no EVs (summer) | 114.59 | 176.91 | 162.67 |
| Active power losses with EVs (summer) | 74.96 | 38.85 | 260.36 |
| Reactive power losses with EVs (summer) | 849.14 | 1315.17 | 1105.95 |
| Active power losses with no EVs (winter) | 29.01 | 114.83 | 96.37 |
| Reactive power losses with no EVs (winter) | 198.73 | 313.99 | 284.14 |
| Active power losses with EVs (winter) | 98.05 | 511.05 | 346.57 |
| Reactive power losses with EVs (winter) | 1044.15 | 1676.93 | 1398.9 |
| Power Losses (kW, kVar) | Power Line Supplied from Sovata and Fântânele | Power Line Supplied from Sovata | Power Line Supplied from Fântânele |
|---|---|---|---|
| Active power losses with no EVs (summer) | 14.89 | 50.52 | 40.24 |
| Reactive power losses with no EVs (summer) | 120.91 | 183.36 | 168.57 |
| Active power losses with EVs (summer) | 15.59 | 53.90 | 42.59 |
| Reactive power losses with EVs (summer) | 128.64 | 195.46 | 179.04 |
| Active power losses with no EVs (winter) | 33.08 | 131.72 | 110.24 |
| Reactive power losses with no EVs (winter) | 210.04 | 360.39 | 324.98 |
| Active power losses with EVs (winter) | 34.13 | 137.29 | 114.48 |
| Reactive power losses with EVs (winter) | 237.42 | 377.50 | 340.96 |
| Bus | Bus Voltage Level for Power Line Supplied from Sovata and Fântânele (%) | Bus Voltage Level for Power Line Supplied from Sovata (%) | Bus Voltage Level for Power Line Supplied from Fântânele (%) |
|---|---|---|---|
| Sovata (no EVs in summer) | 100 | 100 | 97.01 |
| Fântânele (no EVs in summer) | 100 | 96.64 | 100 |
| BL2 (no EVs in summer) | 98.32 | 97.77 | 96.07 |
| BL17 (no EVs in summer) | 97.93 | 95.48 | 97.27 |
| BL18 (no EVs in summer) | 96.84 | 93.8 | 96.71 |
| Sovata (EVs in summer) | 100 | 100 | 94.14 |
| Fântânele (EVs in summer) | 100 | 92.39 | 100 |
| BL2 (EVs in summer) | 97.53 | 96.24 | 92.42 |
| BL17 (EVs in summer) | 96.8 | 91.1 | 95.03 |
| BL18 (EVs in summer) | 95.81 | 88.75 | 95 |
| Sovata (no EVs in winter) | 100 | 100 | 96.04 |
| Fântânele (no EVs in winter) | 100 | 95.52 | 100 |
| BL2 (no EVs in winter) | 97.78 | 97.05 | 94.37 |
| BL17 (no EVs in winter) | 97.27 | 93.98 | 96.1 |
| BL18 (no EVs in winter) | 95.8 | 91.69 | 95.26 |
| Sovata (EVs in winter) | 100 | 100 | 93.06 |
| Fântânele (EVs in winter) | 100 | 91.04 | 100 |
| BL2 (EVs in winter) | 96.64 | 95.41 | 90.84 |
| BL17 (EVs in winter) | 96.07 | 89.28 | 93.94 |
| BL18 (EVs in winter) | 94.65 | 86.15 | 93.66 |
| Bus | Bus Voltage Level for Power Line Supplied from Sovata and Fântânele (%) | Bus Voltage Level for Power Line Supplied from Sovata (%) | Bus Voltage Level for Power Line Supplied from Fântânele (%) |
|---|---|---|---|
| Sovata (no EVs in summer) | 100 | 100 | 97.66 |
| Fântânele (no EVs in summer) | 100 | 97.16 | 100 |
| BL2 (no EVs in summer) | 98.31 | 97.91 | 96.38 |
| BL17 (no EVs in summer) | 97.94 | 95.98 | 97.35 |
| BL18 (no EVs in summer) | 96.77 | 94.25 | 96.5 |
| Sovata (EVs in summer) | 100 | 100 | 97.61 |
| Fântânele (EVs in summer) | 100 | 97.09 | 100 |
| BL2 (EVs in summer) | 98.3 | 97.89 | 96.33 |
| BL17 (EVs in summer) | 97.92 | 95.91 | 97.32 |
| BL18 (EVs in summer) | 96.75 | 94.17 | 96.48 |
| Sovata (no EVs in winter) | 100 | 100 | 95.76 |
| Fântânele (no EVs in winter) | 100 | 95.2 | 100 |
| BL2 (no EVs in winter) | 97.63 | 96.84 | 93.38 |
| BL17 (no EVs in winter) | 97.09 | 93.55 | 95.83 |
| BL18 (no EVs in winter) | 95.5 | 91.08 | 94.92 |
| Sovata (EVs in winter) | 100 | 100 | 95.7 |
| Fântânele (EVs in winter) | 100 | 95.1 | 100 |
| BL2 (EVs in winter) | 97.62 | 96.81 | 93.9 |
| BL17 (EVs in winter) | 97.06 | 93.46 | 95.78 |
| BL18 (Evs in winter) | 95.48 | 90.97 | 94.89 |
| Power Losses (kW, kVar) | Power Line Supplied from Sovata and Fântânele | Power Line Supplied from Sovata | Power Line Supplied from Fântânele |
|---|---|---|---|
| Active power losses with no EVs (summer) | 9.2 | 27 | 18.45 |
| Reactive power losses with no EVs (summer) | 194.05 | 227.45 | 213.86 |
| Active power losses with EVs (summer) | 46.56 | 237.67 | 134.19 |
| Reactive power losses with EVs (summer) | 923.82 | 1275.15 | 1092.44 |
| Active power losses with no EVs (winter) | 28.56 | 98.78 | 77.75 |
| Reactive power losses with no EVs (winter) | 232.6 | 360.66 | 326.21 |
| Active power losses with EVs (winter) | 96.8 | 462.39 | 307.14 |
| Reactive power losses with EVs (winter) | 1117.97 | 1789.72 | 1502.08 |
| Power Losses (kW, kVar) | Power Line Supplied from Sovata and Fântânele | Power Line Supplied from Sovata | Power Line Supplied from Fântânele |
|---|---|---|---|
| Active power losses with no EVs (summer) | 10.39 | 25.8 | 18.97 |
| Reactive power losses with no EVs (summer) | 255.65 | 284.37 | 272.7 |
| Active power losses with EVs (summer) | 10.49 | 26.67 | 19.14 |
| Reactive power losses with EVs (summer) | 262.91 | 295.45 | 280.51 |
| Active power losses with no EVs (winter) | 26.37 | 91.63 | 71 |
| Reactive power losses with no EVs (winter) | 228.23 | 347.86 | 314.08 |
| Active power losses with EVs (winter) | 27.29 | 96.21 | 74.08 |
| Reactive power losses with EVs (winter) | 238.85 | 364.87 | 328.42 |
| Bus | Bus Voltage Level for Power Line Supplied from Sovata and Fântânele (%) | Bus Voltage Level for Power Line Supplied from Sovata (%) | Bus Voltage Level for Power Line Supplied from Fântânele (%) |
|---|---|---|---|
| Sovata (no EVs in summer) | 100 | 100 | 98.35 |
| Fântânele (no EVs in summer) | 100 | 97.78 | 100 |
| BL2 (no EVs in summer) | 98.4 | 98.09 | 97.03 |
| BL17 (no EVs in summer) | 98.1 | 96.56 | 97.68 |
| BL18 (no EVs in summer) | 96.76 | 94.79 | 96.57 |
| Sovata (EVs in summer) | 100 | 100 | 96.15 |
| Fântânele (EVs in summer) | 100 | 94.29 | 100 |
| BL2 (EVs in summer) | 97.63 | 96.8 | 94.39 |
| BL17 (EVs in summer) | 97.01 | 92.95 | 96 |
| BL18 (EVs in summer) | 95.74 | 90.52 | 95.27 |
| Sovata (no EVs in winter) | 100 | 100 | 96.74 |
| Fântânele (no EVs in winter) | 100 | 96.01 | 100 |
| BL2 (no EVs in winter) | 97.66 | 97.09 | 94.94 |
| BL17 (no EVs in winter) | 97.14 | 94.35 | 96.3 |
| BL18 (no EVs in winter) | 95.46 | 91.87 | 95.08 |
| Sovata (EVs in winter) | 100 | 100 | 94.4 |
| Fântânele (EVs in winter) | 100 | 92.23 | 100 |
| BL2 (EVs in winter) | 96.83 | 95.67 | 92.06 |
| BL17 (EVs in winter) | 95.96 | 90.35 | 94.45 |
| BL18 (EVs in winter) | 94.29 | 87.03 | 93.59 |
| Bus | Bus Voltage Level for Power Line Supplied from Sovata and Fântânele (%) | Bus Voltage Level for Power Line Supplied from Sovata (%) | Bus Voltage Level for Power Line Supplied from Fântânele (%) |
|---|---|---|---|
| Sovata (no EVs in summer) | 100 | 100 | 98.57 |
| Fântânele (no EVs in summer) | 100 | 97.98 | 100 |
| BL2 (no EVs in summer) | 98.42 | 98.14 | 97.24 |
| BL17 (no EVs in summer) | 98.15 | 96.75 | 97.78 |
| BL18 (no EVs in summer) | 96.75 | 94.96 | 96.59 |
| Sovata (EVs in summer) | 100 | 100 | 98.52 |
| Fântânele (EVs in summer) | 100 | 97.9 | 100 |
| BL2 (EVs in summer) | 98.41 | 98.12 | 97.19 |
| BL17 (EVs in summer) | 98.13 | 96.68 | 97.75 |
| BL18 (EVs in summer) | 96.73 | 94.87 | 96.56 |
| Sovata (no EVs in winter) | 100 | 100 | 96.84 |
| Fântânele (no EVs in winter) | 100 | 96.12 | 100 |
| BL2 (no EVs in winter) | 97.69 | 97.14 | 95.06 |
| BL17 (no EVs in winter) | 97.18 | 94.47 | 96.37 |
| BL18 (no EVs in winter) | 95.51 | 92.01 | 95.14 |
| Sovata (EVs in winter) | 100 | 100 | 96.79 |
| Fântânele (EVs in winter) | 100 | 96.04 | 100 |
| BL2 (EVs in winter) | 97.68 | 97.12 | 95.01 |
| BL17 (EVs in winter) | 97.16 | 94.4 | 96.34 |
| BL18 (EVs in winter) | 95.49 | 91.92 | 95.11 |
| Power Losses (kW, kVar) | Power Line Supplied from Sovata and Fântânele | Power Line Supplied from Sovata | Power Line Supplied from Fântânele |
|---|---|---|---|
| Active power losses with no EVs (summer) | 13.02 | 27.07 | 23.21 |
| Reactive power losses with no EVs (summer) | 329.38 | 354.03 | 346.12 |
| Active power losses with EVs (summer) | 36.72 | 177.89 | 88.999 |
| Reactive power losses with EVs (summer) | 1039.6 | 1313.58 | 1152.02 |
| Active power losses with no EVs (winter) | 22.88 | 79.8 | 60.32 |
| Reactive power losses with no EVs (winter) | 219.78 | 324.88 | 293.08 |
| Active power losses with EVs (winter) | 84.9 | 412.65 | 266.01 |
| Reactive power losses with EVs (winter) | 1082.77 | 1687.45 | 1417.35 |
| Power Losses (kW, kVar) | Power Line Supplied from Sovata and Fântânele | Power Line Supplied from Sovata | Power Line Supplied from Fântânele |
|---|---|---|---|
| Active power losses with no EVs (summer) | 13.16 | 27.25 | 23.47 |
| Reactive power losses with no EVs (summer) | 332.67 | 357.33 | 349.51 |
| Active power losses with EVs (summer) | 13.08 | 27.37 | 23 |
| Reactive power losses with EVs (summer) | 338.45 | 365.21 | 356.35 |
| Active power losses with no EVs (winter) | 22.79 | 79.4 | 60.17 |
| Reactive power losses with no EVs (winter) | 216.79 | 321.16 | 289.84 |
| Active power losses with EVs (winter) | 23.62 | 83.56 | 62.93 |
| Reactive power losses with EVs (winter) | 227.01 | 337.22 | 303.41 |
| Bus | Bus Voltage Level for Power Line Supplied from Sovata and Fântânele (%) | Bus Voltage Level for Power Line Supplied from Sovata (%) | Bus Voltage Level for Power Line Supplied from Fântânele (%) |
|---|---|---|---|
| Sovata (no EVs in summer) | 100 | 100 | 98.86 |
| Fântânele (no EVs in summer) | 100 | 98.26 | 100 |
| BL2 (no EVs in summer) | 98.5 | 98.25 | 97.56 |
| BL17 (no EVs in summer) | 98.26 | 97.05 | 97.96 |
| BL18 (no EVs in summer) | 96.84 | 95.3 | 96.71 |
| Sovata (EVs in summer) | 100 | 100 | 96.7 |
| Fântânele (EVs in summer) | 100 | 94.82 | 100 |
| BL2 (EVs in summer) | 97.74 | 96.99 | 94.96 |
| BL17 (EVs in summer) | 97.17 | 93.5 | 96.32 |
| BL18 (EVs in summer) | 95.82 | 91.1 | 95.43 |
| Sovata (no EVs in winter) | 100 | 100 | 97.03 |
| Fântânele (no EVs in winter) | 100 | 96.32 | 100 |
| BL2 (no EVs in winter) | 97.77 | 97.24 | 95.3 |
| BL17 (no EVs in winter) | 97.28 | 94.72 | 96.52 |
| BL18 (no EVs in winter) | 95.63 | 92.32 | 95.28 |
| Sovata (EVs in winter) | 100 | 100 | 94.72 |
| Fântânele (EVs in winter) | 100 | 92.59 | 100 |
| BL2 (EVs in winter) | 96.94 | 95.84 | 92.46 |
| BL17 (EVs in winter) | 96.11 | 90.78 | 94.7 |
| BL18 (EVs in winter) | 94.48 | 87.57 | 93.82 |
| Bus | Bus Voltage Level for Power Line Supplied from Sovata and Fântânele (%) | Bus Voltage Level For Power Line Supplied from Sovata (%) | Bus Voltage Level for Power Line Supplied from Fântânele (%) |
|---|---|---|---|
| Sovata (no EVs in summer) | 100 | 100 | 98.87 |
| Fântânele (no EVs in summer) | 100 | 98.26 | 100 |
| BL2 (no EVs in summer) | 98.5 | 98.26 | 97.56 |
| BL17 (no EVs in summer) | 98.26 | 97.06 | 97.97 |
| BL18 (no EVs in summer) | 96.84 | 95.3 | 96.71 |
| Sovata (EVs in summer) | 100 | 100 | 98.82 |
| Fântânele (EVs in summer) | 100 | 98.19 | 100 |
| BL2 (EVs in summer) | 98.49 | 98.23 | 97.51 |
| BL17 (EVs in summer) | 98.24 | 96.99 | 97.94 |
| BL18 (EVs in summer) | 96.82 | 95.22 | 96.68 |
| Sovata (no EVs in winter) | 100 | 100 | 97.04 |
| Fântânele (no EVs in winter) | 100 | 96.33 | 100 |
| BL2 (no EVs in winter) | 97.78 | 97.26 | 95.32 |
| BL17 (no EVs in winter) | 97.3 | 94.74 | 96.54 |
| BL18 (no EVs in winter) | 95.66 | 92.36 | 95.31 |
| Sovata (EVs in winter) | 100 | 100 | 96.99 |
| Fântânele (EVs in winter) | 100 | 96.26 | 100 |
| BL2 (EVs in winter) | 97.77 | 97.23 | 95.26 |
| BL17 (EVs in winter) | 97.28 | 94.66 | 96.5 |
| BL18 (EVs in winter) | 95.64 | 92.27 | 95.29 |
| Power Losses (kW, kVar) | Power Line Supplied from Sovata and Fântânele | Power Line Supplied from Sovata | Power Line Supplied from Fântânele |
|---|---|---|---|
| Active power losses with no EVs (summer) | 10.6 | 24.99 | 19.08 |
| Reactive power losses with no EVs (summer) | 266.14 | 292.67 | 282.26 |
| Active power losses with EVs (summer) | 39.59 | 198.67 | 104.04 |
| Reactive power losses with EVs (summer) | 983 | 1284.37 | 1114.78 |
| Active power losses with no EVs (winter) | 29.13 | 101.05 | 79.08 |
| Reactive power losses with no EVs (winter) | 241.65 | 373.36 | 337.12 |
| Active power losses with EVs (winter) | 97.65 | 467.11 | 309.3 |
| Reactive power losses with EVs (winter) | 1136.48 | 1818.74 | 1524.67 |
| Power Losses (kW, kVar) | Power Line Supplied from Sovata and Fântânele | Power Line Supplied from Sovata | Power Line Supplied from Fântânele |
|---|---|---|---|
| Active power losses with no EVs (summer) | 9.17 | 26.4 | 18.12 |
| Reactive power losses with no EVs (summer) | 198.67 | 231.02 | 217.76 |
| Active power losses with EVs (summer) | 9.4 | 27.83 | 18.76 |
| Reactive power losses with EVs (summer) | 206 | 240.65 | 226.11 |
| Active power losses with no EVs (winter) | 32.11 | 110.85 | 88.12 |
| Reactive power losses with no EVs (winter) | 251.16 | 394.73 | 357.09 |
| Active power losses with EVs (winter) | 33.16 | 116.04 | 91.67 |
| Reactive power losses with EVs (winter) | 262.37 | 413.14 | 372.55 |
| Bus | Bus Voltage Level for Power Line Supplied from Sovata and Fântânele (%) | Bus Voltage Level for Power Line Supplied from Sovata (%) | Bus Voltage Level for Power Line Supplied from Fântânele (%) |
|---|---|---|---|
| Sovata (no EVs in summer) | 100 | 100 | 98.67 |
| Fântânele (no EVs in summer) | 100 | 98.08 | 100 |
| BL2 (no EVs in summer) | 98.46 | 98.2 | 97.36 |
| BL17 (no EVs in summer) | 98.2 | 96.88 | 97.86 |
| BL18 (no EVs in summer) | 96.82 | 95.12 | 96.67 |
| Sovata (EVs in summer) | 100 | 100 | 96.49 |
| Fântânele (EVs in summer) | 100 | 94.62 | 100 |
| BL2 (EVs in summer) | 97.7 | 96.92 | 94.75 |
| BL17 (EVs in summer) | 97.12 | 93.3 | 96.21 |
| BL18 (EVs in summer) | 95.8 | 90.9 | 95.38 |
| Sovata (no EVs in winter) | 100 | 100 | 96.69 |
| Fântânele (no EVs in winter) | 100 | 95.95 | 100 |
| BL2 (no EVs in winter) | 97.62 | 97.04 | 94.86 |
| BL17 (no EVs in winter) | 97.09 | 94.26 | 96.23 |
| BL18 (no EVs in winter) | 95.37 | 91.71 | 94.98 |
| Sovata (EVs in winter) | 100 | 100 | 94.35 |
| Fântânele (EVs in winter) | 100 | 92.15 | 100 |
| BL2 (EVs in winter) | 96.78 | 95.61 | 91.97 |
| BL17 (EVs in winter) | 95.9 | 90.24 | 94.38 |
| BL18 (EVs in winter) | 94.19 | 86.84 | 93.48 |
| Bus | Bus Voltage Level for Power Line Supplied from Sovata and Fântânele (%) | Bus Voltage Level for Power Line Supplied from Sovata (%) | Bus Voltage Level for Power Line Supplied from Fântânele (%) |
|---|---|---|---|
| Sovata (no EVs in summer) | 100 | 100 | 98.38 |
| Fântânele (no EVs in summer) | 100 | 97.81 | 100 |
| BL2 (no EVs in summer) | 98.41 | 98.1 | 97.07 |
| BL17 (no EVs in summer) | 98.11 | 96.6 | 97.7 |
| BL18 (no EVs in summer) | 96.77 | 94.83 | 96.59 |
| Sovata (EVs in summer) | 100 | 100 | 98.33 |
| Fântânele (EVs in summer) | 100 | 97.74 | 100 |
| BL2 (EVs in summer) | 98.4 | 98.08 | 97.02 |
| BL17 (EVs in summer) | 98.09 | 96.53 | 97.67 |
| BL18 (EVs in summer) | 96.76 | 94.75 | 96.57 |
| Sovata (no EVs in winter) | 100 | 100 | 96.56 |
| Fântânele (no EVs in winter) | 100 | 95.8 | 100 |
| BL2 (no EVs in winter) | 97.57 | 96.97 | 94.69 |
| BL17 (no EVs in winter) | 97.02 | 94.08 | 96.13 |
| BL18 (no EVs in winter) | 95.29 | 91.49 | 94.88 |
| Sovata (EVs in winter) | 100 | 100 | 96.51 |
| Fântânele (EVs in winter) | 100 | 95.72 | 100 |
| BL2 (EVs in winter) | 97.55 | 96.94 | 94.64 |
| BL17 (EVs in winter) | 97 | 94 | 96.09 |
| BL18 (EVs in winter) | 95.27 | 91.4 | 94.85 |
| Power Losses (kW, kVar) | Power Line Supplied from Sovata and Fântânele | Power Line Supplied from Sovata | Power Line Supplied from Fântânele |
|---|---|---|---|
| Active power losses with no EVs (summer) | 14.26 | 49.03 | 36.95 |
| Reactive power losses with no EVs (summer) | 145.2 | 207.63 | 189.67 |
| Active power losses with EVs (summer) | 67.71 | 333.63 | 212.43 |
| Reactive power losses with EVs (summer) | 914.26 | 1387.95 | 1175.29 |
| Active power losses with no EVs (winter) | 33.59 | 133.84 | 111.98 |
| Reactive power losses with no EVs (winter) | 230.35 | 366.22 | 330.1 |
| Active power losses with EVs (winter) | 106.11 | 554.54 | 377.12 |
| Reactive power losses with EVs (winter) | 1111.02 | 1805.03 | 1499.43 |
| Power Losses (kW, kVar) | Power Line Supplied from Sovata and Fântânele | Power Line Supplied from Sovata | Power Line Supplied from Fântânele |
|---|---|---|---|
| Active power losses with no EVs (summer) | 14.93 | 50.97 | 39.89 |
| Reactive power losses with no EVs (summer) | 129.71 | 193.4 | 177.25 |
| Active power losses with EVs (summer) | 15.61 | 54.32 | 42.18 |
| Reactive power losses with EVs (summer) | 137.69 | 205.74 | 187.93 |
| Active power losses with no EVs (winter) | 29.5 | 116.83 | 98.01 |
| Reactive power losses with no EVs (winter) | 202.06 | 319.46 | 288.97 |
| Active power losses with EVs (winter) | 30.48 | 122.04 | 101.6 |
| Reactive power losses with EVs (winter) | 212.04 | 335.46 | 302.38 |
| Bus | Bus Voltage Level for Power Line Supplied from Sovata and Fântânele (%) | Bus Voltage Level for Power Line Supplied from Sovata (%) | Bus Voltage Level for Power Line Supplied from Fântânele (%) |
|---|---|---|---|
| Sovata (no EVs in summer) | 100 | 100 | 97.66 |
| Fântânele (no EVs in summer) | 100 | 97.1 | 100 |
| BL2 (no EVs in summer) | 98.2 | 97.8 | 96.27 |
| BL17 (no EVs in summer) | 97.82 | 95.81 | 97.23 |
| BL18 (no EVs in summer) | 96.51 | 93.93 | 96.23 |
| Sovata (EVs in summer) | 100 | 100 | 95.41 |
| Fântânele (EVs in summer) | 100 | 93.52 | 100 |
| BL2 (EVs in summer) | 97.43 | 96.47 | 93.55 |
| BL17 (EVs in summer) | 96.71 | 92.09 | 95.5 |
| BL18 (EVs in summer) | 95.46 | 89.52 | 94.9 |
| Sovata (no EVs in winter) | 100 | 100 | 95.73 |
| Fântânele (no EVs in winter) | 100 | 95.16 | 100 |
| BL2 (no EVs in winter) | 97.61 | 96.82 | 93.93 |
| BL17 (no EVs in winter) | 97.06 | 93.5 | 95.79 |
| BL18 (no EVs in winter) | 95.46 | 91.01 | 94.88 |
| Sovata (EVs in winter) | 100 | 100 | 92.71 |
| Fântânele (EVs in winter) | 100 | 90.6 | 100 |
| BL2 (EVs in winter) | 96.75 | 95.15 | 90.33 |
| BL17 (EVs in winter) | 95.84 | 88.68 | 93.6 |
| BL18 (EVs in winter) | 94.27 | 85.29 | 93.23 |
| Bus | Bus Voltage Level for Power Line Supplied from Sovata and Fântânele (%) | Bus Voltage Level for Power Line Supplied from Sovata (%) | Bus Voltage Level for Power Line Supplied from Fântânele (%) |
|---|---|---|---|
| Sovata (no EVs in summer) | 100 | 100 | 97.64 |
| Fântânele (no EVs in summer) | 100 | 97.11 | 100 |
| BL2 (no EVs in summer) | 98.26 | 97.86 | 96.31 |
| BL17 (no EVs in summer) | 97.88 | 95.89 | 97.28 |
| BL18 (no EVs in summer) | 96.65 | 94.09 | 96.38 |
| Sovata (EVs in summer) | 100 | 100 | 97.59 |
| Fântânele (EVs in summer) | 100 | 97.04 | 100 |
| BL2 (EVs in summer) | 98.25 | 97.83 | 96.26 |
| BL17 (EVs in summer) | 97.87 | 95.81 | 97.25 |
| BL18 (EVs in summer) | 96.64 | 94.01 | 96.36 |
| Sovata (no EVs in winter) | 100 | 100 | 96.01 |
| Fântânele (no EVs in winter) | 100 | 95.48 | 100 |
| BL2 (no EVs in winter) | 97.77 | 97.02 | 94.33 |
| BL17 (no EVs in winter) | 97.25 | 93.93 | 96.07 |
| BL18 (no EVs in winter) | 95.76 | 91.62 | 95.22 |
| Sovata (EVs in winter) | 100 | 100 | 95.94 |
| Fântânele (EVs in winter) | 100 | 95.39 | 100 |
| BL2 (EVs in winter) | 97.75 | 96.99 | 94.26 |
| BL17 (EVs in winter) | 97.23 | 93.84 | 96.03 |
| BL18 (EVs in winter) | 95.74 | 91.51 | 95.19 |
| Power Losses (kW, kVar) | Power Line Supplied from Sovata and Fântânele | Power Line Supplied from Sovata | Power Line Supplied from Fântânele |
|---|---|---|---|
| Active power losses with no EVs (summer) | 12.89 | 49.53 | 42.15 |
| Reactive power losses with no EVs (summer) | 87.82 | 134.83 | 124.32 |
| Active power losses with EVs (summer) | 66.87 | 346.54 | 230.52 |
| Reactive power losses with EVs (summer) | 779.79 | 1190.62 | 1009.52 |
| Active power losses with no EVs (winter) | 20.56 | 80.02 | 67.8 |
| Reactive power losses with no EVs (winter) | 140.52 | 218 | 199.96 |
| Active power losses with EVs (winter) | 82.39 | 427.51 | 287.9 |
| Reactive power losses with EVs (winter) | 912.21 | 1430.28 | 1203.18 |
| Power Losses (kW, kVar) | Power Line Supplied from Sovata and Fântânele | Power Line Supplied from Sovata | Power Line Supplied from Fântânele |
|---|---|---|---|
| Active power losses with no EVs (summer) | 12.17 | 46.73 | 39.78 |
| Reactive power losses with no EVs (summer) | 82.95 | 127.21 | 117.36 |
| Active power losses with EVs (summer) | 12.8 | 49.9 | 42.02 |
| Reactive power losses with EVs (summer) | 89.34 | 136.95 | 125.8 |
| Active power losses with no EVs (winter) | 19.34 | 75.11 | 63.69 |
| Reactive power losses with no EVs (winter) | 132.09 | 204.6 | 187.82 |
| Active power losses with EVs (winter) | 20.13 | 79.18 | 66.54 |
| Reactive power losses with EVs (winter) | 140.14 | 217.07 | 198.55 |
| Bus | Bus Voltage Level for Power Line Supplied from Sovata and Fântânele (%) | Bus Voltage Level For Power Line Supplied from Sovata (%) | Bus Voltage Level for Power Line Supplied from Fântânele (%) |
|---|---|---|---|
| Sovata (no EVs in summer) | 100 | 100 | 97.39 |
| Fântânele (no EVs in summer) | 100 | 97.07 | 100 |
| BL2 (no EVs in summer) | 98.53 | 98.05 | 96.3 |
| BL17 (no EVs in summer) | 98.19 | 96.06 | 97.43 |
| BL18 (no EVs in summer) | 97.24 | 94.61 | 96.9 |
| Sovata (EVs in summer) | 100 | 100 | 94.56 |
| Fântânele (EVs in summer) | 100 | 92.91 | 100 |
| BL2 (EVs in summer) | 97.76 | 96.57 | 93.03 |
| BL17 (EVs in summer) | 97.09 | 91.8 | 95.45 |
| BL18 (EVs in summer) | 96.26 | 89.72 | 95.51 |
| Sovata (no EVs in winter) | 100 | 100 | 96.68 |
| Fântânele (no EVs in winter) | 100 | 96.26 | 100 |
| BL2 (no EVs in winter) | 98.14 | 97.53 | 95.29 |
| BL17 (no EVs in winter) | 97.71 | 94.98 | 96.73 |
| BL18 (no EVs in winter) | 96.49 | 93.1 | 96.04 |
| Sovata (EVs in winter) | 100 | 100 | 93.77 |
| Fântânele (EVs in winter) | 100 | 91.94 | 100 |
| BL2 (EVs in winter) | 97.33 | 95.97 | 91.89 |
| BL17 (EVs in winter) | 96.56 | 90.49 | 94.66 |
| BL18 (EVs in winter) | 95.42 | 87.88 | 94.55 |
| Bus | Bus Voltage Level for Power Line Supplied from Sovata and Fântânele (%) | Bus Voltage Level for Power Line Supplied from Sovata (%) | Bus Voltage Level for Power Line Supplied from Fântânele (%) |
|---|---|---|---|
| Sovata (no EVs in summer) | 100 | 100 | 97.46 |
| Fântânele (no EVs in summer) | 100 | 97.15 | 100 |
| BL2 (no EVs in summer) | 98.57 | 98.11 | 96.4 |
| BL17 (no EVs in summer) | 98.24 | 96.18 | 97.5 |
| BL18 (no EVs in summer) | 97.32 | 94.77 | 96.99 |
| Sovata (EVs in summer) | 100 | 100 | 97.4 |
| Fântânele (EVs in summer) | 100 | 97.06 | 100 |
| BL2 (EVs in summer) | 98.56 | 98.08 | 96.34 |
| BL17 (EVs in summer) | 98.23 | 96.09 | 97.46 |
| BL18 (EVs in summer) | 97.31 | 94.67 | 96.96 |
| Sovata (no EVs in winter) | 100 | 100 | 96.78 |
| Fântânele (no EVs in winter) | 100 | 96.38 | 100 |
| BL2 (no EVs in winter) | 98.19 | 97.6 | 95.44 |
| BL17 (no EVs in winter) | 97.78 | 95.14 | 96.83 |
| BL18 (no EVs in winter) | 96.6 | 93.32 | 96.17 |
| Sovata (EVs in winter) | 100 | 100 | 96.72 |
| Fântânele (EVs in winter) | 100 | 96.29 | 100 |
| BL2 (EVs in winter) | 98.18 | 97.58 | 95.37 |
| BL17 (EVs in winter) | 97.76 | 95.05 | 96.8 |
| BL18 (EVs in winter) | 96.58 | 93.22 | 96.14 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dulău, L.I.; Bică, D. Impact of Electric Vehicles on a Power Line with Photovoltaic Power Plants Connected. Machines 2022, 10, 102. https://doi.org/10.3390/machines10020102
Dulău LI, Bică D. Impact of Electric Vehicles on a Power Line with Photovoltaic Power Plants Connected. Machines. 2022; 10(2):102. https://doi.org/10.3390/machines10020102
Chicago/Turabian StyleDulău, Lucian Ioan, and Dorin Bică. 2022. "Impact of Electric Vehicles on a Power Line with Photovoltaic Power Plants Connected" Machines 10, no. 2: 102. https://doi.org/10.3390/machines10020102
APA StyleDulău, L. I., & Bică, D. (2022). Impact of Electric Vehicles on a Power Line with Photovoltaic Power Plants Connected. Machines, 10(2), 102. https://doi.org/10.3390/machines10020102





