Hairpin Windings for Electric Vehicle Motors: Modeling and Investigation of AC Loss-Mitigating Approaches
Abstract
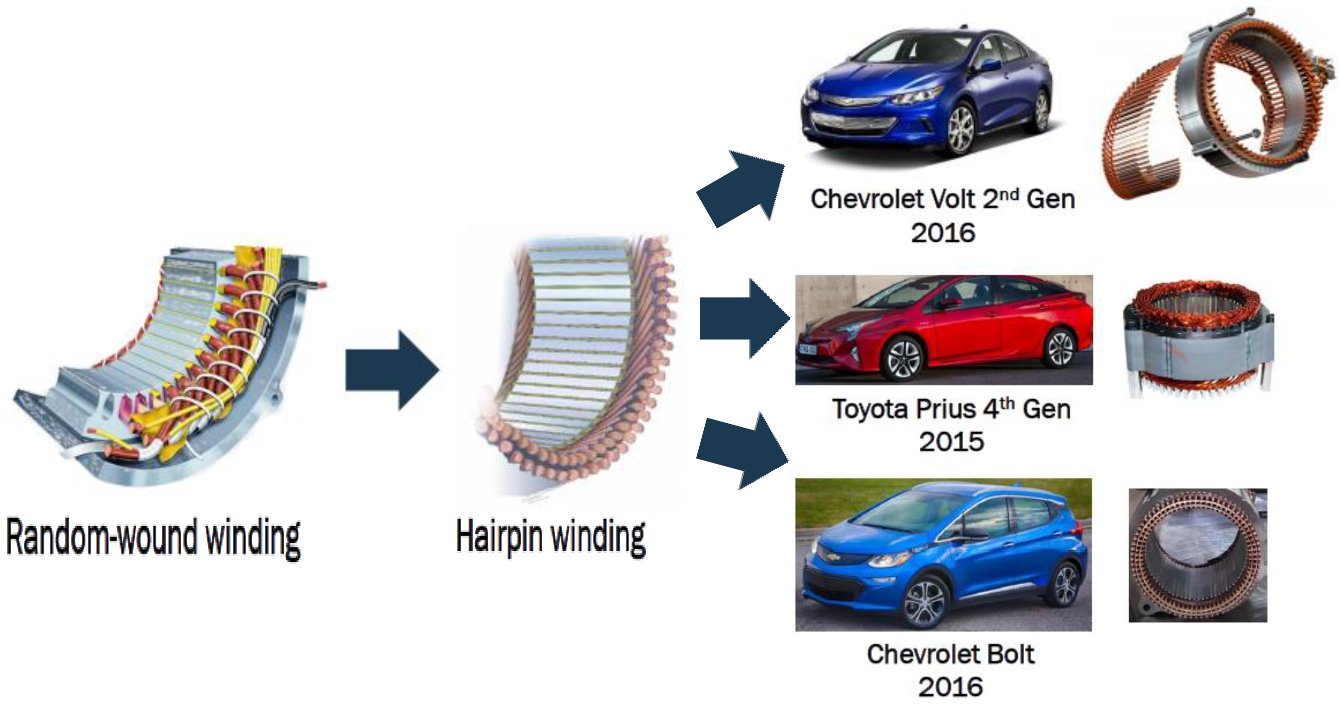
1. Introduction
2. Basic Design Rules of Hairpin Winding and Case Study
2.1. Hairpin Winding Design Principle
- The number of slots per pole pair must be greater than one to provide the possibility of generating electro-motive force (EMF);
- The number of slots per phase per parallel path must be an integer;
- The number of pole pairs per parallel path is equal to 2k, where k is an integer;
- The number of slots per phase divided by the greatest common divisor of the number of slots, and the number of pole pairs (GCD (slots, pole pairs)) must be an integer;
- The number of conductors in the slot must be even.
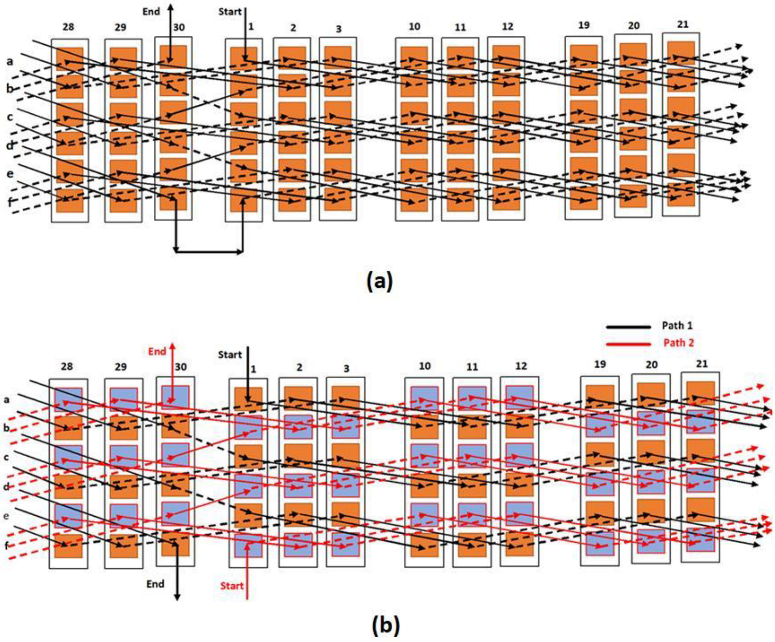
- Layer arrangement rules: In the presence of parallel paths, the wires belonging to one similar path should be placed in all layers of the slot to provide the same inductances for all parallel paths;
- Slot per pole arrangement rule: To make sure that all parallel paths generate the same EMF, the wire that belongs to one similar path should be distributed in all slots per pole per phase.
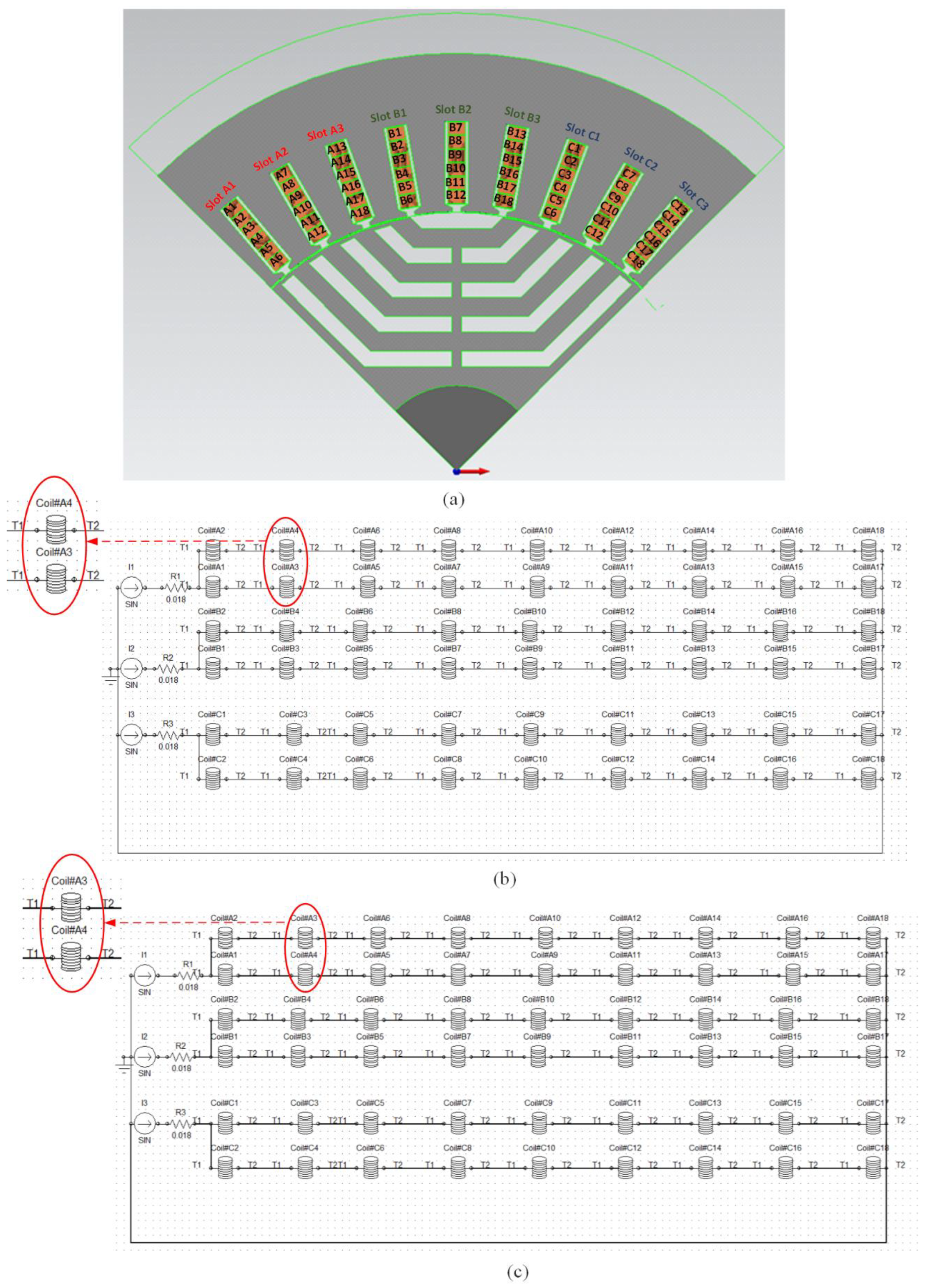
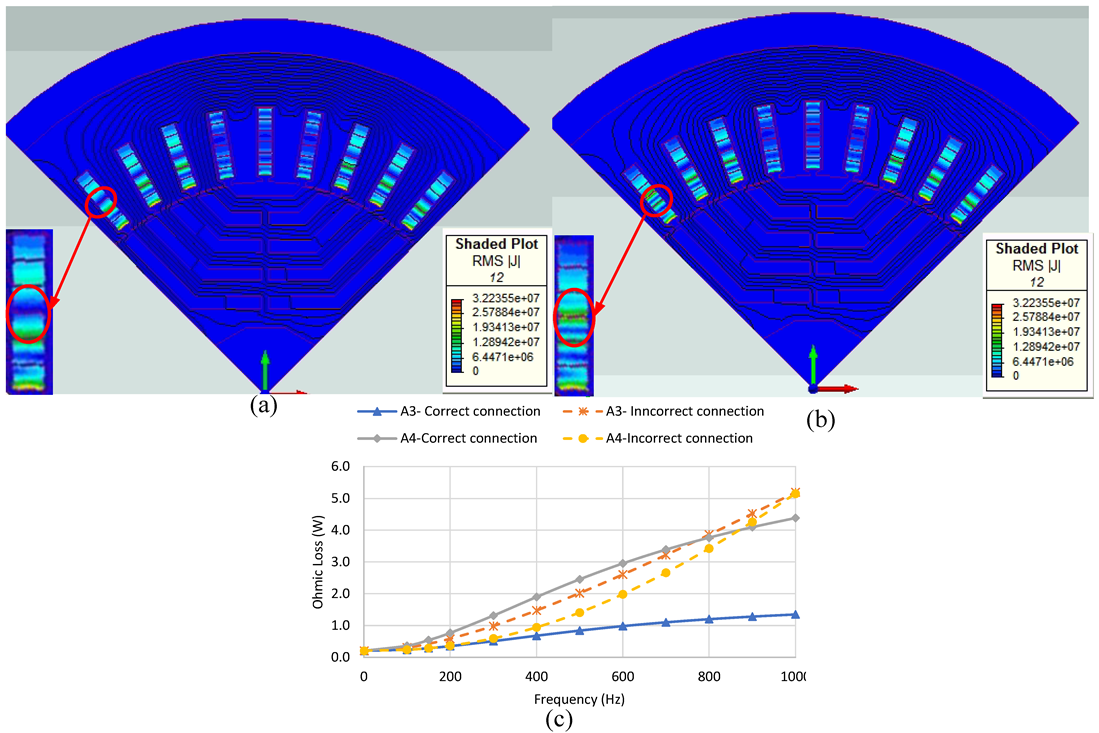
2.2. Additional AC Losses
3. Number of Conductor Layers
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Ghahfarokhi, P.S.; Podgornovs, A.; Kallaste, A.; Vaimann, T.; Belahcen, A.; Cardoso, A.J.M. Oil Spray Cooling with Hairpin Windings in High-Performance Electric Vehicle Motors. In Proceedings of the 28th International Workshop on Electric Drives: Improving Reliability of Electric Drives (IWED), Moscow, Russia, 27–29 January 2021; pp. 1–5. [Google Scholar]
- Preci, E.; Valente, G.; Galassini, A.; Yuan, X.; Degano, M.; Gerada, D.; Buticchi, G.; Gerada, C. Experimental Statistical Method Predicting AC Losses on Random Windings and PWM Effect Evaluation. IEEE Trans. Energy Convers. 2020, 36, 2287–2296. [Google Scholar] [CrossRef]
- Ghahfarokhi, P.S.; Podgornovs, A.; Cardoso, A.J.M.; Kallaste, A.; Belahcen, A.; Vaimann, T. Hairpin Windings Manufacturing, Design, and AC Losses Analysis Approaches for Electric Vehicle Motors. In Proceedings of the 2021 11th International Electric Drives Production Conference (EDPC), Erlangen, Germany, 7–9 December 2021; pp. 1–7. [Google Scholar]
- Ju, X.; Cheng, Y.; Du, B.; Yang, M.; Yang, D.; Cui, S. AC Loss Analysis and Measurement of a Hybrid Transposed Hairpin Winding for EV Traction Machines. IEEE Trans. Ind. Electron. 2022, 1–12. [Google Scholar] [CrossRef]
- Lamichhane, T.N.; Sethuraman, L.; Dalagan, A.; Wang, H.; Keller, J.; Paranthaman, M.P. Additive manufacturing of soft magnets for electrical machines—A review. Mater. Today Phys. 2020, 15, 100255. [Google Scholar] [CrossRef]
- Ghahfarokhi, P.S.; Podgornovs, A.; Kallaste, A.; Cardoso, A.J.; Belahcen, A.; Vaimann, T.; Tiismus, H.; Asad, B. Opportunities and Challenges of Utilizing Additive Manufacturing Approaches in Thermal Management of Electrical Machines. IEEE Access. 2021, 9, 36368–36381. [Google Scholar] [CrossRef]
- Arzillo, A.; Nuzzo, S.; Braglia, P.; Franceschini, G.; Barater, D.; Gerada, D.; Gerada, C. An analytical approach for the design of innovative hairpin winding layouts. In Proceedings of the 2020 International Conference on Electrical Machines, ICEM 2020, Gothenburg, Sweden, 23–26 August 2020; pp. 1534–1539. [Google Scholar]
- Xue, S. Maximising E-Machine Efficiency with Hairpin Windings; White Paper (Report); motor design Ltd.: Wrexham, UK, 2021; pp. 1–7. [Google Scholar]
- Zhao, Y.; Li, D.; Pei, T.; Qu, R. Overview of the rectangular wire windings AC electrical machine. CES Trans. Electr. Mach. Syst. 2019, 3, 160–169. [Google Scholar] [CrossRef]
- Bianchi, N.; Berardi, G. Analytical Approach to Design Hairpin Windings in High Performance Electric Vehicle Motors. In Proceedings of the 2018 IEEE Energy Conversion Congress and Exposition, ECCE 2018, Portland, OR, USA, 23–27 September 2018; pp. 4398–4405. [Google Scholar]
- Liu, C.; Xu, Z.; Gerada, D.; Li, J.; Gerada, C.; Chong, Y.C.; Popescu, M.; Goss, J.; Staton, D.; Zhang, H. Experimental Investigation on Oil Spray Cooling with Hairpin Windings. IEEE Trans. Ind. Electron. 2020, 67, 7343–7353. [Google Scholar] [CrossRef]
- Sano, S.; Yashiro, T.; Takizawa, K.; Mizutani, T. Development of new motor for compact-class hybrid vehicles. World Electr. Veh. J. 2016, 8, 443–449. [Google Scholar] [CrossRef]
- Berardi, G.; Nategh, S.; Bianchi, N.; Thioliere, Y. A Comparison Between Random and Hairpin Winding in E-mobility Applications. In Proceedings of the IECON 2020 The 46th Annual Conference of the IEEE Industrial Electronics Society, Singapore, 18–21 October 2020; pp. 815–820. [Google Scholar]
- Preci, E.; Nuzzo, S.; Valente, G.; Gerada, D.; Barater, D.; Degano, M.; Buticchi, G.; Gerada, C. Segmented Hairpin Topology for Reduced Losses at High Frequency Operations. IEEE Trans. Transp. Electrif. 2021, 8, 688–698. [Google Scholar] [CrossRef]
- Berardi, G.; Bianchi, N. Design Guideline of an AC Hairpin Winding. In Proceedings of the 2018 23rd International Conference on Electrical Machines, ICEM 2018, Alexandroupoli, Greece, 3–6 September 2018; pp. 2444–2450. [Google Scholar]
- Zou, T.; Gerada, D.; La Rocca, A.; Moslemin, M.; Cairns, A.; Cui, M.; Bardalai, A.; Zhang, F.; Gerada, C. A Comprehensive Design Guideline of Hairpin Windings for High Power Density Electric Vehicle Traction Motors. IEEE Trans. Transp. Electrif. 2022, 8, 3578–3593. [Google Scholar] [CrossRef]
- Rosu, M.; Zhou, P.; Lin, D.; Ionel, D.M.; Popescu, M.; Blaabjerg, F.; Rallabandi, V.; Staton, D. Multiphysics Simulation by Design for Electrical Machines, Power Electronics and Drives, 1st ed.; Wiley-IEEE Press: West Sussex, UK, 2017. [Google Scholar]
- Pyrhönen, J.; Jokinen, T.; Hrabovcová, V. Design of Rotating Electrical Machines; Wiley: West Sussex, UK, 2008. [Google Scholar]
- Ghahfarokhi, P.S.; Podgornovs, A.; Cardoso, A.J.M.; Kallaste, A.; Belahcen, A.; Vaimann, T. AC Losses Analysis Approaches for Electric Vehicle Motors with Hairpin Winding Configuration. In Proceedings of the IECON Proceedings (Industrial Electronics Conference), Toronto, ON, Canada, 13–16 October 2021. [Google Scholar]
- Mellor, P.; Wrobel, R.; Simpson, N. AC losses in high frequency electrical machine windings formed from large section conductors. In Proceedings of the 2014 IEEE Energy Conversion Congress and Exposition, ECCE 2014, Pittsburgh, PA, USA, 14–18 September 2014; pp. 5563–5570. [Google Scholar]


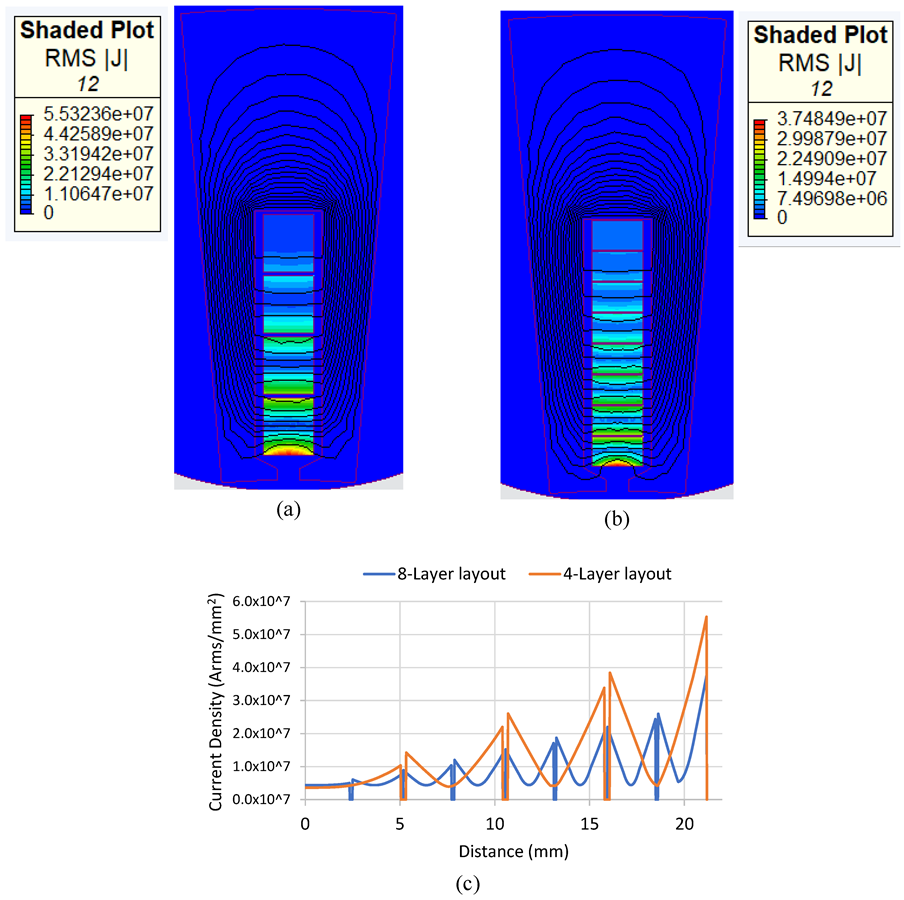

| Parameter | Symbol | Quantity |
|---|---|---|
| Pole pitch | τ | 106.2 mm |
| Machine stack length | L | 156.1 mm |
| Air gap thickness | g | 0.4 mm |
| Rotor outer diameter of the rotor | Dro | 135.2 mm |
| Slot width | bs1 | 6 mm |
| Slot active height | hs | 21.7 mm |
| Teeth width | bts | 6.2 mm |
| Yoke width | hss | 19.8 mm |
| Stator inner diameter | Dsi | 136 mm |
| Stator outer diameter | Dso | 219 mm |
| Number of conductors per slot | nw | 6 |
| Conductor height | hc | 3.4 mm |
| Conductor width | wc | 4.5 mm |
| Slot filling factor | kf | 0.7 |
| Configuration | Width (mm) | Height (mm) | Current (Amp) |
|---|---|---|---|
| Two-layer | 3.4 | 10.2 | 288.6 |
| Four-layer | 3.4 | 5.1 | 144.3 |
| Six-layer | 3.4 | 4.5 | 96.2 |
| Eight-layer | 3.4 | 2.55 | 72.15 |
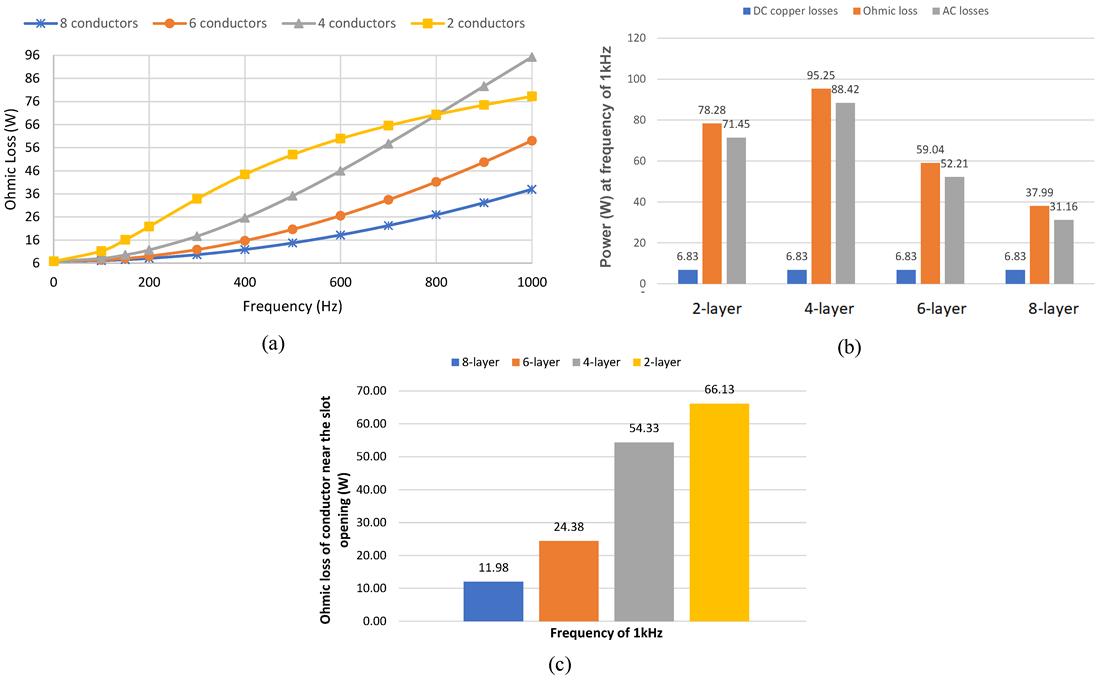
| Frequency (Hz) | Ohmic Losses (W) | |||
|---|---|---|---|---|
| 2-Layer | 4-Layer | 6-Layer | 8-Layer | |
| 1 | 6.83 | 6.83 | 6.83 | 6.83 |
| 100 | 11.29 | 8.08 | 7.40 | 7.15 |
| 200 | 21.89 | 11.76 | 9.09 | 8.11 |
| 300 | 33.85 | 17.69 | 11.88 | 9.70 |
| 400 | 44.44 | 25.60 | 15.76 | 11.93 |
| 500 | 53.04 | 35.16 | 20.68 | 14.77 |
| 600 | 59.93 | 46.00 | 26.59 | 18.23 |
| 700 | 65.58 | 57.74 | 33.45 | 22.30 |
| 800 | 70.35 | 70.06 | 41.19 | 26.96 |
| 900 | 74.52 | 82.65 | 49.74 | 32.19 |
| 1000 | 78.28 | 95.25 | 59.04 | 37.99 |
| Frequency (Hz) | Ohmic Losses (W) | |||
|---|---|---|---|---|
| 2-Layer | 4-Layer | 6-Layer | 8-Layer | |
| 1 | 1.00 | 1.00 | 1.00 | 1.00 |
| 100 | 1.65 | 1.18 | 1.08 | 1.05 |
| 200 | 3.20 | 1.72 | 1.33 | 1.19 |
| 300 | 4.95 | 2.59 | 1.74 | 1.42 |
| 400 | 6.50 | 3.75 | 2.31 | 1.75 |
| 500 | 7.76 | 5.14 | 3.03 | 2.16 |
| 600 | 8.77 | 6.73 | 3.89 | 2.67 |
| 700 | 9.59 | 8.45 | 4.89 | 3.26 |
| 800 | 10.29 | 10.25 | 6.03 | 3.94 |
| 900 | 10.90 | 12.09 | 7.28 | 4.71 |
| 1000 | 11.45 | 13.94 | 8.64 | 5.56 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shams Ghahfarokhi, P.; Podgornovs, A.; Cardoso, A.J.M.; Kallaste, A.; Belahcen, A.; Vaimann, T. Hairpin Windings for Electric Vehicle Motors: Modeling and Investigation of AC Loss-Mitigating Approaches. Machines 2022, 10, 1029. https://doi.org/10.3390/machines10111029
Shams Ghahfarokhi P, Podgornovs A, Cardoso AJM, Kallaste A, Belahcen A, Vaimann T. Hairpin Windings for Electric Vehicle Motors: Modeling and Investigation of AC Loss-Mitigating Approaches. Machines. 2022; 10(11):1029. https://doi.org/10.3390/machines10111029
Chicago/Turabian StyleShams Ghahfarokhi, Payam, Andrejs Podgornovs, Antonio J. Marques Cardoso, Ants Kallaste, Anouar Belahcen, and Toomas Vaimann. 2022. "Hairpin Windings for Electric Vehicle Motors: Modeling and Investigation of AC Loss-Mitigating Approaches" Machines 10, no. 11: 1029. https://doi.org/10.3390/machines10111029
APA StyleShams Ghahfarokhi, P., Podgornovs, A., Cardoso, A. J. M., Kallaste, A., Belahcen, A., & Vaimann, T. (2022). Hairpin Windings for Electric Vehicle Motors: Modeling and Investigation of AC Loss-Mitigating Approaches. Machines, 10(11), 1029. https://doi.org/10.3390/machines10111029











