Bionic Hovering Micro-Aerial Vehicle Using Array-Spiracle Wings
Abstract
1. Introduction
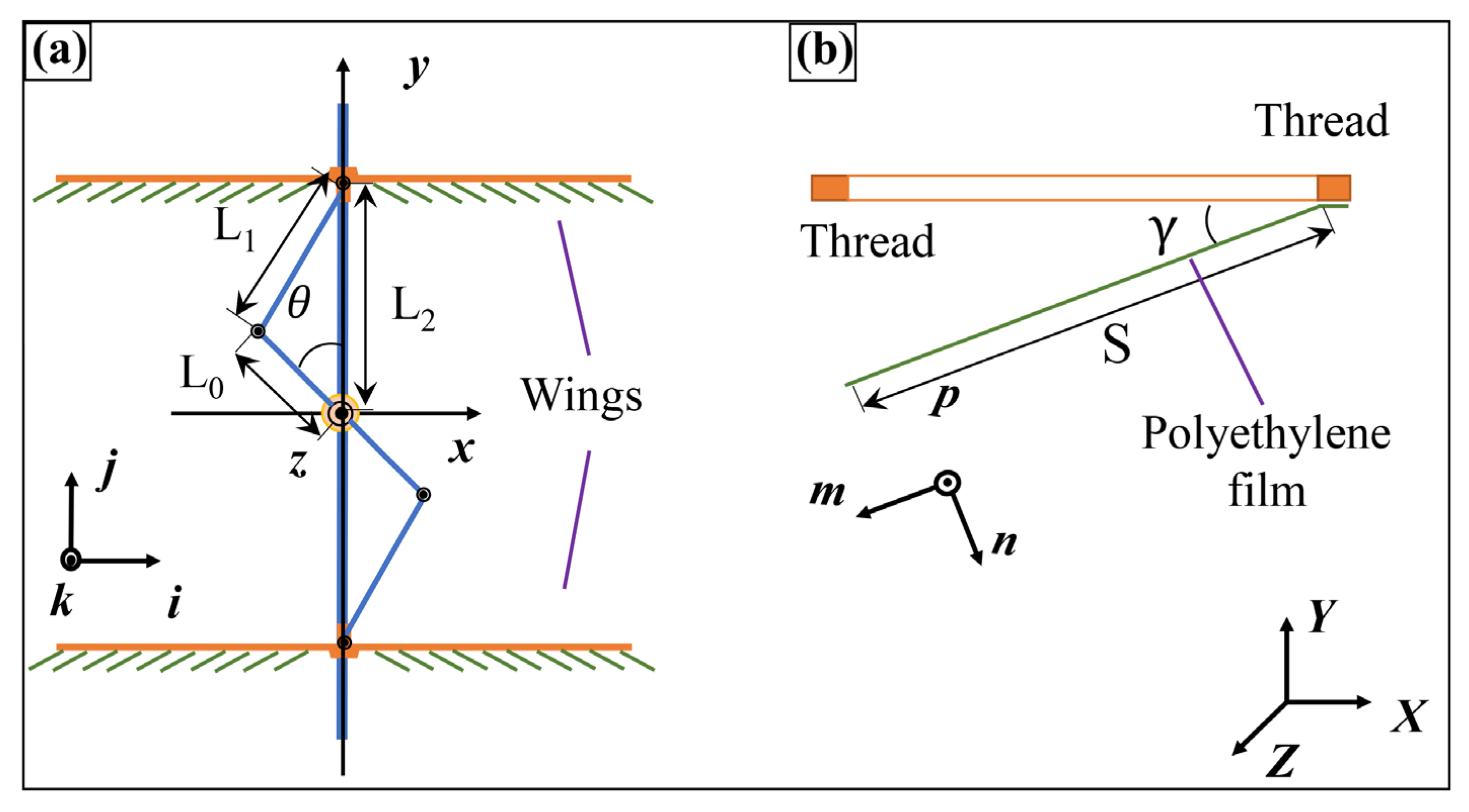
2. Micro-Aerial Vehicle Design and Kinematics
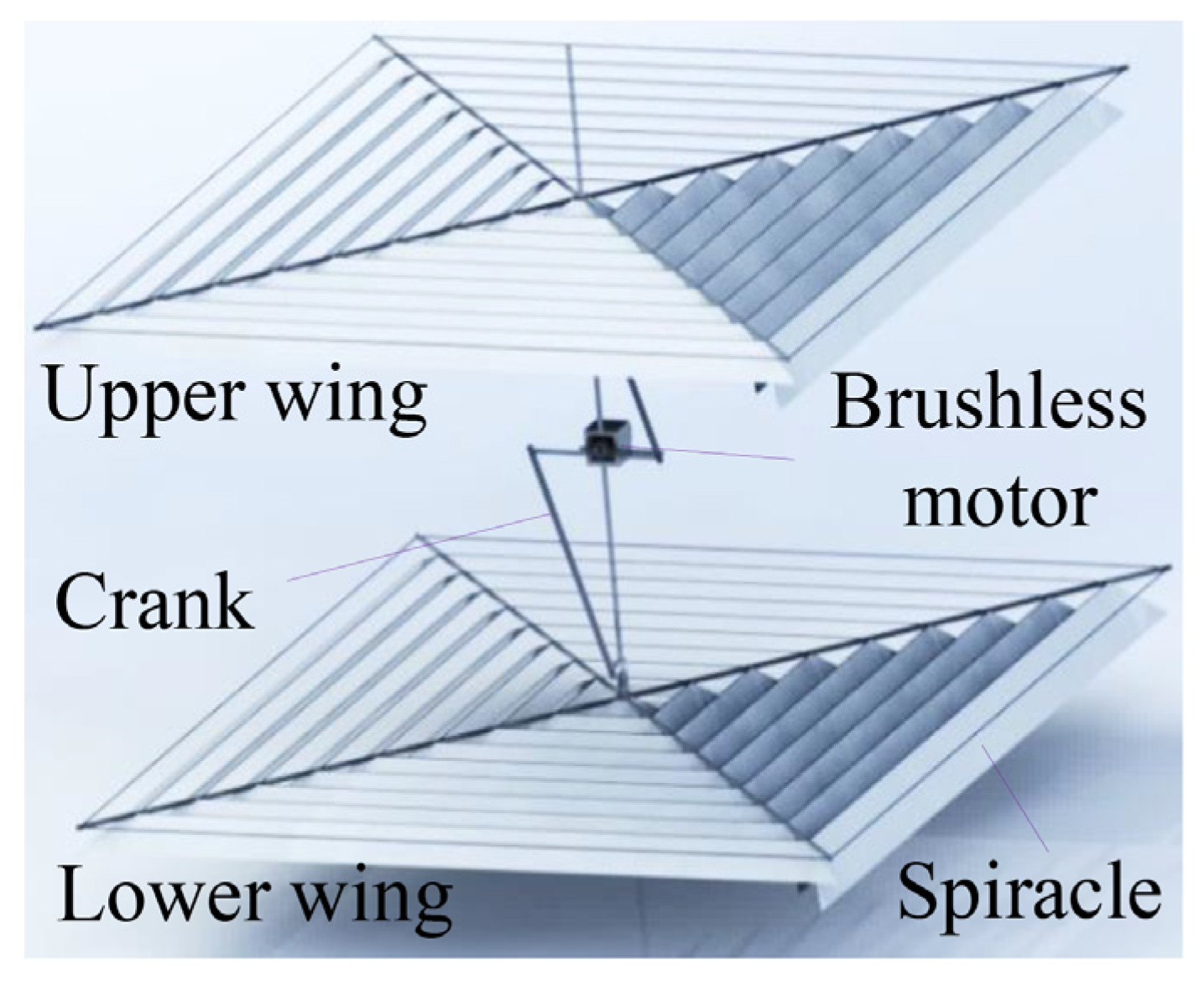
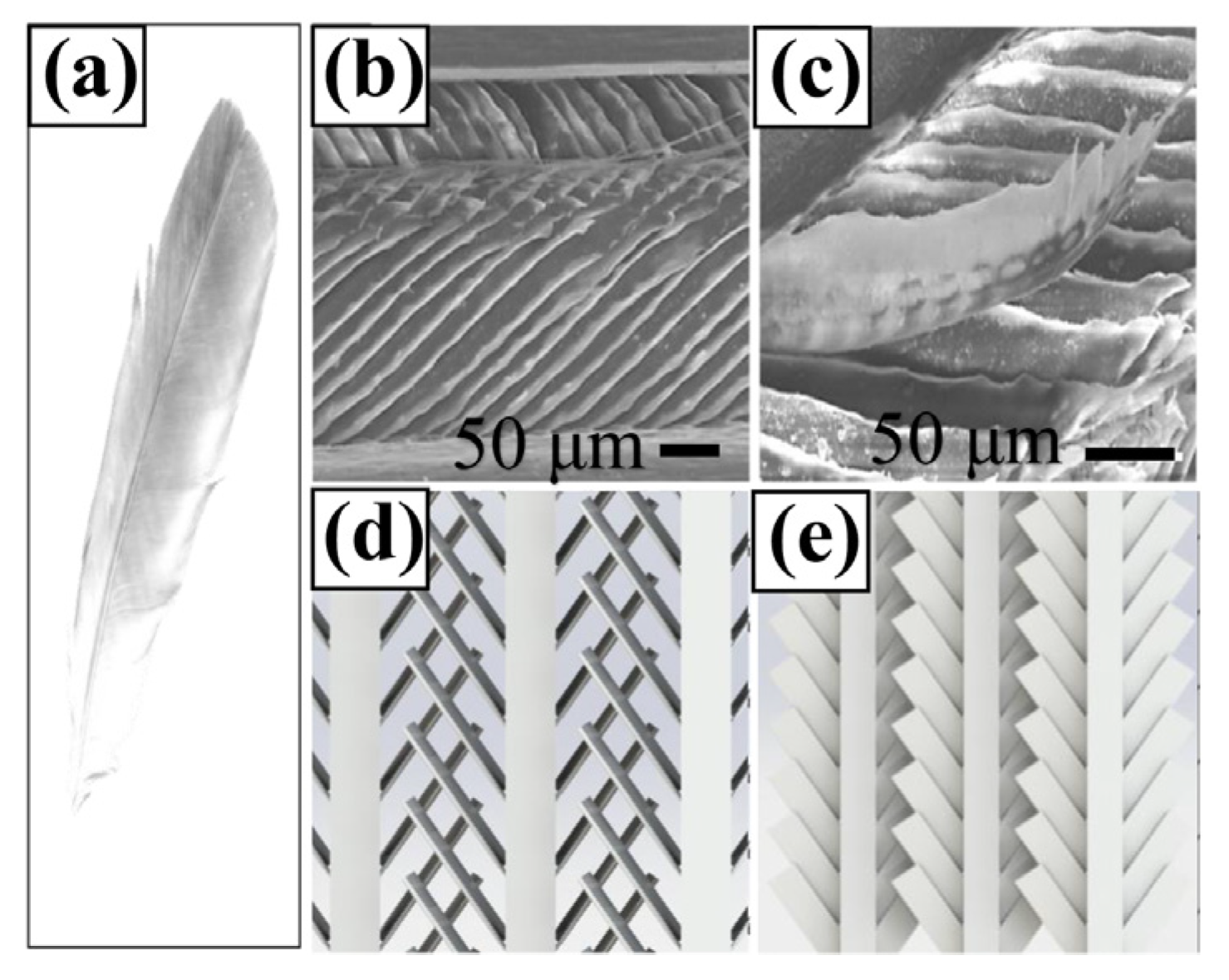
2.1. Bird Feather-like Array of Spiracle Wings Design
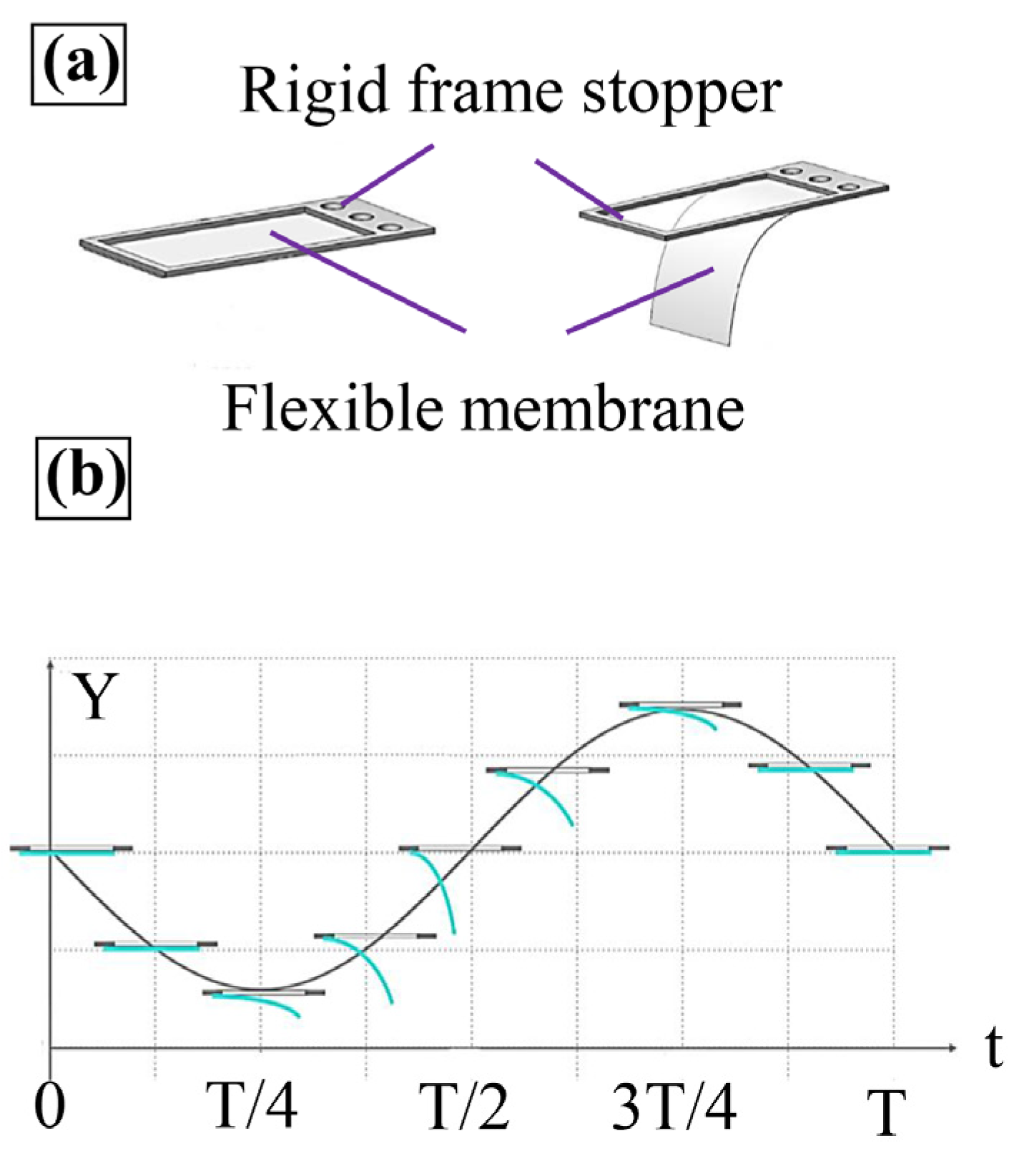
2.2. Wing Design
2.3. Wing Motion Design
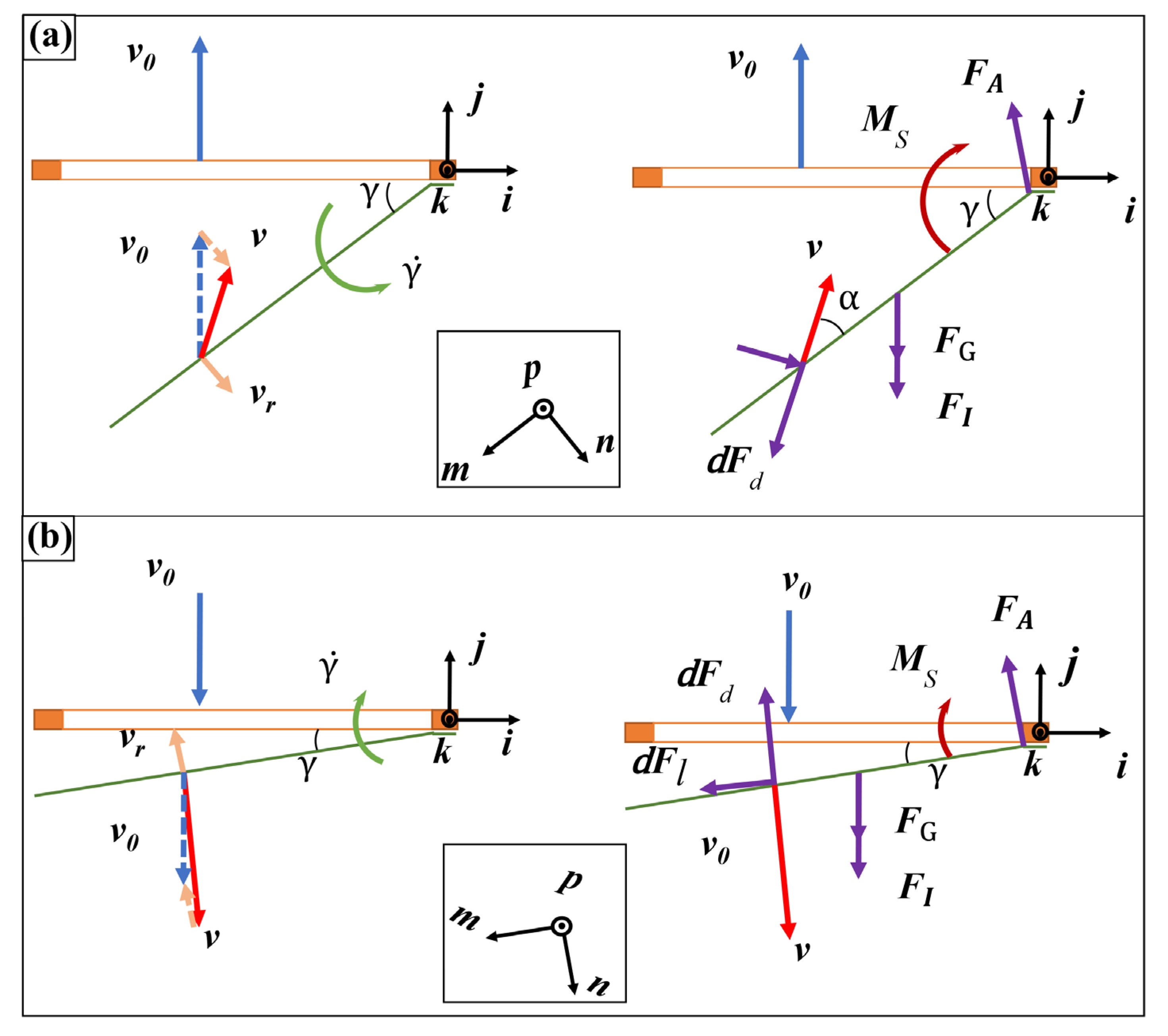
3. Kinematic and Aerodynamic Model of the Spiracle Unit
4. Experimental Results
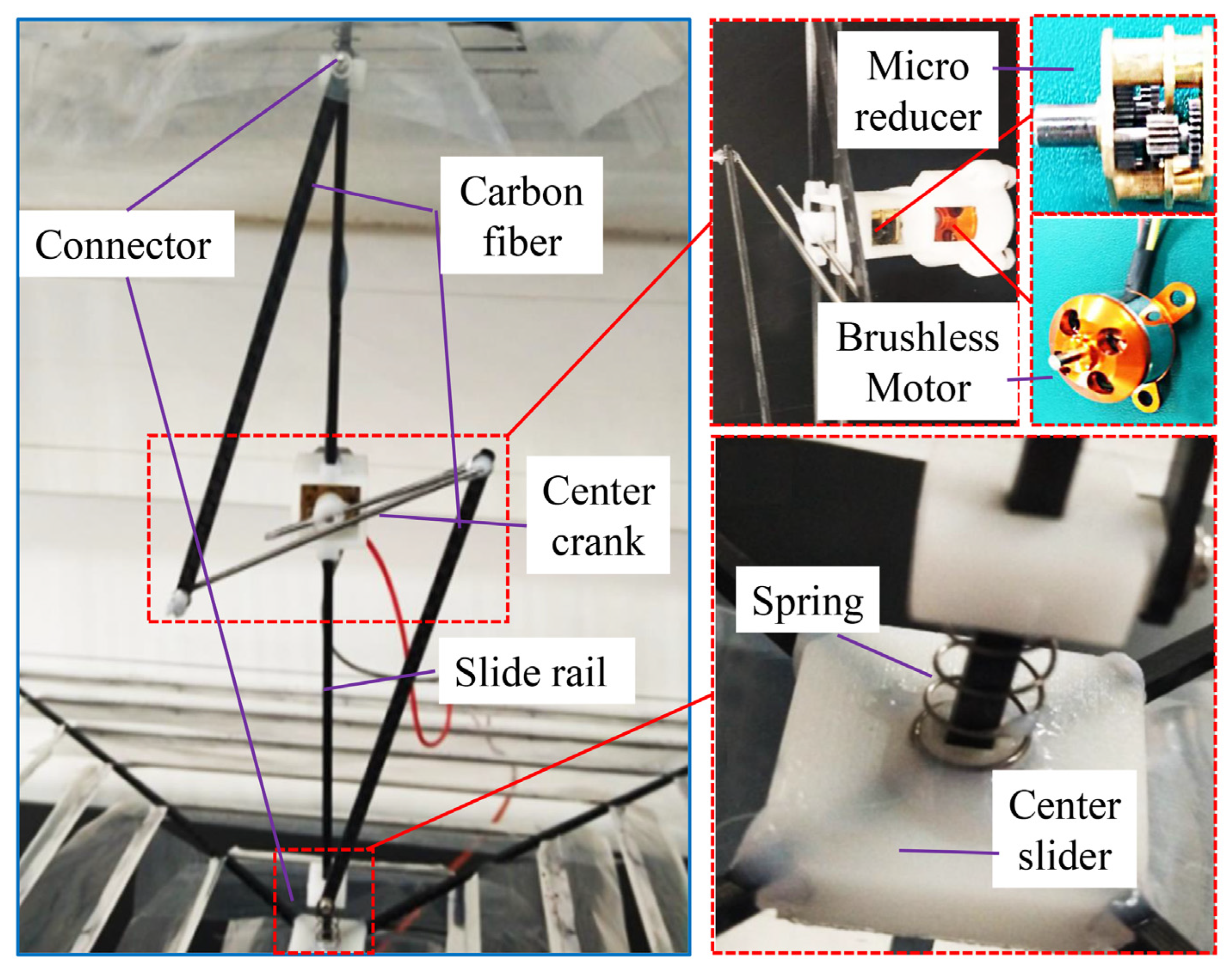
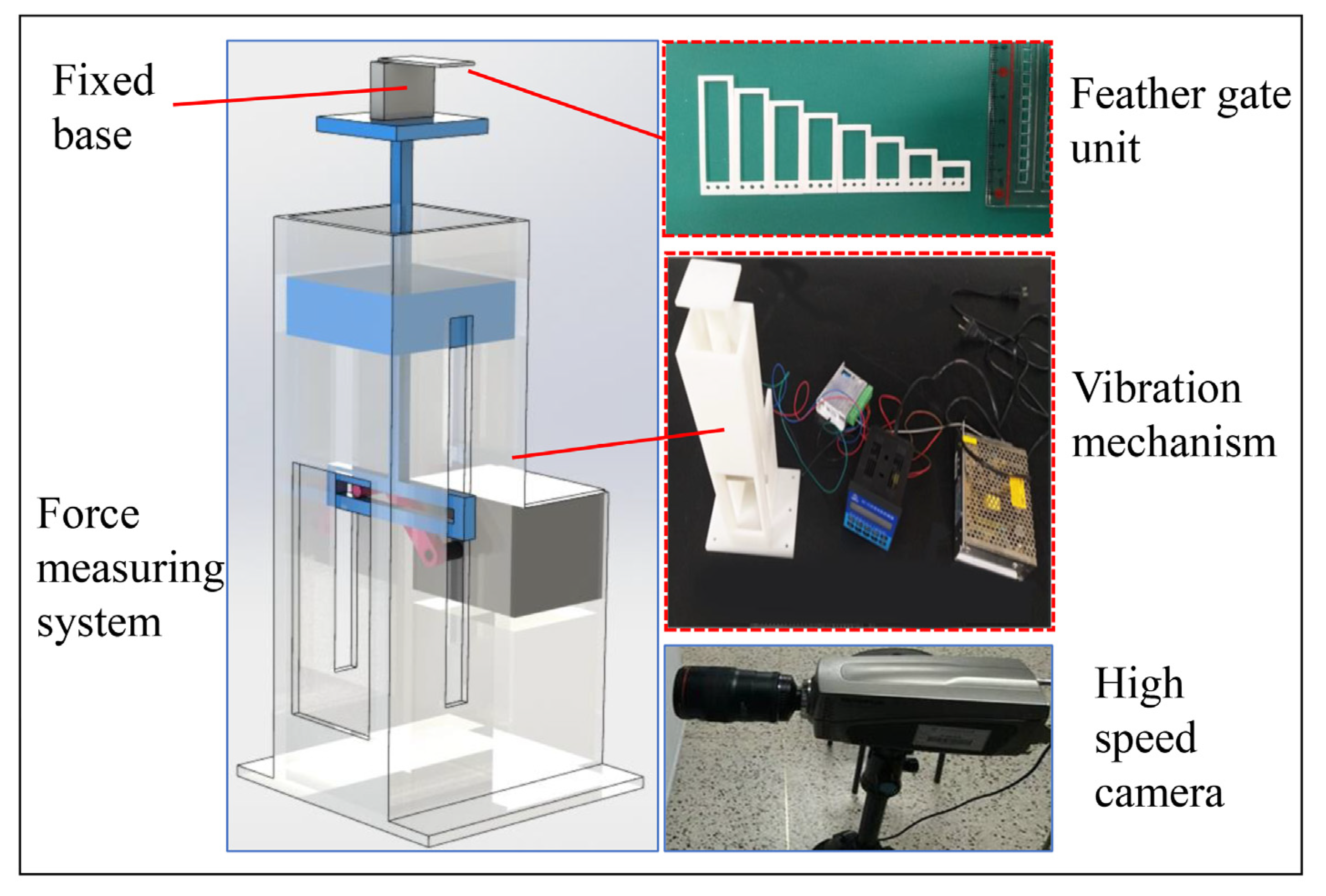
4.1. Construction of the Test System
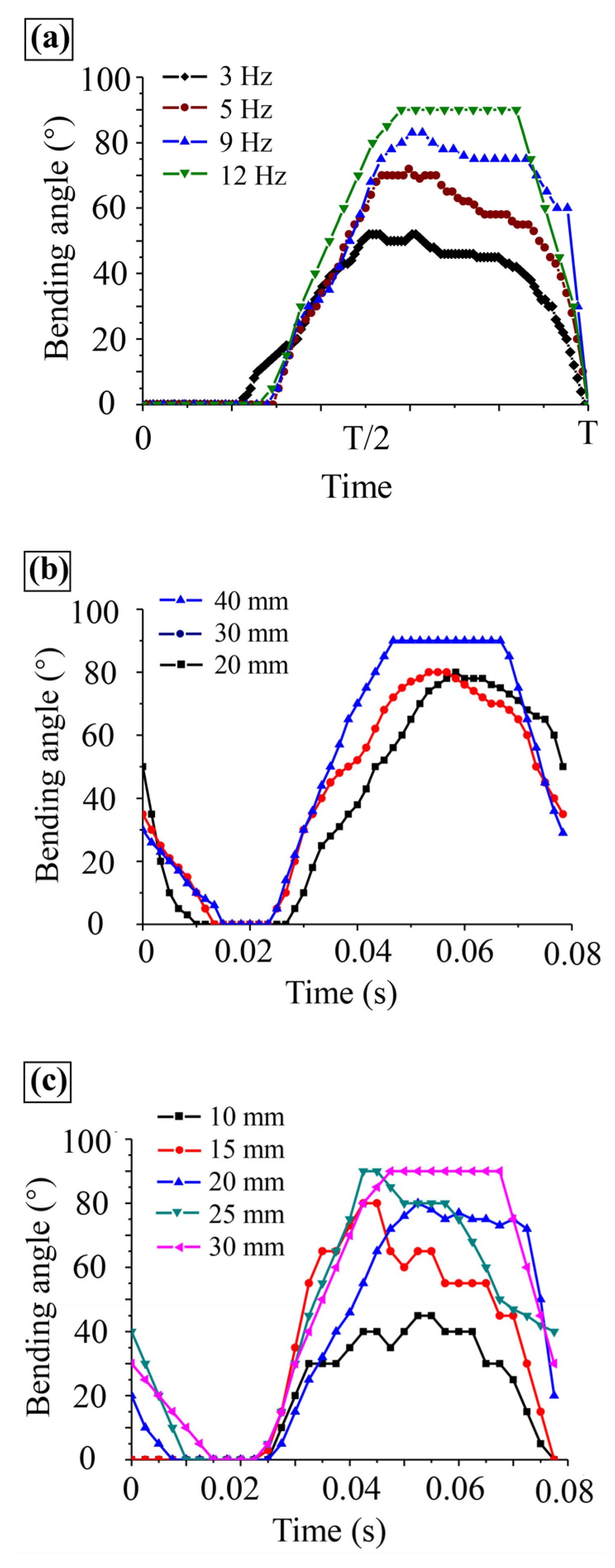
4.2. Numerical Calculation and Verification of the Aerodynamic Theoretical Model
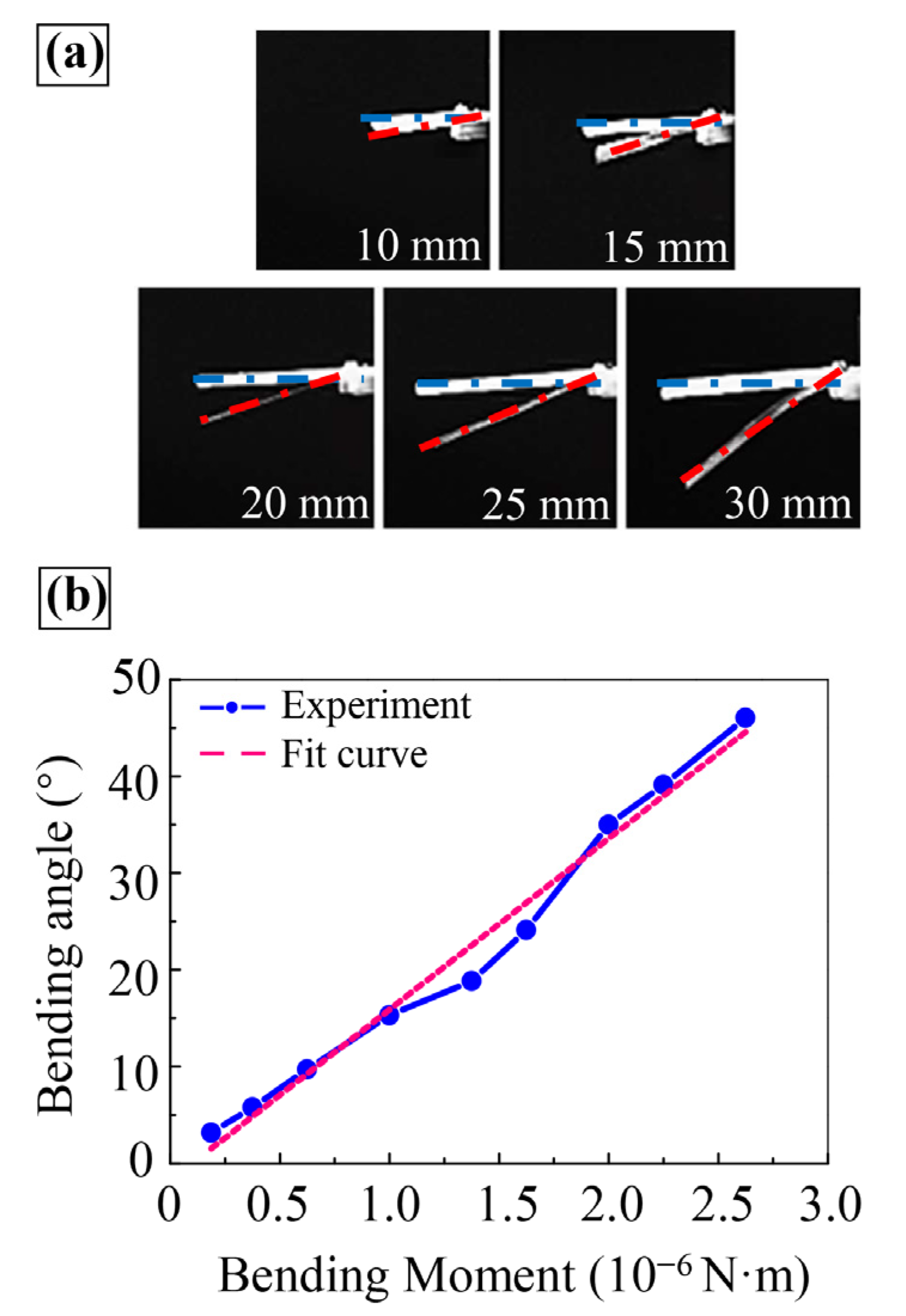
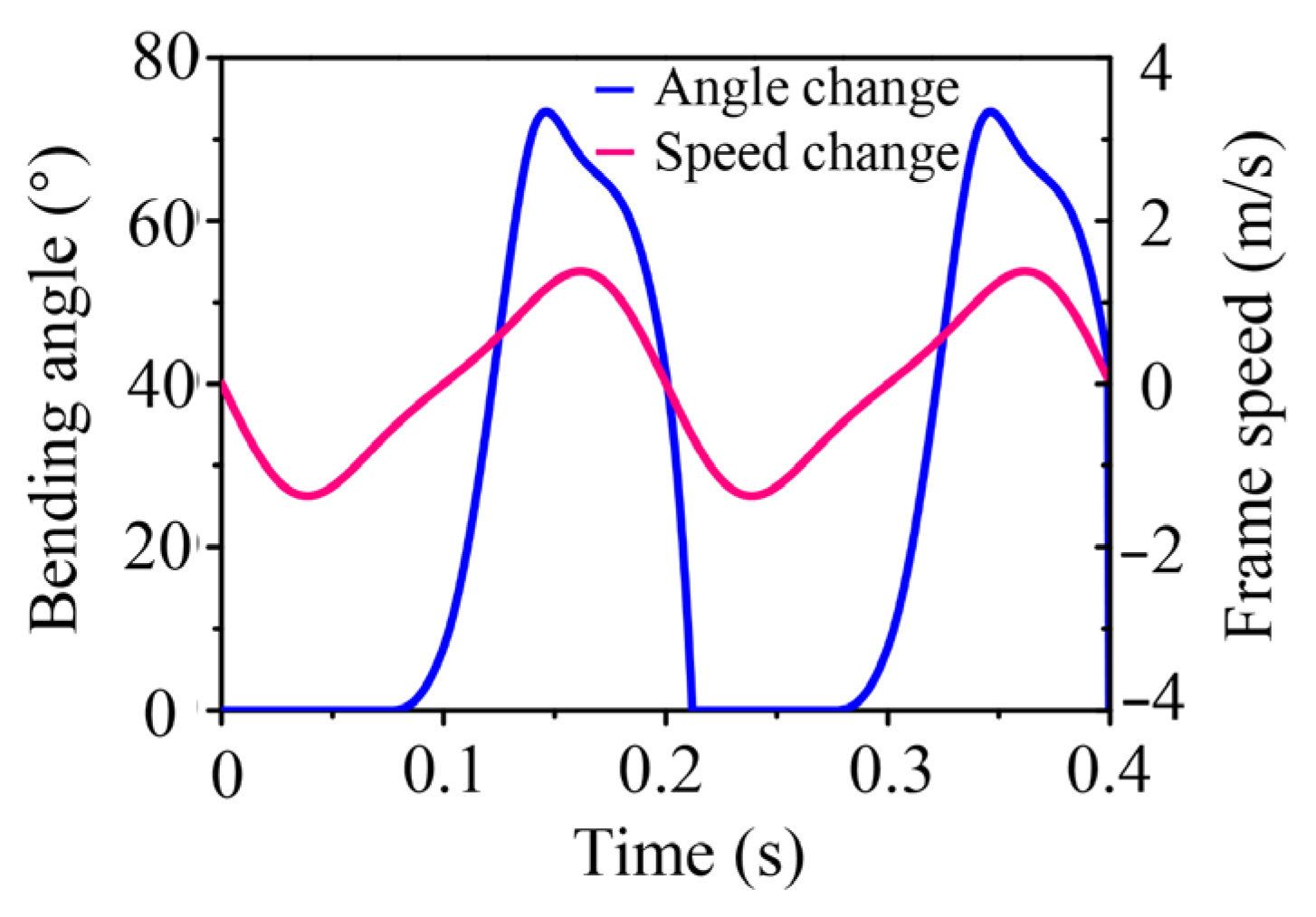
4.3. Experimental Analysis of Spiracle Opening and Closing Characteristics
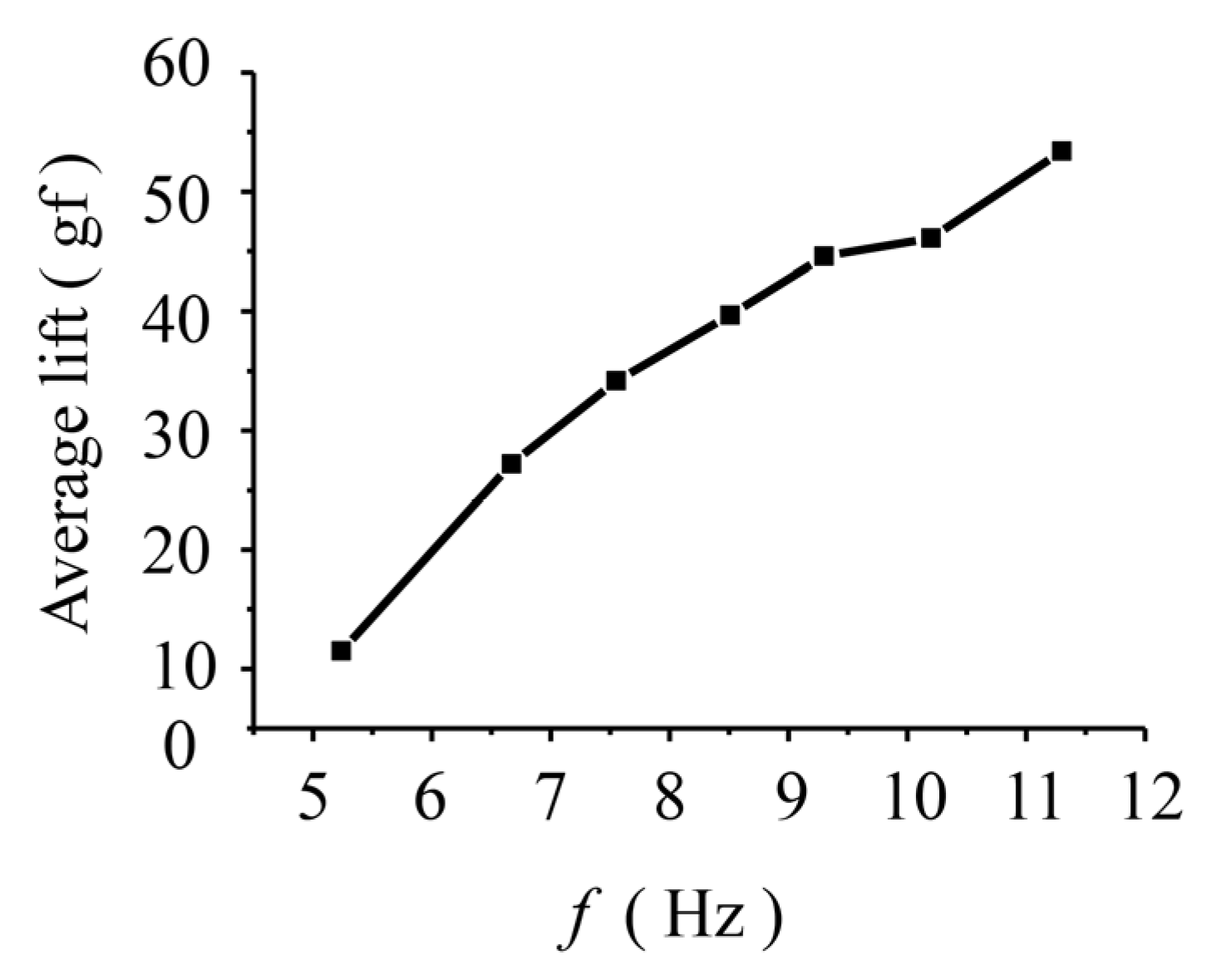
4.4. Prototype Manufacturing and Flight Experiments
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Lehmann, F.-O.; Pick, S. The aerodynamic benefit of wing–wing interaction depends on stroke trajectory in flapping insect wings. J. Exp. Biol. 2007, 210, 1362–1377. [Google Scholar] [CrossRef] [PubMed]
- Sane, S.P. Steady or Unsteady? Uncovering the Aerodynamic Mechanisms of Insect Flight. J. Exp. Biol. 2011, 214, 349–351. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Ellington, C.P. The novel aerodynamics of insect flight: Applications to micro-air vehicles. J. Exp. Biol. 1999, 202, 3439–3448. [Google Scholar] [CrossRef] [PubMed]
- Zhu, Y.; He, X.; Zhang, P.; Guo, G.; Zhang, X. Perching and Grasping Mechanism Inspired by a Bird’s Claw. Machines 2022, 10, 656. [Google Scholar] [CrossRef]
- Deng, H.; Xiao, S.; Huang, B.; Yang, L.; Xiang, X.; Ding, X. Design optimization and experimental study of a novel mechanism for a hover-able bionic flapping-wing micro air vehicle. Bioinspir. Biomim. 2020, 16, 026005. [Google Scholar] [CrossRef]
- Platzer, M.F.; Jones, K.D.; Young, J.; Lai, J.C.S. Flapping Wing Aerodynamics: Progress and Challenges. AIAA J. 2008, 46, 2136–2149. [Google Scholar] [CrossRef]
- Mackenzie, D. A Flapping of Wings. Science 2012, 335, 1430–1433. [Google Scholar] [CrossRef] [PubMed]
- Nguyen, Q.V.; Truong, Q.T.; Park, H.C.; Goo, N.S.; Byun, D. Measurement of Force Produced by an Insect-Mimicking Flapping-Wing System. J. Bionic Eng. 2010, 7, S94–S102. [Google Scholar] [CrossRef]
- Keennon, M.; Klingebiel, K.; Won, H. Development of the Nano Hummingbird: A Tailless Flapping Wing Micro Air Vehicle. In Proceedings of the 50th AIAA Aerospace Sciences Meeting including the New Horizons Forum and Aerospace Exposition, Nashville, Tennessee, 9–12 January 2012; American Institute of Aeronautics and Astronautics: Reston, VA, USA, 2012. [Google Scholar]
- Shyy, W.; Aono, H.; Kang, C.K.; Liu, H. An Introduction to Flapping Wing Aerodynamics: Rigid Fixed-Wing Aerodynamics; Cambridge University Press: Cambridge, UK, 2013; pp. 42–89. [Google Scholar]
- Steltz, E.; Avadhanula, S.; Fearing, R.S. High lift force with 275 Hz wing beat in MFI. In Proceedings of the 2007 IEEE/RSJ International Conference on Intelligent Robots and Systems, San Diego, CA, USA, 29 October–2 November 2007; pp. 3987–3992. [Google Scholar]
- Jafferis, N.T.; Helbling, E.F.; Karpelson, M.; Wood, R.J. Untethered flight of an insect-sized flapping-wing microscale aerial vehicle. Nature 2019, 570, 491–495. [Google Scholar] [CrossRef]
- Coleman, D.A.; Benedict, M.; Amp, T.A.; Hrishikeshavan, V.; Chopra, I. Design, Development and Flight-Testing of a Robotic Hummingbird; Vertical Flight Society: Fairfax, VA, USA, 2015. [Google Scholar]
- Nguyen, Q.V.; Park, H.C.; Goo, N.S.; Byun, D. Characteristics of a Beetle’s Free Flight and a Flapping-Wing System that Mimics Beetle Flight. J. Bionic Eng. 2010, 7, 77–86. [Google Scholar] [CrossRef]
- Quoc-Viet, N.; Woei Leong, C.; Marco, D. An Insect-Inspired Flapping Wing Micro Air Vehicle with Double Wing Clap-Fling Effects and Capability of Sustained Hovering; Proc. SPIE: Bellingham, WA USA, 2015; p. 94290U. [Google Scholar]
- Nguyen, Q.-V.; Chan, W.L.; Debiasi, M. Hybrid Design and Performance Tests of a Hovering Insect-inspired Flapping-wing Micro Aerial Vehicle. J. Bionic Eng. 2016, 13, 235–248. [Google Scholar] [CrossRef]
- Zhou, X.; Zhang, D.; Huang, Z.; Song, X.; Liu, H.; Feng, L. Liftoff of a New Hovering Oscillating-wing Micro Aerial Vehicle. J. Bionic Eng. 2021, 18, 649–661. [Google Scholar] [CrossRef]
- de Croon, G.C.H.E.; Groen, M.A.; De Wagter, C.; Remes, B.; Ruijsink, R.; van Oudheusden, B.W. Design, aerodynamics and autonomy of the DelFly. Bioinspir. Biomim. 2012, 7, 025003. [Google Scholar] [CrossRef] [PubMed]
- Karásek, M.; Muijres, F.T.; De Wagter, C.; Remes, B.D.W.; de Croon, G.C.H.E. A tailless aerial robotic flapper reveals that flies use torque coupling in rapid banked turns. Science 2018, 361, 1089–1094. [Google Scholar] [CrossRef] [PubMed]
- Ristroph, L.; Childress, S. Stable hovering of a jellyfish-like flying machine. J. R. Soc. Interface 2014, 11, 20130992. [Google Scholar] [CrossRef]
- Fang, F.; Ho, K.L.; Ristroph, L.; Shelley, M.J. A computational model of the flight dynamics and aerodynamics of a jellyfish-like flying machine. J. Fluid Mech. 2017, 819, 621–655. [Google Scholar] [CrossRef]
- Guo, S.; Li, H.; Zhou, C.; Zhang, Y.L.; He, Y.; Wu, J.H. Analysis and experiment of a bio-inspired flyable micro flapping wing rotor. Aerosp. Sci. Technol. 2018, 79, 506–517. [Google Scholar] [CrossRef]
- Tanaka, K.; Suzuki, R.; Emaru, T.; Higashi, Y.; Wang, H.O. Development of a Cyclogyro-Based Flying Robot With Variable Attack Angle Mechanisms. IEEE/ASME Trans. Mechatron. 2007, 12, 565–570. [Google Scholar] [CrossRef]
- Hara, N.; Tanaka, K.; Ohtake, H.; Wang, H.O. Development of a Flying Robot with Pantograph-based Variable Wing Mechanism. In Proceedings of the 2007 IEEE International Conference on Robotics and Automation, Roma, Italy, 10–14 April; 2007; pp. 349–354. [Google Scholar]
- Chang, E.; Matloff, L.Y.; Stowers, A.K.; Lentink, D. Soft biohybrid morphing wings with feathers underactuated by wrist and finger motion. Sci. Robot. 2020, 5, eaay1246. [Google Scholar] [CrossRef]
- Matloff, L.Y.; Chang, E.; Feo, T.J.; Jeffries, L.; Stowers, A.K.; Thomson, C.; Lentink, D. How flight feathers stick together to form a continuous morphing wing. Science 2020, 367, 293–297. [Google Scholar] [CrossRef]
- Sullivan, T.N.; Chon, M.; Ramachandramoorthy, R.; Roenbeck, M.R.; Hung, T.-T.; Espinosa, H.D.; Meyers, M.A. Reversible Attachment with Tailored Permeability: The Feather Vane and Bioinspired Designs. Adv. Funct. Mater. 2017, 27, 1702954. [Google Scholar] [CrossRef]
- Müller, W.; Patone, G. Air transmissivity of feathers. J. Exp. Biol. 1998, 201, 2591–2599. [Google Scholar] [CrossRef] [PubMed]
- Behbahani, S.B.; Tan, X. Bio-inspired flexible joints with passive feathering for robotic fish pectoral fins. Bioinspir. Biomim. 2016, 11, 036009. [Google Scholar] [CrossRef] [PubMed]
- Wang, Z.J.; Birch, J.M.; Dickinson, M.H. Unsteady forces and flows in low Reynolds number hovering flight: Two-dimensional computations vs robotic wing experiments. J. Exp. Biol. 2004, 207, 449–460. [Google Scholar] [CrossRef] [PubMed]





| Condition Parameter | Representative Symbol | Substitute Value |
|---|---|---|
| Density of air | 1.293 kg/m3 | |
| Wing vibration cycle | T | 0.2 s |
| Length of rotating rod | 40 mm | |
| Swing bar length | 90 mm | |
| Film length | L | 30 mm |
| Film width | b | 10 mm |
| Film thickness | h | 1.25 × 10−5 m |
| Film Density | 1720 kg/m3 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhou, X.; Song, X.; Zhang, D.; Liu, Y. Bionic Hovering Micro-Aerial Vehicle Using Array-Spiracle Wings. Machines 2022, 10, 1016. https://doi.org/10.3390/machines10111016
Zhou X, Song X, Zhang D, Liu Y. Bionic Hovering Micro-Aerial Vehicle Using Array-Spiracle Wings. Machines. 2022; 10(11):1016. https://doi.org/10.3390/machines10111016
Chicago/Turabian StyleZhou, Xiangcong, Xiaogang Song, Deyuan Zhang, and Yanqiang Liu. 2022. "Bionic Hovering Micro-Aerial Vehicle Using Array-Spiracle Wings" Machines 10, no. 11: 1016. https://doi.org/10.3390/machines10111016
APA StyleZhou, X., Song, X., Zhang, D., & Liu, Y. (2022). Bionic Hovering Micro-Aerial Vehicle Using Array-Spiracle Wings. Machines, 10(11), 1016. https://doi.org/10.3390/machines10111016






