Research on a Measurement Method for Middle-Infrared Radiation Characteristics of Aircraft
Abstract
:1. Introduction
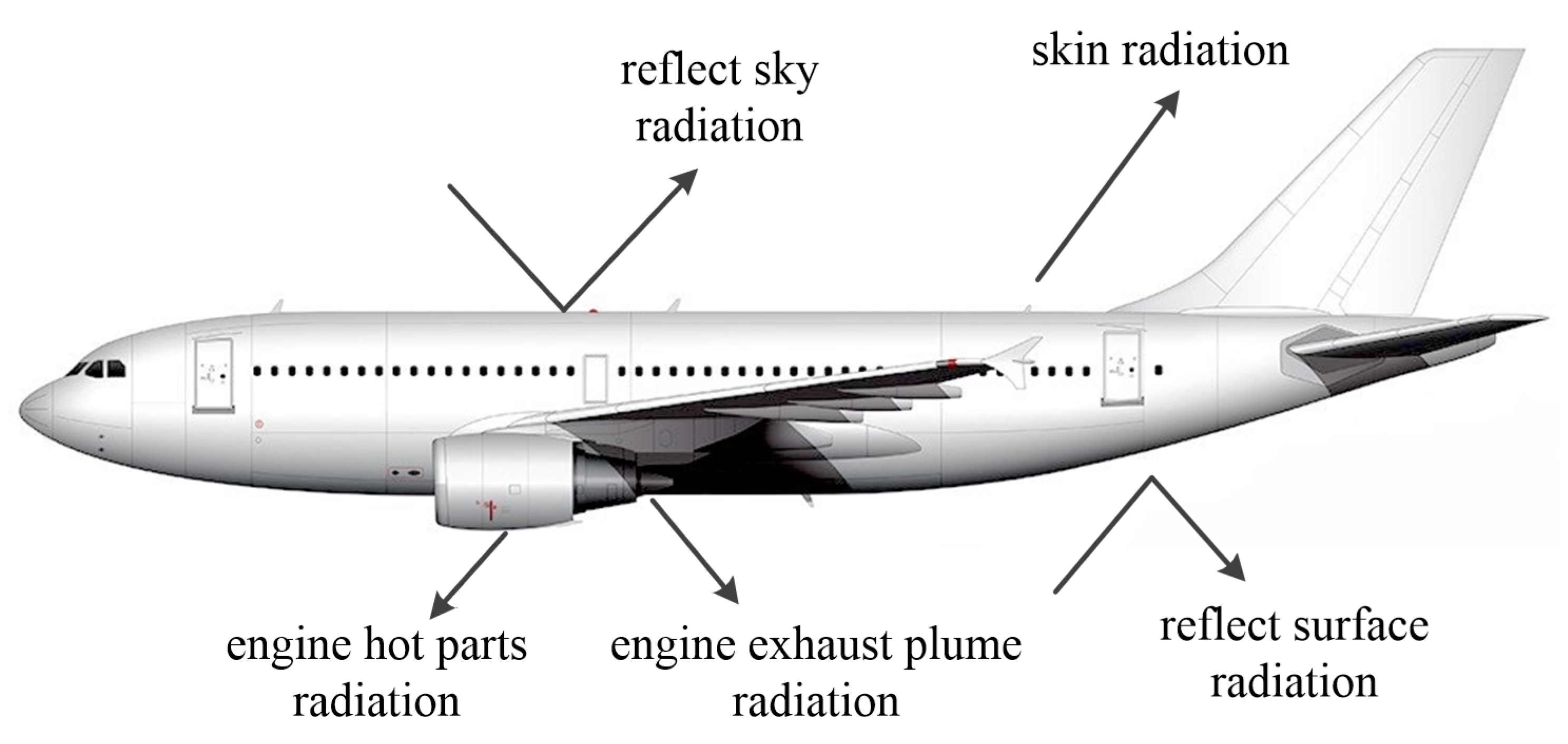
2. Infrared Radiation Characteristics of Aircraft
2.1. Aircraft Skin Infrared Radiation
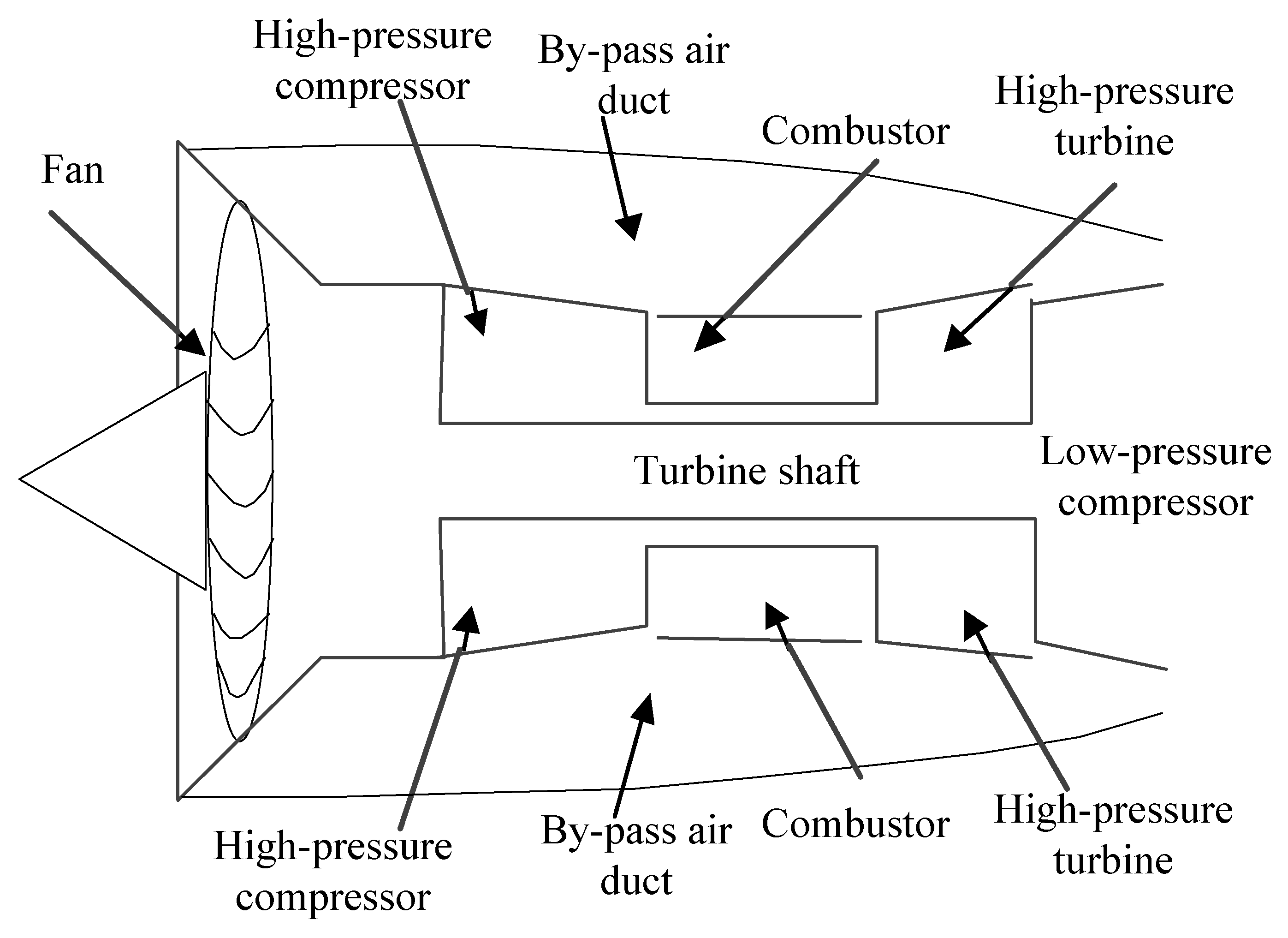
2.2. Engine Hot Parts’ Infrared Radiation
2.3. Reflected Solar Radiation
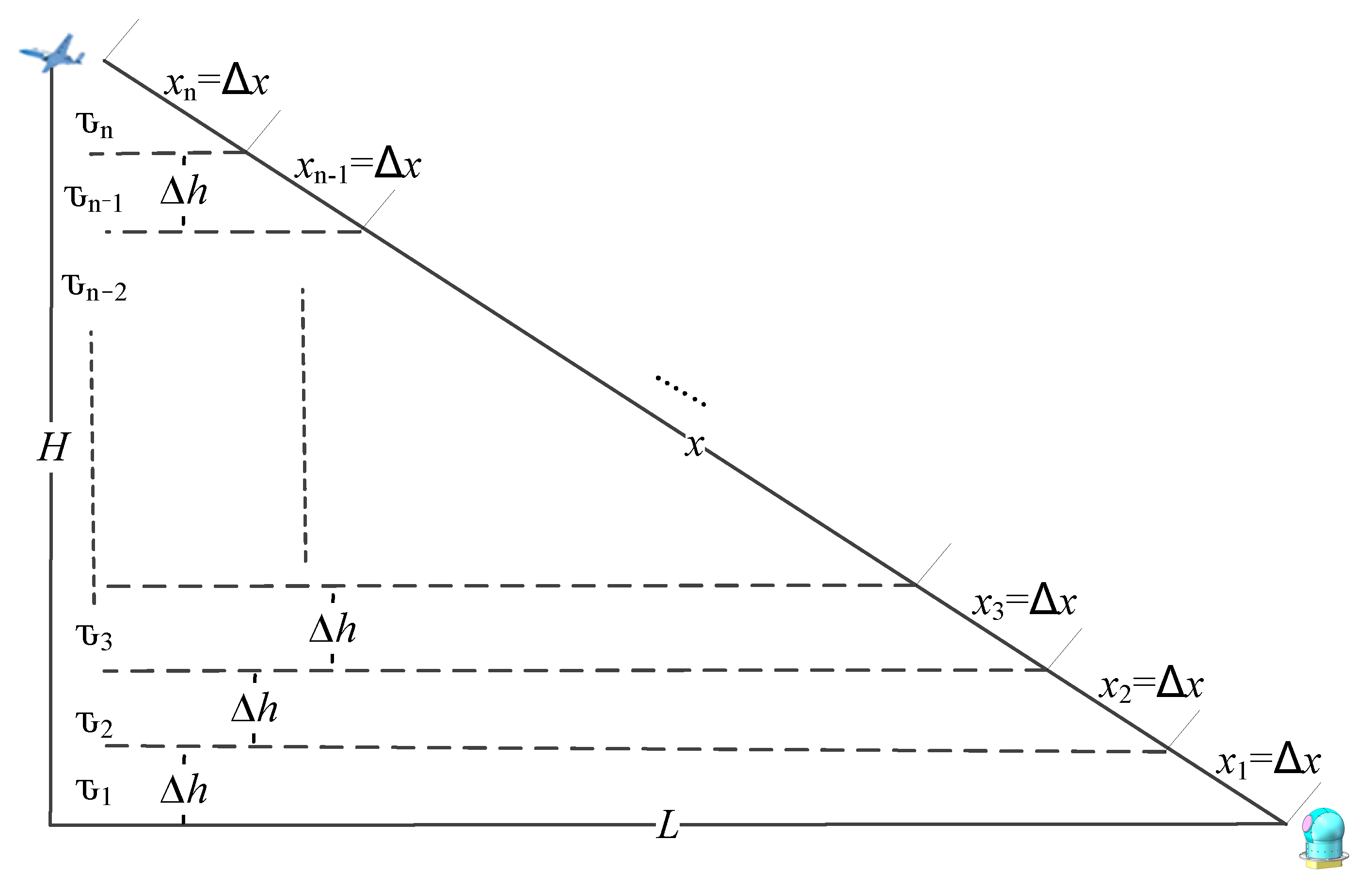
3. Atmospheric Transmission Model
3.1. Absorption of H2O Molecules
3.2. Absorption of CO2 Molecules
3.3. Scattering of Aerosols and Particles
4. Theoretical Model of Temperature Measurement
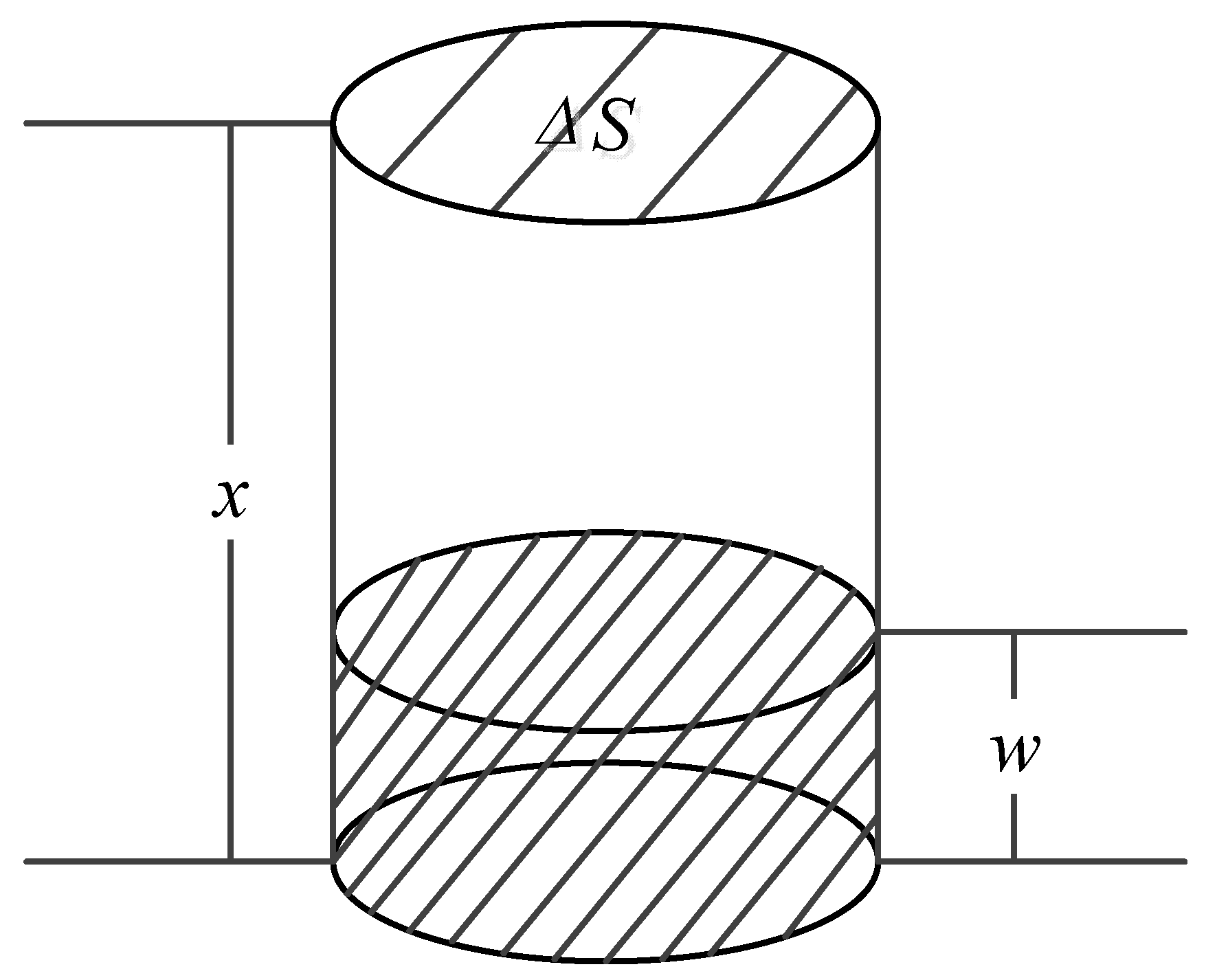
4.1. Background Radiation
4.2. Absolute Radiometric Calibration Model
4.3. Temperature Inversion Model
5. Radiation Characteristic Measurement and Analysis
5.1. Experimental Conditions
5.2. Temperature Inversion
- We first calculated the atmospheric transmittance of each band and substituted it into the objective function ;
- Second, we calculated the entrance pupil radiance of the target signal by the absolute radiometric calibration model;
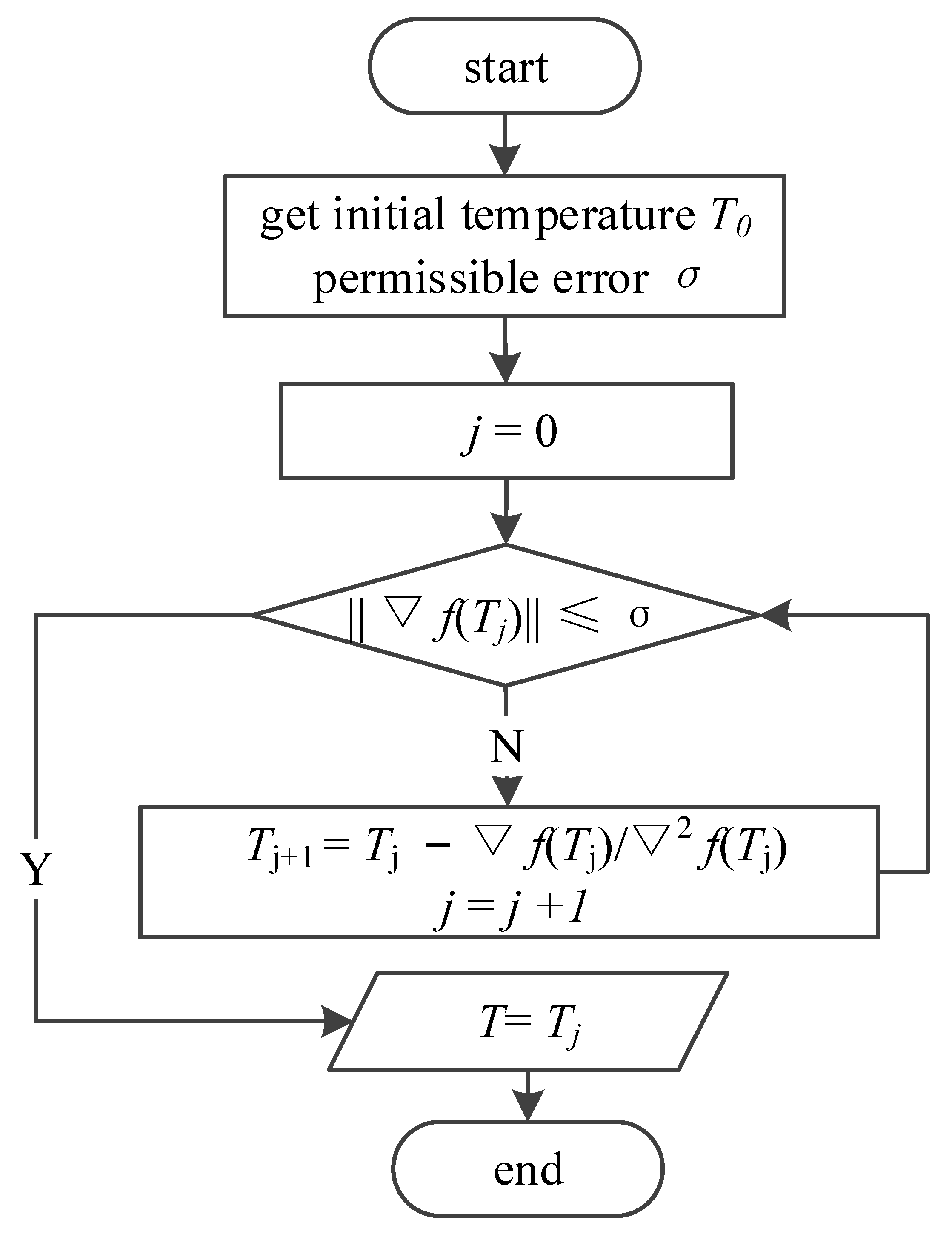
- Third, the quasi-Newton method was used to find the optimal solution of the objective function .
5.3. Measurement Uncertainty Analysis
6. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Kou, T.; Zhou, Z.; Liu, H.; Yang, Z. Correlation between infrared radiation characteristic signals and target maneuvering modes. Acta Opt. Sin. 2018, 38, 37–45. [Google Scholar]
- Du, S.; Yang, J.; Zeng, K.; Gao, Y.; Chen, S. Discussion on the infrared stealthy technique of aircraft. Electron. Warf. 2010, 133, 41–45. [Google Scholar]
- Wang, C. Detecting and tracking technology about IR stealth target. Infrared Laser Eng. 2006, 35 (Suppl. S1), 127–132. [Google Scholar]
- Li, W.; Yan, S.; Hu, L.; Wu, Y.; Wang, C.; Ouyang, Y. A review of false alarm suppression technology for infrared early warning satellite. Infrared Technol. 2020, 42, 115–120. [Google Scholar]
- Mao, X.; Hu, H.; Huang, K.; Liang, X. Calculation method for airplane IR radiation and atmospheric transmittance. J. Beijing Univ. Aeronaut. Astronaut. 2009, 35, 1228. [Google Scholar]
- Kang, L.Z.; Zhao, J.S.; Li, Z.H. The overview of the research work developments on infrared signature of aircraft. Infrared Technol. 2017, 39, 105–115. [Google Scholar]
- Zong, J.; Zhang, J.; Liu, D. Infrared Radiation Characteristics of the Stealth Aircraft. Acta Photonica Sin. 2011, 40, 289–294. [Google Scholar] [CrossRef]
- Liu, Y.; Liu, X. Research on technology of ground-based infrared radiation feature measurement for space target. Acta Opt. Sin. 2014, 34, 123–129. [Google Scholar]
- Wang, X.; Gao, S.; Jin, L.; Li, Z.; Li, F. Multi-band Infrared Radiation Characterization and Simulation Analysis for Aerial Target. Acta Photonica Sin. 2020, 49, 511002. [Google Scholar] [CrossRef]
- Han, Y.; Xuan, Y. Effect of atmospheric transmission on IR radiation of feature target and background. J. Appl. Opt. 2002, 4, 8–11. [Google Scholar]
- Liu, J.; Gong, G.; Han, L.; Gao, D. Modeling and simulation of airplane infrared characteristic. Infrared Laser Eng. 2011, 40, 1209–1213. [Google Scholar]
- Yang, C.; Cao, L.; Zhang, J. Measurement of infrared radiation for target airplane based on real-time atmospheric correction. Opt. Precis. Eng. 2014, 22, 1751–1759. [Google Scholar] [CrossRef]
- Yang, C.; Zhang, J.; Cao, L. Infrared radiation measurement based on real-time correction. J. Infrared Millim. Waves 2011, 30, 284–288. [Google Scholar] [CrossRef]
- Sun, Z.; Chang, S.; Zhu, W. Radiometric calibration method for large aperture infrared system with broad dynamic range. Appl. Opt. 2015, 54, 4659–4666. [Google Scholar] [CrossRef]
- Huang, W.; Ji, H. Effect of reflected background radiation by skin on infrared signature of subsonic aircraft (I): Methodology. Infrared Laser Eng. 2015, 44, 1699–1703. [Google Scholar]
- Huang, W.; Ji, H. Effect of reflected background radiation by skin on infrared signature of subsonic aircraft (II): Application. Infrared Laser Eng. 2015, 44, 2039–2043. [Google Scholar]
- Li, J.; Tong, Z.; Wang, C.; Chai, D.; Zhang, Z.B. IR radiation characteristics analysis and vulnerability assessment of aircraft. Laser Infrared 2013, 43, 180–185. [Google Scholar]
- Chen, B.; Fang, Y.; Xu, X. Study on the IR radiation of aeroplane. Aero Weapon. 2005, 4, 30–32. [Google Scholar]
- Wang, C.; Tong, Z.; Lu, Y.; Chai, D. Study on airplane’s infrared radiation characteristics. Laser Infrared 2011, 41, 996–1001. [Google Scholar]
- Chen, G. The development of civil high-bypass turbofans. J. Aerosp. Power 2019, 3, 56–61. [Google Scholar]
- Xia, J.; Yang, Q.; Wang, H.; Wu, Y. Development analysis to after burner combustion technology of turbofan. J. Aerosp. Power 2020, 4, 17–21. [Google Scholar]
- Wang, Y.; Chen, Z.L. Analysis of temperature field in nacelle for a turbofan engine. Eng. Test 2015, 55, 35. [Google Scholar]
- Zhang, Z.; Tong, Z.; Wang, C.; Li, J. Modeling and simulation of airplane infrared characteristic along the sight line. Laser Infrared 2013, 43, 890–895. [Google Scholar]
- Wu, H. Research in to theoretical calculation method on engineering of transmittance of infrared radiation through atmosphere. Opt. Precis. Eng. 1998, 6, 120–125. [Google Scholar]
- Kang, D.; Cheng, B.; Gao, J. Different algorithm method of ground-air infrared detection range. Electro-Opt. Technol. Appl. 2009, 24, 29–32. [Google Scholar]
- Zhang, J.; Fang, X. Infrared Physics; Xidian University Press: Xi’an, China, 2004. [Google Scholar]
- Liu, L.; Dong, S.; Yu, Q.; Tan, H. Atmospheric mean transmittance in wavelength interval 0.1 μm from infrared 1 to 14 μm, (II) transmittance of water vapor. J. Harbin Inst. Technol. 1999, 6, 75–78. [Google Scholar]
- Liu, L.; Dong, S.; Yu, Q.; Tan, H. Atmospheric mean transmittance in wavelength interval 0.1 μm from infrared 1 to 14 μm (II) transmittance of carbon dioxide. J. Harbin Inst. Technol. 1998, 5, 9–13. [Google Scholar]
- Lu, Y. Simple method to calculate the atmosphere transmittance of infrared radiation on slanting route. Infrared Laser Eng. 2007, 4 (Suppl. S2), 423–426. [Google Scholar]
- Li, N.; Yang, C. Radiation calibration for 3–5μm infrared detector. Infrared Laser Eng. 2012, 41, 858–864. [Google Scholar]
- Liu, B.; Zheng, W.; Li, H. Research progress in measurement technology of material surface emissivity. Infrared Technol. 2018, 40, 725–732. [Google Scholar]
- Wang, Z.; Liu, Z.; Xia, X. Measurement Error and Uncertainty Evaluation; Science Press: Beijing, China, 2008. [Google Scholar]
- Sun, Z.; Wu, H.; Yuan, W.; Wen, Z. Calculation Method and Practice; Southeast University Press: Nanjing, China, 2011. [Google Scholar]
- Pierce, A.K.; Allen, R.G. The Solar Spectrum Between 0.3 and 10 μm. In The Solar Output and Its Variation; Colorado Associated University Press: Boulder, CO, USA, 1977. [Google Scholar]
- Jursa, A.S. Handbook of Geophysics and the Space Environment, 4th ed.; Air Force Systems Command; U.S. Department of Commerce National Technical Information Service: Springfield, VA, USA, 1985.
- Yuan, Z. The effect and correction of blackbody radiation emissivity on the accuracy for radiation thermometry. Acta Metrol. Sin. 2007, 28, 19–22. [Google Scholar]
- Ma, J.; Wen, M. Skin Radiation Measurement Method of High Altitude Aircraft Based on Long Wave Infrared Light. Infrared Technol. 2021, 43, 284–291. [Google Scholar]

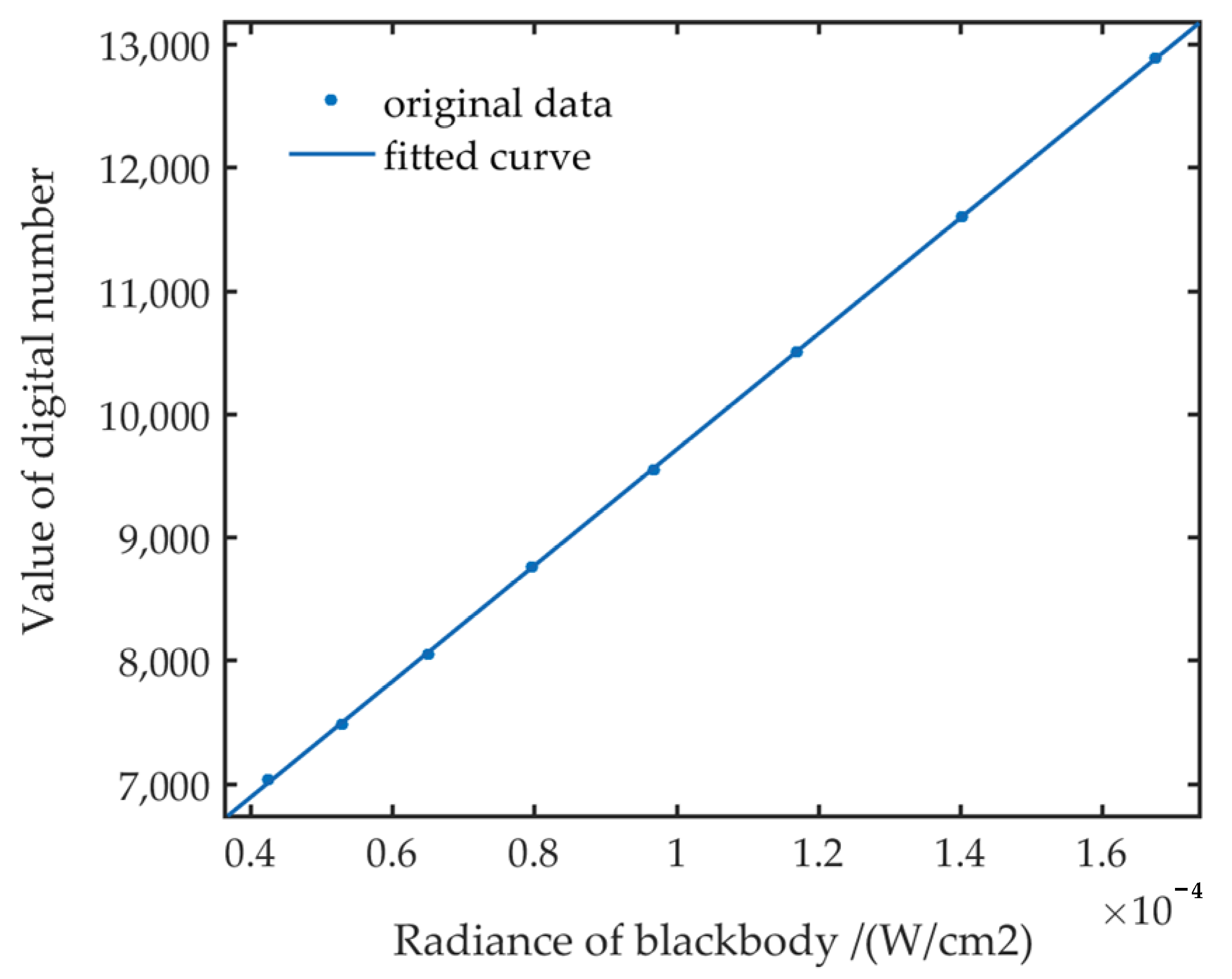

| K | B | RMSE | |
|---|---|---|---|
| 3.7 | −0.009342 | 0.9394 | 0.0001799 |
| 3.8 | −0.005108 | 0.9747 | 0.0003843 |
| 3.9 | −0.002059 | 0.9672 | 0.0002266 |
| 4.0 | −0.001522 | 1.046 | 0.0002771 |
| 4.1 | −0.001672 | 1.033 | 0.0002825 |
| 4.2 | −0.001967 | 1.008 | 0.0001799 |
| 4.3 | −0.003412 | 1.024 | 0.0003843 |
| 4.4 | −0.006245 | 0.9621 | 0.0004364 |
| 4.5 | −0.01069 | 0.8924 | 0.0006834 |
| 4.6 | −0.03198 | 0.7301 | 0.002214 |
| 4.7 | −0.05147 | 0.6721 | 0.002514 |
| 4.8 | −0.1096 | 0.5430 | 0.003284 |
| A | D | RMSE | |
|---|---|---|---|
| 3.7 | −0.0001819 | 0.5540 | 0.0002727 |
| 3.8 | −0.0004674 | 0.6484 | 0.0005531 |
| 3.9 | −0.0002748 | 1.254 | 0.0001910 |
| 4.0 | −0.002860 | 1.035 | 0.0002863 |
| 4.1 | −0.2080 | 0.3545 | 0.005455 |
| 4.2 | −0.9906 | 0.0080 | 0.01117 |
| 4.3 | −0.9786 | 0.0637 | 0.02359 |
| 4.4 | −0.5363 | 0.2544 | 0.05889 |
| 4.5 | −0.005162 | 0.9883 | 0.0004551 |
| 4.6 | −0.001919 | 0.9714 | 0.0002216 |
| 4.7 | −0.009497 | 0.9446 | 0.001027 |
| 4.8 | −0.009232 | 0.5967 | 0.003324 |
| Number of Layer | Atmospheric Pressure/hpa | Temperature/K | Relative Humidity/% | Saturated Water Vapor Content/g·(cm3)−1 |
|---|---|---|---|---|
| 29 | 685.2222 | 278.1854 | 29.7408 | 7.0809 |
| 28 | 696.3333 | 279.1531 | 30.5621 | 7.5911 |
| 27 | 707.4444 | 280.2053 | 30.7385 | 8.2073 |
| 26 | 718.5556 | 281.3268 | 30.3619 | 8.7861 |
| 25 | 729.6667 | 282.4782 | 29.6096 | 9.4842 |
| 24 | 740.7778 | 283.6197 | 28.6605 | 10.2182 |
| 23 | 751.8889 | 284.7116 | 27.6929 | 11.0661 |
| 22 | 763.0000 | 285.7376 | 26.7791 | 11.8898 |
| 21 | 774.1111 | 286.7389 | 25.7333 | 12.6950 |
| 20 | 785.2222 | 287.7575 | 24.3524 | 13.4914 |
| 19 | 796.3333 | 288.7984 | 22.5393 | 14.4903 |
| 18 | 807.4444 | 289.8520 | 20.2653 | 15.5576 |
| 17 | 818.5556 | 290.8794 | 17.8935 | 16.5653 |
| 16 | 829.6667 | 291.8237 | 16.0223 | 17.7032 |
| 15 | 840.7778 | 292.6548 | 14.7151 | 18.5310 |
| 14 | 851.8889 | 293.3754 | 13.3739 | 19.3996 |
| 13 | 863.0000 | 293.975 | 12.2206 | 20.2660 |
| 12 | 874.1111 | 294.4066 | 13.5674 | 20.8110 |
| 11 | 885.2222 | 294.6472 | 19.2452 | 21.0906 |
| 10 | 896.3333 | 294.8249 | 27.0594 | 21.2951 |
| 9 | 907.4444 | 295.1062 | 33.7232 | 21.6279 |
| 8 | 918.5556 | 295.5460 | 38.0968 | 22.2015 |
| 7 | 929.6667 | 296.1275 | 40.4114 | 23.1203 |
| 6 | 940.7778 | 296.8157 | 40.8428 | 24.2205 |
| 6 | 951.8889 | 297.5547 | 39.4749 | 24.6124 |
| 5 | 963.0000 | 298.1789 | 37.5592 | 25.3487 |
| 4 | 974.1111 | 298.260 | 39.1968 | 25.5416 |
| 3 | 985.2222 | 299.3304 | 48.9127 | 28.2848 |
| 2 | 996.3333 | 299.9225 | 71.2322 | 29.1840 |
| 1 | 1007.444 | 300.0181 | 88.5324 | 29.3277 |
| 3.7 | 0.7929 | 4.3 | 1.8464 × 10−3 |
| 3.8 | 0.8235 | 4.4 | 2.5026 × 10−7 |
| 3.9 | 0.8515 | 4.5 | 0.1697 |
| 4.0 | 0.8359 | 4.6 | 0.6249 |
| 4.1 | 0.7080 | 4.7 | 0.4523 |
| 4.2 | 0.1334 | 4.8 | 0.3880 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Deng, X.; Wang, Y.; Han, G.; Xue, T. Research on a Measurement Method for Middle-Infrared Radiation Characteristics of Aircraft. Machines 2022, 10, 44. https://doi.org/10.3390/machines10010044
Deng X, Wang Y, Han G, Xue T. Research on a Measurement Method for Middle-Infrared Radiation Characteristics of Aircraft. Machines. 2022; 10(1):44. https://doi.org/10.3390/machines10010044
Chicago/Turabian StyleDeng, Xuan, Yueming Wang, Guicheng Han, and Tianru Xue. 2022. "Research on a Measurement Method for Middle-Infrared Radiation Characteristics of Aircraft" Machines 10, no. 1: 44. https://doi.org/10.3390/machines10010044
APA StyleDeng, X., Wang, Y., Han, G., & Xue, T. (2022). Research on a Measurement Method for Middle-Infrared Radiation Characteristics of Aircraft. Machines, 10(1), 44. https://doi.org/10.3390/machines10010044







