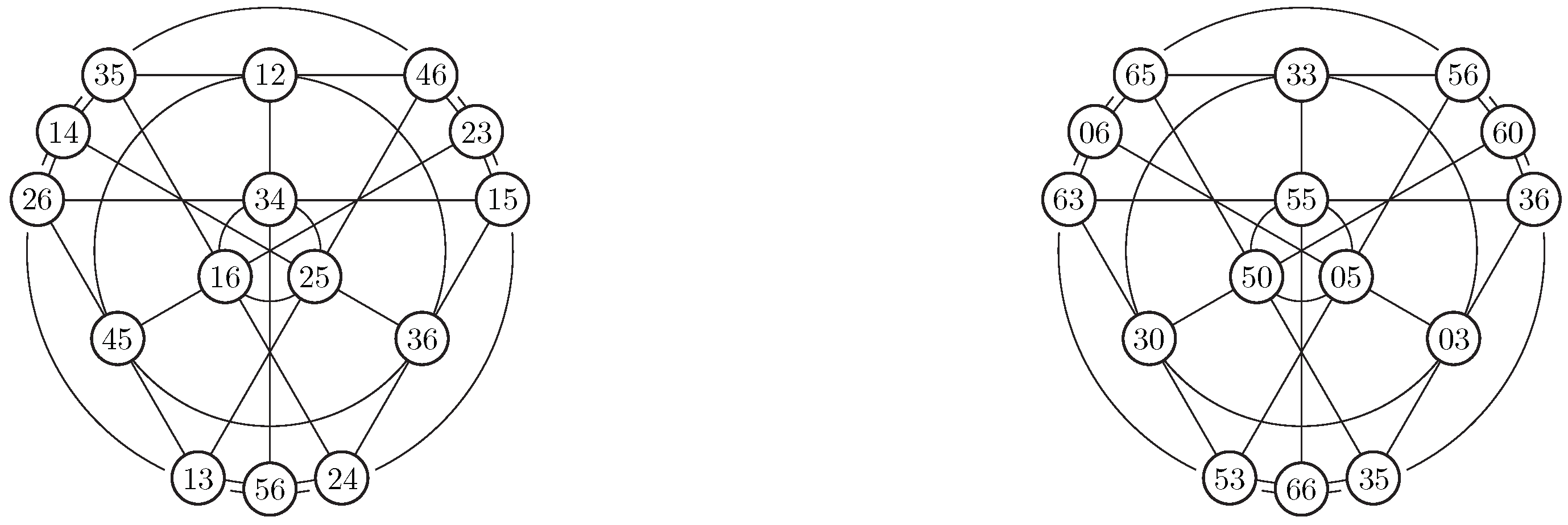Let
R be a finite associative ring with unity (1), and
its free left module. The set
is called a cyclic submodule of
.
is called
free if the mapping
is injective. A pair/vector (
)
is called
unimodular over
R if there exist
such that
. It is well-known (see, for example, [
1]) that if
is unimodular, then
is free. A great majority of finite rings have the property that all their free
’s are generated by unimodular vectors. Here we shall consider a specific ring where this is not true—that is, a ring that also features free
’s containing no unimodular vector. In what follows, we shall call such free cyclic submodules “non-unimodular”. Our ring
R is a non-commutative one of order 16, defined as follows:
Labeling the 16 matrices as follows:
we see that 0 is the additive identity, 1 is the multiplicative identity, and the only invertible elements are 1, 2, 4, and 7. The ring features two (two-sided) maximal ideals, namely:
and
and its Jacobson radical reads:
From the above-given matrix representation of
R, we find that
contains nine distinct free cyclic submodules generated by non-unimodular vectors, which are listed in
Table 1.
We shall show that the way in which these free cyclic submodules are interwoven is intricately related to the structure of the generalized quadrangle of order two, the doily. To this end, we employed a duad-syntheme model of the latter (see, for example, [
2]). Take a six-element set
. Let us call a two-element subset of
S a duad, and a set of three duads forming a partition of
S a syntheme. Then, the point-line incidence structure whose points are 15 duads and whose lines are 15 synthemes, with incidence being containment, is isomorphic to the doily. The structure of the doily is illustrated in
Figure 1 (left). Here, the points of the doily are represented by circles, labeled by duads, and its lines are represented by nine straight segments, three concentric circles, and three arcs of circles; one can readily check that each line corresponds to a syntheme. Next, we employ the following bijection between the 15 duads and 15 nontrivial vectors
, where
and
:
We thus get a new labeling of the points of the doily in terms of these particular non-unimodular vectors of
, as illustrated in
Figure 1 (right). From a comparison of the latter figure with
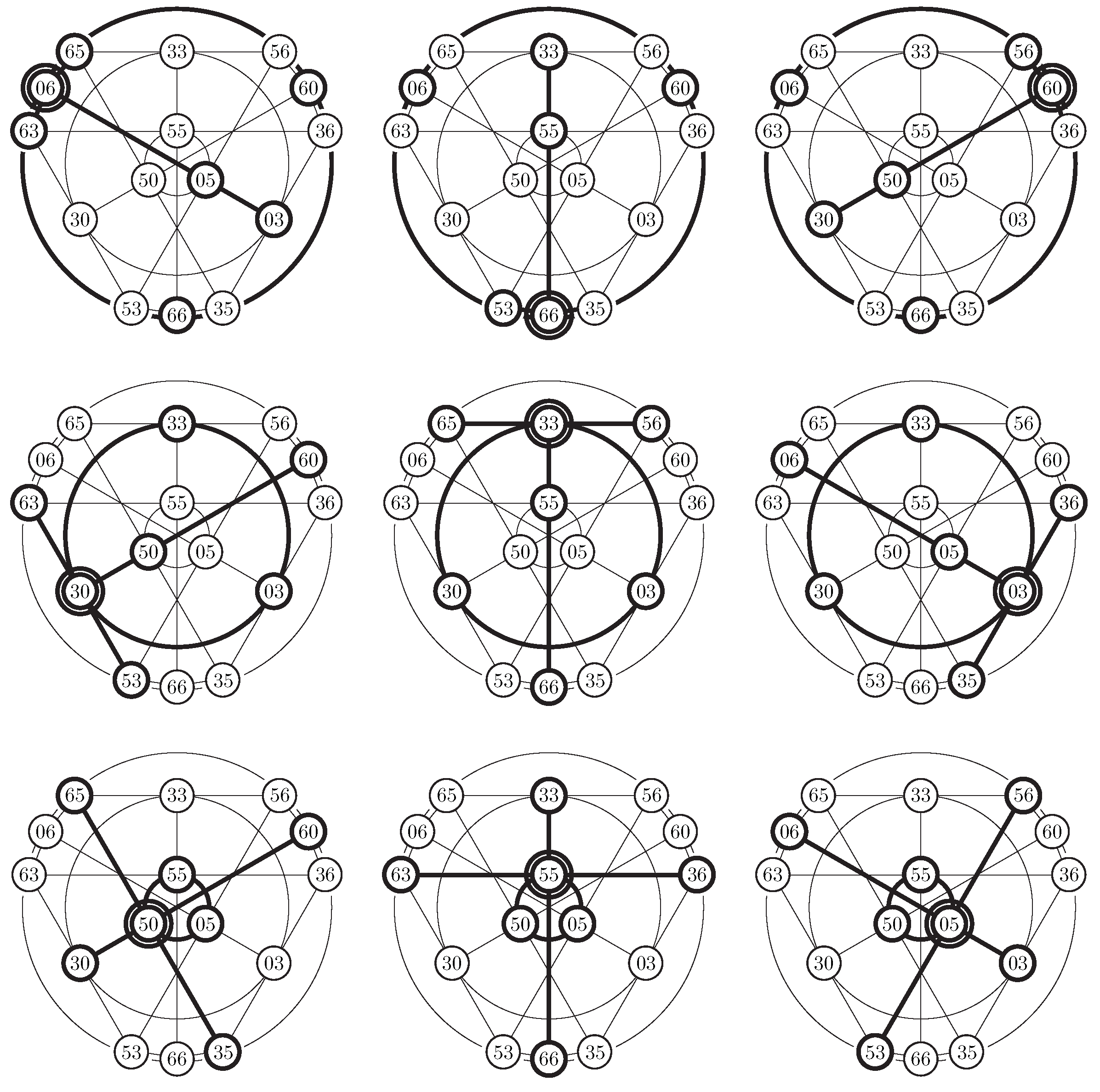
Table 1, it follows that each submodule shares with the doily seven vectors, forming three concurrent lines—as depicted in
Figure 2. From this figure, one can easily discern that six lines of the doily have a different status than the other nine, as each of them belongs to three submodules. Given the fact that each point of concurrence belongs to two such lines, the nine points and the six lines are found to form inside the doily a point-line incidence structure isomorphic to the generalized quadrangle of type GQ(2,1) (see [
2]).
A complete view of the relation between individual submodules is outlined in
Figure 3. The pronounced automorphism of order three of the figure stems from the fact that the submodules form three disjoint triples according to the number of shared vectors. One further observes that all vectors lying on our submodules acquire values from the ideal
. It can readily be verified that a completely analogous geometric structure is obtained if we take the free
right module, in which case the corresponding vectors have entries from the ideal
. Obviously, the two structures share the same doily, as its points are labeled by vectors from
. At this point it is well worth recalling the existence of a similar geometrical structure in the case of the smallest ring of ternions and its three-dimensional free left (and also right) module [
3]. There, the associated geometry, referred to as the “Fano-snowflake”, has its center isomorphic to the Fano plane, a generalized triangle of order two (see also [
4] for generalization to an arbitrary ring of ternions).
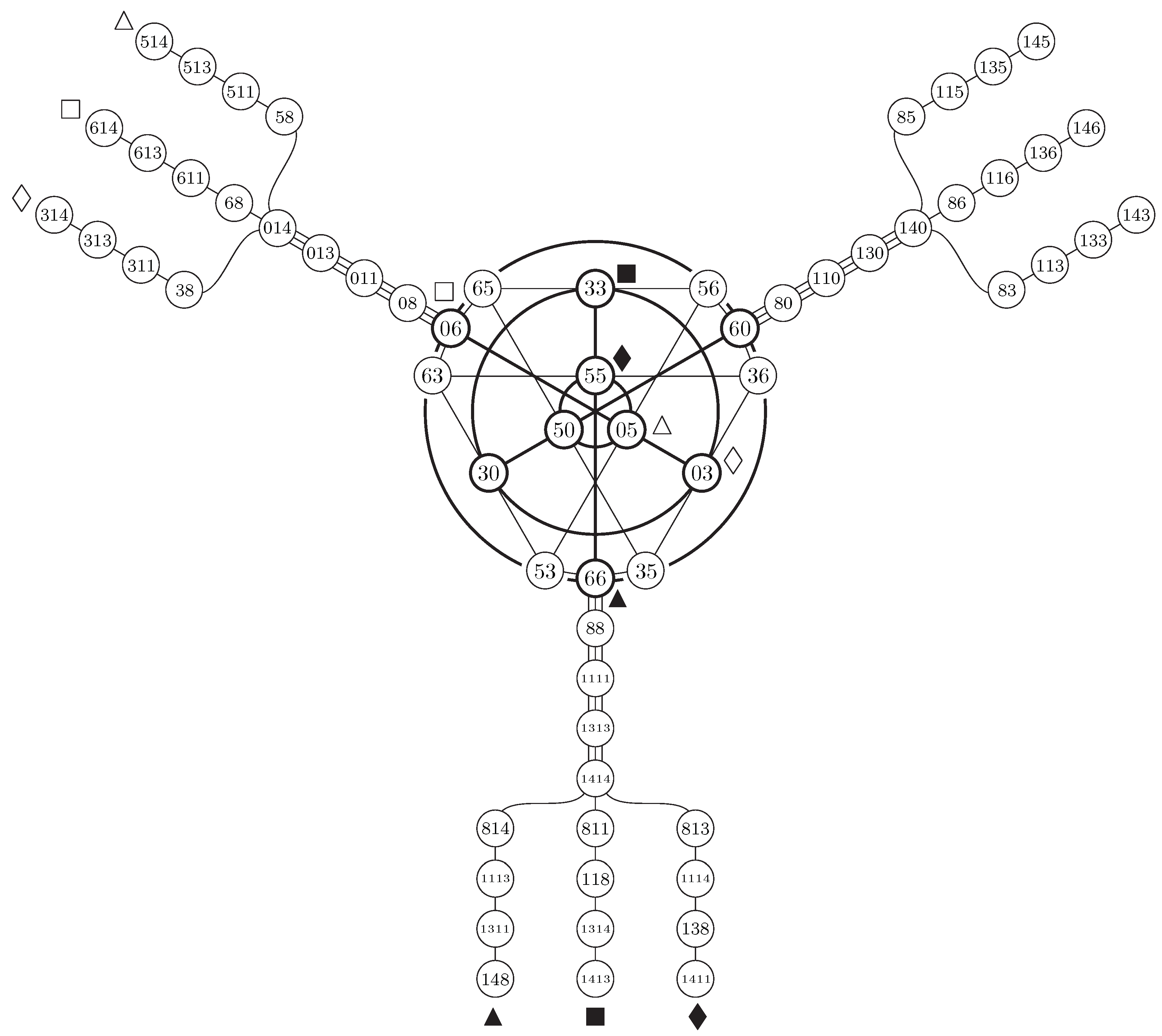
The occurrence of the doily in this remarkable nonunimodular ring-theoretic setting is quite intriguing. We checked, case by case, all non-commutative associative rings with unity up to order 31 inclusive and found, up to the isomorphism, no other ring having a similar property. However, there does exist a ring of order 32 which is a promising candidate in this respect. This particular ring features 21 non-unimodular free cyclic submodules whose mutual relations seem to be expressible in terms of the structure of the split Cayley hexagon of order two; the latter is, like the doily, a generalized polygon and a distinguished subgeometry of the symplectic polar space of type at that. We, therefore, surmise that the “phenomenon” described above is an example of a more general mathematical rule linking ring geometry and polar spaces (or generalized polygons).
This doily-based setting is also of considerable interest in view of possible physical applications. For example, among the finite geometric concepts relevant for the theory of quantum information, the doily—though in various disguises—has been recognized to play the foremost role. Firstly, being isomorphic to the symplectic polar space of type
, it underlies the commutation relations between the elements of the two-qubit Pauli group [
5] and provides us with the simplest settings for observable proofs of quantum contextuality. Secondly, being isomorphic to a non-singular quadric of type
, it also lies in the heart of a remarkable magic three-qubit Veldkamp line of form theories of gravity and its four-qubit extensions [
6]. Finally, being a subquadrangle of a generalized quadrangle of type GQ(2, 4), it enters in an essential way certain black-hole entropy formulas and the so-called black-hole/qubit correspondence [
7].
It is, in particular, the first aspect of the above-mentioned three ones that our non-unimodular doily has a particular link to. For if we label its 15 points by the 15 elements (identity disregarded) of the two-qubit Pauli group in such a way that each of its 15 lines features three pairwise commuting elements, then each of the ten labeled copies of GQ(2,1) (also known in the physics community as Mermin “magic” squares) contained in the doily furnishes an observable-based proof of quantum contextuality (see, e.g., Section 2 and Figure 3 of [
6] for a particularly illustrative description of this quantum phenomenon). It is quite appealing to realize that not only does our non-unimodular geometry exhibit the doily, but it also
selects in the latter a particular copy of GQ(2,1) (see
Figure 3). Moreover, it has recently been shown [
8] that this contextual character of GQ(2,1) is preserved also for doilies embedded in arbitrary symplectic polar spaces of type
and, thus, labeled by elements of the arbitrary
N-qubit Pauli group,
.