A Two Dimensional Discrete Mollification Operator and the Numerical Solution of an Inverse Source Problem
Abstract
1. Introduction
2. The 2D Discrete Mollification Operator (2DDM Operator)
2.1. One-Dimensional Discrete Mollification
2.2. Mollification Weights
2.3. Two-Dimensional Discrete Mollification
2.4. Abstract Setting
- ,
- outside ,
- .
- 1.
- Consistency
- 2.
- Stability: If is a Lipschitz continuous approximation of g so that , then:
3. The Inverse Source Problem
3.1. Direct Problem
3.2. Inverse Problem
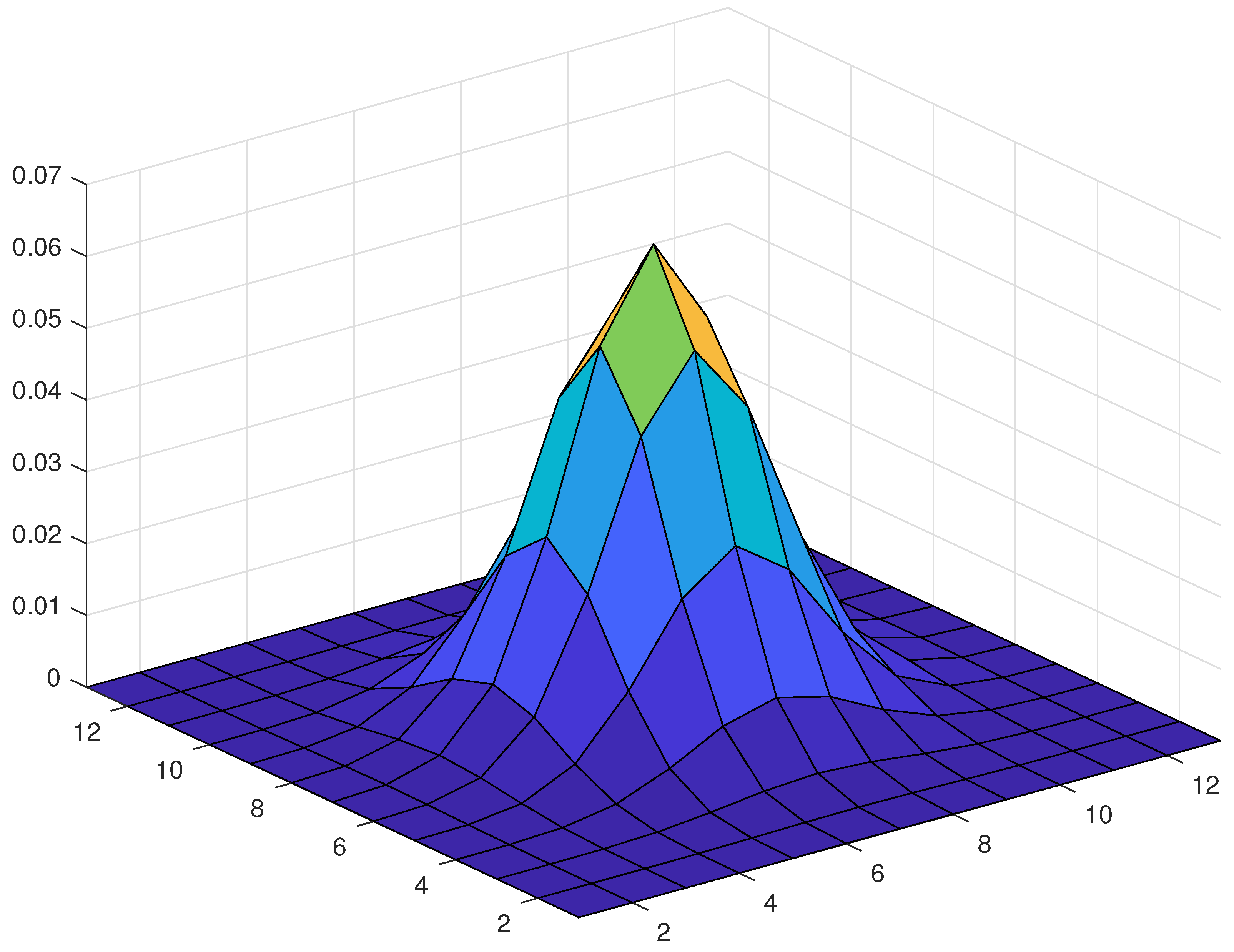
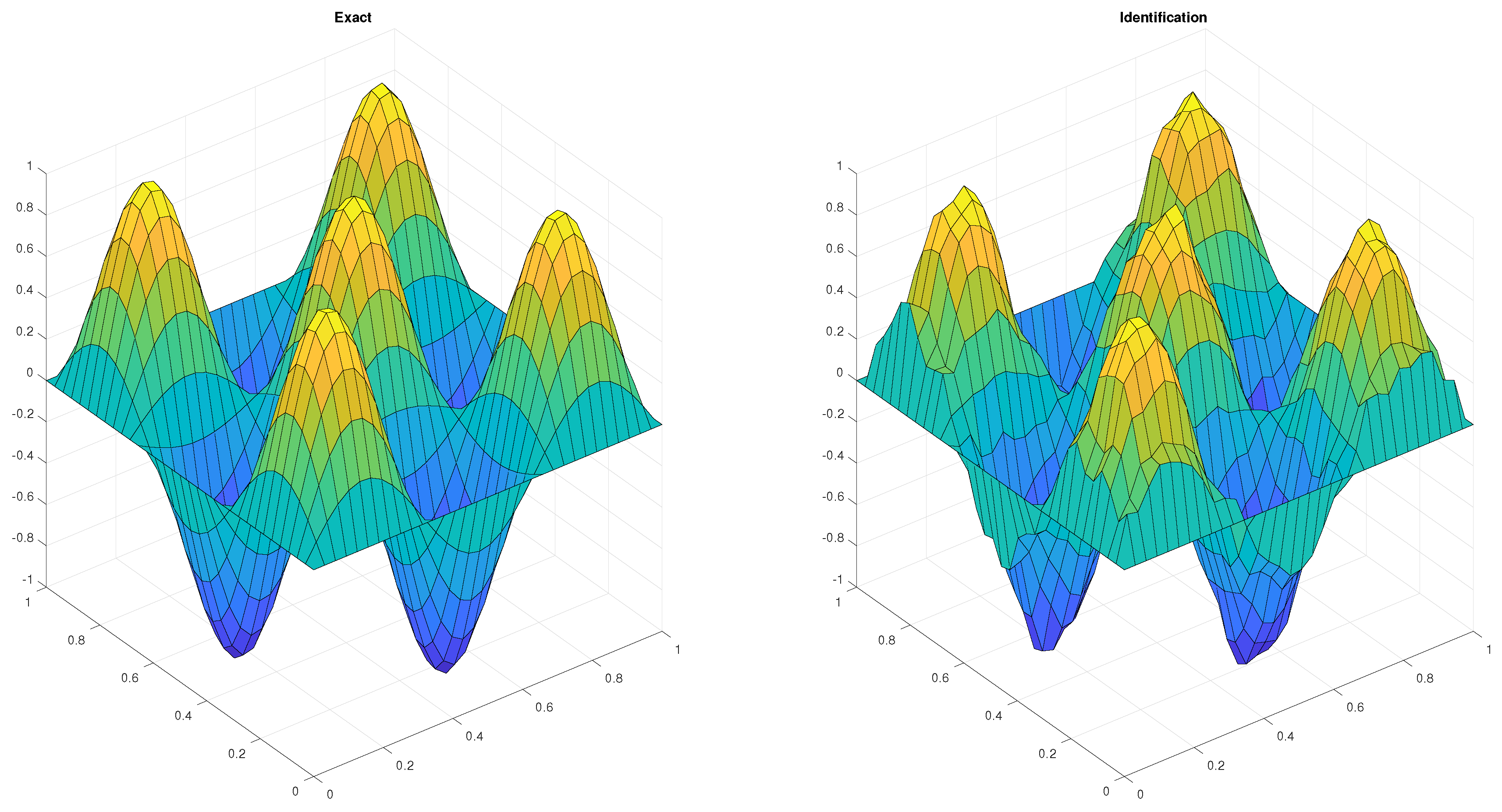
4. Numerical Results
Example 1
Example 2
5. Discussion and Final Comments
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Acosta, C.D.; Mejía, C.E. Stable Computations by Discrete Mollification; Universidad Nacional de Colombia: Bogotá, Colombia, 2014. [Google Scholar]
- Murio, D.A. Mollification and Space Marching. In Inverse Engineering Handbook; Woodbury, K., Ed.; CRC Press: Boca Raton, FL, USA, 2002. [Google Scholar]
- Acosta, C.D.; Mejía, C.E. Stabilization of explicit methods for convection diffusion equations by discrete mollification. Comput. Math. Appl. 2008, 55, 368–380. [Google Scholar] [CrossRef]
- Acosta, C.D.; Buerger, R. Difference schemes stabilized by discrete mollification for degenerate parabolic equations in two space dimensions. IMA J. Numer. Anal. 2012, 32, 1509–1540. [Google Scholar] [CrossRef]
- Shi, C.; Wang, C.; Wei, T. Convolution regularization method for backward problems of linear parabolic equations. Appl. Numer. Math. 2016, 108, 143–156. [Google Scholar] [CrossRef]
- Garshasbi, M.; Dastour, H. Estimation of unknown boundary functions in an inverse heat conduction problem using a mollified marching scheme. Numer. Algorithms 2015, 68, 769–790. [Google Scholar] [CrossRef]
- Mejía, C.E.; Piedrahita, A. Solution of a time fractional inverse advection-dispersion problem by discrete mollification. Rev. Colomb. Mat. 2017, 51, 83–102. [Google Scholar] [CrossRef]
- Yang, F.; Fu, C.; Li, X. A mollification regularization method for unknown source in time-fractional diffusion equation. Int. J. Comput. Mat. 2014, 91, 1516–1534. [Google Scholar] [CrossRef]
- Beatson, R.; Bui, H.Q. Mollification formulas and implicit smoothing. Adv. Comput. Mat. 2007, 27, 125–149. [Google Scholar] [CrossRef]
- Yi, Z.; Murio, D.A. Identification of Source Terms in 2-D IHCP. Comput. Math. Appl. 2004, 47, 1517–1533. [Google Scholar] [CrossRef]
- Ma, Y.; Prakash, P.; Deiveegan, A. Generalized Tikhonov methods for an inverse source problem of the time-fractional diffusion equation. Chaos Solitons Fract. 2018, 108, 39–48. [Google Scholar] [CrossRef]
- Nguyen, H.T.; Le, D.L.; Nguyen, V.T. Regularized solution of an inverse source problem for a time fractional diffusion equation. Appl. Math. Model. 2016, 40, 8244–8264. [Google Scholar] [CrossRef]
- Wei, T.; Wang, J. A modified quasi-boundary value method for an inverse source problem of the time-fractional diffusion equation. Appl. Numer. Math. 2014, 78, 95–111. [Google Scholar] [CrossRef]
- Mejía, C.E.; Piedrahita, A. A finite difference approximation of a two dimensional time fractional advection-dispersion problem. arXiv 2018, arXiv:1807.07393v1. [Google Scholar]
- Sakamoto, K.; Yamamoto, M. Inverse source problem with a final overdetermination for a fractional diffusion equation. Math. Control Relat. Fields 2011, 1, 509–518. [Google Scholar] [CrossRef]
- Jiang, D.; Li, Z.; Liu, Y.; Yamamoto, M. Weak unique continuation property and a related inverse source problem for time-fractional diffusion-advection equations. Inverse Probl. 2017, 33, 055013. [Google Scholar] [CrossRef]
- Zhan, S.; Murio, D.A. Surface fitting and numerical gradient computation by discrete mollification. Comput. Math. Appl. 1999, 37, 145–159. [Google Scholar] [CrossRef]
- Podlubny, I. Fractional Differential Equations; Academic Press: Cambridge, MA, USA, 1999. [Google Scholar]
- Voyiadjis, G.Z.; Sumelka, W. Brain modelling in the framework of anisotropic hyperelasticity with time fractional damage evolution governed by the Caputo-Almeida fractional derivative. J. Mech. Behav. Biomed. Mater. 2019, 89, 209–216. [Google Scholar] [CrossRef] [PubMed]
- Fomin, S.; Chugunov, V.; Hashida, T. Application of fractional differential equations for modeling the anomalous diffusion of contaminant from fracture into porous rock matrix with bordering alteration zone. Transp. Porous Med. 2010, 81, 187–205. [Google Scholar] [CrossRef]
- Sakamoto, K.; Yamamoto, M. Initial value/boundary value problems for fractional diffusion-wave equations and applications to some inverse problems. J. Math. Anal. Appl. 2011, 382, 426–447. [Google Scholar] [CrossRef]
- Odibat, Z. Approximations of fractional integrals and Caputo fractional derivatives. Appl. Math. Comput. 2006, 178, 527–533. [Google Scholar] [CrossRef]
- Sumelka, W.; Szajek, K.; Lodygowski, T. Plain strain and plane stress elasticity under fractional continuum mechanics. Arch. Appl. Mech. 2015, 85, 1527–1544. [Google Scholar] [CrossRef]
- Zhuang, P.; Liu, F. Finite difference approximation for two-dimensional time fractional diffusion equation. J. Algorithms Comput. Technol. 2007, 1, 1–16. [Google Scholar] [CrossRef]
| 1 | 8.4272 | 7.8640 | ||||
| 2 | 6.0387 | 1.9262 | 5.4438 | |||
| 3 | 4.5556 | 2.3772 | 3.3291 | 1.2099 | ||
| 4 | 3.6266 | 2.4003 | 6.9440 | 8.7275 | 4.7268 | |
| 5 | 3.0028 | 2.2625 | 9.6723 | 2.3430 | 3.2095 | 2.4798 |
| 0.1 | 0.5 | 0.7 | 0.9 | |
|---|---|---|---|---|
| 0.11237258 | 0.11341573 | 0.11482934 | 0.11338589 |
| 0.005 | 0.01 | 0.025 | 0.05 | |
|---|---|---|---|---|
| 3 | 4 | 5 | 6 | |
| 0.05634342 | 0.11141561 | 0.17683141 | 0.24996681 |
| 0.1 | 0.5 | 0.7 | 0.9 | |
|---|---|---|---|---|
| 0.18679846 | 0.18330355 | 0.19129097 | 0.19253567 |
| 0.005 | 0.01 | 0.025 | 0.05 | |
|---|---|---|---|---|
| 3 | 4 | 5 | 6 | |
| 0.0715818 | 0.12090887 | 0.19295488 | 0.26167402 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Echeverry, M.D.; Mejía, C.E. A Two Dimensional Discrete Mollification Operator and the Numerical Solution of an Inverse Source Problem. Axioms 2018, 7, 89. https://doi.org/10.3390/axioms7040089
Echeverry MD, Mejía CE. A Two Dimensional Discrete Mollification Operator and the Numerical Solution of an Inverse Source Problem. Axioms. 2018; 7(4):89. https://doi.org/10.3390/axioms7040089
Chicago/Turabian StyleEcheverry, Manuel D., and Carlos E. Mejía. 2018. "A Two Dimensional Discrete Mollification Operator and the Numerical Solution of an Inverse Source Problem" Axioms 7, no. 4: 89. https://doi.org/10.3390/axioms7040089
APA StyleEcheverry, M. D., & Mejía, C. E. (2018). A Two Dimensional Discrete Mollification Operator and the Numerical Solution of an Inverse Source Problem. Axioms, 7(4), 89. https://doi.org/10.3390/axioms7040089





