Convergence Analysis for System of Cayley Generalized Variational Inclusion on q-Uniformly Banach Space

Abstract

This paper is devoted to the analysis of a system of generalized variational inclusion problems involving $\alpha$-averaged and Cayley operators within the framework of a q-uniformly smooth Banach space. We demonstrate that the problem can be reformulated as an equivalent fixed-point equation and propose an iterative method based on the fixed-point approach to obtain the solution. Furthermore, we establish the existence of solutions and analyze the convergence properties of the proposed algorithm under suitable conditions. To validate the effectiveness of the proposed iterative method, we provide a numerical result supported by a computational graph and a convergence plot, illustrating its performance and efficiency.

1. Introduction

Variational inclusion problems constitute a fundamental aspect of numerous areas within mathematics and its applications, including optimization, control theory, and economics [1,2,3,4]. These problems are concerned with determining elements in a given space that fulfill prescribed inclusion conditions, often involving sophisticated operators that model complex systems. Among these, averaged and Cayley operators are particularly significant due to their deep theoretical underpinnings and effectiveness in solving nonlinear problems.

The generalization of variational inclusion problems broadens the classical framework by introducing more general classes of operators and relaxed conditions, allowing for the modeling of complex and realistic phenomena in applied mathematics. However, this increased flexibility also brings additional analytical and computational challenges, especially in the presence of nonlinear and multivalued operators.

A key tool in addressing such complexities is the concept of averaged operators, originally introduced by Baillon et al. [5] in the study of asymptotic behavior of non-expansive mappings and semigroups. Averaged operators play a vital role in the analysis of optimization problems, variational inequalities, and dynamical systems due to their desirable convergence and stability properties. These operators underpin many iterative methods used in solving fixed-point problems and monotone inclusions.

For further developments and generalized frameworks of variational inclusions, readers may refer to the works in [6,7,8,9,10], where various extensions involving duality mappings, generalized accretive operators, and coupled inclusion problems are explored.

The resolvent operator technique, introduced by Hassouni and Moudafi [10] in 1994, has become a fundamental tool for studying variational inequalities involving maximal monotone and single-valued mappings, collectively known as variational inclusions. Building on this, the Cayley operator, which utilizes the resolvent operator [11,12,13], was further investigated by Fang and Huang in 2004, providing deeper insights into the subject.The Cayley transform plays a pivotal role in linear algebra by mapping skew-symmetric matrices to special orthogonal matrices. In Hilbert spaces, it extends naturally to linear operators, maintaining essential structural features. As a homographic transformation, it has far-reaching applications in real, complex, and quaternionic analysis.

The averaged operator offers a robust framework for constructing non-expansive mappings [14,15], which play a crucial role in establishing convergence results. In parallel, the Cayley operator, grounded in accretive operator theory, enables the reformulation of variational inclusion problems as fixed-point problems, thus simplifying their analysis. Together, these operators form a cohesive foundation for addressing generalized variational inclusions, supporting strong and weak convergence analysis in q-uniformly smooth Banach spaces. Meanwhile, fixed-point theory remains a vibrant area of research, with a steadily increasing number of studies devoted to its advancement in recent years. Given the strong connection between variational inequality/inclusion problems and fixed-point problems, many researchers have focused on creating a unified approach that bridges these two areas.

This work explores a system of generalized variational inclusion problems formulated in the setting of q-uniformly smooth Banach spaces. These spaces generalize the traditional concept of uniformly smooth Banach spaces by introducing a q-dependent modulus of smoothness, which characterizes how duality mappings interact with geometric convexity. Such a framework is particularly advantageous for studying algorithms that exploit the smoothness and convex structure of the functional space. For additional discussions, refer to [7,16,17,18].

In this paper, we examine a generalized variational inclusion problem that incorporates both $\alpha$-averaged operators and Cayley operators within a q-uniformly smooth Banach space. A central contribution of this study is establishing the equivalence between this inclusion problem and a corresponding fixed-point formulation.

2. Basic Concepts

Let $(E,\parallel ·\parallel )$ be a Banach space, i.e., a complete normed vector space. Its dual, $(E^{*},\parallel ·\parallel )$, is the space of all bounded linear functionals on E, and is itself a Banach space. In a q-uniformly smooth Banach space E, we denote the norm by $\parallel ·\parallel$, the duality pairing between E and $E^{*}$ by $\langle ·,·\rangle$, and the power set of E by $2^E$.

The generalized duality mapping, ${\mathcal{J}}_q:E\to 2^{E^{*}}$, is defined as follows:

$${\mathcal{J}}_q{\left(x\right)=\{f\in E:\langle x,f\rangle =\parallel x\parallel }^q\mathrm{and}\parallel f\parallel ={\parallel x\parallel }^{q-1}\},\forall x\in E.$$

For $q=2$, the generalized duality mapping coincides with the normalized duality mapping. Additionally, if the space E is uniformly smooth, then ${\mathcal{J}}_q$ is single-valued.

A Banach space is said to be q-uniformly smooth if

$${\rho }_E\left(t\right)\le k{t}^q,$$

where $q>1$, $k>0$ are constants, and ${\rho }_E\left(t\right)$ denotes the modulus of smoothness of the space E.

Definition 1 ([1]).

Let $P:E\to E$ be a single valued mapping. Then, P is said to be:

1. 

Accretive, if for all $x,y\in E$, the following inequality holds:

$$\langle P\left(x\right)-P\left(y\right),{\mathcal{J}}_q(x-y)\rangle \ge 0;$$

2. 

Strictly accretive, if for all $x,y\in E$,

$$\langle P\left(x\right)-P\left(y\right),{\mathcal{J}}_q(x-y)\rangle >0,$$

with equality if and only if $x=y$;

3. 

Strongly accretive, if there exists a constant $r>0$ such that

$$\langle P\left(x\right)-P\left(y\right),{\mathcal{J}}_q(x-y)\rangle \ge r{\parallel x-y\parallel }^q,\forall x,y\in E;$$

4. 

Lipschitz continuous, if there exists a constant ${\lambda }_P>0$ such that

$$\parallel P\left(x\right)-P\left(y\right)\parallel \le {\lambda }_P\parallel x-y\parallel ,\forall x,y\in E;$$

5. 

Relaxed Lipschitz continuous, if there exists a constant $c>0$ such that

$$\langle P\left(x\right)-P\left(y\right),{\mathcal{J}}_q(x-y)\rangle \le -c{\parallel x-y\parallel }^q,\forall x,y\in E.$$

Definition 2.

A multi-valued mapping $M:E\to 2^E$ is said to be accretive if for all $x,y\in E$,

$$\langle u-v,{\mathcal{J}}_q(x-y)\rangle \ge 0,\forall u\in M\left(x\right),v\in M\left(y\right).$$

Definition 3.

An operator $T:E\to E$ is called α-averaged if for $\alpha \in (0,1)$,

$$T=(1-\alpha )I+\alpha N,$$

where $N:E\to E$ is a non-expansive operator and I is an identity operator.

Definition 4.

A mapping $F:E\times E\to E$ is said to be Lipschitz continuous with respect to the first argument if there exists a constant $L>0$ such that

$$\begin{array}{c}\hfill \parallel F(x_1,·)-F(x_2,·)\parallel \le L\parallel x_1-x_2\parallel \forall x_1,x_2\in E.\end{array}$$

Similarly, we can define the Lipschitz continuity in the second argument.

Definition 5 ([11,13]).

The generalized resolvent operator $R_{P,\lambda }^M:E\to E$ is given by

$$R_{P,\lambda }^M\left(x\right)={[P+\lambda M]}^{-1}\left(x\right),\forall x\in E\mathit{and}\lambda >0,$$

(1)

where $P:E\to E$ is a single-valued mapping and $M:E\to 2^E$ is a multi-valued P-accretive mapping.

Definition 6.

The generalized Cayley operator $C_{P,\lambda }^M:E\to E$ is defined as

$$C_{P,\lambda }^M\left(x\right)=\left[2R_{P,\lambda }^M-P\right]\left(x\right),\forall x\in E,\lambda >0,$$

(2)

where $R_{P,\lambda }^M$ is a resolvent operator defined by (1), $P:E\to E$ is a single-valued mapping, and $M:E\to 2^E$ is a P-accretive multi-valued mapping.

Proposition 1.

Assume that E denotes a uniformly smooth Banach space. Then, E is said to be q-uniformly smooth if and only if there exists a constant $C_q$ such that for all $x,y\in E$, the following inequality holds:

$${\parallel x+y\parallel }^q\le {\parallel x\parallel }^q+q\langle y,{\mathcal{J}}_q\left(x\right)\rangle +C_q{\parallel y\parallel }^q.$$

Proposition 2

([11,13]). Let $M_1,M_2:E\to 2^E$ be multi-valued mappings, and let $P_1,P_2:E\to E$ be strongly accretive operators with constants r and s, respectively. Then, for any $\sigma ,\tau >0$, the generalized resolvent operators $R_{P_1,\sigma }^{M_1}$ and $R_{P_2,\tau }^{M_2}$, defined as in (1), are Lipschitz continuous on E with Lipschitz constants ${\displaystyle \frac{1}{r}}$ and ${\displaystyle \frac{1}{s}}$, respectively, where $r,s>0$.

$$\begin{array}{c}\hfill ∥R_{P_1,\sigma }^{M_1}\left(x\right)-R_{P_1,\sigma }^{M_1}\left(y\right)∥\le {\displaystyle \frac{1}{r}}\parallel x-y\parallel ,\end{array}$$

(3)

$$\begin{array}{c}\hfill ∥R_{P_2,\tau }^{M_2}\left(x\right)-R_{P_2,\tau }^{M_2}\left(y\right)∥\le {\displaystyle \frac{1}{s}}\parallel x-y\parallel .\end{array}$$

(4)

Proposition 3

([11,13]). Let $M_1,M_2:E\to 2^E$ be multi-valued mappings, and let $P_1,P_2:E\to E$ be strongly accretive and Lipschitz-continuous operators with constants $r,s>0$ and Lipschitz constants ${\lambda }_{P_1}$ and ${\lambda }_{P_2}$, respectively. Then, the generalized Cayley operators $C_{P_1,\sigma }^{M_1}$ and $C_{P_2,\tau }^{M_2}:E\to E$, defined by (2), are Lipschitz continuous with constants ${\theta }_1={\displaystyle \frac{2}{r}}+{\lambda }_{P_1}$ and ${\theta }_2={\displaystyle \frac{2}{s}}+{\lambda }_{P_2}$, respectively. Specifically,

$$\begin{array}{cc}\hfill ∥C_{P_1,\sigma }^{M_1}\left(x\right)-C_{P_1,\sigma }^{M_1}\left(y\right)∥& \le {\theta }_1\parallel x-y\parallel ,\hfill \end{array}$$

(5)

$$\begin{array}{cc}\hfill ∥C_{P_2,\tau }^{M_2}\left(x\right)-C_{P_2,\tau }^{M_2}\left(y\right)∥& \le {\theta }_2\parallel x-y\parallel .\hfill \end{array}$$

(6)

Lemma 1.

Let $T:E\to E$ be an α-averaged operator, and let $N:E\to E$ be a relaxed Lipschitz-continuous operator with constant ${\lambda }_N$. Then, for all $x,y\in E$, the following inequality holds:

$$\parallel T\left(x\right)-T\left(y\right)\parallel \le {\gamma }_{\mathsf{\Theta }}\parallel x-y\parallel ,$$

where ${\gamma }_{\mathsf{\Theta }}=\sqrt[q]{{(1-\alpha )}^q+C_q{\alpha }^q+q\alpha (1-\alpha ){\lambda }_N}$.

Proof. 

Since N is relaxed Lipschitz, and using Proposition 1,

$$\begin{array}{ccc}\hfill {\parallel T\left(x\right)-T\left(y\right)\parallel }^q& =& {\parallel \left[(1-\alpha )(I\left(x\right)-I\left(y\right))\right]+\left[\alpha (N\left(x\right)-N\left(y\right))\right]\parallel }^q\hfill \\ & \le & {(1-\alpha )}^q{\parallel (I\left(x\right)-I\left(y\right))\parallel }^q+C_q{\alpha }^q{\parallel N\left(x\right)-N\left(y\right)\parallel }^q\hfill \\ & & +q\alpha (1-\alpha )\langle N\left(x\right)-N\left(y\right),J_q(x-y)\rangle \hfill \\ & \le & {(1-\alpha )}^q{\parallel (I\left(x\right)-I\left(y\right))\parallel }^q+C_q{\alpha }^q{\parallel x-y\parallel }^q\hfill \\ & & +q\alpha (1-\alpha )\langle N\left(x\right)-N\left(y\right),J_q(x-y)\rangle \hfill \\ & \le & {(1-\alpha )}^q{\parallel x-y\parallel }^q+C_q{\alpha }^q{\parallel x-y\parallel }^q+q\alpha (1-\alpha ){\lambda }_N{\parallel x-y\parallel }^q\hfill \\ & \le & [{(1-\alpha )}^q+C_q{\alpha }^q+q\alpha (1-\alpha ){\lambda }_N]{\parallel x-y\parallel }^q\hfill \\ & \le & \sqrt[q]{\left({(1-\alpha )}^q+C_q{\alpha }^q+q\alpha (1-\alpha ){\lambda }_N\right)}\parallel x-y\parallel .\hfill \end{array}$$

which implies that

$$\begin{array}{c}\hfill \parallel T\left(x\right)-T\left(y\right)\parallel \le {\gamma }_{\mathsf{\Theta }}\parallel x-y\parallel .\end{array}$$

□

3. Problem and Its Fixed Point Formulations

Let $T:E\to E$ be a single-valued mapping. Consider $M_1,M_2:E\to 2^E$ as maximal multivalued mappings and, for positive constants $\sigma ,\tau >0$, let the generalized Cayley operators $C_{P_1,\sigma }^{M_1},C_{P_2,\tau }^{M_2}:E\to E$ be well defined. Furthermore, let $F_1,F_2:E\times E\to E$ be mappings. Given this setup, we consider the following system of generalized variational inclusion problems that involve the $\alpha$-averaged operator and Cayley operator:

Find $x,y\in E$ such that

$$\begin{array}{c}\hfill 0\in F_1\left(T\left(x\right),C_{P_1,\sigma }^{M_1}\left(y\right)\right)+M_1\left(g\left(x\right)\right),\end{array}$$

(7)

$$\begin{array}{c}\hfill 0\in F_2\left(T\left(y\right),C_{P_2,\tau }^{M_2}\left(x\right)\right)+M_2\left(g\left(y\right)\right).\end{array}$$

(8)

If $F_1(T\left(x\right),C_{P_1,\sigma }^{M_1}\left(y\right))=F_2(T\left(y\right),C_{P_2,\tau }^{M_2}\left(x\right))\equiv 0$ and $g(·)=I$ is the identity mapping, then problems (7) and (8) simplify to the task of finding $x,y\in E$ such that $0\in M_1\left(x\right)$ and $0\in M_2\left(y\right)$, which corresponds to a classical inclusion problem commonly encountered in various applied fields.

If $M_1\left(g(·)\right)=\partial \psi \left(g(·)\right)$, where $\partial \psi (·)$ denotes the subdifferential of a proper, convex, and lower semi-continuous function $\psi :E\to \mathbb{R}\cup \{+\infty \}$, then problems (7) and (8) are equivalent to finding $x\in E$ such that

$$〈F_1(T\left(u\right),C_{P_1,\sigma }^{M_1}\left(x\right)),g\left(u\right)-g\left(x\right)〉+\psi \left(g\left(v\right)\right)-\psi \left(g\left(x\right)\right)\ge 0,\forall x\in E,$$

which is referred to as a mixed variational inequality problem [19].

Moreover, if $\partial \psi \left(g\right(·\left)\right)$ corresponds to the indicator function of a closed convex subset of E, then the problem reduces to finding $x\in E$ such that

$$〈F_1(T\left(u\right),C_{P_1,\sigma }^{M_1}\left(x\right)),g\left(u\right)-g\left(x\right)〉\ge 0,$$

which is known as a bidirectional variational inequality [19].

Lemma 2.

The system of the generalized variational inclusion problem involving an α-averaged operator and Cayley operator has a unique solution $(x,y)\in E\times E$ if and only if the below conditions hold:

$$\begin{array}{c}\hfill g\left(x\right)=R_{P_1,\sigma }^{M_1}\left[P_1\left(g\left(x\right)\right)-\sigma F_1(T\left(x\right),C_{P_1,\sigma }^{M_1}\left(y\right))\right],\end{array}$$

(9)

$$\begin{array}{c}\hfill g\left(y\right)=R_{P_2,\tau }^{M_2}\left[P_2\left(g\left(y\right)\right)-\tau F_2(T\left(y\right),C_{P_2,\tau }^{M_2}\left(x\right))\right].\end{array}$$

(10)

Proof. 

Let $w=P_1\left(g\left(x\right)\right)-\sigma F_1(T\left(x\right),C_{P_1,\sigma }^{M_1}\left(y\right)).$ From (9), we have

$$\begin{array}{ccc}\hfill g\left(x\right)& =& R_{P_1,\sigma }^{M_1}\left[w\right]\hfill \\ & \Rightarrow & g\left(x\right)={[P_1+\sigma M_1]}^{-1}\left(w\right)\hfill \\ & \Rightarrow & P_1\left(g\left(x\right)\right)+\sigma M_1\left(g\left(x\right)\right)\ni w.\hfill \end{array}$$

Substituting $w=P_1\left(g\left(x\right)\right)-\sigma F_1(T\left(x\right),C_{P_1,\sigma }^{M_1}\left(y\right)),$ we obtain

$$\begin{array}{c}\hfill P_1\left(g\left(x\right)\right)+\sigma M_1\left(g\left(x\right)\right)\ni P_1\left(g\left(x\right)\right)-\sigma F_1(T\left(x\right),C_{P_1,\sigma }^{M_1}\left(y\right)),\end{array}$$

which implies that

$$\begin{array}{c}\hfill 0\in F_1(T\left(x\right),C_{P_1,\sigma }^{M_1}\left(y\right))+M_1\left(g\left(x\right)\right).\end{array}$$

Conversely,

$$\begin{array}{ccc}\hfill 0& \in & F_1(T\left(x\right),C_{P_1,\sigma }^{M_1}\left(y\right))+M_1\left(g\left(x\right)\right)\hfill \\ & \Rightarrow & -\sigma F_1(T\left(x\right),C_{P_1,\sigma }^{M_1}\left(y\right))\in \sigma M_1\left(g\left(x\right)\right)\hfill \\ & \Rightarrow & (P_1\left(g\left(x\right)\right)-\sigma F_1(T\left(x\right),C_{P_1,\sigma }^{M_1}\left(y\right)))\in (P_1\left(g\left(x\right)\right)+\sigma M_1\left(g\left(x\right)\right))\hfill \\ & \Rightarrow & \left(P_1\left(g\left(x\right)\right)-\sigma F_1(T\left(x\right),C_{P_1,\sigma }^{M_1}\left(y\right))\right)\in [P_1+\sigma M_1]\left(g\left(x\right)\right)\hfill \\ & \Rightarrow & g\left(x\right)={[P_1+\sigma M_1]}^{-1}\left[P_1\left(g\left(x\right)\right)-\sigma F_1(T\left(x\right),C_{P_1,\sigma }^{M_1}\left(y\right))\right]\hfill \\ & \Rightarrow & g\left(x\right)=R_{P_1,\sigma }^{M_1}\left[P_1\left(g\left(y\right)\right)-\sigma F_1(T\left(x\right),C_{P_1,\sigma }^{M_1}\left(y\right))\right].\hfill \end{array}$$

In a similar way, we can show that

$$g\left(y\right)=R_{P_2,\tau }^{M_2}\left[P_2\left(g\left(y\right)\right)-\tau F_2(T\left(y\right),C_{P_2,\tau }^{M_2}\left(x\right))\right].$$

□

4. Main Result

In this section, we discuss the existence of solution of (7) and (8).

Theorem 1.

Consider the mappings $F_1,F_2:E\times E\to E$, where $F_1$ is ${\alpha }_1$-Lipschitz continuous in its first argument and ${\mu }_1$-Lipschitz continuous in its second argument, while $F_2$ is ${\alpha }_2$-Lipschitz continuous in its first argument and ${\mu }_2$-Lipschitz continuous in its second argument. Let $g,P_1,P_2:E\to E$ be single-valued mappings such that g is ${\lambda }_g$-Lipschitz continuous, and $P_1$ and $P_2$ are ${\lambda }_{P_1}$ and ${\lambda }_{P_2}$-Lipschitz continuous, respectively. Moreover, $P_1$ and $P_2$ are also r-strongly and s-strongly accretive, respectively. Suppose $M_1,M_2:E\to 2^E$ are multivalued mappings, and their associated resolvent operators $R_{P_1,\sigma }^{M_1}$ and $R_{P_2,\tau }^{M_2}$ are ${\displaystyle \frac{1}{r}}$- and ${\displaystyle \frac{1}{s}}$-Lipschitz continuous, respectively. Furthermore, let $T:E\to E$ be an α-averaged operator, and let the Cayley operators $C_{P_1,\sigma }^{M_1}$ and $C_{P_2,\tau }^{M_2}$ be ${\theta }_1$- and ${\theta }_2$-Lipschitz continuous, respectively. Assume that the following condition holds:

$$\left.\begin{array}{c}\hfill \sqrt[q]{1-qr+C_q{\lambda }_g^q}+{\displaystyle \frac{{\lambda }_{P_1}{\lambda }_g}{r}}+{\displaystyle \frac{\sigma {\alpha }_1{\gamma }_{\mathsf{\Theta }}}{r}}+{\displaystyle \frac{\tau {\mu }_2{\theta }_2}{s}}<1,\\ \hfill \sqrt[q]{1-qs+C_q{\lambda }_g^q}+{\displaystyle \frac{{\lambda }_{P_2}{\lambda }_g}{s}}+{\displaystyle \frac{\tau {\alpha }_2{\gamma }_{\mathsf{\Theta }}}{s}}+{\displaystyle \frac{\sigma {\mu }_1{\theta }_1}{r}}<1,\end{array}\right\}$$

(11)

where ${\theta }_1={\displaystyle \frac{2}{r}}+{\lambda }_{P_1}$ and ${\theta }_2={\displaystyle \frac{2}{s}}+{\lambda }_{P_2}$; then, the system of generalized variational inclusion problem involving the α-averaged operator and Cayley operator (7), (8) admits a unique solution.

Proof. 

For each $(x,y)\in E\times E$, we now define the mappings $Q_{\sigma },Q_{\tau }:E\times E\to E$ as

$$\begin{array}{c}\hfill Q_{\sigma }(x,y)=x-g\left(x\right)+R_{P_1,\sigma }^{M_1}\left[P_1\left(g\left(x\right)\right)-\sigma F_1(T\left(x\right),C_{P_1,\sigma }^{M_1}\left(y\right)\right],\end{array}$$

(12)

$$\begin{array}{c}\hfill Q_{\tau }(x,y)=y-g\left(y\right)+R_{P_2,\tau }^{M_2}\left[P_2\left(g\left(y\right)\right)-\tau F_2(T\left(y\right),C_{P_2,\tau }^{M_2}\left(x\right)\right].\end{array}$$

(13)

Using (12) and the Lipschitz continuity of g, $P_1$, $R_{P_1,\sigma }^{M_1}$, and $C_{P_1,\sigma }^{M_1}$, we have the following:

$$\begin{array}{cc}\hfill & \parallel Q_{\sigma }(x_1,y_1)-Q_{\sigma }(x_2,y_2)\parallel \hfill \\ \hfill =& ∥x_1-g\left(x_1\right)+R_{P_1,\sigma }^{M_1}\left[P_1\left(g\left(x_1\right)\right)-\sigma F_1(T\left(x_1\right),C_{P_1,\sigma }^{M_1}\left(y_1\right)\right]\hfill \\ \hfill & -\left(x_2-g\left(x_2\right)+R_{P_1,\sigma }^{M_1}\left[P_1\left(g\left(x_2\right)\right)-\sigma F_1(T\left(x_2\right),C_{P,\sigma }^{M_1}\left(y_2\right)\right]\right)∥\hfill \\ \hfill =& ∥x_1-x_2-\left(g\left(x_1\right)-g\left(x_2\right)\right)+\left(R_{P_1,\sigma }^{M_1}\left[P_1\left(g\left(x_1\right)\right)-\sigma F_1(T\left(x_1\right),C_{P_1,\sigma }^{M_1}\left(y_1\right)\right]\right)\hfill \\ \hfill & -\left(R_{P_1,\sigma }^{M_1}\left[P_1\left(g\left(x_2\right)\right)-\sigma F_1(T\left(x_2\right),C_{P_1,\sigma }^{M_1}\left(y_2\right)\right]\right)∥\hfill \\ \hfill \le & ∥x_1-x_2-(g\left(x_1\right)-g\left(x_2\right))∥+∥R_{P_1,\sigma }^{M_1}\left[P_1\left(g\left(x_1\right)\right)-\sigma F_1(T\left(x_1\right),C_{P_1,\sigma }^{M_1}\left(y_1\right)\right]\hfill \\ \hfill & -\left(R_{P_1,\sigma }^{M_1}\left[P_1\left(g\left(x_2\right)\right)-\sigma F_1(T\left(x_2\right),C_{P_1,\sigma }^{M_1}\left(y_2\right)\right]\right)∥.\hfill \end{array}$$

Using Lemma 1, and given that g is accretive and Lipschitz continuous, we have the following.

$$\begin{array}{ccc}\hfill {∥x_1-x_2-\left(g\left(x_1\right)-g\left(x_2\right)\right)∥}^q& \le & \parallel x_1-x_2{\parallel }^q-q\langle g\left(x_1\right)-g\left(x_2\right),{\mathcal{J}}_q(x_1-x_2)\rangle \hfill \\ \hfill & & +C_q{\parallel g\left(x_1\right)-g\left(x_2\right)\parallel }^q\hfill \\ \hfill & \le & \parallel x_1-x_2{\parallel }^q-qr\parallel x_1-x_2{\parallel }^q+C_q{\lambda }_g^q{\parallel x_1-x_2\parallel }^q\hfill \\ \hfill & \le & (1-qr+C_q{\lambda }_g^q){\parallel x_1-x_2\parallel }^q\hfill \\ \hfill & \le & \sqrt[q]{1-qr+C_q{\lambda }_g^q}\parallel x_1-x_2\parallel .\hfill \end{array}$$

(14)

Using (14), we have

$$\begin{array}{cc}\hfill & \parallel Q_{\sigma }(x_1,y_1)-Q_{\sigma }(x_2,y_2)\parallel \hfill \\ \hfill \le & \sqrt[q]{1-qr+C_q{\lambda }_g^q}\parallel x_1-x_2\parallel +{\displaystyle \frac{1}{r}}∥\left[P_1\left(g\left(x_1\right)\right)-\sigma F_1(T\left(x_1\right),C_{P_1,\sigma }^{M_1}\left(y_1\right))\right]\hfill \\ \hfill & -\left[P_1\left(g\left(x_2\right)\right)-\sigma F_1(T\left(x_2\right),C_{P_1,\sigma }^{M_1}\left(y_2\right))\right]∥\hfill \\ \hfill \le & \sqrt[q]{1-qr+C_q{\lambda }_g^q}\parallel x_1-x_2\parallel +{\displaystyle \frac{1}{r}}∥P_1\left(g\left(x_1\right)\right)-P_1\left(g\left(x_2\right)\right)∥\hfill \\ \hfill & +{\displaystyle \frac{\sigma }{r}}∥F_1(T\left(x_1\right),C_{P_1,\sigma }^{M_1}\left(y_1\right))-F_1(T\left(x_2\right),C_{P_1,\sigma }^{M_1}\left(y_2\right))∥\hfill \\ \hfill \le & \sqrt[q]{1-qr+C_q{\lambda }_g^q}\parallel x_1-x_2\parallel +{\displaystyle \frac{{\lambda }_{P_1}}{r}}\parallel g\left(x_1\right)-g\left(x_2\right)\parallel \hfill \\ \hfill & +{\displaystyle \frac{\sigma }{r}}∥F_1(T\left(x_1\right),C_{P_1,\sigma }^{M_1}\left(y_1\right))-F_1(T\left(x_1\right),C_{P_1,\sigma }^{M_1}\left(y_2\right))\hfill \\ \hfill & +F_1(T\left(x_1\right),C_{P_1,\sigma }^{M_1}\left(y_2\right))-F_1(T\left(x_2\right),C_{P_1,\sigma }^{M_1}\left(y_2\right))∥\hfill \\ \hfill \le & \sqrt[q]{1-qr+C_q{\lambda }_g^q}\parallel x_1-x_2\parallel +{\displaystyle \frac{{\lambda }_{P_1}{\lambda }_g}{r}}\parallel x_1-x_2\parallel +{\displaystyle \frac{\sigma }{r}}∥F_1(T\left(x_1\right),C_{P_1,\sigma }^{M_1}\left(y_1\right))\hfill \\ \hfill & -F_1(T\left(x_1\right),C_{P_1,\sigma }^{M_1}\left(y_2\right))+F_1(T\left(x_1\right),C_{P_1,\sigma }^{M_1}\left(y_2\right))-F_1(T\left(x_2\right),C_{P,\sigma }^{M_1}\left(y_2\right))∥\hfill \\ \hfill \le & \sqrt[q]{1-qr+C_q{\sigma }_g^q}\parallel x_1-x_2\parallel +{\displaystyle \frac{{\lambda }_{P_1}{\lambda }_g}{r}}\parallel x_1-x_2\parallel +{\displaystyle \frac{\sigma }{r}}∥F_1(T\left(x_1\right),C_{P_1,\sigma }^{M_1}\left(y_1\right))\hfill \\ \hfill & -F_1(T\left(x_1\right),C_{P_1,\sigma }^{M_1}\left(y_2\right))∥+{\displaystyle \frac{\sigma }{r}}∥F_1(T\left(x_1\right),C_{P_1,\sigma }^{M_1}\left(y_2\right))-F_1(T\left(x_2\right),C_{P_1,\sigma }^{M_1}\left(y_2\right))∥.\hfill \end{array}$$

Using the Lipschitz continuity of $F_1$ in the first and second arguments, we have the following:

$$\begin{array}{cc}\hfill \parallel Q_{\sigma }(x_1,y_1)-Q_{\sigma }(x_2,y_2)\parallel \le & \sqrt[q]{1-qr+C_q{\lambda }_g^q}∥x_1-x_2∥+{\displaystyle \frac{{\lambda }_{P_1}{\lambda }_g}{r}}∥x_1-x_2∥\hfill \\ \hfill & +{\displaystyle \frac{\lambda {\mu }_1}{r}}∥C_{P_1,\sigma }^{M_1}\left(y_1\right)-C_{P_1,\sigma }^{M_1}\left(y_2\right)∥+{\displaystyle \frac{\sigma {\alpha }_1}{r}}∥T\left(x_1\right)-T\left(x_2\right)∥\hfill \\ \hfill \le & \sqrt[q]{1-qr+C_q{\lambda }_g^q}∥x_1-x_2∥+{\displaystyle \frac{{\lambda }_{P_1}{\lambda }_g}{r}}∥x_1-x_2∥\hfill \\ \hfill & +{\displaystyle \frac{\sigma {\mu }_1{\theta }_1}{r}}∥y_1-y_2∥+{\displaystyle \frac{\sigma {\alpha }_1{\gamma }_{\mathsf{\Theta }}}{r}}∥x_1-x_2∥\hfill \\ \hfill \le & \left(\sqrt[q]{1-qr+C_q{\lambda }_g^q}+{\displaystyle \frac{{\lambda }_{P_1}{\lambda }_g}{r}}+{\displaystyle \frac{\sigma {\alpha }_1{\gamma }_{\mathsf{\Theta }}}{r}}\right)∥x_1-x_2∥\hfill \end{array}$$

(15)

$$\begin{array}{cc}\hfill & +{\displaystyle \frac{\sigma {\mu }_1{\theta }_1}{r}}∥y_1-y_2∥.\hfill \end{array}$$

(16)

Similarly, we obtain the following:

$$\begin{array}{cc}\hfill & \parallel Q_{\tau }(x_1,y_1)-Q_{\tau }(x_2,y_2)\parallel \hfill \\ & \le \left(\sqrt[q]{1-qs+C_q{\lambda }_g^q}+{\displaystyle \frac{{\lambda }_{P_2}{\lambda }_g}{s}}+{\displaystyle \frac{\tau {\alpha }_2{\gamma }_{\mathsf{\Theta }}}{s}}\right)\parallel y_1\parallel y_2\parallel +{\displaystyle \frac{\tau {\mu }_2{\theta }_2}{s}}\parallel x_1-x_2\parallel .\hfill \end{array}$$

(17)

Adding (15) and (17), we can deduce that

$$\begin{array}{cc}\hfill & \parallel Q_{\sigma }(x_1,y_1)-Q_{\sigma }(x_2,y_2)\parallel +\parallel Q_{\tau }(x_1,y_1)-Q_{\tau }(x_2,y_2)\parallel \hfill \\ \hfill \le & \left(\sqrt[q]{1-qr+C_q{\lambda }_g^q}+{\displaystyle \frac{{\lambda }_{P_1}{\lambda }_g}{r}}+{\displaystyle \frac{\sigma {\alpha }_1{\gamma }_{\mathsf{\Theta }}}{r}}\right)\parallel x_1-x_2\parallel +{\displaystyle \frac{\sigma {\mu }_1{\theta }_1}{r}}\parallel y_1-y_2\parallel \hfill \\ \hfill & +\left(\sqrt[q]{1-qs+C_q{\lambda }_g^q}+{\displaystyle \frac{{\lambda }_{P_2}{\lambda }_g}{s}}+{\displaystyle \frac{\tau {\alpha }_2{\gamma }_{\mathsf{\Theta }}}{s}}\right)\parallel y_1-y_2\parallel +{\displaystyle \frac{\tau {\mu }_2{\theta }_2}{s}}\parallel x_1-x_2\parallel \hfill \\ \hfill \le & \left(\sqrt[q]{1-qr+C_q{\lambda }_g^q}+{\displaystyle \frac{{\lambda }_{P_1}{\lambda }_g}{r}}+{\displaystyle \frac{\sigma {\alpha }_1{\gamma }_{\mathsf{\Theta }}}{r}}+{\displaystyle \frac{\tau {\mu }_2{\theta }_2}{s}}\right)\parallel x_1-x_2\parallel \hfill \\ \hfill & +\left(\sqrt[q]{1-qs+C_q{\lambda }_g^q}+{\displaystyle \frac{{\lambda }_{P_2}{\lambda }_g}{s}}+{\displaystyle \frac{\tau {\alpha }_2{\gamma }_{\mathsf{\Theta }}}{s}}+{\displaystyle \frac{\sigma {\mu }_1{\theta }_1}{r}}\right)\parallel y_1-y_2\parallel \hfill \end{array}$$

$$\parallel Q_{\sigma }(x_1,y_1)-Q_{\sigma }(x_2,y_2)\parallel +\parallel Q_{\tau }(x_1,y_1)-Q_{\tau }(x_2,y_2)\parallel \le \mathsf{\Theta }\left[\parallel x_1-x_2\parallel +\parallel y_1-y_2\parallel \right],$$

where

$$\begin{array}{c}\hfill \mathsf{\Theta }=max\left\{\sqrt[q]{1-qr+C_q{\lambda }_g^q}+{\displaystyle \frac{{\lambda }_{P_1}{\lambda }_g}{r}}+{\displaystyle \frac{\sigma {\alpha }_1{\gamma }_{\mathsf{\Theta }}}{r}}+{\displaystyle \frac{\tau {\mu }_2{\theta }_2}{s}},\right.\\ \hfill \left.\sqrt[q]{1-qs+C_q{\lambda }_g^q}+{\displaystyle \frac{{\lambda }_{P_2}{\lambda }_g}{s}}+{\displaystyle \frac{\tau {\alpha }_2{\gamma }_{\mathsf{\Theta }}}{s}}+{\displaystyle \frac{\sigma {\mu }_1{\theta }_1}{r}}\right\}.\end{array}$$

Since we know that $E\times E$ is a Banach space, we introduced $Q_{\sigma ,\tau }:E\times E\to E\times E$, and we can deduce that

$$Q_{\sigma ,\tau }(x,y)=(Q_{\sigma }(x,y),Q_{\tau }(x,y)),\forall (x,y)\in E\times E.$$

From Condition (11), we have $0<\mathsf{\Theta }<1$; therefore,

$$\parallel Q_{\sigma ,\tau }(x_1,y_1)-Q_{\sigma ,\tau }(x_2,y_2)\parallel \le \mathsf{\Theta }\parallel (x_1,y_1)-(x_2,x_2)\parallel .$$

(18)

From the above condition, it is clear that $Q_{\sigma ,\tau }$ is a contraction mapping. By applying the Banach contraction principle, it follows that there exists a unique solution $(x,y)\in E\times E$ such that

$$Q_{\sigma ,\tau }(x,y)=(x,y).$$

Therefore,

$$g\left(x\right)=R_{P_1,\sigma }^{M_1}\left[P_1\left(g\left(x\right)\right)-\sigma F_1(T\left(x\right),C_{P_1,\sigma }^{M_1}\left(y\right))\right],$$

$$g\left(y\right)=R_{P_2,\tau }^{M_2}\left[P_2\left(g\left(y\right)\right)-\tau F_2(T\left(y\right),C_{P_2,\tau }^{M_2}\left(x\right))\right].$$

Therefore, based on Lemma 1, $(x,y)$ is the unique solution of the system of the generalized variational inclusion problem involving the $\alpha$-averaged operator and Cayley operator.    □

5. Algorithm and Convergence Result

Theorem 2.

Let all the conditions of Theorem 1 be satisfied. Then, the sequences $\left\{x_{n+1}\right\}$ and $\left\{y_{n+1}\right\}$, generated by Algorithm 1, converge to x and y, respectively, where $(x,y)$ represents the unique solution of the generalized variational inclusion problem involving the α-averaged operator and the Cayley operator.

Algorithm 1: System of resolvent-based iterative method.

For the initial points $x_0,y_0\in E$, we compute the following schemes: $$\begin{array}{c}\hfill g\left(x_{n+1}\right)=(1-\rho )g\left(x_n\right)+\rho R_{P_1,\sigma }^{M_1}\left[P_1\left(g\left(x_n\right)\right)-\sigma F_1(T\left(x_n\right),C_{P_1,\sigma }^{M_1}\left(y_n\right)\right],\end{array}$$ (19) $$\begin{array}{c}\hfill g\left(y_{n+1}\right)=(1-\rho )g\left(y_n\right)+\rho R_{P_2,\tau }^{M_2}\left[P_2\left(g\left(y_n\right)\right)-\tau F_2(T\left(y_n\right),C_{P_2,\tau }^{M_2}\left(x_n\right)\right],\end{array}$$ (20) where $\tau ,\sigma >0$, $\rho \in [0,1]$ and $n=0,1,2\dots$, which is known as the system of resolvent-based iterative method.

Proof. 

According to Theorem 1, the system of the generalized variational inclusion problem involving the $\alpha$-averaged operator and Cayley operator admits a unique solution $(x,y)\in E\times E.$ Therefore, based on Lemma 1, we have the following:

$$\begin{array}{c}\hfill g\left(\tilde{x}\right)=(1-\rho )g\left(\tilde{x}\right)+\rho R_{P_1,\sigma }^{M_1}\left[P_1\left(g\left(\tilde{x}\right)\right)-\sigma F_1(T\left(\tilde{x}\right),C_{P_1,\sigma }^{M_1}\left(\tilde{y}\right))\right],\\ \hfill g\left(\tilde{y}\right)=(1-\rho )g\left(\tilde{y}\right)+\rho R_{P_2,\tau }^{M_2}\left[P_2\left(g\left(\tilde{y}\right)\right)-\tau F_2(T\left(\tilde{y}\right),C_{P_2,\tau }^{M_2}\left(\tilde{x}\right))\right].\end{array}$$

Now, using (19), Algorithm 1, and the Lipschitz continuity of $g,R_{P_1,\sigma }^{M_1},C_{P_1,\sigma }^{M_1}$ and $F_1,$ we have the following:

$$\begin{array}{cc}\hfill ∥g\left(x_{n+1}\right)-g\left(\tilde{x}\right)∥& =∥(1-\rho )g\left(x_n\right)+\rho R_{P_1,\sigma }^{M_1}\left[P_1\left(g\left(x_n\right)\right)-\sigma F_1(T\left(x_n\right),C_{P_1,\sigma }^{M_1}\left(y_n\right))\right]\hfill \\ \hfill & -\left\{(1-\rho )g\left(\tilde{x}\right)+\rho R_{P_1,\sigma }^{M_1}\left[P_1\left(g\left(\tilde{x}\right)\right)-\sigma F_1(T\left(\tilde{x}\right),C_{P_1,\sigma }^{M_1}\left(\tilde{y}\right))\right]\right\}∥\hfill \\ \hfill & \le (1-\rho )∥g\left(x_n\right)-g\left(\tilde{x}\right)∥+\rho ∥R_{P_1,\sigma }^{M_1}\left[P_1\left(g\left(x_n\right)\right)-\sigma F_1(T\left(x_n\right),C_{P_1,\sigma }^{M_1}\left(y_n\right))\right]\hfill \\ \hfill & -R_{P_1,\sigma }^{M_1}\left[P_1\left(g\left(\tilde{x}\right)\right)-\sigma F_1(T\left(\tilde{x}\right),C_{P_1,\sigma }^{M_1}\left(\tilde{y}\right))\right]∥\hfill \\ \hfill & \le {\lambda }_g(1-\rho )∥x_n-\tilde{x}∥+{\displaystyle \frac{\rho }{r}}∥P_1\left(g\left(x_n\right)\right)-\sigma F_1(T\left(x_n\right),C_{P_1,\sigma }^{M_1}\left(y_n\right))\hfill \\ \hfill & -\left[P_1\left(g\left(\tilde{x}\right)\right)-\sigma F_1(T\left(\tilde{x}\right),C_{P_1,\sigma }^{M_1}\left(\tilde{y}\right))\right]∥\hfill \\ \hfill & \le {\lambda }_g(1-\rho )∥x_n-\tilde{x}∥+{\displaystyle \frac{\rho }{r}}∥P_1\left(g\left(x_n\right)\right)-P_1\left(g\left(\tilde{x}\right)\right)∥\hfill \\ \hfill & +{\displaystyle \frac{\rho \sigma }{r}}∥F_1(T\left(x_n\right),C_{P_1,\sigma }^{M_1}\left(y_n\right))-F_1(T\left(\tilde{x}\right),C_{P_1,\sigma }^{M_1}\left(\tilde{y}\right))∥\hfill \\ \hfill & \le {\lambda }_g(1-\rho )∥x_n-\tilde{x}∥+{\displaystyle \frac{\rho {\lambda }_{P_1}{\lambda }_g}{r}}∥x_n-\tilde{x}∥\hfill \\ \hfill & +{\displaystyle \frac{\rho \sigma }{r}}∥F_1(T\left(x_n\right),C_{P_1,\sigma }^{M_1}\left(y_n\right))-F_1(T\left(x_n\right),C_{P_1,\sigma }^{M_1}\left(\tilde{y}\right))\hfill \\ \hfill & +F_1(T\left(x_n\right),C_{P_1,\sigma }^{M_1}\left(\tilde{y}\right))-F_1(T\left(\tilde{x}\right),C_{P_1,\sigma }^{M_1}\left(\tilde{y}\right))∥\hfill \\ \hfill & \le {\lambda }_g(1-\rho )∥x_n-\tilde{x}∥+{\displaystyle \frac{\rho {\lambda }_{P_1}{\lambda }_g}{r}}∥x_n-\tilde{x}∥\hfill \\ \hfill & +{\displaystyle \frac{\rho \sigma {\mu }_1}{r}}∥C_{P_1,\sigma }^{M_1}\left(y_n\right)-C_{P_1,\sigma }^{M_1}\left(\tilde{y}\right)∥+{\displaystyle \frac{\rho \sigma {\alpha }_1}{r}}∥T\left(x_n\right)-T\left(\tilde{x}\right)∥\hfill \\ \hfill & \le \left({\lambda }_g(1-\rho )+{\displaystyle \frac{\rho {\lambda }_{P_1}{\lambda }_g}{r}}\right)∥x_n-\tilde{x}∥+{\displaystyle \frac{\rho \sigma {\mu }_1{\theta }_1}{r}}∥y_n-\tilde{y}∥+{\displaystyle \frac{\rho \sigma {\alpha }_1{\gamma }_{\mathsf{\Theta }}}{r}}∥x_n-\tilde{x}∥\hfill \\ \hfill & \le \left({\lambda }_g(1-\rho )+{\displaystyle \frac{\rho {\lambda }_{P_1}{\lambda }_g}{r}}+{\displaystyle \frac{\rho \sigma {\alpha }_1{\gamma }_{\mathsf{\Theta }}}{r}}\right)∥x_n-\tilde{x}∥+{\displaystyle \frac{\rho \sigma {\mu }_1{\theta }_1}{r}}∥y_n-\tilde{y}∥.\hfill \end{array}$$

Using (20), Algorithm 1, and the Lipschitz continuity of $g,R_{P_2,\tau }^{M_2},C_{P_2,\tau }^{M_2}$ and $F_2$, we obtain the following:

$$\begin{array}{ccc}\hfill \parallel g\left(y_{n+1}\right)-g\left(\tilde{y}\right)\parallel & \le & \left({\lambda }_g(1-\rho )+{\displaystyle \frac{\rho {\lambda }_{P_2}{\lambda }_g}{s}}+{\displaystyle \frac{\rho \tau {\alpha }_2{\gamma }_{\mathsf{\Theta }}}{s}}\right)\parallel y_n-\tilde{y}\parallel \hfill \\ & & +{\displaystyle \frac{\rho \tau {\mu }_2{\theta }_2}{s}}\parallel x_n-\tilde{x}\parallel .\hfill \end{array}$$

(21)

Given that g is strongly accretive, characterized by the constant ${\delta }_g$, we have the following:

$$\begin{array}{ccc}\hfill \parallel g\left(y_{n+1}\right)-g\left(\tilde{y}\right)\parallel \parallel y_{n+1}-\tilde{y}{\parallel }^{q-1}& =& \parallel g\left(y_{n+1}\right)-g\left(\tilde{y}\right)\parallel {\mathcal{J}}_q(y_{n+1}-\tilde{y})\parallel \hfill \\ & \ge & \langle g\left(y_{n+1}\right)-g\left(\tilde{y}\right),{\mathcal{J}}_q(y_{n+1}-\tilde{y})\rangle \hfill \\ & \ge & {\delta }_g{\parallel y_{n+1}-\tilde{y}\parallel }^q.\hfill \end{array}$$

Therefore,

$$\parallel y_{n+1}-\tilde{y}\parallel \le {\displaystyle \frac{1}{{\delta }_g}}\parallel g\left(y_{n+1}\right)-g\left(\tilde{y}\right)\parallel .$$

(22)

Using a similar approach,

$$\parallel x_{n+1}-\tilde{x}\parallel \le {\displaystyle \frac{1}{{\delta }_g}}\parallel g\left(x_{n+1}\right)-g\left(\tilde{x}\right)\parallel .$$

(23)

Using (22) and (23), we have the following:

$$\begin{array}{c}\hfill \parallel x_{n+1}-\tilde{x}\parallel \le {\displaystyle \frac{1}{{\delta }_g}}\left[\left({\lambda }_g(1-\rho )+{\displaystyle \frac{\rho {\lambda }_{P_1}{\lambda }_g}{r}}+{\displaystyle \frac{\rho \sigma {\alpha }_1{\gamma }_{\mathsf{\Theta }}}{r}}\right)\parallel x_n-\tilde{x}\parallel +{\displaystyle \frac{\rho \sigma {\mu }_1{\theta }_1}{r}}\parallel y_n-\tilde{y}\parallel \right]\end{array}$$

(24)

$$\begin{array}{c}\hfill \parallel y_{n+1}-\tilde{y}\parallel \le {\displaystyle \frac{1}{{\delta }_g}}\left[\left({\lambda }_g(1-\rho )+{\displaystyle \frac{\rho {\lambda }_{P_2}{\lambda }_g}{s}}+{\displaystyle \frac{\rho \tau {\alpha }_2{\gamma }_{\mathsf{\Theta }}}{s}}\right)\parallel y_n-\tilde{y}\parallel +{\displaystyle \frac{\rho \tau {\mu }_2{\theta }_2}{s}}\parallel x_n-\tilde{x}\parallel \right]\end{array}$$

(25)

Adding (24) and (25), we have the following:

$$\begin{array}{cc}\hfill & \parallel x_{n+1}-\tilde{x}\parallel +\parallel y_{n+1}-\tilde{y}\parallel \hfill \\ \hfill \le & {\displaystyle \frac{1}{{\delta }_g}}\left[\left({\lambda }_g(1-\rho )+{\displaystyle \frac{\rho {\lambda }_{P_1}{\lambda }_g}{r}}+{\displaystyle \frac{\rho \sigma {\alpha }_1{\gamma }_{\mathsf{\Theta }}}{r}}\right)\parallel x_n-\tilde{x}\parallel +{\displaystyle \frac{\rho \sigma {\mu }_1{\theta }_1}{r}}\parallel y_n-\tilde{y}\parallel \right]\hfill \\ \hfill & +{\displaystyle \frac{1}{{\delta }_g}}\left[\left({\lambda }_g(1-\rho )+{\displaystyle \frac{\rho {\lambda }_{P_2}{\lambda }_g}{s}}+{\displaystyle \frac{\rho \tau {\alpha }_2{\gamma }_{\mathsf{\Theta }}}{s}}\right)\parallel y_n-\tilde{y}\parallel +{\displaystyle \frac{\rho \tau {\mu }_2{\theta }_2}{s}}\parallel x_n-\tilde{x}\parallel \right]\hfill \\ \hfill \le & {\displaystyle \frac{1}{{\delta }_g}}\left[\left({\lambda }_g(1-\rho )+{\displaystyle \frac{\rho {\lambda }_{P_1}{\lambda }_g}{r}}+{\displaystyle \frac{\rho \sigma {\alpha }_1{\gamma }_{\mathsf{\Theta }}}{r}}+{\displaystyle \frac{\rho \tau {\mu }_2{\theta }_2}{s}}\right)\right]\parallel x_n-\tilde{x}\parallel \hfill \\ \hfill & +{\displaystyle \frac{1}{{\delta }_g}}\left[\left({\lambda }_g(1-\rho )+{\displaystyle \frac{\rho {\lambda }_{P_2}{\lambda }_g}{s}}+{\displaystyle \frac{\rho \tau {\alpha }_2{\gamma }_{\mathsf{\Theta }}}{s}}+{\displaystyle \frac{\rho \sigma {\mu }_1{\theta }_1}{r}}\right)\right]\parallel y_n-\tilde{y}\parallel .\hfill \end{array}$$

Let us take the following:

$$\begin{array}{ccc}\hfill {\mathsf{\Theta }}^{\prime }& =& max\left\{{\displaystyle \frac{1}{{\delta }_g}}\left[{\lambda }_g(1-\rho )+{\displaystyle \frac{\rho {\lambda }_{P_1}{\lambda }_g}{r}}+{\displaystyle \frac{\rho \sigma {\alpha }_1{\gamma }_{\mathsf{\Theta }}}{r}}+{\displaystyle \frac{\rho \tau {\mu }_2{\theta }_2}{s}}\right],\right.\hfill \\ & & \left.{\displaystyle \frac{1}{{\delta }_g}}\left[{\lambda }_g(1-\rho )+{\displaystyle \frac{\rho {\lambda }_{P_2}{\lambda }_g}{s}}+{\displaystyle \frac{\rho \tau {\alpha }_2{\gamma }_{\mathsf{\Theta }}}{s}}+{\displaystyle \frac{\rho \sigma {\mu }_1{\theta }_1}{r}}\right]\right\}.\hfill \end{array}$$

Therefore,

$$\begin{array}{ccc}\hfill \parallel x_{n+1}-\tilde{x}\parallel +\parallel y_{n+1}\tilde{y}\parallel & \le & {\mathsf{\Theta }}^{\prime }\left[\parallel x_n-\tilde{x}\parallel +\parallel y_n-\tilde{y}\parallel \right].\hfill \end{array}$$

Since the product space $E\times E$ is complete and the iteration process satisfies a contraction condition with constant ${\mathsf{\Theta }}^{\prime }$, the Banach contraction mapping principle guarantees the existence and uniqueness of the fixed point $(x,y)$. By applying the contraction inequality repeatedly, we obtain the following:

$$\parallel x_{n+k}-\tilde{x}\parallel +\parallel y_{n+k}-\tilde{y}\parallel \le {\left({\mathsf{\Theta }}^{\prime }\right)}^k\left(\parallel x_n-\tilde{x}\parallel +\parallel y_n-\tilde{y}\parallel \right),\mathrm{for}\mathrm{all}\mathrm{integers}k>0.$$

Given that $0<{\mathsf{\Theta }}^{\prime }<1$, we have ${lim}_{k\to \infty }{\left({\mathsf{\Theta }}^{\prime }\right)}^k=0$.

Consequently,

$$\underset{k\to \infty }{lim}\left(\parallel x_{n+k}-\tilde{x}\parallel +\parallel y_{n+k}-\tilde{y}\parallel \right)=0.$$

Therefore, the sequence $(x_n,y_n)$ converges strongly to the unique solution $(x,y)$ in the space $E\times E$ as $n\to \infty$.    □

Theorem 3.

Suppose all the conditions of the Theorem 1 are satisfied. Then, the sequences $\left\{x_{n+1}\right\},\left\{y_{n+1}\right\}$ defined by the Algorithm 2 converge to x and y, respectively, where $(x,y)$ is the unique solution to the system of the generalized variational inclusion problem involving the α-averaged operator and Cayley operator.

Algorithm 2: Coupled resolvent-based iterative method.

For $x_0,y_0\in E$, we compute $\left\{x_{n+1}\right\}$,$\left\{y_{n+1}\right\}$ based on the following iterative schemes: $$\begin{array}{c}\hfill x_{n+1}=(1-\omega )x_n+\omega \left\{x_n-g\left(x_n\right)+R_{P_1,\sigma }^{M_1}\left[P_1\left(g\left(x_n\right)\right)-\sigma F_1(T\left(x_n\right),C_{P_1,\sigma }^{M_1}\left(y_n\right))\right]\right\},\end{array}$$ (26) $$\begin{array}{c}\hfill y_{n+1}=(1-\omega )y_n+\omega \left\{y_n-g\left(y_n\right)+R_{P_2,\sigma }^{M_2}\left[P_2\left(g\left(y_n\right)\right)-\tau F_2(T\left(y_n\right),C_{P_2,\tau }^{M_2}\left(x_n\right))\right]\right\},\end{array}$$ (27) where $\tau ,\sigma >0$, $\omega \in [0,1]$ and $n=0,1,2\dots$.

Proof. 

Applying Algorithm 2, we have the following:

$$\begin{array}{ccc}\hfill \parallel x_{n+1}-x_n\parallel & =& \parallel (1-\omega )x_n+\omega \left\{x_n-g\left(x_n\right)+R_{P_1,\sigma }^{M_1}\left[P_1\left(g\left(x_n\right)\right)-\sigma F_1(T\left(x_n\right),C_{P_1,\sigma }^{M_1}\left(y_n\right))\right]\right\}\hfill \\ & & -(1-\omega )x_{n-1}+\omega \left\{x_{n-1}-g\left(x_{n-1}\right)\right.\hfill \\ & & \left.+R_{P_1,\sigma }^{M_1}\left[P_1\left(g\left(x_{n-1}\right)\right)-\sigma F_1(T\left(x_{n-1}\right),C_{P_1,\sigma }^{M_1}\left(y_{n-1}\right))\right]\right\}\parallel \hfill \\ & \le & (1-\omega )\parallel x_n-x_{n-1}\parallel +\omega \parallel x_n-x_{n-1}-(g\left(x_n\right)-g\left(x_{n-1}\right)\parallel \hfill \\ & & +\omega ∥R_{P_1,\sigma }^{M_1}\left[P_1\left(g\left(x_n\right)\right)-\sigma F_1(T\left(x_n\right),C_{P_1,\sigma }^{M_1}\left(y_n\right))\right]\hfill \\ & & -R_{P_1,\sigma }^{M_1}\left[P_1\left(g\left(x_{n-1}\right)\right)-\sigma F_1(T\left(x_{n-1}\right),C_{P_1,\sigma }^{M_1}\left(y_{n-1}\right))\right]∥.\hfill \end{array}$$

Using (14), we have the following:

$$\begin{array}{cc}\hfill & \parallel x_{n+1}-x_n\parallel \hfill \\ \hfill \le & (1-\omega )\parallel x_n-x_{n-1}\parallel +\omega \sqrt[q]{1-qr+C_q{\lambda }_g^q}\parallel x_n-x_{n-1}\parallel \hfill \\ \hfill & +{\displaystyle \frac{\omega }{r}}\parallel P_1\left(g\left(x_n\right)\right)-P_1\left(g\left(x_{n-1}\right)\right)\parallel +{\displaystyle \frac{\sigma \omega }{r}}\parallel F_1(T\left(x_n\right),C_{P_1,\sigma }^{M_1}\left(y_n\right))\hfill \\ \hfill & -F_1(T\left(x_{n-1}\right),C_{P_1,\sigma }^{M_1}\left(y_{n-1}\right))\parallel \hfill \\ \hfill \le & (1-\omega )\parallel x_n-x_{n-1}\parallel +\omega \sqrt[q]{1-qr+C_q{\lambda }_g^q}\parallel x_n-x_{n-1}\parallel +{\displaystyle \frac{\omega {\lambda }_{P_1}{\lambda }_g}{r}}\parallel x_n-x_{n-1}\parallel \hfill \\ \hfill & +{\displaystyle \frac{\omega \sigma {\mu }_1{\theta }_1}{r}}\parallel y_n-y_{n-1}\parallel +{\displaystyle \frac{\omega \sigma {\alpha }_1{\gamma }_{\mathsf{\Theta }}}{r}}\parallel x_n-x_{n-1}\parallel \hfill \end{array}$$

(28)

$$\begin{array}{cc}\hfill \le & \left(1-\omega +\omega \sqrt[q]{1-qr+C_q{\lambda }_g^q}+{\displaystyle \frac{\omega {\lambda }_{P_1}{\lambda }_g}{r}}+{\displaystyle \frac{\omega \sigma {\alpha }_1{\gamma }_{\mathsf{\Theta }}}{r}}\right)\parallel x_n-x_{n-1}\parallel \hfill \end{array}$$

(29)

$$\begin{array}{cc}\hfill & +{\displaystyle \frac{\omega \sigma {\mu }_1{\theta }_1}{r}}\parallel y_n-y_{n-1}\parallel \hfill \end{array}$$

(30)

Using Algorithm (2) and using (4), (6) we have the following:

$$\begin{array}{ccc}\hfill \parallel y_{n+1}-y_n\parallel & \le & \left((1-\omega +\omega \sqrt[q]{1-qr+C_q{\lambda }_g^q}+{\displaystyle \frac{\omega {\lambda }_{P_2}{\lambda }_g}{s}}+{\displaystyle \frac{\omega \tau {\alpha }_2{\gamma }_{\mathsf{\Theta }}}{s}}\right)\parallel x_n-x_{n-1}\parallel \hfill \\ & & +{\displaystyle \frac{\omega \tau {\mu }_2{\theta }_2}{s}}\parallel y_n-y_{n-1}\parallel \hfill \end{array}$$

(31)

Adding (29) and (31), we have the following:

$$\begin{array}{cc}\hfill & \parallel x_{n+1}-x_n\parallel +\parallel y_{n+1}-y_n\parallel \hfill \\ \hfill \le & \left(1-\omega +\omega \sqrt[q]{1-qr+C_q{\lambda }_g^q}+{\displaystyle \frac{\omega {\lambda }_{P_1}{\lambda }_g}{r}}+{\displaystyle \frac{\omega \sigma {\alpha }_1{\gamma }_{\mathsf{\Theta }}}{r}}\right)\parallel x_n-x_{n-1}\parallel \hfill \\ \hfill & +{\displaystyle \frac{\omega \sigma {\mu }_1{\theta }_1}{r}}\parallel y_n-y_{n-1}\parallel \hfill \\ \hfill & +\left(1-\omega +\omega \sqrt[q]{1-qr+C_q{\lambda }_g^q}+{\displaystyle \frac{\omega {\lambda }_{P_2}{\lambda }_g}{s}}+{\displaystyle \frac{\omega \tau {\alpha }_2{\gamma }_{\mathsf{\Theta }}}{s}}\right)\parallel y_n-y_{n-1}\parallel \hfill \\ \hfill & +{\displaystyle \frac{\omega \tau {\mu }_2{\theta }_2}{s}}\parallel x_n-x_{n-1}\parallel \hfill \\ \hfill \le & \left(1-\omega +\omega \sqrt[q]{1-qr+C_q{\lambda }_g^q}+{\displaystyle \frac{\omega {\lambda }_{P_1}{\lambda }_g}{r}}+{\displaystyle \frac{\omega \sigma {\alpha }_1{\gamma }_{\mathsf{\Theta }}}{r}}+{\displaystyle \frac{\omega \tau {\mu }_2{\theta }_2}{s}}\right)\parallel x_n-x_{n-1}\parallel \hfill \\ \hfill & +\left(1-\omega +\omega \sqrt[q]{1-qr+C_q{\lambda }_g^q}+{\displaystyle \frac{\omega {\lambda }_{P_2}{\lambda }_g}{s}}+{\displaystyle \frac{\omega \tau {\alpha }_2{\gamma }_{\mathsf{\Theta }}}{s}}+{\displaystyle \frac{\omega \sigma {\mu }_1{\theta }_1}{r}}\right)\parallel y_n-y_{n-1}\parallel .\hfill \end{array}$$

Let us take the following:

$$\begin{array}{c}\hfill {\mathsf{\Phi }}^{\prime }=max\left\{1-\omega +\omega \sqrt[q]{1-qr+C_q{\lambda }_g^q}+{\displaystyle \frac{\omega {\lambda }_{P_1}{\lambda }_g}{r}}+{\displaystyle \frac{\omega \sigma {\alpha }_1{\gamma }_{\mathsf{\Theta }}}{r}}+{\displaystyle \frac{\omega \tau {\mu }_2{\theta }_2}{s}},\right.\\ \hfill \left.1-\omega +\omega \sqrt[q]{1-qr+C_q{\lambda }_g^q}+{\displaystyle \frac{\omega {\lambda }_{P_2}{\lambda }_g}{s}}+{\displaystyle \frac{\omega \tau {\alpha }_2{\gamma }_{\mathsf{\Theta }}}{s}}+{\displaystyle \frac{\omega \sigma {\mu }_1{\theta }_1}{r}}\right\}.\end{array}$$

Therefore,

$$\begin{array}{c}\hfill \parallel x_{n+1}-x_n\parallel +\parallel y_{n+1}-y_n\parallel \le {\mathsf{\Phi }}^{\prime }[\parallel x_n-x_{n-1}\parallel +\parallel y_n-y_{n-1}\parallel ].\end{array}$$

Since $0<{\mathsf{\Phi }}^{\prime }<1$, we can say that $\left\{x_n\right\},\left\{y_n\right\}$ are a Cauchy sequence; thus, $x_n\to x,y_n\to y$. Therefore, $(x_n,y_n)\to (x,y)\in E\times E$ as $n\to \infty .$ □

6. Numerical Result

To verify the applicability of the theorem, we present a numerical example implemented using MATLAB 2024a. The results are illustrated through a computational table and a corresponding convergence graph.

Example 1.

Suppose $E=\mathbb{R}$ is equipped with the usual inner product, and consider the multi-valued mappings $M_1,M_2:\mathbb{R}\to 2^{\mathbb{R}}$ defined by the following:

$$M_1\left(x\right)=\left\{{\displaystyle \frac{4x}{9}}\right\},M_2\left(x\right)=\left\{{\displaystyle \frac{8x}{9}}\right\}.$$

Additionally, let the functions $g,N,P_1,P_2:\mathbb{R}\to \mathbb{R}$ be given as follows:

$$g\left(x\right)={\displaystyle \frac{x}{5}},N\left(x\right)={\displaystyle \frac{-2x}{3}},P_1\left(x\right)={\displaystyle \frac{x}{2}}+1,P_2\left(x\right)={\displaystyle \frac{2x}{5}}+2.$$

Moreover, let $F_1,F_2:\mathbb{R}\times \mathbb{R}\to \mathbb{R}$ be functions defined by

$$F_1(x,y)={\displaystyle \frac{3x}{10}}+{\displaystyle \frac{y}{10}}and{F}_2(x,y)={\displaystyle \frac{x}{5}}+{\displaystyle \frac{2y}{5}}.$$

Now, $F_1(x,y)={\displaystyle \frac{3x}{10}}+{\displaystyle \frac{y}{10}}$. We calculate the Lipschitz constant in the first argument as follows:

$$\begin{array}{c}\hfill \parallel F_1(x_1,y_1)-F_1(x_2,y_1)\parallel =∥\left({\displaystyle \frac{3x_1}{10}}+{\displaystyle \frac{y_1}{10}}\right)-\left({\displaystyle \frac{3x_2}{10}}+{\displaystyle \frac{y_1}{10}}\right)∥\le {\displaystyle \frac{1}{4}}\parallel x_1-x_2\parallel .\end{array}$$

Therefore, ${\alpha }_1={\displaystyle \frac{1}{4}}$. In a similar way, we find the Lipschitz constant ${\alpha }_2={\displaystyle \frac{1}{7}},{\mu }_1={\displaystyle \frac{1}{11}},{\mu }_2={\displaystyle \frac{2}{7}}$. It is easy to verify that g is Lipschitz continuous with the constant ${\lambda }_g={\displaystyle \frac{1}{9}}$ and N is $-{\displaystyle \frac{1}{3}}$ relaxed Lipschitz.Assume $T:\mathbb{R}\to \mathbb{R}$ is a $(1-{\displaystyle \frac{5\alpha }{3}})$-averaged operator, and we calculate it as follows:

$$\begin{array}{ccc}\hfill T\left(x\right)=(1-\alpha )I\left(x\right)+\alpha N\left(x\right)& =& \left(1-{\displaystyle \frac{5\alpha }{3}}\right)x.\hfill \end{array}$$

Sippose $P_1,P_2:\mathbb{R}\to \mathbb{R}$ are defined by

$$P_1\left(x\right)={\displaystyle \frac{x}{2}}+1,P_2\left(x\right)={\displaystyle \frac{2x}{5}}+2,$$

we have

$$\begin{array}{ccc}\hfill \parallel P_1\left(x\right)-P_2\left(x\right)\parallel & =& ∥\left({\displaystyle \frac{x}{2}}+1\right)-\left({\displaystyle \frac{y}{2}}+1\right)∥={\displaystyle \frac{1}{2}}\parallel x-y\parallel \le {\displaystyle \frac{2}{7}}\parallel x-y\parallel ;\hfill \end{array}$$

that is, the Lipschitz constant ${\lambda }_{P_1}={\displaystyle \frac{2}{7}}$, and similarly, the Lipschitz constant ${\lambda }_{P_2}={\displaystyle \frac{3}{8}}$. Moreover,

$$\begin{array}{ccc}\hfill \langle P_1\left(x\right)-P_1\left(y\right),x-y\rangle & =& 〈\left({\displaystyle \frac{x}{2}}+1\right)-\left({\displaystyle \frac{y}{2}}+1\right),x-y〉\hfill \\ & =& {\displaystyle \frac{1}{2}}\parallel x-y\parallel \ge {\displaystyle \frac{2}{5}}\parallel x-y\parallel ;\hfill \end{array}$$

that is, $P_1$ is strongly accretive, and we have $r={\displaystyle \frac{2}{5}}$. Similarly, $P_2$ is strongly accretive, such that $s={\displaystyle \frac{3}{10}}$.

Now, we will calculate the values of Resolvent operators and Cayley operators for $\sigma =0.05.$

For $\sigma =0.05$, we obtain

$$R_{P_1,\sigma }^{M_1}\left(x\right)={\displaystyle \frac{90}{47}}(x-1),and{C}_{P_1,\sigma }^{M_1}\left(x\right)=2R_{P_1,\sigma }^{M_1}\left(x\right)-P_1\left(x\right)={\displaystyle \frac{313}{94}}x-{\displaystyle \frac{227}{47}}.$$

Moreover, the Lipschitz constants of the Resolvent operator $R_{P_2,\tau }^{M_2}$ and the Cayley operator $C_{P_2,\tau }^{M_2}$ are as follows:

$$\begin{array}{c}\hfill ∥R_{P_1,\sigma }^{M_1}\left(x\right)-R_{P_1,\sigma }^{M_1}\left(y\right)∥=∥{\displaystyle \frac{90}{47}}(x-1)-{\displaystyle \frac{90}{47}}(y-1)∥={\displaystyle \frac{90}{47}}\parallel x-y\parallel \le {\displaystyle \frac{85}{51}}\parallel x-y\parallel ,\end{array}$$

and,

$$\begin{array}{c}\hfill ∥C_{P_1,\sigma }^{M_1}\left(x\right)-C_{P_1,\sigma }^{M_1}\left(y\right)∥=∥{\displaystyle \frac{313}{94}}x-{\displaystyle \frac{227}{47}}-\left({\displaystyle \frac{313}{94}}y-{\displaystyle \frac{227}{47}}\right)∥\le {\displaystyle \frac{290}{101}}\parallel x-y\parallel .\end{array}$$

Similarly, for $\tau =0.05$, we calculate the Resolvent operator $R_{P_2,\tau }^{M_2}$ and Cayley operator $C_{P_2,\tau }^{M_2}$ given by

$$R_{P_2,\tau }^{M_2}\left(x\right)={\displaystyle \frac{45}{20}}\left(x-2\right)and{C}_{P_2,\lambda }^{M_2}\left(x\right)={\displaystyle \frac{41}{10}}x-11.$$

Also,

$$\begin{array}{ccc}\hfill ∥R_{P_2,\tau }^{M_2}\left(x\right)-R_{P_2,\tau }^{M_2}\left(y\right)∥& \le & {\displaystyle \frac{35}{14}}\parallel x-y\parallel ,\hfill \\ \hfill ∥C_{P_2,\tau }^{M_2}\left(x\right)-C_{P_2,\tau }^{M_2}\left(y\right)∥& \le & {\displaystyle \frac{38}{15}}\parallel x-y\parallel .\hfill \end{array}$$

7. Convergences Graphs and Tables

This section presents numerical results analyzing the convergence of the proposed algorithms. Detailed tables compare iteration counts and computational outcomes for different initial values, while graphical visualizations illustrate the convergence behavior.

Table 1. Computational results for various initial values $(x_0,y_0)\mathrm{for}\alpha =0.20\mathrm{and}\rho =0.30$.

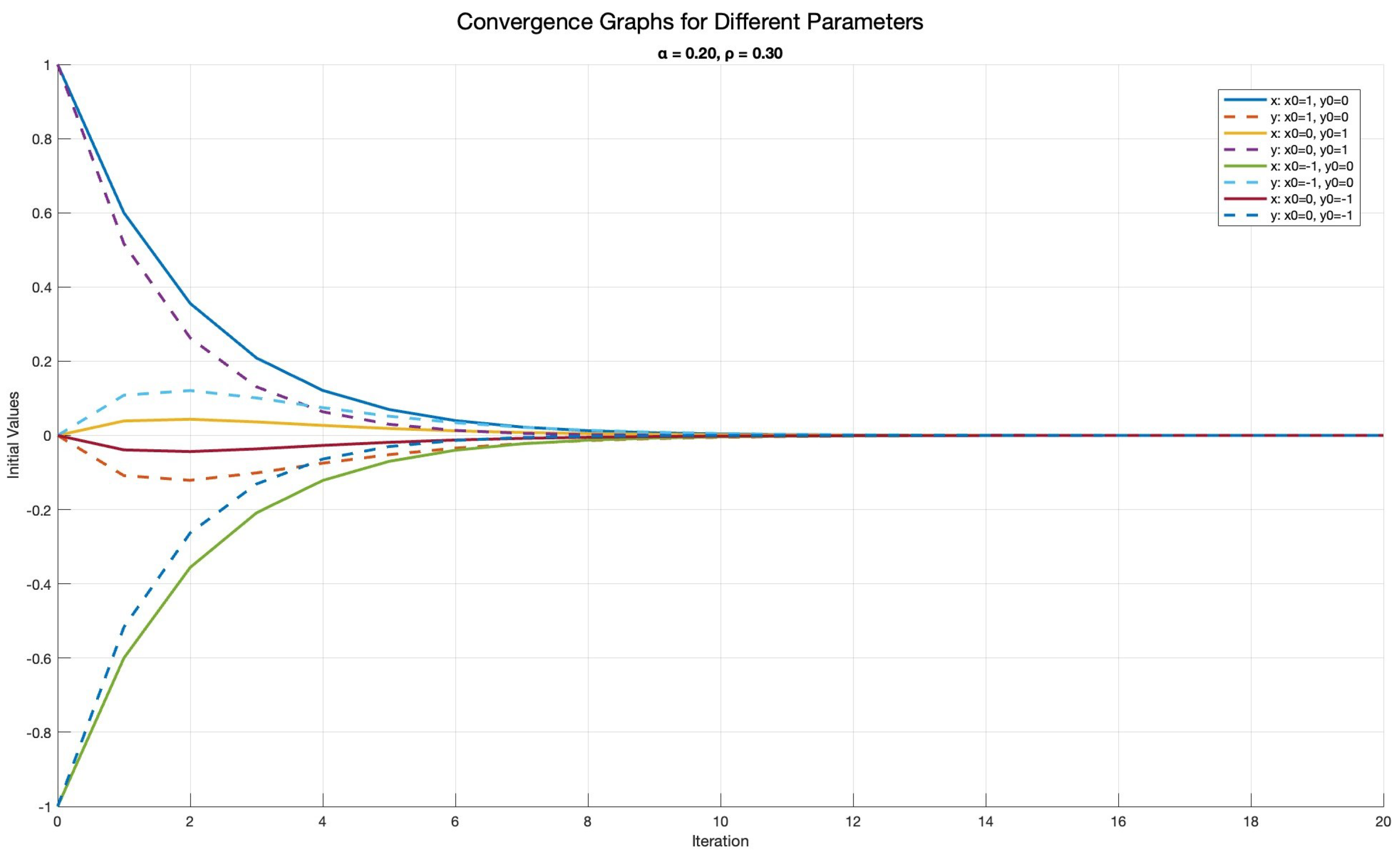
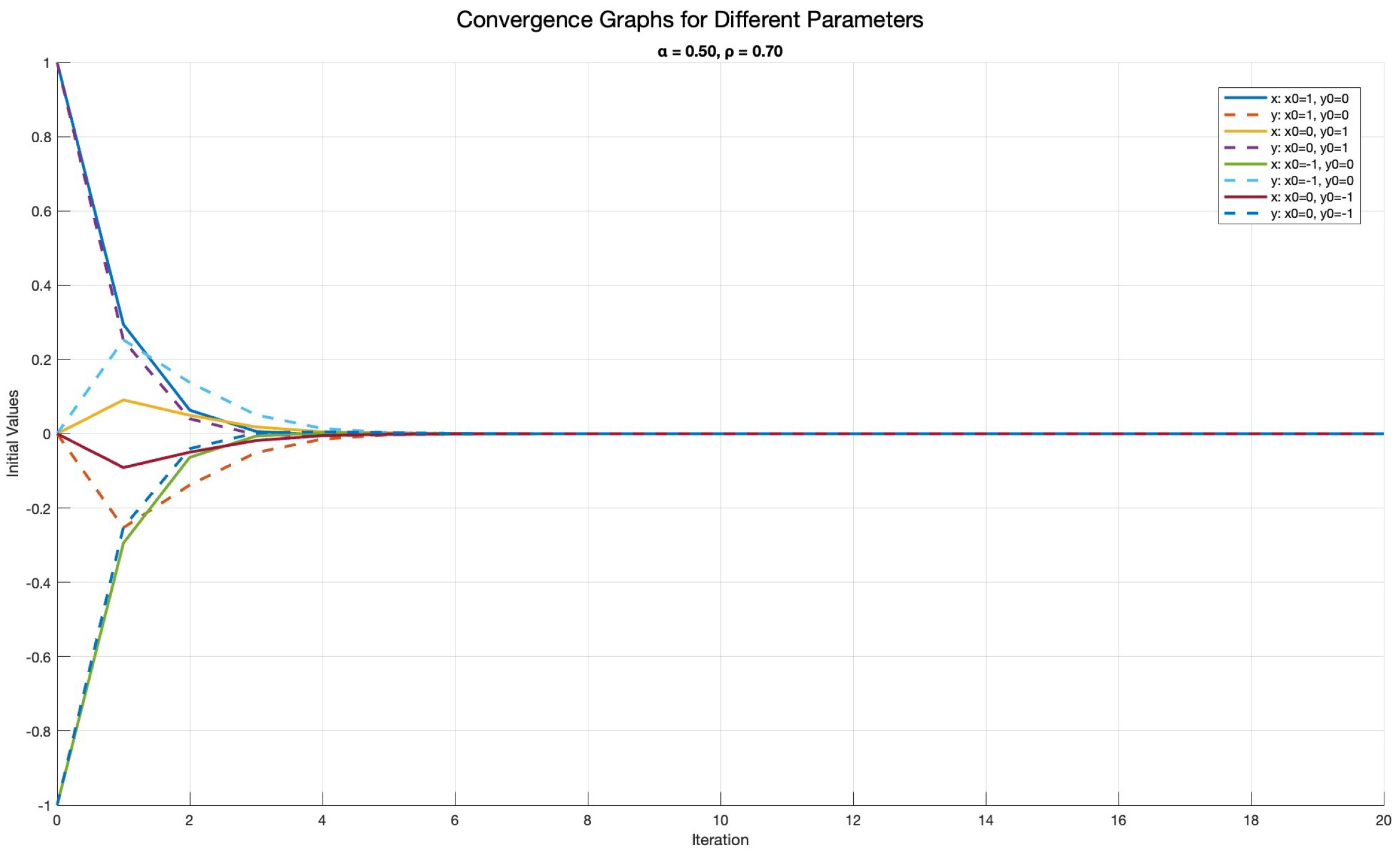
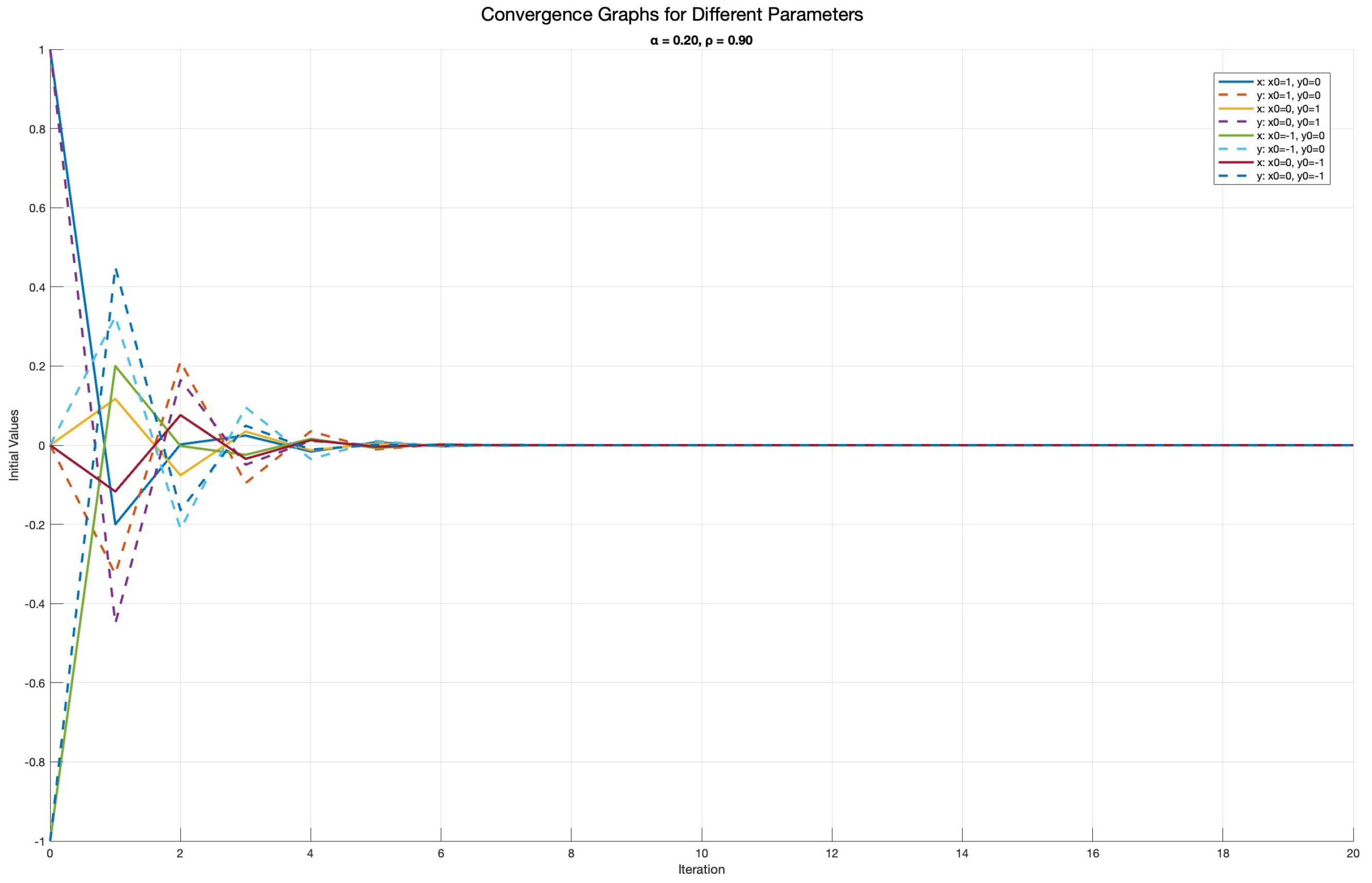
Iteration	Initial ${\mathit{x}}_0$	Initial ${\mathit{y}}_0$	$\mathit{\alpha }$	$\mathit{\rho }$	${\mathit{x}}_{\mathit{n}}$	${\mathit{y}}_{\mathit{n}}$
1	1	0	0.20	0.30	0.6000	−0.1083
5	1	0	0.20	0.30	0.0699	−0.0518
13	1	0	0.20	0.30	6.2912 $\times {10}^{-04}$	−0.0011
1	0	1	0.20	0.30	0.0390	0.5167
5	0	1	0.20	0.30	0.0186	0.0300
12	0	1	0.20	0.30	6.5987 $\times {10}^{-04}$	−2.4232 $\times {10}^{-04}$
1	−1	0	0.20	0.30	−0.6000	0.1083
5	−1	0	0.20	0.30	−0.0699	0.0518
13	−1	0	0.20	0.30	−6.2912 $\times {10}^{-04}$	0.0011
1	0	−1	0.20	0.30	−0.0390	−0.5167
5	0	−1	0.20	0.30	−0.0186	−0.0300
13	0	−1	0.20	0.30	−3.8647 $\times {10}^{-04}$	1.9668 $\times {10}^{-04}$

,

Table 2. Computational results for various initial values $(x_0,y_0)\mathrm{for}\alpha =0.50\mathrm{and}\rho =0.70$.

Iteration	Initial ${\mathit{x}}_0$	Initial ${\mathit{y}}_0$	$\mathit{\alpha }$	$\mathit{\rho }$	${\mathit{x}}_{\mathit{n}}$	${\mathit{y}}_{\mathit{n}}$
1	1	0	0.50	0.70	0.2942	−0.2528
3	1	0	0.50	0.70	0.0061	−0.0507
6	1	0	0.50	0.70	−8.8867 $\times {10}^{-04}$	−1.8741 $\times {10}^{-04}$
1	0	1	0.50	0.70	0.0910	0.2514
3	0	1	0.50	0.70	0.0183	−0.0024
6	0	1	0.50	0.70	6.7466 $\times {10}^{-05}$	−9.2038 $\times {10}^{-04}$
1	−1	0	0.50	0.70	−0.2942	0.2528
3	−1	0	0.50	0.70	−0.0061	0.0507
6	−1	0	0.50	0.70	8.8867 $\times {10}^{-04}$	1.8741 $\times {10}^{-04}$
1	0	−1	0.50	0.70	−0.0910	−0.2514
3	0	−1	0.50	0.70	−0.0183	0.0024
7	0	−1	0.50	0.70	6.3908 $\times {10}^{-05}$	2.4843 $\times {10}^{-04}$

,

Table 3. Computational results for various initial values $(x_0,y_0)\mathrm{for}\alpha =0.70\mathrm{and}\rho =0.90$.

Iteration	Initial ${\mathit{x}}_0$	Initial ${\mathit{y}}_0$	$\mathit{\alpha }$	$\mathit{\rho }$	${\mathit{x}}_{\mathit{n}}$	${\mathit{y}}_{\mathit{n}}$
1	1	0	0.70	0.90	0.2875	−0.3250
4	1	0	0.70	0.90	−0.0141	−0.0292
8	1	0	0.70	0.90	−1.0794 $\times {10}^{-04}$	6.2448 $\times {10}^{-04}$
1	0	1	0.70	0.90	0.1170	0.3625
4	0	1	0.70	0.90	0.0105	−0.0073
8	0	1	0.70	0.90	−2.2481 $\times {10}^{-04}$	−2.5205 $\times {10}^{-04}$
1	−1	0	0.70	0.90	−0.2875	0.3250
4	−1	0	0.70	0.90	0.0141	0.0292
7	−1	0	0.70	0.90	7.8873 $\times {10}^{-04}$	−0.0010
1	0	−1	0.70	0.90	−0.1170	−0.3625
4	0	−1	0.70	0.90	−0.0105	0.0073
7	0	−1	0.70	0.90	3.6560 $\times {10}^{-04}$	0.0010

and

Table 4. Computational results for various initial values $(x_0,y_0)\mathrm{for}\alpha =0.20\mathrm{and}\rho =0.90$.

Iteration	Initial ${\mathit{x}}_0$	Initial ${\mathit{y}}_0$	$\mathit{\alpha }$	$\mathit{\rho }$	${\mathit{x}}_{\mathit{n}}$	${\mathit{y}}_{\mathit{n}}$
1	1	0	0.20	0.90	−0.2000	−0.3250
4	1	0	0.20	0.90	−0.0161	0.0352
7	1	0	0.20	0.90	8.2084 $\times {10}^{-04}$	−1.9626 $\times {10}^{-04}$
1	0	1	0.20	0.90	0.1170	−0.4500
4	0	1	0.20	0.90	−0.0127	0.0110
6	0	1	0.20	0.90	−8.6052 $\times {10}^{-04}$	−8.6711 $\times {10}^{-04}$
1	−1	0	0.20	0.90	0.2000	0.3250
4	−1	0	0.20	0.90	0.0161	−0.0352
7	−1	0	0.20	0.90	−8.2084 $\times {10}^{-04}$	1.9626 $\times {10}^{-04}$
1	0	−1	0.20	0.90	−0.1170	0.4500
4	0	−1	0.20	0.90	0.0127	−0.0110
6	0	−1	0.20	0.90	8.6052 $\times {10}^{-04}$	8.6711 $\times {10}^{-04}$

, along with their corresponding Figure 1, Figure 2, Figure 3 and Figure 4, display the computational results for various initial values $(x_0,y_0)$ under fixed parameters $\alpha$ and $\rho$ respectively. In all cases, the values of $x_n$ and $y_n$ tend toward zero as the number of iterations increases, regardless of the initial conditions. This consistent convergence behaviour, also evident in the accompanying graphs, highlights the stability and effectiveness of the iterative method under the chosen parameters.

8. Conclusions

This work introduced and studied a unified framework for solving generalized variational inclusion problems in q-uniformly smooth Banach spaces. By exploiting the properties of both $\alpha$-averaged and Cayley operators, the proposed variational inclusion problems are reformulated as fixed-point problems, offering a unified perspective that enhances both theoretical understanding and computational feasibility. The developed algorithm, supported by a detailed convergence analysis, proves to be practically effective across a wide range of variational inclusion scenarios. Furthermore, a numerical result is provided to support our main result and validate the proposed algorithm using MATLAB programming, demonstrating the rapid convergence of the mathematical model and its effectiveness in achieving optimal solutions. These results emphasize the strong synergy between averaged and Cayley operator theory, laying the groundwork for the development of more advanced models with promising applications in fields such as optimization, control theory, and related areas. Future investigations may explore further generalizations of such problems, refined convergence schemes, and parallel or distributed algorithms tailored to large-scale systems.