Classification of the Second Minimal Orbits in the Sharkovski Ordering
Abstract
1. Introduction
2. Main Results
- 1.
- is mapped to ; and is mapped to except for one point; or
- 2.
- is mapped to ; and is mapped to except for one point.
3. Preliminary Results
- 1.
- The digraph contains a loop: such that .
- 2.
- , and such that ; moreover, it is always possible to choose unless m is even and , and it is always possible to choose unless .
- 3.
- If , , then and such that .
- 4.
- The digraph of a cycle with period contains a subgraph for any .
4. Proofs of Theorems 3 and 4
- Case :
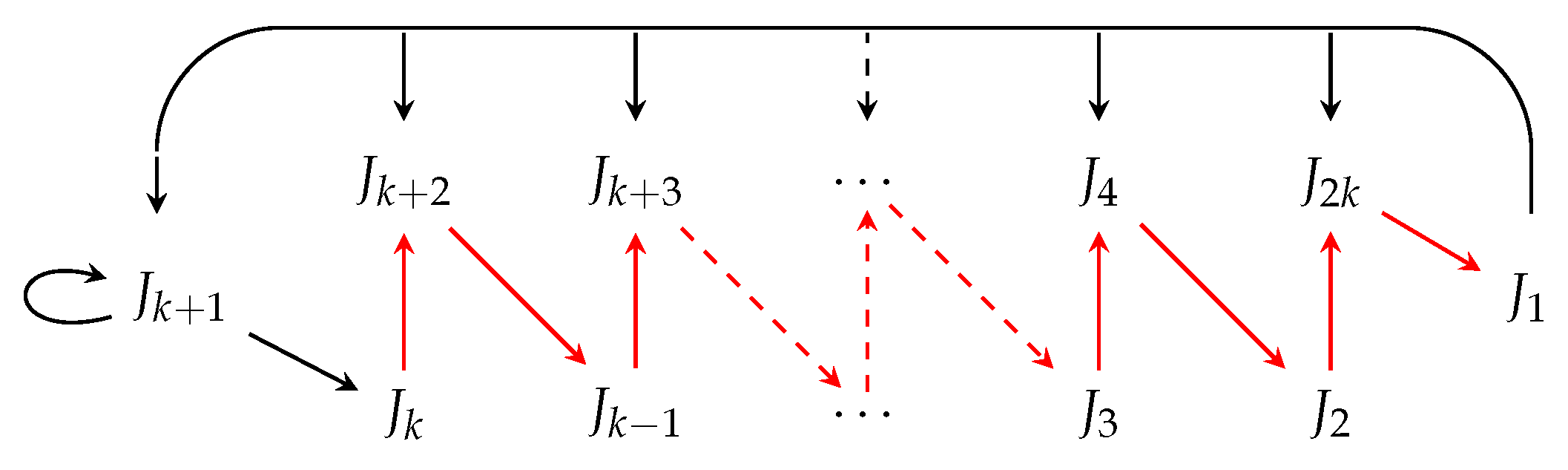
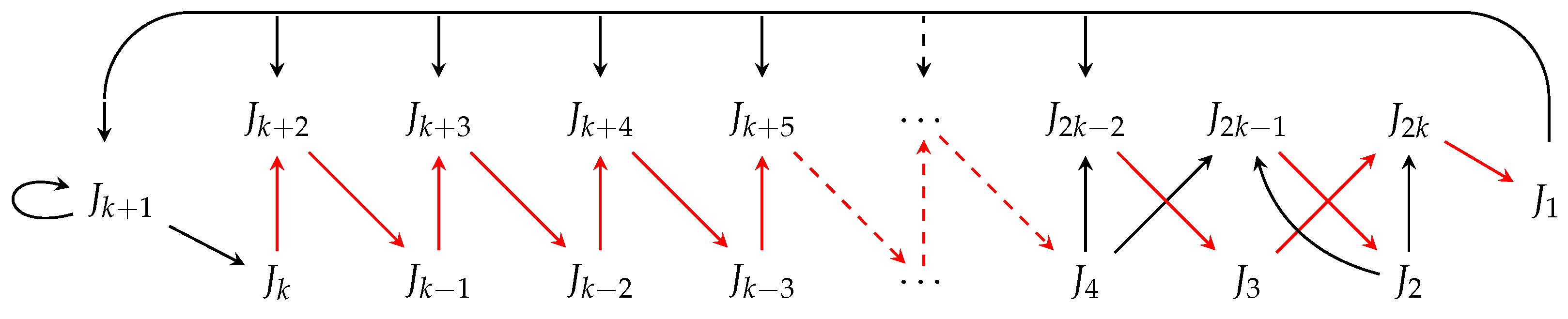
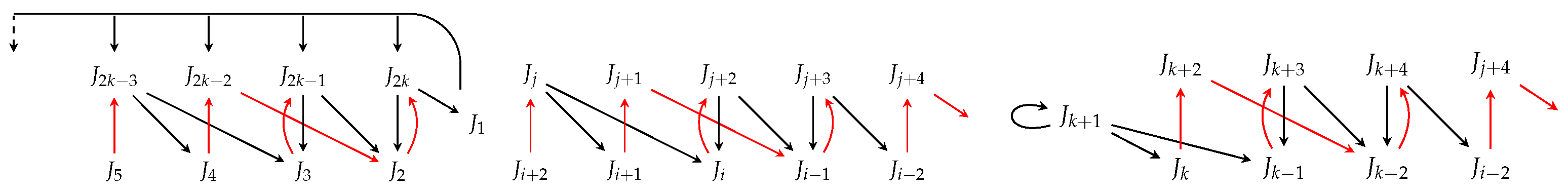
- Case : ; This produces a simple positive type -orbit given in (10) and Figure 4 with topological structure max-min-max. Next, we analyze the digraph to show that there are no primitive cycles of even length , which would imply by Straffin’s lemma, an existence of odd periodic orbit of length . From Lemma 3, it then follows that the P-linearization of the orbit (10) presents an example of a continuous map with a second minimal -orbit. We split the analysis into two cases:
- (a)
- Consider primitive cycles that contain . Without any loss of generality, choose as the starting vertex. First, assume that the cycle does not start with chain . Since any such cycle can be formed only by adding on to the starting vertex pairs . Therefore, the length of the cycle (by counting twice) will always be an odd number. Conversely, if the cycle starts with chain , then to close it at , the smallest required even length is .
- (b)
- Consider primitive cycles that do not contain . Obviously, such a cycle does not contain or since these vertices have red edges connecting them all the way to . Additionally, this cycle cannot contain or since is the only vertex (besides itself) with a directed edge to , and is the only vertex with a directed edge to . This leaves 4 vertices: . Since , and , , any cycle formed by these four vertices will consist of a starting vertex followed (or ending vertex preceded) by pairs , added arbitrarily many times, and hence no cycles of even length can be produced.
- Case : , then we have the period 4-suborbit, a contradiction.
- Case : .
- Case : , then we have the period 2-suborbit , a contradiction.
- Case : ; This produces a simple positive type -orbit given in (11) and Figure 5 with topological structure max-min-max. We repeat the argument from Case . First, we analyze the digraph to show that there are no primitive cycles of even length . We split the analysis into two cases:
- (a)
- Consider primitive cycles that contain . Without any loss of generality, choose as a starting vertex. First, assume that the cycle starts with the edge , with j taking any value between and . Since any such cycle can be formed only by adding to starting vertex pairs . Therefore, the length of the cycle (by counting twice) will be always an odd number. If the cycle starts with the edge , then the only difference from the previous case will be the addition of the pairs and/or arbitrarily many times. Hence, only cycles of odd length will be produced. Conversely, if the cycle starts with the chain or , then to close it at , the smallest required even length is .
- (b)
- Consider a primitive cycle that does not contain . Obviously, such a cycle does not contain or since these vertices have red edges connecting them all the way to . Additionally, this cycle cannot contain since is the only vertex (besides itself) with a directed edge to . This leaves 3 vertices: connected as . Therefore, this triple can only produce cycles when pairs and are added to a starting vertex. Therefore, no cycle of even length can be produced.
- 1.
- position when ,
- 2.
- positions or for ,
- 3.
- and position when .
- is inserted arbitrarily to the left of .
- is inserted arbitrarily to the right of .
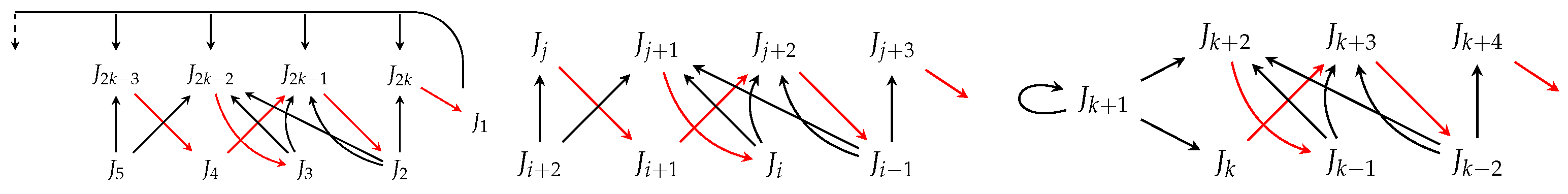
- Case (1): Choosing ; this leads to a valid second minimal orbit with the topological structure min-max-min and the associated digraph is presented in Figure 11 and the cyclic permutation is listed in (14). Next, we analyze the digraph to show that there are no primitive cycles of even length , which would imply by Straffin’s lemma an existence of odd periodic orbit of length . From Lemm 3, it then follows that the P-linearization of the orbit (14) presents an example of a continuous map with a second minimal -orbit.
- (a)
- Consider primitive cycles that contain . Without any loss of generality, choose as the starting vertex. First, assume that the cycle does not contain . Since , any such cycle can be formed only by adding to starting vertex pairs , . Therefore, the length of the cycle (by counting twice) will be always an odd number. Conversely, if the cycle contains , then to close it at , the smallest required even length is .
- (b)
- Consider primitive cycles that do not contain , but contain . Without any loss of generality, choose as the starting vertex. First, assume that the cycle does not contain . Since any such cycle can be formed only by adding to starting vertex pairs . Therefore, the length of the cycle (by counting twice) will always be an odd number. Conversely, if the cycle contains , then to close it at , the smallest required even length is . Finally, it is easy to see that by excluding and from the cycle, due to the red edges, all the vertices but must also be excluded, and cycle at is the only possibility.
- Case : ; this leads to a valid second minimal orbit with the topological structure min-max and the associated digraph is presented in Figure 12 and the cyclic permutation is listed in (15). Next, we prove as in previous case that there are no primitive cycles of even length , and, therefore, according to Lemms 3, P-linearization of the orbit (14) presents an example of a continuous map with a second minimal -orbit.
- (a)
- Consider primitive cycles that contain . Without any loss of generality, choose as the starting vertex. First, assume that the cycle does not contain . Since any such cycle can be formed only by adding to starting vertex pairs . Therefore, the length of the cycle (by counting twice) will be always an odd number. Conversely, if the cycle contains , then to close it at , the smallest required even length is .
- (b)
- Consider primitive cycles that do not contain , but contain . Without any loss of generality, choose as the starting vertex. First, assume that the cycle does not contain . Since any such cycle can be formed only by adding to starting vertex pairs . Therefore, the length of the cycle (by counting twice) will be always an odd number. Conversely, if the cycle contains , then to close it at , the smallest required even length is . Finally, it is easy to see that by excluding and from the cycle, due to the red edges, all the vertices but must be also excluded, and cycle at is the only possibility.
- Case : . The produced cyclic permutation contains the subgraph . According to Straffin’s lemma this subgraph implies the existence of a period 3-orbit, which is a contradiction.
- If -suborbit , a contradiction.
- If
- (a)
- If -suborbit , a contradiction.
- (b)
- If , but we have a second minimal orbit given in (14) with topological structure min-max-min, observe that this is the same as (14) from setting , and so the settings share a cyclic permutation. This is expected since to move from setting to , only the location of is changed and so, in this particular case, the digraph remains unchanged as we simply swap the intervals and .
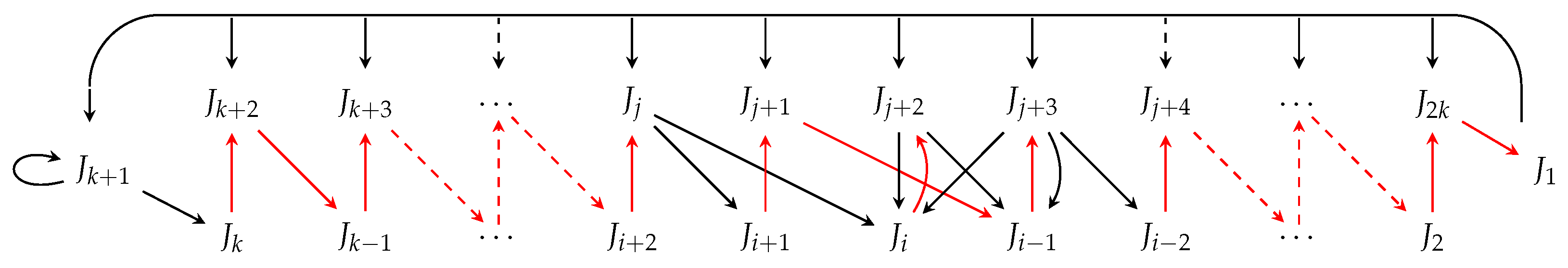
- This leads to a valid second minimal orbit with the topological structure max-min and the associated digraph is presented in Figure 14 and the cyclic permutation is listed in (18). Next, we prove that there are no primitive cycles of even length , and therefore according to Lemma 3, P-linearization of the orbit (18) presents an example of a continuous map with a second minimal -orbit.
- (a)
- Consider primitive cycles that contain . Without any loss of generality, choose as the starting vertex. First, assume that the cycle does not contain . Since and , any such cycle can be formed only by adding to starting vertex pairs , . Therefore, the length of the cycle (by counting twice) will be always an odd number. Conversely, if the cycle contains , then to close it at , the smallest required even length is .
- (b)
- Consider primitive cycles that do not contain . It is easy to see that by excluding from the cycle, due to the red edges, all the vertices but must also be excluded, and the cycle at is the only possibility.
- . This produces a second minimal orbit with topological structure max-min and the associated digraph is presented in Figure 15 and the cyclic permutation is listed in (19). As in previous cases, we prove that there are no primitive cycles of even length , and therefore according to Lemma 3, P-linearization of the orbit (19) presents an example of a continuous map with a second minimal -orbit.
- (a)
- Consider primitive cycles that contain . Without any loss of generality, choose as the starting vertex. First, assume that the cycle does not contain . Since (if ) and , , , , any such cycle can be formed only by adding to starting vertex pairs , , , Therefore, the length of the cycle (by counting twice) will always be an odd number. Conversely, if the cycle contains , then to close it at the smallest required even length is .
- (b)
- Consider primitive cycles that do not contain . It is easy to see that by excluding from the cycle, due to the red edges, all the vertices but must be also excluded, and a cycle at and a cycle formed by and are the only possibilities.
- If
- (a)
- If -suborbit , a contradiction.
- (b)
- If , we have a second minimal orbit given in (15) with topological structure min-max, shared with setting .
- (c)
- If -suborbit , a contradiction.
- If
- (a)
- If and -suborbit , a contradiction.
- (b)
- If and , then for , we have the primitive subgraphLemma 2 implies the existence of a -periodic orbit, which is a contradiction. For , we have the subgraphwhich leads to a 3-orbit, a contradiction.
- (c)
- If -suborbit , a contradiction.
- (d)
- If , then for , we have the subgraphLemma 2 implies the existence of a -periodic orbit, which is a contradiction. For , we have the subgraphwhich leads to a 3-orbit, a contradiction.
- If
- (a)
- If and -suborbit , a contradiction.
- (b)
- If and , we have a second minimal orbit given in (18) with topological structure max-min, shared with setting .
- (c)
- If -suborbit , a contradiction.
- (d)
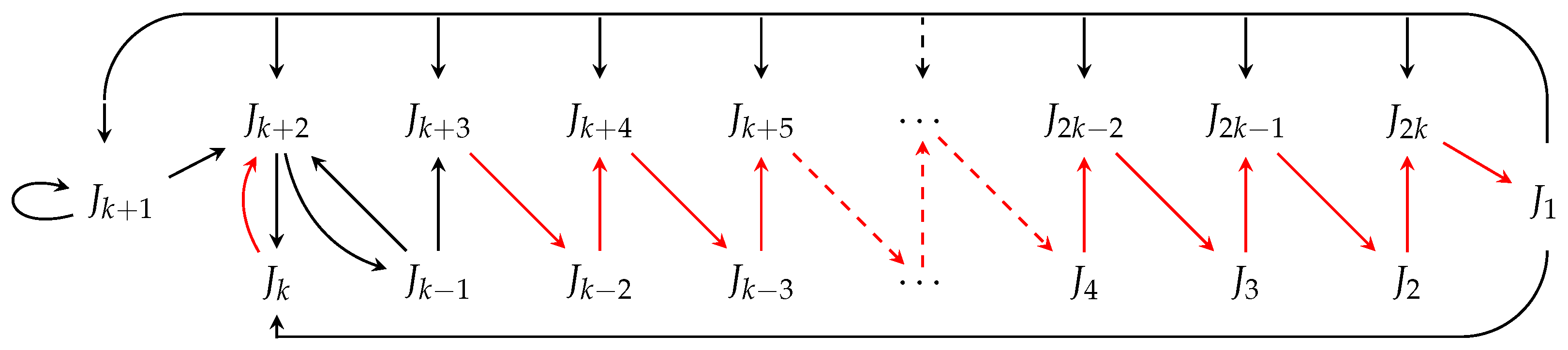
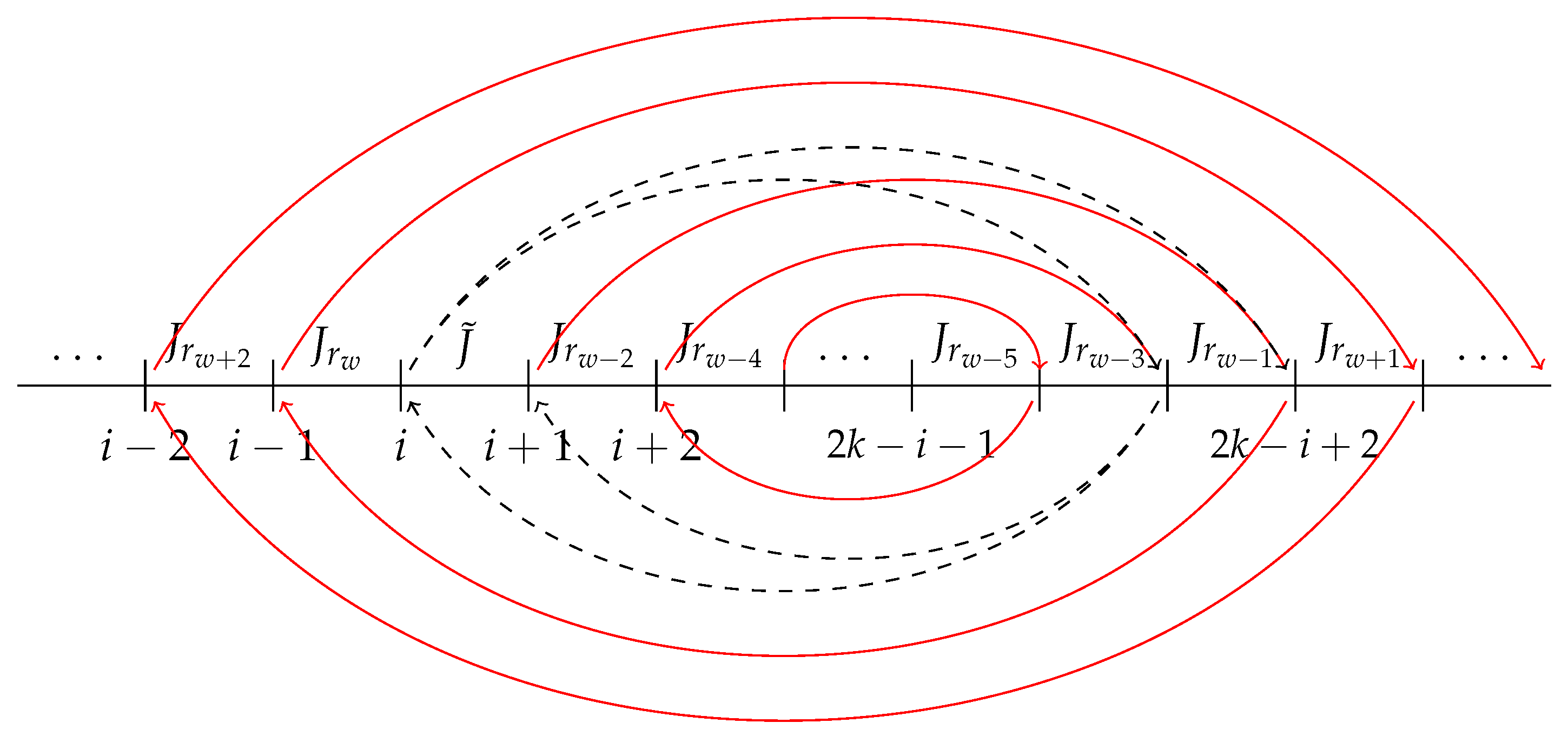
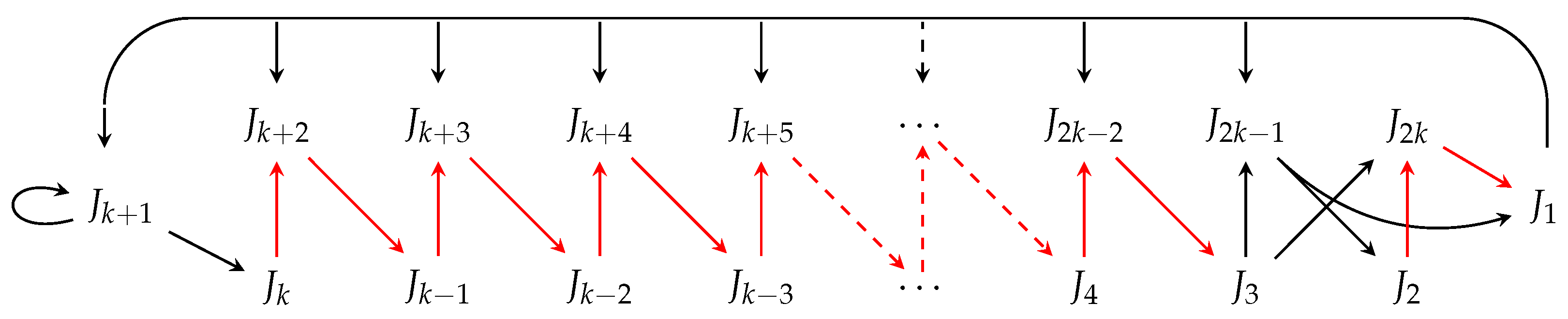
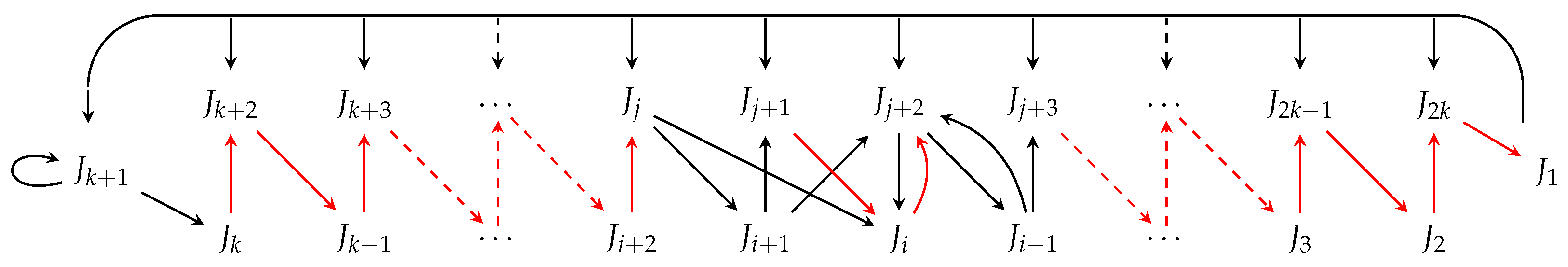
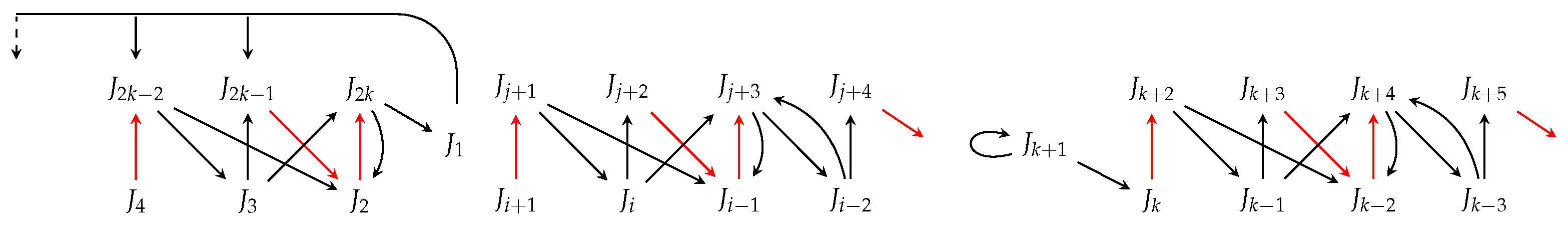
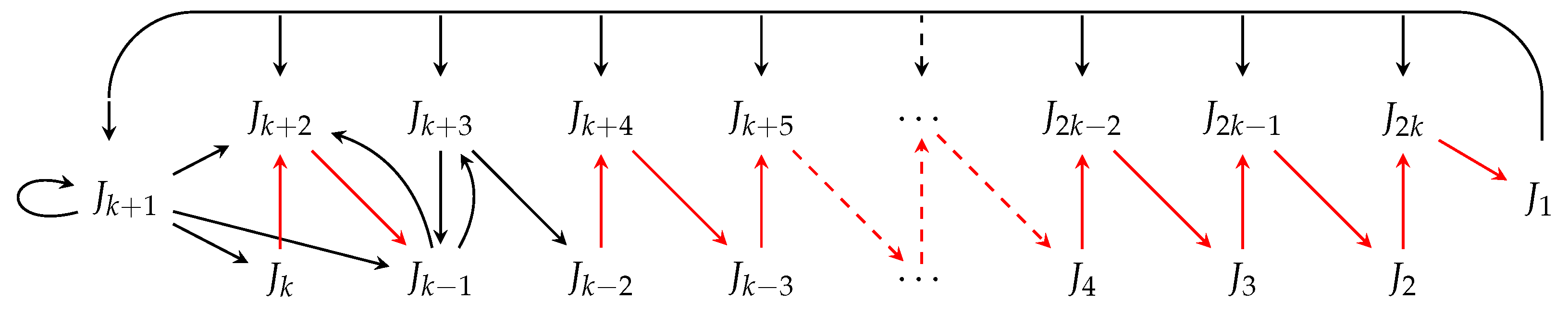
- If , we have a second minimal orbit given in (22) with topological structure max-min-max, and the associated digraph is presented in Figure 16. Next we prove as in previous lemma that there are no primitive cycles of even length , and therefore according to Lemma 3, P-linearization of the orbit (22) presents an example of a continuous map with a second minimal -orbit.
- i.
- Consider primitive cycles that contain . Without any loss of generality, choose as the starting vertex. First, assume that the cycle does not contain . Since and , , any such cycle can be formed only by adding to starting vertex pairs , . Therefore, the length of the cycle (by counting twice) will be always an odd number. Conversely, if the cycle contains , then to close it at , the smallest required even length is .
- ii.
- Consider primitive cycles that do not contain . It is easy to see that by excluding from the cycle, due to the red edges, all the vertices but must be also excluded, and a cycle at and a cycle formed by and are the only possibilities.
- If
- (a)
- If and -suborbit , a contradiction.
- (b)
- If and -suborbit , a contradiction.
- (c)
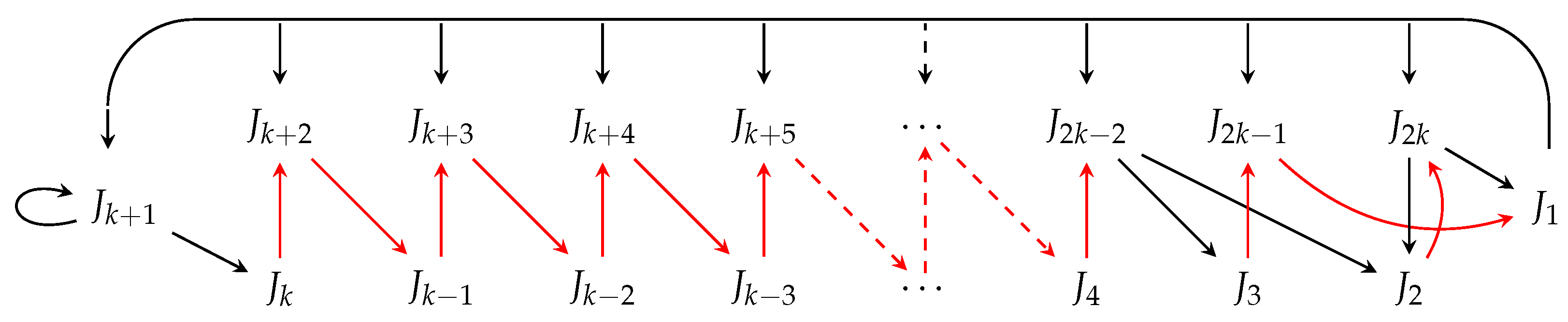
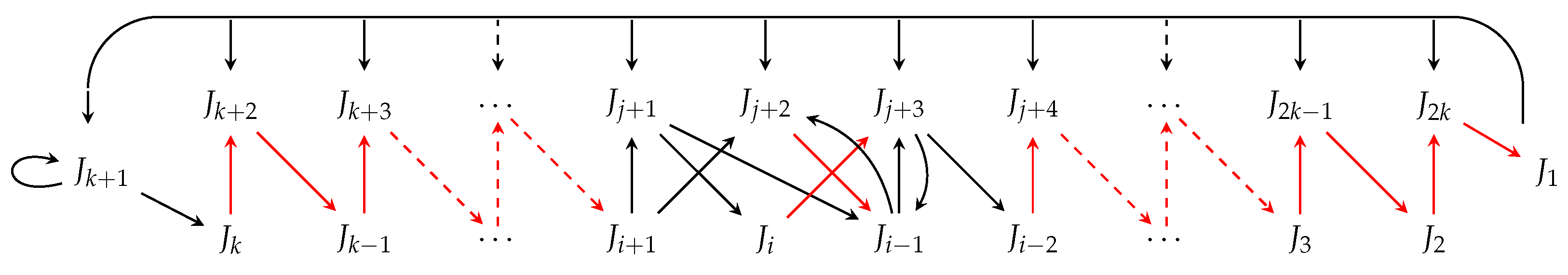
- If , we have a second minimal orbit given in (23) with topological structure max-min-max, and the associated digraph is presented in Figure 17. Next, we prove as in previous cases that there are no primitive cycles of even length , and therefore according to Lemma 3, P-linearization of the orbit (23) presents an example of a continuous map with a second minimal -orbit.
- (i)
- Consider primitive cycles that contain . Without any loss of generality, choose as the starting vertex. First, assume that the cycle does not contain . Since any such cycle can be formed only by adding to starting vertex pairs . Therefore, the length of the cycle (by counting twice) will be always an odd number. Conversely, if the cycle contains , then to close it at , the smallest required even length is .
- (ii)
- Consider primitive cycles that do not contain . It is easy to see that by excluding from the cycle, due to the red edges, all the vertices but must be also excluded, and a cycle at and a cycle formed by and are the only possibilities.
- (d)
- If -suborbit , a contradiction.
- If
- (a)
- If -suborbit , a contradiction.
- (b)
- If -suborbit , a contradiction.
- (c)
- If
- (a)
- If -suborbit , a contradiction.
- (b)
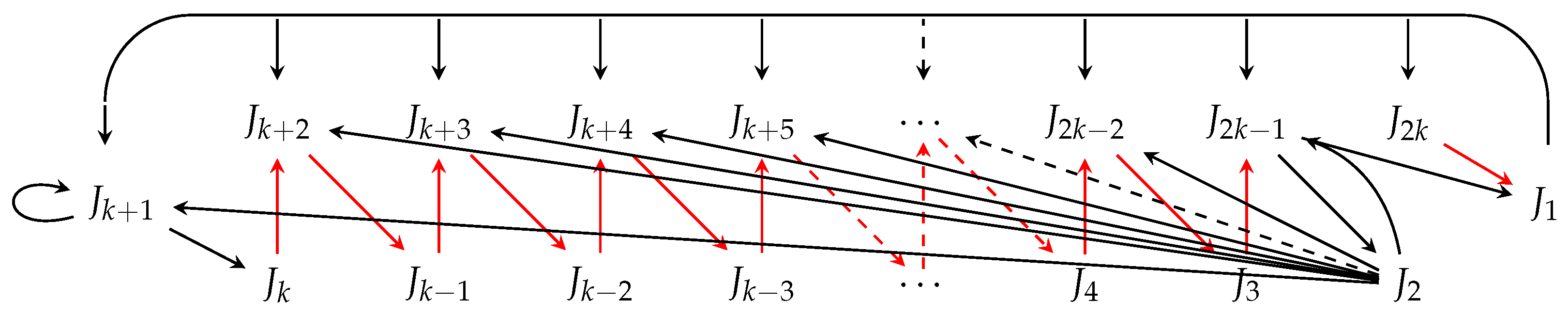
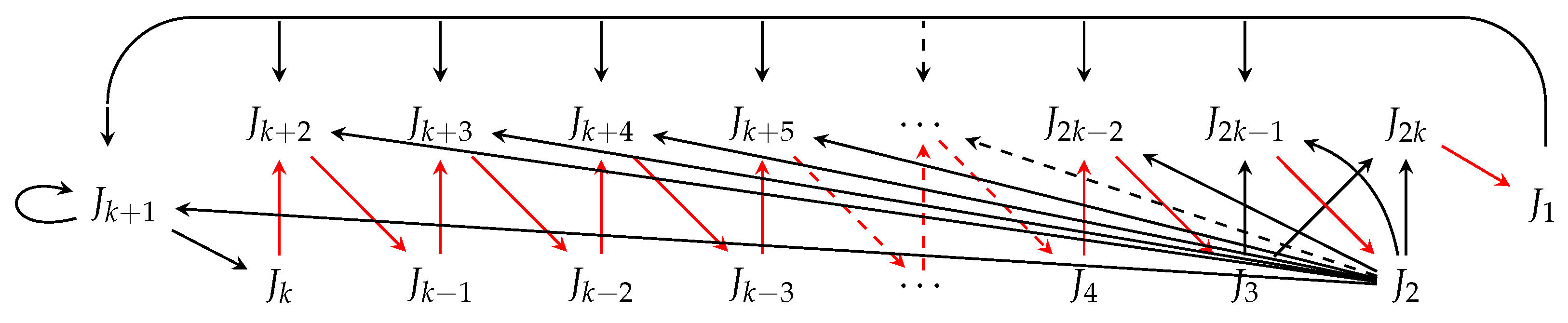
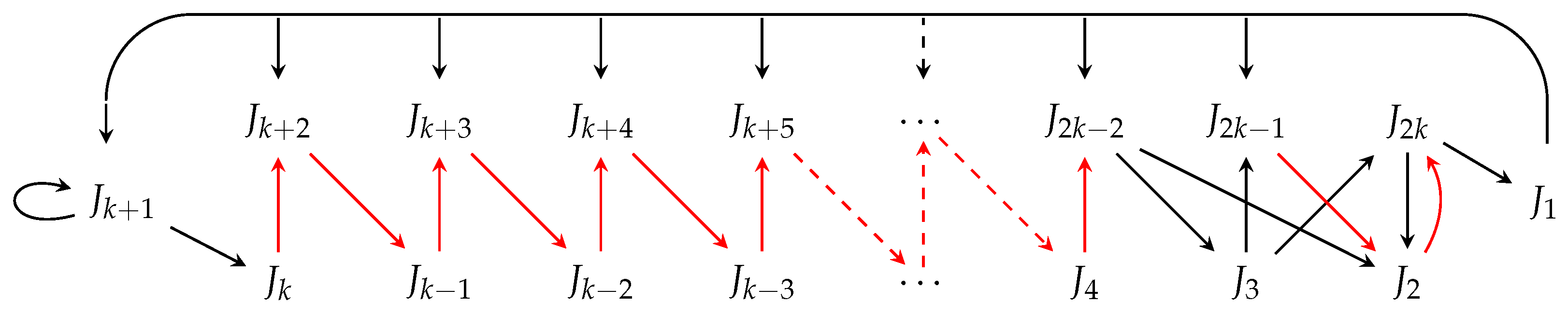
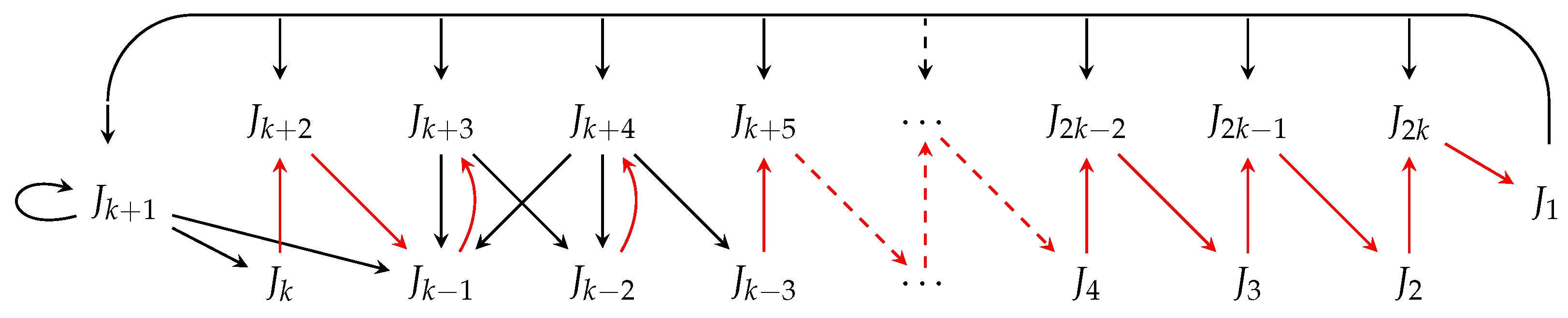
- If or we have the closed 2-suborbit . So we must have . Following the proof of Lemma 5, it follows that for , the digraph of the cyclic permutation contains a primitive subgraphand for , the digraph of the cyclic permutation contains a primitive subgraphboth of which have length . By Lemma 2, a periodic orbit of period must exist, which is a contradiction.
- (c)
- If or there is a period 4-suborbit , . Thus, . By repeating the argument of the previous case, we prove the existence of the -orbit, which is a contradiction.
- If
- If
- (a)
- If -suborbit , a contradiction.
- (b)
- If and -suborbit , a contradiction.
- (c)
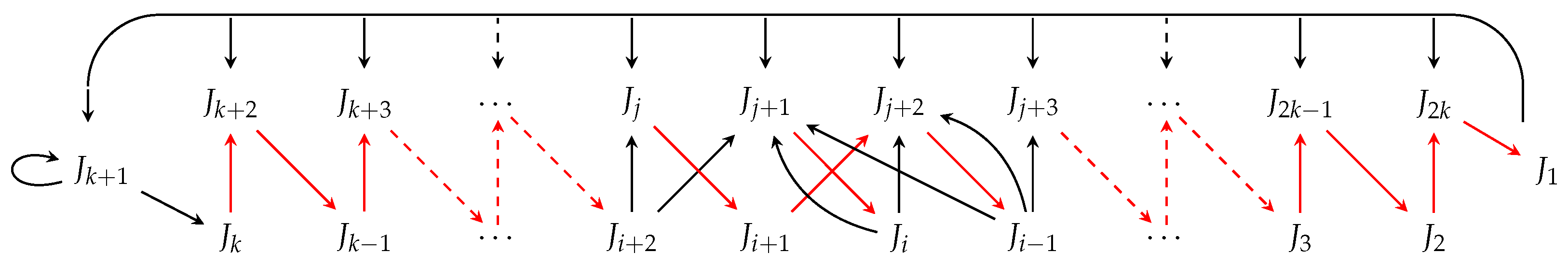
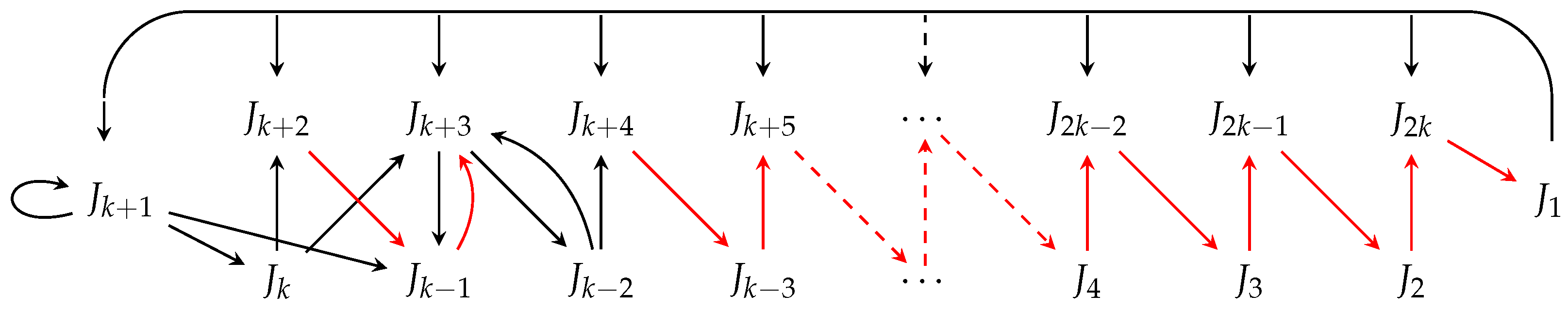
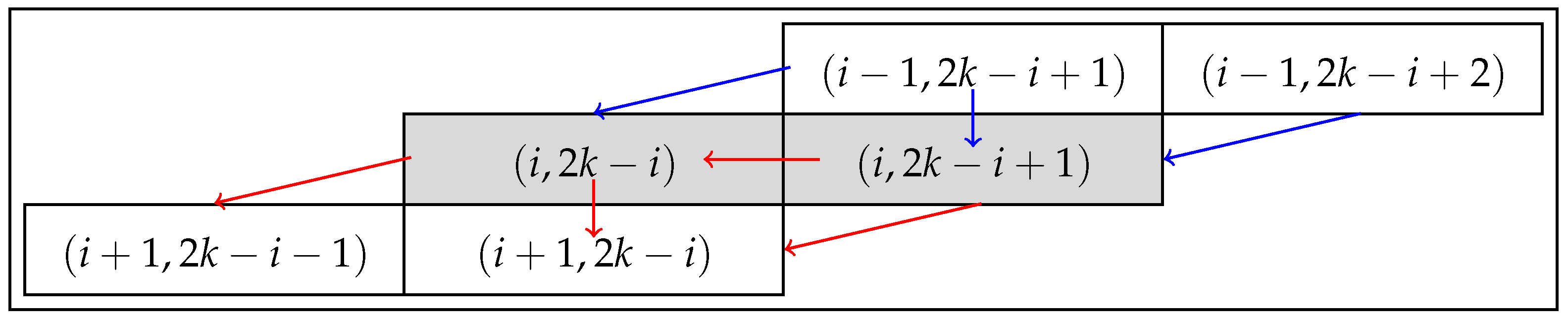
- Consider primitive cycles that contain . Without any loss of generality, choose a starting vertex as . First, assume that the cycle does not contain . Due to presence of red edges, any such cycle can be formed only by successfully adding to starting vertex pairs , where , . Therefore, the length of the cycle (by counting twice) will be always an odd number. Conversely, if the cycle contains , then, besides the new pair or there is a possibility to add just alone due to the loop at , and hence, to build a primitive subgraph of even length. However, the smallest required even length is , and, therefore, no odd orbits of a period smaller than can be produced.
- Consider primitive cycles that do not contain . Since and are only edges directed to , we have to exclude from the primitive cycle unless it is a loop at . However, then, any primitive cycle formed by the remaining intervals can be formed by adding some of the indicated pairs to the starting vertex, and, therefore, all are of odd length.
- If -suborbit , a contradiction.
- If
- (a)
- (b)
- If -suborbit , a contradiction.
- (c)
- If and -suborbit , a contradiction.
- (d)
- If and . Following the proof of the Lemma 5, it follows that for , the digraph of the cyclic permutation contains a primitive subgraphand for , the digraph of the cyclic permutation contains a primitive subgraphboth of which have length . By Lemma 2, a periodic orbit of period must exist, which is a contradiction.
- If
- (a)
- (b)
- If -suborbit , a contradiction.
- (c)
- If and . This implies a cyclic permutation whose digraph contains a primitive subgraph of length . The proof coincides with the proof given above in the case (2d). By Lemma 2, a periodic orbit of period must exist, which is a contradiction.
- (d)
- If and -suborbit , a contradiction.
- If
- (a)
- If -suborbit , a contradiction.
- (b)
- If , we have a second minimal orbit given in (33) with topological structure max-min-max.
- (c)
- If and -suborbit , a contradiction.
- (d)
- If
- (a)
- If -suborbit , a contradiction.
- (b)
- If , then for we have the primitive subgraphof length . Lemma 2 implies the existence of -orbit, which is a contradiction.
- (c)
- If and , we have a second minimal orbit given in (36) with topological structure max-min-max-min-max.
- (d)
- If and -suborbit , a contradiction.
- If
- If -suborbit , a contradiction.
- If
- (a)
- If -suborbit , a contradiction.
- (b)
- If , then for we have the primitive subgraphof length which leads to contradiction as in case (1b).
- (c)
- If and , we have a second minimal orbit given in (39) with topological structure max-min-max-min-max.
- (d)
- If and -suborbit , a contradiction.
5. Conclusions
- Second minimal and minimal -orbits () are simple, meaning that elements on the right and left of the central element of the orbit are mapped to each other except one point. We call these positive type (or negative type) if the exceptional element is on the right (or on the left) of the central point. Cyclic permutations and digraphs of all the negative-type simple orbits are inverses of all the positive-type simple orbits, respectively.
- Simple positive-type second minimal -orbits are either Stefan orbits, or have one of the types, each with a unique digraph and cyclic permutation. Their inverses represent all second minimal -orbits of the simple negative type.
- The constructive proof presented in this paper revealed explicitly the cyclic permutations and digrahs of all second minimal -orbits (), as well as their topological structure (Table 1).
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Sharkovsky, A. Coexistence of cycles of a continuous transofrmation of a line into itself. Ukr. Math. Zh. 1964, 16, 61–71. [Google Scholar]
- Block, L.; Coppel, W.A. Dynamics in One Dimension; Springer: Berlin/Heidelberg, Germany, 1992. [Google Scholar]
- Sharkovsky, A.N.; Maistrenko, Y.L.; Romanenko, E.Y. Difference Equations and Theor Applications; Kluwer Academic Publishers: Dordrecht, The Netherlands, 1986. [Google Scholar]
- Blokh, A.M.; Sharkovsky, O.M. Sharkovsky Ordering; Springer: Cham, Switzerland, 2022; Volume 8, p. 109. [Google Scholar]
- Block, L.; Guckenhimer, J.; Misiurewicz, M.; Young, L. Periodic points and topological entropy of one dimensional maps. In Proceedings of the International Conference on Global Theory of Dynamical Systems, Evanston, IL, USA, 18–22 June 1979; pp. 18–34. [Google Scholar]
- Stefan, P. A theorem of Sharkovskii on the existence of periodic orbits of continuous endomorphisms of the real line. Commun. Math. Phys. 1977, 54, 237–248. [Google Scholar] [CrossRef]
- Alseda, L.; Llibre, J.; Serra, R. Minimal periodic orbits for continuous maps of the interval. Trans. Am. Math. Soc. 1984, 286, 595–627. [Google Scholar] [CrossRef]
- Block, L.; Coppel, W. Stratification of continuous maps of an interval. Trans. Am. Math. Soc. 1986, 297, 587–604. [Google Scholar] [CrossRef]
- Abdulla, A.U.; Abdulla, R.U.; Abdulla, U.G. On the minimal 2(2k + 1)-orbits of the continuous endomorphisms on the real line with application in chaos theory. J. Differ. Equations Appl. 2013, 19, 1395–1416. [Google Scholar] [CrossRef]
- Llibre, J.; Sa, A. Minimal set of periods for continuous self-maps of the eight space. Fixed Point Theory Algorithms Sci. Eng. 2021, 2021, 3. [Google Scholar] [CrossRef]
- Abdulla, U.G.; Abdulla, R.U.; Abdulla, M.U.; Iqbal, N.H. Second minimal orbits, Sharkovski ordering and universality in chaos. Int. J. Bifurc. Chaos 2017, 27, 1730018. [Google Scholar] [CrossRef]
- Feigenbaum, M. Qualitative universality for a class of nonlinear transformations. J. Stat. Phys. 1978, 19, 25–52. [Google Scholar] [CrossRef]
- Feigenbaum, M. The universal metric properties of nonlinear transformations. J. Stat. Phys. 1979, 21, 669–706. [Google Scholar] [CrossRef]
- Feigenbaum, M. Universal behavior in nonlinear systems. Physica 1983, 7, 16–39. [Google Scholar]
- Collet, P.; Eckmann, J.-P.; Lanford, O. Universal properties of maps on an interval. Commun. Math. Phys. 1980, 76, 211–254. [Google Scholar] [CrossRef]
- Campanino, M.; Epstein, H. On the existence of Feigenbaum’s fixed point. Commun. Math. Phys. 1981, 79, 261–302. [Google Scholar] [CrossRef]
- Lanford, O.E. Remarks on the accumulation of period-doubling bifurcations. In Mathematical Problems in Theoretical Physics, Proceedings of the International Conference Mathematics and Physics, Lausanne, Switzerland, 20–25 August 1979; Springer: Berlin/Heidelberg, Germany, 2005; pp. 340–342. [Google Scholar]
- Collet, P.; Eckmann, J.-P. Iterated Maps on the Interval as Dynamical Systems; Birkhauser: Boston, MA, USA, 1980. [Google Scholar]
- Metropolis, N.; Stein, M.L.; Stein, P. On finite limit sets for transformations of the unit interval. J. Comb. Theory 1973, 15, 25–44. [Google Scholar] [CrossRef]
- Milnor, J.; Thurston, W. On iterated maps of the interval. Dyn. Syst. 1988, 1342, 465–563. [Google Scholar]
- Bernhardt, C. Simple permutations with order a power of two. Ergod. Theory Dyn. Syst. 1984, 4, 179–186. [Google Scholar] [CrossRef]
- Acosta-Humanez, P.B. Genealogia de permutaciones simples de orden una potencia de dos. Columbiana Mat. 2008, 2, 1–14. [Google Scholar]
- Acosta-Humanez, P.B.; Martinez-Casablanco, O.E. Simple permutations with order 4n + 2 by means of pasting and reversing. Qual. Theory Dyn. Syst. 2016, 15, 181–210. [Google Scholar] [CrossRef]
- Straffin, P.D. Periodic points of continuous functions. Math. Mag. 1978, 51, 99–105. [Google Scholar] [CrossRef]
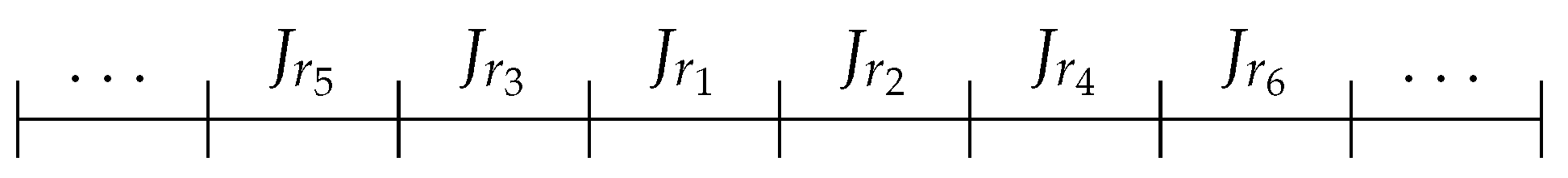
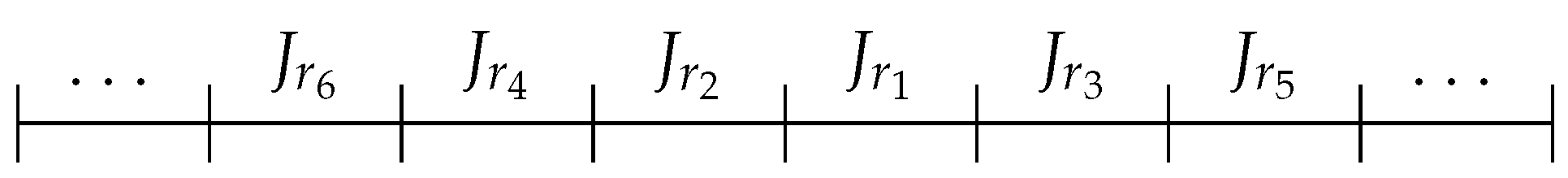

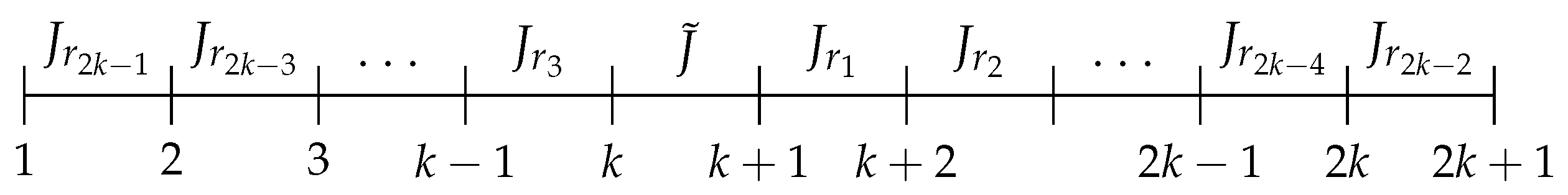



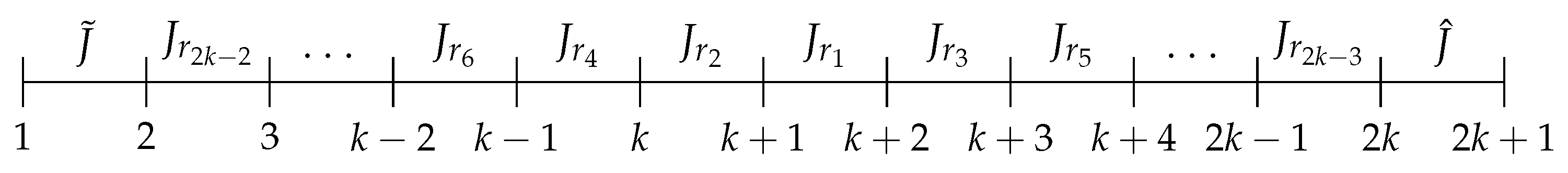



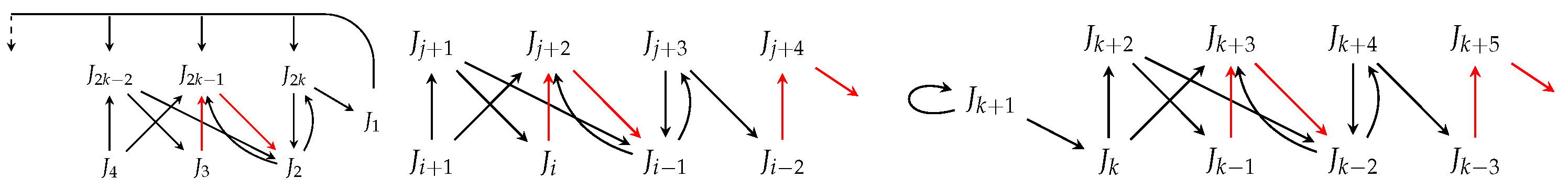






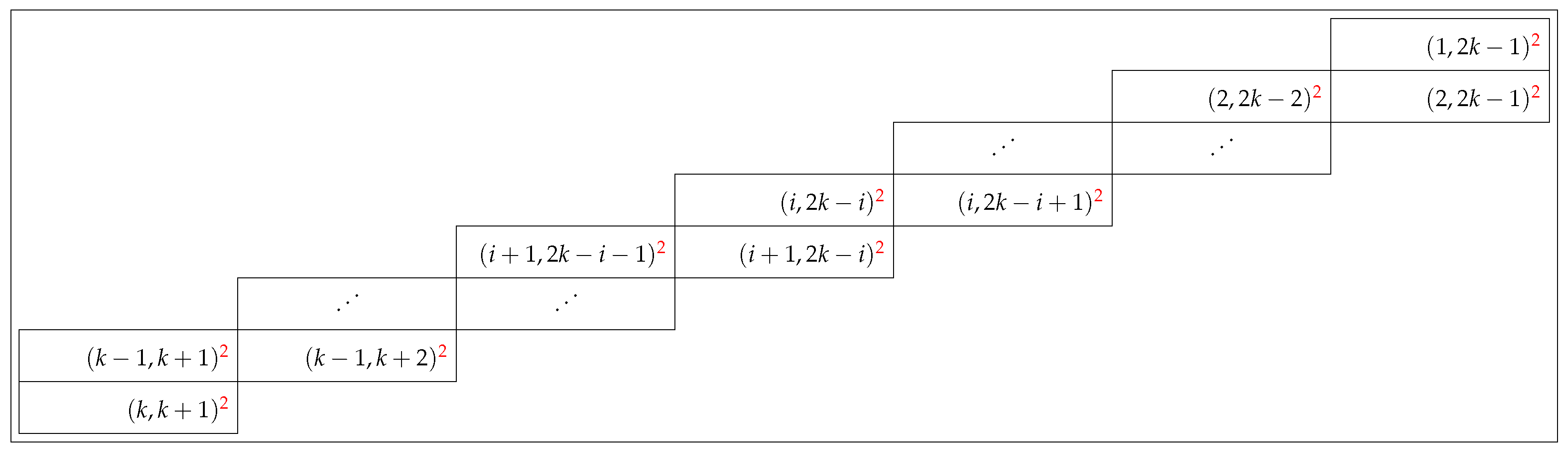
| Topological Structure | Count |
|---|---|
| max | 1 |
| min-max | 1 |
| min-max-min | 1 |
| max-min | 2 |
| max-min-max | |
| max-min-max-min-max |
| Topological Structure | Count | Permutation |
|---|---|---|
| max | 1 | (23) |
| min-max | 1 | (15) |
| min-max-min | 1 | (14) |
| max-min | 2 | (18),(19) |
| max-min-max | (11),(22),(38),(26),(29),(33),(34) | |
| max-min-max-min-max | (36),(27),(31),(28),(32) |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Abdulla, U.G.; Iqbal, N.H.; Abdulla, M.U.; Abdulla, R.U. Classification of the Second Minimal Orbits in the Sharkovski Ordering. Axioms 2025, 14, 222. https://doi.org/10.3390/axioms14030222
Abdulla UG, Iqbal NH, Abdulla MU, Abdulla RU. Classification of the Second Minimal Orbits in the Sharkovski Ordering. Axioms. 2025; 14(3):222. https://doi.org/10.3390/axioms14030222
Chicago/Turabian StyleAbdulla, Ugur G., Naveed H. Iqbal, Muhammad U. Abdulla, and Rashad U. Abdulla. 2025. "Classification of the Second Minimal Orbits in the Sharkovski Ordering" Axioms 14, no. 3: 222. https://doi.org/10.3390/axioms14030222
APA StyleAbdulla, U. G., Iqbal, N. H., Abdulla, M. U., & Abdulla, R. U. (2025). Classification of the Second Minimal Orbits in the Sharkovski Ordering. Axioms, 14(3), 222. https://doi.org/10.3390/axioms14030222





