Epimorphisms Amalgams and Po-Unitary Pomonoids

Abstract

This paper proves that the special pomonoid amalgam with a quasi po-unitary or almost po-unitary core U is strongly poembeddable. The techniques used to establish these results involve Isbell’s zigzag theorem and its descriptions in terms of the pomonoid dominions.

1. Introduction

The subject of unitary subsemigroups has been recognized as being connected to the issue of whether semigroup amalgams can be embedded, following Howie’s foundational research in [1]. This has been extended to almost unitary subsemigroups, again by Howie [2], regarding the embeddability of semigroup amalgams. Renshaw [3] further applied Howie’s work to quasi unitary subsemigroups. Al Subaiei and Renshaw [4] generalized it to posemigroups and established various connections with flatness properties and amalgamation in pomonoids. N. Alam and N. M. Khan (see [5,6]) used the technique of semigroup dominions and obtained results concerning the embeddability of special semigroup amalgams for almost unitary and quasi unitary semigroups, respectively. In this work, we will generalize their findings in [5,6] to the category of pomonoids, exploring new properties and implications within this wider category.

A monoid S is called a partially ordered monoid, or a pomonoid, if it is equipped with a partial-order relation ≤ that is compatible with the binary operation on S.

$$\forall s,t,s^{\prime }\in S,s\le t\Rightarrow s^{\prime }s\le s^{\prime }t\mathrm{and}s{s}^{\prime }\le t{s}^{\prime }.$$

Among the most natural examples of pomonoids are $({\mathbb{N}}^0,+)$ and $(\mathbb{N},.)$, where both pomonoids are equipped with the natural order. A mapping $f:S⟶T$, where S and T are pomonoids, is referred to as a pomonoid morphism if f is a monoid morphism that is also monotone, i.e., $s\le s^{\prime }$ implies $f(s)\le f(s^{\prime })$. A pomonoid morphism $f:S⟶T$ is said to be an epimorphism (epi for short) if, whenever $g,h:T⟶W$ are pomonoid morphisms, such that $g\circ f=h\circ f$, it implies that $g=h$. It is a well-known fact that every surjective morphism is an epimorphism in any category, but in the category of pomonoids, the converse does not hold. In the category of pomonoids, the natural embedding i of $({\mathbb{N}}^0,+)$ into $(\mathbb{Z},+)$, where both pomonoids are equipped with the natural order, is an epimorphism.

J. R. Isbell in [7] introduced the notion of dominion and related it to epimorphism. In the category of semigroups, due to the famous Isbell’s zigzag theorem, this notion has been helpful for the investigation of epimorphism-related problems (see [8,9]). The systematic study of epimorphism and dominions has been initiated by N. Sohail [10] and B. Al Subaie [11]. N. Sohail [12] obtained the analog of Isbell’s zigzag theorem for pomonoids. Ahanger, Shah and Khan [13] successfully extended many of the classical results of N. M. Khan [14] and P. M. Higgins [15] in the category of posemigroups using the zigzag theorem.

Let U be a subpomonoid of a pomonoid S and $d\in S$. When for all pomonoids T and all pomonoid morphisms $\alpha ,\beta :S⟶T,$ we have that

$$\forall u\in U,\alpha (u)=\beta (u)\Rightarrow \alpha (d)=\beta (d),$$

and then, we say that d is dominated by U. The dominion of U in S is the set of all elements of S dominated by U and it is denoted by $\widehat{Do{m}_S}(U)$. One can easily prove that $U\subseteq \widehat{Do{m}_S}(U)\subseteq S$. If $U=\widehat{Do{m}_S}(U)$, we conclude that U is closed in S, and U is said to be absolutely closed if $U=\widehat{Do{m}_S}(U)$ for every pomonoid S that contains U as a subpomonoid. For a subpomonoid U of a pomonoid S, let $Do{m}_S(U)$ denote the monoid dominion of U in S. One can easily observe that $Do{m}_S(U)\subseteq \widehat{Do{m}_S}(U)$. Therefore, if U is closed in S as a pomonoid, then it is also closed in S as a monoid.

We call the triple $U=[S_i;U;{\phi }_i]$ a pomonoid amalgam if it consists of a pomonoid U (referred to as the core of the amalgam), a family of pomonoids $\{S_i:i\in I\}$, as well as a set of order embeddings ${\phi }_i:U⟶S_i$ for each $i\in I$. An amalgam is said to be weakly embeddable (resp. poembeddable) in a pomonoid Z whenever there are pomonoid monomorphisms (resp. order embeddings) ${\theta }_i:S_i⟶Z$ such that ${\theta }_i{\phi }_i={\theta }_j{\phi }_j$ for every $i\ne j$. Furthermore, the amalgam is strongly embeddable (resp. poembeddable) in Z when ${\theta }_i(S_i)\cap {\theta }_j(S_j)={\theta }_i{\phi }_i(U)$. Whenever there exists an order isomorphism $\varphi :S_1⟶S_2$ such that $\varphi {\phi }_1={\phi }_2$, then we refer to the pomonoid amalgam $[U;S_1,S_2]$ as a special amalgam. It is evident from [16] that a pomonoid amalgam is (weakly, strongly) embeddable (resp. poembeddable) in a pomonoid if and only if it is naturally (weakly, strongly) embeddable (resp. poembeddable) in its free product.

The following result establishes the relation between pomonoid amalgams and dominions of Pomonoids.

Theorem 1

([11], Theorem 4.1.1). Let ${\theta }_1$ and ${\theta }_2$ be the natural maps from $S_1$ and $S_2$, respectively, into the free product of the ordered amalgam $[U,S_1,S_2]$. Also, let U be a subpomonoid of pomonoids $S_1$ and $S_2$, and let $\psi :S_1⟶S_2$ be an order isomorphism. Then, $\widehat{Do{m}_S}(U)={\theta }_1^{-1}({\theta }_1(S_1)\cap {\theta }_2(S_2)).$

Thus, we can conclude that the special pomonoid amalgam $[U;S;S^{\prime }]$ is strongly poembeddable if and only if U is closed in S.

The characterization of posemigroup dominion established by Sohail and Tart [17] is known as the zigzag theorem for posemigroups and is described as follows.

Theorem 2

([17], Theorem 5). Let U be a subpomonoid of a pomonoid S. Then, $d\in \widehat{Dom}(U,S)$ if and only if $d\in U$ or

$$\begin{array}{cc}\hfill d& \le x_1{u}_0,u_0\le u_1{y}_1\hfill \\ \hfill x_i{u}_{2i-1}& \le x_{i+1}{u}_{2i},u_{2i}{y}_i\le u_{2i+1}{y}_{i+1}\hfill \\ \hfill x_n{u}_{2n-1}& \le u_{2n},u_{2n}{y}_n\le d;\hfill \end{array}$$

(1)

$$\begin{array}{cc}\hfill v_0& \le s_1{v}_1,d\le v_0{t}_1\hfill \\ \hfill s_j{v}_{2j}& \le s_{j+1}{v}_{2j+1}{v}_{2j-1}{t}_j\le v_{2j}{t}_{j+1}\hfill \\ \hfill s_m{v}_{2m}& \le d,v_{2m-1}{t}_m\le v_{2m},\hfill \end{array}$$

(2)

where,

$$i=2,\dots ,n-1,j=2,\dots ,m-1,$$

$$u_0,\dots ,u_{2n},v_0,\dots ,v_{2m}\in U,$$

$$x_1,\dots ,x_n,y_1,\dots ,y_n,s_1,\dots ,s_m,t_1,\dots ,t_m\in S.$$

The inequalities outlined above are referred to as zigzag in S over U with value d. The first scheme of the zigzag inequalities (1) produces

$$d\le x_1{u}_0\le x_1{u}_1{y}_1\le x_2{u}_2{y}_1\le \cdots \le x_n{u}_{2n-1}{y}_n\le u_{2n}{y}_n\le d.$$

This means that

$$d=x_1{u}_0=x_1{u}_1{y}_1=x_2{u}_2{y}_1=\cdots =x_n{u}_{2n-1}{y}_n=u_{2n}{y}_n.$$

(3)

Similarly, the second scheme (2) of the zigzag inequalities produces

$$d\le v_0{t}_1\le s_1{v}_1{t}_1\le s_1{v}_2{t}_2\le \cdots \le s_m{v}_{2m-1}{t}_m\le s_m{v}_{2m}\le d;$$

whence

$$d=v_0{t}_1=s_1{v}_1{t}_1=s_1{v}_2{t}_2=\cdots =s_m{v}_{2m-1}{t}_m=s_m{v}_{2m}.$$

(4)

2. Epimorphisms–Amalgams and Quasi Po-Unitary Pomonoids

This section will examine the relationship between quasi po-unitary pomonoids and the embeddability of special pomonoid amalgams. We have established that when U is quasi po-unitary in S, the special pomonoid amalgam is strongly poembeddable. In fact, this relation has been demonstrated for the unordered case.

Theorem 3

([6]). Let U be a quasi unitary submonoid of a monoid S, and then U is closed in S.

In the category of pomonoids (see [4,11]), unitary properties have been extended to various types of unitary structures, leading to a refined order relation. Let U be a subpomonoid of a pomonoid S. Then, the following can be stated:

1

U is upper strongly left po-unitary (USLPU) in S if $v\le us\Rightarrow s\in U$;

2

U is lower strongly left po-unitary (LSLPU) in S if $u^{\prime }s\le v^{\prime }\Rightarrow s\in U$;

3

U is strongly left po-unitary (SLPU) in S if $v\le us$ or $u^{\prime }s\le v^{\prime }\Rightarrow s\in U$;

4

U is left po-unitary (LPU) in S if $v\le u_1{s}_1,u_1^{\prime }{s}_1\le u_2{s}_2,\dots ,u_n^{\prime }{s}_n\le v^{\prime }\Rightarrow s_1,s_2,\dots ,s_n\in U$;

5

U is left unitary (LU) in S if $us=v\Rightarrow s\in U$.

where $v,v^{\prime },u,u^{\prime },u_1,u_1^{\prime },\dots ,u_n,u_n^{\prime }\in U$ and $s,s_1,s_2,\dots ,s_n\in S$. Next, we further extend these properties to quasi po-unitary-type properties.

Let U be the subpomonoid of a pomonoid S. Let us assume that there is a $(U,U)$-morphism $\varphi :S⟶S$ such that ${\varphi }^2\le \varphi$ and $\varphi (1)=1$. We say as follows:

6

U is upper strongly left quasi po-unitary (USLQPU) in S if $v\le us\Rightarrow \varphi (s)\in U$;

7

U is lower strongly left quasi po-unitary (LSLQPU) in S if $u^{\prime }s\le v^{\prime }\Rightarrow \varphi (s)\in U$;

8

U is strongly left quasi po-unitary (SLQPU) in S if $v\le us$ or $u^{\prime }s\le v^{\prime }\Rightarrow \varphi (s)\in U$;

9

U is left quasi po-unitary (LQPU) in S if $v\le u_1{s}_1,u_1^{\prime }{s}_1\le u_2{s}_2,\dots ,u_n^{\prime }{s}_n\le v^{\prime }\Rightarrow \varphi (s_1),\varphi (s_2),\dots ,\varphi (s_n)\in U$;

10

U is left quasi unitary (LQU) in S if $us=v\Rightarrow \varphi (s)\in U$.

Versions for these conditions for the right-hand and both sides are defined in a comparable way. The two-sided version is equivalent to both the left-handed and right-handed versions.

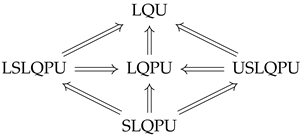
The following diagram indicates the implications among these classes of semigroups.

Further; if U is any of the above subpomonoids of a pomonoid S with $\varphi :S⟶S$ as a $(U,U)$-morphism; then for any $u\in U$,

$$\varphi (u)=\varphi (u1)=u\varphi (1)=u1=u.$$

(5)

Therefore, for every $u\in U$ and $s\in S$, we have

$$\varphi (us)=u\varphi (s),\varphi (su)=\varphi (s)u.$$

(6)

Theorem 4.

Let U be a subpomonoid of a pomonoid S. The subpomonoid U is closed in S whenever U is an LQPU in S.

Proof. 

Let U be a subpomonoid of a pomonoid S such that U is LQPU in S. Take any $d\in \widehat{Do{m}_S}(U)\setminus U$. By Theorem 2, d has zigzag inequalities of the type (1) and (2). From the left columns in schemes (1) and (2), respectively, we have

$$\begin{array}{cc}\hfill u_0& =\varphi (u_0)(\mathrm{by} \mathrm{Equation} (5))\hfill \\ \hfill & \le \varphi (u_1{y}_1)(\mathrm{by} \mathrm{zigzag} \mathrm{inequalities} (1))\hfill \\ \hfill & =u_1\varphi (y_1)(\mathrm{by} \mathrm{Equation} (6)).\hfill \end{array}$$

Therefore,

$$u_0\le u_1\varphi (y_1).$$

(7)

$$\begin{array}{cc}\hfill u_2\varphi (y_1)& =\varphi (u_2{y}_1)(\mathrm{by} \mathrm{Equation} (6))\hfill \\ \hfill & \le \varphi (u_3{y}_2)(\mathrm{by} \mathrm{zigzag} \mathrm{inequalities} (1))\hfill \\ \hfill & =u_3\varphi (y_2)(\mathrm{by} \mathrm{Equation} (6)).\hfill \end{array}$$

Therefore,

$$u_2\varphi (y_1)\le u_3\varphi (y_2).$$

(8)

Continuing in this fashion, we have

$$u_{2i}\varphi (y_i)\le u_{2i+1}\varphi (y_{i+1}),1\le i\le n-1.$$

(9)

And so, by using Equation (6) and the zigzag inequalities (1), we obtain

$$u_{2n}\varphi (y_n)=\varphi (u_{2n}{y}_n)\le \varphi (d)=1\varphi (d).$$

(10)

$$\begin{array}{cc}\hfill 1\varphi (d)& =\varphi (d)(\mathrm{by} \mathrm{Equation} (6))\hfill \\ \hfill & \le \varphi (v_0{t}_1)(\mathrm{by} \mathrm{zigzag} \mathrm{inequalities} (2))\hfill \\ \hfill & =v_0\varphi (t_1)(\mathrm{by} \mathrm{Equation} (6)).\hfill \end{array}$$

Therefore,

$$\varphi (d)\le v_0\varphi (t_1).$$

(11)

Once again,

$$\begin{array}{cc}\hfill v_1\varphi (t_1)& =\varphi (v_1{t}_1)(\mathrm{by} \mathrm{Equation} (6))\hfill \\ \hfill & \le \varphi (v_2{t}_2)(\mathrm{by} \mathrm{zigzag} \mathrm{inequalities} (2))\hfill \\ \hfill & =v_2\varphi (t_2)(\mathrm{by} \mathrm{Equation} (6)).\hfill \end{array}$$

So,

$$v_1\varphi (t_1)\le v_2\varphi (t_2).$$

(12)

Continuing in this fashion, we have

$$v_{2j-1}\varphi (t_j)\le v_{2j}\varphi (t_{j+1}),1\le j\le m-1.$$

(13)

Finally, by using Equation (6), zigzag inequalities (2), and Equation (5), we obtain

$$v_{2m-1}\varphi (t_m)=\varphi (v_{2m-1}{t}_m)\le \varphi (v_{2m})=v_{2m}.$$

(14)

As ${\varphi }^2\le \varphi$ and U is LQPU in S, we obtain that all of

$$\varphi (y_1),\varphi (y_2)\dots ,\varphi (y_n),\varphi (d)\mathrm{and}\varphi (t_1),\varphi (t_2),\dots ,\varphi (t_m)\in U.$$

Now,

$$\begin{array}{cc}\hfill d& =x_1{u}_0(\mathrm{by} \mathrm{zigzag} \mathrm{inequalities} (3))\hfill \\ \hfill & =x_1\varphi (u_0)(\mathrm{by} \mathrm{inequality} (7))\hfill \\ \hfill & \le x_1{u}_1\varphi (y_1)(\mathrm{by} \mathrm{zigzag} \mathrm{inequalities} (1))\hfill \\ \hfill & \le x_2{u}_2\varphi (y_1)(\mathrm{by} \mathrm{inequality} (8))\hfill \\ \hfill & \le x_2{u}_3\varphi (y_2)(\mathrm{by} \mathrm{zigzag} \mathrm{inequalities} (1))\hfill \\ \hfill ⋮& \hfill \\ \hfill & \le x_i{u}_{2i-1}\varphi (y_i)\hfill \\ \hfill & \le x_{i+1}{u}_{2i}\varphi (y_i)(\mathrm{by} \mathrm{zigzag} \mathrm{inequalities} (1))\hfill \\ \hfill & \le x_{i+1}{u}_{2i+1}\varphi (y_{i+1})(\mathrm{by} \mathrm{inequality} (9))\hfill \\ \hfill ⋮& \hfill \\ \hfill & \le x_n{u}_{2n-1}\varphi (y_n)\hfill \\ \hfill & \le u_{2n}\varphi (y_n)(\mathrm{by} \mathrm{zigzag} \mathrm{inequalities} (1))\hfill \\ \hfill & \le \varphi (d)(\mathrm{by} \mathrm{inequality} (10))\hfill \\ \hfill & \le v_0\varphi (t_1)(\mathrm{by} \mathrm{inequality} (11))\hfill \\ \hfill & \le s_1{v}_1\varphi (t_1)(\mathrm{by} \mathrm{zigzag} \mathrm{inequalities} (2))\hfill \\ \hfill & \le s_1{v}_2\varphi (t_2)(\mathrm{by} \mathrm{inequality} (12))\hfill \\ \hfill ⋮& \hfill \\ \hfill & \le s_j{v}_{2j-1}\varphi (t_j)\hfill \\ \hfill & \le s_j{v}_{2j}\varphi (t_{j+1})(\mathrm{by} \mathrm{inequality} (13))\hfill \\ \hfill & \le s_{j+1}{v}_{2j+1}\varphi (t_{j+1})(\mathrm{by} \mathrm{zigzag} \mathrm{inequalities} (2))\hfill \\ \hfill ⋮& \hfill \\ \hfill & \le s_m{v}_{2m-1}\varphi (t_m)\hfill \\ \hfill & \le s_m{v}_{2m}(\mathrm{by} \mathrm{inequality} (14))\hfill \\ \hfill & =d(\mathrm{by} \mathrm{zigzag} \mathrm{inequalities} (4)).\hfill \end{array}$$

Hence, $d=\varphi (d)\in U$, as required. □

In a dual manner, we can establish the following.

Theorem 5.

Let U be a subpomonoid of a pomonoid S. If U is RQPU in S, then U is closed in S.

A subpomonoid U of a pomonoid S is quasi po-unitary (QPU) in S if it is both LQPU and RQPU in S.

The following results are now immediate.

Corollary 1.

A quasi po-unitary subpomonoid U of a pomonoid S is closed in S.

Corollary 2.

Let U be a subpomonoid of a pomonoid S. If U is either SLQPU, LSLQPU, or USLQPU in S, then it is closed in S.

Dually, we may prove the following.

Corollary 3.

Let U be a subpomonoid of a pomonoid S. If U is either SRQPU, LSRQPU, or USRQPU in S, then it is closed in S.

From Theorem 1 and Corollary 1, we can conclude the following.

Corollary 4.

The special pomonoid amalgam with a QPU core U is strongly poembeddable.

3. Epimorphisms–Amalgams and Almost Po-Unitary Pomonoids

This section explores the connection between almost po-unitary pomonoids and the poembeddability of special pomonoid amalgams. We have demonstrated that when U is almost po-unitary in S, the special pomonoid amalgam is strongly poembeddable. Moreover, this relationship has been established for the unordered case.

Theorem 6

([5]). Let U be a submonoid of a monoid S. The submonoid U is closed in S whenever U is almost-unitary in S.

Let S be a pomonoid and $\lambda ,\rho :S⟶S$ be the maps. The map $\lambda$ is called a left translation of S if the following conditions are satisfied:

(ALPU1)

$\lambda (st)=\lambda (s)t$ for all $s,t\in S$;

(ALPU2)

$\lambda$ is monotone.

In contrast, the map $\rho$ is referred to as a right translation if the following conditions are satisfied:

(ALPU3)

$\rho (st)=s\rho (t)$ for all $s,t\in S$;

(ALPU4)

$\rho$ is monotone.

A left translation $\lambda$ and a right translation $\rho$ are called linked if for every $s,t\in S$, the following applies:

(ALPU5)

$s\lambda (t)=\rho (s)t$,

and the pair $(\lambda ,\rho )$ is called a bitranslation of S.

Let U be a nonempty subset of the pomonoid S. The subset U is called an almost left po-unitary (ALPU) in S whenever there exist mappings $\lambda ,\rho :S⟶S$ (called associated mappings) that satisfy the following:

(ALPU6)

$(\lambda ,\rho )$ is called a bitranslation of S;

(ALPU7)

$\lambda \circ \rho =\rho \circ \lambda$ (i.e., $\lambda$ commutes with $\rho$);

(ALPU8)

$\lambda \le {\lambda }^2$ and $\rho \le {\rho }^2$;

(ALPU9)

$\lambda {\mid }_U=\rho {\mid }_U=1_U$;

(ALPU10)

U is left po-unitary in $\rho (\lambda (S))$.

Dually, we can define an almost right po-unitary subset of S. A subset U of S is said to be almost po-unitary in S if it is both ALPU and ARPU in S.

As in the unordered case, we have the following (see Lemma 4.7 in [3]).

Lemma 1.

Let U be the ALPU subset of a pomonoid S. Then, U is LQPU.

Lemma 2.

Let U be the ALPU subset of a pomonoid S. Then, $\rho (\lambda (S))$ is a subpomonoid of S containing U.

Proof. 

Clearly, $U=\rho (\lambda (U))\subseteq \rho (\lambda (S))$ and so $1\in \rho (\lambda (S))$. Let $a,b\in S$, then

$$\begin{array}{cc}\rho (\lambda (a))\rho (\lambda (b))\hfill & \hfill =\rho (\rho (\lambda (a))\lambda (b))(\mathrm{by} \mathrm{ALPU3})\\ \hfill & \hfill =\rho (\lambda (\rho (a))\lambda (b))(\mathrm{by} \mathrm{ALPU7})\\ \hfill & \hfill =\rho (\lambda (\rho (a)\lambda (b)))(\mathrm{by} \mathrm{ALPU1}),\end{array}$$

that is, $\rho (\lambda (a))\rho (\lambda (b))\in \rho (\lambda (S))$, as required. □

In view of Lemma 2 condition (ALPU10), the definition of ALPU makes sense.

Theorem 7.

Let U be a subpomonoid of a pomonoid S. If U is ALPU in S, then U is closed in S.

Proof. 

Let U be a subpomonoid of a pomonoid S such that U is ALPU in S. Take any $d\in \widehat{Do{m}_S}(U)\backslash U$. By Theorem 2, d has zigzag inequalities of the type (2). Therefore, we have

$$\begin{array}{cc}\hfill u_0& =\rho (u_0)(\mathrm{by}(\mathrm{ALPU9}))\hfill \\ \hfill & \le \rho (u_1{y}_1)(\mathrm{by} \mathrm{zigzag} \mathrm{inequalities} (1) \mathrm{and} (\mathrm{ALPU4}))\hfill \\ \hfill & =u_1\rho (y_1)(\mathrm{by} (\mathrm{ALPU3}))\hfill \\ \hfill & =\rho (u_1)\rho (y_1)(\mathrm{by} (\mathrm{ALPU9}))\hfill \\ \hfill & =u_1\lambda (\rho (y_1))(\mathrm{by} (\mathrm{ALPU5}))\hfill \\ \hfill & =u_1\rho (\lambda (y_1))(\mathrm{by} (\mathrm{ALPU7})).\hfill \end{array}$$

Therefore,

$$u_0\le u_1\rho (\lambda (y_1)).$$

(15)

Next, we have

$$\begin{array}{cc}\hfill u_2\rho (\lambda (y_1))& =u_2\lambda (\rho (y_1))(\mathrm{by} (\mathrm{ALPU7}))\hfill \\ \hfill & =\rho (u_2)\rho (y_1)(\mathrm{by} (\mathrm{ALPU5}))\hfill \\ \hfill & =u_2\rho (y_1)(\mathrm{by} (\mathrm{ALPU9}))\hfill \\ \hfill & =\rho (u_2{y}_1)(\mathrm{by} (\mathrm{ALPU3}))\hfill \\ \hfill & \le \rho (u_3{y}_2)(\mathrm{by} \mathrm{zigzag} \mathrm{inequalities} (1) \mathrm{and} (\mathrm{ALPU4}))\hfill \\ \hfill & =u_3\rho (y_2)(\mathrm{by} (\mathrm{ALPU3}))\hfill \\ \hfill & =\rho (u_3)\rho (y_2)(\mathrm{by} (\mathrm{ALPU9}))\hfill \\ \hfill & =u_3\lambda (\rho (y_2))(\mathrm{by} (\mathrm{ALPU5}))\hfill \\ \hfill & =u_3\rho (\lambda (y_2))(\mathrm{by} (\mathrm{ALPU7})).\hfill \end{array}$$

Thus, we have

$$u_2\rho (\lambda (y_1))\le u_3\rho (\lambda (y_2)).$$

(16)

Continuing in this fashion, we have

$$u_{2i}\rho (\lambda (y_i))\le u_{2i+1}\rho (\lambda (y_{i+1}))\mathrm{where}1\le i\le n-1.$$

(17)

Also,

$$\begin{array}{cc}\hfill u_{2n}\rho (\lambda (y_n))& =u_{2n}\lambda (\rho (y_n))(\mathrm{by} (\mathrm{ALPU7}))\hfill \\ \hfill & =\rho (u_{2n})\rho (y_n)(\mathrm{by} (\mathrm{ALPU5}))\hfill \\ \hfill & =u_{2n}\rho (y_n)(\mathrm{by} (\mathrm{ALPU9}))\hfill \\ \hfill & =\rho (u_{2n}{y}_n)(\mathrm{by} (\mathrm{ALPU3}))\hfill \\ \hfill & \le \rho (d)(\mathrm{by} \mathrm{zigzag} \mathrm{inequalities} (1) \mathrm{and} (\mathrm{ALPU4}))\hfill \\ \hfill & \le \rho (v_0{t}_1)(\mathrm{by} \mathrm{zigzag} \mathrm{inequalities} (2) \mathrm{and} (\mathrm{ALPU4}))\hfill \\ \hfill & =v_0\rho (t_1)(\mathrm{by} (\mathrm{ALPU3}))\hfill \\ \hfill & =\rho (v_0)\rho (t_1)(\mathrm{by} (\mathrm{ALPU9}))\hfill \\ \hfill & =v_0\lambda (\rho (t_1))(\mathrm{by} (\mathrm{ALPU5}))\hfill \\ \hfill & =v_0\rho (\lambda (t_1)).\hfill \end{array}$$

We obtain the inequality

$$u_{2n}\rho (\lambda (y_n))\le v_0\rho (\lambda (t_1)).$$

(18)

And

$$\begin{array}{cc}\hfill v_1\rho (\lambda (t_1))& =v_1\lambda (\rho (t_1))(\mathrm{by} (\mathrm{ALPU7}))\hfill \\ \hfill & =\rho (v_1)\rho (t_1)(\mathrm{by} (\mathrm{ALPU5}))\hfill \\ \hfill & =v_1\rho (t_1)(\mathrm{by} (\mathrm{ALPU9}))\hfill \\ \hfill & =\rho (v_1{t}_1)(\mathrm{by} (\mathrm{ALPU3}))\hfill \\ \hfill & \le \rho (v_2{t}_2)(\mathrm{by} \mathrm{zigzag} \mathrm{inequalities} (2) \mathrm{and} (\mathrm{ALPU4}))\hfill \\ \hfill & =v_2\rho (t_2)(\mathrm{by} (\mathrm{ALPU3}))\hfill \\ \hfill & =\rho (v_2)\rho (t_2)(\mathrm{by} (\mathrm{ALPU9}))\hfill \\ \hfill & =v_2\lambda (\rho (t_2))(\mathrm{by} (\mathrm{ALPU5}))\hfill \\ \hfill & =v_2\rho (\lambda (t_2))(\mathrm{by} (\mathrm{ALPU7})).\hfill \end{array}$$

This gives the inequality

$$v_1\rho (\lambda (t_1))\le v_2\rho (\lambda (t_2)).$$

(19)

Continuing like this, we have

$$v_{2j-1}\rho (\lambda (t_j))\le v_{2j}\rho (\lambda (t_{j+1})),\mathrm{where}1\le j\le m-1.$$

(20)

Finally, we have

$$\begin{array}{cc}\hfill v_{2m-1}\rho (\lambda (t_m))& =v_{2m-1}\lambda (\rho (t_m))(\mathrm{by} (\mathrm{ALPU7}))\hfill \\ \hfill & =\rho (v_{2m-1})\rho (t_m)(\mathrm{by} (\mathrm{ALPU5}))\hfill \\ \hfill & =v_{2m-1}\rho (t_m)(\mathrm{by} (\mathrm{ALPU9}))\hfill \\ \hfill & =\rho (v_{2m-1}{t}_m)(\mathrm{by} (\mathrm{ALPU3}))\hfill \\ \hfill & \le \rho (v_{2m})(\mathrm{by} \mathrm{zigzag} \mathrm{inequalities} (2) \mathrm{and} (\mathrm{ALPU4}))\hfill \\ \hfill & =v_{2m}(\mathrm{by} (\mathrm{ALPU9})).\hfill \end{array}$$

Therefore, we obtain the inequality

$$v_{2m-1}\rho (\lambda (t_m))\le v_{2m}.$$

(21)

Since U is LPU in $\rho (\lambda (S))$, it follows that all of $\rho (\lambda (y_1)),\rho (\lambda (y_2)),\dots ,\rho (\lambda (y_n))$ and $\rho (\lambda (t_1)),\rho (\lambda (t_2)),\dots ,\rho (\lambda (t_m))$ are in U.

Now,

$$\begin{array}{cc}\hfill d& =x_1{u}_0(\mathrm{by} \mathrm{zigzag} \mathrm{inequalities} (3))\hfill \\ \hfill & \le x_1{u}_1\rho (\lambda (y_1))(\mathrm{by} \mathrm{inequality} (15))\hfill \\ \hfill & \le x_2{u}_2\rho (\lambda (y_1))(\mathrm{by} \mathrm{zigzag} \mathrm{inequalities} (1))\hfill \\ \hfill & \le x_2{u}_3\rho (\lambda (y_2))(\mathrm{by} \mathrm{inequality} (16))\hfill \\ \hfill & ⋮\hfill \\ \hfill & \le x_i{u}_{2i-1}\rho (\lambda (y_i))\hfill \\ \hfill & \le x_{i+1}{u}_{2i}\rho (\lambda (y_i))(\mathrm{by} \mathrm{zigzag} \mathrm{inequalities} (1))\hfill \\ \hfill & \le x_{i+1}{u}_{2i+1}\rho (\lambda (y_{i+1}))(\mathrm{by} \mathrm{inequality} (17))\hfill \\ \hfill & ⋮\hfill \\ \hfill & \le x_n{u}_{2n-1}\rho (\lambda (y_n))\hfill \\ \hfill & \le u_{2n}\rho (\lambda (y_n))(\mathrm{by} \mathrm{zigzag} \mathrm{inequalities} (1))\hfill \\ \hfill & \le v_0\rho (\lambda (t_1))(\mathrm{by} \mathrm{inequality} (18)).\hfill \\ \hfill & \le s_1{v}_1\rho (\lambda (t_1))(\mathrm{by} \mathrm{zigzag} \mathrm{inequalities} (2))\hfill \\ \hfill & \le s_1{v}_2\rho (\lambda (t_2))(\mathrm{by} \mathrm{inequality} (19))\hfill \\ \hfill ⋮\\ \hfill & \le s_j{v}_{2j-1}\rho (\lambda (t_j))\hfill \\ \hfill & \le s_j{v}_{2j}\rho (\lambda (t_{j+1}))(\mathrm{by} \mathrm{inequality} (20))\hfill \\ \hfill & \le s_{j+1}{v}_{2j+1}\rho (\lambda (t_{j+1}))(\mathrm{by} \mathrm{zigzag} \mathrm{inequalities} (2))\hfill \\ \hfill ⋮\\ \hfill & \le s_m{v}_{2m-1}\rho (\lambda (t_m))\hfill \\ \hfill & \le s_m{v}_{2m}(\mathrm{by} \mathrm{inequality} (21))\hfill \\ \hfill & =d(\mathrm{by} \mathrm{zigzag} \mathrm{inequations} (4)).\hfill \end{array}$$

Thus, $v_0\rho (\lambda (t_1))\le d\le v_0\rho (\lambda (t_1))$ and, so $d=v_0\rho (\lambda (t_1))$. Since $\rho (\lambda (t_1))\in U$, it follows that $d\in U$ as required. □

Conversely, we can demonstrate the following.

Theorem 8.

Let U be a subpomonoid of a pomonoid S. If U is ARPU in S, then it is closed in S.

Thus, the next result is immediate.

Corollary 5.

Let U be a subpomonoid of a pomonoid S. If U is APU in S, then it is closed in S.

From Theorem 1 and Corollary 5, we can conclude the following.

Corollary 6.

The special pomonoid amalgam with an APU core U is strongly poembeddable.