A Verifiable Multi-Secret Sharing Scheme for Hierarchical Access Structure
Abstract
1. Introduction
- These are single secret sharing schemes.
- For every new secret, a new share has to be generated for every participant after the reconstruction of the previous secret
- Private channels are essential for the communication between dealers and participants and among the participants.
- These schemes are not capable of identifying the cheater.
- Efficiency through YCH Scheme Integration: Our proposed hierarchical secret sharing scheme leverages the YCH scheme at each level, optimizing computational resources and reducing the number of public values required.
- Enhanced Security with Cheater Detection: We introduce mechanisms to swiftly identify both malicious dealers and dishonest participants, ensuring the integrity of the secret-sharing process.
- Dual Functionality of Participant Shares: Participants’ shares in our scheme serve dual purposes: facilitating secret reconstruction and enabling verification processes, enhancing overall scheme flexibility.
- Secure Communication Independent Verification: Utilizing two-variable one-way functions, our scheme eliminates the dependency on secure channels for share transmission, ensuring robustness against interception.
2. Related Work
- It supports the hierarchical access structures as discussed in the first paragraph of the current section. It improves and enhances the applicability of the hierarchical access structure.
- It supports weighted threshold secret sharing as discussed in the second paragraph without any exponential operation and extra burden.
- It supports secret sharing with multi-stage, multi-level properties with verification of shares lacking in schemes discussed in the third and fourth paragraphs.
- In the last paragraph of this section, novel schemes are discussed. These schemes do not explain security features such as correctness and forward/backward secrecy.
3. Preliminaries/Foundations
3.1. Polynomial Interpolation
- Polynomial can be utilized to plot a curve using the points that satisfy the same polynomial.
- We can determine polynomials of a fixed degree by knowing the points that satisfy the polynomials. The number of such points must be at least one greater than the degree of polynomial.
3.2. Shamir’s () Secret Sharing Scheme
- Learning of t or more pieces makes S uniquely determined.
- Learning of any or fewer pieces leaves S totally unpredictable.
- At least 2 points are necessary to draw a line. (i.e., one point is not sufficient)
- At least 3 points are necessary to draw a parabola (i.e., less than 3 points are not sufficient)
- Similarly, it takes at least ‘t’ points to draw a polynomial of degree ‘’ (i.e., less than t points are not sufficient).
- Select a prime number Q
- Randomly select a function
- Compute (i, g(i)) corresponding to the ith shareholder,
- These points (i, g(i)) are distributed securely to n shareholders/participants
- Compute Lagrange’s interpolating polynomial using t shares
- In this way, we obtain the polynomial in the formwhere = S is the secret.
3.3. Hierarchical Access Structure
3.4. Overview of YCH Scheme
- -scheme, where t is for the threshold and n is for the number of participants.
- denotes the k secrets to be shared.
- n secret shadows are randomly chosen by the dealer and distributed to the participants through a secure channel.
- A random value ‘r’ is chosen.
- A 2-variable 1-way function h(r,), . is chosen.
- (Number of secrets is less than the threshold)
- (a)
- A prime number ‘Q’ is chosen by the dealer.
- (b)
- The dealer choose a polynomial mod Q. Degree of polynomial is where,
- (c)
- are the secrets to be shared and are random numbers.
- (d)
- (e)
- For every ith participant the dealer computes mod Q
- (f)
- Publish .
- (Number of secrets is greater than the threshold)
- (a)
- A prime number Q and a polynomial are chosen by the dealer. The degree of the polynomial is . Where are the secrets.
- (b)
- For i = 1 to n. is computed.
- (c)
- For . is computed
- (d)
- calculated values like are publicly published.
- (e)
- are also published in public.
- Each participant uses his/her share to compute (for i = 1 to t)
- The polynomial is determined as follows:
- (Number of secrets is less than the threshold)
- For (Number of secrets is greater than the threshold)
- From the above equations, we get the secrets
3.5. 2-Variable 1-Way Function
- when r and s are given is easily computable. But for a given s and , it is very difficult to compute r.
- It is hard to compute when there is no knowledge of s.
- For the given s, it is hard to find two different values and that satisfy the situation .
- It is tough to compute s, for the given r and .
- If we have pairs of r and , it is difficult to find for which .
4. Identification of Problem and Motivation
Contribution
- Efficient Parallel Reconstruction: The YCH scheme allows for parallel reconstruction of multiple secrets, enhancing efficiency in scenarios requiring simultaneous access to different shared secrets.
- Dynamic Distribution of Secrets: Our scheme supports dynamic determination of the number of secrets to be distributed, providing flexibility in managing shared information.
- Optimized Resource Utilization: It minimizes storage requirements and computing time by utilizing fewer public values, making it more efficient compared to traditional schemes.
- Cheater Identification: Any participant can identify dishonest behavior, whether from the dealer or other participants, ensuring the integrity of the sharing process.
- Secure Communication Elimination: There is no dependency on secure channels between the dealer and participants for share transmission, simplifying implementation and reducing overhead.
- Invalid Share Detection: The scheme includes mechanisms to detect and reject invalid shares, enhancing overall security against fraudulent activities.
5. Proposed Scheme
5.1. Overview
5.2. Initialization
- Number of participants is n.
- The number of levels is m. They are .
- Each level is associated with a , access structure.
- Dealer chooses n shares , , .
5.3. Distribution
- The number of secrets k is less than the threshold
- A number of secrets k is more than the threshold.
- (number of secret is less than or equal to )
- (a)
- A prime number Q is chosen by the dealer.
- (b)
- The dealer constructs polynomial mod Q. The degree of the polynomial is (). Let,where are the secrets,
- (c)
- , are randomly chosen numbers.
- (d)
- For the ith participant the dealer computes , .
- (e)
- Publish .
- (Number of secrets is greater than ).
- (a)
- A prime number Q is chosen.
- (b)
- Dealer constructs a polynomial of degree . Letwhere are the secrets.
- (c)
- For . is Computed.
- (d)
- For . is Computed
- (e)
- calculated values like are made public
- (f)
- are published in public.
- Calculate actual share () and pseudo share () for the ith participant of level l using the following formulas:where g is a one-way function in which denotes the share of th participant of level l
- Calculate the actual share () and pseudo share () of the ith participant of upper level u using the formula:where is the identifier for ith element of uth level.
- and (if needed) are distributed to each participant using a secure channel.
- All , r values are published.
5.4. Reconstruction
- for
- for
5.5. Verification
- 1.
- Pseudo share is distributed to participants securely by the dealer.
- 2.
- Each ith participant uses his actual share () in a two-variable one-way function with random variable r. Let that two-way variable function be .
- 3.
- Calculated values made public.
- 4.
- Public share is also published.
- 5.
- Each participant computes the actual share of each participant
- 6.
- Using that actual share, is calculated for participant.
- 7.
- If is equal to the already public value of then the participant share is valid; otherwise, the actual share of the participant is not valid.
- 8.
- In a similar way, an individual participant will be able to check the legitimacy of his/her share given by the dealer.
5.6. Example
5.6.1. Distribution
5.6.2. Reconstruction
- A particular level (here level 2) has a sufficient number of participants.
- A particular level has fewer participants than the upper-level participant and takes part in the reconstruction of the secret.
6. Security and Performance Analysis
6.1. Adversary Model
- Insider Adversary, these are legitimate shareholders who acquired shares from the dealer.
- Outsider Adversary, the external adversary is an attacker who does not own any of the dealer’s shares but may try to gain unauthorized access.
6.2. Security Analysis
6.2.1. Formal Security Analysis (Random Oracle Model)
- We assume the proposed scheme as the set of tuples, where Int stands for the initialization/setup phase, dist stands for distribution and Rec is for the recovery phase.
- PP (Public parameters) =
- p: prime number p > n such that p is at least bits long
- H: a hash function,
- : Set of participants
- t: Threshold
- There is an adversary containing a set of participants and threshold value t.
6.2.2. Informal Security Analysis
- Correctness: In the verification phase, each participant computes the actual share of other participants using that actual share, is calculated for th participant. If is equal to the already public value of then the participant share is valid otherwise the actual share of the participant is not valid.
- Forward secrecy: keys like can only be computed or stored by members of the closed communication group; if a member leaves the group, the departing member will be unable to access the content of future conversations.
- Perfectness: We use Shamir’s (t, n) secret sharing scheme for share distribution at each level in the proposed scheme. It is well known that fewer than t participants in Shamir’s (t, n) secret-sharing scheme cannot reconstruct the secret. Hence, our scheme is also perfect.
- Fairness of secret sharing One desirable quality in secret sharing is Fairness, which indicates that if one member obtains the secret, the other participants are not harmed. Halpern and Teague [53] were the first to offer rational cryptographic protocols in 2004. They pointed out that any method for reassembling secrets with a well-known upper constraint on the running duration is unstable and that parties will not submit anything in the final round since they have no reason to do so because the other participant does. Regrettably, earlier secret-sharing systems necessitated numerous rounds with high overheads. Zhang et al. [26,27] explain the scheme. Fairness but leave out the access mechanism. To summarize, existing fairness schemes necessitate a trusted third party or many rounds of communication. The proposed scheme provides Fairness without the dependency on a third party and extra overheads in communication.
- Freshness of keys: In the proposed scheme, all the exchangeable values depend upon the random bi-variate polynomial. It makes pseudo-share, and shares are always fresh. Hence, it is impossible to impersonate a member by recording a previously used key.
- Eavesdropping attack In the proposed scheme, each ith participant uses his actual share () in a two-variable one-way function with random variable r which makes it independent of public parameters. Therefore, an adversary will not be able to know any secret information from communication parameters. So, the proposed scheme is safe from eavesdropping activities.
- Cheating identification In the proposed scheme, an individual participant can check the legitimacy of his/her share given by the dealer. Thus, the proposed scheme provides cheating identification.
- Verifiability In the reconstruction phase, participants can verify shares received from other participants and dealers. On the other hand, a dealer can also verify the participant’s shares. Thus, the proposed scheme provides verifiability.
- Unconditional security While exploring the security analysis, both types of adversaries are considered with their capacity to the full extent.
6.3. Performance Analysis
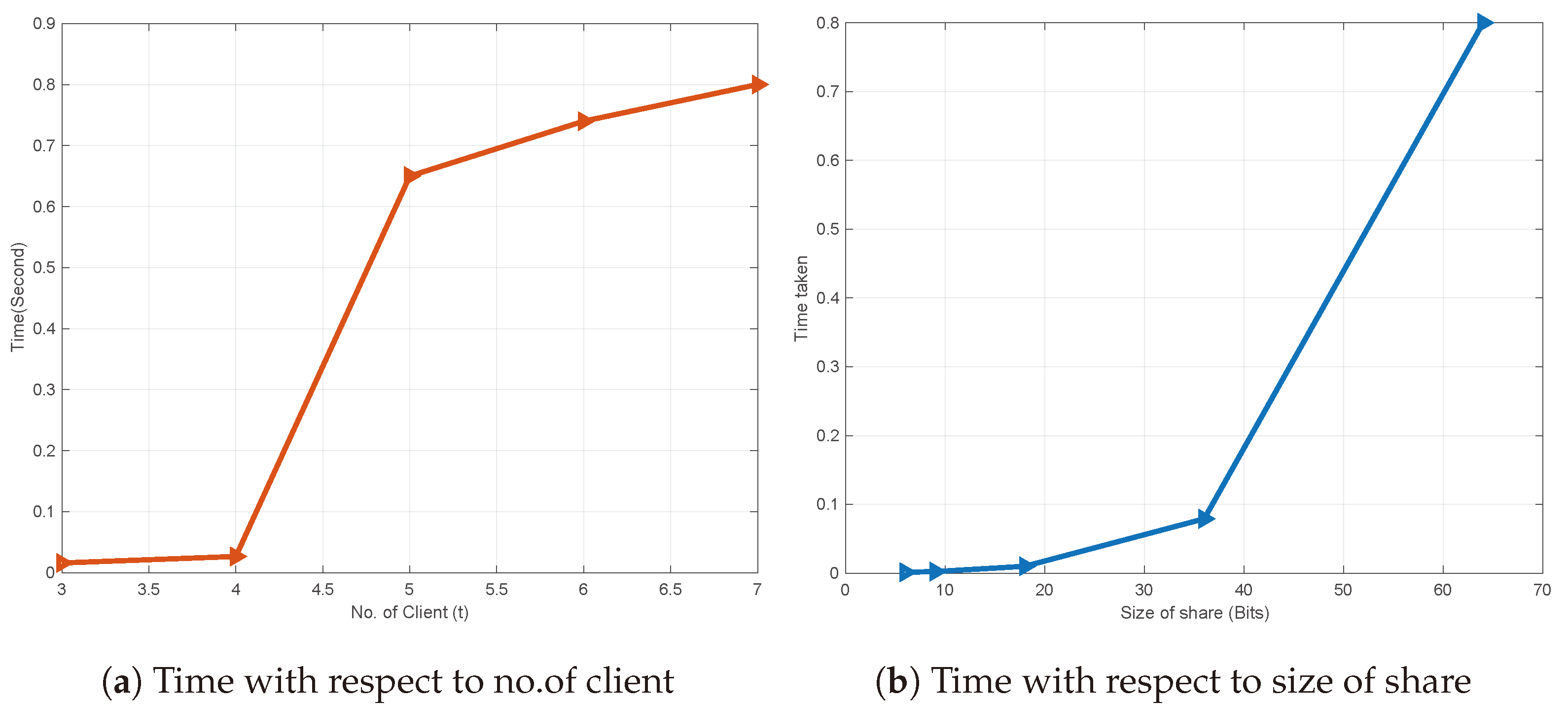
6.3.1. Evaluation of Stealth Share
6.3.2. Computational Cost
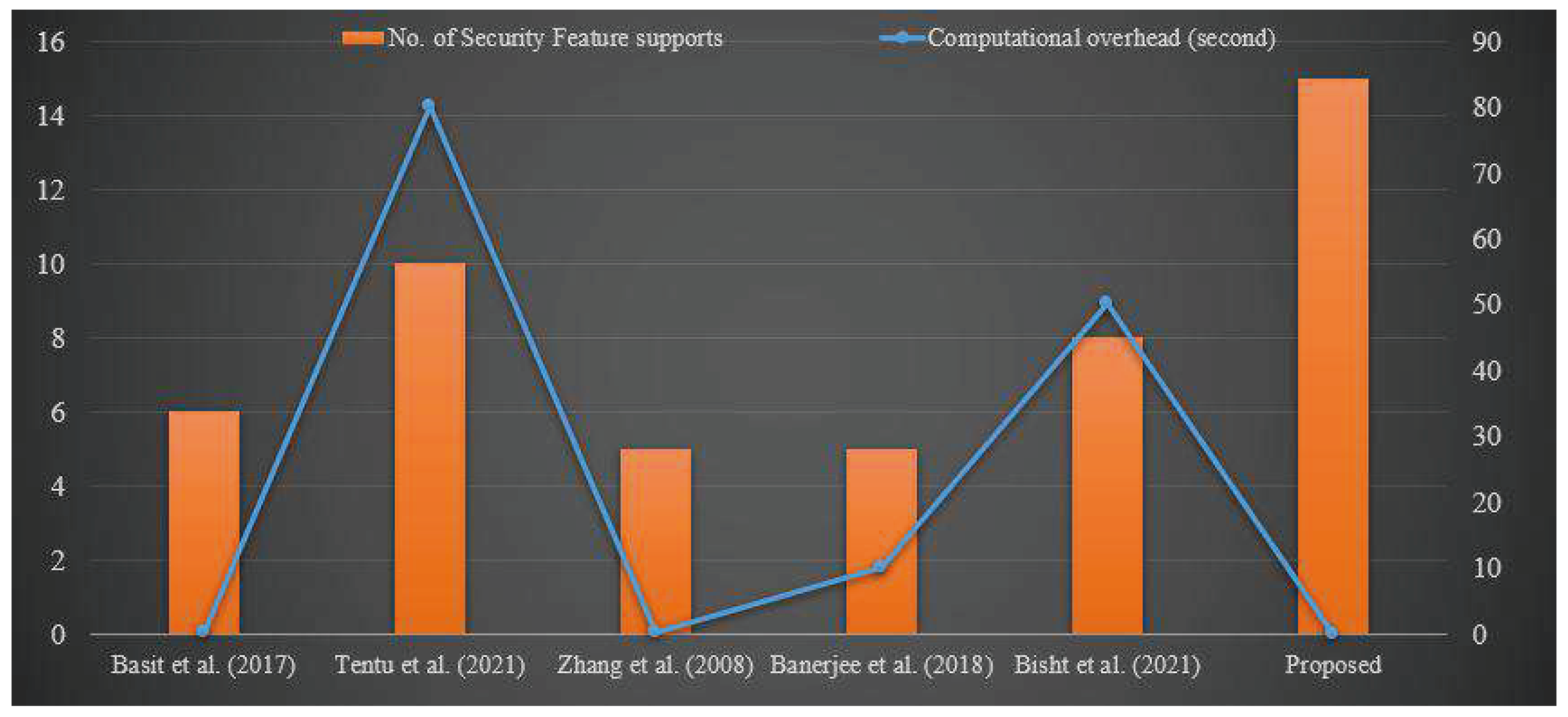
7. Comparison with Existing Schemes
8. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Gutte, V.S.; Parasar, D. Sailfish invasive weed optimization algorithm for multiple image sharing in cloud computing. Int. J. Intell. Syst. 2022, 37, 4190–4213. [Google Scholar] [CrossRef]
- Wang, X.; Zhang, X.; Zu, C.; Yang, Z.; Bian, G.; Zhang, Y.; Ruan, W.; Wu, B.; Wu, X.; Yuan, L. An accurate cloud-based indoor localization system with low latency. Int. J. Intell. Syst. 2022, 37, 4794–4809. [Google Scholar] [CrossRef]
- Ren, P.; Li, F.; Wang, Y.; Zhou, H.; Liu, P. Ipsadas: Identity-privacy-aware secure and anonymous data aggregation scheme. Int. J. Intell. Syst. 2022, 37, 5290–5324. [Google Scholar] [CrossRef]
- Bufalo, M.; Bufalo, D.; Orlando, G. Some properties of the computation of the modular inverse with applications in cryptography. Computation 2023, 11, 70. [Google Scholar] [CrossRef]
- Chen, H.-Y.; Wu, Z.-Y.; Chen, T.-L.; Huang, Y.-M.; Liu, C.-H. Security privacy and policy for cryptographic based electronic medical information system. Sensors 2021, 21, 713. [Google Scholar] [CrossRef]
- Hazzazi, M.M.; Attuluri, S.; Bassfar, Z.; Joshi, K. A novel cipher-based data encryption with galois field theory. Sensors 2023, 23, 3287. [Google Scholar] [CrossRef]
- Hernández-Álvarez, L.; Bullón, Pérez, J.J.; Batista, F.K.; Queiruga-Dios, A. Security threats and cryptographic protocols for medical wearables. Mathematics 2022, 10, 886. [Google Scholar] [CrossRef]
- Jara-Vera, V.; Sánchez-Ávila, C. Some notes on a formal algebraic structure of cryptology. Mathematics 2021, 9, 2183. [Google Scholar] [CrossRef]
- Masood, F.; Ahmad, J.; Shah, S.A.; Jamal, S.S.; Hussain, I. A novel hybrid secure image encryption based on julia set of fractals and 3d lorenz chaotic map. Entropy 2020, 22, 274. [Google Scholar] [CrossRef]
- Rauf, A.; Wang, Z.; Sajid, H.; Ali Tahir, M. Secure route-obfuscation mechanism with information-theoretic security for internet of things. Sensors 2020, 20, 4221. [Google Scholar] [CrossRef]
- Richter, M.; Bertram, M.; Seidensticker, J.; Tschache, A. A mathematical perspective on post-quantum cryptography. Mathematics 2022, 10, 2579. [Google Scholar] [CrossRef]
- Tito-Corrioso, O.; Borges-Quintana, M.; Borges-Trenard, M.A.; Rojas, O.; Sosa-Gómez, G. On the fitness functions involved in genetic algorithms and the cryptanalysis of block ciphers. Entropy 2023, 25, 261. [Google Scholar] [CrossRef]
- Shamir, A. How to share a secret. Commun. ACM 1979, 22, 612–613. [Google Scholar] [CrossRef]
- Blakley, G.R. Safeguarding cryptographic keys. In Proceedings of the 1979 International Workshop on Managing Requirements Knowledge (MARK), New York, NY, USA, 4–7 June 1979; IEEE Computer Society: New York, NY, USA, 1979; pp. 313–318. [Google Scholar]
- Simmons, G.J. How to (really) share a secret. In Conference on the Theory and Application of Cryptography; Springer: New York, NY, USA, 1988; pp. 390–448. [Google Scholar]
- Asmuth, C.; Bloom, J. A modular approach to key safeguarding. IEEE Trans. Inf. Theory 1983, 29, 208–210. [Google Scholar] [CrossRef]
- He, J.; Dawson, E. Multistage secret sharing based on one-way function. Electron. Lett. 1994, 30, 1591–1592. [Google Scholar] [CrossRef]
- He, J.; Dawson, E. Multisecret-sharing scheme based on one-way function. Electron. Lett. 1995, 31, 93–95. [Google Scholar] [CrossRef]
- Chien, H.Y.; Jan, J.K.; Tseng, Y.M. A practical (t, n) multi-secret sharing scheme. IEICE Trans. Fundam. Electron. Commun. Comput. Sci. 2000, 83, 2762–2765. [Google Scholar]
- Choc, B.; Goldwasser, S.; Micali, S.; Awerbuch, B. Verifiable secret sharing and achieving simultaneity in the presence of faults. In Annual Symposium on Foundations of Computer Science (Proceedings); Elsevier: Amsterdam, The Netherlands, 1985; pp. 383–395. [Google Scholar]
- Tompa, M.; Woll, H. How to share a secret with cheaters. J. Cryptol. 1989, 1, 133–138. [Google Scholar] [CrossRef]
- Harn, L.; Hsu, C.-F. (t, n) Multi-secret sharing scheme based on bivariate polynomial. Wirel. Pers. Commun. 2017, 95, 1495–1504. [Google Scholar] [CrossRef]
- Harn, L.; Hsu, C.-F.; Xia, Z.; Zhou, J. How to share secret efficiently over networks. Secur. Commun. Netw. 2017, 2017, 5437403. [Google Scholar] [CrossRef]
- Ballico1, E.; Boato, G.; Fontanari, C.; Granelli, F. Hierarchical secret sharing in ad hoc networks through birkhoff interpolation. In Advances in Computer, Information, and Systems Sciences, and Engineering: Proceedings of IETA 2005, TeNe 2005, EIAE 2005; Springer: Dordrecht, The Netherlands, 2006; pp. 157–164. [Google Scholar]
- Ma, C.; Cheng, R. Key management based on hierarchical secret sharing in ad-hoc networks. In Information Security and Cryptology: Third SKLOIS Conference, Inscrypt 2007, Xining, China, August 31–September 5, 2007, Revised Selected Papers 3; Springer: Berlin/Heidelberg, Germany, 2008; pp. 182–191. [Google Scholar]
- Zhang, E.; Li, M.; Yiu, S.-M.; Du, J.; Zhu, J.-Z.; Jin, G.-G. Fair hierarchical secret sharing scheme based on smart contract. Inf. Sci. 2021, 546, 166–176. [Google Scholar] [CrossRef]
- Zhang, Y.; Liu, Z.; Huang, G. Sure interpolation and its application to hierarchical threshold secret sharing scheme. In Proceedings of the 2008 International Symposium on Computer Science and Computational Technology, Shanghai, China, 20–22 December 2008; IEEE: Piscataway, NJ, USA, 2008; Volume 1, pp. 447–450. [Google Scholar]
- Yang, C.-C.; Chang, T.-Y.; Hwang, M.-S. A (t, n) multi-secret sharing scheme. Appl. Math. Comput. 2004, 151, 483–490. [Google Scholar] [CrossRef]
- Blundo, C.; De Santis, A.; Stinson, D.R.; Vaccaro, U. Graph decompositions and secret sharing schemes. J. Cryptol. 1995, 8, 39–64. [Google Scholar] [CrossRef]
- Padró, C.; Sáez, G. Secret sharing schemes with bipartite access structure. IEEE Trans. Inf. Theory 2000, 46, 2596–2604. [Google Scholar]
- Tentu, A.N.; Bhavani, K.; Basit, A.; Venkaiah, V.C. Sequential (t, n) multi secret sharing scheme for level-ordered access structure. Int. J. Inf. Technol. 2021, 13, 2265–2275. [Google Scholar] [CrossRef]
- Brickell, E.F. Some ideal secret sharing schemes. In Workshop on the Theory and Application of of Cryptographic Techniques; Springer: Berlin/Heidelberg, Germany, 1989; pp. 468–475. [Google Scholar]
- Lin, C.; Harn, L.; Yea, D. Ideal hierarchical (t, n) secret sharing schemes. In Proceedings of the Fifth International Conference on Information Assurance and Security (IAS09), Xi’an, China, 18–20 August 2009; Citeseer: Princeton, NJ, USA, 2009. [Google Scholar]
- Chanu, O.B.; Tentu, A.N.; Venkaiah, V.C. Multi-stage multi-secret sharing schemes based on chinese remainder theorem. In Proceedings of the 2015 International Conference on Advanced Research in Computer Science Engineering & Technology (ICARCSET 2015), Unnao, India, 6–7 March 2015; pp. 1–6. [Google Scholar]
- Basit, A.; Kumar, N.C.; Venkaiah, V.C.; Moiz, S.A.; Tentu, A.N.; Naik, W. Multi-stage multi-secret sharing scheme for hierarchical access structure. In Proceedings of the 2017 International Conference on Computing, Communication and Automation (ICCCA), Greater Noida, India, 5–6 May 2017; IEEE: Piscataway, NJ, USA, 2017; pp. 557–563. [Google Scholar]
- Banerjee, S.; Gupta, D.S.; Biswas, G. Hierarchy-based cheating detection and cheater identification in secret sharing schemes. In Proceedings of the 2018 4th International Conference on Recent Advances in Information Technology (RAIT), Dhanbad, India, 15–17 March 2018; IEEE: Piscataway, NJ, USA, 2018; pp. 1–6. [Google Scholar]
- Liu, Y.; Zhang, F.; Zhang, J. Attacks to some verifiable multi-secret sharing schemes and two improved schemes. Inf. Sci. 2016, 329, 524–539. [Google Scholar] [CrossRef]
- Yuan, J.; Yang, J.; Wang, C.; Jia, X.; Fu, F.-W.; Xu, G. A new efficient hierarchical multi-secret sharing scheme based on linear homogeneous recurrence relations. Inf. Sci. 2022, 592, 36–49. [Google Scholar] [CrossRef]
- Bisht, K.; Deshmukh, M. A novel approach for multilevel multi-secret image sharing scheme. J. Supercomput. 2021, 77, 12157–12191. [Google Scholar] [CrossRef]
- Prashanti, G.; Bhat, M.N. Cheating identifiable polynomial based secret sharing scheme for audio and image. Multimed. Tools Appl. 2024, 83, 403–423. [Google Scholar] [CrossRef]
- Chattopadhyay, A.K.; Saha, S.; Nag, A.; Nandi, S. Secret sharing: A comprehensive survey, taxonomy and applications. Comput. Sci. Rev. 2024, 51, 100608. [Google Scholar] [CrossRef]
- Kamal, A.A.A.M.; Iwamura, K. Privacy preserving multi-party multiplication of polynomials based on (k, n) threshold secret sharing. ICT Express 2023, 9, 875–881. [Google Scholar] [CrossRef]
- Liu, C.; Min, S.; Pang, Y.; Chai, Y. The meshfree radial point interpolation method (RPIM) for wave propagation dynamics in non-homogeneous media. Mathematics 2023, 11, 523. [Google Scholar] [CrossRef]
- Chen, C.S.; Noorizadegan, A.; Young, D.L.; Chen, C.S. On the selection of a better radial basis function and its shape parameter in interpolation problems. Appl. Math. Comput. 2023, 442, 127713. [Google Scholar] [CrossRef]
- Dupac, M. Mathematical modeling and simulation of the inverse kinematic of a redundant robotic manipulator using azimuthal angles and spherical polar piecewise interpolation. Math. Comput. Simul. 2023, 209, 282–298. [Google Scholar] [CrossRef]
- Sauer, T.; Xu, Y. On multivariate Lagrange interpolation. Math. Comput. 1995, 64, 1147–1170. [Google Scholar] [CrossRef]
- Nevai, P. Mean convergence of Lagrange interpolation. III. Trans. Am. Math. Soc. 1984, 282, 669–698. [Google Scholar] [CrossRef]
- Gautschi, W. On mean convergence of extended Lagrange interpolation. J. Comput. Appl. Math. 1992, 43, 19–35. [Google Scholar] [CrossRef]
- Herranz, J.; Sáez, G. New results on multipartite access structures. IEE Proc.-Inf. Secur. 2006, 153, 153–162. [Google Scholar] [CrossRef]
- Dolev, D.; Yao, A. On the security of public key protocols. IEEE Trans. Inf. Theory 1983, 29, 198–208. [Google Scholar] [CrossRef]
- Herranz, J.; Ruiz, A.; Sáez, G. New results and applications for multi-secret sharing schemes. Des. Codes Cryptogr. 2014, 73, 841–864. [Google Scholar] [CrossRef]
- Mashhadi, S. Toward a formal proof for multi-secret sharing in the random oracle model. Inf. Secur. J. A Glob. Perspect. 2020, 29, 244–249. [Google Scholar] [CrossRef]
- Halpern, J.; Teague, V. Rational secret sharing and multiparty computation. In Proceedings of the Thirtysixth Annual ACM Symposium on Theory of Computing, Chicago, IL, USA, 13–16 June 2004; pp. 623–632. [Google Scholar]
- Li, C.; Dong, M.; Xin, X.; Li, J.; Chen, X.B.; Ota, K. Efficient privacy-preserving in IoMT with blockchain and lightweight secret sharing. IEEE Internet Things J. 2023, 10, 22051–22064. [Google Scholar] [CrossRef]
- Buchanan, W.J. Shamir Secret Sharing (SSS). Asecuritysite.com. 2024. Available online: https://asecuritysite.com/shares (accessed on 30 April 2024).
- Wu, S.; Hsu, C.; Xia, Z.; Zhang, J.; Wu, D. Symmetric-bivariate-polynomial-based lightweight authenticated group key agreement for industrial internet of things. J. Internet Technol. 2020, 21, 1969–1979. [Google Scholar]
- Knuth, D.E. The Art of Computer Programming; Pearson Education: London, UK, 2005. [Google Scholar]
- Security and So Many Things—Asecuritysite.com. Available online: https://asecuritysite.com/ (accessed on 30 April 2024).
- Chen, Q.; Tang, C.; Lin, Z. Efficient explicit constructions of multipartite secret sharing schemes. IEEE Trans. Inf. Theory 2021, 68, 601–631. [Google Scholar] [CrossRef]
- SageMath. Use SageMath Online—Cocalc.com. Available online: https://cocalc.com/features/sage (accessed on 30 April 2024).
| Existing Literature | Main Methodology | Positive Aspects | Weaknesses |
|---|---|---|---|
| Basit et al. [35], Chanu et al. [34] | Multi-stage, Multi-level Secret Sharing | Supports complex hierarchical access with verification of shares. | Issues with fairness and perfectness (Bisht [39]); lacks complete security features. |
| Blundo et al. [29] | Graph-based Access Structure | Provides flexible access control based on graph relationships. | Specific to graph-based scenarios; may not generalize well. |
| Pardo et al. [30] | Bipartite Access Structure | Simplifies access control into two distinct groups. | Limited to scenarios with clear two-part division. |
| Tentu et al. [31] | Multipartite Access Structure | Allows for multiple subsets with varied access rights. | Complexity increases with the number of subsets. |
| Shamir et al. [13] | Weighted Threshold Secret Sharing | Incorporates weighted shares for different participant capabilities. | Inefficient due to multiple shares per participant. |
| Shamir et al. [13], Blakley et al. [14] | Hierarchical Secret Sharing | Supports hierarchical access structures; applicable to groups with uniform privileges. | Lacks fairness in hierarchical structure (Banerjee [36]); inefficient with non-singular matrices (Brickell [32]). |
| Banerjee et al. [36], Bisht et al. [39] | Novel Schemes | Introduces efficient schemes with homogenous recurrence relations. | Often lacks security features like correctness and backward secrecy. |
| Comparison (Security) among the Existing Scheme | ||||||
|---|---|---|---|---|---|---|
| Property | Basit et al. [35] | Banerjee et al. [36] | Zhang et al. [26] | Tentu et al. [31] | Chen et al. [59] | Proposed Scheme |
| Hierarchy | Yes | Yes | No | Yes | Yes | yes |
| Reusable | Yes | Yes | Yes | No | No | Yes |
| Multi-secret | Multi | Multi | Multi | Single | Single | Multi |
| Based on | LPI | LPI | YCH | LPI | Polynomial | YCH |
| Ideal and Perfect | Yes | Yes | Yes | Yes | Yes | Yes |
| Cheating detection | partially | Conditional | Complete | Partially | Complete | Compete |
| Correctness | No | No | No | No | yes | yes |
| Forward secrecy | No | No | No | No | No | yes |
| Fairness | No | No | Yes | No | yes | yes |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Alam, I.; Alali, A.S.; Ali, S.; Asri, M.S.M. A Verifiable Multi-Secret Sharing Scheme for Hierarchical Access Structure. Axioms 2024, 13, 515. https://doi.org/10.3390/axioms13080515
Alam I, Alali AS, Ali S, Asri MSM. A Verifiable Multi-Secret Sharing Scheme for Hierarchical Access Structure. Axioms. 2024; 13(8):515. https://doi.org/10.3390/axioms13080515
Chicago/Turabian StyleAlam, Irfan, Amal S. Alali, Shakir Ali, and Muhammad S. M. Asri. 2024. "A Verifiable Multi-Secret Sharing Scheme for Hierarchical Access Structure" Axioms 13, no. 8: 515. https://doi.org/10.3390/axioms13080515
APA StyleAlam, I., Alali, A. S., Ali, S., & Asri, M. S. M. (2024). A Verifiable Multi-Secret Sharing Scheme for Hierarchical Access Structure. Axioms, 13(8), 515. https://doi.org/10.3390/axioms13080515







