A Proportional–Integral Observer-Based Dynamic Event-Triggered Consensus Protocol for Nonlinear Positive Multi-Agent Systems

Abstract

This paper investigates the state estimation and event-triggered control for positive nonlinear multi-agent systems. Firstly, a proportional–integral observer is established to estimate the states of the considered nonlinear positive multi-agent systems based on the matrix decomposition method. Then, a dynamic event-triggered mechanism is constructed, and a control protocol is proposed based on the proportional–integral observer and event-triggered mechanism. By combining linear programming with linear co-positive Lyapunov functions, the considered multi-agent systems are guaranteed to be positive and achieve consensus. Moreover, by introducing three new variables and a finite vector, the final convergence point can be changed based on the given vector. Finally, two illustrative examples demonstrate the validity of the proposed theoretical results.

1. Introduction

A massive amount of research has been conducted in recent decades on multi-agent systems (MASs) due to their enormous potential in a wide range of fields, such as energy management [1], manufacturing systems [2], robotics [3], and sensor networks [4]. As a special subclass of MASs, the so-called positive multi-agent systems (PMASs) are also widely applied in specific domains where the variables are typically non-negative, including greenhouse monitoring [5], multiple vehicle systems [6], epidemic transmission processes [7], etc. Like MASs, the consensus problem is also the fundamental task of PMASs, which involves all agents converging to a common state or reaching an agreement through local interactions. There are numerous studies on the consensus issue of general MASs from different research directions, including finite-time consensus [8], leaderless consensus [9], leader-following consensus [10], event-triggered consensus [11], cluster consensus [12], etc. However, when dealing with the consensus problem of PMASs, the existing results on general MASs cannot be applied directly since the positivity constraint must be taken into consideration.

It should be noted that the energy resources of each individual agent are often limited, which conflicts with the need for frequent communication between agents. In contrast with the traditional periodic (or time-triggered) control, which is widely used in most existing research on the consensus of MASs, the event-triggered strategy has attracted increasing attention due to its low communication burden [13]. A review of the development of event-triggered control can be found in the paper [14]. Comparisons between event-triggered and periodic strategies were conducted in [15,16], and the results have shown that the event-triggered mechanism offers some advantages over the time-triggered mechanism. The work in [17] addressed the problem of stabilizing embedded processor control tasks by introducing an event-triggered strategy, wherein control actuation is only triggered when the predefined error threshold is exceeded. The study [18] proposed a combined measurement approach named distributed event-triggered control such that the control only happens when each agent’s own threshold is triggered. Recently, the dynamic event-triggered mechanism has been adapted to handle the output consensus problem of heterogeneous linear multi-agent systems with a directed communication topology, as in [19]. As a result of the work [20], a gain-scheduled state observer and a switching threshold event-triggered strategy were developed to handle the output feedback consensus tracking control for nonlinear MASs with sensor failures. Despite significant advancements in event-triggered strategies for general and linear MASs, there is still a lack of research when it comes to nonlinear PMASs. The positivity constraints increase the difficulty, making it challenging to directly apply existing results. Additionally, nonlinearity is very common in practical situations. This paper explores dynamic event-triggered control strategies specifically designed for nonlinear PMASs to address these challenges.

On the other hand, research on PMASs is mostly predicated on the assumption that the state of the systems is measurable. However, state data sometimes may be challenging to measure due to technical limitations or high costs in practice. To handle this issue, observers have been introduced to estimate the states of agents. In the existing literature, the proportional observer (Luenberger observer) [21] has gained significant attention in the past few decades, with numerous research achievements. A distributed observer has been designed for a class of leader-following nonlinear multi-agent systems in [22]; the designed observer was cooperative with a distributed control law to solve the consensus problem of nonlinear autonomous systems. The work in [23] proposed an intermediate observer to estimate the system states, actuator faults, and sensor faults of nonlinear MASs with actuator faults and sensor faults. Consider the time-varying and non-uniform input delay: The literature [24] constructs a finite-time distributed observer to estimate the state information of each follower in high-order nonlinear MASs. Benefiting from the extra integral terms in its design structure, the proportional–integral observer (PIO) [25] strategy can utilize both historical and current information. This provides the PIO the capability to increase the degrees of freedom for parameter selection, reducing the sensitivity of parameters variations and improving the dynamic performance. The research conducted by [26] proposed a type of generalized PIO observer for descriptor linear systems; the designed observer possesses the separation property, which is an excellent attribute for observers. Taking the faults and unknown inputs into consideration for linear descriptor systems, a proportional–multi-integral observer was designed in [27]; the proposed observer has good robustness to the variations of the parameters. The authors in [28] proposed a state estimation method in the form of a PIO to handle the mask of online battery states’ estimation. The PIO can also be applied in machine learning to estimate the states of recurrent neural networks [29]. In [30], the PIO was utilized to make sure that the dynamics of Markov switching memristive neural networks has stochastic finite-time boundedness while meeting the desired $H_{\infty }$ performance index. However, to the best of our knowledge, the design problem of the PIO for PMASs has not yet been studied. This constitutes the primary motivation for our research, as we aim to develop and investigate effective PIO design strategies specifically tailored to PMASs.

While significant advancements have been made in the field of event-triggered control strategies and observer design for general (non-positive) and linear MASs, the specific challenges and complexities associated with nonlinear PMASs remain largely unexplored. This issue is particularly important given the prevalence of nonlinearity in practical applications and the unique difficulties introduced by positivity constraints. Addressing these challenges requires novel approaches and innovative solutions, which this paper aims to provide.

Motivated by the above-mentioned considerations, this paper investigates the PIO-based dynamic event-triggered consensus protocol of certain types of nonlinear PMASs. First, a positive PIO is constructed to estimate the states of the considered nonlinear PMASs. Then, an event-triggered mechanism is established, and a control protocol is proposed based on the designed PIO and event-triggered mechanism. Using a linear programming approach and co-positive Lyapunov functions, the designed control protocol can guarantee the positivity and consensus of nonlinear PMASs. Moreover, by introducing three new variables, the final convergence point can be aligned depending on a given vector rather than converging to a zero point. The main contributions of this paper include the following: (i) the innovative construction of a positive PIO for nonlinear PMASs; (ii) the development of a new PIO-based dynamic event-triggered protocol framework, which effectively manages positivity constraints and nonlinearity; (iii) the increased flexibility of the convergence point achieved by introducing new variables, enhancing the practical relevance of our approach.

This paper is organized as follows: Section 2 introduces some preliminary thoughts about positive systems and graph theory. Section 3 presents the main results of positive proportional–integral observer design and observer-based dynamic event-triggered control synthesis. Two illustrative examples are given to verify the proposed theoretical results in Section 4. Section 5 concludes the paper with some discussion on potential future research directions.

Notation 1.

As stated in this paper, $\mathbb{R}$ refers to real numbers, ${\mathbb{R}}^n$ indicates n-dimensional vectors, and ${\mathbb{R}}^{m\times n}$ designates the space of $m\times n$ matrices, respectively. The ith row and jth column entry of matrix A are denoted by $A^{\left(ij\right)}$. Specify $I_n$ to denote an identity matrix of order n. An nth-order vector with all elements being 1 is denoted by ${\mathbf{1}}_n$, and ${\mathbf{1}}_n^{\left(i\right)}$ means the column vector with the ı-th element being 1 and other entries all being 0, i.e., ${\mathbf{1}}_n={(\underset{n}{\underbrace{1,1,\cdots ,1}})}^{\top }$ and ${\mathbf{1}}_n^{\left(i\right)}={(\underset{i-1}{\underbrace{0,\cdots ,0}},1,\underset{n-i}{\underbrace{0,\cdots ,0}})}^{\top }$. For a given vector $v\in {\mathbb{R}}^n$, the i-th element is represented by $v_i$, and $v\succ 0(⪰0)$ means that $v_i>0(\ge 0),i=1,2,\cdots ,n$. ${\nu }_{i_{\iota }}$ represents the ı-th element of the i-th vector in the vector set $\nu =\{{\nu }_1,{\nu }_2,\cdots ,{\nu }_n\}$, where ı specifies the position within the vector ${\nu }_i$. Consider a matrix $A\in {\mathbb{R}}^{p\times q},A\succ 0(⪰0)$ meaning that $a_{ij}>0(\ge 0)$. Given two same-order matrices $A,B$, $A-B\succ 0(⪰0)$ means that $a_{ij}>b_{ij}(a_{ij}\ge b_{ij})$. The symbol ⊗ stands for the Kronecker product.

2. Problem Formulation and Preliminaries

2.1. Positive System Theory

The discrete nonlinear multi-agent systems considered in the paper can be described as:

$$\begin{array}{c}{x}_i(k+1)=Af\left(x_i\left(k\right)\right)+B{u}_i\left(k\right),\hfill \\ y_i\left(k\right)=Cg\left(x_i\left(k\right)\right),\hfill \end{array}$$

(1)

where $x_i\left(k\right)\in {\mathbb{R}}^n$, $u_i\left(k\right)\in {\mathbb{R}}^m$, and $y_i\left(k\right)\in {\mathbb{R}}^s$, $i\in \{1,2,\cdots ,N\}$ denote the state, control input, and the output of the ith agent, respectively. $A\in {\mathbb{R}}^{n\times n}$, $B\in {\mathbb{R}}^{n\times m}$, and $C\in {\mathbb{R}}^{s\times n}$ are the system matrices. $f\left(x_i\left(k\right)\right)={(f_1\left(x_{i_1}\left(k\right)\right),f_2\left(x_{i_2}\left(k\right)\right),\cdots ,f_n\left(x_{i_n}\left(k\right)\right))}^{\top }$, and $g\left(x_i\left(k\right)\right)={(g_1\left(x_{i_1}\left(k\right)\right),g_2\left(x_{i_2}\left(k\right)\right),\cdots ,g_n\left(x_{i_n}\left(k\right)\right))}^{\top }$ are the nonlinear functions. Throughout this paper, there are some assumptions and definitions:

Assumption 1.

The system matrices satisfy that $A⪰0,B⪰0$, and $C⪰0$.

Assumption 2.

The nonlinear functions $f\left(x_i\left(k\right)\right)$ and $g\left(x_i\left(k\right)\right)$ satisfy the following sector conditions:

$$\begin{array}{c}{\varrho }_1{x}_{i_{\iota }}^2\left(k\right)\le f_i\left(x_{i_{\iota }}\left(k\right)\right)x_{i_{\iota }}\left(k\right)\le {\varrho }_2{x}_{i_{\iota }}^2\left(k\right),{\sigma }_1{x}_{i_{\iota }}^2\left(k\right)\le g_i\left(x_{i_{\iota }}\left(k\right)\right)x_{i_{\iota }}\left(k\right)\le {\sigma }_2{x}_{i_{\iota }}^2\left(k\right),\hfill \end{array}$$

(2)

where $i=1,2,\cdots ,N,\iota =1,2,\cdots ,n,0\le {\varrho }_1\le {\varrho }_2,0\le {\sigma }_1\le {\sigma }_2,$ and $f_i\left(0\right)=0.$

Definition 1 

([31,32]). System (1) is considered positive if and only if, with any given non-negative initial state and non-negative input, $x_i\left(k\right)⪰0$ and $y_i\left(k\right)⪰0$ are satisfied for all $k\in \mathbb{N}.$

Definition 2.

For a given PMAS (1), the following statements are equivalent:

(i)

The system achieves consensus;

(ii)

The system satisfies that $\underset{k\to \infty }{lim}\left|\right|x_i\left(k\right)-x_j\left(k\right){\left|\right|}_1=0,\forall i,j\in \{1,2,\cdots ,N\}$.

Lemma 1

([31,32]). The positivity of the system $x(k+1)=Ax\left(k\right)$ is preserved if and only if $A⪰0$.

Lemma 2

([31,32]). If system $x(k+1)=Ax\left(k\right)$ is positive, the following statements are equivalent:

(i)

A is a Schur matrix.

(ii)

The system exhibits stability.

(iii)

There exists a vector $v\in {\mathbb{R}}_{+}^n$ such that $(A^{\top }-I)v\prec 0.$

(iv)

There exists a constant c and a Lyapunov function $V\left(k\right)$ such that $\underset{k\to \infty }{lim}\Delta V\left(k\right)<c.$

Remark 1.

Lemma 2 presents a nuanced definition of bounded consensus within the framework of positive systems, offering a deviation from the traditional concept of asymptotic consensus. The bounded consensus is characterized by the system’s states evolving towards a fixed constant value, rather than diminishing to zero. By introducing this concept, the lemma broadens the horizon for understanding the system dynamics, providing a more flexible approach to achieving consensus in positive systems.

2.2. Graph Theory

In this paper, an undirected graph is utilized to depict the communication topology among the N agents in system (1), which is denoted by $\mathcal{G}=(\mathcal{V},\mathcal{E})$, which consists of a node set $\mathcal{V}\in \{{\mathcal{V}}_1,{\mathcal{V}}_2,\cdots ,{\mathcal{V}}_N\}$ and an edge set $\mathcal{E}=\left\{({\mathcal{V}}_i,{\mathcal{V}}_j)\right\},i,j\in \{1,2,\cdots ,N\}$. The adjacency matrix is denoted as $\mathcal{A}$, where ${\mathcal{A}}^{\left(ii\right)}=0$ and ${\mathcal{A}}^{\left(ij\right)}={\mathcal{A}}^{\left(ji\right)}=1$ if $({\mathcal{V}}_i,{\mathcal{V}}_j)\in \mathcal{E}$; otherwise, ${\mathcal{A}}^{\left(ij\right)}=0$. Obviously, $\mathcal{A}$ is a symmetric matrix. The neighbor nodes of node ${\mathcal{V}}_i$ are denoted by ${\mathcal{N}}_i:=\{{\mathcal{V}}_j:({\mathcal{V}}_i,{\mathcal{V}}_j)\in \mathcal{E}\}$. Diagonal matrix $\mathcal{D}$ denotes the degree matrix of $\mathcal{G}$, where ${\mathcal{D}}^{\left(ii\right)}={\sum }_{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}$. The corresponding Laplacian matrix is defined as $\mathcal{L}=\mathcal{D}-\mathcal{A}$, where ${\mathcal{A}}^{\left(ij\right)}=-{\mathcal{A}}^{\left(ij\right)}$ and ${\mathcal{A}}^{\left(ii\right)}={\mathcal{D}}^{\left(ii\right)}$.

Lemma 3

([33]). The real parts of all eigenvalues of $\mathcal{L}$ are all non-negative. Zero is one of the eigenvalues of $\mathcal{L}$, with $\mathbf{1}$ as the associated right eigenvector.

Lemma 4

([34,35]). Zero is a simple eigenvalue of $\mathcal{L}$ if and only if graph $\mathcal{G}$ possesses a directed spanning tree.

2.3. Problem Formulation

The objectives of this paper consist of two parts:(i) design a PIO to effectively estimate the states of systems (1) and (ii), based on the designed PIO, construct an event-triggered control protocol such that system (1) can reach a consensus.

3. Main Results

This section is split into three segments. The first segment analyzes the positivity of the considered nonlinear PMASs under the assumption. The PIO design is proposed in the second segment. The third segment presents the event-triggered mechanism and corresponding control protocol based on the proposed observer and event-triggered mechanism.

3.1. Positivity Analysis

Lemma 5.

With Assumption 2 in place, system (1) is positive if and only if Assumption 1 is satisfied.

Proof. 

Sufficiency. Suppose that $x_i\left(0\right)⪰0$ and the set of indices that satisfies $x_i^{\left(\iota \right)}\left(0\right)=0$ is denoted by $\Omega .$ It follows that

$$\begin{array}{c}{x}_i^{\left(\iota \right)}\left(1\right)=\sum _{j\notin \Omega }{A}^{\left(\iota j\right)}{f}_j\left(x_i^{\left(j\right)}\left(0\right)\right)+\sum _{j=1}^m{B}^{\left(\iota j\right)}{u}_i^{\left(j\right)}\left(0\right).\hfill \end{array}$$

(3)

From Assumption 2, it follows that $f_j\left(x_i^{\left(j\right)}\left(0\right)\right)\ge 0.$ By Assumption 1, one can obtain that $A^{\left(\iota j\right)}\ge 0$ and $B^{\left(\iota j\right)}⪰0$. Hence, it follows that $x_i\left(1\right)\ge 0$ for given $u_i\left(0\right)⪰0$. Similarly, it can be deduced that $y_i\left(1\right)\ge 0$. Consequently, it is evident that $x_i\left(1\right)⪰0,y_i\left(1\right)⪰0$ for given $x_i\left(0\right)⪰0$ and $u_i\left(0\right)⪰0$. Furthermore, it can be obtained that $x_i\left(k\right)⪰0,y_i\left(k\right)⪰0$.

Necessity. Suppose that the system (1) is positive. Let $x\left(0\right)=0$, then $x\left(1\right)=B{u}_i\left(0\right)$. Since $x\left(1\right)⪰0$ for any given $u_i\left(0\right)⪰0$, then $B⪰0$ according to Lemma 2. To prove that $A⪰0$, let $u_i\left(0\right)=0$. Suppose that there exists an element of matrix A satisfying that $A^{\left(\iota j\right)}<0$; this results in

$$\begin{array}{c}{x}_i^{\left(\iota \right)}(k+1)=\sum _{j=1,j\ne j}^n{A}^{\left(\iota j\right)}{f}_j\left(x_i^{\left(j\right)}\left(k\right)\right)+A^{\left(\iota j\right)}{f}_j\left(x_i^{\left(j\right)}\left(k\right)\right).\hfill \end{array}$$

(4)

It can be seen that it is possible to make $x_i^{\left(\iota \right)}(k+1)<0$ if $A^{\left(\iota j\right)}$ is kept as small as possible, which leads to a contradiction with the system’s positivity. Therefore, $A⪰0.$ By the same argument, it can be obtained that $C⪰0,E⪰0.$ □

3.2. Positive Proportional–Integral Observer

In this section, some conditions will be provided for the design of the PIO for PMASs. The designed PIO is described as follows:

$$\begin{array}{c}{\widehat{x}}_i(k+1)=A\widehat{f}\left({\widehat{x}}_i\left(k\right)\right)+L_P({\widehat{y}}_i\left(k\right)-y_i\left(k\right))+F{v}_i\left(k\right)+B{u}_i\left(k\right),\hfill \\ v_i(k+1)=\alpha v_i\left(k\right)+(1-\alpha )L_I({\widehat{y}}_i\left(k\right)-y_i\left(k\right)),\hfill \\ {\widehat{y}}_i\left(k\right)=C\widehat{g}\left({\widehat{x}}_i\left(k\right)\right),\hfill \end{array}$$

(5)

where ${\vartheta }_1{\widehat{x}}_{i_{\iota }}^2\left(k\right)\le \widehat{f}\left({\widehat{x}}_{i_{\iota }}\left(k\right)\right){\widehat{x}}_{i_{\iota }}\left(k\right)\le {\vartheta }_2{\widehat{x}}_{i_{\iota }}^2\left(k\right),{\varsigma }_1{\widehat{x}}_{i_{\iota }}^2\left(k\right)\le \widehat{g}\left({\widehat{x}}_{i_{\iota }}\left(k\right)\right){\widehat{x}}_{i_{\iota }}\left(k\right)\le {\varsigma }_2{\widehat{x}}_{i_{\iota }}^2\left(k\right)$ and $\alpha \in [0,1].$ ${\widehat{x}}_i\left(k\right)\in {\mathbb{R}}^n,\widehat{y}\left(k\right)\in {\mathbb{R}}^s$, and $v_i\left(k\right)\in {\mathbb{R}}^n$ denote the state estimation value, the output of the observer, and the integral parts, respectively. $L_P,L_I$, and F are the gain matrices to be determined. The estimate error is defined as:

$$e_i\left(k\right)={\widehat{x}}_i\left(k\right)-x_i\left(k\right),$$

(6)

which yields

$$\begin{array}{c}{e}_i(k+1)={\widehat{x}}_i(k+1)-x_i(k+1)\hfill \\ =A\widehat{f}\left({\widehat{x}}_i\left(k\right)\right)+L_P({\widehat{y}}_i\left(k\right)-y_i\left(k\right))+F{v}_i\left(k\right)+B{u}_i\left(k\right)-Af\left(x_i\left(k\right)\right)-B{u}_i\left(k\right)\hfill \\ =A(\widehat{f}\left({\widehat{x}}_i\left(k\right)\right)-f\left(x_i\left(k\right)\right))+L_{P}C(\widehat{g}\left({\widehat{x}}_i\left(k\right)\right)-g\left(x_i\left(k\right)\right))+F{v}_i\left(k\right).\hfill \end{array}$$

(7)

Define ${\tilde{x}}_i(k+1):={\left(x_i^{\top }(k+1)e_i^{\top }(k+1)v_i^{\top }(k+1)\right)}^{\top }$; based on the system (1), the designed observer (5), and the estimate error (6), the following augment systems can be obtained:

$$\begin{array}{c}{\tilde{x}}_i(k+1)=\left(\begin{array}{c}Af\left(x_i\left(k\right)\right)+B{u}_i\left(k\right)\\ A(\widehat{f}\left({\widehat{x}}_i\left(k\right)\right)-f\left(x_i\left(k\right)\right))+L_{P}C(\widehat{g}\left({\widehat{x}}_i\left(k\right)\right)-g\left(x_i\left(k\right)\right))+F{v}_i\left(k\right)\\ \alpha v_i\left(k\right)+(1-\alpha )L_{I}C(\widehat{g}\left({\widehat{x}}_i\left(k\right)\right)-g\left(x_i\left(k\right)\right))\end{array}\right).\hfill \end{array}$$

(8)

The task is to derive the estimation ${\widehat{x}}_i\left(k\right)$ of the state $x_i\left(k\right)$ in such a way that the ${\widehat{x}}_i\left(k\right)$ are non-negative and the error of the estimate remains stable.

Theorem 1.

If there exist constants $0\le {\varrho }_1\le {\varrho }_2$, $0\le {\sigma }_1\le {\sigma }_2$, $0\le {\vartheta }_1\le {\vartheta }_2$, $0\le {\varsigma }_1\le {\varsigma }_2$, ${\sigma }_2\le {\varsigma }_1$, $\alpha \in [0,1]$, ${\mathbb{R}}^n$ vectors ${\nu }_1\succ 0$, ${\nu }_2\succ 0$, ${\nu }_3\succ 0$, ${\delta }_F^{\left(\iota \right)}\succ 0$, and ${\overline{\delta }}_F\succ 0$ and ${\mathbb{R}}^s$ vectors ${\delta }_{LI}^{\left(\iota \right)}\succ 0$, ${\overline{\delta }}_{LI}\succ 0$, ${\delta }_{LP}^{\left(\iota \right)}\succ 0$, and ${\overline{\delta }}_{LP}\succ 0$ such that

$$\begin{array}{c}({\vartheta }_1-{\varrho }_2){\mathbf{1}}_n^{\top }{\nu }_{2}A+({\varsigma }_1-{\sigma }_2)\sum _{\iota =1}^n{\mathbf{1}}_n^{\left(\iota \right)}{\delta }_{LP}^{\left(\iota \right)\top }C⪰0,\hfill \end{array}$$

(9a)

$$\begin{array}{c}{\vartheta }_1{\mathbf{1}}_n^{\top }{\nu }_{2}A+{\varsigma }_1\sum _{\iota =1}^n{\mathbf{1}}_n^{\left(\iota \right)}{\delta }_{LP}^{\left(\iota \right)\top }C⪰0,\hfill \end{array}$$

(9b)

$$\begin{array}{c}{\delta }_{LP}^{\left(\iota \right)}⪯{\overline{\delta }}_{LP},{\delta }_{LI}^{\left(\iota \right)}⪯{\overline{\delta }}_{LI},{\delta }_F^{\left(\iota \right)}⪯{\overline{\delta }}_F,\iota =1,2,\cdots ,n,\hfill \end{array}$$

(9c)

$$\begin{array}{c}{\varrho }_2{A}^{\top }{\nu }_1+({\vartheta }_2-{\varrho }_1)A^{\top }{\nu }_2+({\varsigma }_2-{\sigma }_1)C^{\top }{\overline{\delta }}_{LP}+(1-\alpha )({\varsigma }_2-{\sigma }_1)C^{\top }{\overline{\delta }}_{LI}-{\nu }_1\prec 0,\hfill \end{array}$$

(9d)

$$\begin{array}{c}{\vartheta }_2{A}^{\top }{\nu }_2+{\varsigma }_2{C}^{\top }{\overline{\delta }}_{LP}+(1-\alpha ){\varsigma }_2{C}^{\top }{\overline{\delta }}_{LI}-{\nu }_2\prec 0,\hfill \end{array}$$

(9e)

$$\begin{array}{c}{\overline{\delta }}_F+(\alpha -1){\nu }_3\prec 0.\hfill \end{array}$$

(9f)

hold, then the designed PI observer (5) remains positive and the error system (6) stays stable, where the observer gain matrices are given by

$$\begin{array}{c}{L}_P=\frac{{\sum }_{\iota =1}^n{\mathbf{1}}_n^{\left(\iota \right)}{\delta }_{LP}^{\left(\iota \right)\top }}{{\mathbf{1}}_n^{\top }{\nu }_2},L_I=\frac{{\sum }_{\iota =1}^n{\mathbf{1}}_n^{\left(\iota \right)}{\delta }_{LI}^{\left(\iota \right)\top }}{{\mathbf{1}}_n^{\top }{\nu }_3},F=\frac{{\sum }_{\iota =1}^n{\mathbf{1}}_n^{\left(\iota \right)}{\delta }_F^{\left(\iota \right)\top }}{{\mathbf{1}}_n^{\top }{\nu }_2}.\hfill \end{array}$$

(10)

Proof. 

First, we consider the positivity. Based on Assumptions 1 and 2, for any ${\tilde{x}}_i\left(k_0\right)⪰0$, this gives that

$$\begin{array}{c}{\tilde{x}}_i(k_0+1)\hfill \\ ⪰\left(\begin{array}{c}A{\varrho }_1{x}_i\left(k_0\right)+B{u}_i\left(k_0\right)\\ A({\vartheta }_1{e}_i\left(k_0\right)+({\vartheta }_1-{\varrho }_2)x_i\left(k_0\right))+L_{P}C({\varsigma }_1{e}_i\left(k_0\right)+({\varsigma }_1-{\sigma }_2)x_i\left(k_0\right))+F{v}_i\left(k_0\right)\\ \alpha v_i\left(k_0\right)+(1-\alpha )L_{I}C({\varsigma }_1{e}_i\left(k_0\right)+({\varsigma }_1-{\sigma }_2)x_i\left(k_0\right))\end{array}\right)\hfill \\ =\left(\begin{array}{ccc}{\varrho }_{1}A& \mathbf{0}& \mathbf{0}\\ ({\vartheta }_1-{\varrho }_2)A+({\varsigma }_1-{\sigma }_2)L_{P}C& {\vartheta }_{1}A+{\varsigma }_1{L}_{P}C& F\\ (1-\alpha )({\varsigma }_1-{\sigma }_2)L_{I}C& (1-\alpha ){\varsigma }_1{L}_{I}C& \alpha I\end{array}\right)\left(\begin{array}{c}{x}_i\left(k_0\right)\\ e_i\left(k_0\right)\\ v_i\left(k_0\right)\end{array}\right)+\left(\begin{array}{c}B\\ \mathbf{0}\\ \mathbf{0}\end{array}\right)u_i\left(k_0\right)\hfill \\ =\tilde{A}{\tilde{x}}_i\left(k_0\right)+\tilde{B}{u}_i\left(k_0\right),\hfill \end{array}$$

(11)

where

$$\tilde{A}=\left(\begin{array}{ccc}{\varrho }_{1}A& \mathbf{0}& \mathbf{0}\\ ({\vartheta }_1-{\varrho }_2)A+({\varsigma }_1-{\sigma }_2)L_{P}C& {\vartheta }_{1}A+{\varsigma }_1{L}_{P}C& F\\ (1-\alpha )({\varsigma }_1-{\sigma }_2)L_{I}C& (1-\alpha ){\varsigma }_1{L}_{I}C& \alpha I\end{array}\right),\tilde{B}=\left(\begin{array}{c}B\\ \mathbf{0}\\ \mathbf{0}\end{array}\right).$$

(12)

Since $0\le {\varrho }_1\le {\varrho }_2,{\delta }_F\succ 0$, ${\sigma }_2\le {\varsigma }_1$, and $\alpha \in [0,1]$, one has ${\varrho }_{1}A⪰0$, $F⪰0$, $(1-\alpha )({\varsigma }_1-{\sigma }_2)L_{I}C⪰0$, and $(1-\alpha ){\varsigma }_1{L}_{I}C⪰0$. Based on (9a) and (10), it can be obtained that $({\vartheta }_1-{\varrho }_2)A+({\varsigma }_1-{\sigma }_2)L_{P}C⪰0$. Similarly, it is easy to derive that ${\vartheta }_{1}A+{\varsigma }_1{L}_{P}C⪰0$ from (9b) and (10). Consequently, it can be concluded that $\tilde{A}⪰0.$ Then, the positivity of ${\tilde{x}}_i(k_0+1)$ can be guaranteed by Lemma 1 under Assumption 1. It is not difficult to verify that ${\tilde{x}}_i\left(k\right)⪰0$ for any initial state ${\tilde{x}}_i\left(k_0\right)⪰0$ by recursive deduction. Therefore, the system (8) is positive by Definition 1. Thus, the designed PI observer (5) is positive.

Next, we will analyze the stability of the systems. Choose a co-positive Lyapunov function candidate $V\left(k\right)={\tilde{x}}_i^{\top }\left(k\right)\nu$, where $\nu ={\left({\nu }_1^{\top }{\nu }_2^{\top }{\nu }_3^{\top }\right)}^{\top }$. Since

$$\begin{array}{c}{\tilde{x}}_i(k+1)⪯\left(\begin{array}{c}A{\varrho }_2{x}_i\left(k\right)+B{u}_i\left(k\right)\\ A({\vartheta }_2{e}_i\left(k\right)+({\vartheta }_2-{\varrho }_1)x_i\left(k\right))+L_{P}C({\varsigma }_2{e}_i\left(k\right)+({\varsigma }_2-{\sigma }_1)x_i\left(k\right))+F{v}_i\left(k\right)\\ \alpha v_i\left(k\right)+(1-\alpha )L_{I}C({\varsigma }_2{e}_i\left(k\right)+({\varsigma }_2-{\sigma }_1)x_i\left(k\right))\end{array}\right)\hfill \end{array}$$

$$\begin{array}{c}=\left(\begin{array}{ccc}{\varrho }_{2}A& \mathbf{0}& \mathbf{0}\\ ({\vartheta }_2-{\varrho }_1)A+({\varsigma }_2-{\sigma }_1)L_{P}C& {\vartheta }_{2}A+{\varsigma }_2{L}_{P}C& F\\ (1-\alpha )({\varsigma }_2-{\sigma }_1)L_{I}C& (1-\alpha ){\varsigma }_2{L}_{I}C& \alpha I\end{array}\right)\left(\begin{array}{c}{x}_i\left(k\right)\\ e_i\left(k\right)\\ v_i\left(k\right)\end{array}\right)+\left(\begin{array}{c}B\\ \mathbf{0}\\ \mathbf{0}\end{array}\right)u_i\left(k\right)\hfill \\ =\check{A}{\tilde{x}}_i\left(k\right)+\tilde{B}{u}_i\left(k\right),\hfill \end{array}$$

(13)

where

$$\check{A}=\left(\begin{array}{ccc}{\varrho }_{2}A& \mathbf{0}& \mathbf{0}\\ ({\vartheta }_2-{\varrho }_1)A+({\varsigma }_2-{\sigma }_1)L_{P}C& {\vartheta }_{2}A+{\varsigma }_2{L}_{P}C& F\\ (1-\alpha )({\varsigma }_2-{\sigma }_1)L_{I}C& (1-\alpha ){\varsigma }_2{L}_{I}C& \alpha I\end{array}\right).$$

(14)

As a consequence, it can be inferred that

$$\begin{array}{c}\Delta V\left(k\right)=V(k+1)-V\left(k\right)={\tilde{x}}_i^{\top }(k+1)\nu -{\tilde{x}}_i^{\top }\left(k\right)\nu \hfill \\ \le (\check{A}{\tilde{x}}_i\left(k\right)-{\tilde{x}}_i^{\top }\left(k\right))\nu ={\tilde{x}}_i^{\top }\left(k\right)(\check{A}-I)\nu ;\hfill \end{array}$$

(15)

combined with (14), this gives

$$\begin{array}{c}({\check{A}}^{\top }-I)\nu =\left(\begin{array}{c}{\varrho }_2{A}^{\top }{\nu }_1+({\vartheta }_2-{\varrho }_1)A^{\top }{\nu }_2+({\varsigma }_2-{\sigma }_1)C^{\top }{L}_P^{\top }{\nu }_2+(1-\alpha )({\varsigma }_2-{\sigma }_1)C^{\top }{L}_I^{\top }{\nu }_3-{\nu }_1\\ {\vartheta }_2{A}^{\top }{\nu }_2+{\varsigma }_2{C}^{\top }{L}_P^{\top }{\nu }_2+(1-\alpha ){\varsigma }_2{C}^{\top }{L}_I^{\top }{\nu }_3-{\nu }_2\\ F^{\top }{\nu }_2+\alpha {\nu }_3-{\nu }_3\end{array}\right).\hfill \end{array}$$

By (9c) and (10), it follows that

$$\begin{array}{c}{L}_P^{\top }{\nu }_2=\frac{{\left({\sum }_{\iota =1}^n{\mathbf{1}}_n^{\left(\iota \right)}{\delta }_{LP}^{\left(\iota \right)\top }\right)}^{\top }}{{\mathbf{1}}_n^{\top }{\nu }_2}{\nu }_2⪯\frac{{\overline{\delta }}_{LP}{\mathbf{1}}_n^{\left(\iota \right)\top }}{{\mathbf{1}}_n^{\top }{\nu }_2}{\nu }_2={\overline{\delta }}_{LP},\hfill \\ L_I^{\top }{\nu }_2=\frac{{\left({\sum }_{\iota =1}^n{\mathbf{1}}_n^{\left(\iota \right)}{\delta }_{LI}^{\left(\iota \right)\top }\right)}^{\top }}{{\mathbf{1}}_n^{\top }{\nu }_3}{\nu }_3⪯\frac{{\overline{\delta }}_{LI}{\mathbf{1}}_n^{\left(\iota \right)\top }}{{\mathbf{1}}_n^{\top }{\nu }_3}{\nu }_3={\overline{\delta }}_{LI},\hfill \\ F^{\top }{\nu }_2=\frac{{\left({\sum }_{\iota =1}^n{\mathbf{1}}_n^{\left(\iota \right)}{\delta }_F^{\left(\iota \right)\top }\right)}^{\top }}{{\mathbf{1}}_n^{\top }{\nu }_2}{\nu }_2⪯\frac{{\overline{\delta }}_F{\mathbf{1}}_n^{\left(\iota \right)\top }}{{\mathbf{1}}_n^{\top }{\nu }_2}{\nu }_2={\overline{\delta }}_F.\hfill \end{array}$$

(16)

Together with (9d)–(9f) and (16), it can be derived that

$$\begin{array}{cc}\hfill & {\varrho }_2{A}^{\top }{\nu }_1+({\vartheta }_2-{\varrho }_1)A^{\top }{\nu }_2+({\varsigma }_2-{\sigma }_1)C^{\top }{L}_P^{\top }{\nu }_2+(1-\alpha )({\varsigma }_2-{\sigma }_1)C^{\top }{L}_I^{\top }{\nu }_3-{\nu }_1\hfill \\ \hfill & ⪯{\varrho }_2{A}^{\top }{\nu }_1+({\vartheta }_2-{\varrho }_1)A^{\top }{\nu }_2+({\varsigma }_2-{\sigma }_1)C^{\top }{\overline{\delta }}_{LP}+(1-\alpha )({\varsigma }_2-{\sigma }_1)C^{\top }{\overline{\delta }}_{LI}-{\nu }_1\prec 0,\hfill \end{array}$$

(17)

$$\begin{array}{cc}\hfill & {\vartheta }_2{A}^{\top }{\nu }_2+{\varsigma }_2{C}^{\top }{L}_P^{\top }{\nu }_2+(1-\alpha ){\varsigma }_2{C}^{\top }{L}_I^{\top }{\nu }_3-{\nu }_2\hfill \\ \hfill & ⪯{\vartheta }_2{A}^{\top }{\nu }_2+{\varsigma }_2{C}^{\top }{\overline{\delta }}_{LP}+(1-\alpha ){\varsigma }_2{C}^{\top }{\overline{\delta }}_{LI}-{\nu }_2\prec 0,\hfill \end{array}$$

(18)

$$\begin{array}{c}{F}^{\top }{\nu }_2+\alpha {\nu }_3-{\nu }_3⪯{\overline{\delta }}_F+\alpha {\nu }_3-{\nu }_3\prec 0.\hfill \end{array}$$

(19)

From (15) and (17)–(19), it can be obtained that $\Delta V\left(k\right)\le 0$. The proof is complete. □

Remark 2.

Different from the widely used proportional observer, the designed observer (5) has two additional gain matrices F and $L_I$. These matrices provide higher degrees of freedom for the design of the observer and allow for a more refined adjustment of the observer’s response to the considered system.

Remark 3.

Compared with the PIO designed for general systems (non-positive) in [30,36], an additional parameter α is introduced to the integral part of the designed PIO. The introduced parameter α can tune the integral part to guarantee the positivity of the observer. It should be noted that the selection of α and its impact on the system are still open issues. Furthermore, the co-positive Lyapunov function and linear programming are utilized in this paper, which have shown the effectiveness of handling the control synthesis of positive systems in [37].

3.3. Dynamic Event-Triggered Observer-Based Control Protocol

Define the observer measurement errors:

$$\begin{array}{cc}{ϵ}_i\left(k\right)={\widehat{x}}_i\left({\kappa }_{\iota }\right)-{\widehat{x}}_i\left(k\right)\hfill & k\in [{\kappa }_{\iota },{\kappa }_{\iota }+1,\cdots ,{\kappa }_{\iota +1}-1).\hfill \end{array}$$

(20)

Consider the following dynamic event-triggered mechanism:

$$\begin{array}{c}\left|\right|{ϵ}_i{\left(k\right)\left|\right|}_1\le \beta \left(k\right)\left|\right|{\widehat{x}}_i\left(k\right){\left|\right|}_1,\hfill \end{array}$$

(21)

where

$$\begin{array}{c}\beta (k+1)=\epsilon \beta \left(k\right)+(\beta \left(k\right)-\Lambda )\left(\right|\left|{ϵ}_i\left(k\right)\right|{|}_1-\overline{\beta }\left|\right|{\widehat{x}}_i\left(k\right)\left|{|}_1\right),\hfill \end{array}$$

(22)

with $0<\Lambda <\beta \left(0\right)<\overline{\beta }$, and $\epsilon ,\Lambda ,\overline{\beta }$ are given constants. Thus, the triggering instants $\{{\kappa }_0,{\kappa }_1,\cdots \}$ are generated by

$$\begin{array}{c}{\kappa }_0=0,\hfill \\ {\kappa }_i=\underset{k\in \mathbb{N}}{inf}\{k>{\kappa }_{\iota }:\left|\right|{ϵ}_i{\left(k\right)\left|\right|}_1>\beta \left(k\right)\left|\right|{\widehat{x}}_i{\left(k\right)\left|\right|}_1\}.\hfill \end{array}$$

(23)

Using the PI observer (5) and the dynamic event-triggered mechanism (21), the control protocol is constructed as follows:

$$\begin{array}{c}{u}_i\left(k\right)=K_S{\widehat{x}}_i\left({\kappa }_{\iota }\right)+K_P\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}({\widehat{x}}_i\left({\kappa }_{\iota }\right)-{\widehat{x}}_j\left({\kappa }_{\iota }\right))+K_C{x}_c,\hfill \end{array}$$

(24)

where $K_S,K_P$, and $K_C$ are gain matrices to be designed and $x_c$ is a given vector.

Theorem 2.

If there exist constants $0<\mu <1$, $0\le {\varrho }_1\le {\varrho }_2$, $0\le {\sigma }_1\le {\sigma }_2$, $0\le {\vartheta }_1\le {\vartheta }_2$, $0\le {\varsigma }_1\le {\varsigma }_2$, ${\sigma }_2⪯{\varsigma }_1$, $0<\Lambda <\overline{\beta },\mu >0,\rho >0,0<\underline{\theta }<\overline{\theta }$, $\alpha \in [0,1]$, and ${\sigma }_2\le {\varsigma }_1$, ${\mathbb{R}}^n$ vectors ${\nu }_1\succ 0$, ${\nu }_2\succ 0$, ${\nu }_3\succ 0$, ${\delta }_F^{\left(\iota \right)}\succ 0$, ${\underline{\delta }}_F\succ 0$, ${\delta }_{kc}^{\left(j\right)}\succ 0$, ${\delta }_{ks}^{+\left(j\right)}\succ 0$, ${\underline{\delta }}_{ks}^{+}\succ 0$, ${\delta }_{kp}^{+\left(j\right)}\succ 0$, ${\underline{\delta }}_{kp}^{+}\succ 0$, ${\delta }_{ks}^{-\left(j\right)}\prec 0$, ${\overline{\delta }}_{ks}^{-}\prec 0$, ${\delta }_{kp}^{-\left(j\right)}\prec 0$, and ${\overline{\delta }}_{kp}^{-}\prec 0$, and ${\mathbb{R}}^s$ vectors ${\delta }_{li}^{\left(\iota \right)}\succ 0$, ${\overline{\delta }}_{li}\succ 0$, ${\delta }_{lp}^{\left(\iota \right)}\succ 0$, and ${\overline{\delta }}_{lp}\succ 0$ such that

$$\begin{array}{c}B\sum _{j=1}^m{\mathbf{1}}_m^{\left(j\right)}{\delta }_{ks}^{+\left(j\right)\top }\Psi +B\sum _{j=1}^m{\mathbf{1}}_m^{\left(j\right)}{\delta }_{ks}^{-\left(j\right)\top }\Phi +d_{min}B\sum _{j=1}^m{\mathbf{1}}_m^{\left(j\right)}{\delta }_{kp}^{+\left(j\right)\top }\Psi \\ +d_{max}B\sum _{j=1}^m{\mathbf{1}}_m^{\left(j\right)}{\delta }_{kp}^{-\left(j\right)\top }\Phi ⪰0,\end{array}$$

(25a)

$$\begin{array}{c}B\sum _{j=1}^m{\mathbf{1}}_m^{\left(j\right)}{\delta }_{kp}^{+\left(j\right)\top }\Phi +B\sum _{j=1}^m{\mathbf{1}}_m^{\left(j\right)}{\delta }_{kp}^{-\left(j\right)\top }\Psi ⪯0,\hfill \end{array}$$

(25b)

$$\begin{array}{c}({\vartheta }_1-{\varrho }_2){\mathbf{1}}_n^{\top }{\nu }_{2}A+({\varsigma }_1-{\sigma }_2)\sum _{\iota =1}^n{\mathbf{1}}_n^{\left(\iota \right)\top }{\delta }_{lp}^{\left(n\right)}C⪰0,\hfill \end{array}$$

(25c)

$$\begin{array}{c}{\vartheta }_1{\mathbf{1}}_n^{\top }{\nu }_{2}A+{\varsigma }_1\sum _{\iota =1}^n{\mathbf{1}}_n^{\left(\iota \right)}{\delta }_{lp}^{\left(\iota \right)\top }C⪰0,\hfill \end{array}$$

(25d)

$$\begin{array}{c}{\mathbf{1}}_m^{\top }{B}^{\top }{\nu }_1{\varrho }_{1}A+B\sum _{j=1}^m{\mathbf{1}}_m^{\left(j\right)}{\delta }_{ks}^{+\left(j\right)\top }\Psi +B\sum _{j=1}^m{\mathbf{1}}_m^{\left(j\right)}{\delta }_{ks}^{-\left(j\right)\top }\Phi \\ +2\overline{\beta }{d}_{max}(B\sum _{j=1}^m{\mathbf{1}}_m^{\left(j\right)}{\delta }_{kp}^{-\left(j\right)\top }{\mathbf{1}}_{n\times n}-B\sum _{j=1}^m{\mathbf{1}}_m^{\left(j\right)}{\delta }_{kp}^{+\left(j\right)\top }{\mathbf{1}}_{n\times n})\\ +l_{max}(B\sum _{j=1}^m{\mathbf{1}}_m^{\left(j\right)}{\delta }_{kp}^{+\left(j\right)\top }\Phi +B\sum _{j=1}^m{\mathbf{1}}_m^{\left(j\right)}{\delta }_{kp}^{-\left(j\right)\top }\Psi )\\ +(\underline{\theta }-1)\left(B\sum _{j=1}^m{\mathbf{1}}_m^{\left(j\right)}{\delta }_{kc}^{\left(j\right)\top }\right)-{\mathbf{1}}_m^{\top }{B}^{\top }{\nu }_1{I}_n⪰0,\end{array}$$

(25e)

$$\begin{array}{c}({\varrho }_2{A}^{\top }-I_n){\nu }_1+\Phi {\overline{\delta }}_{ks}^{+}+{\overline{\delta }}_{ks}^{-}-{\mathbf{1}}_{n\times n}{\underline{\delta }}_{ks}^{-}+2\overline{\beta }{d}_{max}({\mathbf{1}}_{n\times n}{\overline{\delta }}_{kp}^{+}-{\mathbf{1}}_{n\times n}{\underline{\delta }}_{kp}^{-})\\ +({\vartheta }_2-{\varrho }_1)A^{\top }{\nu }_2+({\varsigma }_2-{\sigma }_1)C^{\top }{\overline{\delta }}_{lp}+(1-\alpha )({\varsigma }_2-{\sigma }_1)\left(C^{\top }{\overline{\delta }}_{li}\right)+(1-\mu ){\nu }_1\prec 0,\end{array}$$

(25f)

$$\begin{array}{c}\Phi {\overline{\delta }}_{ks}^{+}+{\overline{\delta }}_{ks}^{-}-{\mathbf{1}}_{n\times n}{\underline{\delta }}_{ks}^{-}+2\overline{\beta }{d}_{max}({\mathbf{1}}_{n\times n}{\overline{\delta }}_{kp}^{+}-{\mathbf{1}}_{n\times n}{\underline{\delta }}_{kp}^{-})\\ +{\vartheta }_2{A}^{\top }{\nu }_2+{\varsigma }_2{C}^{\top }{\overline{\delta }}_{lp}-{\nu }_2+{\varsigma }_2(1-\alpha )\left(C^{\top }{\overline{\delta }}_{li}\right)+(1-\mu ){\nu }_2\prec 0,\end{array}$$

(25g)

$$\begin{array}{c}{\overline{\delta }}_f+(\alpha -\mu ){\nu }_3\prec 0,\end{array}$$

(25h)

$$\begin{array}{c}{\varrho }_2{A}^{\top }{\nu }_1+\Phi {\overline{\delta }}_{ks}^{+}+{\overline{\delta }}_{ks}^{-}-{\mathbf{1}}_{n\times n}{\underline{\delta }}_{ks}^{-}+2\overline{\beta }{d}_{max}({\mathbf{1}}_{n\times n}{\overline{\delta }}_{kp}^{+}-{\mathbf{1}}_{n\times n}{\underline{\delta }}_{ks}^{-})+(N\overline{\theta }-1){\overline{\delta }}_{kc}\\ +({\vartheta }_2-{\varrho }_1)A^{\top }{\nu }_2+({\varsigma }_2-{\sigma }_1)C^{\top }{\overline{\delta }}_{lp}+(1-\alpha )({\varsigma }_2-{\sigma }_1)\left(C^{\top }{\overline{\delta }}_{li}\right)-(\rho +1){\nu }_1\prec 0,\end{array}$$

(25i)

$$\begin{array}{c}{\underline{\delta }}_{ks}^{-}⪯{\delta }_{ks}^{-\left(j\right)}⪯{\overline{\delta }}_{ks}^{-},{\underline{\delta }}_{ks}^{+}⪯{\delta }_{ks}^{+\left(j\right)}⪯{\overline{\delta }}_{ks}^{+},{\underline{\delta }}_{kp}^{-}⪯{\delta }_{kp}^{-\left(j\right)}⪯{\overline{\delta }}_{kp}^{-},{\underline{\delta }}_{kp}^{+}⪯{\delta }_{kp}^{+\left(j\right)}⪯{\overline{\delta }}_{kp}^{+},\\ {\underline{\delta }}_{lp}⪯{\delta }_{lp}^{\left(\iota \right)}⪯{\overline{\delta }}_{lp},{\underline{\delta }}_{li}⪯{\delta }_{li}^{\left(\iota \right)}⪯{\overline{\delta }}_{li},\underline{\theta }⪯{\theta }_i⪯\overline{\theta },\end{array}$$

(25j)

hold, where $\Phi =I_n+\overline{\beta }{\mathbf{1}}_{n\times n}$, $\Psi =I_n-\overline{\beta }{\mathbf{1}}_{n\times n}$, ${\displaystyle d_{max}=\underset{1\le i\le n}{max}{\mathcal{D}}^{\left(ii\right)}}$, ${\displaystyle d_{min}=\underset{1\le i\le n}{min}{\mathcal{D}}^{\left(ii\right)}}$, and ${\displaystyle l_{max}=\underset{1\le i,j\le n}{max}{\mathcal{L}}^{\left(ij\right)}}$, then under the observer-based dynamic event-triggered PID protocol (24) with

$$\begin{array}{c}{K}_S^{+}=\frac{{\sum }_{j=1}^m{\mathbf{1}}_m^{\left(j\right)}{\delta }_{ks}^{+\left(j\right)\top }}{{\mathbf{1}}_m^{\top }{B}^{\top }{\nu }_1},K_S^{-}=\frac{{\sum }_{j=1}^m{\mathbf{1}}_m^{\left(j\right)}{\delta }_{ks}^{-\left(j\right)\top }}{{\mathbf{1}}_m^{\top }{B}^{\top }{\nu }_1},K_P^{+}=\frac{{\sum }_{j=1}^m{\mathbf{1}}_m^{\left(j\right)}{\delta }_{kp}^{+\left(j\right)\top }}{{\mathbf{1}}_m^{\top }{B}^{\top }{\nu }_1},K_P^{-}=\frac{{\sum }_{j=1}^m{\mathbf{1}}_m^{\left(j\right)}{\delta }_{kp}^{-\left(j\right)\top }}{{\mathbf{1}}_m^{\top }{B}^{\top }{\nu }_1},\hfill \\ K_C=\frac{{\sum }_{j=1}^m{\mathbf{1}}_m^{\left(j\right)}{\delta }_{kc}^{\left(j\right)\top }}{{\mathbf{1}}_m^{\top }{B}^{\top }{\nu }_1},L_I=\frac{{\sum }_{\iota =1}^n{\mathbf{1}}_n^{\left(\iota \right)}{\delta }_{li}^{\left(\iota \right)\top }}{{\mathbf{1}}_n^{\top }{\nu }_3},L_P=\frac{{\sum }_{\iota =1}^n{\mathbf{1}}_n^{\left(\iota \right)}{\delta }_{lp}^{\left(\iota \right)\top }}{{\mathbf{1}}_n^{\top }{\nu }_2},F=\frac{{\sum }_{\iota =1}^n{\mathbf{1}}_n^{\left(\iota \right)}{\delta }_f^{\left(\iota \right)\top }}{{\mathbf{1}}_n^{\top }{\nu }_2},\hfill \end{array}$$

(26)

the system is positive and stable.

Proof. 

First, we consider the positivity. Using the dynamic event-triggered condition (21), it follows that

$$\begin{array}{c}\left|\right|{ϵ}_i\left(k_0\right){\left|\right|}_1\le \beta \left(k_0\right)\left|\right|{\widehat{x}}_i\left(k_0\right){\left|\right|}_1,\hfill \end{array}$$

(27)

for any initial condition $x_i\left(k_0\right)⪰0$. Noting the fact that $0<\beta \left(k\right)<\overline{\beta }$, it can be inferred that

$$\begin{array}{c}-\overline{\beta }{\mathbf{1}}_{n\times n}{\widehat{x}}_i\left(k_0\right)⪯{ϵ}_i\left(k_0\right)⪯\overline{\beta }{\mathbf{1}}_{n\times n}{\widehat{x}}_i\left(k_0\right).\hfill \end{array}$$

(28)

By (20), (24), and (28), one can obtain that

$$\begin{array}{c}{x}_i(k_0+1)⪰{\varrho }_{1}A{x}_i\left(k_0\right)+(B{K}_S^{+}(I_n-\overline{\beta }{\mathbf{1}}_{n\times n})+B{K}_S^{-}(I_n+\overline{\beta }{\mathbf{1}}_{n\times n})){\widehat{x}}_i\left(k_0\right)\hfill \\ +B{K}_P^{+}(I_n-\overline{\beta }{\mathbf{1}}_{n\times n})\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{\widehat{x}}_i\left(k_0\right)-B{K}_P^{+}(I_n+\overline{\beta }{\mathbf{1}}_{n\times n})\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{\widehat{x}}_j\left(k_0\right)\hfill \\ +B{K}_P^{-}(I_n+\overline{\beta }{\mathbf{1}}_{n\times n})\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{\widehat{x}}_i\left(k_0\right)-B{K}_P^{-}(I_n-\overline{\beta }{\mathbf{1}}_{n\times n})\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{\widehat{x}}_j\left(k_0\right)\hfill \\ +B{K}_C{x}_{ic}\hfill \\ =({\varrho }_{1}A+B{K}_S^{+}\Psi +B{K}_S^{-}\Phi )x_i\left(k_0\right)+(B{K}_S^{+}\Psi +B{K}_S^{-}\Phi )e_i\left(k_0\right)\hfill \\ +B{K}_P^{+}\Psi \sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{x}_i\left(k_0\right)-B{K}_P^{+}\Phi \sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{x}_j\left(k_0\right)+B{K}_P^{-}\Phi \sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{x}_i\left(k_0\right)\hfill \\ -B{K}_P^{-}\Psi \sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{x}_j\left(k_0\right)+B{K}_P^{+}\Psi \sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{e}_i\left(k_0\right)-B{K}_P^{+}\Phi \sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{e}_j\left(k_0\right)\hfill \\ +B{K}_P^{-}\Phi \sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{e}_i\left(k_0\right)-B{K}_P^{-}\Psi \sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{e}_j\left(k_0\right)+B{K}_C{x}_{ic},\hfill \end{array}$$

(29)

$$\begin{array}{c}{e}_i(k_0+1)\hfill \\ ⪰(({\vartheta }_1-{\varrho }_2)A+({\varsigma }_1-{\sigma }_2)L_{P}C)x_i\left(k_0\right)+({\vartheta }_{1}A+{\varsigma }_1{L}_{P}C)e_i\left(k_0\right)+F{v}_i\left(k_0\right),\hfill \end{array}$$

(30)

and

$$\begin{array}{c}{v}_i(k_0+1)⪰(1-\alpha )({\varsigma }_1-{\sigma }_2)L_{I}C{x}_i\left(k_0\right)+{\varsigma }_1(1-\alpha )L_{I}C{e}_i\left(k_0\right)+\alpha v_i\left(k_0\right).\hfill \end{array}$$

(31)

Define ${\tilde{x}}_i\left(k\right):={\left(x_i^{\top }\left(k\right)e_i^{\top }\left(k\right)v_i^{\top }\left(k\right)\right)}^{\top }$ and $\tilde{\mathcal{X}}\left(k\right)={({\tilde{x}}_1^{\top }\left(k\right){\tilde{x}}_2^{\top }\left(k\right)\cdots {\tilde{x}}_N^{\top }\left(k\right))}^{\top }$; it can be derived from (29)–(31) that

$$\begin{array}{c}\mathcal{X}(k_0+1)\hfill \\ ⪰\left(\begin{array}{c}\left(\begin{array}{ccc}{\varrho }_{1}A+B{K}_S^{+}\Psi +B{K}_S^{-}\Phi & B{K}_S^{+}\Psi +B{K}_S^{-}\Phi & \mathbf{0}\\ ({\vartheta }_1-{\varrho }_2)A+({\varsigma }_1-{\sigma }_2)L_{P}C& {\vartheta }_{1}A+{\varsigma }_1{L}_{P}C& F\\ (1-\alpha )({\varsigma }_1-{\sigma }_2)L_{I}C& {\varsigma }_1(1-\alpha )L_{I}C& \alpha I_n\end{array}\right)\left(\begin{array}{c}{x}_1\left(k_0\right)\\ e_1\left(k_0\right)\\ v_1\left(k_0\right)\end{array}\right)\\ ⋮\\ \left(\begin{array}{ccc}{\varrho }_{1}A+B{K}_S^{+}\Psi +B{K}_S^{-}\Phi & B{K}_S^{+}\Psi +B{K}_S^{-}\Phi & \mathbf{0}\\ ({\vartheta }_1-{\varrho }_2)A+({\varsigma }_1-{\sigma }_2)L_{P}C& {\vartheta }_{1}A+{\varsigma }_1{L}_{P}C& F\\ (1-\alpha )({\varsigma }_1-{\sigma }_2)L_{I}C& {\varsigma }_1(1-\alpha )L_{I}C& \alpha I_n\end{array}\right)\left(\begin{array}{c}{x}_N\left(k_0\right)\\ e_N\left(k_0\right)\\ v_N\left(k_0\right)\end{array}\right)\end{array}\right)+\left(\begin{array}{c}B{K}_C{x}_{1c}\\ \mathbf{0}\\ \mathbf{0}\\ ⋮\\ B{K}_C{x}_{Nc}\\ \mathbf{0}\\ \mathbf{0}\end{array}\right)\hfill \end{array}$$

$$\begin{array}{c}+\left(\begin{array}{c}\left(\begin{array}{c}(B{K}_P^{+}\Psi +B{K}_P^{-}\Phi )\sum _{j\in {\mathcal{N}}_1}{\mathcal{A}}^{\left(1j\right)}{x}_1\left(k_0\right)-(B{K}_P^{+}\Phi +B{K}_P^{-}\Psi )\sum _{j\in {\mathcal{N}}_1}{\mathcal{A}}^{\left(1j\right)}{x}_j\left(k_0\right)\\ +(B{K}_P^{+}\Psi +B{K}_P^{-}\Phi )\sum _{j\in {\mathcal{N}}_1}{\mathcal{A}}^{\left(1j\right)}{e}_1\left(k_0\right)-(B{K}_P^{+}\Phi +B{K}_P^{-}\Psi )\sum _{j\in {\mathcal{N}}_1}{\mathcal{A}}^{\left(1j\right)}{e}_j\left(k_0\right)\end{array}\right)\\ \mathbf{0}\\ \mathbf{0}\\ ⋮\\ \left(\begin{array}{c}(B{K}_P^{+}\Psi +B{K}_P^{-}\Phi )\sum _{j\in {\mathcal{N}}_N}{\mathcal{A}}^{\left(Nj\right)}{x}_N\left(k_0\right)-(B{K}_P^{+}\Phi +B{K}_P^{-}\Psi )\sum _{j\in {\mathcal{N}}_N}{\mathcal{A}}^{\left(Nj\right)}{x}_j\left(k_0\right)\\ +(B{K}_P^{+}\Psi +B{K}_P^{-}\Phi )\sum _{j\in {\mathcal{N}}_N}{\mathcal{A}}^{\left(Nj\right)}{e}_N\left(k_0\right)-(B{K}_P^{+}\Phi +B{K}_P^{-}\Psi )\sum _{j\in {\mathcal{N}}_N}{\mathcal{A}}^{\left(Nj\right)}{e}_j\left(k_0\right)\end{array}\right)\\ \mathbf{0}\\ \mathbf{0}\end{array}\right)\hfill \\ ={\mathbb{A}}_1\mathcal{X}\left(k_0\right)+{\mathbb{C}}_1(X_C\otimes {\mathbf{1}}_3),\hfill \end{array}$$

where

$$\begin{array}{c}{\mathbb{A}}_1^{\left(ii\right)}=\left(\begin{array}{ccc}\left(\begin{array}{c}{\varrho }_{1}A+B{K}_S^{+}\Psi +B{K}_S^{-}\Phi \\ +(B{K}_P^{+}\Psi +B{K}_P^{-}\Phi )\sum _{j\in {\mathcal{N}}_i}^N{\mathcal{A}}^{\left(ij\right)}\end{array}\right)& \left(\begin{array}{c}B{K}_S^{+}\Psi +B{K}_S^{-}\Phi \\ +(B{K}_P^{+}\Psi +B{K}_P^{-}\Phi )\sum _{j\in {\mathcal{N}}_i}^N{\mathcal{A}}^{\left(ij\right)}\end{array}\right)& \mathbf{0}\\ ({\vartheta }_1-{\varrho }_2)A+({\varsigma }_1-{\sigma }_2)L_{P}C& {\vartheta }_{1}A+{\varsigma }_1{L}_{P}C& F\\ (1-\alpha )({\varsigma }_1-{\sigma }_2)L_{I}C& {\varsigma }_1(1-\alpha )L_{I}C& \alpha I_n\end{array}\right),\hfill \end{array}$$

$$\begin{array}{c}{\mathbb{A}}_1^{\left(ij\right)}=\left(\begin{array}{ccc}-{\mathcal{A}}^{\left(ij\right)}(B{K}_P^{+}\Phi +B{K}_P^{-}\Psi )& -{\mathcal{A}}^{\left(ij\right)}(B{K}_P^{+}\Phi +B{K}_P^{-}\Psi )& \mathbf{0}\\ \mathbf{0}& \mathbf{0}& \mathbf{0}\\ \mathbf{0}& \mathbf{0}& \mathbf{0}\end{array}\right),\hfill \\ {\mathbb{C}}_1^{\left(ii\right)}=\left(\begin{array}{ccc}B{K}_C& \mathbf{0}& \mathbf{0}\\ \mathbf{0}& \mathbf{0}& \mathbf{0}\\ \mathbf{0}& \mathbf{0}& \mathbf{0}\end{array}\right),{\mathbb{C}}_1^{\left(ij\right)}=\mathbf{0}.\hfill \end{array}$$

Using (25a) and (25c)–(25g) gives that ${\mathbb{A}}_1^{\left(ii\right)}⪰0$. Similarly, ${\mathbb{A}}_1^{\left(ij\right)}⪰0$ can be obtained by (25b). Consequently, it follows that ${\mathbb{A}}_1⪰0$. Since ${\delta }_{kc}^{\left(\iota \right)}\succ 0$, it is easy to concluded that ${\mathbb{C}}_1⪰0$. Therefore, the positivity of system $\mathcal{X}\left(k\right)$ can be guaranteed by Lemma 1 under Assumption 1.

Afterwards, the stability analysis is conducted by introducing the following variables:

$$\begin{array}{c}{\xi }_i\left(k\right)=\sum _{\iota =1}^N{\theta }_{\iota }{x}_{\iota }\left(k\right)-x_i\left(k\right)-x_{ic},\hfill \\ {\varpi }_i\left(k\right)=\sum _{\iota =1}^N{\theta }_{\iota }{e}_{\iota }\left(k\right)-e_i\left(k\right),\hfill \end{array}$$

(32)

and

$$\begin{array}{c}{\zeta }_i\left(k\right)=\sum _{\iota =1}^N{\theta }_{\iota }{v}_{\iota }\left(k\right)-v_i\left(k\right).\hfill \end{array}$$

(33)

where ${\theta }_{\iota }>1,\theta ={({\theta }_1{\theta }_2\cdots {\theta }_N)}^{\top }=\lambda r$, $r={(r_1,r_2,\cdots ,r_3)}^{\top }$ is the left eigenvector of $\mathcal{L}$ associated with a zero eigenvalue. Let $\xi \left(k\right)={({\xi }_1^{\top }\left(k\right){\xi }_2^{\top }\left(k\right)\cdots {\xi }_N^{\top }\left(k\right))}^{\top }$, $\varpi \left(k\right)={({\varpi }_1^{\top }\left(k\right){\varpi }_2^{\top }\left(k\right)\cdots {\varpi }_N^{\top }\left(k\right))}^{\top }$, $\zeta \left(k\right)={({\zeta }_1^{\top }\left(k\right){\zeta }_2^{\top }\left(k\right)\cdots {\zeta }_N^{\top }\left(k\right))}^{\top }$, $X\left(k\right)={(x_1^{\top }\left(k\right)x_2^{\top }\left(k\right)\cdots x_N^{\top }\left(k\right))}^{\top }$, $\widehat{X}\left(k\right)=({\widehat{x}}_1^{\top }\left(k\right){\widehat{x}}_2^{\top }\left(k\right)\cdots {\widehat{x}}_N^{\top }\left(k\right))$, $E\left(k\right)={(e_1^{\top }\left(k\right)e_2^{\top }\left(k\right)\cdots e_N^{\top }\left(k\right))}^{\top }$, $X_C={(x_{1c}^{\top }{x}_{2c}^{\top }\cdots x_{Nc}^{\top })}^{\top }$, $\mathsf{V}\left(k\right)=(v_1^{\top }\left(k\right)v_2^{\top }\left(k\right)\cdots v_N^{\top }\left(k\right))$, and $W\left(k\right)=(w_1^{\top }\left(k\right)w_2^{\top }\left(k\right)\cdots w_N^{\top }\left(k\right))$; it is easy to obtain that

$$\begin{array}{c}\xi \left(k\right)=\Theta X\left(k\right)-X_C,\varpi \left(k\right)=\Theta E\left(k\right),\zeta \left(k\right)=\Theta \mathsf{V}\left(k\right),\hfill \end{array}$$

(34)

where $\Theta ={\mathbf{1}}_N{\theta }^{\top }\otimes I_n-I_{Nn}.$ Construct the following augmented system:

$$\begin{array}{cc}\hfill & \tilde{\mathcal{X}}(k+1)=\left(\begin{array}{c}\xi (k+1)\\ \varpi (k+1)\\ \zeta (k+1)\end{array}\right)=\left(\begin{array}{c}\Theta X(k+1)-X_C\\ \Theta E(k+1)\\ \Theta \mathsf{V}(k+1)\end{array}\right)\hfill \end{array}.$$

(35)

Consider the positivity of the new augmented system (35); it is easy to obtain that

$$\begin{array}{c}X(k+1)⪰\left(\begin{array}{c}\left(\begin{array}{c}({\varrho }_{1}A+B{K}_S^{+}\Psi +B{K}_S^{-}\Phi )x_1\left(k_0\right)+(B{K}_S^{+}\Psi +B{K}_S^{-}\Phi )e_1\left(k_0\right)\\ +(B{K}_P^{+}\Psi +B{K}_P^{-}\Phi )\sum _{j\in {\mathcal{N}}_1}{\mathcal{A}}^{\left(1j\right)}{x}_1\left(k_0\right)-(B{K}_P^{+}\Phi +B{K}_P^{-}\Psi )\sum _{j\in {\mathcal{N}}_1}{\mathcal{A}}^{\left(1j\right)}{x}_j\left(k_0\right)\\ +(B{K}_P^{+}\Psi +B{K}_P^{-}\Phi )\sum _{j\in {\mathcal{N}}_1}{\mathcal{A}}^{\left(1j\right)}{e}_1\left(k_0\right)-(B{K}_P^{+}\Phi +B{K}_P^{-}\Psi )\sum _{j\in {\mathcal{N}}_1}{\mathcal{A}}^{\left(1j\right)}{e}_j\left(k_0\right)+B{K}_C{x}_{ic}\end{array}\right)\\ ⋮\\ \left(\begin{array}{c}({\varrho }_{1}A+B{K}_S^{+}\Psi +B{K}_S^{-}\Phi )x_N\left(k_0\right)+(B{K}_S^{+}\Psi +B{K}_S^{-}\Phi )e_N\left(k_0\right)\\ +(B{K}_P^{+}\Psi +B{K}_P^{-}\Phi )\sum _{j\in {\mathcal{N}}_N}{\mathcal{A}}^{\left(Nj\right)}{x}_N\left(k_0\right)-(B{K}_P^{+}\Phi +B{K}_P^{-}\Psi )\sum _{j\in {\mathcal{N}}_N}{\mathcal{A}}^{\left(Nj\right)}{x}_j\left(k_0\right)\\ +(B{K}_P^{+}\Psi +B{K}_P^{-}\Phi )\sum _{j\in {\mathcal{N}}_N}{\mathcal{A}}^{\left(Nj\right)}{e}_N\left(k_0\right)-(B{K}_P^{+}\Phi +B{K}_P^{-}\Psi )\sum _{j\in {\mathcal{N}}_N}{\mathcal{A}}^{\left(Nj\right)}{e}_j\left(k_0\right)+B{K}_C{x}_{Nc}\end{array}\right)\end{array}\right),\hfill \end{array}$$

$$\begin{array}{c}E(k+1)⪰\left(\begin{array}{c}({\vartheta }_{1}A+{\varsigma }_1{L}_{P}C)e_1\left(k\right)+(({\vartheta }_1-{\varrho }_2)A+({\varsigma }_1-{\sigma }_2)L_{P}C)x_1\left(k\right)+F{\nu }_1\left(k\right)\\ ⋮\\ ({\vartheta }_{1}A+{\varsigma }_1{L}_{P}C)e_N\left(k\right)+(({\vartheta }_1-{\varrho }_2)A+({\varsigma }_1-{\sigma }_2)L_{P}C)x_N\left(k\right)+F{v}_N\left(k\right)\end{array}\right),\hfill \end{array}$$

and

$$\begin{array}{c}\mathsf{V}(k+1)⪰\hfill \end{array}\left(\begin{array}{c}\alpha v_1\left(k\right)+(1-\alpha )L_{I}C({\varsigma }_1{e}_1\left(k\right)+({\varsigma }_1-{\sigma }_2)x_1\left(k\right))\\ ⋮\\ \alpha v_N\left(k\right)+(1-\alpha )L_{I}C({\varsigma }_1{e}_N\left(k\right)+({\varsigma }_1-{\sigma }_2)x_N\left(k\right))\end{array}\right).$$

Define $\overline{\mathcal{D}}=:diag\{d_{max},\cdots ,d_{max}\}\in {\mathbb{R}}^N$. Then, it can be shown that

$$\begin{array}{c}X(k+1)⪰(I_N\otimes ({\varrho }_{1}A+B{K}_S^{+}\Psi +B{K}_S^{-}\Phi )+2\overline{\beta }\overline{\mathcal{D}}\otimes (B{K}_P^{-}{\mathbf{1}}_{n\times n}-B{K}_P^{+}{\mathbf{1}}_{n\times n})\hfill \\ +\mathcal{L}\otimes (B{K}_P^{+}\Phi +B{K}_P^{-}\Psi ))X\left(k\right)+(I_N\otimes (B{K}_S^{+}\Psi +B{K}_S^{-}\Phi )+2\overline{\beta \mathcal{D}}\otimes (B{K}_P^{-}{\mathbf{1}}_{n\times n}\hfill \\ -B{K}_P^{+}{\mathbf{1}}_{n\times n})+\mathcal{L}\otimes (B{K}_P^{+}\Phi +B{K}_P^{-}\Psi ))E\left(k\right)+(I_N\otimes \left(B{K}_C\right))X_C,\hfill \end{array}$$

(36)

$$\begin{array}{c}E(k+1)⪰(I_N\otimes ({\vartheta }_{1}A+{\varsigma }_1{L}_{P}C))E\left(k\right)+(I_N\otimes (({\vartheta }_1-{\varrho }_2)A\hfill \\ +({\varsigma }_1-{\sigma }_2)L_{P}C\left)\right)X\left(k\right)+(I_N\otimes F)\mathsf{V}\left(k\right),\hfill \end{array}$$

(37)

and

$$\begin{array}{c}\mathsf{V}(k+1)⪰{\varsigma }_1(1-\alpha )(I_N\otimes \left(L_{I}C\right))E\left(k\right)\hfill \\ +(1-\alpha )({\varsigma }_1-{\sigma }_2)(I_N\otimes \left(L_{I}C\right))X\left(k\right)+\alpha \mathsf{V}\left(k\right).\hfill \end{array}$$

(38)

With the consideration of $\mathcal{L}{\mathbf{1}}_N{\theta }^{\top }=0$ and ${\mathbf{1}}_N{\theta }^{\top }\mathcal{L}=\lambda {\mathbf{1}}_N{r}^{\top }\mathcal{L}=0$, it can be concluded that

$$\begin{array}{c}({\mathbf{1}}_N{\theta }^{\top }\otimes I_n-I_{Nn})(I_N\otimes ({\varrho }_{1}A+B{K}_S^{+}\Psi +B{K}_S^{-}\Phi )+2\overline{\beta \mathcal{D}}\otimes (B{K}_P^{-}{\mathbf{1}}_{n\times n}-B{K}_P^{+}{\mathbf{1}}_{n\times n})+\mathcal{L}\otimes (B{K}_P^{+}\Phi +B{K}_P^{-}\Psi ))\hfill \\ ={\mathbf{1}}_N{\theta }^{\top }\otimes ({\varrho }_{1}A+B{K}_S^{+}\Psi +B{K}_S^{-}\Phi )+2\overline{\beta }{\mathbf{1}}_N{\theta }^{\top }\overline{\mathcal{D}}\otimes (B{K}_P^{-}{\mathbf{1}}_{n\times n}-B{K}_P^{+}{\mathbf{1}}_{n\times n})+{\mathbf{1}}_N{\theta }^{\top }\mathcal{L}\otimes (B{K}_P^{+}\Phi +B{K}_P^{-}\Psi )\hfill \\ -(I_N\otimes ({\varrho }_{1}A+B{K}_S^{+}\Psi +B{K}_S^{-}\Phi )+2\overline{\beta \mathcal{D}}\otimes (B{K}_P^{-}{\mathbf{1}}_{n\times n}-B{K}_P^{+}{\mathbf{1}}_{n\times n})+\mathcal{L}\otimes (B{K}_P^{+}\Phi +B{K}_P^{-}\Psi ))\hfill \\ ={\mathbf{1}}_N{\theta }^{\top }\otimes ({\varrho }_{1}A+B{K}_S^{+}\Psi +B{K}_S^{-}\Phi )+2\overline{\beta \mathcal{D}}{\mathbf{1}}_N{\theta }^{\top }\otimes (B{K}_P^{-}{\mathbf{1}}_{n\times n}-B{K}_P^{+}{\mathbf{1}}_{n\times n})+\mathcal{L}{\mathbf{1}}_N{\theta }^{\top }\otimes (B{K}_P^{+}\Phi +B{K}_P^{-}\Psi )\hfill \\ -(I_N\otimes ({\varrho }_{1}A+B{K}_S^{+}\Psi +B{K}_S^{-}\Phi )+2\overline{\beta \mathcal{D}}\otimes (B{K}_P^{-}{\mathbf{1}}_{n\times n}-B{K}_P^{+}{\mathbf{1}}_{n\times n})+\mathcal{L}\otimes (B{K}_P^{+}\Phi +B{K}_P^{-}\Psi ))\hfill \\ =(I_N\otimes ({\varrho }_{1}A+B{K}_S^{+}\Psi +B{K}_S^{-}\Phi )+2\overline{\beta \mathcal{D}}\otimes (B{K}_P^{-}{\mathbf{1}}_{n\times n}-B{K}_P^{+}{\mathbf{1}}_{n\times n})+\mathcal{L}\otimes (B{K}_P^{+}\Phi +B{K}_P^{-}\Psi ))({\mathbf{1}}_N{\theta }^{\top }\otimes I_n-I_{Nn}),\hfill \end{array}$$

On this basis, it can be derived that

$$\begin{array}{c}\xi (k+1)⪰({\mathbf{1}}_N{\theta }^{\top }\otimes I_n-I_{Nn})(I_N\otimes ({\varrho }_{1}A+B{K}_S^{+}\Psi +B{K}_S^{-}\Phi )+2\overline{\beta \mathcal{D}}\otimes (B{K}_P^{-}{\mathbf{1}}_{n\times n}-B{K}_P^{+}{\mathbf{1}}_{n\times n})\hfill \\ +\mathcal{L}\otimes (B{K}_P^{+}\Phi +B{K}_P^{-}\Psi ))X\left(k\right)+(I_N\otimes (B{K}_S^{+}\Psi +B{K}_S^{-}\Phi )+2\overline{\beta \mathcal{D}}\otimes (B{K}_P^{-}{\mathbf{1}}_{n\times n}-B{K}_P^{+}{\mathbf{1}}_{n\times n})\hfill \\ +\mathcal{L}\otimes (B{K}_P^{+}\Phi +B{K}_P^{-}\Psi ))E\left(k\right)+(I_N\otimes \left(B{K}_C\right))X_C-X_C\hfill \\ =(I_N\otimes ({\varrho }_{1}A+B{K}_S^{+}\Psi +B{K}_S^{-}\Phi )+2\overline{\beta \mathcal{D}}\otimes (B{K}_P^{-}{\mathbf{1}}_{n\times n}-B{K}_P^{+}{\mathbf{1}}_{n\times n})+\mathcal{L}\otimes (B{K}_P^{+}\Phi +B{K}_P^{-}\Psi ))\hfill \\ +\xi \left(k\right)+(I_N\otimes (B{K}_S^{+}\Psi +B{K}_S^{-}\Phi )+2\overline{\beta \mathcal{D}}\otimes (B{K}_P^{-}{\mathbf{1}}_{n\times n}-B{K}_P^{+}{\mathbf{1}}_{n\times n})+\mathcal{L}\otimes (B{K}_P^{+}\Phi +B{K}_P^{-}\Psi ))\varpi \left(k\right)\hfill \\ +(I_N\otimes ({\varrho }_{1}A+B{K}_S^{+}\Psi +B{K}_S^{-}\Phi )+2\overline{\beta \mathcal{D}}\otimes (B{K}_P^{-}{\mathbf{1}}_{n\times n}-B{K}_P^{+}{\mathbf{1}}_{n\times n})+\mathcal{L}\otimes (B{K}_P^{+}\Phi +B{K}_P^{-}\Psi )\hfill \\ +({\mathbf{1}}_N{\theta }^{\top }-I_N)\otimes \left(B{K}_C\right)-I_{Nn})X_C,\hfill \end{array}$$

(39)

$$\begin{array}{c}\varpi (k+1)⪰(I_N\otimes (({\vartheta }_1-{\varrho }_2)A+({\varsigma }_1-{\sigma }_2)L_{P}C))\xi \left(k\right)+(I_N\otimes ({\vartheta }_{1}A\hfill \\ +{\varsigma }_1{L}_{P}C\left)\right)\varpi \left(k\right)+(I_N\otimes F)\zeta \left(k\right)+(I_N\otimes (({\vartheta }_1-{\varrho }_2)A+({\varsigma }_1-{\sigma }_2)L_{P}C))X_C,\hfill \end{array}$$

(40)

and

$$\begin{array}{c}\zeta (k+1)⪰{\varsigma }_1(1-\alpha )(I_N\otimes \left(L_{I}C\right))\varpi \left(k\right)+(1-\alpha )({\varsigma }_1-{\sigma }_2)(I_N\otimes \left(L_{I}C\right))\xi \left(k\right)\hfill \\ +\alpha \zeta \left(k\right)+(1-\alpha )({\varsigma }_1-{\sigma }_2)(I_N\otimes \left(L_{I}C\right))X_C.\hfill \end{array}$$

(41)

By (36)–(41), one can obtain that

$$\begin{array}{c}\tilde{\mathcal{X}}(k+1)\hfill \end{array}⪰\left(\begin{array}{c}\left(\begin{array}{c}(I_N\otimes ({\varrho }_{1}A+B{K}_S^{+}\Psi +B{K}_S^{-}\Phi )+2\overline{\beta \mathcal{D}}\otimes (B{K}_P^{-}{\mathbf{1}}_{n\times n}-B{K}_P^{+}{\mathbf{1}}_{n\times n})+\mathcal{L}\otimes (B{K}_P^{+}\Phi +B{K}_P^{-}\Psi ))\xi \left(k\right)\\ +(I_N\otimes (B{K}_S^{+}\Psi +B{K}_S^{-}\Phi )+2\overline{\beta \mathcal{D}}\otimes (B{K}_P^{-}{\mathbf{1}}_{n\times n}-B{K}_P^{+}{\mathbf{1}}_{n\times n})+\mathcal{L}\otimes (B{K}_P^{+}\Phi +B{K}_P^{-}\Psi ))\varpi \left(k\right)\\ +(I_N\otimes ({\varrho }_{1}A+B{K}_S^{+}\Psi +B{K}_S^{-}\Phi )+2\overline{\beta \mathcal{D}}\otimes (B{K}_P^{-}{\mathbf{1}}_{n\times n}-B{K}_P^{+}{\mathbf{1}}_{n\times n})+\mathcal{L}\otimes (B{K}_P^{+}\Phi +B{K}_P^{-}\Psi )\\ +({\mathbf{1}}_N{\theta }^{\top }-I_N)\otimes \left(B{K}_C\right)-I_{Nn})X_C\end{array}\right)\\ \left(\begin{array}{c}(I_N\otimes (({\vartheta }_1-{\varrho }_2)A+({\varsigma }_1-{\sigma }_2)L_{P}C))\xi \left(k\right)+(I_N\otimes ({\vartheta }_{1}A+{\varsigma }_1{L}_{P}C))\varpi \left(k\right)\\ +(I_N\otimes F)\zeta \left(k\right)+(I_N\otimes (({\vartheta }_1-{\varrho }_2)A+({\varsigma }_1-{\sigma }_2)L_{P}C))X_C\end{array}\right)\\ \left(\begin{array}{c}{\varsigma }_1(1-\alpha )(I_N\otimes \left(L_{I}C\right))\varpi \left(k\right)+(1-\alpha )({\varsigma }_1-{\sigma }_2)(I_N\otimes \left(L_{I}C\right))\xi \left(k\right)\\ +\alpha \zeta \left(k\right)+(1-\alpha )({\varsigma }_1-{\sigma }_2)(I_N\otimes \left(L_{I}C\right))X_C\end{array}\right)\end{array}\right)$$

$$\begin{array}{c}=\left(\begin{array}{ccc}\left(\begin{array}{c}{I}_N\otimes ({\varrho }_{1}A+B{K}_S^{+}\Psi +B{K}_S^{-}\Phi \\ +2\overline{\beta \mathcal{D}}\otimes (B{K}_P^{-}{\mathbf{1}}_{n\times n}-B{K}_P^{+}{\mathbf{1}}_{n\times n})\\ +\mathcal{L}\otimes (B{K}_P^{+}\Phi +B{K}_P^{-}\Psi )\end{array}\right)& \left(\begin{array}{c}{I}_N\otimes (B{K}_S^{+}\Psi +B{K}_S^{-}\Phi +\\ 2\overline{\beta \mathcal{D}}\otimes (B{K}_P^{-}{\mathbf{1}}_{n\times n}-B{K}_P^{+}{\mathbf{1}}_{n\times n})\\ +\mathcal{L}\otimes (B{K}_P^{+}\Phi +B{K}_P^{-}\Psi )\end{array}\right)& \mathbf{0}\\ I_N\otimes (({\vartheta }_1-{\varrho }_2)A+({\varsigma }_1-{\sigma }_2)L_{P}C)& I_N\otimes ({\vartheta }_{1}A+{\varsigma }_1{L}_{P}C)& I_N\otimes F\\ (1-\alpha )({\varsigma }_1-{\sigma }_2)(I_N\otimes \left(L_{I}C\right))& {\varsigma }_1(1-\alpha )(I_N\otimes \left(L_{I}C\right))& \alpha I_{Nn}\end{array}\right)\left(\begin{array}{c}\xi \left(k\right)\\ \varpi \left(k\right)\\ \zeta \left(k\right)\end{array}\right)\hfill \\ +\left(\begin{array}{c}\left(\begin{array}{c}{I}_N\otimes ({\varrho }_{1}A+B{K}_S^{+}\Psi +B{K}_S^{-}\Phi )+2\overline{\beta \mathcal{D}}\otimes (B{K}_P^{-}{\mathbf{1}}_{n\times n}-B{K}_P^{+}{\mathbf{1}}_{n\times n})\\ +\mathcal{L}\otimes (B{K}_P^{+}\Phi +B{K}_P^{-}\Psi )+({\mathbf{1}}_N{\theta }^{\top }-I_N)\otimes \left(B{K}_C\right)-I_{Nn}\end{array}\right)\\ I_N\otimes (({\vartheta }_1-{\varrho }_2)A+({\varsigma }_1-{\sigma }_2)L_{P}C)\\ (1-\alpha )({\varsigma }_1-{\sigma }_2)(I_N\otimes \left(L_{I}C\right))\end{array}\right)\otimes X_C={\mathbb{A}}_2\tilde{\mathcal{X}}\left(k\right)+{\mathbb{C}}_2\otimes X_C.\hfill \end{array}$$

where

$$\begin{array}{c}{\mathbb{A}}_2=\left(\begin{array}{ccc}\left(\begin{array}{c}{I}_N\otimes ({\varrho }_{1}A+B{K}_S^{+}\Psi +B{K}_S^{-}\Phi \\ +2\overline{\beta \mathcal{D}}\otimes (B{K}_P^{-}{\mathbf{1}}_{n\times n}-B{K}_P^{+}{\mathbf{1}}_{n\times n})\\ +\mathcal{L}\otimes (B{K}_P^{+}\Phi +B{K}_P^{-}\Psi )\end{array}\right)& \left(\begin{array}{c}{I}_N\otimes (B{K}_S^{+}\Psi +B{K}_S^{-}\Phi +\\ 2\overline{\beta \mathcal{D}}\otimes (B{K}_P^{-}{\mathbf{1}}_{n\times n}-B{K}_P^{+}{\mathbf{1}}_{n\times n})\\ +\mathcal{L}\otimes (B{K}_P^{+}\Phi +B{K}_P^{-}\Psi )\end{array}\right)& \mathbf{0}\\ I_N\otimes (({\vartheta }_1-{\varrho }_2)A+({\varsigma }_1-{\sigma }_2)L_{P}C)& I_N\otimes ({\vartheta }_{1}A+{\varsigma }_1{L}_{P}C)& I_N\otimes F\\ (1-\alpha )({\varsigma }_1-{\sigma }_2)(I_N\otimes \left(L_{I}C\right))& {\varsigma }_1(1-\alpha )(I_N\otimes \left(L_{I}C\right))& \alpha I_{Nn}\end{array}\right),\hfill \end{array}$$

$$\begin{array}{c}{\mathbb{C}}_2=\left(\begin{array}{c}\left(\begin{array}{c}{I}_N\otimes ({\varrho }_{1}A+B{K}_S^{+}\Psi +B{K}_S^{-}\Phi )+2\overline{\beta \mathcal{D}}\otimes (B{K}_P^{-}{\mathbf{1}}_{n\times n}-B{K}_P^{+}{\mathbf{1}}_{n\times n})\\ +\mathcal{L}\otimes (B{K}_P^{+}\Phi +B{K}_P^{-}\Psi )+({\mathbf{1}}_N{\theta }^{\top }-I_N)\otimes \left(B{K}_C\right)-I_{Nn}\end{array}\right)\\ I_N\otimes (({\vartheta }_1-{\varrho }_2)A+({\varsigma }_1-{\sigma }_2)L_{P}C)\\ (1-\alpha )({\varsigma }_1-{\sigma }_2)(I_N\otimes \left(L_{I}C\right))\end{array}\right).\hfill \end{array}$$

Using (25a)–(25d), it is derived that ${\mathbb{A}}_2⪰0$, while (25c) and (25e) give that ${\mathbb{C}}_2⪰0.$ Then, the introduced variables $\xi \left(k\right),\varpi \left(k\right)$, and $\zeta \left(k\right)$ can remain positive by Lemma 1. Next, we analyze the stability of system (35), since

$$\begin{array}{c}{x}_i(k_0+1)⪯{\varrho }_{2}A{x}_i\left(k_0\right)+B{K}_S^{+}{\widehat{x}}_i\left(k_0\right)+B{K}_S^{-}{\widehat{x}}_i\left(k_0\right)+\overline{\beta }B{K}_S^{+}{\mathbf{1}}_{n\times n}{\widehat{x}}_i\left(k_0\right)\hfill \\ -\overline{\beta }B{K}_S^{-}{\mathbf{1}}_{n\times n}{\widehat{x}}_i\left(k_0\right)+B{K}_P^{+}\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{\widehat{x}}_i\left(k_0\right)+B{K}_P^{-}\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{\widehat{x}}_i\left(k_0\right)\hfill \\ -B{K}_P^{+}\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{\widehat{x}}_j\left(k_0\right)-B{K}_P^{-}\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{\widehat{x}}_j\left(k_0\right)+\overline{\beta }B{K}_P^{+}{\mathbf{1}}_{n\times n}\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{\widehat{x}}_i\left(k_0\right)\hfill \\ -\overline{\beta }B{K}_P^{-}{\mathbf{1}}_{n\times n}\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{\widehat{x}}_i\left(k_0\right)+\overline{\beta }B{K}_P^{+}{\mathbf{1}}_{n\times n}\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{\widehat{x}}_j\left(k_0\right)\hfill \\ -\overline{\beta }B{K}_P^{-}{\mathbf{1}}_{n\times n}\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{\widehat{x}}_j\left(k_0\right)+B{K}_C{x}_{ic}\hfill \\ =({\varrho }_{2}A+B{K}_S^{+}\Phi +B{K}_S^{-}\Psi )x_i\left(k_0\right)+(B{K}_S^{+}\Phi +B{K}_S^{-}\Psi )e_i\left(k_0\right)+B{K}_C{x}_{ic}\hfill \\ +B{K}_P^{+}\Phi \sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{x}_i\left(k_0\right)-B{K}_P^{+}\Psi \sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{x}_j\left(k_0\right)+B{K}_P^{-}\Psi \sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{x}_i\left(k_0\right)\hfill \\ -B{K}_P^{-}\Phi \sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{x}_j\left(k_0\right)+B{K}_P^{+}\Phi \sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{e}_i\left(k_0\right)-B{K}_P^{+}\Psi \sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{e}_j\left(k_0\right)\hfill \\ +B{K}_P^{-}\Psi \sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{e}_i\left(k_0\right)-B{K}_P^{-}\Phi \sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{e}_j\left(k_0\right).\hfill \end{array}$$

(42)

It is evident that

$$\begin{array}{c}X(k+1)⪯\left(\begin{array}{c}\left(\begin{array}{c}({\varrho }_{2}A+B{K}_S^{+}\Phi +B{K}_S^{-}\Psi )x_1\left(k\right)+(B{K}_S^{+}\Phi +B{K}_S^{-}\Psi )e_1\left(k\right)\\ +(B{K}_P^{+}\Phi +B{K}_P^{-}\Psi )\sum _{j\in {\mathcal{N}}_1}{\mathcal{A}}^{\left(1j\right)}{x}_1\left(k\right)-(B{K}_P^{+}\Psi +B{K}_P^{-}\Phi )\sum _{j\in {\mathcal{N}}_1}{\mathcal{A}}^{\left(1j\right)}{x}_j\left(k\right)\\ +(B{K}_P^{+}\Phi +B{K}_P^{-}\Psi )\sum _{j\in {\mathcal{N}}_1}{\mathcal{A}}^{\left(1j\right)}{e}_1\left(k\right)-(B{K}_P^{+}\Psi +B{K}_P^{-}\Phi )\sum _{j\in {\mathcal{N}}_1}{\mathcal{A}}^{\left(1j\right)}{e}_j\left(k\right)+B{K}_C{x}_{ic}\end{array}\right)\\ ⋮\\ \left(\begin{array}{c}({\varrho }_{2}A+B{K}_S^{+}\Phi +B{K}_S^{-}\Psi )x_1\left(k\right)+(B{K}_S^{+}\Phi +B{K}_S^{-}\Psi )e_N\left(k\right)\\ +(B{K}_P^{+}\Phi +B{K}_P^{-}\Psi )\sum _{j\in {\mathcal{N}}_N}{\mathcal{A}}^{\left(Nj\right)}{x}_N\left(k\right)-(B{K}_P^{+}\Psi +B{K}_P^{-}\Phi )\sum _{j\in {\mathcal{N}}_N}{\mathcal{A}}^{\left(Nj\right)}{x}_j\left(k\right)\\ +(B{K}_P^{+}\Phi +B{K}_P^{-}\Psi )\sum _{j\in {\mathcal{N}}_N}{\mathcal{A}}^{\left(Nj\right)}{e}_N\left(k\right)-(B{K}_P^{+}\Psi +B{K}_P^{-}\Phi )\sum _{j\in {\mathcal{N}}_N}{\mathcal{A}}^{\left(Nj\right)}{e}_j\left(k\right)+B{K}_C{x}_{Nc}\end{array}\right)\end{array}\right),\hfill \end{array}$$

$$\begin{array}{c}E(k+1)⪯\left(\begin{array}{c}({\vartheta }_{2}A+{\varsigma }_2{L}_{P}C)e_1\left(k\right)+(({\vartheta }_2-{\varrho }_1)A+({\varsigma }_2-{\sigma }_1)L_{P}C)x_1\left(k\right)+F{v}_1\left(k\right)\\ ⋮\\ ({\vartheta }_{2}A+{\varsigma }_2{L}_{P}C)e_N\left(k\right)+(({\vartheta }_2-{\varrho }_1)A+({\varsigma }_2-{\sigma }_1)L_{P}C)x_N\left(k\right)+F{v}_N\left(k\right)\end{array}\right),\hfill \end{array}$$

and

$$\begin{array}{c}\mathsf{V}(k+1)⪯\left(\begin{array}{c}\alpha v_1\left(k\right)+(1-\alpha )L_{I}C({\varsigma }_2{e}_1\left(k\right)+({\varsigma }_2-{\sigma }_1)x_1\left(k\right))\\ ⋮\\ \alpha v_N\left(k\right)+(1-\alpha )L_{I}C({\varsigma }_2{e}_N\left(k\right)+({\varsigma }_2-{\sigma }_1)x_N\left(k\right))\end{array}\right).\hfill \end{array}$$

This can be rewritten as

$$\begin{array}{c}X(k+1)⪯(I_N\otimes ({\varrho }_{2}A+B{K}_S^{+}\Phi +B{K}_S^{-}\Psi )+2\overline{\beta \mathcal{D}}\otimes (B{K}_P^{+}{\mathbf{1}}_{n\times n}-B{K}_P^{-}{\mathbf{1}}_{n\times n})\\ +\mathcal{L}\otimes (B{K}_P^{+}\Psi +B{K}_P^{-}\Phi ))X\left(k\right)+(I_N\otimes (B{K}_S^{+}\Phi +B{K}_S^{-}\Psi )+2\overline{\beta \mathcal{D}}\otimes (B{K}_P^{+}{\mathbf{1}}_{n\times n}\\ -B{K}_P^{-}{\mathbf{1}}_{n\times n})+\mathcal{L}\otimes (B{K}_P^{+}\Psi +B{K}_P^{-}\Phi ))E\left(k\right)+(I_N\otimes \left(B{K}_C\right))X_C,\end{array}$$

(43)

$$\begin{array}{c}E(k+1)⪯(I_N\otimes ({\vartheta }_{2}A+{\varsigma }_2{L}_{P}C))E\left(k\right)\\ +(I_N\otimes (({\vartheta }_2-{\varrho }_1)A+({\varsigma }_2-{\sigma }_1)L_{P}C))X\left(k\right)+(I_N\otimes F)\mathsf{V}\left(k\right),\end{array}$$

(44)

and

$$\begin{array}{c}\mathsf{V}(k+1)={\varsigma }_2(1-\alpha )(I_N\otimes \left(L_{I}C\right))E\left(k\right)\\ +(1-\alpha )({\varsigma }_2-{\sigma }_1)(I_N\otimes \left(L_{I}C\right))X\left(k\right)+\alpha I_{Nn}\mathsf{V}\left(k\right).\end{array}$$

(45)

This results in

$$\begin{array}{c}\xi (k+1)⪯(I_N\otimes ({\varrho }_{2}A+B{K}_S^{+}\Phi +B{K}_S^{-}\Psi )+2\overline{\beta \mathcal{D}}\otimes (B{K}_P^{+}{\mathbf{1}}_{n\times n}-B{K}_P^{-}{\mathbf{1}}_{n\times n})\\ +\mathcal{L}\otimes (B{K}_P^{+}\Psi +B{K}_P^{-}\Phi ))\xi \left(k\right)+(I_N\otimes (B{K}_S^{+}\Phi +B{K}_S^{-}\Psi )+2\overline{\beta \mathcal{D}}\otimes (B{K}_P^{+}{\mathbf{1}}_{n\times n}\\ -B{K}_P^{-}{\mathbf{1}}_{n\times n})+\mathcal{L}\otimes (B{K}_P^{+}\Psi +B{K}_P^{-}\Phi ))\varpi \left(k\right)+(I_N\otimes ({\varrho }_{2}A+B{K}_S^{+}\Phi +B{K}_S^{-}\Psi )\\ +2\overline{\beta \mathcal{D}}\otimes (B{K}_P^{+}{\mathbf{1}}_{n\times n}-B{K}_P^{-}{\mathbf{1}}_{n\times n})+\mathcal{L}\otimes (B{K}_P^{+}\Psi +B{K}_P^{-}\Phi )\\ +({\mathbf{1}}_N{\theta }^{\top }-I_N)\otimes \left(B{K}_C\right)-I_{Nn})X_C,\end{array}$$

(46)

$$\begin{array}{c}\varpi (k+1)⪯(I_N\otimes (({\vartheta }_2-{\varrho }_1)A+({\varsigma }_2-{\sigma }_1)L_{P}C))\xi \left(k\right)+(I_N\otimes ({\vartheta }_{2}A\\ +{\varsigma }_2{L}_{P}C\left)\right)\varpi \left(k\right)+(I_N\otimes F)\zeta \left(k\right)+(I_N\otimes (({\vartheta }_2-{\varrho }_1)A+({\varsigma }_2-{\sigma }_1)L_{P}C))X_C,\end{array}$$

(47)

and

$$\begin{array}{c}\zeta (k+1)⪯{\varsigma }_2(1-\alpha )(I_N\otimes \left(L_{I}C\right))\varpi \left(k\right)+(1-\alpha )({\varsigma }_2-{\sigma }_1)(I_N\otimes \left(L_{I}C\right))\xi \left(k\right)\\ +\alpha \zeta \left(k\right)+(1-\alpha )({\varsigma }_2-{\sigma }_1)(I_N\otimes \left(L_{I}C\right))X_C.\end{array}$$

(48)

By (46)–(48), it can be inferred that

$$\begin{array}{c}\tilde{\mathcal{X}}(k+1)\hfill \\ ⪯\left(\begin{array}{c}\left(\begin{array}{c}(I_N\otimes ({\varrho }_{2}A+B{K}_S^{+}\Phi +B{K}_S^{-}\Psi )+2\overline{\beta }\overline{\mathcal{D}}\otimes (B{K}_P^{+}{\mathbf{1}}_{n\times n}-B{K}_P^{-}{\mathbf{1}}_{n\times n})+\mathcal{L}\otimes (B{K}_P^{+}\Psi +B{K}_P^{-}\Phi ))\xi \left(k\right)\\ +(I_N\otimes (B{K}_S^{+}\Phi +B{K}_S^{-}\Psi )+2\overline{\beta \mathcal{D}}\otimes (B{K}_P^{+}{\mathbf{1}}_{n\times n}-B{K}_P^{-}{\mathbf{1}}_{n\times n})+\mathcal{L}\otimes (B{K}_P^{+}\Psi +B{K}_P^{-}\Phi ))\varpi \left(k\right)\\ +(I_N\otimes ({\varrho }_{2}A+B{K}_S^{+}\Phi +B{K}_S^{-}\Psi )+2\overline{\beta \mathcal{D}}\otimes (B{K}_P^{+}{\mathbf{1}}_{n\times n}-B{K}_P^{-}{\mathbf{1}}_{n\times n})+\mathcal{L}\otimes (B{K}_P^{+}\Psi +B{K}_P^{-}\Phi )\\ +({\mathbf{1}}_N{\theta }^{\top }-I_N)\otimes \left(B{K}_C\right)-I_{Nn})X_C\end{array}\right)\\ \left(\begin{array}{c}(I_N\otimes (({\vartheta }_2-{\varrho }_1)A+({\varsigma }_2-{\sigma }_1)L_{P}C))\xi \left(k\right)+(I_N\otimes ({\vartheta }_{2}A+{\varsigma }_2{L}_{P}C))\varpi \left(k\right)\\ +(I_N\otimes F)\zeta \left(k\right)+(I_N\otimes (({\vartheta }_2-{\varrho }_1)A+({\varsigma }_2-{\sigma }_1)L_{P}C))X_C\end{array}\right)\\ \left(\begin{array}{c}{\varsigma }_2(1-\alpha )(I_N\otimes \left(L_{I}C\right))\varpi \left(k\right)+(1-\alpha )({\varsigma }_2-{\sigma }_1)(I_N\otimes \left(L_{I}C\right))\xi \left(k\right)\\ +\alpha \zeta \left(k\right)+(1-\alpha )({\varsigma }_2-{\sigma }_1)(I_N\otimes \left(L_{I}C\right))X_C\end{array}\right)\end{array}\right)\hfill \\ =\left(\begin{array}{ccc}\left(\begin{array}{c}{I}_N\otimes ({\varrho }_{2}A+B{K}_S^{+}\Phi +B{K}_S^{-}\Psi )\\ +2\overline{\beta \mathcal{D}}\otimes (B{K}_P^{+}{\mathbf{1}}_{n\times n}-B{K}_P^{-}{\mathbf{1}}_{n\times n})\\ +\mathcal{L}\otimes (B{K}_P^{+}\Psi +B{K}_P^{-}\Phi ))\end{array}\right)& \left(\begin{array}{c}{I}_N\otimes (B{K}_S^{+}\Phi +B{K}_S^{-}\Psi )\\ +2\overline{\beta \mathcal{D}}\otimes (B{K}_P^{+}{\mathbf{1}}_{n\times n}-B{K}_P^{-}{\mathbf{1}}_{n\times n})\\ +\mathcal{L}\otimes (B{K}_P^{+}\Psi +B{K}_P^{-}\Phi ))\end{array}\right)& \mathbf{0}\\ I_N\otimes (({\vartheta }_2-{\varrho }_1)A+({\varsigma }_2-{\sigma }_1)L_{P}C)& I_N\otimes ({\vartheta }_{2}A+{\varsigma }_2{L}_{P}C)& I_N\otimes F\\ (1-\alpha )({\varsigma }_2-{\sigma }_1)(I_N\otimes \left(L_{I}C\right))& {\varsigma }_2(1-\alpha )(I_N\otimes \left(L_{I}C\right))& \alpha I_{Nn}\end{array}\right)\left(\begin{array}{c}\xi \left(k\right)\\ \varpi \left(k\right)\\ \zeta \left(k\right)\end{array}\right)\hfill \\ +\left(\begin{array}{ccc}\left(\begin{array}{c}{I}_N\otimes ({\varrho }_{2}A+B{K}_S^{+}\Phi +B{K}_S^{-}\Psi )\\ +2\overline{\beta \mathcal{D}}\otimes (B{K}_P^{+}{\mathbf{1}}_{n\times n}-B{K}_P^{-}{\mathbf{1}}_{n\times n})\\ +\mathcal{L}\otimes (B{K}_P^{+}\Psi +B{K}_P^{-}\Phi )\\ +({\mathbf{1}}_N{\theta }^{\top }-I_N)\otimes \left(B{K}_C\right)-I_{Nn}\end{array}\right)& \mathbf{0}& \mathbf{0}\\ \mathbf{0}& \left(\begin{array}{c}{I}_N\otimes (({\vartheta }_2-{\varrho }_1)A\\ +({\varsigma }_2-{\sigma }_1)L_{P}C)\end{array}\right)& \mathbf{0}\\ \mathbf{0}& \mathbf{0}& (1-\alpha )({\varsigma }_2-{\sigma }_1)(I_N\otimes \left(L_{I}C\right))\end{array}\right)\left(\begin{array}{c}{X}_C\\ X_C\\ X_C\end{array}\right)\hfill \\ ={\mathbb{A}}_3\tilde{\mathcal{X}}\left(k\right)+{\mathbb{C}}_3({\mathbf{1}}_3\otimes X_C),\hfill \end{array}$$

where

$$\begin{array}{c}{\mathbb{A}}_3=\left(\begin{array}{ccc}\left(\begin{array}{c}{I}_N\otimes ({\varrho }_{2}A+B{K}_S^{+}\Phi +B{K}_S^{-}\Psi )\\ +2\overline{\beta \mathcal{D}}\otimes (B{K}_P^{+}{\mathbf{1}}_{n\times n}-B{K}_P^{-}{\mathbf{1}}_{n\times n})\\ +\mathcal{L}\otimes (B{K}_P^{+}\Psi +B{K}_P^{-}\Phi ))\end{array}\right)& \left(\begin{array}{c}{I}_N\otimes (B{K}_S^{+}\Phi +B{K}_S^{-}\Psi )\\ +2\overline{\beta \mathcal{D}}\otimes (B{K}_P^{+}{\mathbf{1}}_{n\times n}-B{K}_P^{-}{\mathbf{1}}_{n\times n})\\ +\mathcal{L}\otimes (B{K}_P^{+}\Psi +B{K}_P^{-}\Phi ))\end{array}\right)& \mathbf{0}\\ I_N\otimes (({\vartheta }_2-{\varrho }_1)A+({\varsigma }_2-{\sigma }_1)L_{P}C)& I_N\otimes ({\vartheta }_{2}A+{\varsigma }_2{L}_{P}C)& I_N\otimes F\\ (1-\alpha )({\varsigma }_2-{\sigma }_1)(I_N\otimes \left(L_{I}C\right))& {\varsigma }_2(1-\alpha )(I_N\otimes \left(L_{I}C\right))& \alpha I_{Nn}\end{array}\right)\hfill \end{array},$$

and

$$\begin{array}{c}{\mathbb{C}}_3=\left(\begin{array}{ccc}\left(\begin{array}{c}{I}_N\otimes ({\varrho }_{2}A+B{K}_S^{+}\Phi +B{K}_S^{-}\Psi )\\ +2\overline{\beta \mathcal{D}}\otimes (B{K}_P^{+}{\mathbf{1}}_{n\times n}-B{K}_P^{-}{\mathbf{1}}_{n\times n})\\ +\mathcal{L}\otimes (B{K}_P^{+}\Psi +B{K}_P^{-}\Phi )\\ +({\mathbf{1}}_N{\theta }^{\top }-I_N)\otimes \left(B{K}_C\right)-I_{Nn}\end{array}\right)& \mathbf{0}& \mathbf{0}\\ \mathbf{0}& \left(\begin{array}{c}{I}_N\otimes (({\vartheta }_2-{\varrho }_1)A\\ +({\varsigma }_2-{\sigma }_1)L_{P}C)\end{array}\right)& \mathbf{0}\\ \mathbf{0}& \mathbf{0}& (1-\alpha )({\varsigma }_2-{\sigma }_1)(I_N\otimes \left(L_{I}C\right))\end{array}\right)\hfill \end{array}.$$

Define $\nu ={({\tilde{\nu }}_1^{\top },{\tilde{\nu }}_2^{\top },{\tilde{\nu }}_3^{\top })}^{\top }$, where ${\tilde{\nu }}_1={\mathbf{1}}_N\otimes {\nu }_1,{\tilde{\nu }}_2={\mathbf{1}}_N\otimes {\nu }_2$, and ${\tilde{\nu }}_3={\mathbf{1}}_N\otimes {\nu }_3$. Choose a Lyapunov function candidate $V\left(k\right)={\tilde{\mathcal{X}}}^{\top }\left(k\right)\nu ={\xi }^{\top }\left(k\right){\tilde{\nu }}_1+{\varpi }^{\top }\left(k\right){\tilde{\nu }}_2+{\zeta }^{\top }\left(k\right){\tilde{\nu }}_3$. Then, it can be deduced that

$$\begin{array}{cc}\Delta V\left(k\right)\hfill & =V(k+1)-V\left(k\right)={\tilde{\mathcal{X}}}^{\top }(k+1)\nu -{\tilde{\mathcal{X}}}^{\top }\left(k\right)\nu \hfill \\ \hfill & ={\tilde{\mathcal{X}}}^{\top }\left(k\right){\mathbb{A}}_3^{\top }\nu +{({\mathbf{1}}_3\otimes X_C)}^{\top }{\mathbb{C}}_3^{\top }\nu -{\tilde{\mathcal{X}}}^{\top }\left(k\right)\nu \hfill \\ \hfill & ={\tilde{\mathcal{X}}}^{\top }\left(k\right)({\mathbb{A}}_3^{\top }-I_{3N})\nu +{({\mathbf{1}}_3\otimes X_C)}^{\top }{\mathbb{C}}_3^{\top }\nu .\hfill \end{array}$$

(49)

This results in

$$\begin{array}{c}{\tilde{\mathcal{X}}}^{\top }\left(k\right)({\mathbb{A}}_3^{\top }-I)\nu ={\xi }^{\top }\left(k\right)\left(\right(I_N\otimes ({\varrho }_2{A}^{\top }+\Phi K_S^{+\top }{B}^{\top }+\Psi K_S^{-\top }{B}^{\top })+2\overline{\beta \mathcal{D}}\otimes ({\mathbf{1}}_{n\times n}{K}_P^{+\top }{B}^{\top }-{\mathbf{1}}_{n\times n}{K}_P^{-\top }{B}^{\top })\hfill \\ +\mathcal{L}\otimes (\Psi K_P^{+\top }{B}^{\top }+\Phi K_P^{-\top }{B}^{\top }))-I_{Nn}){\tilde{\nu }}_1+{\varpi }^{\top }\left(k\right)(I_N\otimes (\Phi K_S^{+\top }{B}^{\top }+\Psi K_S^{-\top }{B}^{\top }+2\overline{\beta \mathcal{D}}\otimes ({\mathbf{1}}_{n\times n}{K}_P^{+\top }{B}^{\top }\hfill \\ -{\mathbf{1}}_{n\times n}{K}_P^{-\top }{B}^{\top })+\mathcal{L}\otimes (\Psi K_P^{+\top }{B}^{\top }+\Phi K_P^{-\top }{B}^{\top })\left)\right){\tilde{\nu }}_1+{\xi }^{\top }\left(k\right)(I_N\otimes (({\vartheta }_2-{\varrho }_1)A^{\top }+({\varsigma }_2-{\sigma }_1)C^{\top }{L}_P^{\top })){\tilde{\nu }}_2\hfill \\ +{\varpi }^{\top }\left(k\right)(I_N\otimes ({\vartheta }_2{A}^{\top }+{\varsigma }_2{C}^{\top }{L}_P^{\top })-I_{Nn}){\tilde{\nu }}_2+{\zeta }^{\top }\left(k\right)(I_N\otimes F^{\top }){\tilde{\nu }}_2+{\xi }^{\top }\left(k\right)\left((1-\alpha )({\varsigma }_2-{\sigma }_1)(I_N\otimes \left(C^{\top }{L}_I^{\top }\right))\right){\tilde{\nu }}_3\hfill \\ +{\varpi }^{\top }\left(k\right)\left({\varsigma }_2(1-\alpha )(I_N\otimes \left(C^{\top }{L}_I^{\top }\right))\right){\tilde{\nu }}_3+(\alpha -1){\zeta }^{\top }\left(k\right){\tilde{\nu }}_3,\hfill \end{array}$$

(50)

and

$$\begin{array}{c}{({\mathbf{1}}_3\otimes X_C)}^{\top }{\mathbb{C}}_3^{\top }\nu =X_C^{\top }(I_N\otimes ({\varrho }_2{A}^{\top }+\Phi K_S^{+\top }{B}^{\top }+\Psi K_S^{-\top }{B}^{\top })+2\overline{\beta \mathcal{D}}\otimes ({\mathbf{1}}_{n\times n}{K}_P^{+\top }{B}^{\top }-{\mathbf{1}}_{n\times n}{K}_P^{-\top }{B}^{\top })\hfill \\ +\mathcal{L}\otimes (\Psi K_P^{+\top }{B}^{\top }+\Phi K_P^{-\top }{B}^{\top })+(\theta {\mathbf{1}}_N^{\top }-I_N)\otimes \left(K_C^{\top }{B}^{\top }\right)-I_{Nn}){\tilde{\nu }}_1+X_C^{\top }(I_N\otimes (({\vartheta }_2-{\varrho }_1)A^{\top }\hfill \\ +({\varsigma }_2-{\sigma }_1)C^{\top }{L}_P^{\top }\left)\right){\tilde{\nu }}_2+X_C^{\top }\left((1-\alpha )({\varsigma }_2-{\sigma }_1)(I_N\otimes \left(C^{\top }{L}_I^{\top }\right))\right){\tilde{\nu }}_3.\hfill \end{array}$$

(51)

From (26) and (25j), it can be obtained that

$$\begin{array}{c}{\underline{\delta }}_{ks}^{+}⪯K_S^{+\top }{B}^{\top }{\nu }_1=\frac{{\left({\sum }_{j=1}^m{\mathbf{1}}_m^{\left(j\right)}{\delta }_{ks}^{+\left(\iota \right)\top }\right)}^{\top }}{{\mathbf{1}}_m^{\top }{B}^{\top }{\nu }_1}{B}^{\top }{\nu }_1⪯{\overline{\delta }}_{ks}^{+},\\ {\underline{\delta }}_{ks}^{-}⪯K_S^{-\top }{B}^{\top }{\nu }_1=\frac{{\left({\sum }_{j=1}^m{\mathbf{1}}_m^{\left(j\right)}{\delta }_{ks}^{-\left(\iota \right)\top }\right)}^{\top }}{{\mathbf{1}}_m^{\top }{B}^{\top }{\nu }_1}{B}^{\top }{\nu }_1⪯{\overline{\delta }}_{ks}^{-},\\ {\underline{\delta }}_{kp}^{+}⪯K_P^{+\top }{B}^{\top }{\nu }_1=\frac{{\left({\sum }_{j=1}^m{\mathbf{1}}_m^{\left(j\right)}{\delta }_{kp}^{+\left(\iota \right)\top }\right)}^{\top }}{{\mathbf{1}}_m^{\top }{B}^{\top }{\nu }_1}{B}^{\top }{\nu }_1⪯{\overline{\delta }}_{kp}^{+},\\ {\underline{\delta }}_{kp}^{-}⪯K_P^{-\top }{B}^{\top }{\nu }_1=\frac{{\left({\sum }_{j=1}^m{\mathbf{1}}_m^{\left(j\right)}{\delta }_{kp}^{-\left(\iota \right)\top }\right)}^{\top }}{{\mathbf{1}}_m^{\top }{B}^{\top }{\nu }_1}{B}^{\top }{\nu }_1⪯{\overline{\delta }}_{kp}^{-},\\ {\underline{\delta }}_{kc}⪯K_C^{\top }{B}^{\top }{\nu }_1=\frac{{\left({\sum }_{j=1}^m{\mathbf{1}}_m^{\left(j\right)}{\delta }_{kc}^{\left(\iota \right)\top }\right)}^{\top }}{{\mathbf{1}}_m^{\top }{B}^{\top }{\nu }_1}{B}^{\top }{\nu }_1⪯{\overline{\delta }}_{kc},\\ {\underline{\delta }}_{li}⪯L_I^{\top }{\nu }_3=\frac{{\left({\sum }_{\iota =1}^n{\mathbf{1}}_n^{\left(\iota \right)}{\delta }_{li}^{\left(\iota \right)\top }\right)}^{\top }}{{\mathbf{1}}_n^{\top }{\nu }_3}{\nu }_3⪯{\overline{\delta }}_{li},\\ {\underline{\delta }}_{lp}⪯L_P^{\top }{\nu }_2=\frac{{\left({\sum }_{\iota =1}^n{\mathbf{1}}_n^{\left(\iota \right)}{\delta }_{lp}^{\left(\iota \right)\top }\right)}^{\top }}{{\mathbf{1}}_n^{\top }{\nu }_2}{\nu }_2⪯{\overline{\delta }}_{lp},\\ {\underline{\delta }}_f⪯F^{\top }{\nu }_2=\frac{{\left({\sum }_{\iota =1}^n{\mathbf{1}}_n^{\left(\iota \right)}{\delta }_f^{\left(\iota \right)\top }\right)}^{\top }}{{\mathbf{1}}_n^{\top }{\nu }_2}{\nu }_2⪯{\overline{\delta }}_f.\end{array}$$

(52)

Combined with (50)–(52), one can obtain that

$$\begin{array}{c}{\tilde{\mathcal{X}}}^{\top }\left(k\right)({\mathbb{A}}_3^{\top }-I)\nu ⪯{\xi }^{\top }\left(k\right)({\mathbf{1}}_N\otimes ({\varrho }_2{A}^{\top }{\nu }_1+\Phi {\overline{\delta }}_{ks}^{+}+{\overline{\delta }}_{ks}^{-}-{\mathbf{1}}_{n\times n}{\underline{\delta }}_{ks}^{-})+2\overline{\beta }{d}_{max}{\mathbf{1}}_N\otimes ({\mathbf{1}}_{n\times n}{\overline{\delta }}_{kp}^{+}-{\mathbf{1}}_{n\times n}{\underline{\delta }}_{kp}^{-})-{\nu }_1))\hfill \\ +{\varpi }^{\top }\left(k\right)({\mathbf{1}}_N\otimes (\Phi {\overline{\delta }}_{ks}^{+}+{\overline{\delta }}_{ks}^{-}-{\mathbf{1}}_{n\times n}{\underline{\delta }}_{ks}^{-})+2\overline{\beta }{d}_{max}{\mathbf{1}}_N\otimes ({\mathbf{1}}_{n\times n}{\overline{\delta }}_{kp}^{+}-{\mathbf{1}}_{n\times n}{\underline{\delta }}_{kp}^{-})))+(\alpha -1){\zeta }^{\top }\left(k\right)({\mathbf{1}}_N\otimes {\nu }_3)\hfill \\ +{\xi }^{\top }\left(k\right)({\mathbf{1}}_N\otimes (({\vartheta }_2-{\varrho }_1)A^{\top }{\nu }_2+({\varsigma }_2-{\sigma }_1)C^{\top }{\overline{\delta }}_{lp}))+{\varpi }^{\top }\left(k\right)({\mathbf{1}}_N\otimes ({\vartheta }_2{A}^{\top }{\nu }_2+{\varsigma }_2{C}^{\top }{\overline{\delta }}_{lp}-{\nu }_2))\hfill \\ +{\zeta }^{\top }\left(k\right)({\mathbf{1}}_N\otimes {\overline{\delta }}_f)+{\xi }^{\top }\left(k\right)(1-\alpha )({\varsigma }_2-{\sigma }_1)({\mathbf{1}}_N\otimes \left(C^{\top }{\overline{\delta }}_{li}\right))+{\varpi }^{\top }\left(k\right)\left({\varsigma }_2(1-\alpha )({\mathbf{1}}_N\otimes \left(C^{\top }{\overline{\delta }}_{li}\right))\right),\hfill \end{array}$$

(53)

and

$$\begin{array}{c}{({\mathbf{1}}_3\otimes X_C)}^{\top }{\mathbb{C}}_3^{\top }\nu ⪯X_C^{\top }({\mathbf{1}}_N\otimes ({\varrho }_2{A}^{\top }{\nu }_1+\Phi {\overline{\delta }}_{ks}^{+}+{\overline{\delta }}_{ks}^{-}-{\mathbf{1}}_{n\times n}{\underline{\delta }}_{ks}^{-})+2\overline{\beta }{d}_{max}{\mathbf{1}}_N\otimes ({\mathbf{1}}_{n\times n}{\overline{\delta }}_{kp}^{+}-{\mathbf{1}}_{n\times n}{\underline{\delta }}_{ks}^{-})\hfill \\ +(N\theta -{\mathbf{1}}_N)\otimes {\overline{\delta }}_{kc}-{\mathbf{1}}_N\otimes {\nu }_1+{\mathbf{1}}_N\otimes (({\vartheta }_2-{\varrho }_1)A^{\top }{\nu }_2+({\varsigma }_2-{\sigma }_1)C^{\top }{\overline{\delta }}_{lp})+(1-\alpha )({\varsigma }_2-{\sigma }_1)({\mathbf{1}}_N\otimes \left(C^{\top }{\overline{\delta }}_{li}\right))).\hfill \end{array}$$

(54)

Using (49), (53), and (54) results in

$$\begin{array}{c}\Delta V\left(k\right)\le {\xi }^{\top }\left(k\right)({\mathbf{1}}_N\otimes (({\varrho }_2{A}^{\top }-I_n){\nu }_1+\Phi {\overline{\delta }}_{ks}^{+}+{\overline{\delta }}_{ks}^{-}-{\mathbf{1}}_{n\times n}{\underline{\delta }}_{ks}^{-})+2\overline{\beta }{d}_{max}{\mathbf{1}}_N\otimes ({\mathbf{1}}_{n\times n}{\overline{\delta }}_{kp}^{+}-{\mathbf{1}}_{n\times n}{\underline{\delta }}_{kp}^{-}))\hfill \\ +{\mathbf{1}}_N\otimes (({\vartheta }_2-{\varrho }_1)A^{\top }{\nu }_2+({\varsigma }_2-{\sigma }_1)C^{\top }{\overline{\delta }}_{lp})+(1-\alpha )({\varsigma }_2-{\sigma }_1)({\mathbf{1}}_N\otimes \left(C^{\top }{\overline{\delta }}_{li}\right)))+{\varpi }^{\top }\left(k\right)({\mathbf{1}}_N\otimes (\Phi {\overline{\delta }}_{ks}^{+}\hfill \\ +{\overline{\delta }}_{ks}^{-}-{\mathbf{1}}_{n\times n}{\underline{\delta }}_{ks}^{-})+2\overline{\beta }{d}_{max}{\mathbf{1}}_N\otimes ({\mathbf{1}}_{n\times n}{\overline{\delta }}_{kp}^{+}-{\mathbf{1}}_{n\times n}{\underline{\delta }}_{kp}^{-})+{\mathbf{1}}_N\otimes ({\vartheta }_2{A}^{\top }{\nu }_2+{\varsigma }_2{C}^{\top }{\overline{\delta }}_{lp}-{\nu }_2)\hfill \\ +{\varsigma }_2(1-\alpha )({\mathbf{1}}_N\otimes \left(C^{\top }{\overline{\delta }}_{li}\right)))+{\zeta }^{\top }\left(k\right)({\mathbf{1}}_N\otimes {\overline{\delta }}_f+(\alpha -1)({\mathbf{1}}_N\otimes {\nu }_3))\hfill \\ +X_C^{\top }({\mathbf{1}}_N\otimes ({\varrho }_2{A}^{\top }{\nu }_1+\Phi {\overline{\delta }}_{ks}^{+}+{\overline{\delta }}_{ks}^{-}-{\mathbf{1}}_{n\times n}{\underline{\delta }}_{ks}^{-})+2\overline{\beta }{d}_{max}{\mathbf{1}}_N\otimes ({\mathbf{1}}_{n\times n}{\overline{\delta }}_{kp}^{+}-{\mathbf{1}}_{n\times n}{\underline{\delta }}_{ks}^{-})+(N\theta -{\mathbf{1}}_N)\otimes {\overline{\delta }}_{kc}\hfill \\ -{\mathbf{1}}_N\otimes {\nu }_1+{\mathbf{1}}_N\otimes (({\vartheta }_2-{\varrho }_1)A^{\top }{\nu }_2+({\varsigma }_2-{\sigma }_1)C^{\top }{\overline{\delta }}_{lp})+(1-\alpha )({\varsigma }_2-{\sigma }_1)({\mathbf{1}}_N\otimes \left(C^{\top }{\overline{\delta }}_{li}\right))).\hfill \end{array}$$

(55)

Using (25f)–(25i), it can be concluded that

$$\begin{array}{c}\Delta V\left(k\right)<-(1-\mu ){\tilde{\mathcal{X}}}^{\top }\left(k\right)\nu +\rho X_C^{\top }{\nu }_1.\hfill \end{array}$$

(56)

It is easy to derive from (56) that $V(k+1)<\mu V\left(k\right)+\rho X_C^{\top }{\nu }_1<\cdots <{\mu }^{k+1}V\left(0\right)$$k+\frac{1-{\mu }^{k+1}}{1-\mu }\rho X_C^{\top }{\nu }_1$, and this leads to

$$\begin{array}{c}\underset{k\to \infty }{lim}V\left(k\right)=\frac{1}{1-\mu }\rho X_C^{\top }{\nu }_1.\hfill \end{array}$$

(57)

□

To maintain readability, some detailed steps and intermediate calculations of this proof are provided in Appendix A. These additional details include (29), (42), (50), and (51), which provide a more comprehensive understanding of the proof process.

Remark 4.

The designed control strategy (24) is based on the proposed dynamic event-triggered mechanism (21). This makes the controller dynamically adjust its actions based on real-time system states and events, rather than relying on periodic updates. As stated in the Introduction, the introduction of an event-triggered approach eliminates the need for controller operations when the system states change, leading to lower resource consumption and significantly extending the lifespan of agents.

Remark 5.

There are three controller gain matrices $K_s,K_p$, and $K_c$ in the designed controller (24), which play vital roles in fine-tuning the system’s operational dynamics. Specifically, $K_s$ is instrumental in adjusting the state estimation of the agent itself, enabling a precise self-assessment of its operational state. $K_p$, on the other hand, facilitates the interaction of state estimation between agents, thereby enhancing collaborative performance and system-wide coherence. Lastly, $K_c$ is dedicated to adjusting the final convergence point of the system, ensuring that this value is contingent upon a given constant term, rather than converging to a null point.

Remark 6.

By introducing finite-value vectors $x_{ic}$ and variables (32) and (33), the final convergence point depends on the given vector, rather than converging to a zero point, which increases the practicability of the proposed control protocol. By assigning a unique $x_{ic}$ to each agent, the system facilitates the convergence of each agent to distinct values, rather than enforcing uniformity, where all agents converge to the same point. This capability is crucial in scenarios where varied states or outcomes are desirable among different agents.

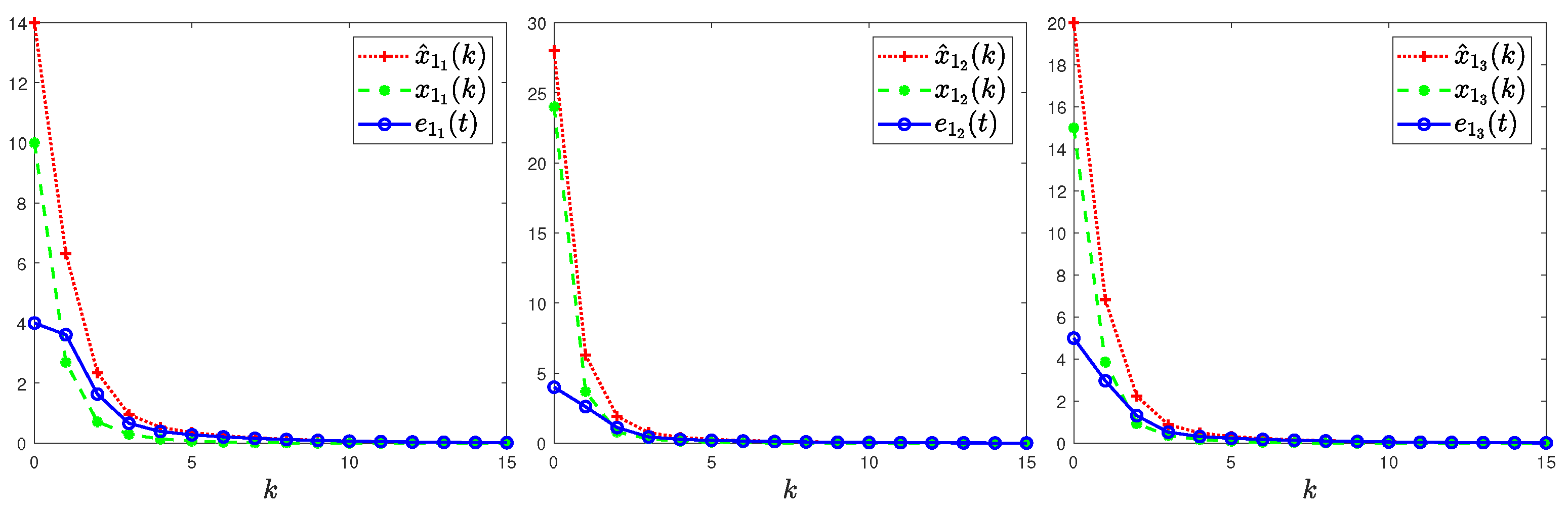
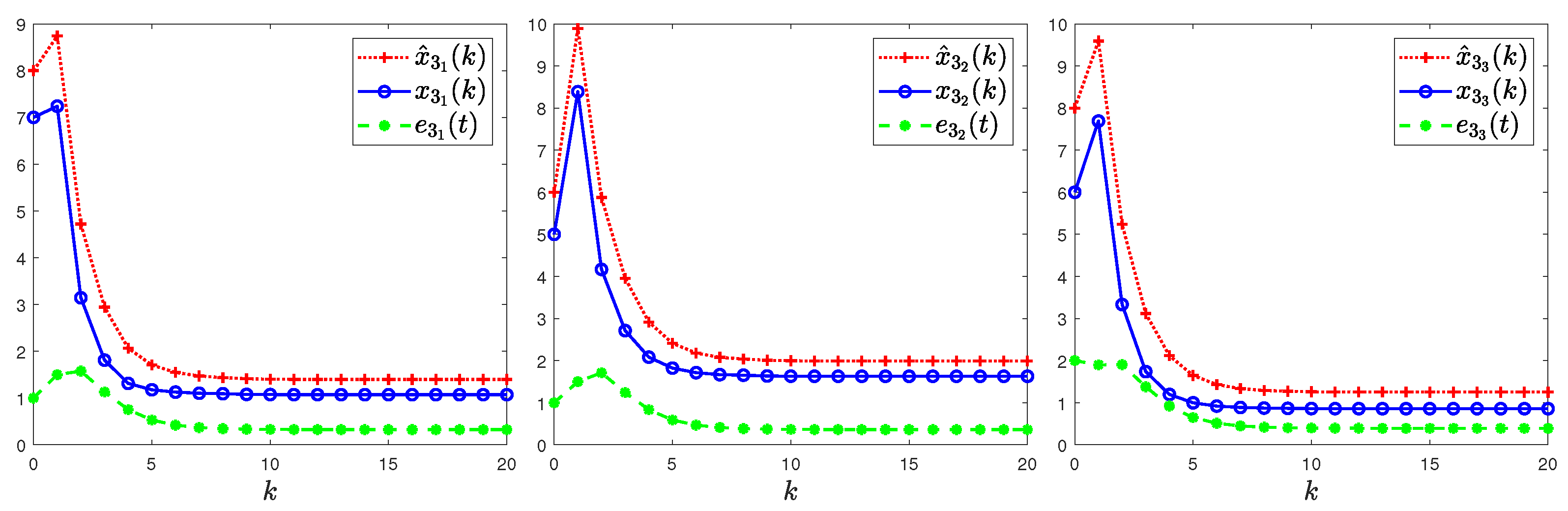
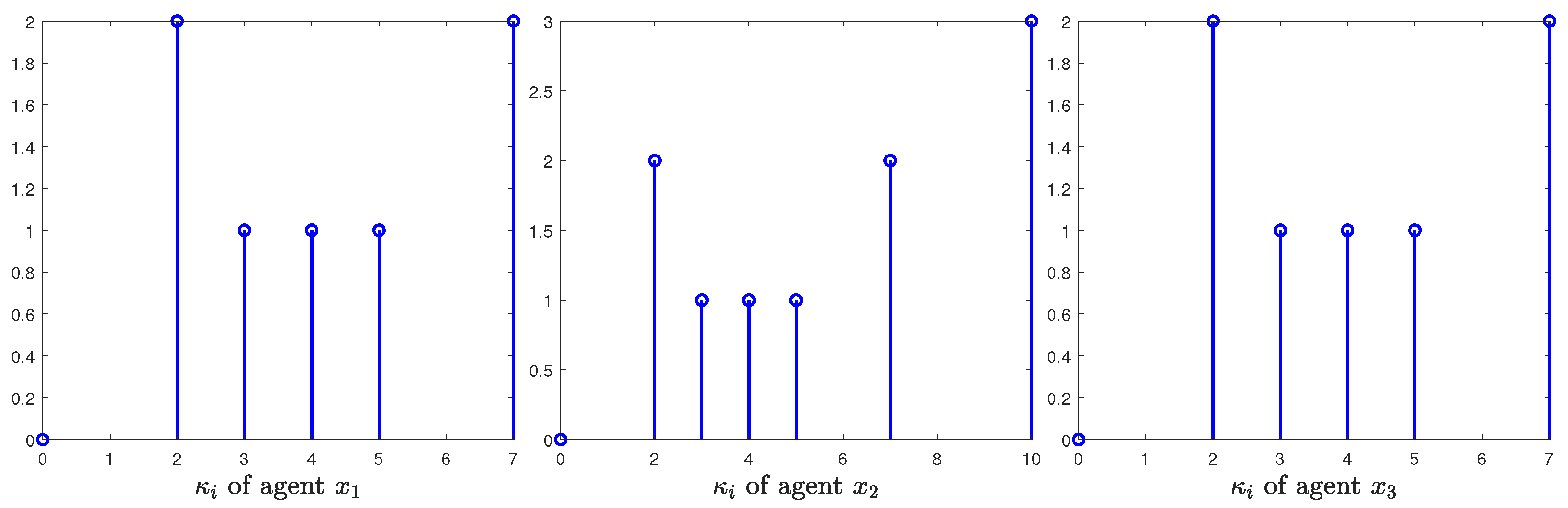
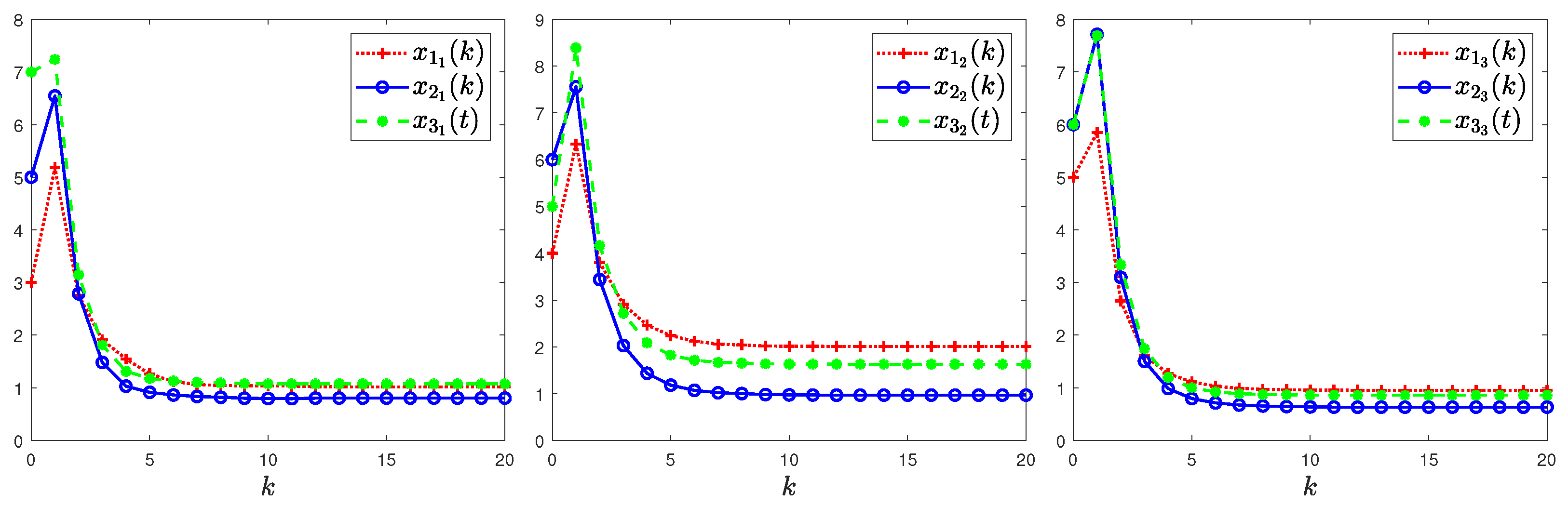
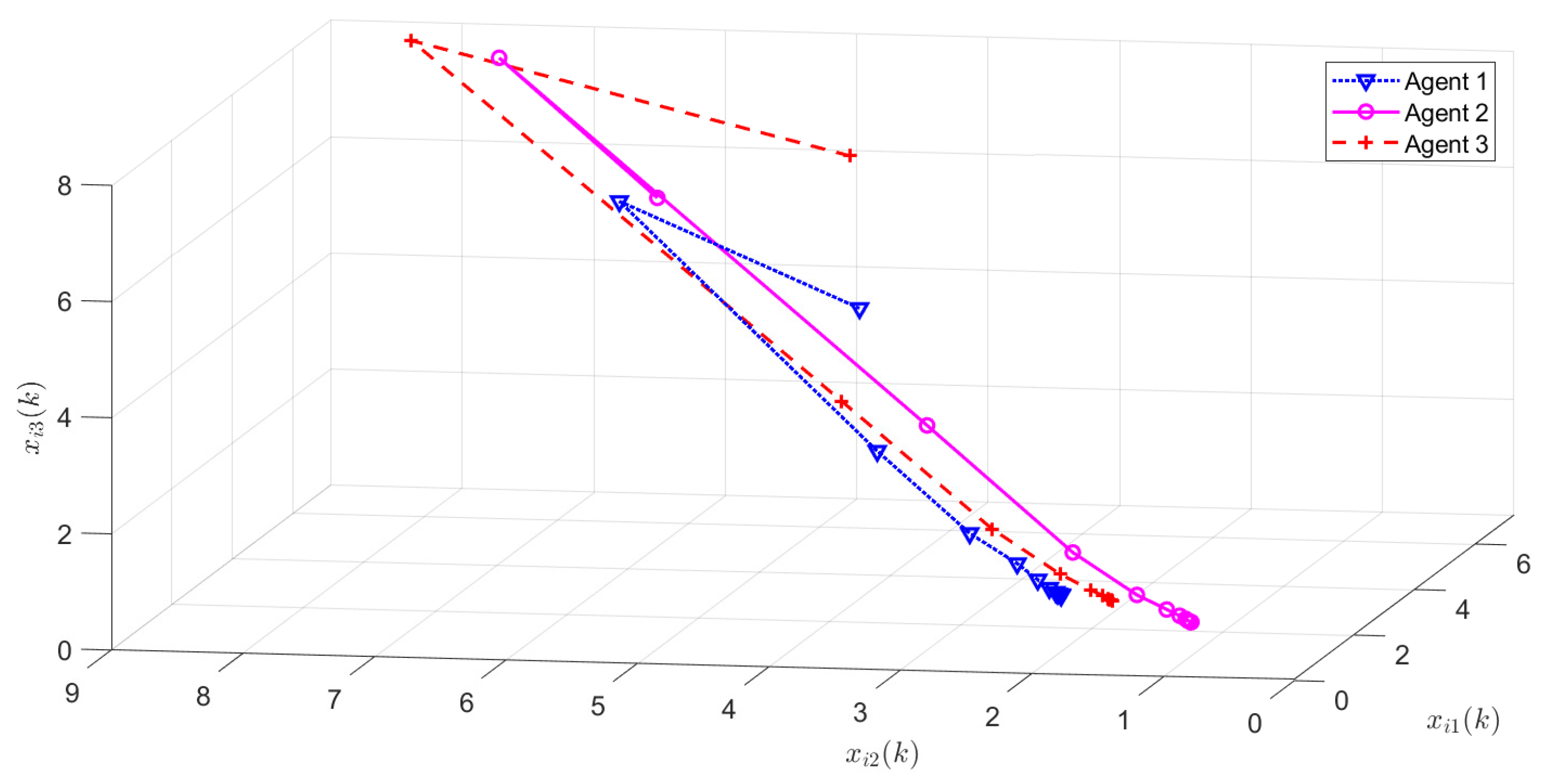
4. Illustrative Examples

Two numerical examples are provided in this section to illustrate the theoretical conclusions.

Example 1.

The systems matrices of the system (1) are given as:

$$\begin{array}{c}A=\left(\begin{array}{ccc}0.5& 0.1& 0.1\\ 0.2& 0.3& 0.2\\ 0.2& 0.2& 0.4\end{array}\right),B=\left(\begin{array}{cc}0.04& 0.04\\ 0.03& 0.05\\ 0.02& 0.06\end{array}\right),C=\left(\begin{array}{ccc}0.04& 0.03& 0.07\\ 0.02& 0.01& 0.05\end{array}\right).\hfill \end{array}$$

According to the original and observer systems, the nonlinear functions are as follows: $f\left(x\right)=0.3x+\frac{x}{5x^2+5},\widehat{f}\left(x\right)=0.4x+\frac{x}{2x^2+2},g\left(x\right)=0.1x+\frac{x}{5x^2+5},\widehat{g}\left(x\right)=0.3x+\frac{3x}{10x^2+10}$. Then, we set ${\varrho }_1=0.30,{\varrho }_2=0.40,{\sigma }_1=0.1,{\sigma }_2=0.2,{\vartheta }_1=0.4,{\vartheta }_2=0.5,{\varsigma }_1=0.3,{\varsigma }_2=0.35,\alpha =0.3$. The initial states and initial estimated states of all agents are as follows: $x_1\left(0\right)={(30.0,40.0,50.0)}^{\top },x_2\left(0\right)={(50.0,61.0,62.0)}^{\top },x_3\left(0\right)={(70.0,54.0,65.0)}^{\top },{\widehat{x}}_1\left(0\right)={(40.0,60.0,80.0)}^{\top }$, ${\widehat{x}}_2\left(0\right)={(85.0,83.0,88.0)}^{\top }$, ${\widehat{x}}_3\left(0\right)={(94.0,98.0,80.0)}^{\top },v_1\left(0\right)=v_2\left(0\right)=v_3\left(0\right)=\mathbf{0}$. By solving the conditions presented in Theorem 1, the vectors that satisfy the conditions and the gain matrices $L_p$, $L_I$, and F of observer (5) can be obtained.

$$\begin{array}{c}{\nu }_1=\left(\begin{array}{c}409.451\\ 182.734\\ 238.676\end{array}\right),{\nu }_2=\left(\begin{array}{c}199.7807\\ 129.5348\\ 149.5892\end{array}\right),{\nu }_2=\left(\begin{array}{c}257.4571\\ 257.4571\\ 257.4571\end{array}\right),{\overline{\delta }}_F=\left(\begin{array}{c}135.7607\\ 135.7607\\ 135.7607\end{array}\right),\hfill \end{array}$$

$$\begin{array}{c}{\overline{\delta }}_{li}=\left(\begin{array}{c}166.2572\\ 172.3710\end{array}\right),{\overline{\delta }}_{lp}=\left(\begin{array}{c}166.2049\\ 172.1265\end{array}\right),L_P=\left(\begin{array}{cc}0.1716& 0.1718\\ 0.1693& 0.1704\\ 0.1679& 0.1691\end{array}\right),\hfill \end{array}$$

$$\begin{array}{c}{L}_I=\left(\begin{array}{cc}0.0993& 0.1023\\ 0.0993& 0.1023\\ 0.0993& 0.1023\end{array}\right),F=\left(\begin{array}{ccc}0.1354& 0.1354& 0.1354\\ 0.1354& 0.1354& 0.1354\\ 0.1354& 0.1354& 0.1354\end{array}\right).\hfill \end{array}$$

The simulations of the system states, observer states, and observer errors are shown in Figure 1, Figure 2 and Figure 3. From the figures, it is easily observed that the designed PI observer (5) offers accurate estimations of the states of the considered systems (1). The rapid convergence of observer errors towards zero highlights the efficiency of the PI observer in correcting discrepancies between actual and estimated states.

Figure 1. The simulations of $x,\widehat{x},$ and e of the 1st agent.

Figure 2. The simulations of $x\left(k\right),\widehat{x}\left(k\right),$ and $e\left(k\right)$ of the 2nd agent.

Figure 3. The simulations of $x\left(k\right),\widehat{x}\left(k\right),$ and $e\left(k\right)$ of the 3rd agent.

Example 2.

Suppose that there are three agents in the system and the communication typology is modeled by an undirected graph. The adjacency matrix degree matrix and corresponding Laplacian matrix are

$$\begin{array}{c}\mathcal{A}=\left(\begin{array}{ccc}2& 1& 1\\ 1& 2& 1\\ 1& 1& 2\end{array}\right),\mathcal{D}=\left(\begin{array}{ccc}2& 0& 0\\ 0& 2& 0\\ 0& 0& 2\end{array}\right),\mathcal{L}=\left(\begin{array}{ccc}2& -1& -1\\ -1& 2& -1\\ -1& -1& 2\end{array}\right).\hfill \end{array}$$

It can be demonstrated that $N=3,d_{max}=l_{max}=2,d_{min}=1$. The system matrices of the PMASs are given as:

$$\begin{array}{c}A=\left(\begin{array}{ccc}0.4& 0.3& 0.4\\ 0.4& 0.5& 0.3\\ 0.2& 0.5& 0.6\end{array}\right),B=\left(\begin{array}{cc}0.2& 0.04\\ 0.03& 0.5\\ 0.02& 0.06\end{array}\right),C=\left(\begin{array}{ccc}0.8& 0.2& 0.6\\ 0.1& 0.3& 1\end{array}\right).\hfill \end{array}$$

The nonlinear functions and the corresponding sector conditions’ parameters of the original system and observer system are chosen same as in Example 1. Choose $\alpha =0.2,\Lambda =0.1,\beta \left(0\right)=0.2,\overline{\beta }=0.3,\mu =2.6,\rho =481,\underline{\theta }=1.01,\overline{\theta }=1.2,\epsilon =0.5$. The initial values required are given as $x_1\left(0\right)={(3.0,4.0,5.0)}^{\top },x_2\left(0\right)={(5.0,6.0,6.0)}^{\top },x_3\left(0\right)={(7.0,5.0,6.0)}^{\top },{\widehat{x}}_1\left(0\right)={(4,6,8)}^{\top },{\widehat{x}}_2\left(0\right)={(7,8,7)}^{\top },{\widehat{x}}_i\left(0\right)={(8,6,8)}^{\top }$, $x_{1c}={(0.002,0.003,0.004)}^{\top }$, $x_{2c}={(0.001,0.002,0.001)}^{\top }$, $x_{1c}={(0.001,0.001,0.003)}^{\top },v_1\left(0\right)=v_2\left(0\right)=v_3\left(0\right)=\mathbf{0}.$ Based on the feasible solutions of the conditions in Theorem 2, the vectors that satisfy the conditions and the gain matrices $L_p$, $L_I$, and F of observer (5) can be obtained.

$$\begin{array}{c}{\nu }_1=\left(\begin{array}{c}6.6079\\ 8.2832\\ 45.0657\end{array}\right),{\nu }_2=\left(\begin{array}{c}5.9825\\ 5.4041\\ 6.9702\end{array}\right),{\nu }_3=\left(\begin{array}{c}234.6972\\ 234.6972\\ 234.6972\end{array}\right),{\underline{\delta }}_F=\left(\begin{array}{c}106.9258\\ 106.9258\\ 106.9258\end{array}\right),\end{array}$$

$$\begin{array}{c}{\underline{\delta }}_{ks}^{+}=\left(\begin{array}{c}0.1915\\ 0.1878\\ 0.1781\end{array}\right),{\overline{\delta }}_{ks}^{-}=\left(\begin{array}{c}-0.0027\\ -0.0029\\ -0.0029\end{array}\right),{\underline{\delta }}_{kp}^{+}=\left(\begin{array}{c}0.000061\\ 0.000057\\ 0.000056\end{array}\right),{\overline{\delta }}_{kp}^{-}=\left(\begin{array}{c}-0.0033\\ -0.0035\\ -0.0036\end{array}\right),\end{array}$$

$$\begin{array}{c}{L}_P=\left(\begin{array}{cc}0.3175& 0.2250\\ 0.3175& 0.2251\\ 0.3188& 0.2233\end{array}\right),L_I=\left(\begin{array}{cc}0.0034& 0.0034\\ 0.0034& 0.0034\\ 0.0034& 0.0034\end{array}\right),\end{array}$$

$$\begin{array}{c}{K}_s^{+}=\left(\begin{array}{ccc}0.0584& 0.0569& 0.0573\\ 0.0561& 0.0563& 0.0563\end{array}\right),K_s^{-}=\left(\begin{array}{ccc}-0.0008& -0.0009& -0.0008\\ -0.0009& -0.0010& -0.0010\end{array}\right),\\ K_p^{+}=\left(\begin{array}{ccc}0.000022& 0.000024& 0.000023\\ 0.000010& 0.000013& 0.000013\end{array}\right),K_n^{-}=\left(\begin{array}{ccc}-0.0022& -0.3667& -0.0893\\ -0.0009& -0.0008& -0.0008\end{array}\right),\end{array}$$

$$\begin{array}{c}{K}_C=\left(\begin{array}{ccc}49.2410& 69.7231& 514.7106\\ 46.7387& 55.3958& 516.3974\end{array}\right),F=\left(\begin{array}{ccc}16.4427& 16.4427& 16.4427\\ 16.4427& 16.4427& 16.4427\\ 15.8696& 15.8696& 15.8696\end{array}\right).\end{array}$$

Based on the given initial values and parameters, as well as the computed gain matrices, the simulation results of the nonlinear positive multi-agent system (1) with a PI observer-based control mechanism (24) are shown in Figure 4, Figure 5, Figure 6, Figure 7, Figure 8 and Figure 9. It can be seen that the designed observer (5) provides appropriate estimations of the considered system (1). Moreover, Figure 4, Figure 5 and Figure 6 also illustrate that, under the designed observer-based dynamic event-triggered control mechanism (24), by introducing the constant vectors $x_{ic}$, the system states and observer states ultimately converge to a fixed value rather than zero. Such a design is both rational and essential in applications where it is necessary for the system to stabilize at specific operational points rather than at a zero state. Figure 7 illustrates the dynamic triggering times and intervals for each agent, highlighting that the intervals at which control actions are taken vary among agents and do not follow a fixed periodic pattern. This leads to a more responsive and resource-efficient system. The state components and trajectories of the three agents are depicted in Figure 8 and Figure 9. It can be seen that each agent converges to its own distinct value. This outcome illustrates the system’s ability to achieve consensus, enhancing robustness and adaptability in real-world scenarios. By converging to distinct values, the agents demonstrate a form of consensus that allows the system to remain stable and effective under diverse conditions.

Figure 4. The simulations of $x\left(k\right),\widehat{x}\left(k\right),$ and $e\left(k\right)$ of the 1st agent.

Figure 5. The simulations of $x\left(k\right),\widehat{x}\left(k\right),$ and $e\left(k\right)$ of the 2nd agent.

Figure 6. The simulations of $x\left(k\right),\widehat{x}\left(k\right),$ and $e\left(k\right)$ of the 3rd agent.

Figure 7. The trigger signal of each agent.

Figure 8. The the state components of the three agents.

Figure 9. The state trajectories of the three agents.

5. Conclusions and Future Work

In this paper, we introduced a positive proportional–integral observer (PIO)-based dynamic event-triggered protocol to address the consensus problem in nonlinear positive multi-agent systems (PMASs). Our contributions include the following three parts: the development of a positive PIO for improved state estimation under positivity constraints; the integration of this PIO with a dynamic event-triggered mechanism to reduce communication overhead while ensuring consensus; the introduction of variables that enhance the flexibility of the convergence point. In the future, it will be interesting to improve the PIO for different kinds of PMASs with various conditions. Comparing the performance of the designed PIO with other forms of observers is meaningful. Moreover, some parameters need to be specified in the proposed results; it is vital to determine them in a more flexible way.

Appendix A.1. Additional Details for Step (29)

$$\begin{array}{c}{x}_i(k_0+1)=Af\left(x_i\left(k_0\right)\right)+B{K}_S{\widehat{x}}_i\left({\kappa }_{\iota }\right)+B{K}_P\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}({\widehat{x}}_i\left({\kappa }_{\iota }\right)-{\widehat{x}}_j\left({\kappa }_{\iota }\right))+B{K}_C{x}_{ic}\hfill \\ =Af\left(x_i\left(k_0\right)\right)+B{K}_S({ϵ}_i\left(k_0\right)+{\widehat{x}}_i\left(k_0\right))+B{K}_P\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}({ϵ}_i\left(k_0\right)+{\widehat{x}}_i\left(k_0\right)\hfill \\ -({ϵ}_j\left(k_0\right)+{\widehat{x}}_j\left(k_0\right)))+B{K}_C{x}_{ic}\hfill \\ =Af\left(x_i\left(k_0\right)\right)+B{K}_S{\widehat{x}}_i\left(k_0\right)+B{K}_S{ϵ}_i\left(k_0\right)+B{K}_P\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}({\widehat{x}}_i\left(k_0\right)-{\widehat{x}}_j\left(k_0\right))\hfill \\ +B{K}_P\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}({ϵ}_i\left(k_0\right)-{ϵ}_j\left(k_0\right))+B{K}_C{x}_{ic}\hfill \\ =Af\left(x_i\left(k_0\right)\right)+B{K}_S{\widehat{x}}_i\left(k_0\right)+B{K}_P\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{\widehat{x}}_i\left(k_0\right)-B{K}_P\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{\widehat{x}}_j\left(k_0\right)\hfill \\ +B{K}_S{ϵ}_i\left(k_0\right)+B{K}_P\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{ϵ}_i\left(k_0\right)-B{K}_P\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{ϵ}_j\left(k_0\right)+B{K}_C{x}_{ic}\hfill \\ =Af\left(x_i\left(k_0\right)\right)+B{K}_S^{+}{\widehat{x}}_i\left(k_0\right)+B{K}_S^{-}{\widehat{x}}_i\left(k_0\right)+B{K}_P^{+}\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{\widehat{x}}_i\left(k_0\right)\hfill \\ +B{K}_P^{-}\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{\widehat{x}}_i\left(k_0\right)-B{K}_P^{+}\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{\widehat{x}}_j\left(k_0\right)-B{K}_P^{-}\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{\widehat{x}}_j\left(k_0\right)\hfill \\ +B{K}_S^{+}{ϵ}_i\left(k_0\right)+B{K}_S^{-}{ϵ}_i\left(k_0\right)+B{K}_P^{+}\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{ϵ}_i\left(k_0\right)+B{K}_P^{-}\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{ϵ}_i\left(k_0\right)\hfill \\ -B{K}_P^{+}\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{ϵ}_j\left(k_0\right)-B{K}_P^{-}\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{ϵ}_j\left(k_0\right)+B{K}_C{x}_{ic}\hfill \\ ⪰{\varrho }_{1}A{x}_i\left(k_0\right)+B{K}_S^{+}{\widehat{x}}_i\left(k_0\right)+B{K}_S^{-}{\widehat{x}}_i\left(k_0\right)-\overline{\beta }B{K}_S^{+}{\mathbf{1}}_{n\times n}{\widehat{x}}_i\left(k_0\right)+\overline{\beta }B{K}_S^{-}{\mathbf{1}}_{n\times n}{\widehat{x}}_i\left(k_0\right)\hfill \\ +B{K}_P^{+}\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{\widehat{x}}_i\left(k_0\right)+B{K}_P^{-}\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{\widehat{x}}_i\left(k_0\right)-B{K}_P^{+}\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{\widehat{x}}_j\left(k_0\right)\hfill \\ -B{K}_P^{-}\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{\widehat{x}}_j\left(k_0\right)-\overline{\beta }B{K}_P^{+}{\mathbf{1}}_{n\times n}\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{\widehat{x}}_i\left(k_0\right)+\overline{\beta }B{K}_P^{-}{\mathbf{1}}_{n\times n}\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{\widehat{x}}_i\left(k_0\right)\hfill \\ -\overline{\beta }B{K}_P^{+}{\mathbf{1}}_{n\times n}\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{\widehat{x}}_j\left(k_0\right)+\overline{\beta }B{K}_P^{-}{\mathbf{1}}_{n\times n}\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{\widehat{x}}_j\left(k_0\right)+B{K}_C{x}_{ic}\hfill \\ ={\varrho }_{1}A{x}_i\left(k_0\right)+(B{K}_S^{+}(I_n-\overline{\beta }{\mathbf{1}}_{n\times n})+B{K}_S^{-}(I_n+\overline{\beta }{\mathbf{1}}_{n\times n})){\widehat{x}}_i\left(k_0\right)+B{K}_C{x}_{ic}\hfill \\ +B{K}_P^{+}(I_n-\overline{\beta }{\mathbf{1}}_{n\times n})\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{\widehat{x}}_i\left(k_0\right)-B{K}_P^{+}(I_n+\overline{\beta }{\mathbf{1}}_{n\times n})\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{\widehat{x}}_j\left(k_0\right)\hfill \\ +B{K}_P^{-}(I_n+\overline{\beta }{\mathbf{1}}_{n\times n})\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{\widehat{x}}_i\left(k_0\right)-B{K}_P^{-}(I_n-\overline{\beta }{\mathbf{1}}_{n\times n})\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{\widehat{x}}_j\left(k_0\right)\hfill \\ ={\varrho }_{1}A{x}_i\left(k_0\right)+(B{K}_S^{+}(I_n-\overline{\beta }{\mathbf{1}}_{n\times n})+B{K}_S^{-}(I_n+\overline{\beta }{\mathbf{1}}_{n\times n}))(x_i\left(k_0\right)+e_i\left(k_0\right))\hfill \\ +B{K}_P^{+}(I_n-\overline{\beta }{\mathbf{1}}_{n\times n})\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}(x_i\left(k_0\right)+e_i\left(k_0\right))-B{K}_P^{+}(I_n+\overline{\beta }{\mathbf{1}}_{n\times n})\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}(x_j\left(k_0\right)\hfill \\ +e_j\left(k_0\right))+B{K}_P^{-}(I_n+\overline{\beta }{\mathbf{1}}_{n\times n})\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}(x_i\left(k_0\right)+e_i\left(k_0\right))-B{K}_P^{-}(I_n-\overline{\beta }{\mathbf{1}}_{n\times n})\hfill \\ \sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}(x_j\left(k_0\right)+e_j\left(k_0\right))+B{K}_C{x}_{ic}\hfill \\ =({\varrho }_{1}A+B{K}_S^{+}(I_n-\overline{\beta }{\mathbf{1}}_{n\times n})+B{K}_S^{-}(I_n+\overline{\beta }{\mathbf{1}}_{n\times n}))x_i\left(k_0\right)+(B{K}_S^{+}(I_n-\overline{\beta }{\mathbf{1}}_{n\times n})\hfill \\ +B{K}_S^{-}(I_n+\overline{\beta }{\mathbf{1}}_{n\times n}))e_i\left(k_0\right)+B{K}_P^{+}(I_n-\overline{\beta }{\mathbf{1}}_{n\times n})\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{x}_i\left(k_0\right)\hfill \\ -B{K}_P^{+}(I_n+\overline{\beta }{\mathbf{1}}_{n\times n})\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{x}_j\left(k_0\right)+B{K}_P^{-}(I_n+\overline{\beta }{\mathbf{1}}_{n\times n})\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{x}_i\left(k_0\right)\hfill \\ -B{K}_P^{-}(I_n-\overline{\beta }{\mathbf{1}}_{n\times n})\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{x}_j\left(k_0\right)+B{K}_P^{+}(I_n-\overline{\beta }{\mathbf{1}}_{n\times n})\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{e}_i\left(k_0\right)\hfill \\ -B{K}_P^{+}(I_n+\overline{\beta }{\mathbf{1}}_{n\times n})\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{e}_j\left(k_0\right)+B{K}_P^{-}(I_n+\overline{\beta }{\mathbf{1}}_{n\times n})\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{e}_i\left(k_0\right)\hfill \\ -B{K}_P^{-}(I_n-\overline{\beta }{\mathbf{1}}_{n\times n})\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{e}_j\left(k_0\right)+B{K}_C{x}_{ic}\hfill \\ =({\varrho }_{1}A+B{K}_S^{+}\Psi +B{K}_S^{-}\Phi )x_i\left(k_0\right)+(B{K}_S^{+}\Psi +B{K}_S^{-}\Phi )e_i\left(k_0\right)\hfill \\ +B{K}_P^{+}\Psi \sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{x}_i\left(k_0\right)-B{K}_P^{+}\Phi \sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{x}_j\left(k_0\right)+B{K}_P^{-}\Phi \sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{x}_i\left(k_0\right)\hfill \\ -B{K}_P^{-}\Psi \sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{x}_j\left(k_0\right)+B{K}_P^{+}\Psi \sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{e}_i\left(k_0\right)-B{K}_P^{+}\Phi \sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{e}_j\left(k_0\right)\hfill \\ +B{K}_P^{-}\Phi \sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{e}_i\left(k_0\right)-B{K}_P^{-}\Psi \sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{e}_j\left(k_0\right)+B{K}_C{x}_{ic}.\hfill \end{array}$$

Appendix A.2. Additional Details for Step (42)

$$\begin{array}{c}{x}_i(k_0+1)=Af\left(x_i\left(k_0\right)\right)+B{K}_S{\widehat{x}}_i\left({\kappa }_{\iota }\right)+B{K}_P\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}({\widehat{x}}_i\left({\kappa }_{\iota }\right)-{\widehat{x}}_j\left({\kappa }_{\iota }\right))+B{K}_C{x}_{ic}\hfill \\ =Af\left(x_i\left(k_0\right)\right)+B{K}_S({ϵ}_i\left(k_0\right)+{\widehat{x}}_i\left(k_0\right))+B{K}_P\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}({ϵ}_i\left(k_0\right)+{\widehat{x}}_i\left(k_0\right)\hfill \\ -({ϵ}_j\left(k_0\right)+{\widehat{x}}_j\left(k_0\right)))+B{K}_C{x}_{ic}\hfill \\ =Af\left(x_i\left(k_0\right)\right)+B{K}_S{\widehat{x}}_i\left(k_0\right)+B{K}_S{ϵ}_i\left(k_0\right)+B{K}_P\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}({\widehat{x}}_i\left(k_0\right)-{\widehat{x}}_j\left(k_0\right))\hfill \\ +B{K}_P\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}({ϵ}_i\left(k_0\right)-{ϵ}_j\left(k_0\right))+B{K}_C{x}_{ic}\hfill \\ =Af\left(x_i\left(k_0\right)\right)+B{K}_S{\widehat{x}}_i\left(k_0\right)+B{K}_P\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{\widehat{x}}_i\left(k_0\right)-B{K}_P\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{\widehat{x}}_j\left(k_0\right)\hfill \\ +B{K}_S{ϵ}_i\left(k_0\right)+B{K}_P\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{ϵ}_i\left(k_0\right)-B{K}_P\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{ϵ}_j\left(k_0\right)+B{K}_C{x}_{ic}\hfill \\ =Af\left(x_i\left(k_0\right)\right)+B{K}_S^{+}{\widehat{x}}_i\left(k_0\right)+B{K}_S^{-}{\widehat{x}}_i\left(k_0\right)+B{K}_S^{+}{ϵ}_i\left(k_0\right)+B{K}_S^{-}{ϵ}_i\left(k_0\right)+B{K}_C{x}_{ic}\hfill \\ +B{K}_P^{+}\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{\widehat{x}}_i\left(k_0\right)+B{K}_P^{-}\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{\widehat{x}}_i\left(k_0\right)-B{K}_P^{+}\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{\widehat{x}}_j\left(k_0\right)\hfill \\ -B{K}_P^{-}\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{\widehat{x}}_j\left(k_0\right)+B{K}_P^{+}\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{ϵ}_i\left(k_0\right)+B{K}_P^{-}\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{ϵ}_i\left(k_0\right)\hfill \end{array}$$

$$\begin{array}{c}-B{K}_P^{+}\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{ϵ}_j\left(k_0\right)-B{K}_P^{-}\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{ϵ}_j\left(k_0\right)\hfill \\ ⪯{\varrho }_{2}A{x}_i\left(k_0\right)+B{K}_S^{+}{\widehat{x}}_i\left(k_0\right)+B{K}_S^{-}{\widehat{x}}_i\left(k_0\right)+\overline{\beta }B{K}_S^{+}{\mathbf{1}}_{n\times n}{\widehat{x}}_i\left(k_0\right)\hfill \\ -\overline{\beta }B{K}_S^{-}{\mathbf{1}}_{n\times n}{\widehat{x}}_i\left(k_0\right)+B{K}_P^{+}\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{\widehat{x}}_i\left(k_0\right)+B{K}_P^{-}\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{\widehat{x}}_i\left(k_0\right)\hfill \\ -B{K}_P^{+}\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{\widehat{x}}_j\left(k_0\right)-B{K}_P^{-}\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{\widehat{x}}_j\left(k_0\right)+\overline{\beta }B{K}_P^{+}{\mathbf{1}}_{n\times n}\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{\widehat{x}}_i\left(k_0\right)\hfill \\ -\overline{\beta }B{K}_P^{-}{\mathbf{1}}_{n\times n}\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{\widehat{x}}_i\left(k_0\right)+\overline{\beta }B{K}_P^{+}{\mathbf{1}}_{n\times n}\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{\widehat{x}}_j\left(k_0\right)\hfill \\ -\overline{\beta }B{K}_P^{-}{\mathbf{1}}_{n\times n}\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{\widehat{x}}_j\left(k_0\right)+B{K}_C{x}_{ic}\hfill \\ ={\varrho }_{2}A{x}_i\left(k_0\right)+(B{K}_S^{+}(I_n+\overline{\beta }{\mathbf{1}}_{n\times n})+B{K}_S^{-}(I_n-\overline{\beta }{\mathbf{1}}_{n\times n})){\widehat{x}}_i\left(k_0\right)\hfill \\ +B{K}_P^{+}(I_n+\overline{\beta }{\mathbf{1}}_{n\times n})\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{\widehat{x}}_i\left(k_0\right)-B{K}_P^{+}(I_n-\overline{\beta }{\mathbf{1}}_{n\times n})\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{\widehat{x}}_j\left(k_0\right)\hfill \\ +B{K}_P^{-}(I_n-\overline{\beta }{\mathbf{1}}_{n\times n})\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{\widehat{x}}_i\left(k_0\right)-B{K}_P^{-}(I_n+\overline{\beta }{\mathbf{1}}_{n\times n})\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{\widehat{x}}_j\left(k_0\right)+B{K}_C{x}_{ic}\hfill \\ ={\varrho }_{2}A{x}_i\left(k_0\right)+(B{K}_S^{+}(I_n+\overline{\beta }{\mathbf{1}}_{n\times n})+B{K}_S^{-}(I_n-\overline{\beta }{\mathbf{1}}_{n\times n}))(x_i\left(k_0\right)+e_i\left(k_0\right))\hfill \\ +B{K}_P^{+}(I_n+\overline{\beta }{\mathbf{1}}_{n\times n})\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}(x_i\left(k_0\right)+e_i\left(k_0\right))-B{K}_P^{+}(I_n-\overline{\beta }{\mathbf{1}}_{n\times n})\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}(x_j\left(k_0\right)\hfill \\ +e_j\left(k_0\right))+B{K}_P^{-}(I_n-\overline{\beta }{\mathbf{1}}_{n\times n})\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}(x_i\left(k_0\right)+e_i\left(k_0\right))\hfill \\ -B{K}_P^{-}(I_n+\overline{\beta }{\mathbf{1}}_{n\times n})\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}(x_j\left(k_0\right)+e_j\left(k_0\right))+B{K}_C{x}_{ic}\hfill \\ =({\varrho }_{2}A+B{K}_S^{+}(I_n+\overline{\beta }{\mathbf{1}}_{n\times n})+B{K}_S^{-}(I_n-\overline{\beta }{\mathbf{1}}_{n\times n}))x_i\left(k_0\right)+(B{K}_S^{+}(I_n+\overline{\beta }{\mathbf{1}}_{n\times n})\hfill \\ +B{K}_S^{-}(I_n-\overline{\beta }{\mathbf{1}}_{n\times n}))e_i\left(k_0\right)+B{K}_P^{+}(I_n+\overline{\beta }{\mathbf{1}}_{n\times n})\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{x}_i\left(k_0\right)\hfill \\ -B{K}_P^{+}(I_n-\overline{\beta }{\mathbf{1}}_{n\times n})\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{x}_j\left(k_0\right)+B{K}_P^{-}(I_n-\overline{\beta }{\mathbf{1}}_{n\times n})\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{x}_i\left(k_0\right)\hfill \\ -B{K}_P^{-}(I_n+\overline{\beta }{\mathbf{1}}_{n\times n})\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{x}_j\left(k_0\right)+B{K}_P^{+}(I_n+\overline{\beta }{\mathbf{1}}_{n\times n})\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{e}_i\left(k_0\right)\hfill \\ -B{K}_P^{+}(I_n-\overline{\beta }{\mathbf{1}}_{n\times n})\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{e}_j\left(k_0\right)+B{K}_P^{-}(I_n-\overline{\beta }{\mathbf{1}}_{n\times n})\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{e}_i\left(k_0\right)\hfill \\ -B{K}_P^{-}(I_n+\overline{\beta }{\mathbf{1}}_{n\times n})\sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{e}_j\left(k_0\right)+B{K}_C{x}_{ic}\hfill \\ =({\varrho }_{2}A+B{K}_S^{+}\Phi +B{K}_S^{-}\Psi )x_i\left(k_0\right)+(B{K}_S^{+}\Phi +B{K}_S^{-}\Psi )e_i\left(k_0\right)+B{K}_C{x}_{ic}\hfill \\ +B{K}_P^{+}\Phi \sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{x}_i\left(k_0\right)-B{K}_P^{+}\Psi \sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{x}_j\left(k_0\right)+B{K}_P^{-}\Psi \sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{x}_i\left(k_0\right)\hfill \\ -B{K}_P^{-}\Phi \sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{x}_j\left(k_0\right)+B{K}_P^{+}\Phi \sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{e}_i\left(k_0\right)-B{K}_P^{+}\Psi \sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{e}_j\left(k_0\right)\hfill \\ +B{K}_P^{-}\Psi \sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{e}_i\left(k_0\right)-B{K}_P^{-}\Phi \sum _{j\in {\mathcal{N}}_i}{\mathcal{A}}^{\left(ij\right)}{e}_j\left(k_0\right).\hfill \end{array}$$

Appendix A.3. Additional Details for Step (50)

$$\begin{array}{c}{\tilde{\mathcal{X}}}^{\top }\left(k\right)({\mathbb{A}}_3^{\top }-I)\nu \hfill \\ ={\left(\begin{array}{c}\xi \left(k\right)\\ \varpi \left(k\right)\\ \zeta \left(k\right)\end{array}\right)}^{\top }{\left(\begin{array}{ccc}\left(\begin{array}{c}{I}_N\otimes ({\varrho }_{2}A+B{K}_S^{+}\Phi +B{K}_S^{-}\Psi \\ +2\overline{\beta \mathcal{D}}\otimes (B{K}_P^{+}{\mathbf{1}}_{n\times n}-B{K}_P^{-}{\mathbf{1}}_{n\times n})\\ +\mathcal{L}\otimes (B{K}_P^{+}\Psi +B{K}_P^{-}\Phi ))-I_{Nn}\end{array}\right)& \left(\begin{array}{c}{I}_N\otimes (B{K}_S^{+}\Phi +B{K}_S^{-}\Psi \\ +2\overline{\beta }\overline{\mathcal{D}}\otimes (B{K}_P^{+}{\mathbf{1}}_{n\times n}-B{K}_P^{-}{\mathbf{1}}_{n\times n})\\ +\mathcal{L}\otimes (B{K}_P^{+}\Psi +B{K}_P^{-}\Phi ))\end{array}\right)& \mathbf{0}\\ I_N\otimes (({\vartheta }_2-{\varrho }_1)A+({\varsigma }_2-{\sigma }_1)L_{P}C)& I_N\otimes ({\vartheta }_{2}A+{\varsigma }_2{L}_{P}C)-I_{Nn}& I_N\otimes F\\ (1-\alpha )({\varsigma }_2-{\sigma }_1)(I_N\otimes \left(L_{I}C\right))& {\varsigma }_2(1-\alpha )(I_N\otimes \left(L_{I}C\right))& (\alpha -1)I_{Nn}\end{array}\right)}^{\top }\left(\begin{array}{c}{\tilde{\nu }}_1\\ {\tilde{\nu }}_2\\ {\tilde{\nu }}_3\end{array}\right)\hfill \\ ={\xi }^{\top }\left(k\right)\left(\right(I_N\otimes ({\varrho }_2{A}^{\top }+\Phi K_S^{+\top }{B}^{\top }+\Psi K_S^{-\top }{B}^{\top })+2\overline{\beta \mathcal{D}}\otimes ({\mathbf{1}}_{n\times n}{K}_P^{+\top }{B}^{\top }-{\mathbf{1}}_{n\times n}{K}_P^{-\top }{B}^{\top })\hfill \\ +\mathcal{L}\otimes (\Psi K_P^{+\top }{B}^{\top }+\Phi K_P^{-\top }{B}^{\top }))-I_{Nn}){\tilde{\nu }}_1+{\varpi }^{\top }\left(k\right)(I_N\otimes (\Phi K_S^{+\top }{B}^{\top }+\Psi K_S^{-\top }{B}^{\top }+2\overline{\beta \mathcal{D}}\otimes ({\mathbf{1}}_{n\times n}{K}_P^{+\top }{B}^{\top }\hfill \\ -{\mathbf{1}}_{n\times n}{K}_P^{-\top }{B}^{\top })+\mathcal{L}\otimes (\Psi K_P^{+\top }{B}^{\top }+\Phi K_P^{-\top }{B}^{\top })\left)\right){\tilde{\nu }}_1+{\xi }^{\top }\left(k\right)(I_N\otimes (({\vartheta }_2-{\varrho }_1)A^{\top }+({\varsigma }_2-{\sigma }_1)C^{\top }{L}_P^{\top })){\tilde{\nu }}_2\hfill \\ +{\varpi }^{\top }\left(k\right)(I_N\otimes ({\vartheta }_2{A}^{\top }+{\varsigma }_2{C}^{\top }{L}_P^{\top })-I_{Nn}){\tilde{\nu }}_2+{\zeta }^{\top }\left(k\right)(I_N\otimes F^{\top }){\tilde{\nu }}_2+{\xi }^{\top }\left(k\right)\left((1-\alpha )({\varsigma }_2-{\sigma }_1)(I_N\otimes \left(C^{\top }{L}_I^{\top }\right))\right){\tilde{\nu }}_3\hfill \\ +{\varpi }^{\top }\left(k\right)\left({\varsigma }_2(1-\alpha )(I_N\otimes \left(C^{\top }{L}_I^{\top }\right))\right){\tilde{\nu }}_3+(\alpha -1){\zeta }^{\top }\left(k\right){\tilde{\nu }}_3.\hfill \end{array}$$

Appendix A.4. Additional Details for Step (51)

$$\begin{array}{c}{({\mathbf{1}}_3\otimes X_C)}^{\top }{\mathbb{C}}_3^{\top }\nu \hfill \\ ={\left(\begin{array}{c}{X}_C\\ X_C\\ X_C\end{array}\right)}^{\top }{\left(\begin{array}{ccc}\left(\begin{array}{c}{I}_N\otimes ({\varrho }_{2}A+B{K}_S^{+}\Phi +B{K}_S^{-}\Psi )\\ +2\overline{\beta \mathcal{D}}\otimes (B{K}_P^{+}{\mathbf{1}}_{n\times n}-B{K}_P^{-}{\mathbf{1}}_{n\times n})\\ +\mathcal{L}\otimes (B{K}_P^{+}\Psi +B{K}_P^{-}\Phi )\\ +({\mathbf{1}}_N{\theta }^{\top }-I_N)\otimes \left(B{K}_C\right)-I_{Nn}\end{array}\right)& \mathbf{0}& \mathbf{0}\\ \mathbf{0}& \left(\begin{array}{c}{I}_N\otimes (({\vartheta }_2-{\varrho }_1)A\\ +({\varsigma }_2-{\sigma }_1)L_{P}C)\end{array}\right)& \mathbf{0}\\ \mathbf{0}& \mathbf{0}& (1-\alpha )({\varsigma }_2-{\sigma }_1)(I_N\otimes \left(L_{I}C\right))\end{array}\right)}^{\top }\left(\begin{array}{c}{\tilde{\nu }}_1\\ {\tilde{\nu }}_2\\ {\tilde{\nu }}_3\end{array}\right)\hfill \end{array}$$

$$\begin{array}{c}=X_C^{\top }(I_N\otimes ({\varrho }_2{A}^{\top }+\Phi K_S^{+\top }{B}^{\top }+\Psi K_S^{-\top }{B}^{\top })+2\overline{\beta \mathcal{D}}\otimes ({\mathbf{1}}_{n\times n}{K}_P^{+\top }{B}^{\top }-{\mathbf{1}}_{n\times n}{K}_P^{-\top }{B}^{\top })\hfill \\ +\mathcal{L}\otimes (\Psi K_P^{+\top }{B}^{\top }+\Phi K_P^{-\top }{B}^{\top })+(\theta {\mathbf{1}}_N^{\top }-I_N)\otimes \left(K_C^{\top }{B}^{\top }\right)-I_{Nn}){\tilde{\nu }}_1+X_C^{\top }(I_N\otimes (({\vartheta }_2-{\varrho }_1)A^{\top }\hfill \\ +({\varsigma }_2-{\sigma }_1)C^{\top }{L}_P^{\top }\left)\right){\tilde{\nu }}_2+X_C^{\top }\left((1-\alpha )({\varsigma }_2-{\sigma }_1)(I_N\otimes \left(C^{\top }{L}_I^{\top }\right))\right){\tilde{\nu }}_3.\hfill \end{array}$$