On Topologies Induced by Ideals, Primals, Filters and Grills

Abstract

In this paper, one-to-one correspondences and equivalences between ideals, primals, filters and grills are introduced. It is shown that the local functions and the topological spaces induced by them are the same. From this point of view, the topological properties of one topology can be derived from the topological properties that are valid in the corresponding topology.

1. Introduction

Many topologies with important applications in mathematics have been defined using some additional mathematical structures. These structures include grills, ideals, filters, and primals. These classical structures are undoubtedly some of the most important objects in topology.

A very interesting area of application for these additional structures is the technique of induced topologies; namely, the creation of a new topology from a given topology derived from ideals, primals, filters, and grills.

The notion of ideal topological spaces was studied by Kuratowski [1] and Vaidyanathaswamy [2], a famous method of creating a new topology derived from an ideal. Roughly speaking, if an ideal is given on a topological space, a new topology (called an ideal topology) can be obtained by an ideal-associated local function or its dual operator. This concept was further investigated in various directions by Jankovic and Hamlett [3,4,5,6], Dontchev et al. [7], Mandal and Mukherjee [8] and Mukherjee et al. [9]. Properties such as the decomposition of continuity, separation axioms, connectedness, compactness, resolvability, and the compatibility of topology with an ideal are central research topics of ideal topological spaces. The classical result is that if a given set is locally of the first category at every point, then it is of the first category proven by Banach [10] for metric spaces and extended to arbitrary spaces by Kuratowski [1]. Oxtoby [11] proved that the union of open sets in the first category is also of the first category. Similar results were found by Kaniewski et al. for an ideal topological space [12].

The other classical structure of general topology in the literature is the notion of grills. These were introduced by Choquet [13] and further developed by Mandal [14], Roy and Mukherjee [15,16,17], Roy and et al. [18], Thorn [19] and many others. The main idea is based on a similar course to grill-associated topology. A few operators were introduced, and the basic properties of these operators and induced topologies were studied. Also, the suitability of topology for a given grill was defined. Grill topological spaces are still a focus of research; see Azzam et al. [20], Kalaivani et al. [21] and Rajasekaran et al. [22].

Recently, Acharjee et al. [23] introduced a new type of structure called primals. They defined the notion of primal topological space by utilizing two new operators and investigated many fundamental properties of this new structure and these two operators. Moreover, the notion of a primal is a dual grill structure. Furthermore, some new studies have been developed regarding primal topological spaces since the introduction of primals; for more details, see [24,25,26,27].

Filters that are used in general topology to characterize important concepts such as continuity, initial and final structures, compactness, etc., were introduced by Henri Cartan [28]. They allow for a general theory of convergence in topological spaces when sequences do not suffice. For the purposes of our article, it is sufficient to state that ideals and filters are mutually dual concepts. Similarly, grills and primals are dual. This fact is stated in the literature, but the equality of local functions induced by primals, grills, filters, and ideals has rarely been explicitly proven. The exceptions to this are Kandil et al. [29] and Renukadevi [30], where the equality of local functions generated by ideals and grills is proven. Also, a new topology can be induced from a grill and a filter on the same set; see Modak [31,32]. At the end of this article, we will provide some other applications, where topological properties characterized by filters can be replaced by other structures; see Modak et al. [33] and Selim et al. [34].

In general, we can consider four systems, namely an ideal $\mathcal{I}$, a primal $\mathcal{P}$, a filter $\mathcal{F}$ and a grill $\mathcal{G}$, on a topological space $(X,\tau )$ as in Definition 1. The derivation of a new topology that is finer than the original topology $\tau$ is as follows: The local functions $A_{\mathcal{I}}^{*}$, $A_{\mathcal{P}}^{*}$, $A_{\mathcal{F}}^{*}$ and $A_{\mathcal{G}}^{*}$ in Definition 1 are derived from $\mathcal{I},\mathcal{P},\mathcal{F}$, and $\mathcal{G}$, and $\tau$ defines the Kuratowski closure operators $c{l}_{\mathcal{I}}$, $c{l}_{\mathcal{P}}$, $c{l}_{\mathcal{F}}$ and $c{l}_{\mathcal{G}}$; see Definition 3. In the final step, a new topology on X is defined, and denoted by ${\tau }_{\mathcal{I}}$, ${\tau }_{\mathcal{P}}$, ${\tau }_{\mathcal{F}}$ and ${\tau }_{\mathcal{G}}$ (see Theorem 9). If we look at the achieved results, we can see a striking similarity. In fact, the local functions and topologies generated in this way are equivalent.

The main concept of this article is as follows: Using correspondence between two systems (Theorems 1–6), it is possible to define their equivalence in Definition 2. Two equivalent systems generate the same topology (Theorem 10), and the results achieved in one topology can be used in a topology determined by an equivalent system. In the last section, we will show the application of this equivalence on examples of compatibility and codense topologies.

Definition 1. 

Let X be a non-empty set. The non-empty systems $\mathcal{I}$, $\mathcal{P}$, $\mathcal{F}$ and $\mathcal{G}$ of subsets of X are respectively called an ideal, a primal, a filter and a grill on X if they satisfy the following conditions in order:

$$\begin{array}{cc}\left(1\right)X\notin \mathcal{I}\hfill & \left(1\right)X\notin \mathcal{P}\hfill \\ \left(2\right)A\in \mathcal{I}\mathrm{and}B\subset A\Rightarrow B\in \mathcal{I}\hfill & \left(2\right)A\in \mathcal{P}\mathrm{and}B\subset A\Rightarrow B\in \mathcal{P}\hfill \\ \left(3\right)A\in \mathcal{I}\mathrm{and}B\in \mathcal{I}\Rightarrow A\cup B\in \mathcal{I}\hfill & \left(3\right)A\cap B\in \mathcal{P}\Rightarrow A\in \mathcal{P}\mathrm{or}B\in \mathcal{P}\hfill \\ \\ \left(1\right)\varnothing \notin \mathcal{F}\hfill & \left(1\right)\varnothing \notin \mathcal{G}\hfill \\ \left(2\right)A\in \mathcal{F}\mathrm{and}A\subset B\Rightarrow B\in \mathcal{F}\hfill & \left(2\right)A\in \mathcal{G}\mathrm{and}A\subset B\Rightarrow B\in \mathcal{G}\hfill \\ \left(3\right)A\in \mathcal{F}\mathrm{and}B\in \mathcal{F}\Rightarrow A\cap B\in \mathcal{F}\hfill & \left(3\right)A\cup B\in \mathcal{G}\Rightarrow A\in \mathcal{G}\mathrm{or}B\in \mathcal{G}\hfill \end{array}$$

Furthermore, if τ is a topology on X and $\tau \left(x\right)=\{U\in \tau :x\in U\}$, for $A\subset X$ we define four local functions that map the set A in turn into sets with respect to the above-defined ideal, primal, filter and grill.

• $\begin{array}{c}{A}_{\mathcal{I}}^{*}=\{x\in X:A\cap U\notin \mathcal{I}\mathrm{for}\mathrm{every}U\in \tau \left(x\right)\},\hfill \\ A_{\mathcal{P}}^{*}=\{x\in X:(X\backslash A)\cup (X\backslash U)\in \mathcal{P}\mathrm{for}\mathrm{every}U\in \tau \left(x\right)\},\hfill \\ A_{\mathcal{F}}^{*}=\{x\in X:(X\backslash A)\cup (X\backslash U)\notin \mathcal{F}\mathrm{for}\mathrm{every}U\in \tau \left(x\right)\},\hfill \\ A_{\mathcal{G}}^{*}=\{x\in X:A\cap U\in \mathcal{G}\mathrm{for}\mathrm{every}U\in \tau \left(x\right)\}.\hfill \end{array}$

If $(X,\tau )$ is a topological space with an ideal $\mathcal{I}$, a primal $\mathcal{P}$, a filter $\mathcal{F}$, and a grill $\mathcal{G}$ on X, then the triplets $(X,\tau ,\mathcal{I})$, $(X,\tau ,\mathcal{P})$, $(X,\tau ,\mathcal{F})$ and $(X,\tau ,\mathcal{G})$ are an ideal topological space, a primal topological space, a filter topological space and a grill topological space, respectively. Let $\mathbb{I},\mathbb{P},\mathbb{F},\mathbb{G}$ be the families of all ideals, primals, filters and grills on X, respectively. Put $\mathbb{X}=\mathbb{I}\cup \mathbb{P}\cup \mathbb{F}\cup \mathbb{G}$. If $\mathcal{Z}\in \mathbb{X}$, then $(X,\tau ,\mathcal{Z})$ is a $\mathcal{Z}$-topological space.

2. Main Results

In the following two sections, we present sixteen operators ${\mathsf{H}}_{{\mathbb{Z}}_2}^{{\mathbb{Z}}_1}$ (Theorems 1–6) between the system pairs ${\mathbb{Z}}_1,{\mathbb{Z}}_2\in \{\mathbb{I},\mathbb{P},\mathbb{F},\mathbb{G}\}$ and study their properties and compositions. Next, the equivalence between ${\mathcal{Z}}_1$ and ${\mathcal{Z}}_2\in \mathbb{X}$ is defined (Definition 2). The equality of local functions and the equality of the generated topologies are proven, provided that ${\mathcal{Z}}_1$ and ${\mathcal{Z}}_2$ are equivalent.

We define four identity operators as follows:

• $\begin{array}{cc}{\mathsf{H}}_{\mathbb{I}}^{\mathbb{I}}:\mathbb{I}\to \mathbb{I}\mathrm{by}{\mathsf{H}}_{\mathbb{I}}^{\mathbb{I}}\left(\mathcal{I}\right)=\mathcal{I},\hfill & {\mathsf{H}}_{\mathbb{P}}^{\mathbb{P}}:\mathbb{P}\to \mathbb{P}\mathrm{by}{\mathsf{H}}_{\mathbb{P}}^{\mathbb{P}}\left(\mathcal{P}\right)=\mathcal{P},\hfill \\ {\mathsf{H}}_{\mathbb{F}}^{\mathbb{F}}:\mathbb{F}\to \mathbb{F}\mathrm{by}{\mathsf{H}}_{\mathbb{F}}^{\mathbb{F}}\left(\mathcal{F}\right)=\mathcal{F},\hfill & {\mathsf{H}}_{\mathbb{G}}^{\mathbb{G}}:\mathbb{G}\to \mathbb{G}\mathrm{by}{\mathsf{H}}_{\mathbb{G}}^{\mathbb{G}}\left(\mathcal{G}\right)=\mathcal{G}.\hfill \end{array}$

In the next six theorems, the proofs of items (1)–(10) are the direct consequences of the operators’ definitions and they are left to the reader. The rest will be proven.

Theorem 1. 

Let $\mathcal{I}$ and $\mathcal{P}$ be an ideal and a primal on X, respectively. Define

• ${\mathsf{H}}_{\mathbb{P}}^{\mathbb{I}}:\mathbb{I}\to \mathbb{P}$ by ${\mathsf{H}}_{\mathbb{P}}^{\mathbb{I}}\left(\mathcal{I}\right)=\{A\subset X:X\backslash A\notin \mathcal{I}\}$,
  ${\mathsf{H}}_{\mathbb{I}}^{\mathbb{P}}:\mathbb{P}\to \mathbb{I}$ by ${\mathsf{H}}_{\mathbb{I}}^{\mathbb{P}}\left(\mathcal{P}\right)=\{A\subset X:X\backslash A\notin \mathcal{P}\}$.

Then, ${\mathsf{H}}_{\mathbb{P}}^{\mathbb{I}}\left(\mathcal{I}\right)$ and ${\mathsf{H}}_{\mathbb{I}}^{\mathbb{P}}\left(\mathcal{P}\right)$ are a primal and an ideal on X, respectively.

$\begin{array}{cc}\left(1\right)A\in {\mathsf{H}}_{\mathbb{P}}^{\mathbb{I}}\left(\mathcal{I}\right)\iff X\backslash A\notin \mathcal{I}& \left(5\right)A\in {\mathsf{H}}_{\mathbb{I}}^{\mathbb{P}}\left(\mathcal{P}\right)\iff X\backslash A\notin \mathcal{P}\\ \left(2\right)A\notin {\mathsf{H}}_{\mathbb{P}}^{\mathbb{I}}\left(\mathcal{I}\right)\iff X\backslash A\in \mathcal{I}& \left(6\right)A\notin {\mathsf{H}}_{\mathbb{I}}^{\mathbb{P}}\left(\mathcal{P}\right)\iff X\backslash A\in \mathcal{P}\\ \left(3\right)X\backslash A\in {\mathsf{H}}_{\mathbb{P}}^{\mathbb{I}}\left(\mathcal{I}\right)\iff A\notin \mathcal{I}& \left(7\right)X\backslash A\in {\mathsf{H}}_{\mathbb{I}}^{\mathbb{P}}\left(\mathcal{P}\right)\iff A\notin \mathcal{P}\\ \left(4\right)X\backslash A\notin {\mathsf{H}}_{\mathbb{P}}^{\mathbb{I}}\left(\mathcal{I}\right)\iff A\in \mathcal{I}& \left(8\right)X\backslash A\notin {\mathsf{H}}_{\mathbb{I}}^{\mathbb{P}}\left(\mathcal{P}\right)\iff A\in \mathcal{P}\\ \\ \left(9\right){\mathsf{H}}_{\mathbb{P}}^{\mathbb{I}}\left({\mathsf{H}}_{\mathbb{I}}^{\mathbb{P}}\right(\mathcal{P}\left)\right)={\mathsf{H}}_{\mathbb{P}}^{\mathbb{P}}\left(\mathcal{P}\right)=\mathcal{P}& \left(10\right){\mathsf{H}}_{\mathbb{I}}^{\mathbb{P}}\left({\mathsf{H}}_{\mathbb{P}}^{\mathbb{I}}\right(\mathcal{I}\left)\right)={\mathsf{H}}_{\mathbb{I}}^{\mathbb{I}}\left(\mathcal{I}\right)=\mathcal{I}\\ \left(11\right)A_{{\mathsf{H}}_{\mathbb{P}}^{\mathbb{I}}\left(\mathcal{I}\right)}^{*}=A_{\mathcal{I}}^{*}& \left(12\right)A_{{\mathsf{H}}_{\mathbb{I}}^{\mathbb{P}}\left(\mathcal{P}\right)}^{*}=A_{*}^{\mathcal{P}}\end{array}$

Proof. 

We prove that ${\mathsf{H}}_{\mathbb{P}}^{\mathbb{I}}\left(\mathcal{I}\right)$ is a primal.

• Since $\varnothing \in \mathcal{I}$, $X\notin {\mathsf{H}}_{\mathbb{P}}^{\mathbb{I}}\left(\mathcal{I}\right)$.
• Let $A\in {\mathsf{H}}_{\mathbb{P}}^{\mathbb{I}}\left(\mathcal{I}\right)$ and $B\subset A$. Then, $X\backslash A\notin \mathcal{I}$. Since $X\backslash A\subset X\backslash B$ and $\mathcal{I}$ is an ideal, $X\backslash B\notin \mathcal{I}$, so $B\in {\mathsf{H}}_{\mathbb{P}}^{\mathbb{I}}\left(\mathcal{I}\right)$.
• Let $A\cap B\in {\mathsf{H}}_{\mathbb{P}}^{\mathbb{I}}\left(\mathcal{I}\right)$. Then, $(X\backslash A)\cup (X\backslash B)=X\backslash A\cap B\notin \mathcal{I}$. Since $\mathcal{I}$ is an ideal, $X\backslash A$ or $X\backslash B$ is not from $\mathcal{I}$. So, $A\in {\mathsf{H}}_{\mathbb{P}}^{\mathbb{I}}\left(\mathcal{I}\right)$ or $B\in {\mathsf{H}}_{\mathbb{P}}^{\mathbb{I}}\left(\mathcal{I}\right)$. That means ${\mathsf{H}}_{\mathbb{P}}^{\mathbb{I}}\left(\mathcal{I}\right)$ is a primal.

We prove that ${\mathsf{H}}_{\mathbb{I}}^{\mathbb{P}}\left(\mathcal{P}\right)$ is an ideal.

• Since $\varnothing \in \mathcal{P}$, $X\notin {\mathsf{H}}_{\mathbb{I}}^{\mathbb{P}}\left(\mathcal{P}\right)$.
• Let $A\in {\mathsf{H}}_{\mathbb{I}}^{\mathbb{P}}\left(\mathcal{P}\right)$ and $B\subset A$. Then, $X\backslash A\notin \mathcal{P}$. Since $X\backslash A\subset X\backslash B$ and $\mathcal{P}$ is a primal, $X\backslash B\notin \mathcal{P}$, so $B\in {\mathsf{H}}_{\mathbb{I}}^{\mathbb{P}}\left(\mathcal{P}\right)$.
• Let $A,B\in {\mathsf{H}}_{\mathbb{I}}^{\mathbb{P}}\left(\mathcal{P}\right)$. Then, $X\backslash A\notin \mathcal{P}$, $X\backslash B\notin \mathcal{P}$ and $(X\backslash A)\cap (X\backslash B)=X\backslash A\cup B\notin \mathcal{P}$. So $A\cup B\in {\mathsf{H}}_{\mathbb{I}}^{\mathbb{P}}\left(\mathcal{P}\right)$. That means ${\mathsf{H}}_{\mathbb{I}}^{\mathbb{P}}\left(\mathcal{P}\right)$ is an ideal.

(11): $x\in A_{{\mathsf{H}}_{\mathbb{P}}^{\mathbb{I}}\left(\mathcal{I}\right)}^{*}$ if and only if $(X\backslash A)\cup (X\backslash U)=X\backslash A\cap U\in {\mathsf{H}}_{\mathbb{P}}^{\mathbb{I}}\left(\mathcal{I}\right)$ for any neighborhood U of x. Then, the last holds if and only if $A\cap U\notin \mathcal{I}$. Finally, the last holds if and only if $x\in A_{\mathcal{I}}^{*}$.

(12): $x\in A_{{\mathsf{H}}_{\mathbb{I}}^{\mathbb{P}}\left(\mathcal{P}\right)}^{*}$ if and only if $A\cap U\notin {\mathsf{H}}_{\mathbb{I}}^{\mathbb{P}}\left(\mathcal{P}\right)$ for any neighborhood U of x. Then, the last holds if and only if $X\backslash A\cap U=(X\backslash A)\cup (X\backslash U)\in \mathcal{P}$. Finally, the last holds if and only if $x\in A_{\mathcal{P}}^{*}$.  □

Theorem 2. 

Let $\mathcal{I}$ and $\mathcal{G}$ be an ideal and a grill on X, respectively. Define

• ${\mathsf{H}}_{\mathbb{G}}^{\mathbb{I}}:\mathbb{I}\to \mathbb{G}$ by ${\mathsf{H}}_{\mathbb{G}}^{\mathbb{I}}\left(\mathcal{I}\right)=\{A\subset X:A\notin \mathcal{I}\}$,
  ${\mathsf{H}}_{\mathbb{I}}^{\mathbb{G}}:\mathbb{G}\to \mathbb{I}$ by ${\mathsf{H}}_{\mathbb{I}}^{\mathbb{G}}\left(\mathcal{G}\right)=\{A\subset X:A\notin \mathcal{G}\}$.

Then, ${\mathsf{H}}_{\mathbb{G}}^{\mathbb{I}}\left(\mathcal{I}\right)$ and ${\mathsf{H}}_{\mathbb{I}}^{\mathbb{G}}\left(\mathcal{G}\right)$ are a grill and an ideal on X, respectively.

$\begin{array}{cc}\left(1\right)A\in {\mathsf{H}}_{\mathbb{G}}^{\mathbb{I}}\left(\mathcal{I}\right)\iff A\notin \mathcal{I}\hfill & \left(5\right)A\in {\mathsf{H}}_{\mathbb{I}}^{\mathbb{G}}\left(\mathcal{G}\right)\iff A\notin \mathcal{G}\hfill \\ \left(2\right)A\notin {\mathsf{H}}_{\mathbb{G}}^{\mathbb{I}}\left(\mathcal{I}\right)\iff A\in \mathcal{I}\hfill & \left(6\right)A\notin {\mathsf{H}}_{\mathbb{I}}^{\mathbb{G}}\left(\mathcal{G}\right)\iff A\in \mathcal{G}\hfill \\ \left(3\right)X\backslash A\in {\mathsf{H}}_{\mathbb{G}}^{\mathbb{I}}\left(\mathcal{I}\right)\iff X\backslash A\notin \mathcal{I}\hfill & \left(7\right)X\backslash A\in {\mathsf{H}}_{\mathbb{I}}^{\mathbb{G}}\left(\mathcal{G}\right)\iff X\backslash A\notin \mathcal{G}\hfill \\ \left(4\right)X\backslash A\notin {\mathsf{H}}_{\mathbb{G}}^{\mathbb{I}}\left(\mathcal{I}\right)\iff X\backslash A\in \mathcal{I}\hfill & \left(8\right)X\backslash A\notin {\mathsf{H}}_{\mathbb{I}}^{\mathbb{G}}\left(\mathcal{G}\right)\iff X\backslash A\in \mathcal{G}\hfill \\ \\ \left(9\right){\mathsf{H}}_{\mathbb{G}}^{\mathbb{I}}\left({\mathsf{H}}_{\mathbb{I}}^{\mathbb{G}}\left(\mathcal{G}\right)\right)={\mathsf{H}}_{\mathbb{G}}^{\mathbb{G}}\left(\mathcal{G}\right)=\mathcal{G}\hfill & \left(10\right){\mathsf{H}}_{\mathbb{I}}^{\mathbb{G}}\left({\mathsf{H}}_{\mathbb{G}}^{\mathbb{I}}\left(\mathcal{I}\right)\right)={\mathsf{H}}_{\mathbb{I}}^{\mathbb{I}}\left(\mathcal{I}\right)=\mathcal{I}\hfill \\ \left(11\right)A_{{\mathsf{H}}_{\mathbb{G}}^{\mathbb{I}}\left(\mathcal{I}\right)}^{*}=A_{\mathcal{I}}^{*}\hfill & \left(12\right)A_{{\mathsf{H}}_{\mathbb{I}}^{\mathbb{G}}\left(\mathcal{G}\right)}^{*}=A_{\mathcal{G}}^{*}\hfill \end{array}$

Proof. 

We prove that ${\mathsf{H}}_{\mathbb{G}}^{\mathbb{I}}\left(\mathcal{I}\right)$ is a grill.

• Since $\varnothing \in \mathcal{I}$, $\varnothing \notin {\mathsf{H}}_{\mathbb{G}}^{\mathbb{I}}\left(\mathcal{I}\right)$.
• Let $A\in {\mathsf{H}}_{\mathbb{G}}^{\mathbb{I}}\left(\mathcal{I}\right)$ and $A\subset B$. Then, $A\notin \mathcal{I}$. Since $\mathcal{I}$ is an ideal, $B\notin \mathcal{I}$, so $B\in {\mathsf{H}}_{\mathbb{G}}^{\mathbb{I}}\left(\mathcal{I}\right)$.
• Let $A\cup B\in {\mathsf{H}}_{\mathbb{G}}^{\mathbb{I}}\left(\mathcal{I}\right)$. Then, $A\cup B\notin \mathcal{I}$. Since $\mathcal{I}$ is an ideal, A or B is not from $\mathcal{I}$. So, $A\in {\mathsf{H}}_{\mathbb{G}}^{\mathbb{I}}\left(\mathcal{I}\right)$ or $B\in {\mathsf{H}}_{\mathbb{G}}^{\mathbb{I}}\left(\mathcal{I}\right)$. That means ${\mathsf{H}}_{\mathbb{G}}^{\mathbb{I}}\left(\mathcal{I}\right)$ is a grill.

We prove that ${\mathsf{H}}_{\mathbb{I}}^{\mathbb{G}}\left(\mathcal{G}\right)$ is an ideal.

• Since $X\in \mathcal{G}$, $X\notin {\mathsf{H}}_{\mathbb{I}}^{\mathbb{G}}\left(\mathcal{G}\right)$.
• Let $A\in {\mathsf{H}}_{\mathbb{I}}^{\mathbb{G}}\left(\mathcal{G}\right)$ and $B\subset A$. Then, $A\notin \mathcal{G}$. Since $\mathcal{G}$ is a grill, $B\notin \mathcal{G}$, so $B\in {\mathsf{H}}_{\mathbb{I}}^{\mathbb{G}}\left(\mathcal{G}\right)$.
• Let $A,B\in {\mathsf{H}}_{\mathbb{I}}^{\mathbb{G}}\left(\mathcal{G}\right)$. Then, $A,B\notin \mathcal{G}$. Since $\mathcal{G}$ is a grill, $A\cup B\notin \mathcal{G}$. So, $A\cup B\in {\mathsf{H}}_{\mathbb{I}}^{\mathbb{G}}\left(\mathcal{G}\right)$. That means ${\mathsf{H}}_{\mathbb{I}}^{\mathbb{G}}\left(\mathcal{G}\right)$ is an ideal.

(11): $x\in A_{{\mathsf{H}}_{\mathbb{G}}^{\mathbb{I}}\left(\mathcal{I}\right)}^{*}$ if and only if $A\cap U\in {\mathsf{H}}_{\mathbb{G}}^{\mathbb{I}}\left(\mathcal{I}\right)$ for any neighborhood U of x. Then, the last holds if and only if $A\cap U\notin \mathcal{I}$. Finally, the last holds if and only if $x\in A_{\mathcal{I}}^{*}$.

(12): $x\in A_{{\mathsf{H}}_{\mathbb{I}}^{\mathbb{G}}\left(\mathcal{G}\right)}^{*}$ if and only if $A\cap U\notin {\mathsf{H}}_{\mathbb{I}}^{\mathbb{G}}\left(\mathcal{G}\right)$ for any neighborhood U of x. Then, the last holds if and only if $A\cap U\in \mathcal{G}$ and if and only if $x\in A_{\mathcal{G}}^{*}$.  □

Theorem 3. 

Let $\mathcal{F}$ and $\mathcal{G}$ be a filter and a grill on X, respectively. Define

• ${\mathsf{H}}_{\mathbb{G}}^{\mathbb{F}}:\mathbb{F}\to \mathbb{G}$ by ${\mathsf{H}}_{\mathbb{G}}^{\mathbb{F}}\left(\mathcal{F}\right)=\{A\subset X:X\backslash A\notin \mathcal{F}\}$,
  ${\mathsf{H}}_{\mathbb{F}}^{\mathbb{G}}:\mathbb{G}\to \mathbb{F}$ by ${\mathsf{H}}_{\mathbb{F}}^{\mathbb{G}}\left(\mathcal{G}\right)=\{A\subset X:X\backslash A\notin \mathcal{G}\}$.

Then, ${\mathsf{H}}_{\mathbb{G}}^{\mathbb{F}}\left(\mathcal{F}\right)$, and ${\mathsf{H}}_{\mathbb{F}}^{\mathbb{G}}\left(\mathcal{G}\right)$ are a grill and a filter on X, respectively.

$\begin{array}{cc}\left(1\right)A\in {\mathsf{H}}_{\mathbb{G}}^{\mathbb{F}}\left(\mathcal{F}\right)\iff X\backslash A\notin \mathcal{F}\hfill & \left(5\right)A\in {\mathsf{H}}_{\mathbb{F}}^{\mathbb{G}}\left(\mathcal{G}\right)\iff X\backslash A\notin \mathcal{G}\hfill \\ \left(2\right)A\notin {\mathsf{H}}_{\mathbb{G}}^{\mathbb{F}}\left(\mathcal{F}\right)\iff X\backslash A\in \mathcal{F}\hfill & \left(6\right)A\notin {\mathsf{H}}_{\mathbb{F}}^{\mathbb{G}}\left(\mathcal{G}\right)\iff X\backslash A\in \mathcal{G}\hfill \\ \left(3\right)X\backslash A\in {\mathsf{H}}_{\mathbb{G}}^{\mathbb{F}}\left(\mathcal{F}\right)\iff A\notin \mathcal{F}\hfill & \left(7\right)X\backslash A\in {\mathsf{H}}_{\mathbb{F}}^{\mathbb{G}}\left(\mathcal{G}\right)\iff A\notin \mathcal{G}\hfill \\ \left(4\right)X\backslash A\notin {\mathsf{H}}_{\mathbb{G}}^{\mathbb{F}}\left(\mathcal{F}\right)\iff A\in \mathcal{F}\hfill & \left(8\right)X\backslash A\notin {\mathsf{H}}_{\mathbb{F}}^{\mathbb{G}}\left(\mathcal{G}\right)\iff A\in \mathcal{G}\hfill \\ \\ \left(9\right){\mathsf{H}}_{\mathbb{G}}^{\mathbb{F}}\left({\mathsf{H}}_{\mathbb{F}}^{\mathbb{G}}\left(\mathcal{G}\right)\right)={\mathsf{H}}_{\mathbb{G}}^{\mathbb{G}}\left(\mathcal{G}\right)=\mathcal{G}\hfill & \left(10\right){\mathsf{H}}_{\mathbb{F}}^{\mathbb{G}}\left({\mathsf{H}}_{\mathbb{G}}^{\mathbb{F}}\left(\mathcal{F}\right)\right)={\mathsf{H}}_{\mathbb{F}}^{\mathbb{F}}\left(\mathcal{F}\right)=\mathcal{F}\hfill \\ \left(11\right)A_{{\mathsf{H}}_{\mathbb{G}}^{\mathbb{F}}\left(\mathcal{F}\right)}^{*}=A_{\mathcal{F}}^{*}\hfill & \left(12\right)A_{{\mathsf{H}}_{\mathbb{F}}^{\mathbb{G}}\left(\mathcal{G}\right)}^{*}=A_{\mathcal{G}}^{*}\hfill \end{array}$

Proof. 

We prove that ${\mathsf{H}}_{\mathbb{G}}^{\mathbb{F}}\left(\mathcal{F}\right)$ is a grill.

• Since $X\in \mathcal{F}$, $\varnothing \notin {\mathsf{H}}_{\mathbb{G}}^{\mathbb{F}}\left(\mathcal{F}\right)$.
• Let $A\in {\mathsf{H}}_{\mathbb{G}}^{\mathbb{F}}\left(\mathcal{F}\right)$ and $A\subset B$. Then, $X\backslash A\notin \mathcal{F}$. Since $\mathcal{F}$ is a filter and $X\backslash B\subset X\backslash A$, $X\backslash B\notin \mathcal{F}$, so $B\in {\mathsf{H}}_{\mathbb{G}}^{\mathbb{F}}\left(\mathcal{F}\right)$.
• Let $A\cup B\in {\mathsf{H}}_{\mathbb{G}}^{\mathbb{F}}\left(\mathcal{F}\right)$. Then, $X\backslash A\cup B=(X\backslash A)\cap (X\backslash B)\notin \mathcal{F}$. Since $\mathcal{F}$ is an filter, $X\backslash A$ or $X\backslash B$ is not from $\mathcal{F}$. So, $A\in {\mathsf{H}}_{\mathbb{G}}^{\mathbb{F}}\left(\mathcal{I}\right)$ or $B\in {\mathsf{H}}_{\mathbb{G}}^{\mathbb{F}}\left(\mathcal{I}\right)$. That means ${\mathsf{H}}_{\mathbb{G}}^{\mathbb{F}}\left(\mathcal{I}\right)$ is a grill.

We prove that ${\mathsf{H}}_{\mathbb{F}}^{\mathbb{G}}\left(\mathcal{G}\right)$ is a filter.

• Since $X\in \mathcal{G}$, $\varnothing \notin {\mathsf{H}}_{\mathbb{F}}^{\mathbb{G}}\left(\mathcal{G}\right)$.
• Let $A\in {\mathsf{H}}_{\mathbb{F}}^{\mathbb{G}}\left(\mathcal{G}\right)$ and $A\subset B$. Then, $X\backslash A\notin \mathcal{G}$. Since $\mathcal{G}$ is a grill and $X\backslash B\subset X\backslash A$, $X\backslash B\notin \mathcal{G}$, so $B\in {\mathsf{H}}_{\mathbb{F}}^{\mathbb{G}}\left(\mathcal{G}\right)$.
• Let $A,B\in {\mathsf{H}}_{\mathbb{F}}^{\mathbb{G}}\left(\mathcal{G}\right)$. Then, $X\backslash A\notin \mathcal{G}$, $X\backslash B\notin \mathcal{G}$. Then, $(X\backslash A)\cup (X\backslash B)=X\backslash A\cap B\notin \mathcal{G}$. So, $A\cap B\in {\mathsf{H}}_{\mathbb{F}}^{\mathbb{G}}\left(\mathcal{G}\right)$. That means ${\mathsf{H}}_{\mathbb{F}}^{\mathbb{G}}\left(\mathcal{G}\right)$ is a filter.

(11): $x\in A_{{\mathsf{H}}_{\mathbb{G}}^{\mathbb{F}}\left(\mathcal{F}\right)}^{*}$ if and only if $A\cap U\in {\mathsf{H}}_{\mathbb{G}}^{\mathbb{F}}\left(\mathcal{F}\right)$ for any neighborhood U of x. Then, the last holds if and only if $X\backslash A\cap U\notin \mathcal{F}$. Finally, the last holds if and only if $x\in A_{\mathcal{F}}^{*}$.

(12): $x\in A_{{\mathsf{H}}_{\mathbb{F}}^{\mathbb{G}}\left(\mathcal{G}\right)}^{*}$ if and only if $X\backslash A\cap U\notin {\mathsf{H}}_{\mathbb{F}}^{\mathbb{G}}\left(\mathcal{G}\right)$ for any neighborhood U of x. Then, the last holds if and only if $A\cap U\in \mathcal{G}$. Finally, the last holds if and only if $x\in A_{\mathcal{G}}^{*}$.  □

Theorem 4. 

Let $\mathcal{F}$ and $\mathcal{P}$ be a filter and a primal on X, respectively. Define

• ${\mathsf{H}}_{\mathbb{P}}^{\mathbb{F}}:\mathbb{F}\to \mathbb{P}$ by ${\mathsf{H}}_{\mathbb{P}}^{\mathbb{F}}\left(\mathcal{F}\right)=\{A\subset X:A\notin \mathcal{F}\}$,
  ${\mathsf{H}}_{\mathbb{F}}^{\mathbb{P}}:\mathbb{P}\to \mathbb{F}$ by ${\mathsf{H}}_{\mathbb{F}}^{\mathbb{P}}\left(\mathcal{P}\right)=\{A\subset X:A\notin \mathcal{P}\}$.

Then, ${\mathsf{H}}_{\mathbb{P}}^{\mathbb{F}}\left(\mathcal{F}\right)$ and ${\mathsf{H}}_{\mathbb{F}}^{\mathbb{P}}\left(\mathcal{P}\right)$ are a primal and a filter on X, respectively.

$\begin{array}{cc}\left(1\right)A\in {\mathsf{H}}_{\mathbb{P}}^{\mathbb{F}}\left(\mathcal{F}\right)\iff A\notin \mathcal{F}\hfill & \left(5\right)A\in {\mathsf{H}}_{\mathbb{F}}^{\mathbb{P}}\left(\mathcal{P}\right)\iff A\notin \mathcal{P}\hfill \\ \left(2\right)A\notin {\mathsf{H}}_{\mathbb{P}}^{\mathbb{F}}\left(\mathcal{F}\right)\iff A\in \mathcal{F}\hfill & \left(6\right)A\notin {\mathsf{H}}_{\mathbb{F}}^{\mathbb{P}}\left(\mathcal{P}\right)\iff A\in \mathcal{P}\hfill \\ \left(3\right)X\backslash A\in {\mathsf{H}}_{\mathbb{P}}^{\mathbb{F}}\left(\mathcal{F}\right)\iff X\backslash A\notin \mathcal{F}\hfill & \left(7\right)X\backslash A\in {\mathsf{H}}_{\mathbb{F}}^{\mathbb{P}}\left(\mathcal{P}\right)\iff X\backslash A\notin \mathcal{P}\hfill \\ \left(4\right)X\backslash A\notin {\mathsf{H}}_{\mathbb{P}}^{\mathbb{F}}\left(\mathcal{F}\right)\iff X\backslash A\in \mathcal{F}\hfill & \left(8\right)X\backslash A\notin {\mathsf{H}}_{\mathbb{F}}^{\mathbb{P}}\left(\mathcal{P}\right)\iff X\backslash A\in \mathcal{P}\hfill \\ \\ \left(9\right){\mathsf{H}}_{\mathbb{P}}^{\mathbb{F}}\left({\mathsf{H}}_{\mathbb{F}}^{\mathbb{P}}\right(\mathcal{P}\left)\right)={\mathsf{H}}_{\mathbb{P}}^{\mathbb{P}}\left(\mathcal{P}\right)=\mathcal{P}\hfill & \left(10\right){\mathsf{H}}_{\mathbb{F}}^{\mathbb{P}}\left({\mathsf{H}}_{\mathbb{P}}^{\mathbb{F}}\right(\mathcal{P}\left)\right)={\mathsf{H}}_{\mathbb{F}}^{\mathbb{F}}\left(\mathcal{F}\right)=\mathcal{F}\hfill \\ \left(11\right)A_{{\mathsf{H}}_{\mathbb{F}}^{\mathbb{F}}\left(\mathcal{F}\right)}^{*}=A_{\mathcal{F}}^{*}\hfill & \left(12\right)A_{{\mathsf{H}}_{\mathbb{F}}^{\mathbb{P}}\left(\mathcal{P}\right)}^{*}=A_{\mathcal{P}}^{*}\hfill \end{array}$

Proof. 

We prove that ${\mathsf{H}}_{\mathbb{P}}^{\mathbb{F}}\left(\mathcal{F}\right)$ is a primal.

• Since $X\in \mathcal{F}$, $X\notin {\mathsf{H}}_{\mathbb{P}}^{\mathbb{F}}\left(\mathcal{F}\right)$.
• Let $A\in {\mathsf{H}}_{\mathbb{P}}^{\mathbb{F}}\left(\mathcal{F}\right)$ and $B\subset A$. Then, $A\notin \mathcal{F}$. Since $\mathcal{F}$ is a filter, $B\notin \mathcal{F}$, so $B\in {\mathsf{H}}_{\mathbb{P}}^{\mathbb{F}}\left(\mathcal{F}\right)$.
• Let $A\cap B\in {\mathsf{H}}_{\mathbb{P}}^{\mathbb{F}}\left(\mathcal{F}\right)$. Then $A\cap B\notin \mathcal{F}$. Since $\mathcal{F}$ is a filter, A or B is not from $\mathcal{F}$. So, $A\in {\mathsf{H}}_{\mathbb{P}}^{\mathbb{F}}\left(\mathcal{F}\right)$ or $B\in {\mathsf{H}}_{\mathbb{P}}^{\mathbb{F}}\left(\mathcal{F}\right)$. That means ${\mathsf{H}}_{\mathbb{P}}^{\mathbb{F}}\left(\mathcal{F}\right)$ is a primal.

We prove that ${\mathsf{H}}_{\mathbb{F}}^{\mathbb{P}}\left(\mathcal{P}\right)$ is a filter.

• Since $\varnothing \in \mathcal{P}$, $\varnothing \notin {\mathsf{H}}_{\mathbb{F}}^{\mathbb{P}}\left(\mathcal{P}\right)$.
• Let $A\in {\mathsf{H}}_{\mathbb{F}}^{\mathbb{P}}\left(\mathcal{P}\right)$ and $A\subset B$. Then, $A\notin \mathcal{P}$. Since $\mathcal{P}$ is a primal, $B\notin \mathcal{P}$, so $B\in {\mathsf{H}}_{\mathbb{F}}^{\mathbb{P}}\left(\mathcal{P}\right)$.
• Let $A,B\in {\mathsf{H}}_{\mathbb{F}}^{\mathbb{P}}\left(\mathcal{P}\right)$. Then, $A,B\notin \mathcal{P}$. Since $\mathcal{P}$ is a primal, $A\cap B\notin \mathcal{P}$. So, $A\cap B\in {\mathsf{H}}_{\mathbb{F}}^{\mathbb{P}}\left(\mathcal{P}\right)$. That means ${\mathsf{H}}_{\mathbb{F}}^{\mathbb{P}}\left(\mathcal{P}\right)$ is a filter.

(11): $x\in A_{{\mathsf{H}}_{\mathbb{P}}^{\mathbb{F}}\left(\mathcal{F}\right)}^{*}$ if and only if $(X\backslash A)\cup (X\backslash U)=X\backslash A\cap U\in {\mathsf{H}}_{\mathbb{P}}^{\mathbb{F}}\left(\mathcal{F}\right)$ for any neighborhood U of x. Then, the last holds if and only if $X\backslash A\cap U\notin \mathcal{F}$. Finally, the last holds if and only if $x\in A_{\mathcal{F}}^{*}$.

(12): $x\in A_{{\mathsf{H}}_{\mathbb{F}}^{\mathbb{P}}\left(\mathcal{P}\right)}^{*}$ if and only if $X\backslash A\cap U\notin {\mathsf{H}}_{\mathbb{F}}^{\mathbb{P}}\left(\mathcal{P}\right)$ for any neighborhood U of x. Then, the last holds if and only if $X\backslash A\cap U=(X\backslash A)\cup (X\backslash U)\in \mathcal{P}$. Finally, the last holds if and only if $x\in A_{\mathcal{P}}^{*}$.  □

Theorem 5. 

Let $\mathcal{G}$ and $\mathcal{P}$ be a grill and a primal on X, respectively. Define

• ${\mathsf{H}}_{\mathbb{P}}^{\mathbb{G}}:\mathbb{G}\to \mathbb{P}$ by ${\mathsf{F}}_{\mathbb{P}}^{\mathbb{G}}\left(\mathcal{G}\right)=\{A\subset X:X\backslash A\in \mathcal{G}\}$,
  ${\mathsf{H}}_{\mathbb{G}}^{\mathbb{P}}:\mathbb{P}\to \mathbb{G}$ by ${\mathsf{H}}_{\mathbb{G}}^{\mathbb{P}}\left(\mathcal{P}\right)=\{A\subset X:X\backslash A\in \mathcal{P}\}$.

Then, ${\mathsf{H}}_{\mathbb{P}}^{\mathbb{G}}\left(\mathcal{G}\right)$ and ${\mathsf{H}}_{\mathbb{G}}^{\mathbb{P}}\left(\mathcal{P}\right)$ are a primal and a grill on X, respectively.

$\begin{array}{cc}\left(1\right)A\in {\mathsf{H}}_{\mathbb{P}}^{\mathbb{G}}\left(\mathcal{G}\right)\iff X\backslash A\in \mathcal{G}\hfill & \left(5\right)A\in {\mathsf{H}}_{\mathbb{G}}^{\mathbb{P}}\left(\mathcal{P}\right)\iff X\backslash A\in \mathcal{P}\hfill \\ \left(2\right)A\notin {\mathsf{H}}_{\mathbb{P}}^{\mathbb{G}}\left(\mathcal{G}\right)\iff X\backslash A\notin \mathcal{G}\hfill & \left(6\right)A\notin {\mathsf{H}}_{\mathbb{G}}^{\mathbb{P}}\left(\mathcal{P}\right)\iff X\backslash A\notin \mathcal{P}\hfill \\ \left(3\right)X\backslash A\in {\mathsf{H}}_{\mathbb{P}}^{\mathbb{G}}\left(\mathcal{G}\right)\iff A\in \mathcal{G}\hfill & \left(7\right)X\backslash A\in {\mathsf{H}}_{\mathbb{G}}^{\mathbb{P}}\left(\mathcal{P}\right)\iff A\in \mathcal{P}\hfill \\ \left(4\right)X\backslash A\notin {\mathsf{H}}_{\mathbb{P}}^{\mathbb{G}}\left(\mathcal{G}\right)\iff A\notin \mathcal{G}\hfill & \left(8\right)X\backslash A\notin {\mathsf{H}}_{\mathbb{G}}^{\mathbb{P}}\left(\mathcal{P}\right)\iff A\notin \mathcal{P}\hfill \\ \\ \left(9\right){\mathsf{H}}_{\mathbb{P}}^{\mathbb{G}}\left({\mathsf{H}}_{\mathbb{G}}^{\mathbb{P}}\right(\mathcal{P}\left)\right)={\mathsf{H}}_{\mathbb{P}}^{\mathbb{P}}\left(\mathcal{P}\right)=\mathcal{P}\hfill & \left(10\right){\mathsf{H}}_{\mathbb{G}}^{\mathbb{P}}\left({\mathsf{H}}_{\mathbb{P}}^{\mathbb{G}}\right(\mathcal{P}\left)\right)={\mathsf{H}}_{\mathbb{G}}^{\mathbb{G}}\left(\mathcal{G}\right)=\mathcal{G}\hfill \\ \left(11\right)A_{{\mathsf{H}}_{\mathbb{P}}^{\mathbb{G}}\left(\mathcal{G}\right)}^{*}=A_{\mathcal{G}}^{*}\hfill & \left(12\right)A_{{\mathsf{H}}_{\mathbb{G}}^{\mathbb{P}}\left(\mathcal{P}\right)}^{*}=A_{\mathcal{P}}^{*}\hfill \end{array}$

Proof. 

We prove that ${\mathsf{H}}_{\mathbb{P}}^{\mathbb{G}}\left(\mathcal{G}\right)$ is a primal.

• Since $\varnothing \notin \mathcal{G}$, $X\notin {\mathsf{H}}_{\mathbb{P}}^{\mathbb{G}}\left(\mathcal{G}\right)$.
• Let $A\in {\mathsf{H}}_{\mathbb{P}}^{\mathbb{G}}\left(\mathcal{G}\right)$ and $B\subset A$. Then, $X\backslash A\in \mathcal{G}$. Since $\mathcal{G}$ is a grill and $X\backslash A\subset X\backslash B$, $X\backslash B\in \mathcal{G}$, so $B\in {\mathsf{H}}_{\mathbb{P}}^{\mathbb{G}}\left(\mathcal{G}\right)$.
• Let $A\cap B\in {\mathsf{H}}_{\mathbb{P}}^{\mathbb{G}}\left(\mathcal{G}\right)$. Then, $X\backslash A\cap B=(X\backslash A)\cup (X\backslash B)\in \mathcal{G}$. Since $\mathcal{G}$ is a grill, $X\backslash A$ or $X\backslash B$ is from $\mathcal{G}$. So, $A\in {\mathsf{H}}_{\mathbb{P}}^{\mathbb{G}}\left(\mathcal{G}\right)$ or $B\in {\mathsf{H}}_{\mathbb{P}}^{\mathbb{G}}\left(\mathcal{G}\right)$. That means ${\mathsf{H}}_{\mathbb{P}}^{\mathbb{G}}\left(\mathcal{G}\right)$ is a primal.

We prove that ${\mathsf{H}}_{\mathbb{G}}^{\mathbb{P}}\left(\mathcal{P}\right)$ is a grill.

• Since $X\notin \mathcal{P}$, $\varnothing \notin {\mathsf{H}}_{\mathbb{G}}^{\mathbb{P}}\left(\mathcal{P}\right)$.
• Let $A\in {\mathsf{H}}_{\mathbb{G}}^{\mathbb{P}}\left(\mathcal{P}\right)$ and $A\subset B$. Then, $X\backslash A\in \mathcal{P}$. Since $\mathcal{P}$ is a primal and $X\backslash B\subset X\backslash A$, $X\backslash B\in \mathcal{P}$, so $B\in {\mathsf{H}}_{\mathbb{G}}^{\mathbb{P}}\left(\mathcal{P}\right)$.
• Let $A\cup B\in {\mathsf{H}}_{\mathbb{G}}^{\mathbb{P}}\left(\mathcal{P}\right)$. Then, $X\backslash A\cup B=(X\backslash A)\cap (X\backslash B)\in \mathcal{P}$. Since $\mathcal{P}$ is a primal, $X\backslash A$ or $X\backslash B$ is from $\mathcal{P}$. So, $A\in {\mathsf{H}}_{\mathbb{G}}^{\mathbb{P}}\left(\mathcal{P}\right)$ or $B\in {\mathsf{H}}_{\mathbb{G}}^{\mathbb{P}}\left(\mathcal{P}\right)$. That means ${\mathsf{H}}_{\mathbb{G}}^{\mathbb{P}}\left(\mathcal{P}\right)$ is a grill.

(11): $x\in A_{{\mathsf{H}}_{\mathbb{P}}^{\mathbb{G}}\left(\mathcal{G}\right)}^{*}$ if and only if $(X\backslash A)\cup (X\backslash U)=X\backslash A\cap U\in {\mathsf{H}}_{\mathbb{P}}^{\mathbb{G}}\left(\mathcal{G}\right)$ for any neighborhood U of x. Then, the last holds if and only if $A\cap U\in \mathcal{G}$. Finally, the last holds if and only if $x\in A_{\mathcal{G}}^{*}$.

(12): $x\in A_{{\mathsf{H}}_{\mathbb{G}}^{\mathbb{P}}\left(\mathcal{P}\right)}^{*}$ if and only if $A\cap U\in {\mathsf{H}}_{\mathbb{G}}^{\mathbb{P}}\left(\mathcal{P}\right)$ for any neighborhood U of x. Then, the last holds if and only if $X\backslash A\cap U=(X\backslash A)\cup (X\backslash U)\in \mathcal{P}$. Finally, the last holds if and only if $x\in A_{\mathcal{P}}^{*}$.  □

Theorem 6. 

Let $\mathcal{F}$ and $\mathcal{I}$ be a filter and a ideal on X, respectively. Define

• ${\mathsf{H}}_{\mathbb{I}}^{\mathbb{F}}:\mathbb{F}\to \mathbb{I}$ by ${\mathsf{H}}_{\mathbb{I}}^{\mathbb{F}}\left(\mathcal{F}\right)=\{A\subset X:X\backslash A\in \mathcal{F}\}$,
  ${\mathsf{H}}_{\mathbb{F}}^{\mathbb{I}}:\mathbb{I}\to \mathbb{F}$ by ${\mathsf{H}}_{\mathbb{F}}^{\mathbb{I}}\left(\mathcal{I}\right)=\{A\subset X:X\backslash A\in \mathcal{I}\}$.

Then, ${\mathsf{H}}_{\mathbb{I}}^{\mathbb{F}}\left(\mathcal{F}\right)$ and ${\mathsf{H}}_{\mathbb{F}}^{\mathbb{I}}\left(\mathcal{I}\right)$ are an ideal and a filter on X, respectively.

$\begin{array}{cc}\left(1\right)A\in {\mathsf{H}}_{\mathbb{I}}^{\mathbb{F}}\left(\mathcal{F}\right)\iff X\backslash A\in \mathcal{F}\hfill & \left(5\right)A\in {\mathsf{H}}_{\mathbb{F}}^{\mathbb{I}}\left(\mathcal{I}\right)\iff X\backslash A\in \mathcal{I}\hfill \\ \left(2\right)A\notin {\mathsf{H}}_{\mathbb{I}}^{\mathbb{F}}\left(\mathcal{F}\right)\iff X\backslash A\notin \mathcal{F}\hfill & \left(6\right)A\notin {\mathsf{H}}_{\mathbb{F}}^{\mathbb{I}}\left(\mathcal{I}\right)\iff X\backslash A\notin \mathcal{I}\hfill \\ \left(3\right)X\backslash A\in {\mathsf{H}}_{\mathbb{I}}^{\mathbb{F}}\left(\mathcal{F}\right)\iff A\in \mathcal{F}\hfill & \left(7\right)X\backslash A\in {\mathsf{H}}_{\mathbb{F}}^{\mathbb{I}}\left(\mathcal{I}\right)\iff A\in \mathcal{I}\hfill \\ \left(4\right)X\backslash A\notin {\mathsf{H}}_{\mathbb{I}}^{\mathbb{F}}\left(\mathcal{F}\right)\iff A\notin \mathcal{F}\hfill & \left(8\right)X\backslash A\notin {\mathsf{H}}_{\mathbb{F}}^{\mathbb{I}}\left(\mathcal{I}\right)\iff A\notin \mathcal{I}\hfill \\ \\ \left(9\right){\mathsf{H}}_{\mathbb{I}}^{\mathbb{F}}\left({\mathsf{H}}_{\mathbb{F}}^{\mathbb{I}}\right(\mathcal{I}\left)\right)={\mathsf{H}}_{\mathbb{I}}^{\mathbb{I}}\left(\mathcal{I}\right)=\mathcal{I}\hfill & \left(10\right){\mathsf{H}}_{\mathbb{F}}^{\mathbb{I}}\left({\mathsf{H}}_{\mathbb{I}}^{\mathbb{F}}\right(\mathcal{F}\left)\right)={\mathsf{H}}_{\mathbb{F}}^{\mathbb{F}}\left(\mathcal{F}\right)=\mathcal{F}\hfill \\ \left(11\right)A_{{\mathsf{H}}_{\mathbb{I}}^{\mathbb{F}}\left(\mathcal{I}\right)}^{*}=A_{\mathcal{F}}^{*}\hfill & \left(12\right)A_{{\mathsf{H}}_{\mathbb{F}}^{\mathbb{I}}\left(\mathcal{I}\right)}^{*}=A_{\mathcal{I}}^{*}\hfill \end{array}$

Proof. 

We prove that ${\mathsf{H}}_{\mathbb{I}}^{\mathbb{F}}\left(\mathcal{F}\right)$ is an ideal.

• Since $\varnothing \notin \mathcal{F}$, $X\notin {\mathsf{H}}_{\mathbb{I}}^{\mathbb{F}}\left(\mathcal{F}\right)$.
• Let $A\in {\mathsf{H}}_{\mathbb{I}}^{\mathbb{F}}\left(\mathcal{F}\right)$ and $B\subset A$. Then, $X\backslash A\in \mathcal{F}$. Since $X\backslash A\subset X\backslash B$ and $\mathcal{F}$ is a filter, $X\backslash B\in \mathcal{F}$, so $B\in {\mathsf{H}}_{\mathbb{I}}^{\mathbb{F}}\left(\mathcal{F}\right)$.
• Let $A,B\in {\mathsf{H}}_{\mathbb{I}}^{\mathbb{F}}\left(\mathcal{F}\right)$. Then, $X\backslash A,X\backslash B\in \mathcal{F}$. Since $\mathcal{F}$ is a filter, $(X\backslash A)\cap (X\backslash B)=X\backslash A\cup B\in \mathcal{F}$. So, $A\cup B\in {\mathsf{H}}_{\mathbb{I}}^{\mathbb{F}}\left(\mathcal{F}\right)$. That means ${\mathsf{H}}_{\mathbb{I}}^{\mathbb{F}}\left(\mathcal{F}\right)$ is an ideal.

We prove that ${\mathsf{H}}_{\mathbb{F}}^{\mathbb{I}}\left(\mathcal{I}\right)$ is a filter.

• Since $X\notin \mathcal{I}$, $\varnothing \notin {\mathsf{H}}_{\mathbb{F}}^{\mathbb{I}}\left(\mathcal{I}\right)$.
• Let $A\in {\mathsf{H}}_{\mathbb{F}}^{\mathbb{I}}\left(\mathcal{I}\right)$ and $A\subset B$. Then, $X\backslash A\in \mathcal{I}$. Since $X\backslash B\subset X\backslash A$ and $\mathcal{I}$ is an ideal, $X\backslash B\in \mathcal{I}$, so $B\in {\mathsf{H}}_{\mathbb{F}}^{\mathbb{I}}\left(\mathcal{I}\right)$.
• Let $A,B\in {\mathsf{H}}_{\mathbb{F}}^{\mathbb{I}}\left(\mathcal{I}\right)$. Then, $X\backslash A,X\backslash B\in \mathcal{I}$. Since $\mathcal{I}$ is an ideal, $(X\backslash A)\cup (X\backslash B)=X\backslash A\cap B\in \mathcal{I}$. So, $A\cap B\in {\mathsf{H}}_{\mathbb{F}}^{\mathbb{I}}\left(\mathcal{I}\right)$. That means ${\mathsf{H}}_{\mathbb{F}}^{\mathbb{I}}\left(\mathcal{I}\right)$ is a filter.

(11): $x\in A_{{\mathsf{H}}_{\mathbb{I}}^{\mathbb{F}}\left(\mathcal{F}\right)}^{*}$ if and only if $A\cap U\notin {\mathsf{H}}_{\mathbb{I}}^{\mathbb{F}}\left(\mathcal{F}\right)$ for any neighborhood U of x. Then, the last holds if and only if $X\backslash A\cap U\notin \mathcal{F}$. Finally, the last holds if and only if $x\in A_{\mathcal{F}}^{*}$.

(12): $x\in A_{{\mathsf{H}}_{\mathbb{F}}^{\mathbb{I}}\left(\mathcal{I}\right)}^{*}$ if and only if $X\backslash A\cap U\notin {\mathsf{H}}_{\mathbb{F}}^{\mathbb{I}}\left(\mathcal{I}\right)$ for any neighborhood U of x. Then, the last holds if and only if $A\cap U\notin \mathcal{I}$. Finally, the last holds if and only if $x\in A_{\mathcal{I}}^{*}$.  □

Example 1. 

In this example, we give a few ideals and the equivalent systems.

(1)

${\mathcal{I}}_0=\{\varnothing \}$

(2)

${\mathcal{I}}_f$, the ideal of all finite subsets of X (X-infinite)

(3)

${\mathcal{I}}_c$, the ideal of all countable subsets of X (X-uncountable)

(4)

${\mathcal{I}}_a=\{A\subset X:a\notin A\}$, an excluded point ideal on X ($a\in X$)

(5)

${\mathcal{I}}_{nd}$, the family of all nowhere dense subsets of $(X,\tau )$. Recall the set A is nowhere dense, if $int\left(cl\right(A\left)\right)=\varnothing$. A set which is not nowhere dense is called somewhere dense. In other words, A is somewhere dense if there exists a non-empty open set G such that $G\subset cl\left(A\right)$.

The equivalent systems:

(1)

${\mathsf{H}}_{\mathbb{P}}^{\mathbb{I}}\left({\mathcal{I}}_0\right)$, the primal of all proper subsets of X

${\mathsf{H}}_{\mathbb{G}}^{\mathbb{I}}\left({\mathcal{I}}_0\right)$, the grill of all non-empty subsets of X

${\mathsf{H}}_{\mathbb{F}}^{\mathbb{I}}\left({\mathcal{I}}_0\right)=\left\{X\right\}$

(2)

${\mathsf{H}}_{\mathbb{P}}^{\mathbb{I}}\left({\mathcal{I}}_f\right)=\{A\subset X:X\backslash A$ is infinite}-primal

${\mathsf{H}}_{\mathbb{G}}^{\mathbb{I}}\left({\mathcal{I}}_f\right)=\{A\subset X:A$ is infinite}-grill

${\mathsf{H}}_{\mathbb{F}}^{\mathbb{I}}\left({\mathcal{I}}_f\right)=\{A\subset X:X\backslash A$ is finite}-filter of all co-finite sets

(3)

${\mathsf{H}}_{\mathbb{P}}^{\mathbb{I}}\left({\mathcal{I}}_c\right)=\{A\subset X:X\backslash A$ is uncountable}-primal

${\mathsf{H}}_{\mathbb{G}}^{\mathbb{I}}\left({\mathcal{I}}_c\right)=\{A\subset X:A$ is uncountable}-grill

${\mathsf{H}}_{\mathbb{F}}^{\mathbb{I}}\left({\mathcal{I}}_c\right)=\{A\subset X:X\backslash A$ is countable}-filter of all co-countable sets

(4)

${\mathsf{H}}_{\mathbb{P}}^{\mathbb{I}}\left({\mathcal{I}}_a\right)=\{A\subset X:a\notin A\}={\mathcal{I}}_a$-primal

${\mathsf{H}}_{\mathbb{G}}^{\mathbb{I}}\left({\mathcal{I}}_a\right)=\{A\subset X:a\in A$}-grill

${\mathsf{H}}_{\mathbb{F}}^{\mathbb{I}}\left({\mathcal{I}}_a\right)=\{A\subset X:a\in A$}-filter

(5)

${\mathsf{H}}_{\mathbb{P}}^{\mathbb{I}}\left({\mathcal{I}}_{nd}\right)=\{A\subset X:X\backslash A$ is somewhere dense}-primal

${\mathsf{H}}_{\mathbb{G}}^{\mathbb{I}}\left({\mathcal{I}}_{nd}\right)=\{A\subset X:A$ is somewhere dense}-grill

${\mathsf{H}}_{\mathbb{F}}^{\mathbb{I}}\left({\mathcal{I}}_{nd}\right)=\{A\subset X:X\backslash A$ is nowhere dense}-filter

Proposition 1. 

Let ${\mathbb{Z}}_1,{\mathbb{Z}}_2\in \{\mathbb{I},\mathbb{P},\mathbb{F},\mathbb{G}\}$. If ${\mathbb{Z}}_1\ne {\mathbb{Z}}_2$, then each of the twelve operators ${\mathsf{H}}_{{\mathbb{Z}}_2}^{{\mathbb{Z}}_1}$ from Theorems 1–6 is bijective and ${\left({\mathsf{H}}_{{\mathbb{Z}}_2}^{{\mathbb{Z}}_1}\right)}^{-1}={\mathsf{H}}_{{\mathbb{Z}}_1}^{{\mathbb{Z}}_2}$. Consequently, ${\mathsf{H}}_{{\mathbb{Z}}_2}^{{\mathbb{Z}}_1}$ and ${\mathsf{H}}_{{\mathbb{Z}}_1}^{{\mathbb{Z}}_2}$ are mutually inverse, so ${\mathsf{H}}_{{\mathbb{Z}}_1}^{{\mathbb{Z}}_2}\circ {\mathsf{H}}_{{\mathbb{Z}}_2}^{{\mathbb{Z}}_1}={\mathsf{H}}_{{\mathbb{Z}}_1}^{{\mathbb{Z}}_1}$.

Proof. 

Proposition 1 follows from items (9) and (10) of Theorems 1–6.  □

Proposition 2. 

${\mathsf{H}}_{\mathbb{F}}^{\mathbb{P}}\circ {\mathsf{H}}_{\mathbb{P}}^{\mathbb{I}}={\mathsf{H}}_{\mathbb{F}}^{\mathbb{I}}$, ${\mathsf{H}}_{\mathbb{I}}^{\mathbb{G}}\circ {\mathsf{H}}_{\mathbb{G}}^{\mathbb{F}}={\mathsf{H}}_{\mathbb{I}}^{\mathbb{F}}$, ${\mathsf{H}}_{\mathbb{G}}^{\mathbb{F}}\circ {\mathsf{H}}_{\mathbb{F}}^{\mathbb{P}}={\mathsf{H}}_{\mathbb{G}}^{\mathbb{P}}$, ${\mathsf{H}}_{\mathbb{P}}^{\mathbb{I}}\circ {\mathsf{H}}_{\mathbb{I}}^{\mathbb{G}}={\mathsf{H}}_{\mathbb{P}}^{\mathbb{G}}$.

Proof. 

$A\in {\mathsf{H}}_{\mathbb{F}}^{\mathbb{P}}\left({\mathsf{H}}_{\mathbb{P}}^{\mathbb{I}}\left(\mathcal{I}\right)\right)$, and ${\mathsf{H}}_{\mathbb{I}}^{\mathbb{G}}\left({\mathsf{H}}_{\mathbb{G}}^{\mathbb{F}}\left(\mathcal{F}\right)\right)\iff$$A\notin {\mathsf{H}}_{\mathbb{P}}^{\mathbb{I}}\left(\mathcal{I}\right)$, and ${\mathsf{H}}_{\mathbb{G}}^{\mathbb{F}}\left(\mathcal{F}\right)$⇔$X\backslash A\in \mathcal{I}$, and $\mathcal{F}\iff A\in {\mathsf{H}}_{\mathbb{F}}^{\mathbb{I}}\left(\mathcal{I}\right)$, and ${\mathsf{H}}_{\mathbb{I}}^{\mathbb{F}}\left(\mathcal{F}\right)$, respectively. So, ${\mathsf{H}}_{\mathbb{F}}^{\mathbb{P}}\circ {\mathsf{H}}_{\mathbb{P}}^{\mathbb{I}}={\mathsf{H}}_{\mathbb{F}}^{\mathbb{I}}$, ${\mathsf{H}}_{\mathbb{I}}^{\mathbb{G}}\circ {\mathsf{H}}_{\mathbb{G}}^{\mathbb{F}}={\mathsf{H}}_{\mathbb{I}}^{\mathbb{F}}$.

$A\in {\mathsf{H}}_{\mathbb{G}}^{\mathbb{F}}\left({\mathsf{H}}_{\mathbb{F}}^{\mathbb{P}}\left(\mathcal{P}\right)\right)$, and ${\mathsf{H}}_{\mathbb{P}}^{\mathbb{I}}\left({\mathsf{H}}_{\mathbb{I}}^{\mathbb{G}}\left(\mathcal{G}\right)\right)\iff$$X\backslash A\notin {\mathsf{H}}_{\mathbb{F}}^{\mathbb{P}}\left(\mathcal{P}\right)$, and ${\mathsf{H}}_{\mathbb{I}}^{\mathbb{G}}\left(\mathcal{G}\right)\iff$$X\backslash A\in \mathcal{P}$, and $\mathcal{G}\iff$$A\in {\mathsf{H}}_{\mathbb{G}}^{\mathbb{P}}\left(\mathcal{P}\right)$, and ${\mathsf{H}}_{\mathbb{P}}^{\mathbb{G}}\left(\mathcal{G}\right)$, respectively. So, ${\mathsf{H}}_{\mathbb{G}}^{\mathbb{F}}\circ {\mathsf{H}}_{\mathbb{F}}^{\mathbb{P}}={\mathsf{H}}_{\mathbb{G}}^{\mathbb{P}}$, ${\mathsf{H}}_{\mathbb{P}}^{\mathbb{I}}\circ {\mathsf{H}}_{\mathbb{I}}^{\mathbb{G}}={\mathsf{H}}_{\mathbb{P}}^{\mathbb{G}}$.  □

Proposition 3. 

Let $\mathbb{Z},{\mathbb{Z}}_1,{\mathbb{Z}}_2\in \{\mathbb{I},\mathbb{P},\mathbb{F},\mathbb{G}\}$. The following conditions are equivalent:

• (1) ${\mathsf{H}}_{{\mathbb{Z}}_2}^{\mathbb{Z}}\circ {\mathsf{H}}_{\mathbb{Z}}^{{\mathbb{Z}}_1}={\mathsf{H}}_{{\mathbb{Z}}_2}^{{\mathbb{Z}}_1}$  (2) ${\mathsf{H}}_{{\mathbb{Z}}_1}^{\mathbb{Z}}\circ {\mathsf{H}}_{\mathbb{Z}}^{{\mathbb{Z}}_2}={\mathsf{H}}_{{\mathbb{Z}}_1}^{{\mathbb{Z}}_2}$
• (3) ${\mathsf{H}}_{\mathbb{Z}}^{{\mathbb{Z}}_2}\circ {\mathsf{H}}_{{\mathbb{Z}}_2}^{{\mathbb{Z}}_1}={\mathsf{H}}_{\mathbb{Z}}^{{\mathbb{Z}}_1}$  (4) ${\mathsf{H}}_{{\mathbb{Z}}_1}^{{\mathbb{Z}}_2}\circ {\mathsf{H}}_{{\mathbb{Z}}_2}^{\mathbb{Z}}={\mathsf{H}}_{{\mathbb{Z}}_1}^{\mathbb{Z}}$
• (5) ${\mathsf{H}}_{{\mathbb{Z}}_2}^{{\mathbb{Z}}_1}\circ {\mathsf{H}}_{{\mathbb{Z}}_1}^{\mathbb{Z}}={\mathsf{H}}_{{\mathbb{Z}}_2}^{\mathbb{Z}}$  (6) ${\mathsf{H}}_{\mathbb{Z}}^{{\mathbb{Z}}_1}\circ {\mathsf{H}}_{{\mathbb{Z}}_1}^{{\mathbb{Z}}_2}={\mathsf{H}}_{\mathbb{Z}}^{{\mathbb{Z}}_2}$

Proof. 

$\left(1\right)\iff \left(2\right)$:

${\mathsf{H}}_{{\mathbb{Z}}_2}^{\mathbb{Z}}\circ {\mathsf{H}}_{\mathbb{Z}}^{{\mathbb{Z}}_1}={\mathsf{H}}_{{\mathbb{Z}}_2}^{{\mathbb{Z}}_1}$⇔${({\mathsf{H}}_{{\mathbb{Z}}_2}^{\mathbb{Z}}\circ {\mathsf{H}}_{\mathbb{Z}}^{{\mathbb{Z}}_1})}^{-1}={\left({\mathsf{H}}_{{\mathbb{Z}}_2}^{{\mathbb{Z}}_1}\right)}^{-1}$⇔${\mathsf{H}}_{{\mathbb{Z}}_1}^{\mathbb{Z}}\circ {\mathsf{H}}_{\mathbb{Z}}^{{\mathbb{Z}}_2}={\mathsf{H}}_{{\mathbb{Z}}_1}^{{\mathbb{Z}}_2}$

$\left(1\right)\iff \left(3\right)$:

${\mathsf{H}}_{{\mathbb{Z}}_2}^{\mathbb{Z}}\circ {\mathsf{H}}_{\mathbb{Z}}^{{\mathbb{Z}}_1}={\mathsf{H}}_{{\mathbb{Z}}_2}^{{\mathbb{Z}}_1}$⇔${\mathsf{H}}_{\mathbb{Z}}^{{\mathbb{Z}}_1}={\left({\mathsf{H}}_{{\mathbb{Z}}_2}^{\mathbb{Z}}\right)}^{-1}\circ {\mathsf{H}}_{{\mathbb{Z}}_2}^{{\mathbb{Z}}_1}\iff {\mathsf{H}}_{\mathbb{Z}}^{{\mathbb{Z}}_1}={\mathsf{H}}_{\mathbb{Z}}^{{\mathbb{Z}}_2}\circ {\mathsf{H}}_{{\mathbb{Z}}_2}^{{\mathbb{Z}}_1}$

$\left(3\right)\iff \left(4\right)$:

${\mathsf{H}}_{\mathbb{Z}}^{{\mathbb{Z}}_2}\circ {\mathsf{H}}_{{\mathbb{Z}}_2}^{{\mathbb{Z}}_1}={\mathsf{H}}_{\mathbb{Z}}^{{\mathbb{Z}}_1}$⇔${({\mathsf{H}}_{\mathbb{Z}}^{{\mathbb{Z}}_2}\circ {\mathsf{H}}_{{\mathbb{Z}}_2}^{{\mathbb{Z}}_1})}^{-1}={\left({\mathsf{H}}_{\mathbb{Z}}^{{\mathbb{Z}}_1}\right)}^{-1}$⇔${\mathsf{H}}_{{\mathbb{Z}}_1}^{{\mathbb{Z}}_2}\circ {\mathsf{H}}_{{\mathbb{Z}}_2}^{\mathbb{Z}}={\mathsf{H}}_{{\mathbb{Z}}_1}^{\mathbb{Z}}$

$\left(1\right)\iff \left(5\right)$:

${\mathsf{H}}_{{\mathbb{Z}}_2}^{\mathbb{Z}}\circ {\mathsf{H}}_{\mathbb{Z}}^{{\mathbb{Z}}_1}={\mathsf{H}}_{{\mathbb{Z}}_2}^{{\mathbb{Z}}_1}$⇔${\mathsf{H}}_{{\mathbb{Z}}_2}^{\mathbb{Z}}={\mathsf{H}}_{{\mathbb{Z}}_2}^{{\mathbb{Z}}_1}\circ {\left({\mathsf{H}}_{\mathbb{Z}}^{{\mathbb{Z}}_1}\right)}^{-1}\iff {\mathsf{H}}_{{\mathbb{Z}}_2}^{\mathbb{Z}}={\mathsf{H}}_{{\mathbb{Z}}_2}^{{\mathbb{Z}}_1}\circ {\mathsf{H}}_{{\mathbb{Z}}_1}^{\mathbb{Z}}$

$\left(5\right)\iff \left(6\right)$:

${\mathsf{H}}_{{\mathbb{Z}}_2}^{{\mathbb{Z}}_1}\circ {\mathsf{H}}_{{\mathbb{Z}}_1}^{\mathbb{Z}}={\mathsf{H}}_{{\mathbb{Z}}_2}^{\mathbb{Z}}$⇔${({\mathsf{H}}_{{\mathbb{Z}}_2}^{{\mathbb{Z}}_1}\circ {\mathsf{H}}_{{\mathbb{Z}}_1}^{\mathbb{Z}})}^{-1}={\left({\mathsf{H}}_{{\mathbb{Z}}_2}^{\mathbb{Z}}\right)}^{-1}$⇔${\mathsf{H}}_{\mathbb{Z}}^{{\mathbb{Z}}_1}\circ {\mathsf{H}}_{{\mathbb{Z}}_1}^{{\mathbb{Z}}_2}={\mathsf{H}}_{\mathbb{Z}}^{{\mathbb{Z}}_2}$□

Proposition 4. 

Let $\mathbb{Z},{\mathbb{Z}}_1,{\mathbb{Z}}_2\in \{\mathbb{I},\mathbb{P},\mathbb{F},\mathbb{G}\}$. Then, for 64 possibilities, the equation ${\mathsf{H}}_{{\mathbb{Z}}_2}^{\mathbb{Z}}\circ {\mathsf{H}}_{\mathbb{Z}}^{{\mathbb{Z}}_1}={\mathsf{H}}_{{\mathbb{Z}}_2}^{{\mathbb{Z}}_1}$ holds.

Proof. 

For Propositions 2 and 3, the equation ${\mathsf{H}}_{{\mathbb{Z}}_2}^{\mathbb{Z}}\circ {\mathsf{H}}_{\mathbb{Z}}^{{\mathbb{Z}}_1}={\mathsf{H}}_{{\mathbb{Z}}_2}^{{\mathbb{Z}}_1}$ holds for 24 possibilities ($\mathbb{Z},{\mathbb{Z}}_1$ and ${\mathbb{Z}}_2$ are mutually different):

$$\begin{gathered} {\mathsf{H}}_{\mathbb{F}}^{\mathbb{P}}\circ {\mathsf{H}}_{\mathbb{P}}^{\mathbb{I}}={\mathsf{H}}_{\mathbb{F}}^{\mathbb{I}}{\mathsf{H}}_{\mathbb{I}}^{\mathbb{G}}\circ {\mathsf{H}}_{\mathbb{G}}^{\mathbb{F}}={\mathsf{H}}_{\mathbb{I}}^{\mathbb{F}}{\mathsf{H}}_{\mathbb{G}}^{\mathbb{F}}\circ {\mathsf{H}}_{\mathbb{F}}^{\mathbb{P}}={\mathsf{H}}_{\mathbb{G}}^{\mathbb{P}}{\mathsf{H}}_{\mathbb{P}}^{\mathbb{I}}\circ {\mathsf{H}}_{\mathbb{I}}^{\mathbb{G}}={\mathsf{H}}_{\mathbb{P}}^{\mathbb{G}} \\ {\mathsf{H}}_{\mathbb{I}}^{\mathbb{P}}\circ {\mathsf{H}}_{\mathbb{P}}^{\mathbb{F}}={\mathsf{H}}_{\mathbb{I}}^{\mathbb{F}}{\mathsf{H}}_{\mathbb{F}}^{\mathbb{G}}\circ {\mathsf{H}}_{\mathbb{G}}^{\mathbb{I}}={\mathsf{H}}_{\mathbb{F}}^{\mathbb{I}}{\mathsf{H}}_{\mathbb{P}}^{\mathbb{F}}\circ {\mathsf{H}}_{\mathbb{F}}^{\mathbb{G}}={\mathsf{H}}_{\mathbb{P}}^{\mathbb{G}}{\mathsf{H}}_{\mathbb{G}}^{\mathbb{I}}\circ {\mathsf{H}}_{\mathbb{I}}^{\mathbb{P}}={\mathsf{H}}_{\mathbb{G}}^{\mathbb{P}} \\ {\mathsf{H}}_{\mathbb{P}}^{\mathbb{F}}\circ {\mathsf{H}}_{\mathbb{F}}^{\mathbb{I}}={\mathsf{H}}_{\mathbb{P}}^{\mathbb{I}}{\mathsf{H}}_{\mathbb{G}}^{\mathbb{I}}\circ {\mathsf{H}}_{\mathbb{I}}^{\mathbb{F}}={\mathsf{H}}_{\mathbb{G}}^{\mathbb{F}}{\mathsf{H}}_{\mathbb{F}}^{\mathbb{G}}\circ {\mathsf{H}}_{\mathbb{G}}^{\mathbb{P}}={\mathsf{H}}_{\mathbb{F}}^{\mathbb{P}}{\mathsf{H}}_{\mathbb{I}}^{\mathbb{P}}\circ {\mathsf{H}}_{\mathbb{P}}^{\mathbb{G}}={\mathsf{H}}_{\mathbb{I}}^{\mathbb{G}} \\ {\mathsf{H}}_{\mathbb{I}}^{\mathbb{F}}\circ {\mathsf{H}}_{\mathbb{F}}^{\mathbb{P}}={\mathsf{H}}_{\mathbb{I}}^{\mathbb{P}}{\mathsf{H}}_{\mathbb{F}}^{\mathbb{I}}\circ {\mathsf{H}}_{\mathbb{I}}^{\mathbb{G}}={\mathsf{H}}_{\mathbb{F}}^{\mathbb{G}}{\mathsf{H}}_{\mathbb{P}}^{\mathbb{G}}\circ {\mathsf{H}}_{\mathbb{G}}^{\mathbb{F}}={\mathsf{H}}_{\mathbb{P}}^{\mathbb{F}}{\mathsf{H}}_{\mathbb{G}}^{\mathbb{P}}\circ {\mathsf{H}}_{\mathbb{P}}^{\mathbb{I}}={\mathsf{H}}_{\mathbb{G}}^{\mathbb{I}} \\ {\mathsf{H}}_{\mathbb{F}}^{\mathbb{I}}\circ {\mathsf{H}}_{\mathbb{I}}^{\mathbb{P}}={\mathsf{H}}_{\mathbb{F}}^{\mathbb{P}}{\mathsf{H}}_{\mathbb{I}}^{\mathbb{F}}\circ {\mathsf{H}}_{\mathbb{F}}^{\mathbb{G}}={\mathsf{H}}_{\mathbb{I}}^{\mathbb{G}}{\mathsf{H}}_{\mathbb{G}}^{\mathbb{P}}\circ {\mathsf{H}}_{\mathbb{P}}^{\mathbb{F}}={\mathsf{H}}_{\mathbb{G}}^{\mathbb{F}}{\mathsf{H}}_{\mathbb{P}}^{\mathbb{G}}\circ {\mathsf{H}}_{\mathbb{G}}^{\mathbb{I}}={\mathsf{H}}_{\mathbb{P}}^{\mathbb{I}} \\ {\mathsf{H}}_{\mathbb{P}}^{\mathbb{I}}\circ {\mathsf{H}}_{\mathbb{I}}^{\mathbb{F}}={\mathsf{H}}_{\mathbb{P}}^{\mathbb{F}}{\mathsf{H}}_{\mathbb{G}}^{\mathbb{F}}\circ {\mathsf{H}}_{\mathbb{F}}^{\mathbb{I}}={\mathsf{H}}_{\mathbb{G}}^{\mathbb{I}}{\mathsf{H}}_{\mathbb{F}}^{\mathbb{P}}\circ {\mathsf{H}}_{\mathbb{P}}^{\mathbb{G}}={\mathsf{H}}_{\mathbb{F}}^{\mathbb{G}}{\mathsf{H}}_{\mathbb{I}}^{\mathbb{G}}\circ {\mathsf{H}}_{\mathbb{G}}^{\mathbb{P}}={\mathsf{H}}_{\mathbb{I}}^{\mathbb{P}} \end{gathered}$$

Other cases for ${\mathbb{Z}}_1\ne {\mathbb{Z}}_2$ are trivial:

${\mathsf{H}}_{{\mathbb{Z}}_1}^{{\mathbb{Z}}_1}\circ {\mathsf{H}}_{{\mathbb{Z}}_1}^{{\mathbb{Z}}_2}={\mathsf{H}}_{{\mathbb{Z}}_1}^{{\mathbb{Z}}_2}$ (12 possibilities), ${\mathsf{H}}_{{\mathbb{Z}}_2}^{{\mathbb{Z}}_1}\circ {\mathsf{H}}_{{\mathbb{Z}}_1}^{{\mathbb{Z}}_1}={\mathsf{H}}_{{\mathbb{Z}}_2}^{{\mathbb{Z}}_1}$ (12 possibilities),

${\mathsf{H}}_{{\mathbb{Z}}_1}^{{\mathbb{Z}}_2}\circ {\mathsf{H}}_{{\mathbb{Z}}_2}^{{\mathbb{Z}}_1}={\mathsf{H}}_{{\mathbb{Z}}_1}^{{\mathbb{Z}}_1}$ (12 possibilities), ${\mathsf{H}}_{{\mathbb{Z}}_1}^{{\mathbb{Z}}_1}\circ {\mathsf{H}}_{{\mathbb{Z}}_1}^{{\mathbb{Z}}_1}={\mathsf{H}}_{{\mathbb{Z}}_1}^{{\mathbb{Z}}_1}$ (4 possibilities).

□

For a composition of finitely many operators, the domain (co-domain) of the resulting operator is equal to the domain (co-domain) of the first (last) operator and the value of the local function is independent of the operators, as the following theorem states.

Theorem 7. 

Let ${\mathbb{Z}}_k\in \{\mathbb{I},\mathbb{P},\mathbb{F},\mathbb{G}\}$, $k=1,\cdots ,n$, and $\mathcal{Z}\in {\mathbb{Z}}_1$. Then,

$${\mathsf{H}}_{{\mathbb{Z}}_n}^{{\mathbb{Z}}_{n-1}}\circ {\mathsf{H}}_{{\mathbb{Z}}_{n-1}}^{{\mathbb{Z}}_{n-2}}\circ \cdots \circ {\mathsf{H}}_{{\mathbb{Z}}_3}^{{\mathbb{Z}}_2}\circ {\mathsf{H}}_{{\mathbb{Z}}_2}^{{\mathbb{Z}}_1}={\mathsf{H}}_{{\mathbb{Z}}_n}^{{\mathbb{Z}}_1}$$

$$A_{\left({\mathsf{H}}_{{\mathbb{Z}}_n}^{{\mathbb{Z}}_{n-1}}\circ {\mathsf{H}}_{{\mathbb{Z}}_{n-1}}^{{\mathbb{Z}}_{n-2}}\circ \cdots \circ {\mathsf{H}}_{{\mathbb{Z}}_3}^{{\mathbb{Z}}_2}\circ {\mathsf{H}}_{{\mathbb{Z}}_2}^{{\mathbb{Z}}_1}\right)\left(\mathcal{Z}\right)}^{*}=A_{{\mathsf{H}}_{{\mathbb{Z}}_n}^{{\mathbb{Z}}_1}\left(\mathcal{Z}\right)}^{*}=A_{\mathcal{Z}}^{*}$$

Consequently,

(1)

$A_{\mathcal{I}}^{*}=A_{{\mathsf{H}}_{\mathbb{I}}^{\mathbb{I}}\left(\mathcal{I}\right)}^{*}=A_{{\mathsf{H}}_{\mathbb{P}}^{\mathbb{I}}\left(\mathcal{I}\right)}^{*}=A_{{\mathsf{H}}_{\mathbb{G}}^{\mathbb{I}}\left(\mathcal{I}\right)}^{*}=A_{{\mathsf{H}}_{\mathbb{F}}^{\mathbb{I}}\left(\mathcal{I}\right)}^{*}$

(2)

$A_{\mathcal{P}}^{*}=A_{{\mathsf{H}}_{\mathbb{P}}^{\mathbb{P}}\left(\mathcal{P}\right)}^{*}=A_{{\mathsf{H}}_{\mathbb{I}}^{\mathbb{P}}\left(\mathcal{P}\right)}^{*}=A_{{\mathsf{H}}_{\mathbb{F}}^{\mathbb{P}}\left(\mathcal{P}\right)}^{*}=A_{{\mathsf{H}}_{\mathbb{G}}^{\mathbb{P}}\left(\mathcal{P}\right)}^{*}$

(3)

$A_{\mathcal{F}}^{*}=A_{{\mathsf{H}}_{\mathbb{F}}^{\mathbb{F}}\left(\mathcal{F}\right)}^{*}=A_{{\mathsf{H}}_{\mathbb{G}}^{\mathbb{F}}\left(\mathcal{F}\right)}^{*}=A_{{\mathsf{H}}_{\mathbb{P}}^{\mathbb{F}}\left(\mathcal{F}\right)}^{*}=A_{{\mathsf{H}}_{\mathbb{I}}^{\mathbb{F}}\left(\mathcal{F}\right)}^{*}$

(4)

$A_{\mathcal{G}}^{*}=A_{{\mathsf{H}}_{\mathbb{G}}^{\mathbb{G}}\left(\mathcal{G}\right)}^{*}=A_{{\mathsf{H}}_{\mathbb{I}}^{\mathbb{G}}\left(\mathcal{G}\right)}^{*}=A_{{\mathsf{H}}_{\mathbb{F}}^{\mathbb{G}}\left(\mathcal{G}\right)}^{*}=A_{{\mathsf{H}}_{\mathbb{P}}^{\mathbb{G}}\left(\mathcal{G}\right)}^{*}$

Proof. 

For the first equation, we use mathematical induction. If $n=3$, it follows from Proposition 4. Suppose the equation holds for $n>3$. Then,

${\mathsf{H}}_{{\mathbb{Z}}_{n+1}}^{{\mathbb{Z}}_n}\circ {\mathsf{H}}_{{\mathbb{Z}}_n}^{{\mathbb{Z}}_{n-1}}\circ {\mathsf{H}}_{{\mathbb{Z}}_{n-1}}^{{\mathbb{Z}}_{n-2}}\circ \cdots \circ {\mathsf{H}}_{{\mathbb{Z}}_3}^{{\mathbb{Z}}_2}\circ {\mathsf{H}}_{{\mathbb{Z}}_2}^{{\mathbb{Z}}_1}={\mathsf{H}}_{{\mathbb{Z}}_{n+1}}^{{\mathbb{Z}}_n}\circ {\mathsf{H}}_{{\mathbb{Z}}_n}^{{\mathbb{Z}}_1}={\mathsf{H}}_{{\mathbb{Z}}_{n+1}}^{{\mathbb{Z}}_1}$, as per Proposition 4. The second equation follows from the first one and from Theorems 1–6.  □

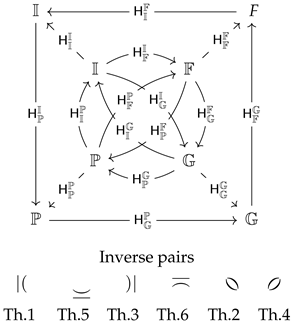
The set $\{{\mathsf{H}}_{{\mathbb{Z}}_2}^{{\mathbb{Z}}_1}:{\mathbb{Z}}_1,{\mathbb{Z}}_2\in \{\mathbb{I},\mathbb{P},\mathbb{F},\mathbb{G}\}\}$ consisting of 16 operators is enclosed under the composition. The results are interpreted by the next diagram.

3. Applications

We have defined 16 operators, which can be expressed by one notation ${\mathsf{H}}_{{\mathbb{Z}}_2}^{{\mathbb{Z}}_1}$, where ${\mathbb{Z}}_1,{\mathbb{Z}}_2\in \{\mathbb{I},\mathbb{P},\mathbb{F},\mathbb{G}\}$. Between the members of ${\mathbb{Z}}_1$ and the members of ${\mathbb{Z}}_2$, we can define an equivalence as the next definition states. Note that if $\mathcal{Z}\in \mathbb{X}$, then $\mathcal{Z}\in \mathbb{Z}$ for some $\mathbb{Z}\in \{\mathbb{I},\mathbb{P},\mathbb{F},\mathbb{G}\}$.

Definition 2. 

Let ${\mathcal{Z}}_1,{\mathcal{Z}}_2\in \mathbb{X}$. ${\mathcal{Z}}_1$ is equivalent to ${\mathcal{Z}}_2$ if ${\mathsf{H}}_{{\mathbb{Z}}_2}^{{\mathbb{Z}}_1}\left({\mathcal{Z}}_1\right)={\mathcal{Z}}_2$ and ${\mathsf{H}}_{{\mathbb{Z}}_1}^{{\mathbb{Z}}_2}\left({\mathcal{Z}}_2\right)={\mathcal{Z}}_1$, where ${\mathcal{Z}}_1\in {\mathbb{Z}}_1$, ${\mathcal{Z}}_2\in {\mathbb{Z}}_2$ and ${\mathbb{Z}}_1,{\mathbb{Z}}_2\in \{\mathbb{I},\mathbb{P},\mathbb{F},\mathbb{G}\}$. This relation is denoted by ${\mathcal{Z}}_1\sim {\mathcal{Z}}_2$.

Lemma 1. 

For any $\mathcal{Z}\in \mathbb{X}$, $\mathcal{Z}\sim {\mathsf{H}}_{{\mathbb{Z}}_2}^{{\mathbb{Z}}_1}\left(\mathcal{Z}\right)$, where $\mathcal{Z}\in {\mathbb{Z}}_1$ and ${\mathbb{Z}}_1,{\mathbb{Z}}_2\in \{\mathbb{I},\mathbb{P},\mathbb{F},\mathbb{G}\}$. Moreover, ∼ is an equivalence relation.

Proof. 

Since ${\mathsf{H}}_{{\mathbb{Z}}_2}^{{\mathbb{Z}}_1}\left(\mathcal{Z}\right)={\mathsf{H}}_{{\mathbb{Z}}_2}^{{\mathbb{Z}}_1}\left(\mathcal{Z}\right)$ and $\mathcal{Z}={\mathsf{H}}_{{\mathbb{Z}}_1}^{{\mathbb{Z}}_2}\left({\mathsf{H}}_{{\mathbb{Z}}_2}^{{\mathbb{Z}}_1}\left(\mathcal{Z}\right)\right)$, $\mathcal{Z}\sim {\mathsf{H}}_{{\mathbb{Z}}_2}^{{\mathbb{Z}}_1}\left(\mathcal{Z}\right)$. It is clear that ∼ is reflexive and symmetric. Let ${\mathcal{Z}}_1\sim {\mathcal{Z}}_2\sim {\mathcal{Z}}_3$. Then, ${\mathcal{Z}}_2={\mathsf{H}}_{{\mathbb{Z}}_2}^{{\mathbb{Z}}_1}\left({\mathcal{Z}}_1\right)$ and ${\mathcal{Z}}_3={\mathsf{H}}_{{\mathbb{Z}}_3}^{{\mathbb{Z}}_2}\left({\mathcal{Z}}_2\right)={\mathsf{H}}_{{\mathbb{Z}}_3}^{{\mathbb{Z}}_2}\left({\mathsf{H}}_{{\mathbb{Z}}_2}^{{\mathbb{Z}}_1}\left({\mathcal{Z}}_1\right)\right)={\mathsf{H}}_{{\mathbb{Z}}_3}^{{\mathbb{Z}}_1}\left({\mathcal{Z}}_1\right)$ and ${\mathcal{Z}}_1={\mathsf{H}}_{{\mathbb{Z}}_1}^{{\mathbb{Z}}_3}\left({\mathcal{Z}}_3\right)$, so ${\mathcal{Z}}_1\sim {\mathcal{Z}}_3$.  □

In the next section, we define a dual operator $A_{\mathcal{Z}}^{▷}$ (see Hamlett et al. [4]) with the operator $A_{\mathcal{Z}}^{*}$, a closure $c{l}_{\mathcal{Z}}(·)$ and an interior $in{t}_{\mathcal{Z}}(·)$ operator.

Definition 3. 

Let $\mathcal{Z}\in \mathbb{X}$. Define the operators

$A_{\mathcal{Z}}^{▷}=X\backslash {(X\backslash A)}_{\mathcal{Z}}^{*},$

$c{l}_{\mathcal{Z}}\left(A\right)=A\cup A_{\mathcal{Z}}^{*},$

$in{t}_{\mathcal{Z}}\left(A\right)=A\cap A_{\mathcal{Z}}^{▷}.$

Lemma 2. 

Let ${\mathcal{Z}}_1,{\mathcal{Z}}_2\in \mathbb{X}$. If ${\mathcal{Z}}_1\sim {\mathcal{Z}}_2$, then $A_{{\mathcal{Z}}_2}^{*}=A_{{\mathcal{Z}}_1}^{*}$, $A_{{\mathcal{Z}}_2}^{▷}=A_{{\mathcal{Z}}_1}^{▷}$, $c{l}_{{\mathcal{Z}}_2}\left(A\right)=c{l}_{{\mathcal{Z}}_1}\left(A\right)$, $in{t}_{{\mathcal{Z}}_2}\left(A\right)=in{t}_{{\mathcal{Z}}_1}\left(A\right)$. Consequently, if $\mathcal{I}\sim \mathcal{P}\sim \mathcal{F}\sim \mathcal{G}$, then $A_{\mathcal{I}}^{*}=A_{\mathcal{P}}^{*}=A_{\mathcal{F}}^{*}=A_{\mathcal{G}}^{*}$.

Proof. 

Suppose ${\mathcal{Z}}_1\sim {\mathcal{Z}}_2$. Then, ${\mathsf{H}}_{{\mathbb{Z}}_2}^{{\mathbb{Z}}_1}\left({\mathcal{Z}}_1\right)={\mathcal{Z}}_2$. As per Theorem 7, $A_{{\mathcal{Z}}_2}^{*}=A_{{\mathsf{H}}_{{\mathbb{Z}}_2}^{{\mathbb{Z}}_1}\left({\mathcal{Z}}_1\right)}^{*}=A_{{\mathcal{Z}}_1}^{*}$. The other equalities are obvious.  □

Remark 1. 

It is well known (see, for example, Jankovic and Hamlett [5]) that if an ideal topology ${\tau }_{\mathcal{I}}$, derived from a topology τ on a set X and an ideal $\mathcal{I}$ on X, is defined by a Kuratowski closure operator $c{l}_{\mathcal{I}}\left(A\right)=A\cup A_{\mathcal{I}}^{*}$, then ${\tau }_{\mathcal{I}}$ is finer then τ. A base for ${\tau }_{\mathcal{I}}$ is described as $\beta (\tau ,\mathcal{I})=\{G\backslash A:G\in \tau ,A\in \mathcal{I}\}$ and ${\tau }_{\mathcal{I}}=\{A\subset X:c{l}_{\mathcal{I}}(X\backslash A)=X\backslash A\}$.

In the literature, we can find many properties of local functions. The designation of operators is different. For example, $A_{\mathcal{P}}^{*}=A_{\mathcal{P}}^{\diamond }$, $A_{\mathcal{P}}^{▷}=\Psi \left(A\right)$ in Al-Omari et al. [24], or $A_{\mathcal{G}}^{*}={\Phi }_{\mathcal{G}}\left(A\right)$, $A_{\mathcal{G}}^{▷}={\Gamma }_{\mathcal{G}}\left(A\right)$ in Roy et al. [18]. We will list some of them below regardless of what system $\mathcal{Z}\in \mathbb{Z}$ they apply to.

Theorem 8. 

Let $\mathcal{Z}\in \mathbb{X}$. Then,

(1) 

${\varnothing }_{\mathcal{Z}}^{*}=\varnothing$,

(2) 

If $A\subset B$, then $A_{\mathcal{Z}}^{*}\subset B_{\mathcal{Z}}^{*}$,

(3) 

${(A\cup B)}_{\mathcal{Z}}^{*}=A_{\mathcal{Z}}^{*}\cup B_{\mathcal{Z}}^{*}$,

(4) 

$A_{\mathcal{Z}}^{*}\backslash B_{\mathcal{Z}}^{*}={(A\backslash B)}_{\mathcal{Z}}^{*}\backslash B_{\mathcal{Z}}^{*}$,

(5) 

${\left(A_{\mathcal{Z}}^{*}\right)}_{\mathcal{Z}}^{*}\subset A_{\mathcal{Z}}^{*}$,

(6) 

If $A\subset B$, then $A_{\mathcal{Z}}^{▷}\subset B_{\mathcal{Z}}^{▷}$,

(7) 

$A_{\mathcal{Z}}^{▷}\subset {\left(A_{\mathcal{Z}}^{▷}\right)}_{\mathcal{Z}}^{▷}$,

(8) 

${(A\cap B)}_{\mathcal{Z}}^{▷}=A_{\mathcal{Z}}^{▷}\cap B_{\mathcal{Z}}^{▷}$.

Proof. 

Let $\mathcal{I}:={\mathsf{H}}_{\mathbb{I}}^{\mathbb{Z}}\left(\mathcal{Z}\right)$. Then $A_{\mathcal{I}}^{*}=A_{{\mathsf{H}}_{\mathbb{I}}^{\mathbb{Z}}\left(\mathcal{Z}\right)}^{*}=A_{\mathcal{Z}}^{*}$ as per Theorem 7. Since all items hold for $\mathcal{I}\in \mathbb{I}$ (see, Jankovic and Hamlett [5]), they hold for $\mathcal{Z}\in \mathbb{P}\cup \mathbb{F}\cup \mathbb{G}$ analogously.  □

Theorem 9. 

Let $\mathcal{Z}\in \mathbb{X}$. A family ${\tau }_{\mathcal{Z}}=\{A\subset X:c{l}_{\mathcal{Z}}(X\backslash A)=X\backslash A\}$ is a topology finer then τ and the next conditions are equivalent:

(1) 

$A\in {\tau }_{\mathcal{Z}}$,

(2) 

$A\subset A_{\mathcal{Z}}^{▷}\left(A\right)$,

(3) 

$in{t}_{\mathcal{Z}}\left(A\right)=A$,

(4) 

$c{l}_{\mathcal{Z}}(X\backslash A)\subset X\backslash A$,

(5) 

${(X\backslash A)}_{\mathcal{Z}}^{*}\subset X\backslash A$.

Proof. 

Since $c{l}_{\mathcal{Z}}\left(A\right)=A\cup A_{\mathcal{Z}}^{*}$ is a Kuratowski operator, ${\tau }_{\mathcal{Z}}$ is a topology.

Let $G\in \tau$. Then, $F:=X\backslash G$ is closed in $(X,\tau )$. Put $\mathcal{I}:={\mathsf{H}}_{\mathbb{I}}^{\mathbb{Z}}\left(\mathcal{Z}\right)$. Then, $F_{\mathcal{I}}^{*}=F_{{\mathsf{H}}_{\mathbb{I}}^{\mathbb{Z}}\left(\mathcal{Z}\right)}^{*}=F_{\mathcal{Z}}^{*}$ as per Theorem 7. Since F is closed, $F_{\mathcal{Z}}^{*}=F_{\mathcal{I}}^{*}\subset F$. That means $c{l}_{\mathcal{Z}}\left(F\right)=F\cup F_{\mathcal{Z}}^{*}=F$, so $cl(X\backslash G)=X\backslash G$. That means ${\tau }_{\mathcal{Z}}$ is finer then τ.

(1) ⇒ (2): $A\in {\tau }_{\mathcal{Z}}$⇒${(X\backslash A)}_{\mathcal{Z}}^{*}\subset X\backslash A$⇒ $X\backslash {(X\backslash A)}_{\mathcal{Z}}^{*}\supset A$⇒ $A^{▷}\supset A$

(2) ⇒ (3): $A\subset A_{\mathcal{Z}}^{▷}\left(A\right)$⇒ $A\cap A_{\mathcal{Z}}^{▷}=A$⇒$in{t}_{\mathcal{Z}}=A$

(3) ⇒ (4): $c{l}_{\mathcal{Z}}(X\backslash A)=(X\backslash A)\cup {(X\backslash A)}_{\mathcal{Z}}^{*}=(X\backslash A)\cup (X\backslash A_{\mathcal{Z}}^{▷})=X\backslash (A\cap A_{\mathcal{Z}}^{▷})=$

$X\backslash in{t}_{\mathcal{Z}}\left(A\right)=X\backslash A$⇒$c{l}_{\mathcal{Z}}(X\backslash A)\subset X\backslash A$

(4) ⇒ (5): $c{l}_{\mathcal{Z}}(X\backslash A)=(X\backslash A)\cup {(X\backslash A)}_{\mathcal{Z}}^{*}\subset X\backslash A$⇒ ${(X\backslash A)}_{\mathcal{Z}}^{*}\subset X\backslash A$

(5) ⇒ (1): $c{l}_{\mathcal{Z}}(X\backslash A)=(X\backslash A)\cup {(X\backslash A)}_{\mathcal{Z}}^{*}\subset X\backslash A$. Inclusion $c{l}_{\mathcal{Z}}(X\backslash A)\supset X\backslash A$

is clear. That means $c{l}_{\mathcal{Z}}(X\backslash A)=X\backslash A$, so $A\in {\tau }_{\mathcal{Z}}$.  □

Theorem 10. 

Let ${\mathcal{Z}}_1,{\mathcal{Z}}_2\in \mathbb{X}$. If ${\mathcal{Z}}_1\sim {\mathcal{Z}}_2$, then ${\tau }_{{\mathcal{Z}}_1}={\tau }_{{\mathcal{Z}}_2}$. Consequently, if $\mathcal{I}\sim \mathcal{P}\sim \mathcal{F}\sim \mathcal{G}$, then ${\tau }_{\mathcal{I}}={\tau }_{\mathcal{P}}={\tau }_{\mathcal{F}}={\tau }_{\mathcal{G}}$. A simple base for the open sets of ${\tau }_{\mathcal{I}},{\tau }_{\mathcal{P}},{\tau }_{\mathcal{F}}$ and ${\tau }_{\mathcal{G}}$ is described as follows:

$\beta (\tau ,\mathcal{I})=\{G\backslash A:G\in \tau ,A\in \mathcal{I}\}$,

$\beta (\tau ,\mathcal{P})=\{G\cap A:G\in \tau ,A\notin \mathcal{P}\}$,

$\beta (\tau ,\mathcal{F})=\{G\cap A:G\in \tau ,A\in \mathcal{F}\}$,

$\beta (\tau ,\mathcal{G})=\{G\backslash A:G\in \tau ,A\notin \mathcal{G}\}$, respectively.

Proof. 

The equality ${\tau }_{{\mathcal{Z}}_1}={\tau }_{{\mathcal{Z}}_2}$ follows from Lemma 2.

As per Remark 1, $\beta (\tau ,\mathcal{I})=\{G\backslash A:G\in \tau ,A\in \mathcal{I}\}$ is a base for ${\tau }_{\mathcal{I}}$. $H\in \beta (\tau ,\mathcal{I})$ if and only if $H=G\backslash A=G\cap (X\backslash A)$, where $G\in \tau$ and $A\in \mathcal{I}$ if and only if $H=G\cap B$, where $G\in \tau$ and $B:=X\backslash A\notin \mathcal{P}$ (as per Theorem 1 (4)), so $H\in \beta (\tau ,\mathcal{P})$ if and only if $H=G\cap B$, where $G\in \tau$ and $B\in \mathcal{F}$ (as per Theorem 4 (5)), so $H\in \beta (\tau ,\mathcal{F})$ if and only if $H=G\backslash A=G\backslash (X\backslash B)$, where $G\in \tau$ and $X\backslash B\notin \mathcal{G}$ (by Theorem 3 (4)), so $H\in \beta (\tau ,\mathcal{G})$.  □

Definition 4. 

A set A is $\mathcal{I}$-small ($\mathcal{P}$-small, $\mathcal{F}$-small, $\mathcal{G}$-small) if $A\in \mathcal{I}$ ($X\backslash A\notin \mathcal{P}$, $X\backslash A\in \mathcal{F}$, $A\notin \mathcal{G}$) and A is locally $\mathcal{I}$-small ($\mathcal{P}$-small, $\mathcal{F}$-small, $\mathcal{G}$-small) if for any $a\in A$ there is a set $U\in \tau$ containing a such that $A\cap U$ is $\mathcal{I}$-small ($\mathcal{P}$-small, $\mathcal{F}$-small, $\mathcal{G}$-small). Let $\mathcal{Z}\in \mathbb{X}$. A topology τ is said to be compatible with $\mathcal{Z}$ if any locally $\mathcal{Z}$-small set is $\mathcal{Z}$-small, denoted by $\mathcal{Z}\sim \tau$. Note the different meanings of the two designations. $\mathcal{Z}\sim \tau$ is a relation between a system from $\mathbb{X}$ and a topology. On the other hand, ${\mathcal{Z}}_1\sim {\mathcal{Z}}_2$ is a relation between two systems from $\mathbb{X}$ as per Definition 2.

Remark 2. 

Let $\mathcal{I}\sim \mathcal{P}\sim \mathcal{F}\sim \mathcal{G}$. Then, $\mathcal{I}={\mathsf{H}}_{\mathbb{I}}^{\mathbb{P}}\left(\mathcal{P}\right)$, $\mathcal{F}={\mathsf{H}}_{\mathbb{F}}^{\mathbb{P}}\left(\mathcal{P}\right)$ $\mathcal{G}={\mathsf{H}}_{\mathbb{G}}^{\mathbb{F}}\left(\mathcal{F}\right)$. So, $A\in \mathcal{I}\iff X\backslash A\notin \mathcal{P}\iff X\backslash A\in \mathcal{F}\iff A\notin \mathcal{G}$. That means if ${\mathcal{Z}}_1,{\mathcal{Z}}_2\in \mathbb{X}$ and ${\mathcal{Z}}_1\sim {\mathcal{Z}}_2$, then a set A is ${\mathcal{Z}}_1$-small (locally ${\mathcal{Z}}_1$-small) if and only if A is ${\mathcal{Z}}_2$-small (locally ${\mathcal{Z}}_2$-small) and ${\mathcal{Z}}_1\sim \tau$ if and only if ${\mathcal{Z}}_2\sim \tau$. Consequently,

(1) 

If $\mathcal{I}\sim \tau$, then ${\mathsf{H}}_{\mathbb{P}}^{\mathbb{I}}\left(\mathcal{I}\right)\sim \tau ,{\mathsf{H}}_{\mathbb{F}}^{\mathbb{I}}\left(\mathcal{I}\right)\sim \tau ,{\mathsf{H}}_{\mathbb{G}}^{\mathbb{I}}\left(\mathcal{I}\right)\sim \tau$.

(2) 

If $\mathcal{P}\sim \tau$, then ${\mathsf{H}}_{\mathbb{F}}^{\mathbb{P}}\left(\mathcal{P}\right)\sim \tau ,{\mathsf{H}}_{\mathbb{G}}^{\mathbb{P}}\left(\mathcal{P}\right)\sim \tau ,{\mathsf{H}}_{\mathbb{I}}^{\mathbb{P}}\left(\mathcal{P}\right)\sim \tau$.

(3) 

If $\mathcal{F}\sim \tau$, then ${\mathsf{H}}_{\mathbb{G}}^{\mathbb{F}}\left(\mathcal{F}\right)\sim \tau ,{\mathsf{H}}_{\mathbb{I}}^{\mathbb{F}}\left(\mathcal{F}\right)\sim \tau ,{\mathsf{H}}_{\mathbb{P}}^{\mathbb{F}}\left(\mathcal{F}\right)\sim \tau$.

(4) 

If $\mathcal{G}\sim \tau$, then ${\mathsf{H}}_{\mathbb{I}}^{\mathbb{G}}\left(\mathcal{G}\right)\sim \tau ,{\mathsf{H}}_{\mathbb{P}}^{\mathbb{G}}\left(\mathcal{G}\right)\sim \tau ,{\mathsf{H}}_{\mathbb{F}}^{\mathbb{G}}\left(\mathcal{G}\right)\sim \tau$.

In the theory of ideal topological spaces, there are several characterizations of compatibility. For $(X,\tau ,\mathcal{I})$, the most common equivalent conditions are as follows (see, for example, Hamlett and Jankovic [4] and Jankovic and Hamlett [5]).

Theorem 11. 

The following are equivalent:

(1) 

$\mathcal{I}\sim \tau$,

(2) 

for every $A\subset X$, if $A\cap A_{\mathcal{I}}^{*}=\varnothing$, then $A\in \mathcal{I}$,

(3) 

for every $A\subset X$, $A\backslash A_{\mathcal{I}}^{*}\in \mathcal{I}$,

(4) 

for every $A\subset X$, if $(X\backslash A)\cup {(X\backslash A)}_{\mathcal{I}}^{▷}=X$, then $A\in \mathcal{I}$,

(5) 

for every $A\subset X$, $A_{\mathcal{I}}^{▷}\backslash A\in \mathcal{I}$.

Regardless of the concept we work with, compatibility can be characterized by another equivalent system.

Theorem 12. 

Let ${\mathcal{Z}}_1,{\mathcal{Z}}_2\in \mathbb{X}$. If ${\mathcal{Z}}_1\sim {\mathcal{Z}}_2$, then the following are equivalent:

(1) 

${\mathcal{Z}}_1\sim \tau$,

(2) 

for every $A\subset X$, if $A\cap A_{{\mathcal{Z}}_2}^{*}=\varnothing$, then A is ${\mathcal{Z}}_2$-small,

(3) 

for every $A\subset X$, $A\backslash A_{{\mathcal{Z}}_2}^{*}$ is ${\mathcal{Z}}_2$-small,

(4) 

for every $A\subset X$, if $(X\backslash A)\cup {(X\backslash A)}_{{\mathcal{Z}}_2}^{▷}=X$, then A is ${\mathcal{Z}}_2$-small,

(5) 

for every $A\subset X$, $A_{{\mathcal{Z}}_2}^{▷}\backslash A$ is ${\mathcal{Z}}_2$-small.

Proof. 

Let ${\mathcal{I}}_1:={\mathsf{H}}_{\mathbb{I}}^{{\mathbb{Z}}_1}\left({\mathcal{Z}}_1\right)$, where ${\mathcal{Z}}_1\in {\mathbb{Z}}_1\in \{\mathbb{P},\mathbb{F},\mathbb{G}\}$. Then, ${\mathcal{I}}_1\sim {\mathcal{Z}}_1\sim {\mathcal{Z}}_2$. As per Remark 2, ${\mathcal{Z}}_1\sim \tau$ if and only if ${\mathcal{I}}_1\sim \tau$ and A is ${\mathcal{I}}_1$-small if and only if A is ${\mathcal{Z}}_2$-small. Moreover, as per Lemma 2, $A_{{\mathcal{I}}_1}^{*}=A_{{\mathcal{Z}}_1}^{*}=A_{{\mathcal{Z}}_2}^{*}$, $A_{{\mathcal{I}}_1}^{▷}=A_{{\mathcal{Z}}_1}^{▷}=A_{{\mathcal{Z}}_2}^{▷}$. Then, the following are equivalent (note $\left(\mathrm{ii}\right)\iff \left(2\right),\left(\mathrm{iii}\right)\iff \left(3\right),\left(\mathrm{iv}\right)\iff \left(4\right),(\mathrm{v})\iff (5)$):

(1)

${\mathcal{Z}}_1\sim \tau$,

(i)

${\mathcal{I}}_1\sim \tau$,

(ii)

for every $A\subset X$, if $A\cap A_{{\mathcal{I}}_1}^{*}=\varnothing$, then A is ${\mathcal{I}}_1$-small,

(iii)

for every $A\subset X$, $A\backslash A_{{\mathcal{I}}_1}^{*}$ is ${\mathcal{I}}_1$-small,

(iv)

for every $A\subset X$, if $(X\backslash A)\cup {(X\backslash A)}_{{\mathcal{I}}_1}^{▷}=X$, then A is ${\mathcal{I}}_1$-small,

(v)

for every $A\subset X$, $A_{{\mathcal{I}}_1}^{▷}\backslash A$ is ${\mathcal{I}}_1$-small.

(2)

for every $A\subset X$, if $A\cap A_{{\mathcal{Z}}_2}^{*}=\varnothing$, then A is ${\mathcal{Z}}_2$-small,

(3)

for every $A\subset X$, $A\backslash A_{{\mathcal{Z}}_2}^{*}$ is ${\mathcal{Z}}_2$-small,

(4)

for every $A\subset X$, if $(X\backslash A)\cup {(X\backslash A)}_{{\mathcal{Z}}_2}^{▷}=X$, then A is ${\mathcal{Z}}_2$-small,

(5)

for every $A\subset X$, $A_{{\mathcal{Z}}_2}^{▷}\backslash A$ is ${\mathcal{Z}}_2$-small.

□

Remark 3. 

Using the notion of compatibility in ideal topological space, the corresponding notion can be introduced for any system $\mathcal{Z}$ in the following way. A topology τ is compatible with a primal $\mathcal{P}$, a grill $\mathcal{G}$ and a filter $\mathcal{F}$ if τ is compatible with the corresponding ideals ${\mathsf{H}}_{\mathbb{I}}^{\mathbb{P}}\left(\mathcal{P}\right)$, ${\mathsf{H}}_{\mathbb{I}}^{\mathbb{G}}\left(\mathcal{G}\right)$ and ${\mathsf{H}}_{\mathbb{I}}^{\mathbb{F}}\left(\mathcal{F}\right)$, respectively. Note that in a primal case (see Al-Omari et al. [24]), compatibility is called “a topology suitable for a primal”. Other examples of applications are given at the end of this section.

Definition 5. 

A set A is $\mathcal{I}$-big ($\mathcal{P}$-big, $\mathcal{F}$-big, $\mathcal{G}$-big) if A is not $\mathcal{I}$-small ($\mathcal{P}$-small, $\mathcal{F}$-small, $\mathcal{G}$-small), and equivalently $A\notin \mathcal{I}$ ($X\backslash A\in \mathcal{P}$, $X\backslash A\notin \mathcal{F}$, $A\in \mathcal{G}$). Let $\mathcal{Z}\in \mathbb{Z}$. A topology τ is $\mathcal{Z}$-codense if any non-empty open set is $\mathcal{Z}$-big.

Remark 4. 

Let $\mathcal{I}\sim \mathcal{P}\sim \mathcal{F}\sim \mathcal{G}$. Then, $\mathcal{I}={\mathsf{H}}_{\mathbb{I}}^{\mathbb{P}}\left(\mathcal{P}\right)$, $\mathcal{F}={\mathsf{H}}_{\mathbb{F}}^{\mathbb{P}}\left(\mathcal{P}\right)$, $\mathcal{G}={\mathsf{H}}_{\mathbb{G}}^{\mathbb{F}}\left(\mathcal{F}\right)$. So, $A\notin \mathcal{I}\iff X\backslash A\in \mathcal{P}\iff X\backslash A\notin \mathcal{F}\iff A\in \mathcal{G}$. That means if ${\mathcal{Z}}_1,{\mathcal{Z}}_2\in \mathbb{X}$ and ${\mathcal{Z}}_1\sim {\mathcal{Z}}_2$, a set A is ${\mathcal{Z}}_1$-big if and only if A is ${\mathcal{Z}}_2$-big and τ is ${\mathcal{Z}}_1$-codense if and only if τ is ${\mathcal{Z}}_2$-codense.

From the theory of ideal topological spaces, we can obtain the following characterization.

Theorem 13. 

τ is $\mathcal{I}$-codense if and only if $X_{\mathcal{I}}^{*}=X$.

The property of being $\mathcal{Z}$-codense can be characterized by another equivalent system as the next theorem shows.

Theorem 14. 

Let ${\mathcal{Z}}_1,{\mathcal{Z}}_2\in \mathbb{X}$. If ${\mathcal{Z}}_1\sim {\mathcal{Z}}_2$, then the following are equivalent:

(1) 

τ is ${\mathcal{Z}}_1$-codense,

(2) 

$X_{{\mathcal{Z}}_2}^{*}=X$,

(3) 

${\varnothing }_{{\mathcal{Z}}_2}^{▷}=\varnothing$.

Proof. 

Let $\mathcal{I}:={\mathsf{H}}_{\mathbb{I}}^{{\mathbb{Z}}_2}\left({\mathcal{Z}}_2\right)$, where ${\mathcal{Z}}_2\in {\mathbb{Z}}_2\in \{\mathbb{P},\mathbb{F},\mathbb{G}\}$. Then, ${\mathcal{Z}}_2\sim \mathcal{I}$. As per Remark 4 and Theorem 13, τ is ${\mathcal{Z}}_1$-codense if and only if τ is ${\mathcal{Z}}_2$-codense, and τ is ${\mathcal{Z}}_2$-codense if and only if τ is $\mathcal{I}$-codense. The last holds if and only if $X=X_{\mathcal{I}}^{*}=X_{{\mathsf{H}}_{\mathbb{I}}^{{\mathbb{Z}}_2}\left({\mathcal{Z}}_2\right)}^{*}=X_{{\mathcal{Z}}_2}^{*}$ (as per Theorem 7), so $\left(1\right)\iff \left(2\right)$. The equivalence $\left(2\right)\iff \left(3\right)$ follows from equation ${\varnothing }_{{\mathcal{Z}}_2}^{▷}=X\backslash X_{{\mathcal{Z}}_2}^{*}$.  □

Finally, one more application in topology is presented. Many topological properties can be characterized by filters. Equivalent characterization can be performed by grills, primals and ideals in the following way. A primal $\mathcal{P}$, a grill $\mathcal{G}$ and an ideal $\mathcal{I}$ converge to a point x if the corresponding filters ${\mathsf{H}}_{\mathbb{F}}^{\mathbb{P}}\left(\mathcal{P}\right)$, ${\mathsf{H}}_{\mathbb{F}}^{\mathbb{G}}\left(\mathcal{G}\right)$ and ${\mathsf{H}}_{\mathbb{F}}^{\mathbb{I}}\left(\mathcal{I}\right)$ converge to x. Moreover, $\mathcal{P}$, $\mathcal{G}$ and $\mathcal{I}$ are referred to as an ultraprimal, an ultragrill and an ultraideal if the corresponding filters ${\mathsf{H}}_{\mathbb{F}}^{\mathbb{P}}\left(\mathcal{P}\right)$, ${\mathsf{H}}_{\mathbb{F}}^{\mathbb{G}}\left(\mathcal{G}\right)$ and ${\mathsf{H}}_{\mathbb{F}}^{\mathbb{I}}\left(\mathcal{I}\right)$ are the ultrafilters. For example, a topological space is Hausdorff (compact) if and only if each primal, grill and ideal have at most one limit (each ultraprimal, ultragrill and ultraideal converges to at least one point); see Modak et al. [33] and Selim et al. [34].

Another alternative approach can be used in cases of $\mathcal{I}$-convergence of sequences in metric spaces, where $\mathcal{I}$ is an ideal of subsets of the set $\mathbb{N}$ of positive integers; see Kostyrko et al. [35]. Let $(X,d)$ be a fixed metric space and $\mathcal{I}$ be an ideal of subsets of $\mathbb{N}$. A sequence ${\left\{x_n\right\}}_{n\in \mathbb{N}}$ of elements of X is said to be $\mathcal{I}$-convergent to $a\in X$ if for each $ϵ>0$ the set $A\left(ϵ\right)=\{n\in \mathbb{N}:d(x_n,a)\ge ϵ\}$ belongs to $\mathcal{I}$. The element a is called the $\mathcal{I}$-limit of the sequence ${\left\{x_n\right\}}_{n\in \mathbb{N}}$. Equivalent characterization can be performed by primals, grills and ideals in the following way. Let $\mathcal{P}$, $\mathcal{G}$ and $\mathcal{F}$ be a primal, a grill and a filter, respectively. A sequence ${\left\{x_n\right\}}_{n\in \mathbb{N}}$ is said to be $\mathcal{P}$-convergent, $\mathcal{G}$-convergent or $\mathcal{F}$-convergent to $a\in X$, if for each $ϵ>0$ the set $\mathbb{N}\backslash A\left(ϵ\right)=\{n\in \mathbb{N}:d(x_n,a)<ϵ\}$ does not belong to $\mathcal{P}$, $A\left(ϵ\right)$ does not belong to $\mathcal{G}$ and $\mathbb{N}\backslash A\left(ϵ\right)=\{n\in \mathbb{N}:d(x_n,a)<ϵ\}$ belongs to $\mathcal{F}$, respectively. In a similar way, other concepts such as ${\mathcal{I}}^{*}$-convergence, ${\mathcal{I}}^{*}$-limit points and ${\mathcal{I}}^{*}$-cluster points (see Kostyrko et al. [35]) can be introduced with respect to $\mathcal{Z}\in \mathbb{P}\cup \mathbb{G}\cup \mathbb{F}$. A notion of admissible ideals is important in the study of various relationships. An ideal $\mathcal{I}$ is called admissible if any finite set is in $\mathcal{I}$. An equivalent definition for $\mathcal{P},\mathcal{G},\mathcal{F}$ is as follows. $\mathcal{P}$, $\mathcal{G}$ and $\mathcal{F}$ are admissible if and only if the corresponding ideals ${\mathsf{H}}_{\mathbb{I}}^{\mathbb{P}}\left(\mathcal{P}\right)$, ${\mathsf{H}}_{\mathbb{I}}^{\mathbb{G}}\left(\mathcal{G}\right)$ and ${\mathsf{H}}_{\mathbb{I}}^{\mathbb{F}}\left(\mathcal{F}\right)$ are admissible, respectively, and equivalently, if any co-finite set is not in $\mathcal{P}$, any finite set is not in $\mathcal{G}$ and any co-finite set is in $\mathcal{F}$, respectively.

4. Direction of Further Research

In this section, we would like to point out possible directions for further research. On the basis of proven facts, we could say that the results established in topological spaces with grills, primals and filters are nothing but the results established in ideal topological spaces. The question is whether it is possible to achieve comparable results derived from more general systems. Before we introduce a general concept, let us recall some trivial cases. Definition 1 applies only to non-trivial systems. In the following remark, we deal with the trivial cases.

Remark 5. 

If $\mathcal{I}=\mathcal{P}=\mathcal{G}=\mathcal{F}=\varnothing$, then $\mathcal{I}$, $\mathcal{P}$, $\mathcal{G}$, $\mathcal{F}$ are vacuously an ideal, a primal, a grill and a filter, respectively. If $X\in \mathcal{I}$, $X\in \mathcal{P}$, $\varnothing \in \mathcal{G}$, $\varnothing \in \mathcal{F}$, then $\mathcal{I}=\mathcal{P}=\mathcal{G}=\mathcal{F}=2^X$ (power set of X). Then, the following is easy to check. The systems $\mathcal{I}=2^X$, $\mathcal{P}=\varnothing$, $\mathcal{G}=\varnothing$ and $\mathcal{F}=2^X$ are mutually equivalent; all local functions are equal to ∅ and all induced topologies are discrete. The systems $\mathcal{I}=\varnothing$, $\mathcal{P}=2^X$, $\mathcal{G}=2^X$, $\mathcal{F}=\varnothing$ are mutually equivalent, and all local functions are equal to X. They do not induce topologies. The only open set is ∅.

Let us try to derive a local function from a system on which we do not impose any conditions.

Let $\mathcal{E}$ be a system of subsets of X. For a subset A of a topological space $(X,\tau )$, we define the set $\mathcal{E}\left(A\right)$ of all points $x\in X$ such that for any neighborhood U of x, the intersection $U\cap A$ contains a set form $\mathcal{E}$. A triplet $(X,\tau ,\mathcal{E})$ is called a cluster topological space; see Matejdes [36], Matejdes [37] and Thangamariappan and Renukadevi [38], where one can find the basic notions of cluster topological space.

For a trivial case $\mathcal{E}=\varnothing$ ($\varnothing \in \mathcal{E}$), we have $\mathcal{E}\left(A\right)=\varnothing$ ($\mathcal{E}\left(A\right)=X$) for any $A\subset X$. In the sequel, we suppose $\mathcal{E}$ is not trivial, i.e., $\mathcal{E}$ is a non-empty system of non-empty subsets of X.

Remark 6. 

Specially, if $\mathcal{I}$ is an ideal on X, then a cluster system ${\mathcal{E}}_{\mathcal{I}}=\{Z\subset X:Z\notin \mathcal{I}\}$ leads to $\mathcal{I}$-local function and ${\mathcal{E}}_{\mathcal{I}}\left(A\right)=A_{\mathcal{I}}^{*}$. If $\mathcal{I}=2^X$, then $\mathcal{E}=\varnothing$ and ${\mathcal{E}}_{\mathcal{I}}\left(A\right)=A_{\mathcal{I}}^{*}=\varnothing$ for any $A\subset X$. If $\mathcal{I}=\varnothing$, then $\mathcal{E}=2^X$ and ${\mathcal{E}}_{\mathcal{I}}\left(A\right)=A_{\mathcal{I}}^{*}=X$ for any $A\subset X$.

Despite the generality of the definition of a cluster system, the following lemma copies the known properties of $\mathcal{I}$-local function. Let us mention some of them (compare with Theorem 8).

Lemma 3 

([36,37,38]).

(1) 

$\mathcal{E}(\varnothing )=\varnothing$,

(2) 

if $A\subset B$, then $\mathcal{E}\left(A\right)\subset \mathcal{E}\left(B\right)$,

(3) 

$\mathcal{E}\left(A\right)\cup \mathcal{E}\left(B\right)\subset \mathcal{E}(A\cup B)$,

(4) 

$\mathcal{E}\left(A\right)\subset cl\left(A\right)$ and $\mathcal{E}\left(A\right)$ is closed,

(5) 

$\mathcal{E}\left(\mathcal{E}\right(A\left)\right)\subset \mathcal{E}\left(A\right)$,

(6) 

if ${\mathcal{E}}_1\subset {\mathcal{E}}_2$, then ${\mathcal{E}}_1\left(A\right)\subset {\mathcal{E}}_2\left(A\right)$,

In a cluster topological setting, we can introduce the following classification of sets.

Definition 6 

([36,37,38]). A set $A\subset X$ is called

(1) 

$\mathcal{E}$-closed ($\mathcal{E}$-open, $\mathcal{E}$-dense in itself, $\mathcal{E}$-perfect), if $\mathcal{E}\left(A\right)\subset A$ ($X\backslash A$ is $\mathcal{E}$-closed, $A\subset \mathcal{E}\left(A\right)$, $\mathcal{E}\left(A\right)=A$).

(2) 

$\mathcal{E}$-scattered, if A contains no set from $\mathcal{E}$.

(3) 

locally $\mathcal{E}$-scattered at a point $x\in X$, if there is an open set U containing x such that $U\cap A$ is $\mathcal{E}$-scattered (i.e., $x\notin \mathcal{E}\left(A\right)$).

(4) 

locally $\mathcal{E}$-scattered, if A is $\mathcal{E}$-scattered at any point from A (i.e., $A\cap \mathcal{E}\left(A\right)=\varnothing$).

(5) 

$\mathcal{E}$-nowhere dense, if for any non-empty open set U there is a non-empty open subset H of U such that $H\cap A$ contains no set from $\mathcal{E}$.

An ideal $\mathcal{I}$ represents small sets and a set not belonging to $\mathcal{I}$ is understood as large. A cluster system also has a similar interpretation. A set from $\mathcal{E}$ is understood as large. This means that any set containing a set from $\mathcal{E}$ is also large. On the other hand, a set containing no set from $\mathcal{E}$ (i.e., an $\mathcal{E}$-scattered set) is small. For example, a set A is $\mathcal{I}$-small (in the sense of Definition 4) if and only if A is ${\mathcal{E}}_{\mathcal{I}}$-scattered. If we use the terminology of [12], a set A locally belongs to $\mathcal{I}$ (i.e., $A\cap A_{\mathcal{I}}^{*}=\varnothing$) if and only if A is locally ${\mathcal{E}}_{\mathcal{I}}$-scattered (i.e., $A\cap {\mathcal{E}}_{\mathcal{I}}\left(A\right)=\varnothing$).

Despite the many results achieved in [36,37,38]), among other directions of research we could include relationships between the properties $B_1$, $B_2$ and $B_3$ inspired by a very interesting article [12] that deals with three abstract versions of the Banach category theorem for an arbitrary ideal which are mutually equivalent for the ideal of meager sets.

Property $B_1$: If A is a $\mathcal{E}$-nowhere dense set, then A is $\mathcal{E}$-scattered.

Property $B_2$: If A is A locally $\mathcal{E}$-scattered, then A is $\mathcal{E}$-scattered.

Property $B_3$: The union of any open $\mathcal{E}$-scattered sets is $\mathcal{E}$-scattered.

It is clear $B_1\Rightarrow B_2$. Other mutual relations are left for further research (see analogies in [12]).

5. Conclusions

The main result of this work is based on the unification of approaches to the creation of new topologies derived from ideals, primals, filters and grills. Each approach has its equivalent counterparts in other approaches, and results valid in one approach can be used in others according to the following scheme. Definitions, theorems and proof methods in a space $(X,\tau ,\mathcal{Z})$, $\mathcal{Z}\in \mathbb{X}$ are valid and usable in the other three spaces $(X,\tau ,{\mathsf{H}}_{{\mathbb{Z}}_1}^{{\mathbb{Z}}_2}\left(\mathcal{Z}\right))$, where $\mathcal{Z}\in {\mathbb{Z}}_2\in \{\mathbb{I},\mathbb{P},\mathbb{F},\mathbb{G}\}$ and ${\mathbb{Z}}_1\in \{\mathbb{I},\mathbb{P},\mathbb{F},\mathbb{G}\}$, ${\mathbb{Z}}_1\ne {\mathbb{Z}}_2$. From this point of view, many proofs do not need to be obtained, and it is enough to obtain them only once in one space. Due to the fact that ideals are the most widespread set of systems, we can say that the results established in topological spaces with grills, primals and filters are nothing but the results established in ideal topological spaces. On the other hand, such diversity, even if equivalent, can be a stimulus for further research and applications.