Exact Solutions to Fractional Schrödinger–Hirota Equation Using Auxiliary Equation Method

Abstract

In this paper, we consider the fractional Schrödinger–Hirota (FSH) equation in the sense of a conformable fractional derivative. Through a traveling wave transformation, we change the FSH equation to an ordinary differential equation. We obtain several exact solutions through the auxiliary equation method, including soliton, exponential and periodic solutions, which are useful to analyze the behaviors of the FSH equation. We show that the auxiliary equation method improves the speed of the discovery of exact solutions.

1. Introduction

The Schrödinger equation, also known as the Schrödinger wave equation, is a fundamental partial differential equation (PDE) in quantum mechanics. It describes the motion of microscopic particles by combining the concept of matter waves with the wave equation [1]. The Hirota equation is a nonlinear partial differential equation (NLPDE). It describes nonlinear wave behavior in certain physical systems [2]. There are also some papers about the Schrödinger equation and Hirota equation from the perspective of differential geometry [3,4]. The Gaussian curvature, mean curvature and other geometric properties of the soliton surfaces and hypersurfaces of the equations in Euclidean and Minkowski spaces are studied in [5,6,7,8].

The Schrödinger–Hirota (SH) equation combines the Schrödinger equation with the Hirota equation. It is an NLPDE with a dispersion term, which can capture the nonlinear coupling effects in some complex nonlinear wave phenomena. However, with the advancement of technology, especially in the fields of high-precision measurement and ultrafast optics, traditional models have shown their limitations. Therefore, the demand for the exploration of more complex systems has prompted us to seek more precise mathematical tools to describe the behavior of light waves. As a generalization of the integer-order SH equation, the fractional Schrödinger–Hirota (FSH) equation has been proven to be a powerful tool in describing the propagation behavior of light waves in optical fibers. The fractional derivatives enable us to describe the effects of non-local and non-Markov processes on light wave propagation more accurately [9], therefore providing new possibilities for the understanding and design of novel fiber optic devices. In this paper, we focus on the FSH equation; it is given as follows [1]:

$$i{D}_t^{\alpha }u+a{D}_x^{\beta }{D}_x^{\beta }u+{b\left|u\right|}^{2}u+i(c{D}_x^{\beta }{D}_x^{\beta }{D}_x^{\beta }u+{d\left|u\right|}^2{D}_x^{\beta }u)=0,$$

(1)

where x is the spatial variable, t is the time variable and $u=u(x,t)$ is the complex short wave envelope. $a,b,c$ and d are constants, where a is the spatio-temporal dispersion, b is the Landau coefficient, c is the third-order dispersion and d is the nonlinear dispersion. $D_t^{\alpha }$, $D_x^{\beta }$ are the modified fractional derivatives; $\alpha ,\beta$ are constants satisfying $0<\alpha ,\beta \le 1$.

The exact solutions of the FSH equation are crucial in understanding the dynamic behavior of complex systems. There are many methods used to find the exact solutions of the FSH equation, such as the Hirota bilinear method [10], Jacobi elliptic function expansion method [11], Darboux transform method [12] and so on [13].

In this paper, we obtain some exact solutions of (1) through the traveling wave transformation [14] and auxiliary equation method [15]. We also give the figures and contour plots of the amplitudes of these solutions. When studying the solutions and properties of these equations, differential geometry tools such as manifolds, connections and curvatures can be used [16,17,18,19]. The geometric structures of the manifolds [20] can be used in analyzing the behaviors of the solutions. We hope to reveal the influence of fractional derivatives on the propagation behaviors of light waves through these exact solutions.

The auxiliary equation method used in this paper is highly efficient in solving certain NLPDEs. It converts an NLPDE into a lower-dimensional ODE, which reduces the difficulty in solving equations. Appropriate auxiliary equations can systematically generate multiple solutions and verify the structures of the solutions, therefore providing a powerful exploration tool for the analysis of the properties of the solution space.

2. Preliminaries

2.1. Conformable Fractional Derivatives

Firstly, we review the definition and properties of conformable fractional derivatives.

Definition 1

([21]). For a function $f:[0,+\infty )\to \mathbf{R}$, its conformable fractional derivative of order $\alpha$ at t is defined by

$$D_t^{\alpha }f\left(t\right)=\underset{\epsilon \to 0}{lim}{\displaystyle \frac{f(t-\epsilon t^{1-\alpha })-f\left(t\right)}{\epsilon }},$$

(2)

where $t>0$ and $0<\alpha \le 1$. If the limit above exists, f is said to be differentiable of order $\alpha$ at t. If f is differentiable of order α in the interval $(0,s)$ for some $s>0$, and $\underset{t\to 0}{lim}{D}_t^{\alpha }f\left(t\right)$ exists, we define the conformable fractional derivative of order $\alpha$ of f at 0 to be $D_t^{\alpha }f\left(0\right)=\underset{t\to 0}{lim}{D}_t^{\alpha }f\left(t\right)$.

By definition, $D_t^{1}f\left(t\right)$ is simply the normal derivative $f^{\prime }\left(t\right)$ of f when $t>0$ and the normal right-hand derivative $f_{+}^{\prime }\left(t\right)$ of f when $t=0$.

Lemma 1

([21]). Let $0<\alpha \le 1$, $0<t\le 1$, $f\left(t\right)$ and $g\left(t\right)$ be differentiable of order α at $t>0$. Then,

1. 

$D_t^{\alpha }\left(\lambda \right)=0$ for any constant λ;

2. 

$D_t^{\alpha }{t}^{\mu }=\mu t^{\mu -\alpha }$ for any $\mu \in \mathbf{R}$;

3. 

$D_t^{\alpha }(af\left(t\right)+bg\left(t\right))=a{D}_t^{\alpha }f\left(t\right)+b{D}_t^{\alpha }g\left(t\right)$ for any $a,b\in \mathbf{R}$;

4. 

$D_t^{\alpha }\left(f\left(t\right)g\left(t\right)\right)=g\left(t\right)D_t^{\alpha }f\left(t\right)+f\left(t\right)D_t^{\alpha }g\left(t\right)$;

5. 

$D_t^{\alpha }{\displaystyle \frac{f\left(t\right)}{g\left(t\right)}}={\displaystyle \frac{g\left(t\right)D_t^{\alpha }f\left(t\right)+f\left(t\right)D_t^{\alpha }g\left(t\right)}{g^2\left(t\right)}}$;

6. 

When $f\left(t\right)$ is differentiable in the normal sense, $D_t^{\alpha }f\left(t\right)=t^{1-\alpha }{f}^{\prime }\left(t\right)$.

2.2. Auxiliary Equation Method

Here, we introduce the basic idea of the auxiliary equation method briefly [22]. We consider an NLPDE as

$$F(u,u_t,u_x,u_{xt},u_{xx},\cdots )=0,$$

(3)

F is the polynomial of the shown variables. It contains the nonlinear term of the unknown function and the linear highest derivative term.

Step 1. Then, we consider the following transformation:

$$u(x,t)=u\left(\xi \right),\xi =kx-vt,$$

(4)

where $\xi =kx-vt$ is the phase of the traveling wave, and the wave number k and wave speed v are constants. With the derivative substitution

$${\displaystyle \frac{\partial }{\partial t}}⟶-v{\displaystyle \frac{d}{d\xi }},{\displaystyle \frac{\partial }{\partial x}}⟶k{\displaystyle \frac{d}{d\xi }},$$

substituting (4) into (3) yields

$$G(u,u_{\xi },u_{\xi \xi },u_{\xi \xi \xi },\cdots )=0,$$

(5)

where G is the polynomial of the shown variables.

Step 2. Assume that the solution of (5) is given as follows:

$$u\left(\xi \right)=\sum _{i=-N}^N{m}_i{\phi }^i\left(\xi \right),$$

(6)

where $m_i$$(-N\le i\le N)$ are unknown constants to be determined. N is called the equilibrium constant, which can be determined by the homogeneous equilibrium principle. In detail, the linear derivative term of the highest order and the nonlinear term of the highest power in Equation (5) cancel each other out. A new auxiliary equation satisfying the following conditions is defined in [15].

$${\left({\phi }^{\prime }\left(\xi \right)\right)}^2=p_2{\phi }^2\left(\xi \right)+p_4{\phi }^4\left(\xi \right)+p_6{\phi }^6\left(\xi \right),$$

(7)

where $p_2,p_4$ and $p_6$ are real parameters. Some solutions of (7) have been given in Table 1 of [23].

Step 3. Substituting (6) and the auxiliary Equation (7) into Equation (5), making the coefficients of each power equal to zero, we obtain a system of nonlinear algebraic equations with $m_i(i=\cdots ,-2,-1,0,1,2,\cdots ),k,v,p_2,$$p_4,p_6$ as unknowns.

Step 4. The computer algebra system can be used to solve the system of nonlinear algebraic equations obtained in Step 3. Substituting the solutions into (6), the exact solution of Equation (3) is obtained by transformation (4).

3. Application of Auxiliary Equation Method to FSH Equation

The traveling wave transformation for the FSH Equation (1) is performed as follows.

$$u(x,t)=\psi \left(\xi \right){\mathrm{e}}^{i\theta },\xi =k{\displaystyle \frac{x^{\beta }}{\beta }}-\upsilon {\displaystyle \frac{t^{\alpha }}{\alpha }},\theta =r{\displaystyle \frac{x^{\beta }}{\beta }}-\omega {\displaystyle \frac{t^{\alpha }}{\alpha }},$$

(8)

where $\omega$ is the wave number of the soliton, and k, r, $\upsilon$ and $\omega$ are constants that need to be determined. Substituting (8) into (1) yields the following.

Re:

$$(\omega -a{r}^2+c{r}^3)\psi +(b-dr){\psi }^3+(a{k}^2-3c{k}^{2}r){\psi }^{″}=0.$$

(9)

Im:

$$(-\upsilon +2akr-3ck{r}^2){\psi }^{\prime }+dk{\psi }^2{\psi }^{\prime }+c{k}^3{\psi }^{″\prime }=0.$$

(10)

Integrating (10) and taking the constant as zero, we have the following result.

$$(-\upsilon +2akr-3ck{r}^2)\psi +{\displaystyle \frac{dk}{3}}{\psi }^3+c{k}^3{\psi }^{″}=0.$$

(11)

By balancing (9) and (11) with homogeneous power, the following formula can be obtained.

$${\displaystyle \frac{\omega -a{r}^2+c{r}^3}{-\upsilon +2akr-3ck{r}^2}}={\displaystyle \frac{b-dr}{\frac{dk}{3}}}={\displaystyle \frac{a-3cr}{ck}}.$$

(12)

From Equation (12), we have

$$d={\displaystyle \frac{3bc}{a}},\upsilon ={\displaystyle \frac{2a^{2}kr-ck\omega -8ack{r}^2+8ck{r}^3}{a-3cr}}.$$

(13)

Then, we consider (9) under the constraints of Equation (13). By using the homogeneous balance principle in (9), we have $N+2=3N$, i.e., $N=1$. Considering all of the above conditions, the scheme is presented in the representative form as follows.

$$\psi \left(\xi \right)=m_{-1}{\phi }^{-1}\left(\xi \right)+m_0+m_1\phi \left(\xi \right),$$

(14)

where $\phi \left(\xi \right)$ satisfies the auxiliary Equation (7). Substituting (14) into (9), we can obtain the following algebraic equation system.

$$\begin{array}{c}\hfill \left\{\begin{array}{cc}& (\omega -a{r}^2+c{r}^3)m_0+(b-dr)m_0^3+6(b-dr)m_{-1}{m}_0{m}_1=0,\hfill \\ & (b-rd)m_{-1}^3=0,\hfill \\ & 3(b-dr)m_{-1}^2{m}_0=0,\hfill \\ & (\omega -a{r}^2+c{r}^3)m_{-1}+3(b-dr)m_{-1}{m}_0^2+3(b-dr)m_{-1}^2{m}_1\hfill \\ & +k^2(a-3cr)m_{-1}{p}_2=0,\hfill \\ & (\omega -a{r}^2+c{r}^3)m_1+3(b-dr)m_0^2{m}_1+3(b-dr)m_{-1}^2{m}_{-1}{m}_1^2\hfill \\ & +k^2(a-3cr)m_1{p}_2=0,\hfill \\ & (b-dr)m_1^3+2k^2(a-3cr)m_1{p}_4+k^2(3cr-a)m_{-1}{p}_6=0,\hfill \\ & 3(b-dr)m_0{m}_1^2=0,\hfill \\ & 3k^2(a-3cr)m_1{p}_6=0.\hfill \end{array}\right.\end{array}$$

(15)

A group of solutions of (15) are obtained as follows.

$$m_{-1}=0,m_0=0,m_1=\pm {\displaystyle \frac{i\sqrt{2}k\sqrt{a-3cr}\sqrt{p_4}}{\sqrt{b-dr}}},$$

$$p_6=0,\omega =a{r}^2-c{r}^3-a{k}^2{p}_2+3c{k}^{2}r{p}_2.$$

Therefore, we obtain exact solutions of (14) as

$$\psi \left(\xi \right)=\pm {\displaystyle \frac{i\sqrt{2}k\sqrt{a-3cr}\sqrt{p_4}}{\sqrt{b-dr}}}\phi \left(\xi \right).$$

(16)

4. Exact Solutions of FSH Equation

4.1. Method for Derivation of Exact Solutions

For Equation (7), let ${\phi }^2\left(\xi \right)=F\left(\xi \right)$; then, $\phi \left(\xi \right)=\pm \sqrt{F\left(\xi \right)}$. Substituting

$${\displaystyle \frac{d\phi }{d\xi }}=\pm {\displaystyle \frac{1}{2\sqrt{F}}}{\displaystyle \frac{dF}{d\xi }}$$

into (7), we have

$${\left(\pm {\displaystyle \frac{F^{\prime }}{2\sqrt{F}}}\right)}^2=p_{2}F+p_4{F}^2+p_6{F}^3,$$

(17)

i.e.,

$${\left(F^{\prime }\right)}^2=4p_2{F}^2+4p_4{F}^3+4p_6{F}^4.$$

(18)

The problem of solving Equation (7) becomes the problem of solving the following Equation (19).

$${\left(F^{\prime }\right)}^2=c_2{F}^2+c_3{F}^3+c_4{F}^4,$$

(19)

where $c_2=4p_2,c_3=4p_4,c_4=4p_6$.

Devising the following transformation

$$F\left(\xi \right)={\displaystyle \frac{4f^2\left(\xi \right)-c_2}{c_3-4\sqrt{c_4}f\left(\xi \right)}},$$

(20)

and substituting it into Equation (19), we have

$${\left({\displaystyle \frac{dF}{d\xi }}\right)}^2-c_2{F}^2-c_3{F}^3-c_4{F}^4={\displaystyle \frac{16\left(\frac{df}{d\xi }-f^2+\frac{c_2}{4}\right)\left(\frac{df}{d\xi }+f^2-\frac{c_2}{4}\right)H\left(f\right)}{{(4\sqrt{c_2}f-c_3)}^4}},$$

$$H\left(f\right)=16c_4{f}^4-16c_3\sqrt{c_4}{f}^3+(4c_3^2+8c_2{c}_4)f^2-4c_2{c}_3\sqrt{c_4}f+c_2^2{c}_4.$$

Therefore, $F\left(\xi \right)$ is a solution of Equation (19) if and only if $f\left(\xi \right)$ is a solution of the following Riccati equation:

$$f^{\prime }\left(\xi \right)=f^2\left(\xi \right)-{\displaystyle \frac{1}{4}}{c}_2.$$

(21)

This indicates that the Bäcklund transformation of Equation (19) and the nonlinear superposition formula of the solution can be indirectly derived from that of the Riccati Equation (21) through the transformation (20).

For example, integrating Equation (21), we obtain a solution

$$f\left(\xi \right)=-{\displaystyle \frac{\sqrt{c_2}}{2}}\tanh \left[{\displaystyle \frac{\sqrt{c_2}}{2}}\xi \right],$$

and substitute it into Equation (20), and we have

$$F\left(\xi \right)={\displaystyle \frac{4f^2\left(\xi \right)-c_2}{c_3-4\sqrt{c_4}f\left(\xi \right)}}={\displaystyle \frac{4{\left(-\frac{\sqrt{c_2}}{2}\tanh \left[\frac{\sqrt{c_2}}{2}\xi \right]\right)}^2-c_2}{c_3-4\sqrt{c_4}\left(-\frac{\sqrt{c_2}}{2}\tanh \left[\frac{\sqrt{c_2}}{2}\xi \right]\right)}}.$$

For ${\tanh }^2\left(\xi \right)+{\mathrm{sech}}^2\left(\xi \right)=1$, we have

$$F\left(\xi \right)={\displaystyle \frac{-c_2{\mathrm{sech}}^2\left[\frac{\sqrt{c_2}}{2}\xi \right]}{c_3+2\sqrt{c_2{c}_4}\tanh \left[\frac{\sqrt{c_2}}{2}\xi \right]}},$$

so

$$\phi \left(\xi \right)=\pm \sqrt{F\left(\xi \right)}=\pm \sqrt{{\displaystyle \frac{-c_2{\mathrm{sech}}^2\left[\frac{\sqrt{c_2}}{2}\xi \right]}{c_3+2\sqrt{c_2{c}_4}\tanh \left[\frac{\sqrt{c_2}}{2}\xi \right]}}}.$$

For $p_6=0$ and $c_4=0$, we have

$$\phi \left(\xi \right)=\pm \sqrt{-{\displaystyle \frac{p_2}{p_4}}}\mathrm{sech}\left[\sqrt{p_2}\xi \right].$$

This is the first solution ${\phi }_1\left(\xi \right)$ that we give in Section 4.2. The exact solutions of the Riccati Equation (21) are well known; similarly, we can obtain other exact solutions of (1), which we give in Section 4.2.

4.2. Six Exact Solutions of FSH Equation

In this section, we give six exact solutions of (1) using the method introduced in Section 4.1. The solutions of (7) have also been provided in [23,24]. Here, we take

$$m_{-1}=0,m_0=0,m_1=\pm {\displaystyle \frac{i\sqrt{2}k\sqrt{a-3cr}\sqrt{p_4}}{\sqrt{b-dr}}},$$

$$p_6=0,\omega =a{r}^2-c{r}^3-a{k}^2{p}_2+3c{k}^{2}r{p}_2.$$

We divide the coefficients of (7) into three cases.

Case 1. When $p_2>0$ and $\epsilon =\pm 1$, Equation (7) has the following solutions:

$${\phi }_1\left(\xi \right)=\pm \mathrm{sech}\left[\sqrt{p_2}\left(k{\displaystyle \frac{x^{\beta }}{\beta }}-\upsilon {\displaystyle \frac{t^{\alpha }}{\alpha }}\right)-{\xi }_0\right]{\left({\displaystyle \frac{-p_2{p}_4}{p_4^2-p_2{p}_6{\left(1+\epsilon \tanh \left[\sqrt{p_2}\left(k\frac{x^{\beta }}{\beta }-\upsilon \frac{t^{\alpha }}{\alpha }\right)\right]\right)}^2}}\right)}^{{\displaystyle \frac{1}{2}}}.$$

$${\phi }_2\left(\xi \right)=\pm \mathrm{csch}\left[\sqrt{p_2}\left(k{\displaystyle \frac{x^{\beta }}{\beta }}-\upsilon {\displaystyle \frac{t^{\alpha }}{\alpha }}\right)-{\xi }_0\right]{\left({\displaystyle \frac{p_2{p}_4}{p_4^2-p_2{p}_6{\left(1+\epsilon \coth \left[\sqrt{p_2}\left(k\frac{x^{\beta }}{\beta }-\upsilon \frac{t^{\alpha }}{\alpha }\right)\right]\right)}^2}}\right)}^{{\displaystyle \frac{1}{2}}}.$$

$${\phi }_3\left(\xi \right)=\pm 4{\left({\displaystyle \frac{p_2{\mathrm{e}}^{2\epsilon \sqrt{p_2}\left(k\frac{x^{\beta }}{\beta }-\upsilon \frac{t^{\alpha }}{\alpha }\right)}}{{\left({\mathrm{e}}^{2\epsilon \sqrt{p_2}\left(k\frac{x^{\beta }}{\beta }-\upsilon \frac{t^{\alpha }}{\alpha }\right)}-4p_4\right)}^2-64p_2{p}_6}}\right)}^{{\displaystyle \frac{1}{2}}}.$$

Hence, Equation (1) has the following solutions, respectively:

$$u_1(x,t)=\pm {\displaystyle \frac{i\sqrt{2}k\sqrt{a-3cr}\sqrt{p_4}}{\sqrt{b-dr}}}{\phi }_1\left(\xi \right)\exp \left[i\left(r{\displaystyle \frac{x^{\beta }}{\beta }}-\omega {\displaystyle \frac{t^{\alpha }}{\alpha }}\right)\right].$$

$$u_2(x,t)=\pm {\displaystyle \frac{i\sqrt{2}k\sqrt{a-3cr}\sqrt{p_4}}{\sqrt{b-dr}}}{\phi }_2\left(\xi \right)\exp \left[i\left(r{\displaystyle \frac{x^{\beta }}{\beta }}-\omega {\displaystyle \frac{t^{\alpha }}{\alpha }}\right)\right].$$

$$u_3(x,t)=\pm {\displaystyle \frac{i\sqrt{2}k\sqrt{a-3cr}\sqrt{p_4}}{\sqrt{b-dr}}}{\phi }_3\left(\xi \right)\exp \left[i\left(r{\displaystyle \frac{x^{\beta }}{\beta }}-\omega {\displaystyle \frac{t^{\alpha }}{\alpha }}\right)\right].$$

Case 2. When $p_2>0$, $\Delta =p_4^2-4p_2{p}_6>0$ and $\epsilon =\pm 1$, Equation (7) has the following solution:

$${\phi }_4\left(\xi \right)=\pm {\left({\displaystyle \frac{2p_2}{\epsilon \sqrt{\Delta }\cosh \left[2\sqrt{p_2}\left(k\frac{x^{\beta }}{\beta }-\upsilon \frac{t^{\alpha }}{\alpha }\right)\right]-p_4}}\right)}^{{\displaystyle \frac{1}{2}}}.$$

Hence, Equation (1) has the following solution:

$$u_4(x,t)=\pm {\displaystyle \frac{i\sqrt{2}k\sqrt{a-3cr}\sqrt{p_4}}{\sqrt{b-dr}}}{\phi }_4\left(\xi \right)\exp \left[i\left(r{\displaystyle \frac{x^{\beta }}{\beta }}-\omega {\displaystyle \frac{t^{\alpha }}{\alpha }}\right)\right].$$

Case 3. When $p_2<0$, $\Delta =p_4^2-4p_2{p}_6>0$ and $\epsilon =\pm 1$, Equation (7) has the following solutions:

$$\begin{array}{c}\hfill {\phi }_5\left(\xi \right)=\pm {\left({\displaystyle \frac{2p_2}{\epsilon \sqrt{\Delta }cos\left[2\sqrt{-p_2}\left(k\frac{x^{\beta }}{\beta }-\upsilon \frac{t^{\alpha }}{\alpha }\right)\right]-p_4}}\right)}^{{\displaystyle \frac{1}{2}}}.\\ \hfill {\phi }_6\left(\xi \right)=\pm {\left({\displaystyle \frac{2p_2}{\epsilon \sqrt{\Delta }sin\left[2\sqrt{-p_2}\left(k\frac{x^{\beta }}{\beta }-\upsilon \frac{t^{\alpha }}{\alpha }\right)\right]-p_4}}\right)}^{{\displaystyle \frac{1}{2}}}.\end{array}$$

Hence, Equation (1) has the following solutions, respectively:

$$\begin{array}{c}\hfill u_5(x,t)=\pm {\displaystyle \frac{i\sqrt{2}k\sqrt{a-3cr}\sqrt{p_4}}{\sqrt{b-dr}}}{\phi }_5\left(\xi \right)\exp \left[i\left(r{\displaystyle \frac{x^{\beta }}{\beta }}-\omega {\displaystyle \frac{t^{\alpha }}{\alpha }}\right)\right].\\ \hfill u_6(x,t)=\pm {\displaystyle \frac{i\sqrt{2}k\sqrt{a-3cr}\sqrt{p_4}}{\sqrt{b-dr}}}{\phi }_6\left(\xi \right)\exp \left[i\left(r{\displaystyle \frac{x^{\beta }}{\beta }}-\omega {\displaystyle \frac{t^{\alpha }}{\alpha }}\right)\right].\end{array}$$

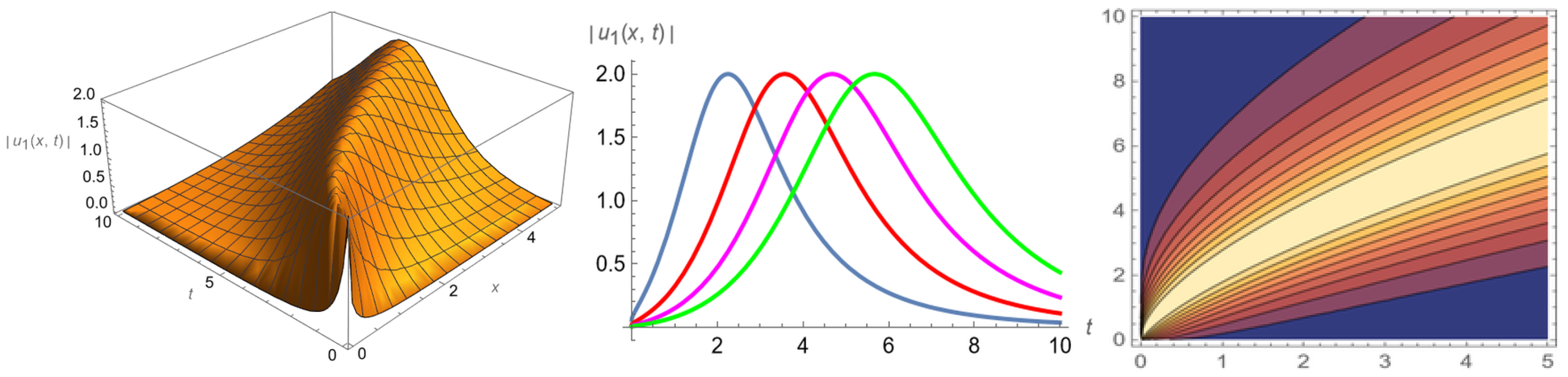
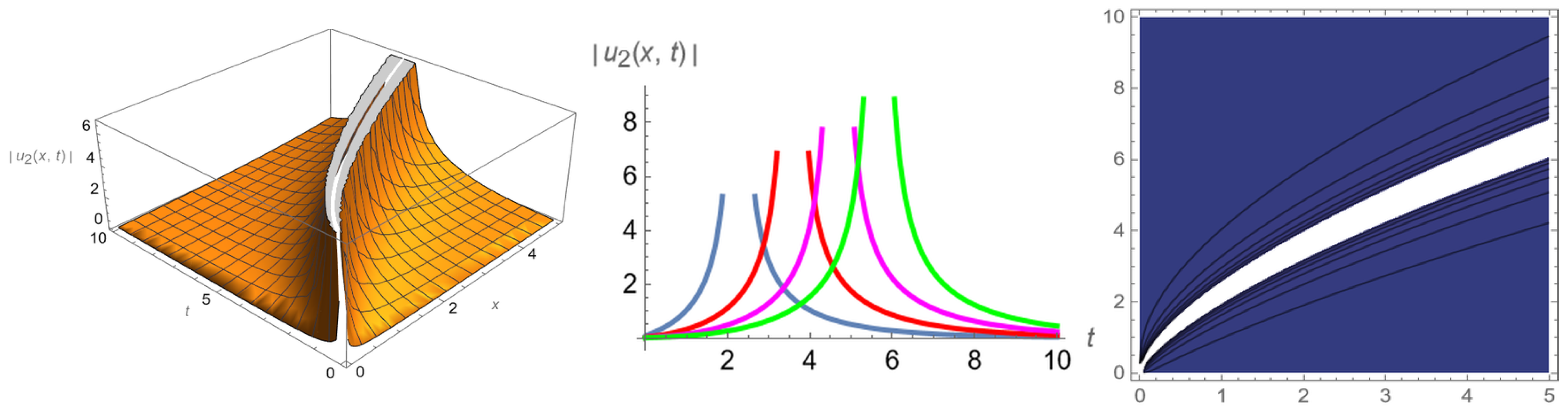
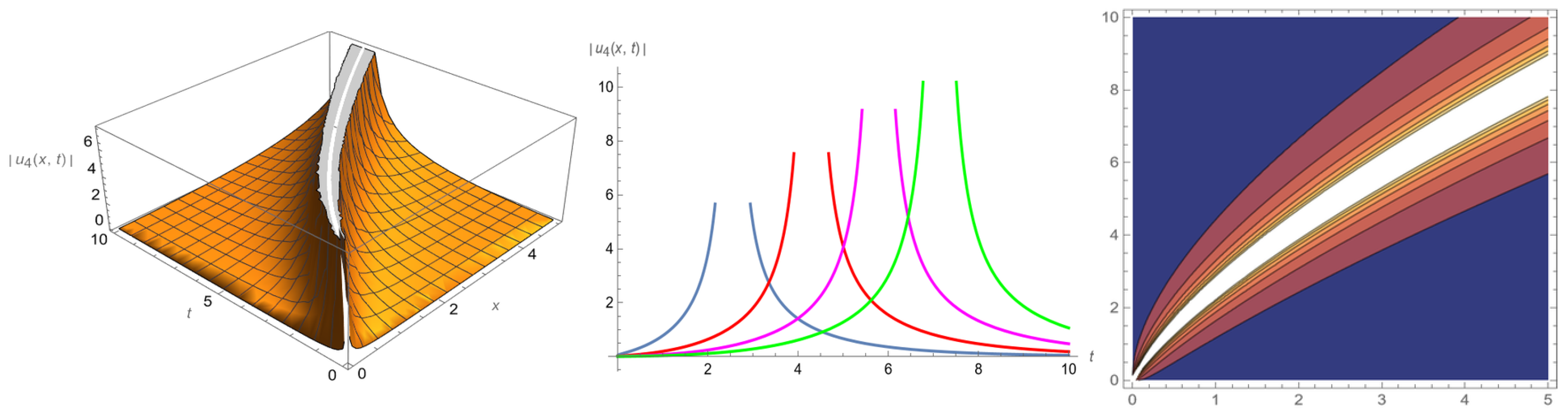
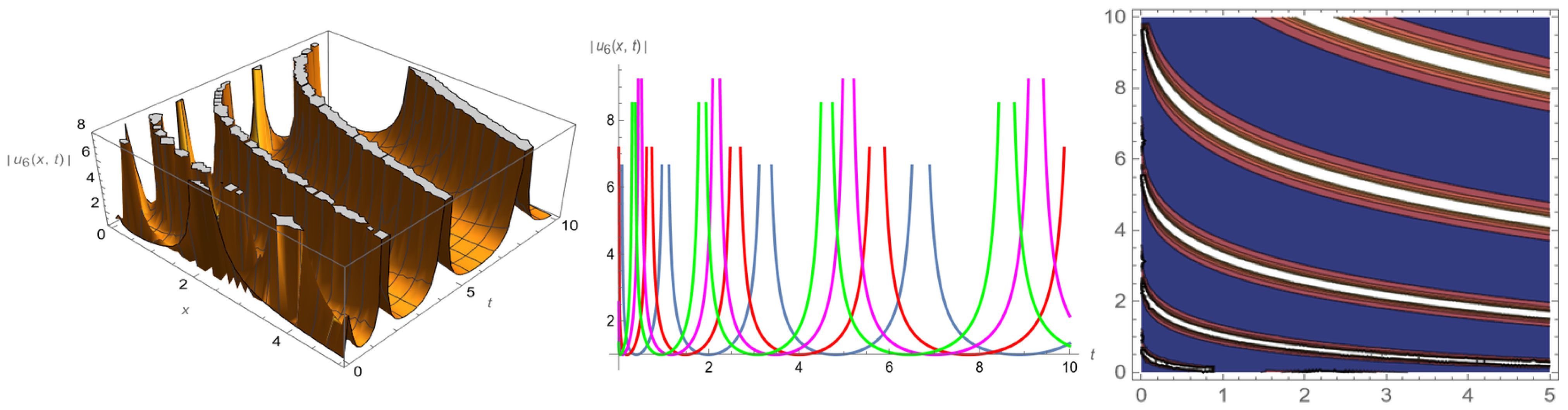
4.3. Physical and Geometry Interpretation

In this section, by selecting appropriate parameter values, we render amplitude images of the soliton, exponential and periodic solutions in the form of 3D, 2D and contour plots. Figure 1 represents the bright soliton $|u_1(x,t)|$ with parameters $\alpha ={\displaystyle \frac{1}{2}},\beta ={\displaystyle \frac{1}{3}},a=b=c=k=r=\upsilon =1,d=3,\omega =4,p_2=2,p_4=-3,\epsilon =1,{\xi }_0=0$. Figure 2 represents the hyperbolic function solution $|u_2(x,t)|$ with parameters $\alpha ={\displaystyle \frac{1}{2}},\beta ={\displaystyle \frac{1}{3}},a=b=c=k=r=\upsilon =1,d=3,\omega =4,p_2=2,p_4=1,\epsilon =1,{\xi }_0=0$. Figure 3 represents the exponential solution $|u_3(x,t)|$ with parameters $\alpha ={\displaystyle \frac{1}{2}},\beta ={\displaystyle \frac{1}{3}},a=b=c=r=1,d=3,\omega =4,p_2=1,p_4=1,k=\sqrt{2},\epsilon =1,\upsilon =\sqrt{2}$. Figure 4 represents the soliton solution $|u_4(x,t)|$ with parameters $\alpha ={\displaystyle \frac{1}{2}},\beta ={\displaystyle \frac{1}{3}},a=b=c=k=r=\upsilon =1,d=3,\omega =4,p_2=2,p_4=-1,\epsilon =1$. Figure 5 and Figure 6 represent the periodic solutions $|u_5(x,t)|$ and $|u_6(x,t)|$ with parameters $\alpha ={\displaystyle \frac{1}{2}},\beta ={\displaystyle \frac{1}{3}},a=c=k=r=1,b=2,d=6,\upsilon =\omega =-2,p_2=-1,p_4=1,\epsilon =-1$.

In quantum mechanics, the amplitude (modulus) of a solution is usually related to the probability density or strength of the system. It can be used to describe specific quantum states or dynamic behaviors. For example, in quantum computing, quantum simulation or quantum field theory, the information of the amplitude distribution is crucial in understanding the behavior of quantum systems, predicting experimental results and conducting theoretical analysis.

Figure 1 and Figure 4 show that the amplitude of the soliton waves does not change with time. They only move forward along the space axis with the specific wave velocities and propagation directions. Figure 2 and Figure 3 show that the amplitude of the wave gradually increases with the change in the spatial position. Figure 5 and Figure 6 show that the periodic wave moves left or right along the space axis with a constant amplitude.

5. Conclusions

From the above discussion, we see that the auxiliary equation method is an effective way to obtain exact traveling wave solutions of fractional NLPDEs. The results given in our paper show that the method can generate multiple solutions for the given equation, thus enriching the diversity of the solutions.

The auxiliary equation method not only plays a significant role in obtaining exact solutions of NLPDEs, but can also be widely applied in other fields. For example, it can be used to optimize the loss functions in machine learning models by improving the convergence speed and accuracy of the algorithm. When dealing with nonlinear and multivariable systems, it can help to design more accurate and efficient controllers. When simulating complex fluid flows and turbulent phenomena, it can be used to simplify the calculations and improve the simulation accuracy. When studying the mechanical properties of new materials, it can be used to analyze the stress–strain relationships and other key performance indicators.

Although the auxiliary equation method is highly effective in two-dimensional problems, in higher-dimensional problems, the complexity of the auxiliary equation and the solution structures increases greatly, which means that it may no longer be directly applicable or may require more complex techniques to be implemented. For some PDEs, the solution found by the auxiliary equation method may not be unique or may not satisfy all conditions. This requires further mathematical analysis to verify the existence and uniqueness of the solution. The auxiliary equation method is mainly used to find general solutions; when applying boundary and initial conditions to the solution, it may require further processing in combination with other methods.