Numerical Reconstruction of the Source in Dynamical Boundary Condition of Laplace’s Equation
Abstract
1. Introduction
2. The Forward Problem
3. Basic Identities
4. Inverse Problems
4.1. Solution of
4.2. Solution of
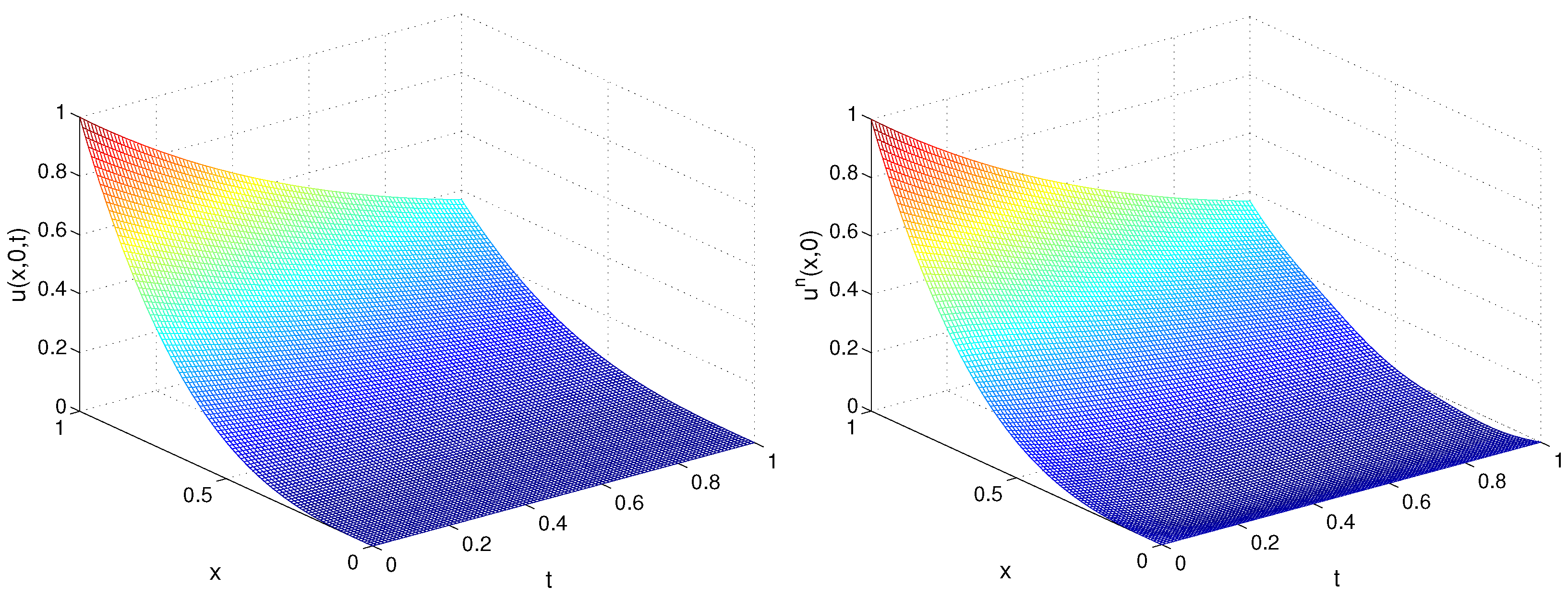
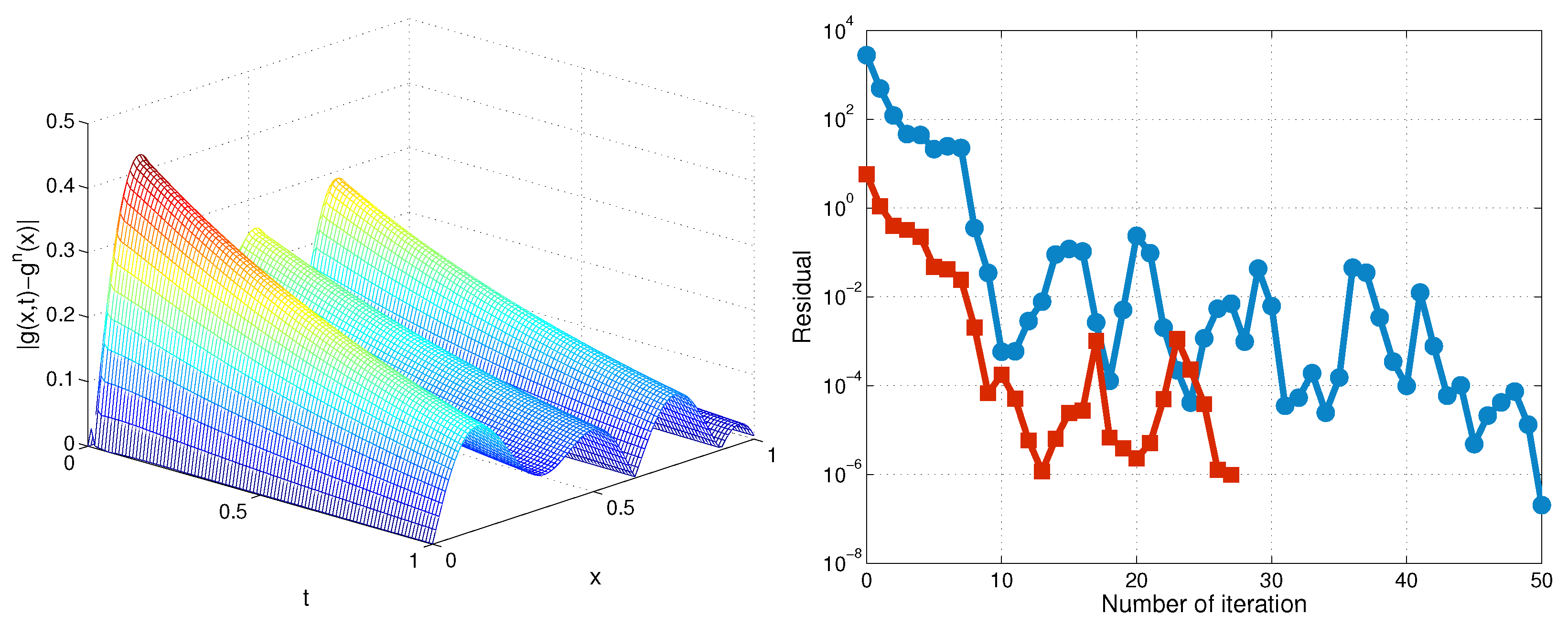
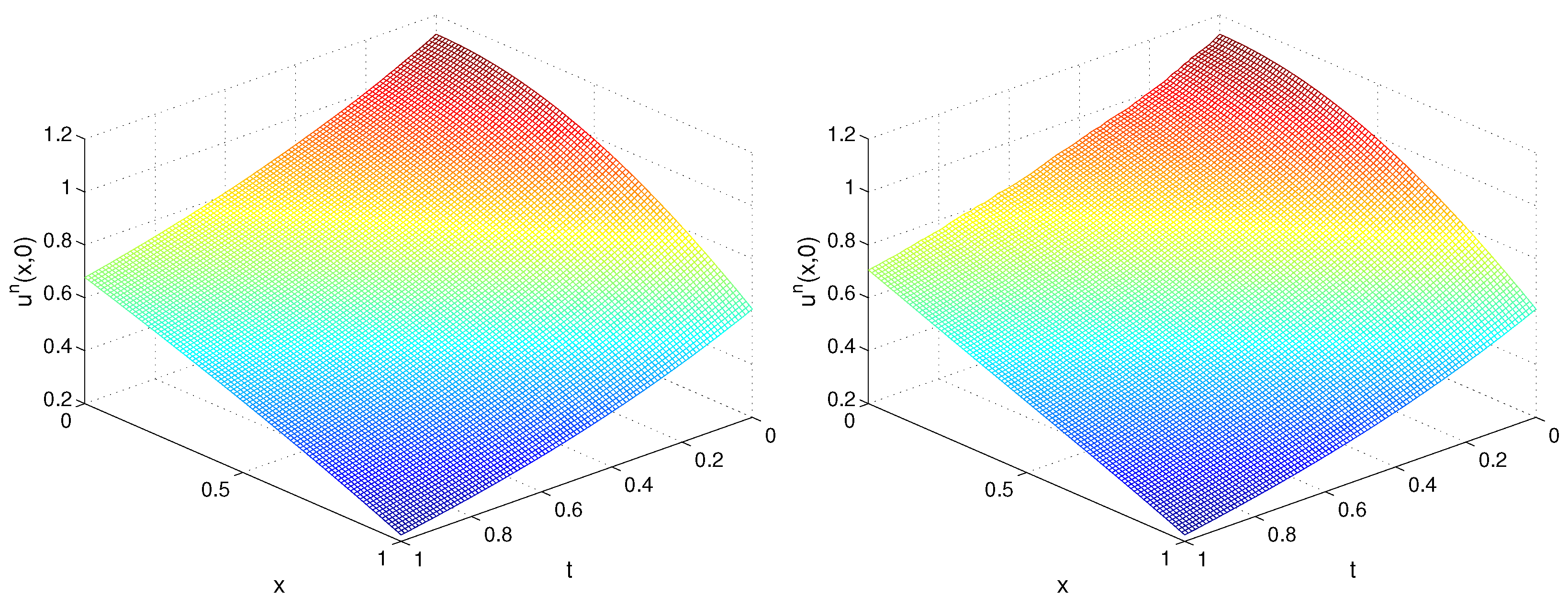
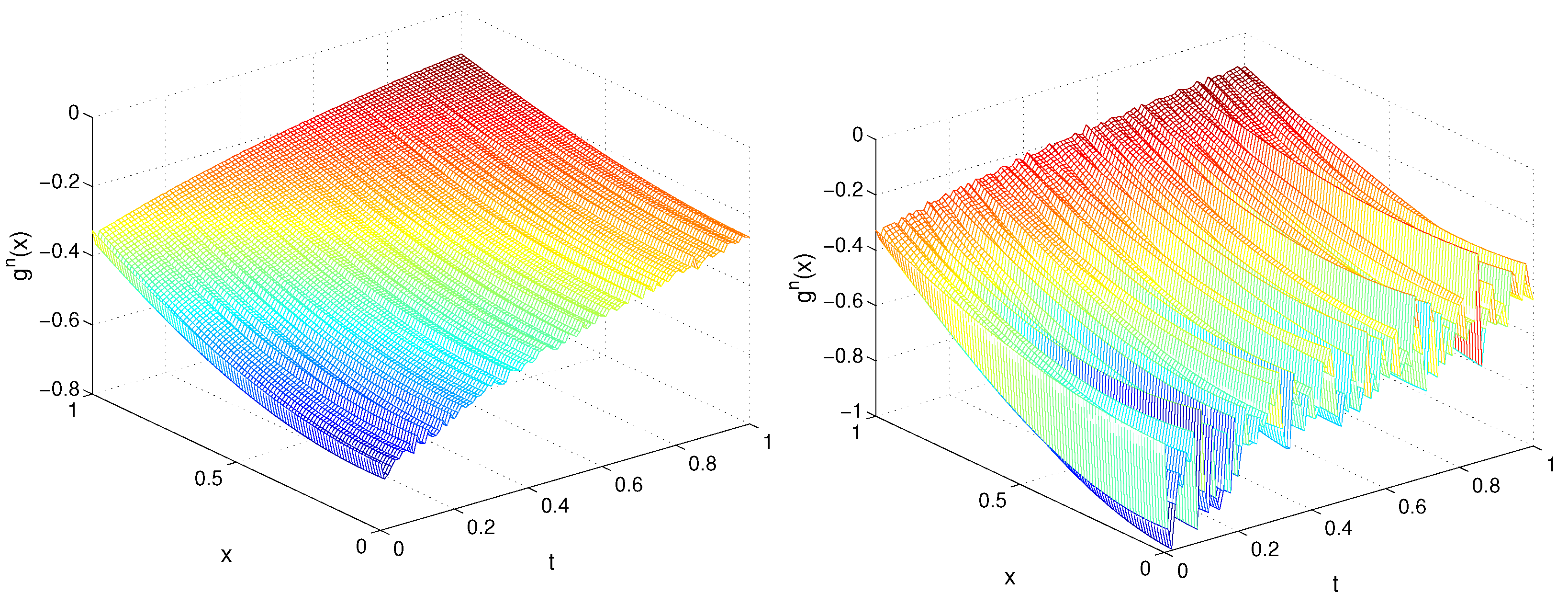
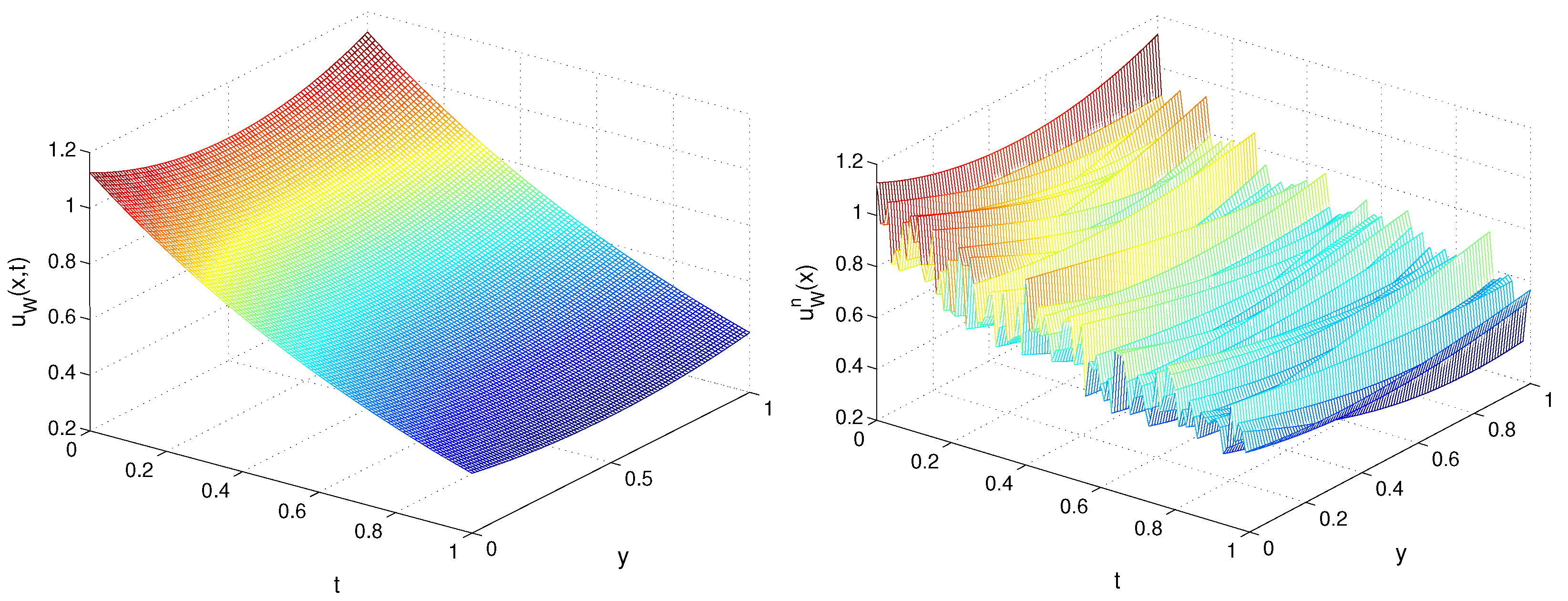
5. Numerical Results
- TP1: ;
- TP2: .
6. Discussion
7. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Crank, J. The Mathematics of Diffusion; Clarendon Press: Oxford, UK, 1973. [Google Scholar]
- Gröger, K. Initial boundary value problems from semiconductor device theory. Z. Angew. Math. Mech. 1978, 67, 345–355. [Google Scholar] [CrossRef]
- Langer, R.E. A problem in diffusion or in the flow of heat for a solid in contact with a fluid. Tohoka Math. J. 1972, 35, 260–275. [Google Scholar]
- Fila, M.; Quittner, P. Global solutions of the Laplace equation with a nonlinear dynamical boundary condition. Math. Appl. Sci. 1997, 20, 1325–1333. [Google Scholar] [CrossRef]
- Koleva, M.N.; Vulkov, L.G. Blow-up of continuous and semilinear solutions to elliptic equations with semilinear dynamical boundary conditions of parabolic type. J. Comp. Appl. Math. 2007, 202, 414–434. [Google Scholar] [CrossRef][Green Version]
- Jovanovic, B.S.; Vulkov, L.G. Convergence of difference schemes for the Poisson equation dynamical interface conditions. Comput. Methods Appl. Math. 2003, 3, 177–188. [Google Scholar] [CrossRef]
- Jovanovic, B.S.; Vulkov, L.G. Convergence of finite difference schemes for the Poisson’s equation with a dynamic boundary condition. Comput. Methods Appl. Math. 2005, 45, 275–284. [Google Scholar]
- Vabishchevich, P.N. Numerical solution of a problem for the elliptic equation with unsteady boundary conditions. Matem Model. 1995, 7, 49–60. (In Russian) [Google Scholar]
- Isakov, V. Inverse Source Problems; AMS: Providence, RI, USA, 1989. [Google Scholar]
- Grysa, K.; Macia̧g, A. Identifying heat source intensity in treatment of cancerous tumor using therapy based on local hyperthermia—The Trefftz method approachs. J. Therm. Biol. 2019, 84, 16–25. [Google Scholar] [CrossRef]
- Hasanoglu, A.; Romanov, V.G. Introduction to Inverse Problems for Differential Equations, 1st ed.; Springer: Cham, Switzerland, 2017; 261p. [Google Scholar]
- Kabanikhin, S.I. Inverse and Ill-Posed Problems; DeGruyer: Berlin, Germany, 2011. [Google Scholar]
- Lesnic, D. Inverse Problems with Applications in Science and Engineering; CRC Pres: Abingdon, UK, 2021; p. 349. [Google Scholar]
- Samarskii, A.A.; Vabishchevich, P.N. Numerical Methods for Solving Inverse Problems in Mathematical Physics; de Gruyter: Berlin, Germany, 2007; 438p. [Google Scholar]
- Ait Ben Hassi, E.M.; Chorfi, S.-E.; Maniar, L. Identification of source terms in heat equation with dynamic boundary conditions. Math. Meth. Appl. Sci. 2022, 45, 2364–2379. [Google Scholar] [CrossRef]
- Ivanov, D.K.; Kolesov, A.E.; Vabischevich, P.N. Numerical method for recovering the piecewise constant right-hand side function of an alliptic equation from a partial boundary observation data. J. Phys. Conf. Ser. 2021, 2092, 012006. [Google Scholar] [CrossRef]
- Liu, C.-S. A BIEM using the Treftz test functions for solving the inverse Cauchy and source recovery problems. Engn. Anal. Bound. Elem. 2016, 62, 177–185. [Google Scholar] [CrossRef]
- Liu, C.-S.; Cheng, C.-W. A global boundary integral equation method for recovering space-time dependent heat source. Int. J. Heat Mass Transf. 2016, 92, 1034–1040. [Google Scholar] [CrossRef]
- Yu, W. Well-posednes of determining the source term of elliptic equation. Bull. Austral. Math. Soc. 1994, 50, 383–398. [Google Scholar] [CrossRef][Green Version]
- Tikhonov, A.N.; Goncharsky, A.V.; Stepanov, V.V.; Yagola, A.G. Numerical Methods for the Solution of Ill-Posed Problems, 1st ed.; Springer: Dordrecht, The Netherlands, 1995; 253p. [Google Scholar]
- Tikhonov, A.N.; Arsenin, V.Y. Solutions of Ill-Posed Problems; V. H. Winston & Sons: Washington, DC, USA; John Wiley & Sons: New York, NY, USA, 1977; 258p, (Translated from Russian). [Google Scholar]
- Belgacem, F.B. Why is the Cauchy problem severely ill-posed? Inverse Probl. 2007, 23, 823–836. [Google Scholar] [CrossRef]
- Cheng, J.; Hon, Y.C.; Wei, T.; Yamamoto, M. Numerical computation of a Cauchy problem for Laplace’s equation. Z. Angew. Math. Mech. 2001, 81, 665–674. [Google Scholar] [CrossRef]
- Joachimiak, M.; Ciałkowski, M.; Fra̧ckowiak, A. Stable method for solving the Cauchy problem with the use of Chebyshev polynomials. Int. J. Numer. Methods Heat Fluid Flow 2019, 30, 1441–1456. [Google Scholar] [CrossRef]
- Joachimiak, M.; Joachimiak, D.; Ciałkowski, M. Investigation on thermal loads in steady-state conditions with the use of the solution to the inverse problem. Heat Transf. Eng. 2023, 44, 963–969. [Google Scholar] [CrossRef]
- El Hajji, M.; Jday, F. Boundary data completion for a diffusion-reaction equation based on the minimization of an energy error functional using conjugate gradient method. Punjab Univ. J. Math. 2019, 51, 25–43. [Google Scholar]
- Kirkeby, A. Feynman’s inverse problem. arXiv 2023. [Google Scholar] [CrossRef]
- Alessandrini, G. A small collection of open problems. Rend. Istit. Mat. Univ. Trieste 2020, 52, 591–600. [Google Scholar]
- Rundell, R. Some inverse problems for elliptic equations. Appl. Anal. Int. J. 1988, 28, 67–78. [Google Scholar] [CrossRef]
- Slodička, M.; Lesnic, D. Determination of the Robin coefficient in a nonlinear boundary condition for a steady-state problem. Math. Meth. Appl. Sci. 2009, 32, 1311–1324. [Google Scholar] [CrossRef]
- Engl, H.W.; Leitão, A. A Mann iterative regularization method for elliptic Cauchy problems. Numer. Funct. Anal. Optim. 2001, 22, 861–884. [Google Scholar] [CrossRef][Green Version]
- Kozlov, V.A.E.; Maz’ya, V.G.; Fomin, A.V. An iterative method for solving the Cauchy problem for elliptic equation. Comput. Math. Phys. 1991, 31, 45–52. [Google Scholar]
- Gong, R.; Wang, M.; Huang, Q.; Zhang, Y. Inverse Cauchy problems: Revisit and a new approach. arXiv 2022. [Google Scholar] [CrossRef]
- Jaoua, M.; Chaabane, S.; Elhechmi, C.; Leblond, J.; Mahjoub, M.; Partington, J. On some robust algorithms for Robin inverse problem. Rev. Arima 2008, 9, 287–307. [Google Scholar]
- Shirzadi, A.; Takhtabnoos, F. A local meshless method for Cauchy problem of elliptic PDEs in annulus domains. Inverse Probl. Sci. Eng. 2016, 24, 729–743. [Google Scholar] [CrossRef]
- Liu, C.-S.; Wang, F. A meshless method for solving the nonlinear inverse Cauchy problem of elliptic type equation in a doubly-connected domain. Comput. Math. Appl. 2018, 76, 1837–1852. [Google Scholar] [CrossRef]
- Wang, L.; Qian, Z.; Wang, Z.; Gao, Y.; Peng, Y. An efficient radial basis collocation method for the boundary condition identification of the inverse wave problem. Int. J. Appl. Mech. 2018, 10, 1850010. [Google Scholar] [CrossRef]
- Wang, L.; Wang, Z.; Qian, Z. A meshfree method for inverse wave propagation using collocation and radial basis functions. Comput. Methods Appl. Mech. Engrg. 2017, 322, 311–350. [Google Scholar]
- Hu, M.; Wang, L.; Yang, F.; Zhou, Y. Weighted radial basis collocation method for the nonlinear inverse Helmholtz problems. Mathematics 2023, 11, 662. [Google Scholar] [CrossRef]
- Ciałkowski, M.; Olejnik, A.; Joachimiak, M.; Grysa, K.; Fra̧ckowiak, A. Cauchy type nonlinear inverse problem in a two-layer area. Int. J. Numer. Methods Heat Fluid Flow 2021, 32, 313–331. [Google Scholar] [CrossRef]
- Karageorghis, A.; Lesnic, D.; Marin, L. A survey of applications of the MFS to inverse problems. Inverse Probl. Sci. Eng. 2011, 19, 309–336. [Google Scholar] [CrossRef]
- Liu, C.-S.; Qu, W.; Zhang, Y. Numerically solving twofold ill-posed inverse problems of heat equation by the adjoint Trefftz method. Numer. Heat Transf. Part B 2018, 73, 48–61. [Google Scholar] [CrossRef]
- Chorfi, S.E.; El Guermai, G.; Maniar, L.; Zouhair, W. Numerical identification of initial temperatures in heat equation with dynamic boundary conditions. Mediterr. J. Math. 2023, 20, 256. [Google Scholar] [CrossRef]
- Constantin, A.; Escher, J. Global solutions for quasilinear parabolic problems. J. Evol. Equations 2002, 2, 97–111. [Google Scholar] [CrossRef]
- Craig, W. A Course on Partial Differential Equations. Amer. Math. Soc. 2018, 197, 205. [Google Scholar]
- Esher, J. Smooth solutions of nonlinear elliptic systems with dynamic boundary conditions. In: Evolution equations, control theory, and biomathematics (Han sur Lesse, 1991). Lect. Notes Pure Appl. Math. 1994, 155, 173–183. [Google Scholar]
- Yin, Z. Global existence for elliptic equations with dynamic boundary conditions. Arch. Math. 2003, 81, 567–574. [Google Scholar] [CrossRef]
- Liu, C.-S. A highly accurate MCTM for inverse Cauchy problems of Laplace equation in arbitrary plane domains. Comput. Model. Eng. Sci. 2008, 35, 91–111. [Google Scholar]
- Liu, C.-S. A modified collocation Trefftz method for the inverse Cauchy problem of Laplace equation. Eng. Anal. Bound. Elem. 2008, 32, 778–785. [Google Scholar] [CrossRef]



| m | Max. Error | Max. Error | |||
|---|---|---|---|---|---|
| 0.01 | 1 | 6.8956 | 1.3360 | ||
| 0.005 | 1 | 4.3177 | 0.6754 | 2.4273 | 2.4606 |
| 0.0025 | 1 | 2.1914 | 0.9784 | 3.4441 | 2.8171 |
| 0.00125 | 1 | 1.1003 | 0.9940 | 5.0705 | 2.7639 |
| 0.000625 | 1 | 5.5081 | 0.9983 | 8.1055 | 2.6452 |
| 0.0003125 | 1 | 2.7551 | 0.9995 | 1.4465 | 2.4864 |
| 0.00015625 | 1 | 1.3777 | 0.9998 | 2.8862 | 2.3253 |
| 0.000078125 | 1 | 6.8888 | 0.9999 | 6.2998 | 2.1958 |
| 0.01 | 3 | 4.3634 | 3.4898 | ||
| 0.005 | 3 | 3.4898 | 0.3223 | 1.8095 | 0.9476 |
| 0.0025 | 3 | 1.9202 | 0.8619 | 1.5003 | 0.2703 |
| 0.00125 | 3 | 1.0576 | 0.8605 | 3.9079 | 1.9407 |
| 0.000625 | 3 | 5.4102 | 0.9670 | 9.9157 | 1.9786 |
| 0.0003125 | 3 | 2.7232 | 0.9904 | 2.5132 | 1.9802 |
| 0.00015625 | 3 | 1.3642 | 0.9972 | 6.2961 | 1.9970 |
| 0.000078125 | 3 | 6.8247 | 0.9992 | 1.5335 | 2.0376 |
| m | Max. Error | Max. Error | Iter | |
|---|---|---|---|---|
| = 0.02 | ||||
| 0.01 | 1 | 6.3069 | 1.1115 | 4.842 |
| 2 | 2.0456 | 9.1980 | 9.149 | |
| 3 | 4.0123 | 2.3232 | 15.733 | |
| 0.005 | 1 | 1.6550 | 2.0769 | 3.821 |
| 2 | 3.2464 | 1.5258 | 8.940 | |
| 3 | 4.3991 | 7.9018 | 19.010 | |
| 0.01 | 1 | 4.4107 | 7.3444 | 6.594 |
| 2 | 1.1629 | 5.2861 | 17.574 | |
| 3 | 3.9689 | 2.1198 | 21.306 | |
| 0.005 | 1 | 4.4905 | 3.9252 | 7.139 |
| 2 | 1.3193 | 7.0087 | 17.920 | |
| 3 | 4.0288 | 6.0438 | 24.303 | |
| m | Max. Error | Max. Error | Iter | |
|---|---|---|---|---|
| 0.01 | 10 | 4.3234 | 1.9103 | 40.763 |
| 15 | 3.0266 | 1.6096 | 47.208 | |
| 20 | 2.7671 | 1.4172 | 51.000 | |
| 0.005 | 10 | 4.2076 | 1.8547 | 44.582 |
| 15 | 2.9288 | 1.5733 | 51.553 | |
| 20 | 2.7508 | 1.3997 | 60.816 |
| Max. error | Max. Error | Max. Error | |||
|---|---|---|---|---|---|
| Iter | |||||
| 0.02 | 1 | 1.8693 | 4.2198 | 1.9027 | 13.821 |
| 2 | 2.6226 | 3.7340 | 2.8543 | 15.772 | |
| 3 | 2.4577 | 8.0663 | 2.8665 | 19.841 | |
| 0.2 | 1 | 5.8913 | 6.2845 | 2.6709 | 13.862 |
| 2 | 2.3012 | 2.4847 | 1.5154 | 15.861 | |
| 3 | 2.1429 | 6.2469 | 2.1894 | 19.594 |
| Max. Error | Max. Error | Max. Error | ||
|---|---|---|---|---|
| Iter | ||||
| 10 | 1.7842 | 1.6864 | 2.5069 | 39.267 |
| 15 | 1.4291 | 1.4003 | 1.1296 | 41.733 |
| 20 | 1.2674 | 1.4083 | 1.9546 | 48.831 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Koleva, M.N.; Vulkov, L.G. Numerical Reconstruction of the Source in Dynamical Boundary Condition of Laplace’s Equation. Axioms 2024, 13, 64. https://doi.org/10.3390/axioms13010064
Koleva MN, Vulkov LG. Numerical Reconstruction of the Source in Dynamical Boundary Condition of Laplace’s Equation. Axioms. 2024; 13(1):64. https://doi.org/10.3390/axioms13010064
Chicago/Turabian StyleKoleva, Miglena N., and Lubin G. Vulkov. 2024. "Numerical Reconstruction of the Source in Dynamical Boundary Condition of Laplace’s Equation" Axioms 13, no. 1: 64. https://doi.org/10.3390/axioms13010064
APA StyleKoleva, M. N., & Vulkov, L. G. (2024). Numerical Reconstruction of the Source in Dynamical Boundary Condition of Laplace’s Equation. Axioms, 13(1), 64. https://doi.org/10.3390/axioms13010064






